Protolytic Equilibria in Organized Solutions: Ionization and Tautomerism of Fluorescein Dyes and Related Indicators in Cetyltrimethylammonium Chloride Micellar Solutions at High Ionic Strength of the Bulk Phase
Abstract
:1. Introduction
- (i).
- Ion exchange between Cl− ions and components of buffer solutions is negligible;
- (ii).
- The Cl− concentrations in the bulk and in the Stern region are coming closer;
- (iii).
- The value is getting extremely low;
- (iv).
- Using of high enough HCl concentrations is possible under condition of constant ionic strength (HCl + KCl);
- (v).
- In contrast to micelles of non-ionic surfactants (where 0), where compounds of various hydrophobicity can more or less deeply sink in the polyoxyethylene “mantle”, in the micelles of chosen type compounds are localized in more compact Stern region, thus being in relatively identical conditions;
- (vi).
- (vii).
- The s of nitrofluoresceins are rather low (even in 50 mass % ethanol some of values are quite small [47]), and while studying them in solutions of cationic surfactants at low bulk ionic strength the values would have been extremely low and practically unavailable.
- (viii).
- Analogous systems with 4.00 M NaBr of KBr turned out to be less stable over time and with small temperature fluctuations.
2. Experimental Section
3. Results
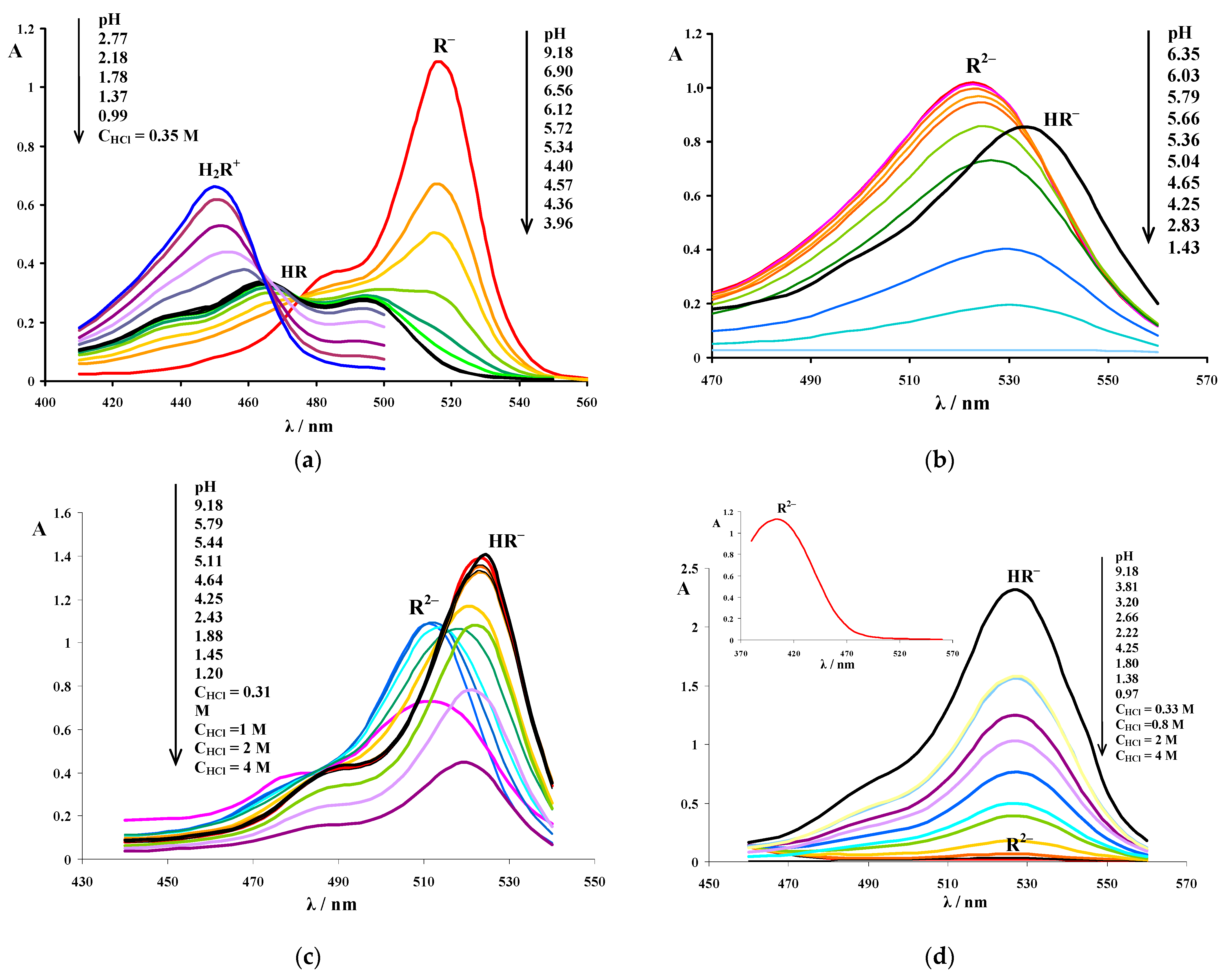
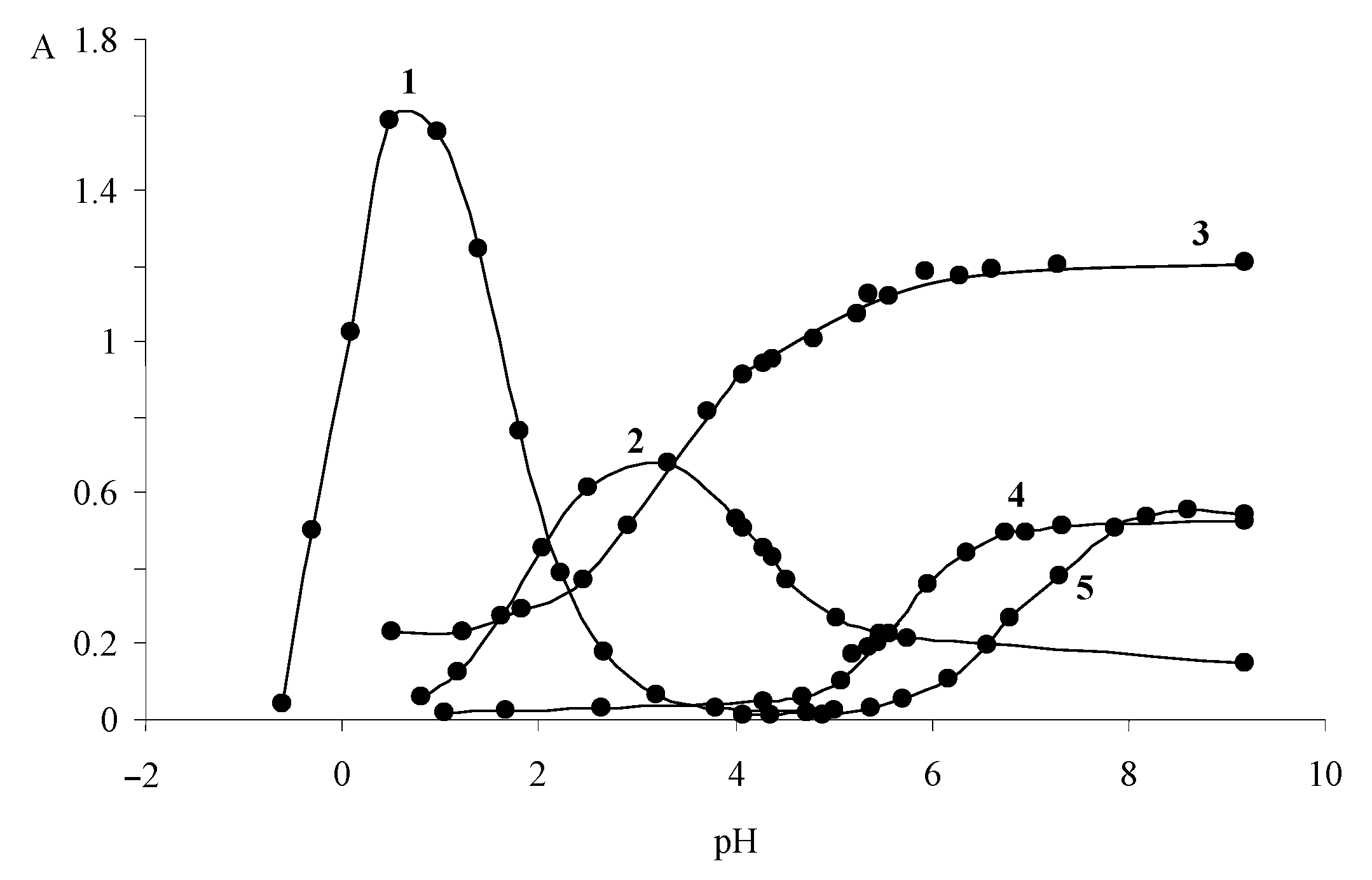
3.1. Determination of the Apparent Ionization Constants
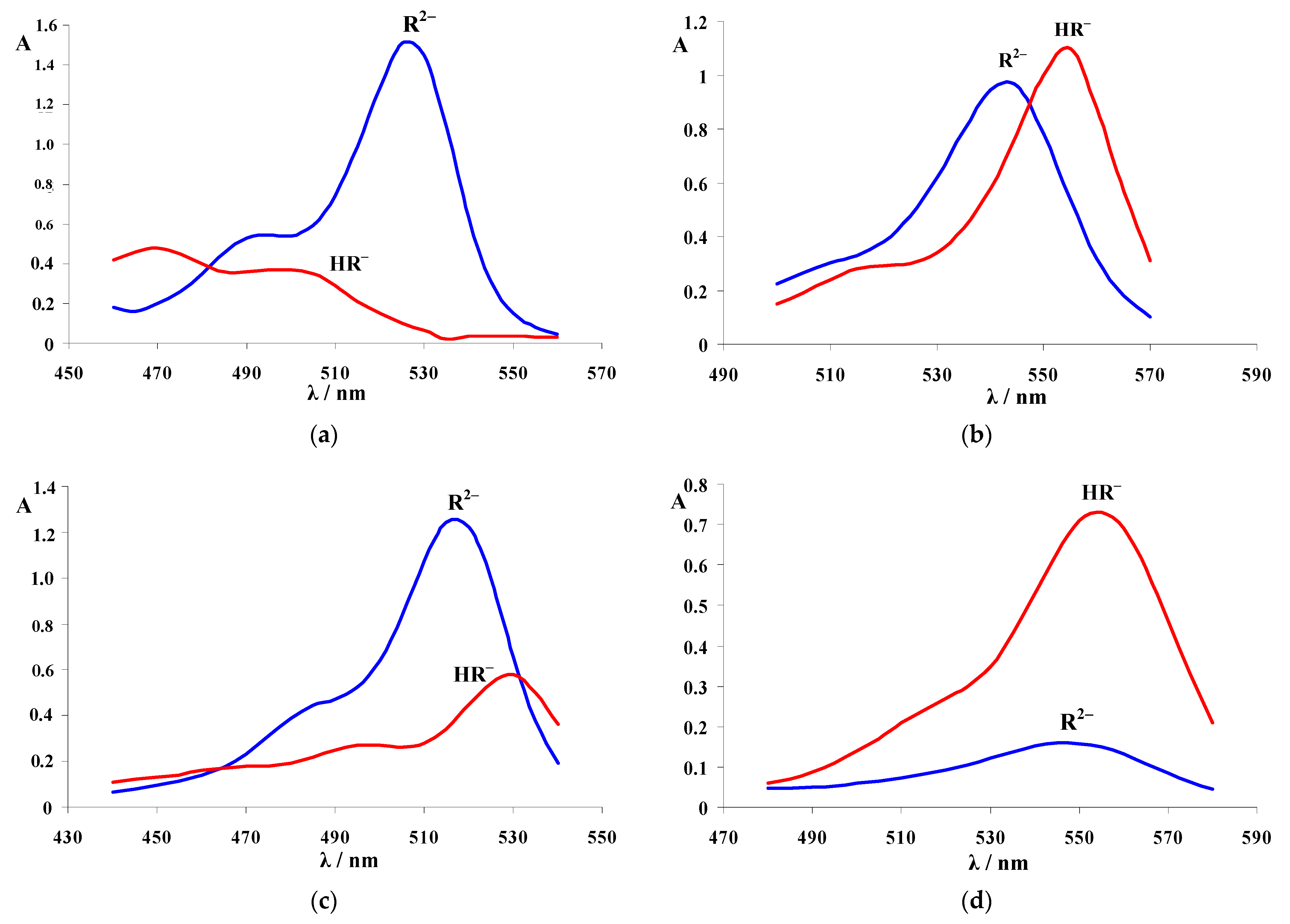
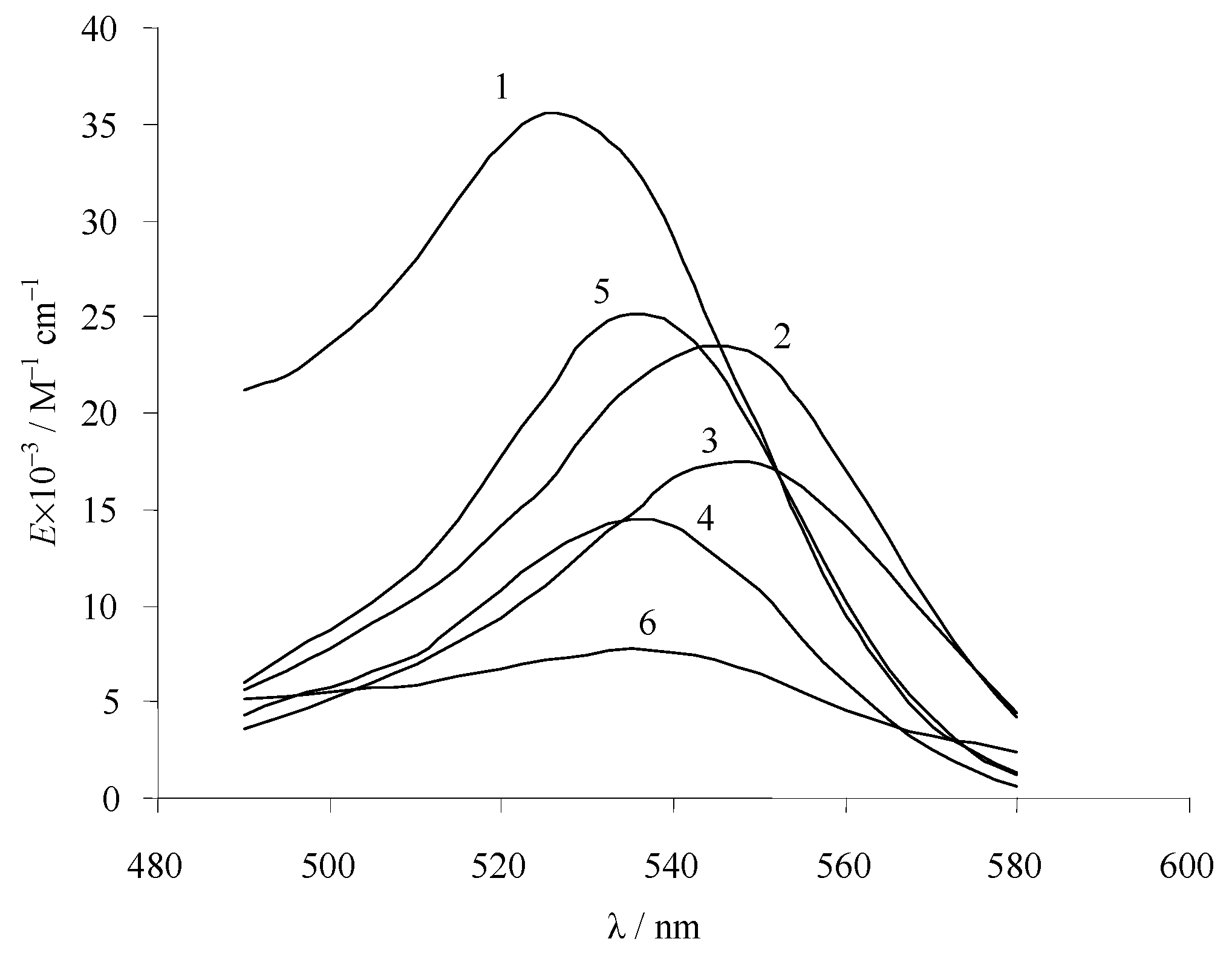
3.2. Absorption Spectra of Ionic and Molecular Forms
4. Discussion
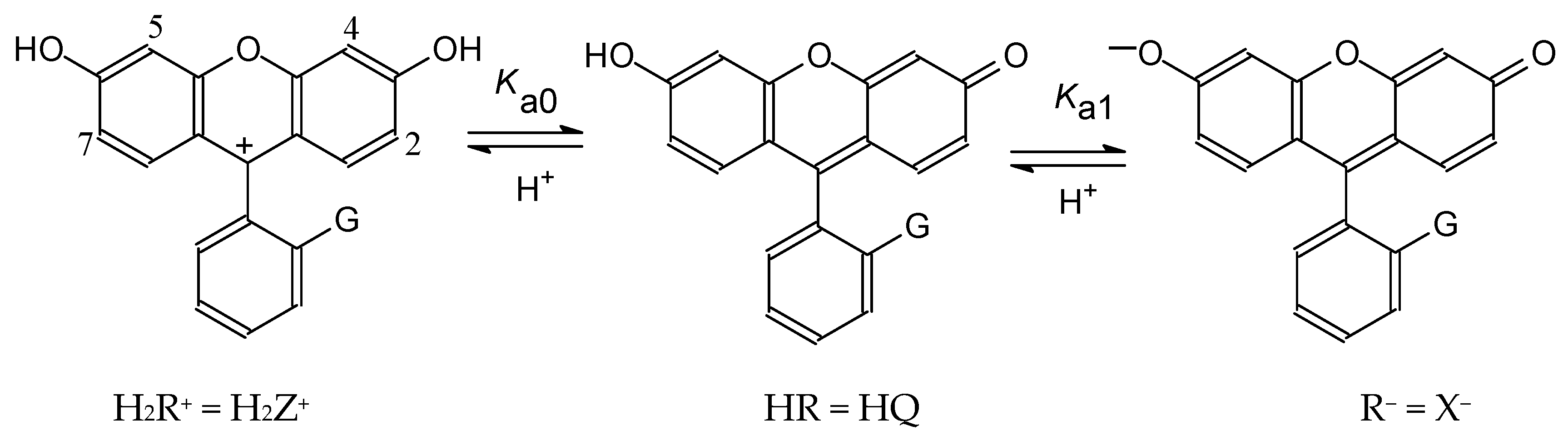
4.1. Ionization of Fluoresceins with Blocked Carboxylic Group and Similar Compounds
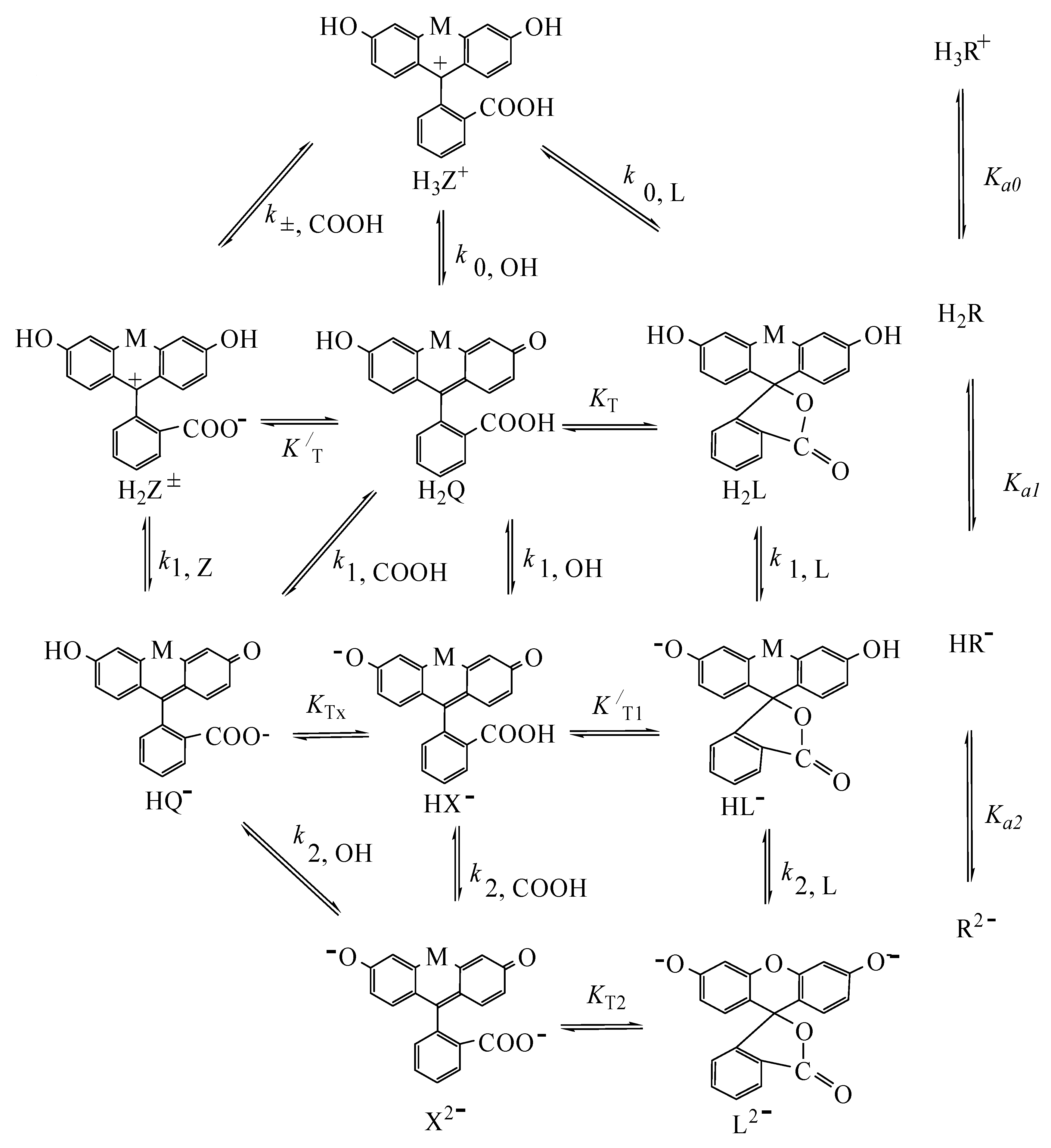
4.2. Detailed Scheme of Protolytic Equilibria in the Micellar Pseudophase
4.3. Classification of Fluorescein Dyes According to the Type of Tautomerism of Anions
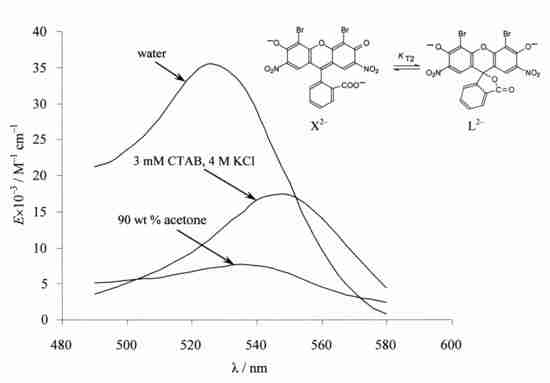
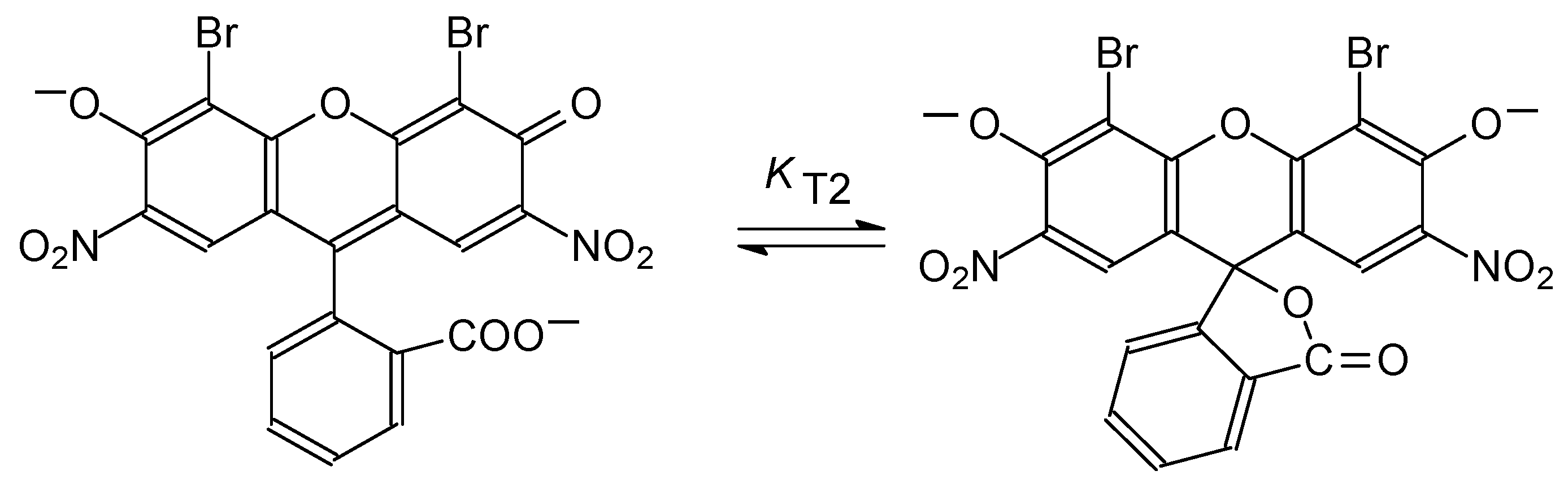
4.4. Chain-Ring Tautomerism of the Dianion of 4,5-dibromo-2,7-dinitrofluorescein
4.5. Dependence of the Ratio of the Values of the Stepwise Ionization Constants on the Character of Tautomerism
4.6. Solvation Properties of the CTAC–4.0 M KCl System
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Shinoda, K. The significance and characteristics of organized solutions. J. Phys. Chem. 1985, 89, 2429–2431. [Google Scholar] [CrossRef]
- Hartley, G.S. Organised structures in soap solutions. Nature 1949, 163, 767–768. [Google Scholar] [CrossRef]
- Romsted, L.S. (Ed.) Surfactants Science and Technology: Retrospects and Prospects; CRC Press Taylor & Francis Group: Boca Raton, FL, USA, 2014; p. 580. [Google Scholar]
- Lacerda, C.D.; Andrade, M.F.C.; Pessoa, P.S.; Prado, F.M.; Pires, P.A.R.; Pinatto-Botelho, M.F.; Wodtke, F.; Dos Santos, A.A.; Dias, L.G.; Lima, F.S.; et al. Experimental mapping of a pH gradient from a positively charged micellar interface to bulk solution. Colloids Surf. A Physicochem. Eng. Asp. 2021, 611, 125770. [Google Scholar] [CrossRef]
- Farafonov, V.S.; Lebed, A.V. Developing and validating a set of all-atom potential models for sodium dodecyl sulfate. J. Chem. Theory Comp. 2017, 13, 2742–2750. [Google Scholar] [CrossRef] [PubMed]
- Lukanov, B.; Firoozabadi, A. Specific ion effects on the self-assembly of ionic surfactants: A molecular thermodynamic theory of micellization with dispersion forces. Langmuir 2014, 30, 6373–6383. [Google Scholar] [CrossRef] [PubMed]
- Mchedlov-Petrossyan, N.O.; Kleshchevnikova, V.N. Influence of the cetyltrimethylammonium chloride micellar pseudophase on the protolytic equilibria of oxyxanthene dyes at high bulk phase ionic strength. J. Chem. Soc. Faraday Trans. 1994, 90, 629–640. [Google Scholar] [CrossRef]
- Mchedlov-Petrossyan, N.O. Protolytic equilibrium in lyophilic nano-sized dispersions: Differentiating influence of the pseudophase and salt effects. Pure Appl. Chem. 2008, 80, 1459–1510. [Google Scholar] [CrossRef]
- Grieser, F.; Drummond, C.J. The physicochemical properties of self-assembled surfactant aggregates as determined by some molecular spectroscopic probe techniques. J. Phys. Chem. 1988, 92, 5580–5593. [Google Scholar] [CrossRef]
- Fernandez, M.S.; Fromherz, P. Lipoid pH indicators as probes of electrical potential and polarity in micelles. J. Phys. Chem. 1977, 81, 1755–1761. [Google Scholar] [CrossRef]
- Funasaki, N. The effect of the solvent property of the surfactant micelle on the dissociation constants of weak electrolytes. Nippon Kagaku Kaishi 1976, 5, 722–726. [Google Scholar] [CrossRef]
- Mchedlov-Petrossyan, N.O.; Vodolazkaya, N.A.; Kamneva, N.N. Acid-base equilibrium in aqueous micellar solutions of surfactants. In Micelles: Structural Biochemistry, Formation and Functions & Usage; Bradburn, D., Bittinger, J., Eds.; Nova Publishers: New York, NY, USA, 2013; pp. 1–71. [Google Scholar]
- Kibblewhite, J.; Drummond, C.J.; Grieser, F.; Thistlethwaite, P.J. Lipoidal eosin and fluorescein derivatives as probes of the electrostatic characteristics of self-assembled surfactant/water interfaces. J. Phys. Chem. 1989, 93, 7464–7473. [Google Scholar] [CrossRef]
- Brown, L.; Halling, P.J.; Johnston, G.A.; Suckling, C.J.; Valivety, R.V. The synthesis of some water insoluble dyes for the measurement of pH in water immiscible solvents. J. Chem. Soc. Perkin Trans. I 1990, 1, 3349–3353. [Google Scholar] [CrossRef]
- Vodolazkaya, N.A.; Gurina, Y.A.; Salamanova, N.V.; Mchedlov-Petrossyan, N.O. Spectroscopic study of acid-base ionization and tautomerism of fluorescein dyes in direct microemulsions at high bulk ionic strength. J. Mol. Liq. 2009, 145, 188–196. [Google Scholar] [CrossRef]
- De Freitas, C.F.; Vanzin, D.; Braga, T.L.; Pellosi, D.S.; Batistella, V.R.; Caetano, W.; Hioka, N. Multivariate analysis of protolytic and tautomeric equilibria of Erythrosine B and its ester derivatives in ionic and non-ionic micelles. J. Mol. Liq. 2020, 313, 113320. [Google Scholar] [CrossRef]
- De Freitas, C.F.; Estevão, B.M.; Pellosi, D.S.; Scarminio, I.S.; Caetano, W.; Hioka, N.; Batistella, V.R. Chemical equilibria of Eosin Y and its synthetic ester derivatives in non-ionic and ionic micellar environments. J. Mol. Liq. 2020, 114794, in press. [Google Scholar] [CrossRef]
- De Freitas, C.F.; da Rocha, N.L.; Pereverzieff, I.S.; Batistela, V.R.; Malacarne, L.C.; Hioka, N.; Caetano, W. Potential of triblock copolymers Pluronic® P-84 and F-108 with erythrosine B and its synthetic ester derivatives for photodynamic applications. J. Mol. Liq. 2020, 322, 114904. [Google Scholar] [CrossRef]
- Pereira, P.C.S.; Costa, P.F.A.; Pellosi, D.S.; Calori, I.R.; Vilsinski, B.H.; Estevão, B.M.; Hioka, N.; Caetano, W. Photophysical properties and interaction studies of Rose Bengal derivatives with biomimetic systems based in micellar aqueous solutions. J. Mol. Liq. 2017, 230, 674–685. [Google Scholar] [CrossRef]
- Haugland, R.P. Handbook of Fluorescent Probes and Research Products, 9th ed.; Molecular Probes, Inc.: Eugene, OR, USA, 2002; p. 966. [Google Scholar]
- Urano, Y.; Kamiya, M.; Kanda, K.; Ueno, T.; Hirose, K.; Nagano, T. Evolution of fluorescein as a platform for finely tunable fluorescence probes. J. Am. Chem. Soc. 2005, 127, 4888–4894. [Google Scholar] [CrossRef]
- Han, J.; Burgess, K. Fluorescent indicators for intracellular pH. Chem. Rev. 2010, 110, 2709–2728. [Google Scholar] [CrossRef]
- Li, X.; Gao, X.; Shi, W.; Ma, H. Design strategies for water-soluble small molecular chromogenic and fluorogenic probes. Chem. Rev. 2014, 114, 590–659. [Google Scholar] [CrossRef]
- Lavis, L.D. Teaching old dyes new tricks: Biological probes built from fluoresceins and rhodamines. Annu. Rev. Biochem. 2017, 86, 825–843. [Google Scholar] [CrossRef]
- Hirabayashi, K.; Hanaoka, K.; Takayanagi, T.; Toki, Y.; Egawa, T.; Kamiya, M.; Komatsu, T.; Ueno, T.; Terai, T.; Yoshida, K.; et al. Analysis of chemical equilibrium of silicon-substituted fluorescein and its application to develop a scaffold for red fluorescent probes. Anal. Chem. 2015, 87, 9061–9069. [Google Scholar] [CrossRef] [PubMed]
- Schröder, C.R.; Weidgans, B.M.; Klimant, I. pH Fluorosensors for use in marine systems. Analyst 2005, 130, 907–916. [Google Scholar] [CrossRef] [PubMed]
- McLoughlin, C.K.; Kotroni, E.; Bregnhøj, M.; Rotas, G.; Vougioukalakis, G.C.; Ogilby, P.R. Oxygen- and pH-dependent photophysics of fluorinated fluorescein derivatives: Non-symmetrical vs. symmetrical fluorination. Sensors 2020, 20, 5172. [Google Scholar] [CrossRef] [PubMed]
- Niazi, A.; Yazdanipour, A.; Ghasemi, J.; Amini, A.; Bozorgzad, S.; Kubista, M. Spectrophotometric investigation of the acidity constants of fluorescein in various water-organic solvent media. Chem. Eng. Comm. 2008, 195, 1257–1268. [Google Scholar] [CrossRef]
- Batistela, V.R.; Pellosi, D.S.; Souza, F.D.; Costa, W.F.; Santin, S.M.O.; Souza, V.R.; Oliveira, H.P.M.; Scarminio, I.S.; Hioka, N. pKa determinations of xanthene derivates in aqueous solutions by multivariate analysis applied to UV–Vis spectrophotometric data. Spectrochim. Acta Part A 2011, 79, 889–897. [Google Scholar] [CrossRef]
- Vanzin, D.; Freitas, C.F.; Pellosi, D.S.; Batistela, V.R.; Machado, A.E.H.; Pontes, R.M.; Caetano, W.; Hioka, N. Experimental and computational studies studies of protolytic and tautometic equilibria of Erythrosin B and Eosin Y in water/DMSO. RSC Adv. 2016, 6, 110312–110328. [Google Scholar] [CrossRef]
- Mchedlov-Petrossyan, N.O.; Cheipesh, T.A.; Shekhovtsov, S.V.; Redko, A.N.; Rybachenko, V.I.; Omelchenko, I.V.; Shishkin, O.V. Ionization and tautomerism of methyl fluorescein and related dyes. Spectrochim. Acta A Mol. Biomol. Spectrosc. 2015, 150, 151–161. [Google Scholar] [CrossRef]
- Greeneltch, N.G.; Davis, A.S.; Valley, N.A.; Casadio, F.; Schatz, G.C.; Van Duyne, R.P.; Shah, N.C. Near-infrared surface-enhanced Raman spectroscopy (NIR-SERS) for the identification of eosin y: Theoretical calculations and evaluation of two different nanoplasmonic substrates. J. Phys. Chem. A 2012, 116, 11863–11869. [Google Scholar] [CrossRef]
- Crovetto, L.; Orte, A.; Paredes, J.M.; Resa, S.; Valverde, J.; Castello, F.; Miguel, D.; Cuerva, J.M.; Talavera, E.M.; Alvarez-Pez, J.M. Photophysics of a live-cell-marker, red silicon-substituted xanthene dye. J. Phys. Chem. A. 2015, 119, 10854–10862. [Google Scholar] [CrossRef]
- Hwang, J.Y.; Shim, S.; Hwang, G.T. 4′,5′-Bis(dimethylamino)fluorescein exhibits pH-dependent emission behavior distinct from that of fluorescein. Asian J. Org. Chem. 2018, 7, 150–154. [Google Scholar] [CrossRef] [Green Version]
- Hwang, J.Y.; Lee, J.-Y.; Cho, C.-W.; Choi, W.; Lee, Y.; Shim, S.; Hwang, G.T. 5-Bromo-4′,5′-bis(dimethylamino)fluorescein: Synthesis and photophysical studies. Molecules 2018, 23, 219. [Google Scholar] [CrossRef] [Green Version]
- Orte, A.; Ruedas-Rama, M.J.; Paredes, J.M.; Crovetto, L.; Alvarez-Pez, J.M. Dynamics of water-in-oil nanoemulsions revealed by fluorescence lifetime correlation spectroscopy. Langmuir 2011, 27, 12792–12799. [Google Scholar] [CrossRef]
- Mchedlov-Petrossyan, N.O.; Vodolazkaya, N.A.; Surov, Y.N.; Samoylov, D.V. 2,4,5,7–Tetranitrofluorescein in solutions: Novel type of tautomerism in hydroxyxanthene series as detected by various spectral methods. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2005, 61, 2747–2760. [Google Scholar] [CrossRef]
- Mchedlov-Petrossyan, N.O.; Steinbach, K.; Vodolazkaya, N.A.; Samoylov, D.V.; Shekhovtsov, S.V.; Omelchenko, I.V.; Shishkin, O.V. The molecular structure of anionic species of 2,4,5,7-tetranitrofluorescein as studied by ESI, NMR, and X-ray techniques. Coloration Technol. 2018, 134, 390–399. [Google Scholar] [CrossRef]
- Mchedlov-Petrossyan, N.O.; Vodolazkaya, N.A.; Gurina, Y.A.; Sun, W.-C.; Gee, K.R. Medium effects on the prototropic equilibria of fluorescein fluoro derivatives in true and organized solution. J. Phys. Chem. B 2010, 114, 4551–4564. [Google Scholar] [CrossRef]
- Mchedlov-Petrossyan, N.O.; Cheipesh, T.A.; Vodolazkaya, N.A. Acid-base dissociation and tautomerism of two aminofluorescein dyes in solution. J. Mol. Liq. 2017, 225, 696–705. [Google Scholar] [CrossRef]
- Mchedlov-Petrossyan, N.O.; Cheipesh, T.A.; Shekhovtsov, S.V.; Ushakova, E.V.; Roshal, A.D.; Omelchenko, I.V. Aminofluoresceins vs. fluorescein: Ascertained new unusual features of tautomerism and dissociation of hydroxyxanthene dyes in solution. J. Phys. Chem. A 2019, 123, 8845–8859. [Google Scholar] [CrossRef] [PubMed]
- Lebed, A.V.; Biryukov, A.V.; Mchedlov-Petrossyan, N.O. A quantum-chemical study of tautomeric equilibria of fluorescein dyes in DMSO. Chem. Heterocycl. Comp. 2014, 50, 336–348. [Google Scholar] [CrossRef]
- Yao, H.; Jockusch, R.A. Fluorescence and electronic action spectroscopy of mass-selected gas-phase fluorescein, 2′,7′-dichlorofluorescein, and 2′,7′-difluorofluorescein ions. J. Phys. Chem. A 2013, 117, 1351–1359. [Google Scholar] [CrossRef]
- Tanabe, T.; Saito, M.; Noda, K.; Starikov, E.B. Molecular structure conversion of fluorescein monoanions in an electrostatic storage ring. Eur. Phys. J. D 2012, 66, 1–8. [Google Scholar] [CrossRef]
- Horke, D.A.; Chatterley, A.S.; Bull, J.N.; Verlet, J.R.R. Time-resolved photodetachment anisotropy: Gas-phase rotational and vibrational dynamics of the fluorescein anion. J. Phys. Chem. Lett. 2015, 6, 189–194. [Google Scholar] [CrossRef] [Green Version]
- Tamburello-Luca, A.A.; Herbert, P.; Antoine, R.; Brevet, P.F.; Girault, H.H. Optical surface second harmonic generation study of the two acid-base equilibria of eosin b at air/water interface. Langmuir 1997, 13, 4428–4434. [Google Scholar] [CrossRef]
- Samoilov, D.V.; Mchedlov-Petrosyan, N.O.; Martynova, V.P.; El’tsov, A.V. Protolytic equilibria of fluorescein nitro derivatives. Russ. J. Gen. Chem. 2000, 70, 1259–1271. [Google Scholar]
- Mchedlov-Petrosyan, N.O.; Vodolazkaya, N.A.; Martynova, V.P.; Samoilov, D.V.; El’tsov, A.V. Protolytic properties of thiofluorescein and its derivatives. J. Gen. Chem. 2002, 72, 785–792. [Google Scholar] [CrossRef]
- Guo, Z.-j.; Miyoshi, H.; Komoyoji, T.; Haga, T.; Fujita, T. Quantitative analysis with physicochemical substituent and molecular parameters of uncoupling activity of substituted diarylamines. Biochim. Biophys. Acta 1991, 1059, 91–98. [Google Scholar] [CrossRef]
- Mchedlov-Petrossyan, N.O.; Farafonov, V.S.; Cheipesh, T.A.; Shekhovtsov, S.V.; Nerukh, D.A.; Lebed, A.V. In search of an optimal acid-base indicator for examining surfactant micelles: Spectrophotometric studies and molecular dynamics simulations. Colloids Surf. A Physicochem. Eng. Asp. 2019, 565, 97–107. [Google Scholar] [CrossRef] [Green Version]
- Machado, V.G.; Stock, R.I.; Reichardt, C. Pyridinium N-phenolate betaine dyes. Chem. Rev. 2014, 114, 10429–10475. [Google Scholar] [CrossRef] [PubMed]
- Drummond, C.J.; Grieser, F.; Healy, T.W. A single spectroscopic probe for the determination of both the interfacial solvent properties and electrostatic surface potential of model lipid membranes. Faraday Discuss. 1986, 81, 95–106. [Google Scholar] [CrossRef]
- Drummond, C.J.; Grieser, F.; Healy, T.W. Effect of electrolyte on the mean interfacial solveny and electrostatic characteristic of cationic micelles. Chem. Phys. Lett. 1987, 140, 493–498. [Google Scholar] [CrossRef]
- Healy, T.W.; Drummond, C.J.; Grieser, F.; Murray, B.S. Electrostatic surface potential and critical micelle concentration relationship for ionic micelles. Langmuir 1990, 6, 506–508. [Google Scholar] [CrossRef]
- Yakubovskaya, A.G.; Vodolazkaya, N.A.; Mchedlov-Petrossyan, N.O. Ionic equilibria of acid/base indicators in micellar media. Ionization of dinitrophenols in aqueous solutions of cationic and zwitterionic surfactants. Kharkov Univ. Bull. Chem. Ser. 2006, 14, 217–229. [Google Scholar]
- Kholin, Y.V. CLINP Program. Available online: http://www.bestnet.kharkov.ua/kholin/clinp.html (accessed on 30 January 2020).
- Mchedlov-Petrossyan, N.O.; Kukhtik, V.I.; Bezugliy, V.D. Dissociation, tautomerism and electroreduction of xanthene and sulfonephthalein dyes in N,N-dimethylformamide and other solvents. J. Phys. Org. Chem. 2003, 16, 380–397. [Google Scholar] [CrossRef]
- Markuszewski, R.; Diehl, H. The infrared spectra and structures of the three solid forms of fluorescein and related compounds. Talanta 1980, 27, 937–946. [Google Scholar] [CrossRef]
- Anthoni, U.; Christophersen, C.; Nielsen, P.H.; Püschl, A.; Schaumburg, K. Structure of red and orange fluorescein. Struct. Chem. 1995, 6, 161–165. [Google Scholar] [CrossRef]
- Fompeydie, D.; Levillain, P. Équilibre entre formes structurales de l’éosine et de la fluorescéine moléculaires. Influence des solvants. Bull. Soc. Chem. Fr. 1980, 11–12, 459–465. [Google Scholar]
- Klonis, N.; Sawyer, W.H. Spectral properties of the prototropic forms of fluorescein in aqueous solutions. J. Fluoresc. 1996, 6, 147–157. [Google Scholar] [CrossRef]
- Vodolazkaya, N.A.; Kleshchevnikova, Y.A.; Mchedlov-Petrossyan, N.O. Differentiating impact of the AOT-stabilized droplets of water-in-octane microemulsions as examined using halogenated fluoresceins as molecular probes. J. Mol. Liq. 2013, 187, 381–388. [Google Scholar] [CrossRef]
- Mchedlov-Petrossyan, N.O.; Bryleva, E.Y.; Vodolazkaya, N.A.; Dissanayake, A.A.; Ford, W.T. The nature of cationic poly(propylenimine) dendrimers in aqueous solutions as studied using versatile indicator dyes. Langmuir 2008, 24, 5689–5699. [Google Scholar] [CrossRef] [PubMed]
- Cheipesh, T.A.; Zagorulko, E.S.; Mchedlov-Petrossyan, N.O.; Rodik, R.V.; Kalchenko, V.I. The difference between the aggregates of a short-tailed and a long-tailed cationic calix[4]arene in water as detected using fluorescein dyes. J. Mol. Liq. 2014, 193, 232–238. [Google Scholar] [CrossRef]
- Bogdanova, L.N.; Mchedlov-Petrossyan, N.O.; Vodolazkaya, N.A.; Lebed, A.V. The influence of β–cyclodextrin on acid-base and tautomeric equilibrium of fluorescein dyes in aqueous solution. Carbohydr. Res. 2010, 345, 1882–1890. [Google Scholar] [CrossRef]
- Kölbel, H. Quantitative Untersuchungen über den Speicherungsmechanismus von Rhodamin B, Eosin und Neutralrot in Hefezellen. Zeitschrift für Naturforschung B 1948, 3, 442–453. [Google Scholar] [CrossRef] [Green Version]
- Scheibe, G.; Bruck, D. Zusammenhang physikalischer und chemischer Eigenschaften bei der Bildung Van der Waals’scher Molekeln. Z. Elektrochem. 1950, 54, 403–412. [Google Scholar]
- Birkedal-Hansen, H. Eosin staining of gelatine. Histochemie 1973, 36, 73–87. [Google Scholar] [CrossRef]
- Mchedlov-Petrossyan, N.O.; Adamovich, L.P.; Nikishina, L.E. Ionization constants and tautomeric equilibrium of eosin in aqueous solution. Russ. J. Anal. Chem. 1980, 36, 1495–1502. [Google Scholar]
- Birge, R.; Acree, S.F. On the quinonephenolate theory of indicators. A spectrophotometric method for measuring the concentrations of the quinoidal and lactoidal salts and the equilibrium and affinity constants of the phenolphthaleins and phenolenolsulfonphthaleins. J. Am. Chem. Soc. 1919, 41, 1031–1050. [Google Scholar] [CrossRef] [Green Version]
- Thiel, A.; Diehl, R. Beiträge zur systematischen Indikatorenkunde. Marburg. Sitzungsber. 1927, 62, 471–546. [Google Scholar] [CrossRef]
- Mchedlov-Petrossyan, N.O. Acidic properties and structure of phthalein indicators in solution. Zhurn. Anal. Khim. (Russ. J. Anal. Chem.) 1986, 41, 1771–1779. [Google Scholar]
- Kunimoto, K.-K.; Sugiura, H.; Kato, T.; Senda, H.; Kuwae, A.; Hanai, K. Molecular structure and vibrational spectra of phenolphthalein and its dianion. Spectrochim. Acta A 2001, 57, 265–271. [Google Scholar] [CrossRef]
- Mchedlov-Petrossyan, N.O.; Ivanov, V.V. Effect of the solvent on the absorption spectra and protonation of fluorescein dye anions. Russ. J. Phys. Chem. A 2007, 81, 112–115. [Google Scholar] [CrossRef]
- Vodolazkaya, N.A.; Salamanova, N.V.; Mchedlov-Petrossyan, N.O. Protolytic equilibrium of thiofluorescein in water-organic mixtures. Ukr. Chem. J. 2008, 74, 3–8. [Google Scholar]
- Shekhovtsov, S.V.; Mchedlov-Petrossyan, N.O.; Kamneva, N.N.; Gromovoy, T.Y. New orange dyes: Nitroderivatives of sulfonefluorescein. Kharkov Univ. Bull. Chem. Ser. 2014, 24, 7–18. [Google Scholar]
- Mchedlov-Petrossyan, N.O.; Laguta, A.N.; Shekhovtsov, S.V.; Eltsov, S.V.; Cheipesh, T.A.; Omelchenko, I.V.; Shishkin, O.V. Dinitrophenolsulfonephthalein: An acid-base indicator dye with unusual properties. Coloration Technol. 2017, 133, 135–144. [Google Scholar] [CrossRef]
- Vereshchagin, A.N. Inductive Effect; Nauka: Moscow, Russian, 1987; p. 328. (In Russian) [Google Scholar]
- Hibbert, F.; Hunte, K.P.P. Rates of proton transfer for meso-tetraphenylporphyrin in 90% dimethyl sulphoxide–water (v/v). Chem. Commun. 1975, 728–729. [Google Scholar] [CrossRef]
- Hibbert, F.; Hunte, K.P.P. Kinetic and equilibrium studies of the protonation of meso-tetraphenylporphirin in dimethyl sulphoxide–water. J. Chem. Soc. Perkin Trans. II 1977, 2, 1624–1628. [Google Scholar] [CrossRef]
- Mchedlov-Petrossyan, N.O.; Romanenko, A.V.; Nikishina, L.E. Acid-base equilibria of phenolphthalein in aqueous solutions. Zhurn. Anal. Khim. (Russ. J. Anal. Chem.) 1984, 39, 1395–1403. [Google Scholar]
- Guo, Z.J.; Miyoshi, H.; Nagatani, K.; Komoyoji, T.; Haga, T.; Fujita, T. Correlation of the acid dissociation constants of some multisubstituted diphenyl- and phenylpyridylamines. J. Org. Chem. 1991, 56, 3692–3700. [Google Scholar] [CrossRef]
- Krygowski, T.M.; Wrona, P.K.; Zielkowska, U.; Reichardt, C. Empirical parameters of Lewis acidity and basicity for aqueous binary solvent mixtures. Tetrahedron 1985, 41, 4519–4527. [Google Scholar] [CrossRef]
- Kalidas, C.; Hefter, G.; Marcus, Y. Gibbs energies of transfer of cations from water to mixed aqueous organic solvents. Chem. Rev. 2000, 100, 819–852. [Google Scholar] [CrossRef]
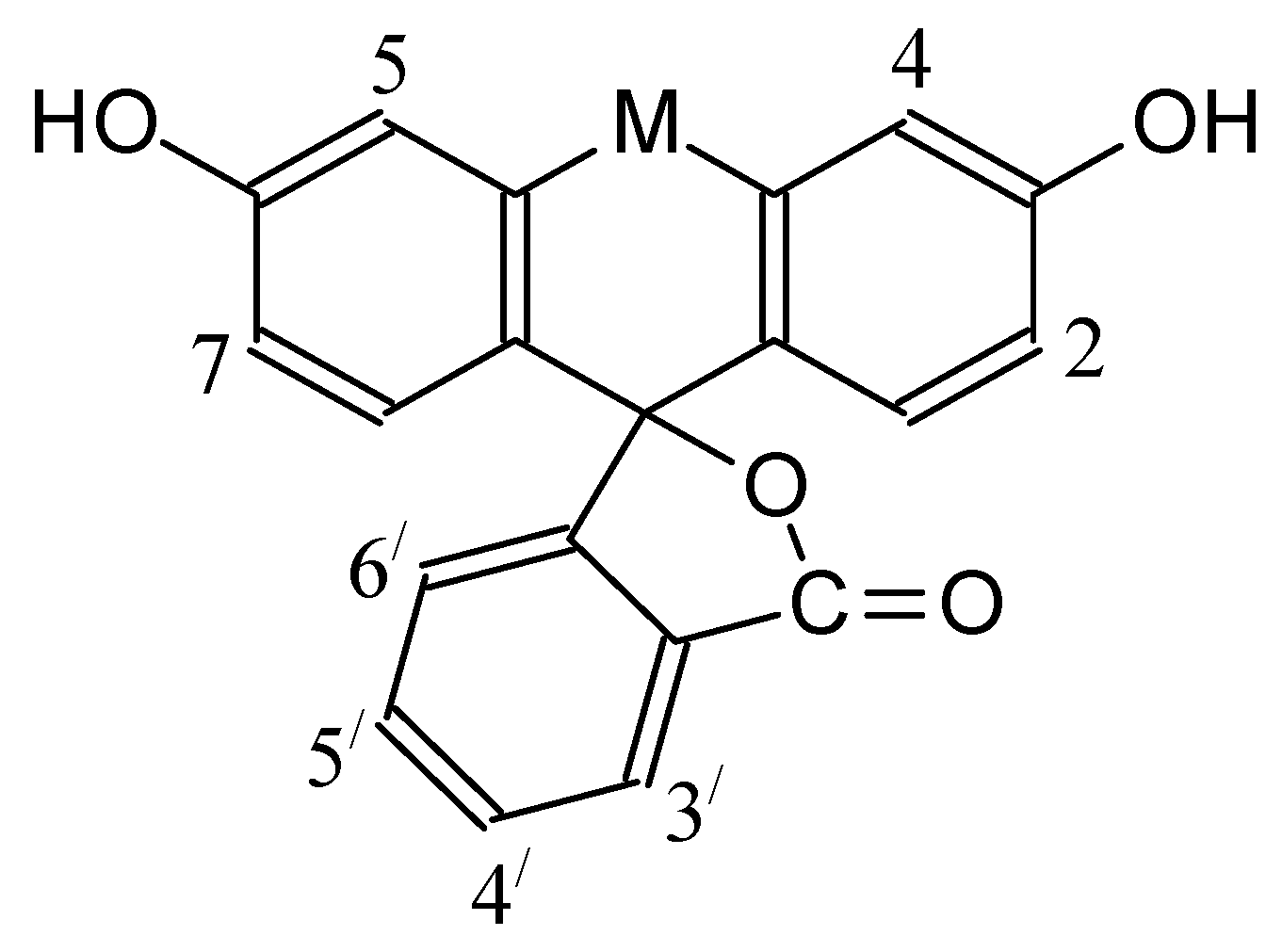
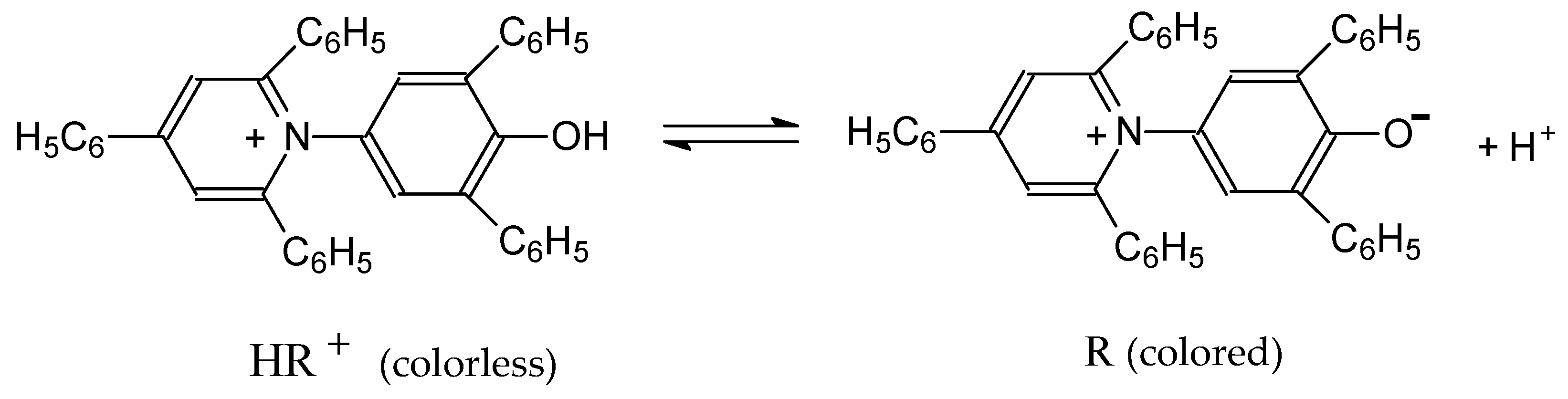


| Dye | |||
|---|---|---|---|
| Fluorescein a | 0.60 ± 0.10 | 6.41 ± 0.10 | 7.17 ± 0.06 |
| Thiofluorescein | 0.55 ± 0.09 | 7.67 ± 0.06 | 7.77 ± 0.12 |
| Sulfonefluorescein a | — | 2.33 ± 0.05 | 7.00 ± 0.01 |
| 6-Hydroxy-9-phenylfluorone | 1.98 ± 0.04 | 6.67 ± 0.03 | — |
| Ethyl fluorescein | 1.86 ± 0.02 | 6.59 ± 0.03 | — |
| n-Decyl fluorescein b | 2.13 ± 0.01 | 6.61 ± 0.07 | — |
| n-Hexadecyl fluorescein | 1.58 ± 0.04 | 7.06 ± 0.09 | — |
| 2,4,5,7-Tetrabromofluorescein (eosin) a | — | 1.83 ± 0.07 | 5.76 ± 0.06 |
| 2,4,5,7-Tetrabromothiofluorescein | — | 1.69 ± 0.02 | 6.07 ± 0.03 |
| Ethyl eosin a | — | 1.11 ± 0.03 | — |
| n-Decyl eosin b | — | 1.18 ± 0.05 | — |
| 3′,4′,5′,6′-Tetrabromofluorescein | — c | 6.66 ± 0.03 | 7.27 ± 0.02 |
| 2,7-Dichlorofluorescein a | <−0.50 d | 5.50 ± 0.05 | 5.79 ± 0.06 |
| 4,5-Dibromofluorescein | — | 5.34 ± 0.05 | 6.00 ± 0.04 |
| 4,5-Dinitrofluorescein | — | 4.04 ± 0.02 | 4.92 ± 0.03 |
| 4,5-Dinitro-N-ethylazafluorescein | — | 3.10 ± 0.01 | 5.21 ± 0.02 |
| 4,5-Dinitrothiofluorescein | — | 4.88 ± 0.02 | 4.91 ± 0.04 |
| 4-Nitrothiofluorescein | — | 5.74 ± 0.04 | 6.56 ± 0.02 |
| 4,5-Dinitro-2,7-dibromofluorescein | — | −0.4 ± 0.2 c | 4.63 ± 0.06 |
| 4,5-Dibromo-2-nitrofluorescein | — | 3.92 ± 0.02 | 5.50 ± 0.02 |
| 4,5-Dibromo-2,7-dinitrofluorescein | — | 1.83 ± 0.02 | 4.35 ± 0.02 |
| 2,4,5,7-Tetranitrofluorescein e | — | 0.16 ± 0.03 | 1.45 ± 0.02 |
| Bromophenol blue b | — | — | 3.86 ± 0.03 |
| Bromocresol green b | — | — | 5.13 ± 0.01 |
| Bromocresol purple b | — | — | 7.02 ± 0.02 |
| Bromothymol blue b | — | — | 8.26 ± 0.01 |
| Phenol red b | — | — | 8.71 ± 0.03 |
| ortho-Cresol red b | — | — | 9.46 ± 0.01 |
| meta-Cresol purple b | — | — | 9.72 ± 0.03 |
| Thymol blue b | — | 1.25 ± 0.04 | 10.47 ± 0.02 |
| 2,4-Dinitrophenol (csurf = 0.01 M) f | — | 3.57 ± 0.03 | — |
| 2,5-Dinitrophenol (csurf = 0.01 M) f | — | 4.60 ± 0.05 | — |
| 2,6-Dinitrophenol (csurf = 0.01 M) f | — | 2.51 ± 0.02 | — |
| 2,6-Dinitro-4-n-dodecylphenol g | — | 2.20 ± 0.02 | — |
| 2,6-Diphenyl-4-(2,4,6-triphenylpyridinium-1-yl) phenolate b | 8.60 ± 0.02 | — | — |
| N,N′-Di-n-octadecyl rhodamine (csurf = 0.01 M) b | 3.94 ± 0.09 | — | — |
| Dye | , nm | |
|---|---|---|
| HR | R− | |
| 6-Hydroxy-9-phenylfluorone | 465, 495 | 517 |
| Ethyl fluorescein | 465, 495 | 517 |
| n-Decyl fluorescein | 465, 490–495 | 516.5 |
| Ethyl eosin | 485.5 | 542.5 |
| HR− | R2− | |
| Fluorescein | 455, 480 | 500 |
| Sulfonefluorescein | 460, 490 | 512 |
| Thiofluorescein | 500, 520 | 524 |
| 3′,4′,5′,6′-Tetrabromofluorescein | 470, 495–500 | 526.5 |
| 2,7-Dichlorofluorescein | 525 | 515 |
| 4,5-Dibromofluorescein | 495–500, 530 | 517 |
| 2,4,5,7-Tetrabromofluorescein (eosin) | 538–540 | 526.5 |
| 2,4,5,7-Tetrabromothiofluorescein | 555 | 543 |
| 4,5-Dinitrofluorescein | 510 | 502 |
| 4,5-Dinitro-N-ethylazafluorescein | 495 | 483.5 |
| 4,5-Dinitrothiofluorescein | 535 | 522.5 |
| 4,5-Dinitro-2,7-dibromofluorescein | 525 | 511 |
| 4,5-Dibromo-2-nitrofluorescein | 535 | 527 |
| 4,5-Dibromo-2,7-dinitrofluorescein | 555 | 547 |
| 2,4,5,7-Tetranitrofluorescein | 525 | 405 |
| Type of Tautomerism. Predominating Forms of Anions | Dye | |||
|---|---|---|---|---|
| Fluorescein type | Sulfonefluorescein | 2.33 | 7.00 | 4.67 a |
| Fluorescein | 6.41 | 7.17 | 0.76 | |
| 3′,4′,5′,6′-Tetrabromofluorescein | 6.66 | 7.27 | 0.61 | |
| Thiofluorescein | 7.67 | 7.77 | 0.10 | |
| 4-Nitrothiofluorescein | 5.74 | 6.56 | 0.82 | |
| Intervening type | 2,7-Dichlorofluorescein | 5.50 | 5.79 | 0.29 |
| 4,5-Dibromofluorescein | 5.34 | 6.00 | 0.66 | |
| Eosin type | Eosin | 1.83 | 5.76 | 3.93 |
| Thioeosin | 1.69 | 6.07 | 4.38 | |
| 4,5-Dinitro-N-ethylazafluorescein | 3.10 | 5.21 | 2.11 | |
| 4,5-Dinitrofluorescein | 4.04 | 4.92 | 0.88 | |
| 4,5-Dinitrothiofluorescein | 4.88 | 4.91 | 0.03 | |
| 4,5-Dinitro-2,7-dibromofluorescein b | −0.40 | 4.63 | 5.03 | |
| Lactoid anions type | 4,5-Dinitro-2,7-dibromofluorescein b | −0.40 | 4.63 | 5.03 |
| 4,5–Dibromo–2,7–dinitrofluorescein | 1.83 | 4.35 | 2.52 | |
| 4,5–Dibromo–2–nitrofluorescein | 3.92 | 5.50 | 1.58 | |
| 2,4,5,7-Tetranitrofluorescein | 0.16 | 1.45 | 1.29 |
| Dye | |
|---|---|
| Ethyl fluorescein; n-decyl fluorescein; a 6-hydroxy-9-phenylfluorone; n-hexadecyl fluorescein | 6.59; 6.61; 6.67; 7.06 |
| 4,5-Dibromofluorescein; 2,7-dichlorofluorescein | 4.5; 4.1 |
| 4,5-Dinitrofluorescein | 2.6 |
| Eosin; b ethyl eosin b | 1.40; 1.11 |
| 4,5-Dibromo-2,7-dinitrofluorescein; 4,5-dinitro-2,7-dibromofluorescein | below 0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mchedlov-Petrossyan, N.O.; Vodolazkaya, N.A. Protolytic Equilibria in Organized Solutions: Ionization and Tautomerism of Fluorescein Dyes and Related Indicators in Cetyltrimethylammonium Chloride Micellar Solutions at High Ionic Strength of the Bulk Phase. Liquids 2021, 1, 1-24. https://doi.org/10.3390/liquids1010001
Mchedlov-Petrossyan NO, Vodolazkaya NA. Protolytic Equilibria in Organized Solutions: Ionization and Tautomerism of Fluorescein Dyes and Related Indicators in Cetyltrimethylammonium Chloride Micellar Solutions at High Ionic Strength of the Bulk Phase. Liquids. 2021; 1(1):1-24. https://doi.org/10.3390/liquids1010001
Chicago/Turabian StyleMchedlov-Petrossyan, Nikolay O., and Natalya A. Vodolazkaya. 2021. "Protolytic Equilibria in Organized Solutions: Ionization and Tautomerism of Fluorescein Dyes and Related Indicators in Cetyltrimethylammonium Chloride Micellar Solutions at High Ionic Strength of the Bulk Phase" Liquids 1, no. 1: 1-24. https://doi.org/10.3390/liquids1010001
APA StyleMchedlov-Petrossyan, N. O., & Vodolazkaya, N. A. (2021). Protolytic Equilibria in Organized Solutions: Ionization and Tautomerism of Fluorescein Dyes and Related Indicators in Cetyltrimethylammonium Chloride Micellar Solutions at High Ionic Strength of the Bulk Phase. Liquids, 1(1), 1-24. https://doi.org/10.3390/liquids1010001