Clothing Thermophysiological Comfort: A Textile Science Perspective
Abstract
:1. Introduction
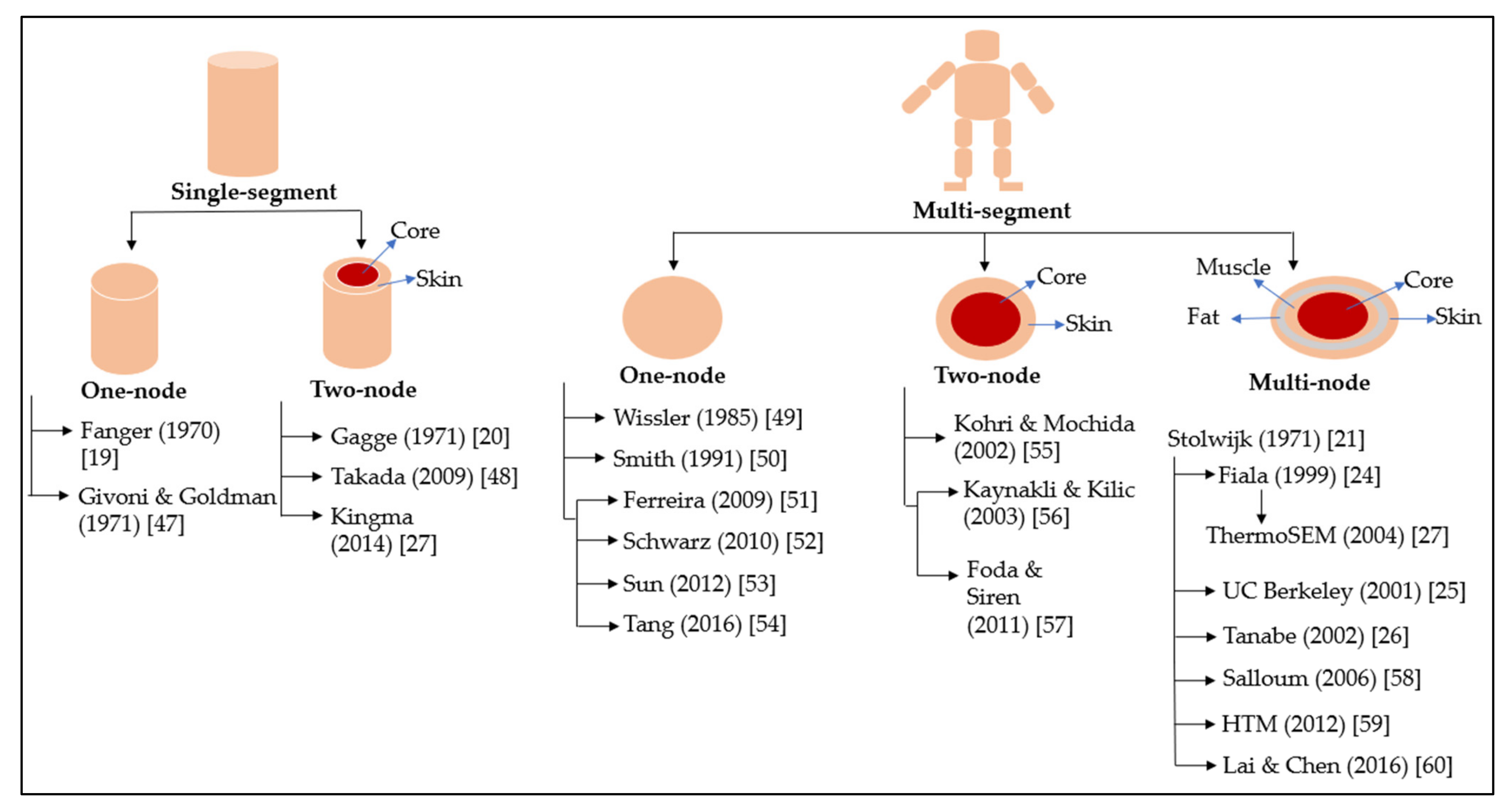
2. Thermophysiological Comfort Prediction Models
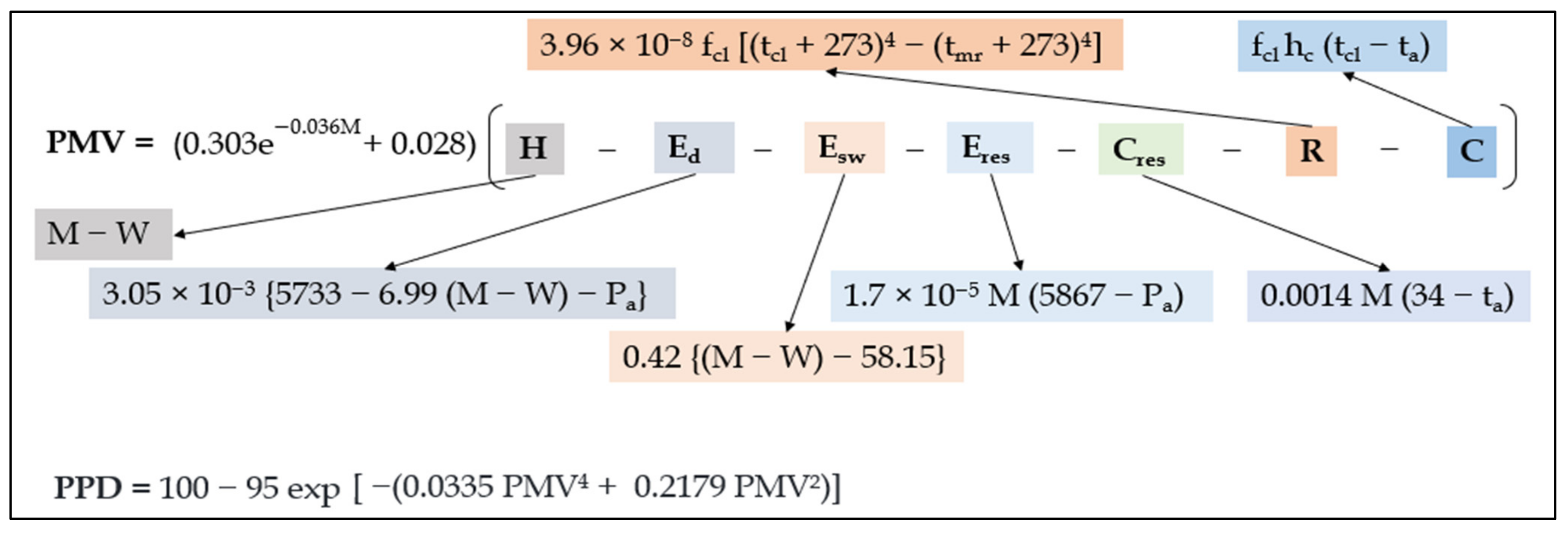
2.1. Heat Balance Equation
2.2. Thermoregulation in a Human Body
3. Physics of Heat and Moisture Transfer in the Skin–Clothing–Environment System
3.1. Heat and Sweat Generation in the Human Body
3.2. Heat and Moisture Transfer in the Skin–Clothing–Environment System
3.2.1. Sensible Heat Transfer
Sensible Heat Transfer in the Enclosed Air Layer (or Microclimate)
Sensible Heat Transfer through the Clothing Layer
Sensible Heat Transfer in the Boundary Air Layer
3.2.2. Latent Heat Transfer
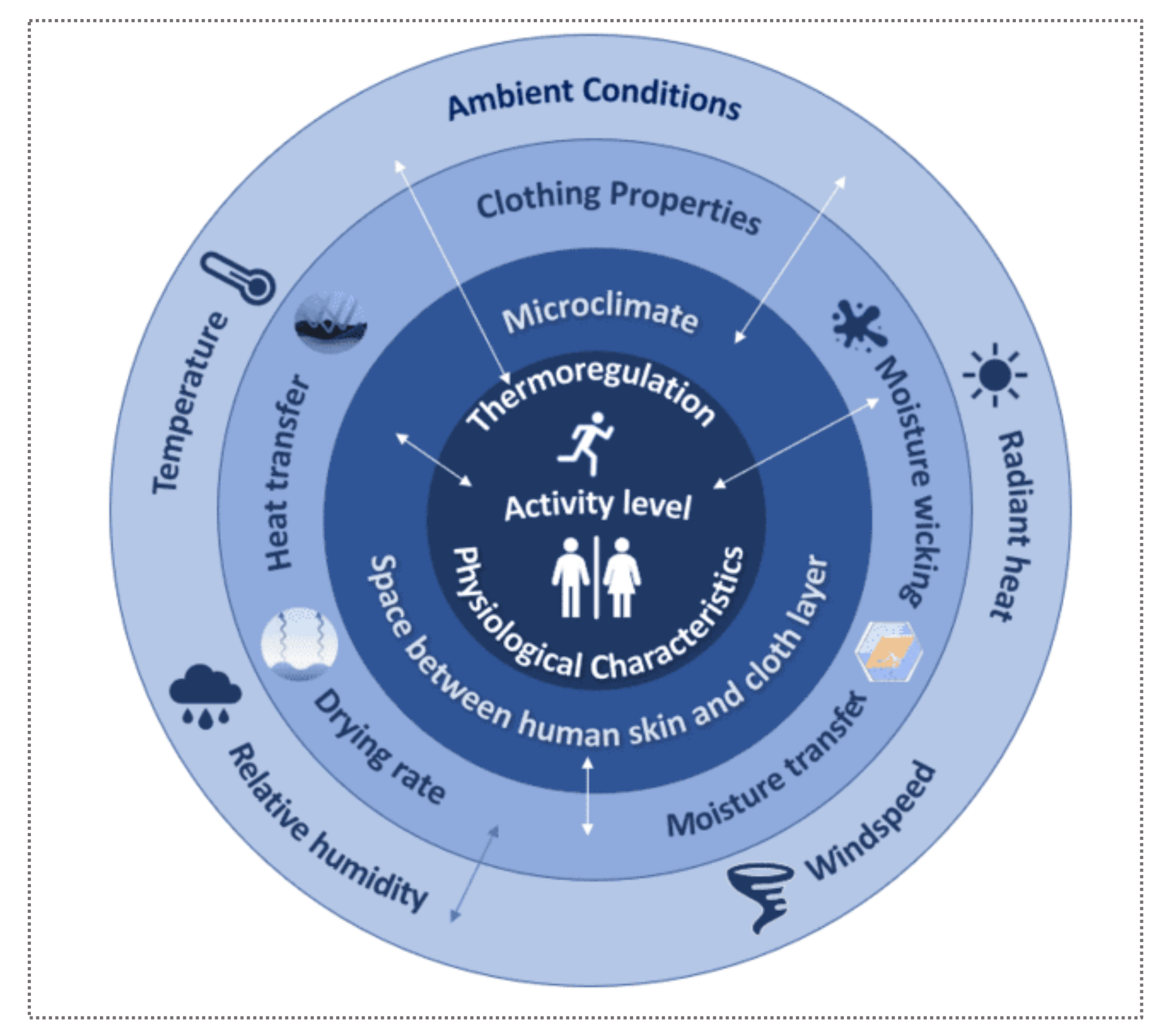
4. Factors Controlling Thermophysiological Comfort
4.1. Effect of the Physiological Characteristics and Processes
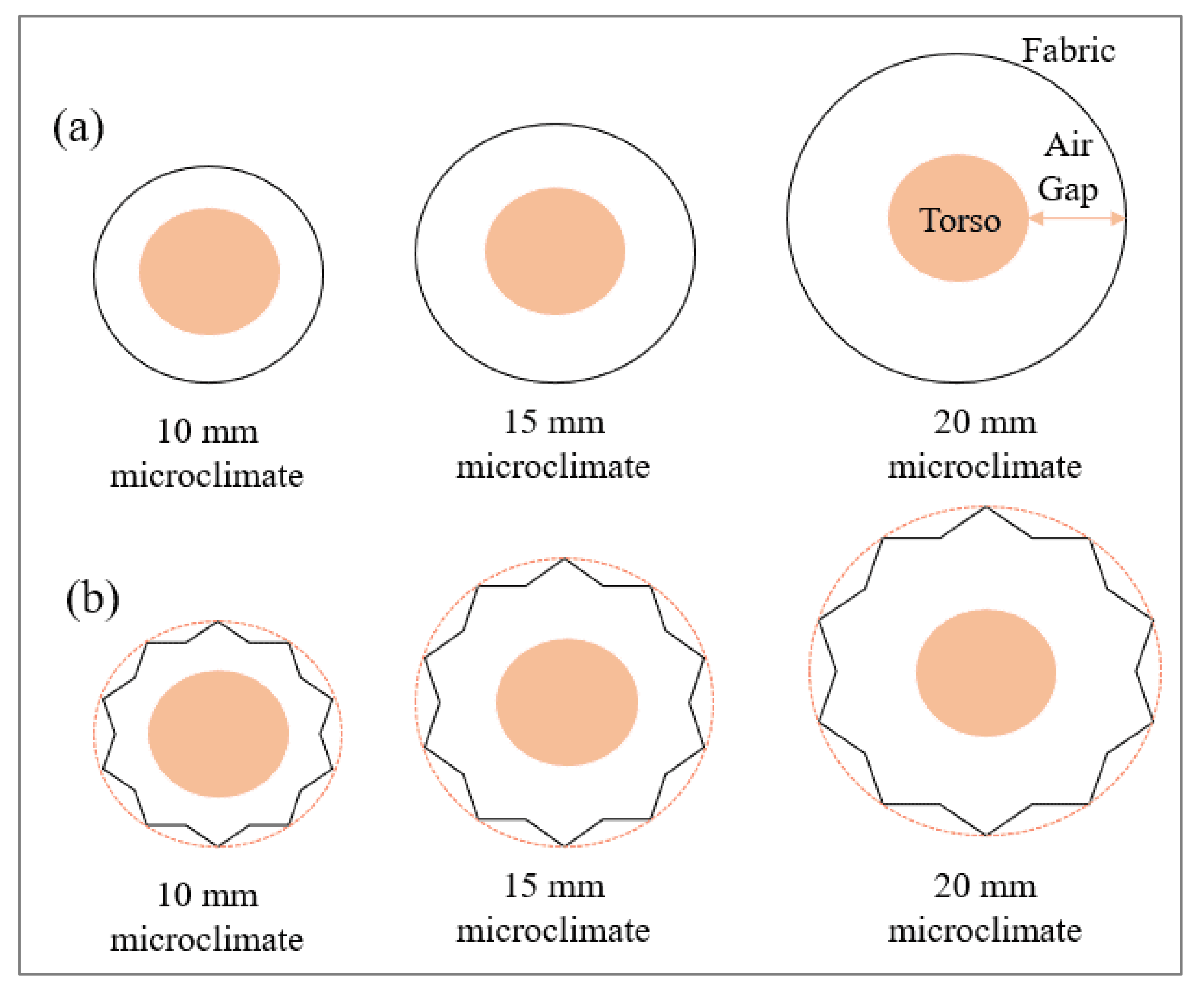
4.2. Effect of the Microclimate Layer
4.3. Effect of Clothing Properties
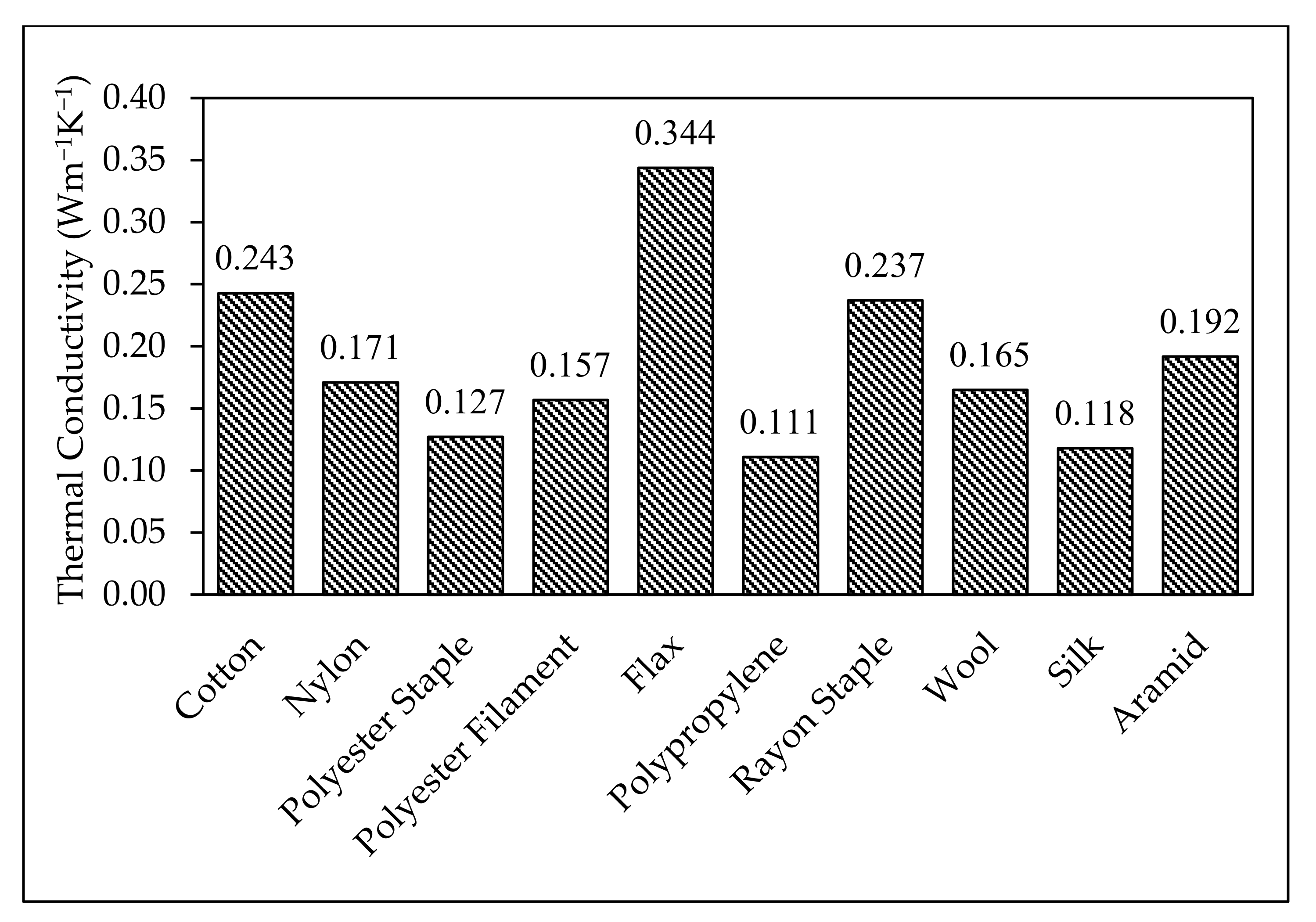
4.3.1. Effect of the Fiber Properties
4.3.2. Effect of Yarn Properties
4.3.3. Effect of Fabric Properties
4.4. Effect of Ambient Conditions
4.5. Effect of Body Movement
5. Materials and Advanced Technologies for Superior Thermophysiological Comfort
5.1. Fiber Selection and Engineering
5.2. Finishes for Improved Thermophysiological Comfort
5.3. Radiative Cooling and Heating Technologies
5.4. Smart Textiles for Superior Thermophysiological Comfort
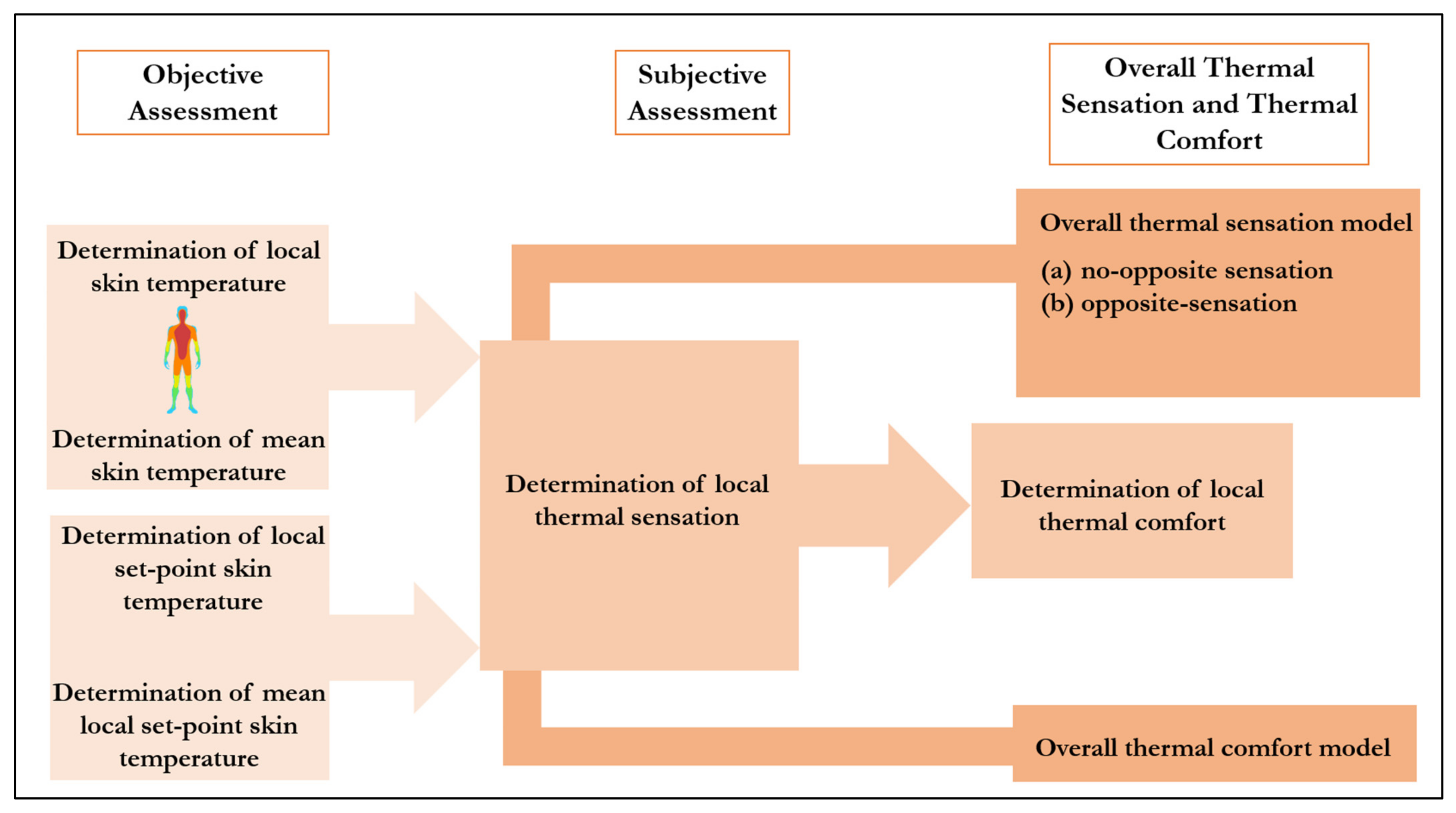
6. Human Thermophysiological Comfort Assessment
6.1. Subjective Assessment
6.2. Objective Assessment
6.3. Relationship between Subjective and Objective Assessment of Thermophysiological Comfort
7. Clothing Thermophysiological Comfort Assessment
7.1. Subjective Assessment
7.2. Objective Assessment
7.2.1. Sweating-Guarded Hot Plate
7.2.2. Thermal Sweating Manikins
7.2.3. Sweating Cylindrical Torso
- ▪
- Total supplied sweat = (sweat present on the skin + sweat absorbed by the fabric + evaporated sweat + dripped sweat) [33].
- ▪
- Skin wettedness = body surface area covered by sweat/total body surface area.
- ▪
- Sweat evaporative efficiency (ηevap) = total evaporated sweat from the skin and cloth/total supplied sweat.
8. Summary and Key Insights
8.1. Research Gaps and Future Directions
8.2. Thermophysiological Comfort Conceptual Model
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Parsons, K.C. Human Thermal Comfort; CRC Press: Boca Raton, FL, USA; Taylor & Francis Group: Abingdon, UK, 2020. [Google Scholar]
- Angelova, R.A. Textiles and Human Thermophysiological Comfort in the Indoor Environment; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar] [CrossRef]
- Ding, D.; Tang, T.; Song, G.; McDonald, A. Characterizing the Performance of a Single-Layer Fabric System through a Heat and Mass Transfer Model—Part I: Heat and Mass Transfer Model. Text. Res. J. 2011, 81, 398–411. [Google Scholar] [CrossRef]
- Joshi, A.; Wang, F.; Kang, Z.; Yang, B.; Zhao, D. A Three-Dimensional Thermoregulatory Model for Predicting Human Thermophysiological Responses in Various Thermal Environments. Build. Environ. 2022, 207, 108506. [Google Scholar] [CrossRef]
- Havenith, G.; Fiala, D. Thermal Indices and Thermophysiological Modeling for Heat Stress. Compr. Physiol. 2015, 6, 255–302. [Google Scholar] [PubMed]
- Havenith, G. Heat Balance When Wearing Protective Clothing. Ann. Occup. Hyg. 1999, 43, 296–298. [Google Scholar] [CrossRef]
- Holmér, I. Protective Clothing in Hot Environments. Ind. Health 2006, 44, 404–413. [Google Scholar] [CrossRef]
- Havenith, G.; Hartog, E.A.D.; Martini, S. Heat Stress in Chemical Protective Clothing: Porosity and Vapour Resistance. Ergonomics 2011, 54, 497–507. [Google Scholar] [CrossRef]
- Holmér, I. Protective Clothing and Heat Stress. Ergonomics 1995, 38, 166–182. [Google Scholar] [CrossRef]
- Parsons, K.C. Human Thermal Environments; Taylor & Francis: Abingdon, UK, 2002. [Google Scholar]
- Song, G. Improving Comfort in Clothing; Woodhead Publishing Limited: Cambridge, UK, 2011. [Google Scholar]
- Psikuta, A.; Allegrini, J.; Koelblen, B.; Bogdan, A.; Annaheim, S.; Martínez, N.; Derome, D.; Carmeliet, J.; Rossi, R.M. Thermal Manikins Controlled by Human Thermoregulation Models for Energy Efficiency and Thermal Comfort Research—A Review. Renew. Sustain. Energy Rev. 2017, 78, 1315–1330. [Google Scholar] [CrossRef]
- Alba, B.K.; Castellani, J.W.; Charkoudian, N. Cold-induced Cutaneous Vasoconstriction in Humans: Function, Dysfunction and the Distinctly Counterproductive. Exp. Physiol. 2019, 104, 1202–1214. [Google Scholar] [CrossRef]
- Haman, F.; Blondin, D.P. Shivering Thermogenesis in Humans: Origin, Contribution and Metabolic Requirement. Temperature 2017, 4, 217–226. [Google Scholar] [CrossRef]
- Luginbuehl, I.; Bissonnette, B. Thermal Regulation. In Elsevier eBooks; Saunders Elsevier: Philadelphia, PA, USA, 2009; pp. 557–567. [Google Scholar] [CrossRef]
- Chaplin, G.; Jablonski, N.G.; Sussman, R.W.; Kelley, E.A. The Role of Piloerection in Primate Thermoregulation. Folia Primatol. 2013, 85, 1–17. [Google Scholar] [CrossRef] [PubMed]
- Pisacane, V.L.; Kuznetz, L.H.; Logan, J.S.; Clark, J.B.; Wissler, E.H. Thermoregulatory Models of Space Shuttle and Space Station Activities. Aviat. Space Environ. Med. 2007, 78 (Suppl. 4), A48–A55. [Google Scholar]
- Katić, K.; Li, R.; Zeiler, W. Thermophysiological Models and Their Applications: A Review. Build. Environ. 2016, 106, 286–300. [Google Scholar] [CrossRef]
- Fanger, P.O. Thermal Comfort: Analysis and Applications in Environmental Engineering; Danish Technical Press: Copenhagen, Denmark, 1970. [Google Scholar]
- Gagge, A.P.; Stowijk, J.A.J.; Nishi, Y. An Effective Temperature Scale Based on a Simple Model of Human Physiological Regulatory Response. ASHRAE Trans. 1971, 77, 247–262. [Google Scholar]
- Stolwijk, J.A. A Mathematical Model of Physiological Temperature Regulation in Man; National Aeronautics and Space Administration: Washington, DC, USA, 1971. [Google Scholar]
- Guedes, J.C. Mathematical Modelling of Human Thermoregulation in Hot Environments, University of Porto. 2016. Available online: https://core.ac.uk/download/pdf/302916501.pdf (accessed on 7 June 2023).
- Basdanis, T.; Tatsios, G.; Valougeorgis, D. Human Thermophysiological Models: Quantification of Uncertainty in the Output Quantities of the Passive System Due to Uncertainties in the Control Equations of the Active System via the Monte Carlo Method. J. Therm. Biol. 2021, 100, 103045. [Google Scholar] [CrossRef]
- Fiala, D.; Lomas, K.J.; Stohrer, M. A Computer Model of Human Thermoregulation for a Wide Range of Environmental Conditions: The Passive System. J. Appl. Physiol. 1999, 87, 1957–1972. [Google Scholar] [CrossRef]
- Huizenga, C.; Hui, Z.; Arens, E. A Model of Human Physiology and Comfort for Assessing Complex Thermal Environments. Build. Environ. 2001, 36, 691–699. [Google Scholar] [CrossRef]
- Tanabe, S.; Kobayashi, K.; Nakano, J.; Ozeki, Y.; Konishi, M. Evaluation of Thermal Comfort Using Combined Multi-Node Thermoregulation (65MN) and Radiation Models and Computational Fluid Dynamics (CFD). Energy Build. 2002, 34, 637–646. [Google Scholar] [CrossRef]
- Kingma, B.R.M.; Vosselman, M.J.; Frijns, A.J.H.; van Steenhoven, A.A.; van Marken Lichtenbelt, W.D. Incorporating Neurophysiological Concepts in Mathematical Thermoregulation Models. Int. J. Biometeorol. 2014, 58, 87–99. [Google Scholar] [CrossRef]
- Joshi, A. Comprehensive Model for Heat and Mass Transfer in Human Skin-Clothing-Environment System; Université de Haute-Alsace: Mulhouse, France, 2019. [Google Scholar]
- Psikuta, A.; Richards, M.; Fiala, D. Single-Sector Thermophysiological Human Simulator. Physiol. Meas. 2008, 29, 181–192. [Google Scholar] [CrossRef]
- ASHRAE 55; Thermal Environmental Conditions for Human Occupancy. American Society of Heating Refrigerating and Air-Conditioning Engineers: Atlanta, GA, USA, 2017.
- ISO 7730; Ergonomics of the Thermal Environment—Analytical Determination and Interpretation of Thermal Comfort Using Calculation of the PMV and PPD Indices and Local Thermal Comfort Criteria. International Organization for Standardization: Geneva, Switzerland, 2005.
- ISO 18640-1; Protective Clothing for Firefighters—Physiological Impact—Part 1: Measurement of Coupled Heat and Moisture Transfer with the Sweating Torso. International Organization for Standardization: Geneva, Switzerland, 2018.
- Guan, M.; Annaheim, S.; Camenzind, M.; Li, J.; Mandal, S.; Psikuta, A.; Rossi, R.M. Moisture Transfer of the Clothing–Human Body System during Continuous Sweating under Radiant Heat. Text. Res. J. 2019, 89, 4537–4553. [Google Scholar] [CrossRef]
- Guthrie, J.C. The Integral and Differential Heats of Sorption of Water by Cellulose. J. Text. Inst. Trans. 1949, 40, T489–T504. [Google Scholar] [CrossRef]
- Kim, H.-A. Moisture Vapor Permeability and Thermal Wear Comfort of Ecofriendly Fiber-Embedded Woven Fabrics for High-Performance Clothing. Materials 2021, 14, 6205. [Google Scholar] [CrossRef] [PubMed]
- Morton, W.; Hearle, J.S. Physical Properties of Textile Fibres; Heinemann: London, UK, 2008. [Google Scholar]
- Noman, M.T.; Petru, M. Effect of Sonication and Nano TiO 2 on Thermophysiological Comfort Properties of Woven Fabrics. ACS Omega 2020, 5, 11481–11490. [Google Scholar] [CrossRef]
- Hes, L. Non-Destructive Determination of Comfort Parameters during Marketing of Functional Garments and Clothing. Indian J. Fibre Text. Res. 2008, 33, 239–245. [Google Scholar]
- Croitoru, C.; Nastase, I.; Bode, F.; Meslem, A.; Dogeanu, A. Thermal Comfort Models for Indoor Spaces and Vehicles—Current Capabilities and Future Perspectives. Renew. Sustain. Energy Rev. 2015, 44, 304–318. [Google Scholar] [CrossRef]
- ISO 14505-3; Ergonomics of the Thermal Environment—Evaluation of Thermal Environments in Vehicles—Part 3: Evaluation of Thermal Comfort Using Human Subjects. International Organization for Standardization: Geneva, Switzerland, 2006.
- ISO 9886; Ergonomics-Evaluation of Thermal Strain by Physiological Measurements. International Organization for Standardization: Geneva, Switzerland, 2004.
- ISO 11092; Textiles—Physiological Effects—Measurement of Thermal and Water Vapour Resistance under Steady-State Conditions (Sweating Guarded Hotplate Test. International Organization for Standardization: Geneva, Switzerland, 2014.
- ASTM E96/E96M; Standard Test Methods for Water Vapor Transmission of Materials. ASTM International: London, UK, 2017.
- AATCC 195; Test Method for Liquid Moisture Management Properties of Textile Fabrics. American Association of Textile Chemists and Colorists: Research Triangle Park, NC, USA, 2017.
- ASTM D737; Standard Test Method for Air Permeability of Textile Fabrics. ASTM International: London, UK, 2018.
- ASTM D7984; Standard Test Method for Measurement of Thermal Effusivity of Fabrics Using a Modified Transient Plane Source (MTPS) Instrument. ASTM International: London, UK, 2021.
- Givoni, B.; Goldman, R.F. Predicting Metabolic Energy Cost. J. Appl. Physiol. 1971, 30, 429–433. [Google Scholar] [CrossRef]
- Takada, S.; Kobayashi, H.; Matsushita, T. Thermal Model of Human Body Fitted with Individual Characteristics of Body Temperature Regulation. Build. Environ. 2009, 44, 463–470. [Google Scholar] [CrossRef]
- Wissler, E.H. Heat Transfer in Medicine and Biology; Shitzer, A., Eberhart, R.C., Eds.; Plenum Press: New York, USA, 1985; Volume 1, pp. 325–373. [Google Scholar]
- Smith, C.E. A Transient, Three-Dimensional Model of the Human Thermal System; Kansas State University: Kansas, NY, USA, 1991. [Google Scholar]
- Ferreira, M.S.; Yanagihara, J.I. A Transient Three-Dimensional Heat Transfer Model of the Human Body. Int. Commun. Heat Mass Transf. 2009, 36, 718–724. [Google Scholar] [CrossRef]
- Schwarz, M.; Krueger, M.W.; Busch, H.-J.; Benk, C.; Heilmann, C. Model-Based Assessment of Tissue Perfusion and Temperature in Deep Hypothermic Patients. IEEE Trans. Biomed. Eng. 2010, 57, 1577–1586. [Google Scholar] [CrossRef]
- Sun, X.; Eckels, S.J.; Zheng, Z.C. An Improved Thermal Model of the Human Body. Hvac Res. 2012, 18, 323–338. [Google Scholar] [CrossRef]
- Tang, Y.; He, Y.; Shao, H.; Ji, C. Assessment of Comfortable Clothing Thermal Resistance Using a Multi-Scale Human Thermoregulatory Model. Int. J. Heat Mass Transf. 2016, 98, 568–583. [Google Scholar] [CrossRef]
- Kohri, I.; Mochida, T. Evaluation Method of Thermal Comfort in a Vehicle with a Dispersed Two-Node Model Part 1—Development of Dispersed Two-Node Model. J. Human Environ. Syst. 2002, 6, 19–29. [Google Scholar] [CrossRef]
- Kaynakli, O.; Unver, U.; Kilic, M. Evaluating Thermal Environments for Sitting and Standing Posture. Int. Commun. Heat Mass Transf. 2003, 30, 1179–1188. [Google Scholar] [CrossRef]
- Foda, E.; Sirén, K. A New Approach Using the Pierce Two-Node Model for Different Body Parts. Int. J. Biometeorol. 2010, 55, 519–532. [Google Scholar] [CrossRef]
- Salloum, M.; Ghaddar, N.; Ghali, K. A New Transient Bioheat Model of the Human Body and Its Integration to Clothing Models. Int. J. Therm. Sci. 2007, 46, 371–384. [Google Scholar] [CrossRef]
- Dongmei, P.; Mingyin, C.; Shiming, D.; Minglu, Q. A Four-Node Thermoregulation Model for Predicting the Thermal Physiological Responses of a Sleeping Person. Build. Environ. 2012, 52, 88–97. [Google Scholar] [CrossRef]
- Lai, D.; Chen, Q. A Two-Dimensional Model for Calculating Heat Transfer in the Human Body in a Transient and Non-Uniform Thermal Environment. Energy Build. 2016, 118, 114–122. [Google Scholar] [CrossRef]
- Fanger, P.O. Assessment of Man’s Thermal Comfort in Practice. Occup. Environ. Med. 1973, 30, 313–324. [Google Scholar] [CrossRef]
- Kang, Z.; Wang, F.; Udayraj. An Advanced Three-Dimensional Thermoregulation Model of the Human Body: Development and Validation. Int. Commun. Heat Mass Transf. 2019, 107, 34–43. [Google Scholar] [CrossRef]
- Broday, E.; de Paula Xavier, A.; de Oliveira, R. Comparative Analysis of Methods for Determining the Clothing Surface Temperature (TCL) in Order to Provide a Balance between Man and the Environment. Int. J. Ind. Ergon. 2017, 57, 80–87. [Google Scholar] [CrossRef]
- Fiala, D.; Lomas, K.J.; Stohrer, M. Computer Prediction of Human Thermoregulatory and Temperature Responses to a Wide Range of Environmental Conditions. Int. J. Biometeorol. 2001, 45, 143–159. [Google Scholar] [CrossRef] [PubMed]
- Mert, E.; Psikuta, A.; Bueno, M.-A.; Rossi, R.M. Effect of Heterogenous and Homogenous Air Gaps on Dry Heat Loss through the Garment. Int. J. Biometeorol. 2015, 59, 1701–1710. [Google Scholar] [CrossRef]
- Awais, M.; Krzywinski, S.; Wendt, E. A Novel Modeling and Simulation Approach for the Prediction of Human Thermophysiological Comfort. Text. Res. J. 2021, 91, 691–705. [Google Scholar] [CrossRef]
- Gagge, A.P.; Burton, A.C.; Bazett, H.C. A Practical System of Units for the Description of the Heat Exchange of Man with His Environment. Science 1941, 94, 428–430. [Google Scholar] [CrossRef] [PubMed]
- Joshi, A.; Psikuta, A.; Bueno, M.-A.; Annaheim, S.; Rossi, R.M. Analytical Clothing Model for Sensible Heat Transfer Considering Spatial Heterogeneity. Int. J. Therm. Sci. 2019, 145, 105949. [Google Scholar] [CrossRef]
- Ekici, C. A Review of Thermal Comfort and Method of Using Fanger’s PMV Equation. In 5th International Symposium on Measurement, Analysis and Modeling of Human Functions; Curran Associates Inc.: Vancouver, BC, Canada, 2003. [Google Scholar]
- Krucińska, I.; Skrzetuska, E.; Kowalski, K. Application of a Thermal Mannequin to the Assessment of the Heat Insulating Power of Protective Garments for Premature Babies. Autex Res. J. 2019, 19, 134–146. [Google Scholar] [CrossRef]
- Crandall, C.G. Mechanisms and Controllers of Eccrine Sweating in Humans. Front. Biosci. 2010, S2, 94. [Google Scholar] [CrossRef]
- Eyolfson, D.A.; Tikuisis, P.; Xu, X.; Weseen, G.; Giesbrecht, G.G. Measurement and Prediction of Peak Shivering Intensity in Humans. Eur. J. Appl. Physiol. 2001, 84, 100–106. [Google Scholar] [CrossRef]
- Bergman, T.L.; Lavine, A.S.; Incropera, F.P.; DeWitt, D.P. Introduction to Heat and Mass Transfer; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Aziz, K.; Holst, P.H.; Karra, P.S. Natural Convection In Porous Media. In Proceedings of the 19th Annual Technical Meeting of the Petroleum Society of Canada, Petroleum Society of Canada. Calgary, AB, Canada, 8 May 1968. [Google Scholar]
- Çengel, Y.A.; Ghajar, A.J. Heat Transfer and Mass Transfer: Fundamentals and Applications; McGraw-Hill Higher Education: New York, USA, 2015. [Google Scholar]
- Hu, Y.; Topolkaraev, V.; Hiltner, A.; Baer, E. Measurement of Water Vapor Transmission Rate in Highly Permeable Films. J. Appl. Polym. Sci. 2001, 81, 1624–1633. [Google Scholar] [CrossRef]
- Schweiker, M.; Huebner, G.M.; Kingma, B.R.M.; Kramer, R.; Pallubinsky, H. Drivers of Diversity in Human Thermal Perception—A Review for Holistic Comfort Models. Temperature 2018, 5, 308–342. [Google Scholar] [CrossRef] [PubMed]
- Rewitz, K.; Müller, D. Influence of Gender, Age and BMI on Human Physiological Response and Thermal Sensation for Transient Indoor Environments with Displacement Ventilation. Build. Environ. 2022, 219, 109045. [Google Scholar] [CrossRef]
- Van Someren, E.J.W. Thermoregulation and Aging. Am. J. Physiol. Regul. Integr. Comp. Physiol. 2007, 292, R99–R102. [Google Scholar] [CrossRef] [PubMed]
- Blatteis, C.M. Age-Dependent Changes in Temperature Regulation–A Mini Review. Gerontology 2012, 58, 289–295. [Google Scholar] [CrossRef] [PubMed]
- van Hoof, J. Forty Years of Fanger’s Model of Thermal Comfort: Comfort for All? Indoor Air 2008, 18, 182–201. [Google Scholar] [CrossRef] [PubMed]
- Kingma, B.; van Marken Lichtenbelt, W. Energy Consumption in Buildings and Female Thermal Demand. Nat. Clim. Chang. 2015, 5, 1054–1056. [Google Scholar] [CrossRef]
- Rupp, R.F.; Vásquez, N.G.; Lamberts, R. A Review of Human Thermal Comfort in the Built Environment. Energy Build. 2015, 105, 178–205. [Google Scholar] [CrossRef]
- Rennie, D.W. Tissue Heat Transfer in Water: Lessons from the Korean Divers. Med. Sci. Sports Exerc. 1988, 20 (Suppl. S1), S177–S184. [Google Scholar] [CrossRef]
- Speakman, J.R. Obesity and Thermoregulation. Handb. Clin. Neurol. 2018, 156, 431–443. [Google Scholar] [CrossRef]
- Ding, D.; Tian Tang, T.; Song, G.; McDonald, A. Characterizing the Performance of a Single-Layer Fabric System through a Heat and Mass Transfer Model—Part II: Thermal and Evaporative Resistances. Text. Res. J. 2011, 81, 945–958. [Google Scholar] [CrossRef]
- Santos, M.S.; Oliveira, D.; Campos, J.B.L.M.; Mayor, T.S. Numerical Analysis of the Flow and Heat Transfer in Cylindrical Clothing Microclimates–Influence of the Microclimate Thickness Ratio. Int. J. Heat Mass Transf. 2018, 117, 71–79. [Google Scholar] [CrossRef]
- Belal, S.A. Understanding Textiles for a Merchandiser; BMN3 Foundation: Dhaka, Bangladesh, 2009. [Google Scholar]
- Hatch, K.L. Textile Science; West Publishing Co.: St Paul, MN, USA, 1993. [Google Scholar]
- Cimilli, S.; Nergis, B.U.; Candan, C.; Özdemir, M. A Comparative Study of Some Comfort-Related Properties of Socks of Different Fiber Types. Text. Res. J. 2010, 80, 948–957. [Google Scholar] [CrossRef]
- Aisyah, H.A.; Paridah, M.T.; Sapuan, S.M.; Ilyas, R.A.; Khalina, A.; Nurazzi, N.M.; Lee, S.H.; Lee, C.H. A Comprehensive Review on Advanced Sustainable Woven Natural Fibre Polymer Composites. Polymers 2021, 13, 471. [Google Scholar] [CrossRef] [PubMed]
- Özdil, N.; Marmaralı, A.; Kretzschmar, S.D. Effect of Yarn Properties on Thermal Comfort of Knitted Fabrics. Int. J. Therm. Sci. 2007, 46, 1318–1322. [Google Scholar] [CrossRef]
- Aydın, İ.S.; Kertmen, M.; Marmarali, A. Evaluating the Effect of Spinning Systems on Thermal Comfort Properties of Modal Fabrics. IOP Conf. Ser. Mater. Sci. Eng. 2017, 254, 182011. [Google Scholar] [CrossRef]
- Spencer, D.J. Knitting Technology: A Comprehensive Handbook and Practical Guide; Woodhead Publishing: Sawston, UK, 2001. [Google Scholar]
- Ahmad, S.; Ahmad, F.; Afzal, A.; Rasheed, A.; Mohsin, M.; Ahmad, N. Effect of Weave Structure on Thermo-Physiological Properties of Cotton Fabrics. Autex Res. J. 2015, 15, 30–34. [Google Scholar] [CrossRef]
- Fourt, L.; Harrist, M. Diffusion of Water Vapor Through Textiles. Text. Res. J. 1947, 17, 256–263. [Google Scholar] [CrossRef]
- Badr, A.A.; Hassanin, A.; Moursey, M. Influence of Tencel/Cotton Blends on Knitted Fabric Performance. Alex. Eng. J. 2016, 55, 2439–2447. [Google Scholar] [CrossRef]
- Ward, K. Crystallinity of Cellulose and Its Significance for the Fiber Properties. Text. Res. J. 1950, 20, 363–372. [Google Scholar] [CrossRef]
- Kim, J.O.; Spivak, S.M. Dynamic Moisture Vapor Transfer Through Textiles. Text. Res. J. 1994, 64, 112–121. [Google Scholar] [CrossRef]
- Li, Y.; Holcombe, B.V.; Apcar, F. Moisture Buffering Behavior of Hygroscopic Fabric During Wear. Text. Res. J. 1992, 62, 619–627. [Google Scholar] [CrossRef]
- Trifol, J.; Plackett, D.; Szabo, P.; Daugaard, A.E.; Giacinti Baschetti, M. Effect of Crystallinity on Water Vapor Sorption, Diffusion, and Permeation of PLA-Based Nanocomposites. ACS Omega 2020, 5, 15362–15369. [Google Scholar] [CrossRef]
- Duan, Z.; Thomas, N.L. Water Vapour Permeability of Poly(Lactic Acid): Crystallinity and the Tortuous Path Model. J. Appl. Phys. 2014, 115, 064903. [Google Scholar] [CrossRef]
- Kwon, Y.-J.; Park, J.-B.; Jeon, Y.-P.; Hong, J.-Y.; Park, H.-S.; Lee, J.-U. A Review of Polymer Composites Based on Carbon Fillers for Thermal Management Applications: Design, Preparation, and Properties. Polymers 2021, 13, 1312. [Google Scholar] [CrossRef] [PubMed]
- Feng, T.; He, J.; Rai, A.; Hun, D.; Liu, J.; Shrestha, S.S. Size Effects in the Thermal Conductivity of Amorphous Polymers. Phys. Rev. Appl. 2020, 14, 044023. [Google Scholar] [CrossRef]
- Bui, H.; Sebaibi, N.; Boutouil, M.; Levacher, D. Determination and Review of Physical and Mechanical Properties of Raw and Treated Coconut Fibers for Their Recycling in Construction Materials. Fibers 2020, 8, 37. [Google Scholar] [CrossRef]
- Zhang, C.; Wang, F. Comfort Management of Fibrous Materials. In Handbook of Fibrous Materials; Wiley: Hoboken, NJ, USA, 2020; pp. 857–887. [Google Scholar] [CrossRef]
- Szurgot, M. On the Specific Heat Capacity and Thermal Capacity of Meteorites. In Proceedings of the 42nd Lunar and Planetary Science Conference, The Woodlands, TX, USA, 7–11 March 2011. [Google Scholar]
- Tyagi, G.; Madhusoodhanan, P. Effect of Fibre Cross-Sectional Shape on Handle Characteristics of Polyester-Viscose and Polyester-Cotton Ring and MJS Yarn Fabrics. Indian J. Fibre Text. Res. (IJFTR) 2006, 31, 496–500. [Google Scholar]
- Ravandi, S.A.H.; Valizadeh, M. Properties of Fibers and Fabrics That Contribute to Human Comfort. In Improving Comfort in Clothing; Elsevier: Amsterdam, The Netherlands, 2011; pp. 61–78. [Google Scholar] [CrossRef]
- Guan, M.; Psikuta, A.; Camenzind, M.; Li, J.; Mandal, S.; Michel Rossi, R.; Annaheim, S. Effect of Perspired Moisture and Material Properties on Evaporative Cooling and Thermal Protection of the Clothed Human Body Exposed to Radiant Heat. Text. Res. J. 2019, 89, 3663–3676. [Google Scholar] [CrossRef]
- Atasağun, H.G.; Okur, A.; Psikuta, A.; Rossi, R.M.; Annaheim, S. Determination of the Effect of Fabric Properties on the Coupled Heat and Moisture Transport of Underwear–Shirt Fabric Combinations. Text. Res. J. 2018, 88, 1319–1331. [Google Scholar] [CrossRef]
- Hussain, U.; Younis, F.B.; Usman, F.; Hussain, T.; Ahmed, F. Comfort and Mechanical Properties of Polyester/Bamboo and Polyester/Cotton Blended Knitted Fabric. J. Eng. Fibers Fabr. 2015, 10, 155892501501000. [Google Scholar] [CrossRef]
- Prakash, C.; Ramakrishnan, G. Effect of Blend Proportion on Thermal Behaviour of Bamboo Knitted Fabrics. J. Text. Inst. 2013, 104, 907–913. [Google Scholar] [CrossRef]
- Atalie, D.; Tesema, A.F.; Rotich, G.K. Effect of Weft Yarn Twist Level on Thermal Comfort of 100 per Cent Cotton Woven Fabrics. Res. J. Text. Appar. 2018, 22, 180–194. [Google Scholar] [CrossRef]
- Majumdar, P.K. Process Control in Ring and Rotor Spinning. In Process Control in Textile Manufacturing; Elsevier: Amsterdam, The Netherlands, 2013; pp. 191–224. [Google Scholar] [CrossRef]
- Basu, A. Progress in air-jet spinning. Text. Prog. 1999, 29, 1–38. [Google Scholar] [CrossRef]
- Das, A.; Alagirusamy, R. Dynamic Heat and Mass Transmission. In Science in Clothing Comfort; Woodhead Publishing India Pvt Limited: New Delhi, India, 2010; pp. 136–138. [Google Scholar] [CrossRef]
- Stoffberg, M.E.; Hunter, L.; Botha, A. The Effect of Fabric Structural Parameters and Fiber Type on the Comfort-Related Properties of Commercial Apparel Fabrics. J. Nat. Fibers 2015, 12, 505–517. [Google Scholar] [CrossRef]
- Dalbaşı, E.S.; Süpüren Mengüç, G.; Özgüney, A.T.; Özdil, N. The Effect of Softeners on Thermal Comfort and Wetting Properties of Bamboo Viscose and Bamboo/Cotton Blended Fabrics. J. Nat. Fibers 2022, 19, 1700–1714. [Google Scholar] [CrossRef]
- Dias, T.; Delkumburewatte, G.B. Changing Porosity of Knitted Structures by Changing Tightness. Fibers Polym. 2008, 9, 76–79. [Google Scholar] [CrossRef]
- Bibi, N.; Abdullah Makhdoom, M.U. Prediction of Fabric Porosity from Yarn Diameter and Effect of Different Spinning Techniques and Process on Porosity. Int. J. Sci. Res. Publ. (IJSRP) 2021, 11, 699–706. [Google Scholar] [CrossRef]
- Hoque, M.S.; Hossain, M.J.; Rahman, M.M.; Rashid, M.M. Fiber Types and Fabric Structures Influence on Weft Knitted Fabrics. Heliyon 2022, 8, e09605. [Google Scholar] [CrossRef] [PubMed]
- Chowdhary, U.; Islam, M.R. Pre-Post Wash Wicking Behavior, Moisture Transfer, and Water Repellency of Plain, Twill and Satin Weaves. J. Text. Sci. Fash. Technol. 2019, 2, 1–13. [Google Scholar] [CrossRef]
- Limeneh, D.Y.; Ayele, M.; Tesfaye, T.; Liyew, E.Z.; Tesema, A.F. Effect of Weave Structure on Comfort Property of Fabric. J. Nat. Fibers 2020, 19, 4148–4155. [Google Scholar] [CrossRef]
- Tabor, J.; Chatterjee, K.; Ghosh, T.K. Smart Textile-Based Personal Thermal Comfort Systems: Current Status and Potential Solutions. Adv. Mater. Technol. 2020, 5, 1901155. [Google Scholar] [CrossRef]
- Zhu, F.L.; Feng, Q.Q. Recent Advances in Textile Materials for Personal Radiative Thermal Management in Indoor and Outdoor Environments. Int. J. Therm. Sci. 2021, 165, 106899. [Google Scholar] [CrossRef]
- Wichmann, J.; Andersen, Z.; Ketzel, M.; Ellermann, T.; Loft, S. Apparent Temperature and Cause-Specific Emergency Hospital Admissions in Greater Copenhagen, Denmark. PLoS ONE 2011, 6, e22904. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.K. Handbook of Air Conditioning and Refrigeration; McGraw-Hill: New York, NY, USA, 2001. [Google Scholar]
- Legg, R. Properties of Humid Air. In Air Conditioning System Design; Elsevier: Amsterdam, The Netherlands, 2017; pp. 1–28. [Google Scholar] [CrossRef]
- Tartarini, F.; Schiavon, S.; Cheung, T.; Hoyt, T. CBE Thermal Comfort Tool: Online Tool for Thermal Comfort Calculations and Visualizations. SoftwareX 2020, 12, 100563. [Google Scholar] [CrossRef]
- Teitelbaum, E.; Jayathissa, P.; Miller, C.; Meggers, F. Design with Comfort: Expanding the Psychrometric Chart with Radiation and Convection Dimensions. Energy Build. 2020, 209, 109591. [Google Scholar] [CrossRef]
- Huang, J.; Chen, Y. Effects of Air Temperature, Relative Humidity, and Wind Speed on Water Vapor Transmission Rate of Fabrics. Text. Res. J. 2010, 80, 422–428. [Google Scholar] [CrossRef]
- Belding, H.S.; Russell, H.D.; Darling, R.C.; Folk, G.E. Analysis of Factors Concerned in Maintaining Energy Balance for Dressed Men in Extreme Cold; Effects of Activity on the Protective Value and Comfort of an Arctic Uniform. Am. J. Physiol. Leg. Content 1947, 149, 223–239. [Google Scholar] [CrossRef]
- Vogt, J.J.; Meyer, J.P.; Candas, V.; Libert, J.P.; Sagot, J.C. Pumping Effects on Thermal Insulation of Clothing Worn by Human Subjects. Ergonomics 1983, 26, 963–974. [Google Scholar] [CrossRef] [PubMed]
- Nielsen, R.; Olesen, B.W.; Fanger, P.O. Effect of Physical Activity and Air Velocity on the Thermal Insulation of Clothing. Ergonomics 1985, 28, 1617–1631. [Google Scholar] [CrossRef]
- Ismail, N.; Ghaddar, N.; Ghali, K. Electric Circuit Analogy of Heat Losses of Clothed Walking Human Body in Windy Environment. Int. J. Therm. Sci. 2018, 127, 105–116. [Google Scholar] [CrossRef]
- Ismail, N.; Ghaddar, N.; Ghali, K. Determination of Segmental and Overall Ventilation of Clothed Walking Human by Means of Electric Circuit Analogy. Text. Res. J. 2016, 88, 586–601. [Google Scholar] [CrossRef]
- Zhu, G.; Kremenakova, D.; Wang, Y.; Militky, J. Air Permeability of Polyester Nonwoven Fabrics. Autex Res. J. 2015, 15, 8–12. [Google Scholar] [CrossRef]
- Joshi, A.; Psikuta, A.; Bueno, M.-A.; Annaheim, S.; Rossi, R.M. Effect of Movement on Convection and Ventilation in a Skin-Clothing-Environment System. Int. J. Therm. Sci. 2021, 166, 106965. [Google Scholar] [CrossRef]
- Fisher, C.H. History of Natural Fibers. J. Macromol. Sci. 1981, 15, 1345–1375. [Google Scholar] [CrossRef]
- Cook, J.G. Handbook of Textile Fibres: Man-Made Fibres; Woodhead Pub Limited: Cambridge, UK, 1984. [Google Scholar]
- Ahmad, S.; Rasheed, A.; Nawab, Y. Fibers for Technical Textiles; Springer Nature: Berlin/Heidelberg, Germany, 2020. [Google Scholar]
- Zhang, Y.F.; Sun, B.K. Development of Outlast Fiber and Study about Its Character of Thermoregulation. Adv. Mater. Res. 2012, 557–559, 979–982. [Google Scholar] [CrossRef]
- Gulhane, S.S.; Raichurkar, P.P. Developments in Profile Filament Yarn for Enhancing Moisture Management Properties of Fabrics. Man Made Text. India 2020, XLVIII, 88–92. [Google Scholar]
- Sperling, L.H. Introduction to Physical Polymer Science; Wiley: Hoboken, NJ, USA, 2005. [Google Scholar] [CrossRef]
- Plante, A.M.; Holcombe, B.V.; Stephens, L.G. Fiber Hygroscopicity and Perceptions of Dampness. Text. Res. J. 1995, 65, 293–298. [Google Scholar] [CrossRef]
- Senthilkumar, M.; Sampath, M.B.; Ramachandran, T. Moisture Management in an Active Sportswear: Techniques and Evaluation—A Review Article. J. Inst. Eng. (India) Ser. E 2012, 93, 61–68. [Google Scholar] [CrossRef]
- Jhanji, Y.; Gupta, D.; Kothari, V.K. Thermo-Physiological Properties of Polyester–Cotton Plated Fabrics in Relation to Fibre Linear Density and Yarn Type. Fash. Text. 2015, 2, 16. [Google Scholar] [CrossRef]
- Islam, M.R.; Chowdhary, U. Relative Color Pickup of Three Different Knits and Predictive Dyeing Recipe Formulation. Int. J. Polym. Text. Eng. 2019, 6, 1–16. [Google Scholar] [CrossRef]
- Laitala, K.; Klepp, I.; Henry, B. Does Use Matter? Comparison of Environmental Impacts of Clothing Based on Fiber Type. Sustainability 2018, 10, 2524. [Google Scholar] [CrossRef]
- Russell, I.M. Sustainable Wool Production and Processing. In Sustainable Textiles; Elsevier: Amsterdam, The Netherlands, 2009; pp. 63–87. [Google Scholar] [CrossRef]
- Allafi, F.; Hossain, M.S.; Lalung, J.; Shaah, M.; Salehabadi, A.; Ahmad, M.I.; Shadi, A. Advancements in Applications of Natural Wool Fiber: Review. J. Nat. Fibers 2022, 19, 497–512. [Google Scholar] [CrossRef]
- Laing, R.M.; Wilson, C.A.; Gore, S.E.; Carr, D.J.; Niven, B.E. Determining the Drying Time of Apparel Fabrics. Text. Res. J. 2007, 77, 583–590. [Google Scholar] [CrossRef]
- Kumar, S.; Boominathan, S.K.; Raj, D.V.K. Comparative Analysis on Thermo-Physiological Behavior of Eri Silk, Wool and Bamboo Knitted Fabrics Toward Sportswear. J. Nat. Fibers 2022, 19, 7702–7713. [Google Scholar] [CrossRef]
- Troynikov, O.; Wardiningsih, W. Moisture Management Properties of Wool/ Polyester and Wool/Bamboo Knitted Fabrics for the Sportswear Base Layer. Text. Res. J. 2011, 81, 621–631. [Google Scholar] [CrossRef]
- Hossaini, F.A. Customer Quality Analysis of Outdoor Clothing, Mälardalen University. 2011. Available online: http://www.diva-portal.org/smash/get/diva2:431565/fulltext01 (accessed on 7 June 2023).
- Jeon, E.; Yoo, S.; Kim, E. Psychophysical Determination of Moisture Perception in High-Performance Shirt Fabrics in Relation to sweating Level. Ergonomics 2011, 54, 576–586. [Google Scholar] [CrossRef]
- Hatch, K.L.; Woo, S.S.; Barker, R.L.; Radhakrishnaiah, P.; Markee, N.L.; Maibach, H.I. In Vivo Cutaneous and Perceived Comfort Response to Fabric. Text. Res. J. 1990, 60, 405–412. [Google Scholar] [CrossRef]
- Adler, M.M.; Walsh, W.K. Mechanisms of Transient Moisture Transport Between Fabrics. Text. Res. J. 1984, 54, 334–343. [Google Scholar] [CrossRef]
- Hsieh, Y.-L. Liquid Transport in Fabric Structures. Text. Res. J. 1995, 65, 299–307. [Google Scholar] [CrossRef]
- Varshney, R.K.; Kothari, V.K.; Dhamija, S. A Study on Thermophysiological Comfort Properties of Fabrics in Relation to Constituent Fibre Fineness and Cross-Sectional Shapes. J. Text. Inst. 2010, 101, 495–505. [Google Scholar] [CrossRef]
- Kohan, M.I. The History and Development of Nylon-66. In High Performance Polymers: Their Origin and Development; Springer: Dordrecht, The Netherlands, 1986; pp. 19–37. [Google Scholar] [CrossRef]
- Chen, J. Synthetic Textile Fibers. In Textiles and Fashion; Elsevier: Amsterdam, The Netherlands, 2015; pp. 79–95. [Google Scholar] [CrossRef]
- Adamu, B.F.; Gao, J. Comfort Related Woven Fabric Transmission Properties Made of Cotton and Nylon. Fash. Text. 2022, 9, 8. [Google Scholar] [CrossRef]
- Anas, M.S.; Awais, H.; Ali Hamdani, S.T.; Shaker, K.; Azam, Z.; Nawab, Y. Investigating the Thermo-Physiological Comfort Properties of Weft-Knitted Smart Structures Having a Negative Poisson’s Ratio. Adv. Mater. Sci. Eng. 2022, 2022, 1896634. [Google Scholar] [CrossRef]
- Kumar, P.S.; Suganya, S. Introduction to Sustainable Fibres and Textiles. In Sustainable Fibres and Textiles; Elsevier: Amsterdam, The Netherlands, 2017; pp. 1–18. [Google Scholar] [CrossRef]
- Colom, X.; Carrillo, F. Crystallinity Changes in Lyocell and Viscose-Type Fibres by Caustic Treatment. Eur. Polym. J. 2002, 38, 2225–2230. [Google Scholar] [CrossRef]
- Kayseri, G.Ö.; Bozdo, F. Performance Properties of Regenerated Cellulose Fibers. Tekst. Ve Konfeksiyon 2010, 20, 208–212. [Google Scholar]
- Prakash, C.; Ramakrishnan, G.; Koushik, C.V. Effect of Blend Proportion on Moisture Management Characteristics of Bamboo/Cotton Knitted Fabrics. J. Text. Inst. 2013, 104, 1320–1326. [Google Scholar] [CrossRef]
- Brito, M.D.; Carbone, V.; Blanquart, C.M. Towards a Sustainable Fashion Retail Supply Chain in Europe: Organisation and Performance. Int. J. Prod. Econ. 2008, 114, 534–553. [Google Scholar] [CrossRef]
- Stana-Kleinschek, K.; Ribitsch, V.; Kreze, T.; Fras, L. Determination of the Adsorption Character of Cellulose Fibres Using Surface Tension and Surface Charge. Mater. Res. Innov. 2002, 6, 13–18. [Google Scholar] [CrossRef]
- Parajuli, P.; Acharya, S.; Rumi, S.S.; Hossain, M.T.; Abidi, N. Regenerated Cellulose in Textiles: Rayon, Lyocell, Modal and Other Fibres. In Fundamentals of Natural Fibres and Textiles; Elsevier: Amsterdam, The Netherlands, 2021; pp. 87–110. [Google Scholar] [CrossRef]
- Smole, M.S.; Peršin, Z.; Kreže, T.; Kleinschek, K.S.; Ribitsch, V.; Neumayer, S. X-Ray Study of Pre-Treated Regenerated Cellulose Fibres. Mater. Res. Innov. 2003, 7, 275–282. [Google Scholar] [CrossRef]
- Kreze, T.; Strnad, S.; Stana-Kleinschek, K.; Ribitsch, V. Influence of Aqueous Medium on Mechanical Properties of Conventional and New Environmentally Friendly Regenerated Cellulose Fibers. Mater. Res. Innov. 2001, 4, 107–114. [Google Scholar] [CrossRef]
- Öztürk, H.B.; Okubayashi, S.; Bechtold, T. Splitting Tendency of Cellulosic Fibers. Part 2: Effects of Fiber Swelling in Alkali Solutions. Cellulose 2006, 13, 403–409. [Google Scholar] [CrossRef]
- Ozdemir, H. Permeability and Wicking Properties of Modal and Lyocell Woven Fabrics Used for Clothing. J. Eng. Fibers Fabr. 2017, 12, 155892501701200. [Google Scholar] [CrossRef]
- Eryuruk, S.H.; Kalaoglu, F. Analysis of the Performance Properties of Knitted Fabrics Containing Elastane. Int. J. Cloth. Sci. Technol. 2016, 28, 463–479. [Google Scholar] [CrossRef]
- Manshahia, M.; Das, A. Thermophysiological Comfort Characteristics of Plated Knitted Fabrics. J. Text. Inst. 2013, 105, 509–519. [Google Scholar] [CrossRef]
- Shobanasree, P.C.; Prakash, C.; Kumar, M.R.; Lokesh, K.V. Effect of Elastane Plating on Physical & Thermal Comfort Properties of Lyocell Single Jersey Knit Fabric with Different Loop Length. J. Nat. Fibers 2022, 19, 11574–11581. [Google Scholar]
- Öner, E.; Okur, A. Thermophysiological Comfort Properties of Selected Knitted Fabrics and Design of T-Shirts. J. Text. Inst. 2015, 106, 1403–1414. [Google Scholar] [CrossRef]
- Atasağun, H.G.; Öner, E.; Okur, A.; Beden, A.R. A Comprehensive Study on the General Performance Properties of Viloft-Blended Knitted Fabrics. J. Text. Inst. 2015, 106, 523–535. [Google Scholar] [CrossRef]
- Krithika, U.S.M.; Sampath, M.B.; Prakash, C.; Senthilkumar, M. Moisture Management Finish on Woven Fabrics. Indian J. Fibre Text. Res. 2019, 44, 486–491. [Google Scholar]
- Sampath, M.B.; Senthilkumar, M. Effect of Moisture Management Finish on Comfort Characteristics of Microdenier Polyester Knitted Fabrics. J. Ind. Text. 2009, 39, 163–173. [Google Scholar] [CrossRef]
- Sampath, M.B.; Prakash, C.; Senthilkumar, M. Influence of Moisture Management Finish on Comfort Characteristics of Knitted Fabrics Made from Different Yarns. Indian J. Fibre Text. Res. 2020, 45, 102–108. [Google Scholar] [CrossRef]
- Liu, J.; Wang, W.; Yu, D.; Zhao, K. Durable Moisture-Wicking and Fast-Dry Polyester Fabric Prepared by UV-Induced Click Reaction. Fibers Polym. 2020, 21, 111–118. [Google Scholar] [CrossRef]
- Holmes, D.A. Waterproof Breathable Fabrics. In Handbook of Technical Textiles; Elsevier: Amsterdam, The Netherlands, 2000; pp. 282–315. [Google Scholar] [CrossRef]
- Schindler, W.D.; Hauser, P.J. Chemical Finishing of Textiles; Woodhead Publishing in Textiles: Cambridge, UK, 2004. [Google Scholar]
- Tama, D.; Catarino, A.; Abreu, M.J. Evaluating the Effect of Water-Repellent Finishing on Thermal Insulation Properties of Rowing Shirts Using a Thermal Manikin. Tekst. Ve Konfeksiyon 2019, 29, 279–288. [Google Scholar] [CrossRef]
- Zhou, H.; Wang, H.; Niu, H.; Gestos, A.; Wang, X.; Lin, T. Fluoroalkyl Silane Modified Silicone Rubber/Nanoparticle Composite: A Super Durable, Robust Superhydrophobic Fabric Coating. Adv. Mater. 2012, 24, 2409–2412. [Google Scholar] [CrossRef] [PubMed]
- Yang, M.; Liu, W.; Jiang, C.; Liu, C.; He, S.; Xie, Y.; Wang, Z. Robust Fabrication of Superhydrophobic and Photocatalytic Self-Cleaning Cotton Textile Based on TiO2 and Fluoroalkylsilane. J. Mater. Sci. 2019, 54, 2079–2092. [Google Scholar] [CrossRef]
- Hays, H.L.; Mathew, D.; Chapman, J. Fluorides and Fluorocarbons Toxicity. In StatPearls; StatePearls Publishing: Treasure Island, FL, USA, 2022. [Google Scholar]
- Vethandamoorthy, D.; Mandawala, E.; Bandara, W. Development of Silicone-Based Water-Resistant, Chemical Resistant, Moisture Absorbent and Non-Ignitable Fabric. Int. J. Sci. Eng. Res. 2019, 10, 346. [Google Scholar]
- Cheng, X.-W.; Liang, C.-X.; Guan, J.-P.; Yang, X.-H.; Tang, R.-C. Flame Retardant and Hydrophobic Properties of Novel Sol-Gel Derived Phytic Acid/Silica Hybrid Organic-Inorganic Coatings for Silk Fabric. Appl. Surf. Sci. 2018, 427, 69–80. [Google Scholar] [CrossRef]
- Özek, H.Z. Silicone-Based Water Repellents. In Waterproof and Water Repellent Textiles and Clothing; Elsevier: Amsterdam, The Netherlands, 2018; pp. 153–189. [Google Scholar] [CrossRef]
- Mohseni, M.; Far, H.S.; Hasanzadeh, M.; Golovin, K. Non-Fluorinated Sprayable Fabric Finish for Durable and Comfortable Superhydrophobic Textiles. Prog. Org. Coat. 2021, 157, 106319. [Google Scholar] [CrossRef]
- De, P.; Sankhe, M.D.; Chaudhari, S.S.; Mathur, M.R. UV-Resist, Water-Repellent Breathable Fabric as Protective Textiles. J. Ind. Text. 2005, 34, 209–222. [Google Scholar] [CrossRef]
- Yoo, S.; Barker, R.L. Moisture Management Properties of Heat-Resistant Workwear Fabrics— Effects of Hydrophilic Finishes and Hygroscopic Fiber Blends. Text. Res. J. 2004, 74, 995–1000. [Google Scholar] [CrossRef]
- Chen, Q.; Fan, J.T.; Sarkar, M.K.; Bal, K. Plant-Based Biomimetic Branching Structures in Knitted Fabrics for Improved Comfort-Related Properties. Text. Res. J. 2011, 81, 1039–1048. [Google Scholar] [CrossRef]
- Guan, X.; Wang, X.; Huang, Y.; Zhao, L.; Sun, X.; Owens, H.; Lu, J.R.; Liu, X. Smart Textiles with Janus Wetting and Wicking Properties Fabricated by Graphene Oxide Coatings. Adv. Mater. Interfaces 2021, 8, 2001427. [Google Scholar] [CrossRef]
- Zeng, C.; Wang, H.; Zhou, H.; Lin, T. Directional Water Transport Fabrics with Durable Ultra-High One-Way Transport Capacity. Adv. Mater. Interfaces 2016, 3, 1600036. [Google Scholar] [CrossRef]
- Prabu, G.; Chattopadhyay, S.K.; Patil, P.G.; Arputharaj, A.; Mandhyan, P.; Prasad, G.K.; Vivekanandan, M.; Hadge, G.B.; Nadanathangam, V. Moisture Management Finish on Cotton Fabric by Electrospraying. Text. Res. J. 2017, 87, 2154–2165. [Google Scholar] [CrossRef]
- Tong, J.K.; Huang, X.; Boriskina, S.V.; Loomis, J.; Xu, Y.; Chen, G. Infrared-Transparent Visible-Opaque Fabrics for Wearable Personal Thermal Management. ACS Photon. 2015, 2, 769–778. [Google Scholar] [CrossRef]
- Wang, J.; Dai, G.; Huang, J. Thermal Metamaterial: Fundamental, Application, and Outlook. iScience 2020, 23, 101637. [Google Scholar] [CrossRef]
- Hsu, P.-C.; Song, A.Y.; Catrysse, P.B.; Liu, C.; Peng, Y.; Xie, J.; Fan, S.; Cui, Y. Radiative Human Body Cooling by Nanoporous Polyethylene Textile. Science 2016, 353, 1019–1023. [Google Scholar] [CrossRef]
- Ke, Y.; Wang, F.; Xu, P.; Yang, B. On the Use of a Novel Nanoporous Polyethylene (NanoPE) Passive Cooling Material for Personal Thermal Comfort Management under Uniform Indoor Environments. Build. Environ. 2018, 145, 85–95. [Google Scholar] [CrossRef]
- Catrysse, P.B.; Song, A.Y.; Fan, S. Photonic Structure Textile Design for Localized Thermal Cooling Based on a Fiber Blending Scheme. ACS Photonics 2016, 3, 2420–2426. [Google Scholar] [CrossRef]
- Cai, L.; Song, A.Y.; Li, W.; Hsu, P.; Lin, D.; Catrysse, P.B.; Liu, Y.; Peng, Y.; Chen, J.; Wang, H.; et al. Spectrally Selective Nanocomposite Textile for Outdoor Personal Cooling. Adv. Mater. 2018, 30, 1802152. [Google Scholar] [CrossRef]
- Yue, X.; Zhang, T.; Yang, D.; Qiu, F.; Wei, G.; Zhou, H. Multifunctional Janus Fibrous Hybrid Membranes with Sandwich Structure for On-Demand Personal Thermal Management. Nano Energy 2019, 63, 103808. [Google Scholar] [CrossRef]
- Liang, J.; Wu, J.; Guo, J.; Li, H.; Zhou, X.; Liang, S.; Qiu, C.-W.; Tao, G. Radiative Cooling for Passive Thermal Management towards Sustainable Carbon Neutrality. Natl. Sci. Rev. 2023, 10, nwac208. [Google Scholar] [CrossRef]
- Simms, D.L.; Hinkley, P.L. Protective Clothing Against Flames and Heat; Fire Research Notes 324; Fire Research Station: Boreham Wood, Herts, UK, 1959. [Google Scholar]
- Cai, G.; Wang, H.; Luo, Z.; Wang, X. Study on Thermal Insulation Properties of Aluminised Aramid Fabrics. Fibres Text. East. Eur. 2015, 23, 52–56. [Google Scholar]
- Zhu, F.; Feng, Q. Preparation, Thermal Properties and Permeabilities of Aluminum-coated Fabrics Destined for Thermal Radiation Protective Clothing. Fire Mater. 2020, 44, 844–853. [Google Scholar] [CrossRef]
- Shi, N.N.; Tsai, C.-C.; Camino, F.; Bernard, G.D.; Yu, N.; Wehner, R. Keeping Cool: Enhanced Optical Reflection and Radiative Heat Dissipation in Saharan Silver Ants. Science 2015, 349, 298–301. [Google Scholar] [CrossRef]
- Yamada, J. Radiative Properties of Fibers with Non-Circular Cross Sectional Shapes. J. Quant. Spectrosc. Radiat. Transf. 2002, 73, 261–272. [Google Scholar] [CrossRef]
- Sun, Y.; Ji, Y.; Javed, M.; Li, X.; Fan, Z.; Wang, Y.; Cai, Z.; Xu, B. Preparation of Passive Daytime Cooling Fabric with the Synergistic Effect of Radiative Cooling and Evaporative Cooling. Adv. Mater. Technol. 2022, 7, 2100803. [Google Scholar] [CrossRef]
- Zhang, Y.; Yu, J. In Situ Formation of SiO 2 Nanospheres on Common Fabrics for Broadband Radiative Cooling. ACS Appl. Nano Mater. 2021, 4, 11260–11268. [Google Scholar] [CrossRef]
- Hsu, P.-C.; Liu, X.; Liu, C.; Xie, X.; Lee, H.R.; Welch, A.J.; Zhao, T.; Cui, Y. Personal Thermal Management by Metallic Nanowire-Coated Textile. Nano Lett. 2015, 15, 365–371. [Google Scholar] [CrossRef]
- Mccann, J. Build the Perfect Survival Kit: Custom Kits for Adventure, Sport, and Travel; Krause Publications: Iola, Wisconsin, USA, 2005. [Google Scholar]
- Kosiński, S.; Podsiadło, P.; Darocha, T.; Pasquier, M.; Mendrala, K.; Sanak, T.; Zafren, K. Prehospital Use of Ultrathin Reflective Foils. Wilderness Environ. Med. 2022, 33, 134–139. [Google Scholar] [CrossRef]
- Hayes, S.G.; Venkatraman, P. Materials and Technology for Sportswear and Performance Apparel; CRC Press: Boca Raton, FL, USA, 2016. [Google Scholar]
- Cai, L.; Song, A.Y.; Wu, P.; Hsu, P.-C.; Peng, Y.; Chen, J.; Liu, C.; Catrysse, P.B.; Liu, Y.; Yang, A.; et al. Warming up Human Body by Nanoporous Metallized Polyethylene Textile. Nat. Commun. 2017, 8, 496. [Google Scholar] [CrossRef]
- Yue, X.; He, M.; Zhang, T.; Yang, D.; Qiu, F. Laminated Fibrous Membrane Inspired by Polar Bear Pelt for Outdoor Personal Radiation Management. ACS Appl. Mater. Interfaces 2020, 12, 12285–12293. [Google Scholar] [CrossRef]
- Abebe, M.G.; De Corte, A.; Rosolen, G.; Maes, B. Janus-Yarn Fabric for Dual-Mode Radiative Heat Management. Phys. Rev. Appl. 2021, 16, 054013. [Google Scholar] [CrossRef]
- Yue, X.; Zhang, T.; Yang, D.; Qiu, F.; Wei, G.; Lv, Y. A Robust Janus Fibrous Membrane with Switchable Infrared Radiation Properties for Potential Building Thermal Management Applications. J. Mater. Chem. A Mater. 2019, 7, 8344–8352. [Google Scholar] [CrossRef]
- Fang, Y.; Chen, G.; Bick, M.; Chen, J. Smart Textiles for Personalized Thermoregulation. Chem. Soc. Rev. 2021, 50, 9357–9374. [Google Scholar] [CrossRef] [PubMed]
- Wei, W.; Liu, J.; Huang, J.; Cao, F.; Qian, K.; Yao, Y.; Li, W. Recent Advances and Perspectives of Shape Memory Polymer Fibers. Eur. Polym. J. 2022, 175, 111385. [Google Scholar] [CrossRef]
- Chen, W.L.; He, M.M.; Gao, Y.; Wu, X. Temperature-Regulating Properties of Phase Change Fiber Blended Knitted Fabrics after Laundering. Adv. Mater. Res. 2011, 393–395, 397–400. [Google Scholar] [CrossRef]
- Mondal, S. Phase Change Materials for Smart Textiles—An Overview. Appl. Therm. Eng. 2008, 28, 1536–1550. [Google Scholar] [CrossRef]
- Wang, Y.; Yao, J.; Zhu, G.; Militky, J.; Marek, J.; Venkataraman, M.; Zhang, G. A Novel Method for Producing Bi-Component Thermo-Regulating Alginate Fiber from Phase Change Material Microemulsion. Text. Res. J. 2020, 90, 1038–1044. [Google Scholar] [CrossRef]
- Tran, N.H.A.; Kirsten, M.; Cherif, C. New Fibers from PCM Using the Conventional Melt Spinning Process. In Proceedings of the Europe/Africa conference Dresden 2017—Polymer Processing Society PPS; AIP Publishing: College Park, Maryland, USA, 2019; p. 060002. [Google Scholar] [CrossRef]
- Ding, B.; Yu, J. Electrospun Nanofibers for Energy and Environmental Applications; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar] [CrossRef]
- Shin, Y.; Yoo, D.-I.; Son, K. Development of Thermoregulating Textile Materials with Microencapsulated Phase Change Materials (PCM). IV. Performance Properties and Hand of Fabrics Treated with PCM Microcapsules. J. Appl. Polym. Sci. 2005, 97, 910–915. [Google Scholar] [CrossRef]
- Choi, K.; Cho, G.; Kim, P.; Cho, C. Thermal Storage/Release and Mechanical Properties of Phase Change Materials on Polyester Fabrics. Text. Res. J. 2004, 74, 292–296. [Google Scholar] [CrossRef]
- Kim, J.; Cho, G. Thermal Storage/Release, Durability, and Temperature Sensing Properties of Thermostatic Fabrics Treated with Octadecane-Containing Microcapsules. Text. Res. J. 2002, 72, 1093–1098. [Google Scholar] [CrossRef]
- Mazumder, J. Functional Polymers; Springer International Publishing: Berlin/Heidelberg, Germany, 2020. [Google Scholar]
- Zhu, Y.; Hu, J.; Lu, J.; Yeung, L.Y.; Yeung, K. Shape Memory Fiber Spun with Segmented Polyurethane Ionomer. Polym. Adv. Technol. 2008, 19, 1745–1753. [Google Scholar] [CrossRef]
- Kaursoin, J.; Agrawal, A.K. Melt Spun Thermoresponsive Shape Memory Fibers Based on Polyurethanes: Effect of Drawing and Heat-Setting on Fiber Morphology and Properties. J. Appl. Polym. Sci. 2007, 103, 2172–2182. [Google Scholar] [CrossRef]
- Xia, Y.; Zhang, F.; Wang, L.; Liu, Y.; Leng, J. Electrospun Shape-Memory Polymer Fibers and Their Applications. In Electrospun Polymers and Composites; Elsevier: Amsterdam, The Netherlands, 2021; pp. 567–596. [Google Scholar] [CrossRef]
- Xiao, X.; Hu, J. Animal Hairs as Water-Stimulated Shape Memory Materials: Mechanism and Structural Networks in Molecular Assemblies. Sci. Rep. 2016, 6, 26393. [Google Scholar] [CrossRef] [PubMed]
- Hu, J.; Irfan Iqbal, M.; Sun, F. Wool Can Be Cool: Water-Actuating Woolen Knitwear for Both Hot and Cold. Adv. Funct. Mater. 2020, 30, 2005033. [Google Scholar] [CrossRef]
- Wang, L.; Pan, M.; Lu, Y.; Song, W.; Liu, S.; Lv, J. Developing Smart Fabric Systems with Shape Memory Layer for Improved Thermal Protection and Thermal Comfort. Mater. Des. 2022, 221, 110922. [Google Scholar] [CrossRef]
- Memiş, N.K.; Kaplan, S. Wool Fabric Having Thermal Comfort Management Function via Shape Memory Polyurethane Finishing. J. Text. Inst. 2020, 111, 734–744. [Google Scholar] [CrossRef]
- Rousseau, I.A. Challenges of Shape Memory Polymers: A Review of the Progress toward Overcoming SMP’s Limitations. Polym. Eng. Sci. 2008, 48, 2075–2089. [Google Scholar] [CrossRef]
- Wang, Y.; Yu, X.; Liu, R.; Zhi, C.; Liu, Y.; Fan, W.; Meng, J. Shape Memory Active Thermal-Moisture Management Textiles. Compos. Part A Appl. Sci. Manuf. 2022, 160, 107037. [Google Scholar] [CrossRef]
- Shahzad, A.; Jabbar, A.; Irfan, M.; Qadir, M.B.; Ahmad, Z. Electrical Resistive Heating Characterization of Conductive Hybrid Staple Spun Yarns. J. Text. Inst. 2020, 111, 1481–1488. [Google Scholar] [CrossRef]
- Pillai, A.S.; Chandran, A.; Peethambharan, S.K. Joule Heating Fabrics. In Advances in Healthcare and Protective Textiles; Elsevier: Amsterdam, The Netherlands, 2023; pp. 387–421. [Google Scholar] [CrossRef]
- Wu, B.Y.; Alam, M.O.; Chan, Y.C.; Zhong, H.W. Joule Heating Enhanced Phase Coarsening in Sn37Pb and Sn3.5Ag0.5Cu Solder Joints during Current Stressing. J. Electron. Mater. 2008, 37, 469–476. [Google Scholar] [CrossRef]
- Martins, R.d.A. Joule’s 1840 Manuscript on the Production of Heat by Voltaic Electricity. Notes Rec. R. Soc. J. Hist. Sci. 2022, 76, 117–154. [Google Scholar] [CrossRef]
- Doganay, D.; Coskun, S.; Genlik, S.P.; Unalan, H.E. Silver Nanowire Decorated Heatable Textiles. Nanotechnology 2016, 27, 435201. [Google Scholar] [CrossRef] [PubMed]
- Ahn, J.; Gu, J.; Hwang, B.; Kang, H.; Hwang, S.; Jeon, S.; Jeong, J.; Park, I. Printed Fabric Heater Based on Ag Nanowire/Carbon Nanotube Composites. Nanotechnology 2019, 30, 455707. [Google Scholar] [CrossRef] [PubMed]
- Cheng, Y.; Zhang, H.; Wang, R.; Wang, X.; Zhai, H.; Wang, T.; Jin, Q.; Sun, J. Highly Stretchable and Conductive Copper Nanowire Based Fibers with Hierarchical Structure for Wearable Heaters. ACS Appl. Mater. Interfaces 2016, 8, 32925–32933. [Google Scholar] [CrossRef]
- Mahmud, S.; Forcier, P.; Decaens, J.; Dolez, P.I. Controlling the Variability in the Measurement of the Efficiency of Joule Heating Textiles; Institute of Textile Science (ITS) Symposium: Edmonton, AB, Canada, 2023. [Google Scholar]
- Ma, R.; Zhang, Z.; Tong, K.; Huber, D.; Kornbluh, R.; Ju, Y.S.; Pei, Q. Highly Efficient Electrocaloric Cooling with Electrostatic Actuation. Science 2017, 357, 1130–1134. [Google Scholar] [CrossRef]
- Wang, H.; Meng, Y.; Zhang, Z.; Gao, M.; Peng, Z.; He, H.; Pei, Q. Self-Actuating Electrocaloric Cooling Fibers. Adv. Energy Mater. 2020, 10, 1903902. [Google Scholar] [CrossRef]
- Hong, S.; Gu, Y.; Seo, J.K.; Wang, J.; Liu, P.; Meng, Y.S.; Xu, S.; Chen, R. Wearable Thermoelectrics for Personalized Thermoregulation. Sci. Adv. 2019, 5, eaaw0536. [Google Scholar] [CrossRef]
- Snyder, G.J.; Snyder, A.H. Figure of Merit ZT of a Thermoelectric Device Defined from Materials Properties. Energy Environ. Sci 2017, 10, 2280–2283. [Google Scholar] [CrossRef]
- Lee, J.A.; Aliev, A.E.; Bykova, J.S.; de Andrade, M.J.; Kim, D.; Sim, H.J.; Lepró, X.; Zakhidov, A.A.; Lee, J.; Spinks, G.M.; et al. Woven-Yarn Thermoelectric Textiles. Adv. Mater. 2016, 28, 5038–5044. [Google Scholar] [CrossRef]
- Zhang, H.; Huizenga, C.; Arens, E.; Wang, D. Thermal Sensation and Comfort in Transient Non-Uniform Thermal Environments. Eur. J. Appl. Physiol. 2004, 92, 728–733. [Google Scholar] [CrossRef]
- West, A.M.; Havenith, G.; Hodder, S. Are Running Socks Beneficial for Comfort? The Role of the Sock and Sock Fiber Type on Shoe Microclimate and Subjective Evaluations. Text. Res. J. 2021, 91, 1698–1712. [Google Scholar] [CrossRef]
- Filingeri, D.; Havenith, G. Human Skin Wetness Perception: Psychophysical and Neurophysiological Bases. Temperature 2015, 2, 86–104. [Google Scholar] [CrossRef] [PubMed]
- Periyaswamy, T.; Islam, M.R. Tactile Rendering of Textile Materials. J. Text. Sci. Fash. Technol. 2022, 9, 1–10. [Google Scholar] [CrossRef]
- Tichy, H.; Kallina, W. Insect Hygroreceptor Responses to Continuous Changes in Humidity and Air Pressure. J. Neurophysiol. 2010, 103, 3274–3286. [Google Scholar] [CrossRef] [PubMed]
- Tiest, W.M.B.; Kosters, N.D.; Kappers, A.M.L.; Daanen, H.A.M. Haptic Perception of Wetness. Acta Psychol. 2012, 141, 159–163. [Google Scholar] [CrossRef] [PubMed]
- Bentley, I.M. The Synthetic Experiment. Am. J. Psychol. 1900, 11, 405. [Google Scholar] [CrossRef]
- Ackerley, R.; Olausson, H.; Wessberg, J.; McGlone, F. Wetness Perception across Body Sites. Neurosci. Lett. 2012, 522, 73–77. [Google Scholar] [CrossRef]
- Filingeri, D.; Redortier, B.; Hodder, S.; Havenith, G. Thermal and Tactile Interactions in the Perception of Local Skin Wetness at Rest and during Exercise in Thermo-Neutral and Warm Environments. Neuroscience 2014, 258, 121–130. [Google Scholar] [CrossRef]
- Li, Y. Perceptions of Temperature, Moisture and Comfort in Clothing during Environmental Transients. Ergonomics 2005, 48, 234–248. [Google Scholar] [CrossRef]
- Gagge, A.P. A New Physiological Variable Associated with Sensible and Insensible Perspiration. Am. J. Physiol. Leg. Content 1937, 120, 277–287. [Google Scholar] [CrossRef]
- MacRae, B.A.; Annaheim, S.; Spengler, C.M.; Rossi, R.M. Skin Temperature Measurement Using Contact Thermometry: A Systematic Review of Setup Variables and Their Effects on Measured Values. Front. Physiol. 2018, 9, 29. [Google Scholar] [CrossRef] [PubMed]
- Kateb, B.; Yamamoto, V.; Yu, C.; Grundfest, W.; Gruen, J.P. Infrared Thermal Imaging: A Review of the Literature and Case Report. Neuroimage 2009, 47, T154–T162. [Google Scholar] [CrossRef] [PubMed]
- Bongers, C.C.W.G.; Hopman, M.T.E.; Eijsvogels, T.M.H. Using an Ingestible Telemetric Temperature Pill to Assess Gastrointestinal Temperature During Exercise. J. Vis. Exp. 2015, 53258. [Google Scholar] [CrossRef]
- Koo, H.R.; Lee, Y.-J.; Gi, S.; Khang, S.; Lee, J.H.; Lee, J.-H.; Lim, M.-G.; Park, H.-J.; Lee, J.-W. The Effect of Textile-Based Inductive Coil Sensor Positions for Heart Rate Monitoring. J. Med. Syst. 2014, 38, 2. [Google Scholar] [CrossRef]
- Temko, A. Accurate Heart Rate Monitoring During Physical Exercises Using PPG. IEEE Trans. Biomed. Eng. 2017, 64, 2016–2024. [Google Scholar] [CrossRef]
- Chung, M.; Fortunato, G.; Radacsi, N. Wearable Flexible Sweat Sensors for Healthcare Monitoring: A Review. J. R. Soc. Interface 2019, 16, 20190217. [Google Scholar] [CrossRef]
- Mekjavic, I.B.; Yogev, D.; Ciuha, U. Perception of Thermal Comfort during Skin Cooling and Heating. Life 2021, 11, 681. [Google Scholar] [CrossRef]
- Pao, S.-L.; Wu, S.-Y.; Liang, J.-M.; Huang, I.-J.; Guo, L.-Y.; Wu, W.-L.; Liu, Y.-G.; Nian, S.-H. A Physiological-Signal-Based Thermal Sensation Model for Indoor Environment Thermal Comfort Evaluation. Int. J. Environ. Res. Public Health 2022, 19, 7292. [Google Scholar] [CrossRef]
- Schepers, R.J.; Ringkamp, M. Thermoreceptors and Thermosensitive Afferents. Neurosci. Biobehav. Rev. 2010, 34, 177–184. [Google Scholar] [CrossRef]
- Campero, M.; Bostock, H. Unmyelinated Afferents in Human Skin and Their Responsiveness to Low Temperature. Neurosci. Lett. 2010, 470, 188–192. [Google Scholar] [CrossRef]
- Zhang, H.; Arens, E.; Huizenga, C.; Han, T. Thermal Sensation and Comfort Models for Non-Uniform and Transient Environments: Part I: Local Sensation of Individual Body Parts. Build. Environ. 2010, 45, 380–388. [Google Scholar] [CrossRef]
- Guan, Y.; Hosni, M.H.; Jones, B.W.; Gielda, T.P. Investigation of Human Thermal Comfort under Highly Transient Conditions for Automobile Applications–Part 2: Thermal Sensation Modeling. ASHRAE Trans. 2003, 109, 898–907. [Google Scholar]
- Wang, X.L.; Peterson, F.K. Estimating Thermal Transient Comfort. ASHRAE Trans. 1992, 98, 182–188. [Google Scholar]
- Briese, E. Normal Body Temperature of Rats: The Setpoint Controversy. Neurosci. Biobehav. Rev. 1998, 22, 427–436. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.; Arens, E.; Huizenga, C.; Han, T. Thermal Sensation and Comfort Models for Non-Uniform and Transient Environments, Part III: Whole-Body Sensation and Comfort. Build. Environ. 2010, 45, 399–410. [Google Scholar] [CrossRef]
- Zhang, H.; Arens, E.; Huizenga, C.; Han, T. Thermal Sensation and Comfort Models for Non-Uniform and Transient Environments, Part II: Local Comfort of Individual Body Parts. Build. Environ. 2010, 45, 389–398. [Google Scholar] [CrossRef]
- Raccuglia, M.; Hodder, S.; Havenith, G. Human Wetness Perception in Relation to Textile Water Absorption Parameters under Static Skin Contact. Text. Res. J. 2017, 87, 2449–2463. [Google Scholar] [CrossRef]
- ISO 10551; Ergonomics of the Physical Environment—Subjective Judgement Scales for Assessing Physical Environments. International Organization for Standardization: Geneva, Switzerland, 2019.
- Grujic, D.; Geršak, J. Examination of the Relationships between Subjective Clothing Comfort Assessment and Physiological Parameters with Wear Trials. Text. Res. J. 2017, 87, 1522–1537. [Google Scholar] [CrossRef]
- Celcar, D. A Subjective Evaluation of the Thermal Comfort of Clothing Evaluated in Cold Environment. Glas. Hemičara Tehnol. I Ekol. Repub. Srp. 2014, 5, 65–71. [Google Scholar] [CrossRef]
- ASTM F1868; Standard Test Method for Thermal and Evaporative Resistance of Clothing Materials Using a Sweating Hot Plate. ASTM International: London, UK, 2017.
- Rossi, R. Interactions between Protection and Thermal Comfort. In Textiles for Protection; Elsevier: Amsterdam, The Netherlands, 2005; pp. 233–260. [Google Scholar] [CrossRef]
- Huang, J. Sweating Guarded Hot Plate Test Method. Polym. Test. 2006, 25, 709–716. [Google Scholar] [CrossRef]
- Thermetrics. Sweating Guarded Hotplate. Available online: https://thermetrics.com/wp-content/uploads/2020/09/SGHP-Hotplate-Thermetrics.pdf (accessed on 6 June 2023).
- McCullough, E.A. The Use of Thermal Manikins to Evaluate Clothing and Environmental Factors. Elsevier Ergon. Book Ser. 2005, 3, 403–407. [Google Scholar] [CrossRef]
- ASTM F1291; Standard Test Method for Measuring the Thermal Insulation of Clothing Using a Heated Manikin. ASTM International: London, UK, 2016.
- ASTM F2370; Standard Test Method for Measuring the Evaporative Resistance of Clothing Using a Sweating Manikin. ASTM International: London, UK, 2016.
- Lei, Z. Review of Application of Thermal Manikin in Evaluation on Thermal and Moisture Comfort of Clothing. J. Eng. Fibers Fabr. 2019, 14, 155892501984154. [Google Scholar] [CrossRef]
- Psikuta, A. Development of an “Artificial Human” for Clothing Research; De Montfort University: Leicester, UK, 2009. [Google Scholar]
- Psikuta, A.; Weibel, M.; Burke, R.; Hepokoski, M.; Schwenn, T.; Annaheim, S.; Rossi, R.M. A Systematic Approach to the Development and Validation of Adaptive Manikins. Extrem. Physiol. Med. 2015, 4, A15. [Google Scholar] [CrossRef]
- Wang, F. Effect of Body Movement on the Thermophysiological Responses of an Adaptive Manikin and Human Subjects. Measurement 2018, 116, 251–256. [Google Scholar] [CrossRef]
- Albert, M.M.; García, P.D.; Belda, E.B. Development of a new testing protocol to evaluate cooling systems. EXCLI J. 2023, 22, 583–594. [Google Scholar]
- Ostrowski, Z.; Rojczyk, M. Natural Convection Heat Transfer Coefficient for Newborn Baby. Heat Mass Transf. 2018, 54, 2395–2403. [Google Scholar] [CrossRef]
- Kang, I.-H.; Tamura, T. A Study on the Development of an Infant-Sized Movable Sweating Thermal Manikin. J. Hum. Environ. Syst. 2001, 5, 49–56. [Google Scholar] [CrossRef]
- Golden, J.; Brown, T.; Domina, T.; Wroblewski, S.; Kumphai, P. Utilisation of an Infant Sweating Thermal Manikin to Determine Clo Values for Infant Clothing: A Comparison of Predictive Formulas. Int. J. Fash. Des. Technol. Educ. 2021, 14, 272–283. [Google Scholar] [CrossRef]
- Richards, M.; Alison, J.; Devarajan, S.; Bussoli, M.; Gathercole, R.; Jack, A. A Multi-Sector Cylinder For Iso 18640-1, Specially Designed For Studying Clothing Sweat Management, Working in Hot Climates and Dynamic Physiological Control. In Proceedings of the 12th International Manikin and Modelling Meeting (12i3m), St. Gallen, Switzerland, 29–31 August 2018. [Google Scholar]
- Fontana, P.; Saiani, F.; Grütter, M.; Croset, J.-P.; Capt, A.; Camenzind, M.; Morrissey, M.; Rossi, R.M.; Annaheim, S. Exercise Intensity Dependent Relevance of Protective Textile Properties for Human Thermo-Physiology. Text. Res. J. 2017, 87, 1425–1434. [Google Scholar] [CrossRef]
- Psikuta, A.; Wang, L.-C.; Rossi, R.M. Prediction of the Physiological Response of Humans Wearing Protective Clothing Using a Thermophysiological Human Simulator. J. Occup. Environ. Hyg 2013, 10, 222–232. [Google Scholar] [CrossRef]
- Candas, V.; Libert, J.P.; Vogt, J.J. Human Skin Wettedness and Evaporative Efficiency of Sweating. J. Appl. Physiol. 1979, 46, 522–528. [Google Scholar] [CrossRef] [PubMed]
- Guan, M.; Annaheim, S.; Li, J.; Camenzind, M.; Psikuta, A.; Rossi, R.M. Apparent Evaporative Cooling Efficiency in Clothing with Continuous Perspiration: A Sweating Manikin Study. Int. J. Therm. Sci. 2019, 137, 446–455. [Google Scholar] [CrossRef]
- Havenith, G.; Richards, M.G.; Wang, X.; Bröde, P.; Candas, V.; den Hartog, E.; Holmér, I.; Kuklane, K.; Meinander, H.; Nocker, W. Apparent Latent Heat of Evaporation from Clothing: Attenuation and “Heat Pipe” Effects. J. Appl. Physiol. 2008, 104, 142–149. [Google Scholar] [CrossRef] [PubMed]
- Barndt, H.J.; Pierce, J.D., Jr.; Pa, P.U. QMax Relationship to Perceived Comfort as Measured by the Calm Scale; Laboratory for Engineered Human Protection: Philadelphia, PA, USA, 2022. [Google Scholar]
- Wang, F.; Annaheim, S.; Morrissey, M.; Rossi, R.M. Real Evaporative Cooling Efficiency of One-Layer Tight-Fitting Sportswear in a Hot Environment. Scand. J. Med. Sci. Sports 2013, 24, e129–e139. [Google Scholar] [CrossRef]
- McLellan, T.; Selkirk, G. Heat Stress While Wearing Long Pants or Shorts under Firefighting Protective Clothing. Ergonomics 2004, 47, 75–90. [Google Scholar] [CrossRef]
- Lu, Y.; Song, G.; Ackerman, M.Y.; Paskaluk, S.A.; Li, J. A New Protocol to Characterize Thermal Protective Performance of Fabrics against Hot Liquid Splash. Exp. Therm. Fluid Sci. 2013, 46, 37–45. [Google Scholar] [CrossRef]
- Dolez, P.I.; Marsha, S.; McQueen, R.H. Fibers and Textiles for Personal Protective Equipment: Review of Recent Progress and Perspectives on Future Developments. Textiles 2022, 2, 349–381. [Google Scholar] [CrossRef]
- Abedin, F.; DenHartog, E. The Exothermic Effects of Textile Fibers during Changes in Environmental Humidity: A Comparison between ISO:16533 and Dynamic Hot Plate Test Method. Fibers 2023, 11, 47. [Google Scholar] [CrossRef]
- Gao, H.; Deaton, A.S.; Barker, R. A New Test Method for Evaluating the Evaporative Cooling Efficiency of Fabrics Using a Dynamic Sweating Hot Plate. Meas. Sci. Technol. 2022, 33, 125601. [Google Scholar] [CrossRef]
- Renard, M.; Puszkarz, A.K. Modeling of Heat Transfer through Firefighters Multilayer Protective Clothing Using the Computational Fluid Dynamics Assisted by X-Ray Microtomography and Thermography. Materials 2022, 15, 5417. [Google Scholar] [CrossRef]
- Hamilton, S.F.; Luster, T. Bronfenbrenner, Urie. In Encyclopedia of Human Ecology; Miller, J.R., Lerner, R.M., Schiamberg, L.B., Anderson, P.M., Eds.; ABC-CLIO: Santa Barbara, CA, USA, 2003; Volume 1, pp. 84–89. [Google Scholar]
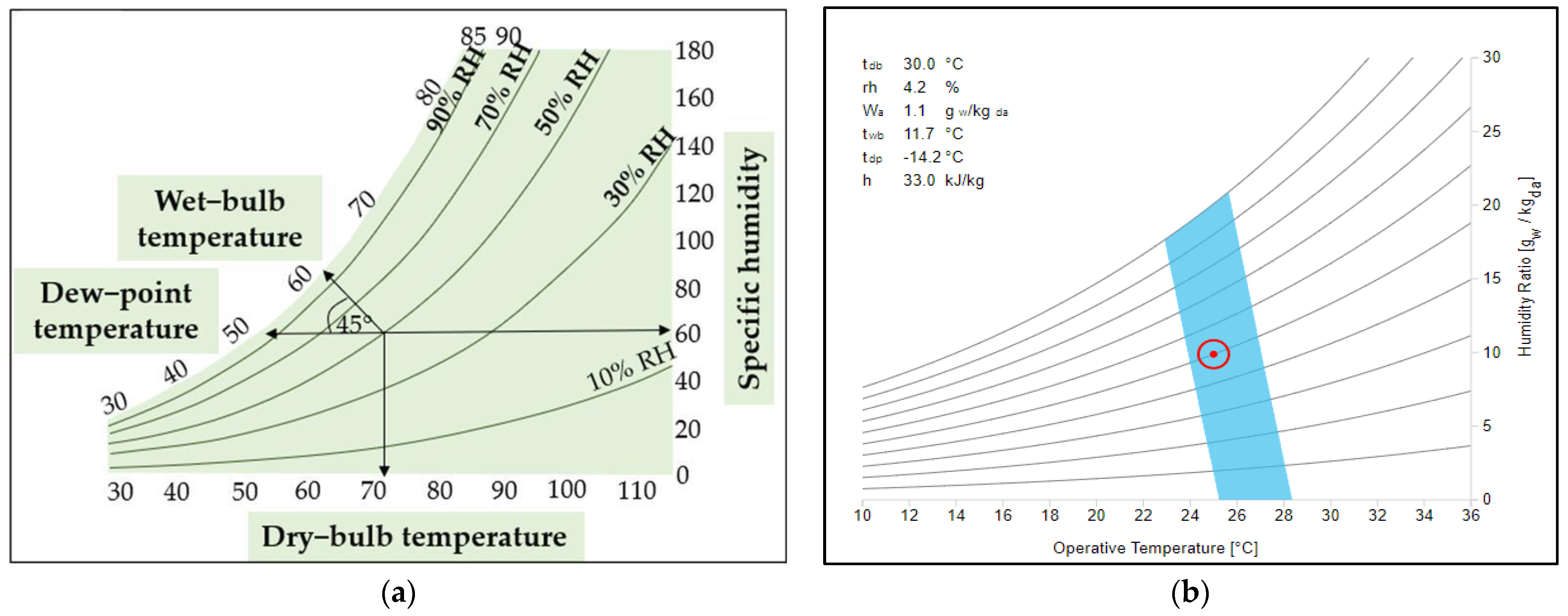
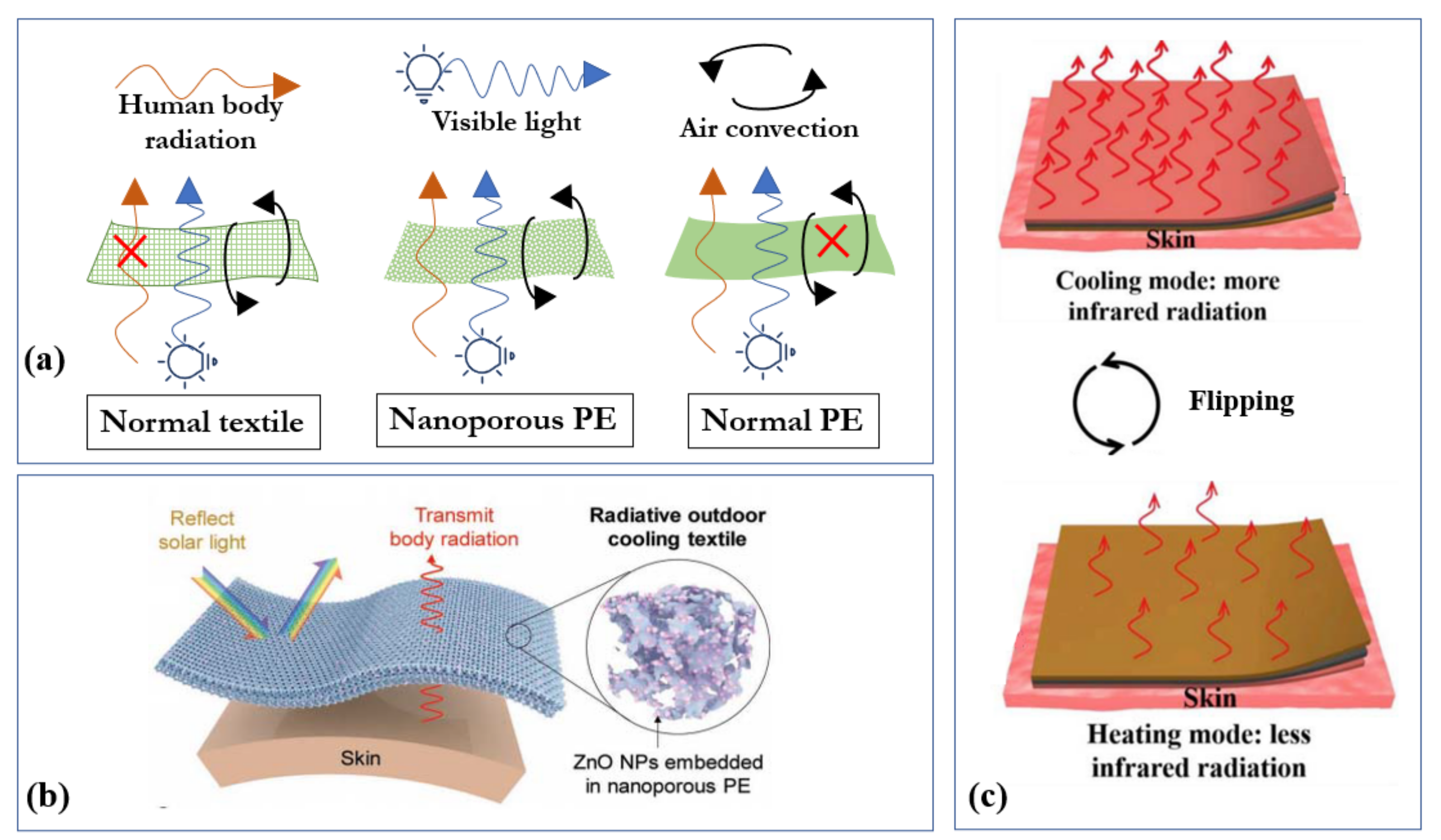
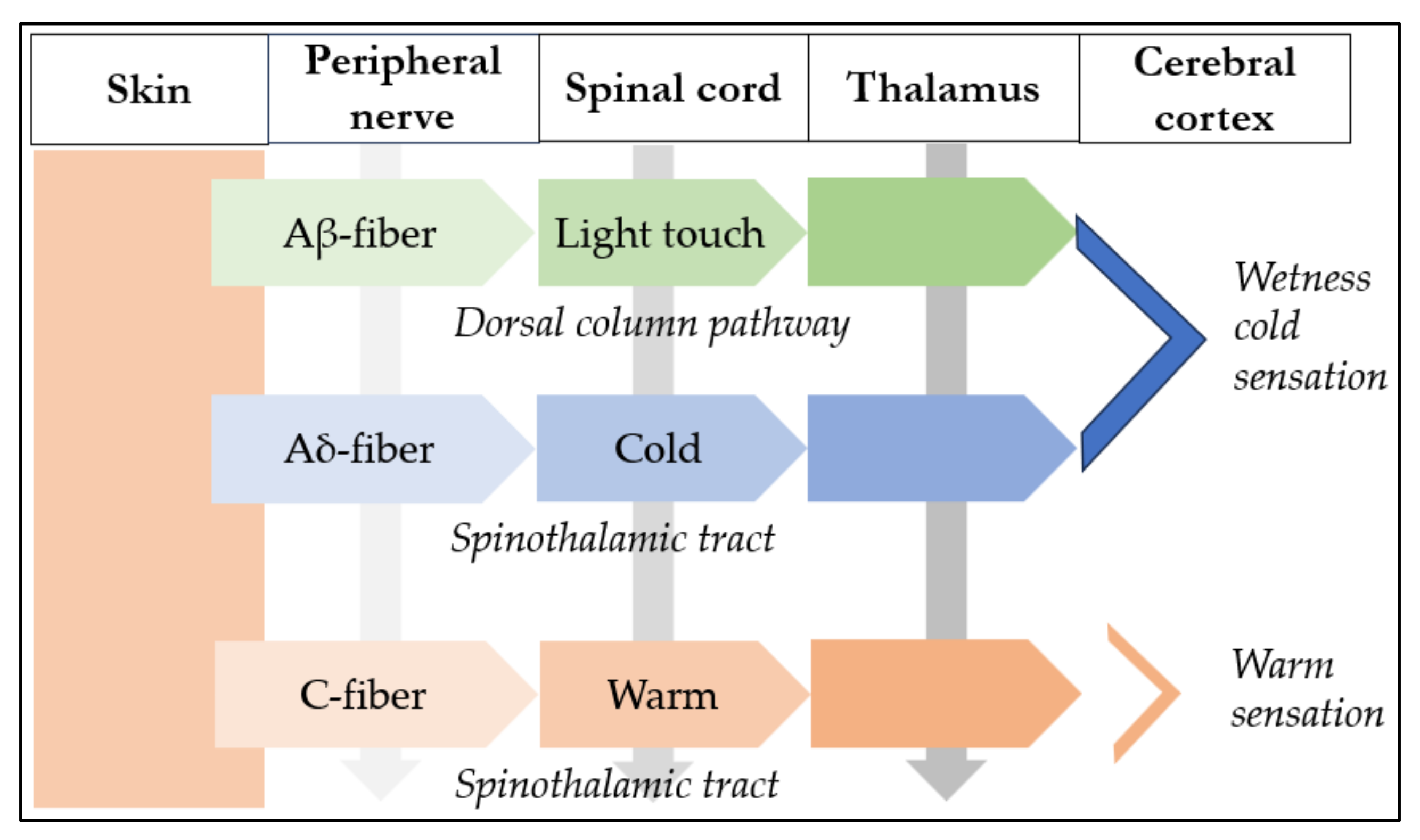
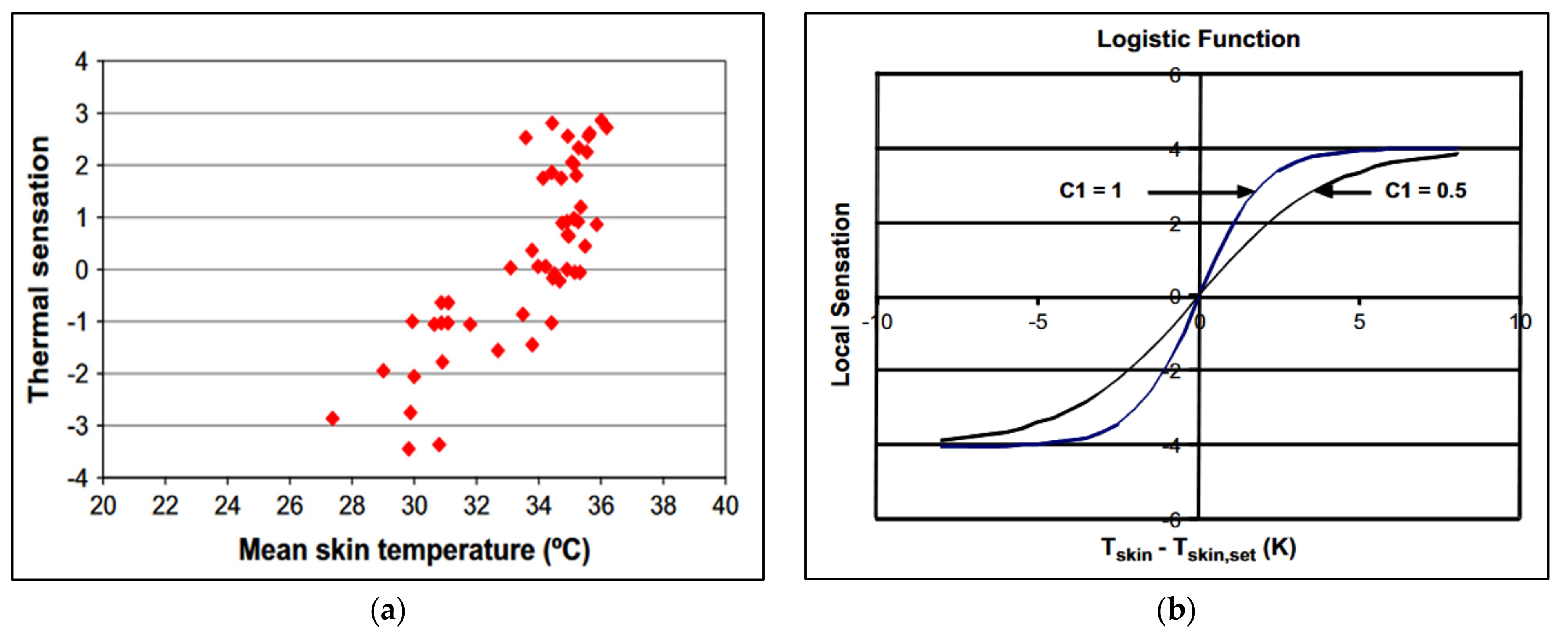

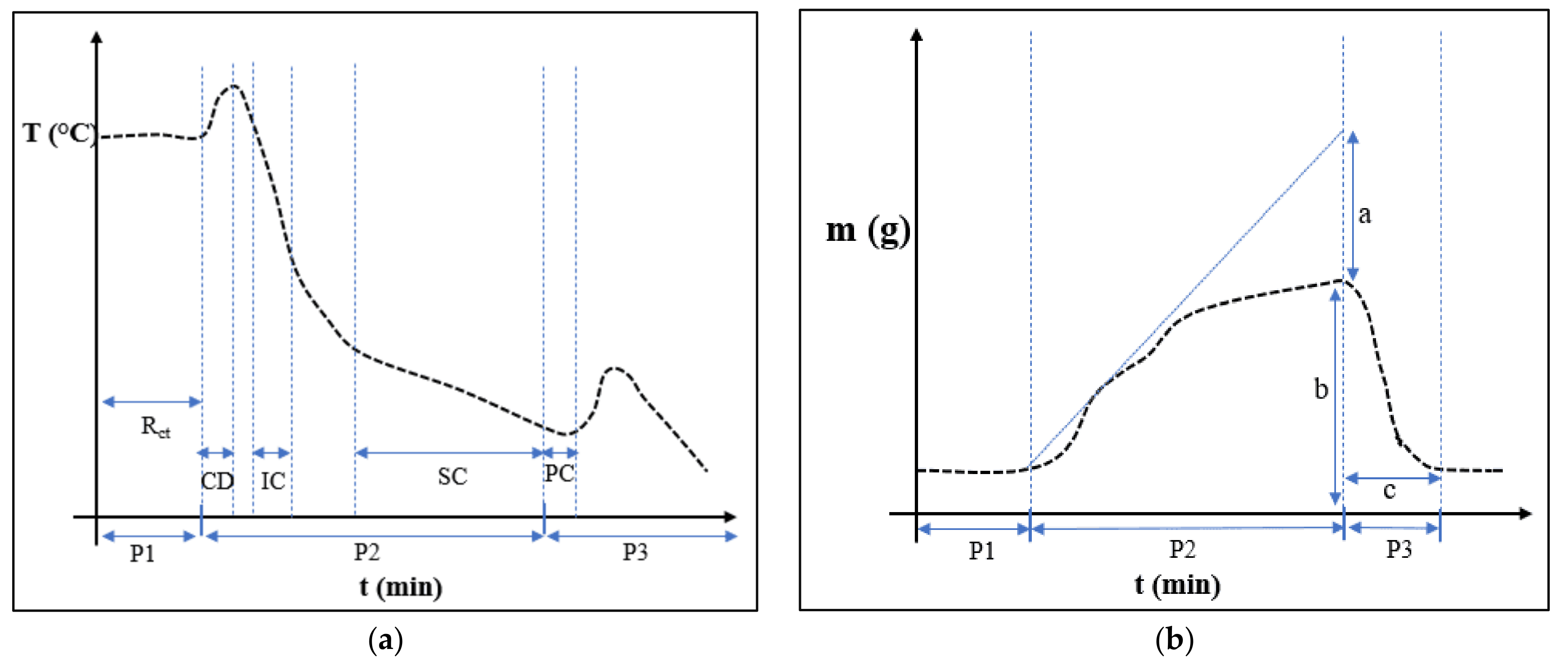
| Process | Equation | Description |
|---|---|---|
| Heat generation within the human body [24,62] | (3) | = Density of human tissue C = Specific heat capacity of the human tissue T = Tissue temperature of the local body considered for the calculation. |
| Heat conduction within the tissue layers [68] | ; TSkin ≥ 37 °C , when TSkin ≤ 27.9 °C (4) , when 27.9 °C < TSkin < 37 °C | K = Thermal conductivity of the tissue layer r = Radius of the tissue layer w = Dimensionless geometry factor when using polar or spherical coordinates |
| Heat generation from basal metabolism [62] | QBasic = b × Qtbm (5) | b = Heat distribution factor of different body parts Qtbm = Total basal metabolic heat |
| Heat generation from shivering [62] | , when TSkin ≥ 36.4 °C , when TSkin ≤ 34.1 °C (6) , when 34.1 °C < TSkin < 36.4 °C | TSkin = Skin temperature |
| Metabolic heat generation due to physical activity [62] | QBasic) (7) | wi = Heat distribution factor of labor work Qtotal = Total heat generation from Tanabe et al.’s [26] model |
| Sweat rate [62] | msw = fsw (T − 37) + fmin (8) | fsw = Sweat factor fmin = Minimum sweat rate |
| Parameter/Process | Equation | Description |
|---|---|---|
| Rayleigh number [68] | Ra = Gr.Pr (10) | β = Thermal expansion coefficient of air = Gravitational force = 9.8 m/s2 dT = Temperature difference between the skin and the inner cloth surface L = Thickness of the microclimate layer TEAL = (TSkin + TFabric)/2; TSkin = Skin temperature TFabric = Inner cloth surface temperature = Kinematic viscosity of air α = Thermal diffusivity of air |
| Conductive heat transfer (EAL) [68] | QK_EAL = KEAL (11) )0.8646 | hK_EAL = Conductive heat transfer coefficient = KEAL/L; KEAL = Thermal conductivity of enclosed air layer |
| Radiative heat transfer (EAL) [68,75] | QR_EAL = (12) | = Skin emissivity = Fabric inner surface emissivity 10−8 Wm−2K−4 hR_EAL = Radiative heat transfer coefficient = |
| Convective heat transfer (EAL) [68] | QC_EAL = hcon_EAL (TSkin − TFabric) (13) | |
| Total heat transfer (EAL) | For Ra < 1708, QEAL_cf = (Conduction + Radiation) (14) For Ra ≥ 1708, QEAL_cf = (Convection + Radiation) (15) | For Ra <1708, QEAL_cf = KEAL + (16) (17) |
| Total thermal resistance (EAL) [68] | (18) | For Ra < 1708: hTotal_EAL = hK_EAL + hR_EAL For Ra ≥ 1708: hTotal_EAL = hcon_EAL + hR_EAL |
| Process | Equation | Description |
|---|---|---|
| Convective heat transfer (BAL) [24,68] | QC_mixed_BAL = hC_mixed_BAL (TFabric –TAir) (20) 0.5 | = Natural convection coefficient = Forced convection coefficient = Mixed convection coefficient = Ambient air speed TFabric = Outer cloth surface temperature TAir = Air temperature |
| Radiative heat transfer (BAL) [68] | QR_BAL = (21) hR_BAL = | = View factor of a body segment with respect to the environment. The term “view factor” indicates how much radiation from the human body is intercepted by a second surface. |
| Total heat transfer (BAL) | (22) | |
| Total thermal resistance (BAL) [68] | (23) hTotal_BAL = hC_mixed_BAL + hR_BAL | |
| Process | Equation | Description |
|---|---|---|
| Evaporative heat transfer (EAL) [28] | λ (28) (29) | = Heat transfer due to diffusion-based vapor transfer = Heat transfer due to vapor convection |
| Water vapor transmission rate (EAL) [3,28] | (30) For Ra ≥ 1708, (31) | Csat = Saturation vapor pressure at the wet surface/skin Cfabric_inner_surf = Partial vapor pressure at the inner surface of the fabric |
| Evaporative resistance (EAL) [28] | (32) (33) | = Vapor diffusion coefficient in the encloser air layer = Vapor convection coefficient in the encloser air layer L = Thickness of the enclosed air layer |
| Evaporative resistance of the fabric layer | ||
| Evaporative resistance of the fabric layer [42] | Ref = (Pskin − Pair)/Qef (34) | Pskin = Saturation vapor pressure at the sweating-guarded hot plate surface Pair = Partial vapor pressure of the ambient air inside the guarded hot plate chamber Qef = Evaporative heat loss through the fabric structure |
| Evaporative heat transfer in the boundary air layer | ||
| Convective heat transfer (BAL) [4] | (35) | LR = Lewis ratio |
| Commercial Name | Genre | Structural Feature | Functionality | Manufacturer |
|---|---|---|---|---|
| Thermolite® | Polyester | Hollow cross-section and convoluted outer surface | Thermal insulation and capillary action | DuPont |
| Tactel® | Nylon 6.6 | Trilobal cross-section | Quick wicking and drying | DuPont |
| Tencel TM | Modal/Lyocell | Microscopic channels between fibrils and hygroscopicity | Moisture absorption and wicking | Lenzing |
| Coolmax® | Polyester | Tetra- or hexa-channel cross-section | Quick wicking and drying | DuPont |
| Viloft® | Viscose | Flat surface with multiple wicking channels | Moisture absorption and wicking | Kelheim Fibers |
| Fieldsensor TM | Polyester | Grooved fiber surface | Quick moisture absorption and evaporation | Toray Industries Inc. |
| Coolpass® | Polyester | Tetra lobbed cross-section | Quick wicking and drying | Coolpass Fabric Technology |
| Hygra | Nylon-coated water-absorbent polymer (WAP) | WAP core and nylon sheath | Quick moisture absorption and desorption | Unitika Limited |
| Killat N | Nylon 6,6 | C-shaped hollow fiber cross-section | Increased thermal insulation and moisture absorption | Kanebo Limited |
| Calculo TM | Polyester | Random cross-section and moisture-wicking finish | Quick moisture absorption and drying | Teijin |
| Octa® | Polyester | Hollow fiber with 6 to 8 channels along the cross-section | Moisture-wicking and drying | Teijin |
| Outlast® | Microencapsulated paraffin wax incorporated into fiber as PCM | Absorbs and releases heat depending on weather conditions | Reduces sweat rate and improves thermal comfort | Heidenheim |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Islam, M.R.; Golovin, K.; Dolez, P.I. Clothing Thermophysiological Comfort: A Textile Science Perspective. Textiles 2023, 3, 353-407. https://doi.org/10.3390/textiles3040024
Islam MR, Golovin K, Dolez PI. Clothing Thermophysiological Comfort: A Textile Science Perspective. Textiles. 2023; 3(4):353-407. https://doi.org/10.3390/textiles3040024
Chicago/Turabian StyleIslam, Md Rashedul, Kevin Golovin, and Patricia I. Dolez. 2023. "Clothing Thermophysiological Comfort: A Textile Science Perspective" Textiles 3, no. 4: 353-407. https://doi.org/10.3390/textiles3040024
APA StyleIslam, M. R., Golovin, K., & Dolez, P. I. (2023). Clothing Thermophysiological Comfort: A Textile Science Perspective. Textiles, 3(4), 353-407. https://doi.org/10.3390/textiles3040024






