Raman Sensor for the Determination of Gas Solubility
Abstract
:1. Introduction
2. Materials and Methods
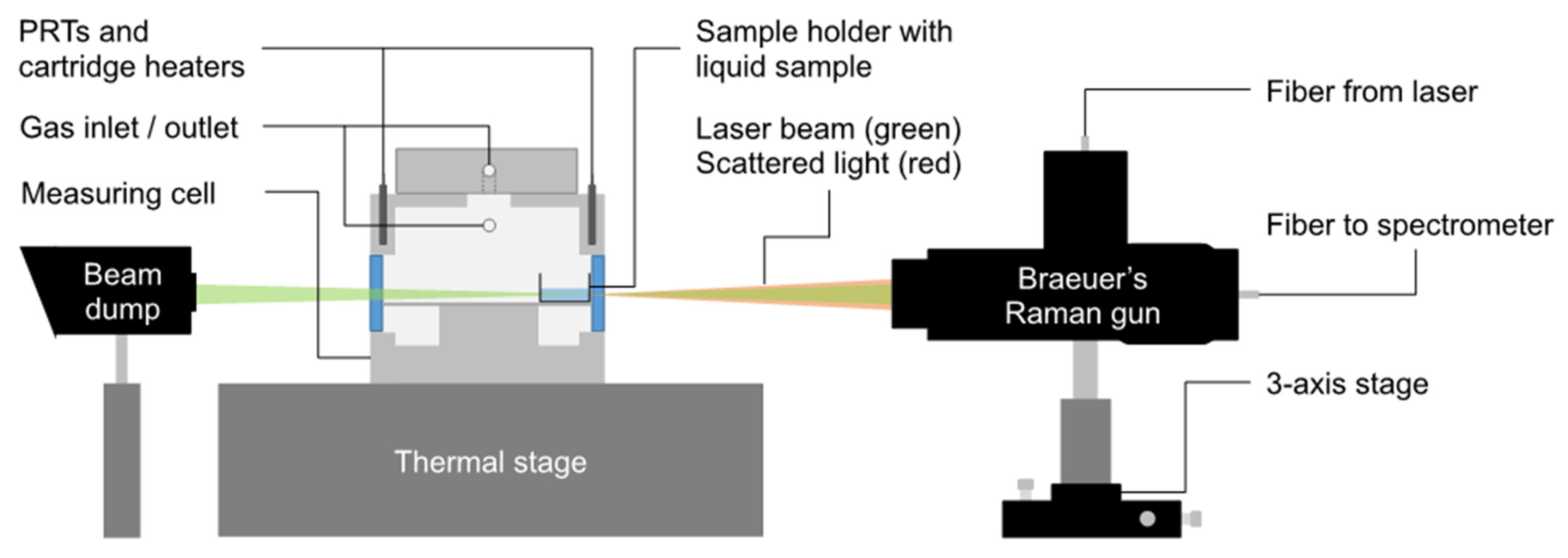
2.1. Experimental Setup
2.2. Experimental Material
2.3. Experimental Procedure
3. Results and Discussion
3.1. Data Analysis
3.2. Uncertainty in Measurement
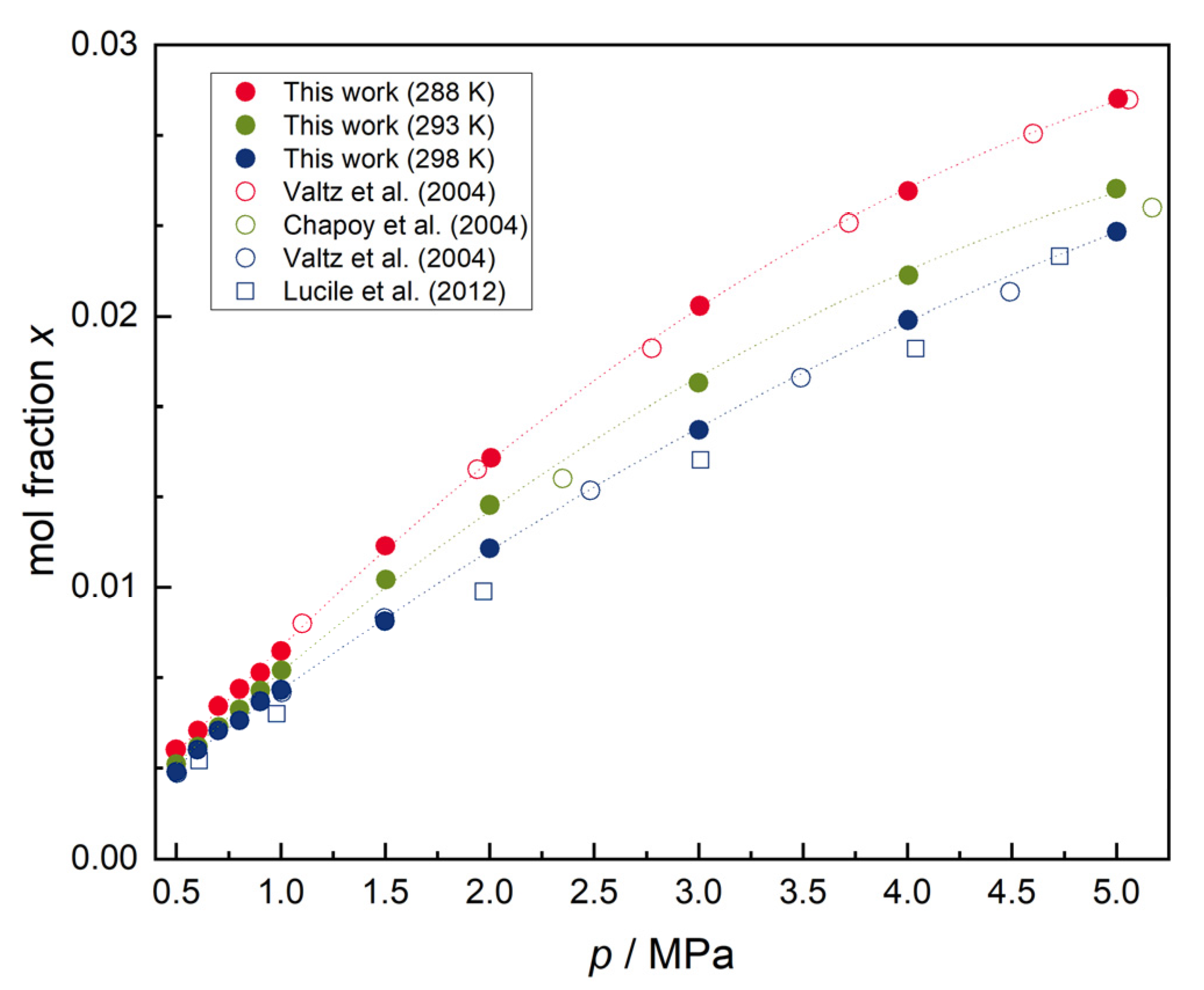
3.3. Results for Solubilty of Carbon Dioxide in Water
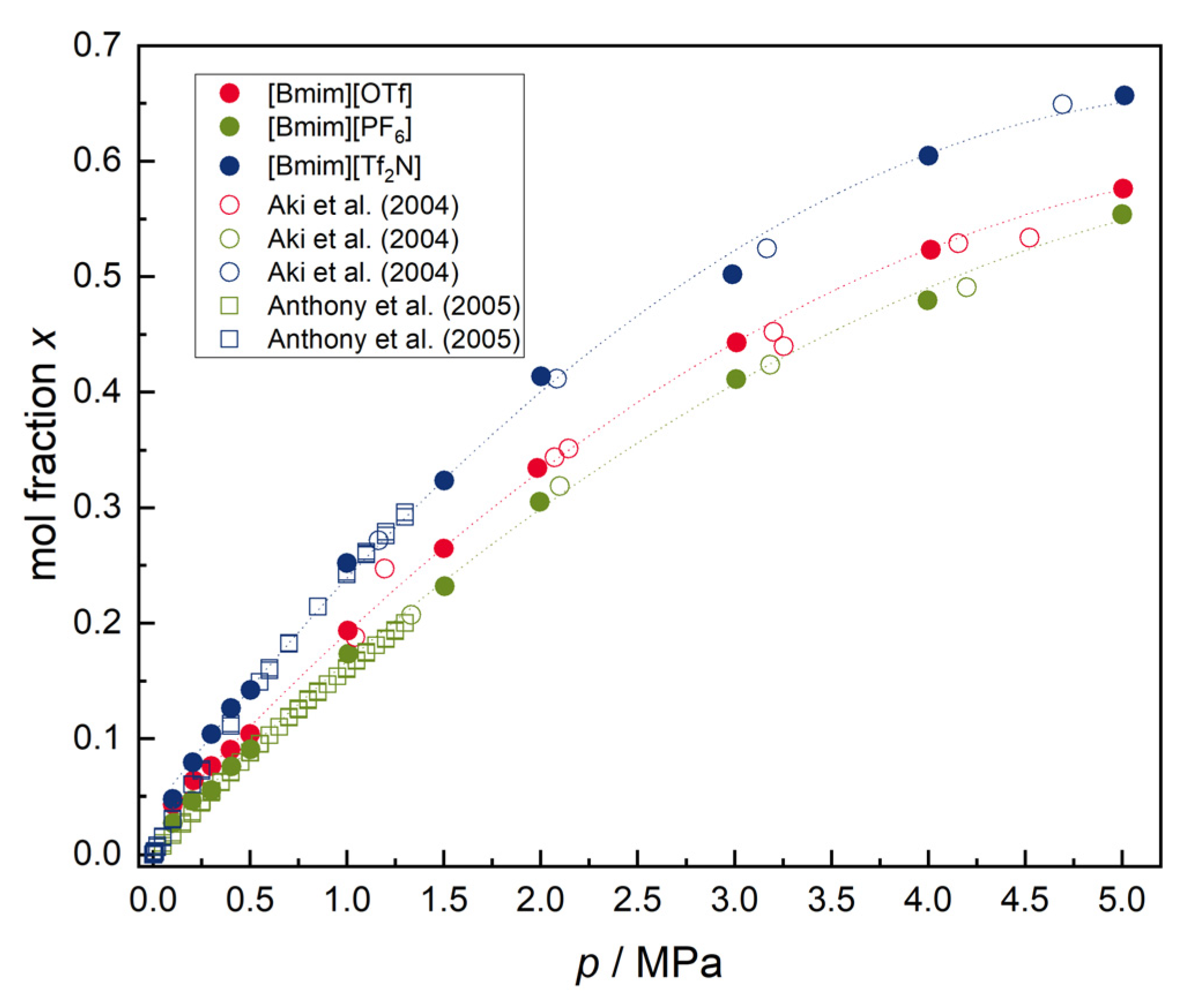
3.4. Results for Solubilty of Carbon Dioxide in Ionic Liquids
4. Conclusions and Outlook
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Water
| T/K | p/MPa | xCO2 | U(xCO2) | U(xCO2)·(xCO2)−1/% |
|---|---|---|---|---|
| 288.170 | 0.49948 | 0.0040 | 0.0002 | 5.002 |
| 288.219 | 0.60390 | 0.0047 | 0.0002 | 4.696 |
| 288.213 | 0.70222 | 0.0056 | 0.0003 | 5.148 |
| 288.226 | 0.80377 | 0.0063 | 0.0003 | 4.268 |
| 288.232 | 0.90201 | 0.0068 | 0.0003 | 4.044 |
| 288.218 | 1.0.0180 | 0.0076 | 0.0003 | 4.182 |
| 288.341 | 1.50218 | 0.0115 | 0.0004 | 3.737 |
| 288.244 | 2.00692 | 0.0148 | 0.0006 | 3.940 |
| 288.245 | 3.00527 | 0.0204 | 0.0008 | 3.686 |
| 288.245 | 4.00375 | 0.0246 | 0.0009 | 3.594 |
| 288.261 | 5.00929 | 0.0280 | 0.0010 | 3.604 |
| T/K | p/MPa | xCO2 | U(xCO2) | U(xCO2)·(xCO2)−1/% |
|---|---|---|---|---|
| 293.288 | 0.50013 | 0.0035 | 0.0002 | 5.918 |
| 293.296 | 0.60432 | 0.0041 | 0.0002 | 4.151 |
| 293.314 | 0.70312 | 0.0049 | 0.0003 | 6.182 |
| 293.315 | 0.80243 | 0.0055 | 0.0003 | 5.193 |
| 293.303 | 0.90190 | 0.0062 | 0.0002 | 3.314 |
| 293.362 | 1.00330 | 0.0069 | 0.0003 | 3.763 |
| 293.305 | 1.50295 | 0.0103 | 0.0004 | 3.880 |
| 293.307 | 2.00047 | 0.0130 | 0.0004 | 3.117 |
| 293.320 | 3.00050 | 0.0170 | 0.0006 | 3.334 |
| 293.317 | 4.00540 | 0.0215 | 0.0006 | 2.899 |
| 293.322 | 5.00079 | 0.0247 | 0.0008 | 3.109 |
| T/K | p/MPa | xCO2 | U(xCO2) | U(xCO2)·(xCO2)−1/% |
|---|---|---|---|---|
| 298.122 | 0.50006 | 0.0032 | 0.0002 | 6.658 |
| 298.137 | 0.60229 | 0.0040 | 0.0002 | 6.078 |
| 298.145 | 0.70124 | 0.0047 | 0.0003 | 6.222 |
| 298.161 | 0.80192 | 0.0051 | 0.0003 | 6.157 |
| 298.154 | 0.90176 | 0.0058 | 0.0004 | 6.554 |
| 298.162 | 1.00143 | 0.0062 | 0.0003 | 5.133 |
| 298.163 | 1.49948 | 0.0088 | 0.0005 | 6.120 |
| 298.137 | 2.00118 | 0.0115 | 0.0007 | 5.813 |
| 298.143 | 3.00088 | 0.0158 | 0.0008 | 5.170 |
| 298.174 | 4.00231 | 0.0199 | 0.0011 | 5.711 |
| 298.182 | 5.00264 | 0.0231 | 0.0012 | 5.229 |
Appendix B. Ionic Liquids
| T/K | p/MPa | xCO2 | U(xCO2) | U(xCO2)·(xCO2)−1/% |
|---|---|---|---|---|
| 293.204 | 0.10185 | 0.0481 | 0.0020 | 4.111 |
| 293.245 | 0.20174 | 0.0622 | 0.0026 | 4.223 |
| 293.247 | 0.29971 | 0.0777 | 0.0032 | 4.102 |
| 293.266 | 0.40085 | 0.0961 | 0.0039 | 4.049 |
| 293.265 | 0.50234 | 0.1110 | 0.0041 | 3.678 |
| 293.258 | 0.99962 | 0.2075 | 0.0077 | 3.720 |
| 293.233 | 1.50541 | 0.2866 | 0.0077 | 2.695 |
| 293.236 | 2.00833 | 0.3590 | 0.0106 | 2.953 |
| 293.223 | 2.99711 | 0.4837 | 0.0131 | 2.702 |
| 293.242 | 4.03906 | 0.5630 | 0.0128 | 2.266 |
| 293.276 | 5.00981 | 0.6161 | 0.0133 | 2.162 |
| T/K | p/MPa | xCO2 | U(xCO2) | U(xCO2)·(xCO2)−1/% |
|---|---|---|---|---|
| 293.266 | 0.10030 | 0.0346 | 0.0011 | 3.205 |
| 293.256 | 0.20045 | 0.0545 | 0.0017 | 3.084 |
| 293.251 | 0.30380 | 0.0648 | 0.0019 | 2.984 |
| 293.229 | 0.40299 | 0.0896 | 0.0025 | 2.793 |
| 293.200 | 0.50020 | 0.1014 | 0.0027 | 2.676 |
| 293.214 | 0.99997 | 0.1986 | 0.0052 | 2.609 |
| 293.215 | 1.50415 | 0.2644 | 0.0065 | 2.471 |
| 293.207 | 2.01386 | 0.3318 | 0.0073 | 2.210 |
| 292.847 | 2.98469 | 0.4458 | 0.0079 | 1.781 |
| 293.026 | 4.00660 | 0.5292 | 0.0076 | 1.442 |
| 293.303 | 5.03079 | 0.6086 | 0.0082 | 1.340 |
| T/K | p/MPa | xCO2 | U(xCO2) | U(xCO2)·(xCO2)−1/% |
|---|---|---|---|---|
| 293.090 | 0.10145 | 0.0586 | 0.0029 | 4.966 |
| 293.279 | 0.20236 | 0.0860 | 0.0042 | 4.873 |
| 293.216 | 0.30252 | 0.1136 | 0.0056 | 4.904 |
| 293.200 | 0.40159 | 0.1440 | 0.0069 | 4.759 |
| 293.199 | 0.50097 | 0.1607 | 0.0075 | 4.641 |
| 293.190 | 0.99947 | 0.2782 | 0.0126 | 4.532 |
| 293.273 | 1.50195 | 0.3578 | 0.0141 | 3.942 |
| 293.180 | 2.01805 | 0.4424 | 0.0167 | 3.785 |
| 293.214 | 3.00150 | 0.5484 | 0.0203 | 3.695 |
| 293.219 | 4.00222 | 0.6194 | 0.0228 | 3.683 |
| 293.206 | 5.00784 | 0.6767 | 0.0242 | 3.578 |
Appendix C. Sample Spectrum of Carbon Dioxide in Water

References
- Somekawa, T.; Takeuchi, T.; Yamanaka, C.; Fujita, M. Raman spectroscopy measurements of CO2 dissolved in water and CO2 bubbles for laser remote sensing in water. In Remote Sensing of the Ocean, Sea Ice, Coastal Waters, and Large Water Regions, Proceedings of the SPIE Remote Sensing, Amsterdam, The Netherlands, 22–25 September 2014; SPIE: Bellingham, WA, USA, 2014; Volume 9240. [Google Scholar] [CrossRef]
- Somekawa, T.; Fujita, M. Raman Spectroscopic Measurements of CO2 Dissolved in Seawater for Laser Remote Sensing in Water. EPJ Web Conf. 2016, 119, 25017. [Google Scholar] [CrossRef] [Green Version]
- Li, L.; Zhang, X.; Luan, Z.; Du, Z.; Xi, S.; Wang, B.; Cao, L.; Lian, C.; Yan, J. In Situ Quantitative Raman Detection of Dissolved Carbon Dioxide and Sulfate in Deep-Sea High-Temperature Hydrothermal Vent Fluids. Geochem. Geophys. Geosyst. 2018, 19, 1809–1823. [Google Scholar] [CrossRef]
- Valtz, A.; Chapoy, A.; Coquelet, C.; Paricaud, P.; Richon, D. Vapour–Liquid Equilibria in the Carbon Dioxide–Water System, Measurement and Modelling from 278.2 to 318.2K. Fluid Phase Equilibria 2004, 226, 333–344. [Google Scholar] [CrossRef]
- Chapoy, A.; Mohammadi, A.H.; Chareton, A.; Tohidi, B.; Richon, D. Measurement and Modeling of Gas Solubility and Literature Review of the Properties for the Carbon Dioxide−Water System. Ind. Eng. Chem. Res. 2004, 43, 1794–1802. [Google Scholar] [CrossRef]
- Lucile, F.; Cézac, P.; Contamine, F.; Serin, J.-P.; Houssin, D.; Arpentinier, P. Solubility of Carbon Dioxide in Water and Aqueous Solution Containing Sodium Hydroxide at Temperatures from (293.15 to 393.15) K and Pressure up to 5 MPa: Experimental Measurements. J. Chem. Eng. Data 2012, 57, 784–789. [Google Scholar] [CrossRef]
- Zhang, J.; Tong, D.; Fennell, P.S.; Trusler, J.P.M. Solubility of CO2 in aqueous amine solutions: A study to select solvents for carbon capture from natural-gas power plant. In Proceedings of the 4th International Gas Processing Symposium; Elsevier: Amsterdam, The Netherlands, 2015; pp. 1–10, ISBN 978-0-444-63461-0. [Google Scholar]
- Kiepe, J.; Horstmann, S.; Fischer, K.; Gmehling, J. Experimental Determination and Prediction of Gas Solubility Data for CO2 + H2O Mixtures Containing NaCl or KCl at Temperatures between 313 and 393 K and Pressures up to 10 MPa. Ind. Eng. Chem. Res. 2002, 41, 4393–4398. [Google Scholar] [CrossRef]
- Koschel, D.; Coxam, J.-Y.; Rodier, L.; Majer, V. Enthalpy and Solubility Data of CO2 in Water and NaCl(Aq) at Conditions of Interest for Geological Sequestration. Fluid Phase Equilibria 2006, 247, 107–120. [Google Scholar] [CrossRef]
- Wu, G.; Cao, E.; Kuhn, S.; Gavriilidis, A. A Novel Approach for Measuring Gas Solubility in Liquids Using a Tube-in-Tube Membrane Contactor. Chem. Eng. Technol. 2017, 40, 2346–2350. [Google Scholar] [CrossRef] [Green Version]
- Wu, H.; Zheng, K.; Wang, G.; Yang, Y.; Li, Y. Modeling of Gas Solubility in Hydrocarbons Using the Perturbed-Chain Statistical Associating Fluid Theory Equation of State. Ind. Eng. Chem. Res. 2019, 58, 12347–12360. [Google Scholar] [CrossRef]
- Christmas, K.M.; Bassingthwaighte, J.B. Equations for O2 and CO2 Solubilities in Saline and Plasma: Combining Temperature and Density Dependences. J. Appl. Physiol. 2017, 122, 1313–1320. [Google Scholar] [CrossRef] [Green Version]
- Lei, Z.; Dai, C.; Chen, B. Gas Solubility in Ionic Liquids. Chem. Rev. 2014, 114, 1289–1326. [Google Scholar] [CrossRef]
- Aki, S.N.V.K.; Mellein, B.R.; Saurer, E.M.; Brennecke, J.F. High-Pressure Phase Behavior of Carbon Dioxide with Imidazolium-Based Ionic Liquids. J. Phys. Chem. B 2004, 108, 20355–20365. [Google Scholar] [CrossRef]
- Anthony, J.L.; Anderson, J.L.; Maginn, E.J.; Brennecke, J.F. Anion Effects on Gas Solubility in Ionic Liquids. J. Phys. Chem. B 2005, 109, 6366–6374. [Google Scholar] [CrossRef] [Green Version]
- Anthony, J.L.; Maginn, E.J.; Brennecke, J.F. Solubilities and Thermodynamic Properties of Gases in the Ionic Liquid 1- n -Butyl-3-Methylimidazolium Hexafluorophosphate. J. Phys. Chem. B 2002, 106, 7315–7320. [Google Scholar] [CrossRef]
- Barry, J.P.; Buck, K.R.; Lovera, C.; Brewer, P.G.; Seibel, B.A.; Drazen, J.C.; Tamburri, M.N.; Whaling, P.J.; Kuhnz, L.; Pane, E.F. The Response of Abyssal Organisms to Low PH Conditions during a Series of CO2-Release Experiments Simulating Deep-Sea Carbon Sequestration. Deep Sea Res. Part II Top. Stud. Oceanogr. 2013, 92, 249–260. [Google Scholar] [CrossRef]
- Leung, D.Y.C.; Caramanna, G.; Maroto-Valer, M.M. An Overview of Current Status of Carbon Dioxide Capture and Storage Technologies. Renew. Sustain. Energy Rev. 2014, 39, 426–443. [Google Scholar] [CrossRef] [Green Version]
- Laizāns, A. Analytical model and simulation of oxygen solubility in wastewater. In Proceedings of the International Scientific Conference, Jelgava, Latvia, 28–30 May 2012; pp. 109–114. [Google Scholar]
- Seewald, J.S.; Doherty, K.W.; Hammar, T.R.; Liberatore, S.P. A New Gas-Tight Isobaric Sampler for Hydrothermal Fluids. Deep Sea Res. Part I Oceanogr. Res. Pap. 2002, 49, 189–196. [Google Scholar] [CrossRef]
- Hallett, J.P.; Welton, T. Room-Temperature Ionic Liquids: Solvents for Synthesis and Catalysis. Chem. Rev. 2011, 111, 3508–3576. [Google Scholar] [CrossRef]
- Blanchard, L.A.; Gu, Z.; Brennecke, J.F. High-Pressure Phase Behavior of Ionic Liquid/CO2 Systems. J. Phys. Chem. B 2001, 105, 2437–2444. [Google Scholar] [CrossRef] [Green Version]
- Chauvin, Y.; Mussmann, L.; Olivier, H. A Novel Class of Versatile Solvents for Two-Phase Catalysis: Hydrogenation, Isomerization, and Hydroformylation of Alkenes Catalyzed by Rhodium Complexes in Liquid 1,3-Dialkylimidazolium Salts. Angew. Chem. Int. Ed. Engl. 1996, 34, 2698–2700. [Google Scholar] [CrossRef]
- Fonseca, I.M.A.; Almeida, J.P.B.; Fachada, H.C. Automated Apparatus for Gas Solubility Measurements. J. Chem. Thermodyn. 2007, 39, 1407–1411. [Google Scholar] [CrossRef] [Green Version]
- Dohrn, R.; Peper, S.; Fonseca, J.M.S. High-Pressure Fluid-Phase Equilibria: Experimental Methods and Systems Investigated (2000–2004). Fluid Phase Equilibria 2010, 288, 1–54. [Google Scholar] [CrossRef]
- Bunsen, R. Ueber das Gesetz der Gasabsorption. Ann. Chem. Pharm. 1855, 93, 1–50. [Google Scholar] [CrossRef]
- Bunsen, R. On the law of absorption of gases. Phil. Mag. Ser. 4 1855, 9, 116–130. [Google Scholar] [CrossRef]
- Bunsen, R. Memoire sur la loi de l’absorption des gaz par les liquides. Ann. Chim. Phys. Ser. 3 1855, 43, 496–507. [Google Scholar]
- Naim, A.B.; Baer, S. Method for Measuring Solubilities of Slightly Soluble Gases in Liquids. Trans. Faraday Soc. 1963, 59, 2735. [Google Scholar] [CrossRef]
- Liu, N.; Aymonier, C.; Lecoutre, Y.; Garrabos, Y.; Marre, S. Microfluidic approach for studying CO2 solubility in water and brine using confocal Raman spectroscopy. Chem. Phys. Lett. 2012, 551, 139–143. [Google Scholar] [CrossRef]
- Caumon, M.-C.; Sterpenich, J.; Randi, A.; Pironon, J. Measuring Mutual Solubility in the H2O–CO2 System up to 200 Bar and 100 °C by in Situ Raman Spectroscopy. Int. J. Greenh. Gas Con. 2016, 47, 63–70. [Google Scholar] [CrossRef]
- Guo, H.; Huang, Y.; Chen, Y.; Zhou, Q. Quantitative Raman Spectroscopic Measurements of CO2 Solubility in NaCl Solution from (273.15 to 473.15) K at p = (10.0, 20.0, 30.0, and 40.0) MPa. J. Chem. Eng. Data 2016, 61, 466–474. [Google Scholar] [CrossRef]
- Wang, Z.; Zhou, Q.; Guo, H.; Yang, P.; Lu, W. Determination of water solubility in supercritical CO2 from 313.15 to 473.15 K and from 10 to 50 MPa by in-situ quantitative Raman spectroscopy. Fluid Phase Equilibr. 2018, 476, 170–178. [Google Scholar] [CrossRef]
- Lipinski, G.; Holzammer, C.; Petermann, M.; Richter, M. Measurement of Sorption Phenomena near Dew Points of Fluid Mixtures: Concept for the Combination of Gravimetric Sorption Analysis and Raman Spectroscopy. Meas. Sci. Technol. 2018, 29, 105501. [Google Scholar] [CrossRef]
- Holzammer, C.; Finckenstein, A.; Will, S.; Braeuer, A.S. How Sodium Chloride Salt Inhibits the Formation of CO2 Gas Hydrates. J. Phys. Chem. B 2016, 120, 2452–2459. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lemmon, E.; Harvey, A.; Hardin, G.; Bell, I.; Huber, M.; McLinden, M. NIST Reference Fluid Thermodynamic and Transport Properties Database (REFPROP), Version 10—SRD 23; National Institute of Standards and Technology: Boulder, CO, USA, 2018. [Google Scholar]
- Span, R.; Beckmüller, R.; Hielscher, S.; Jäger, A.; Mickoleit, E.; Neumann, T.; Pohl, S.; Semrau, B.; Thol, M. TREND. Thermodynamic Reference and Engineering Data 5.0; Ruhr University Bochum: Bochum, Germany, 2020. [Google Scholar]
- Wagner, W.; Pruss, A. International Equations for the Saturation Properties of Ordinary Water Substance. Revised According to the International Temperature Scale of 1990. Addendum to J. Phys. Chem. Ref. Data 16, 893 (1987). J. Phys. Chem. Ref. Data 1993, 22, 783–787. [Google Scholar] [CrossRef] [Green Version]
- Soldatović, D.; Vuksanović, J.; Radović, I.; Višak, Z.; Kijevčanin, M. Excess Molar Volumes and Viscosity Behaviour of Binary Mixtures of Aniline/or N,N-Dimethylaniline with Imidazolium Ionic Liquids Having Triflate or Bistriflamide Anion. J. Chem. Thermodyn. 2017, 109, 137–154. [Google Scholar] [CrossRef] [Green Version]
- Huo, Y.; Xia, S.; Ma, P. Densities of Ionic Liquids, 1-Butyl-3-Methylimidazolium Hexafluorophosphate and 1-Butyl-3-Methylimidazolium Tetrafluoroborate, with Benzene, Acetonitrile, and 1-Propanol at T = (293.15 to 343.15) K. J. Chem. Eng. Data 2007, 52, 2077–2082. [Google Scholar] [CrossRef]
- Kiefer, J. Recent Advances in the Characterization of Gaseous and Liquid Fuels by Vibrational Spectroscopy. Energies 2015, 8, 3165–3197. [Google Scholar] [CrossRef] [Green Version]
- Span, R.; Wagner, W. A New Equation of State for Carbon Dioxide Covering the Fluid Region from the Triple-Point Temperature to 1100 K at Pressures up to 800 MPa. J. Phys. Chem. Ref. Data 1996, 25, 1509–1596. [Google Scholar] [CrossRef] [Green Version]
- Woodward, L.A.; George, J.H.B. Refractive Index Correction in Relative Raman Intensity Measurements. Nature 1951, 167, 193. [Google Scholar] [CrossRef]
- Riazi, M.R.; Roomi, Y.A. Use of the Refractive Index in the Estimation of Thermophysical Properties of Hydrocarbons and Petroleum Mixtures. Ind. Eng. Chem. Res. 2001, 40, 1975–1984. [Google Scholar] [CrossRef]
- Tariq, M.; Forte, P.A.S.; Gomes, M.F.C.; Lopes, J.N.C.; Rebelo, L.P.N. Densities and Refractive Indices of Imidazolium- and Phosphonium-Based Ionic Liquids: Effect of Temperature, Alkyl Chain Length, and Anion. J. Chem. Thermodyn. 2009, 41, 790–798. [Google Scholar] [CrossRef]
- Guide to the Expression of Uncertainty in Measurement, 1st ed.; International Bureau of Weights and Measures; International Organization for Standardization (Eds.) International Organization for Standardization: Genève, Switzerland, 1993; ISBN 978-92-67-10188-0. [Google Scholar]
- Anderson, G.R. The Raman Spectra of Carbon Dioxide in Liquid Water and Water-D2. J. Phys. Chem. 1977, 81, 273–276. [Google Scholar] [CrossRef]
- Wiebe, R.; Gaddy, V.L. The Solubility of Carbon Dioxide in Water at Various Temperatures from 12 to 40° and at Pressures to 500 Atmospheres. Critical Phenomena. J. Am. Chem. Soc. 1940, 62, 815–817. [Google Scholar] [CrossRef]
- Ashcroft, S.J.; Isa, M.B. Effect of Dissolved Gases on the Densities of Hydrocarbons. J. Chem. Eng. Data 1997, 42, 1244–1248. [Google Scholar] [CrossRef]
- Watanabe, H.; Iizuka, K. The Influence of Dissolved Gases on the Density of Water. Metrologia 1985, 21, 19–26. [Google Scholar] [CrossRef]


| Chemical Name | Source | Purity |
|---|---|---|
| Carbon dioxide | Air Liquide | 99.9999% |
| Helium | Air Liquide | 99.9999% |
| 1-Butyl-3-methyl-imidazolium-trifluormethansulfonat | Sigma-Aldrich | ≥95.0% |
| 1-Butyl-3-methyl-imidazolium-hexafluorophosphat | Sigma-Aldrich | ≥97.0% |
| 1-Butyl-3-methylimidazolium-bis(trifluormethylsulfonyl)imid | Sigma-Aldrich | ≥98.0% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lipinski, G.; Richter, M. Raman Sensor for the Determination of Gas Solubility. Physchem 2021, 1, 176-188. https://doi.org/10.3390/physchem1020012
Lipinski G, Richter M. Raman Sensor for the Determination of Gas Solubility. Physchem. 2021; 1(2):176-188. https://doi.org/10.3390/physchem1020012
Chicago/Turabian StyleLipinski, Gregor, and Markus Richter. 2021. "Raman Sensor for the Determination of Gas Solubility" Physchem 1, no. 2: 176-188. https://doi.org/10.3390/physchem1020012
APA StyleLipinski, G., & Richter, M. (2021). Raman Sensor for the Determination of Gas Solubility. Physchem, 1(2), 176-188. https://doi.org/10.3390/physchem1020012







