Striking a Balance between Conservation and Development: A Geospatial Approach to Watershed Prioritisation in the Himalayan Basin
Abstract
:1. Introduction
2. Materials and Methods
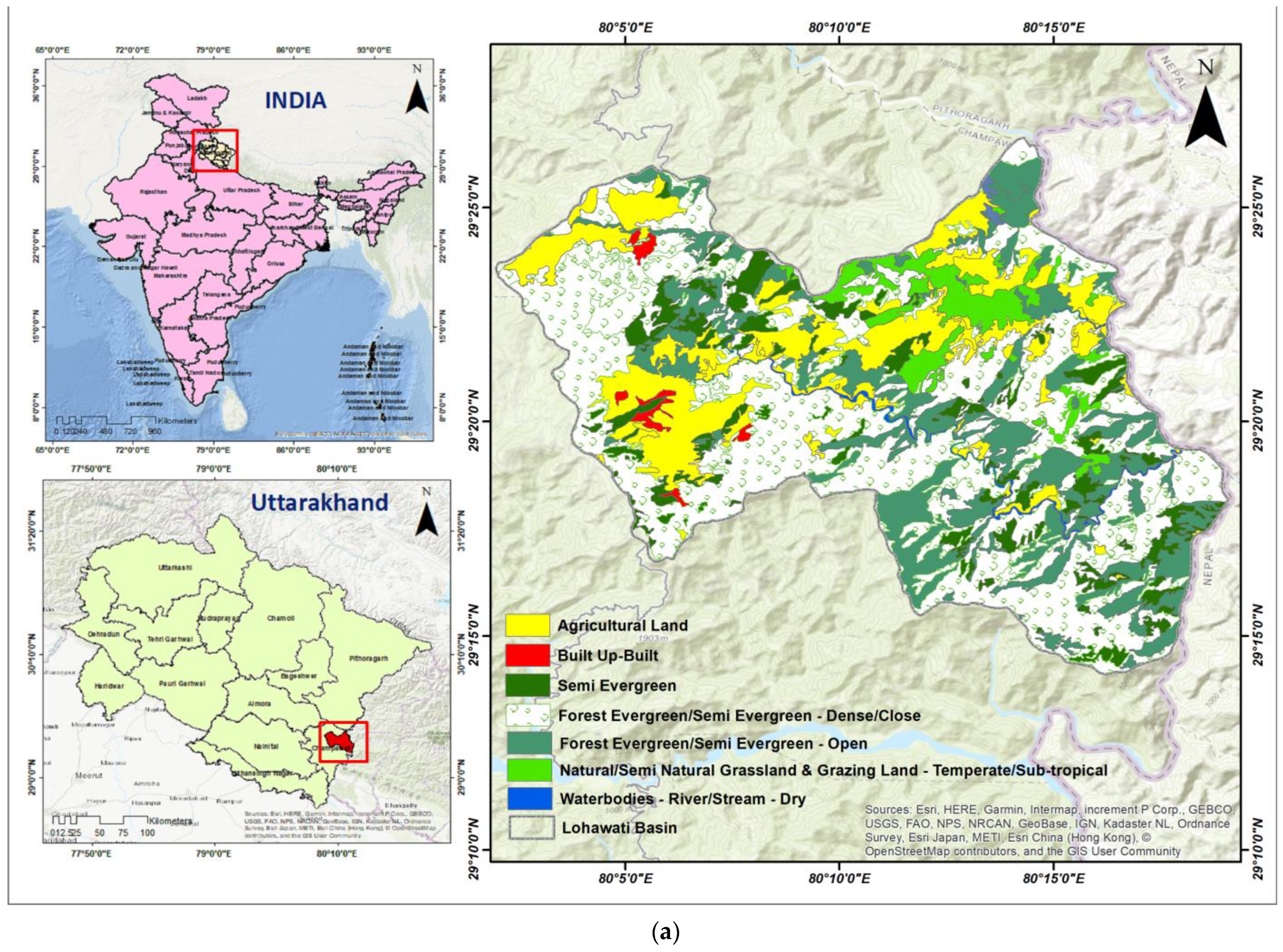
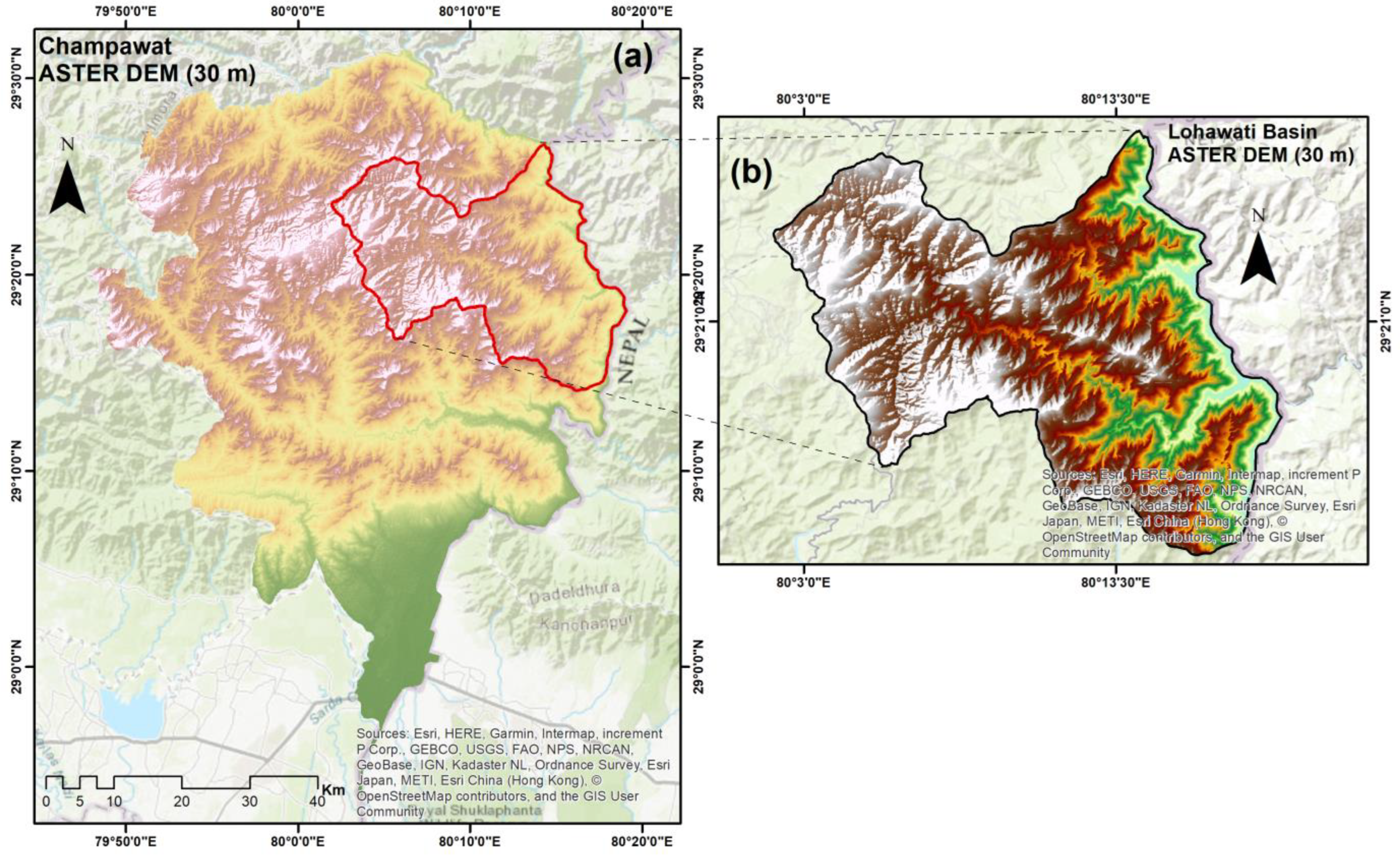
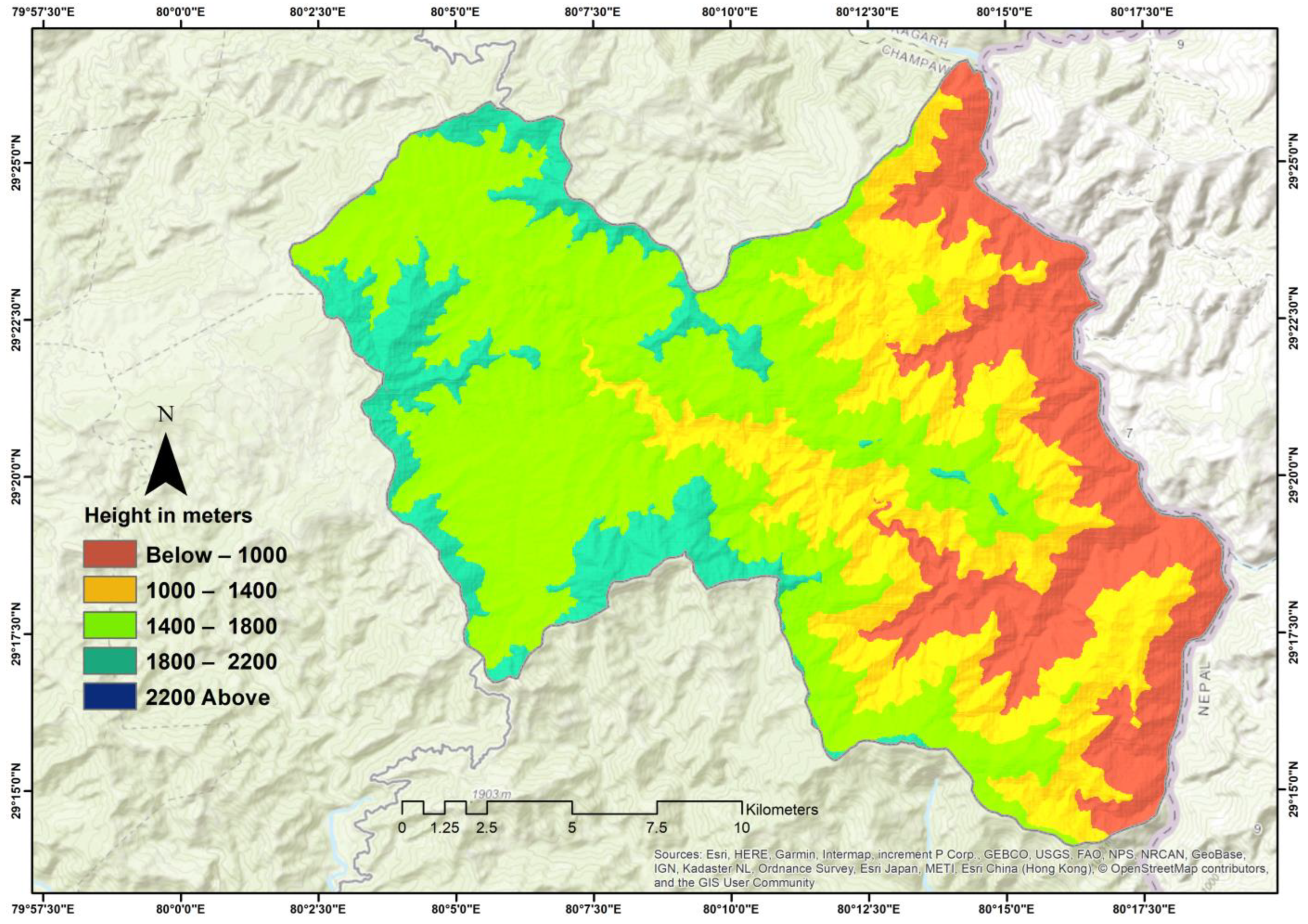
2.1. Description of Study Site
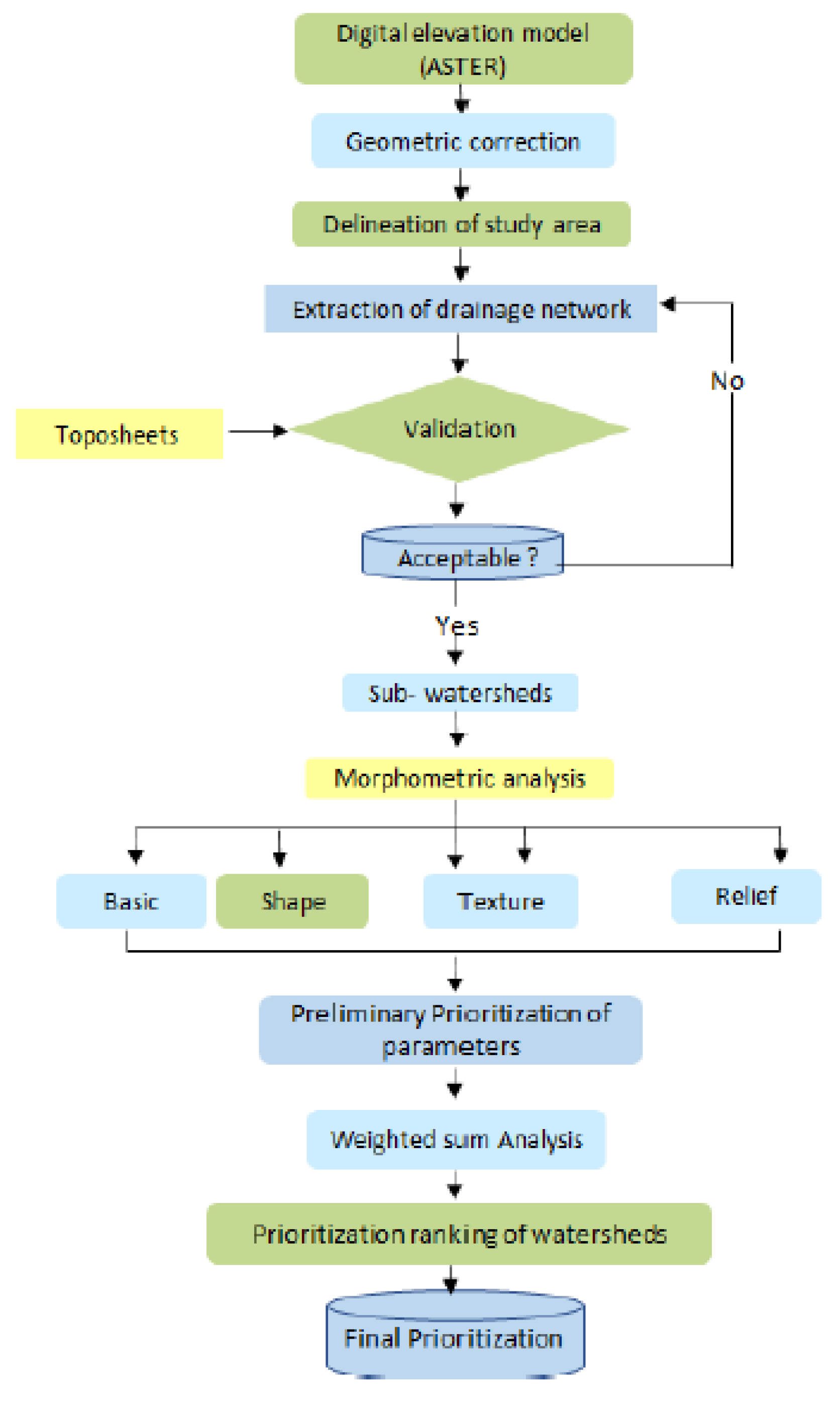
2.2. Data Used and Methodology
2.3. ASTER-30 m
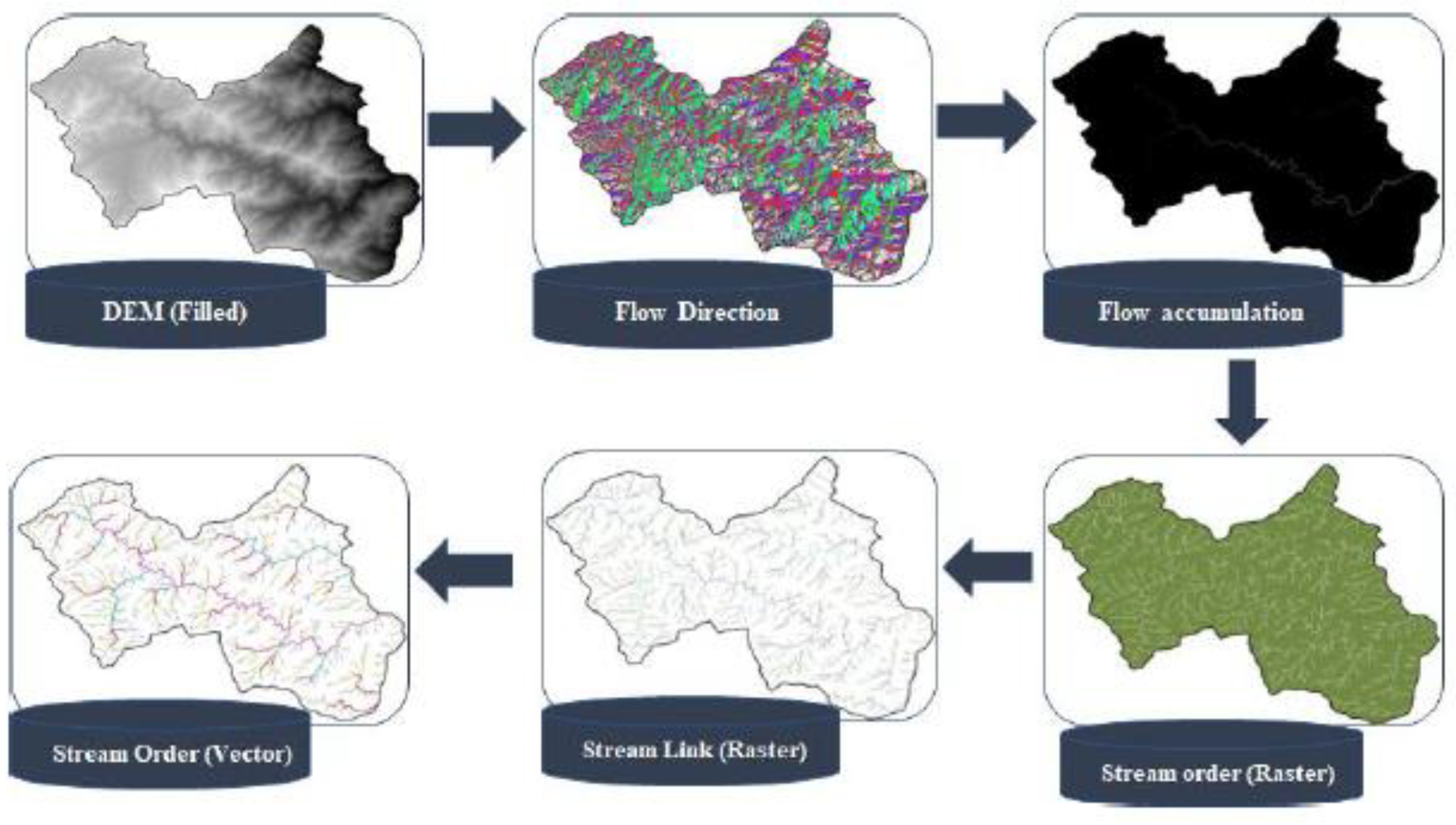
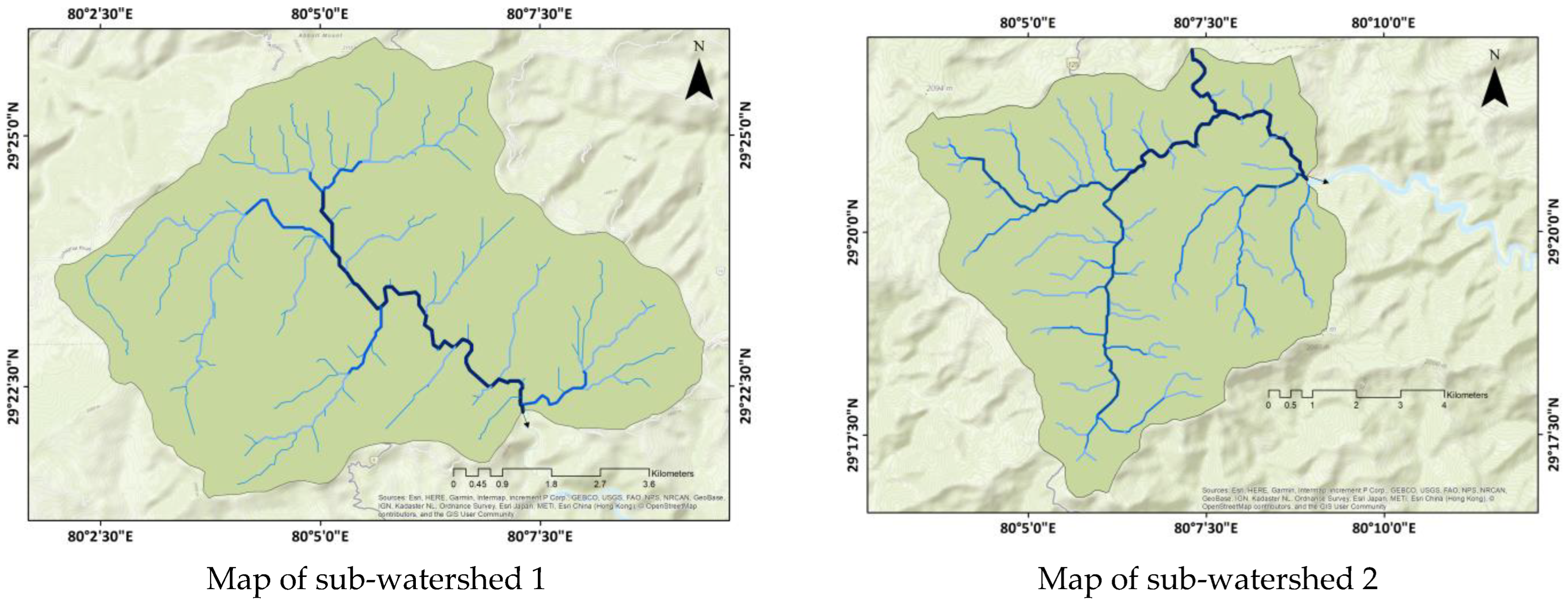
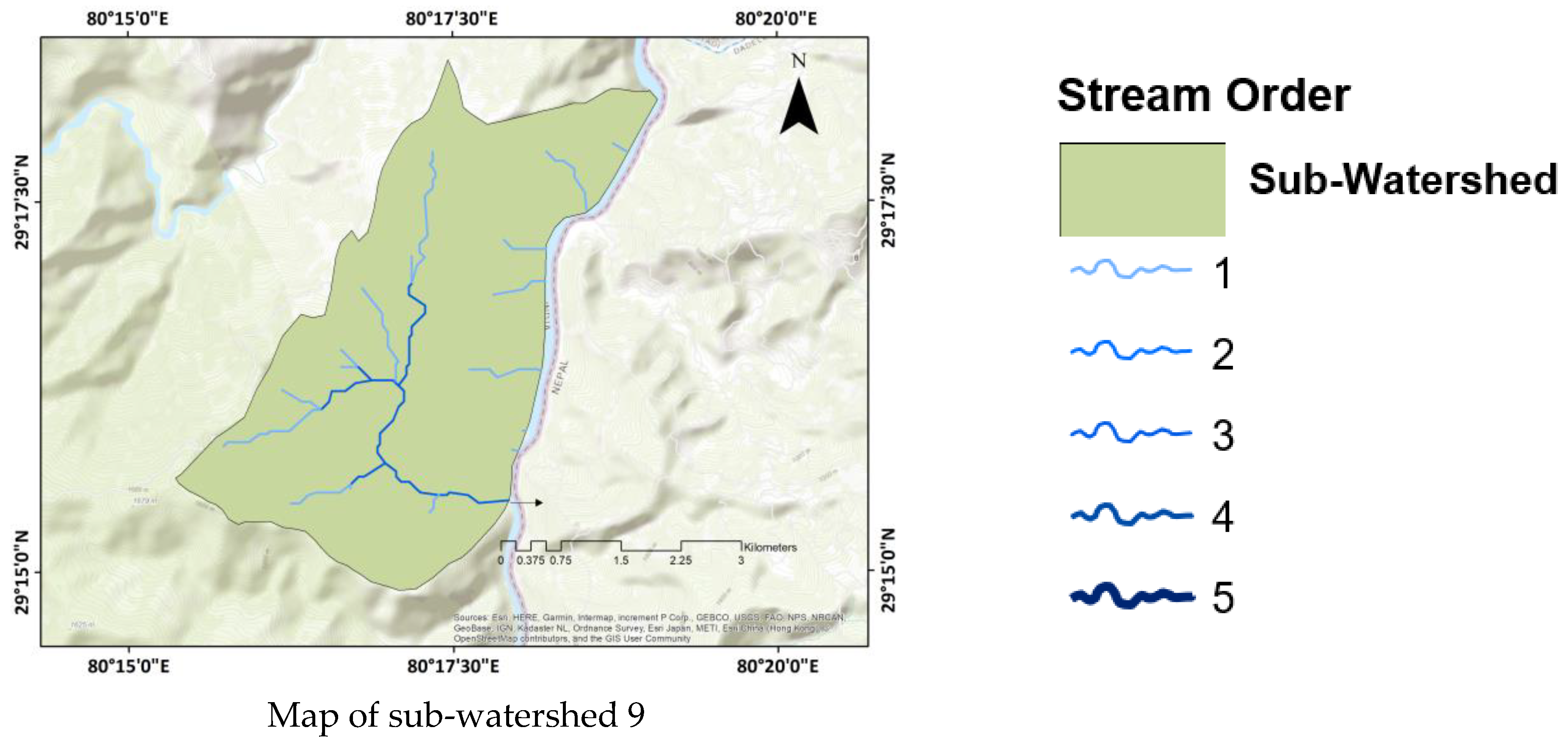
2.4. Extraction of Drainage Network
2.5. Calculation of Morphometric Characteristics
2.6. Prioritisation of Sub-Watersheds
3. Results and Discussion
3.1. Basic Morphometric Parameters
3.2. Shape Morphometric Parameters
3.3. Texture Morphometric Parameters
3.4. Relief Morphometric Parameters
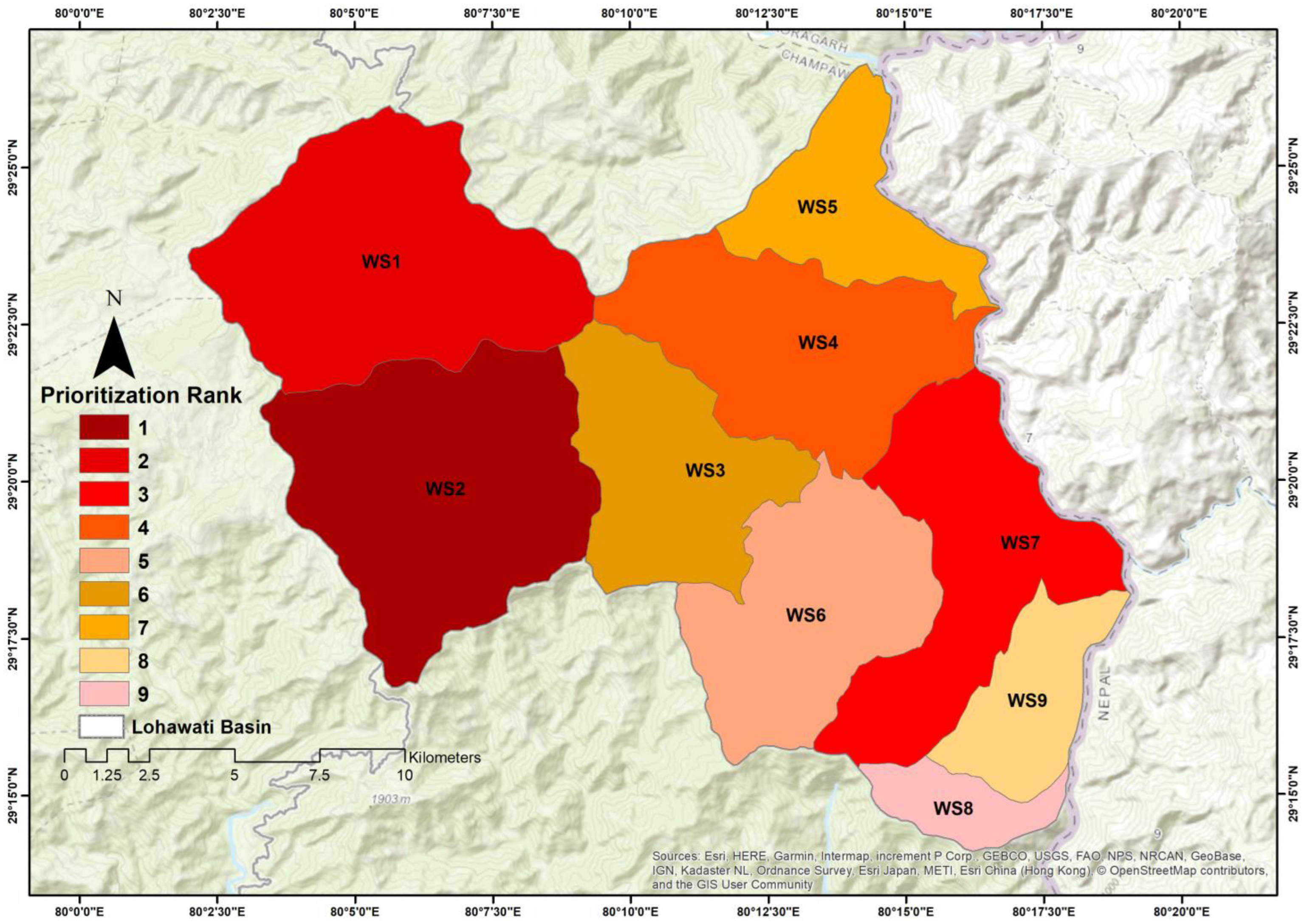
3.5. Prioritisation of the Watersheds
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Chopra, R.; Dhiman, R.D.; Sharma, P.K. Morphometric analysis of sub-watersheds in Gurdaspur district, Punjab using remote sensing and GIS techniques. J. Indian. Soc. Remote Sens. 2005, 33, 531–539. [Google Scholar] [CrossRef]
- Ghosh, M.; Gope, D. Hydro-morphometric characterization and prioritization of sub-watersheds for land and water resource management using fuzzy analytical hierarchical process (FAHP): A case study of upper Rihand watershed of Chhattisgarh State, India. Appl. Water. Sci. 2021, 11, 1–20. [Google Scholar] [CrossRef]
- Prabhakar, A.K.; Singh, K.K.; Lohani, A.K.; Chandniha, S.K. Study of Champua watershed for management of resources by using morphometric analysis and satellite imagery. Appl. Water. Sci. 2019, 9, 127. [Google Scholar] [CrossRef]
- Raj, P.N.; Azeez, P.A. Morphometric analysis of a tropical medium river system: A case from Bharathapuzha River Southern India. Open. J. Modern. Hydrol. 2012, 2, 91–98. [Google Scholar]
- Altaf, F.; Meraj, G.; Romshoo, S.A. Morphometric analysis to infer hydrological behaviour of Lidder watershed, Western Himalaya, India. Geog. J. 2013, 2013, 178021. [Google Scholar] [CrossRef]
- Puno, G.R.; Puno, R.C.C. Watershed conservation prioritization using geomorphometric and land use-land cover parameters. Glob. J. Environ. Sci. Manag. 2019, 5, 279–294. [Google Scholar]
- Jasmin, I.; Mallikarjuna, P. Morphometric analysis of Araniar river basin using remote sensing and geographical information system in the assessment of groundwater potential. Arab. J. Geosci. 2013, 6, 3683–3692. [Google Scholar] [CrossRef]
- Horton, R.E. Drainage-basin characteristics. Trans. Am. Geophy. Union. 1932, 13, 350–361. [Google Scholar] [CrossRef]
- Horton, R.E. An approach toward a physical interpretation of infiltration-capacity. Soil Sci. Soc. Am. J. 1941, 5, 399–417. [Google Scholar] [CrossRef]
- Miller, V.C. Quantitative Geomorphic Study of Drainage Basin Characteristics in the Clinch Mountain area, Virginia and Tennessee; Technical Report; Columbia University: New York, NY, USA, 1953; pp. 389–402. [Google Scholar]
- Schumm, S.A. Evolution of drainage systems and slopes in badlands at Perth Amboy, New Jersey. Geol. Soc. Am. Bull. 1956, 67, 597–646. [Google Scholar] [CrossRef]
- Strahler, A. Statistical Analysis in Geomorphic Research. J. Geol. 1954, 62, 1. [Google Scholar] [CrossRef]
- Strahler, A.N. Quantitative analysis of watershed geomorphology. Trans. Am. Geophy. Union. 1957, 38, 913–920. [Google Scholar] [CrossRef]
- Strahler, A.N. Part II. Quantitative geomorphology of drainage basins and channel networks. In Handbook of Applied Hydrology; McGraw-Hill: New York, NY, USA, 1964; pp. 4–39. [Google Scholar]
- Das, S.; Pardeshi, S.D. ; Pardeshi, S.D. Morphometric analysis of Vaitarna and Ulhas river basins, Maharashtra, India: Using geospatial techniques. Appl. Water. Sci. 2018, 8, 158. [Google Scholar] [CrossRef]
- Ganie, P.A.; Posti, R.; Kumar, P.; Singh, A. Morphometric analysis of a Kosi River Basin, Uttarakhand using geographical information system. Int. J. Multidisc. Current Res. 2016, 4, 1190–1200. [Google Scholar]
- Ganie, P.A.; Posti, R.; Aswal, A.S.; Bharti, V.S.; Sehgal, V.K.; Sarma, D.; Pandey, P.K. A comparative analysis of the vertical accuracy of multiple open-source digital elevation models for the mountainous terrain of the north-western Himalaya. Model. Earth Syst. Environ. 2023, 9, 2723–2743. [Google Scholar] [CrossRef]
- Khatoon, T.; Javed, A. Morphometric Behavior of Shahzad Watershed, Lalitpur District, Uttar Pradesh, India: A Geospatial Approach. J. Geogr. Inf Syst. 2022, 14, 193–220. [Google Scholar] [CrossRef]
- Kumar, L.; Joshi, G.; Agarwal, K.K. Morphometry and Morphostructural Studies of the Parts of Gola River and Kalsa River Basins, Chanphi-Okhalkanda Region, Kumaun Lesser Himalaya, India. Geotectonics. 2020, 54, 410–427. [Google Scholar] [CrossRef]
- Mangan, P.; Haq, M.A.; Baral, P. Morphometric analysis of watershed using remote sensing and GIS—A case study of Nanganji River Basin in Tamil Nadu, India. Arab. J. Geosci. 2019, 12, 202. [Google Scholar] [CrossRef]
- Manjare, B.S.; Padhye, M.A.; Girhe, S.S. Morphometric analysis of a Lower Wardha River sub basin of Maharashtra, India Using ASTER DEM Data and GIS. Proceedings of Geo-Enabling Digital India, 15th ESRI India User Conference, New Delhi, India, 9–11 December 2014; pp. 1–13. [Google Scholar]
- Muhtadi, A.; Aldiano, R.; Leidonald, R. Morphometric characteristics of the Alas-Singkil drainage basins. In IOP Conference Series: Earth and Environmental Science; IOP Publishing: Bristol, UK, 2022; Volume 977, p. 012090. [Google Scholar]
- Soni, S. Assessment of morphometric characteristics of Chakrar watershed in Madhya Pradesh India using geospatial technique. Appl. Water. Sci. 2017, 7, 2089–2102. [Google Scholar] [CrossRef]
- Tassew, B.G.; Belete, M.A.; Miegel, K. Assessment and analysis of morphometric characteristics of Lake Tana sub-basin, Upper Blue Nile Basin, Ethiopia. Int. J. River Basin. Manag. 2021, 21, 195–209. [Google Scholar] [CrossRef]
- Umrikar, B.N. Morphometric analysis of Andhale watershed, Taluka Mulshi, District Pune, India. Appl. Water. Sci. 2017, 7, 2231–2243. [Google Scholar] [CrossRef]
- Moore, I.D.; Grayson, R.B.; Ladson, A.R. Digital terrain modelling: A review of hydrological, geomorphological, and biological applications. Hydrol. Process. 1991, 5, 3–30. [Google Scholar] [CrossRef]
- Bajirao, T.S.; Kumar, P.K.; Kumar, P.K.; Tarate, C.; Bajirao, S. Application of remote sensing and GIS for morphometric analysis of watershed: A Review. Int. J. Chem. Stud. 2019, 7, 709–713. [Google Scholar]
- Choudhari, P.P.; Nigam, G.K.; Singh, S.K.; Thakur, S. Morphometric based prioritization of watershed for groundwater potential of Mula river basin, Maharashtra, India. Geol. Ecol. Landscapes 2018, 2, 256–267. [Google Scholar] [CrossRef]
- Khurana, D.; Rawat, S.S.; Raina, G.; Sharma, R.; Jose, P.G. GIS-Based Morphometric Analysis and Prioritization of Upper Ravi Catchment, Himachal Pradesh, India. In Advances in Water Resources Engineering and Management; Springer: Singapore, 2019; pp. 163–185. [Google Scholar] [CrossRef]
- Harsha, J.; Ravikumar, A.S.; Shivakumar, B.L. Evaluation of morphometric parameters and hypsometric curve of Arkavathy river basin using RS and GIS techniques. Appl. Water Sci. 2020, 10, 86. [Google Scholar] [CrossRef]
- Shivhare, N.; Rahul, A.K.; Omar, P.J.; Chauhan, M.S.; Gaur, S.; Dikshit, P.K.S.; Dwivedi, S.B. Identification of critical soil erosion prone areas and prioritization of micro-watersheds using geoinformatics techniques. Ecol. Eng. 2018, 121, 26–34. [Google Scholar] [CrossRef]
- Pandey, A.; Chowdary, V.M.; Mal, B.C. Identification of critical erosion prone areas in the small agricultural watershed using USLE, GIS and remote sensing. Water Resour. Manag. 2006, 21, 729–746. [Google Scholar] [CrossRef]
- Arabameri, A.; Pradhan, B.; Pourghasemi, H.R.; Rezaei, K. Identification of erosion-prone areas using different multi-criteria decision-making techniques and GIS. Geomat. Nat. Hazards Risk 2018, 9, 1129–1155. [Google Scholar] [CrossRef]
- Jain, P.; Ramsankaran, R. GIS-based integrated multi-criteria modelling framework for watershed prioritisation in India—A demonstration in Marol watershed. J. Hydrol. 2019, 578, 124131. [Google Scholar] [CrossRef]
- Jaiswal, R.; Ghosh, N.; Galkate, R.; Thomas, T. Multi Criteria Decision Analysis (MCDA) for Watershed Prioritization. Aquat. Procedia 2015, 4, 1553–1560. [Google Scholar] [CrossRef]
- Rahaman, S.A.; Ajeez, S.A.; Aruchamy, S.; Jegankumar, R. Prioritization of Sub Watershed Based on Morphometric Characteristics Using Fuzzy Analytical Hierarchy Process and Geographical Information System—A Study of Kallar Watershed, Tamil Nadu. Aquat. Procedia 2015, 4, 1322–1330. [Google Scholar] [CrossRef]
- Rahmati, O.; Haghizadeh, A.; Stefanidis, S. Assessing the Accuracy of GIS-Based Analytical Hierarchy Process for Watershed Prioritization; Gorganrood River Basin, Iran. Water Resour. Manag. 2015, 30, 1131–1150. [Google Scholar] [CrossRef]
- Shivhare, V.; Gupta, C.; Mallick, J.; Singh, C.K. Geospatial modelling for sub-watershed prioritization in Western Himalayan Basin using morphometric parameters. Nat. Hazards 2021, 110, 545–561. [Google Scholar] [CrossRef]
- Toosi, S.R.; Samani, J.M.V. Prioritizing watersheds using a novel hybrid decision model based on fuzzy DEMATEL, fuzzy ANP and fuzzy VIKOR. Water Resour. Manag. 2017, 31, 2853–2867. [Google Scholar] [CrossRef]
- Aher, P.; Adinarayana, J.; Gorantiwar, S. Quantification of morphometric characterization and prioritization for management planning in semi-arid tropics of India: A remote sensing and GIS approach. J. Hydrol. 2014, 511, 850–860. [Google Scholar] [CrossRef]
- Kadam, A.K.; Jaweed, T.H.; Kale, S.S.; Umrikar, B.N.; Sankhua, R.N. Identification of erosion-prone areas using modified morphometric prioritization method and sediment production rate: A remote sensing and GIS approach. Geomat. Nat. Hazards Risk 2019, 10, 986–1006. [Google Scholar] [CrossRef]
- Malik, A.; Kumar, A.; Kandpal, H. Morphometric analysis and prioritization of sub-watersheds in a hilly watershed using weighted sum approach. Arab. J. Geosci. 2019, 12, 118. [Google Scholar] [CrossRef]
- Ayele, G.T.; Teshale, E.Z.; Yu, B.; Rutherfurd, I.D.; Jeong, J. Streamflow and Sediment Yield Prediction for Watershed Prioritization in the Upper Blue Nile River Basin, Ethiopia. Water 2017, 9, 782. [Google Scholar] [CrossRef]
- Bali, Y.P.; Karale, R.L. Sediment Yield Index as a Criterion for Choosing Priority Basins; IAHS-AISH Publication: Paris, France, 1977; pp. 180–188. [Google Scholar]
- Chakraborti, A.K. Sediment yield prediction and prioritization of watershed using remote sensing data. In Proceedings of the 12th Asian Conference on Remote Sensing, Singapore, 30 October–5 November 1991. [Google Scholar]
- Gajbhiye, S.; Sharma, S.K.; Meshram, C. Prioritization of Watershed through Sediment Yield Index Using RS and GIS Approach. Int. J. u- and e-Serv. Sci. Technol. 2014, 7, 47–60. [Google Scholar] [CrossRef]
- Kumar, K.A.; Sandeep, P.; Masilamani, P. Prioritization of Watershed using Sediment Yield Index Method: A Case study of Semi-Arid Ecosystem of South India. Environ. We Int. J. Sci. Tech. 2021, 16, 1–13. [Google Scholar]
- Naqvi, H.R.; Athick, A.M.A.; Ganaie, H.A.; Siddiqui, M.A. Soil erosion planning using sediment yield index method in the Nun Nadi watershed, India. Int. Soil Water Conserv. Res. 2015, 3, 86–96. [Google Scholar] [CrossRef]
- Rajasekhar, M.; Raju, G.S.; Raju, R.S. Morphometric analysis of the Jilledubanderu River Basin, Anantapur District, Andhra Pradesh, India, using geospatial technologies. Groundw. Sustain. Dev. 2020, 11, 100434. [Google Scholar] [CrossRef]
- Ratnam, K.N.; Srivastava, Y.K.; Rao, V.V.; Amminedu, E.; Murthy, K.S.R. Check dam positioning by prioritization of micro-watersheds using SYI model and morphometric analysis—Remote sensing and GIS perspective. J. Indian Soc. Remote Sens. 2005, 33, 25–38. [Google Scholar] [CrossRef]
- Samal, D.R.; Gedam, S.S.; Nagarajan, R. GIS based drainage morphometry and its influence on hydrology in parts of Western Ghats region, Maharashtra, India. Geocarto Int. 2015, 30, 755–778. [Google Scholar] [CrossRef]
- Ayadi, I.; Abida, H.; Djebbar, Y.; Mahjoub, M.R. Sediment yield variability in central Tunisia: A quantitative analysis of its controlling factors. Hydrol. Sci. J.-J. Des Sci. Hydrol. 2010, 55, 446–458. [Google Scholar] [CrossRef]
- Meshram, S.G.; Sharma, S.K. Prioritization of watershed through morphometric parameters: A PCA-based approach. Appl. Water Sci. 2015, 7, 1505–1519. [Google Scholar] [CrossRef]
- Sharma, S.K.; Tignath, S.; Gajbhiye, S.; Patil, R. Application of principal component analysis in grouping geomorphic parameters of Uttela watershed for hydrological modeling. Int. J. Remote Sens. Geosci. 2013, 2, 63–70. [Google Scholar]
- Bharath, A.; Kumar, K.K.; Maddamsetty, R.; Manjunatha, M.; Tangadagi, R.B.; Preethi, S. Drainage morphometry based sub-watershed prioritization of Kalinadi basin using geospatial technology. Environ. Chall. 2021, 5, 100277. [Google Scholar] [CrossRef]
- Farhan, Y.; Anaba, O. A Remote Sensing and GIS Approach for Prioritization of Wadi Shueib Mini-Watersheds (Central Jordan) Based on Morphometric and Soil Erosion Susceptibility Analysis. J. Geogr. Inf. Syst. 2016, 8, 1–19. [Google Scholar] [CrossRef]
- Gajbhiye, S.; Mishra, S.K.; Pandey, A. Prioritizing erosion-prone area through morphometric analysis: An RS and GIS perspective. Appl. Water Sci. 2013, 4, 51–61. [Google Scholar] [CrossRef]
- Mallick, J.; Shivhare, V.; Singh, C.K.; Al Subih, M. Prioritizing Watershed Restoration, Management, and Development Based on Geo-Morphometric Analysis in Asir Region of Saudi Arabia Using Geospatial Technology. Pol. J. Environ. Stud. 2022, 31, 1201–1222. [Google Scholar] [CrossRef] [PubMed]
- Valdiya, K.S. Geology of Kumaun Lesser Himalaya; Wadia Institute of Himalayan Geology: Dehradun, India, 1980; 291p. [Google Scholar]
- Valdiya, K.S. An outline of the structural set-up of Kumaun Himalaya. J. Geol. Soc. India 1979, 20, 145–157. [Google Scholar]
- IMD Indian Meteorological Department. 2022. Available online: https://mausam.imd.gov.in (accessed on 25 September 2022).
- Abrams, M.; Hook, S.; Ramachandran, B. ASTER User Handbook Version 2; Jet Propulsion Laboratory: Pasadena, CA, USA, 2002; Volume 2003. [Google Scholar]
- Ahmed, S.A.; Chandrashekarappa, K.N.; Raj, S.K.; Nischitha, V.; Kavitha, G. Evaluation of morphometric parameters derived from ASTER and SRTM DEM—A study on Bandihole sub-watershed basin in Karnataka. J. Indian Soc. Remote Sens. 2010, 38, 227–238. [Google Scholar] [CrossRef]
- Tarboton, D.G.; Bras, R.L.; Rodriguez-Iturbe, I. On the extraction of channel networks from digital elevation data. Hydrol. Process. 1991, 5, 81–100. [Google Scholar] [CrossRef]
- Horton, R.E. Erosional development of streams and their drainage basins: Hydrophysical approach to quantitative morphology. Bull. Geol. Soc. Am. 1945, 56, 275–370. [Google Scholar] [CrossRef]
- Sameena, M.; Krishnamurthy, J.; Jayaraman, V.; Ranganna, G. Evaluation of drainage networks developed in hard rock ter-rain. Geocarto Int. 2009, 24, 397–420. [Google Scholar] [CrossRef]
- Faniran, A. The index of drainage intensity: A provisional new drainage factor. Austr. J. Sci. 1968, 31, 326–330. [Google Scholar]
- Bhat, S.; Romshoo, S. Digital elevation model based watershed characteristics of upper watersheds of Jhelum basin. J. Appl. Hydro. 2009, 21, 23–34. [Google Scholar]
- Amiri, M.; Pourghasemi, H.R.; Arabameri, A.; Vazirzadeh, A.; Yousefi, H.; Kafaei, S. Prioritization of flood inundation of Ma-harloo Watershed in iran using morphometric parameters analysis and TOPSIS MCDM model. In Spatial Modeling in GIS and R for Earth and Environmental Sciences; Elsevier: Amsterdam, The Netherlands, 2019; pp. 371–390. [Google Scholar]
- Magesh, N.S.; Chandrasekar, N. GIS model-based morphometric evaluation of Tamiraparani subbasin, Tirunelveli district, Tamil Nadu, India. Arab. J. Geosci. 2012, 7, 131–141. [Google Scholar] [CrossRef]
- Costa, J.E. Hydraulics and basin morphometry of the largest flash floods in the conterminous United States. J. Hydrol. 1987, 93, 313–338. [Google Scholar] [CrossRef]
- Sreedevi, P.D.; Owais, S.H.H.K.; Khan, H.H.; Ahmed, S. Morphometric analysis of a watershed of South India using SRTM data and GIS. J. Geol. Soc. India. 2009, 73, 543–552. [Google Scholar] [CrossRef]
- Gregory, K.J.; Walling, D.E. Drainage Basin form and Process a Geomorphological Approach; Edward Arnold: London, UK, 1973; p. 456. [Google Scholar]
- Dar, R.A.; Chandra, R.; Romshoo, S.A. Morphotectonic and lithostratigraphic analysis of intermontane Karewa Basin of Kashmir Himalayas, India. J. Mt. Sci. 2013, 10, 1–15. [Google Scholar] [CrossRef]
- Reddy, G.P.O.; Maji, A.K.; Gajbhiye, K.S. Drainage morphometry and its influence on landform characteristics in a basaltic terrain, Central India—A remote sensing and GIS approach. Int. J. Appl. Earth Obs. Geoinf. 2004, 6, 1–16. [Google Scholar] [CrossRef]
- Yangchan, J.; Jain, A.K.; Tiwari, A.K.; Sood, A. Morphometric analysis of drainage basin through GIS: A case study of Sukhna Lake Watershed in Lower Shiwalik, India. Int. J. Sci. Eng. Res. 2015, 6, 1015–1023. [Google Scholar] [CrossRef]
- Vinutha, D.N.; Janardhana, M.R. Morphometry of The Payaswini Watershed, Coorg District, Karnataka, India, Using Remote Sensing and GIS. Techniques. Int. J. Innov. Res. Sci. Eng. Technol. 2014, 3, 516–524. [Google Scholar]
- Bull, W.B.; McFadden, L.D. Tectonic Geomorphology North and South of the Garlock Fault, California. In Geomorphology in Arid Regions: Proceedings of the Eighth Annual Geomorphology Symposium, State University of New York at Binghamton, Binghamton, NY, USA, 23–24 September 1977; Doehring, D.O., Ed.; George Allen & Unwin Ltd.: Crows Nest, Australia, 1977; pp. 115–138. [Google Scholar]
- Zaz, S.N.; Romshoo, S.A. Assessing the geoindicators of land degradation in the Kashmir Himalayan region, India. Nat. Hazards 2012, 64, 1219–1245. [Google Scholar] [CrossRef]
- Suresh, M.; Sudhakar, S.; Tiwari, K.N.; Chowdary, V.M. Prioritization of watersheds using morphometric parameters and assessment of surface water potential using remote sensing. J. Indian Soc. Remote Sens. 2004, 32, 249–259. [Google Scholar] [CrossRef]
- Melton, M.A. Geometric Properties of Mature Drainage Systems and Their Representation in an E4Phase Space. J. Geol. 1958, 66, 35–54. [Google Scholar] [CrossRef]
- Das, A.K.; Mukherjee, S. Drainage morphometry using satellite data and GIS in Raigad district, Maharashtra. J. Geol. Soc. India 2005, 65, 577–586. [Google Scholar]
- Ganie, P.A.; Posti, R.; Kunal, K.; Kunal, G.; Sarma, D.; Pandey, P.K. Insights into the morphometric characteristics of the Himalayan River using remote sensing and GIS techniques: A case study of Saryu basin, Uttarakhand, India. Appl. Geomat. 2022, 14, 707–730. [Google Scholar] [CrossRef]
- Mesa, L.M. Morphometric analysis of a subtropical Andean basin (Tucumán, Argentina). Environ. Geol. 2006, 50, 1235–1242. [Google Scholar] [CrossRef]
- Chorley, R.J. The drainage basin as the fundamental geomorphic unit. In Water, Earth and Man; Chorley, R.J., Ed.; Methuen: London, UK, 1969; pp. 77–98. [Google Scholar]
- Chow, V.T.; Maidment, D.; Mays, L.W. Applied Hydrology; McGraw-Hill: New York, NY, USA, 1988. [Google Scholar]
- Altın, T.B.; Altın, B.N. Drainage morphometry and its influence on landforms in volcanic terrain, Central Anatolia, Turkey. Procedia-Soc. Behav. Sci. 2011, 19, 732–740. [Google Scholar] [CrossRef]
- Angillieri, M.Y.E. Morphometric analysis of Colangüil river basin and flash flood hazard, San Juan, Argentina. Environ. Geol. 2007, 55, 107–111. [Google Scholar] [CrossRef]
- Potter, K.W.; Faulkner, E.B. Catchment response time as a predictor of flood quantiles. J. Am. Water Resour. Assoc. 1987, 23, 857–861. [Google Scholar] [CrossRef]
- Hadley, R.F.; Schumm, S.A. Sediment Sources and Drainage Basin Characteristics in Upper Cheyenne River Basin; USGS water supply paper-1531 B; Scientific Research: Washington, DC, USA, 1961; p. 198. [Google Scholar]
- Ahnert, F. Functional relationships between denudation, relief, and uplift in large, mid-latitude drainage basins. Am. J. Sci. 1970, 268, 243–263. [Google Scholar] [CrossRef]
- Mustak, S.K.; Baghmar, N.K.; Ratre, C.R. Measurement of Dissection Index of Pairi River Basin Using Remote Sensing and GIS. Natl. Geogr. J. India 2012, 58, 97–106. [Google Scholar]
- Gottschalk, L.C. Reservoir Sedimentation. In Handbook of Applied Hydrology; McGraw-Hill: New York, NY, USA, 1964. [Google Scholar]
- Vijith, H.; Satheesh, R. GIS based morphometric analysis of two major upland sub-watersheds of meenachil river in Kerala. J. Indian Soc. Remote Sens. 2006, 34, 181–185. [Google Scholar] [CrossRef]
- Selvan, M.T.; Ahmad, S.; Rashid, S.M. Analysis of the geomorphometric parameters in high altitude glacierized terrain using SRTM DEM data in Central Himalaya, India. ARPN J. Sci. Technol. 2011, 1, 22–27. [Google Scholar]
- Patton, P.C. Drainage basin morphometry and floods. In Flood Geomorphology; John Wiley & Sons: New York, NY, USA, 1988; pp. 51–64. [Google Scholar]
- Singh, W.R.; Barman, S.; Tirkey, G. Morphometric analysis and watershed prioritization in relation to soil erosion in Dudhnai Watershed. Appl. Water Sci. 2021, 11, 151. [Google Scholar] [CrossRef]
- Jain, M.K.; Das, D. Estimation of Sediment Yield and Areas of Soil Erosion and Deposition for Watershed Prioritization using GIS and Remote Sensing. Water Resour. Manag. 2009, 24, 2091–2112. [Google Scholar] [CrossRef]
- Javed, A.; Khanday, M.Y.; Rais, S. Watershed prioritization using morphometric and land use/land cover parameters: A remote sensing and GIS based approach. J. Geol. Soc. India 2011, 78, 63–75. [Google Scholar] [CrossRef]
- Ganie, P.A.; Posti, R.; Kunal, K.; Kunal, G.; Bharti, V.S.; Sehgal, V.K.; Sarma, D.; Pandey, P.K. Modelling of the Himalayan Mountain river basin through hydro-morphological and compound factor-based approaches using geoinformatics tools. Model. Earth Syst. Environ. 2023, 9, 3053–3084. [Google Scholar] [CrossRef]
- Adhami, M.; Sadeghi, S.H. Sub-watershed prioritization based on sediment yield using game theory. J. Hydrol. 2016, 541, 977–987. [Google Scholar] [CrossRef]
- Balasubramanian, A.; Duraisamy, K.; Thirumalaisamy, S.; Krishnaraj, S.; Yatheendradasan, R.K. Prioritization of subwatersheds based on quantitative morphometric analysis in lower Bhavani basin, Tamil Nadu, India using DEM and GIS techniques. Arab. J. Geosci. 2017, 10, 552. [Google Scholar] [CrossRef]
- Kruse, R.; Schwecke, E.; Heinsohn, J. Uncertainty and Vagueness in Knowledge Based Systems: Numerical Methods; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]



| S. No. | Data Type | Details of Data | Source |
|---|---|---|---|
| 1. | ASTER GDEM | 30 m resolution | http://demex.cr.usgs.gov/DEMEX/. (accessed on 5 July 2022) |
| 2. | SOI toposheets | 62C3, 62C4, and 62C7. | Survey of India, Dehradun, Uttarakhand, India |
| Morphometric Parameters | Symbol | Formula | References |
|---|---|---|---|
| Basic parameters | |||
| Basin area (km2) | A | GIS software (ArcGIS 10.8) analysis | [11] |
| Basin perimeter (km) | P | GIS software (ArcGIS 10.8) analysis | [11] |
| Basin length (km) | Lb | 1.312 × A0.568 | [11] |
| Where Lb = basin length | |||
| A = basin area (km2) | |||
| Stream number | Nu | Number of stream segments | [12] |
| Stream order | U | Hierarchical rank | [14] |
| Stream length (km) | Lu | Length of the stream segment | [65] |
| Mean stream length | Lsm | Lsm = Lu/Nu | [14] |
| Where Lsm = mean stream length | |||
| Lu = total stream length of order “u” | |||
| Nu = total no. of stream segments of order “u” | |||
| Stream length ratio | Rl | Rl = Lu/Lu-1 | [65] |
| Where Rl = stream length ratio | |||
| Lu = total stream length of order “u” | |||
| Lu-1 = total stream length of its next lower-order | |||
| Shape parameters | |||
| Form factor | Ff | Ff = A/Lb2 | [9] |
| Where Ff = form factor | |||
| A = area of the basin (km2) | |||
| Lb2 = square of basin length (km) | |||
| Circulatory ratio | Rc | Rc = 4 × Pi × A/P2 | [10] |
| Where Rc = circulatory ratio | |||
| Pi = “Pi” value, i.e., 3.14 | |||
| A = area of the basin (km2) | |||
| P = perimeter (km) | |||
| Elongation ratio | Re | Re = 2v (A/Pi/Lb) | [13] |
| Where Re = elongation ratio | |||
| Pi = “Pi” value, i.e., 3.14 | |||
| A = area of the basin (km2) | |||
| Lb = basin length (km) | |||
| Shape index | Si | Si = Lb2/A | [65] |
| Where Si = shape index | |||
| Lb = basin length | |||
| A = area of basin | |||
| Shape factor | Sf | Sf = Pu/Pc | [66] |
| Where Sf = shape factor | |||
| Pu = perimeter of the circle of watershed area | |||
| Pc = perimeter of watershed | |||
| Texture parameters | |||
| Length of overland flow (km) | Lg | Lg = 1/2 (Dd) | [65] |
| Where Lg = length of overland flow | |||
| Dd = drainage density | |||
| Drainage density (km km−2) | Dd | Dd = Lu/A | [9] |
| Where Dd = drainage density | |||
| Lu = total stream length of order “u” | |||
| A = area of the basin (km2) | |||
| Bifurcation ratio | Rb | Rb = Nu/Nu + 1 | [11] |
| Where Rb = bifurcation ratio | |||
| Nu = total no. of stream segments of order “u” | |||
| Nu + 1 = number of segments of the next higher-order | |||
| Mean bifurcation ratio | Rbm | Average of bifurcation ratio of all orders | [13] |
| Stream frequency (km−2) | Fs | Fs = Nu/A | [9] |
| Where Fs = stream frequency | |||
| Nu = total no. of streams of all orders | |||
| A = area of the basin (km2) | |||
| Constant of channel maintenance (km2 km−1) | C | C = 1/Dd | [11] |
| Where C = constant of channel maintenance | |||
| Dd = drainage density | |||
| Drainage intensity | Di | Di = Fs/Dd | [28,67] |
| Where Di = drainage Intensity | |||
| Fs = stream frequency | |||
| Dd = drainage density | |||
| Infiltration number | If | If = Fs × Dd | [67] |
| Where If = infiltration number | |||
| Fs = stream frequency | |||
| Dd = drainage density | |||
| Texture ratio | Rt | Rt = N1/P | [11] |
| Where Rt = texture ratio | |||
| N1 = number of 1st order streams | |||
| P = basin perimeter (km) | |||
| Drainage texture | Dt | Dt = Nu/P | [65] |
| Where Dt = drainage texture | |||
| Nu = total number of streams | |||
| P = perimeter (km) | |||
| Compactness coefficient | Cc | Cc = Pc/Pu | [68] |
| Where Pc = perimeter of watershed | |||
| Pu = perimeter of the circle of watershed area | |||
| Relief parameters | |||
| Height of basin mouth (km) | z | GIS analysis/DEM | |
| Maximum height of the basin (km) | Z | GIS analysis/DEM | |
| Total basin relief (km) | H | H = Z − z | [12] |
| Where H = total basin relief | |||
| Z = maximum height of the basin (km) | |||
| z = height of basin mouth (km) | |||
| Relief ratio | Rh | Rh = H/Lb | [11] |
| Where Rh = relief ratio | |||
| H = total relief of the basin (km) | |||
| Lb = basin length (km) | |||
| Relative relief | Rr | Rr = 100 H/P | [11] |
| Where Rr = relative relief | |||
| H = total relief of the basin (km) | |||
| P = perimeter (km) | |||
| Ruggedness number | Rn | Rn = Dd ×H | [14] |
| Where Rn = ruggedness number | |||
| Dd = drainage density | |||
| H = total basin relief (km) | |||
| (a) | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Code | Basin Length (km) | Basin Area (km2) | Basin Perimeter (km) | Stream Order | Total Stream Numbers | Stream Length (km) | Total Stream Length (km) | ||||||||
| U1 | U2 | U3 | U4 | U5 | LU1 | LU2 | LU3 | LU4 | LU5 | ||||||
| WS1 | 12.00 | 59.87 | 34.37 | 71 | 18 | 5 | 1 | 95 | 46.78 | 29.43 | 7.92 | 10.37 | 94.50 | ||
| WS2 | 11.10 | 62.08 | 35.07 | 74 | 19 | 3 | 2 | 1 | 99 | 50.22 | 26.30 | 13.32 | 7.27 | 3.67 | 100.78 |
| WS3 | 9.45 | 34.89 | 29.94 | 43 | 9 | 2 | 1 | 55 | 25.96 | 11.62 | 4.41 | 11.76 | 53.75 | ||
| WS4 | 11.31 | 45.57 | 35.30 | 55 | 14 | 1 | 1 | 71 | 27.30 | 20.96 | 13.72 | 4.67 | 66.65 | ||
| WS5 | 7.43 | 23.42 | 28.13 | 20 | 4 | 1 | 25 | 14.62 | 5.50 | 2.80 | 22.92 | ||||
| WS6 | 9.60 | 40.66 | 31.62 | 45 | 9 | 2 | 1 | 57 | 28.44 | 12.60 | 7.00 | 13.33 | 61.37 | ||
| WS7 | 13.00 | 43.76 | 39.87 | 52 | 11 | 1 | 1 | 65 | 33.82 | 13.27 | 5.38 | 8.79 | 61.26 | ||
| WS8 | 6.00 | 9.26 | 16.38 | 9 | 1 | 1 | 11 | 3.87 | 6.64 | 1.07 | 11.58 | ||||
| WS9 | 7.65 | 17.93 | 20.83 | 17 | 4 | 1 | 22 | 12.28 | 3.54 | 3.78 | 19.6 | ||||
| (b) | |||||||||||||||
| Code | Mean Stream Length | Mean Stream Length (km) | Stream Length Ratio | ||||||||||||
| LU1/NU1 | LU2/NU2 | LU3/NU3 | LU4/NU4 | LU5/NU5 | LU2/LU1 | LU3/LU2 | LU4/LU3 | LU5/LU4 | |||||||
| WS1 | 0.66 | 1.64 | 1.58 | 10.37 | 1.99 | 0.63 | 0.27 | 1.31 | |||||||
| WS2 | 0.68 | 1.38 | 4.44 | 3.635 | 3.67 | 1.76 | 0.52 | 0.51 | 0.55 | 0.50 | |||||
| WS3 | 0.60 | 1.29 | 11.76 | 1.93 | 0.45 | 0.38 | |||||||||
| WS4 | 0.50 | 1.50 | 13.72 | 4.67 | 1.86 | 0.77 | 0.65 | 0.34 | |||||||
| WS5 | 0.73 | 1.38 | 1.07 | 0.38 | 0.51 | ||||||||||
| WS6 | 0.63 | 1.40 | 13.33 | 2.09 | 0.44 | 0.56 | |||||||||
| WS7 | 0.65 | 1.21 | 8.79 | 1.62 | 0.39 | 0.41 | 1.63 | ||||||||
| WS8 | 0.43 | 6.24 | 1.72 | ||||||||||||
| WS9 | 1.47 | 2.89 | 1.83 | 0.29 | 1.06 | ||||||||||
| Code | Elongation Ratio | Circulatory Ratio | Form Factor | Shape Index | Shape Factor |
|---|---|---|---|---|---|
| WS1 | 0.73 | 0.64 | 0.42 | 2.41 | 1.74 |
| WS2 | 0.80 | 0.63 | 0.50 | 1.98 | 1.77 |
| WS3 | 0.71 | 0.49 | 0.39 | 2.56 | 1.17 |
| WS4 | 0.67 | 0.46 | 0.36 | 2.81 | 1.29 |
| WS5 | 0.74 | 0.37 | 0.42 | 2.36 | 0.83 |
| WS6 | 0.75 | 0.51 | 0.44 | 2.27 | 1.29 |
| WS7 | 0.57 | 0.35 | 0.26 | 3.86 | 1.10 |
| WS8 | 0.57 | 0.43 | 0.26 | 3.89 | 0.57 |
| WS9 | 0.62 | 0.52 | 0.31 | 3.26 | 0.86 |
| Code | Stream Frequency (km−2) | Drainage Density (km km−2) | Bifurcation Ratio | Mean Bifurcation Ratio | Infiltration Number | Constant of Channel Maintenance (km2 km−1) | Length of Overland Flow (km) | Drainage Intensity | Texture Ratio | Drainage Texture | Compactness Coefficient | |||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| NU1/ NU2 | NU2/ NU3 | NU3/ NU4 | NU4/ NU5 | |||||||||||
| WS1 | 1.59 | 1.58 | 3.94 | 3.60 | 5 | 3.14 | 2.50 | 0.63 | 0.79 | 1.01 | 2.07 | 2.76 | 0.57 | |
| WS2 | 1.59 | 1.62 | 3.89 | 6.33 | 1.5 | 2 | 3.43 | 2.59 | 0.62 | 0.81 | 0.98 | 2.11 | 2.82 | 0.56 |
| WS3 | 1.58 | 1.54 | 4.78 | 4.50 | 2.32 | 2.43 | 0.65 | 0.77 | 1.02 | 1.44 | 1.84 | 0.86 | ||
| WS4 | 1.56 | 1.46 | 3.93 | 14.00 | 1 | 4.73 | 2.28 | 0.68 | 0.73 | 1.07 | 1.56 | 2.01 | 0.77 | |
| WS5 | 1.07 | 0.98 | 5.00 | 4.00 | 2.25 | 1.04 | 1.02 | 0.49 | 1.09 | 0.71 | 0.89 | 1.20 | ||
| WS6 | 1.40 | 1.51 | 5.00 | 4.50 | 2.38 | 2.12 | 0.66 | 0.75 | 0.93 | 1.42 | 1.80 | 0.78 | ||
| WS7 | 1.49 | 1.40 | 4.73 | 11.00 | 1 | 4.18 | 2.08 | 0.71 | 0.70 | 1.06 | 1.30 | 1.63 | 0.91 | |
| WS8 | 1.19 | 1.25 | 9.00 | 1.00 | 2.50 | 1.49 | 0.80 | 0.63 | 0.95 | 0.55 | 0.67 | 1.77 | ||
| WS9 | 1.23 | 1.09 | 4.25 | 4.00 | 2.06 | 1.34 | 0.91 | 0.55 | 1.12 | 0.82 | 1.06 | 1.16 | ||
| Code | Maximum Basin Relief (km) | Minimum Basin Relief (km) | Total Basin Relief (km) | Relative Relief | Relief Ratio | Ruggedness Number |
|---|---|---|---|---|---|---|
| WS1 | 2.094 | 1.400 | 0.694 | 2.019 | 0.058 | 1.095 |
| WS2 | 2.200 | 1.221 | 0.979 | 2.792 | 0.088 | 1.589 |
| WS3 | 2.192 | 0.999 | 1.193 | 3.985 | 0.126 | 1.838 |
| WS4 | 1.913 | 0.397 | 1.516 | 4.295 | 0.134 | 2.217 |
| WS5 | 1.832 | 0.404 | 1.428 | 5.076 | 0.192 | 1.398 |
| WS6 | 2.004 | 0.641 | 1.363 | 4.311 | 0.142 | 2.057 |
| WS7 | 1.890 | 0.381 | 1.509 | 3.785 | 0.116 | 2.112 |
| WS8 | 1.702 | 0.366 | 1.336 | 8.156 | 0.223 | 1.671 |
| WS9 | 1.634 | 0.358 | 1.276 | 6.126 | 0.167 | 1.395 |
| Watershed | Basin Area | Elongation Ratio | Circulatory Ratio | Form Factor | Shape Index | Shape Factor | Stream Frequency | Drainage Density | Mean Bifurcation Ratio | Length of Overland Flow | Texture Ratio | Drainage Texture | Relief Ratio | Ruggedness Number |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| WS1 | 8 | 6 | 9 | 6 | 4 | 8 | 2 | 2 | 4 | 2 | 2 | 2 | 9 | 9 |
| WS2 | 9 | 9 | 8 | 9 | 1 | 9 | 1 | 1 | 3 | 1 | 1 | 1 | 8 | 6 |
| WS3 | 4 | 5 | 5 | 5 | 5 | 5 | 3 | 3 | 7 | 3 | 4 | 4 | 6 | 4 |
| WS4 | 7 | 4 | 4 | 4 | 6 | 7 | 4 | 5 | 1 | 5 | 3 | 3 | 5 | 1 |
| WS5 | 3 | 7 | 2 | 7 | 3 | 2 | 9 | 9 | 8 | 9 | 8 | 8 | 2 | 7 |
| WS6 | 5 | 8 | 6 | 8 | 2 | 6 | 6 | 4 | 6 | 4 | 5 | 5 | 4 | 3 |
| WS7 | 6 | 2 | 1 | 2 | 8 | 4 | 5 | 6 | 2 | 6 | 6 | 6 | 7 | 2 |
| WS9 | 1 | 1 | 3 | 1 | 9 | 1 | 8 | 7 | 5 | 7 | 9 | 9 | 1 | 5 |
| Basin Area | Elongation Ratio | Circulatory Ratio | Form Factor | Shape Index | Shape Factor | Stream Frequency | Drainage Density | Mean Bifurcation Ratio | Length of Overland Flow | Texture Ratio | Drainage Texture | Relief Ratio | Ruggedness Number | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Basin area | 1.000 | 0.533 | 0.433 | 0.533 | −0.533 | 0.933 | −0.850 | −0.783 | −0.700 | −0.783 | −0.917 | −0.917 | 0.883 | −0.117 |
| Elongation ratio | 0.533 | 1.000 | 0.500 | 1.000 | −1.000 | 0.617 | −0.383 | −0.517 | 0.083 | −0.517 | −0.567 | −0.567 | 0.350 | 0.217 |
| Circulatory ratio | 0.433 | 0.500 | 1.000 | 0.500 | −0.500 | 0.667 | −0.600 | −0.650 | 0.100 | −0.650 | −0.650 | −0.650 | 0.483 | 0.550 |
| Form factor | 0.533 | 1.000 | 0.500 | 1.000 | −1.000 | 0.617 | −0.383 | −0.517 | 0.083 | −0.517 | −0.567 | −0.567 | 0.350 | 0.217 |
| Shape index | −0.533 | −1.000 | −0.500 | −1.000 | 1.000 | −0.617 | 0.383 | 0.517 | −0.083 | 0.517 | 0.567 | 0.567 | −0.350 | −0.217 |
| Shape factor | 0.933 | 0.617 | 0.667 | 0.617 | −0.617 | 1.000 | −0.900 | −0.883 | −0.533 | −0.883 | −0.983 | −0.983 | 0.833 | −0.050 |
| Stream frequency | −0.850 | −0.383 | −0.600 | −0.383 | 0.383 | −0.900 | 1.000 | 0.933 | 0.533 | 0.933 | 0.950 | 0.950 | −0.917 | 0.033 |
| Drainage density | −0.783 | −0.517 | −0.650 | −0.517 | 0.517 | −0.883 | 0.933 | 1.000 | 0.450 | 1.000 | 0.900 | 0.900 | −0.817 | 0.033 |
| Mean bifurcation ratio | −0.700 | 0.083 | 0.100 | 0.083 | −0.083 | −0.533 | 0.533 | 0.450 | 1.000 | 0.450 | 0.517 | 0.517 | −0.533 | 0.550 |
| Length of overland flow | −0.783 | −0.517 | −0.650 | −0.517 | 0.517 | −0.883 | 0.933 | 1.000 | 0.450 | 1.000 | 0.900 | 0.900 | −0.817 | 0.033 |
| Texture ratio | −0.917 | −0.567 | −0.650 | −0.567 | 0.567 | −0.983 | 0.950 | 0.900 | 0.517 | 0.900 | 1.000 | 1.000 | −0.867 | 0.033 |
| drainage texture | −0.917 | −0.567 | −0.650 | −0.567 | 0.567 | −0.983 | 0.950 | 0.900 | 0.517 | 0.900 | 1.000 | 1.000 | −0.867 | 0.033 |
| Relief ratio | 0.883 | 0.350 | 0.483 | 0.350 | −0.350 | 0.833 | −0.917 | −0.817 | −0.533 | −0.817 | −0.867 | −0.867 | 1.000 | 0.033 |
| Ruggedness number | −0.117 | 0.217 | 0.550 | 0.217 | −0.217 | −0.050 | 0.033 | 0.033 | 0.550 | 0.033 | 0.033 | 0.033 | 0.033 | 1.000 |
| Sum of correlations | −1.283 | 0.750 | 0.533 | 0.750 | −0.750 | −1.167 | 1.683 | 1.567 | 2.433 | 1.567 | 1.317 | 1.317 | −1.233 | 2.350 |
| Grand total | 9.833 | 9.833 | 9.833 | 9.833 | 9.833 | 9.833 | 9.833 | 9.833 | 9.833 | 9.833 | 9.833 | 9.833 | 9.833 | 9.833 |
| Prioritisation ranking | −0.131 | 0.076 | 0.054 | 0.076 | −0.076 | −0.119 | 0.171 | 0.159 | 0.247 | 0.159 | 0.134 | 0.134 | −0.125 | 0.239 |
| S. No. | Code | Prioritisation Value | Rank |
|---|---|---|---|
| 1. | WS1 | −5.7876 | 2 |
| 2. | WS2 | −5.8231 | 1 |
| 3. | WS3 | −2.7221 | 6 |
| 4. | WS4 | −3.4580 | 4 |
| 5. | WS5 | −1.7420 | 7 |
| 6. | WS6 | −3.4411 | 5 |
| 7. | WS7 | −3.5703 | 3 |
| 8. | WS8 | 0.1646 | 9 |
| 8. | WS9 | −1.0572 | 8 |
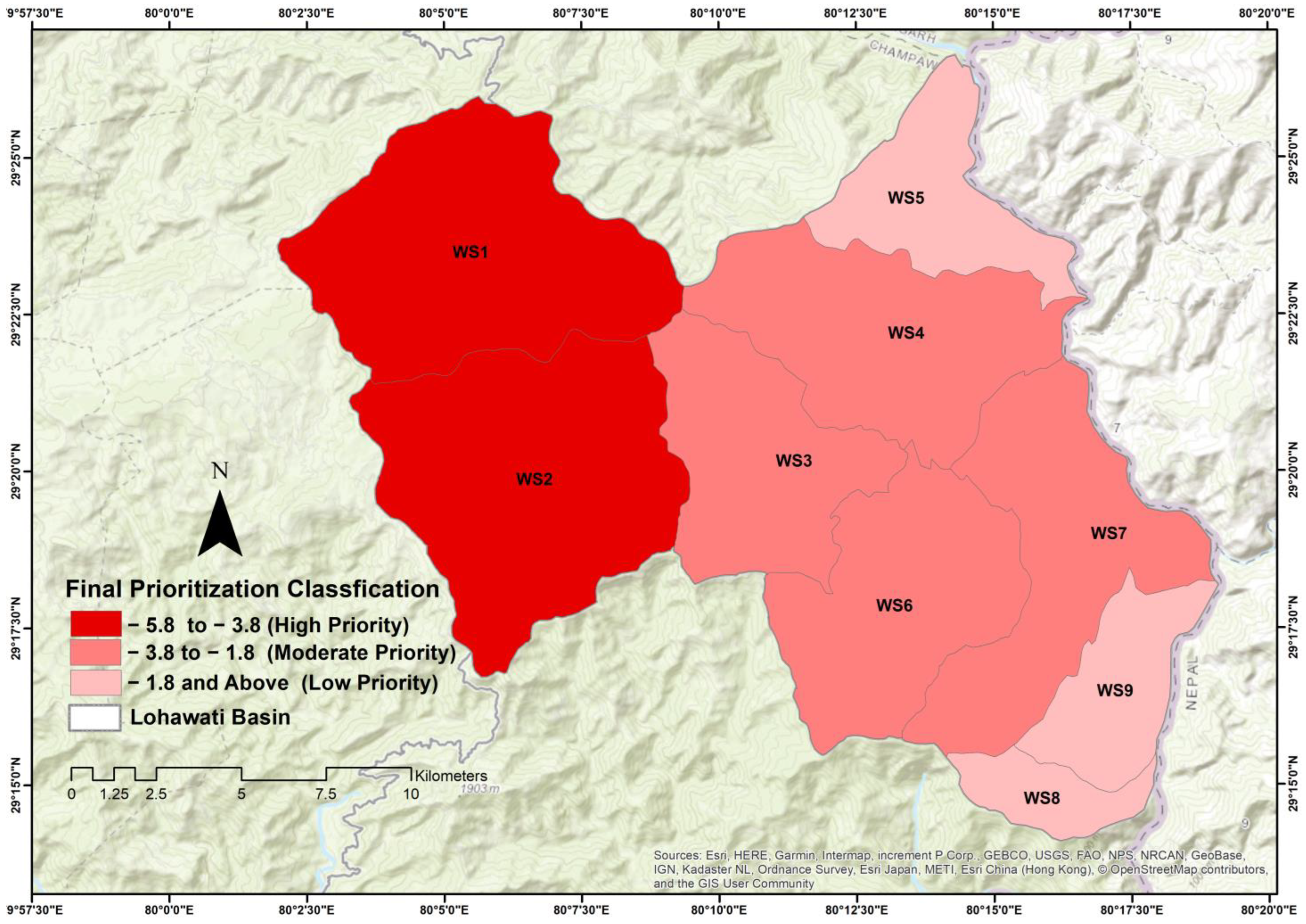
| S. No. | Priority Zone | Priority Rank Range | Watershed Code | Area | Area (%) |
|---|---|---|---|---|---|
| 1. | High | −5.8 to −3.8 | WS1 and WS2 | 121.95 km2 | 36.14 |
| 2. | Moderate | −3.8 to −1.8 | WS3, WS4, WS6, and WS7 | 164.91 km2 | 48.87 |
| 3. | Low | −1.8 and above | WS5, WS8, and WS9 | 50.62 km2 | 15.00 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ganie, P.A.; Posti, R.; Bharti, V.S.; Sehgal, V.K.; Sarma, D.; Pandey, P.K. Striking a Balance between Conservation and Development: A Geospatial Approach to Watershed Prioritisation in the Himalayan Basin. Conservation 2023, 3, 460-490. https://doi.org/10.3390/conservation3040031
Ganie PA, Posti R, Bharti VS, Sehgal VK, Sarma D, Pandey PK. Striking a Balance between Conservation and Development: A Geospatial Approach to Watershed Prioritisation in the Himalayan Basin. Conservation. 2023; 3(4):460-490. https://doi.org/10.3390/conservation3040031
Chicago/Turabian StyleGanie, Parvaiz Ahmad, Ravindra Posti, Vidya Shree Bharti, Vinay Kumar Sehgal, Debajit Sarma, and Pramod Kumar Pandey. 2023. "Striking a Balance between Conservation and Development: A Geospatial Approach to Watershed Prioritisation in the Himalayan Basin" Conservation 3, no. 4: 460-490. https://doi.org/10.3390/conservation3040031
APA StyleGanie, P. A., Posti, R., Bharti, V. S., Sehgal, V. K., Sarma, D., & Pandey, P. K. (2023). Striking a Balance between Conservation and Development: A Geospatial Approach to Watershed Prioritisation in the Himalayan Basin. Conservation, 3(4), 460-490. https://doi.org/10.3390/conservation3040031








