Application of Bilinear Softening Laws and Fracture Toughness of Foamed Concrete
Abstract
:1. Introduction
1.1. Bilinear Softening Law
1.2. Fracture Toughness
2. Experimental Investigations
2.1. Experimental Testing
2.2. Experimental Results
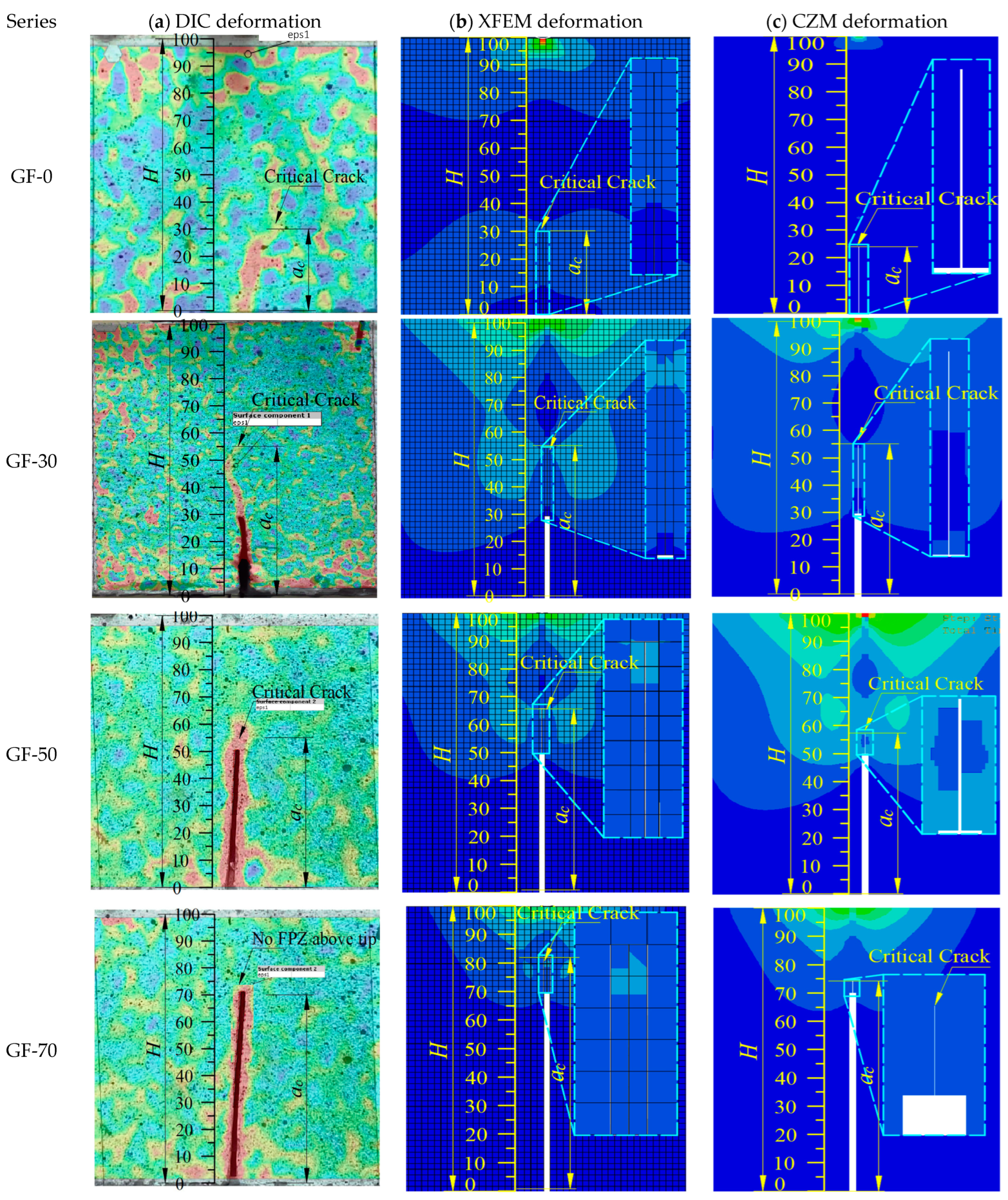
2.3. Digital Image Correlation
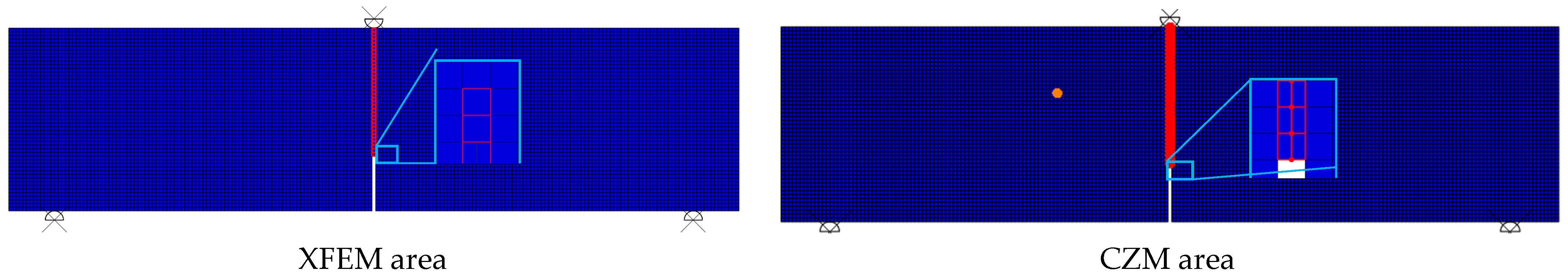
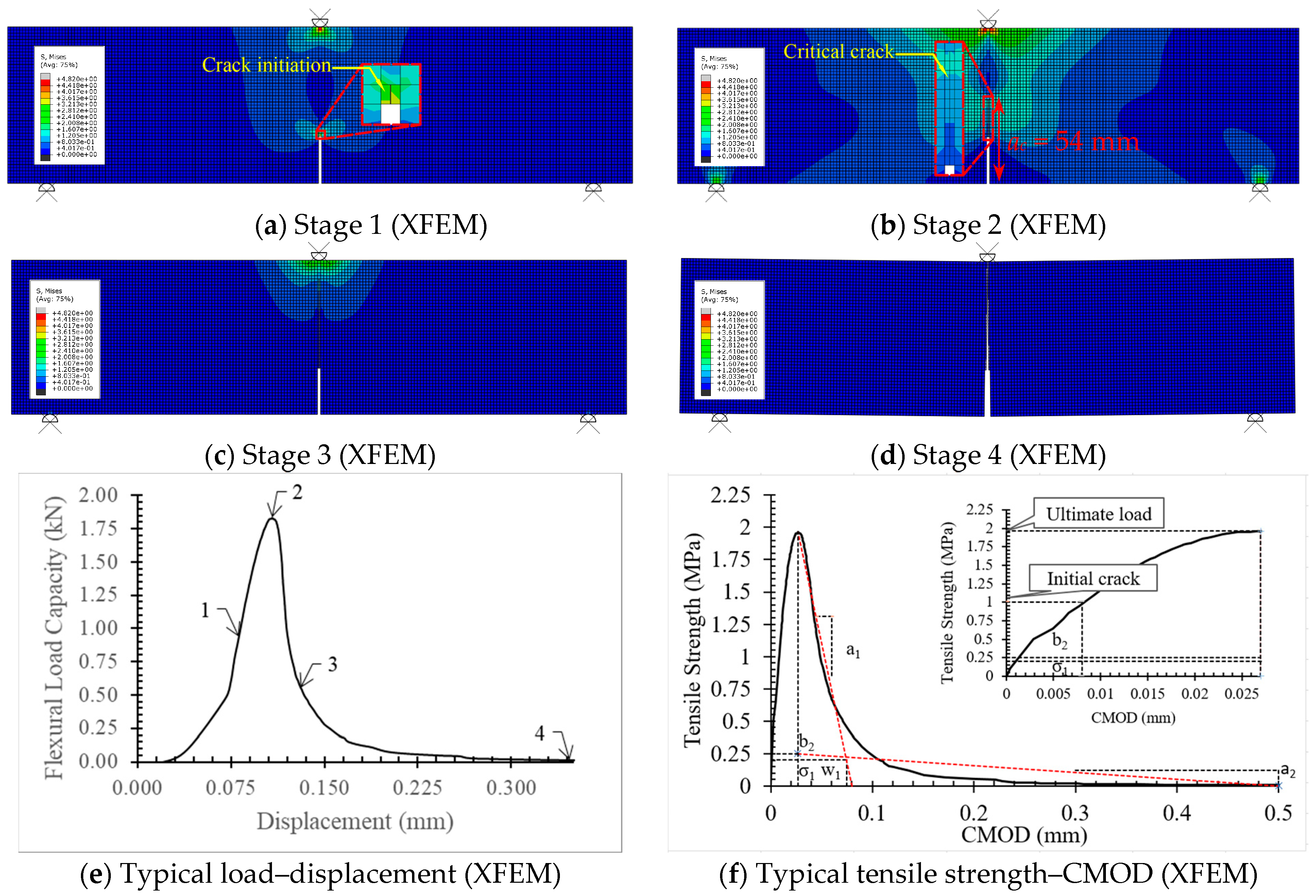
3. Finite Element Study
3.1. Sensitivity Study
3.2. Damage Plot
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| σ1 | kink point tensile stress |
| σ0 | un-notched strength |
| fictitious crack extension length | |
| pre-cut crack length | |
| the critical crack length | |
| w1 | kink point CMOD opening |
| w2 | zero stress point |
| a1 | first softening compliance |
| a2 | second softening compliance |
| b2 | stress of second softening intercept |
| crack tip opening displacement | |
| critical crack tip opening displacement | |
| crack mouth opening displacement | |
| critical crack mouth opening displacement | |
| Ci | initial compliance of the P–CMOD curve |
| E | elastic modulus |
| ft | tensile strength |
| GF | fracture energy |
| GI | Fracture energy mode I |
| GF-0 | specimen without notches |
| GF-30 | specimen with a 30-millimeter notch height |
| GF-50 | specimen with a 50-millimeter notch height |
| GF-70 | specimen with a 70-millimeter notch height |
| specimen height | |
| clip gauge holder thickness | |
| cracking toughness | |
| initial cracking toughness | |
| unstable cracking toughness | |
| Pult | ultimate load |
| Pini | initial crack load |
| v | Poisson’s ratio |
| U0 | softening area |
| mg | specimen weight |
References
- Lee, Y.L.; Tan, C.S.; Lim, S.K.; Mohammad, S.; Lim, J.H. Strength Performance on Different Mix of Cement-Sand Ratio and Sand Condition for Lightweight Foamed Concrete. E3S Web Conf. 2018, 65, 02006. [Google Scholar] [CrossRef] [Green Version]
- Gökçe, H.S.; Hatungimana, D.; Ramyar, K. Effect of Fly Ash and Silica Fume on Hardened Properties of Foam Concrete. Constr. Build. Mater. 2019, 194, 1–11. [Google Scholar] [CrossRef]
- Ahmad, M.R.; Chen, B.; Farasat Ali Shah, S. Investigate the Influence of Expanded Clay Aggregate and Silica Fume on the Properties of Lightweight Concrete. Constr. Build. Mater. 2019, 220, 253–266. [Google Scholar] [CrossRef]
- Wang, X.; Huang, J.; Dai, S.; Ma, B.; Jiang, Q. Investigation of Silica Fume as Foam Cell Stabilizer for Foamed Concrete. Constr. Build. Mater. 2020, 237, 117514. [Google Scholar] [CrossRef]
- Hillerborg, A.; Modéer, M.; Petersson, P.E. Analysis of Crack Formation and Crack Growth in Concrete by Means of Fracture Mechanics and Finite Elements. Cem. Concr. Res. 1976, 6, 773–781. [Google Scholar] [CrossRef]
- Bažant, Z.P. Size Effect in Blunt Fracture: Concrete, Rock, Metal. J. Eng. Mech. 1984, 110, 518–535. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Oh, B.H. Crack Band Theory for Fracture of Concrete. Matériaux Constr. 1983, 16, 155–177. [Google Scholar] [CrossRef] [Green Version]
- Nallathambi, P.; Karihaloo, B.L. Determination of Specimen-Size Independent Fracture Toughness of Plain Concrete. Mag. Concr. Res. 1986, 38, 67–76. [Google Scholar] [CrossRef]
- Bazant, Z.P.; Kazemi, M.T. Determination of fracture energy, process zone longth and brittleness number from size effect, with application to rock and conerete. Int. J. Fract. 1990, 44, 111–131. [Google Scholar] [CrossRef]
- Xu, S.; Reinhardt, H.W. Determination of Double-K Criterion for Crack Propagation in Quasi-brittle fracture, Part II: Experimental investigation of crack propagation. Int. J. Fract. 1999, 98, 111–149. [Google Scholar] [CrossRef]
- Rahman, N.A.; Jaini, Z.M. An Experimental Study on the Fracture Energy of Foamed Concrete Using V-Notched Beams. In InCIEC 2014; Springer: Singapore, 2015; pp. 1–13. [Google Scholar] [CrossRef] [Green Version]
- Kozłowski, M.; Kadela, M.; Kukiełka, A. Fracture Energy of Foamed Concrete Based on Three-Point Bending Test on Notched Beams. Procedia Eng. 2015, 108, 349–354. [Google Scholar] [CrossRef] [Green Version]
- Falliano, D.; De Domenico, D.; Sciarrone, A.; Ricciardi, G.; Restuccia, L.; Tulliani, J.M.C.; Gugliandolo, E. Fracture Behavior of Lightweight Foamed Concrete: The Crucial Role of Curing Conditions. Theor. Appl. Fract. Mech. 2019, 103, 102297. [Google Scholar] [CrossRef]
- Xu, P.; Ma, J.; Zhang, M.; Ding, Y.; Meng, L. Fracture Energy Analysis of Concrete Considering the Boundary Effect of Single-Edge Notched Beams. Adv. Civ. Eng. 2018, 2018, 3067236. [Google Scholar] [CrossRef] [Green Version]
- Ding, Y.; Bai, Y.; Dai, J.; Shi, C. An Investigation of Softening Laws and Fracture Toughness of Slag-Based Geopolymer Concrete. Materials 2020, 13, 5200. [Google Scholar] [CrossRef] [PubMed]
- Bu, J.; Chen, X.; Hu, L.; Yang, H.; Liu, S. Experimental Study on Crack Propagation of Concrete Under Various Loading Rates with Digital Image Correlation Method. Int. J. Concr. Struct. Mater. 2020, 14, 25. [Google Scholar] [CrossRef]
- Qing, L.; Su, Y.; Dong, M.; Cheng, Y.; Li, Y. Size effect on double-K fracture parameters of concrete based on fracture extreme theory. Arch. Appl. Mech. 2021, 91, 427–442. [Google Scholar] [CrossRef]
- Skarzyński, Ł.; Kozicki, J.; Tejchman, J. Application of DIC Technique to Concrete-Study on Objectivity of Measured Surface Displacements. Exp. Mech. 2013, 53, 1545–1559. [Google Scholar] [CrossRef]
- Ohno, K.; Uji, K.; Ueno, A.; Ohtsu, M. Fracture Process Zone in Notched Concrete Beam under Three-Point Bending by Acoustic Emission. Constr. Build. Mater. 2014, 67, 139–145. [Google Scholar] [CrossRef]
- Alam, S.Y.; Saliba, J.; Loukili, A. Fracture Examination in Concrete through Combined Digital Image Correlation and Acoustic Emission Techniques. Constr. Build. Mater. 2014, 69, 232–242. [Google Scholar] [CrossRef] [Green Version]
- Wu, Z.M.; Rong, H.; Zheng, J.J.; Xu, F.; Dong, W. An Experimental Investigation on the FPZ Properties in Concrete Using Digital Image Correlation Technique. Eng. Fract. Mech. 2011, 78, 2978–2990. [Google Scholar] [CrossRef]
- Park, K.; Choi, H.; Paulino, G.H. Assessment of Cohesive Traction-Separation Relationships in ABAQUS: A Comparative Study. Mech. Res. Commun. 2016, 78, 71–78. [Google Scholar] [CrossRef] [Green Version]
- Elices, M.; Guinea, G.V.; Gómez, J.; Planas, J. The cohesive zone model: Advantages, limitations and challenges. Eng. Fract. Mech. 2001, 69, 137–163. [Google Scholar] [CrossRef]
- Freed, Y.; Banks-Sills, L. A New Cohesive Zone Model for Mixed Mode Interface Fracture in Bimaterials. Eng. Fract. Mech. 2008, 75, 4583–4593. [Google Scholar] [CrossRef]
- McGarry, J.P.; Ó Máirtín, É.; Parry, G.; Beltz, G.E. Potential-based and non-potential-based cohesive zone formulations under mixed-mode separation and over-closure. Part I: Theoretical analysis. J. Mech. Phys. Solids 2014, 63, 336–362. [Google Scholar] [CrossRef] [Green Version]
- Faron, A.; Rombach, G.A. Simulation of Crack Growth in Reinforced Concrete Beams Using Extended Finite Element Method. Eng. Fail. Anal. 2020, 116, 104698. [Google Scholar] [CrossRef]
- Campilho, R.D.S.G.; Banea, M.D.; Neto, J.A.B.P.; Da Silva, L.F.M. Modelling adhesive joints with cohesive zone models: Effect of the cohesive law shape of the adhesive layer. Int. J. Adhes. Adhes. 2013, 44, 48–56. [Google Scholar] [CrossRef] [Green Version]
- Yu, Z.; Zhang, J.; Shen, J.; Chen, H. Simulation of Crack Propagation Behavior of Nuclear Graphite by Using XFEM, VCCT and CZM Methods. Nucl. Mater. Energy 2021, 29, 101063. [Google Scholar] [CrossRef]
- Ahmad, H.; Sugiman, S.; Jaini, Z.M.; Omar, A.Z. Numerical Modelling of Foamed Concrete Beam under Flexural Using Traction-Separation Relationship. Lat. Am. J. Solids Struct. 2021, 18, 1–13. [Google Scholar] [CrossRef]
- Park, K.; Paulino, G.H.; Roesler, J.R. Determination of the Kink Point in the Bilinear Softening Model for Concrete. Eng. Fract. Mech. 2008, 75, 3806–3818. [Google Scholar] [CrossRef]
- Petersson, P.E. Crack Growth and Development of Fracture Zones in Plain Concrete and Similar Materials. Ph.D. Thesis, Lund University, Lund, Sweden, 1981. [Google Scholar]
- Wittmann, F.H.; Rokugo, K.; Brühwiler, E.; Mihashi, H.; Simonin, P. Fracture Energy and Strain Softening of Concrete as Determined by Means of Compact Tension Specimens. Mater. Struct. 1988, 21, 21–32. [Google Scholar] [CrossRef]
- Hu, S.; Fan, B. Study on the Bilinear Softening Mode and Fracture Parameters of Concrete in Low Temperature Environments. Eng. Fract. Mech. 2019, 211, 1–16. [Google Scholar] [CrossRef]
- Roelfstra, P.E.; Wittmann, F. Numerical Method to Link Strain Softening with Failure of Concrete. In Fracture Toughness and Fracture Energy of Concrete; Elsevier Science Publishers: Amsterdam, The Netherlands, 1986; pp. 163–175. [Google Scholar]
- Kumar, S.; Barai, S.V. Effect of loading condition, specimen geometry, size-effect and softening function on double-K fracture parameters of concrete. Sadhana-Acad. Proc. Eng. Sci. 2012, 37, 3–15. [Google Scholar] [CrossRef] [Green Version]
- Zhang, X.; Xu, S.; Zheng, S. Experimental measurement of double-K fracture parameters of concrete with small-size aggregates. Front. Archit. Civ. Eng. China 2007, 1, 448–457. [Google Scholar] [CrossRef]
- Yu, K.; Lu, Z. Determining residual double-K fracture toughness of post-fire concrete using analytical and weight function method. Mater. Struct./Mater. Constr. 2014, 47, 839–852. [Google Scholar] [CrossRef]
- Kumar, S.; Barai, S.V. Influence of specimen geometry on determination of double-K fracture parameters of concrete: A comparative study. Int. J. Fract. 2008, 149, 47–66. [Google Scholar] [CrossRef]
- ASTM-C78-02; Standard Test Method for Flexural Strength of Concrete (Using Simple Beam with Third-Point Loading) 1. ASTM International: West Conshohocken, PA, USA, 2002.
- JCI-S-001.2003; Method of Test for Fracture Energy of Concrete by Use of Notched Beam. Japan Concrete Institute Standard: Tokyo, Japan, 2003.
- ASTM C469-02; Standard Test Method for Static Modulus of Elasticity and Poisson’s Ratio of Concrete in Compression. ASTM International: West Conshohocken, PA, USA, 2002.
- Bažant, Z.P. Concrete Fracture Models: Testing and Practice. Eng. Fract. Mech. 2002, 69, 165–205. [Google Scholar] [CrossRef]
- Hillerborg, A. The Theoretical Basis of a Method to Determine the Fracture Energy GF of Concrete. Mater. Struct. 1985, 21, 162. [Google Scholar] [CrossRef]
- Munjiza, A.; John, N.W.M. Mesh Size Sensitivity of the Combined FEM/DEM Fracture and Fragmentation Algorithms. Eng. Fract. Mech. 2001, 69, 281–295. [Google Scholar] [CrossRef]
- More, S.T.; Bindu, R.S. Effect of Mesh Size on Finite Element Analysis of Plate Structure. Int. J. Eng. Sci. Innov. Technol. 2015, 4, 181–185. [Google Scholar]
- Maulana, M.R.; Sugiman, S.; Ahmad, H.; Jaini, Z.M.; Mansor, H. XFEM Modelling and Experimental Observations of Foam Concrete Beam Externally-Bonded with KFRP Sheet. Lat. Am. J. Solids Struct. 2022, 19, 1–12. [Google Scholar] [CrossRef]
- Shen, W.; Liang, G.; Lei, J.; Li, C. Thin-Walled Structures Singular Intensity Factor Method to Estimate Notch Stress and N-SIF in Double Edge V-Notched Plate. Thin-Walled Struct. 2021, 169, 108387. [Google Scholar] [CrossRef]
- Ye, J.; Gong, Y.; Tao, J.; Cao, T.; Zhao, L.; Zhang, J.; Hu, N. Efficiently Determining the R-Curve and Bridging Traction-Separation Relation of Mode I Delamination in a Simple Way. Compos. Struct. 2022, 288, 115388. [Google Scholar] [CrossRef]
- Koslan, M.F.S.; Zaidi, A.M.A.; Othman, M.Z.; Abdullah, S.; Thanakodi, S. The Effect of Mesh Sizing toward Deformation Result in Computational Dynamic Simulation for Blast Loading Application. Mod. Appl. Sci. 2013, 7, 23–28. [Google Scholar] [CrossRef] [Green Version]
- Supar, K.; Ahmad, H. Multi-Holes Configurations of Woven Fabric Kenaf Composite Plates: Experimental Works and 2-D Modelling. J. Mech. Eng. Sci. 2018, 12, 3539–3547. [Google Scholar] [CrossRef]
- Ahmad, H.; Crocombe, A.D.; Smith, P.A. Strength prediction in CFRP woven laminate bolted double-lap joints under quasi-static loading using XFEM. Compos. Part A Appl. Sci. Manuf. 2014, 56, 192–202. [Google Scholar] [CrossRef] [Green Version]









| Binder (kg/m3) | Sand (kg/m3) | SP (kg/m3) | Water (kg/m3) | Foam (liter/m3) | f’c (MPa) | |
|---|---|---|---|---|---|---|
| Cement | SF | |||||
| 1043.91 | 54.94 | 366.28 | 10.99 | 461.52 | 105 | 42.3 |
| Testing Series | Dimension (mm) (l × b × h) | Notch Height (mm) | Standard | Speed Rate |
|---|---|---|---|---|
| Control | 400 × 100 × 100 | Un-notched | ASTM-C78-02 [39] | 0.5 mm/min |
| GF-0 | 400 × 100 × 100 | 0 | JCI-S-001 [40] | 0.1 mm/min |
| GF-30 | 30 | |||
| GF-50 | 50 | |||
| GF-70 | 70 | |||
| E | D150 × 300 | - | ASTM C469-02 [41] | 3 kN/sec |
| Testing Series | Pini (kN) | Pult (kN) | ft (MPa) | σ1 (MPa) | w1 (mm) | w2 (mm) | a1 (mm−1) | a2 (mm−1) | b2 (MPa) |
|---|---|---|---|---|---|---|---|---|---|
| GF-0 | 3.333 | 4.101 | 2.153 | 0.380 | 0.820 | 2.250 | 2.623 | 0.214 | 0.550 |
| ±0.075 | ±0.089 | ±0.105 | ±0.034 | ±0.098 | ±0.052 | ±0.398 | ±0.025 | ±0.104 | |
| GF-30 | 1.384 | 1.752 | 1.877 | 0.317 | 0.650 | 2.000 | 2.612 | 0.240 | 0.433 |
| ±0.135 | ±0.142 | ±0.159 | ±0.057 | ±0.153 | ±0.288 | ±0.398 | ±0.038 | ±0.088 | |
| GF-50 | 0.595 | 0.786 | 1.650 | 0.270 | 0.700 | 2.310 | 2.118 | 0.187 | 0.350 |
| ±0.075 | ±0.052 | ±0.095 | ±0.045 | ±0.115 | ±0.288 | ±0.086 | ±0.045 | ±0.093 | |
| GF-70 | 0.244 | 0.254 | 1.303 | 0.270 | 0.670 | 2.203 | 2.082 | 0.200 | 0.345 |
| ±0.021 | ±0.015 | ±0.058 | ±0.031 | ±0.124 | ±0.288 | ±0.152 | ±0.018 | ±0.101 |
| Testing Series | (mm) | Enotched beam (GPa) | Ecylinder (GPa) | (MPa mm0.5) | (mm) | (mm) | GF (N/mm) | ||
|---|---|---|---|---|---|---|---|---|---|
| GF-0 | 30.213 | 13.1 | 0.426 | 20.557 | 20.131 | 0.178 | 0.178 | 0.016 | |
| ±1.145 | ±0.135 | ±0.847 | ±2.175 | ±1.231 | ±0.011 | ±0.011 | ±0.002 | ||
| GF-30 | 54.459 | 13.2 | 6.907 | 23.186 | 16.278 | 0.123 | 0.075 | 0.015 | |
| ±2.288 | ±0.231 | ±1.172 | ±4.212 | ±3.210 | ±0.015 | ±0.009 | ±0.001 | ||
| GF-50 | 62.716 | 2.363 | ±1.353 | 4.875 | 6.963 | 2.088 | 0.149 | 0.024 | 0.014 |
| ±1.145 | ±0.135 | ±0.847 | ±2.175 | ±1.231 | ±0.011 | ±0.011 | ±0.003 | ||
| GF-70 | - | −0.018 | 3.697 | - | - | 0.157 | - | 0.014 | |
| ±0.000 | ±0.000 | ±0.746 | ±0.001 | ±0.231 | ±0.005 | ±0.000 | ±0.002 | ||
| E (GPa) | v | σ0 (MPa) | GI (N/mm) | TSL |
|---|---|---|---|---|
| 13.0 | 0.28 | 1.652 | 0.015 | MaxPS |
| Testing Series | FEM XFEM Number of | FEM CZM Number of | ||||
|---|---|---|---|---|---|---|
| Elements | Nodes | Integration Points | Elements | Nodes | Integration Points | |
| GF-0 | 10,302 | 10,050 | 4 | 10,100 | 10,404 | 4 |
| GF-30 | 10,135 | 10,404 | 4 | 10,170 | 10,476 | 4 |
| GF-50 | 10,125 | 10,404 | 4 | 10,150 | 10,456 | 4 |
| GF-70 | 10,115 | 10,404 | 4 | 10,130 | 10,436 | 4 |
| Series | TSL | Pini (kN) | Pult (kN) | ft (MPa) | σ1 (MPa) | w1 (mm) | w2 (mm) | a1 (mm−1) | a2 (mm−1) | b2 (MPa) |
|---|---|---|---|---|---|---|---|---|---|---|
| GF-0 | XFEM | 2.563 | 3.974 | 2.086 | 0.230 | 0.070 | 2.217 | 33.030 | 0.424 | 0.250 |
| CZM | 2.985 | 4.928 | 2.587 | 0.350 | 0.150 | 2.274 | 15.830 | 0.467 | 0.500 | |
| GF-30 | XFEM | 0.938 | 1.832 | 1.963 | 0.220 | 0.080 | 2.215 | 22.956 | 0.350 | 0.241 |
| CZM | 1.125 | 2.455 | 2.338 | 0.250 | 0.140 | 2.341 | 33.973 | 0.452 | 0.300 | |
| GF-50 | XFEM | 0.552 | 0.945 | 1.985 | 0.210 | 0.090 | 1.659 | 21.562 | 0.325 | 0.236 |
| CZM | 0.589 | 1.040 | 2.185 | 0.240 | 0.130 | 2.547 | 22.867 | 0.441 | 0.280 | |
| GF-70 | XFEM | 0.215 | 0.330 | 1.928 | 0.200 | 0.190 | 1.348 | 21.795 | 0.375 | 0.268 |
| CZM | 0.258 | 0.340 | 2.166 | 0.235 | 0.160 | 2.942 | 22.207 | 0.410 | 0.410 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maulana, M.R.; Ahmad, H.; Sugiman, S. Application of Bilinear Softening Laws and Fracture Toughness of Foamed Concrete. Constr. Mater. 2023, 3, 287-304. https://doi.org/10.3390/constrmater3030019
Maulana MR, Ahmad H, Sugiman S. Application of Bilinear Softening Laws and Fracture Toughness of Foamed Concrete. Construction Materials. 2023; 3(3):287-304. https://doi.org/10.3390/constrmater3030019
Chicago/Turabian StyleMaulana, Malik Ridwan, Hilton Ahmad, and Sugiman Sugiman. 2023. "Application of Bilinear Softening Laws and Fracture Toughness of Foamed Concrete" Construction Materials 3, no. 3: 287-304. https://doi.org/10.3390/constrmater3030019
APA StyleMaulana, M. R., Ahmad, H., & Sugiman, S. (2023). Application of Bilinear Softening Laws and Fracture Toughness of Foamed Concrete. Construction Materials, 3(3), 287-304. https://doi.org/10.3390/constrmater3030019





