Artificial Ground Freezing—On the Soil Deformations during Freeze–Thaw Cycles
Abstract
1. Introduction
2. Definition of Ground Freezing
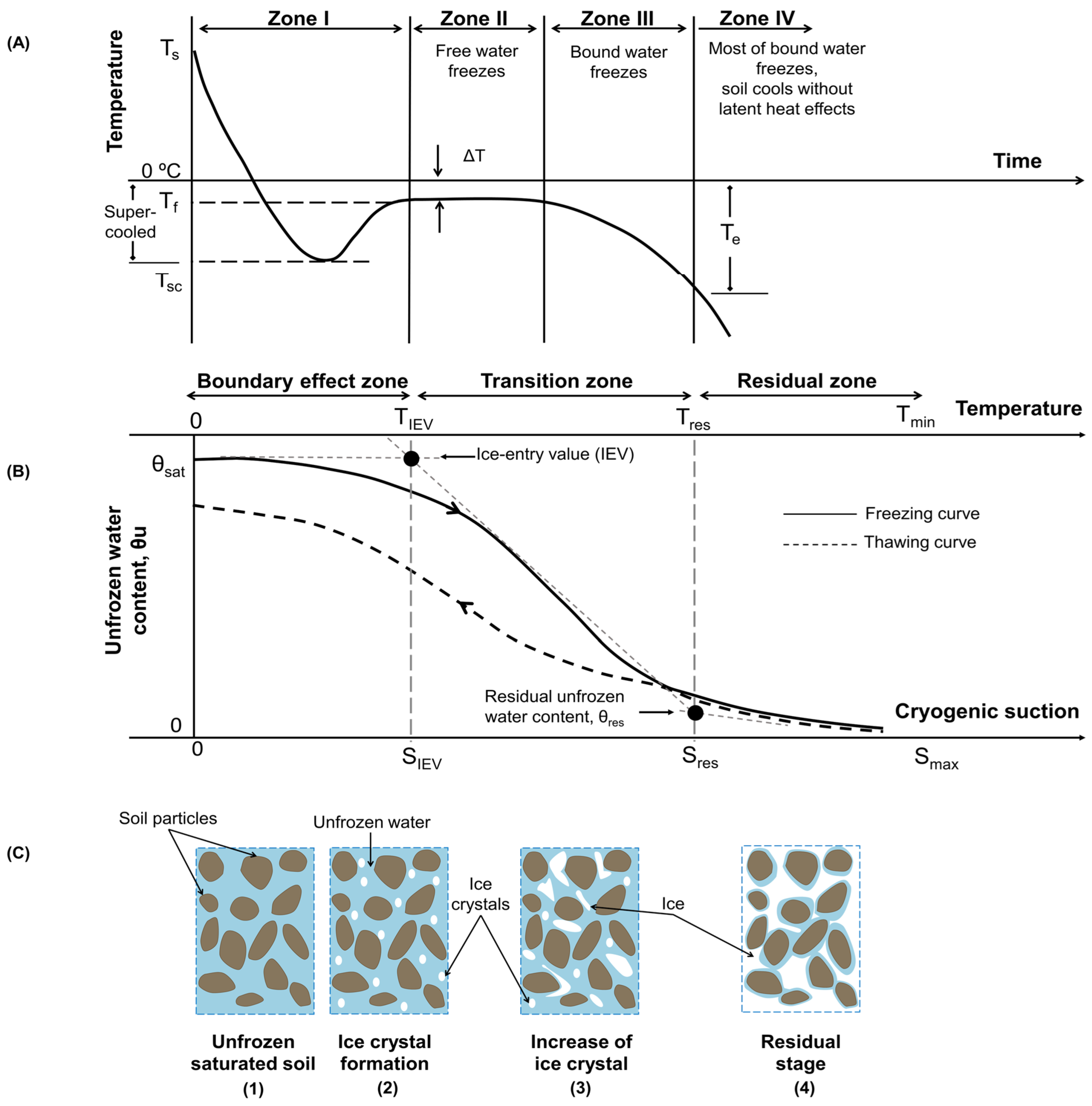
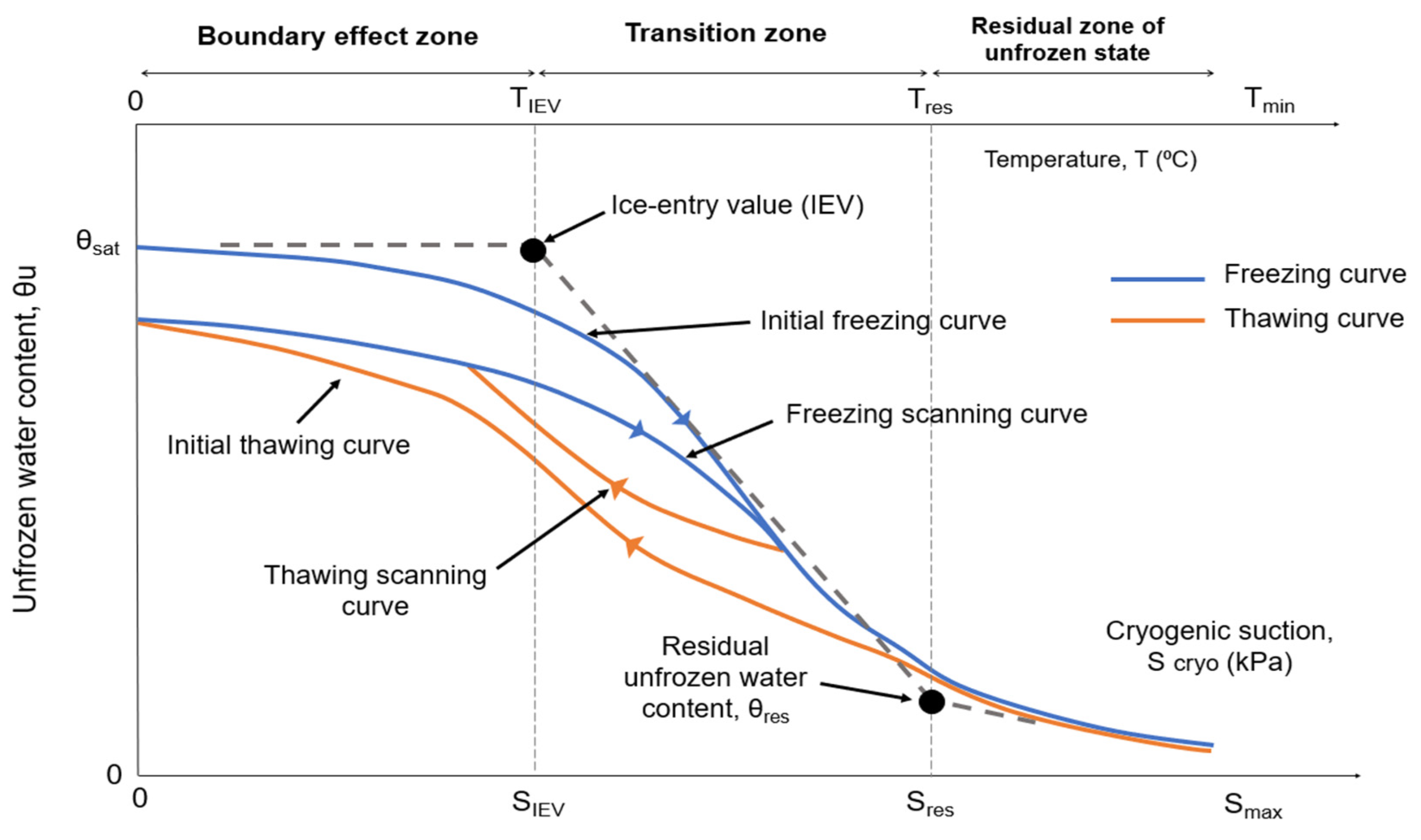
- In Zone I (Figure 1A), the free pore water does not freeze until the temperature drops to a supercooled temperature—Tsc. This is because the supercooled water is in a metastable equilibrium state until nucleation centers cause an abrupt transformation of free water into ice, marking the beginning of Zone II. Nuclei can be aggregates of water molecules or soil particles. Zone I corresponds to the boundary effect zone on the SFCC (Figure 1B) and stage (1) of Figure 1C. Initially, the pores of the saturated soil are filled with liquid water. During the freezing process, the temperature of the pore water decreases, but due to confinement and interaction with soil particles, its freezing point is lower than that of free water. Therefore, even if the soil temperature reaches 0 °C, the pore water will not freeze.
- In Zone II (Figure 1A), ice formation releases latent heat, causing the temperature to rise from Tsc to Tf, the initial freezing temperature. Tf is close to 0 °C for cohesionless soils with low specific surface area, but the temperature decrease (ΔT) can be as much as 5 °C for fine-grained soils [3]. In Zone II, the supercooled water contained in the large interconnected pores is isothermally converted into ice. Zone II corresponds to the transition zone on the SFCC (Figure 1B) and stage (2) of Figure 1C. The beginning of the transition zone is marked by the onset of ice nucleation as the temperature continues to decrease. Small ice crystals begin to form in large pores, replacing existing water. The cryogenic suction at which ice nucleation begins is referred to as the ice entry value (IEV). As the ice continues to grow, it will gradually enter smaller pores. For the SFCC, the IEV is the intersection of the linear extent of the initial straight segment of the SFCC and its central zone, which is characterized by a significant decrease in the amount of remaining unfrozen water. From this point on, the water bound to the grains is gradually converted into ice, as shown in the third part (Zone III) of the curve in Figure 1A.
- Zone III corresponds to stage (3) in Figure 1C. Unlike free pore water, the solidification of bound water in soil is not a uniform, constant temperature process due to the influence of capillarity and absorption forces [17]. The resulting latent heat slows down the temperature decrease until a limit temperature Te (about −70 °C) is reached. At this point, all the free water and most of the bound water (unfrozen water film adsorbed on soil particles) is frozen. For fine-grained soils with high specific surface areas, a significant amount of unfrozen water may still be present at higher negative temperatures.
- Zone IV corresponds to the residual zone on the SFCC (Figure 1B) and stage (4) in Figure 1C. As the temperature decreases, once a certain value is reached, most of the pore water has already been converted into ice, and only a small amount of liquid water remains. This water is found as thin films adsorbed on soil particles and can be referred to as the residual unfrozen water content. At this point, the ice phase is continuous while the water phase is not.
3. Ground Freezing, a Highly Coupled THMC Process
- HT coupling
- HTM coupling
- TC coupling
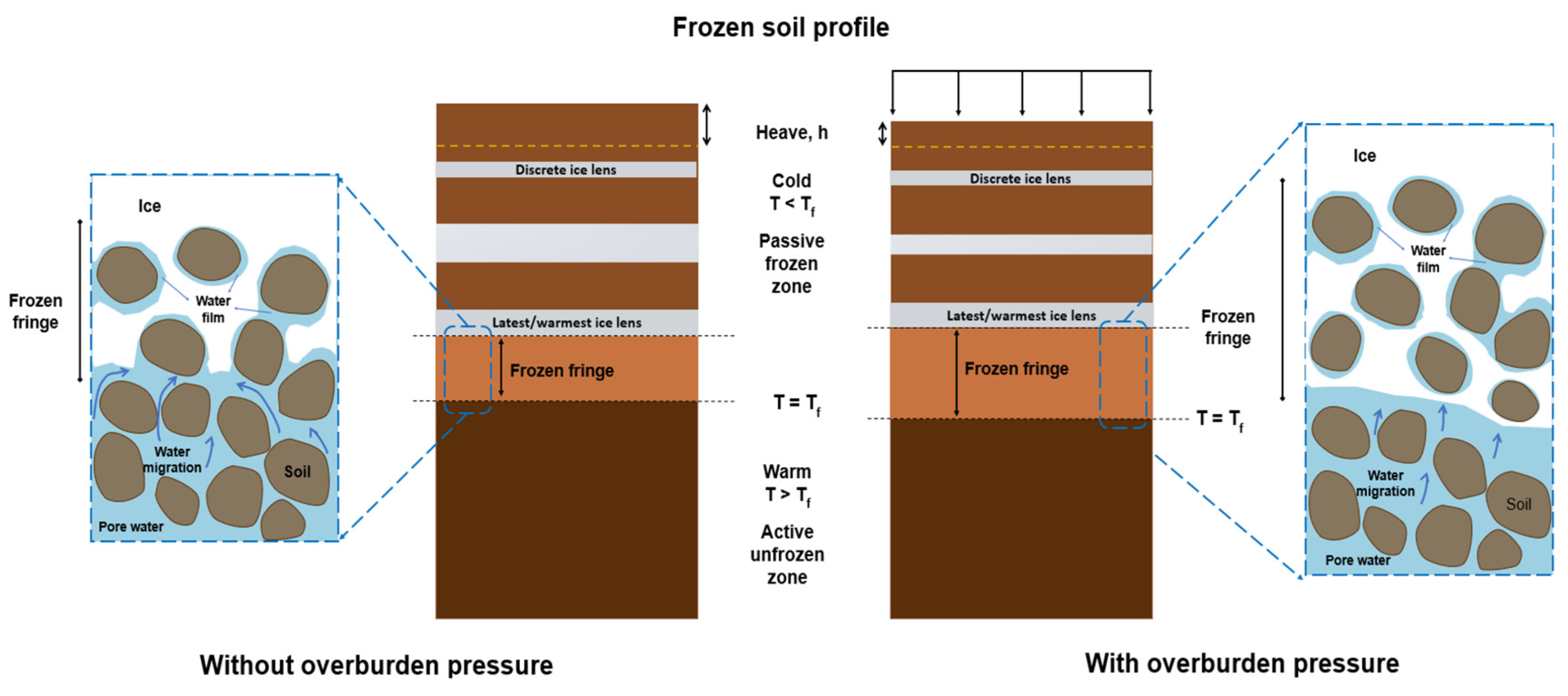
4. Ground Deformations Induced by AGF
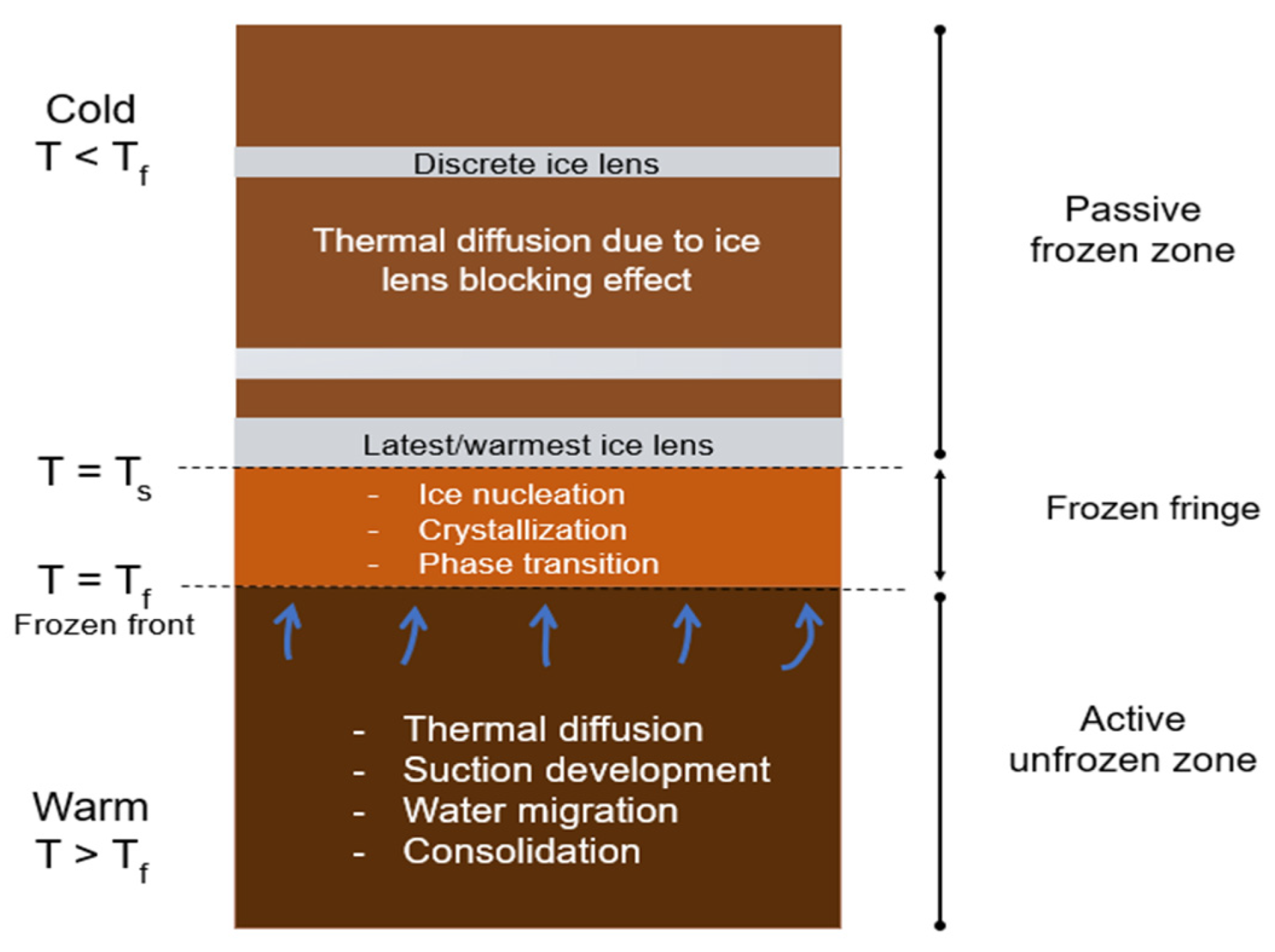
- Passive frozen zone: This region is ice-bound and experiences ice crystal and lens formation. Temperature changes are driven by thermal diffusion mediated by the presence of ice.
- Frozen fringe: This transition zone marks the interface between the frozen and the unfrozen zone. Here, ice and water coexist in soil pores, and complex processes of ice nucleation, crystallization, and phase transition occur.
- Active unfrozen zone: This zone is dominated by thermal diffusion, water migration, suction, and soil consolidation.
- Permeability criteria: Emphasizes the role of frozen fringe permeability linked to segregation temperature [16]. A new lens forms when reduced permeability restricts water flow to existing lenses.
- Soil freezing characteristic curve criteria: Uses the SFCC to determine ice lens initiation conditions, including the segregation temperature [26]. This method identifies the IEV within the SFCC for fine-grained soils.
5. AGF in the Presence of Overburden Pressure
6. Properties and Behavior of Soil after FT Cycles
6.1. Differences behind the Freezing and Thawing Paths
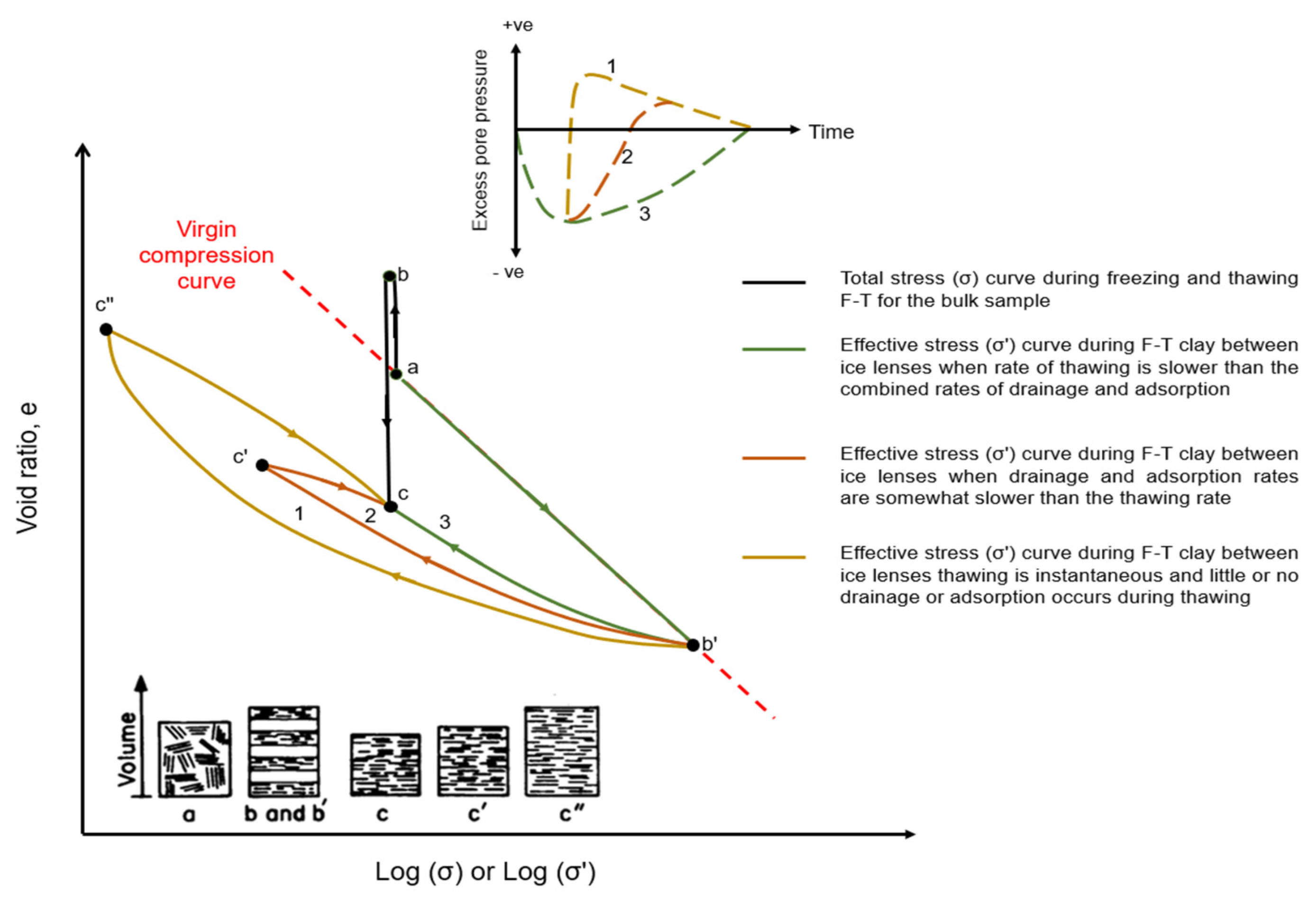
6.2. Stress Path during a Freeze–Thaw Cycle
- Slow thawing (b′-c): This scenario represents a situation where drainage and adsorption can keep pace with the thawing rate. The effective stress path follows b′-c, reaching equilibrium with no excess pore pressure at point c.
- Moderate thawing rate (b′-c′-c): This intermediate scenario occurs when the drainage and adsorption rates are slightly slower than the thawing rate. The soil swells upon thawing, generating excess pore pressure and a significant increase in the volume change (point c′). As the excess pore pressure dissipates, the soil consolidates further to point c.
- Rapid thawing with minimal drainage/adsorption (b′-c″-c): In this case, thawing is very fast, leading to minimal drainage or adsorption. The excess pore pressure can approach the applied stress, causing the effective stress to drop near zero (b′-c″). Over time, this pressure dissipates and the effective stress returns to the applied stress value (point c).
6.3. Modifications in Soil Properties after a Freeze–Thaw Cycle
6.3.1. Influence of FT Cycles on the Physical Properties of Soil
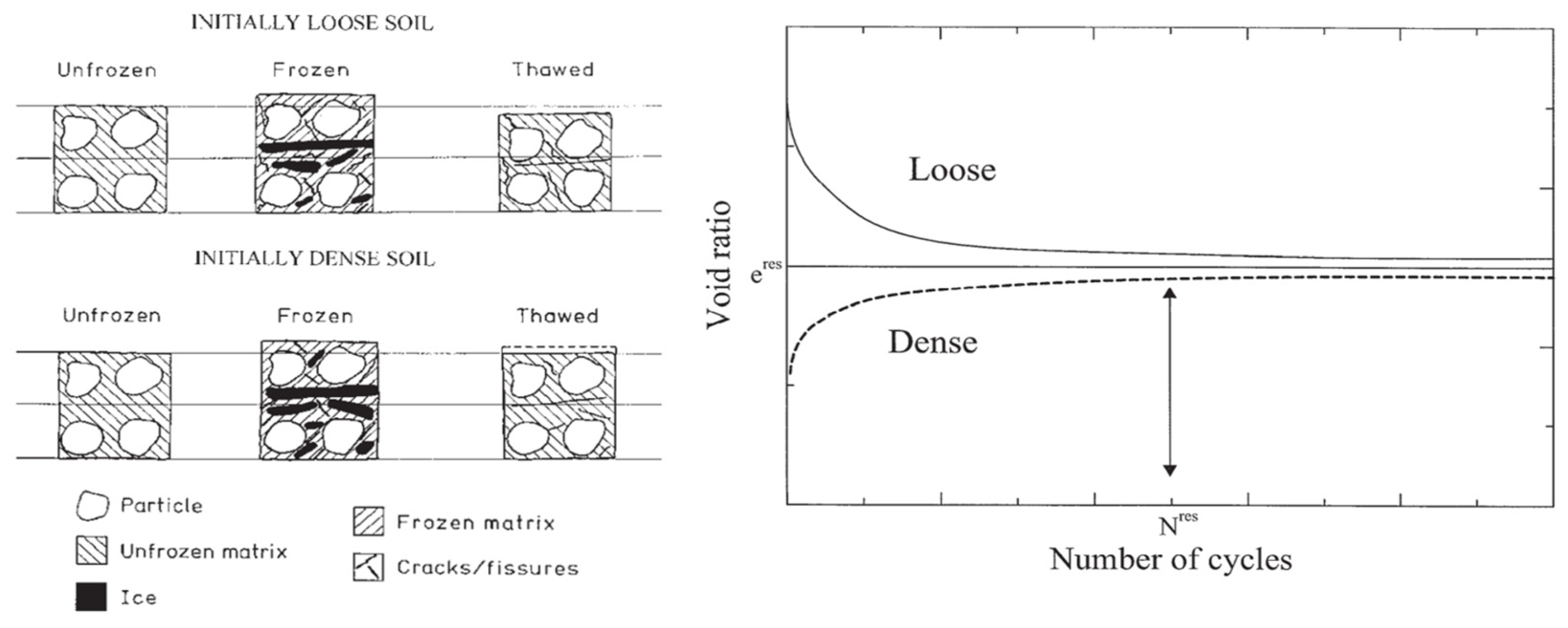
- Soil structure and porosity
- Density and Void Ratio
- Crack formation
6.3.2. Influence of FT Cycles on the Mechanical Properties of Soil
- Stress–strain relationship
- Shear strength parameters
7. Conclusions
- AGF improves soil mechanics and reduces water permeability, creating a more stable environment for construction projects. However, unfrozen water can persist at sub-zero temperatures and migrate in certain soils, forming ice lenses.
- The success of AGF relies on understanding the complex interplay between factors like the temperature, soil composition, and initial ground conditions, which all influence the freezing process. This intricate thermo-hydro-chemo-mechanical process strengthens soil and decreases permeability but can also induce deformations due to water expansion and ice lens formation.
- Frost heave, the upward movement of the ground surface caused by freezing water, has two main contributors: phase change and ice lens formation.
- Overburden pressure, the weight of overlying soil, affects freezing progression and limits ice lens growth by reducing the water flow and lowering the temperature at which ice lenses form.
- Freeze–thaw cycles significantly impact soil properties. In fine-grained soils, FT cycles can lead to over-consolidation, but rapid thawing risks generating high pore pressures and compromising stability.
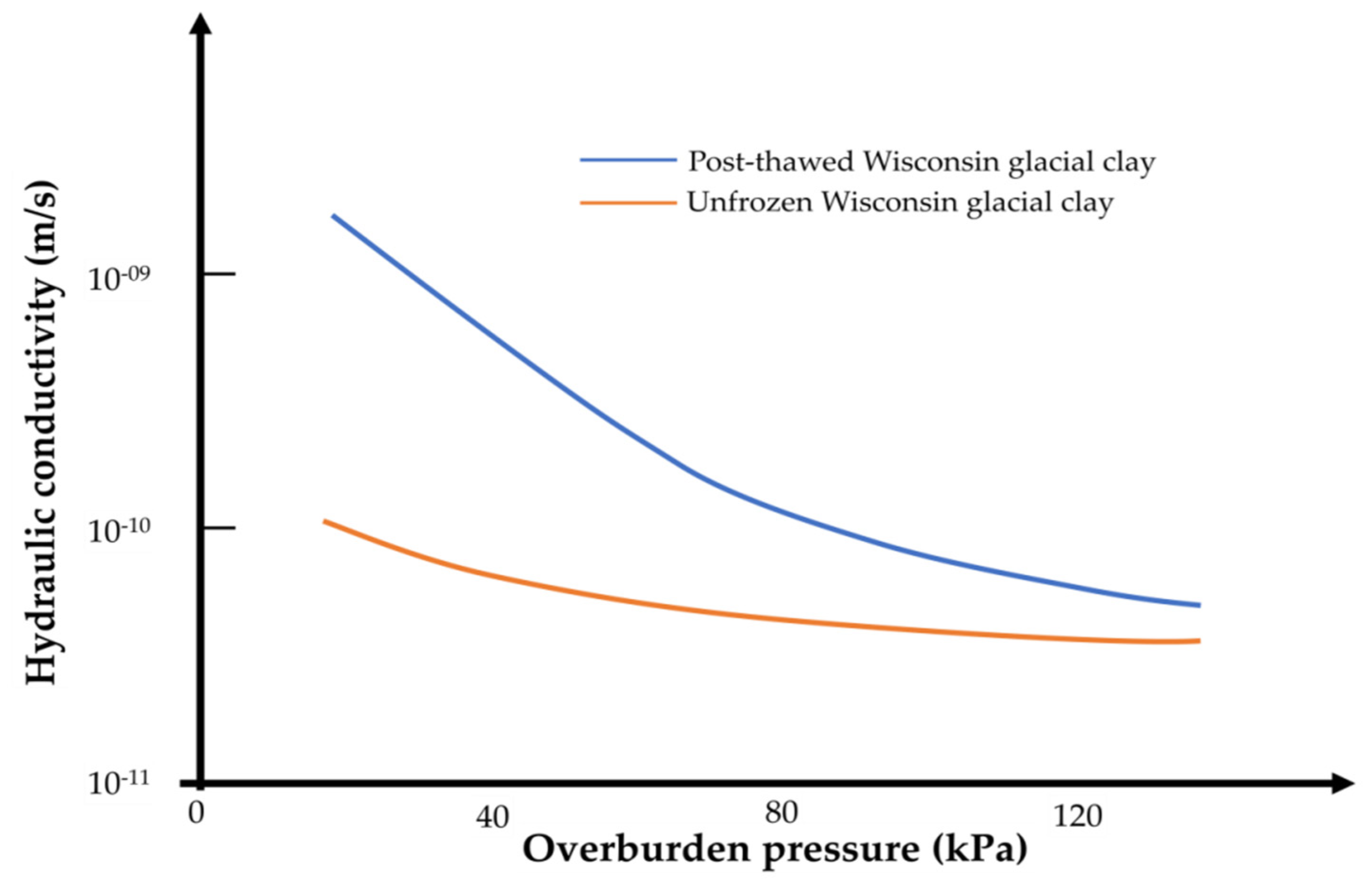
- The freeze–thaw process by itself acts as a form of weathering that influences the mechanical and physical properties of soil at both the microscopic and macroscopic levels. Notably, FT cycles can loosen dense soil, densify loose soil, and increase the overall hydraulic conductivity due to structural changes within the soil matrix. Additionally, these cycles can weaken the soil structure and deteriorate its mechanical performance.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Burt, T.P.; Williams, P.J. Hydraulic conductivity in frozen soils. Earth Surf. Process. 1976, 1, 349–360. [Google Scholar] [CrossRef]
- Harris, J.S. Ground Freezing in Practice; Thomas Telford: London, UK; New York, NY, USA, 1995; ISBN 978-0-7277-1995-9. [Google Scholar]
- Andersland, O.B.; Ladanyi, B. Frozen Ground Engineering Second Edition, 2nd ed.; John Wiley & Sons Canada, Ltd.: Toronto, ON, Canada, 1994; ISBN 978-0-471-61549-1. [Google Scholar]
- Taber, S. Frost Heaving. J. Geol. 1929, 37, 428–461. [Google Scholar] [CrossRef]
- Beskow, G. Soil Freezing and Frost Heaving with Special Application to Roads and Railroads. Swed. Geol. Soc. 1935, 26, 145. [Google Scholar] [CrossRef]
- Johansson, T. Artificial Ground Freezing in Clayey Soils Laboratory and Field Studies of Deformations during Thawing at the Bothnia Line. Ph.D. Thesis, Royal Institute of Technology, Stockholm, Sweden, 2009. [Google Scholar]
- Morgenstern, N.R.; Nixon, J.F. One-dimensional Consolidation of Thawing Soils. Can. Geotech. J. 1971, 8, 558–565. [Google Scholar] [CrossRef]
- Zhang, Z.; Pendin, V.V.; Feng, W.; Zhang, Z. The influence of freeze-thaw cycles on the granulometric composition of Moscow morainic clay. Sci. Cold Arid Reg. 2015, 7, 199–205. [Google Scholar] [CrossRef]
- Adeli Ghareh Viran, P.; Binal, A. Effects of repeated freeze–thaw cycles on physico-mechanical properties of cohesive soils. Arab. J. Geosci. 2018, 11, 250. [Google Scholar] [CrossRef]
- Konrad, J.-M. Physical processes during freeze-thaw cycles in clayey silts. Cold Reg. Sci. Technol. 1989, 16, 291–303. [Google Scholar] [CrossRef]
- Chang, D.K.; Lacy, H.S. Artificial Ground Freezing in Geotechnical Engineering. In Proceedings of the Artificial Ground Freezing in Geotechnical Engineering, Arlington, VA, USA, 14 August 2008; p. 12. [Google Scholar]
- Endo, K. Artificial soil freezing method for subway construction. Civ. Eng./Jpn. 1969, 8, 103–117. [Google Scholar]
- Lunardini, V.J. Heat Transfer in Cold Climates, 1st ed.; Van Nostrand Reinhold Co.: New York, NY, USA, 1981. [Google Scholar]
- Koopmans, R.W.R.; Miller, R.D. Soil Freezing and Soil Water Characteristic Curves. Soil Sci. Soc. Am. J. 1966, 30, 680–685. [Google Scholar] [CrossRef]
- Spaans, E.J.A.; Baker, J.M. The Soil Freezing Characteristic: Its Measurement and Similarity to the Soil Moisture Characteristic. Soil Sci. Soc. Am. J. 1996, 60, 13–19. [Google Scholar] [CrossRef]
- Konrad, J.-M.; Morgenstern, N.R. A mechanistic theory of ice lens formation in fine-grained soils. Can. Geotech. J. 1980, 17, 473–486. [Google Scholar] [CrossRef]
- Tounsi, H. Modélisation THMC de la Congélation Artificielle des Terrains: Application à la Mine de Cigar Lake. Ph.D. Thesis, Université Paris Sciences et Lettres, Paris, France, 2019. [Google Scholar]
- Ren, J. Interpretation of the Frozen Soils Behavior Extending the Mechanics of Unsaturated Soils. Ph.D. Thesis, University of Ottawa, Ottawa, ON, Canada, 2019. Available online: http://ruor.uottawa.ca/handle/10393/39555 (accessed on 11 April 2022).
- Spaans, E.J.A.; Baker, J.M. Examining the use of time domain reflectometry for measuring liquid water content in frozen soil. Water Resources Research. 1995, 31, 2917–2926. [Google Scholar] [CrossRef]
- Smith, M.W.; Tice, A.R. Measurement of the Unfrozen Water Content of Soils: Comparison of NMR (Nuclear Magnetic Resonance) and TDR (Time Domain Reflectometry) Methods; US Army Corps of Engineers Cold Regions Research & Engineering Laboratory: Hanover, NH, USA, 1988; Available online: https://apps.dtic.mil/sti/citations/ADA203082 (accessed on 11 April 2022).
- Tice, A.R.; Oliphant, J.L.; Nakano, Y.; Jenkins, T.F. Relationship between the Ice and Unfrozen Water Phases in Frozen Soil as Determined by Pulsed Nuclear Magnetic Resonance and Physical Desorption Data; U.S. Army Corps of Engineers, Cold Regions Research & Engineering Laboratory: Hanover, NH, USA, 1982; Available online: https://apps.dtic.mil/sti/citations/ADA118486 (accessed on 11 April 2022).
- Watanabe, K.; Mizoguchi, M. Amount of unfrozen water in frozen porous media saturated with solution. Cold Reg. Sci. Technol. 2002, 34, 103–110. [Google Scholar] [CrossRef]
- Kozlowski, T. A comprehensive method of determining the soil unfrozen water curves: 2. Stages of the phase change process in frozen soil–water system. Cold Reg. Sci. Technol. 2003, 36, 81–92. [Google Scholar] [CrossRef]
- Fabbri, A.; Fen-Chong, T.; Azouni, A.; Thimus, J.-F. Investigation of Water to Ice Phase Change in Porous Media by Ultrasonic and Dielectric Measurements. J. Cold Reg. Eng. 2009, 23, 69–90. [Google Scholar] [CrossRef]
- Konrad, J.-M. Sixteenth Canadian Geotechnical Colloquium: Frost heave in soils: Concepts and engineering. Can. Geotech. J. 1994, 31, 223–245. [Google Scholar] [CrossRef]
- Azmatch, T.F. Frost Heave: New Ice Lens Initiation Condition and Hydraulic Conductivity Prediction. Ph.D. Thesis, University of Alberta, Edmonton, AB, Canada, 2013. Available online: https://scholar.archive.org/work/n7xgctztgbb7xoaslebmxelbji (accessed on 24 July 2022).
- Sayles, F.H. Creep of Frozen Sands; US Army CRREL: Hanover, NH, USA, 1968; p. 63. [Google Scholar]
- Nixon, J.F. Thaw–Consolidation of Some Layered Systems. Can. Geotech. J. 1973, 10, 15. [Google Scholar] [CrossRef]
- Hampton, C. Strength and Creep Testing of Artificial Ground Freezing. Ph.D. Thesis, University of Nottingham, Nottingham, UK, 1986. [Google Scholar]
- Dysli, M. Le Gel et Son Action Sur Les Sols et Les Fondations; Presses Polytechniques et Universitaires Romandes (PPUR): Lausann, Switzerland, 1991; ISBN 2-88074-229-3. [Google Scholar]
- Rajaei, P.; Baladi, G. Frost Heave: A Semi-Empirical Model Based on Field Data. In Proceedings of the Cold Regions Engineering 2015, Salt Lake City, UT, USA, 19–22 July 2015; American Society of Civil Engineers: Salt Lake City, UT, USA, 2015; pp. 382–393. [Google Scholar] [CrossRef]
- Zhao, J.; Zhang, P.; Yang, X.; Qi, J. On the uniaxial compression strength of frozen gravelly soils. Cold Reg. Sci. Technol. 2020, 171, 102965. [Google Scholar] [CrossRef]
- Ji, Y.; Zhou, G.; Vandeginste, V.; Zhou, Y. Thermal-hydraulic-mechanical coupling behavior and frost heave mitigation in freezing soil. Bull. Eng. Geol. Environ. 2021, 80, 2701–2713. [Google Scholar] [CrossRef]
- Sweidan, A.H.; Niggemann, K.; Heider, Y.; Ziegler, M.; Markert, B. Experimental study and numerical modeling of the thermo-hydro-mechanical processes in soil freezing with different frost penetration directions. Acta Geotech. 2022, 17, 231–255. [Google Scholar] [CrossRef]
- Vitel, M. Modélisation Thermo-Hydraulique de la Congélation Artificielle des Terrains. Ph.D. Thesis, Ecole Nationale Supérieure des Mines de Paris, Paris, France, 2015. [Google Scholar]
- Penner, E.; Ueda, T. The dependence of frost heaving on load application—Preliminary results. Proc. Int. Symp. Frost Action Soils 1977, 1, 92–101. [Google Scholar]
- Loch, J.P.G.; Kay, B.D. Water Redistribution in Partially Frozen, Saturated Silt Under Several Temperature Gradients and Overburden Loads. Soil Sci. Soc. Am. J. 1978, 42, 400–406. [Google Scholar] [CrossRef]
- Konrad, J.-M.; Morgenstern, N.R. Effects of applied pressure on freezing soils. Can. Geotech. J. 1982, 19, 494–505. [Google Scholar] [CrossRef]
- Yamamoto, Y.; Springman, S.M. Axial compression stress path tests on artificial frozen soil samples in a triaxial device at temperatures just below 0 °C. Can. Geotech. J. 2014, 51, 1178–1195. [Google Scholar] [CrossRef]
- Banin, A.; Anderson, D.M. Effects of Salt Concentration Changes During Freezing on the Unfrozen Water Content of Porous Materials. Water Resour. Res. 1974, 10, 124–128. [Google Scholar] [CrossRef]
- Pitzer, K.S.; Peiper, J.C.; Busey, R.H. Thermodynamic Properties of Aqueous Sodium Chloride Solutions. J. Phys. Chem. Ref. Data 1984, 13, 1–102. [Google Scholar] [CrossRef]
- Bing, H.; Ma, W. Laboratory investigation of the freezing point of saline soil. Cold Reg. Sci. Technol. 2011, 67, 79–88. [Google Scholar] [CrossRef]
- Wan, X.; Lai, Y.; Wang, C. Experimental Study on the Freezing Temperatures of Saline Silty Soils. Permafr. Periglac. Process. 2015, 26, 175–187. [Google Scholar] [CrossRef]
- Xiao, Z.; Lai, Y.; Zhang, M. Study on the freezing temperature of saline soil. Acta Geotech. 2018, 13, 195–205. [Google Scholar] [CrossRef]
- Zhou, J.; Zhao, W.; Tang, Y. Practical prediction method on frost heave of soft clay in artificial ground freezing with field experiment. Tunn. Undergr. Space Technol. 2021, 107, 103647. [Google Scholar] [CrossRef]
- Dash, J.G. Thermomolecular Pressure in Surface Melting: Motivation for Frost Heave. Science 1989, 246, 1591–1593. [Google Scholar] [CrossRef] [PubMed]
- Tiedje, E. The Experimental Characterization and Numerical Modelling of Frost Heave. Ph.D. Thesis, McMaster University, Hamilton, ON, Canada, 2015. [Google Scholar]
- Taber, S. The Mechanics of Frost Heaving. J. Geol. 1930, 38, 303–317. [Google Scholar] [CrossRef]
- Sheng, D.; Zhang, S.; Yu, Z.; Zhang, J. Assessing frost susceptibility of soils using PCHeave. Cold Reg. Sci. Technol. 2013, 95, 27–38. [Google Scholar] [CrossRef]
- Ming, F.; Zhang, Y.; Li, D. Experimental and theoretical investigations into the formation of ice lenses in deformable porous media. Geosci. J. 2016, 20, 667–679. [Google Scholar] [CrossRef]
- Zhang, X.; Zhang, M.; Lu, J.; Pei, W.; Yan, Z. Effect of hydro-thermal behavior on the frost heave of a saturated silty clay under different applied pressures. Appl. Therm. Eng. 2017, 117, 462–467. [Google Scholar] [CrossRef]
- Ji, Y.; Zhou, G.; Hall, M.R.; Vandeginste, V.; Zhao, X. Thermal-Hydraulic-Mechanical Coupling Research on Overburden Pressure Mitigated Ice Lens Growth in the Freezing Soil. KSCE J. Civ. Eng. 2022, 26, 1606–1617. [Google Scholar] [CrossRef]
- Miller, R.D. Freezing and heaving of saturated and unsaturated soils. Highw. Res. Rec. 1972, 393, 1–11. [Google Scholar]
- O’Neill, K.; Miller, R.D. Exploration of a Rigid Ice Model of Frost Heave. Water Resour. Res. 1985, 21, 281–296. [Google Scholar] [CrossRef]
- Akagawa, S. Experimental study of frozen fringe characteristics. Cold Reg. Sci. Technol. 1988, 15, 209–223. [Google Scholar] [CrossRef]
- Xia, D.; Arenson, L.; Biggar, K.; Sego, D. Freezing process in Devon silt-using time-lapse photography. In Proceedings of the 58th Canadian Geotechnical Conference, Saskatoon, SK, Canada, 1 January 2005. [Google Scholar]
- Arenson, L.; Sego, D.; Take, W. Measurement of ice lens growth and soil consolidation during frost penetration using particle image velocimetry (PIV). In Proceedings of the 60th Canadian Geotechnical Conference, Ottawa, ON, Canada, 21 October 2007. [Google Scholar]
- Zhang, L.; Ma, W.; Yang, C.; Wen, Z.; Dong, S. An investigation of pore water pressure and consolidation phenomenon in the unfrozen zone during soil freezing. Cold Reg. Sci. Technol. 2016, 130, 21–32. [Google Scholar] [CrossRef]
- Joudieh, Z. Artificial Ground Freezing: Characterization and Modeling of the Hydromechanical Behavior under Thermal Solicitations. Ph.D. Thesis, Université de Lorraine, Nancy, France, 2024. [Google Scholar]
- Peppin, S.S.L.; Style, R.W. The Physics of Frost Heave and Ice-Lens Growth. Vadose Zone J. 2013, 12, 1–12. [Google Scholar] [CrossRef]
- Henry, K.S. A Review of the Thermodynamics of Frost Heave; Engineer Research and Development Center Hanover NH Cold Regions Research And Engineering Lab: Hanover, Germany, 2000; p. 26. [Google Scholar]
- O’Neill, K.; Miller, R. Numerical Solutions for a Rigid-Ice Model of Secondary Frost Heave; US Army Corps of Engineer: Hanover, NH, USA, 1982; Available online: https://www.semanticscholar.org/paper/Numerical-Solutions-for-a-Rigid-Ice-Model-of-Frost-O%27Neill-Miller/1e8e064cf5f114a8c1eef9ba972894b9794fdd18 (accessed on 5 August 2022).
- Gilpin, R.R. A model for the prediction of ice lensing and frost heave in soils. Water Resour. Res. 1980, 16, 918–930. [Google Scholar] [CrossRef]
- Akagawa, S.; Satoh, M.; Kanie, S.; Mikami, T. Effect of Tensile Strength on Ice Lens Initiation Temperature. In Proceedings of the Cold Regions Engineering 2006, Orono, ME, USA, 23–26 July 2006; pp. 1–12. [Google Scholar] [CrossRef]
- Harlan, R.L. Analysis of coupled heat-fluid transport in partially frozen soil. Water Resour. Res. 1973, 9, 1314–1323. [Google Scholar] [CrossRef]
- Miller, R.D. Frost heaving in non-colloidal soils. In Proceedings of the 3rd International Conference on Permafrost, Edmonton, AB, Canada, 10 July 1978. [Google Scholar]
- Watanabe, K.; Flury, M. Capillary bundle model of hydraulic conductivity for frozen soil. Water Resour. Res. 2008, 44, 9. [Google Scholar] [CrossRef]
- Watanabe, K.; Wake, T. Measurement of unfrozen water content and relative permittivity of frozen unsaturated soil using NMR and TDR. Cold Reg. Sci. Technol. 2009, 59, 34–41. [Google Scholar] [CrossRef]
- Black, P.B. The Rigid Ice Model of Frost Heave and Its Input Functions; Cornell University: Ithaca, NY, USA, 1985. [Google Scholar]
- Ishizaki, T.; Nishio, N. Experimental study of frost heaving of a saturated soil. Tokyo Gas Gijutsu Kenkyusho Hokoku 1988, 88, 65–72. [Google Scholar]
- Sheng, D.; Axelsson, K.; Knutsson, S. Frost Heave due to Ice Lens Formation in Freezing Soils. Hydrol. Res. 1995, 26, 147–168. [Google Scholar] [CrossRef]
- Bronfenbrener, L.; Bronfenbrener, R. Modeling frost heave in freezing soils. Cold Reg. Sci. Technol. 2010, 61, 43–64. [Google Scholar] [CrossRef]
- Nixon, J.F. (Derick) Discrete ice lens theory for frost heave in soils. Can. Geotech. J. 1991, 28, 843–859. [Google Scholar] [CrossRef]
- Ji, Y.; Zhou, G.; Zhou, Y.; Vandeginste, V. Frost heave in freezing soils: A quasi-static model for ice lens growth. Cold Reg. Sci. Technol. 2019, 158, 10–17. [Google Scholar] [CrossRef]
- Nishimura, S.; Gens, A.; Olivella, S.; Jardine, R.J. THM-coupled finite element analysis of frozen soil: Formulation and application. Géotechnique 2009, 59, 159–171. [Google Scholar] [CrossRef]
- Casini, F.; Gens, A.; Olivella, S.; Viggiani, G.M.B. Artificial ground freezing of a volcanic ash: Laboratory tests and modelling. Environ. Geotech. 2016, 3, 141–154. [Google Scholar] [CrossRef]
- Arvidson, W.D.; Morgenstern, N. Water flow induced by soil freezing. Can. Geotech. J. 1977, 14, 237–245. [Google Scholar] [CrossRef]
- Penner, E.; Walton, T. Effects of temperature and pressure on frost heaving. Eng. Geol. 1979, 13, 29–39. [Google Scholar] [CrossRef]
- Konrad, J.-M. Estimation of the segregation potential of fine-grained soils using the frost heave response of two reference soils. Can. Geotech. J. 2005, 42, 38–50. [Google Scholar] [CrossRef]
- Konrad, J.-M. Influence of cooling rate on the temperature of ice lens formation in clayey silts. Cold Reg. Sci. Technol. 1989, 16, 25–36. [Google Scholar] [CrossRef]
- Konrad, J.M. Frost Heave Mechanics. Ph.D. Thesis, University of Alberta, Edmonton, AB, Canada, 1980. [Google Scholar]
- Azmatch, T.F.; Sego, D.C.; Arenson, L.U.; Biggar, K.W. New ice lens initiation condition for frost heave in fine-grained soils. Cold Reg. Sci. Technol. 2012, 82, 8–13. [Google Scholar] [CrossRef]
- Lu, X.; Zhang, F.; Qin, W.; Zheng, H.; Feng, D. Experimental investigation on frost heave characteristics of saturated clay soil under different stress levels and temperature gradients. Cold Reg. Sci. Technol. 2021, 192, 103379. [Google Scholar] [CrossRef]
- Chamberlain, E.J. Overconsolidation effects of ground freezing. Eng. Geol. 1981, 18, 14. [Google Scholar] [CrossRef]
- Chamberlain, E.J.; Gow, A.J. Effect of Freezing and Thawing on the Permeability and Structure of Soils. In Developments in Geotechnical Engineering; Elsevier: Amsterdam, The Netherlands, 1979; Volume 26, pp. 73–92. ISBN 978-0-444-41782-4. [Google Scholar]
- Graham, J.; Au, V.C.S. Effects of freeze–thaw and softening on a natural clay at low stresses. Can. Geotech. J. 1985, 22, 10. [Google Scholar] [CrossRef]
- Bittelli, M.; Flury, M.; Campbell, G.S. A thermodielectric analyzer to measure the freezing and moisture characteristic of porous media. Water Resour. Res. 2003, 39, 10. [Google Scholar] [CrossRef]
- He, H.; Dyck, M. Application of Multiphase Dielectric Mixing Models for Understanding the Effective Dielectric Permittivity of Frozen Soils. Vadose Zone J. 2013, 12, 1–22. [Google Scholar] [CrossRef]
- Tian, H.; Wei, C.; Wei, H.; Zhou, J. Freezing and thawing characteristics of frozen soils: Bound water content and hysteresis phenomenon. Cold Reg. Sci. Technol. 2014, 103, 74–81. [Google Scholar] [CrossRef]
- He, H.; Dyck, M.F.; Si, B.C.; Zhang, T.; Lv, J.; Wang, J. Soil freezing–thawing characteristics and snowmelt infiltration in Cryalfs of Alberta, Canada. Geoderma Reg. 2015, 5, 198–208. [Google Scholar] [CrossRef]
- Homshaw, L.G. Freezing and Melting Temperature Hysteresis of Water in Porous Materials: Application to the Study of Pore Form. J. Soil Sci. 1980, 31, 399–414. [Google Scholar] [CrossRef]
- Anderson, R.; Tohidi, B.; Webber, J.B.W. Gas hydrate growth and dissociation in narrow pore networks: Capillary inhibition and hysteresis phenomena. In Sediment-Hosted Gas Hydrates: New Insights on Natural and Synthetic Systems; Long, D., Lovell, M.A., Rees, J.G., Rochelle, C.A., Eds.; Geological Society of London: London, UK, 2009; Volume 319, pp. 145–159. ISBN 978-1-86239-279-3. [Google Scholar]
- Liu, Z.; Yu, X. Physically Based Equation for Phase Composition Curve of Frozen Soils. Transp. Res. Rec. 2013, 2349, 93–99. [Google Scholar] [CrossRef]
- Zhou, Y.; Zhou, J.; Shi, X.; Zhou, G. Practical models describing hysteresis behavior of unfrozen water in frozen soil based on similarity analysis. Cold Reg. Sci. Technol. 2019, 157, 215–223. [Google Scholar] [CrossRef]
- Kozlowski, T.; Nartowska, E. Unfrozen Water Content in Representative Bentonites of Different Origin Subjected to Cyclic Freezing and Thawing. Vadose Zone J. 2013, 12, 1–11. [Google Scholar] [CrossRef]
- Ren, J.; Vanapalli, S.K. Effect of freeze–thaw cycling on the soil-freezing characteristic curve of five Canadian soils. Vadose Zone J. 2020, 19, e20039. [Google Scholar] [CrossRef]
- Chamberlain, E.J.; Blouin, S.E. Freeze-Thaw Enhancement of the Drainage and Consolidation of Fine-Grained Dredged Material in Confined Disposal Areas: Final Report; Department of Defense, Department of the Army, Corps of Engineers, Waterways Experiment Station, Environmental Effects Laboratory: Vicksburg, MS, USA, 1977. [Google Scholar]
- Othman, M.A.; Benson, C.H. Effect of freeze–thaw on the hydraulic conductivity and morphology of compacted clay. Can. Geotech. J. 1993, 30, 236–246. [Google Scholar] [CrossRef]
- Eigenbrod, K.D.; Knutsson, S.; Sheng, D. Pore-Water Pressures in Freezing and Thawing Fine-Grained Soils. J. Cold Reg. Eng. 1996, 10, 77–92. [Google Scholar] [CrossRef]
- Viklander, P. Compaction and Thaw Deformation of Frozen Soil: Permeability and Structural Effects due to Freezing and Thawing. Ph.D. Thesis, Luleå University of Technology, Luleå, Sweden, 1997. [Google Scholar]
- Han, Y.; Wang, Q.; Wang, N.; Wang, J.; Zhang, X.; Cheng, S.; Kong, Y. Effect of freeze-thaw cycles on shear strength of saline soil. Cold Reg. Sci. Technol. 2018, 154, 42–53. [Google Scholar] [CrossRef]
- Zhai, J.; Zhang, Z.; Melnikov, A.; Zhang, M.; Yang, L.; Jin, D. Experimental Study on the Effect of Freeze—Thaw Cycles on the Mineral Particle Fragmentation and Aggregation with Different Soil Types. Minerals 2021, 11, 913. [Google Scholar] [CrossRef]
- An, R.; Zhang, X.; Kong, L.; Gong, J.; Lei, X. Artificial Ground Freezing Impact on Shear Strength and Microstructure of Granite Residual Soil under an Extremely Low Temperature. Front. Earth Sci. 2021, 9, 772459. [Google Scholar] [CrossRef]
- Viklander, P. Permeability and volume changes in till due to cyclic freeze/thaw. Can. Geotech. J. 1998, 35, 471–477. [Google Scholar] [CrossRef]
- Zhai, J.; Zhang, S.; Zhang, Z.; Melnikov, A.; Li, H. Study on the geometry characteristics of soil primary mineral particles under cryogenic action. Sci. Rep. 2022, 12, 16766. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.-L.; Cui, Z.-D. Effects of freezing-thawing and cyclic loading on pore size distribution of silty clay by mercury intrusion porosimetry. Cold Reg. Sci. Technol. 2018, 145, 185–196. [Google Scholar] [CrossRef]
- Eigenbrod, K.D. Effects of cyclic freezing and thawing on volume changes and permeabilities of soft fine-gained soils. Can. Geotech. J. 1996, 33, 529–537. [Google Scholar] [CrossRef]
- Qi, J.; Ma, W.; Song, C. Influence of freeze–thaw on engineering properties of a silty soil. Cold Reg. Sci. Technol. 2008, 53, 397–404. [Google Scholar] [CrossRef]
- Leroueil, S.; Tardif, J.; Roy, M.; Rochelle, P.L.; Konrad, J.-M. Effects of frost on the mechanical behaviour of Champlain Sea clays. Can. Geotech. J. 1991, 28, 690–697. [Google Scholar] [CrossRef]
- Aubert, J.E.; Gasc-Barbier, M. Hardening of clayey soil blocks during freezing and thawing cycles. Appl. Clay Sci. 2012, 65–66, 1–5. [Google Scholar] [CrossRef]
- Li, G.; Ma, W.; Zhao, S.; Mao, Y.; Mu, Y. Effect of Freeze-Thaw Cycles on Mechanical Behavior of Compacted Fine-Grained Soil. In Sustainable Infrastructure Development in a Changing Cold Environment, Proceedings of Cold Regions Engineering 2012, Quebec City, Canada, 19 August 2012; Morse, B., Doré, G., Eds.; American Society of Civil Engineers: Reston, VA, USA, 2012; pp. 72–81. [Google Scholar] [CrossRef]
- Wang, X.; Li, S.; Sun, Y.; Zhang, C.; Liu, G. Influence of freeze–thaw cycling on the soil mechanical properties of open-pit mine dump under different moisture contents. Environ. Earth Sci. 2021, 80, 279. [Google Scholar] [CrossRef]
- Liu, J.; Chang, D.; Yu, Q. Influence of freeze-thaw cycles on mechanical properties of a silty sand. Eng. Geol. 2016, 210, 23–32. [Google Scholar] [CrossRef]
- Wang, D.; Ma, W.; Niu, Y.; Chang, X.; Wen, Z. Effects of cyclic freezing and thawing on mechanical properties of Qinghai–Tibet clay. Cold Reg. Sci. Technol. 2007, 48, 34–43. [Google Scholar] [CrossRef]
- Yao, X.; Fang, L.; Qi, J.; Yu, F. Study on Mechanism of Freeze-Thaw Cycles Induced Changes in Soil Strength Using Electrical Resistivity and X-Ray Computed Tomography. J. Offshore Mech. Arct. Eng. 2017, 139, 021501. [Google Scholar] [CrossRef]

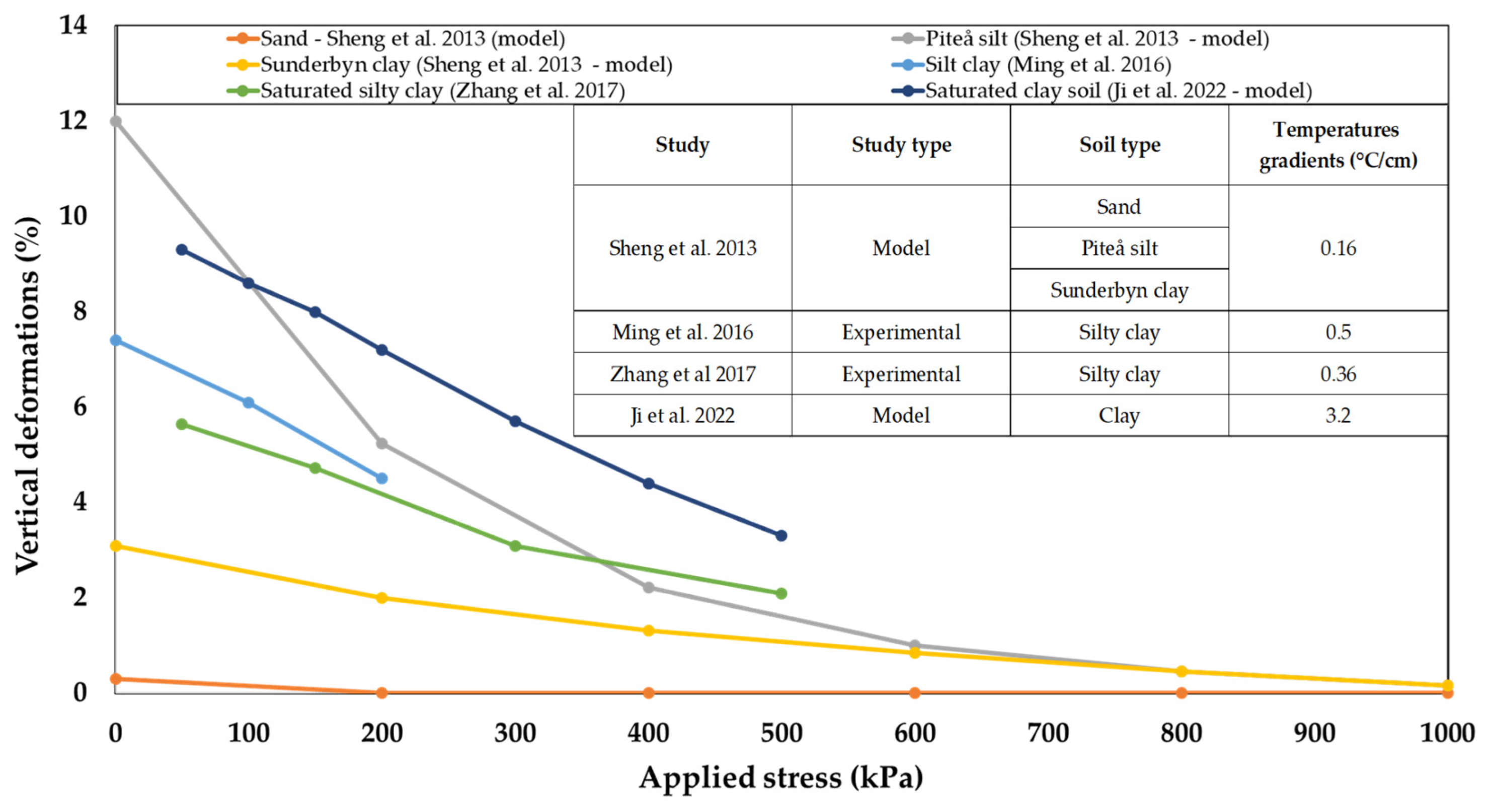

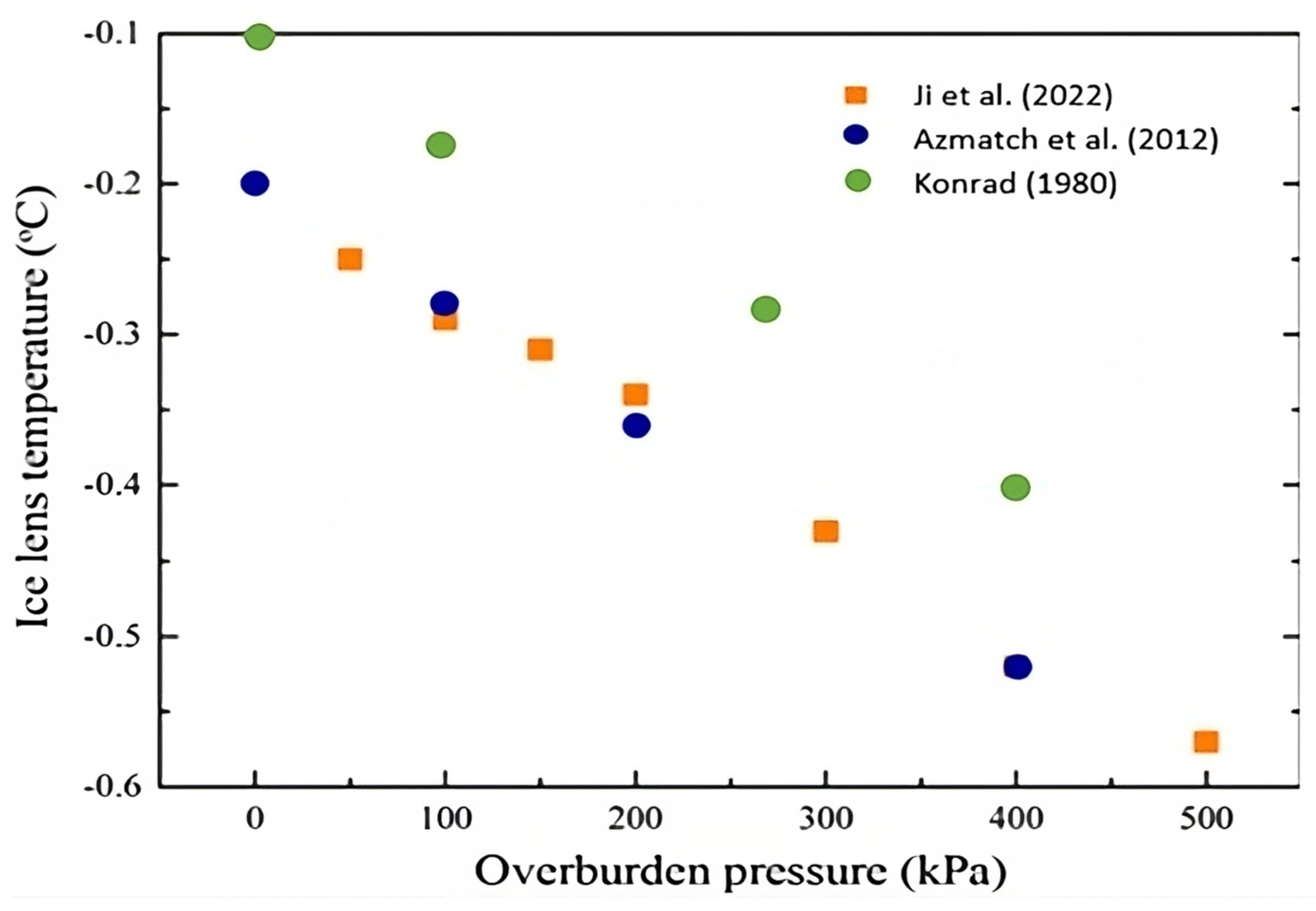
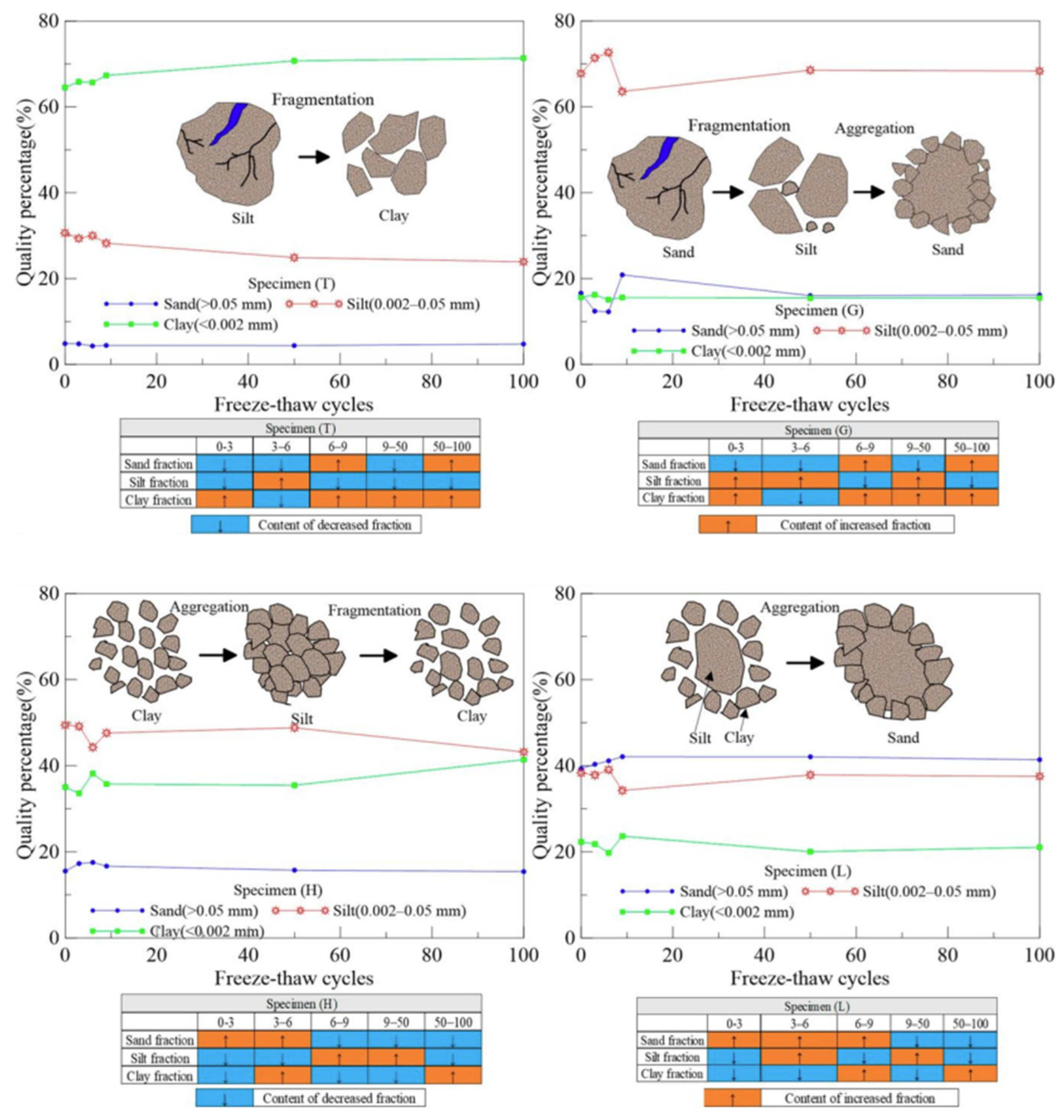
| Mechanism | Explanation | References |
|---|---|---|
| Supercooling of pore water | Despite reaching its freezing point, soil pore water does not always freeze. Instead, pore water is kept in the liquid phase until the temperature drops to temperature Tsc, where water super-cools and freezing is initiated by ice nucleation. On the other hand, the thawing branch displays no rapid changes in θu and no superheating phenomena. | [87,88,89,90] |
| Effect of electrolytes | When soil is frozen, electrolytes are excluded from the ice, increasing the solute concentrations in the remaining pore water. This lowers the freezing point of the remaining pore water and promotes hysteresis. | [87,88,89,90] |
| Pore geometry | Hysteresis may be linked to the different curvatures of the ice–water contact during freezing and thawing. Crystallization initiates in large pores upon freezing. Comparatively, when soil is thawed, pore ice melting begins in small pores and then advances gradually to larger pores. Hence, for the same amount of unfrozen water, the temperature on the thawing branch is higher than that on the freezing branch. The melting branch is, therefore, underneath the freezing branch, hence the hysteresis. | [91,92] |
| Pore blocking: bottleneck effect | The bottleneck effect, which is thought to be the principal reason for the hysteresis frequently seen for the air–water phase transition in soils with a broad range of interconnected pores of diverse geometry (different radii), may also contribute to the hysteresis for the ice-water phase transition in the soil. This effect originates from large pores with narrow necks. | [87,92] |
| Effect of contact angle | The rising contact angle during soil freezing differs from the retreating contact angle during soil thawing. | [93,94] |
| Change in the pore structure | Hysteresis may be caused by the thixotropic property and aging conditions that shape pore size distribution. Soil particles usually relocate from their original locations as soil pores expand due to ice development during the freezing process. Consequently, larger pores would be present than before soil freezing. | [93] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Joudieh, Z.; Cuisinier, O.; Abdallah, A.; Masrouri, F. Artificial Ground Freezing—On the Soil Deformations during Freeze–Thaw Cycles. Geotechnics 2024, 4, 718-741. https://doi.org/10.3390/geotechnics4030038
Joudieh Z, Cuisinier O, Abdallah A, Masrouri F. Artificial Ground Freezing—On the Soil Deformations during Freeze–Thaw Cycles. Geotechnics. 2024; 4(3):718-741. https://doi.org/10.3390/geotechnics4030038
Chicago/Turabian StyleJoudieh, Zeina, Olivier Cuisinier, Adel Abdallah, and Farimah Masrouri. 2024. "Artificial Ground Freezing—On the Soil Deformations during Freeze–Thaw Cycles" Geotechnics 4, no. 3: 718-741. https://doi.org/10.3390/geotechnics4030038
APA StyleJoudieh, Z., Cuisinier, O., Abdallah, A., & Masrouri, F. (2024). Artificial Ground Freezing—On the Soil Deformations during Freeze–Thaw Cycles. Geotechnics, 4(3), 718-741. https://doi.org/10.3390/geotechnics4030038








