Impurity Ferromagnetism of Pd-Fe and Pd-Co Alloys: Ab Initio vs. Experiment †
Abstract
:1. Introduction
2. Materials and Methods
2.1. DFT Calculation Details
2.2. The Experiment Description
3. Results
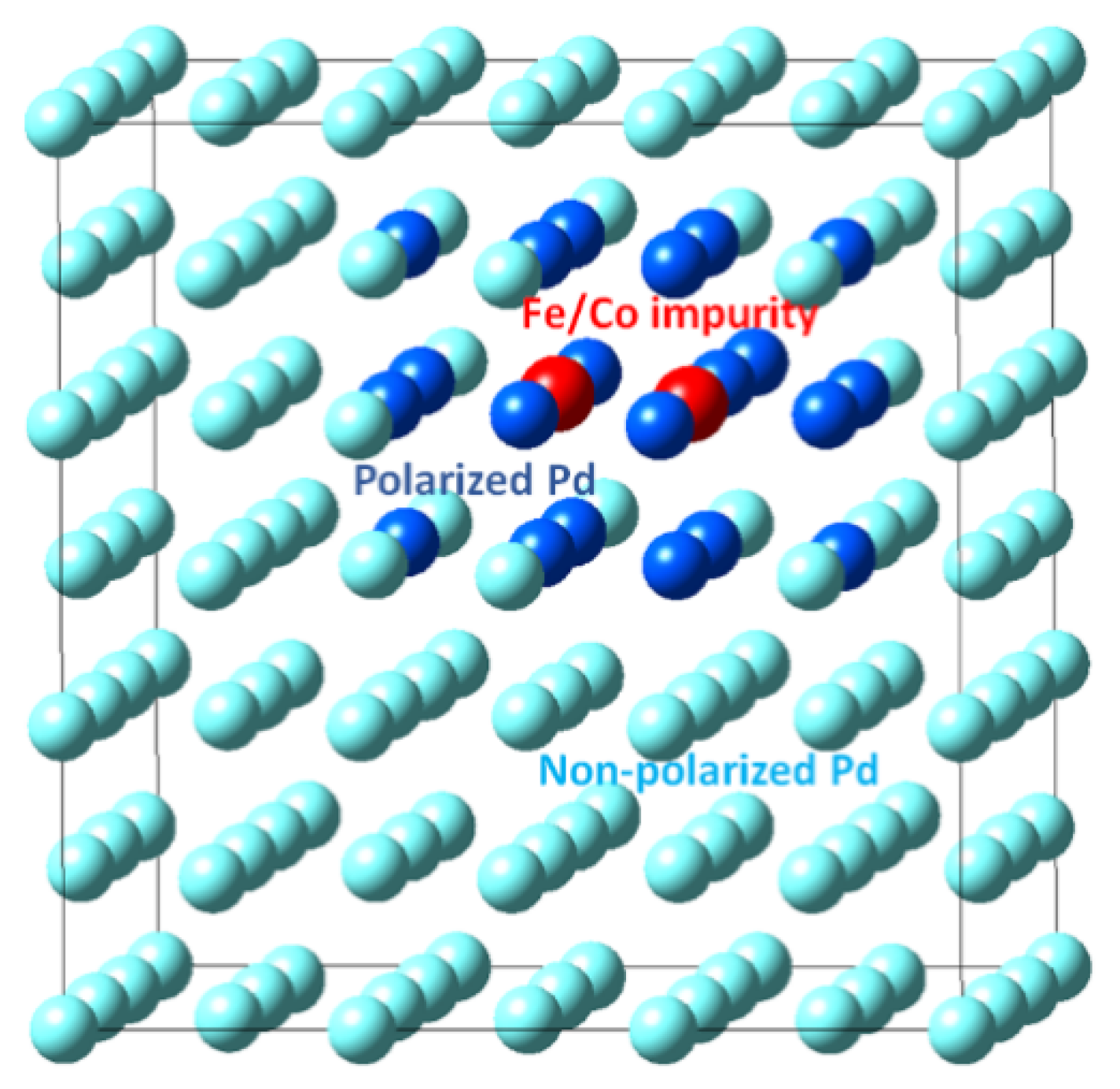
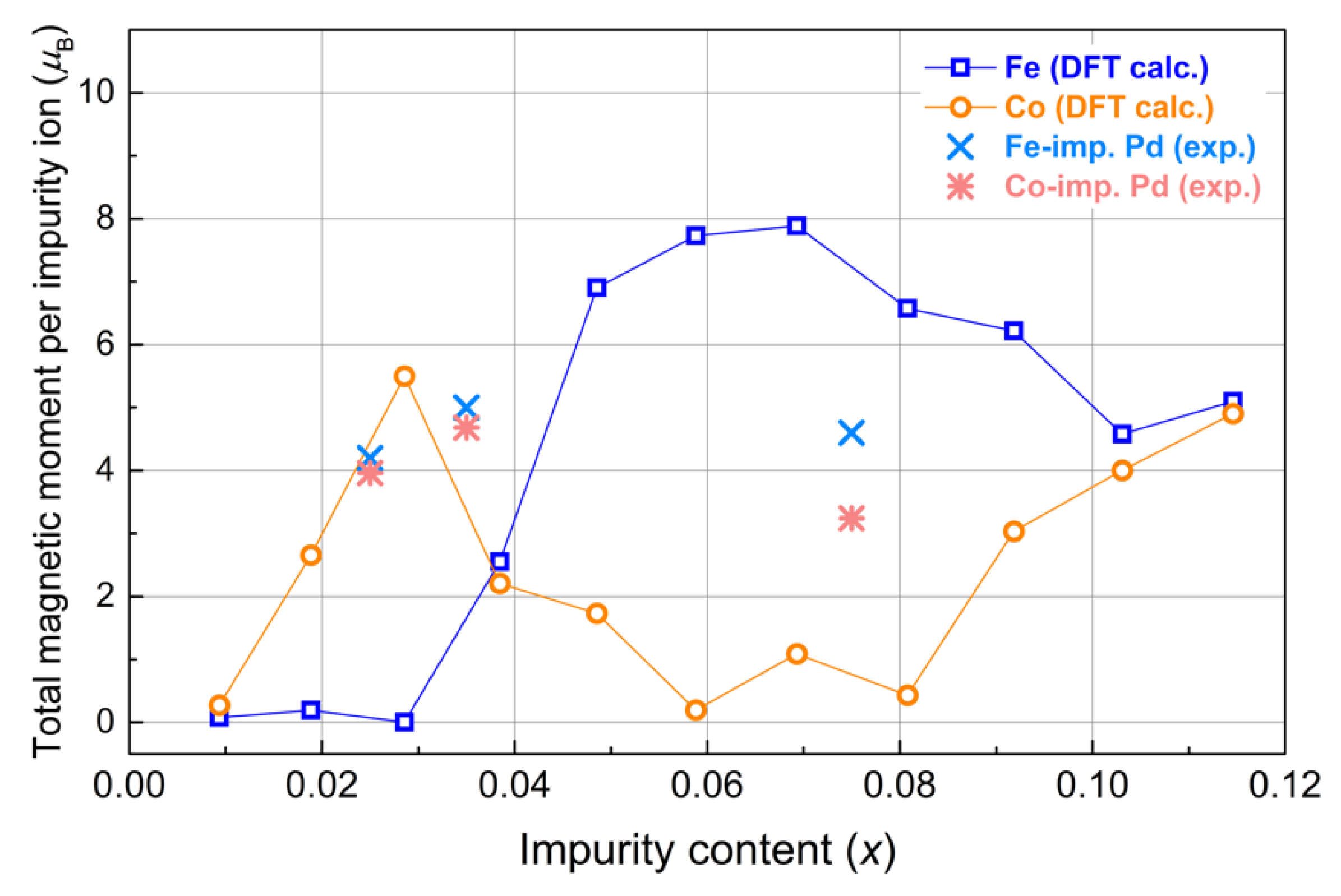
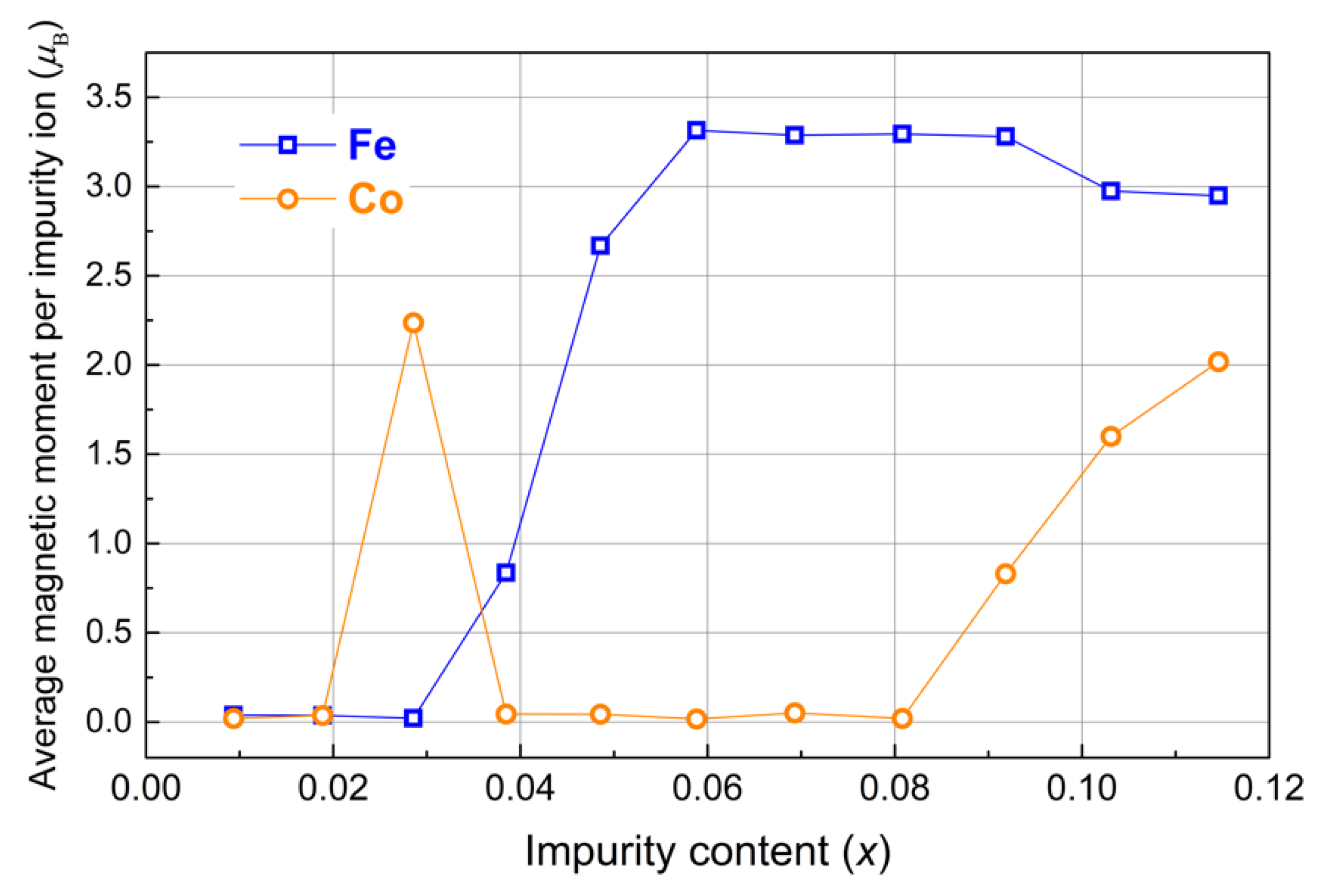
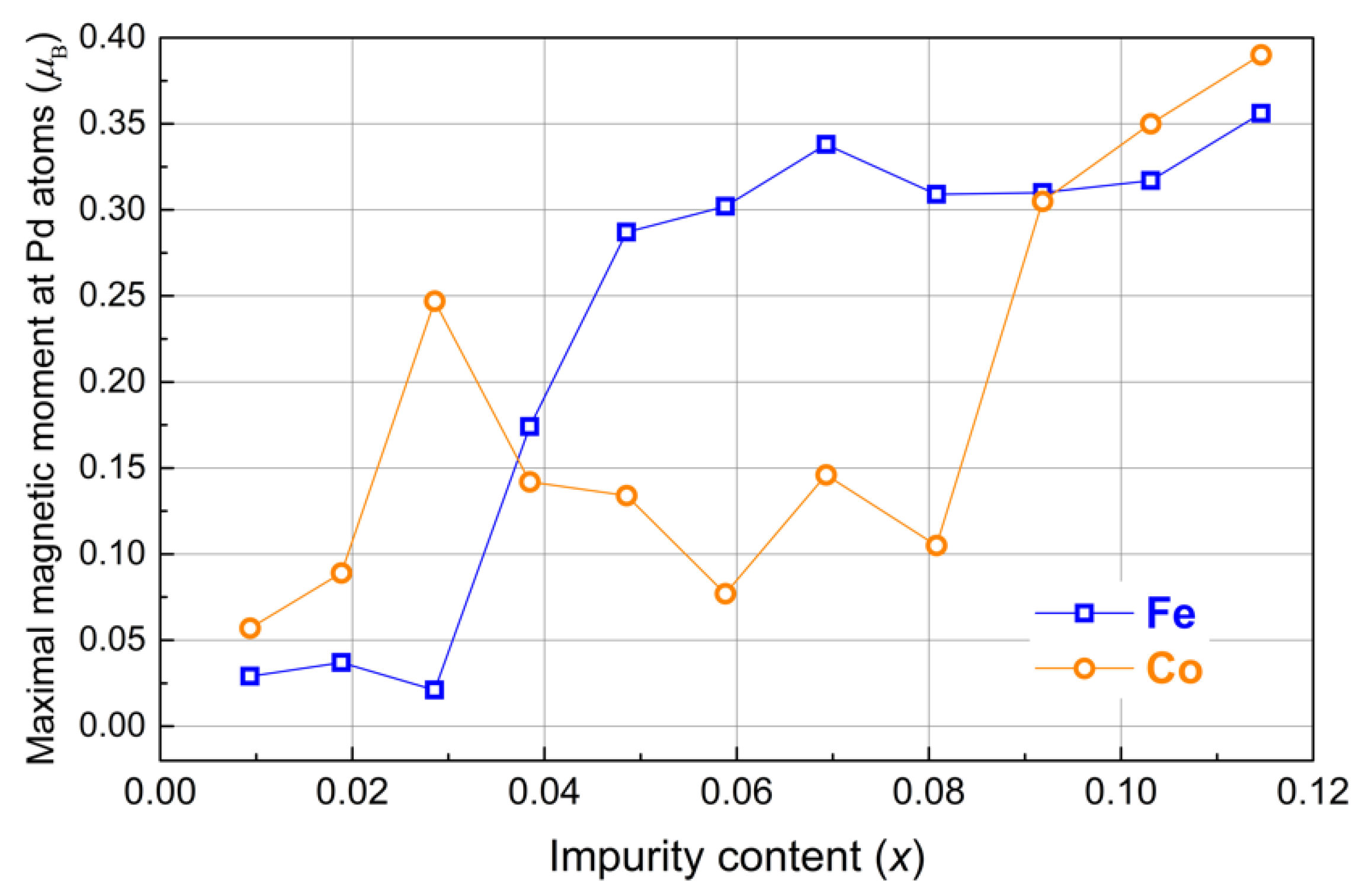
3.1. DFT Calculations
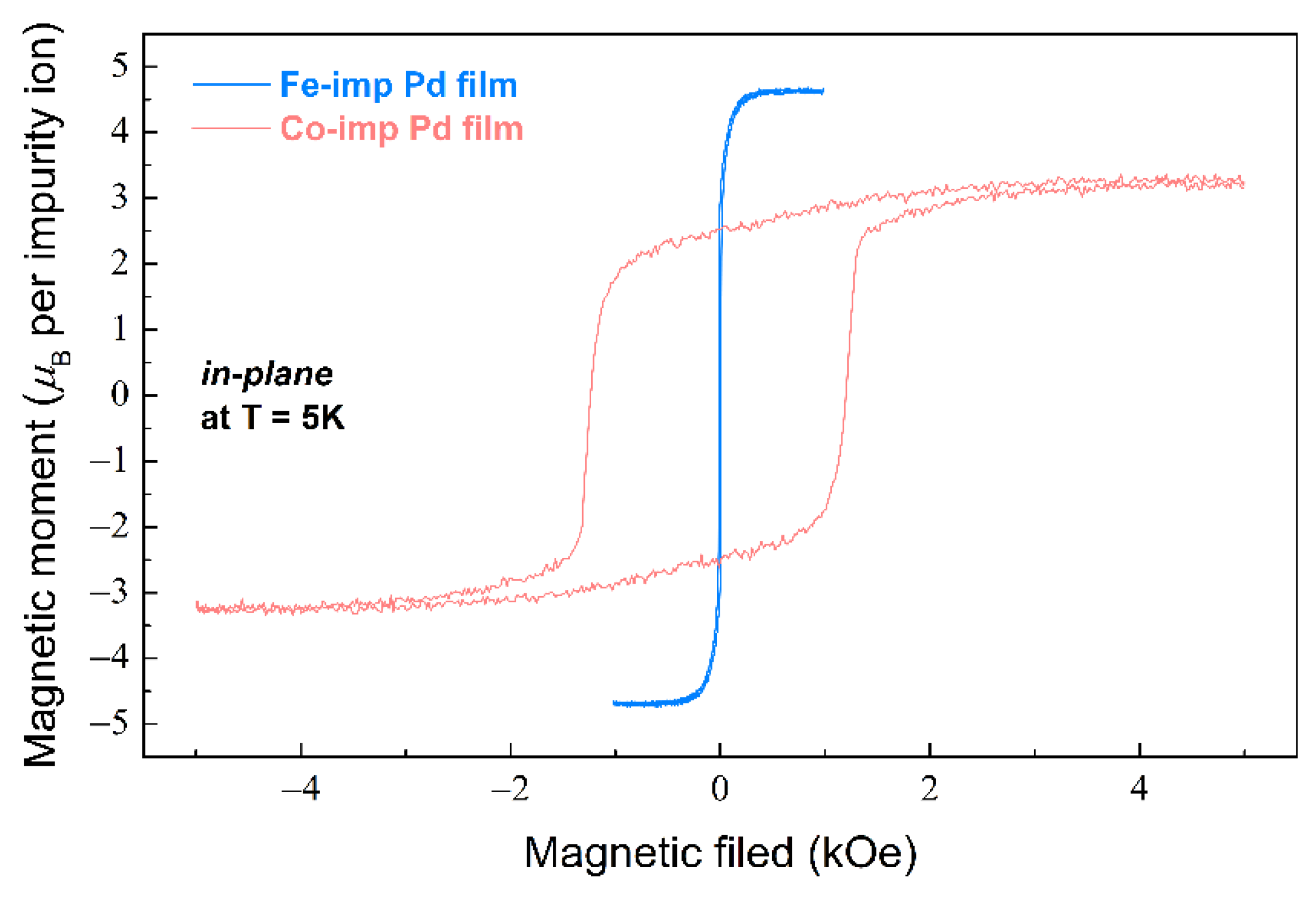
3.2. Magnetostatic Measurements
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Crangle, J. Ferromagnetism in Pd-rich palladium-iron alloys. Philos. Mag. 1960, 5, 335–342. [Google Scholar] [CrossRef]
- Nieuwenhuys, G.J. Magnetic behaviour of cobalt, iron and manganese dissolved in palladium. Adv. Phys. 1975, 24, 515–591. [Google Scholar] [CrossRef]
- Wohlfarth, E.P. Handbook of Magnetic Materials; North-Holland Publishing Company: London, UK, 1980; Volume 1, p. 75. [Google Scholar]
- Ryazanov, V.V. Josephson superconductor—Ferromagnet—Superconductor π-contact as an element of a quantum bit (experiment). Uspekhi Fiz. Nauk 1999, 42, 825–827. [Google Scholar] [CrossRef]
- Larkin, T.I.; Bol’ginov, V.V.; Stolyarov, V.S.; Ryazanov, V.V.; Vernik, I.V.; Tolpygo, S.K.; Mukhanov, O.A. Ferromagnetic Josephson switching device with high characteristic voltage. Appl. Phys. Lett. 2012, 100, 222601. [Google Scholar] [CrossRef] [Green Version]
- Ryazanov, V.V.; Bol’ginov, V.V.; Sobanin, D.S.; Vernik, I.V.; Tolpygo, S.K.; Kadin, A.M.; Mukhanov, O.A. Magnetic Josephson junction technology for digital and memory applications. Phys. Procedia 2012, 36, 35–41. [Google Scholar] [CrossRef] [Green Version]
- Vernik, I.V.; Bol’ginov, V.V.; Bakurskiy, S.V.; Golubov, A.A.; Kupriyanov, M.Y.; Ryazanov, V.V.; Mukhanov, O.A. Magnetic Josephson junctions with superconducting interlayer for cryogenic memory. IEEE Trans. Appl. Supercond. 2013, 23, 1701208. [Google Scholar] [CrossRef] [Green Version]
- Esmaeili, A.; Yanilkin, I.V.; Gumarov, A.I.; Vakhitov, I.R.; Yusupov, R.V.; Tatarsky, D.A.; Tagirov, L.R. Epitaxial thin-film Pd1−xFex alloy—A tunable ferromagnet for superconducting spintronics. Sci. China Mater. 2021, 64, 1246–1255. [Google Scholar] [CrossRef]
- Mohammed, W.M.; Yanilkin, I.V.; Gumarov, A.I.; Kiiamov, A.G.; Yusupov, R.V.; Tagirov, L.R. Epitaxial growth and superconducting properties of thin-film PdFe/VN and VN/PdFe bilayers on MgO(001) substrates. Beilstein J. Nanotechnol. 2020, 11, 807–813. [Google Scholar] [CrossRef]
- Yanilkin, I.V.; Mohammed, W.M.; Gumarov, A.I.; Kiiamov, A.G.; Yusupov, R.V.; Tagirov, L.R. Synthesis, characterization, and magnetoresistive properties of the epitaxial Pd0.96Fe0.04/VN/Pd0.92Fe0.08 superconducting spin-valve heterostructure. Nanomaterials 2021, 11, 64. [Google Scholar] [CrossRef] [PubMed]
- Gumarov, A.I.; Yanilkin, I.V.; Yusupov, R.V.; Kiiamov, A.G.; Tagirov, L.R.; Khaibullin, R.I. Iron-implanted epitaxial palladium thin films: Structure, ferromagnetism and signatures of spinodal decomposition. Mater. Lett. 2021, 305, 130783. [Google Scholar] [CrossRef]
- Gumarov, A.I.; Yanilkin, I.V.; Rodionov, A.A.; Gabbasov, B.F.; Yusupov, R.V.; Aliyev, M.N.; Khaibullin, R.I.; Tagirov, L.R. Manifestations of Spinodal Decomposition into Dilute Pd1-xFex “Phases” in Iron-Implanted Palladium Films: FMR Study. Appl. Magn. Reson. 2022, 53, 875–886. [Google Scholar] [CrossRef]
- Hohenberg, P.; Kohn, W. Inhomogeneous Electron Gas. Phys. Rev. 1964, 136, B864–B871. [Google Scholar] [CrossRef] [Green Version]
- Kohn, W.; Sham, L.J. Self-Consistent Equations Including Exchange and Correlation Effects. Phys. Rev. 1965, 140, A1133–A1138. [Google Scholar] [CrossRef] [Green Version]
- Kresse, G.; Furthmüller, J. Efficiency of ab-initio total energy calculations for metals and semiconductors using a plane-wave basis set. Compur. Mater. Sci. 1996, 6, 15–50. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169–11186. [Google Scholar] [CrossRef]
- Kresse, G.; Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 1999, 59, 1758–1775. [Google Scholar] [CrossRef]
- Materials Design, Inc. MedeA, version 3.4; MedeA is a Registered Trademark of Materials Design, Inc.: San Diego, CA, USA, 2012. [Google Scholar]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [Green Version]
- Blöchl, P.E. Projector augmented-wave method. Phys. Rev. B 1994, 50, 17953–17979. [Google Scholar] [CrossRef] [Green Version]
- Piyanzina, I.; Gumarov, A.; Khaibullin, R.; Tagirov, L. Ab initio Investigation of Impurity Ferromagnetism in the Pd1−xFex Alloys: Concentration and Position Dependences. Crystals 2021, 11, 1257. [Google Scholar] [CrossRef]
- Korenblit, I.Y.; Shender, E.F. Ferromagnetism of disordered systems. Sov. Phys. Uspekhi 1978, 21, 832–851. [Google Scholar] [CrossRef]
- Low, G.G. The electronic structure of some transition metal alloys. Adv. Phys. 1969, 18, 371–400. [Google Scholar] [CrossRef]
- Aldred, A.T.; Rainford, B.D.; Stringfellow, M.W. Magnetic Moment Distribution in Dilute Alloys of Nickel in Palladium. Phys. Rev. Lett. 1970, 24, 897–900. [Google Scholar] [CrossRef]
| Sample | Implanted Ion | Implantation Energy (keV) | Dose (×1016 ions/cm2) |
|---|---|---|---|
| FePd-1 | Fe | 40 | 0.5 |
| FePd-2 | 1.0 | ||
| FePd-3 | 3.0 | ||
| CoPd-1 | Co | 40 | 0.5 |
| CoPd-2 | 1.0 | ||
| CoPd-3 | 3.0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Korableva, A.; Piyanzina, I.; Gumarov, A.; Yanilkin, I.; Khaibullin, R. Impurity Ferromagnetism of Pd-Fe and Pd-Co Alloys: Ab Initio vs. Experiment. Mater. Proc. 2022, 9, 22. https://doi.org/10.3390/materproc2022009022
Korableva A, Piyanzina I, Gumarov A, Yanilkin I, Khaibullin R. Impurity Ferromagnetism of Pd-Fe and Pd-Co Alloys: Ab Initio vs. Experiment. Materials Proceedings. 2022; 9(1):22. https://doi.org/10.3390/materproc2022009022
Chicago/Turabian StyleKorableva, Alexandra, Irina Piyanzina, Amir Gumarov, Igor Yanilkin, and Rustam Khaibullin. 2022. "Impurity Ferromagnetism of Pd-Fe and Pd-Co Alloys: Ab Initio vs. Experiment" Materials Proceedings 9, no. 1: 22. https://doi.org/10.3390/materproc2022009022
APA StyleKorableva, A., Piyanzina, I., Gumarov, A., Yanilkin, I., & Khaibullin, R. (2022). Impurity Ferromagnetism of Pd-Fe and Pd-Co Alloys: Ab Initio vs. Experiment. Materials Proceedings, 9(1), 22. https://doi.org/10.3390/materproc2022009022








