Steady-State and Transient Electrical Properties of Liquid Crystal Cells ^†

Abstract

Rapidly expanding and new applications of liquid crystal materials cover a wide range of technology products. A very incomplete list includes conventional and miniature high-resolution displays, AR/VR glasses, smart windows, dynamic lenses, tunable filters and retarders, electrically controlled sensors, reconfigurable antennas for wireless and space communications, and many other commercially available devices. The aforementioned devices are enabled by the collective reorientation of thermotropic molecular liquid crystals under the action of applied electric fields. The reorientation effects in liquid crystals can be altered by ionic contaminants typically present in mesogenic materials in small quantities. Therefore, information about ions in liquid crystals is very important because it allows for a proper selection of liquid crystal materials and uncompromised performance of liquid crystal devices. This information can be obtained by performing electrical measurements of liquid crystal materials. Measurements of basic electrical parameters (DC conductivity, charge mobility, and ion density) are carried out using sandwich-like liquid crystal cells of finite thickness. Once a cell is filled with liquid crystal materials, interactions between ions and the cell substrates will result in the time dependence of the ion density and DC electrical conductivity until a steady state is reached. In this paper, we show how complementary information about ionic processes in liquid crystal cells can be obtained by analyzing their transient and steady-state electrical properties.

1. Introduction

Contemporary applications of thermotropic liquid crystals are rapidly expanding. In addition to conventional liquid crystal displays (LCDs) [1,2], liquid crystal technologies are very promising for the development of augmented reality (AR) and virtual reality (VR) systems [3,4,5]. Numerous photonic and biomedical devices (filters [6], retarders and spatial light modulators [7], lenses [8], electrically controlled sensors [9], diffractive optics [10], and beam-steering devices [11,12]) rely on tunable optical elements made of liquid crystals. An emerging field of flat optics [13] along with the reconfigurability of plasmonic [14] and meta-devices [15] can also benefit from the tunability of liquid crystal materials. This tunability of liquid crystals enabled a wide range of microwave devices such as resonators, antennas, and phase shifters [16,17]. It should be noted that classical applications of liquid crystals such as switchable smart windows [18,19] and light shutters [20,21,22] are also constantly growing and evolving [23].

The reorientation of liquid crystals under the action of applied electric fields results in the tunability of their physical properties and enables their numerous applications [7]. An electric-field-induced reorientation of liquid crystals can be altered by ions that are always present in thermotropic liquid crystals [24,25]. Ions in liquid crystals can compromise the performance of liquid crystal devices. A well-known screening effect in LCD can lead to image sticking, image flickering, a reduced voltage holding ratio, and an overall slow response [24,25]. Under certain conditions, ions in liquid crystals can give rise to electrohydrodynamic instabilities [26], resulting in strong light scattering and enabling applications such as light shutters and smart windows [18,19,20,21,22].

Regardless the type of application, information about ions in thermotropic liquid crystals is very important. This information can be obtained by performing electrical characterization of liquid crystal materials ([27,28,29], and references therein). The obtained experimental results can be used to evaluate DC electrical conductivity, ion mobility, and ion concentration in liquid crystals [30,31,32].

As a rule, electrical measurements of thermotropic liquid crystals are carried out using sandwich-like cells of a fixed thickness. At the same time, interactions between ions and substrates of a liquid crystal cell can result in dependence of the measured electrical conductivity on the cell thickness [32,33]. Even though the importance of considering interactions between ions and substrates of a liquid crystal cell for a proper interpretation of electrical measurements was emphasized many times ([28], and references therein), only a very limited number of experimental reports can be found [34,35,36]. The experimental results reported in [34,35,36] unambiguously point to the necessity to consider the dependence of the measured electrical conductivity of thermotropic liquid crystals on the cell thickness. A general analysis of such dependence performed recently shows a very rich behavior with the possibility of both monotonous and non-monotonous dependence of DC electrical conductivity on the cell thickness [33]. Both experiments [34,35,36] and modeling [33] assume a steady state. The consideration of time dependence of DC electrical conductivity can reveal transient ionic processes in liquid crystal cells caused by interactions between ions and substrates of the cell. This time dependence is analyzed in the present paper assuming liquid crystal cells of varying thickness.

2. Model

The DC electrical conductivity ${\lambda }_{DC}$ of molecular liquid crystals caused by ionic contaminants is given by Equation (1):

$${\lambda }_{DC}={\displaystyle \sum }_i{q}_i{\mu }_i{n}_i$$

(1)

where $n_i$ is the volume concentration of ions, ${\mu }_i$ is the mobility of the $i$-th ion, and $q_i$ is the charge of the $i$-th ion [24,25]. Assuming two types of fully dissociated symmetric monovalent ions ($q_i=\left|e\right|=1.6\times {10}^{-19}C \left(i=1,2\right)$, $n_1^{+}=n_1^{-}=n_1$, ${\mu }_1={\mu }_1^{+}+{\mu }_1^{-}$, $n_2^{+}=n_2^{-}=n_2$, ${\mu }_2={\mu }_2^{+}+{\mu }_2^{-}$), Equation (1) can be rewritten as Equation (2)

$${\lambda }_{DC}=\left|e\right|\left({\mu }_1{n}_1+{\mu }_2{n}_2\right)$$

(2)

Existing experimental and theoretical results [28,37,38,39,40,41] were used in the development of an elementary model [42,43] for the computation of the bulk concentration of ions in liquid crystal cells. The model considers two ionic processes in liquid crystal cells, namely the capturing of ions by substrates of the cell (the first term of the right-hand side of Equation (3)) and the ion-releasing process when substrates release trapped ions (the second term of the right-hand side of Equation (3)):

$$\frac{d{n}_i}{dt}=-k_{Si}^{a\pm }{n}_i\frac{{\sigma }_{Si}}{d}\left(1-{\mathsf{\Theta }}_{S1}^{\pm }-{\mathsf{\Theta }}_{S2}^{\pm }\right)+k_{Si}^{d\pm }\frac{{\sigma }_{Si}}{d}{\mathsf{\Theta }}_{Si}^{\pm }$$

(3)

Parameters $k_{Si}^{a\pm }$ and $k_{Si}^{d\pm }$ describe the time rate of ion-capturing and ion-releasing processes, respectively. Quantities ${\mathsf{\Theta }}_{Si}^{\pm }$ describe the fractional surface coverage of substrates by the i-th ions (i = 1, 2), ${\sigma }_{Si}$ stands for the surface density of all surface sites of the liquid crystal substrates, and $d$ is the cell thickness.

The conservation of the total number of ions of the i-th type is given by Equation (4):

$$n_{0i}+\frac{{\sigma }_{Si}}{d}{\nu }_{Si}=n_i+\frac{{\sigma }_{Si}}{d}{\mathsf{\Theta }}_{Si}^{\pm }$$

(4)

where ${\nu }_{Sj}$ is the contamination factor of substrates and $d$ is the cell thickness [42,43]. Additional details can be found in [33,42,43]. It should be noted that a perfect alignment of liquid crystal samples is assumed (either planar or homeotropic). To account for the anisotropy of the electrical conductivity of liquid crystals, different values of physical parameters, appropriate for a given alignment, can be used.

3. Results: DC Electrical Conductivity of Liquid Crystal Cells

The DC electrical conductivity of liquid crystal cells can be computed by solving Equations (2)–(4). The most common scenario involves a sandwich-like cell contaminated with ions of one type prior to filling it with liquid crystals that contain ions of another type. Once an empty cell is filled with liquid crystals, some fraction of ions already present in a liquid crystal bulk will be captured by the substrates of the liquid crystal cell. Some ions captured by the substrates can also be released back into the liquid crystal bulk. These ion-capturing/ion-releasing processes will continue until a steady state is reached when the number of ions per unit time captured by substrates is equal to the number of ions per unit time released by the substrates. At the same time, ionic contaminants already present on the substrates of the empty cell will enrich liquid crystals via the ion-releasing process. Some released ions will also be captured by the substrates. These ionic processes will also continue until a steady state is reached. In our recent paper [33], we modeled the DC conductivity of liquid crystal cells as a function of the cell thickness assuming a steady state. Because the present paper is a logical continuation of [33], we use similar physical parameters, as listed in

Table 1. Physical parameters and their values.

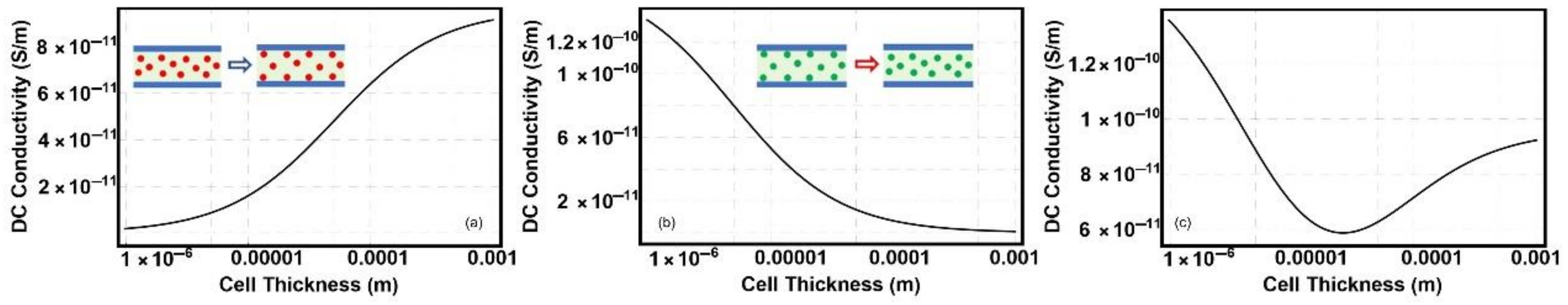
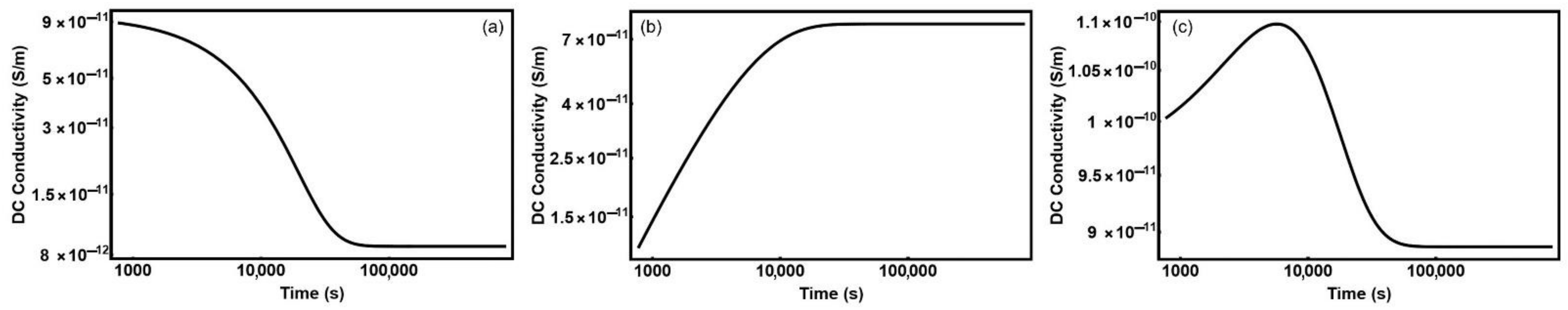
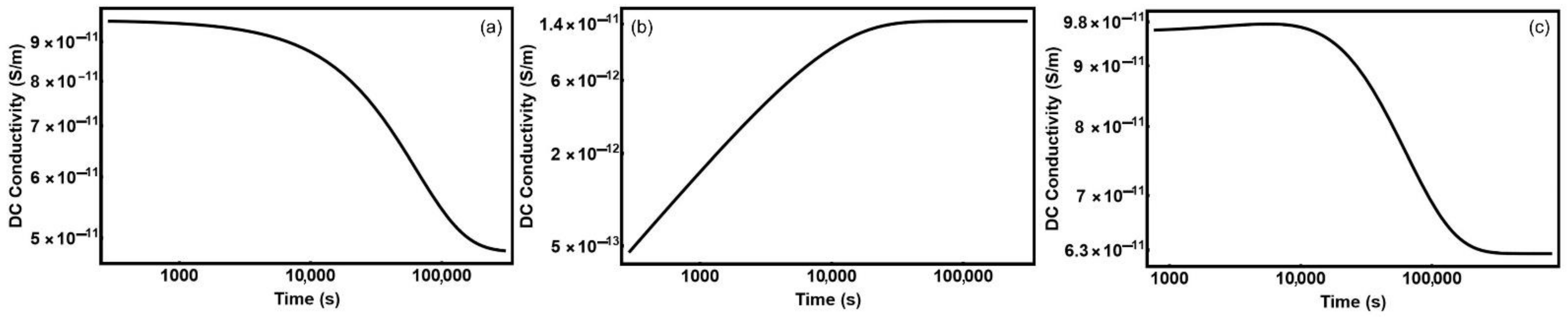
Physical Parameter	Value
$K_1=\frac{k_{S1}^a}{k_{S1}^d}$	10−21 m3 (Figure 1a,c, Figure 2a,c, Figure 3a,c and Figure 4a,c)
$k_{S1}^a$	10−26 m3/s (Figure 1a,c, Figure 2a,c, Figure 3a,c and Figure 4a,c)
$k_{S1}^d$	10−5 s−1 (Figure 1a,c, Figure 2a,c, Figure 3a,c and Figure 4a,c)
$K_2=\frac{k_{S2}^a}{k_{S2}^d}$	10−22 m3 (Figure 1b,c, Figure 2b,c, Figure 3b,c and Figure 4b,c)
$k_{S2}^a$	10−26 m3/s (Figure 1b,c, Figure 2b,c, Figure 3b,c and Figure 4b,c)
$k_{S2}^d$	10−4 s−1 (Figure 1b,c, Figure 2b,c, Figure 3b,c and Figure 4b,c)
${\sigma }_{S1}={\sigma }_{S2}$	5 × 1016 m−2 (Figure 1, Figure 2, Figure 3 and Figure 4)
${\mu }_1={\mu }_2$	10−10 m2/Vs (Figure 1, Figure 2, Figure 3 and Figure 4)
${\nu }_{S1}$	0 (Figure 1a,c, Figure 2a,c, Figure 3a,c and Figure 4a,c)
${\nu }_{S2}$	10−3 (Figure 1b,c, Figure 2b,c, Figure 3b,c and Figure 4b,c)
$n_{01}$	6 × 1018 m−3 (Figure 1a,c, Figure 2a,c, Figure 3a,c and Figure 4a,c)
$n_{02}$	0 (Figure 1b,c, Figure 2b,c, Figure 3b,c and Figure 4b,c)

.

We have already analyzed a steady-state solution in our previous paper [33]. Given a great variety of possible outcomes (the steady-state DC conductivity can depend on the cell thickness in monotonous (either increase or decrease) and non-monotonous (with maximum or minimum) ways), let us consider a non-trivial case of non-monotonous dependence of DC electrical conductivity on the cell thickness shown in Figure 1. According to Figure 1, a fraction of ions already present in liquid crystals prior to filling an empty cell are captured by the substrates (Figure 1a). At the same time, because substrates of the cell were contaminated with other ions, once the cell is filled with liquid crystals, these ions can be released into the liquid crystal bulk, as shown in Figure 1b. The combination of these ionic processes results in a non-monotonous dependence of steady-state DC electrical conductivity on the cell thickness (Figure 1c).

It is interesting to explore the time dependence of the ion-capturing and ion-releasing processes shown in Figure 1. This time dependence can be obtained by solving Equations (2)–(4). The results are shown in Figure 2, Figure 3 and Figure 4.

According to Figure 2, Figure 3 and Figure 4, an interplay between ion-capturing (Figure 2a, Figure 3a and Figure 4a) and ion-releasing (Figure 2b, Figure 3b and Figure 4b) regimes can result in a non-monotonous time dependence of DC conductivity of liquid crystal cells (Figure 2c, Figure 3c and Figure 4c). Interestingly, this time dependence strongly depends on the cell thickness. A rather weak maximum (Figure 2c) for a 1 µm thick cell becomes much stronger for a 5 µm thick cell (Figure 3c), and nearly disappears for a 50 µm thick cell (Figure 4c).

4. Conclusions

Interactions between ions and substrates of a liquid crystal cell can alter DC electrical conductivity in a very non-trivial way. As a result, DC conductivity of liquid crystal cells depends on the cell thickness. The type of this dependence can reveal important information about ions inherently present in liquid crystals and ions brought to liquid crystals by contaminated substrates. Once an empty cell is filled with liquid crystals, the combination of ion-capturing and ion-releasing processes results in time dependence of DC electrical conductivity. This time dependence is a function of the cell thickness (Figure 2, Figure 3 and Figure 4). By analyzing the time dependence of DC electrical conductivity, complementary information about ionic processes in liquid crystal cells can be obtained. Figure 1, Figure 2, Figure 3 and Figure 4 indicate that measuring ions in liquid crystals is a very non-trivial activity. Electrical conductivity of liquid crystals should be measured as a function of the cell thickness and as a function of time.