Enhancing Onshore Wind Tower Foundations: A Comprehensive Automated Design Approach
Abstract
1. Introduction and Literature Survey
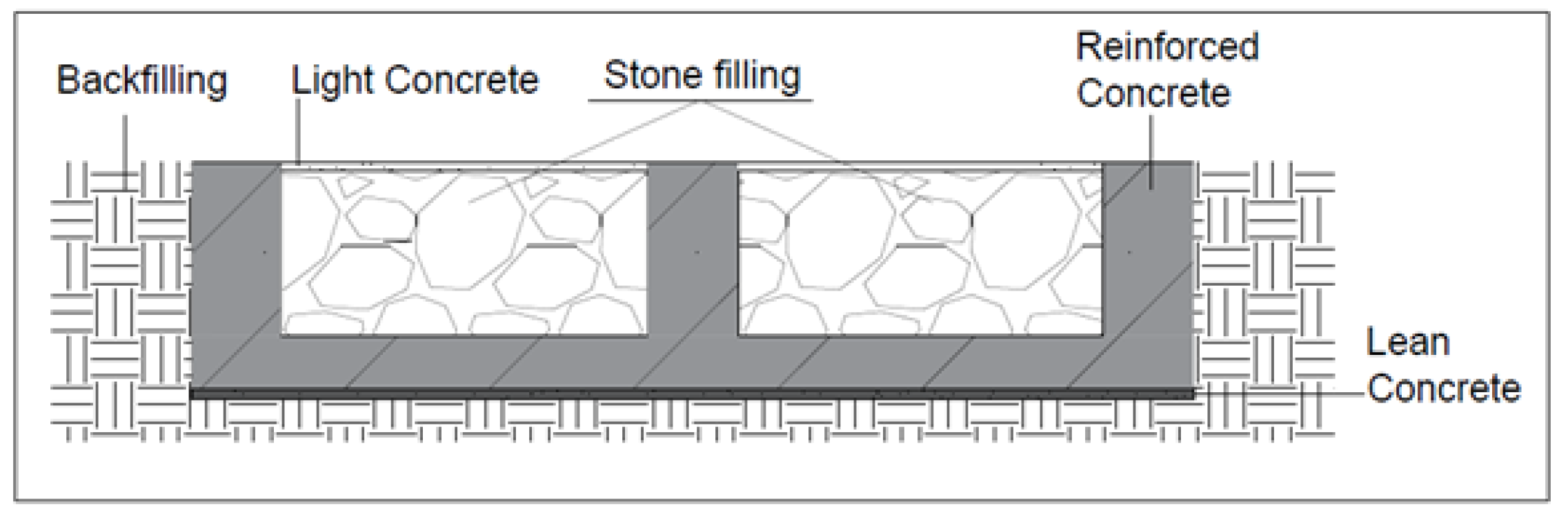
2. The Proposed Design of the Foundation System
3. Development of Proposed Automated Design Approach
3.1. Data Required by the Proposed Design Approach and Setting the Automated Design Problem
3.2. Variables of the Proposed Design
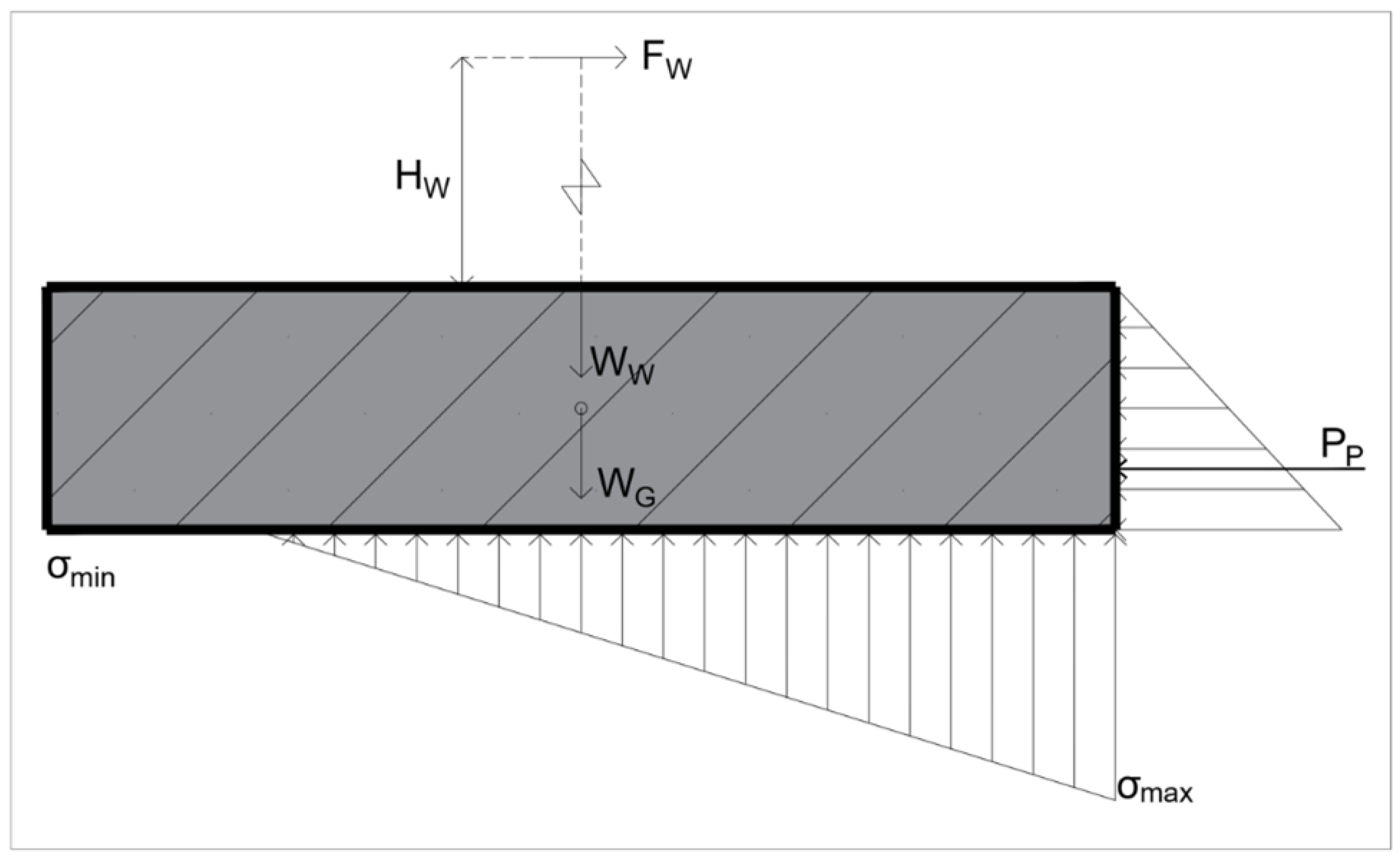
3.3. Analysis of the Forces Applied on the System Wind Turbine—Foundation and Check Analysis
3.4. Design Objective—Criterion of the Proposed Design
4. Design Checks
4.1. Stability Forces along Global y Axis
4.2. Overturn Forces at y Axis
4.3. Flowchart of the Automated Design Process
5. Computational Analysis and Design Evaluation—The Case Study
6. Results and Discussion
6.1. Analysis of the Numerical Investigation for the Wind Turbine Examined (V52-850 kW)
6.2. Impact Analysis of the Proposed Design Approach in Wind Farm Industry in Europe and Worldwide
6.3. Future Trends: Integrating Steel 3D Printing Technology
6.3.1. Advancements in Steel 3D Printing
6.3.2. Benefits for Wind Tower Foundations
6.4. Implementation Challenges
6.5. Comparison with Existing Design Approaches
7. Conclusions
- A pivotal aspect that demands meticulous attention during the design process is the critical check against overturn. Regardless of the fulfillment of other design criteria, the design process cannot be deemed complete unless the overturn check is met. As a result, this check takes precedence, positioned as the primary consideration, with all subsequent checks conducted in a secondary phase. In essence, the entire design process hinges on the successful fulfillment of the overturn check, highlighting its paramount importance in the overall design procedure.
- The most effective configuration for the wind tower foundation in order to undertake the moment applied on the foundation by the wind force applied on the wind turbine is a rectangular layout featuring beams extending in both directions. This optimal shape involves strategically filling voids within the foundation structure with soil material that contribute significantly to meeting the crucial criteria for overturn stability. In incorporating beams in both directions, the foundation gains structural robustness, while filling voids enhances overall stability. This design not only ensures an optimal foundation shape but also plays a pivotal role in satisfying the critical checks against overturn, thereby reinforcing the structural integrity of the wind turbine foundation.
- Upon delineating the foundation as a unified structural system with designated dimensions, the surplus quantity of concrete is determined through an assessment of its resistance against shear forces. Subsequently, a significant reduction of up to 31% is applied to the final concrete quantity specifically at the beam foundation, compared to a full rectangular foundation. This meticulous process not only optimizes the overall material usage but also ensures that the concrete composition aligns seamlessly with the foundation’s structural requirements. The reduction in concrete quantity at the beam foundation represents a targeted and calculated approach to streamline resources while maintaining the foundation’s integrity against shear forces.
- The steel reinforcement is calculated for the beams derived, and as a result, the minimum steel requirements are now implemented on beams and not on the whole foundation. The steel quantity is reduced by 90% at the beam foundation compared to the foundation without beams.
- The cost for the wind tower foundation is calculated for the single foundation and for the beam foundation, taking into consideration the excavation, steel installation, concrete pouring, back filling, compressing, and light concrete cover, and it was derived that the final cost is reduced by 70% and 69% in the cases of rock ground and soft soil, respectively. As far as the construction time is concerned, it will be reduced due to the fact that less reinforcement needs to be installed and less concrete needs to be poured.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| TW | Tera Watt; |
| MW | Mega Watt; |
| GW | Giga Watt; |
| kW | kilo Watt; |
| MU | Money Unit. |
| List of Symbols | |
| Symbol | Description |
| f | Soil internal angle |
| Special weight of the soil | |
| c | Cohesion factor of the soil |
| Maximum soil allowable stress | |
| Minimum soil allowable stress | |
| Wind velocity | |
| Total cost of the wind turbine foundation | |
| Cost of the excavation of the wind turbine foundation | |
| Cost for concrete pouring for the wind turbine foundation | |
| Cost for steel reinforcement of wind turbine foundation | |
| Cost for backfilling of wind turbine foundation | |
| Cost for recycling of excavation material | |
| Wind turbine foundation self-weight | |
| B | Wind turbine width |
| L | Wind turbine length |
| H | Wind turbine height |
| Concrete special weight | |
| Soil passive forces | |
| Horizontal stress applied on the lateral limit of the foundation | |
| Impel coefficient | |
| Vertical stress applied on the lateral limit of the foundation | |
| Special weight of the soil | |
| Total stability moment at the most distanced point of the foundation | |
| Self-weight of the wind turbine | |
| Wind force in the y axis | |
| Size factor | |
| Dynamic factor | |
| Force coefficient | |
| Peak speed pressure at reference height ze | |
| Reference surface | |
| Wind force at x axis | |
| Wind force at the wings in the x axis | |
| Wind force at the tower in the x axis | |
| Wind force at the tower | |
| Total overturn moment at the most distanced point of the foundation in the y axis | |
| Height of the wind turbine | |
| Total stability moment at the most distanced point of the foundation in the x axis | |
| Active moment in the y axis | |
| Strength moment at the foundation | |
| Foundation section area | |
| Concrete strength | |
| Steel reinforcement in the y direction | |
| Steel strength | |
| z | Internal forces lever arm |
| Minimum allowable steel reinforcement | |
| Maximum allowable steel reinforcement | |
| Vertical designed action on the foundation that causes perforation | |
| Vertical action on the foundation that causes perforation | |
| Strength of the foundation against perforation | |
| k | Coefficient depending on static height d |
| Designed concrete strength | |
| d | Static height |
| a | Check distance |
| Shear load on the foundation | |
| Shear strength | |
| Total axial force | |
| A | Area of the applied axial forces |
| t | Soil height |
| Final moment | |
| W | Resistance moment |
| Load applied on the slab beams | |
| Effective width of the slab beam | |
| Reinforcement percentage | |
| Cost for filling the void of the beam foundation with stones | |
| Concrete cost for covering the stones with light concrete | |
References
- Wind Industry Statement on Economic Recovery from COVID-19—Global Wind Energy Council—Gwec.Net. Available online: https://gwec.net/wind-industry-statement-on-economic-recovery-from-covid-19/ (accessed on 27 June 2024).
- Bošnjaković, M.; Katinić, M.; Santa, R.; Marić, D. Wind Turbine Technology Trends. Appl. Sci. 2022, 12, 8653. [Google Scholar] [CrossRef]
- Abdelmoteleb, S.E.; Mendoza, A.S.E.; dos Santos, C.R.; Bachynski-Polić, E.E.; Griffith, D.T.; Oggiano, L. Preliminary Sizing and Optimization of Semisubmersible Substructures for Future Generation Offshore Wind Turbines. J. Phys. Conf. Ser. 2022, 2362, 012001. [Google Scholar] [CrossRef]
- Asim, T.; Islam, S.Z.; Hemmati, A.; Khalid, M.S.U. A Review of Recent Advancements in Offshore Wind Turbine Technology. Energies 2022, 15, 579. [Google Scholar] [CrossRef]
- Al-Sanad, S.; Parol, J.; Wang, L.; Kolios, A. Design optimisation of wind turbine towers with reliability-based calibration of partial safety factors. Energy Rep. 2023, 9, 2548–2556. [Google Scholar] [CrossRef]
- Lagaros, N.D.; Karlaftis, M.G. Life-cycle cost structural design optimization of steel wind towers. Comput. Struct. 2016, 174, 122–132. [Google Scholar] [CrossRef]
- De Anda, J.; Ruiz, S.E.; Bojórquez, E.; Inzunza-Aragon, I. Towards optimal reliability-based design of wind turbines towers using artificial intelligence. Eng. Struct. 2023, 294, 116778. [Google Scholar] [CrossRef]
- Lehmann, P.; Reutter, F.; Tafarte, P. Optimal siting of onshore wind turbines: Local disamenities matter. Resour. Energy Econ. 2023, 74, 101386. [Google Scholar] [CrossRef]
- Tafarte, P.; Lehmann, P. Quantifying trade-offs for the spatial allocation of onshore wind generation capacity—A case study for Germany. Ecol. Econ. 2023, 209, 107812. [Google Scholar] [CrossRef]
- Sun, Y.; Fan, Y.; Lu, J.; Hou, J.; Wang, Y.; Bai, X. Optimal Design Method for Topology of Onshore Wind Power DC Collection System. Dianli Xitong Zidonghua/Automation Electr. Power Syst. 2023, 47, 142–152. [Google Scholar] [CrossRef]
- Kallioras, N.A.; Lagaros, N.D.; Karlaftis, M.G.; Pachy, P. Optimum layout design of onshore wind farms considering stochastic loading. Adv. Eng. Softw. 2015, 88, 8–20. [Google Scholar] [CrossRef]
- Srikakulapu, R.; U, V. Optimal design of collector topology for offshore wind farm based on ant colony optimization approach. In Proceedings of the 2016 IEEE International Conference on Power Electronics, Drives and Energy Systems (PEDES), Trivandrum, India, 14–17 December 2016; pp. 1–6. [Google Scholar] [CrossRef]
- Chen, X.; Xu, J.Z. Structural failure analysis of wind turbines impacted by super typhoon Usagi. Eng. Fail. Anal. 2016, 60, 391–404. [Google Scholar] [CrossRef]
- Lavassas, I.; Nikolaidis, G.; Zervas, P.; Efthimiou, E.; Doudoumis, I.; Baniotopoulos, C. Analysis and design of the prototype of a steel 1-MW wind turbine tower. Eng. Struct. 2003, 25, 1097–1106. [Google Scholar] [CrossRef]
- Matos, R.P.; Pinto, P.L.; Rebelo, C.S.; Gervásio, H.S.; Veljkovic, M. Improved design of tubular wind tower foundations using steel micropiles. Struct. Infrastruct. Eng. 2016, 12, 1038–1050. [Google Scholar] [CrossRef]
- Byron, M.; Denis, K.; Charles, W.C. Loading and dynamic response considerations for the design of wind turbine foundations on South African soils. Geotech. Eng. 2017, 48, 110–117. [Google Scholar]
- IEA. Executive Summary—Renewables 2023—Analysis and Forecast to 2028. International Energy Agency (IEA). 2023. Available online: https://www.iea.org/reports/renewables-2023/executive-summary (accessed on 3 August 2024).
- Lago, B.D.; Flessati, L.; Marveggio, P.; Martinelli, P.; Fraraccio, G.; di Prisco, C.; di Prisco, M. Experimental tests on shallow foundations of onshore wind turbine towers. Struct. Concr. 2022, 23, 2986–3006. [Google Scholar] [CrossRef]
- Satymov, R.; Bogdanov, D.; Breyer, C. Global-local analysis of cost-optimal onshore wind turbine configurations considering wind classes and hub heights. Energy 2022, 256, 124629. [Google Scholar] [CrossRef]
- Shen, Q.; Vahdatikhaki, F.; Voordijk, H.; van der Gucht, J.; van der Meer, L. Metamodel-based generative design of wind turbine foundations. Autom. Constr. 2022, 138, 104233. [Google Scholar] [CrossRef]
- Tian, Y.; Liu, Z.; Xu, X.; Wang, G.; Li, Q.; Zhou, Y.; Cheng, J. Systematic review of research relating to heavy-duty machine tool foundation systems. Adv. Mech. Eng. 2019, 11, 1687814018806106. [Google Scholar] [CrossRef]
- Wu, X.; Hu, W.; Huang, Q.; Chen, C.; Chen, Z.; Blaabjerg, F. Optimized Placement of Onshore Wind Farms Considering Topography. Energies 2019, 12, 2944. [Google Scholar] [CrossRef]
- Gao, Y.; Ma, S.; Wang, T.; Miao, C.; Yang, F. Distributed onshore wind farm siting using intelligent optimization algorithm based on spatial and temporal variability of wind energy. Energy 2022, 258, 124816. [Google Scholar] [CrossRef]
- Loubser, P.; Jacobs, A. Optimised Design of Wind Turbine Gravity Foundations; CRC Press: Boca Raton, FL, USA, 2016; pp. 953–958. [Google Scholar] [CrossRef]
- Gravett, D.Z.; Markou, G. State-of-the-art investigation of wind turbine structures founded on soft clay by considering the soil-foundation-structure interaction phenomenon—Optimization of battered RC piles. Eng. Struct. 2021, 235, 112013. [Google Scholar] [CrossRef]
- AlHamaydeh, M.; Hussain, S. Optimized frequency-based foundation design for wind turbine towers utilizing soil–structure interaction. J. Frankl. Inst. 2011, 348, 1470–1487. [Google Scholar] [CrossRef]
- Tayeh, A.I. Critical review of three selected papers on the design of wind turbine foundations. Int. J. Adv. Eng. Res. Sci. 2019, 6, 21–24. [Google Scholar] [CrossRef][Green Version]
- Gulvanessian, H.; Formichi, P.; Calgaro, J.A.; Harding, G.J. Designers’ Guide to Eurocode 1: Actions on Buildings; Designers’ Guide to Eurocodes; Thomas Telford: London, UK, 2009. [Google Scholar]
- Bungey, J.H.; Mosley, W.H.; Hulse, R. Reinforced Concrete Design to Eurocode 2; Palgrave Macmillan: Basingstoke, UK, 1996. [Google Scholar]
- Investment Costs—wind-energy-the-facts.org. Available online: https://www.wind-energy-the-facts.org/index-43.html (accessed on 27 June 2024).
- Simoncelli, M.; Zucca, M.; Ghilardi, M. Structural health monitoring of an onshore steel wind turbine. J. Civ. Struct. Health Monit. 2024, 14, 1423–1437. [Google Scholar] [CrossRef]


| Wind Turbine | Soil Condition | Wind Conditions |
|---|---|---|
| Total height | Soil internal angle (f), | Wind velocity () |
| Tower height | Special weight of the soil () | |
| Tower diameter at the base | Cohesion factor (c) | |
| Tower diameter reduction | Maximum allowable soil stress () | |
| Wings diameter | Minimum allowable soil stress () |
| Type of Design Check | Importance |
|---|---|
| Overturn resistance | High |
| Bending resistance | Normal |
| Shear resistance | Normal |
| Perforation | Normal |
| Soil stress limits | Normal |
| Wind Tubrine Characteristcs | Value |
|---|---|
| Wind turbine height (Hw) (m) | 86 |
| Wings diameter (Dw) (m) | 52 |
| Wind turbine self-weight without the foundation (Ww) (kN) | 1391.6 |
| Soil Characteristics | Value |
|---|---|
| f (raD) | 28 |
| (kN/m3) | 22 |
| c (kN/m3) | 120 |
| Description | Unit Costs (MU) | Quantity | Cost: Rock (MU) | Cost: Soft Soil (MU) |
|---|---|---|---|---|
| Excavation/m3 (rock) | 18 | 357 | 6.435 | |
| Excavation/m3 (soft) | 13 | 357 | ||
| Concrete price/m3 (rock) | 80 | 357 | 28,600 | |
| Concrete price/m3 (soft) | 100 | 357 | 35,750 | |
| Steel price/kg (kg) | 1.50 | 70,000 | 105,000 | 105,000 |
| Recycling cost/m3 (rock) | 8 | 357 | 2900 | |
| Recycling cost/m3 (soft) (rock) | 5 | 357 | ||
| Total Cost (soft) (rock) | 143,000 | 147,200 |
| Description | Unit Costs (MU) | Quantity | Cost: Rock (MU) | Cost: Soft Soil (MU) |
|---|---|---|---|---|
| Excavation/m3 (rock) | 18 | 357 | 6.35 | |
| Excavation/m3 (soft) | 13 | 357 | 4650 | |
| Concrete price/m3 (rock/soft) | 100 | 245 | 24,500 | 24,500 |
| Steel price/kg (kg) | 1.50 | 5300 | 7900 | 7900 |
| Stone filling/m3 (rock) | 0.003 | 618,550 | 1850 | |
| Stone filling/m3 (soft) | 0.010 | 618,550 | ||
| C12/15 procurement and pouring (m3) | 67 | 25 | 1650 | 1650 |
| Total Cost | 42,300 | 44,900 |
| Single Foundation | Soft Soil | Rock (the Coefficients Are the Percentage of the Cost for Soft Soil) | Status |
|---|---|---|---|
| Excavation Cost | a | ↑ | |
| Concrete Framework, Procurement, and Installation Cost | b | ↓ | |
| Steel Procurement and Installation Cost | c | c | - |
| Recycling of Backfilling Material Cost | d | ↑ |
| Related Cost | Single Foundation | Beam Foundation | Status (Increase, Reduction) |
|---|---|---|---|
| Excavation | a1 | a1 | - |
| Concrete Framework, Procurement, and Installation | b1 | ↓ | |
| Steel Procurement and Installation | c1 | ↓ |
| Related Cost | Single Foundation | Beam Foundation | Status (Increase, Reduction) |
|---|---|---|---|
| Excavation | a2 | a2 | - |
| Concrete Framework, Procurement, and Installation | b2 | ↓ | |
| Steel Procurement and Installation | c2 | ↓ |
| Cost Center | Contribution (Percentage) |
|---|---|
| Turbine | 68–84 |
| Foundation | 1–9 |
| Grid Connection | 2–10 |
| Control Systems | 1–2 |
| Consultancy | 1–3 |
| Land | 1–5 |
| Financial Costs | 1–5 |
| Road | 1–5 |
| Total | 100 |
| Country | Number of Turbines | Total Power (kW) | Cost/Kw (MU) | Total Cost of the Wind Farm (MU) | Foundation Cost Share (7%) (MU) | Saving (69%) (MU) |
|---|---|---|---|---|---|---|
| Belgium | 8 | 16,000 | 1250 | 20,000,000 | 1,400,000 | 966,000 |
| Belgium | 6 | 9000 | 1250 | 11,250,000 | 787,500 | 543,375 |
| Belgium | 1 | 2350 | 1250 | 2,937,500 | 205,625 | 141,881 |
| Belgium | 6 | 9000 | 1250 | 11,250,000 | 787,500 | 543,375 |
| Germany | 1 | 600 | 1300 | 780,000 | 54,600 | 37,674 |
| France | 8 | 5280 | 1200 | 6,336,000 | 443,520 | 306,029 |
| France | 6 | 9000 | 1200 | 10,800,000 | 756,000 | 521,640 |
| France | 5 | 3000 | 1200 | 3,600,000 | 252,000 | 173,880 |
| France | 13 | 9750 | 1200 | 11,700,000 | 819,000 | 565,110 |
| France | 5 | 11,500 | 1200 | 13,800,000 | 966,000 | 666,540 |
| France | 4 | 3000 | 1200 | 3,600,000 | 252,000 | 173,880 |
| France | 10 | 8000 | 1200 | 9,600,000 | 672,000 | 463,680 |
| France | 5 | 7500 | 1200 | 9,000,000 | 630,000 | 434,700 |
| France | 1 | 1500 | 1200 | 1,800,000 | 126,000 | 86,940 |
| France | 12 | 10,200 | 1200 | 12,240,000 | 856,800 | 591,192 |
| France | 2 | 4000 | 1200 | 4,800,000 | 336,000 | 231,840 |
| France | 6 | 9000 | 1200 | 10,800,000 | 756,000 | 521,640 |
| France | 5 | 12,000 | 1200 | 14,400,000 | 1,008,000 | 695,520 |
| France | 2 | 5000 | 1200 | 6,000,000 | 420,000 | 289,800 |
| Total | 164,693,500 | 11,528,545 | 7,954,696 |
| Continent | Country | Number of Turbines | Total Power (kW) | Cost/kW (MU) | Total Cost of the Wind Farm (MU) | Foundation Cost Share (7%) (MU) | Saving (69%) (MU) |
|---|---|---|---|---|---|---|---|
| Oceania | Australia | 20 | 12,000 | 1300 | 15,600,000 | 1,092,000 | 753,480 |
| Oceania | Australia | 20 | 12,000 | 1300 | 15,600,000 | 1,092,000 | 753,480 |
| Europe | Belgium | 8 | 16,000 | 1200 | 19,200,000 | 1,344,000 | 927,360 |
| Europe | Belgium | 6 | 9000 | 1200 | 10,800,000 | 756,000 | 521,640 |
| Europe | Belgium | 1 | 2350 | 1200 | 2,820,000 | 197,400 | 136,206 |
| Europe | Belgium | 6 | 9000 | 1200 | 10,800,000 | 756,000 | 521,640 |
| North America | Canada | 45 | 67,500 | 1350 | 91,125,000 | 6,378,750 | 4,401,338 |
| North America | Canada | 73 | 109,500 | 1350 | 147,825,000 | 10,347,750 | 7,139,948 |
| Europe | France | 8 | 5280 | 1250 | 6,600,000 | 462,000 | 318,780 |
| Europe | France | 6 | 9000 | 1250 | 11,250,000 | 787,500 | 543,375 |
| Europe | France | 5 | 3000 | 1250 | 3,750,000 | 262,500 | 181,125 |
| Europe | France | 5 | 11,500 | 1250 | 14,375,000 | 1,006,250 | 694,313 |
| Europe | France | 10 | 8000 | 1250 | 10,000,000 | 700,000 | 483,000 |
| Europe | France | 7 | 10,500 | 1250 | 13,125,000 | 918,750 | 633,938 |
| Europe | France | 5 | 7500 | 1250 | 9,375,000 | 656,250 | 452,813 |
| Europe | France | 12 | 10,200 | 1250 | 12,750,000 | 892,500 | 615,825 |
| Europe | France | 2 | 4000 | 1250 | 5,000,000 | 350,000 | 241,500 |
| Europe | France | 6 | 9000 | 1250 | 11,250,000 | 787,500 | 543,375 |
| Europe | France | 6 | 9000 | 1250 | 11,250,000 | 787,500 | 543,375 |
| Europe | France | 5 | 12,000 | 1250 | 15,000,000 | 1,050,000 | 724,500 |
| Europe | France | 2 | 5000 | 1250 | 6,250,000 | 437,500 | 301,875 |
| Total | 428,145,000 | 29,970,150 | 20,679,404 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vougioukas, E.; Stamos, A.; Pappa, C.; Lagaros, N.D. Enhancing Onshore Wind Tower Foundations: A Comprehensive Automated Design Approach. CivilEng 2024, 5, 736-759. https://doi.org/10.3390/civileng5030039
Vougioukas E, Stamos A, Pappa C, Lagaros ND. Enhancing Onshore Wind Tower Foundations: A Comprehensive Automated Design Approach. CivilEng. 2024; 5(3):736-759. https://doi.org/10.3390/civileng5030039
Chicago/Turabian StyleVougioukas, Emmanouil, Athanasios Stamos, Charikleia Pappa, and Nikos D. Lagaros. 2024. "Enhancing Onshore Wind Tower Foundations: A Comprehensive Automated Design Approach" CivilEng 5, no. 3: 736-759. https://doi.org/10.3390/civileng5030039
APA StyleVougioukas, E., Stamos, A., Pappa, C., & Lagaros, N. D. (2024). Enhancing Onshore Wind Tower Foundations: A Comprehensive Automated Design Approach. CivilEng, 5(3), 736-759. https://doi.org/10.3390/civileng5030039








