Finite Element In-Depth Verification: Base Displacements of a Spherical Dome Loaded by Edge Forces and Moments
Abstract
:1. Introduction
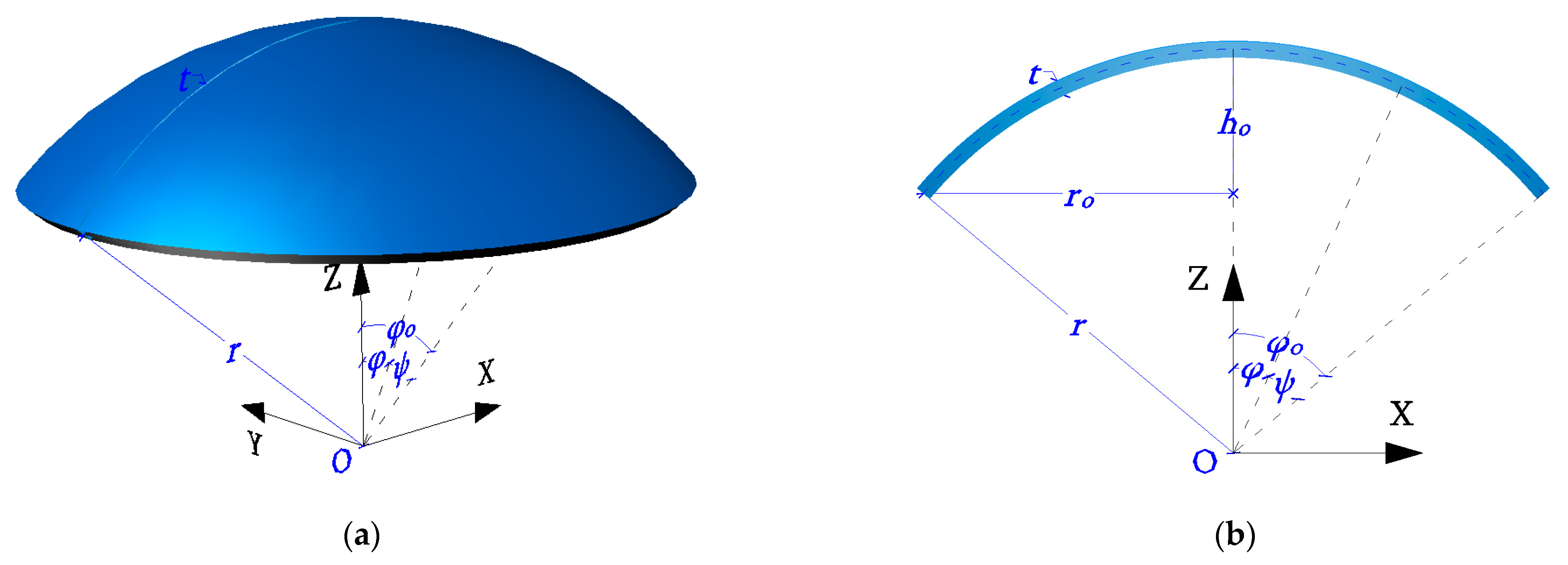
2. Theoretical Background
3. Finite Element Approach
3.1. Finite Element Modelling
3.1.1. Finite Element Type
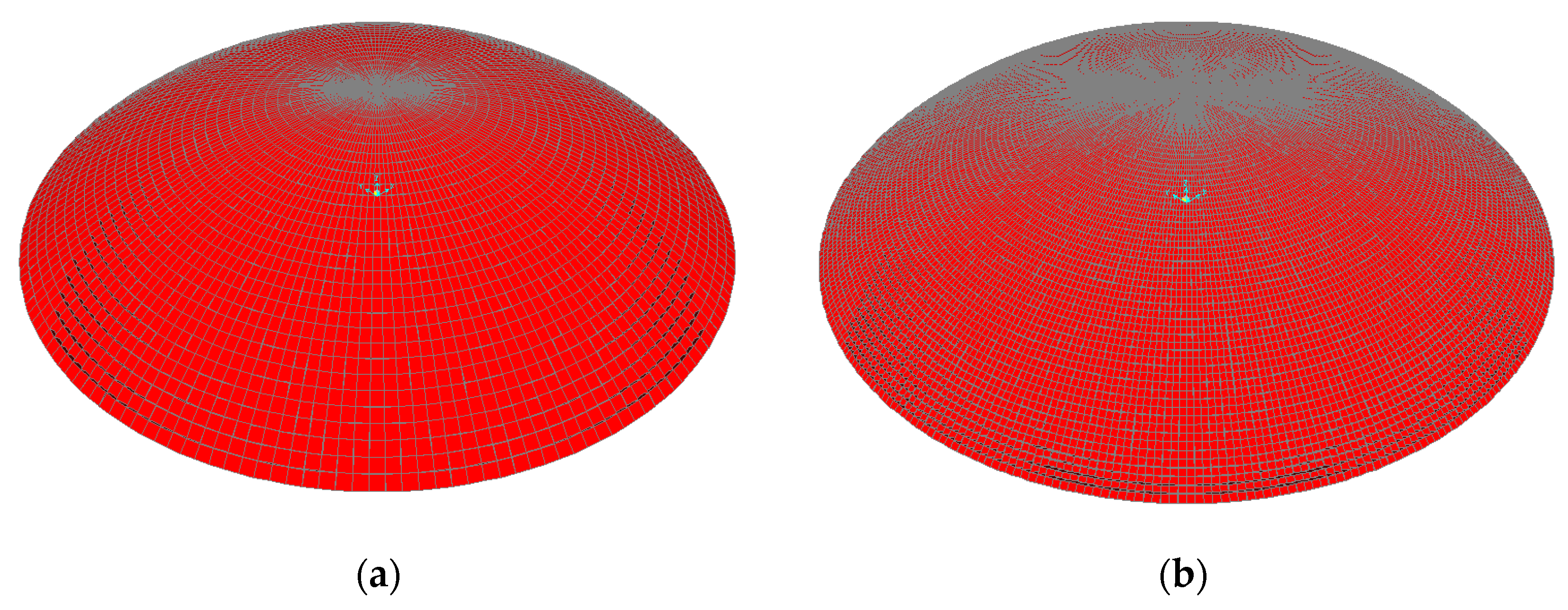
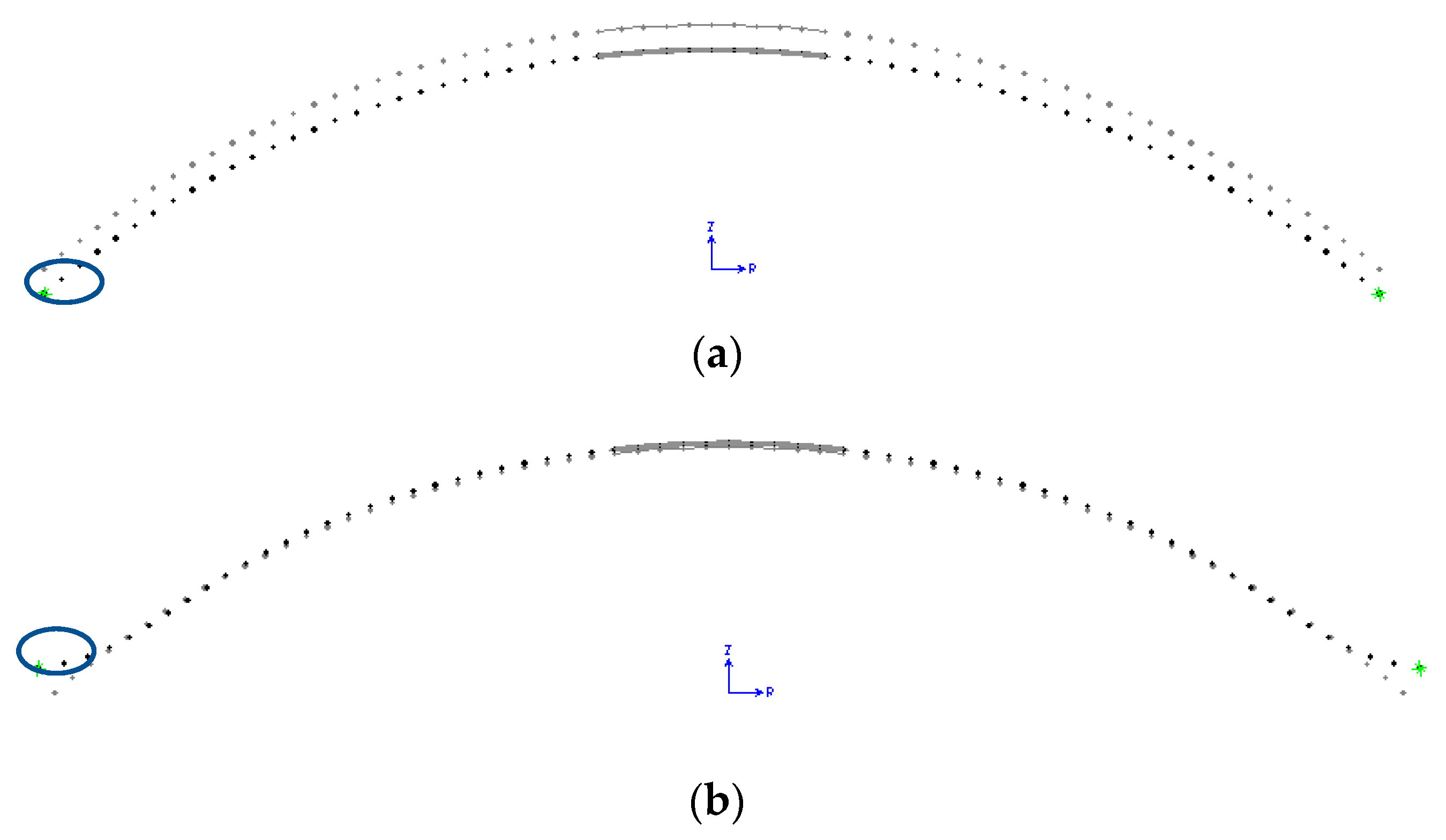
3.1.2. Model Discretization
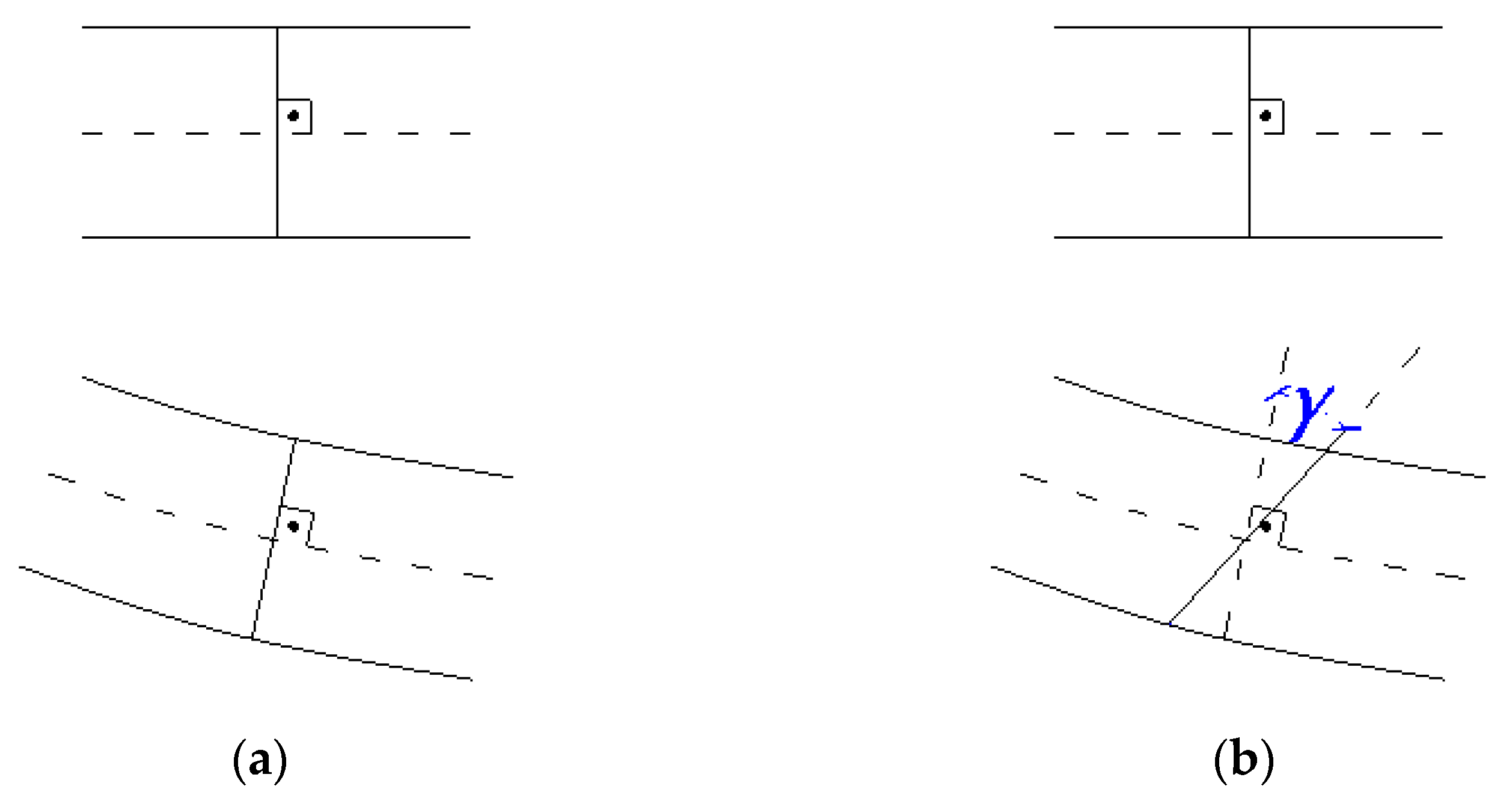
3.1.3. Orientation of Joint Local Coordinate System
3.1.4. Loading Definition
3.1.5. Boundary Conditions
3.1.6. Results’ Interpretation
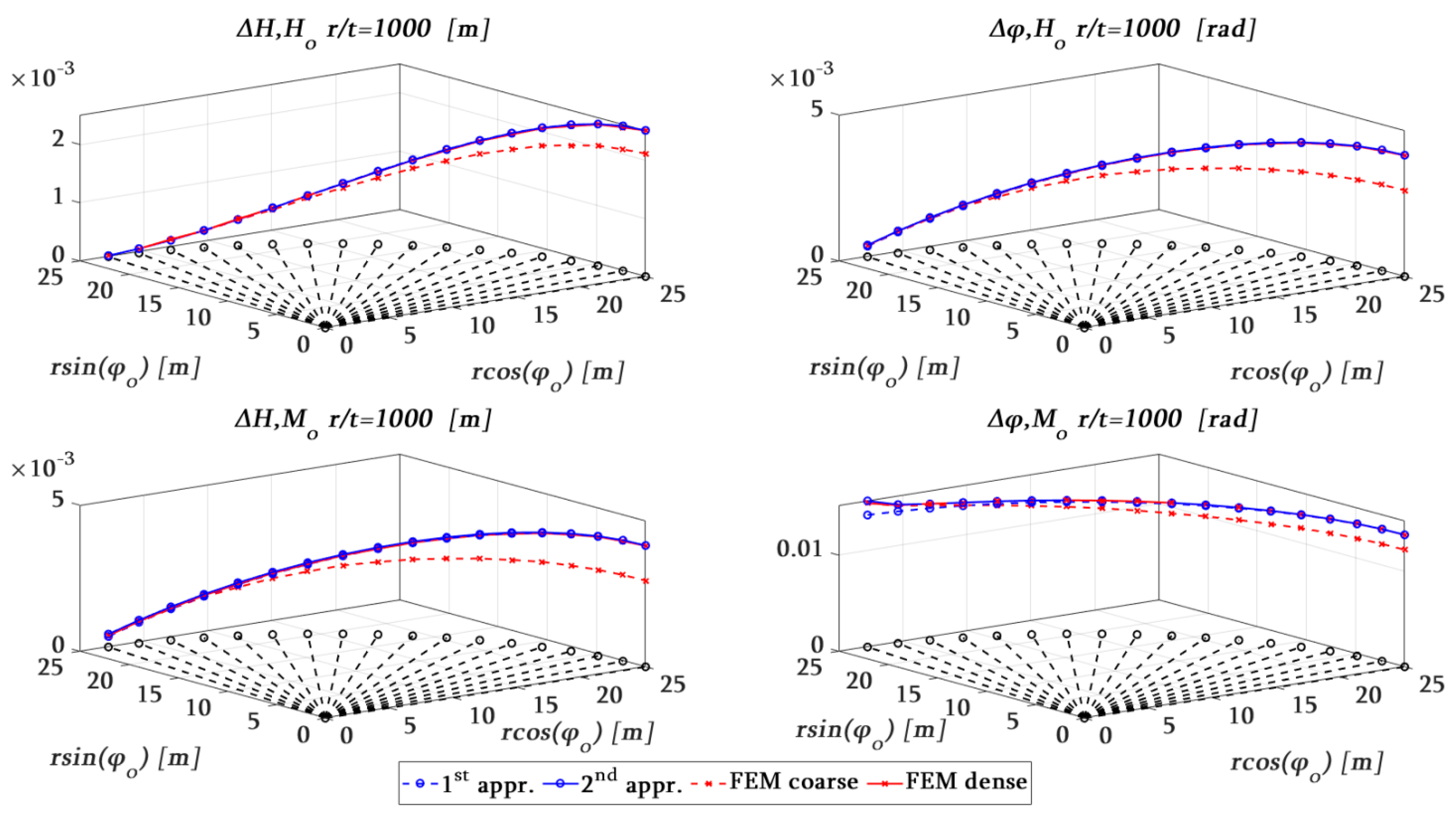
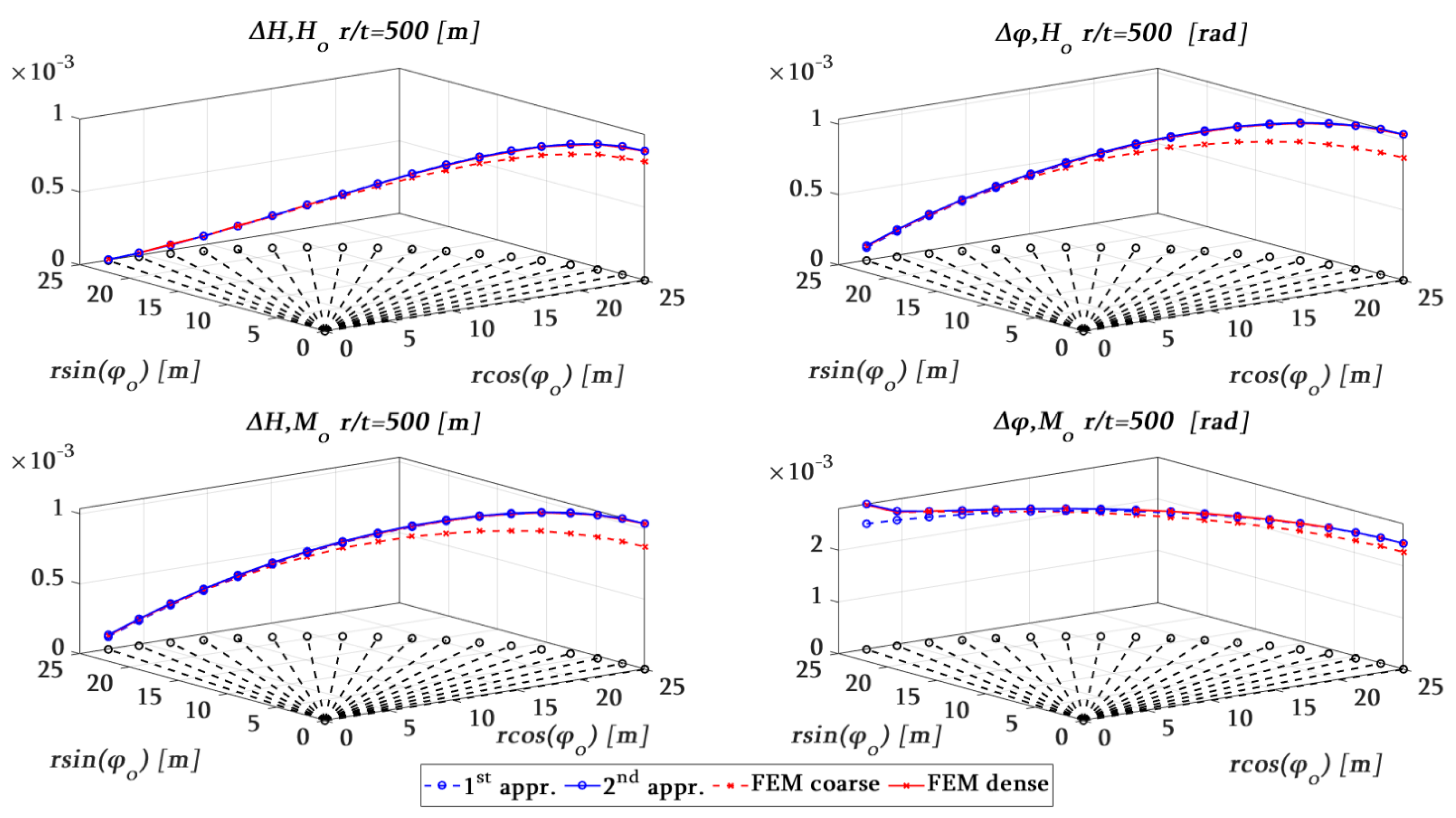
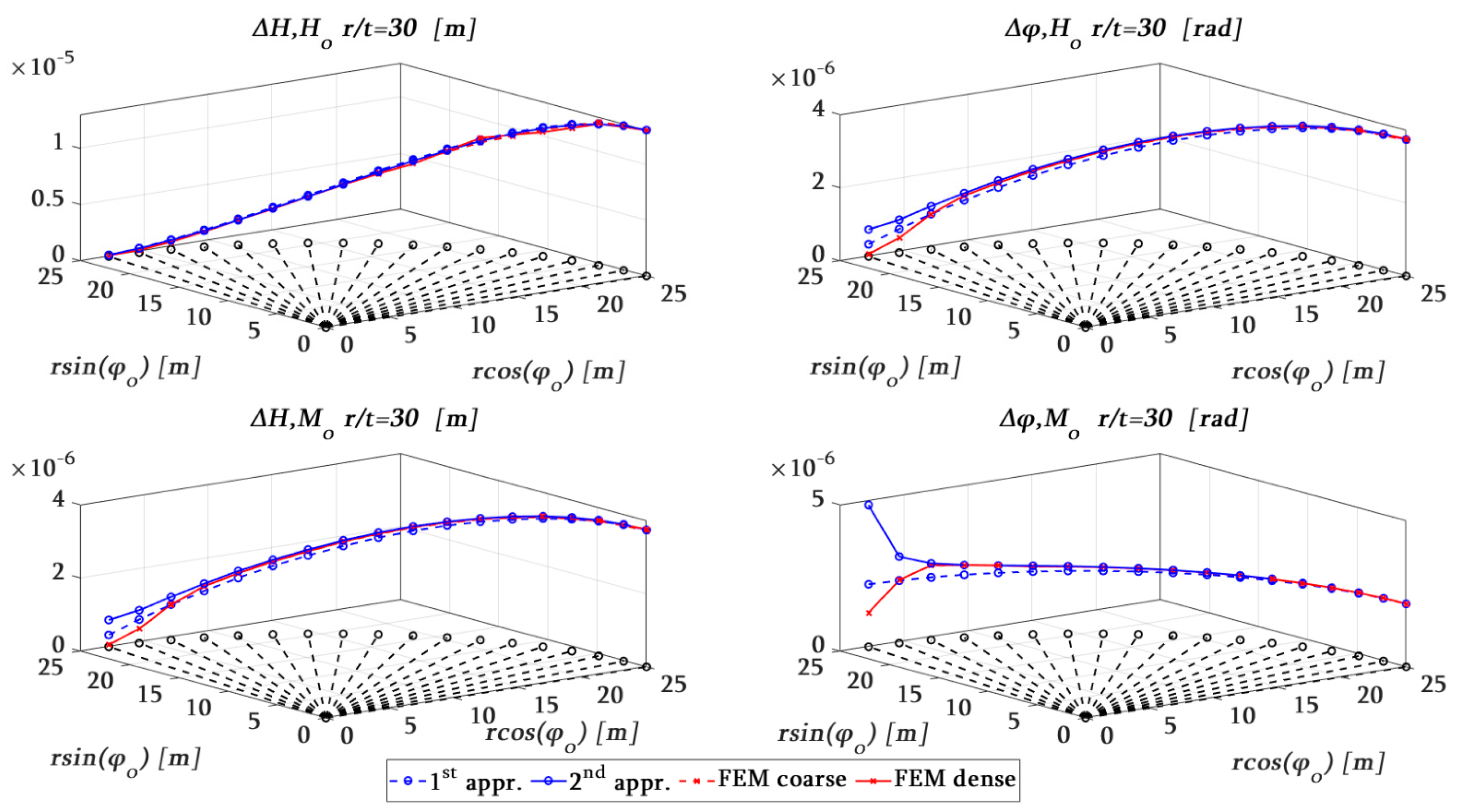
3.2. Comparison between Analytical and Numerical Results
4. Conclusions
- The validation and verification of finite element models implemented to study a specific engineering case are not only crucial but also of vast importance.
- In the absence of laboratory or in situ experiments, the engineer should refer to the results of an analytical approach. The verified finite element model, even under the assumptions of linearity and elasticity, forms a stable basis for its evolution and development under non-linear assumptions.
- Special attention should be given by the engineer on the assumptions by which an analytical approach is governed and on the appropriate available finite element tools.
- The engineer must be fully conversant with the capabilities or restrictions of the available finite element software.
- Two theoretical and analytical approaches have been implemented. The basic difference refers to the dependency of the rotational displacements caused by edge moments, on the value of the roll-down angle for all the considered values of the ratio, .
- As the ratio, becomes larger, or in other words, the shell becomes thicker, the discrepancy between the two analytical approaches is evident not only in rotational but also in translational displacements.
- The numerical results considering the dense finite element discretization approach are in excellent agreement with the analytical ones derived by the second analytical approach for all ratios of .
- The effect of the finite element mesh discretization on numerical results is more pronounced in the case of very thin shells. In particular, for 500 and for the roll-down angle 30°, a dense mesh should be applied. On the contrary, as the shell thickness increases ( 100), no difference between the coarse and dense mesh is detected.
- Differences between the numerical and analytical results are detected for the case of 30, which belongs to the transitional area of definition between “thin” and “thick” shells but only in the range of small values of roll-down angles ( 10°). The behavior of the dome as a “thick shallow” shell cannot be captured by the implementation of homogeneous and thin shell elements. The use of the thick shell formulation or of three-dimensional finite elements is proposed.
- According to the aforementioned conclusions, the main future research direction regards the finite element modeling of “thick shallow” shells by the use of three-dimensional elements.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kwasniewski, L.; Bojanowski, C. Principles of verification and validation. J. Struct. Fire Eng. 2015, 6, 29–40. [Google Scholar] [CrossRef]
- Roache, P. Verification and Validation in Computational Science and Engineering, 1st ed.; Hermosa Publishers: Albuquerque, NM, USA, 1998. [Google Scholar]
- Niemi, H.A. Benchmark computations of stresses in a spherical dome with shell finite elements. SIAM J. Sci. Comput. 2016, 38, B440–B457. [Google Scholar] [CrossRef]
- Pitilakis, K.; Terzi, V. Experimental and theoretical SFSI studies in a model structure in Euroseistest. In Special Topics in Earthquake Geotechnical Engineering. Geotechnical, Geological and Earthquake Engineering, 1st ed.; Sakr, M., Ansal, A., Eds.; Springer: Dordrecht, The Netherlands, 2012; Volume 16, pp. 175–215. [Google Scholar] [CrossRef]
- Manolis, G.D.; Makarios, T.K.; Terzi, V.; Karetsou, I. Mode shape identification of an existing three-story flexible steel stairway as a continuous dynamic system. Theor. Appl. Mech. 2015, 42, 151–166. [Google Scholar] [CrossRef]
- Chandrashekhra, K. Analysis of Thin Concrete Shells, 2nd ed.; New Age International Publishers: Bengaluru, India, 1995. [Google Scholar]
- Sun, G.; Longman, R.W.; Betti, R.; Che, Z.; Xue, S. Observer Kalman filter identification of suspen-dome. Math. Prob. Eng. 2017, 2, 16101534. [Google Scholar] [CrossRef]
- Hussain, N.L.; Mohammed, S.A.; Mansor, A.A. Finite element analysis of large-scale reinforced concrete shell of domes. J. Eng. Sci. Technol. 2020, 15, 782–791. [Google Scholar]
- Kobielak, S.; Zamiar, Z. Oval concrete domes. Arch. Civ. Mech. Eng. 2017, 17, 486–501. [Google Scholar] [CrossRef]
- Sadowski, A.J.; Rotter, J.M. Solid or shell finite elements to model thick cylindrical tubes and shells under global bending. Int. J. Mech. Sci. 2013, 74, 143–153. [Google Scholar] [CrossRef]
- Makarios, T.K. Equivalent torsional-warping stiffness of cores with thin-walled open cross-section using the Vlasov torsion theory. Am. J. Eng. App. Sci. 2023, 16, 44–55. [Google Scholar] [CrossRef]
- Donell, L.H. Stability of Thin-Walled Tubes under Torsion; NACA Report No. 479; National Advisory Committee for Aeronautics: Washington, DC, USA, 1933. [Google Scholar]
- Timoshenko, S.P. History of Strength of Materials, 1st ed.; McGraw-Hill Book Company: New York, NY, USA, 1953. [Google Scholar]
- Novozhilov, V.V. The Theory of Thin Shells, 2nd ed.; P. Noordhoff Ltd.: Groningen, The Netherlands, 1964. [Google Scholar]
- Calladine, C.R. Theory of Shell Structures, 1st ed.; Cambridge University Press: Cambridge, UK, 1983. [Google Scholar]
- Bushnell, D. Computerized Buckling Analysis of Shells, 1st ed.; Martinus Nijhoff Publishers: Mortsel, Belgium, 1985. [Google Scholar]
- Brush, D.O.; Almroth, B.O. Buckling of Bars, Plates and Shells; McGraw-Hill Book Company: New York, NY, USA, 1975. [Google Scholar]
- Seide, P. Small Elastic Deformations of Thin Shells; P. Noordhoff Ltd.: Groningen, The Netherlands, 1975. [Google Scholar]
- Reissner, E. The effect of transverse shear deformation on the bending of elastic plates. J. Appl. Mech. 1945, 12, A69–A77. [Google Scholar] [CrossRef]
- Mindlin, R.D. Influence of rotary inertia and shear on flexural motions of isotropic elastic plates. J. Appl. Mech. 1951, 18, 31–38. [Google Scholar] [CrossRef]
- Euler, L. De motu vibratorio laminarum elasticarum, ubi plures novae vibrationum species hactenus non pertractatae evolvunter. Novi Comment. Acad. Sci. Metrop. 1773, 17, 449–487. [Google Scholar]
- Timoshenko, S.P. On the correction factor for shear of the differential equation for transverse vibrations of bars of uniform cross-section. Lond. Edinb. Dubl. Phil. Mag. 1921, 41, 744–746. [Google Scholar] [CrossRef]
- Elishakoff, I. Who developed the so-called Timoshenko beam theory? Math. Mech. Solids 2020, 25, 97–119. [Google Scholar] [CrossRef]
- Terzi, G.V. Soil-structure interaction effects on the flexural vibrations of a cantilever beam. Appl. Math. Model. 2021, 97, 138–181. [Google Scholar] [CrossRef]
- Vlassov, S. Algemeine Schalentheorie und ihre Anwendung in der Technik; Akademie-Verlag: Berlin, Germany, 1958. [Google Scholar]
- Timoshenko, S.; Woinowsky-Krieger, S. Theory of Plates and Shells; McGraw-Hill: New York, NY, USA, 1959. [Google Scholar]
- Flügge, W. Statik und Dynamik der Schalen; Springer: Berlin/Heidelberg, Germany, 1975. [Google Scholar]
- Heyman, J. Equilibrium of Shell Structures; Oxford University Press: Oxford, UK, 1977. [Google Scholar]
- Mbakogu, F.C.; Pavlović, M.N. Bending and stretching actions in shallow domes. Part 1. Analytical derivations. Thin-Walled Struct. 1996, 26, 61–82. [Google Scholar] [CrossRef]
- Mbakogu, F.C.; Pavlović, M.N. Bending and stretching actions in shallow domes. Part 2. A comparative study of various boundary conditions. Thin-Walled Struct. 1996, 26, 147–158. [Google Scholar] [CrossRef]
- Barsotti, R.; Stagnari, R.; Bennati, S. Searching for admissible thrust surfaces in axial-symmetric masonry domes: Some first explicit solutions. Eng. Struct. 2021, 242, 112547. [Google Scholar] [CrossRef]
- Cusano, C.; Montanino, A.; Olivieri, C.; Paris, V.; Cennamo, C. Graphical and Analytical Quantitative Comparison in the Domes Assessment: The Case of San Francesco di Paola. Appl. Sci. 2021, 11, 3622. [Google Scholar] [CrossRef]
- Bacigalupo, A.; Brencich, A.; Gambarotta, L. A simplified assessment of the dome and drum of the Basilica of S. Maria Assunta in Carignano in Genoa. Eng. Struct. 2013, 56, 749–765. [Google Scholar] [CrossRef]
- Balasubramanian, M.; Sathish Kumar, M.K.; Stalin, B.; Ravichandran, M. Theoretical predictions and experimental investigation on three stage hemispherical dome in superplastic forming process. Mater. Today Proc. 2020, 24, 1424–1433. [Google Scholar] [CrossRef]
- Cusano, C.; Angjeliu, G.; Montanino, A.; Zuccaro, G.; Cennamo, C. Considerations about the static response of masonry domes: A comparison between limit analysis and finite element method. Int. J. Mason. Res. Innov. 2021, 6, 502–528. [Google Scholar] [CrossRef]
- Padovec, Z.; Vondráček, D.; Mareš, T. The analytical and numerical stress analysis of various domes for composite pressure vessels. Appl. Comput. Mech. 2022, 16, 151–166. [Google Scholar] [CrossRef]
- Al-Hashimi, H.; Seibi, A.C.; Molki, A. Experimental Study and Numerical Simulation of Domes under Wind Load. In Proceedings of the ASME 2009 Pressure Vessels and Piping Division Conference, Prague, Czech Republic, 26–30 July 2009; pp. 519–528. [Google Scholar]
- Hamed, E.; Bradford, M.A.; Gilbert, R.I.; Chang, Z.-T. Analytical Model and Experimental Study of Failure Behavior of Thin-Walled Shallow Concrete Domes. J. Struct. Eng. 2011, 137, 88–99. [Google Scholar] [CrossRef]
- Shaheen, Y.B.I.; Eltaly, B.A.; Hanesh, A.A. Experimental and FE simulations of ferrocement domes reinforced with composite materials. Concr. Res. Lett. 2014, 5, 873–887. [Google Scholar]
- Gohari, S.; Sharifi, S.; Burvill, C.; Mouloodi, S.; Izadifar, M.; Thissen, P. Localized failure analysis of internally pressurized laminated ellipsoidal woven GFRP composite domes: Analytical, numerical, and experimental studies. Arch. Civ. Mech. Eng. 2019, 19, 1235–1250. [Google Scholar] [CrossRef]
- Zona, R.; Ferla, P.; Minutolo, V. Limit analysis of conical and parabolic domes based on semi-analytical solution. J. Build. Eng. 2021, 44, 103271. [Google Scholar] [CrossRef]
- Nodargi, N.A.; Bisegna, P. Minimum thrust and minimum thickness of spherical masonry domes: A semi-analytical approach. Eur. J. Mech. A/Solids 2021, 87, 104222. [Google Scholar] [CrossRef]
- Zona, R.; Esposito, L.; Palladino, S.; Totaro, E.; Minutolo, V. Semianalytical Lower-Bound Limit Analysis of Domes and Vaults. Appl. Sci. 2022, 12, 9155. [Google Scholar] [CrossRef]
- Girkman, K. Die Faltwerke. In Flächentragwerke; Springer: Vienna, Austria, 1963. [Google Scholar] [CrossRef]
- Pitkäranta, J.; Babuška, I.; Szabó, B. The Girkmann problem. IACM Expr. 2008, 22, 28. [Google Scholar]
- Szabó, B.; Babuška, I.; Pitkäranta, J.; Nervi, S. The problem of verification with reference to the Girkmann problem. Eng. Comput. 2010, 26, 171–183. [Google Scholar] [CrossRef]
- Niemi, A.H.; Babuška, I.; Pitkäranta, J.; Demkowicz, L. Finite element analysis of the Girkmann problem using the modern hp-version and the classical h-version. Eng. Comput. 2012, 28, 123–134. [Google Scholar] [CrossRef]
- Pitkäranta, J.; Babuška, I.; Szabó, B. The dome and the ring: Verification of an old mathematical model for the design of a stiffned shell roof. Comput. Math. Appl. 2012, 64, 48–72. [Google Scholar] [CrossRef]
- Novozhilov, V.V. Thin Shell Theory; Wolter-Noordhoff: Groningen, The Netherlands, 1970. [Google Scholar]
- Gould, P.L. Analysis of Shells and Plates; Springer: New York, NY, USA, 1988. [Google Scholar]
- Zingoni, A. Shell Structures in Civil and Mechanical Engineering: Theory and Closed-Form Analytical Solutions; Thomas Telford, The Institution of Civil Engineers: London, UK, 1997. [Google Scholar]
- Zingoni, A.; Enoma, N. On the strength and stability of elliptic toroidal domes. Eng. Struct. 2020, 207, 110241. [Google Scholar] [CrossRef]
- Geckeler, J. Über die Festigkeit achsensymmetrischer Schalen. Forschungesarbeiten Auf Dem Geb. Dse Ingenieurwesens 1926, 276, 52. [Google Scholar]
- Hetényi, M. Spherical shells subjected to axial symmetrical bending. Publ. Int. Assoc. Brid. Struct. Eng. 1938, 5, 173. [Google Scholar]
- SAP2000v.24; Computers and Structures, Inc.: Berkeley, CA, USA, 2022.
- Taylor, R.L.; Simo, J.C. Bending and membrane elements for analysis of thick and thin shells. In Proceedings of the NUMEETA, Swansea, Wales, 7–11 January 1985. [Google Scholar]
- Ibrahimbegovic, A.; Wilson, E.L. A unified formulation for triangular and quadrilateral flat shell finite elements with six nodal degrees of freedom. Commun. Appl. Numer. Methods 1991, 7, 1–9. [Google Scholar] [CrossRef]








| E [GPa] | v [−] | [m] | [kN/m] | [kNm/m] | [°] | |
|---|---|---|---|---|---|---|
| 33 | 0.15 | 25 | 1 | 1 | 30, 100, 500, 1000 | 5:5:90 |
| 1st Analytical | FEM Coarse | FEM Dense | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1000 | −0.67 | 3.2 | 1.74 | 8.13 | 17.67 | 6.70 | −0.14 | 2.24 | 0.15 | |||
| 500 | −0.97 | 2.44 | 2.41 | 4.28 | 9.21 | 3.92 | −0.01 | 0.90 | 0.09 | |||
| 100 | −1.84 | 5.44 | 5.44 | 3.29 | 6.43 | 2.84 | 2.70 | 4.99 | 2.05 | |||
| 30 | −1.74 | 9.93 | 9.94 | 6.08 | 10.56 | 6.26 | 6.26 | 10.15 | 6.02 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Terzi, V.G.; Makarios, T.K. Finite Element In-Depth Verification: Base Displacements of a Spherical Dome Loaded by Edge Forces and Moments. Modelling 2024, 5, 37-54. https://doi.org/10.3390/modelling5010003
Terzi VG, Makarios TK. Finite Element In-Depth Verification: Base Displacements of a Spherical Dome Loaded by Edge Forces and Moments. Modelling. 2024; 5(1):37-54. https://doi.org/10.3390/modelling5010003
Chicago/Turabian StyleTerzi, Vasiliki G., and Triantafyllos K. Makarios. 2024. "Finite Element In-Depth Verification: Base Displacements of a Spherical Dome Loaded by Edge Forces and Moments" Modelling 5, no. 1: 37-54. https://doi.org/10.3390/modelling5010003
APA StyleTerzi, V. G., & Makarios, T. K. (2024). Finite Element In-Depth Verification: Base Displacements of a Spherical Dome Loaded by Edge Forces and Moments. Modelling, 5(1), 37-54. https://doi.org/10.3390/modelling5010003







