Simulation of Oil Well Drilling System Using Distributed–Lumped Modelling Technique
Abstract
1. Introduction
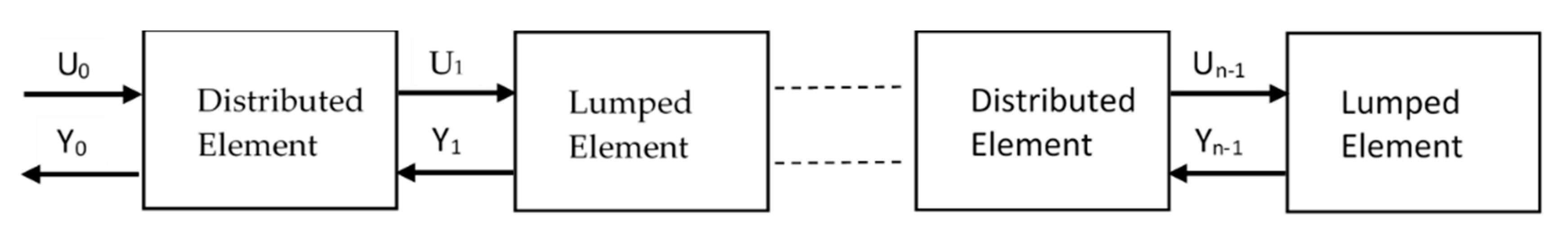
2. Model Description
3. Mathematical Models
4. Lumped Model
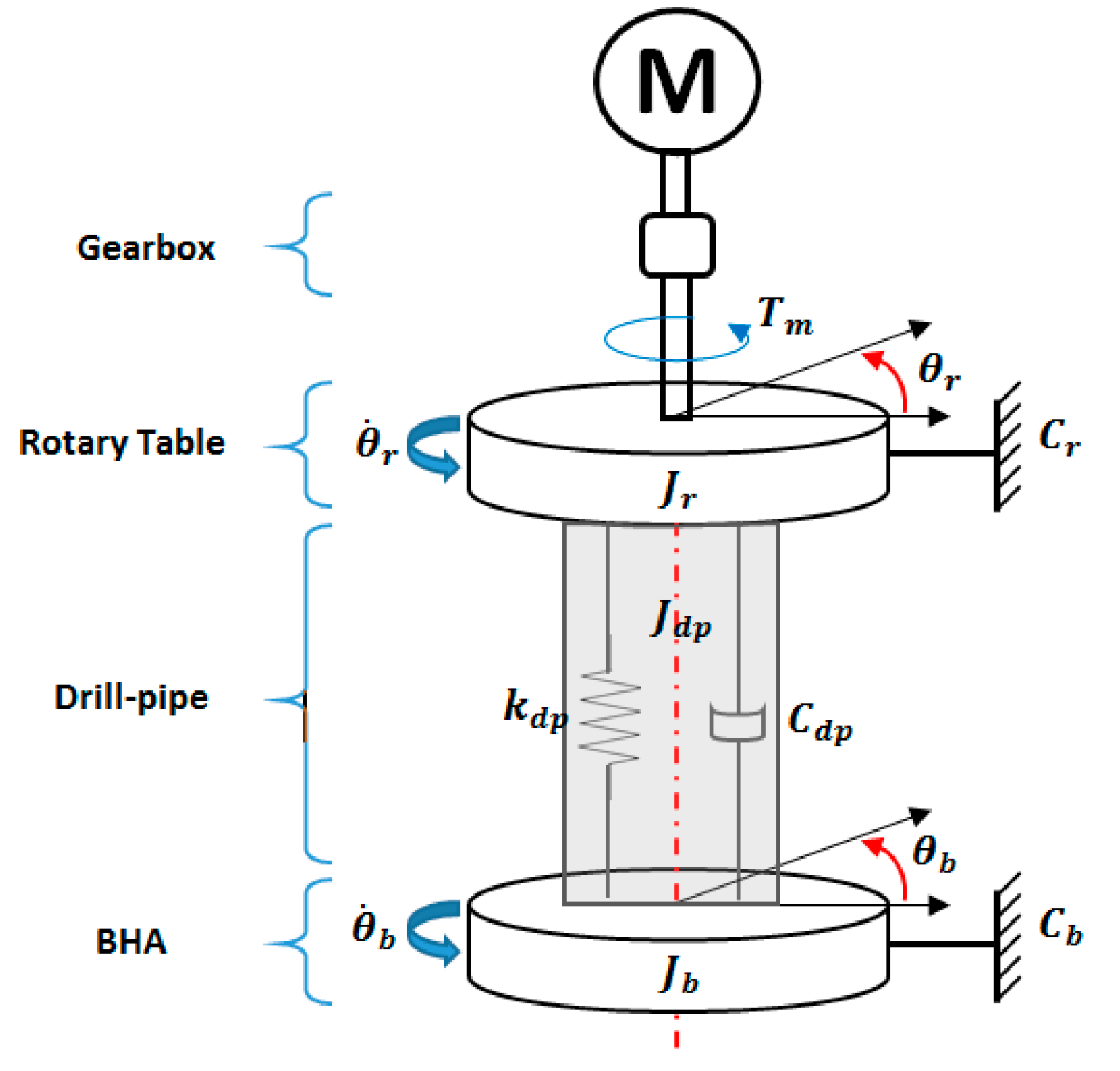
4.1. The Drive System
4.2. The Drill String
4.3. The Cutting Process
5. Frictional Torque on the Bit
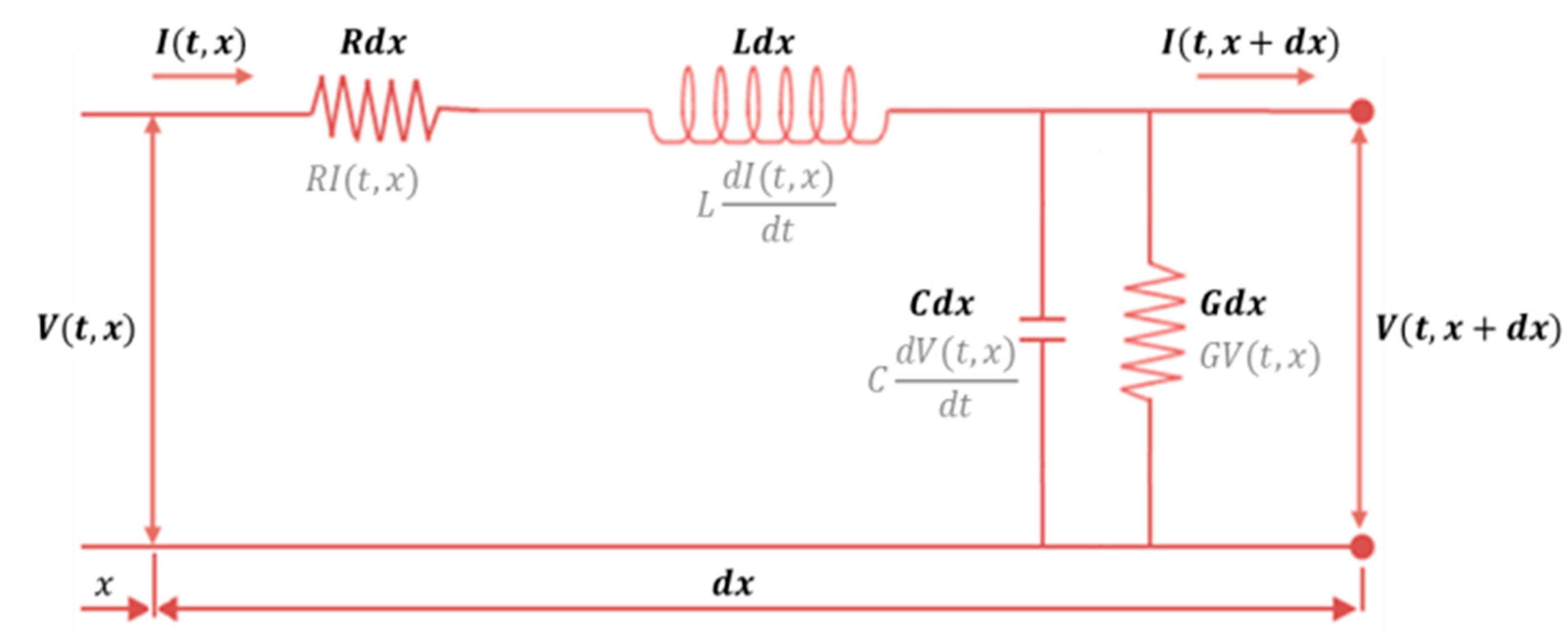
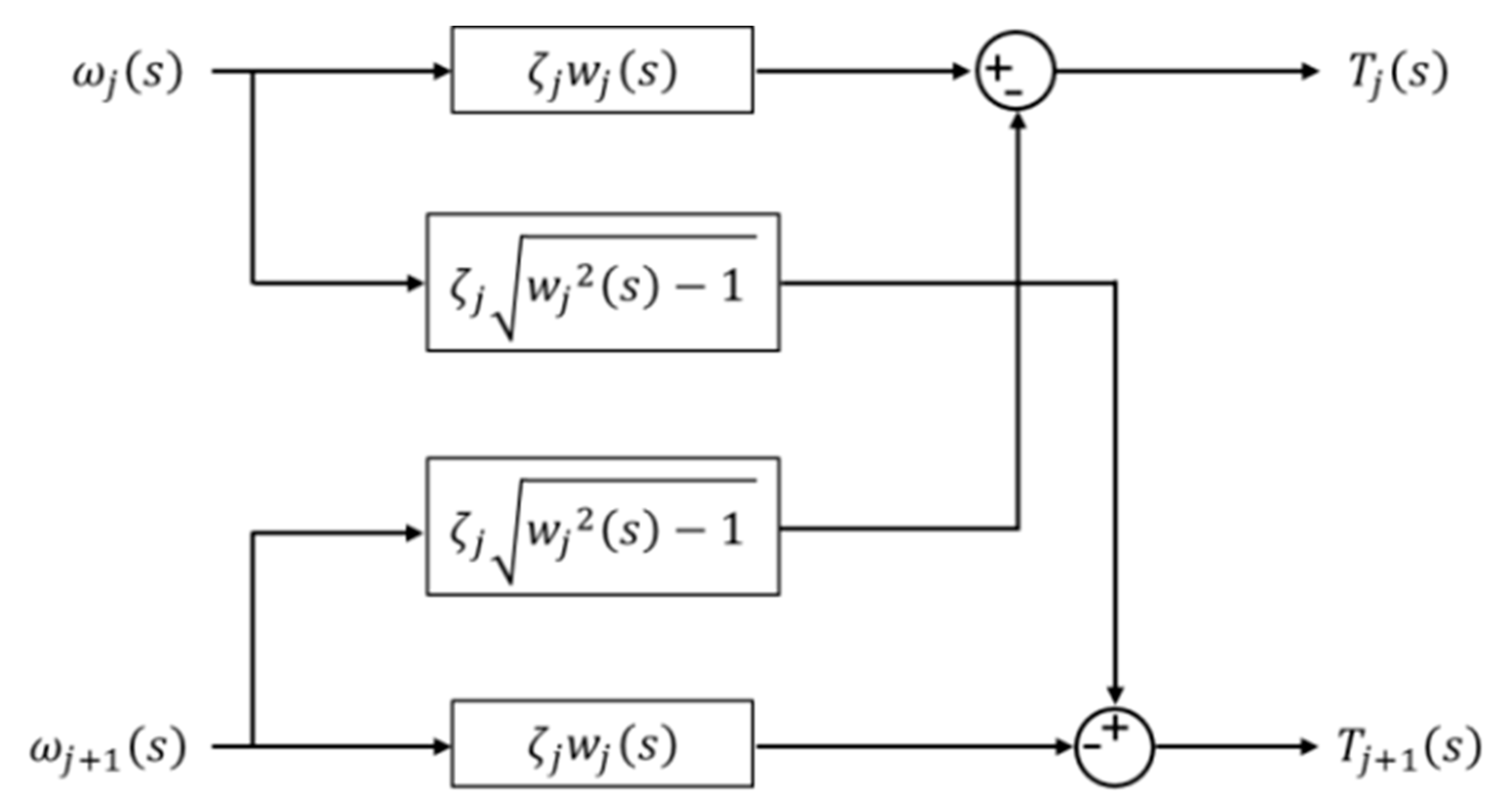
5.1. Distributed Model of the Rotating Shaft
5.2. Torsional Shaft
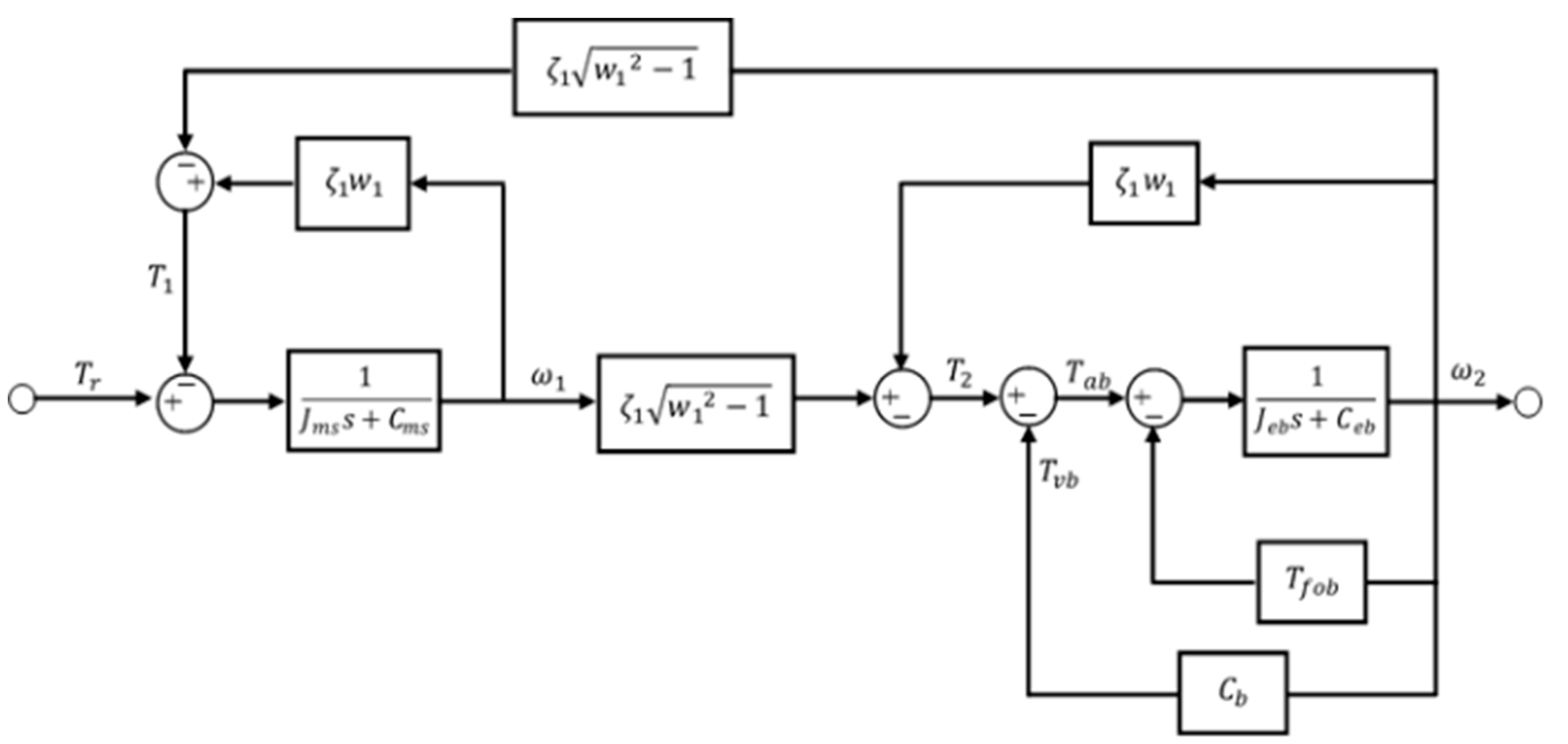
6. Hybrid Model of the Drilling System
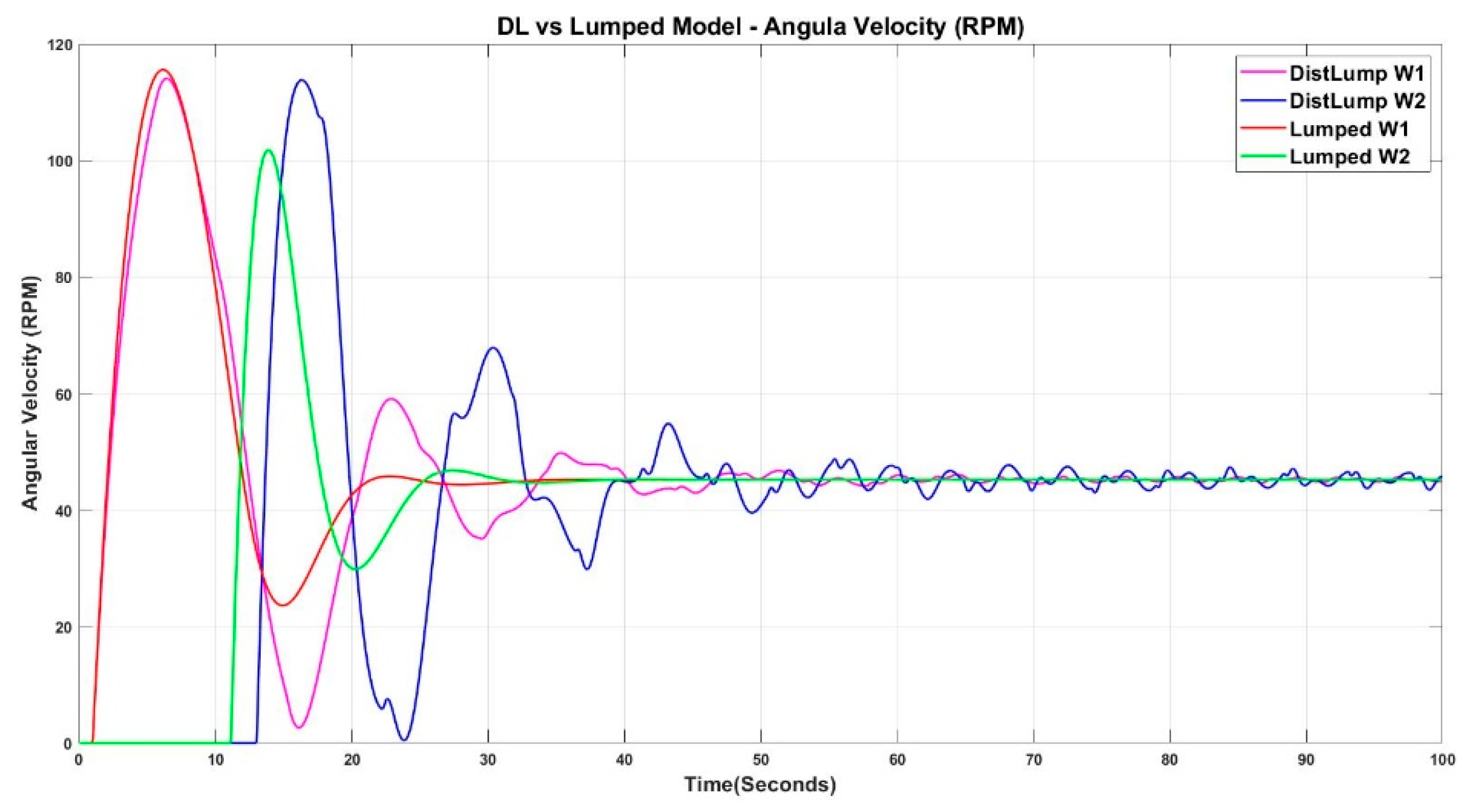
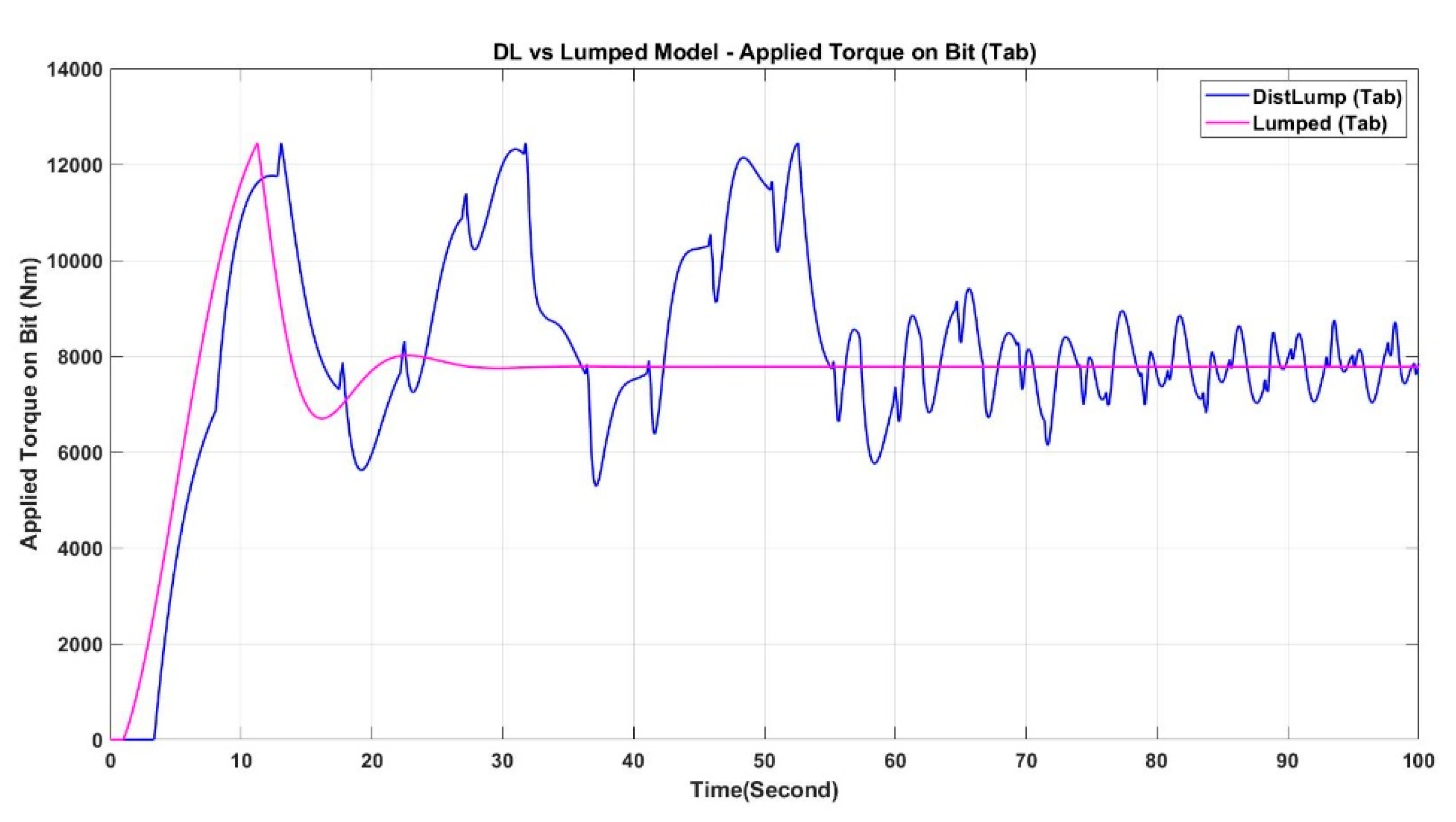
7. Results and Analysis
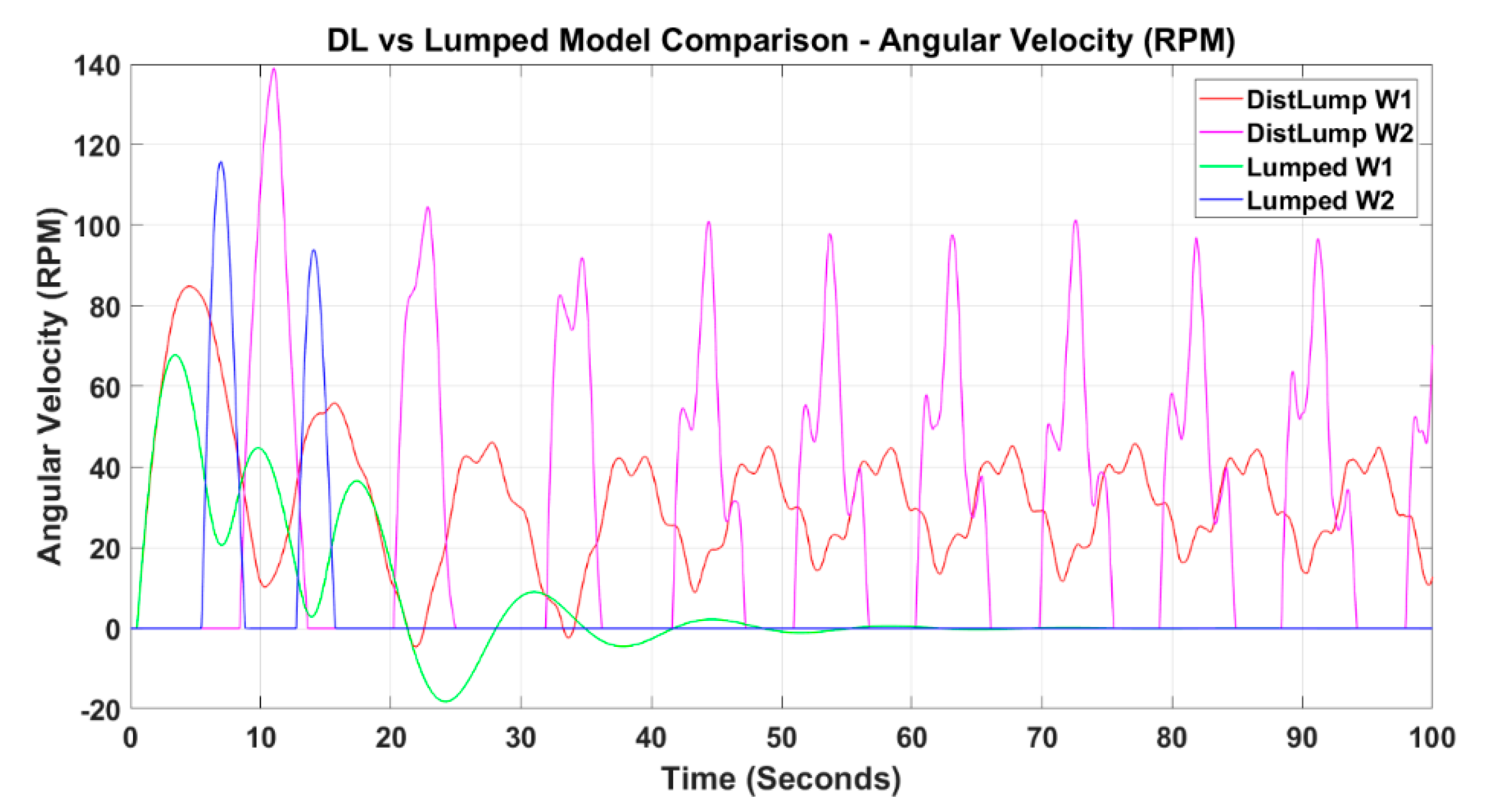
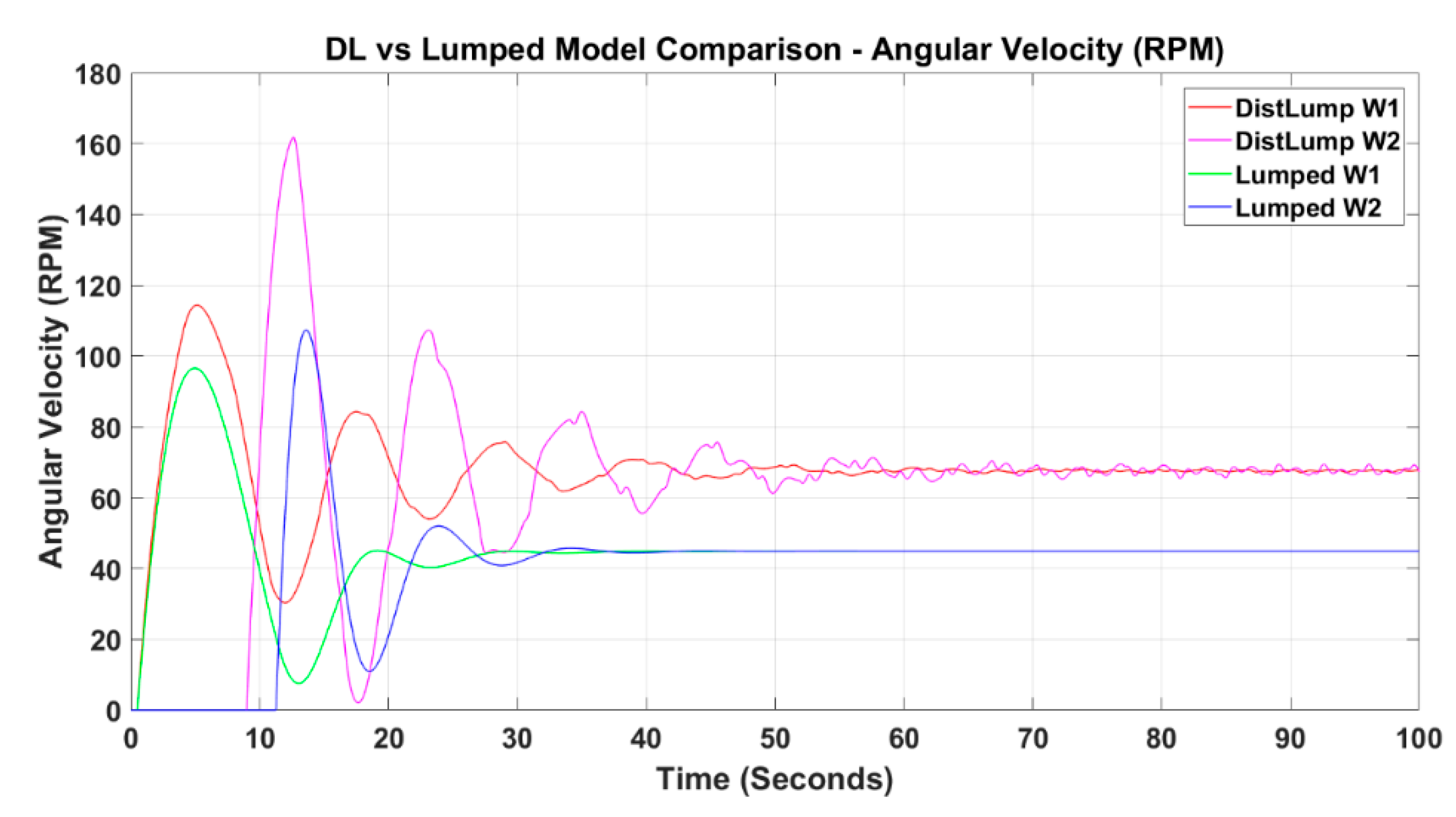
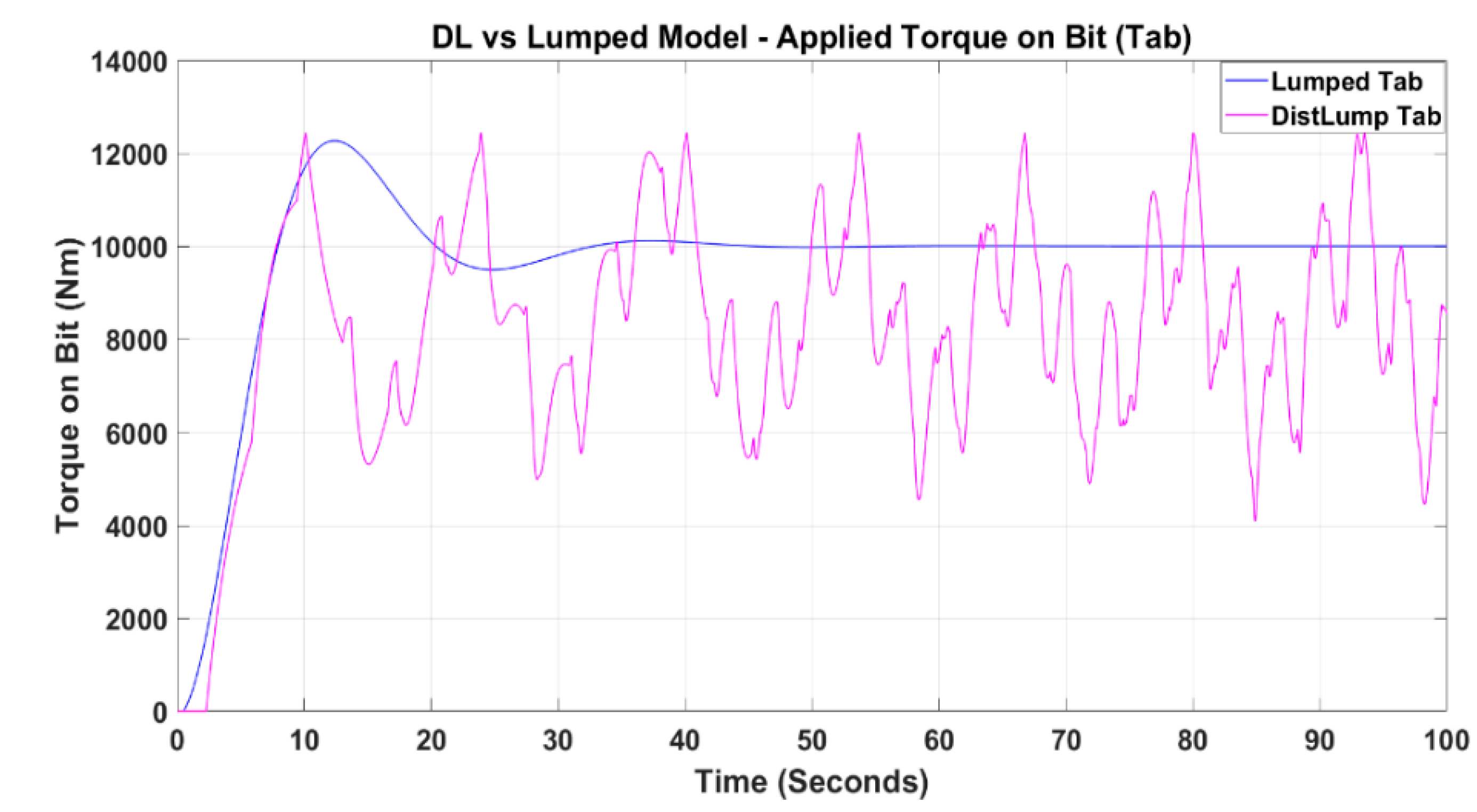
7.1. Comparison of the Lumped Model and the D–L Model
7.2. Simulation for Drill Pipe Length of 2000 m
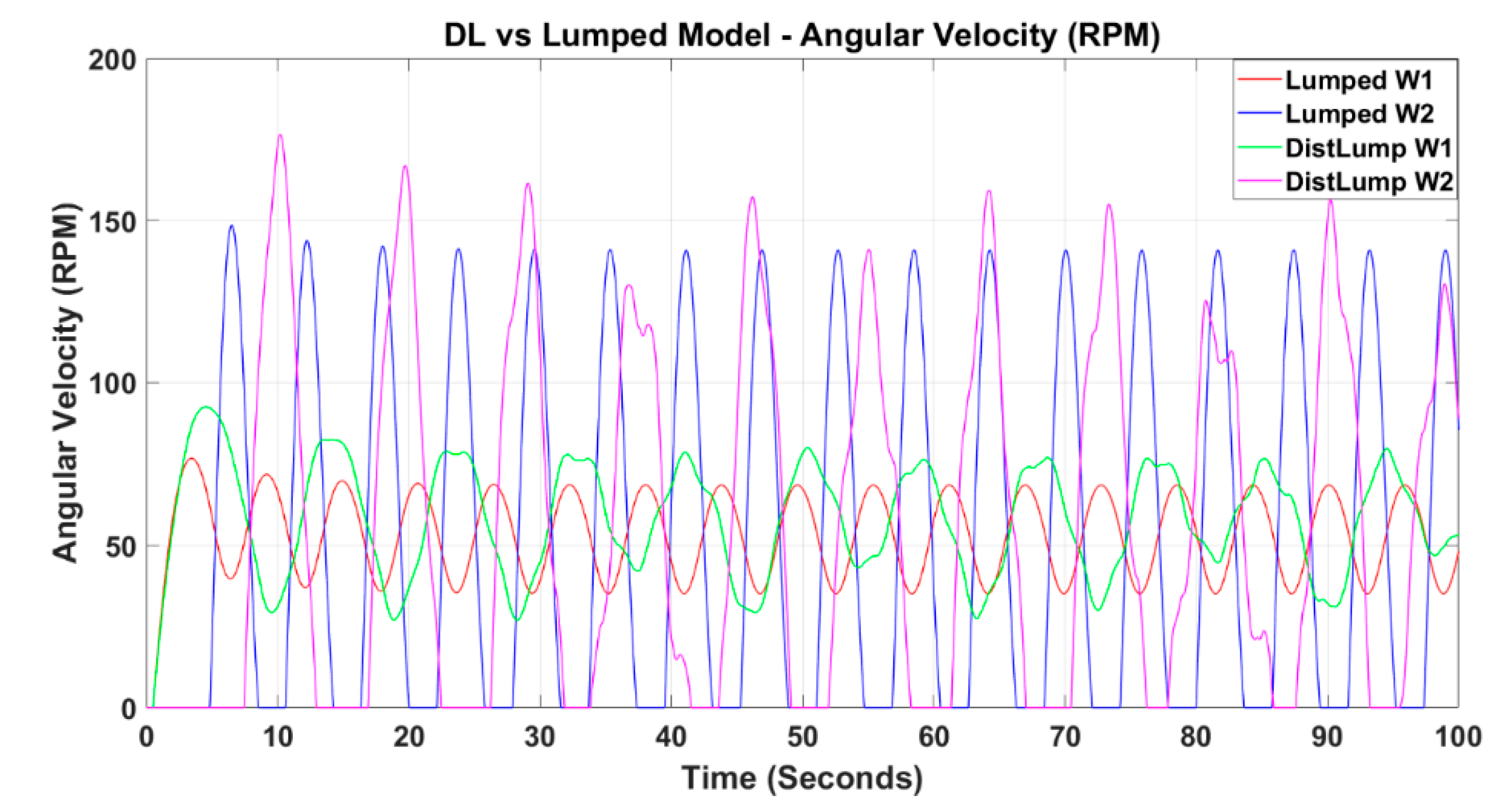
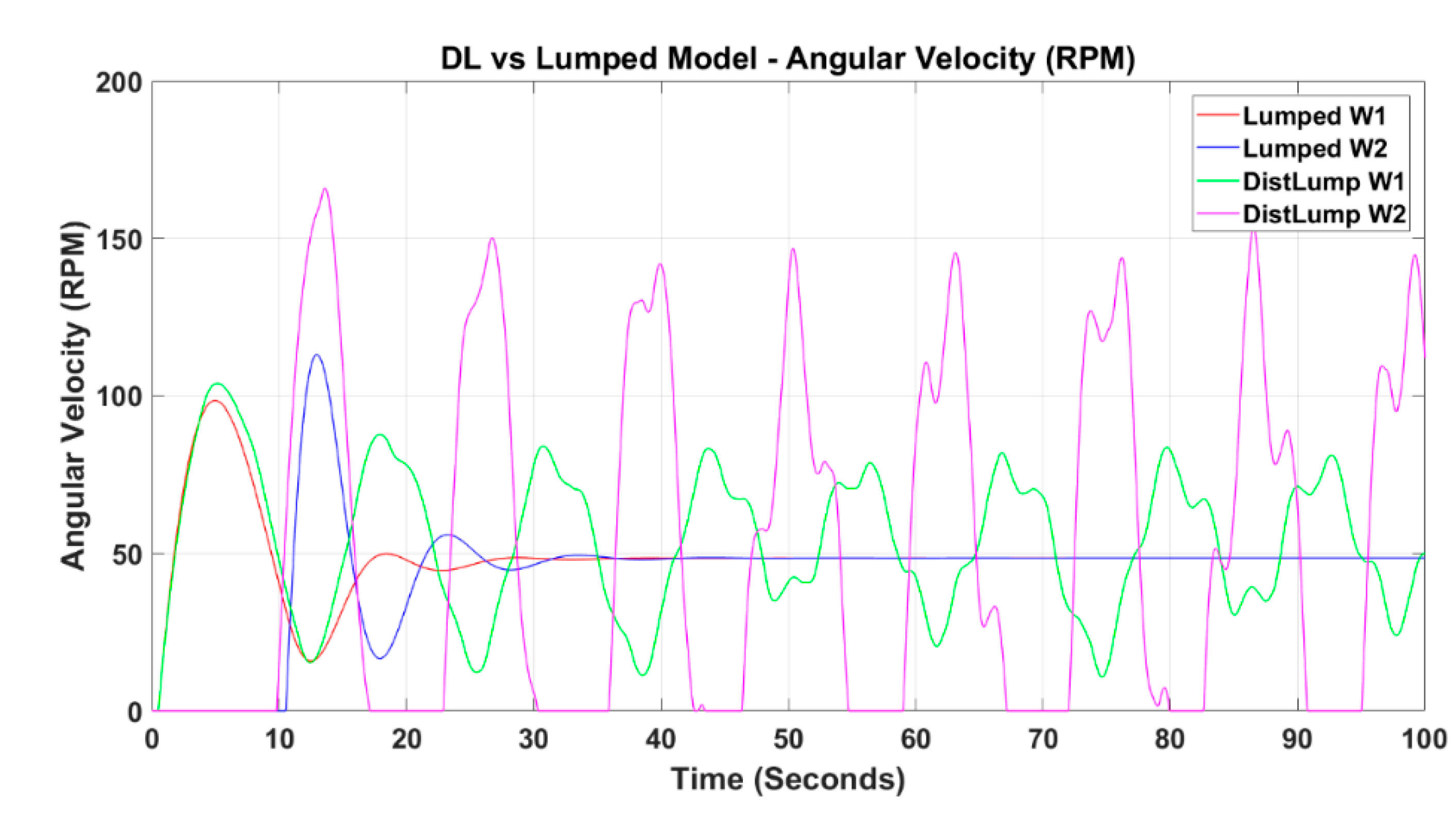
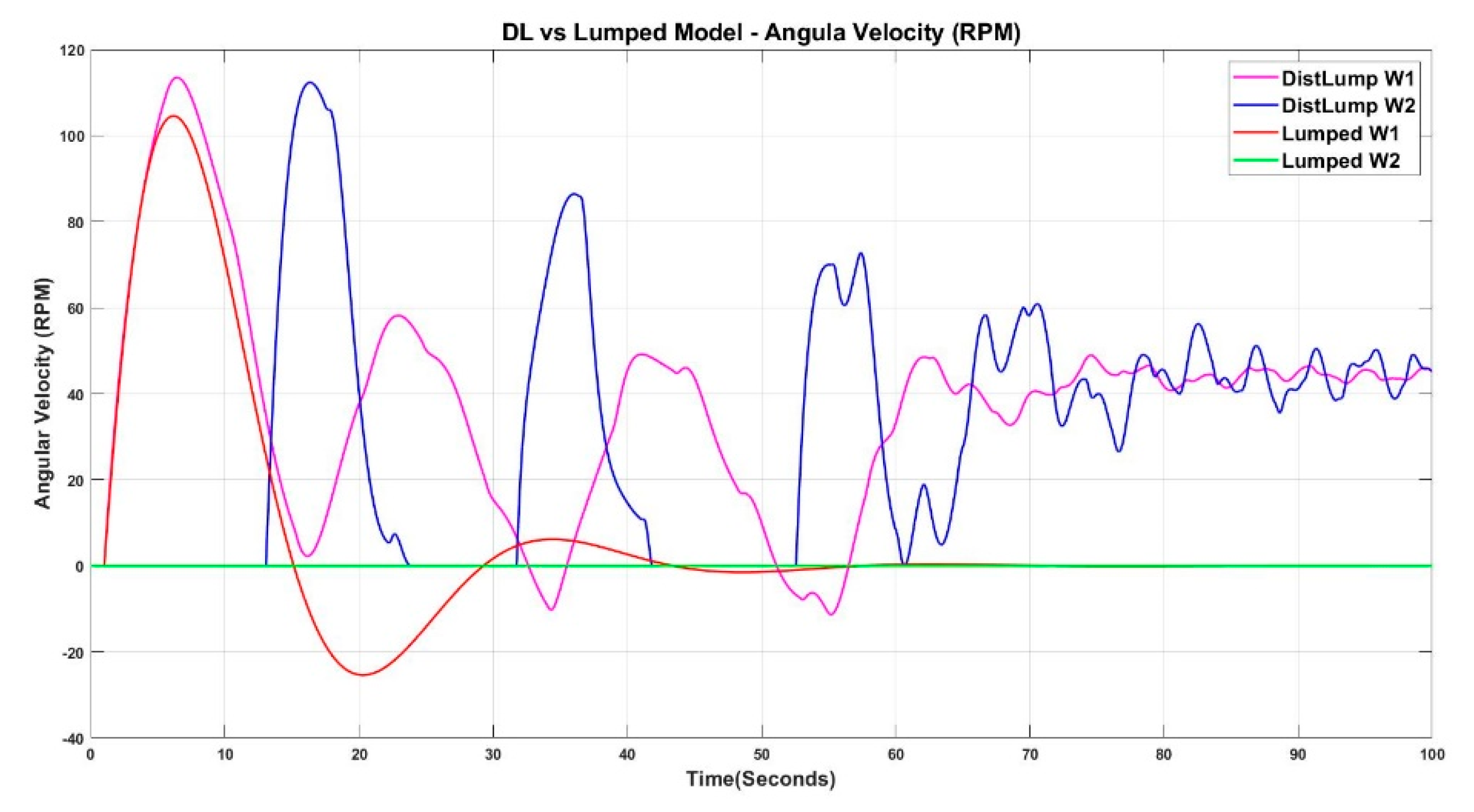
7.3. Simulation for Drill Pipe Length of 5700 m
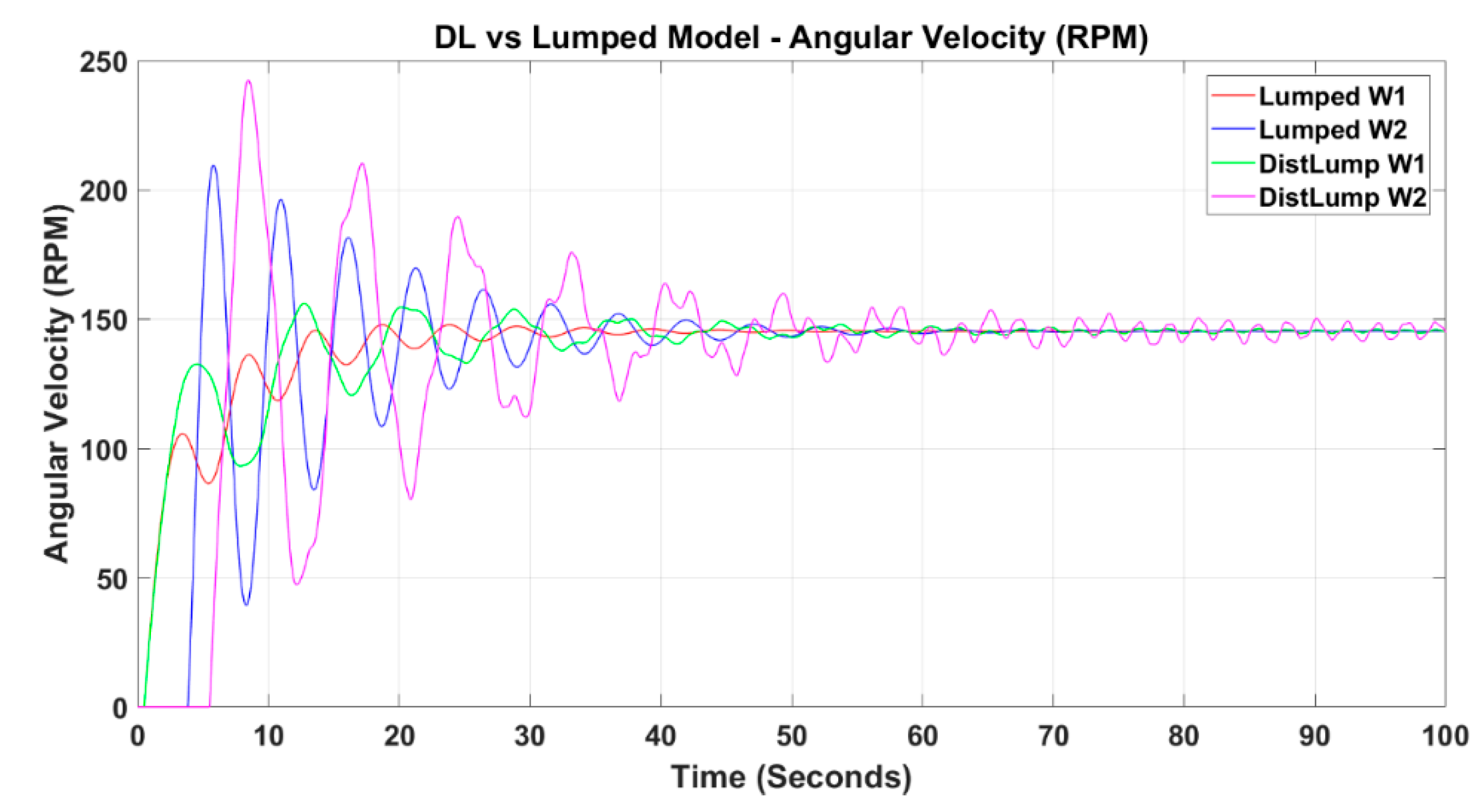
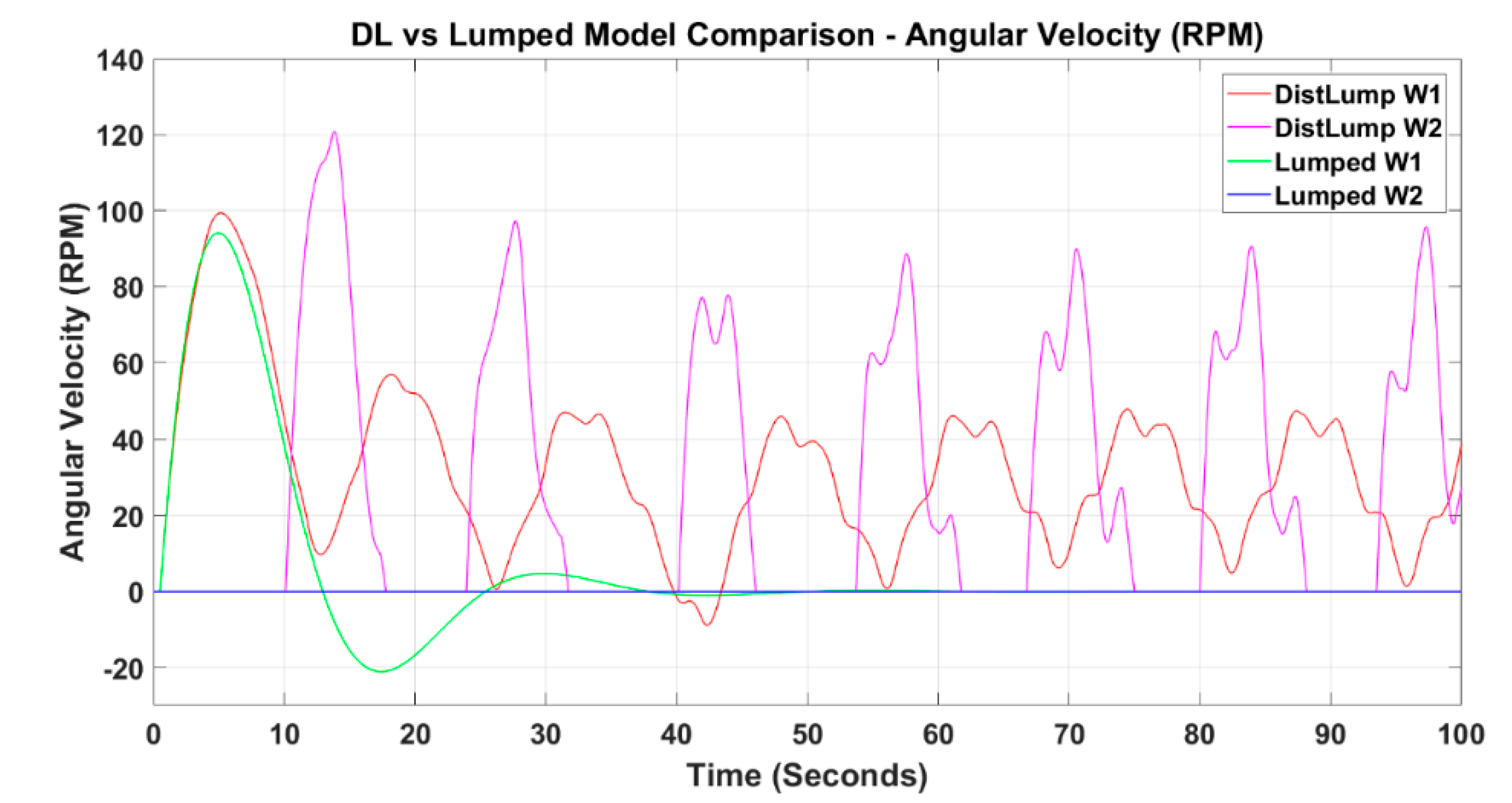
7.4. Simulation for Drill Pipe Length of 7500 m
8. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Capacitance per unit length | |
| Coefficient damping of bearing on the motor side | |
| Coefficient damping of bearing on the load side | |
| Equivalent viscous damping of the drive system | |
| Equivalent viscous damping coefficient along the drill pipe | |
| Damping coefficient of the drill pipe per unit length | |
| Equivalent viscous damping of the bottom hole assembly | |
| Outer diameter of the drill pipe | |
| Inner diameter of the drill pipe | |
| Outer diameter of the drill collar | |
| Inner diameter of the drill collar | |
| Outer diameter of the HWDP | |
| Inner diameter of the HWDP | |
| Conductance per unit length | |
| Shear modulus of steel | |
| Shear modulus of distributed shaft | |
| Current of armature of the motor | |
| Current at distance x and time t | |
| Inertia of distributed shaft | |
| Mass moment of inertia of the motor | |
| Mass moment of inertia of the rotary table | |
| Equivalent mass moment of inertia at motor side | |
| Equivalent mass moment of inertia of the BHA | |
| Mass moment of inertia of the drill string | |
| Mass moment of inertia of the HWDP | |
| Mass moment of inertia of the drill pipe | |
| Mass moment of inertia of the drill collar | |
| Mass moment of inertia of distributed shaft | |
| Mass moment of inertia of load | |
| Shaft polar moment of inertia | |
| The emf constant | |
| Motor constant related to physical properties of the motor | |
| Equivalent torsional stiffness of the drill pipe | |
| Inductance per unit length of electrical transmission line | |
| Armature inductance of the motor | |
| Length of drill pipe | |
| Length of HWDP | |
| Length of torsional distributed shaft | |
| A combined gear ratio of gear box and bevel gear | |
| Armature resistance of the motor | |
| Radius of shaft | |
| Radius of the bit | |
| Resistance per unit length | |
| Torque of motor | |
| Torque of the rotary table | |
| Torque on the bit (TOB) | |
| Viscosity damping torque over the BHA | |
| Friction torque on the bit | |
| External torque applied by drill string on the bit | |
| Static friction torque on the bit | |
| Sliding friction torque (cutting torque) | |
| Input torque to distributed shaft from a prime mover | |
| Input torque to drill pipe from a rotary table | |
| Output torque from distributed shaft to load | |
| Output torque from drill pipe to the BHA | |
| Back electric motive force (back-emf) of armature | |
| Supply armature voltage | |
| Voltage at distance x and time t | |
| Weight on a bit (WOB) | |
| Limit velocity interval | |
| Shear strain | |
| Positive constant defining the decaying velocity of | |
| Propagation constant of the electrical transmission line | |
| Propagation constant of the distributed | |
| Characteristic impedance of electrical transmission line | |
| Characteristic impedance of distributed shaft | |
| Characteristic impedance of the drill pipe | |
| Friction coefficient at the bit | |
| Coulomb friction coefficient | |
| Static friction coefficient | |
| Angular displacement of the motor | |
| Angular displacement of the rotary table | |
| Angular displacement of the bit | |
| Angle of twist | |
| Density of steel | |
| Density of distributed shaft | |
| Angular velocity of the motor shaft | |
| Angular velocity of the rotary table | |
| Angular velocity of the bit | |
| Angular velocity at the inlet of a distributed shaft | |
| Angular velocity at the outlet of a distributed shaft |
Appendix A
| Name | Symbol | Value | Units |
|---|---|---|---|
| Shear Modulus of Steel | N/m2 | ||
| Density of Steel | Kg/m2 | ||
| Radius of Drill Bit | |||
| Weight on Bit | |||
| Static Friction Torque on Bit | 12,446 | ||
| Coulomb Friction Torque on Bit | |||
| Length of the BHA | |||
| Length of HWDP | |||
| Outer Diameter of Drill Pipes | |||
| Inner Diameter of Drill Pipes | |||
| Outer Diameter of Drill Collar | |||
| Inner Diameter of Drill Collar | |||
| Outer Diameter of HWDP | |||
| Inner Diameter of HWDP | |||
| Gear Ratio | |||
| Inertia Mass Moment of Motor | Kgm2 | ||
| Inertia Mass Moment of Rotary Table | |||
| Inertia Mass Moment of Drive System | Kgm2 | ||
| Equivalent Mass Moment of Inertia of the BHA | Kgm2 | ||
| Equivalent Damping Coefficient of Drive System | Nms/rad | ||
| Propagation Constant of Drill Pipe | s/m | ||
| Static Friction Coefficients | |||
| Coulomb Friction Coefficients | |||
| Decaying Constant | |||
| Limit Velocity Interval | RPM | ||
| Inductance of Motor | |||
| Resistance of Motor | |||
| Constant of Motor | |||
| Emf Constant | |||
| Characteristic Impedance of Drill Pipe |
References
- Yigit, A.S.; Christoforou, A.P. Fully coupled vibrations of actively controlled drillstrings. J. Sound Vib. 2003, 267, 1029–1045. [Google Scholar] [CrossRef]
- Sahebkar, S.M.; Ghazavi, M.R.; Khadem, S.E.; Ghayesh, M.H. Nonlinear vibration analysis of an axially moving drillstring system with time dependent axial load and axial velocity in inclined well. Mech. Mach. Theory 2011, 46, 743–760. [Google Scholar] [CrossRef]
- Depouhon, A.; Detournay, E. Instability regimes and self-excited vibrations in deep drilling systems. J. Sound Vib. 2014, 333, 2019–2039. [Google Scholar] [CrossRef]
- Macdonald, K.A.; Bjune, J.V. Failure analysis of drillstrings. Eng. Fail. Anal. 2007, 14, 1641–1666. [Google Scholar] [CrossRef]
- Macdonald, K.A. Failure analysis of drillstring and bottom hole assembly components. Eng. Fail. Anal. 1994, 1, 91–117. [Google Scholar] [CrossRef]
- Tinivella, U.; Giustiniani, M. Numerical simulation of coupled waves in borehole drilling through a BSR. Mar. Pet. Geol. 2013, 44, 34–40. [Google Scholar] [CrossRef]
- Dugundji, J.; Mukhopadhyay, V. Lateral Bending-Torsion Vibrations of a Thin Beam Under Parametric Excitation. J. Appl. Mech. 1973, 40, 693–698. [Google Scholar] [CrossRef]
- Vielsack, P. Lateral bending vibrations of a beam with small pretwist. Eng. Struct. 2000, 22, 691–698. [Google Scholar] [CrossRef]
- Yigit, A.S.; Christoforou, A.P. Coupled Torsional and Bending Vibrations of Actively Controlled Drillstrings. J. Sound Vib. 2000, 234, 67–83. [Google Scholar] [CrossRef]
- Zamanian, M.; Khadem, S.E.; Ghazavi, M.R. Stick-slip oscillations of drag bits by considering damping of drilling mud and active damping system. J. Pet. Sci. Eng. 2007, 59, 289–299. [Google Scholar] [CrossRef]
- Jansen, J.D.; Van Den Steen, L. Active damping of self-excited torsional vibrations in oil well drillstrings. J. Sound Vib. 1995, 179, 647–668. [Google Scholar] [CrossRef]
- Rajnauth, J.; Jagai, T. Reduce Torsional Vibrations and Improve Drilling Operations. Int. J. Appl. Sci. Technol. 2012, 2, 109–122. [Google Scholar]
- Whalley, R. Interconnected spatially distributed system. Trans. Inst. Meas. Control 1990, 12, 262–270. [Google Scholar] [CrossRef]
- Russer, P.; Russer, J.A. Application of the Transmission Line Matrix (TLM) method to EMC problems. In Proceedings of the 2012 Asia-Pacific Symposium on Electromagnetic Compatibility, Singapore, 21–24 May 2012; pp. 141–144. [Google Scholar]
- Hoefer, W.J.R. The Transmission-Line Matrix Method—Theory and Applications. IEEE Trans. Microw. Theory Tech. 1985, 33, 882–893. [Google Scholar] [CrossRef]
- Baker, R. A Primer of Oilwell Drilling: A Basic Text of Oil and Gas Drilling, 6th ed.; Petroleum Extension Service, Continuing & Extended Education, University of Texas at Austin: Austin, TX, USA, 2001. [Google Scholar]
- Bommer, P. A Primer of Oilwell Drilling: A Basic Text of Oil and Gas Drilling, 7th ed.; Petroleum Extension Service, Continuing & Extended Education, University of Texas at Austin: Austin, TX, USA, 2008. [Google Scholar]
- Bowman, I. Well-Drilling Methods, Water-Supply Paper-257; Government Printing Office: Washington, DC, USA, 1911.
- Mitchell, R.F.; Miska, S.Z. Fundamental of Drilling Engineering, SPE Textbook Series; Society of Petroleum Engineers: Oklahoma City, OK, USA, 2011; Volume 12, ISBN 9781555632076. [Google Scholar]
- Baker, H. Oil Field Familiarization: Training Guide; Baker Hughes INTEQ: Houston, TX, USA, 1996. [Google Scholar]
- Brett, J.F. The Genesis of Torsional Drillstring Vibrations, Society of Petroleum Engineers, SPE Drilling Engineering; Society of Petroleum Engineers: Oklahoma City, OK, USA, 1992; pp. 168–174. [Google Scholar]
- Navarro-López, E.M.; Suarez, R. Practical approach to modelling and controlling stick-slip oscillations in oilwell drillstrings. In Proceedings of the IEEE International Conference on Control Applications, Institute of Electrical and Electronics Engineers (IEEE), Taipei, Taiwan, 2–4 September 2004; Volume 2, pp. 1454–1460. [Google Scholar]
- Brett, J.F. The Genesis of Bit-Induced Torsional Drillstring Vibrations. SPE Drill. Eng. 1992, 7, 168–174. [Google Scholar] [CrossRef]
- Navarro-Lopez, E.M.; Suarez, R. Modelling and Analysis of Stick-Slip Behaviour in a Drillstring Under Dry Friction. In Proceedings of the Congress of the Mexican Association of Automatic Control; 2004; Volume 1, pp. 330–335. Available online: https://d1wqtxts1xzle7.cloudfront.net/31021540/enavarro_amca04.pdf?1364097902=&response-content-disposition=inline%3B+filename%3DModelling_and_Analysis_of_Stick_slip_Beh.pdf&Expires=1605156926&Signature=LviT~TRxy4IRtGCEy1ujSp-3-XviugLTC8NLZhugqzZZ2FKeG5Bbv-iOP0HWgdpt0hMnKceW9lvAxri9FrUGQQORZ71UhHbrvxCAscAT5Rf~EqyLFJPwIObZUPZfPqQVhFyn~EVX649GhjkNLhSAEQItk9PiA16xqRtYuP5XnKa3-96qfBN8GDeu6Mc8WqblVKAfXvv3bVw5T3f3I~lvv9-6~Xw8IEyjrMf1Nu8S9cHOms6x3xyeyVzb90k-TB3n5uKfSs-2EsqTywpc7TiKdwiWvHz94onY3G8VPsu0MoRmjX-YQGBHblOXhVYYPGPRBVv1W11H0XENamP-qTb7eQ__&Key-Pair-Id=APKAJLOHF5GGSLRBV4ZA (accessed on 1 June 2020).
- Armstrong-Hélouvry, B.; Dupont, P.; Canudas-De Wit, C. A survey of models, analysis tools and compensation methods for the control of machines with friction. Automatica 1994, 30, 1083–1138. [Google Scholar] [CrossRef]
- Márquez, M.B.S.; Boussaada, I.; Mounier, H.; Niculescu, S.-I. Analysis and Control of Oilwell Drilling Vibrations; Springer Science and Business Media LLC: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Christopoulos, C. The Transmission-Line Modelling Method, Synthesis Lectures on Computational Electromagnetics; Morgan & Claypool. 2006. Available online: https://www.morganclaypool.com/doi/abs/10.2200/S00316ED1V01Y201012CEM027 (accessed on 1 June 2020).
- Whalley, R.; Ebrahimi, M.; Jamil, Z. The torsional response of rotor systems. Proc. Inst. Mech. Eng. J. Mech. Eng. Sci. 2005, 219, 357–380. [Google Scholar] [CrossRef]
- Pavone, D.R.; Desplans, J.P. Application of High Sampling Rate Downhole Measurements for Analysis and Cure of Stick-Slip in Drilling. Soc. Pet. Eng. 1994. [Google Scholar] [CrossRef]
- Jansen, J.D.; Van den Steen, L.; Zachariasen, E. Active Damping of Torsional Drillstring Vibrations With a Hydraulic Top Drive. SPE Drill. Complet. 1995, 10, 250–254. [Google Scholar] [CrossRef]
- Navarro-López, E.M.; Licéaga-Castro, E. Non-desired transitions and sliding-mode control of a multi-DOF mechanical system with stick-slip oscillations. Chaos Solitons Fractals 2009, 41, 2035–2044. [Google Scholar] [CrossRef]







| Name | Symbol | Value | Units |
|---|---|---|---|
| Length of Drill Pipe | 2000 | m | |
| Viscous Damping along with Drill Pipe | 23 | Nms/rad | |
| Viscous Damping along with the bottom hole assembly (BHA) | 50 | Nms/rad | |
| Equivalent Inertia Mass Moment of Drill String | 540.6 | Kgm2 | |
| Drill Pipe Stiffness | 473 | Nms/rad | |
| Propagation Time Associated with Drill Pipe | 0.6281 | s |
| Name | Symbol | Value | Units |
|---|---|---|---|
| Length of Drill Pipe | 5700 | m | |
| Viscous Damping along with Drill Pipe | 85 | Nms/rad | |
| Viscous Damping along with the BHA | 100 | Nms/rad | |
| Equivalent Inertia Mass Moment of Drill String | 655.6 | Kgm2 | |
| Drill Pipe Stiffness | 166 | Nms/rad | |
| Propagation Time Associated with Drill Pipe | 1.79 | s |
| Name | Symbol | Value | Units |
|---|---|---|---|
| Length of Drill Pipe | 7500 | m | |
| Viscous Damping along Drill Pipe | 140 | Nms/rad | |
| Viscous Damping along the BHA | Ceb | 150 | Nms/rad |
| Equivalent Inertia Mass Moment of Drill String | Jes | 711.6 | Kgm2 |
| Drill Pipe Stiffness | Kdp | 126 | Nms/rad |
| Propagation Time Associated with Drill Pipe | td | 2.3553 | s |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Athanasiou, P.; Hadi, Y. Simulation of Oil Well Drilling System Using Distributed–Lumped Modelling Technique. Modelling 2020, 1, 175-197. https://doi.org/10.3390/modelling1020011
Athanasiou P, Hadi Y. Simulation of Oil Well Drilling System Using Distributed–Lumped Modelling Technique. Modelling. 2020; 1(2):175-197. https://doi.org/10.3390/modelling1020011
Chicago/Turabian StyleAthanasiou, Panagiotis, and Yaser Hadi. 2020. "Simulation of Oil Well Drilling System Using Distributed–Lumped Modelling Technique" Modelling 1, no. 2: 175-197. https://doi.org/10.3390/modelling1020011
APA StyleAthanasiou, P., & Hadi, Y. (2020). Simulation of Oil Well Drilling System Using Distributed–Lumped Modelling Technique. Modelling, 1(2), 175-197. https://doi.org/10.3390/modelling1020011




