A Study on the Influence of Tire Speed and Pressure on Measurement Parameters Obtained from High-Speed Tire Uniformity Testing
Abstract
:1. Introduction
2. Tire Uniformity
2.1. Tire Uniformity Definition
2.2. Definition of Tire Uniformity Parameters
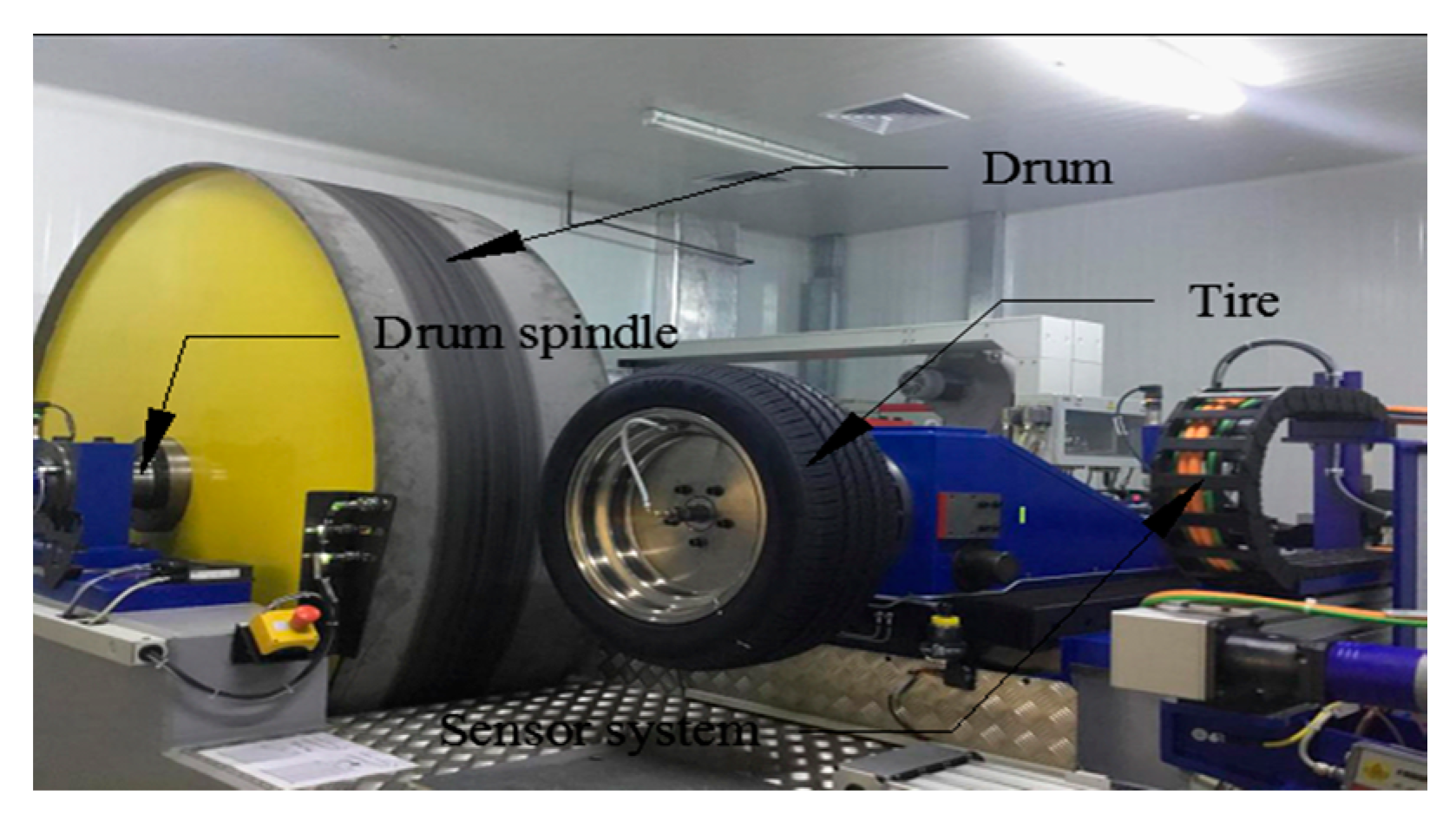
3. High-Speed Uniformity Testing Machine
3.1. Working Principle of the High-Speed Uniformity Testing Machine
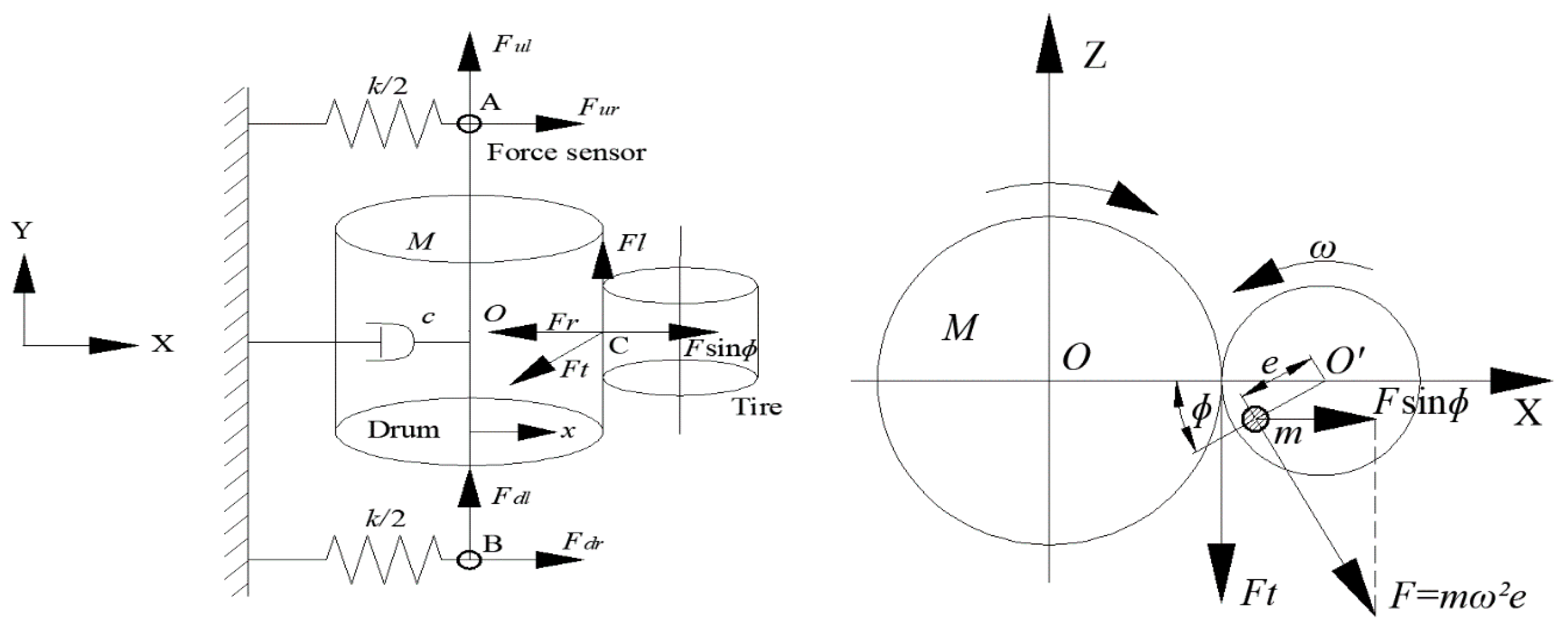
3.2. Mathematical Model of Uniformity Testing Machine
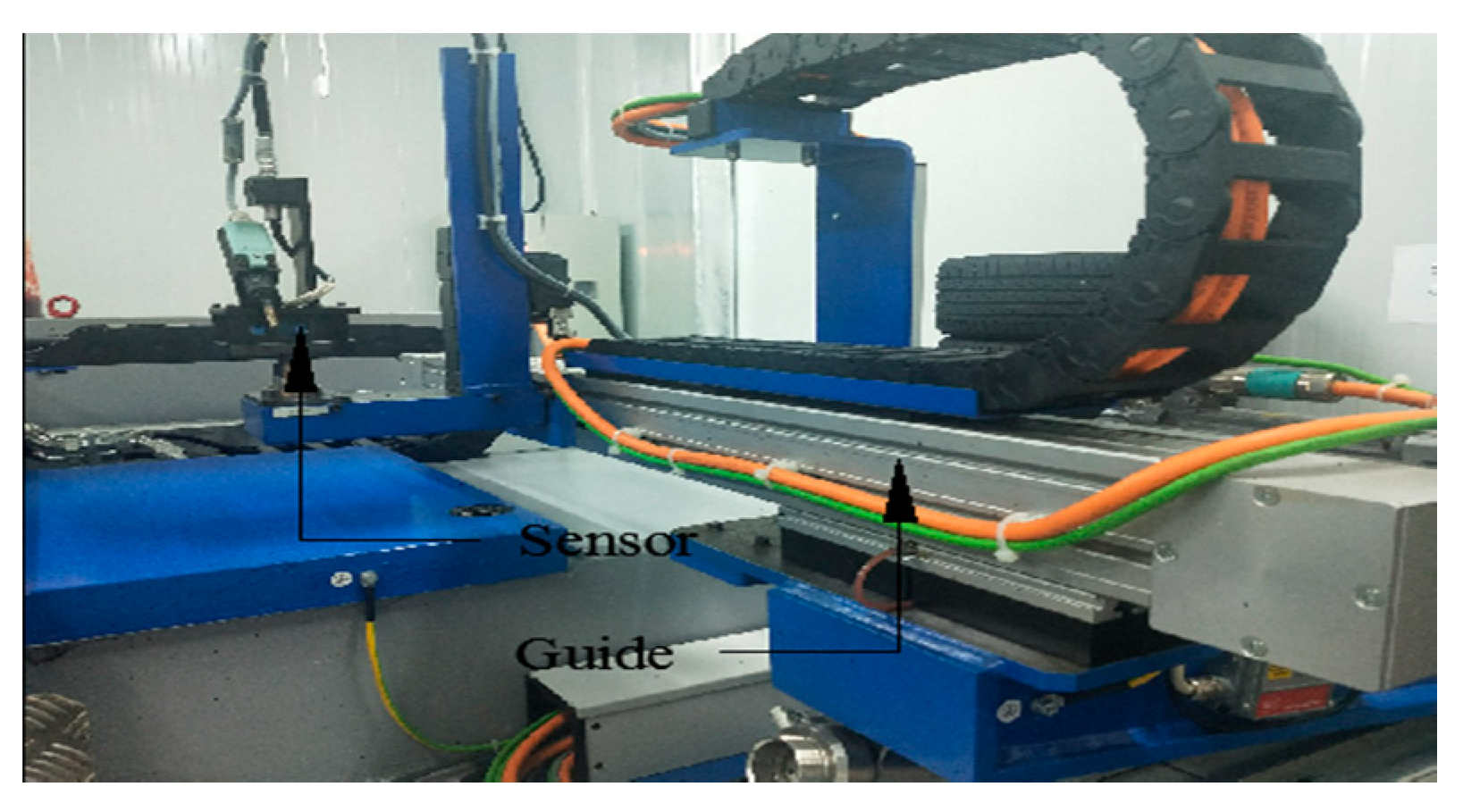
3.3. Uniformity Parameter Signal Acquisition
3.4. Method for Calculating Uniformity Parameters
4. High-Speed Uniformity Test and Analysis of Tires
4.1. Relationship between Velocity and Uniformity Parameters
4.1.1. Speed vs. RFV and H1RFV
4.1.2. Speed vs. LFV and H1LFV
4.1.3. Speed vs. SATV and H1SATV
4.1.4. Speed vs. TFV and H1TFV
4.1.5. Speed vs. OTTV and H1OTTV
4.2. Relationship between Pressure and Uniformity Parameters
4.2.1. Pressure vs. RFV and H1RFV
4.2.2. Pressure vs. LFV and H1LFV
4.2.3. Pressure vs. SATV and H1SATV
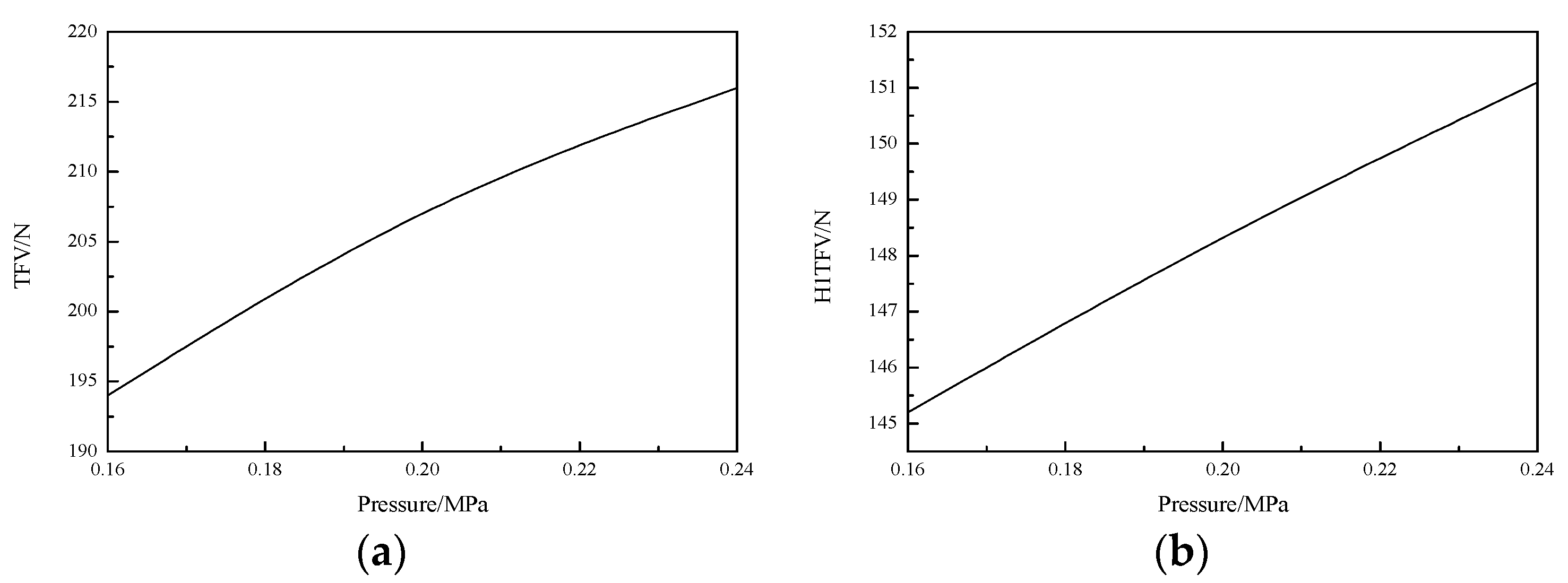
4.2.4. Pressure vs. TFV and H1TFV
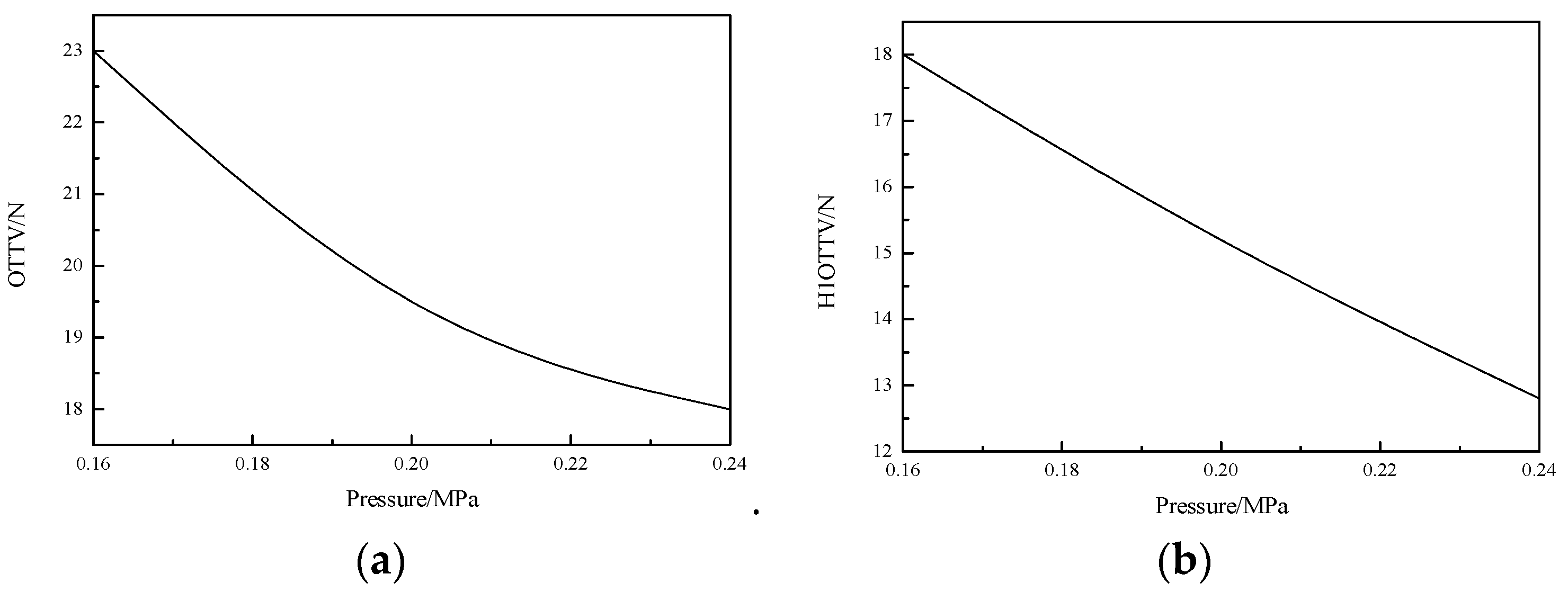
4.2.5. Pressure vs. OTTV and H1OTTV
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Lin, Q.; Ma, T.J. Study on the effect of load and inflation pressure on the measurement of tire uniformity testing machine parameters. Mech. Des. Manuf. 2011, 3, 95–97. [Google Scholar]
- Guan, X.G. Research on Algorithmic Solution and Control Method of Tire Uniformity Testing Machine; Qingdao University of Science and Technology: Qingdao, China, 2006; pp. 3–9. [Google Scholar]
- Zhang, X.L. Research and System Design on Testing and Calibrating Method of Tire High Speed Uniformity; Qingdao University of Science and Technology: Qingdao, China, 2016; pp. 10–16. [Google Scholar]
- Ma, Y.M. Tire uniformity. Rubber Plast. Resour. Util. 2015, 1, 32–40. [Google Scholar]
- Dorfi, H.R. Tyre non-uniformities: Comparison of analytical and numerical tyre models and correlation to experimentally measured data. Veh. Syst. Dyn. 2005, 43, 223–240. [Google Scholar] [CrossRef]
- Ge, H. Study on Matching of Tire Inhomogeneity and Chassis Dynamic Characteristics; Hefei University of Technology: Hefei, China, 2013; pp. 11–15. [Google Scholar]
- Stutts, D.S.; Krousgrill, C.M.; Soedel, W. Parametric excitation of tire-wheel assemblies by a stiffness non-uniformity. J. Sound Vib. 1995, 179, 499–512. [Google Scholar] [CrossRef]
- Ma, M.H. Application Research of Tire Uniformity Testing Machine. China Rubber/Plast. Technol. Equip. 2012, 6, 22–29. [Google Scholar]
- Song, B. Design and Finite Element Analysis of Tire High Speed Uniformity Testing System; Qingdao University of Science and Technology: Qingdao, China, 2015; pp. 26–28. [Google Scholar]
- Jiang, X.Z. High speed tire uniformity testing machine. Adv. Rubber Technol. 2006, 32, 32–38. [Google Scholar]
- Hang, B.L.; Guan, X.G. Data processing and management system of tire uniformity. China Rubber/Plast. Technol. Equip. 2006, 22, 59–61. [Google Scholar]
- Lv, P.W. Study on the Consistency of Measuring Parameters of Different Tire Uniformity Testing Machines; Qingdao University of Science and Technology: Qingdao, China, 2010; pp. 12–15. [Google Scholar]
- Hang, B.L.; Duan, Z.Y. Development of a Two-Dimensional Force Model and Data Analysis for the Tire Uniformity Testing Machine. Adv. Mater. Res. 2011, 221, 343. [Google Scholar] [CrossRef]
- Hang, B.L.; Zhao, M.D. Analysis of influencing factors of radial characteristic parameters of tire uniformity. Rubber Ind. 2011, 58, 146–149. [Google Scholar]
- He, X. Tire/Wheel Uniformity and Related Vehicle Vibration Analysis; Hefei University of Technology: Hefei, China, 2015; pp. 6–10. [Google Scholar]
- Meijuan, Y.; Yuankan, D.; Gall, R.; Rodriguez, N.D. Effects of variations in geometry and material on the non-uniformity of tires. Tire Sci. Technol. 2001, 29, 56–64. [Google Scholar] [CrossRef]
- Yu, S.Y. Tire uniformity and its influencing factors. Tire Ind. 2008, 8, 463–469. [Google Scholar]
- Huo, Z.D.; Jiao, S.W.; Ye, Q. Influence of Curing Process on Radial Runout Value of Truck and Bus Radial Tire. Tire Ind. 2018, 38, 760–761. [Google Scholar]



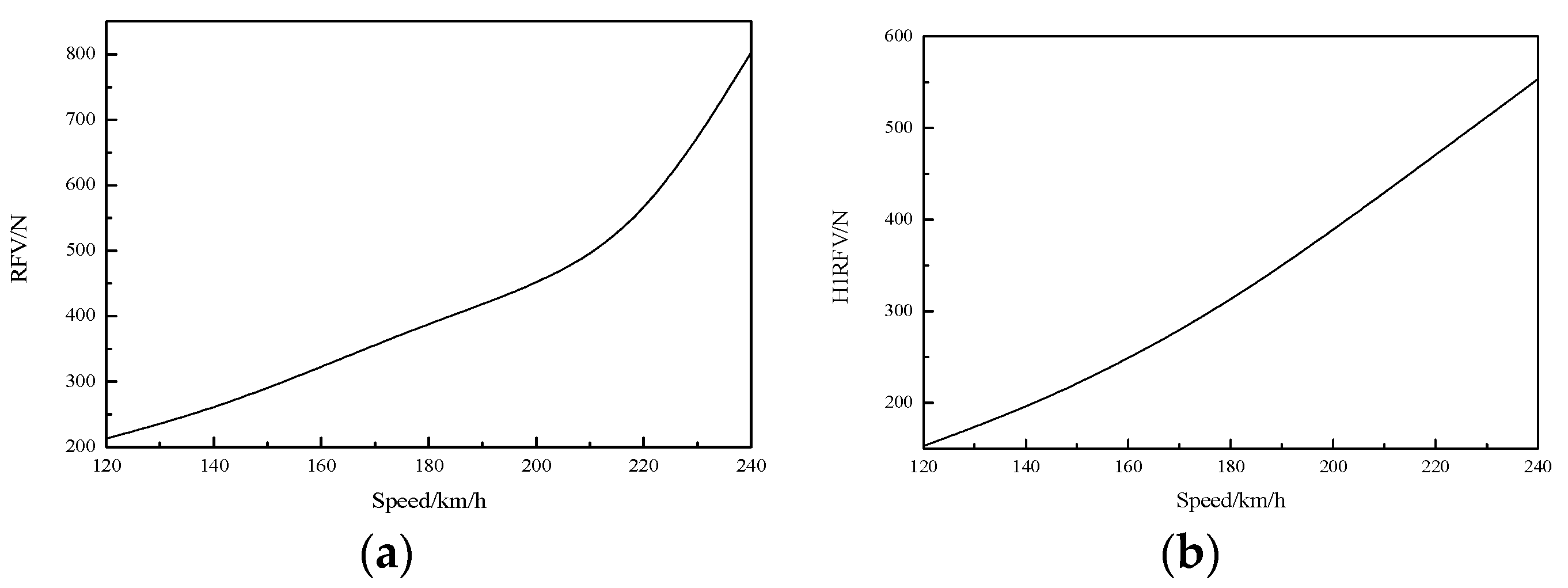

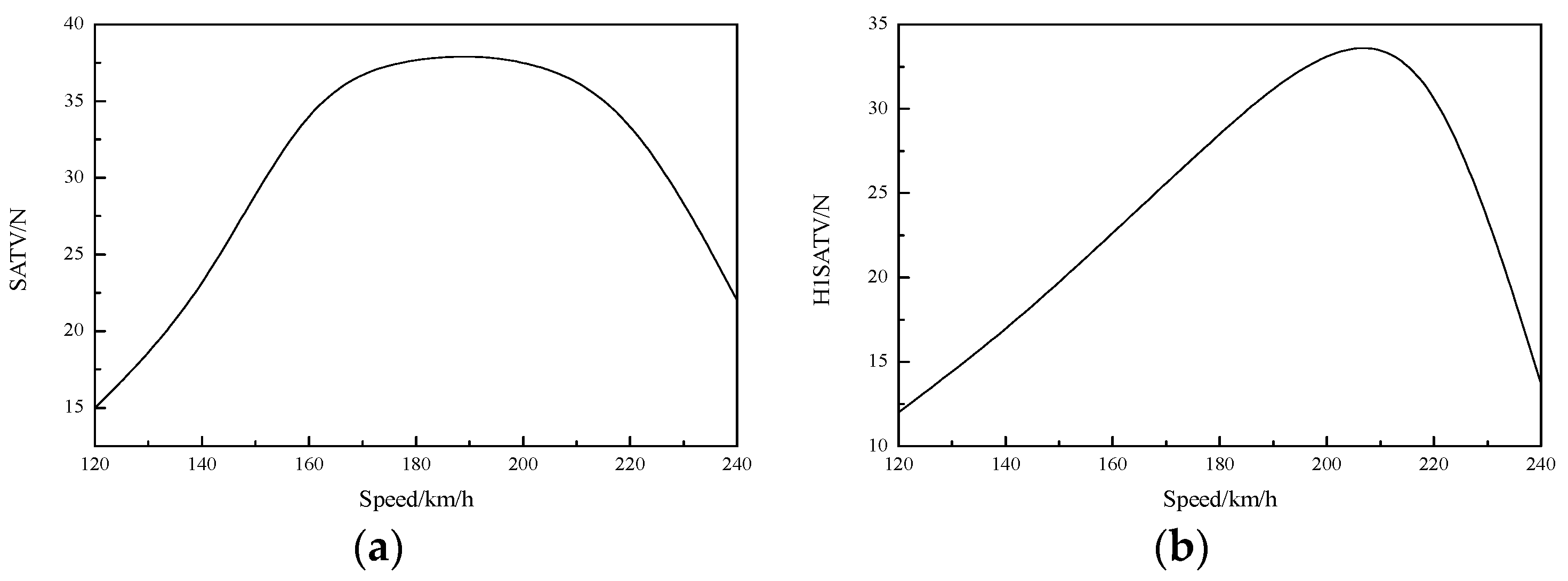
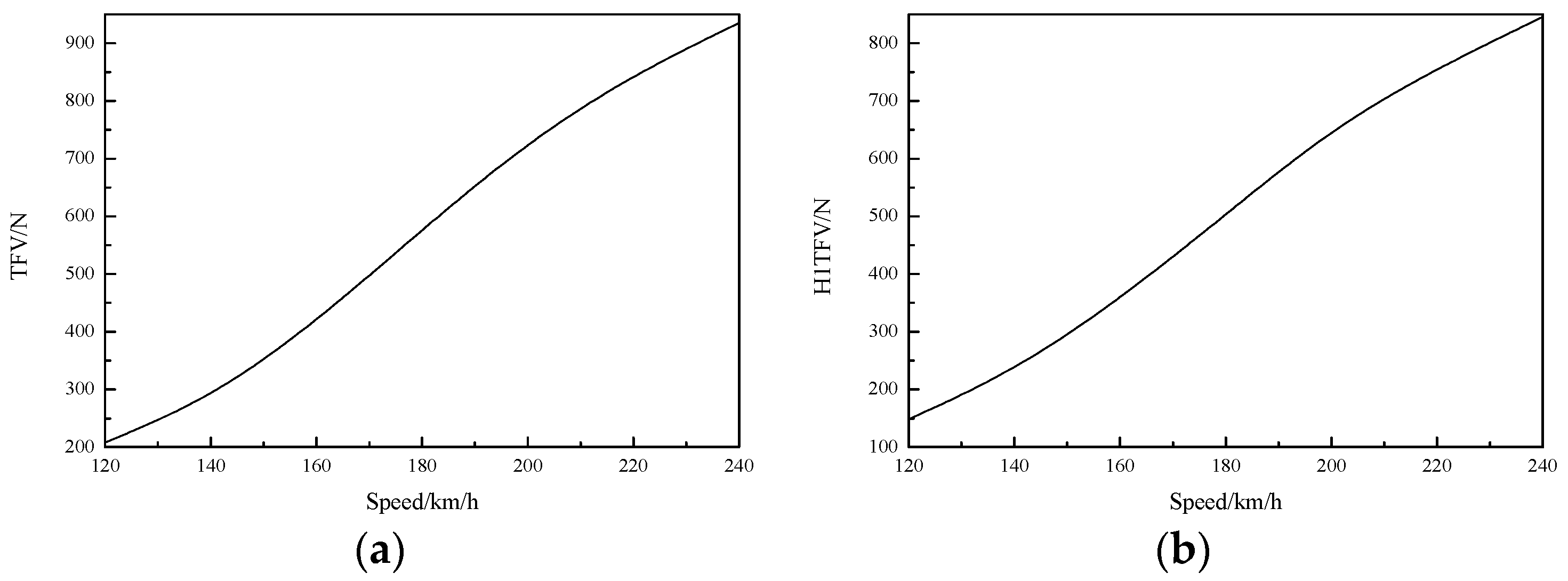

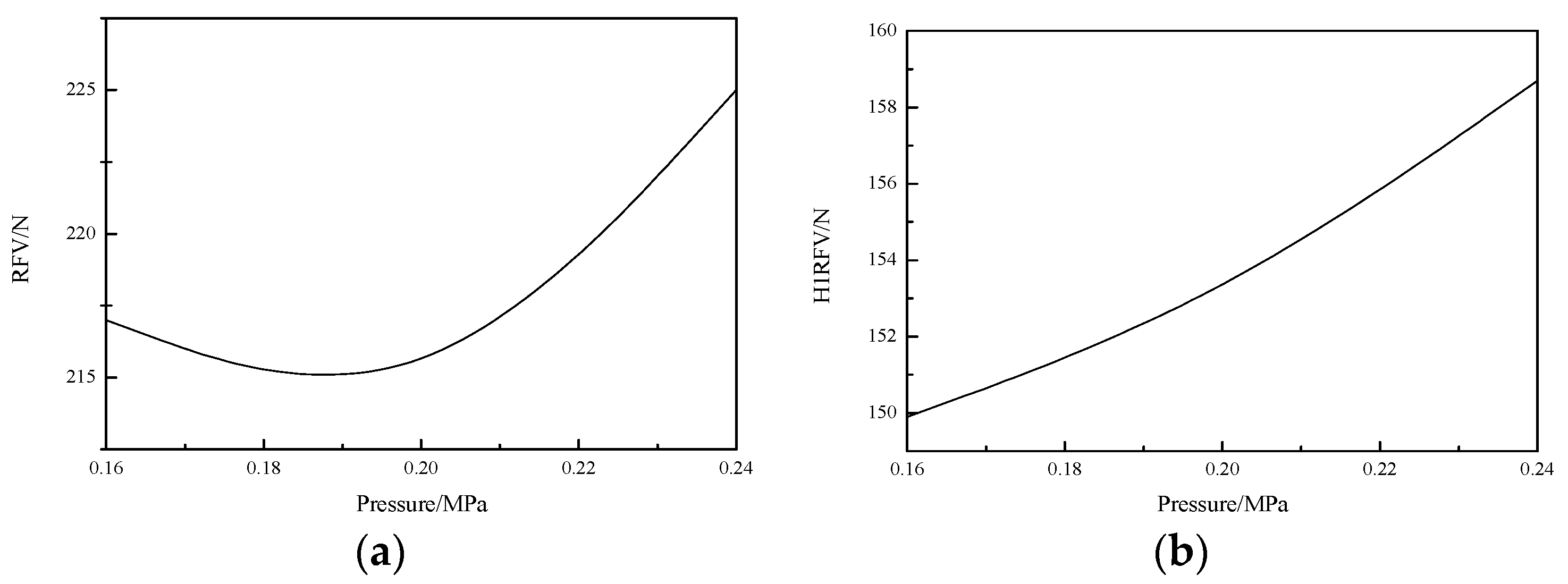

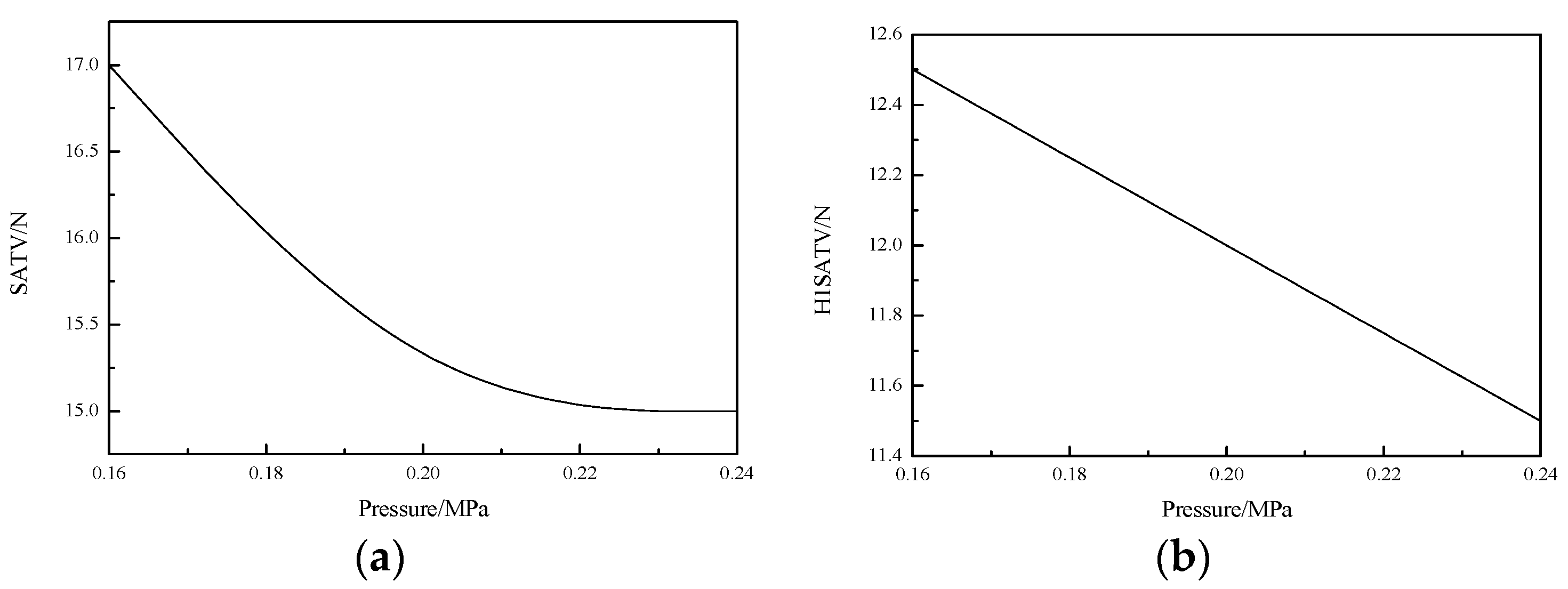
| Speed (km/h) | RFV (N) | LFV (N) | TFV (N) | H1RFV (N) | H1LFV (N) | H1TFV (N) | SATV (N) | OTTV (N) | H1SATV (N) | H1OTTV (N) |
|---|---|---|---|---|---|---|---|---|---|---|
| 120 | 213 | 59 | 208 | 152.9 | 25 | 148.4 | 15 | 19 | 12 | 15.1 |
| 140 | 258 | 43 | 285 | 194.4 | 27.9 | 232.2 | 22 | 27 | 16.8 | 20.3 |
| 160 | 322 | 74 | 417 | 247.1 | 33.2 | 356.4 | 36 | 31 | 22.6 | 27 |
| 180 | 390 | 67 | 577 | 310.9 | 39.2 | 503.2 | 38 | 41 | 28.6 | 35.7 |
| 200 | 446 | 44 | 729 | 388.5 | 34.6 | 652.1 | 38 | 58 | 34 | 48.6 |
| 220 | 538 | 39 | 846 | 470.7 | 11.8 | 757 | 35 | 71 | 34 | 70.5 |
| 240 | 803 | 149 | 935 | 553.4 | 128.8 | 845.5 | 22 | 114 | 13.7 | 114.5 |
| Pressure (MPa) | RFV (N) | LFV (N) | TFV (N) | H1RFV (N) | H1LFV (N) | H1TFV (N) | SATV (N) | OTTV (N) | H1SATV (N) | H1OTTV (N) |
|---|---|---|---|---|---|---|---|---|---|---|
| 0.16 | 217 | 65 | 194 | 149.9 | 34.3 | 145.2 | 17 | 23 | 12.5 | 18 |
| 0.20 | 213 | 59 | 208 | 152.9 | 25 | 148.4 | 15 | 19 | 12 | 15.1 |
| 0.24 | 225 | 52 | 216 | 158.7 | 21.6 | 151.1 | 15 | 18 | 11.5 | 12.8 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Du, M.; Sun, P.; Zhou, S.; Huang, H.; Zhu, J. A Study on the Influence of Tire Speed and Pressure on Measurement Parameters Obtained from High-Speed Tire Uniformity Testing. Vehicles 2020, 2, 559-573. https://doi.org/10.3390/vehicles2030031
Du M, Sun P, Zhou S, Huang H, Zhu J. A Study on the Influence of Tire Speed and Pressure on Measurement Parameters Obtained from High-Speed Tire Uniformity Testing. Vehicles. 2020; 2(3):559-573. https://doi.org/10.3390/vehicles2030031
Chicago/Turabian StyleDu, Meng, Pengfei Sun, Shuiting Zhou, Hongwu Huang, and Jie Zhu. 2020. "A Study on the Influence of Tire Speed and Pressure on Measurement Parameters Obtained from High-Speed Tire Uniformity Testing" Vehicles 2, no. 3: 559-573. https://doi.org/10.3390/vehicles2030031
APA StyleDu, M., Sun, P., Zhou, S., Huang, H., & Zhu, J. (2020). A Study on the Influence of Tire Speed and Pressure on Measurement Parameters Obtained from High-Speed Tire Uniformity Testing. Vehicles, 2(3), 559-573. https://doi.org/10.3390/vehicles2030031




