A Review of the Contribution of Satellite Altimetry and Tide Gauge Data to Evaluate Sea Level Trends in the Adriatic Sea within a Mediterranean and Global Context
Abstract
1. Introduction
2. A View on the Regional Sea Level Variations and Trends for the Eastern Adriatic Sea Coast within the Mediterranean Context
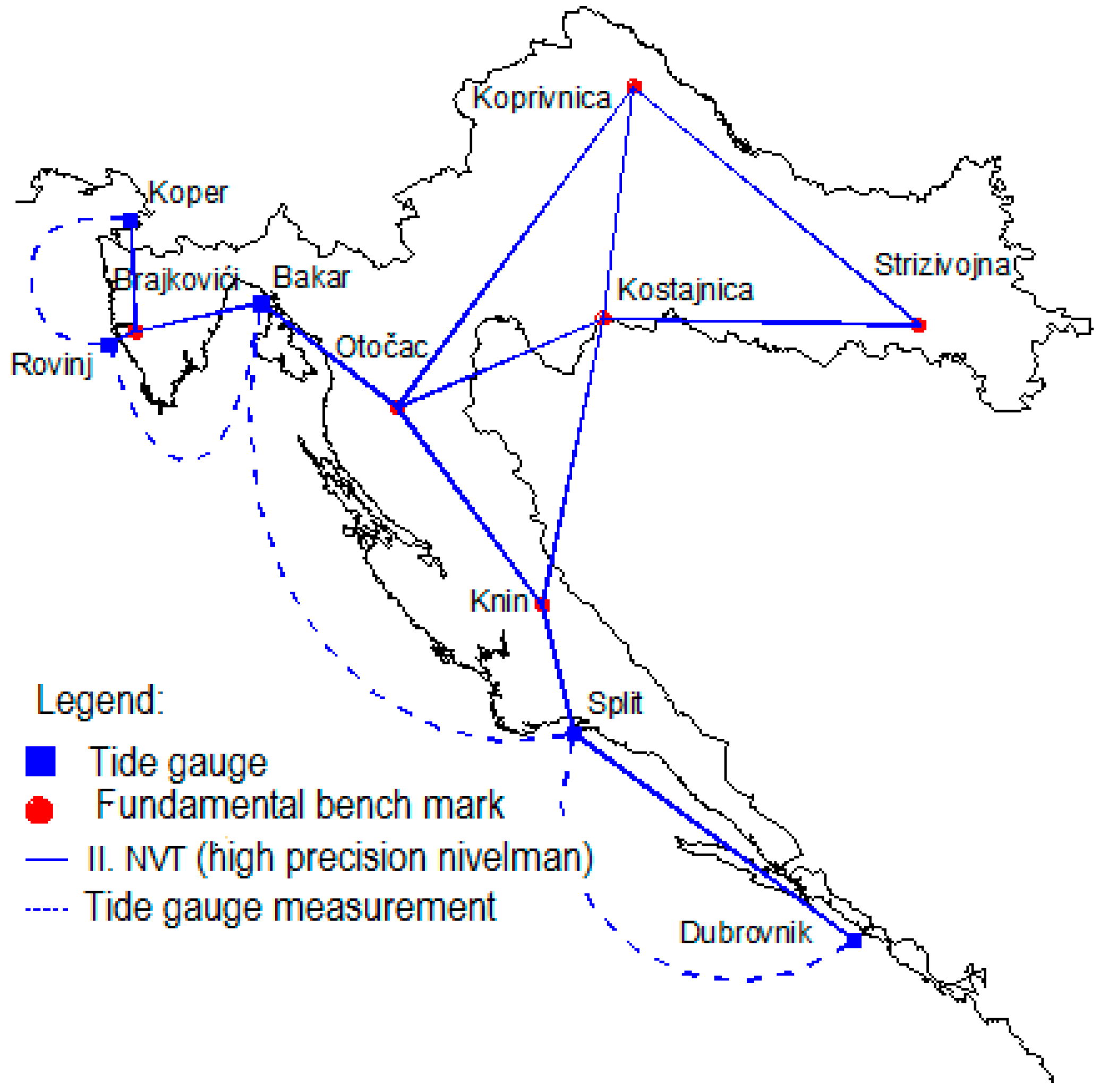
2.1. The Mean Sea Level Determination and Its Use for Setting up a New Height Reference System on the Territory of Croatia
2.1.1. The First Published Paper on the Mean Sea Level in Bakar
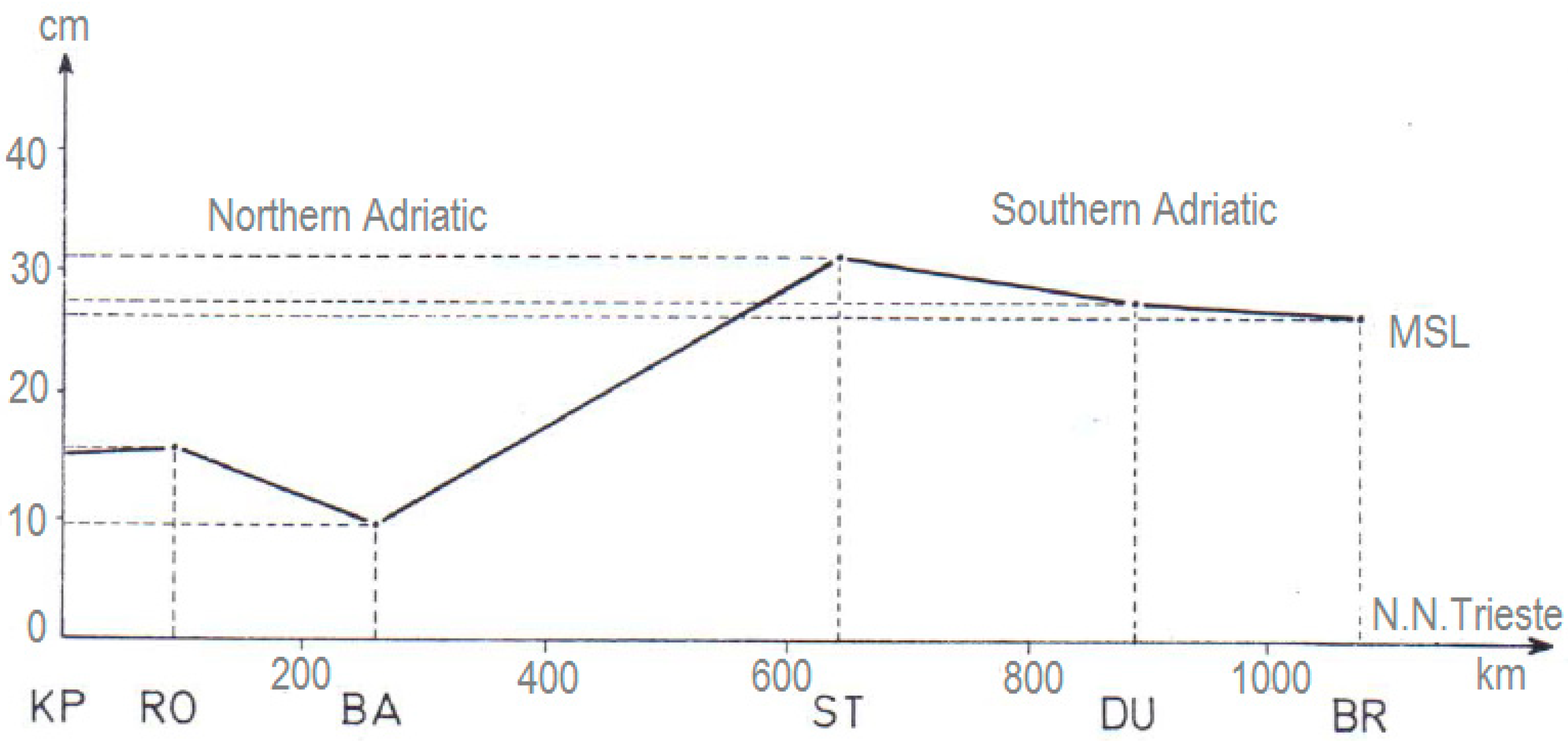
2.1.2. Comparison of the Mean Sea Levels on the Eastern Adriatic Coast
“By knowing these differences, the sea levels of all the tide gauges can be reduced to the basic level of one of them, and in that way, it can be determined whether the mean sea level of the Adriatic is ‘flat’ or not, and if not, what is it”—Kasumović asked himself in [15].
2.1.3. A New Reference Height System of the Republic of Croatia
2.2. Satellite Altimetry Principles and an Example of Sea Level Monitoring on the Eastern Adriatic Sea
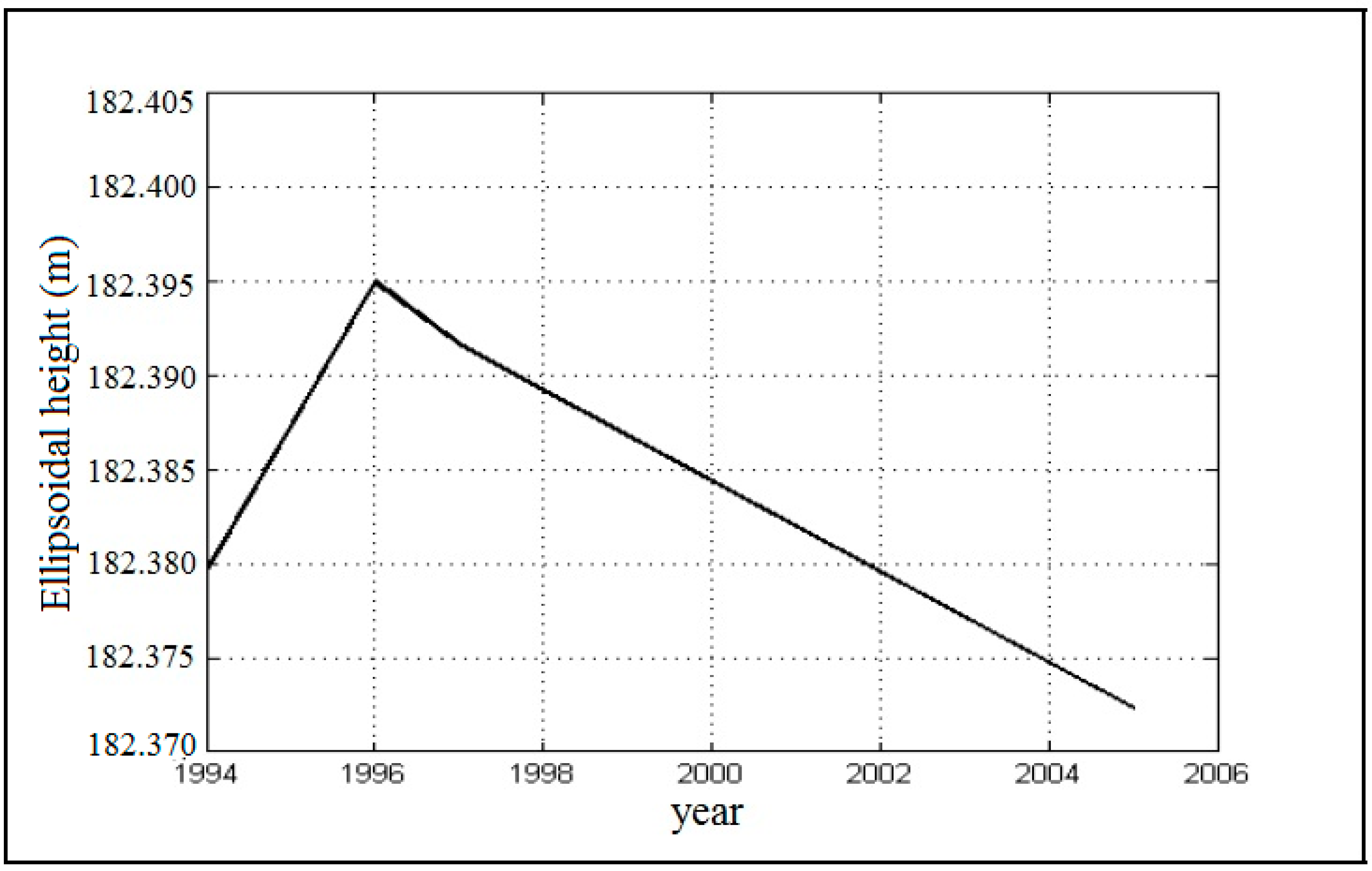
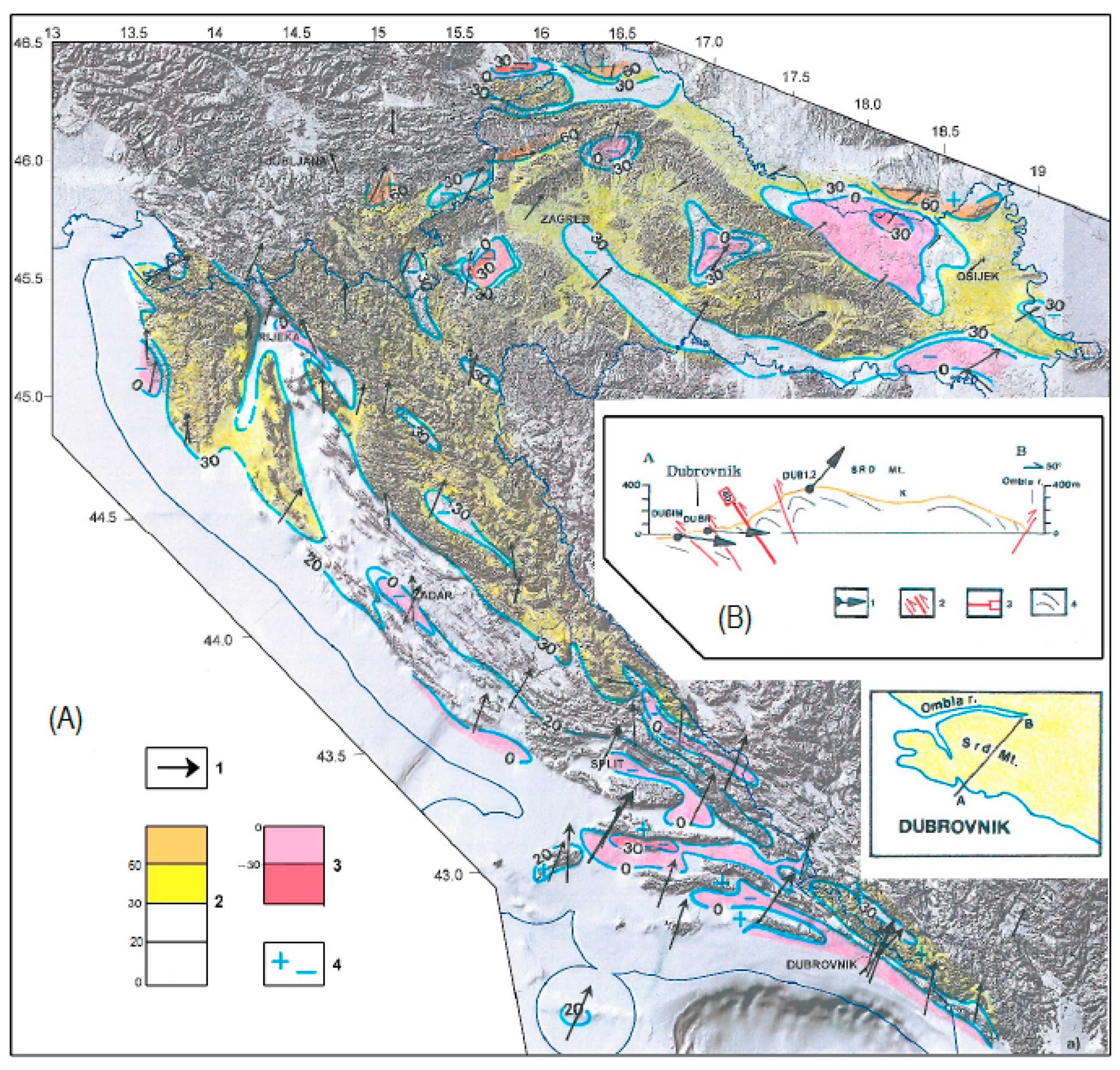
2.3. Determination of Vertical Motion of the Adriatic Crustal Microplate
2.3.1. Application of Geodetic Modelling and GPS (Global Position System)/GNSS (Global Navigation Satellite System) Observations for Determination of Crustal Motions
2.3.2. Application of Satellite Altimetry and TG Sea Level Data for Estimation of Absolute Crustal Motion
2.4. Some Studies of Sea Level Temporal Evolution According to Tide Gauge Observations on the Eastern Adriatic Coast in the Adriatic–Mediterranean Context
2.4.1. Sea Level Analysis Based on Geomorphological Approach and TG Data
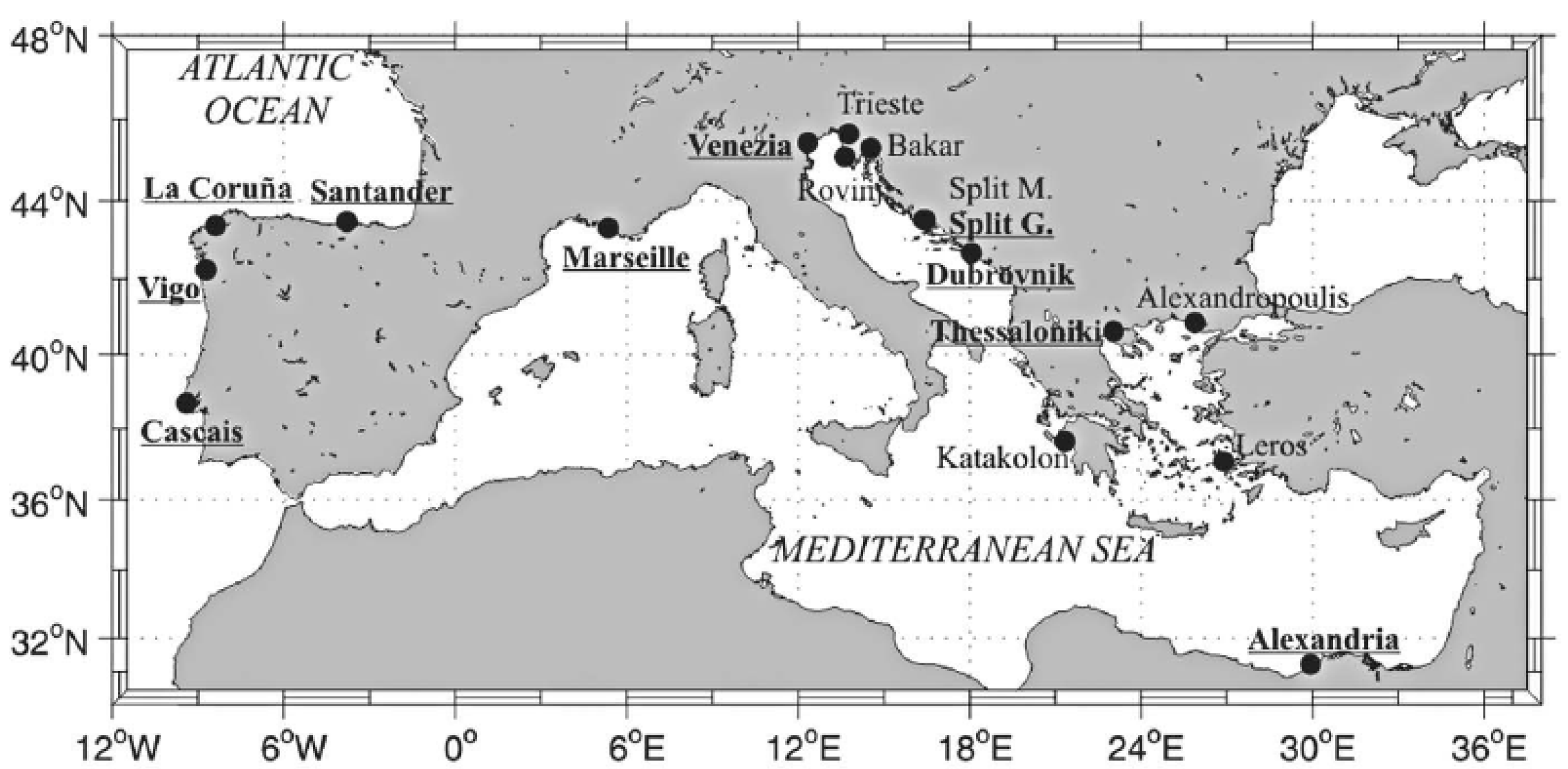
2.4.2. Examples of Sea Level Trend Analysis Using Both Satellite Altimetry and Tide Gauge Data
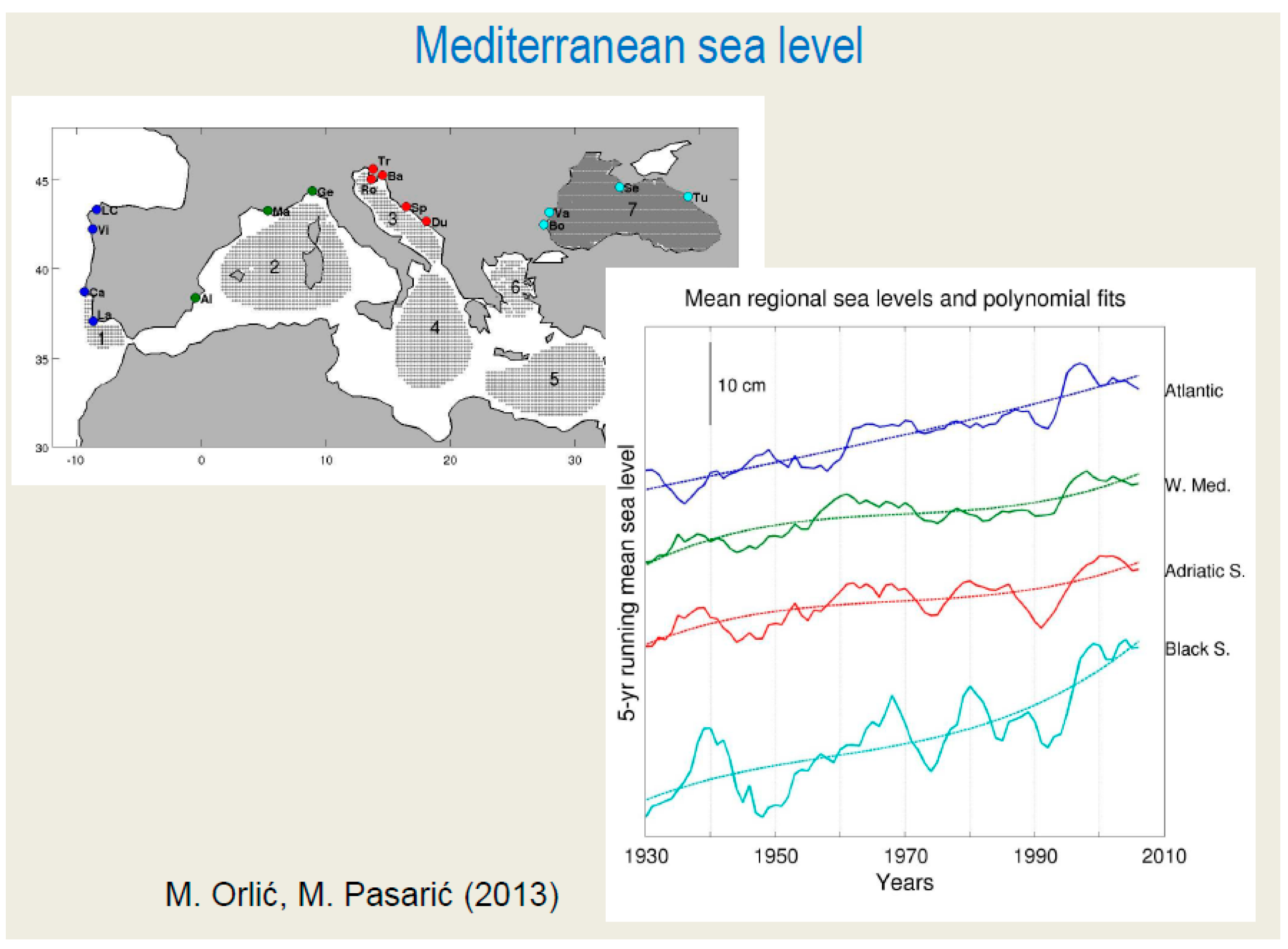
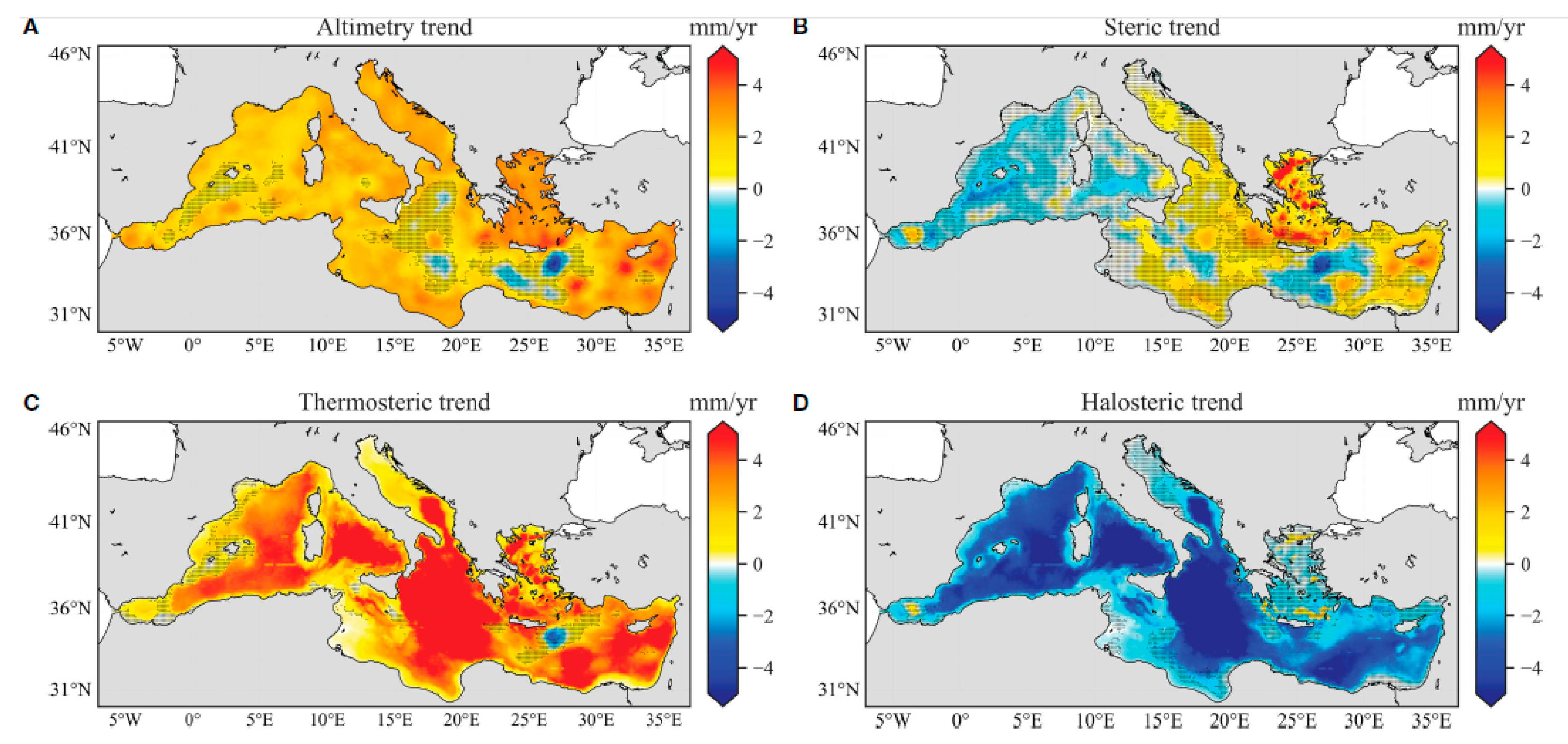
2.4.3. Spatial and Temporal Variability of Sea Level Trends in the Mediterranean Sea for the Period 1993–2019
3. A View on Global Sea Level Trends and Variations
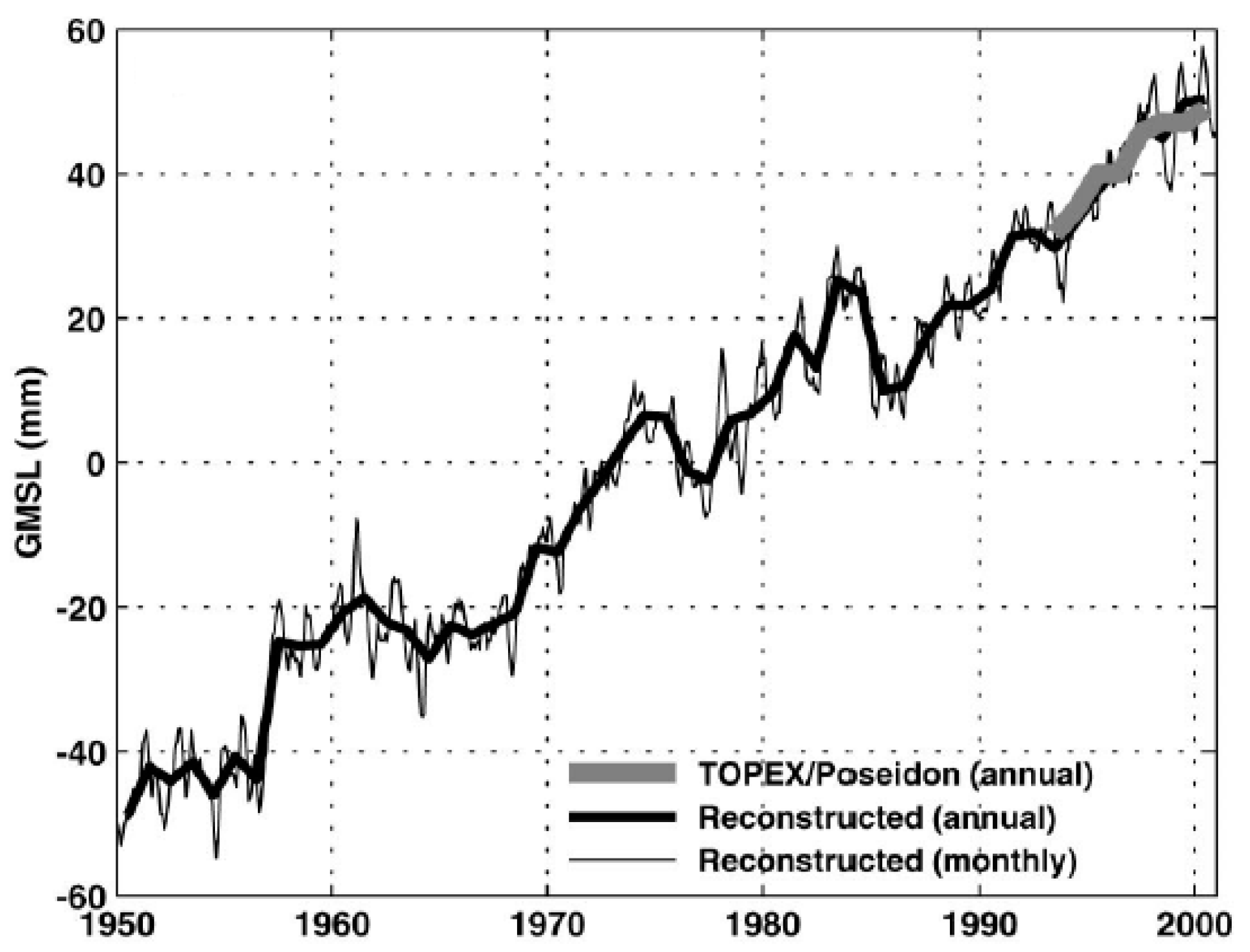
3.1. Estimates of the Regional and Global Sea Level Rise over the 1950–2000 Period
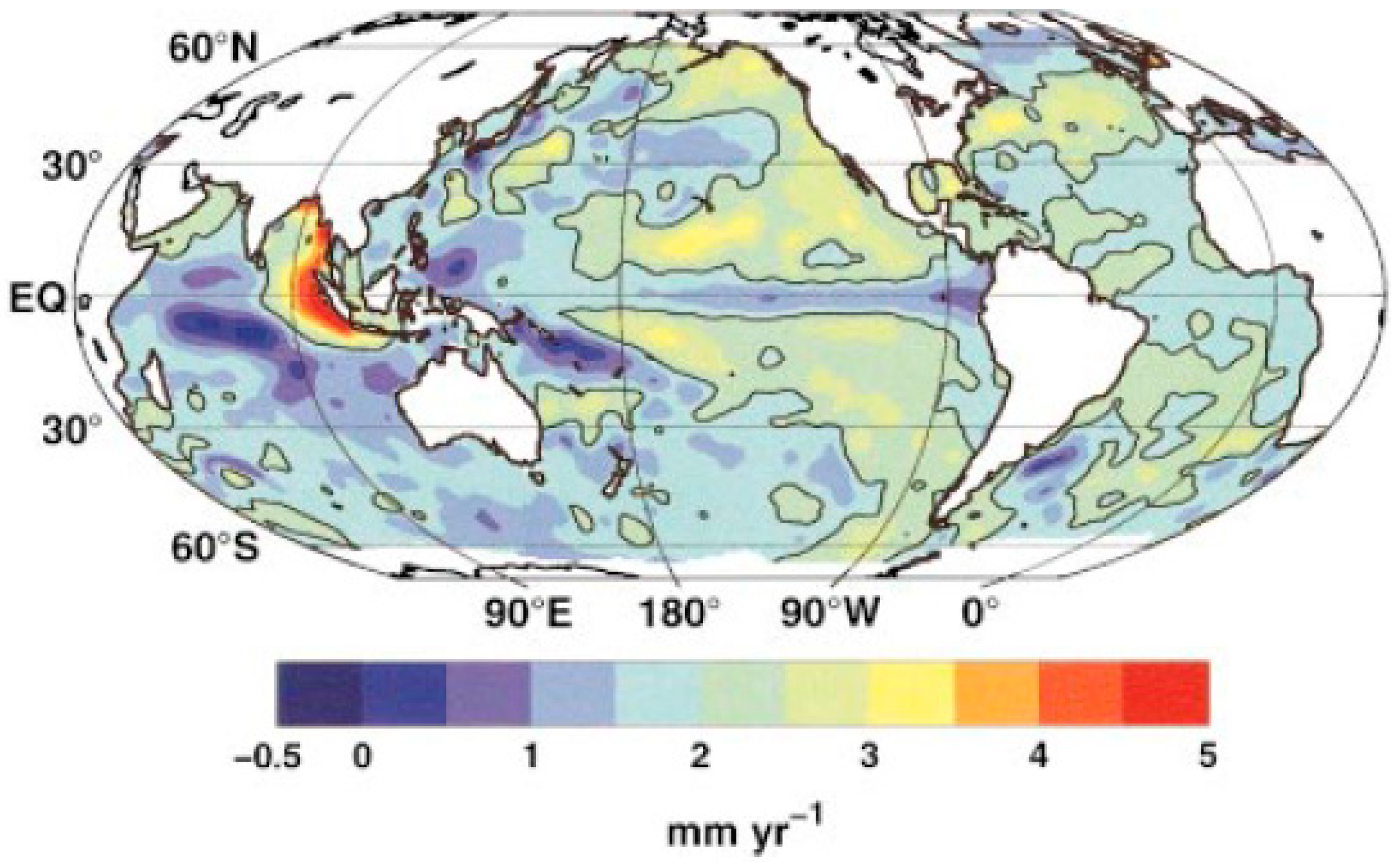
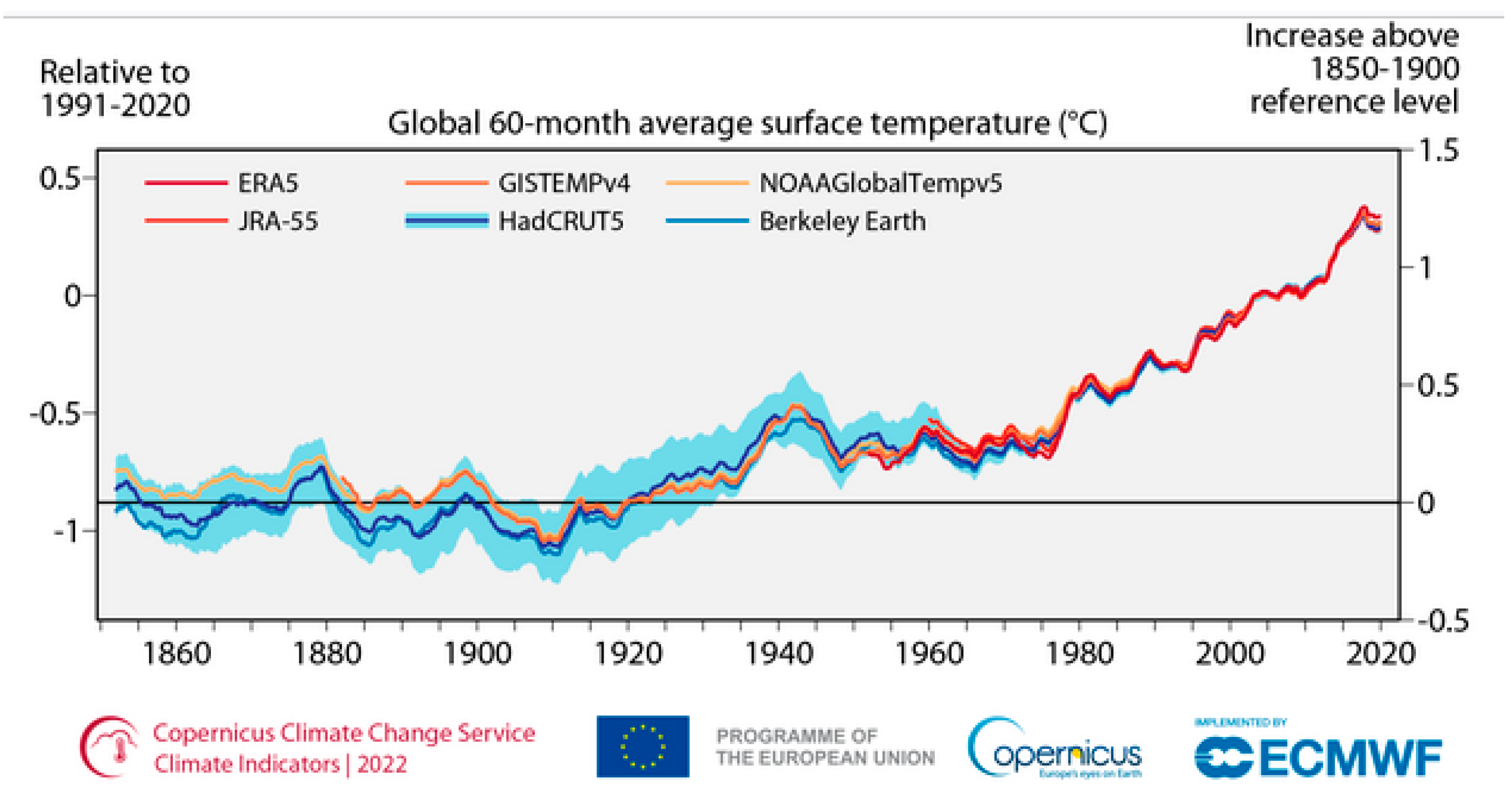
3.2. Sea Level Rise from the Late 19th to the Early 21st Century
3.3. Persistent Acceleration in Global Sea Level Rise since 1960
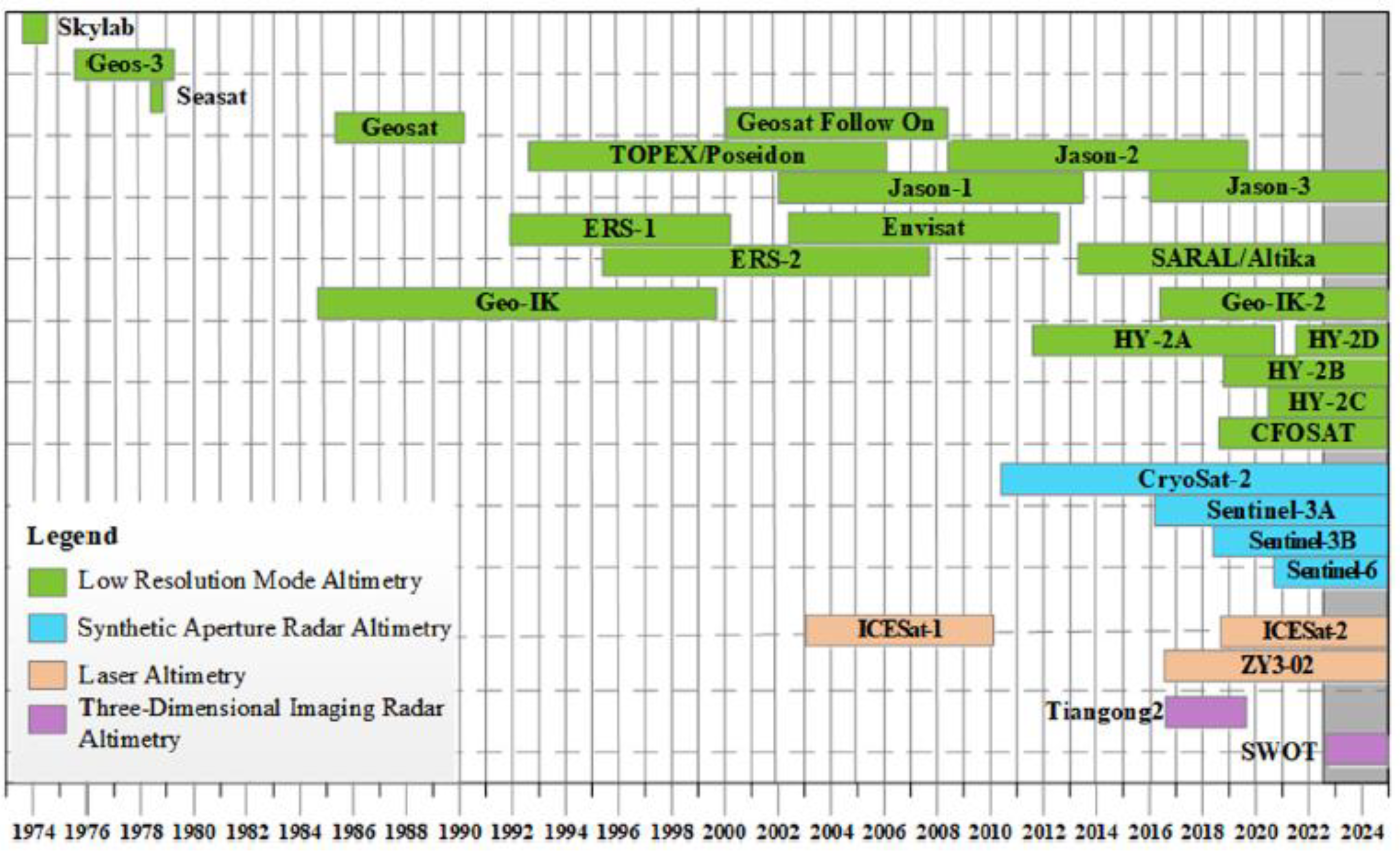
3.4. Recent Achievements in Satellite Altimetry Era
3.5. Semi-Empirical versus Process-Based Approaches to Projecting Future Sea Level Rise
“…found no such linear relationship and that there was considerable uncertainty in the prediction of future sea level rise…”
4. Conclusions
- (1)
- Tide gauge network establishment and data processing standards, including geodetic normal-null (N.N.), i.e., reference geoid surface level determination as reference vertical datums on the eastern Adriatic coast, are discussed.
- (2)
- It has been shown that the quality of TG data is satisfactory for the tide gauge network on the eastern Adriatic coast in the 20th century.
- (3)
- A redefinition of a new geodetic N.N. has recently been recommended to the relevant governmental authorities of Croatia instead of the used geodetic N.N. for the Adriatic Sea based on the sea level data in Trieste for 1875 only.
- (4)
- A comprehensive modelling of Earth’s crustal movements of the Adriatic micro-plate has also been presented.
- (5)
- A rising sea level trend was qualitatively detected in the broader Adriatic Sea area after interannual variations were removed (Figure 15).
- (6)
- On the bases of TG long-term sea level records, after removing crustal movement biases, spatially homogeneous sea level trends, on average about 2.43 mm/year, have been estimated for the eastern Adriatic coast for the period 1974–2018 (Table 4).
- (7)
- On the bases of satellite altimetry data for the whole Adriatic for the period 1993–2019, a positive sea level trend of about 2.6 mm/year was calculated as well (Section 2.4.3, second paragraph).
- (8)
- Application of Empirical Orthogonal Functions (EOFs) for reconstruction of sea level on a regular network (Section 3.1, second paragraph).
- (9)
- For the period 1880–2009, regional sea level rates from about −0.5 mm/year to 5 mm/year (Figure 18) and GMSL rates of about 1.5 mm/year (Section 3.2, second paragraph) were achieved and emphasized the complementarity and value of both satellite and tide gauge sea level data.
- (10)
- The possibility to compare the average mean sea level rise rate of 2.6 mm/year for the Adriatic Sea (Section 2.4.3, second paragraph) for the period 1993–2019 with the GMSL rise rate of 3.3 mm/year (Section 3.4, second paragraph) for the period 1993–2022 can be considered a big achievement.
- (11)
- Semi-empirical estimations of future sea level projections have been shown (Section 3.5). Unfortunately, the same sea level estimation procedure cannot be directly applied within semi-enclosed basins at mid-latitudes, such as the Adriatic and the Mediterranean Sea, at which the halosteric opposite effect was influential, at least in the period 1993–2019 (Section 2.4.3).
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Correction Statement
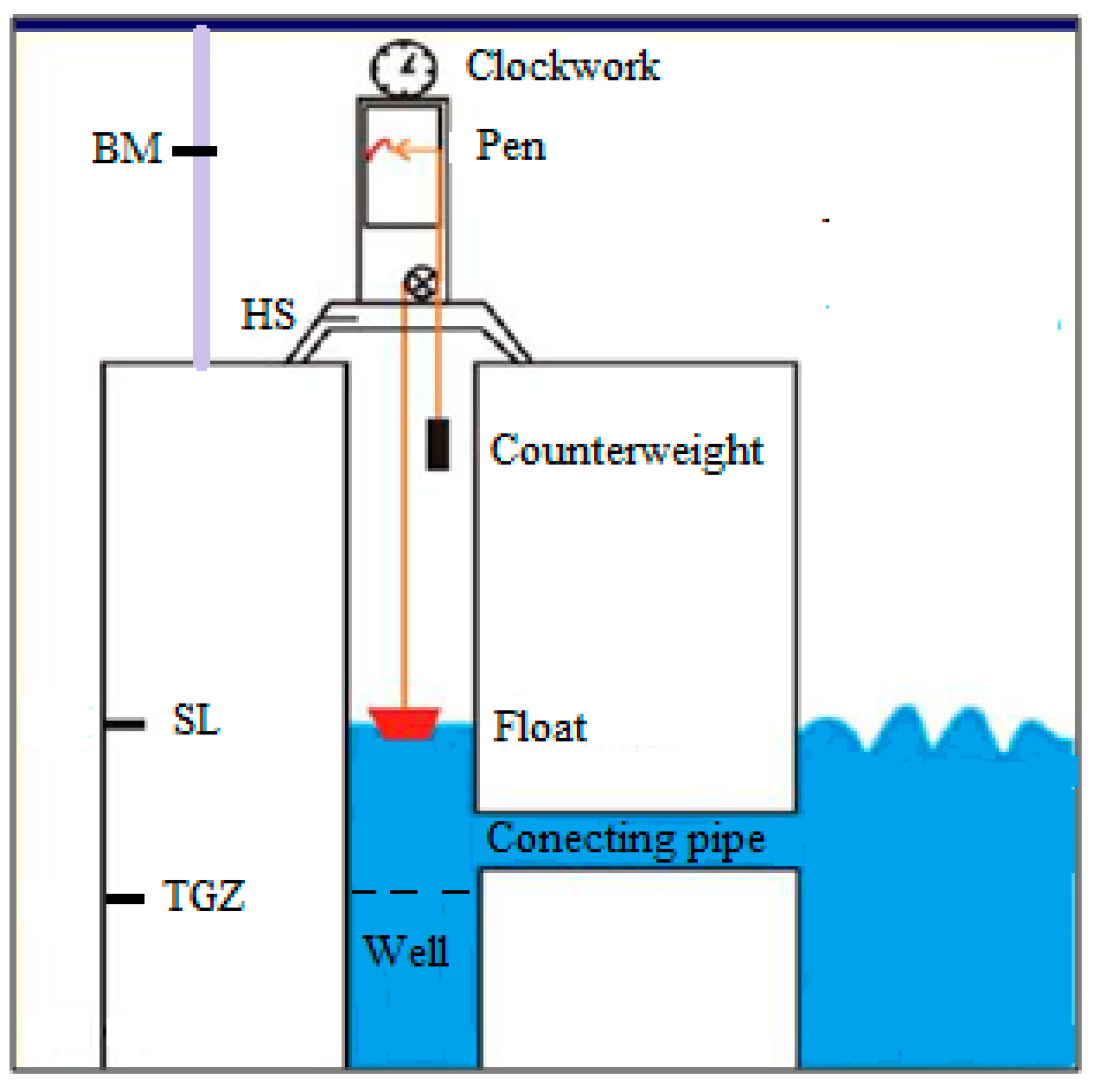
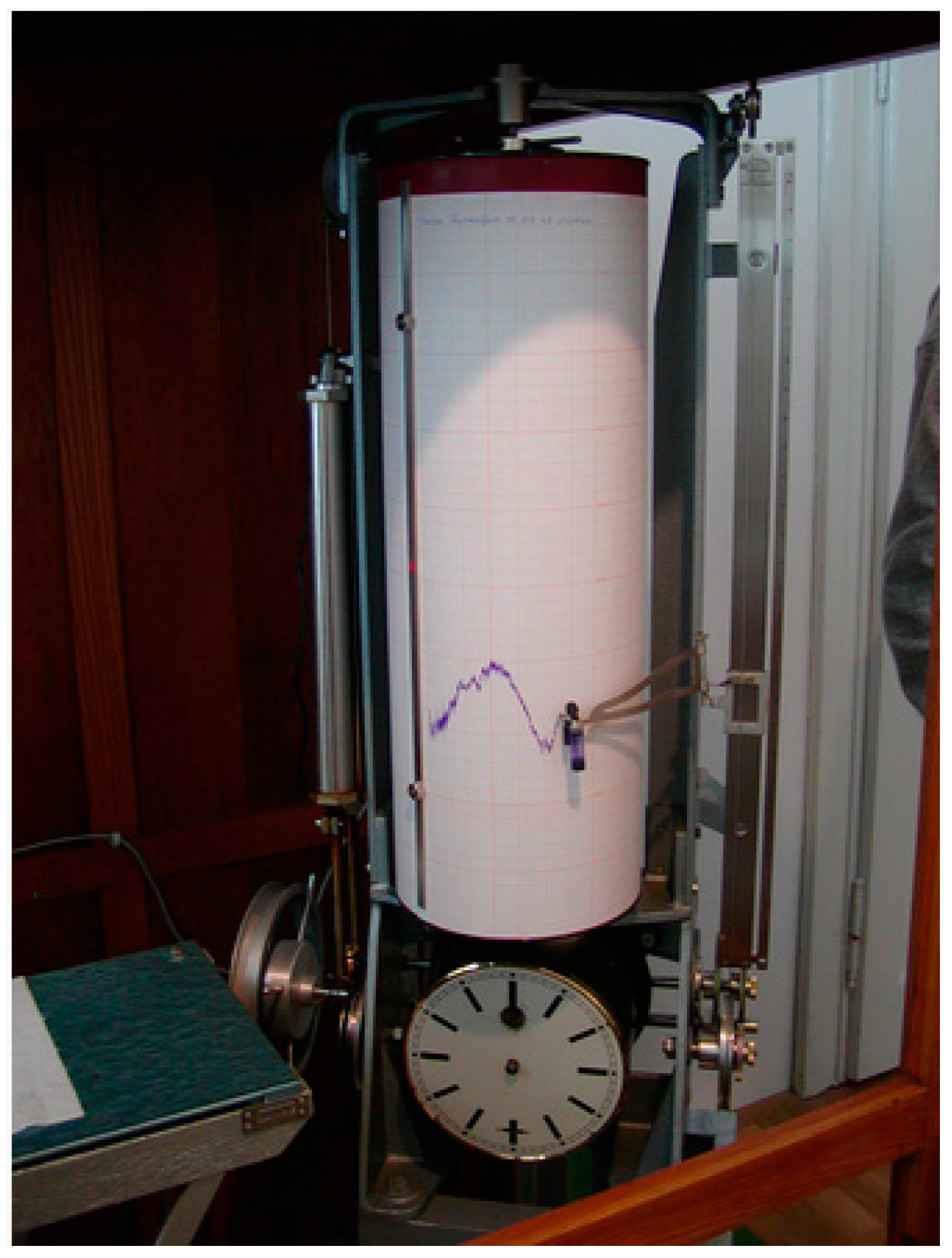
Appendix A. A Scheme of a Conventional Tide Gauge Installed in the Town of Bakar in 1929
References
- Church, J.A.; Woodworth, P.L.; Aarup, T.; Wilson, W.S. Understanding Sea Level Rise and Variability; John Wiley & Sons: Oxford, UK, 2010. [Google Scholar]
- Orlić, M. An Introduction to Physical Oceanography; Element: Zagreb, Croatia, 2022. (In Croatian) [Google Scholar]
- Seeber, G. Satellite Geodesy; Walter de Gruyter GMbH & Co.: Berlin, Germany, 2003. [Google Scholar]
- Rožić, N. Croatian Height Reference System; Faculty of Geodesy University of Zagreb: Zagreb, Croatia, 2019; 256p. [Google Scholar]
- Domijan, N.; Leder, N.; Čupić, S. Vertical datums of the Republic of Croatia. In Proceedings of the Third Croatian Congress on Cadastre, Croatian Geodetic Society, Zagreb, Croatia, 7–9 March 2005; Available online: https://www.hgd1952.hr/images/com_gallery_wd/uploads/3_KongresKatastar/3_KongresKatastar.pdf (accessed on 1 October 2023). (In Croatian).
- Church, J.A.; White, N.J. A 20th century acceleration in global sea level rise. Geophys. Res. Lett. 2006, 33, 1–4. [Google Scholar] [CrossRef]
- Guo, J.; Hwang, C.; Deng, X. Editorial: Application of Satellite Altimetry in Marine Geodesy and Geophysics. Front. Earth Sci. 2022, 10, 910562. [Google Scholar] [CrossRef]
- Global Climate Observing System—GCOS. Implementation Plan for the Global Observing System in Support of the UnitedNations Framework Convention on Climte Change—UNFCCC (2010 Update). GCOS Rep.138. Available online: https://goosocean.org/index.php?option=com_oe&task=viewDocumentRecord&docID=6176 (accessed on 1 November 2023).
- Bojinski, S.; Verstraete, M.; Peterson, T.C.; Richter, C.; Simons, A.; Zemp, M. The concept of essential climate variables in support of climate research, application and policy. Bull. Am. Meteorol. Soc. 2014, 95, 1431–1443. [Google Scholar] [CrossRef]
- Kamranzad, F.; Memarian, H.; Zare, M. Earthquake Risk Assessment for Tehran, Iran. ISPRS Int. J. Geo-Inf. 2020, 9, 430. [Google Scholar] [CrossRef]
- Laurenti, L.; Tinti, E.; Galasso, F.; Franco, L.; Marone, C. Deep learning for laboratory earthquake prediction and autoregressive forecasting of fault zone stress. Earth Planet Sci. Lett. 2020, 598, 117825. [Google Scholar] [CrossRef]
- Lazos, I.; Sboras, S.; Chousianitis, K.; Bitharis, S.; Mouzakiotis, E.; Karastathis, V.; Pikridas, C.; Fotiou, A.; Galanakis, D. Crustal deformation analysis of Thessaly (central Greece) before the March 2021 earth quake sequence near Elassona-Tyrnavos (northern Thessaly). Acta Geodyn. Geomater. 2021, 18, 379–385. [Google Scholar] [CrossRef]
- Santos-reyes, J.; Gouzeva, T.; Santos-reyes, G. Earth quake Risk Perception and Mexico City’s Public Safety. Procedia Eng. 2014, 84, 662–671. [Google Scholar] [CrossRef]
- Kasumović, M. The Mean Level of the Adriatic Sea and the Geodetic Normal Null of Trieste. Rad Geofizičkog zavoda u Zagrebu 1950, 2, 1–22. Available online: https://hrcak.srce.hr/file/432951 (accessed on 1 November 2023).
- Kasumović, M. On mean sea level of Adriatic Sea and its determination. Geod. List 1959, 13, 159–169. Available online: https://hrcak.srce.hr/file/432171 (accessed on 1 November 2023). (In Croatian).
- Jovanović, B. Method Studies of: Sea Depth Observations and Its Data Processing and Definition of Coastal Line from Hidrographic, Geodetic and Marine Aspect. Ph.D. Thesis, Geodetic Faculty of University of Zagreb, Zagreb, Croatia, 1978; 292p. (In Croatian). [Google Scholar]
- Bilajbegović, A.; Marchesini, C. Yugoslav vertical datums and preliminary relationship new Yugoslav nivelman with Austrian and Italian networks. Geod. List 1991, 45, 233–248. Available online: https://hrcak.srce.hr/293670 (accessed on 1 December 2023). (In Croatian).
- Bašić, T. Unique transformation model and new geoid model of the Republic of Croatia. In Report on the Scientific Projects of State Geodetic Administration in Period from 2006 till 2009; Boseiljevac, M., Ed.; State Geodetic Administration: Zagreb, Croatia, 2009; pp. 5–21. Available online: https://www.researchgate.net/publication/228791071 (accessed on 1 October 2023). (In Croatian)
- Dragičević, D.; Pavasović, M.; Bašić, T. Accuracy validation of official Croatian geoid solutions over the area of City of Zagreb. Geofizika 2016, 33, 183–206. [Google Scholar] [CrossRef]
- Esselborn, S.; Rudenko, S.; Schöne, T. Orbit-related sea level errors for TOPEX altimetry at seasonal to decadal time scales. Ocean Sci. 2018, 14, 205–223. [Google Scholar] [CrossRef]
- Marjanović, M.; Bačić, Ž.; Bašić, T. Determination of horizontal and vertical movements of the Adriatic micro-plate on the basis of GPS measurements. In Geodesy for Planet Earth, Proceedings of the 2009 IAG Symposium, Buenos Aires, Argentina, 31 August–4 September 2009; Springer: Berlin, Germany, 2012; Volume 136, pp. 683–688. Available online: https://link.springer.com/chapter/10.1007/978-3-642-20338-1_84 (accessed on 1 October 2023).
- Omstedt, A.; Pettersen, C.; Rodhe, J.; Winsor, P. Baltic Sea climate: 200 yr of data on air temperature, sea level variations, icecover and atmospheric circulation. Clim. Res. 2004, 25, 205–216. [Google Scholar] [CrossRef]
- Hammarklint, T. Swedish Sea Level Series—A Climate Indicator; Swedish Meteorological and Hydrological Institute: Stockholm, Sweden, 2009; Available online: https://www.smhi.se/polopoly_fs/1.8963!/Swedish_Sea_Level_Series_-_A_Climate_Indicator.pdf (accessed on 1 October 2023).
- He, X.; Montillet, J.P.; Fernandes, R.; Melbourne, T.I.; Jiang, W.; Huang, Z. Sea level rise estimation on the pacific coast fromsouthern California to Vancouver island. Remote Sens. 2020, 14, 4339. [Google Scholar] [CrossRef]
- Emery, K.O.; Aubrey, D.G. Sea Levels, Land Levels and Tide Gauges; Springer: New York, NY, USA, 1991; 245p. [Google Scholar]
- Kapsi, I.; Kall, T.; Liibusk, A. Sea Level Rise and Future Projections in the Baltic Sea. J. Mar. Sci. Eng. 2023, 11, 1514. [Google Scholar] [CrossRef]
- Cazenave, A.; Dominh, K.; Ponchaut, F.; Soudarin, L.; Cretaux, J.F.; Le Provost, C. Sea level changes from Topex-Poseidon altimetry and tide gauges and vertical crustal motions from DORIS. Geophys. Res. Lett. 1999, 26, 2077–2080. [Google Scholar] [CrossRef]
- Kuo, C.Y.; Shum, C.K.; Braun, A.; Mitrovica, J.X. Vertical crustal motion determined by satellite altimetry and tide gauge data in Fenoscandia. Geophys. Res. Lett. 2004, 31, L01608. [Google Scholar] [CrossRef]
- Wõppelmann, G.; Marcos, M. Coastal sea level rise in southern Europe and the no climate contribution of vertical land motion. J. Geophys. Res. 2012, 11, C01007. [Google Scholar] [CrossRef]
- De Biasio, F.; Baldin, G.; Vignudelli, S. Revisiting vesrtical land motion and sea level trends in the north-eastern Adriatic Sea using satellite altimetry and tide gauge data. J. Mar. Sci. 2020, 8, 949. [Google Scholar] [CrossRef]
- Surić, M. Reconstructing sea-level changes on the Eastern Adriatic Sea (Croatia)—An overview. Geoadria 2009, 14, 181–199. Available online: https://hrcak.srce.hr/clanak/68320 (accessed on 1 October 2023). [CrossRef]
- Orlić, M. (Ed.) Nulla Dies Sine Observation—150 Years of Geophysical Institute in Zagreb; Geophysical Institute: Zagreb, Croatia, 2011. (In Croatian) [Google Scholar]
- Orlić, M.; Pasarić, M. Sea level of Adriatic Sea and global climate changes. Pomor. Zb. 1994, 32, 481–501. Available online: https://www.croris.hr/crosbi/publikacija/prilog-casopis/182959 (accessed on 1 October 2023). (In Croatian).
- Orlić, M.; Pasarić, M. Sea level changes and crustal movements recorded along the east Adriatic coastal waters. Nuovo C. Della Soc. Ital. Di Fis. C-Geophys. Space Phys. 2000, 23, 351–364. Available online: https://core.ac.uk/download/pdf/294761906.pdf (accessed on 15 November 2023).
- Orlić, M.; Pasarić, M. Is the Mediterranean Sea level rising again? In Rapport du 40e Congres de la CIESM; CIESM: Marseille, France, 2013; p. 205. [Google Scholar]
- Vilibić, I.; Šepić, J.; Pasarić, M.; Orlić, M. The Adriatic Sea: A long-standing laboratory for sea level studies. Pure Appl. Geophys. 2017, 174, 3765–3811. Available online: https://link.springer.com/article/10.1007/s00024-017-1625-8 (accessed on 15 November 2023). [CrossRef]
- Kaplan, A.; Kushnir, Y.; Cane, M.A.; Blumenthal, M.B. Reduced space optimal analysis for historical datasets: 136 years of Atlantic sea surface temperatures. J. Geophys. Res. 1997, 102, 27835–27860. Available online: https://scholar.google.co.th/citations?user=-fMSJMoAAAAJ&hl=ja (accessed on 15 November 2023).
- Chambers, D.P.; Mehlhaff, C.A.; Urban, T.J.; Fujii, D. Low-frequency variations in global mean sea level. J. Geophys. Res. 2002, 107, 3026. [Google Scholar] [CrossRef]
- Church, J.A.; White, N.J.; Coleman, R.; Lambeck, K.; Mitrovica, J.X. Estimates of the regional distribution of sea level rise over the1950–2000 period. J. Clim. 2004, 17, 2609–2625. [Google Scholar] [CrossRef]
- Curch, J.A.; White, N.J. Sea level rise from the late 19th to early 21st century. Surv. Geophys. 2011, 32, 585–602. Available online: https://link.springer.com/article/10.1007/s10712-011-9119-1 (accessed on 15 November 2023). [CrossRef]
- Preisendorfer, R.W. Principal Component Analysis in Meteorology and Oceanography; Elsevier: Amsterdam, The Netherlands, 1988; 425p. [Google Scholar]
- Pandžić, K. Principal component analysis of precipitation in the Adriatic-Pannonian area of Yugoslavia. J. Climatol. 1988, 8, 357–370. [Google Scholar] [CrossRef]
- Pandžić, K.; Trninić, D. The relationship between the Sava River Basin annual precipitation, its discharge and thelarge-scale atmospheric circulation. Theor. Appl. Climatol. 1998, 61, 69–76. [Google Scholar] [CrossRef]
- Pandžić, K.; Likso, T. Eastern Adriatic typical wind patterns and large-scale atmospheric conditions. Int. J. Clim. 2005, 25, 81–98. [Google Scholar] [CrossRef]
- Chen, W.; Shum, C.K.; Forootan, E.; Feng, W.; Zhong, M.; Jia, Y.; Li, W.; Guo, J.; Wang, C.; Li, Q.; et al. Understanding water level changes in the Great Lakes by an ICA-based merging of multi-mission altimetry measurements. Remote Sens. 2022, 14, 5194. [Google Scholar] [CrossRef]
- Dangendorf, S.; Hay, C.; Calafat, F.; Marcos, M.; Piecuch, C.G.; Berk, K.; Jensen, J. Persistent global sea level rise acceleration since the 1960th. Nat. Clim. Chang. 2019, 9, 705–710. Available online: https://www.nature.com/articles/s41558-019-0531-8 (accessed on 20 November 2023). [CrossRef]
- Nerem, R.S.; Beckley, B.D.; Fasullo, J.T.; Hamlington, B.D.; Masters, D.; Mitchum, G.T. Climate change driven accelerated sea level rise detected in the altimeter era. Proc. Nat. Acad. Sci. USA 2018, 115, 2022–2025. [Google Scholar] [CrossRef] [PubMed]
- Watson, C.S.; White, N.J.; Church, J.A.; King, M.A.; Burgette, R.J.; Legresy, B. Unabated global meansea-level rise over the satellite altimeter era. Nat. Clim. Chang. 2015, 5, 565–568. Available online: https://www.nature.com/articles/nclimate2635 (accessed on 15 November 2023). [CrossRef]
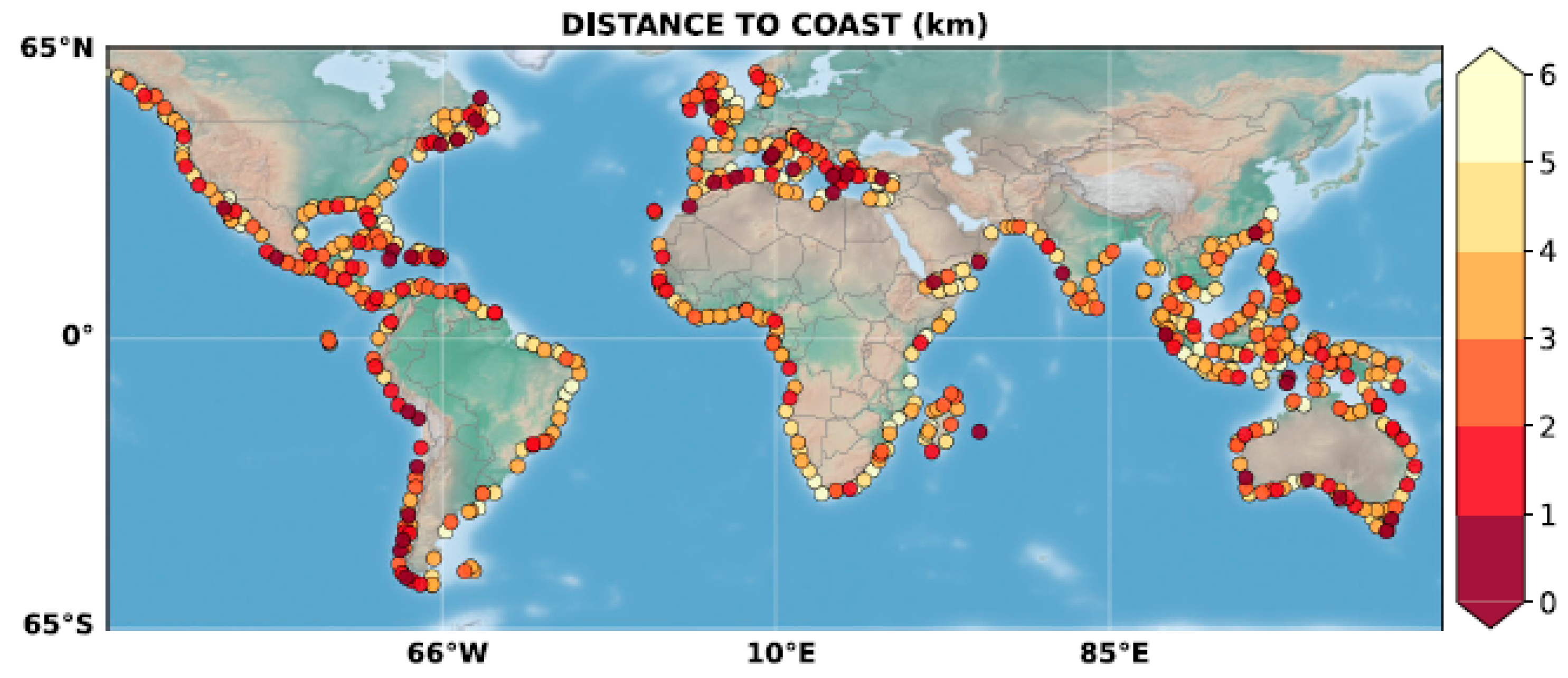
- Cazenave, A.; Gouzenes, Y.; Birol, F.; Leger, F.; Passaro, M.; Calafat, F.M.; Shaw, A.; Nino, F.; Legeais, J.F.; Oelsmann, J.; et al. Sea level along the world’s coastlines can be measured by a network of virtual altimetry stations. Commun. Earth Environ. 2022, 3, 177. Available online: https://www.nature.com/articles/s43247-022-00448-z (accessed on 15 November 2023). [CrossRef]
- Hamlington, B.D.; Gardner, A.S.; Ivins, E.; Lenaerts, J.T.M.; Reager, J.T.; Trossman, D.S.; Zaron, E.D.; Adhikari, S.; Arendt, A.; Aschwanden, A.; et al. Understanding of contemporary regional sea-level change and the implications for the future. Rev. Geophys. 2020, 58, e2019RG000672. [Google Scholar] [CrossRef] [PubMed]
- International Altimetry Team. Altimetry for the future: Building on 25 years of progress. Adv. Space Res. 2021, 68, 319–363. [Google Scholar] [CrossRef]
- Cazenave, A.; Moreira, L. Contemporary sea level changes from global to local scales: Areview. Proc. R. Soc. A 2022, 13, 1893. [Google Scholar] [CrossRef]
- Horwath, M.; Gutknecht, B.D.; Cazenave, A.; Palanisamy, H.K.; Marti, F.; Marzeion, B.; Paul, F.; LeBris, R.; Hogg, A.E.; Otosaka, I.; et al. Global sea level budget and ocean mass budget, with focus on advanced data products and uncertainty. Earth Syst. Sci. Data 2022, 14, 411–447. [Google Scholar] [CrossRef]
- Church, J.A.; Gregory, J.M.; Huybrechts, P.; Kuhn, M.; Lambeck, K.; Nhuan, M.T.; Qin, D.; Woodworth, P.L. Changes in sealevel. In Climate Change 2001: The Scientific Basis. Contribution of Working Group 1 to the Third Assessment Report of the Intergovernmental Panel on Climate Change; Houghton, J.T., Ding, Y., Griggs, D.J., Noguer, M., vander Linden, P., Dai, X., Maskell, K., Johnson, C.I., Eds.; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Meehl, G.A.; Stocker, T.F.; Collins, W.D.; Friedlingstein, P.; Gaye, A.T.; Gregory, J.M.; Kitoh, A.; Knutti, R.; Murphy, J.M.; Noda, A.; et al. Global climate projections. In Climate Change 2007: The Physical Science Basis. Contribution of Working Group 1 to the Fourth Assessment Report of the Intergovernmental Panel on Climate Change; Qin, D., Solomon, S., Manning, M., Marquis, M., Averyt, K., Tignor, M.M.B., Miller, H.L.J., Chen, Z., Eds.; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Rahmstorf, S. A semi-empirical approach to projecting future sea level rise. Science 2007, 315, 368–370. Available online: https://www.science.org/doi/abs/10.1126/science.1135456 (accessed on 15 November 2023). [CrossRef]
- Hologate, S.; Jevrejeva, S.; Woodworth, P.; Brewer, S. Comment on A Semi-Empirical Approach to Projecting Future Sea-Level Rise. Science 135. Available online: https://www.science.org/doi/10.1126/science.1140942 (accessed on 15 November 2023).
- Orlić, M.; Pasarić, Z. Semi-empirical versus process-basedsea level projections for the twenty-first century. Nat. Clim. Chang. 2013, 3, 735–738. Available online: https://www.nature.com/articles/nclimate1877 (accessed on 15 November 2023). [CrossRef]
- Orlić, M.; Pasarić, Z. Some pitfalls of the semi-empirical method used to project sea level. J. Clim. 2015, 28, 3779–3785. [Google Scholar] [CrossRef]
- IPCC. 2021: Summary for Policymakers. In Climate Change 2021: The Physical Science Basis. Contribution of Working Group I to the Sixth Assessment Report of the Intergovernmental Panel on Climate Change; Masson-Delmotte, V.P., Zhai, A., Pirani, S.L., Connors, C., Pean, S., Berger, N., Caud, Y., Chen, L., Goldfarb, M.I., Gomis, M., et al., Eds.; Cambridge University Press: Cambridge, UK, 2021. [Google Scholar]
- Yang, L.; Lin, L.; Fan, L.; Liu, N.; Huang, L.; Xu, Y.; Metikas, S.P.; Jia, Y.; Lin, M. Satellite altimetry: Achievements and futuretrends by a scientometrics analysis. Remote Sens. 2022, 14, 3332. [Google Scholar] [CrossRef]
- Tsimplis, M.N.; Raaicich, F.; Fenoglio-Marc, L.; Shaw, A.G.P.; Marcos, M.; Somot, S.; Bergamasco, A. Recent developments in understanding sea level rise at the Adriatic coast. Phys. Chem. Earth 2012, 40–41, 59–71. Available online: http://eprints.soton.ac.uk/id/eprint/159725 (accessed on 15 November 2023). [CrossRef]
- Barić, A.; Grbec, B.; Bogner, D. Potential implications of sea level rise for Croatia. J. Coast. Res. 2008, 24, 299–305. Available online: https://www.jstor.org/stable/30137836 (accessed on 10 October 2023). [CrossRef]
- Škreb, S. Sea level. Priroda 1936, 26, 271–274. Available online: https://library.foi.hr/dbook/cas.php?B=1&item=S00001&godina=1936&broj=00009&page=271 (accessed on 10 November 2023). (In Croatian).
- Virual Surveyor. Available online: https://support.virtual-surveyor.com/en/support/solutions/articles/1000261346 (accessed on 10 December 2023).
- von Sterneck, R. Kontrolie des Nivellementsdurchdie Flutmessrangaben und die Schwankungen des Meeresspiegels der Adria. In Mitteilungendse K. u. K. Militaägeographischen Institutes in Wien, Bd. XXIV; Military Institute in Vienna: Vienna, Austria, 1904. [Google Scholar]
- Zupan, A. Average sea level for Split in the period 1947–1957. Hidrogr. Godišnjak 1958, 1956–1957, 123–151. (In Croatian) [Google Scholar]
- Izvještaj o mareografskim osmatranjima na Jugoslavenskoj obali Jadranaza 1955., 1956.i 1957. Hydrographic Institute of Yugoslav Navy, Split, Croatia. 1956., 1957.i 1958. Available online: https://www.hhi.hr/en (accessed on 31 December 2022).
- Vilibić, I.; Orlić, M.; Čupić, S.; Domijan, N.; Leder, N.; Mihanović, H.; Pasarić, M.; Pasarić, Z.; Srdelić, M.; Strinić, G. A new approach to sea level observations in Croatia. Geofizika 2005, 22, 21–57. Available online: http://geofizika-journal.gfz.hr/vol_22/vilibic.pdf (accessed on 15 November 2023).
- Omar, K.; Ses, S.; Mustafar, M.A. The Malaysian seas: Variation of sea level observed by tide gauges and satellite altimetry. In Proceedings of the SEAMERGES Final Symposium, Bangkok, Thailand, 28 November–1 December 2005; Available online: http://eprints.utm.my/3518/1/Seamerges-salt-edited.pdf (accessed on 15 October 2023).
- Lumban-Gaol, J.; Vignudelli, S.; Nurjaya, I.W.; Natih, N.M.N.; Sinurat, M.E.; Arhatin, R.E.; Kusumaningrum, E.E. Evaluation of altimetry satellite data products and sea level trends in the Indonesian maritime continent. Earth Environ. Sci. 2021, 944, 012041. Available online: https://iopscience.iop.org/article/10.1088/1755-1315/944/1/012041/meta (accessed on 15 November 2023). [CrossRef]
- Andersen, O.B.; Nielsen, K.; Knudsen, P.; Hughes, C.W.; Bingham, R.; Fenoglio-Marc, L.; Gravelle, M.; Kern, M.; Poloo, S.P. Improving the coastal mean dynamic topography by geodetic combination of tide gauge and satellite altimetry. Mar. Geod. 2018, 41, 517–545. [Google Scholar] [CrossRef]
- Hamden, M.E.; Din, A.H.M.; Wijaya, D.D.; Yusoff, M.Y.M.; Pa’suya, M.F. Regional mean sea surface and mean dynamic topography models around Malesian Seas developed from 27 years of Along-track satellite altimetry data. Front. Earth Sci. 2021, 9, 665878. [Google Scholar] [CrossRef]
- Filmer, M.S.; Hughes, C.W.; Woodworth, P.L.; Featherstone, W.E.; Bingham, R.J. Comparison between geodetic and oceanographic approaches to estimate mean dynamic topography for vertical datum unification: Evaluation at Australian tide gauges. J. Geod. 2018, 92, 1413–1437. Available online: https://link.springer.com/article/10.1007/s00190-018-1131-5 (accessed on 15 November 2023). [CrossRef]
- Pavasović, M.; Đapo, A.; Marjanović, M.; Pribičević, B. Present tectonic dynamics of the geological structural setting of the Eastern Part the Adriatic Region obtained from geodetic and geological data. Appl. Sci. 2021, 11, 5735. [Google Scholar] [CrossRef]
- Dach, R.; Hugentobler, U.; Fridez, P. Bernese GPS Software Version 5.0 Tutorial; Astronomical Institute University of Bern: Bern, Switzerland, 2007; Available online: http://ftp.aiub.unibe.ch/BERN50/DOCU/DOCU50.pdf (accessed on 15 November 2023).
- Altmer, Y.; Bačić, Ž.; Coticchia, A.; Medved, M.; Mulić, M.; Nurce, B. Present-day tectonics in and around the Adriaplate inferred from GPS measurements. In Postcollisional Tectonics and Magmatism in the Mediterranean Region and Asia; Dilek, Y., Pavlides, S., Eds.; Geological Society of America Special Paper 409; Geological Society of America: Bolder, CO, USA, 2006; pp. 43–55. [Google Scholar] [CrossRef]
- Tsimplis, M.; Spada, G.; Marcos, M.; Flemming, N. Multi-decadal sea level trends and land movements in the Mediterranean Sea with estimation of factors perturbing tide gauge data and cumulative uncertainties. Glob. Planet Chang. 2011, 76, 63–76. [Google Scholar] [CrossRef]
- Biondić, B.; Biondić, R. Hydrology of Dinaric Karst in Croatia; University of Zagreb: Zagreb, Croatia, 2014; 341p. (In Croatian) [Google Scholar]
- Pandžić, K.; Cesarec, K.; Grgić, B. An analysis of the relationship between precipitation and discharge fields over a karstic river basin. Int. J. Climatol. 1997, 17, 891–901. [Google Scholar] [CrossRef]
- Tsimplis, M.N.; Baker, T.F. Sea level drop in the MediterraneanSea. An indicator of deep water salinity and temperaturechanges? Geophys. Res. Lett. 2000, 27, 1731–1734. [Google Scholar] [CrossRef]
- Tsimplis, M.N.; Josey, S.A. Forsing of Mediterranean Sea by atmospheric oscillations over the North Atlantic. Geophys. Res. Lett. 2001, 28, 803–806. [Google Scholar] [CrossRef]
- Houghton, J.T.; Meira, L.G.; Callander, B.A.; Harris, N.; Kattenberg, A.; Maskell, K. (Eds.) Climate Change 1995: The Science of Climate Change; Cambridge University Press: Cambridge, UK, 1996; 572p. [Google Scholar]
- Corti, S.; Molteni, F.; Palmer, T.N. Signature of recent climate change in frequencies of natural atmospheric circulation regimes. Nature 1999, 398, 799–802. Available online: https://www.nature.com/articles/19745 (accessed on 10 October 2023).
- Meli, M.; Camargo, C.M.L.; Olivieri, M.; Slangen, A.B.A.; Romangoli, C. Sea level trend variability in the Mediterranean during the1993–2019 period. Front. Mar. Sci. 2023, 10, 1150488. [Google Scholar] [CrossRef]
- Orlić, M. Basic physical properties and processes in theAdriatic Sea. In Velika geogrfija Hrvatske, Knjiga 2, Fizička geografija Hrvatske, Prirodno-geografska osnova razvoja; Školska knjiga; Sveučilište u Zadru, Odjel za geografiju, Školska knjiga: Zagreb, Croatia, 2023; pp. 485–513. (In Croatian) [Google Scholar]
- Orlić, M.; Pasarić, M.; Pasarić, Z. Mediterranean sea level variability in the second half of the twentieth century: A Bayesian approach to closing the budget. Pure Appl. Geophys. 2018, 175, 3973–3988. [Google Scholar] [CrossRef]
- Chen, X.; Zhang, X.; Church, J.A.; Watson, C.S.; King, M.A.; Monselesan, D.; Legresy, B.; Harig, C. The increasing rate of global mean se level rise during 1993–2014. Nat. Clim. Chang. 2017, 7, 492–495. Available online: https://polarice.geo.arizona.edu/papers/Chen.etal.AcceleratingGMSL.NClimate.2017.pdf (accessed on 15 November 2023). [CrossRef]
- Budyko, M.I. On present-day climatic changes. Tellus 1977, 29, 193–204. [Google Scholar] [CrossRef]
- Pandžić, K.; Likso, T.; Bonacci, O. A review of Extreme Air Temperature Analysis in Croatia. Atmosphere 2022, 13, 1893. [Google Scholar] [CrossRef]
- Wang, Y.; Heidarzadeh, M.; Satake, K.; Mulia, I.; Yamada, M. A tsunami warning system based on off shore bottom pressure gauges and data assimilation for Crete Island in the Eastern Mediterranean Basin. J. Geophys. Res. Solid Earth 2020, 125, e2020JB020293. [Google Scholar] [CrossRef]
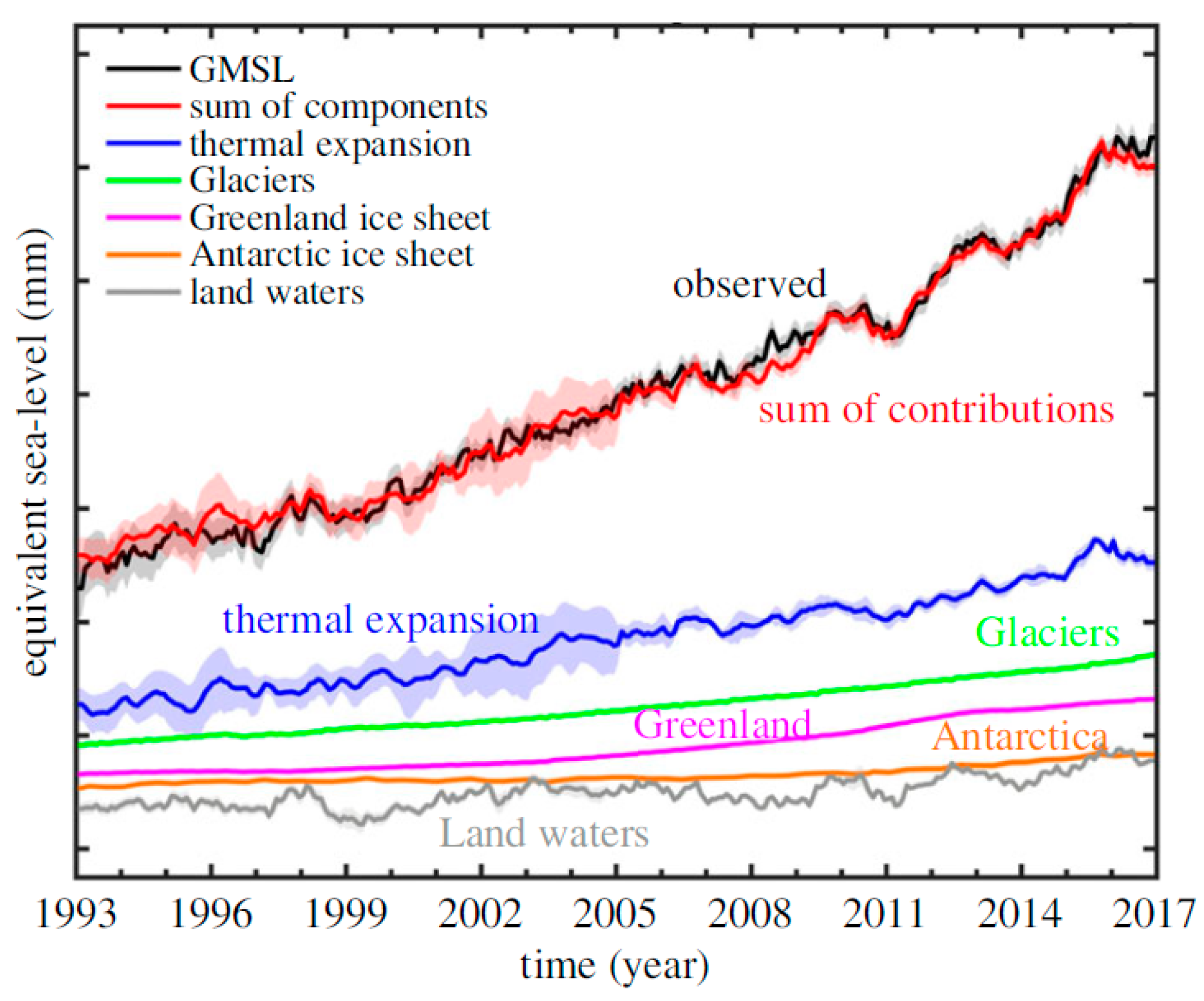
- Frederikse, T.; Landerer, F.; Caron, L.; Adhikari, S.; Parkes, D.; Humphrey, V.W.; Dangendorf, S.; Hogarth, P.; Zanna, L.; Cheng, L.; et al. The causes of sea level rise since 1900. Nature 2020, 584, 393–397. Available online: https://www.nature.com/articles/s41586-020-2591-3 (accessed on 15 November 2023). [CrossRef] [PubMed]
- Noye, B.J. Tide-well systems II: The frequency response of a linear tide-well system. J. Mar. Res. 1974, 32, 155–181. Available online: https://elischolar.library.yale.edu/cgi/viewcontent.cgi?article=2283&context=journal_of_marine_research (accessed on 15 October 2023).











| TG/GPS Stations | TG [Number of Years] | GPS [Number of Sessions] | Vu [mm/year] |
|---|---|---|---|
| Bakar | 62 | 17 | −1.2 |
| Dubrovnik | 47 | 17 | −1.2 |
| Rovinj | 48 | 19 | −0.4 |
| Split | 50 | 17 | −2.1 |
| Zadar | 10 | 11 | −0.9 |
| Station (PSMSL) | Altimeter—TG Classical Approach (1992–2010) | Altimeter—TG This study/Advanced Approach (All Data) | GPS (ULR Solution) | GIA (SELEN) |
|---|---|---|---|---|
| Santander I | −1.62 ± 0.43 | 0.91 ± 0.34 | −0.09 ± 0.23 | −0.55 ± 0.26 |
| La Coruña I | 0.19 ± 0.60 | 0.73 ± 0.33 | −2.19 ± 1.82 | −0.80 ± 0.26 |
| Vigo | 3.64 ± 0.64 | 0.57 ± 0.33 | - | −0.70 ± 0.31 |
| Cascai | 0.43 ± 0.66 | - | 0.18 ± 0.16 | −0.62 ± 0.22 |
| Marselle | −0.23 ± 0.45 | 0.17 ± 0.20 | −0.04 ± 0.25 | −0.59 ± 0.19 |
| Venezia (PDS—Punta Della Salute) | −2.03 ± 1.32 | −1.22 ± 0.19 | 1.40 ± 3.06 | −0.43 ± 0.19 |
| Trieste | −0.31 ± 0.39 | 0.30 ± 0.19 | - | −0.41 ± 0.19 |
| Rovinj | 1.95 ± 0.42 | 0.75 ± 0.19 | - | −0.46 ± 0.20 |
| Bakar | 0.03 ± 0.50 | 0.43 ± 0.18 | - | −0.45 ± 0.20 |
| Split Marjan | −0.44 ± 0.44 | 0.61 ± 0.18 | - | −0.58 ± 0.20 |
| Split Gradska Luka | −0.69 ± 0.45 | 0.70 ± 0.18 | - | −0.57 ± 0.20 |
| Dubrovnik | −0.50 ± 0.38 | 0.32 ± 0.18 | −0.92 ± 0.31 | −0.60 ± 0.17 |
| Katakolon | 0.63 ± 0.47 | 0.06 ± 0.21 | - | −0.84 ± 0.23 |
| Thessaloniki | −1.38 ± 0.47 | −1.96 ± 0.25 | - | −0.63 ± 0.22 |
| Alexandroupolis | 0.01 ± 0.47 | 0.02 ± 0.28 | - | −0.64 ± 0.20 |
| Leros | 3.63 ± 0.51 | 1.28 ± 0.35 | - | −0.80 ± 0.19 |
| Alexandria | 0.37 ± 0.70 | −0.40 ± 0.23 | −0.10 ± 0.50 | −0.51 ± 0.07 |
| Station (PSMSL) | No Corrections | Altimeter—TG Classic | Altimeter—TG Advanced | GPS Corrected (URL Solution) | GIA Corrected (SELEN) |
|---|---|---|---|---|---|
| Santander I | 2.07 ± 0.13 | 0.4 ±5 0.45 | 2.98 ± 0.36 | 1.97 ± 0.26 | 1.52 ± 0.29 |
| La Coruña I | 2.21 ± 0.14 | 2.40 ± 0.62 | 2.94 ± 0.36 | 0.02 ±1.83 | 1.42 ± 0.30 |
| Vigo | 2.35 ± 0.14 | 5.99 ± 0.66 | 2.92 ± 0.36 | - | 1.65 ± 0.34 |
| Cascai | 1.29 ± 0.04 | 1.79 ± 0.66 | - | 1.14 ± 0.19 | 0.35 ± 0.24 |
| Marselle | 1.22 ± 0.04 | 0.99 ± 0.45 | 1.39 ± 0.20 | 1.18 ± 0.25 | 0.63 ± 0.19 |
| Venezia (PDS) | 2.45 ± 0.09 | 0.42 ±1.32 | 1.24 ± 0.21 | 3.85 ± 0.60 | 2.02 ± 0.21 |
| Trieste | 1.28 ± 0.07 | 0.97 ± 0.40 | 1.58 ± 0.20 | - | 0.87 ± 0.20 |
| Rovinj | 0.53 ± 0.17 | 2.48 ± 0.45 | 1.27 ± 0.25 | - | 0.07 ± 0.26 |
| Bakar | 1.03 ± 0.12 | 1.06 ± 0.51 | 1.46 ± 0.22 | - | 0.46 ± 0.26 |
| Split Marjan | 0.64 ± 0.16 | 0.20 ± 0.47 | 1.25 ± 0.24 | - | 0.06 ± 0.26 |
| Split Gradska Luka | 0.64 ± 0.16 | −0.05 ± 0.48 | 1.33 ± 0.24 | - | 0.06 ± 0.26 |
| Dubrovnik | 1.02 ± 0.16 | 0.52 ± 0.41 | 1.33 ± 0.24 | 0.10 ± 0.35 | 0.42 ± 0.23 |
| Katakolon | 1.97 ± 0.22 | 2.60 ± 0.52 | 2.03 ± 0.30 | - | 1.13 ± 0.32 |
| Thessaloniki | 3.88 ± 0.24 | 2.50 ± 0.53 | 1.93 ± 0.34 | - | 3.26 ± 0.33 |
| Alexandroupolis | 2.05 ± 0.27 | 2.06 ± 0.54 | 2.07 ± 0.39 | - | 1.42 ± 0.34 |
| Leros | 0.93 ± 0.24 | 4.56 ± 0.56 | 2.21 ± 0.42 | - | 0.14 ± 0.31 |
| Alexandria | 1.81 ± 0.12 | 2.18 ± 0.74 | 1.41 ± 0.29 | 1.64 ± 0.51 | 1.23 ±0.14 |
| Location | |
|---|---|
| VENEZIA * | 2.33 ± 0.83 |
| VEPTF | 2.37 ± 0.86 |
| TRIESTE * | 2.71 ± 0.75 |
| ROVINJ | 2.29 ± 0.80 |
| SPLIT * | 2.57 ± 0.74 |
| DUBROVNIK | 2.28 ± 0.74 |
| Pooled mean | 2.43 ± 0.80 |
| Sample mean | 2.43 ± 0.18 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pandžić, K.; Likso, T.; Biondić, R.; Biondić, B. A Review of the Contribution of Satellite Altimetry and Tide Gauge Data to Evaluate Sea Level Trends in the Adriatic Sea within a Mediterranean and Global Context. GeoHazards 2024, 5, 112-141. https://doi.org/10.3390/geohazards5010006
Pandžić K, Likso T, Biondić R, Biondić B. A Review of the Contribution of Satellite Altimetry and Tide Gauge Data to Evaluate Sea Level Trends in the Adriatic Sea within a Mediterranean and Global Context. GeoHazards. 2024; 5(1):112-141. https://doi.org/10.3390/geohazards5010006
Chicago/Turabian StylePandžić, Krešo, Tanja Likso, Ranko Biondić, and Božidar Biondić. 2024. "A Review of the Contribution of Satellite Altimetry and Tide Gauge Data to Evaluate Sea Level Trends in the Adriatic Sea within a Mediterranean and Global Context" GeoHazards 5, no. 1: 112-141. https://doi.org/10.3390/geohazards5010006
APA StylePandžić, K., Likso, T., Biondić, R., & Biondić, B. (2024). A Review of the Contribution of Satellite Altimetry and Tide Gauge Data to Evaluate Sea Level Trends in the Adriatic Sea within a Mediterranean and Global Context. GeoHazards, 5(1), 112-141. https://doi.org/10.3390/geohazards5010006