Reconstructing the Snow Avalanche of Coll de Pal 2018 (SE Pyrenees)
Abstract
1. Introduction
2. Materials and Methods
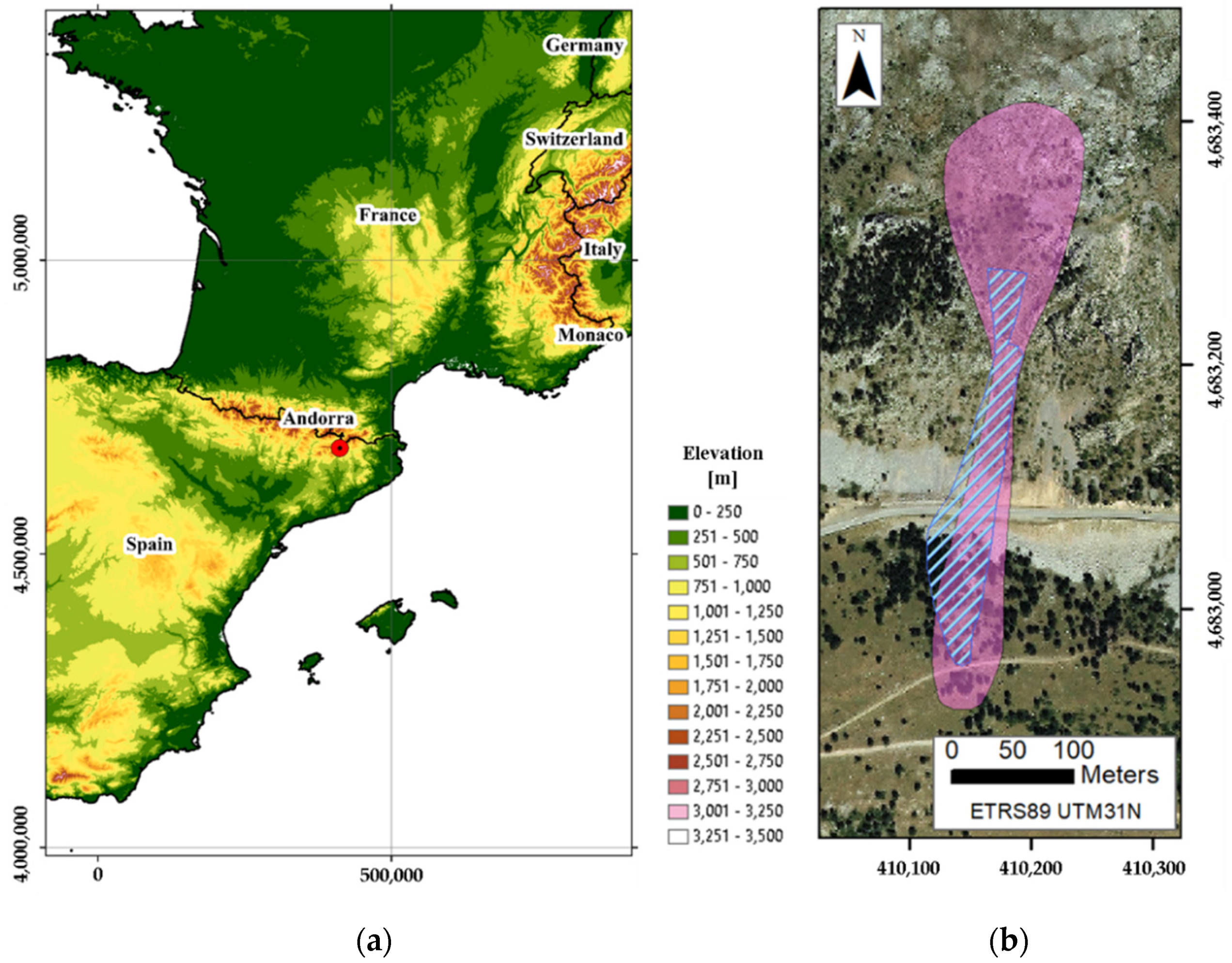
2.1. Coll de Pall Snow Avalanche
2.2. Event Characterization
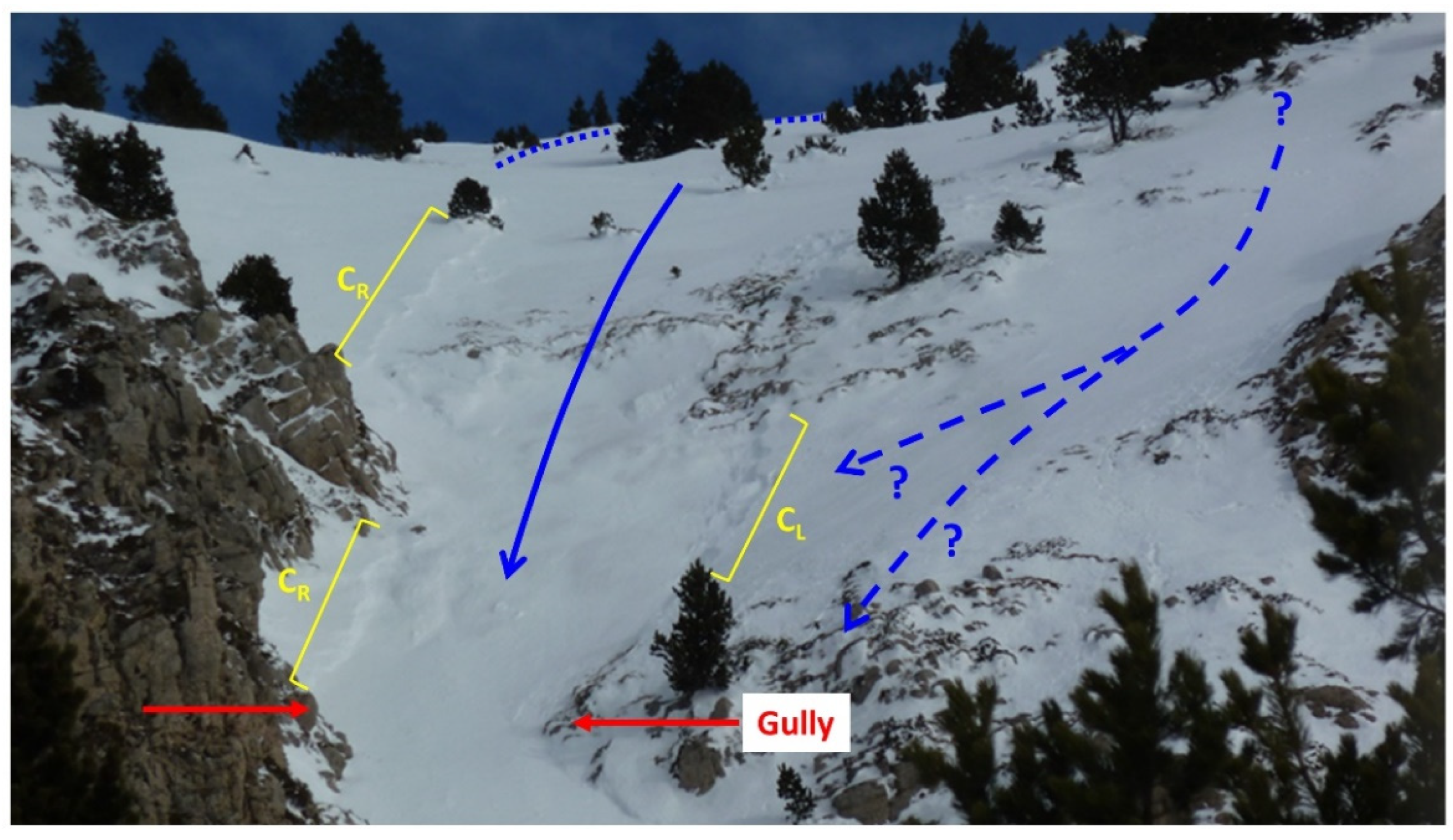
2.2.1. Avalanche Path
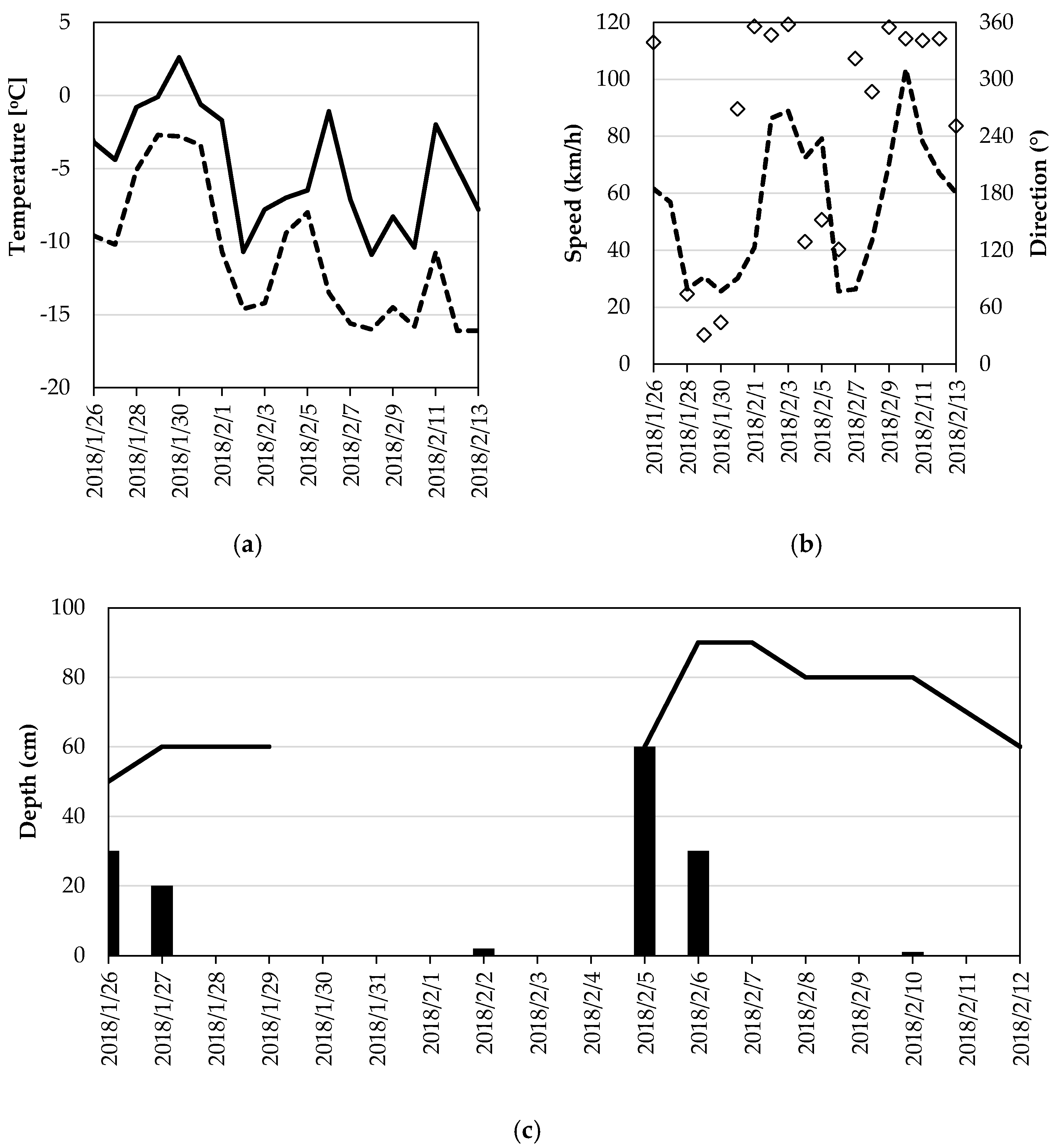
2.2.2. Snow and Meteorological Conditions
2.2.3. Avalanche Characteristics and Behavior
2.2.4. Estimation of the Return Period
2.3. Numerical Modelling
2.3.1. Rheological Model
2.3.2. Discretization and Conditions
2.3.3. Treatment of Wet-Dry Fronts
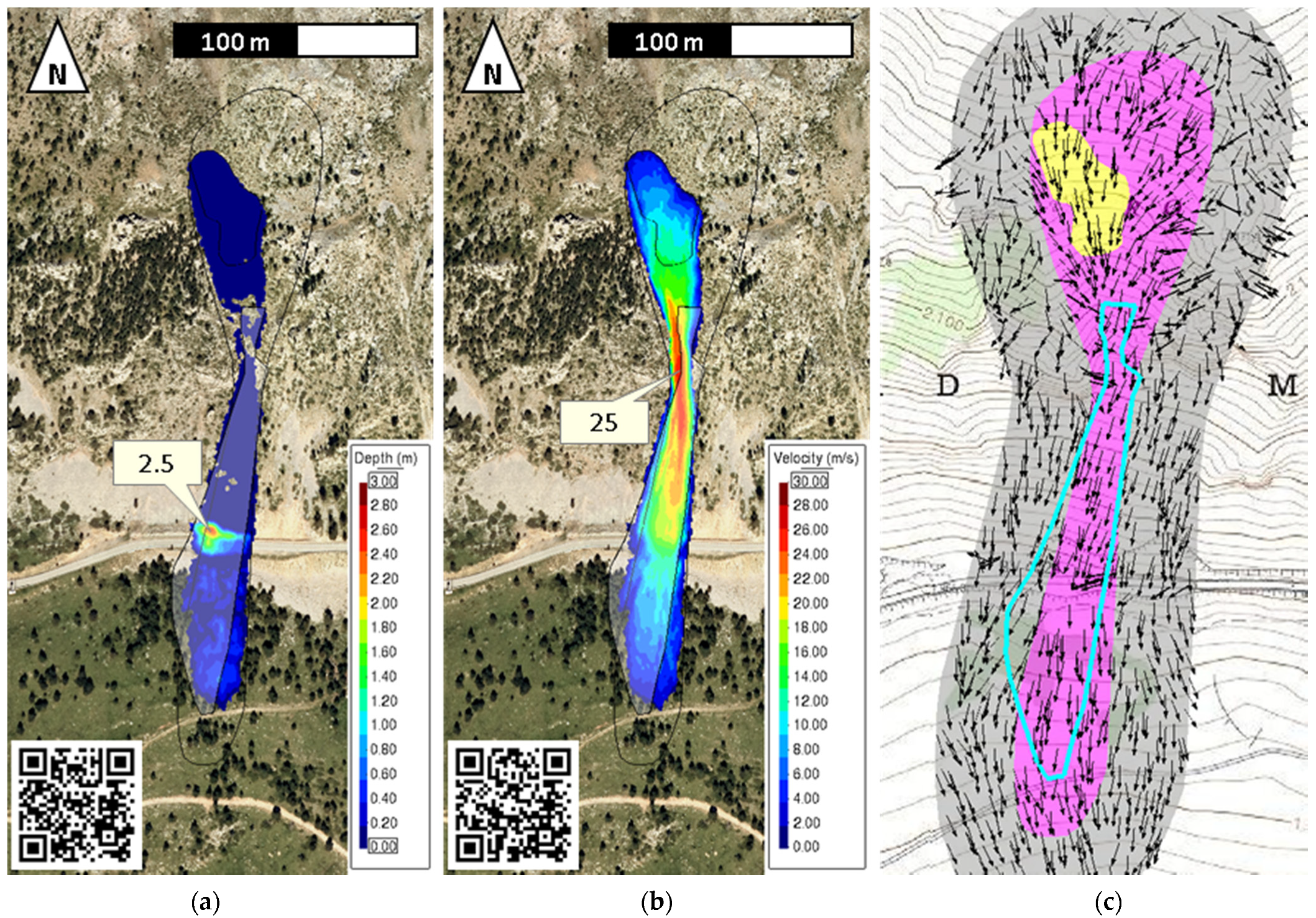
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A

References
- UNISDR. The Human Cost of Weather Related Disasters (1995–2015); United Nations Office for Disaster Risk Reduction (UNISDR): Geneva, Switzerland, 2015. [Google Scholar]
- CRED EM-DAT. The International Disaster Database. Available online: https://www.emdat.be (accessed on 14 February 2021).
- Ferreira, T.M.; Lu, Z. GeoHazards: A New Interdisciplinary Journal Devoted to the Study of Geomorphological Hazards. GeoHazards 2018, 1, 1–2. [Google Scholar] [CrossRef]
- Oller, P.; Muntán, E.; García-Sellés, C.; Furdada, G.; Baeza, C.; Angulo, C. Characterizing major avalanche episodes in space and time in the twentieth and early twenty-first centuries in the Catalan Pyrenees. Cold Reg. Sci. Technol. 2015, 110, 129–148. [Google Scholar] [CrossRef][Green Version]
- Muntán, E.; Garcia, C.; Oller, P.; Marti, G.; Garcia, A.; Gutiérrez, E. Reconstructing snow avalanches in the Southeastern Pyrenees. Nat. Hazards Earth Syst. Sci. 2009, 9, 1599–1612. [Google Scholar] [CrossRef]
- Semakova, E.; Myagkov, S.; Armstrong, R.L. The current state of avalanche risk analysis and hazard mapping in Uzbekistan. In Proceedings of the ISSW 09—International Snow Science Workshop, Davos, Switzerland, 27 September–2 October 2009; pp. 509–513. [Google Scholar]
- Bonsoms, J.; Salvador-Franch, F.; Oliva, M. Snowfall and snow cover evolution in the Eastern Pre-Pyrenees (NE Iberian Peninsula). Cuad. Investig. Geográfica 2021, 47. [Google Scholar]
- Stoffel, M.; Bollschweiler, M.; Butler, D.R.; Luckman, B.H. Tree Rings and Natural Hazards; Stoffel, M., Bollschweiler, M., Butler, D.R., Luckman, B.H., Eds.; Advances in Global Change Research; Springer: Dordrecht, The Netherlands, 2010; Volume 41, ISBN 978-90-481-8735-5. [Google Scholar]
- Aydin, A.; Eker, R. GIS-Based snow avalanche hazard mapping: Bayburt-Aşağı Dere catchment case. J. Environ. Biol. 2017, 38, 937–943. [Google Scholar] [CrossRef]
- Bocchiola, D.; Galizzi, M.; Bombelli, G.M.; Soncini, A. Mapping snow avalanches hazard in poorly monitored areas. The case of Rigopiano avalanche, Apennines of Italy. Nat. Hazards Earth Syst. Sci. Discuss. 2018, 1–31. [Google Scholar] [CrossRef]
- Bonnefoy-Demongeot, M.; Robinet, J.; Bouilloux, J.; Bourova, E.; Richard, D. How to improve snow avalanche observation quality? In Proceedings of the International Snow Science Workshop, Banff, AB, Canada, 29 September–3 October 2014. [Google Scholar]
- Fischer, J.T. A novel approach to evaluate and compare computational snow avalanche simulation. Nat. Hazards Earth Syst. Sci. 2013, 13, 1655–1667. [Google Scholar] [CrossRef]
- Jamieson, B.; Margreth, S.; Jones, A. Application and Limitations of Dynamic Models for Snow Avalanche Hazard Mapping. In Proceedings of the International Snow Science Workshop, Whistler, BC, Canada, 21–27 September 2008; pp. 730–739. [Google Scholar]
- Margreth, S.; Funk, M. Hazard mapping for ice and combined snow/ice avalanches—Two case studies from the Swiss and Italian Alps. Cold Reg. Sci. Technol. 1999, 30, 159–173. [Google Scholar] [CrossRef]
- Bosch, J.; Furdada, G.; Vilaplana, J.M. Estudi del mantell nival i programa de predicció del risc d’allaus al Pirineu de Catalunya. Rev. Dep. Política Territ. Obres Públiques 1991, 28, 34–44. [Google Scholar]
- Gauer, P.; Issler, D.; Lied, K.; Kristensen, K.; Sandersen, F. On snow avalanche flow regimes: Inferences from observations and measurements. In Proceedings of the International Snow Science Workshop, Whistler, BC, Canada, 21–27 September, 2008; pp. 717–723. [Google Scholar]
- Dreier, L.; Bühler, Y.; Steinkogler, W.; Feistl, T.; Christen, M.; Bartelt, P. Modelling Small and Frequent Avalanches. In Proceedings of the International Snow Science Workshop, Banff, AB, Canada, 29 September–3 October 2014; p. 8. [Google Scholar]
- Gubler, H. Measurements and modelling of snow avalanche speeds. Avalanche formation, movement and effects. In Proceedings of the Davos Symposium, September 1986; IAHS Publ.: Oxfordshire, UK, 1987; Volume 162, pp. 405–420. [Google Scholar]
- Gruber, U.; Bartelt, P. Snow avalanche hazard modelling of large areas using shallow water numerical methods and GIS. Environ. Model. Softw. 2007, 22, 1472–1481. [Google Scholar] [CrossRef]
- Stefania, S.; Zugliani, D.; Rosatti, G. Dense snow avalanche modelling with Voellmy rheology: TRENT2D vs. RAMMS2D. In Proceedings of the Vistual Snow Science Workshop—VSSW 2020, Fernie, BC, Canada, 4–6 October 2020. [Google Scholar]
- Sampl, P.; Zwinger, T. Avalanche simulation with SAMOS. Ann. Glaciol. 2004, 38, 393–398. [Google Scholar] [CrossRef]
- Mergili, M.; Fischer, J.T.; Krenn, J.; Pudasaini, S.P.R. avaflow v1, an advanced open-source computational framework for the propagation and interaction of two-phase mass flows. Geosci. Model Dev. 2017, 10, 553–569. [Google Scholar] [CrossRef]
- Maggioni, M.; Barbero, M.; Barpi, F.; Borri-Brunetto, M.; De Biagi, V.; Freppaz, M.; Frigo, B.; Pallara, O.; Chiaia, B. Snow Avalanche Impact Measurements at the Seehore Test Site in Aosta Valley (NW Italian Alps). Geosciences 2019, 9, 471. [Google Scholar] [CrossRef]
- Keylock, C.J.; Barbolini, M. Snow avalanche impact pressure - vulnerability relations for use in risk assessment. Can. Geotech. J. 2011, 38, 227–238. [Google Scholar] [CrossRef]
- Christen, M.; Bartelt, P.; Gruber, U. AVAL-1D: An avalanche dynamics program for the practice. In Proceedings of the International Congress Interpraevent, Pacific Rim, Matsumoto, Japan, 14–18 October 2002; pp. 715–725. [Google Scholar]
- Podolskiy, E.A.; Chambon, G.; Naaim, M.; Gaume, J. A review of finite-element modelling in snow mechanics. J. Glaciol. 2013, 59, 1189–1201. [Google Scholar] [CrossRef]
- López, D.; Cuellar Moro, V.; Díaz Martínez, R. Corrección termodinámica de la difusión numérica del método W-SPH. Ing. del agua 2015, 19, 1. [Google Scholar] [CrossRef]
- López, D.; Marivela, R.; Garrote, L. Smoothed particle hydrodynamics model applied to hydraulic structures: A hydraulic jump test case. J. Hydraul. Res. 2010, 48, 142–158. [Google Scholar] [CrossRef]
- Schraml, K.; Thomschitz, B.; Mcardell, B.W.; Graf, C.; Kaitna, R. Modeling debris-flow runout patterns on two alpine fans with different dynamic simulation models. Nat. Hazards Earth Syst. Sci. 2015, 15, 1483–1492. [Google Scholar] [CrossRef]
- Issler, D.; Harbitz, C.B.; Kristensen, K.; Lied, K.; Moe, A.S.; Barbolini, M.; De Blasio, F.V.; Khazaradze, G.; McElwaine, J.N.; Mears, A.I.; et al. A comparison of avalanche models with data from dry-snow avalanches at Ryggfonn, Norway. In Proceedings of the 11th International Conference and Field Trip on Landslides, Trondheim, Norway, 1–10 September 2005; pp. 173–179. [Google Scholar]
- Christen, M.; Kowalski, J.; Bartelt, P. RAMMS: Numerical simulation of dense snow avalanches in three-dimensional terrain. Cold Reg. Sci. Technol. 2010, 63, 1–14. [Google Scholar] [CrossRef]
- ICGC Database of avalanches in Catalonia (BDAC). Available online: https://www.icgc.cat/en/Public-Administration-and-Enterprises/Tools/Databases-and-catalogues/Database-of-avalanches-in-Catalonia-BDAC (accessed on 9 September 2020).
- Oller, P.; Marturià, J.; Martí, G.; González, J.C.; Martínez, P. Avalanche mapping in the Catalan Pyrenees. Balance and future perspectives. In Proceedings of the 4th ICA Workshop on Mountain Cartography; International Cartographic Association (ICA), Vall de Núria, Spain, 30 September–2 October 2004; pp. 13–22. [Google Scholar]
- Oller, P.; Muntán, E.; Marturià, J.; García, C.; García, A.; Martínez, P. The Avalanche Data in the Catalan Pyrenees, 20 Years of Avalanche Mapping. In Proceedings of the 2006 International Snow Science Workshop, Telluride, CO, USA, 1–6 October 2006; pp. 305–313. [Google Scholar]
- Winkler, K.; Schweizer, J. Comparison of snow stability tests: Extended column test, rutschblock test and compression test. Cold Reg. Sci. Technol. 2009, 59, 217–226. [Google Scholar] [CrossRef]
- McClung, D.; Schaerer, P.A. The Avalanche Handbook; Mountaineers: Seattle, WA, USA, 1993; ISBN 0898863643. [Google Scholar]
- EAWS European Avalanche Warning Services. Available online: https://www.avalanches.org/standards/avalanche-size/ (accessed on 27 April 2021).
- AAA. Snow, Weather, and Avalanches: Observation Guidelines for Avalanche Programs in the United States, 3rd ed.; American Avalanche Association (AAA): Victor, ID, USA, 2016. [Google Scholar]
- Centre de lauegi d’Aran Desencadenament artificial amb Daisybell. Bonaigua 15/01/2010. Available online: https://www.youtube.com/watch?v=5yO-PSTKxCY (accessed on 9 February 2021).
- Burkard, A.A.; Salm, B. Die Bestimmung der Mittleren Anrissmächtigkeit do zur Berechnung von Fliesslawinen; Eidgenössisches Institut für Schnee- und Lawinenforschung: Davos, Switzerland, 1992. [Google Scholar]
- Andrade-Fuentes, C.A. Reconstrucción del alud de Febrero de 2018 en la Carretera BV-4024, Coll de Pal, y Definición y Caracterización del Correspondiente Escenario de Referencia de T100; Universitat de Barcelona—Universitat Autònoma de Barcelona: Bellaterra, Barcelona, 2019. [Google Scholar]
- Bladé, E.; Cea, L.; Corestein, G.; Escolano, E.; Puertas, J.; Vázquez-Cendón, E.; Dolz, J.; Coll, A. Iber: Herramienta de simulación numérica del flujo en ríos. Rev. Int. Métodos Numéricos para Cálculo Diseño Ing. 2014, 30, 1–10. [Google Scholar] [CrossRef]
- Anta Álvarez, J.; Bermúdez, M.; Cea, L.; Suárez, J.; Ures, P.; Puertas, J. Modelización de los impactos por DSU en el río Miño (Lugo). Ing. Agua 2015, 19, 105. [Google Scholar] [CrossRef]
- Bladé, E.; Cea, L.; Corestein, G. Numerical modelling of river inundations. Ing. Agua 2014, 18, 68. [Google Scholar] [CrossRef]
- Sopelana, J.; Cea, L.; Ruano, S. Determinación de la inundación en tramos de ríos afectados por marea basada en la simulación continúa de nivel. Ing. Agua 2017, 21, 231. [Google Scholar] [CrossRef]
- Sopelana, J.; Cea, L.; Ruano, S. A continuous simulation approach for the estimation of extreme flood inundation in coastal river reaches affected by meso- and macrotides. Nat. Hazards 2018, 93, 1337–1358. [Google Scholar] [CrossRef]
- Sanz-Ramos, M.; Olivares Cerpa, G.; Bladé i Castellet, E. Metodología para el análisis de rotura de presas con aterramiento mediante simulación con fondo móvil. Ribagua 2020, 7, 1–10. [Google Scholar] [CrossRef]
- Sanz-Ramos, M.; Bladé, E.; Escolano, E. Optimización del cálculo de la Vía de Intenso Desagüe con criterios hidráulicos. Ing. Agua 2020, 24, 203. [Google Scholar] [CrossRef]
- Sanz-Ramos, M.; Bladé, E.; Dolz, J. Reconstructing the Aznalcóllar mine accident 20 year later. Numerical modelling of the flood. In Proceedings of the River Flow 2020: The 10th Conference on Fluvial Hydraulics, Delft, The Netherlands, 7–10 July 2020; CRC Press: Boca Raton, FL, USA, 2020; pp. 1222–1230, ISBN 9781000294361. [Google Scholar]
- Ruiz-Villanueva, V.; Mazzorana, B.; Bladé, E.; Bürkli, L.; Iribarren-Anacona, P.; Mao, L.; Nakamura, F.; Ravazzolo, D.; Rickenmann, D.; Sanz-Ramos, M.; et al. Characterization of wood-laden flows in rivers. Earth Surf. Process. Landf. 2019, 44, 1694–1709. [Google Scholar] [CrossRef]
- Torralba, A.; Bladé, E.; Oller, P. Implementació d’un model bidimensional per a simulació d’allaus de neu densa. In Proceedings of the V Jornades Tècniques de Neu i Allaus: Pyrenean Symposium on Snow and Avalanches, Ordino, Andorra, 9–11 October 2017. [Google Scholar]
- Sanz-Ramos, M.; Bladé, E.; Torralba, A.; Oller, P. Las ecuaciones de Saint Venant para la modelización de avalanchas de nieve densa. Ing. Agua 2020, 24, 65–79. [Google Scholar] [CrossRef]
- Toro, E.F. Riemann Solvers and Numerical Methods for Fluid Dynamics; Springer: Berlin/Heidelberg, Gernamy, 2009; Volume 40, ISBN 978-3-540-25202-3. [Google Scholar]
- Roe, P.L. A basis for the upwind differencing of the two-dimensional unsteady Euler equations. Numer. Methods Fluid Dyn. 1986, 55–80. [Google Scholar]
- Bladé, E.; Gómez-Valentín, M.; Sánchez-Juny, M.; Dolz, J. Source term treatment of SWEs using the surface gradient upwind method. J. Hydraul. Res. 2012, 50, 447–448. [Google Scholar] [CrossRef]
- Bermúdez, A.; Dervieux, A.; Desideri, J.-A.; Vázquez, M.E. Upwind schemes for the two-dimensional shallow water equations with variable depth using unstructured meshes. Comput. Methods Appl. Mech. Eng. 1998, 155, 49–72. [Google Scholar] [CrossRef]
- Voellmy, A. Über die Zerstörungskraft von Lawinen. Schweiz. Bauztg. 1955, 73, 15. [Google Scholar]
- Bartelt, P.; Salm, B.; Gruber, U. Calculating dense-snow avalanche runout using a Voellmy-fluid model with active/passive longitudinal straining. J. Glaciol. 1999, 45, 242–254. [Google Scholar] [CrossRef]
- Naaim, M.; Durand, Y.; Eckert, N.; Chambon, G. Dense avalanche friction coefficients: Influence of physical properties of snow. J. Glaciol. 2013, 59, 771–782. [Google Scholar] [CrossRef]
- Pudasaini, S.P.; Krautblatter, M. A two-phase mechanical model for rock-ice avalanches. J. Geophys. Res. Earth Surf. 2014, 119, 2272–2290. [Google Scholar] [CrossRef]
- Bartelt, P.; Valero, C.V.; Feistl, T.; Christen, M.; Bühler, Y.; Buser, O. Modelling cohesion in snow avalanche flow. J. Glaciol. 2015, 61, 837–850. [Google Scholar] [CrossRef]
- ICGC Descàrregues. Available online: https://www.icgc.cat/Descarregues (accessed on 25 May 2020).
- Brufau, P.; García-Navarro, P.; Vázquez-Cendón, M.E. Zero mass error using unsteady wetting–drying conditions in shallow flows over dry irregular topography. Int. J. Numer. Methods Fluids 2004, 45, 1047–1082. [Google Scholar] [CrossRef]
- Cea, L.; Puertas, J.; Vázquez-Cendón, M.-E. Depth averaged modelling of turbulent shallow water flow with wet-dry fronts. Arch. Comput. Methods Eng. 2007, 14, 303–341. [Google Scholar] [CrossRef]
- Bartelt, P.; Bühler, Y.; Christen, M.; Deubelbeiss, Y.; Salz, M.; Schneider, M.; Schumacher, L. RAMMS: Avalanche User Manual; WSL Institute for Snow and Avalanche Research SLF: Davos, Switzerland, 2017. [Google Scholar]
- Gauer, P. Comparison of avalanche front velocity measurements and implications for avalanche models. Cold Reg. Sci. Technol. 2014, 97, 132–150. [Google Scholar] [CrossRef]
- Thibert, E.; Bellot, H.; Ravanat, X.; Ousset, F.; Pulfer, G.; Naaim, M.; Hagenmuller, P.; Naaim-Bouvet, F.; Faug, T.; Nishimura, K.; et al. The full-scale avalanche test-site at Lautaret Pass (French Alps). Cold Reg. Sci. Technol. 2015, 115, 30–41. [Google Scholar] [CrossRef]
- Blagovechshenskiy, V.; Eglit, M.; Naaim, M. The calibration of an avalanche mathematical model using field data. Nat. Hazards Earth Syst. Sci. 2002, 2, 217–220. [Google Scholar] [CrossRef]
- Maggioni, M.; Bovet, E.; Dreier, L.; Buehler, Y.; Godone, D.; Bartelt, P.; Freppaz, M.; Chiaia, B.; Segor, V. Influence of summer and winter surface topography on numerical avalanche simulations. In Proceedings of the International Snow Science Workshop; International Snow Science Workshop ISSW 2013At, Grenoble Chamonix-Mont-Blanc: Grenoble, France, 2013; pp. 591–598. [Google Scholar]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sanz-Ramos, M.; Andrade, C.A.; Oller, P.; Furdada, G.; Bladé, E.; Martínez-Gomariz, E. Reconstructing the Snow Avalanche of Coll de Pal 2018 (SE Pyrenees). GeoHazards 2021, 2, 196-211. https://doi.org/10.3390/geohazards2030011
Sanz-Ramos M, Andrade CA, Oller P, Furdada G, Bladé E, Martínez-Gomariz E. Reconstructing the Snow Avalanche of Coll de Pal 2018 (SE Pyrenees). GeoHazards. 2021; 2(3):196-211. https://doi.org/10.3390/geohazards2030011
Chicago/Turabian StyleSanz-Ramos, Marcos, Carlos A. Andrade, Pere Oller, Glòria Furdada, Ernest Bladé, and Eduardo Martínez-Gomariz. 2021. "Reconstructing the Snow Avalanche of Coll de Pal 2018 (SE Pyrenees)" GeoHazards 2, no. 3: 196-211. https://doi.org/10.3390/geohazards2030011
APA StyleSanz-Ramos, M., Andrade, C. A., Oller, P., Furdada, G., Bladé, E., & Martínez-Gomariz, E. (2021). Reconstructing the Snow Avalanche of Coll de Pal 2018 (SE Pyrenees). GeoHazards, 2(3), 196-211. https://doi.org/10.3390/geohazards2030011





