Discrete Element Modelling of Soil Compaction of a Press-Wheel
Abstract
1. Introduction
2. Materials and Methods
2.1. Experiment
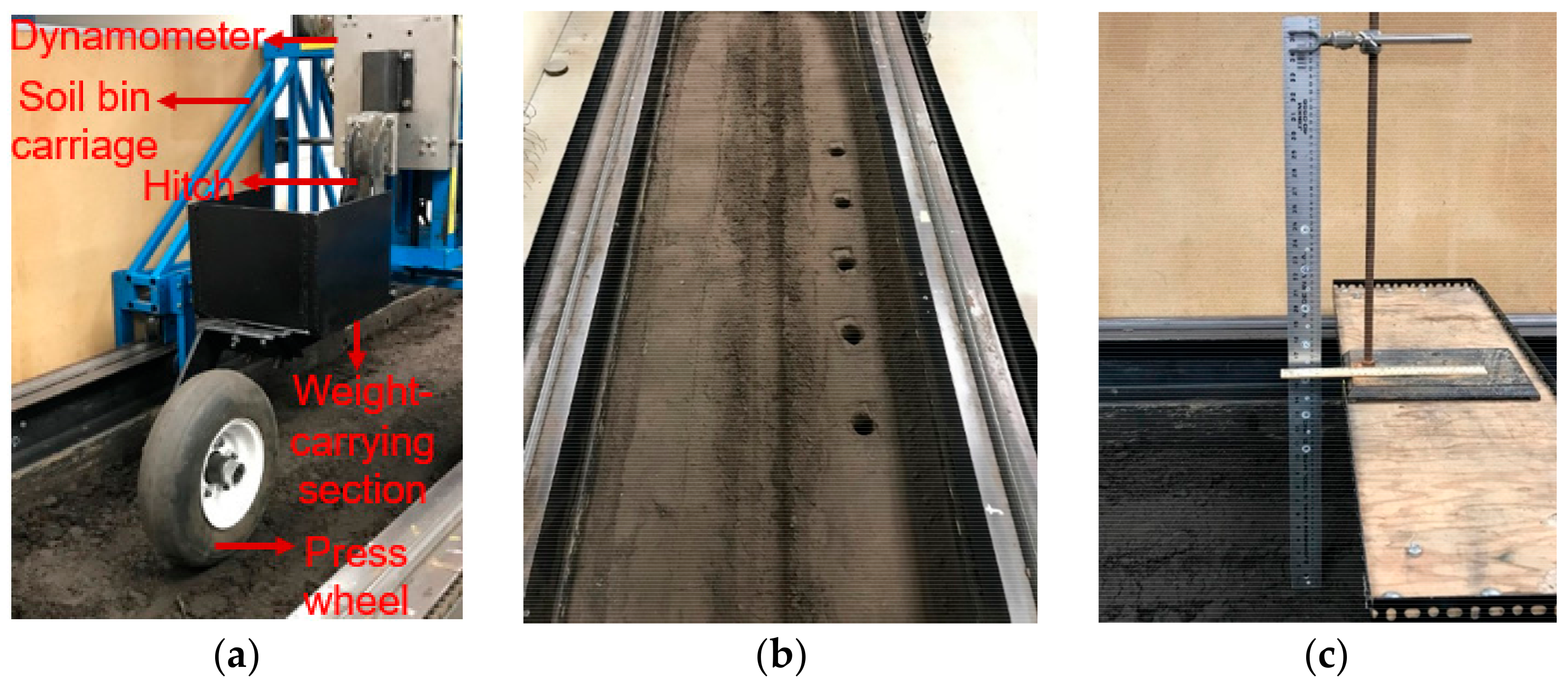
2.1.1. Description of the Press-Wheel, Test Apparatus and Soil Bin
2.1.2. Experimental Design
2.1.3. Measurements
2.1.4. Statistical Analysis
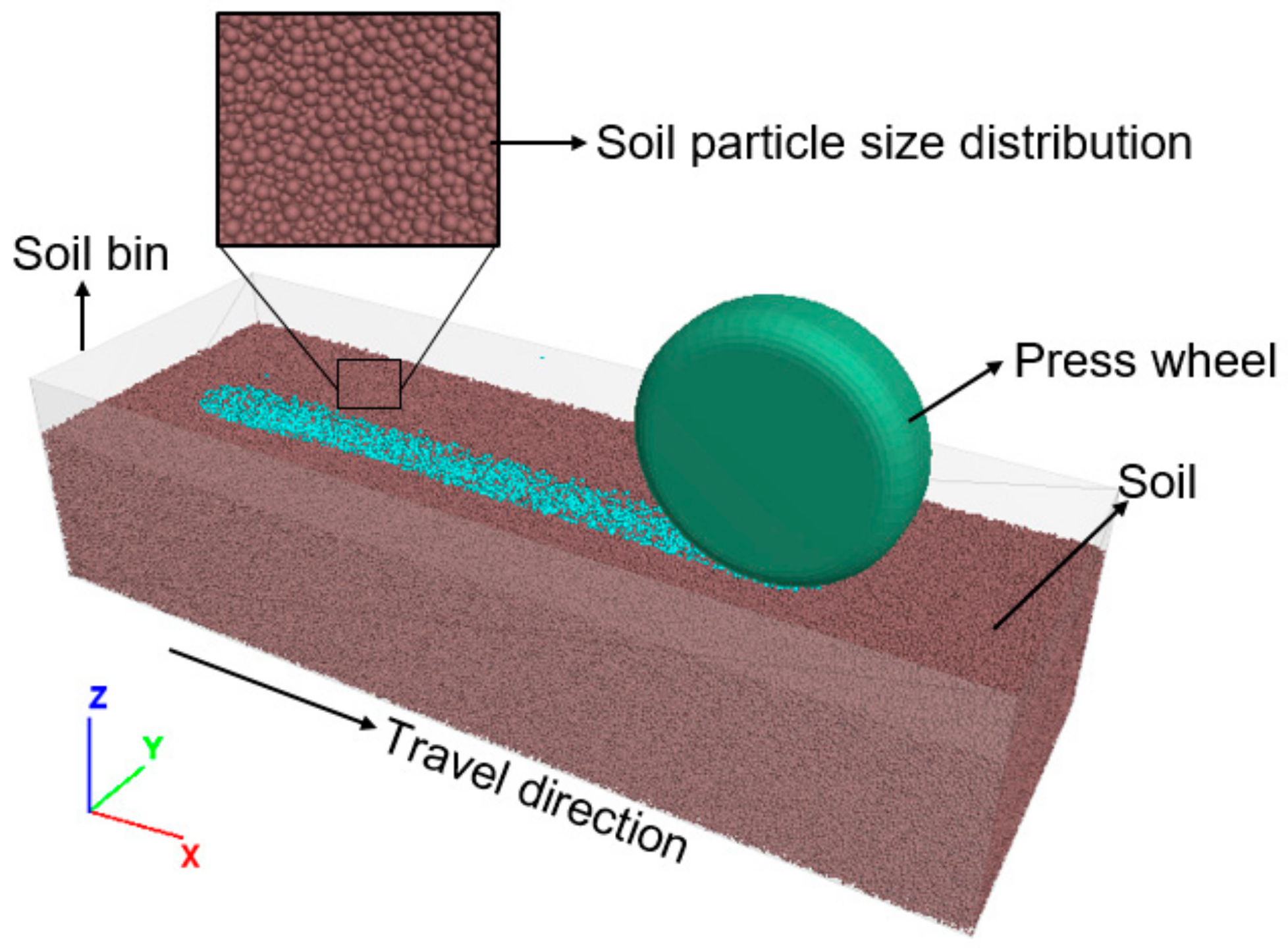
2.2. Soil-Wheel Model Development
2.2.1. Soil Model
2.2.2. Press-Wheel Model and Down Force
2.2.3. Soil–Wheel Interaction Model
2.2.4. Model Parameters
2.3. Model Validation
2.4. Model Application
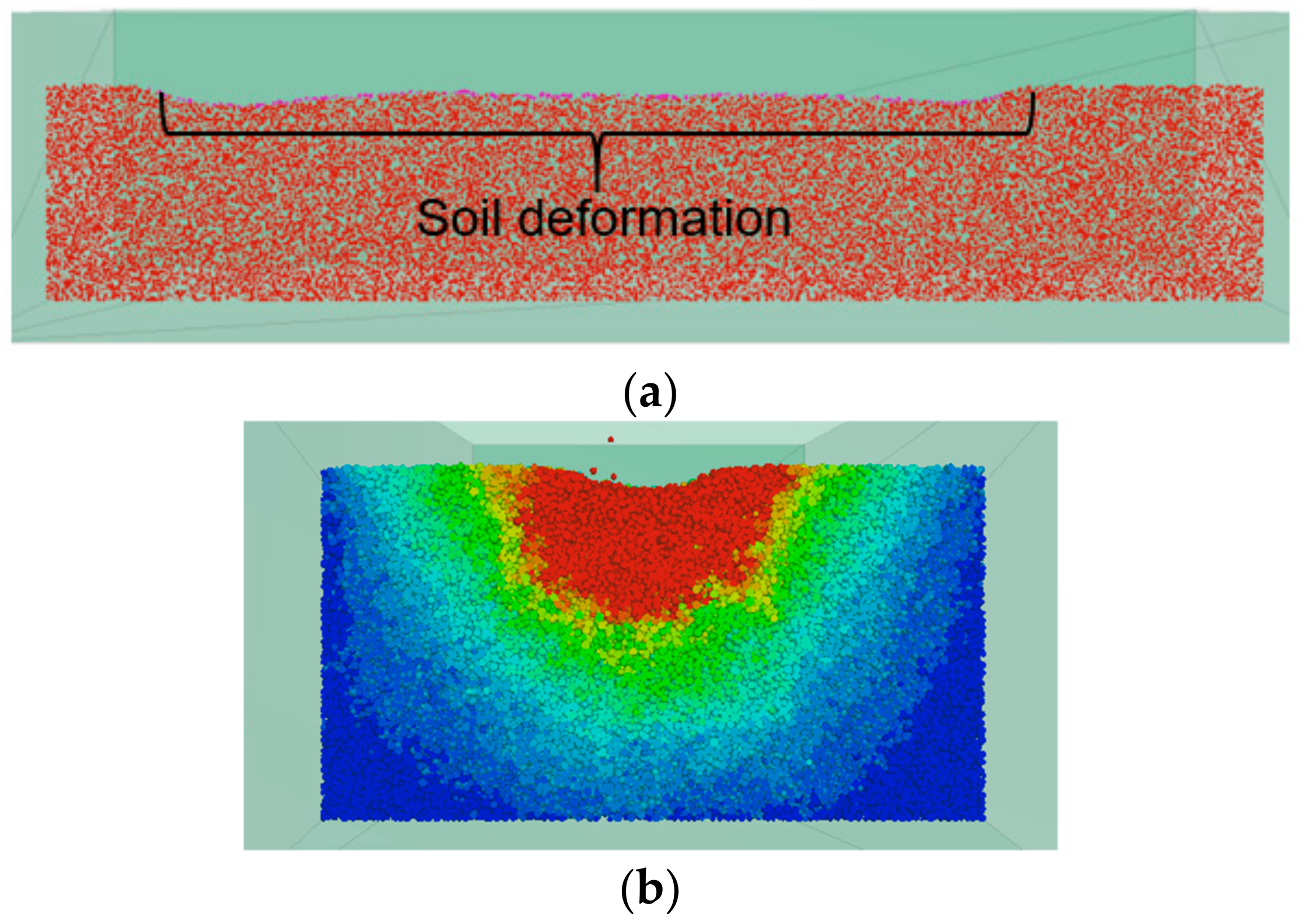
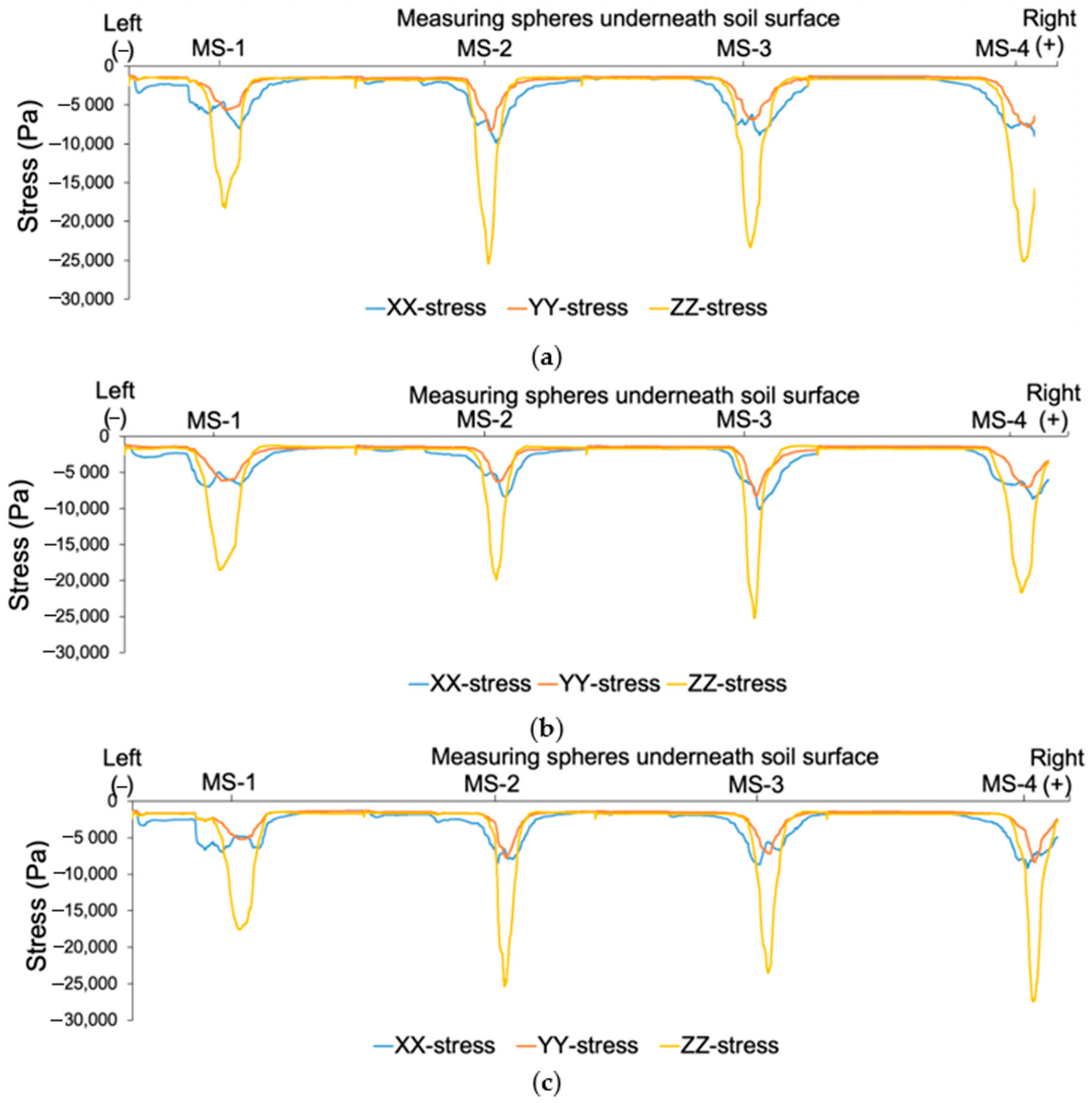
2.4.1. Instantaneous Stress Distribution in Soil
2.4.2. Stress–Sinkage Relationship
2.4.3. Effects of Down Force on the Sinkage
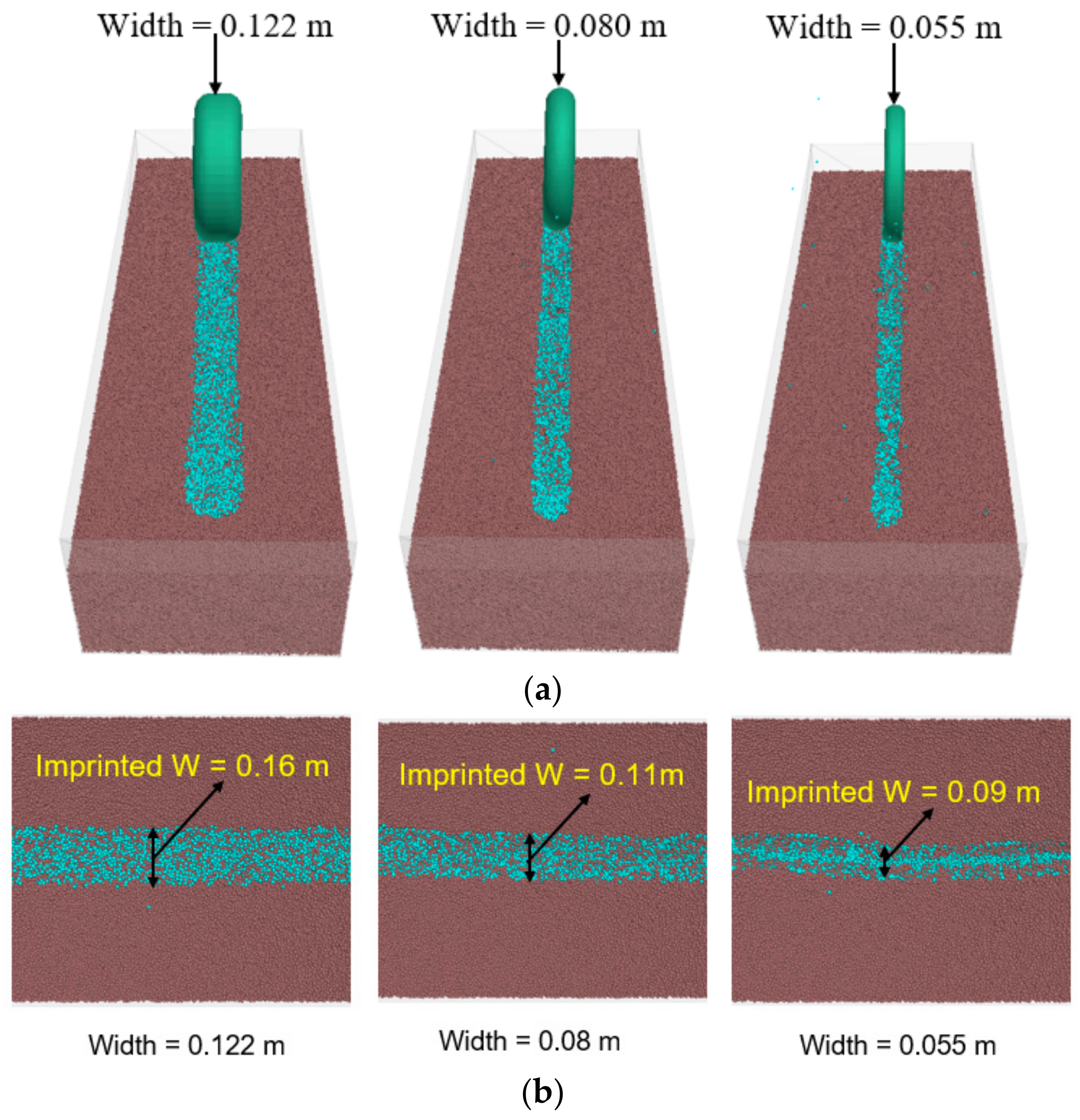
2.4.4. Effects of Press-Wheel Width on Sinkage
3. Results and Discussion
3.1. Experiment Results
3.1.1. Effect of Soil Moisture Content on Change in Bulk Density
3.1.2. Effect of Soil Moisture Content on Sinkage
3.1.3. Effect of Soil Moisture Content on Rolling Resistance
3.2. Model Behaviour Validation Results
3.3. Model Application
3.3.1. Instantaneous Stress Distribution in Soil
3.3.2. Stress–Sinkage Relationship
3.3.3. Effects of Press-Wheel down Force on the Sinkage
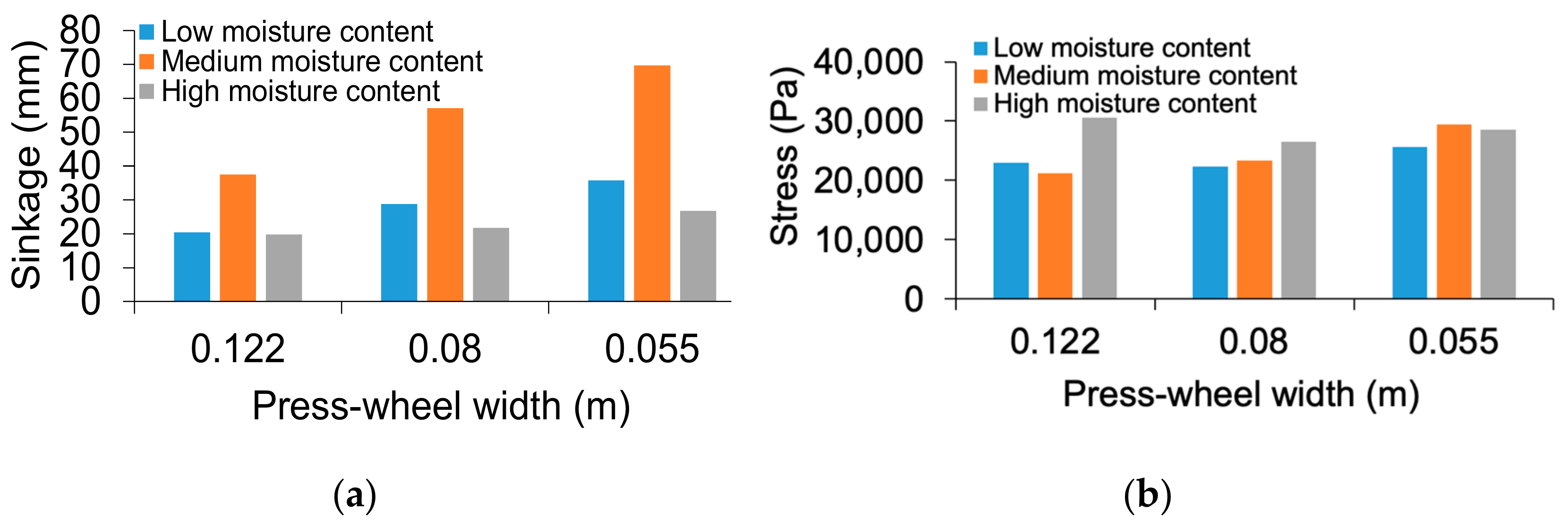
3.3.4. Effects of Press-Wheel Width on the Sinkage
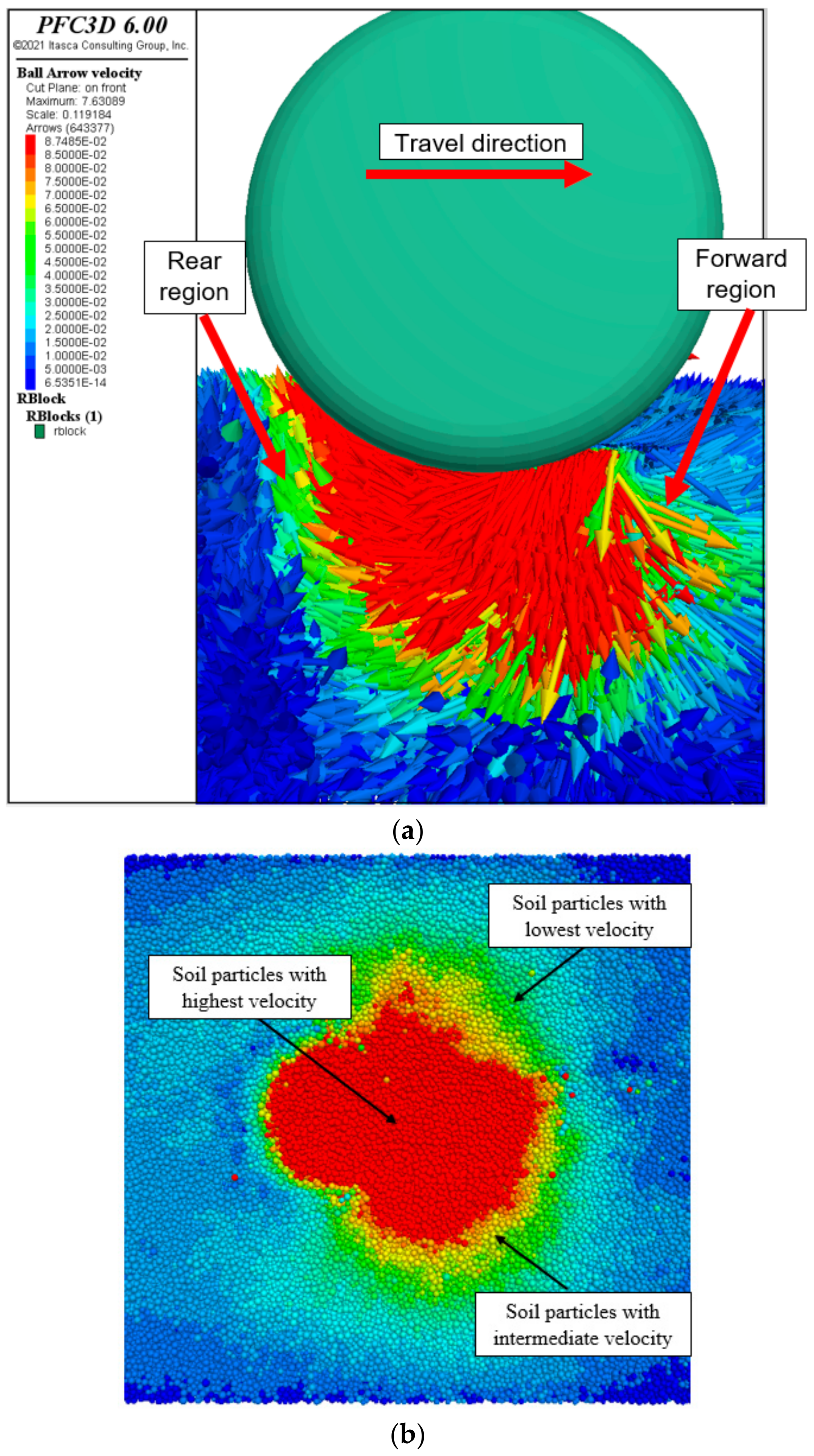
3.3.5. Velocity Flow Distribution of Soil Particles
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bayhan, Y.; Kayisoglu, B.; Gonulol, E. Effect of Soil Compaction on Sunflower Growth. Soil Tillage Res. 2002, 68, 31–38. [Google Scholar] [CrossRef]
- Hamza, M.A.; Anderson, W.K. Soil Compaction in Cropping Systems: A Review of the Nature, Causes and Possible Solutions. Soil Tillage Res. 2005, 82, 121–145. [Google Scholar] [CrossRef]
- Hanna, H.M.; Steward, B.L.; Aldinger, L. Soil Loading Effects of Planter Depth-Gauge Wheels on Early Corn Growth. Appl. Eng. Agric. 2010, 26, 551–556. [Google Scholar] [CrossRef]
- Chen, Y.; Tessier, S.; Irvine, B. Drill and Crop Performances as Affected by Different Drill Configurations for No-till Seeding. Soil Tillage Res. 2004, 77, 147–155. [Google Scholar] [CrossRef]
- Tessier, S.; Saxton, K.E.; Papendick, R.I.; Hyde, G.M. Zero-Tillage Furrow Opener Effects on Seed Environment and Wheat Emergence. Soil Tillage Res. 1991, 21, 347–360. [Google Scholar] [CrossRef]
- Saffih-Hdadi, K.; Défossez, P.; Richard, G.; Cui, Y.J.; Tang, A.M.; Chaplain, V. A Method for Predicting Soil Susceptibility to the Compaction of Surface Layers as a Function of Water Content and Bulk Density. Soil Tillage Res. 2009, 105, 96–103. [Google Scholar] [CrossRef]
- Smith, D.L.O.; Dickson, J.W. Contributions of Vehicle Weight and Ground Pressure to Soil Compaction. J. Agric. Eng. Res. 1990, 46, 13–29. [Google Scholar] [CrossRef]
- Rücknagel, J.; Götze, P.; Hofmann, B.; Christen, O.; Marschall, K. The Influence of Soil Gravel Content on Compaction Behaviour and Pre-Compression Stress. Geoderma 2013, 209–210, 226–232. [Google Scholar] [CrossRef]
- Asoodar, M.A.; Bakhshandeh, A.M.; Afraseabi, H.; Shafeinia, A. Effects of Press Wheel Weight and Soil Moisture at Sowing on Grain Yield. J. Agron. 2006, 5, 278–283. [Google Scholar] [CrossRef]
- McKyes, E. Soil Cutting and Tillage. Dev. Agric. Eng. 1989, 10, 192–221. [Google Scholar] [CrossRef]
- Kotrocz, K.; Kerényi, G. Modeling of the Soil-Rigid Wheel Interaction. Agric. Eng. 2015, 2014, 1–11. [Google Scholar]
- Khot, L.R.; Salokhe, V.M.; Jayasuriya, H.P.W.; Nakashima, H. Experimental Validation of Distinct Element Simulation for Dynamic Wheel-Soil Interaction. J. Terramech. 2007, 44, 429–437. [Google Scholar] [CrossRef]
- Mak, J.; Chen, Y. Simulation of Draft Forces of a Sweep in a Loamy Sand Soil Using the Discrete Element Method. Can. Biosyst. Eng. Genie Biosyst. Can. 2015, 56, 2.1–2.7. [Google Scholar] [CrossRef]
- Fielke, J.; Bayhan, Y. Effect of Set up Parameters for a Dual Tine and Presswheel Seeding Module on Seed Placement and Germination. Tarım Makinaları Bilim. Derg. 2011, 7, 191–197. [Google Scholar]
- O’Sullivan, C. Particulate Discrete Element Modelling; Routledge: London, UK, 2014. [Google Scholar] [CrossRef]
- Murray, S.E.; Chen, Y. Soil Bin Tests and Discrete Element Modeling of a Disc Opener. Can. Biosyst. Eng. Genie Biosyst. Can. 2018, 60, 21–210. [Google Scholar] [CrossRef][Green Version]
- Sadek, M.A.; Chen, Y. Feasibility of Using PFC3D to Simulate Soil Flow Resulting from a Simple Soil-Engaging Tool. Trans. ASABE 2015, 58, 987–996. [Google Scholar] [CrossRef]
- Campbell, G.S. Soil Physics with Basic Transport Models for Soil-Plant Systems. In Developments in Soil Science; Campbell, G.S., Ed.; Elsevier: Amsterdam, The Netherlands, 1985; Volume 14. [Google Scholar]
- Potyondy, D.O.; Cundall, P.A. A Bonded-Particle Model for Rock. Int. J. Rock Mech. Min. Sci. 2004, 41, 1329–1364. [Google Scholar] [CrossRef]
- Nandanwar, M.; Chen, Y. Modeling and Measurements of Triaxial Tests for a Sandy Loam Soil. Can. Biosyst. Eng. 2017, 59, 2.1–2.8. [Google Scholar] [CrossRef]
- Lamandé, M.; Schjønning, P. The Ability of Agricultural Tyres to Distribute the Wheel Load at the Soil-Tyre Interface. J. Terramech. 2008, 45, 109–120. [Google Scholar] [CrossRef]
- Meirion-Griffith, G.; Spenko, M. A Modified Pressure-Sinkage Model for Small, Rigid Wheels on Deformable Terrains. J. Terramech. 2011, 48, 149–155. [Google Scholar] [CrossRef]
- Smith, W.; Melanz, D.; Senatore, C.; Iagnemma, K.; Peng, H. Comparison of Discrete Element Method and Traditional Modeling Methods for Steady-State Wheel-Terrain Interaction of Small Vehicles. J. Terramech. 2014, 56, 61–75. [Google Scholar] [CrossRef]
- Bayhan, Y.; Fielke, J.; Saglam, C. Performance of A Dual Tine And Presswheel Seeding Module for A Range of Speeds, Presswheels and Sowing Tine Alignments. Bulg. J. Agric. Sci. 2015, 21, 454–460. [Google Scholar]
- Johnston, A.M.; Lafond, G.P.; May, W.E.; Hnatowich, G.L.; Hultgreen, G.E. Opener, Packer Wheel and Packing Force Effects on Crop Emergence and Yield of Direct Seeded Wheat, Canola and Field Peas. Can. J. Plant. Sci. 2003, 83, 129–139. [Google Scholar] [CrossRef]
- Zhao, C.; Zang, M. Analysis of Rigid Tire Traction Performance on a Sandy Soil by 3D Finite Element-Discrete Element Method. J. Terramech. 2014, 55, 29–37. [Google Scholar] [CrossRef]




| Model Parameter | Description and Unit | Value |
|---|---|---|
| Ball parameter | ||
| Particle normal stiffness (N/m) | ||
| Particle shear stiffness (N/m) | ||
| βn | Viscous damping coefficient | 1.0 |
| damp | Local damping coefficient | 0.5 |
| Bond parameter | ||
| Bond radius multiplier (dimensionless) | 0.5 | |
| Normal bond stiffness (pa/m) | ||
| Shear bond stiffness (pa/m) | ||
| σ | Normal bond strength (pa) | |
| τ | Shear bond strength (pa) | |
| Moisture Content | Change in Soil Bulk Density (Kg/mm3) | Sinkage (mm) | Rolling Resistance (N) | |||
|---|---|---|---|---|---|---|
| Mean 1 | Standard Deviation | Mean 1 | Standard Deviation | Mean 1 | Standard Deviation | |
| Low | 60.5c | 18.9 | 27.7a | 1.8 | 104.4ab | 8.8 |
| Medium | 249.0a | 38.5 | 26.7a | 1.1 | 89.9b | 18.4 |
| High | 110.0b | 8.9 | 25.2a | 4.2 | 113.6a | 18.8 |
| Moisture Content | Sinkage (mm) | Rolling Resistance (N) | Overall RME * (%) | ||
|---|---|---|---|---|---|
| Measurement | Simulation | Measurement | Simulation | ||
| Low | 27.7 | 20.4 | 104.4 | 104.0 | 13.2 |
| Medium | 26.7 | 38.0 | 82.9 | 80.6 | 22.7 |
| High | 25.2 | 20.3 | 113.6 | 138.6 | 20.8 |
| Moisture Content | Vertical Stress (Pa) | Lateral Stress (Pa) | Horizontal Stress (Pa) | |||
|---|---|---|---|---|---|---|
| Mean | Standard Deviation | Mean | Standard Deviation | Mean | Standard Deviation | |
| Low | 22,800 | 3.3 | 7100 | 1.1 | 9200 | 0.5 |
| Medium | 21,300 | 2.9 | 6800 | 1.0 | 8500 | 1.3 |
| High | 23,300 | 4.2 | 6800 | 1.1 | 8400 | 1.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Acquah, K.; Chen, Y. Discrete Element Modelling of Soil Compaction of a Press-Wheel. AgriEngineering 2021, 3, 278-293. https://doi.org/10.3390/agriengineering3020019
Acquah K, Chen Y. Discrete Element Modelling of Soil Compaction of a Press-Wheel. AgriEngineering. 2021; 3(2):278-293. https://doi.org/10.3390/agriengineering3020019
Chicago/Turabian StyleAcquah, Kobby, and Ying Chen. 2021. "Discrete Element Modelling of Soil Compaction of a Press-Wheel" AgriEngineering 3, no. 2: 278-293. https://doi.org/10.3390/agriengineering3020019
APA StyleAcquah, K., & Chen, Y. (2021). Discrete Element Modelling of Soil Compaction of a Press-Wheel. AgriEngineering, 3(2), 278-293. https://doi.org/10.3390/agriengineering3020019






