The Gamma-G Family: Brief Survey and COVID-19 Application
Abstract
1. Introduction
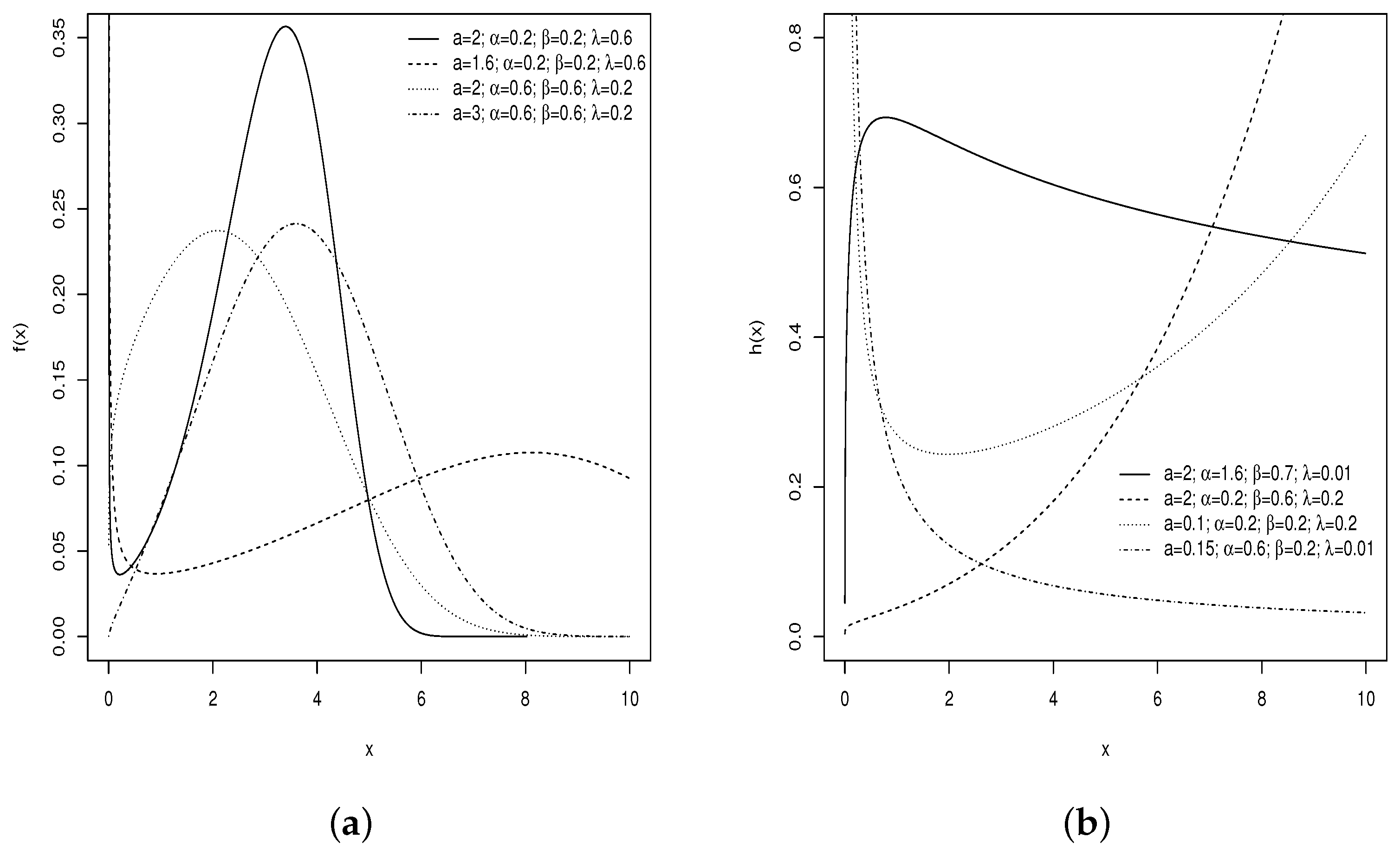
2. The Gamma-G Family
3. The Dual Gamma Generalized Family
4. Simulation
5. Application
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Marshall, A.W.; Olkin, I. A new method for adding a parameter to a family of distributions with application to the exponential and Weibull families. Biometrika 1997, 84, 641–652. [Google Scholar] [CrossRef]
- Eugene, N.; Lee, C.; Famoye, F. Beta-normal distribution and its applications. Commun. Stat.-Theory Methods 2002, 31, 497–512. [Google Scholar] [CrossRef]
- Tlhaloganyang, B.P.; Sengweni, W.; Oluyede, B.O. The Gamma Odd Burr X-G Family of Distributions with Applications. Pak. J. Stat. Oper. Res. 2022, 18, 721–746. [Google Scholar] [CrossRef]
- Mudholkar, G.; Srivastava, D. Exponentiated Weibull family for analyzing bathtub failure-rate data. IEEE Trans. Reliab. 1993, 42, 299–302. [Google Scholar] [CrossRef]
- El-Desouky, B.S.; Mustafa, A.; Al-Garash, S. The beta flexible Weibull distribution. arXiv 2017, arXiv:1703.05757. [Google Scholar]
- Shams, T.M. The Kumaraswamy-generalized Lomax distribution. Middle-East J. Sci. Res. 2013, 17, 641–646. [Google Scholar]
- Zografos, K.; Balakrishnan, N. On families of beta- and generalized gamma-generated distributions and associated inference. Stat. Methodol. 2009, 6, 344–362. [Google Scholar] [CrossRef]
- Ristić, M.M.; Balakrishnan, N. The gamma-exponentiated exponential distribution. J. Stat. Comput. Simul. 2012, 82, 1191–1206. [Google Scholar] [CrossRef]
- Nadarajah, S.; Cordeiro, G.M.; Ortega, E.M.M. The Zografos–Balakrishnan-G family of distributions: Mathematical properties and applications. Commun. Stat.-Theory Methods 2015, 44, 186–215. [Google Scholar] [CrossRef]
- Bourguignon, M.; Cordeiro, G.M. New results on the Ristić-Balakrishnan family of distributions. Commun. Stat.-Theory Methods 2016, 45, 6969–6988. [Google Scholar] [CrossRef]
- Castellares, F.; Lemonte, A.J. A new generalized Weibull distribution generated by gamma random variables. J. Egypt. Math. Soc. 2015, 23, 382–390. [Google Scholar] [CrossRef]
- Castellares, F.; Lemonte, A.J. On the gamma dual Weibull model. Am. J. Math. Manag. Sci. 2016, 35, 124–132. [Google Scholar] [CrossRef]
- Cordeiro, G.M.; Aristizábal, W.D.; Suárez, D.M.; Lozano, S. The Gamma Modified Weibull Distribution. Chil. J. Stat. 2015, 6, 37–48. [Google Scholar]
- Guerra, R.R.; Peña-Ramírez, F.A.; Cordeiro, G.M. The gamma Burr XII distributions: Theory and applications. J. Data Sci. 2017, 15, 467–494. [Google Scholar] [CrossRef]
- Pinho, G.B.; Cordeiro, G.M.; Nobre, J.S. The Gamma-exponentiated Weibull distribution. J. Stat. Theory Appl. 2012, 11, 379–395. [Google Scholar]
- Alzaatreh, A.; Famoye, F.; Lee, C. Gamma-Pareto distribution and its applications. J. Mod. Appl. Stat. Methods 2012, 11, 78–94. [Google Scholar] [CrossRef]
- Torabi, H.; Montazeri Hedesh, N. The gamma-uniform distribution and its applications. Kybernetika 2012, 48, 16–30. [Google Scholar]
- Silva, R.V.; Andrade, T.A.N.; Maciel, D.B.M.; Campos, R.P.S.; Cordeiro, G.M. A New Lifetime Model: The Gamma Extended Fréchet Distribution. J. Stat. Theory Appl. 2013, 12, 39–54. [Google Scholar] [CrossRef]
- Alzaatreh, A.; Knight, K. On the gamma-half normal distribution and its applications. J. Mod. Appl. Stat. Methods 2013, 12, 103–119. [Google Scholar] [CrossRef]
- Ramos, M.W.A.; Cordeiro, G.M.; Marinho, P.R.D.; Dias, C.R.B.; Hamedani, G. The Zografos-Balakrishnan Log-Logistic Distribution: Properties and Applications. J. Stat. Theory Appl. 2013, 12, 225–244. [Google Scholar] [CrossRef]
- Cordeiro, G.M.; Ortega, E.M.M.; Popović, B.V. The gamma-linear failure rate distribution: Theory and applications. J. Stat. Comput. Simul. 2014, 84, 2408–2426. [Google Scholar] [CrossRef]
- Oluyede, B.O.; Huang, S.; Pararai, M. A New Class of Generalized Dagum Distribution with Applications to Income and Lifetime Data. J. Stat. Econom. Methods 2014, 3, 125–151. [Google Scholar]
- Rodrigues, J.A.; Silva, A.P.C.M. The Gamma-Dagum distribution: Definition, properties and application. Rev. Eletrônica Matemática Estatística Foco 2015, 3, 1–7. [Google Scholar]
- Alzaatreh, A.; Famoye, F.; Lee, C. The gamma-normal distribution: Properties and applications. Comput. Stat. Data Anal. 2014, 69, 67–80. [Google Scholar] [CrossRef]
- Alzaatreh, A.; Mansoor, M.; Tahir, M.H.; Zubair, M.; Ghazali, S.A. The gamma half-Cauchy distribution: Properties and applications. Hacet. J. Math. Stat. 2015, 45, 1143–1159. [Google Scholar] [CrossRef]
- Pogány, T.K.; Saboor, A. The Gamma exponentiated exponential-Weibull distribution. Filomat 2016, 30, 3159–3170. [Google Scholar] [CrossRef]
- Khaleel, M.A.; Ibrahim, N.A.; Shitan, M.; Merovci, F. Some properties of gamma Burr type X distribution with application. In AIP Conference Proceedings; AIP Publishing: College Park, MD, USA, 2016; Volume 1739, p. 020087. [Google Scholar] [CrossRef]
- Iriarte, Y.A.; Astorga, J.M.; Bolfarine, H.; Gómez, H.W. Gamma-Maxwell distribution. Commun. Stat.-Theory Methods 2017, 46, 4264–4274. [Google Scholar] [CrossRef]
- Foya, S.; Oluyede, B.O.; Fagbamigbe, A.F.; Makubate, B. The gamma log-logistic Weibull distribution: Model, properties and application. Electron. J. Appl. Stat. Anal. 2017, 10, 206–241. [Google Scholar] [CrossRef]
- Kehinde, O.; Osebi, A.; Ganiyu, D. A New Class of Generalized Burr III Distribution for Lifetime Data. Int. J. Stat. Distrib. Appl. 2017, 4, 6–21. [Google Scholar] [CrossRef]
- Korkmaz, M. A Generalized Skew Slash Distribution via Gamma-normal Distribution. Commun. Stat.-Simul. Comput. 2017, 46, 1647–1660. [Google Scholar] [CrossRef]
- Gomes-Silva, F.; Andrade, T.A.N.; Bourguignon, M. Ristić-Balakrishnan extended exponential distribution. Acta Sci. Technol. 2017, 40, e34963. [Google Scholar] [CrossRef]
- Fagbamigbe, A.F.; Melamu, P.; Oluyede, B.O.; Boikanyo, M. The Ristić and Balakrishnan Lindley-Poisson distribution: Model, theory and application. Afr. Stat. 2018, 13, 1837–1864. [Google Scholar] [CrossRef]
- Ghosh, I.; Hamedani, G.G. The Gamma–Kumaraswamy distribution: An alternative to Gamma distribution. Commun. Stat.-Theory Methods 2018, 47, 2056–2072. [Google Scholar] [CrossRef]
- Akarawak, E.; Adeleke, I.; Okafor, R. The Gamma-Rayleigh Distribution and Applications to Survival Data. Niger. J. Basic Appl. Sci. 2018, 25, 130–142. [Google Scholar] [CrossRef]
- Arshad, R.M.I.; Chesneau, C.; Ghazali, S.A.; Jamal, F.; Mansoor, M. The gamma power half-logistic distribution: Theory and applications. São Paulo J. Math. Sci. 2023, 17, 1142–1169. [Google Scholar] [CrossRef]
- Jimoh, H.; Oluyede, B.O.; Wanduku, D.; Makubate, B. The gamma log-logistic Erlang truncated exponential distribution with applications. Afr. Stat. 2019, 14, 2141–2164. [Google Scholar] [CrossRef]
- Chaturvedi, A.; Vyas, S. Generalized Gamma-Maxwell distribution: Properties and estimation of reliability functions. J. Stat. Manag. Syst. 2019, 22, 1425–1444. [Google Scholar] [CrossRef]
- Oluyede, B.O.; Moakofi, T.; Makubate, B. A new gamma generalized Lindley-log-logistic distribution with applications. Afr. Stat. 2020, 15, 2451–2479. [Google Scholar] [CrossRef]
- Shahid, N.; Khalil, R.; Khokhar, J. Zografos Balakrishnan Power Lindley Distribution. J. Data Sci. 2021, 18, 279–298. [Google Scholar] [CrossRef]
- Reis, L.D.R.; Cordeiro, G.M.; Lima, M.D.C.S. The Gamma-Chen distribution: A new family of distributions with applications. Span. J. Stat. 2021, 2, 23–40. [Google Scholar] [CrossRef]
- Irshad, M.R.; D’cruz, V.; Maya, R. The Zografos-Balakrishnan Lindley Distribution: Properties and Applications. Statistica 2021, 81, 45–64. [Google Scholar]
- Shama, M.; Dey, S.; Altun, E.; Afify, A.Z. The Gamma-Gompertz distribution: Theory and applications. Math. Comput. Simul. 2021, 193, 689–712. [Google Scholar] [CrossRef]
- Vuong, Q.H. Likelihood Ratio Tests for Model Selection and Non-Nested Hypotheses. Econometrica 1989, 57, 307–333. [Google Scholar] [CrossRef]
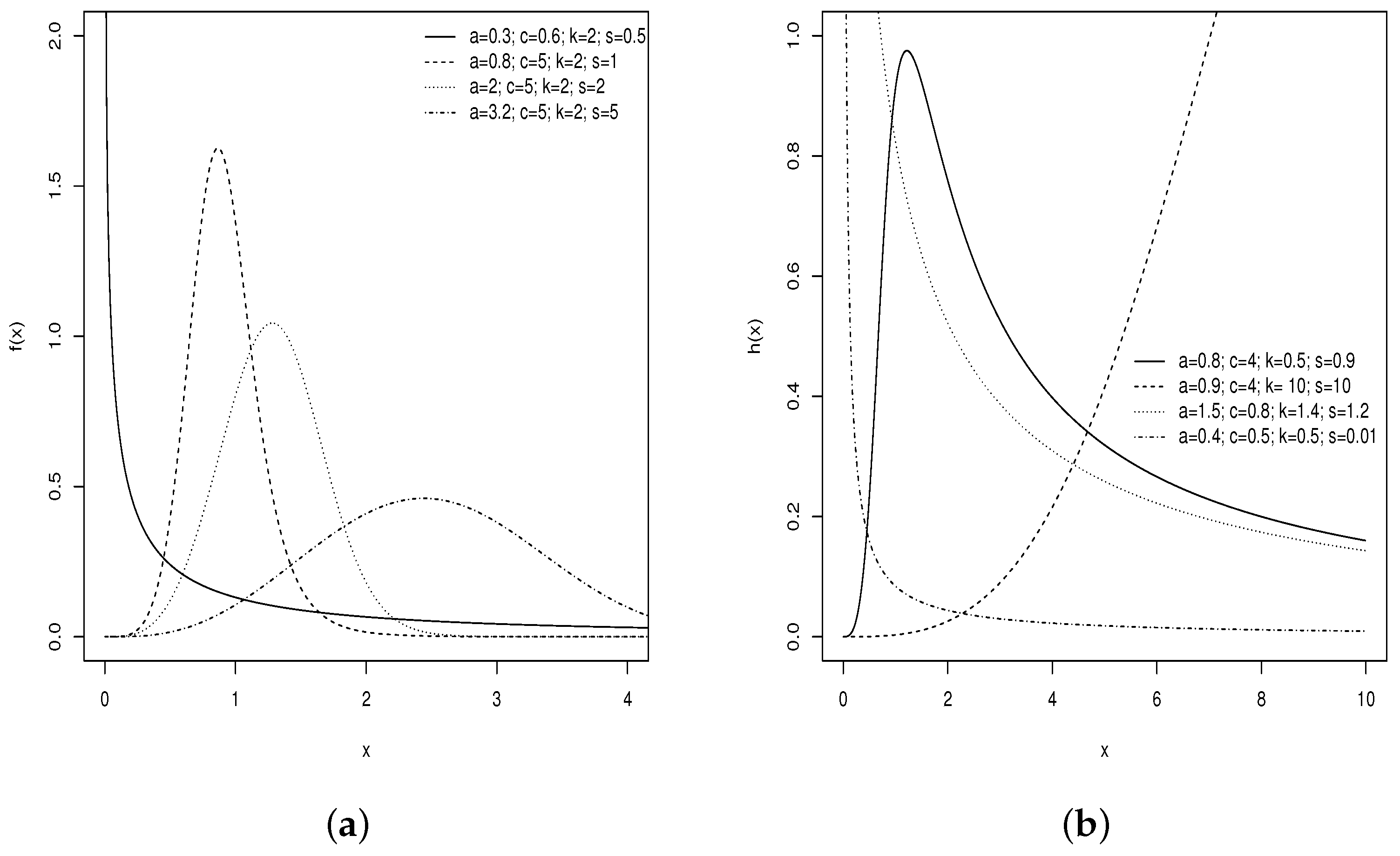

| No. | Distribution | Author(s) |
|---|---|---|
| 1. | Gamma exponentiated exponential | [8] |
| 2. | Gamma exponentiated Weibull | [15] |
| 3. | Gamma Pareto | [16] |
| 4. | Gamma uniform | [17] |
| 5. | Gamma extended Fréchet | [18] |
| 6. | Gamma half normal | [19] |
| 7. | ZB-LL | [20] |
| 8. | Gamma linear failure rate | [21] |
| 9. | Gamma Dagum | [22,23] |
| 10. | Gamma normal | [24] |
| 11. | Gamma generalized Weibull | [11] |
| 12. | Gamma log-normal | [9] |
| 13. | Gamma Weibull | [9,10] |
| 14. | Gamma Gumbel | [9,10] |
| 15. | ZB-MW | [13] |
| 16. | Gamma half-Cauchy | [25] |
| 17. | Gamma logistic | [10] |
| 18. | Gamma exponentiated exponential Weibull | [26] |
| 19. | Gamma Burr type X | [27] |
| 20. | Gamma Maxwell | [28] |
| 21. | Gamma log-logistic Weibull | [29] |
| 22. | RB-BXII | [14] |
| 23. | Gamma Burr III | [30] |
| 24. | Gamma Slash | [31] |
| 25. | Gamma extended exponential | [32] |
| 26. | Gamma Lindley–Poisson | [33] |
| 27. | Gamma Kumaraswamy | [34] |
| 28. | Gamma Rayleigh | [35] |
| 29. | Gamma power half-logistic | [36] |
| 30. | Gamma log-logistic Erlang truncated exponential | [37] |
| 31. | Gamma generalized Maxwell | [38] |
| 32. | Gamma generalized Lindley log-logistic | [39] |
| 33. | Gamma power Lindley | [40] |
| 34. | Gamma Chen | [41] |
| 35. | Gamma Lindley | [42] |
| 36. | Gamma Gompertz | [43] |
| (0.9, 0.6, 1.2, 1.5) | (1.9, 0.4, 0.8, 2.5) | (2.0, 0.2, 0.2, 0.6) | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| AE | Bias | MSE | AE | Bias | MSE | AE | Bias | MSE | ||
| 50 | a | 0.9728 | 0.0728 | 1.0307 | 2.0957 | 0.4197 | 3.8420 | 2.1033 | 0.1033 | 0.6619 |
| 1.0450 | 0.4450 | 2.0660 | 1.2353 | 0.8353 | 4.2478 | 0.2480 | 0.0480 | 0.0501 | ||
| 2.3106 | 1.1106 | 12.8133 | 2.3474 | 1.5474 | 14.7544 | 0.4817 | 0.2817 | 0.3140 | ||
| 1.4252 | −0.0747 | 1.5578 | 2.2546 | −0.2453 | 2.1023 | 0.5540 | −0.0459 | 0.0449 | ||
| 100 | a | 0.9304 | 0.0304 | 0.5110 | 1.9530 | 0.0530 | 2.1756 | 2.0475 | 0.0475 | 0.5442 |
| 0.8391 | 0.2391 | 0.6581 | 0.8094 | 0.4094 | 1.1510 | 0.2387 | 0.0387 | 0.0427 | ||
| 1.9481 | 0.7481 | 4.5213 | 1.7120 | 0.9120 | 4.4432 | 0.3222 | 0.1222 | 0.0972 | ||
| 1.4085 | −0.0914 | 0.8180 | 2.3235 | −0.1764 | 1.2409 | 0.5987 | −0.0012 | 0.0230 | ||
| 200 | a | 0.8961 | −0.0038 | 0.2995 | 1.8995 | −0.0004 | 1.1542 | 1.9906 | −0.0093 | 0.4053 |
| 0.6618 | 0.0618 | 0.1359 | 0.5345 | 0.1345 | 0.1900 | 0.2201 | 0.0201 | 0.0318 | ||
| 1.6463 | 0.4463 | 1.3229 | 1.3082 | 0.5082 | 1.7515 | 0.2511 | 0.0511 | 0.0205 | ||
| 1.4269 | −0.0730 | 0.2520 | 2.3528 | −0.1471 | 0.4407 | 0.6161 | 0.0161 | 0.0106 | ||
| 500 | a | 0.8995 | −0.0004 | 0.2667 | 1.9002 | 0.0002 | 1.0055 | 2.0173 | 0.0173 | 0.3730 |
| 0.6509 | 0.0509 | 0.0947 | 0.4973 | 0.0973 | 0.1134 | 0.2243 | 0.0243 | 0.0275 | ||
| 1.5854 | 0.3854 | 1.0946 | 1.2290 | 0.4290 | 1.3054 | 0.2384 | 0.0384 | 0.0164 | ||
| 1.4175 | −0.0824 | 0.1764 | 2.3720 | −0.1279 | 0.2932 | 0.6113 | 0.0113 | 0.0083 | ||
| (0.9, 2.5, 0.1, 0.2) | (0.5, 7.0, 0.3, 0.4) | (1.5, 3.2, 0.8, 0.1) | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| AE | Bias | MSE | AE | Bias | MSE | AE | Bias | MSE | ||
| 50 | a | 1.1745 | 0.2745 | 0.8229 | 0.5867 | 0.0867 | 0.3093 | 3.4171 | 1.9171 | 20.8649 |
| c | 3.5301 | 1.0301 | 10.8446 | 9.0703 | 2.0703 | 36.0733 | 4.0026 | 0.8026 | 2.2241 | |
| d | 0.1284 | 0.0284 | 0.0147 | 0.3496 | 0.0496 | 0.0481 | 2.1072 | 1.3072 | 24.2399 | |
| s | 0.3119 | 0.1119 | 0.0991 | 0.4308 | 0.0308 | 0.1342 | 0.2113 | 0.1113 | 0.0939 | |
| 100 | a | 1.0775 | 0.1775 | 0.4930 | 0.5820 | 0.0820 | 0.1547 | 2.5576 | 1.0576 | 8.4377 |
| c | 3.1230 | 0.6230 | 4.5324 | 8.1806 | 1.1806 | 17.0091 | 3.6160 | 0.4160 | 0.9437 | |
| d | 0.1162 | 0.0162 | 0.0085 | 0.3147 | 0.0147 | 0.0240 | 1.1418 | 0.3418 | 3.5938 | |
| s | 0.2546 | 0.0546 | 0.0299 | 0.4122 | 0.0122 | 0.0025 | 0.1461 | 0.0461 | 0.0250 | |
| 200 | a | 0.9556 | 0.0556 | 0.2081 | 0.5650 | 0.0650 | 0.0788 | 1.8870 | 0.3870 | 0.9241 |
| c | 2.7397 | 0.2397 | 1.2328 | 7.3936 | 0.3936 | 2.4543 | 3.3788 | 0.1788 | 0.2119 | |
| d | 0.1127 | 0.0127 | 0.0040 | 0.3008 | 0.0008 | 0.0142 | 0.7529 | −0.0470 | 0.1796 | |
| s | 0.2143 | 0.0143 | 0.0046 | 0.4055 | 0.0055 | 0.0007 | 0.1069 | 0.0069 | 0.0008 | |
| 500 | a | 0.9265 | 0.0265 | 0.1413 | 0.5518 | 0.0518 | 0.0596 | 1.8267 | 0.3267 | 0.5674 |
| c | 2.6120 | 0.1120 | 0.2713 | 7.2273 | 0.2273 | 1.2589 | 3.3451 | 0.1451 | 0.1435 | |
| d | 0.1133 | 0.0133 | 0.0034 | 0.3021 | 0.0021 | 0.0106 | 0.7453 | -0.0546 | 0.1428 | |
| s | 0.2084 | 0.0084 | 0.0025 | 0.4045 | 0.0045 | 0.0005 | 0.1047 | 0.0047 | 0.0001 | |
| Mean | Median | SD | Variance | Skewness | Kurtosis | Min. | Max. |
|---|---|---|---|---|---|---|---|
| 55.660 | 31.000 | 54.838 | 3007.3 | 1.180 | 3.612 | 1 | 243 |
| Model | MLEs (SEs) | |||
|---|---|---|---|---|
| ZB-BXII | ||||
| () | () | () | () | |
| RB-BXII | ||||
| () | () | () | () | |
| ZB-MW | ||||
| () | () | () | () | |
| RB-MW | ||||
| () | () | () | () | |
| ZB-LL | ||||
| () | () | () | ||
| RB-LL | ||||
| () | () | () | ||
| BXII | ||||
| () | () | () | ||
| MW | ||||
| () | () | () | ||
| LL | ||||
| () | () | |||
| Model | AIC | CAIC | BIC | HQIC | ||
|---|---|---|---|---|---|---|
| ZB-BXII | 0.260 | 1.663 | ||||
| RB-BXII | ||||||
| ZB-MW | ||||||
| RB-MW | ||||||
| ZB-LL | ||||||
| RB-LL | 1036.393 | 1036.635 | 1044.297 | 1039.594 | ||
| BXII | ||||||
| MW | ||||||
| LL |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cordeiro, G.M.; Ferreira, A.A. The Gamma-G Family: Brief Survey and COVID-19 Application. Stats 2025, 8, 21. https://doi.org/10.3390/stats8010021
Cordeiro GM, Ferreira AA. The Gamma-G Family: Brief Survey and COVID-19 Application. Stats. 2025; 8(1):21. https://doi.org/10.3390/stats8010021
Chicago/Turabian StyleCordeiro, Gauss M., and Alexsandro A. Ferreira. 2025. "The Gamma-G Family: Brief Survey and COVID-19 Application" Stats 8, no. 1: 21. https://doi.org/10.3390/stats8010021
APA StyleCordeiro, G. M., & Ferreira, A. A. (2025). The Gamma-G Family: Brief Survey and COVID-19 Application. Stats, 8(1), 21. https://doi.org/10.3390/stats8010021







