Effect of Slope on the Frequency and Height of Fire Whirls
Abstract
1. Introduction
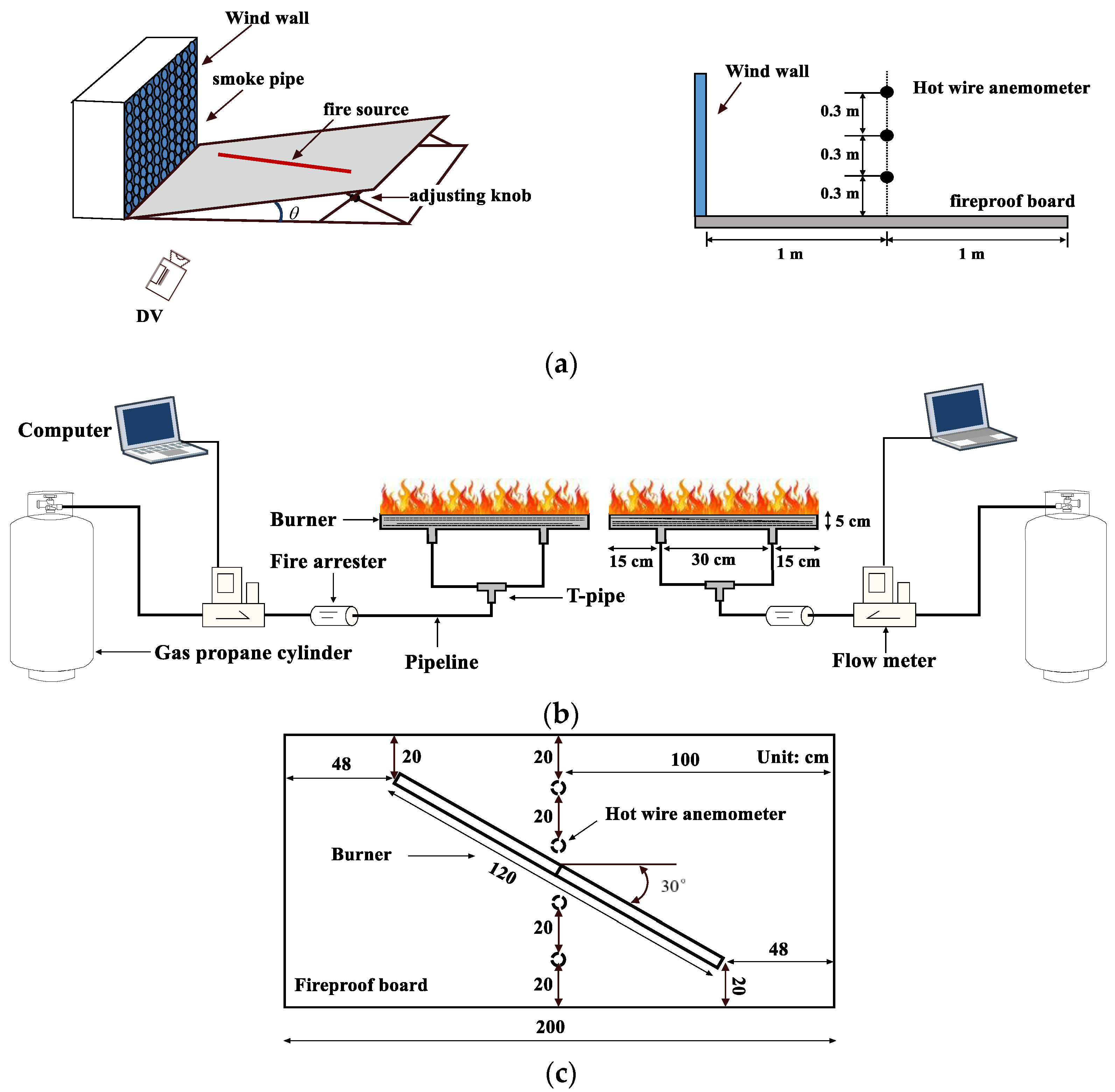
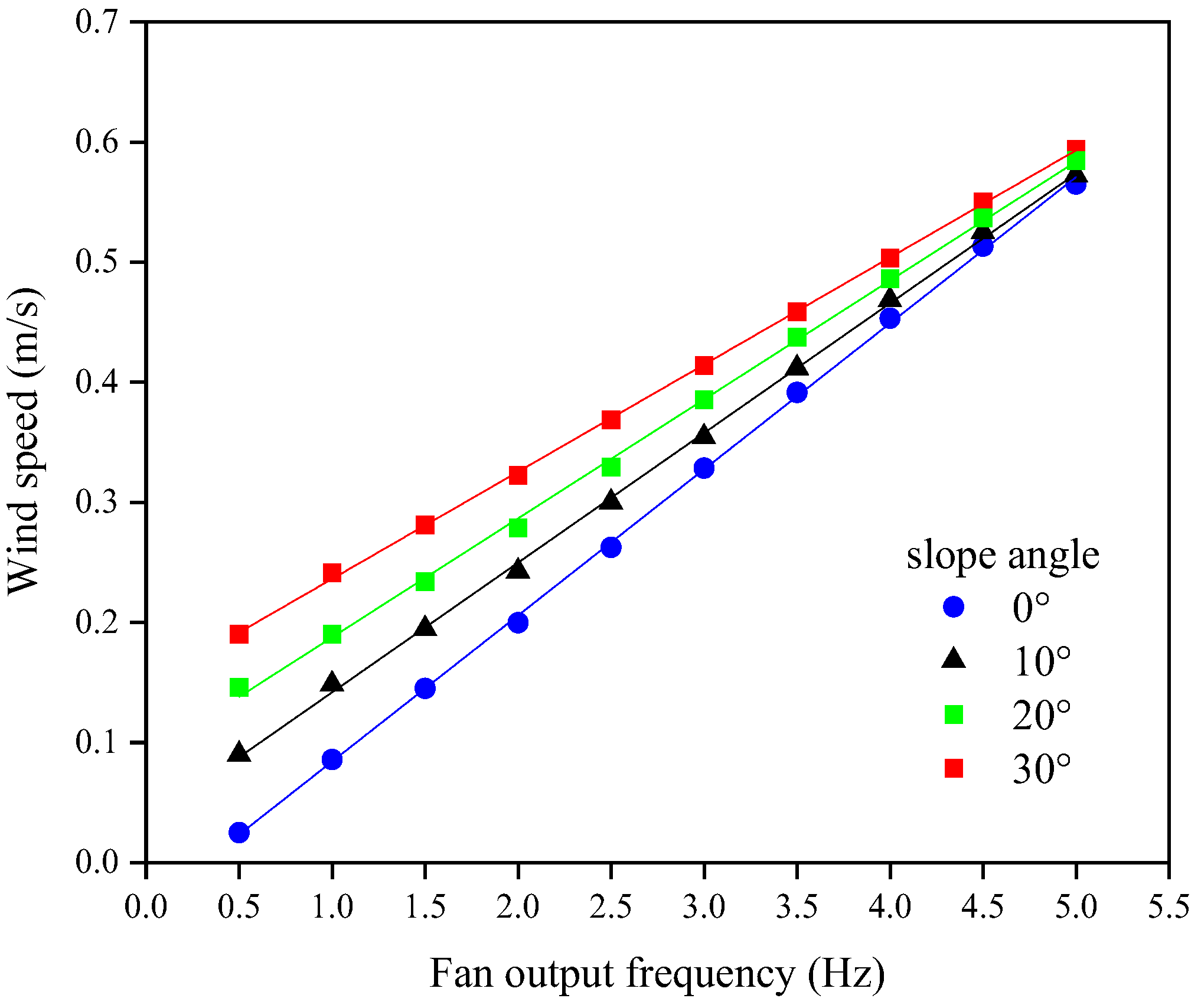
2. Experimental
3. Results and Discussion
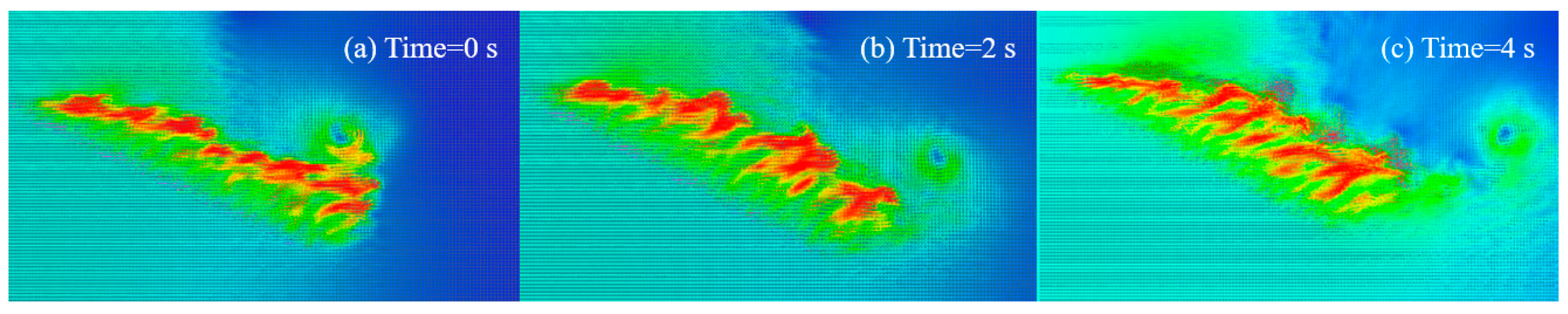
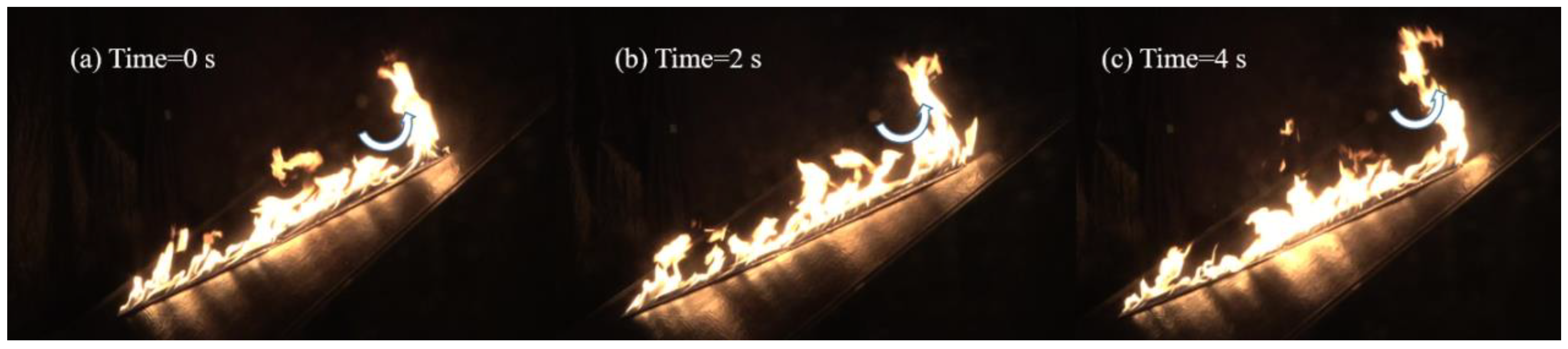
3.1. Experimental Observation
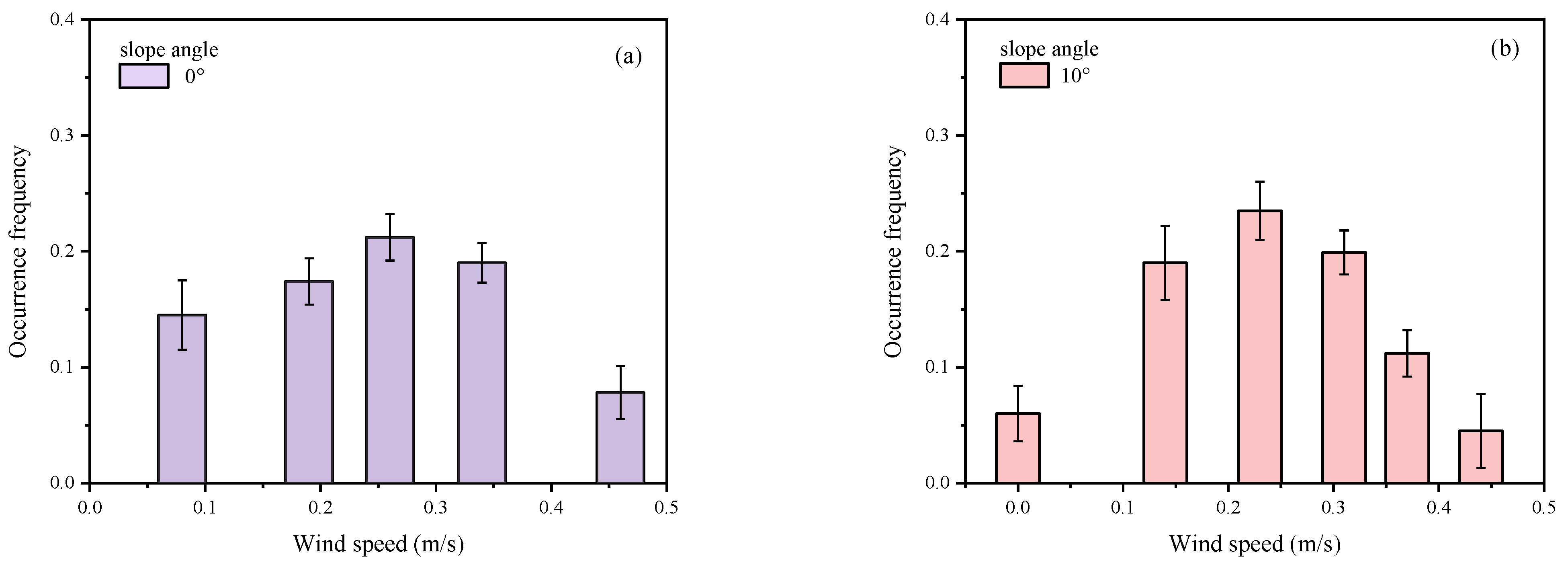
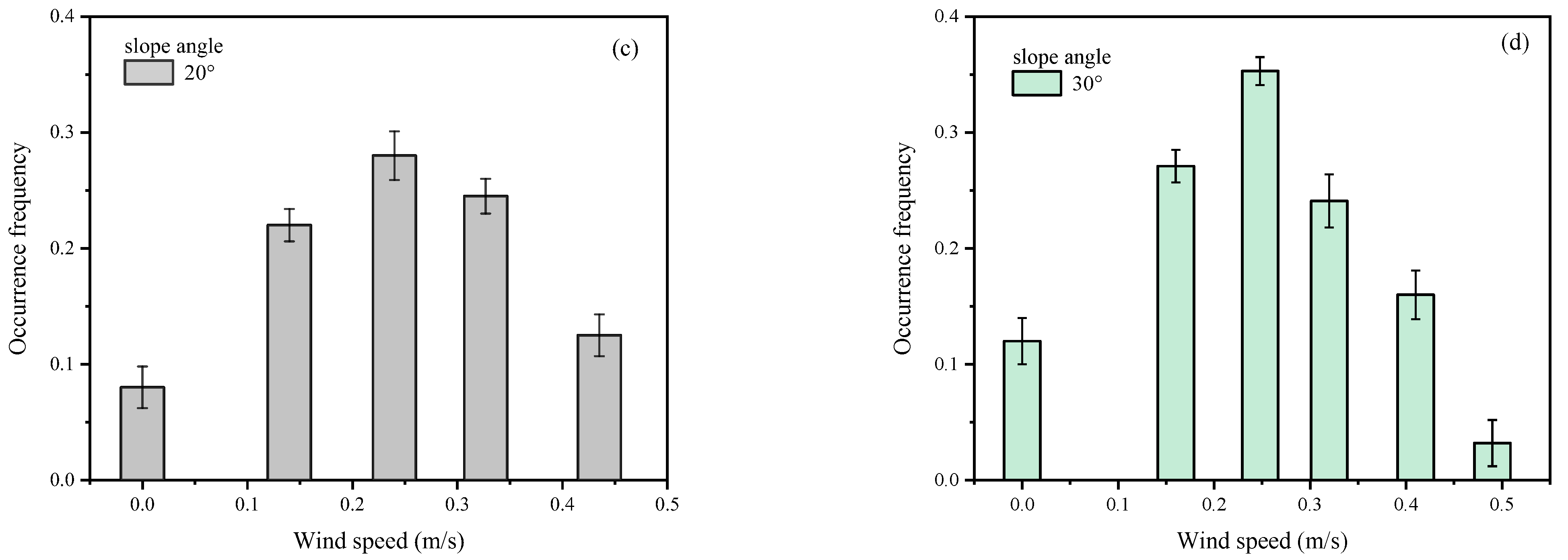
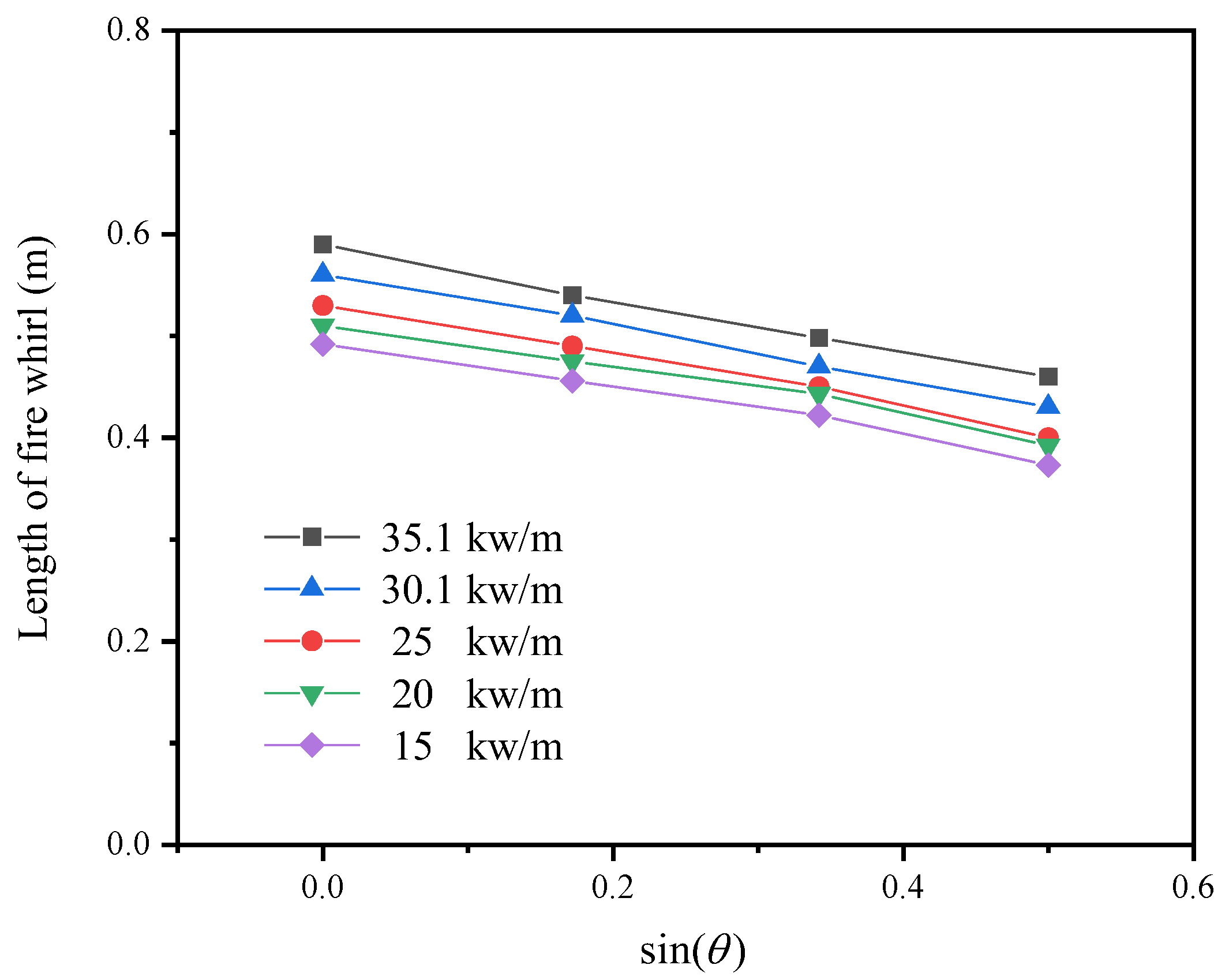
3.2. Fire Whirl Generation Frequency and Height
3.3. Condition and Mechanism of Fire Whirl Formation
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Forthofer, J.M.; Goodrick, S.L. Review of Vortices in Wildland Fire. J. Combust. 2011, 2011, 984363. [Google Scholar] [CrossRef]
- Ghodrat, M.; Shakeriaski, F.; Nelson, D.J.; Simeoni, A. Experimental and Numerical Analysis of Formation and Flame Precession of Fire Whirls: A Review. Fire 2021, 4, 43. [Google Scholar] [CrossRef]
- Kazunori, K. Fire Whirls: Why Are They Tall, and When Do They Occur? J. Combust. Soc. Jpn. 2019, 61, 101–111. [Google Scholar]
- Snow, J.; Scott, R.B. 12b.4 strategic directions for wsr-88d doppler weather surveillance radar in the period Strategic Directions for WSR-88D Doppler Weather Surveillance Radar in the Period 2007–2025. 2022. [Google Scholar]
- Dupuy, J.L.; Maréchal, J.; Portier, D.; Valette, J.C. The effects of slope and fuel bed width on laboratory fire behaviour. Int. J. Wildland Fire 2011, 20, 272. [Google Scholar] [CrossRef]
- Silvani, X.; Morandini, F.; Dupuy, J.-L. Effects of slope on fire spread observed through video images and multiple-point thermal measurements. Exp. Therm. Fluid Sci. 2012, 41, 99–111. [Google Scholar] [CrossRef]
- Weiss, A.D.; Rajamanickam, P.; Coenen, W.; Sánchez, A.L.; Williams, F.A. A model for the constant-density boundary layer surrounding fire whirls. J. Fluid Mech. 2020, 900, A22. [Google Scholar] [CrossRef]
- Liu, N.; Liu, Q.; Deng, Z.; Kohyu, S.; Zhu, J. Burn-out time data analysis on interaction effects among multiple fires in fire arrays. Proc. Combust. Inst. 2007, 31, 2589–2597. [Google Scholar] [CrossRef]
- Kuwana, K.; Sekimoto, K.; Minami, T.; Tashiro, T.; Saito, K. Scale-model experiments of moving fire whirl over a line fire. Proc. Combust. Inst. 2013, 34, 2625–2631. [Google Scholar] [CrossRef]
- Sasaki, T.; Igari, M.; Kuwana, K. Fire whirls behind an L-shaped wall in a crossflow. Combust. Flame 2018, 197, 197–203. [Google Scholar] [CrossRef]
- Kuwana, K.; Sekimoto, K.; Saito, K.; Williams, F.A. Scaling fire whirls. Fire Saf. J. 2008, 43, 252–257. [Google Scholar] [CrossRef]
- Shinohara, M. Vortex strength and size of fire whirls without flames around a long narrow fire source. Fire Saf. J. 2022, 129, 103561. [Google Scholar] [CrossRef]
- Palacios, A.; Bradley, D. Wildfires and the generation of fire whirls. Combust. Flame 2021, 239, 111664. [Google Scholar] [CrossRef]
- Tohidi, A.; Gollner, M.J.; Xiao, H. Fire Whirls. Annu. Rev. Fluid Mech. 2018, 50, 187–213. [Google Scholar] [CrossRef]
- Graham, H.E. Fire-whirlwind formation as favored by topography and upper winds: Fire whirlwinds studied in the lab [reprinted from 1957]. 2003. [Google Scholar]
- Umscheid, M.E.; Monteverdi, J.P.; Davies, J.M. Photographs and Analysis of an Unusually Large and Long-Lived Firewhirl. E J. Sev. Storms Meteorol. 2006, 1, 1–13. [Google Scholar] [CrossRef]
- Morandini, F.; Silvani, X.; Dupuy, J.L.; Susset, A. Fire spread across a sloping fuel bed: Flame dynamics and heat transfers. Combust. Flame 2018, 190, 158–170. [Google Scholar] [CrossRef]
- Himoto, K.; Naruse, T. Probabilistic aspect of fire whirl generation around an L-shaped fire source in a crosswind. Fire Saf. J. 2017, 88, 89–95. [Google Scholar] [CrossRef]
- Zhou, K.; Liu, N.; Yuan, X. Effect of wind on fire whirl over a line fire. Fire Technol. 2016, 52, 865–875. [Google Scholar] [CrossRef]
- Zhou, K.; Liu, N.; Yin, P.; Yuan, X.; Jiang, J. Fire Whirl due to Interaction between Line Fire and Cross Wind. Fire Saf. Sci. 2014, 11, 1420–1429. [Google Scholar] [CrossRef]
- Nelson, R.M. An effective wind speed for models of fire spread. Int. J. Wildland Fire 2002, 11, 153–161. [Google Scholar] [CrossRef]
- Huo, Y.; Chow, W.K.; Chow, C.L. Generation and characteristics of internal fire whirl in a shaft model with two corner slits under microgravity conditions. Adv. Space Res. 2017, 59, 3058–3069. [Google Scholar] [CrossRef]
- Hartl, K.A.; Smits, A.J. Stereo PIV measurements in fire whirls. Exp. Fluids 2019, 60, 17. [Google Scholar] [CrossRef]
- Hartl, K.A.; Smits, A.J. Scaling of a small scale burner fire whirl. Combust. Flame 2016, 163, 202–208. [Google Scholar] [CrossRef]
- Coenen, W.; Kolb, E.J.; Sánchez, A.L.; Williams, F.A. Observed dependence of characteristics of liquid-pool fires on swirl magnitude. Combust. Flame 2019, 205, 1–6. [Google Scholar] [CrossRef]
- Li, S.; Yao, Q.; Law, C.K. The bottom boundary-layer structure of fire whirls. Proc. Combust. Inst. 2019, 37, 4277–4284. [Google Scholar] [CrossRef]
- Chuah, K.H.; Kushida, G. The prediction of flame heights and flame shapes of small fire whirls. Proc. Combust. Inst. 2007, 31, 2599–2606. [Google Scholar] [CrossRef]
- Emmons, H.W.; Ying, S.-J. The fire whirl. Symp. (Int.) Combust. 1967, 11, 475–488. [Google Scholar] [CrossRef]
- Meroney, R.N. Fire whirls, fire tornadoes, and fire storms: Physical and numerical modeling. In Proceedings of the International Workshop on Physical Modelling of Flow and Dispersion Phenomena, Prato, Italy, 3–5 September 2003. [Google Scholar]
- Byram, G.M.; Martin, R.E. The modeling of fire whirlwinds. For. Sci. 1970, 16, 386–399. [Google Scholar]
- Simpson, C.C.; Sharples, J.J.; Evans, J.P. Sensitivity of atypical lateral fire spread to wind and slope. Geophys. Res. Lett. 2016, 43, 1744–1751. [Google Scholar] [CrossRef]
- Chuah, K.H.; Kuwana, K.; Saito, K.; Williams, F.A. Inclined fire whirls. Proc. Combust. Inst. 2011, 33, 2417–2424. [Google Scholar] [CrossRef]



| Layout Types | |||
|---|---|---|---|
| Linear line fire | 0° | 15, 20, 25, 30.1, 35.1 | 0.08, 0.19, 0.24, 0.34, 0.46 |
| 10° | 15, 20, 25, 30.1, 35.1 | 0.14, 0.24, 0.31, 0.37, 0.44 | |
| 20° | 15, 20, 25, 30.1, 35.1 | 0.14, 0.24, 0.34, 0.45 | |
| 30° | 15, 20, 25, 30.1, 35.1 | 0.19, 0.24, 0.32, 0.49 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Zhou, K. Effect of Slope on the Frequency and Height of Fire Whirls. Fire 2023, 6, 189. https://doi.org/10.3390/fire6050189
Wang Y, Zhou K. Effect of Slope on the Frequency and Height of Fire Whirls. Fire. 2023; 6(5):189. https://doi.org/10.3390/fire6050189
Chicago/Turabian StyleWang, Yifan, and Kuibin Zhou. 2023. "Effect of Slope on the Frequency and Height of Fire Whirls" Fire 6, no. 5: 189. https://doi.org/10.3390/fire6050189
APA StyleWang, Y., & Zhou, K. (2023). Effect of Slope on the Frequency and Height of Fire Whirls. Fire, 6(5), 189. https://doi.org/10.3390/fire6050189







