UAV-Assisted Wideband Terahertz Wireless Communications with Time-Delay Phased UPA under Beam Squint
Abstract
:1. Introduction
- Firstly, we analyze the impact of the 2D beam squint effect on the phased UPA and propose a time-delay phased UPA extended from [16], which can be applied in both single-beam large bandwidth communication for single user case and multi-beam large-scale access scenarios for multi-user case.
- Secondly, considering the commonly assumed LoS path in UAV communication, although it can effectively improve gain in traditional ground communication, the presence of beam squint in broadband terahertz communication will seriously affect performance. Therefore, we will apply the time-delay phased UPA studied in unmanned aerial vehicle communication to improve the overall system gain by eliminating or utilizing beam squints, thereby perfectly adapting to UAV communication.
- Thirdly, considering the fact that the beams are more fine-grained in UPA due to the extra vertical dimension, the beam design method of ULA cannot be directly applied to UPA. We further propose a frequency division beam multiple access (FDBMA) strategy to deal with uneven vertical beam distribution. The position of the drone is also variable, making it more suitable for the proposed multi user transmission process.
- Finally, to further enlarge the coverage of the beam in the multi-user case, influence of the beamwidth is also analyzed through antenna dropout.
2. System Model and Beam Squint
2.1. System Model
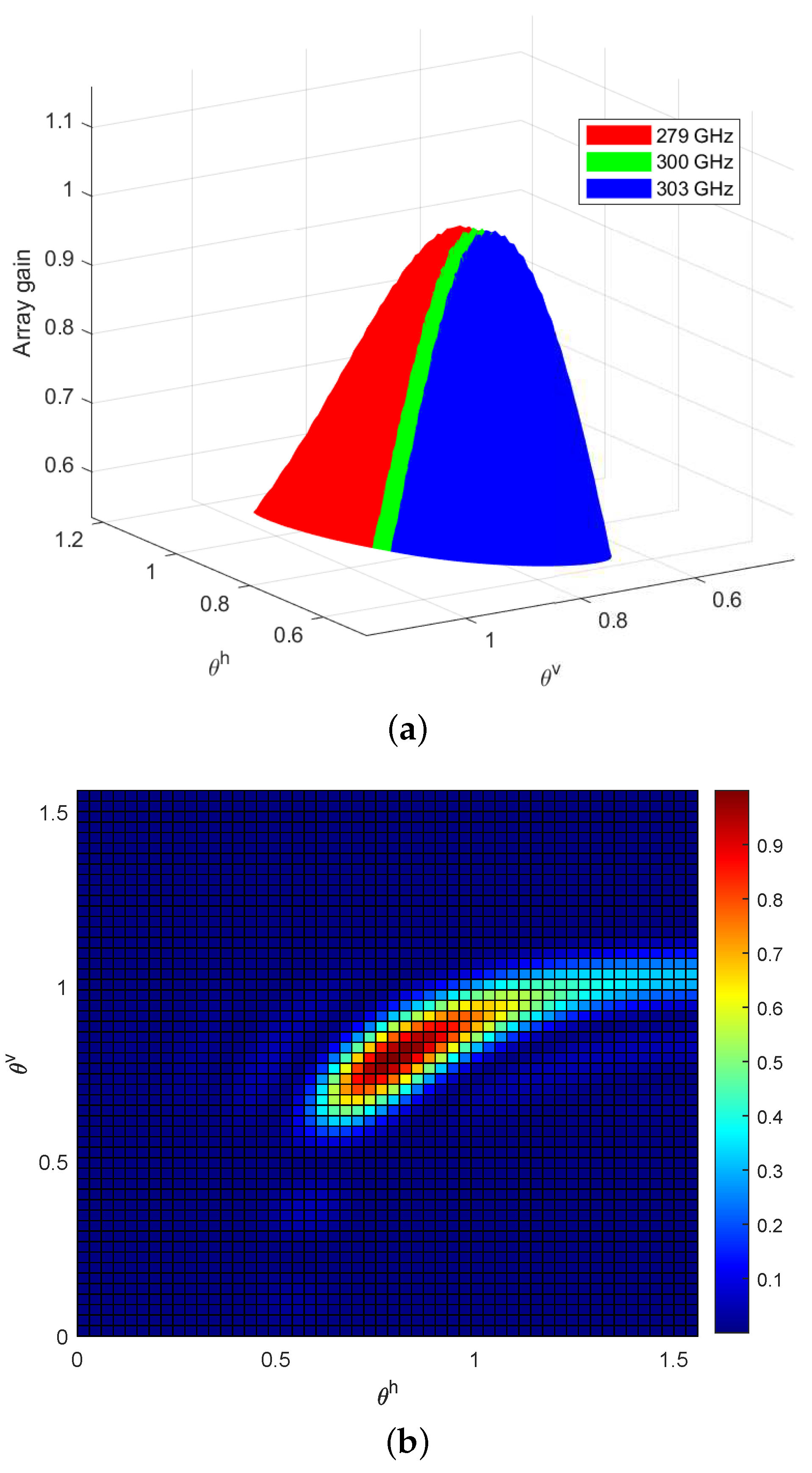
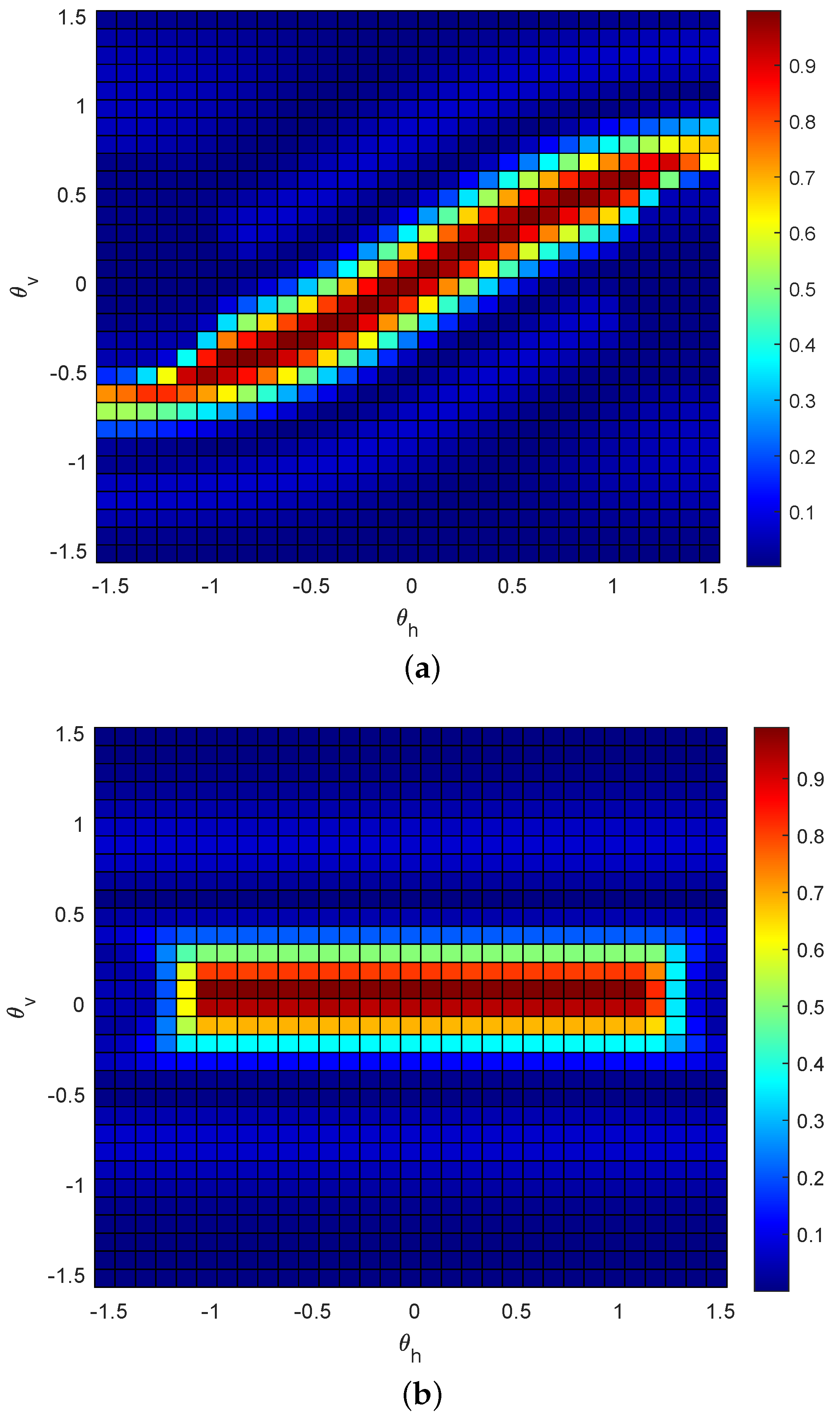
2.2. 2D Beam Squint Effect of the UPA
3. The Proposed Architecture and Applications in UAV Communications
- Eliminate 2D beam squint and increase the array gain at all frequencies to improve the rate of wideband communication (single-beam mode);
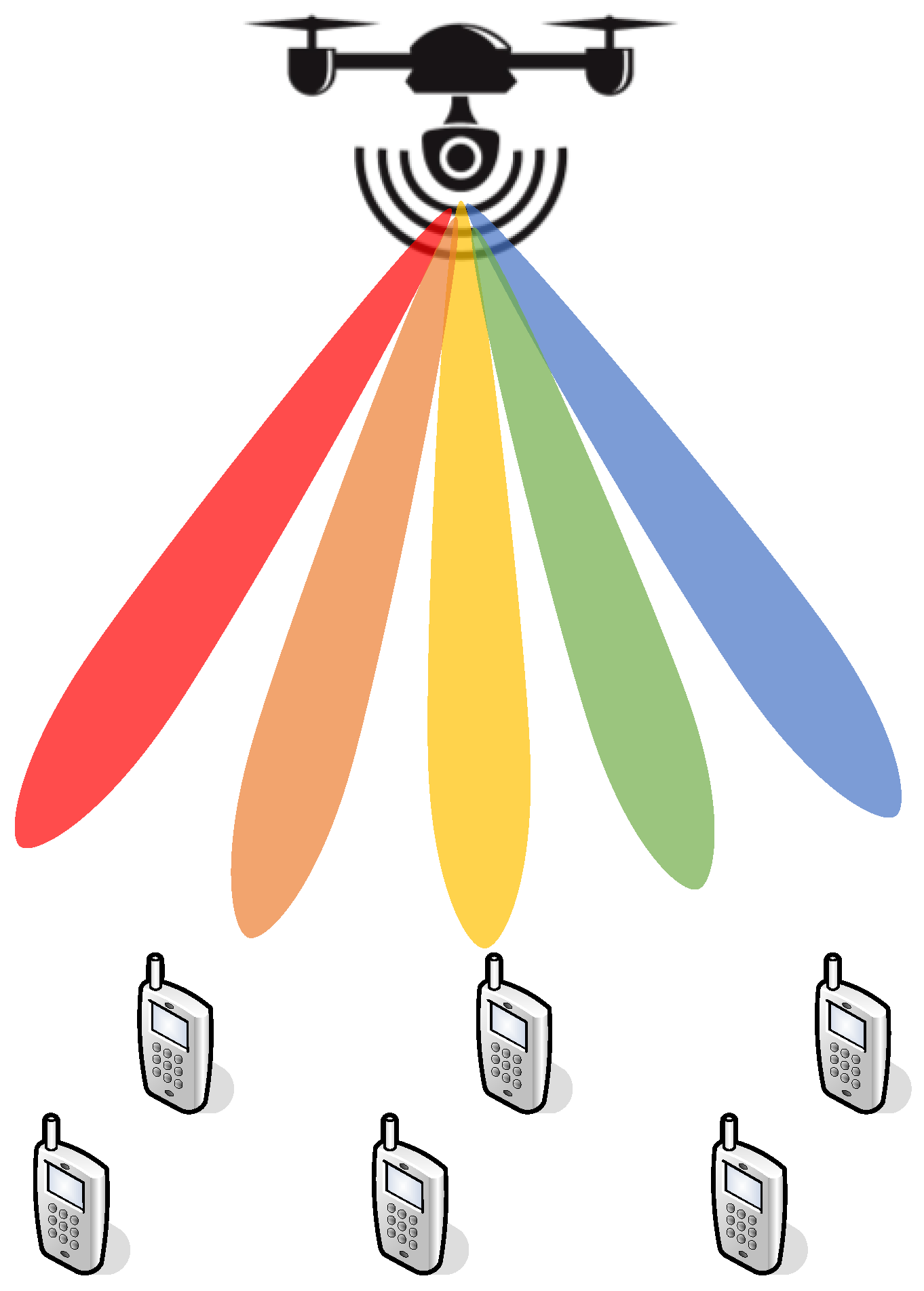
- Enhance the 2D beam squint to increase the coverage and improve system accessibility and rate (multi-beam mode).
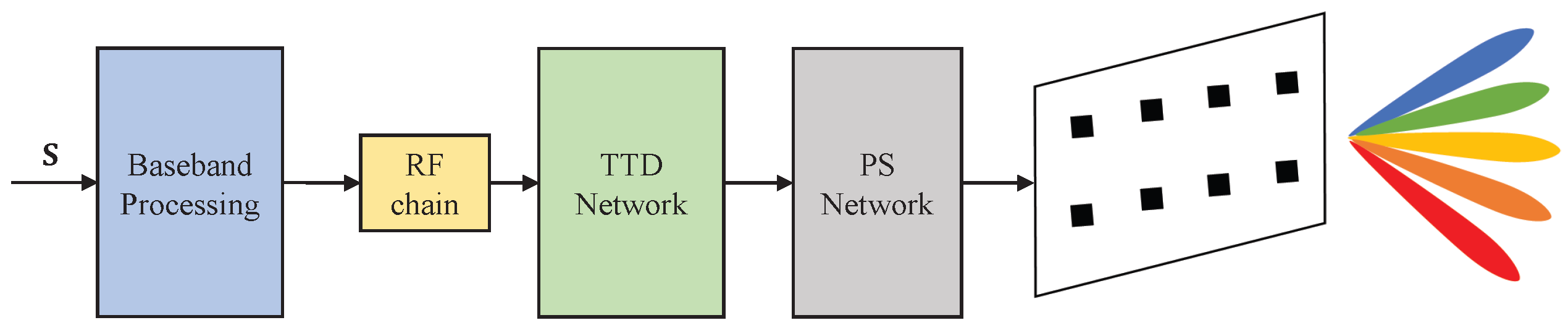
3.1. Time-Delay Phased UPA
3.2. Application Scenarios in UAV Communications
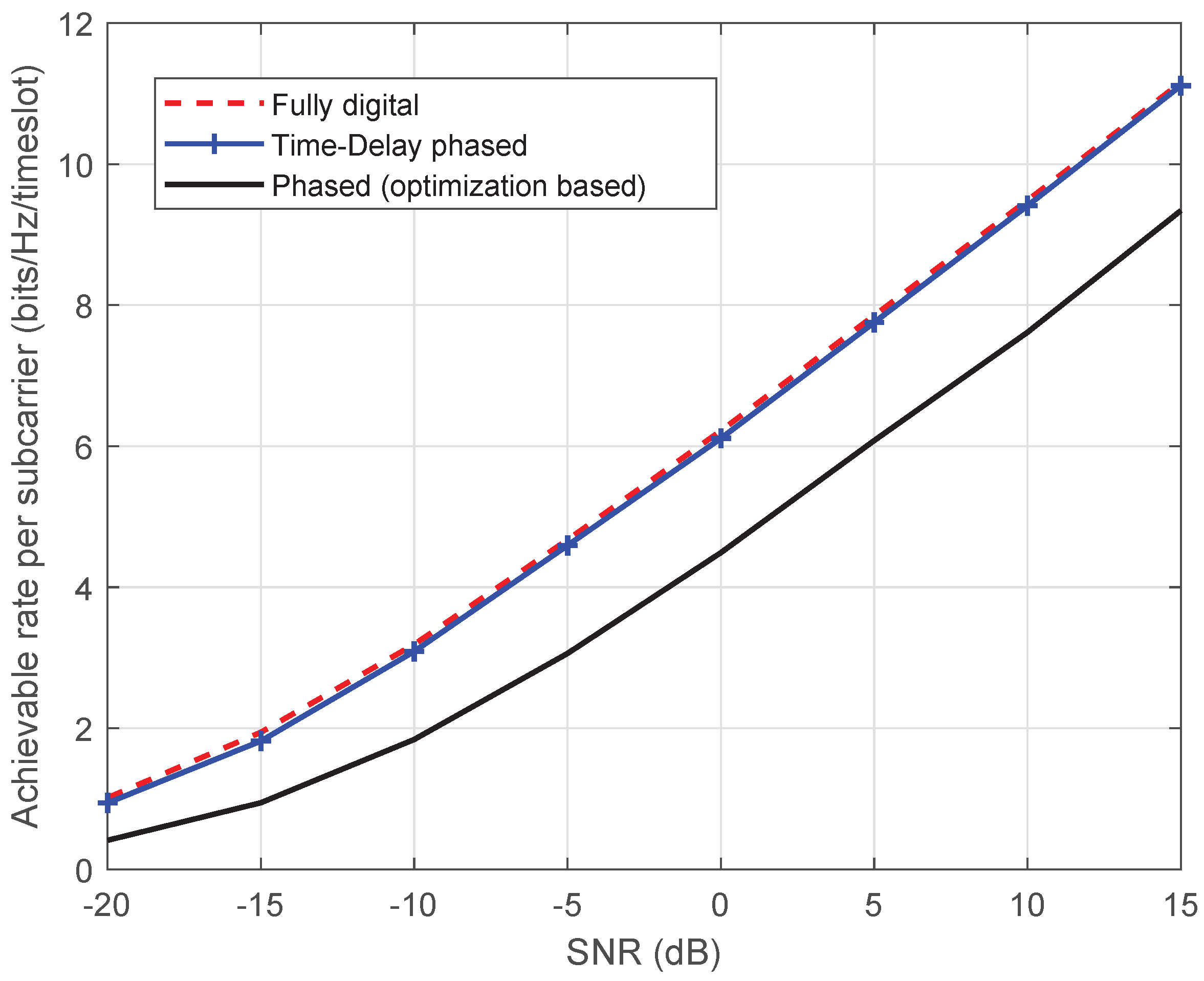
3.2.1. Single User Case
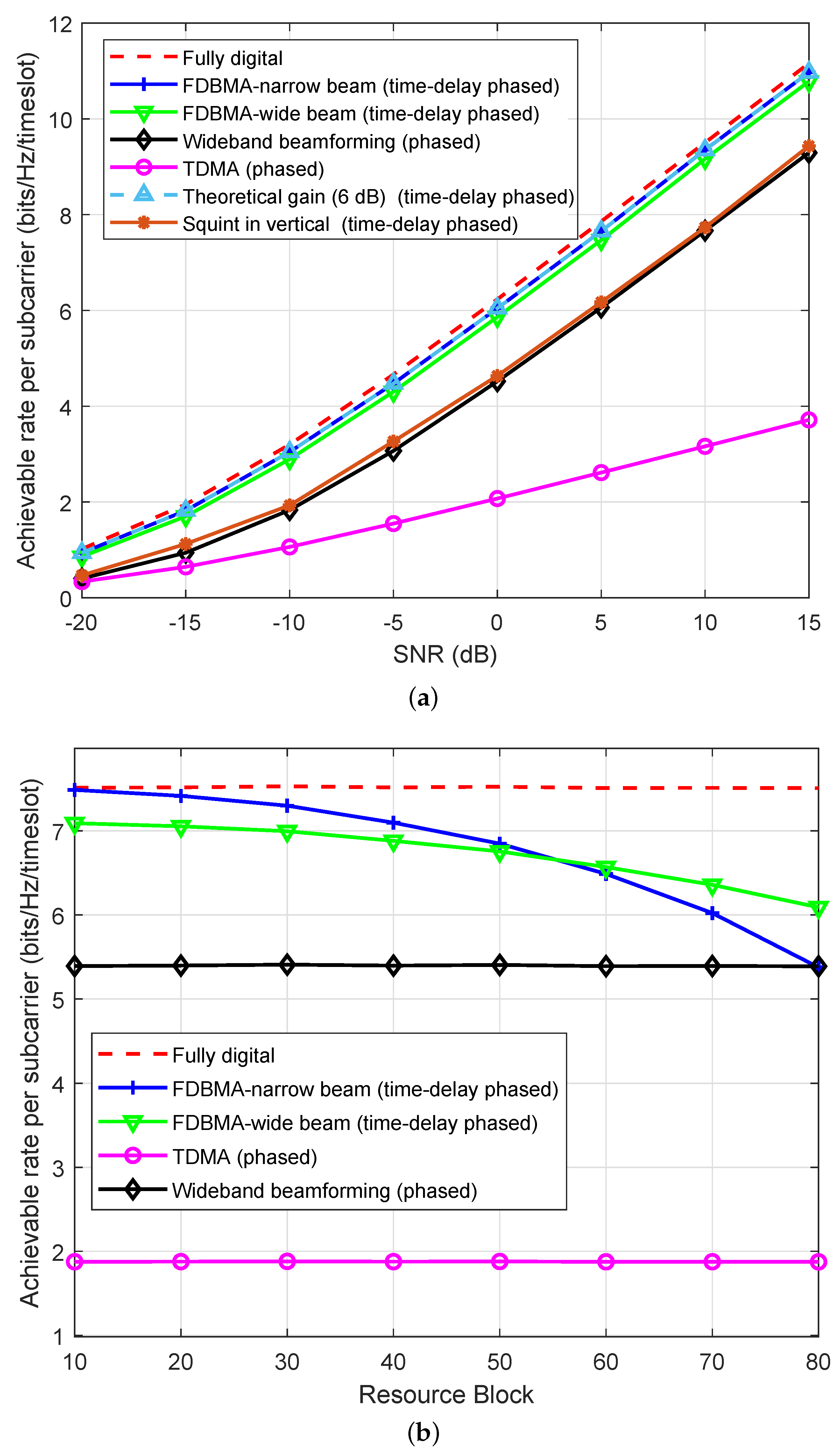
3.2.2. Multi-User Case
4. Simulation Results
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Gui, G.; Liu, M.; Tang, F.; Kato, N.; Adachi, F. 6g: Opening new horizons for integration of comfort, security, and intelligence. IEEE Wirel. Commun. 2020, 27, 126–132. [Google Scholar] [CrossRef]
- Han, C.; Chen, Y. Propagation modeling for wireless communications in the terahertz band. IEEE Commun. Mag. 2018, 56, 96–101. [Google Scholar] [CrossRef]
- Li, Z.; Guan, L.; Li, C.; Radwan, A. A secure intelligent spectrum control strategy for future THz mobile heterogeneous networks. IEEE Commun. Mag. 2018, 56, 116–123. [Google Scholar] [CrossRef]
- Ning, B.; Chen, Z.; Chen, W.; Du, Y.; Fang, J. Terahertz multi-user massive mimo with intelligent reflecting surface: Beam training and hybrid beamforming. IEEE Trans. Veh. Technol. 2021, 70, 1376–1393. [Google Scholar] [CrossRef]
- Raghavan, V.; Subramanian, S.; Cezanne, J.; Sampath, A. Directional beamforming for millimeter-wave MIMO systems. In Proceedings of the 2015 IEEE Global Communications Conference (GLOBECOM)-IEEE Conference, San Diego, CA, USA, 6–10 December 2015; pp. 1–7. [Google Scholar]
- Huang, S.; Zhang, M.; Gao, Y.; Feng, Z. MIMO Radar Aided mmWave Time-Varying Channel Estimation in MU-MIMO V2X Communications. IEEE Trans. Wirel. Commun. 2021, 20, 7581–7594. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, D.; Qiao, D.; Zhang, L. Analysis of Indoor THz Communication Systems With Finite-Bit DACs and ADCs. IEEE Trans. Veh. Technol. 2022, 71, 375–390. [Google Scholar] [CrossRef]
- Li, D.; Qiao, D.; Zhang, L. Achievable Rate of Indoor THz Communication Systems with Finite-Bit ADCs. In Proceedings of the 2018 10th International Conference on Wireless Communications and Signal Processing (WCSP), Hangzhou, China, 18–20 October 2018; pp. 1–6. [Google Scholar]
- Lin, C.; Li, G.Y.L. Terahertz Communications: An Array-of-Subarrays Solution. IEEE Commun. Mag. 2016, 54, 124–131. [Google Scholar] [CrossRef]
- Gao, F.; Wang, B.; Xing, C.; An, J.; Li, G.Y. Wideband beamforming for hybrid massive MIMO terahertz communications. IEEE J. Sel. Areas Commun. 2021, 39, 1725–1740. [Google Scholar] [CrossRef]
- Wang, B.; Gao, F.; Jin, S.; Lin, H.; Li, G.Y. Spatial and frequency wideband effects in millimeter-wave massive mimo systems. IEEE Trans. Signal Process. 2018, 66, 3393–3406. [Google Scholar] [CrossRef]
- Zhao, J.; Liu, J.; Gao, F.; Jia, W.; Zhang, W. Gridless compressed sensing based channel estimation for UAV wideband communications with beam squint. IEEE Trans. Veh. Technol. 2021, 70, 10265–10277. [Google Scholar] [CrossRef]
- Wan, Q.; Fang, J.; Chen, Z.; Li, H. Hybrid precoding and combining for millimeter wave/sub-thz mimo-ofdm systems with beam squint effects. IEEE Trans. Veh. Technol. 2021, 70, 8314–8319. [Google Scholar] [CrossRef]
- Di, B. Reconfigurable holographic metasurface aided wideband ofdm communications against beam squint. IEEE Trans. Veh. Technol. 2021, 70, 5099–5103. [Google Scholar] [CrossRef]
- Boljanovic, V.; Yan, H.; Ghaderi, E.; Heo, D.; Gupta, S.; Cabric, D. Design of millimeter-wave single-shot beam training for true-time-delay array. In Proceedings of the 2020 IEEE 21st International Workshop on Signal Processing Advances in Wireless Communications (SPAWC), Atlanta, GA, USA, 26–29 May 2020; pp. 1–5. [Google Scholar]
- Zhai, B.; Zhu, Y.; Tang, A.; Wang, X. THzPrism: Frequency-based beam spreading for terahertz communication systems. IEEE Wireless Commun. Lett. 2020, 9, 897–900. [Google Scholar] [CrossRef]
- Zhai, B.; Tang, A.; Peng, C.; Wang, X. Ss-ofdma: Spatial-spread orthogonal frequency division multiple access for terahertz networks. IEEE J. Sel. Areas Commun. 2021, 39, 1678–1692. [Google Scholar] [CrossRef]
- Sohrabi, F.; Yu, W. Hybrid analog and digital beamforming for mmWave OFDM large-scale antenna arrays. IEEE J. Sel. Areas Commun. 2017, 35, 1432–1443. [Google Scholar] [CrossRef]
- Song, J.; Choi, J.; Love, D.J. Common codebook millimeter wave beam design: Designing beams for both sounding and communication with uniform planar arrays. IEEE Trans. Commun. 2017, 65, 1859–1872. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, A.; Xia, X.; Xu, K. Learning the structured sparsity: 3-d massive mimo channel estimation and adaptive spatial interpolation. IEEE Trans. Veh. Technol. 2019, 68, 10663–10678. [Google Scholar] [CrossRef]
- Xu, L.; Chen, M.; Chen, M.; Yang, Z.; Chaccour, C.; Saad, W.; Hong, C.S. Joint location, bandwidth and power optimization for thz-enabled uav communications. IEEE Commun. Lett. 2021, 25, 1984–1988. [Google Scholar] [CrossRef]
- Alali, A.; Rawat, D.B.; Liu, C. Trajectory and power optimization in sub-thz band for uav communications. In Proceedings of the ICC 2022—IEEE International Conference on Communications, Seoul, Republic of Korea, 16–20 May 2022; pp. 1–6. [Google Scholar]
- Fan, G.; Sun, J.; Gui, G.; Gacanin, H.; Adebisi, B.; Ohtsuki, T. Fully convolutional neural network-based csi limited feedback for fdd massive mimo systems. IEEE Trans. Cogn. Commun. Netw. 2022, 8, 672–682. [Google Scholar] [CrossRef]
- Ayach, O.E.; Rajagopal, S.; Abu-Surra, S.; Pi, Z.; Heath, R.W. Spatially sparse precoding in millimeter wave MIMO systems. IEEE Trans. Wirel. Commun. 2014, 13, 1499–1513. [Google Scholar] [CrossRef]
- Zhong, W.; Xu, L.; Zhu, Q.; Chen, X.; Zhou, J. MmWave beamforming for UAV communications with unstable beam pointing. China Commun. 2019, 16, 37–46. [Google Scholar] [CrossRef]
- Xia, W.; Semkin, V.; Mezzavilla, M.; Loianno, G.; Rangan, S. Multi-array designs for mmwave and sub-thz communication to uavs. In Proceedings of the 2020 IEEE 21st International Workshop on Signal Processing Advances in Wireless Communications (SPAWC), Atlanta, GA, USA, 26–29 May 2020; pp. 1–5. [Google Scholar]
- Pan, Y.; Wang, K.; Pan, C.; Zhu, H.; Wang, J. Uav-assisted and intelligent reflecting surfaces-supported terahertz communications. IEEE Wirel. Commun. Let. 2021, 10, 1256–1260. [Google Scholar] [CrossRef]
- Huang, H.; Gui, G.; Gacanin, H.; Yuen, C.; Sari, H.; Adachi, F. Deep regularized waveform learning for beam prediction with limited samples in non-cooperative mmwave systems. IEEE Trans. Veh. Technol. 2023, 72, 9614–9619. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, H.; Zheng, Q.; Sari, H. UAV-Assisted Wideband Terahertz Wireless Communications with Time-Delay Phased UPA under Beam Squint. Drones 2023, 7, 608. https://doi.org/10.3390/drones7100608
Huang H, Zheng Q, Sari H. UAV-Assisted Wideband Terahertz Wireless Communications with Time-Delay Phased UPA under Beam Squint. Drones. 2023; 7(10):608. https://doi.org/10.3390/drones7100608
Chicago/Turabian StyleHuang, Hao, Qinghe Zheng, and Hikmet Sari. 2023. "UAV-Assisted Wideband Terahertz Wireless Communications with Time-Delay Phased UPA under Beam Squint" Drones 7, no. 10: 608. https://doi.org/10.3390/drones7100608
APA StyleHuang, H., Zheng, Q., & Sari, H. (2023). UAV-Assisted Wideband Terahertz Wireless Communications with Time-Delay Phased UPA under Beam Squint. Drones, 7(10), 608. https://doi.org/10.3390/drones7100608







