2. Methods
The proposed new rat-tail exposure system is shown in
Figure 1. It is a modification of the existing NIOSH rat-tail model with an addition of a loading device [
3]. The loading device is composed of a loading plate, a set of loading springs, and the fixtures for installing the device on the vibration platform.
The vibration platform can be installed on a shaker or a vibration tool. The loading plate is placed on the middle region (C12–18) of the rat tail that is constrained on the vibration platform. The static force is applied to the tail by loading the plate by compressing the loading springs. The loading plate has a groove with a conical taper, which can conform to the tapering diameter along the tail and secure the tail to the vibration platform.
The rat tail is likely to have a nonlinear force-deformation relationship. However, the dynamic properties of the tail can be locally linearized to reduce the complexity of the system analysis. Hence, the new rat-tail vibration-exposure system under several static forces (F
PS) was simulated using a linear analytical model illustrated in
Figure 2.
The effective mass of the loading plate (MPE) includes the loading plate mass (MP), half of the mass of the loaded tail portion (MR), and that of loading springs (MSprings). The total stiffness of the exposure system (K) includes the tail stiffness (KR) and loading spring stiffness (KS). The total damping value includes the tail damping value (CR) and that of the loading system (CS).
Three biomechanical measures, including vibration stress, strain, and power-absorption density (
VPAD), were considered for the quantification of the tail-vibration exposure. The average static or dynamic stress/pressure at the tail-plate interface was estimated from
where
Lt is the plate length or tail contact length;
bt is the average tail contact width;
FPD is the complex dynamic force acting on the loaded portion of the tail.
FPD was estimated from
where
DP and
DV are the complex vibration displacements of the loading plate and vibration platform, respectively;
VP and
VV are their vibration velocities; and
Ap is the vibration acceleration of the loading plate.
The stress method assumes that the vibration stress at any point in the loaded portion of the tail (
σ) is approximately proportional to the average contact stress. It also assumes that vibration injuries or biological effects are correlated with the stress magnitude and the number of stress cycles. Hence, the vibration exposure dose using the stress method (
Γσ) for each frequency (
f) was calculated from
where
Τ is the time duration (seconds) of the rat-tail vibration exposure;
α is termed as stress frequency weighting;
is the stress-proportional factor; and
Iσ is termed as vibration-stress-dose index, which can be expressed as follows:
where j =
;
TP is the transfer function of the loading plate (=
AP/
AV), where
AV is the vibration acceleration of the platform.
The strain is a measure of the material relative deformation. The maximum static strain of the tail subjected to a static force was estimated from the maximum compression deformation (Δ) and the average diameter of the loaded-tail portion (d
t):
The average dynamic strain for a given static force was expressed as follows:
where
ht is the deformed height of the loaded tail loaded with a static force, which can be measured in a static test.
Similar to the stress method, the strain method assumes that the distributed strain at any point (
ε) is approximately proportional to the average dynamic strain, and the vibration injuries or biological effects are correlated with the strain and the number of strain cycles. Hence, the vibration exposure dose using the strain method (
Γε) can be written as follows:
where
β is termed as strain frequency weighting;
Q is the strain-proportional factor; and
Iε is termed as the vibration-strain-dose index, which is expressed as follows:
Similarly, with the model shown in
Figure 2, the
VPAD method for calculating the dose can be derived as follows:
where
ν is the volume of the loaded tail portion (≈
π∙(0.5d
t)
2∙
Lt); P is the
VPAD proportional factor; and
IVPAD is termed as
VPAD dose index:
A spring-calibration test, a damping test, and a rat-tail test were performed in this study to determine the system parameters, evaluate the feasibility of the new rat-tail model, and explore the characteristics of the exposure-dose indexes. The rat-tail test used a dissected tail obtained from a cadaver rat (the tissue collected post-mortem from the rat in a control group of another study).
4. Discussion and Conclusions
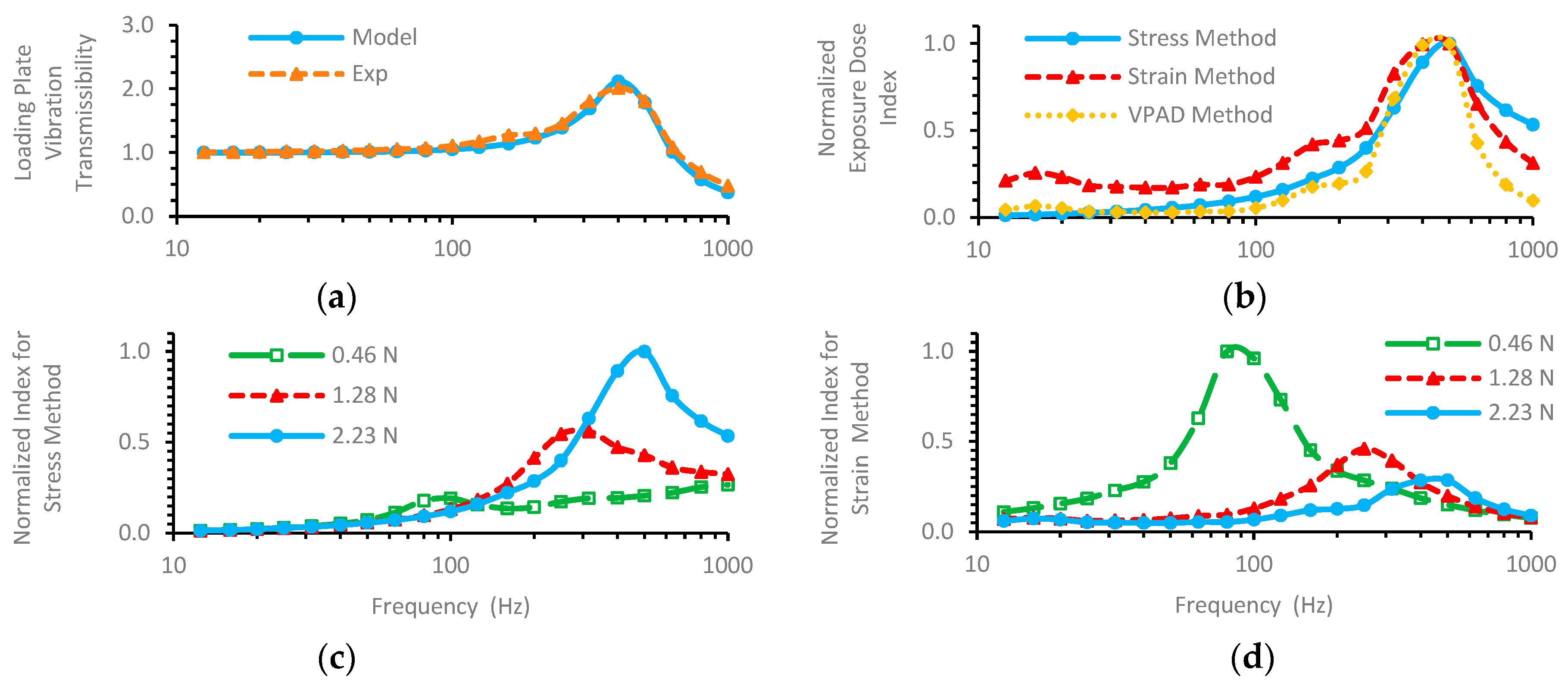
The vibration response of the new rat-tail model worked as expected, as demonstrated by the excellent agreement between the modeling and experimental results (
Figure 3). As the tail mass of the loaded portion (about 0.21 g) is much less than that of the loading plate (>20 g), the dynamic force acting on this portion of the tail during a vibration exposure results primarily from the plate acceleration (≈
MPAP). In this way, the biodynamic responses, such as vibration stress and strain of the tail tissues, can be effectively controlled and quantified by measuring and controlling the loading plate acceleration (
AP). This makes it convenient and reliable to quantify the tail biodynamic responses required to investigate their relationships with biological effects.
The new methodology opens a window to view the mechanisms of the vibration health effects and their influencing factors. For example, the stress- and strain-dose index formulas (Equations (4) and (8)) suggest that the frequency may affect the vibration injuries or biological effects in two different ways: (I) the frequency determines the stress and strain magnitudes; they reach their peak values at the fundamental resonant frequency under each static force, as shown in
Figure 3c,d; and (II) the frequency determines the number of cycles per second. These two different roles may result in different biological effects and/or their different frequency dependencies.
Another unique feature of the new rat-tail model is that the static force applied on the tail can be reliably controlled, which makes it possible to investigate the influences of the combined force and vibration exposures on the biological effects. The results presented in
Figure 3 suggest that the static force affected the vibration stress- and strain-exposure doses differently. This is because the rat tail has a nonlinear mechanical behavior. At a low static force, the tissue stiffness is less than that at a higher static force; as a result, the same dynamic force results in a larger strain at the low static force than that at the higher static force. On the other hand, the vibration stress is independent of the deformation, but it depends primarily on the dynamic force itself (Equation (4)). This finding may help identify and understand the static-force influences on the vibration health effects and their frequency dependencies.
Overall, the new rat-tail model provides a new and effective tool for studying human finger vibration health effects.