Design of a Quasi-Direct-Drive Actuator for Dynamic Motions †
Abstract
:1. Introduction
2. Actuator Design
| Actuator | Dimensions (mm) | Peak Torque (Nm) | Nominal Torque (Nm) | Maximum Speed (rad/s) | Peak Torque Density × Maximum Speed (W/kg) | Weight (Kg) |
|---|---|---|---|---|---|---|
| MIT Cheetah 1 | N/A | 58 | N/A | 6.9 * | 219.29 | 1.825 |
| Mini Cheetah | 96×40 | 17 | 6.9 | 40 | 1360 | 0.5 |
| BIRO | 110×51 | 36 | 17.1 | 19 | 876.9 | 0.78 |
| Stanford Doggo | N/A | 4.8 | 1.51 | 251 | 3675 | 0.28 |
| Proposed actuator | 126×68 | 55.2 | 26.4 | 37.2 | 1086.47 | 1.89 |
3. Experimental Results
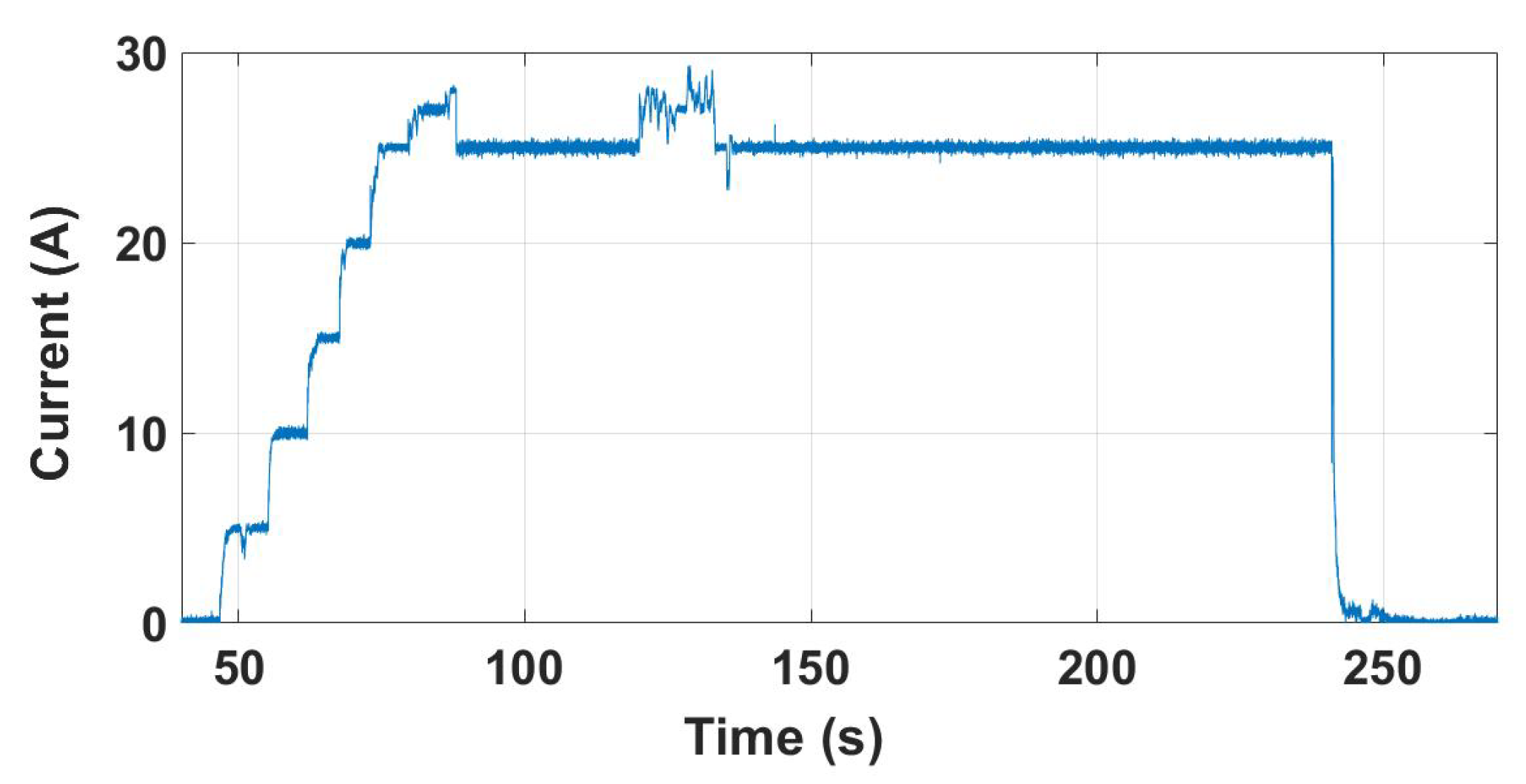
- Nominal Torque Evaluation: The actuator output shaft was attached to a lever with a set of loads weighing about 14 Kg hanging at a 20 cm distance with respect to the joint axis, replicating an external torque of approximately 28 Nm, which is slightly higher than the continuous torque of the actuator. A set of velocity references was commanded until the lever reached the horizontal position, and it was held in that condition. The time history of the current associated with this test is shown in Figure 2.
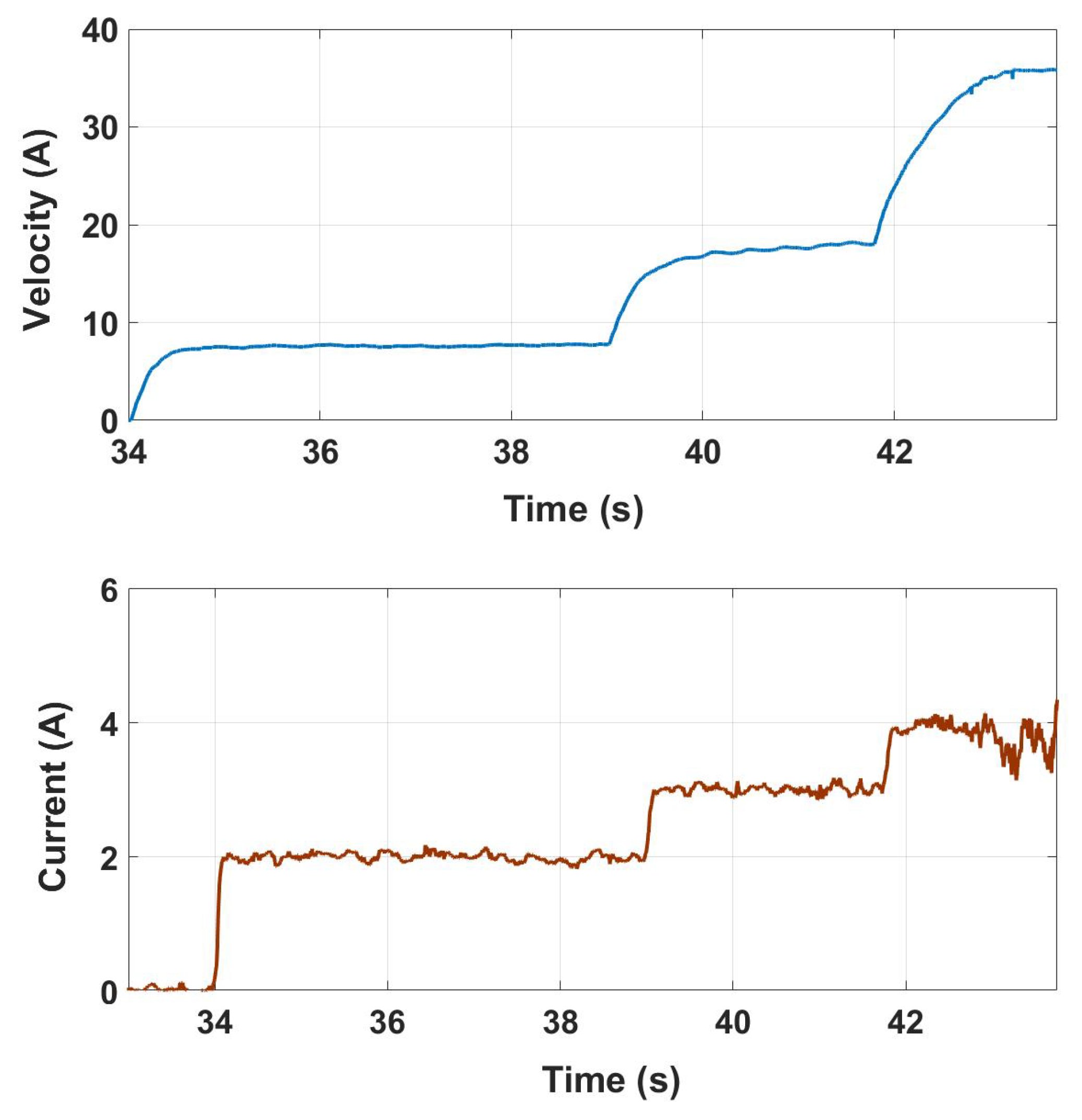
- Maximum Speed Evaluation: The actuator output was free, and a few direct current commands were set to render the maximum velocity with the actuator, which was about 37 rad/s when taking about 4 A to overcome the frictional and other losses. The corresponding time history of the current and velocity is illustrated in Figure 3.
4. Conclusions
References
- Kashiri, N.; Abate, A.; Abram, S.J.; Albu-Schaffer, A.; Clary, P.J.; Daley, M.; Faraji, S.; Furnemont, R.; Garabini, M.; Geyer, H.; et al. An overview on principles for energy efficient robot locomotion. Front. Robot. AI 2018, 5, 129. [Google Scholar] [CrossRef] [PubMed]
- Pratt, G.A.; Williamson, M.M. Series elastic actuators. In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems, Pittsburgh, PA, USA, 5–9 August 1995; Volume 1, pp. 399–406. [Google Scholar]
- Tsagarakis, N.G.; Laffranchi, M.; Vanderborght, B.; Caldwell, D.G. A compact soft actuator unit for small scale human friendly robots. In Proceedings of the IEEE international Conference on Robotics and Automation, Kobe, Japan, 12–17 May 2009; pp. 4356–4362. [Google Scholar]
- Hutter, M.; Remy, C.D.; Hoepflinger, M.A.; Siegwart, R. Scarleth: Design and control of a planar running robot. In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems, San Francisco, CA, USA, 25–30 September 2011; pp. 562–567. [Google Scholar]
- Grioli, G.; Wolf, S.; Garabini, M.; Catalano, M.; Burdet, E.; Caldwell, D.; Carloni, R.; Friedl, W.; Grebenstein, M.; Laffranchi, M.; et al. Variable stiffness actuators: The user’s point of view. Int. J. Robot. Res. 2015, 34, 727–743. [Google Scholar] [CrossRef]
- Laffranchi, M.; Chen, L.; Kashiri, N.; Lee, J.; Tsagarakis, N.G.; Caldwell, D.G. Development and control of a series elastic actuator equipped with a semi active friction damper for human friendly robots. Robot. Auton. Syst. 2014, 62, 1827–1836. [Google Scholar] [CrossRef]
- Kashiri, N.; Caldwell, D.G.; Tsagarakis, N. A self-adaptive variable impedance actuator based on intrinsic non-linear compliance and damping principles. In Proceedings of the 2017 IEEE International Conference on Robotics and Automation (ICRA), Singapore, 29 May–3 June 2017; pp. 1248–1254. [Google Scholar]
- Asada, H.; Youcef-Toumi, K. Direct-Drive Robots: Theory and Practice; MIT Press: Cambridge, MA, USA, 1987. [Google Scholar]
- Carignan, C.R.; Cleary, K.R. Closed-Loop Force Control for Haptic Simulation of Virtual Environments. 2000, pp. 1–14. Available online: https://www.researchgate.net/publication/243766717_Closed-loop_force_control_for_haptic_simulation_of_virtual_environments.
- Kashiri, N.; Malzahn, J.; Tsagarakis, N.G. On the sensor design of torque controlled actuators: A comparison study of strain gauge and encoder-based principles. IEEE Robot. Autom. Lett. 2017, 2, 1186–1194. [Google Scholar] [CrossRef]
- Kashiri, N.; Baccelliere, L.; Muratore, L.; Laurenzi, A.; Ren, Z.; Hoffman, E.M.; Kamedula, M.; Rigano, G.F.; Malzahn, J.; Cordasco, S.; et al. CENTAURO: A hybrid locomotion and high power resilient manipulation platform. IEEE Robot. Autom. Lett. 2019, 4, 1595–1602. [Google Scholar] [CrossRef]
- Seok, S.; Wang, A.; Chuah, M.Y.; Hyun, D.J.; Lee, J.; Otten, D.M.; Lang, J.H.; Kim, S. Design principles for energy-efficient legged locomotion and implementation on the MIT cheetah robot. IEEE/ASME Trans. Mechatronics 2014, 20, 1117–1129. [Google Scholar] [CrossRef]
- Wensing, P.M.; Wang, A.; Seok, S.; Otten, D.; Lang, J.; Kim, S. Proprioceptive actuator design in the MIT Cheetah: Impact mitigation and high-bandwidth physical interaction for dynamic legged robots. IEEE Trans. Robot. 2017, 33, 509–522. [Google Scholar] [CrossRef]
- Ding, Y.; Park, H.W. Design and experimental implementation of a quasi-direct-drive leg for optimized jumping. In Proceedings of the 2017 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Vancouver, BC, Canada, 24–28 September 2017; pp. 300–305. [Google Scholar]
- Katz, B.; Di Carlo, J.; Kim, S. Mini cheetah: A platform for pushing the limits of dynamic quadruped control. In Proceedings of the IEEE International Conference on Robotics and Automation, Montreal, QC, Canada, 20–24 May 2019; pp. 6295–6301. [Google Scholar]
- Yu, S.; Huang, T.H.; Yang, X.; Jiao, C.; Yang, J.; Chen, Y.; Yi, J.; Su, H. Quasi-Direct Drive Actuation for a Lightweight Hip Exoskeleton with High Backdrivability and High Bandwidth. IEEE/ASME Trans. Mechatronics 2020, 25, 1794–1802. [Google Scholar] [CrossRef] [PubMed]
- Kau, N.; Schultz, A.; Ferrante, N.; Slade, P. Stanford doggo: An open-source, quasi-direct-drive quadruped. In Proceedings of the IEEE International Conference on Robotics and Automation, Montreal, QC, Canada, 20–24 May 2019; pp. 6309–6315. [Google Scholar]
- Association, A.G.M.; Institute, A.N.S. Fundamental Rating Factors and Calculation Methods for Involute Spur and Helical Gear Teeth; AGMA Standard; American Gear Manufacturers Association: Alexandria, VA, USA, 2004. [Google Scholar]
- Roozing, W.; Kashiri, N.; Tsagarakis, N.G. Enhanced explosive motion for torque controlled actuators through field weakening control. In Proceedings of the 2018 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Madrid, Spain, 1–5 October 2018; pp. 1–8. [Google Scholar]
- Mohammadnia, M.; Kashiri, N.; Braghin, F.; Tsagarakis, N.G. Flux Regulation for Torque-controlled Robotics Actuators. In Proceedings of the 2019 19th International Conference on Advanced Robotics (ICAR), Belo Horizonte, Brazil, 2–6 December 2019; pp. 93–98. [Google Scholar]
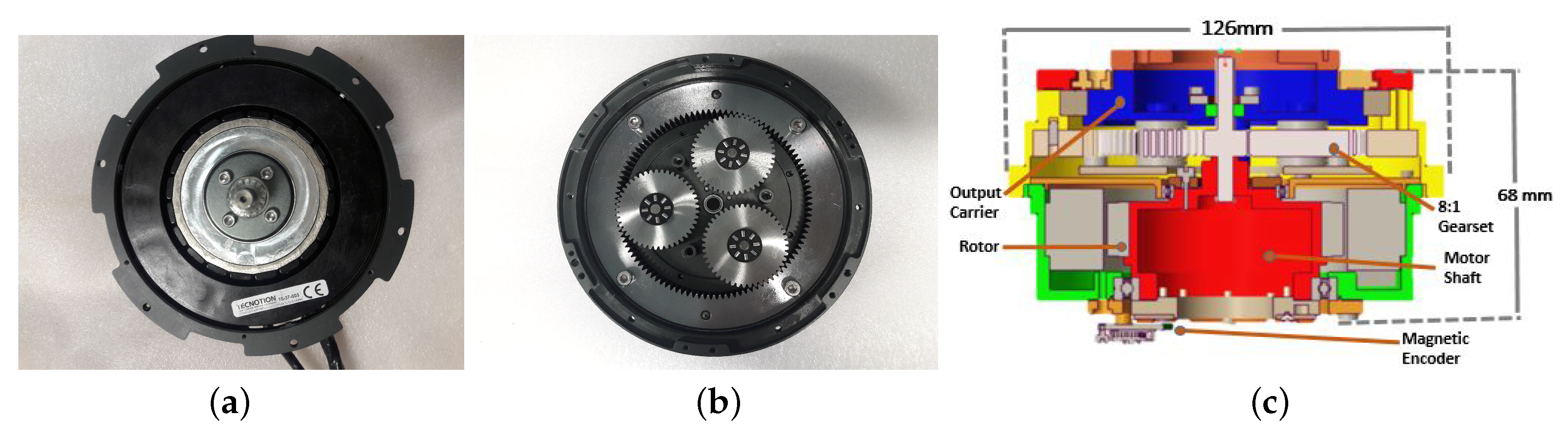
| Gears | Number of Teeth | Pitch Diameter | Module | Load Dist. Factor | Dynamic Factor | Geometry Factor | Max Admissible Torque per Tooth | Max Torque Applied per Tooth |
|---|---|---|---|---|---|---|---|---|
| Pinion | 15 | 12 | 0.8 | 1.3 | 0.75 | 0.29 | 2.34 | 2.3 |
| Planets | 45 | 36 | 0.8 | 1.3 | 0.74 | 0.35 | 8.49 | 6.9 |
| Ring | 105 | 84 | 0.8 | 1.3 | 0.8 | 0.42 | 17.36 | 16.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Singh, A.; Kashiri, N.; Tsagarakis, N. Design of a Quasi-Direct-Drive Actuator for Dynamic Motions. Proceedings 2020, 64, 11. https://doi.org/10.3390/IeCAT2020-08516
Singh A, Kashiri N, Tsagarakis N. Design of a Quasi-Direct-Drive Actuator for Dynamic Motions. Proceedings. 2020; 64(1):11. https://doi.org/10.3390/IeCAT2020-08516
Chicago/Turabian StyleSingh, Akash, Navvab Kashiri, and Nikolaos Tsagarakis. 2020. "Design of a Quasi-Direct-Drive Actuator for Dynamic Motions" Proceedings 64, no. 1: 11. https://doi.org/10.3390/IeCAT2020-08516
APA StyleSingh, A., Kashiri, N., & Tsagarakis, N. (2020). Design of a Quasi-Direct-Drive Actuator for Dynamic Motions. Proceedings, 64(1), 11. https://doi.org/10.3390/IeCAT2020-08516




