New Insights from Econometric Data: An Extended Exergy Analysis (EEA) of the Italian System, 2013–2017 †
Abstract
:1. Introduction
1.1. Exergy and Energy
- Two systems in different thermodynamic states, when physically communicating with each other, provide a potential for producing work.
- “Work” and “Heat” flows represent ways by which two systems attain mutual thermodynamic equilibrium by equalizing their respective thermodynamic state parameters.
- All real processes are irreversible: it is impossible to transform work into (a different type of) work, work into heat, heat into work and heat into heat (at different temperature) with no losses.
- Given that “work” and “heat” are two different forms of energy, the former can ideally be converted entirely into the latter (1 kWhwork −> 1 kWhheat); but the reverse is not true: any heat-to-work transformation requires that a portion of the high-T heat in input be dispersed into the environment as low-T waste heat: 1 kWhheat ≠ 1 kWhwork.
Exergy expresses the amount of work necessary to produce a material in a specified state from components common in the natural environment, in a reversible way, heat being exchanged only with the environment.
The environment can be viewed as a system sufficiently large as to be unaffected by the thermodynamic transformations occurring inside of it, that is its thermodynamic properties (temperature, pressure etc.) remain constant throughout the process.
1.2. Exergy-Based Analysis: Thermoeconomics, Cumulative Exergy Content, Extended Exergy Analysis
- ➢
- Domestic (DO) power-consuming activities for survival and growth of human population.
- ➢
- Extractive (EX) involves the processes of mining and quarrying.
- ➢
- Conversion (CO) includes energy conversion, heat and power plants, oil refineries, other refinery and base chemistry industries.
- ➢
- Industrial (IN) includes all of the manufacturing activities which generate added value to raw materials.
- ➢
- Transportation (TR) covers transportation services, commercial and private.
- ➢
- Tertiary (TE) includes commercial, financial and all the service sector (government, schools, police, etc.).
- ➢
- Agricultural (AG). Harvesting, forestry, fishing.
2. Method
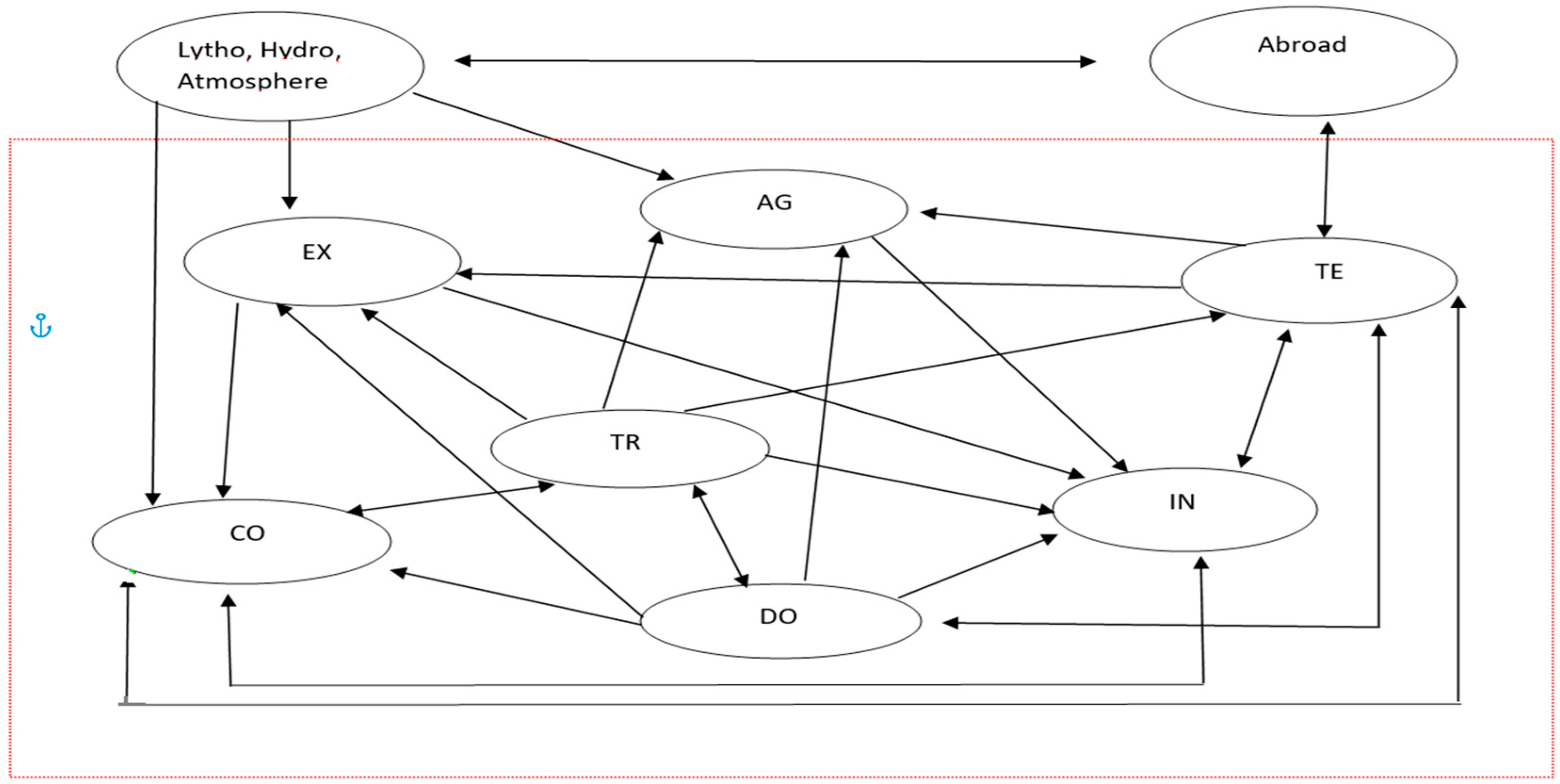
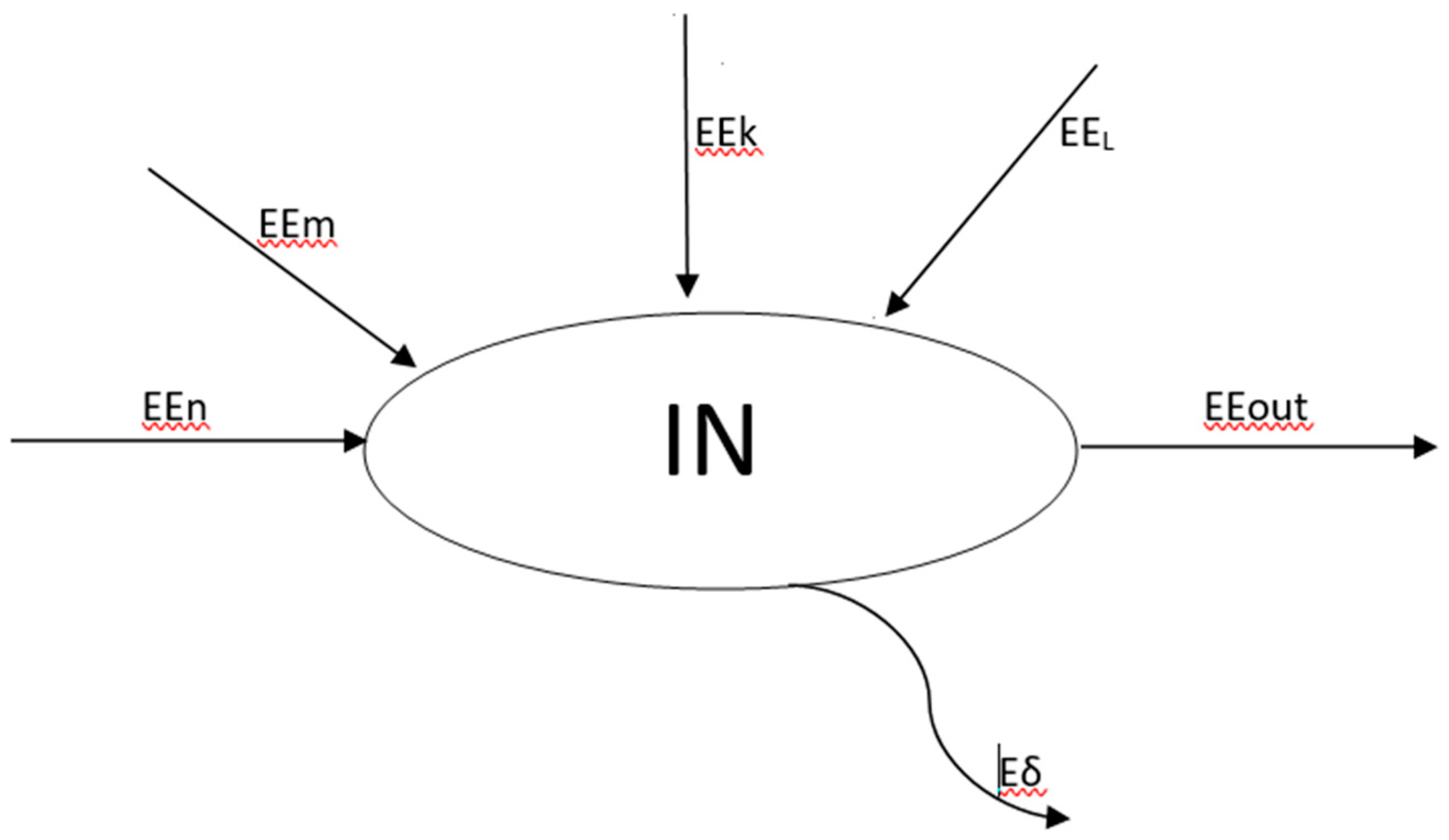
2.1. Sector Classification and EE Fluxes
- ➢
- EX extracts from the environment energy carriers and ores as raw materials, thanks to the energy and services supplied by TE, transports provided by TR, financial investments from TE and workers from DO. Its outputs are conveyed to CO for processing.
- ➢
- CO converts the energy carriers from EX, with their CExC and exergy of externalities, into heat and electrical energy with the generation of by-products as coke and refinery output thanks to contribution from DO, TR and TE. Renewable energy primary inputs (solar, wind, geothermal energy, hydropower) are “extracted” from the environment. The products are sent to TR, TE, IN.
- ➢
- IN generates consumer goods with added value. The products are dispatched to TE to be sold. Its inputs are EE fluxes from DO (workers), TR, AG, energy from CO (distributed by TE), raw materials from EX.
- ➢
- AG receives exergy from DO, TE, TR and the Environment, generating semi-finished products to be sent to IN and partly to DO.
- ➢
- DO supplies labor force to all of the sectors, receiving goods and services from TE, TR and, partially, from AG.
- ➢
- TR receives refinery products from CO, labor from DO and supplies all of the sectors.
- ➢
- TE provides goods and services to all of the sectors: receives the EE of CO and IN commodities and sells them to DO and all of the other sectors (for example, electricity generated in CO is sold by utilities to all of the sectors, charged with their content of EE due to the “production” of such an energy service). The exchanges with the other countries (“abroad”) represent import/export fluxes and are totally mediated in their entirety by TE.
2.2. Collecting Data
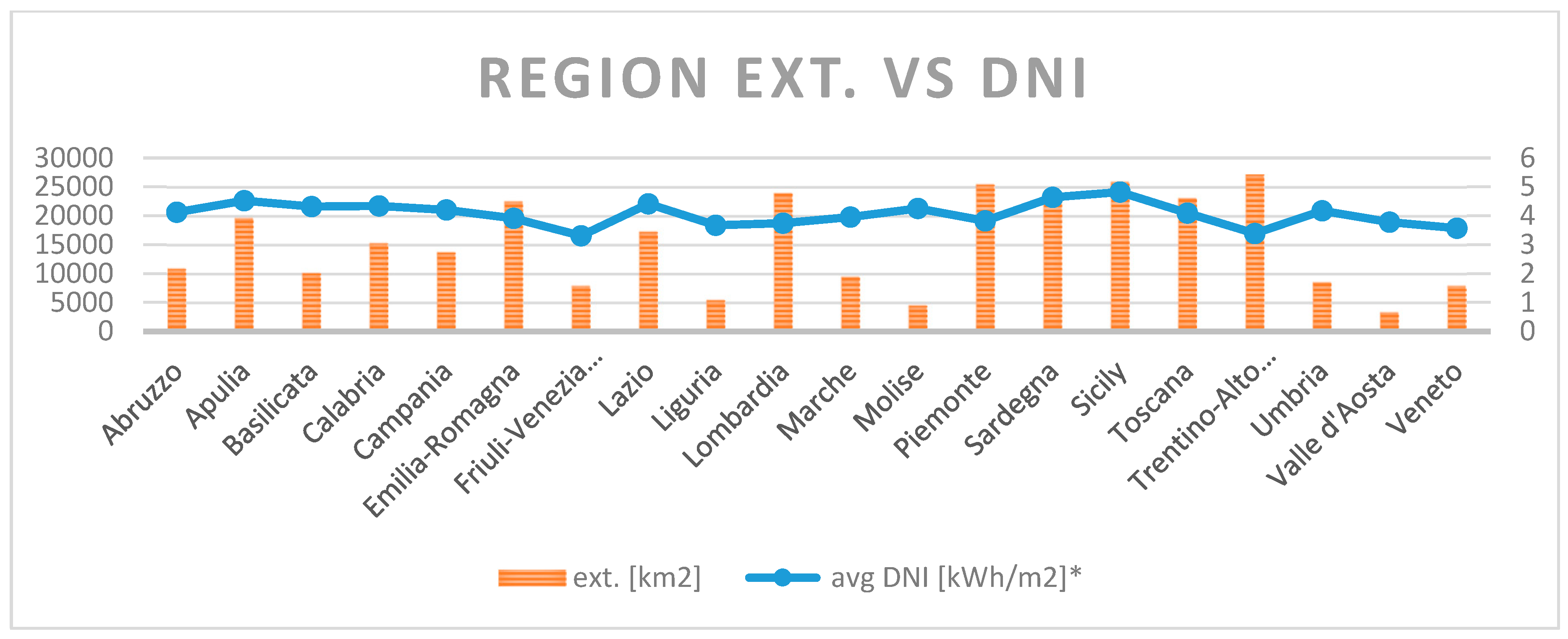
2.2.1. Solar Exergy
2.2.2. Hydraulic Exergy Potential
- ➢
- ➢
- ➢
- A mean temperature of the Italian sea was calculated as the average of the temperature of the seas that bathe the Italian coasts.
- ➢
- The equation developed by Valero et al. [34] was then used to compute the hydraulic specific exergy of each river (neglecting the chemical exergy terms):
2.2.3. Geothermal Exergy
2.2.4. Other Material and Energy Flows
2.3. Computation of the Econometric Factors and Specific Exergy of Labor and Capital
- ➢
- The α factor represents the portion of the input exergy necessary to the survival of the population. It is thus computed as the ratio between the exergy input of the domestic sector and the country total exergy input. Once is known, the specific Extended Exergy of labor is computed as where Nwh is the number of work hours per year.
- ➢
- The second econometric factor, β is computed on the basis of economic data: the economic “Money and Quasi-Money” aggregate M2 and the average salary; β is the ratio between M2 and the total average salary of a given year. In this definition, β is merely a sort of amplification factor that produces wealth only from financial activities: the higher, the more the society is service-based. EEA introduces a correction to this definition, to take into account the so-called financial capital (the amount in excess of the global salaries in the country): the Extended Exergy embodied in one monetary unit is for a given year and is computed as .
3. Results and Discussion
- (a)
- The first econometric coefficient is fairly constant over the time window of observation: it is equal to 4 × 10−4 and indicates that in spite of its high living standards, Italy is an “exergy sparing” country (values of α for different countries for year 2005 as reported in [31]);
- (b)
- The second econometric coefficient also is fairly constant between 2013 and 2017: its values oscillate around 5.2. This indicates that Italy is a country dominated by financial capital (Kf/S = β − 1);
- (c)
- The Extended Exergy of labor is a measure of how many Joules is 1 work hour equivalent to: a higher eeL pertains to more energy-intensive societies. The value for Italy did not change much from 2013 to 2017, being around 70 MJ/hour; the Extended Exergy of capital is a measure of how many monetary units it takes to make up for one work hour. A higher eeK pertains to more affluent societies. The value for Italy did not change much from 2013 to 2017, being around 65 MJ/€;
- (d)
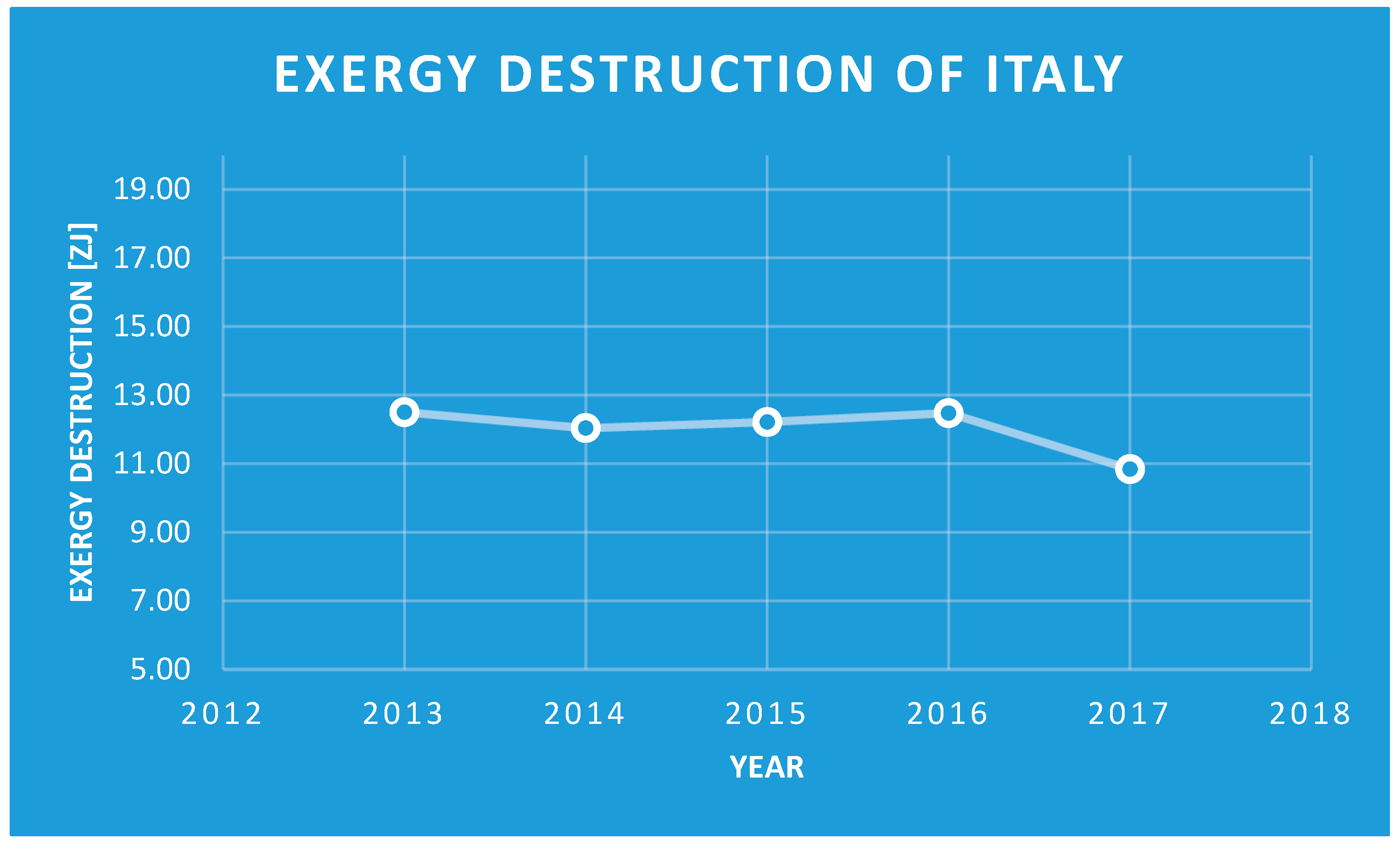
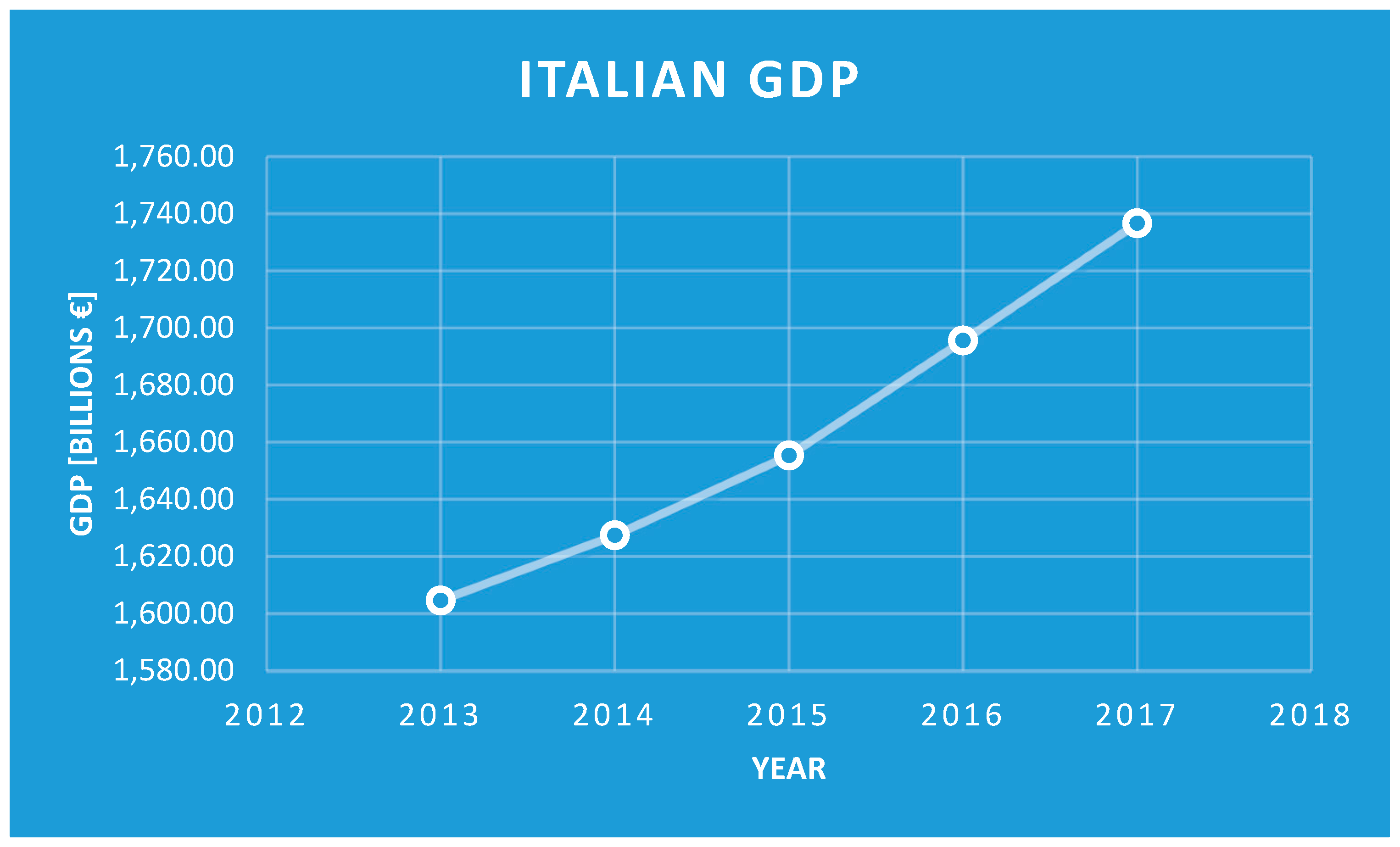
- The Eδ does not correlate with the GDP (Figure 10): the GDP curve is convex and growing, while the Eδ curve is fairly constant around 12 ZJ/year except for 2017 in which there was less destruction in respect to the other years it was compared with. This was an unexpected result worthy of further investigation.
- (e)
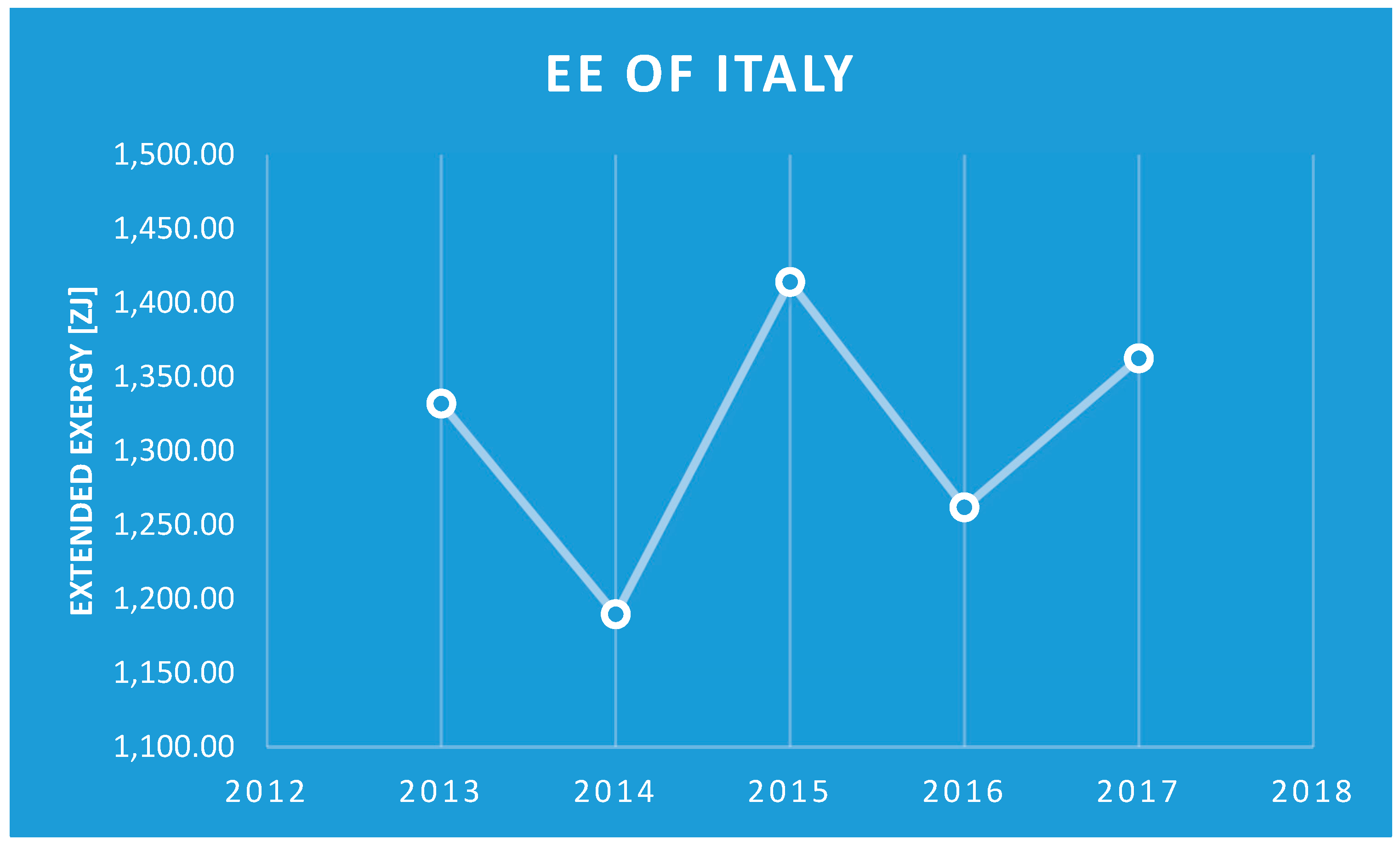
- The Extended Exergy, that is the “cost” of Italian society, is fairly constant, around a value of 1300 ZJ; it is instructive to compare this trend with that of the GDP. Historically, we associate development and wellness with a growth of GDP, so considering Figure 11, one could be led to consider that the Italian society is growing in the “right way”; the problem is that, according to the analysis, the Extended Exergy of the country reached a plateau (Figure 12); this means that the “cost” of the economic and social growth was the same within the years, witnessing the absence of enhanced rational exploitation of the available resources. As a matter of fact, this result suggests that the sustainability of the Italian society did not improve throughout the window of observation.
4. Conclusions
Nomenclature
| CExC | Cumulative Exergy Content |
| Cp | Specific heat, constant pressure |
| DNI | Direct Normal Irradiance |
| Exergy rate | |
| EEA | Extended Exergy Analysis |
| EEenv | Extended Exergy of environment remediation |
| . | Extended Exergy of Labor |
| Extended Exergy of Capital | |
| δ | Exergy destruction rate |
| fc | Carnot factor |
| h | Specific enthalpy |
| Ib | Solar constant |
| M2 | Money + quasi-money circulation |
| PV | Photovoltaics |
| S | Specific entropy |
| T | Temperature |
| TE | Thermo-Economics |
| Greek symbols | |
| α | First econometric factor |
| β | Second econometric factor |
| ηII | Exergy efficiency |
References
- The World Commission on Environment and Development (Brundtland Commission). Our Common Future; Oxford University Press: Oxford, UK, 1987. [Google Scholar]
- Sciubba, E. Our Exergy Footprint: A Thermodynamic Indicator for the Assessment of the Degree of Unsustainability of Energy Systems; Perspectives for Joint Research Projects, Russian Center of Science and Culture; Seminar on Autonomous Energy and Energy Efficiency: Rome, Italy, 2019. [Google Scholar]
- Empresa de Pesquisa Energetica, Ministero de Minas e Energia. Brazilian Energy Balance. 2017. Available online: https://epe.gov.br (accessed on 6 March 2020).
- Eurostat. Energy Balances. Available online: https://ec.europa.eu (accessed on 6 March 2020).
- ISTAT. Energy Balance of Italy. Available online: https://dati.istat.it (accessed on 6 March 2020).
- Rant, Z. Energy Value and Pricing. Strojniski vestnik. J. Mech. Eng. 1995, 1, 4–7. [Google Scholar]
- Rocco, M.V.; Colombo, E.; Sciubba, E. Advances in Exergy Analysis. A Novel Assessment of the Extended Exergy Accounting method. Appl. Energy 2014, 113, 1405–1420. [Google Scholar] [CrossRef]
- Wall, G. Exergy conversion in the Swedish society. Resour. Energy 1987, 9, 55–73. [Google Scholar] [CrossRef]
- Wall, G. Exergy conversion in the Japanese society. Energy 1990, 15, 435–444. [Google Scholar] [CrossRef]
- Hammond, G.P.; Stapleton, A.J. Exergy analysis of the UnitedKingdom energy system. Proc. Inst. Mech. Eng. Part A 2001, 215, 141–162. [Google Scholar] [CrossRef]
- Ertesvåg, I.S. Society exergy analysis: A comparison of different Societies. Energy 2001, 26, 253–270. [Google Scholar] [CrossRef]
- Brockway, P.E.; Barrett, J.R.; Foxon, J.T.; Steinberger, J.K. Divergence of Trend in US and UK aggregate Exergy Efficiencies 1960–2010. Environ. Sci. Technol. 2014, 48, 9874–9881. [Google Scholar] [CrossRef]
- Chen, B.; Chen, J.Q. Exergy Analysis for Resource Conversion of the Chinese Society 1993 Under the Material product System. Energy 2006, 31, 1115–1150. [Google Scholar] [CrossRef]
- Koroneos, C.J.; Nanaki, E.A. Energy and Exergy utilization Assessment of the Greek Transoprt Sector. Resour. Conserv. Recycl. 2008, 52, 700–706. [Google Scholar] [CrossRef]
- Szargut, J.; Ziębik, A.; Stanek, W. Depletion of the Unrestorable Natural Exergy Resources as a Measure of the Ecological Cost. Energy Convers. Manag. 2002, 42, 9–12, 1149–1163. [Google Scholar]
- Sciubba, E. A novel exergetic costing method for determining the optimal allocation of scarce resources. In Proc. Contemporary Problems of Thermal Engineering; Ziebek, A., Ed.; Gliwice, Poland, 1998; pp. 311–324. [Google Scholar]
- Dai, J.; Chen, B.; Sciubba, E. Extended Exergy Based Economic Accounting for the Transportation Sector in China. Renew. Sustain. Energy Rev. 2014, 32, 229–237. [Google Scholar] [CrossRef]
- Sciubba, E.; Bastianoni, S.; Tiezzi, E. Exergy and Extended Exergy accounting of very large complex systems with an application to the province of Siena, Italy. J. Environ. Manag. 2008, 86, 372–382. [Google Scholar] [CrossRef] [PubMed]
- Ertesvåg, I.S. Energy, exergy, and extended-exergy analysis of the Norwegian society 2000. Energy 2005, 30, 649–675. [Google Scholar] [CrossRef]
- Ptasinski, K.J.; Koymans, M.N.; Verspagen, H.H.G. Performance of the Dutch energy sector based on energy, exergy and Extended Exergy accounting. Energy 2006, 31, 3135–3144. [Google Scholar] [CrossRef]
- Feynman, R. The Feynman Lecture on Physics Vol. 1, Chp. 4. Available online: http://feynmanlectures.caltech.edu/I_04.html (accessed on 3 May 2020).
- Petela, R. Engineering Thermodynamics of Thermal Radiation; McGrawHill: New York, NY, USA, 2010. [Google Scholar]
- Moran, M.J.; Shapiro, H.N.; Boettner, D.D.; Bailey, M.B. Fundamentals of Engineering Thermodynamics, 8th ed.; Wiley: Hoboken, NJ, USA, 2014. [Google Scholar]
- Sciubba, E.; Wall, G. A brief commented history of exergy from the beginnings to 2004. Int. J. Thermodyn. 2007, 10, 1–26. [Google Scholar]
- Szargut, J. Exergy Method-Technical and Ecological Applications; WIT Press: Southampton, UK, 2005. [Google Scholar]
- Sayed, Y.M.E. The Thermo-Economics of Energy Conversion; Elsevier: Amsterdam, The Netherlands, 2003. [Google Scholar]
- Gaggioli, R.A.; Rodriguez, L. Second Law Efficiency of a Coal Gasification Process. Can. J. Chem. Eng. 1980, 58, 376–381. [Google Scholar]
- Szargut, J.; Morris, D.R.; Steward, F.R. Exergy Analysis of Thermal, Chemical, and Metallurgical Processes; Hemispere Pub: New York; NY, USA, 1988. [Google Scholar]
- Valero, A.; Usón, S.; Torres, C.; Valero, A. Application of Thermoeconomics to Industrial Ecology. Entropy 2010, 12, 591A–612A. [Google Scholar] [CrossRef]
- Sciubba, E. Beyond Thermoeconomics? The concept of Extended Exergy Analysis and Its Application to the Analysis and Design of Thermal Systems. Exergy Int. J. 2001, 1, 68–84. [Google Scholar] [CrossRef]
- Sciubba, E. A revised calculation of the econometric factors α- and β for the Extended Exergy Accounting method. Ecol. Mod. 2011, 222, 1060–1066. [Google Scholar] [CrossRef]
- Available online: http://globalsolaratlas.info (accessed on 1 February 2020).
- ISTAT. La Superficie dei Comuni, delle Province e delle Regioni Italiane; ISTAT: Chicago, IL, USA, 2013. [Google Scholar]
- Valero, A.; Al Valero, U.J.; Martínez, A. Physical Hydronomics: Application of the exergy analysis to the assessment of environmental costs of water bodies. The case of the inland basins of Catalonia. Energy 2009, 34, 2101–2107. [Google Scholar] [CrossRef]
- Available online:. Available online: http://bacino-adige.it (accessed on 3 March 2020).
- Available online:. Available online: http://arpat.toscana.it (accessed on 3 March 2020).
- Available online:. Available online: http://arpa.veneto.it (accessed on 3 March 2020).
- Available online:. Available online: http://autorita.bacinoserchio.it/ (accessed on 3 March 2020).
- Available online:. Available online: http://idrografico.regione.lazio.it (accessed on 3 March 2020).
- Available online:. Available online: http://webbook.nist.gov (accessed on 5 March 2020).
- Davies, J.H. Global map of solid Earth surface heat flow. Geochem. Geophys. Geosyst. 2013, 14, 4608–4622. [Google Scholar] [CrossRef]
- Available online:. Available online: http://dati.istat.it (accessed on 5 March 2020).
- TERNA. Produzione e Richiesta di Energia Elettrica in Italia dal 1883 al 2018; TERNA: Rome, Italy, 2019. [Google Scholar]
- TERNA. Consumi Energia Elettrica in Italia; TERNA: Rome, Italy, 2019. [Google Scholar]
- GSE. Energia nel Settore Trasporti 2005–2018; GSE: Rome, Italy, 2019. [Google Scholar]
- GSE. Rapporto Statistico FER 2017; GSE: Rome, Italy, 2018. [Google Scholar]
- ISTAT. 6° censimento generale dell’agricoltura-utilizzo della risorsa idrica a fini irrigui in agricoltura; ISTAT: Chicago, IL, USA, 2014. [Google Scholar]
- ISTAT. Utilizzo e Qualità della Risorsa Idrica in Italia; ISTAT: Chicago, IL, USA, 2019. [Google Scholar]
- Nolè, A.; Collalti, A.; Borghetti, M.; Chiesi, M.; Chirici, G.; Magnani, F.; Marras, S.; Maselli, F.; Sirca, C.; Spano, D.; et al. The Role of Managed Forest Ecosystems: A Modelling Based Approach, Environmental Science and Engineering; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar] [CrossRef]
- Available online:. Available online: http://isprambiente.gov.it (accessed on 5 March 2020).
- ISPRA Il piombo nelle munizioni da caccia: Problematiche e possibili soluzioni; ISPRA: Rome, Italy, 2012.
- Sharqawy, M.H.; Zubair, S.M. On Exergy Calculations of Seawater with Applications in Desalination Systems; Int. J. Therm. Sci. 2012, 50, 187–196. [Google Scholar] [CrossRef]

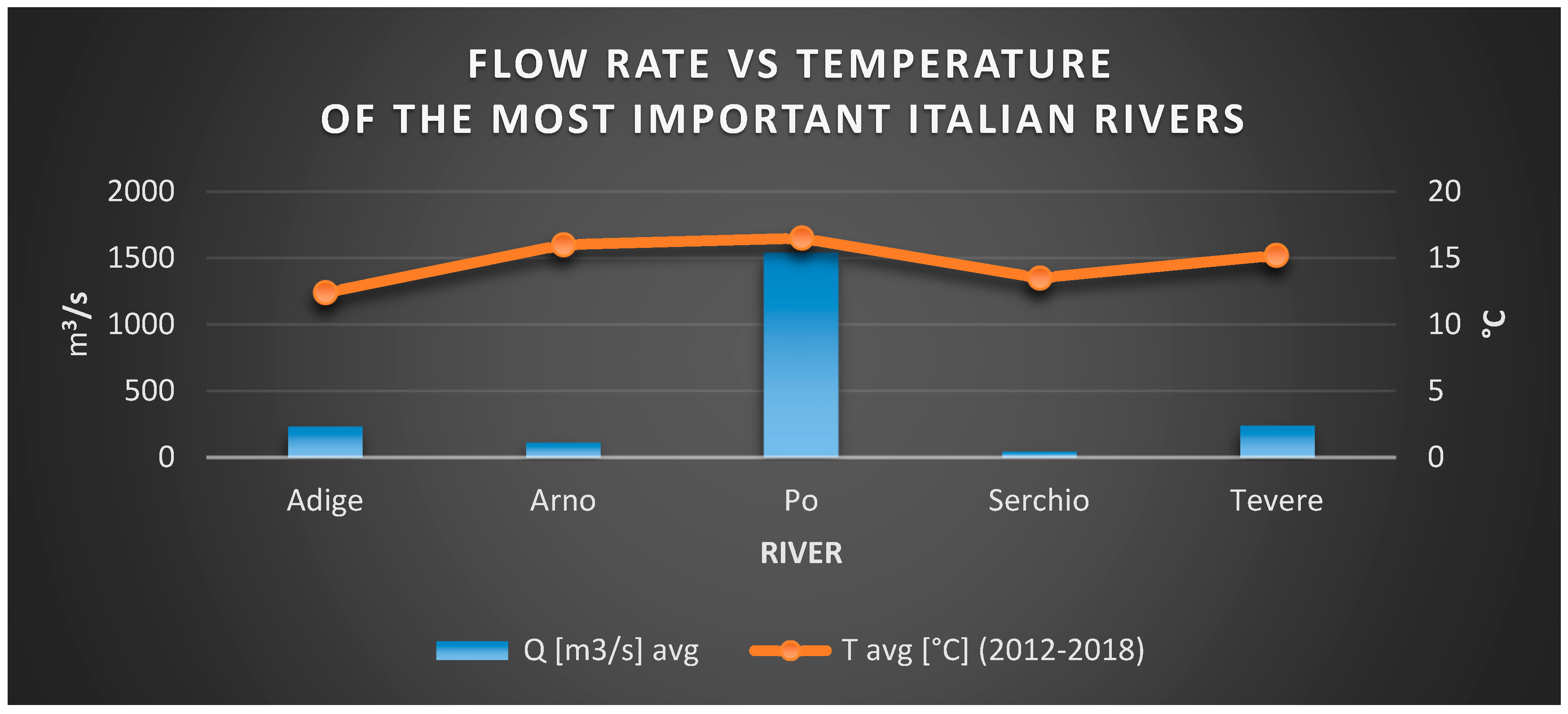
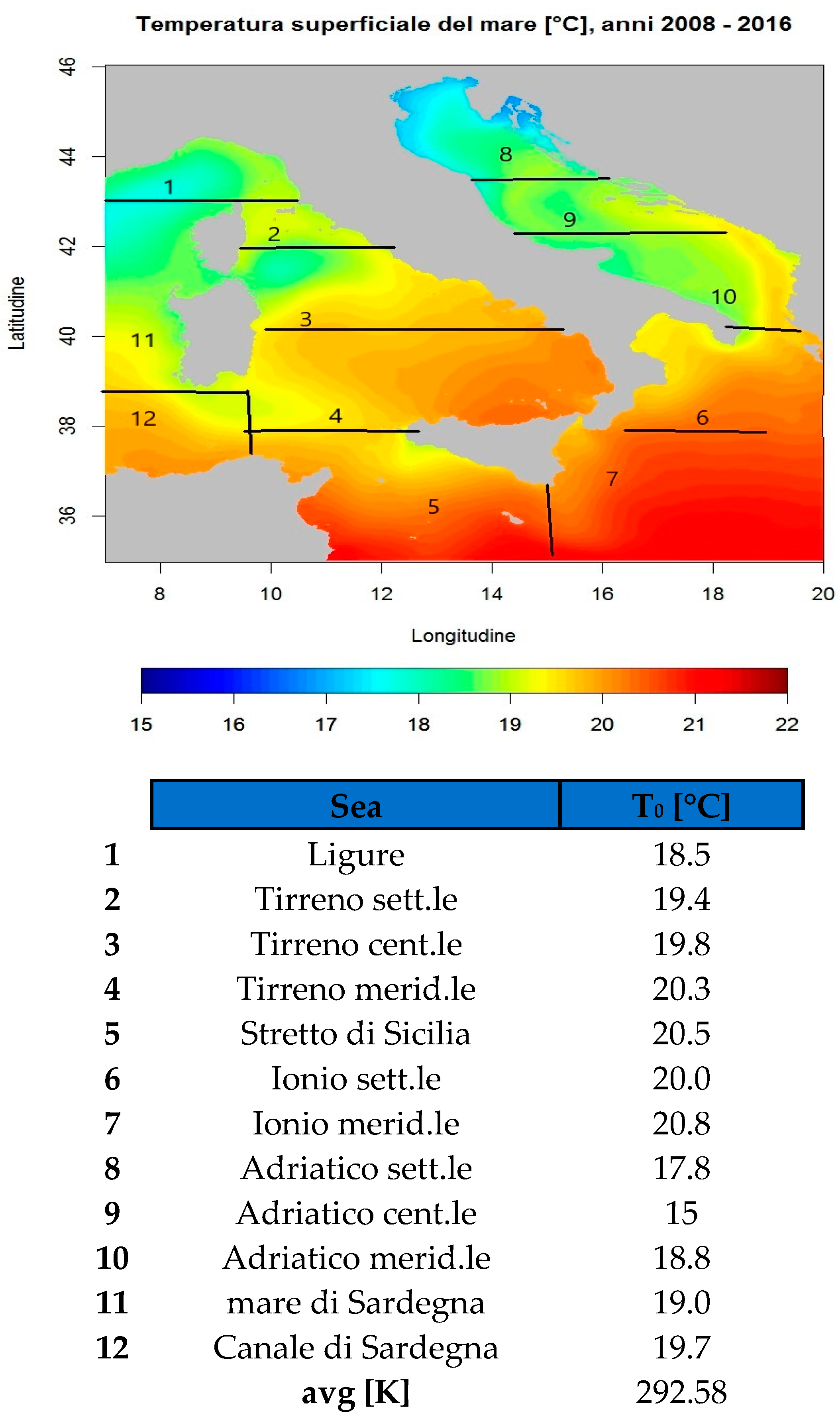
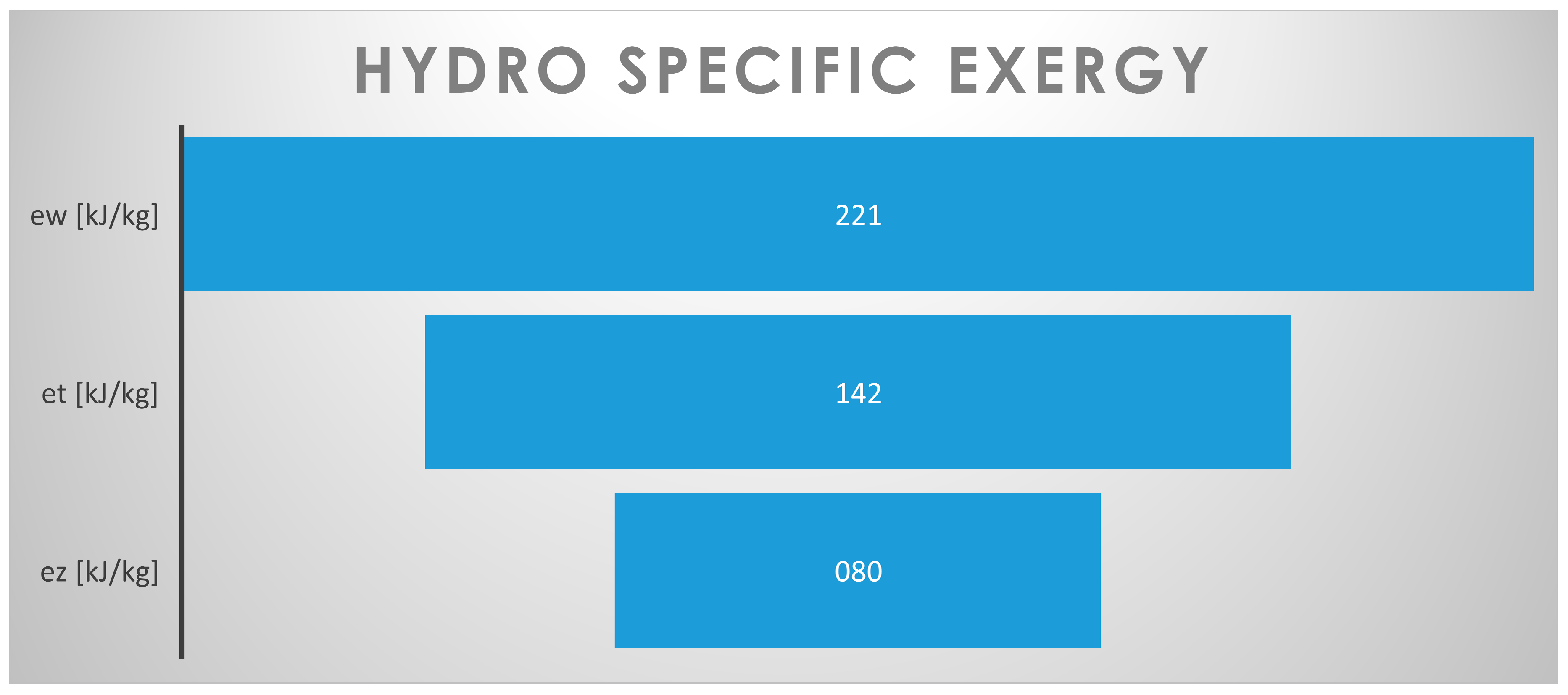

| River | Q avg [m3/s] | T avg [°C](2012–2018) | et [kJ/kg] | ez [kJ/kg] | ew [kJ/kg] | Ew [GW] |
|---|---|---|---|---|---|---|
| Tevere | 240 | 15.20 | 25.40 | 13.80 | 39.20 | 9.40 |
| Serchio | 46 | 13.50 | 35.69 | 14.72 | 50.41 | 2.32 |
| Po | 1540 | 16.50 | 17.59 | 19.62 | 37.21 | 57.23 |
| Arno | 110 | 16.00 | 20.61 | 16.23 | 36.83 | 4.05 |
| Adige | 235 | 12.40 | 42.34 | 15.21 | 57.55 | 13.51 |
| 2017 | EIN [TJ] | EOUT [TJ] | ED [TJ] | EEL [TJ] | EEK [TJ] | EE [TJ] |
| AG | 2,905,903.61 | 1,732,441.10 | 1,173,462.51 | 400,451.72 | 18,640,855.93 | 21,947,211.26 |
| EX | 69,270.50 | 28,010.15 | 41,260.34 | 422,641.02 | 2,174.46 | 494,085.98 |
| IN | 4,211,548.06 | 968,278.84 | 3,243,269.21 | 131,009.99 | 6,148,498.22 | 10,491,056.27 |
| CO | 8,778,510.88 | 4,724,156.23 | 4,054,354.64 | 68,207.93 | 570,731,751.76 | 509,624,525.90 |
| TE | 12,463,938.44 | 10,697,215.70 | 1,766,722.74 | 10,119.53 | 574,185,858.21 | 586,659,916.18 |
| TR | 1,512,342.32 | 388,387.22 | 1,123,955.10 | 3,467.11 | 160,136,961.18 | 161,652,770.61 |
| DO | 1,649,768.67 | 1,035,897.30 | 613,871.38 | −1,035,897.30 | 2,968,488.96 | 3,582,360.34 |
| TOT [TJ] | 28,685,378.88 | 17,841,945.45 | 10,843,433.43 | 0.00 | 1,332,814,588.73 | 1,362,535,864.90 |
| 2016 | EIN [TJ] | EOUT [TJ] | ED [TJ] | EEL [TJ] | EEK [TJ] | EE [TJ] |
| AG | 2,887,089.62 | 1,779,232.17 | 1,107,857.45 | 384,784.55 | 17,040,758.72 | 20,312,632.90 |
| EX | 73,326.31 | 32,032.01 | 41,294.30 | 406,105.73 | 1,974.35 | 481,406.39 |
| IN | 4,947,389.38 | 939,424.50 | 4,007,964.88 | 125,884.39 | 5,499,017.06 | 10,572,290.84 |
| CO | 8,415,800.79 | 4,555,071.14 | 3,860,729.65 | 65,539.38 | 499,290,951.91 | 507,772,292.08 |
| TE | 12,418,922.84 | 10,608,650.80 | 1,810,272.05 | 9,723.62 | 562,765,639.40 | 575,194,285.86 |
| TR | 1,460,256.38 | 401,687.18 | 1,058,569.20 | 3,331.46 | 142,758,547.14 | 144,222,134.99 |
| DO | 1,585,223.57 | 995,369.13 | 589,854.44 | −995,369.13 | 2,739,636.99 | 3,329,491.43 |
| TOT [TJ] | 31,788,008.91 | 19,311,466.94 | 12,476,541.97 | 0.00 | 1,230,096,525.58 | 1,261,884,534.49 |
| 2015 | EIN [TJ] | EOUT [TJ] | ED [TJ] | EEL [TJ] | EEK [TJ] | EE [TJ] |
| AG | 2,940,417.47 | 1,790,296.64 | 1,150,120.84 | 458,248.75 | 19,907,848.46 | 23,306,514.68 |
| EX | 63,189.64 | 13,379.86 | 49,809.78 | 483,640.63 | 2,315.60 | 549,145.87 |
| IN | 4,760,901.76 | 879,050.97 | 3,881,850.79 | 149,918.61 | 5,543,839.70 | 10,454,660.07 |
| CO | 8,250,329.46 | 4,693,211.16 | 3,557,118.30 | 78,052.35 | 563,169,915.75 | 571,498,297.56 |
| TE | 12,408,435.62 | 10,591,634.37 | 1,816,801.25 | 11,580.08 | 634,644,770.81 | 647,064,786.51 |
| TR | 1,484,773.00 | 426,981.31 | 1,057,791.69 | 3,967.52 | 156,483,974.99 | 157,972,715.51 |
| DO | 1,886,587.57 | 1,185,407.93 | 701,179.64 | −1,185,407.93 | 2,560,765.69 | 3,261,945.33 |
| TOT [TJ] | 31,794,634.53 | 19,579,962.24 | 12,214,672.30 | 0.00 | 1,382,313,431.00 | 1,414,108,065.54 |
| 2014 | EIN [TJ] | EOUT [TJ] | ED [TJ] | EEL [TJ] | EEK [TJ] | EE [TJ] |
| AG | 2,960,821.83 | 2,047,404.09 | 913,417.74 | 368,542.81 | 18,263,896.62 | 21,593,261.26 |
| EX | 61,952.45 | 20,389.37 | 41,563.07 | 388,964.02 | 2121.07 | 453,037.53 |
| IN | 5,168,071.30 | 862,206.77 | 4,305,864.53 | 120,570.81 | 3,606,893.72 | 8,895,535.83 |
| CO | 8,268,030.08 | 4,493,085.87 | 3,774,944.21 | 62,772.97 | 491,679,976.73 | 500,010,779.78 |
| TE | 12,017,586.72 | 10,678,869.03 | 1,338,717.69 | 9,313.18 | 498,016,783.11 | 510,043,683.01 |
| TR | 1,512,740.62 | 413,236.00 | 1,099,504.62 | 3,190.84 | 143,650,215.89 | 145,166,147.35 |
| DO | 1,515,470.32 | 953,354.64 | 562,115.68 | −953,354.64 | 2,829,459.95 | 3,391,575.63 |
| TOT [TJ] | 31,504,673.31 | 19,468,545.78 | 12,036,127.53 | 0.00 | 1,158,049,347.09 | 1,189,554,020.40 |
| 2013 | EIN [TJ] | EOUT [TJ] | ED [TJ] | EEL [TJ] | EEK [TJ] | EE [TJ] |
| AG | 2,892,355.64 | 1,991,910.97 | 900,444.68 | 400,758.53 | 18,416,433.35 | 21,709,547.52 |
| EX | 65,804.92 | 22,764.72 | 43,040.20 | 422,964.83 | 2,301.82 | 491,071.57 |
| IN | 5,381,999.01 | 949,041.46 | 4,432,957.55 | 131,110.36 | 6,703,977.00 | 12,217,086.37 |
| CO | 8,317,612.65 | 4,618,955.00 | 3,698,657.65 | 68,260.19 | 529,971,342.64 | 538,357,215.48 |
| TE | 12,365,041.56 | 10,607,832.42 | 1,757,209.14 | 10,127.29 | 597,372,908.36 | 609,748,077.20 |
| TR | 1,456,877.83 | 400,678.69 | 1,056,199.13 | 3,469.77 | 144,359,578.27 | 145,819,925.86 |
| DO | 1,651,032.66 | 1,036,690.96 | 614,341.70 | −1,036,690.96 | 2,960,190.38 | 3,574,532.08 |
| TOT [TJ] | 32,130,724.27 | 19,627,874.22 | 12,502,850.05 | 0.00 | 1,299,786,731.81 | 1,331,917,456.08 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Biondi, A.; Sciubba, E. New Insights from Econometric Data: An Extended Exergy Analysis (EEA) of the Italian System, 2013–2017. Proceedings 2020, 58, 3. https://doi.org/10.3390/WEF-06933
Biondi A, Sciubba E. New Insights from Econometric Data: An Extended Exergy Analysis (EEA) of the Italian System, 2013–2017. Proceedings. 2020; 58(1):3. https://doi.org/10.3390/WEF-06933
Chicago/Turabian StyleBiondi, Alfonso, and Enrico Sciubba. 2020. "New Insights from Econometric Data: An Extended Exergy Analysis (EEA) of the Italian System, 2013–2017" Proceedings 58, no. 1: 3. https://doi.org/10.3390/WEF-06933
APA StyleBiondi, A., & Sciubba, E. (2020). New Insights from Econometric Data: An Extended Exergy Analysis (EEA) of the Italian System, 2013–2017. Proceedings, 58(1), 3. https://doi.org/10.3390/WEF-06933






