Thermal Conversion of Pine Wood and Kinetic Analysis under Oxidative and Non-Oxidative Environments at Low Heating Rate †
Abstract
1. Introduction
2. Materials and Methods
2.1. Material and Sample Preparation
2.2. Thermogravimentric Experiments
2.3. Kinetics Analysis
3. Results and Discussion
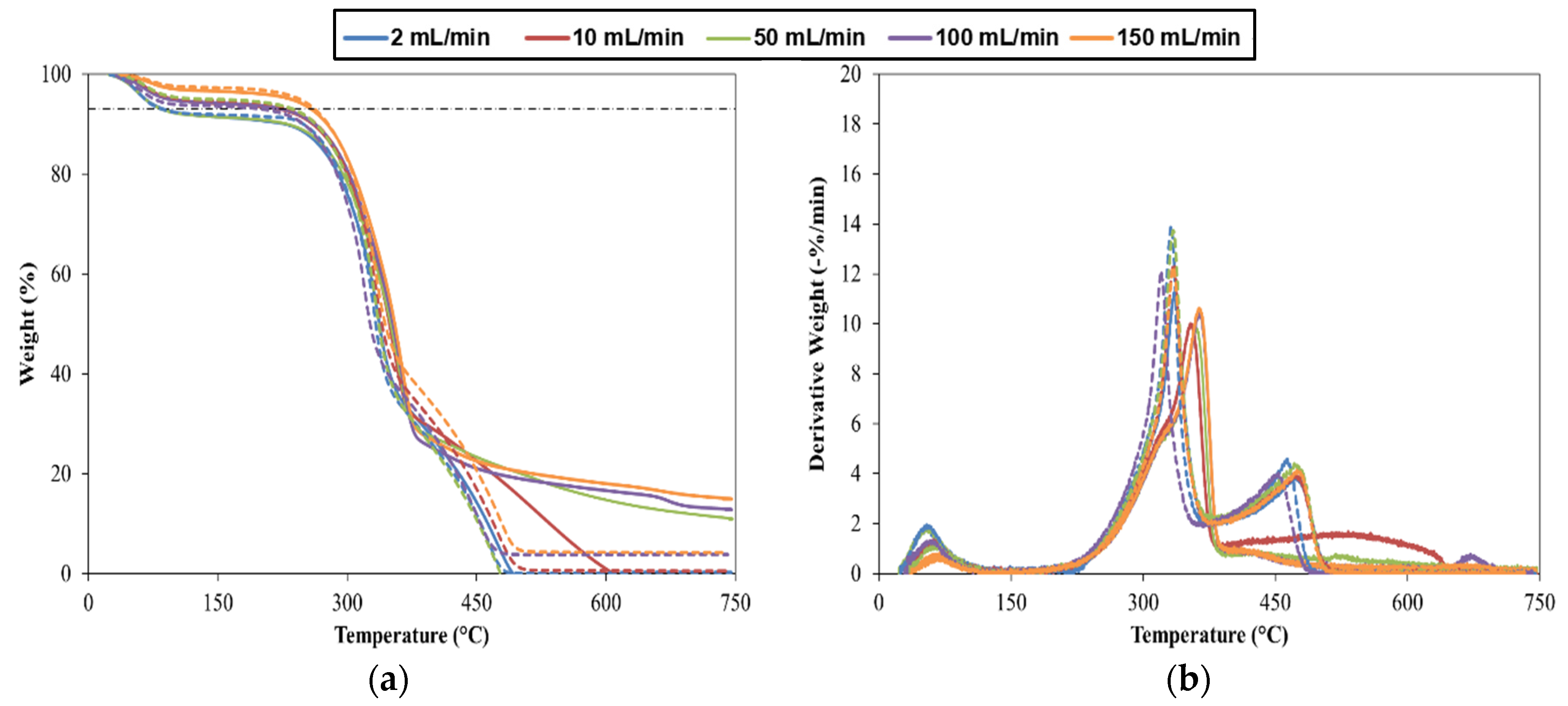
3.1. Thermal Decomposition Analysis
3.2. Kinetics Analysis
4. Conclusions
- It was observed that the thermal decomposition of pine wood particles varied from three to two stages in the presence of an oxidative or inert atmosphere respectively;
- The presence of oxygen changes the mass loss curve mainly at high temperature, around 350 °C, where char reacts with oxygen and promotes the degradation of the remaining mass. Therefore, the gas-phase reactions enhance the thermal conversion when compared with experiments with an inert atmosphere;
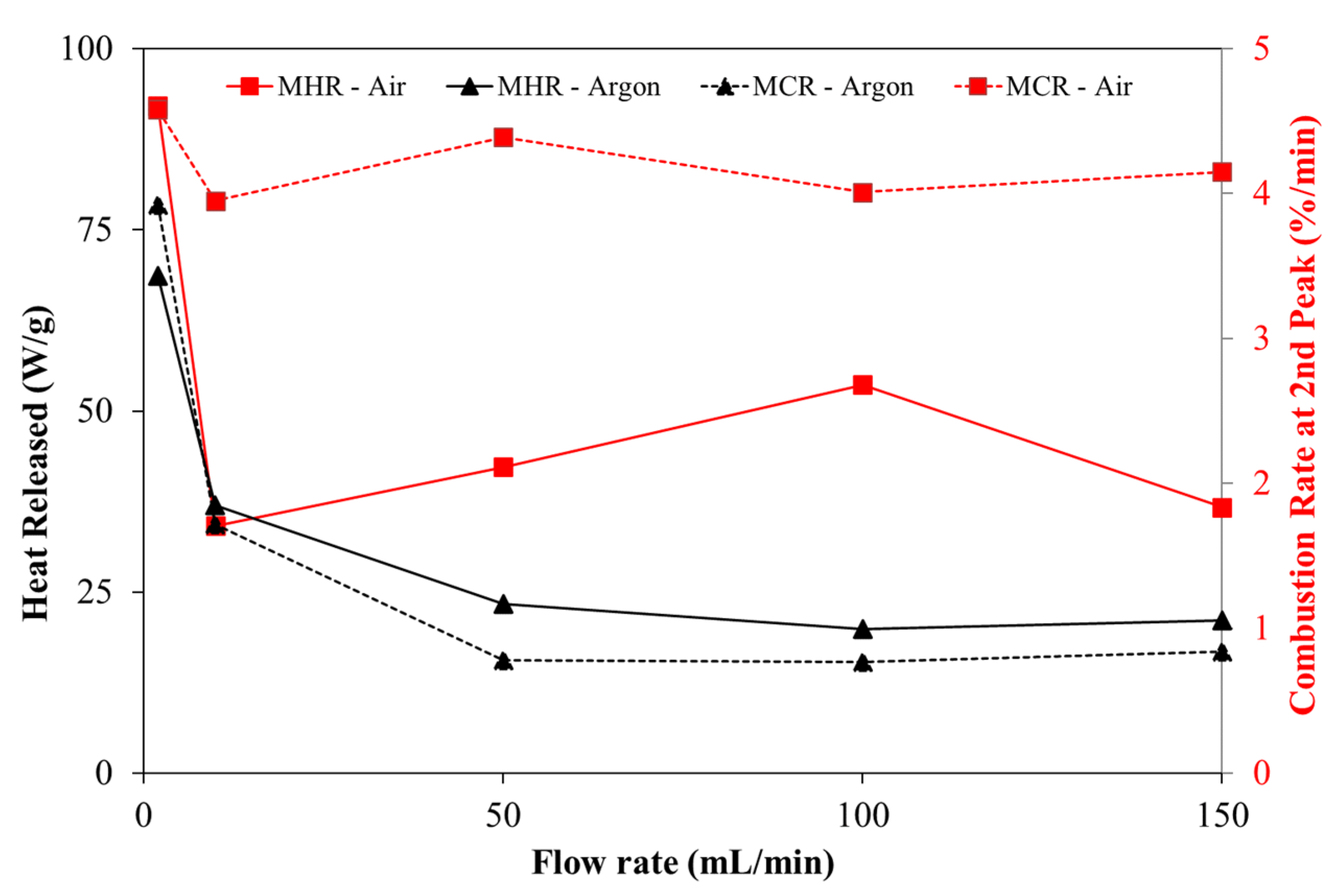
- The maximum value of DTG curves from experiments with the oxidative atmosphere is 15% higher than in an inert atmosphere, and the time to complete the reaction is also higher since the char mass loss continues at a slower rate and this process is associated with the slow degradation of lignin. Additionally, the maximum values of DTG curves were shifted to a lower temperature when compared to the maximum values obtained with the inert atmosphere;
- The average char combustion rate is approximately 5 times higher with the oxidative atmosphere when the flow rate was higher than 10 mL/min;
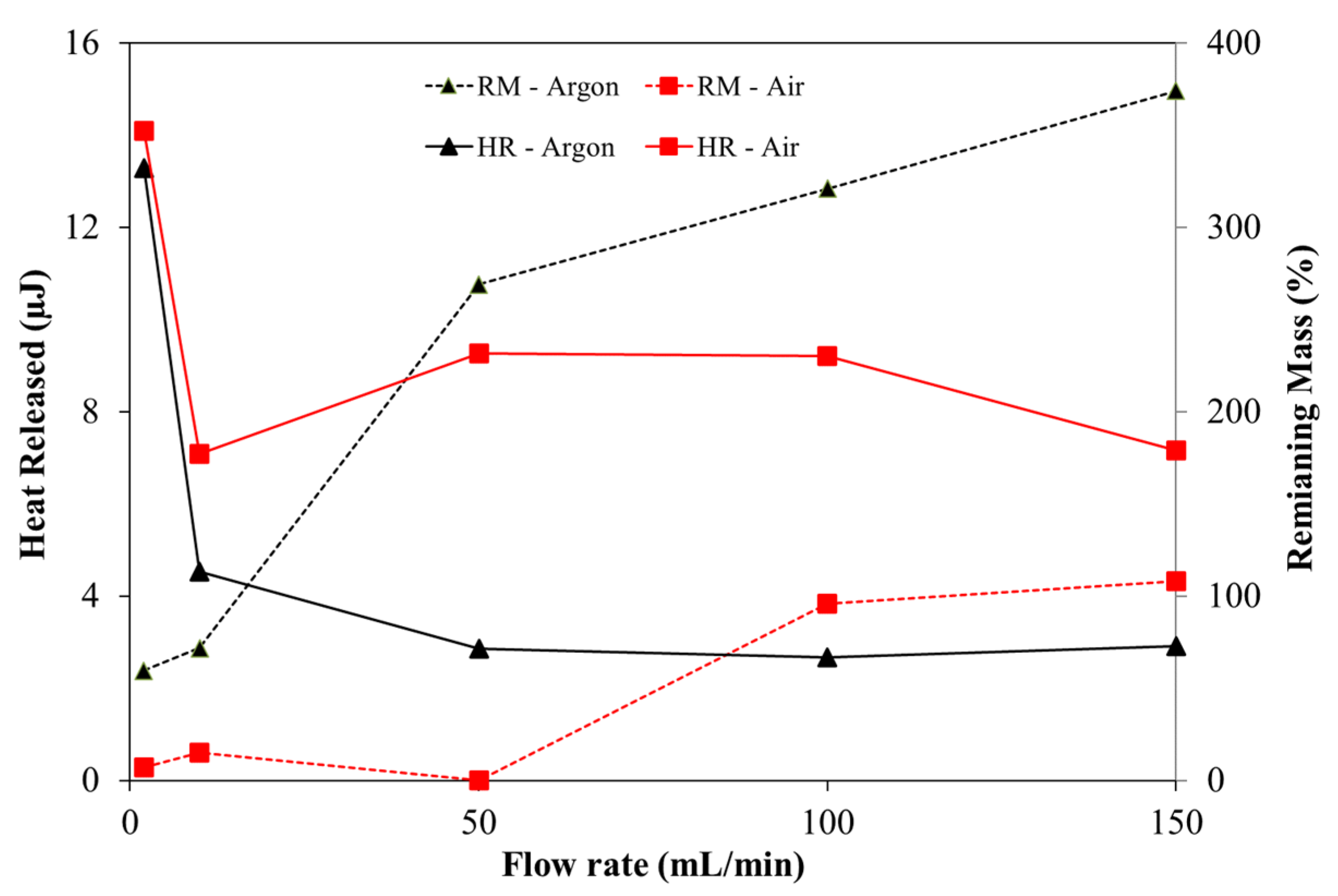
- Regarding the maximum heat released, significant differences were observed as the gas flow rate was increased. However, the heat released in oxidative experiments is up to 3.44 times higher than in an inert atmosphere;
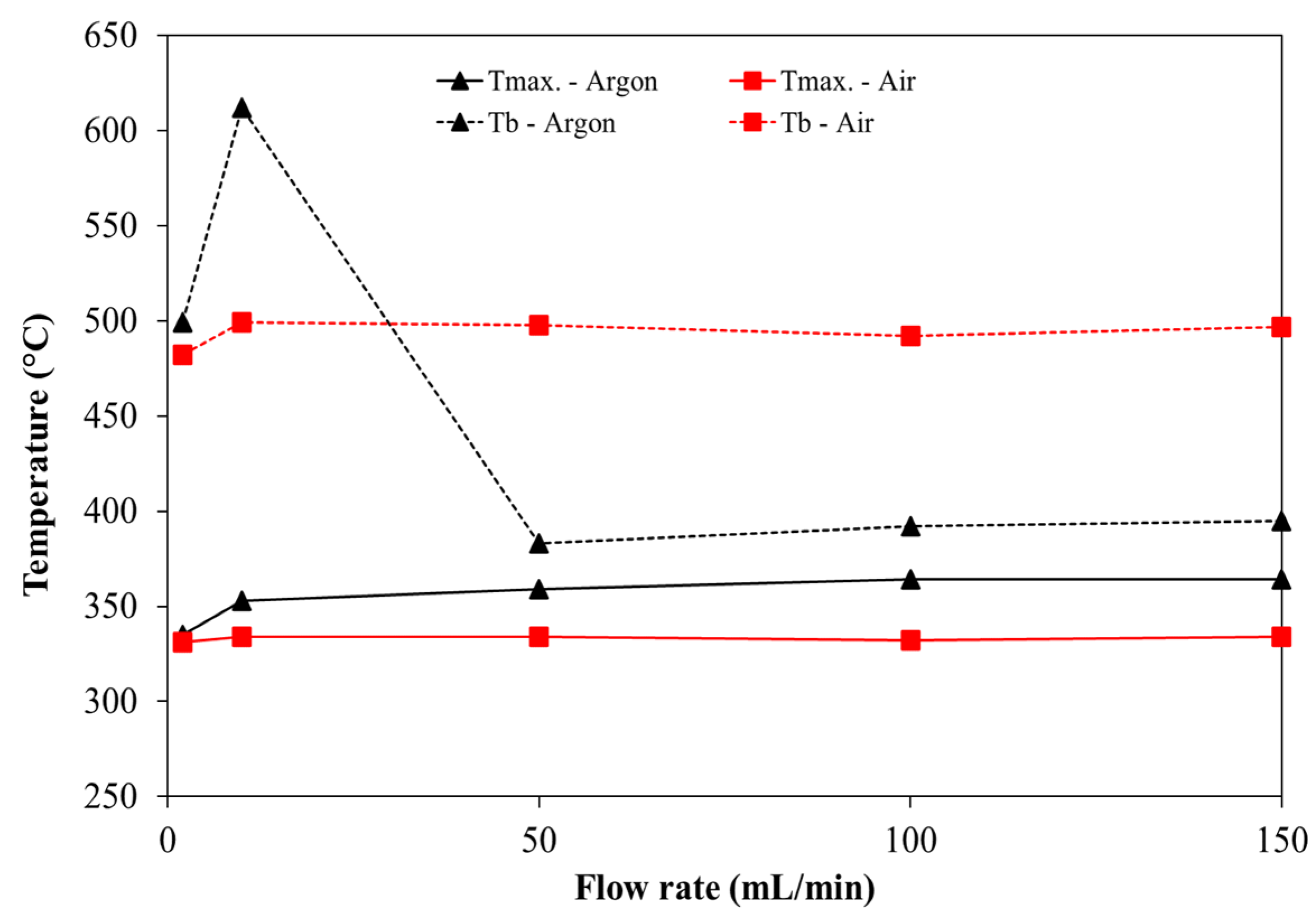
- As a result of the presence of the oxygen, Tmax is lower and Tb is higher than experiments under an inert atmosphere. Regarding the other characteristic temperatures, Tin was not significantly affected by the atmosphere but, Tig is lower around 10 °C for the case where combustion takes place. Therefore, the oxidative environment enhances the ignition of pine and leads to faster weight loss;
- The index D presents higher values which means that pine wood particles are ignited faster than under inert atmosphere. The comprehensive characteristic of the fuel defined by index S also presents higher values for the case where the oxidative atmosphere was applied. On average, its value is 1.7 times higher, which reinforces the more vigorously way that the samples are burned and how char is burned out faster in the experiments with air;
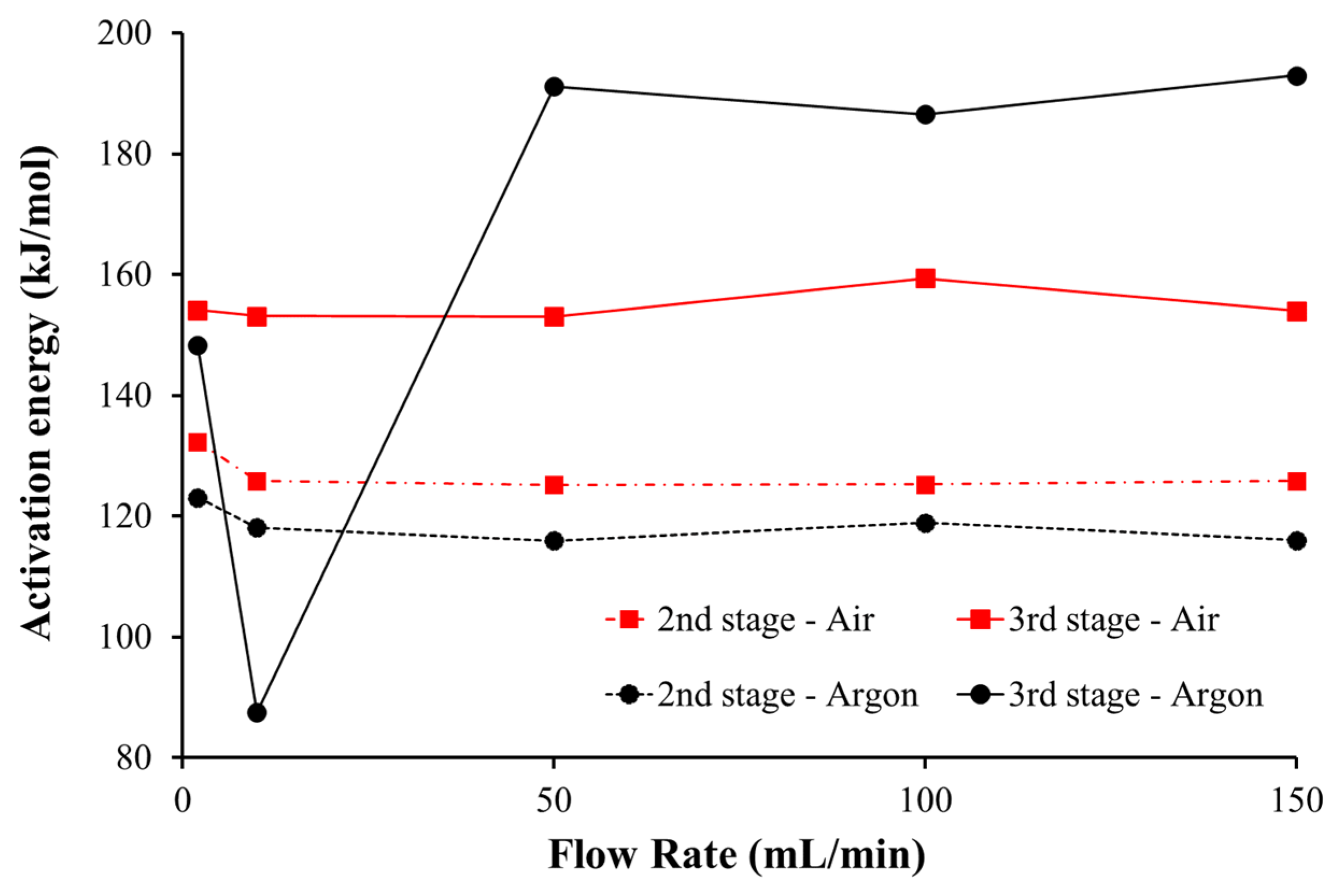
- The kinetics analysis was carried out considering a first-order reaction to each thermal conversion stage and the Coats–Redfern method. Results revealed that the kinetic parameters are affected by the gaseous atmosphere;
- Higher activation energies and, consequently, lower reactivity was obtained under the oxidative atmosphere for the second stage and under the inert atmosphere for the third thermal conversion stage. Although, in the second stage, the differences are not substantially different, this means that the reaction of the volatiles released and combustion are less reactive than the pyrolysis. The opposite trend happens in the last conversion stage at higher flow rates.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| A | pre-exponential factor, min−1 |
| D | ignition index, %/min3 |
| DTG | derivative thermogravimetric |
| E | activation energy, kJ/mol |
| HR | heat released |
| k | rate of the chemical reaction, min−1 |
| m | mass, mg |
| MCR | maximum combustion rate |
| MHR | maximum heat released |
| R | universal gas contant, kJ/(mol.K) |
| RM | remaining mass |
| S | combustion index, %/(min2.K3) |
| t | time, min−1 |
| T | temperature, °C |
| TG | thermogravimetric |
| TGA | thermogravimetric analysis |
| Greek symbols | |
| α | conversion rate, - |
| β | heating rate, °C/min |
| Subscripts and superscripts | |
| 0 | initial |
| a | air |
| avg | average |
| b | burnout |
| f | final |
| ig | ignition |
| in | initial decomposition |
| max | maximum |
| t | time |
References
- Mandova, H.; Leduc, S.; Wang, C.; Wetterlund, E.; Patrizio, P.; Gale, W.; Kraxner, F. Possibilities for CO2 emission reduction using biomass in European integrated steel plants. Biomass Bioenergy 2018, 115, 231–243. [Google Scholar] [CrossRef]
- Malico, I.; Nepomuceno Pereira, R.; Gonçalves, A.C.; Sousa, A.M.O. Current status and future perspectives for energy production from solid biomass in the European industry. Renew. Sustain. Energy Rev. 2019, 112, 960–977. [Google Scholar] [CrossRef]
- Nussbaumer, T. Combustion and Co-combustion of Biomass: Fundamentals, Technologies, and Primary Measures for Emission Reduction. Energy Fuels 2003, 17, 1510–1521. [Google Scholar] [CrossRef]
- White, J.E.; Catallo, W.J.; Legendre, B.L. Biomass pyrolysis kinetics: A comparative critical review with relevant agricultural residue case studies. J. Anal. Appl. Pyrolysis 2011, 91, 1–33. [Google Scholar] [CrossRef]
- Cai, J.; Xu, D.; Dong, Z.; Yu, X.; Yang, Y.; Banks, S.W.; Bridgwater, A.V. Processing thermogravimetric analysis data for isoconversional kinetic analysis of lignocellulosic biomass pyrolysis: Case study of corn stalk. Renew. Sustain. Energy Rev. 2018, 82, 2705–2715. [Google Scholar] [CrossRef]
- Anca-Couce, A.; Tsekos, C.; Retschitzegger, S.; Zimbardi, F.; Funke, A.; Banks, S.; Kraia, T.; Marques, P.; Scharler, R.; de Jong, W.; et al. Biomass pyrolysis TGA assessment with an international round robin. Fuel 2020, 276, 118002. [Google Scholar] [CrossRef]
- Williams, P.T.; Besler, S. The influence of temperature and heating rate on the slow pyrolysis of biomass. Renew. Energy 1996, 7, 233–250. [Google Scholar] [CrossRef]
- Kleinhans, U.; Wieland, C.; Frandsen, F.J.; Spliethoff, H. Ash formation and deposition in coal and biomass fired combustion systems: Progress and challenges in the field of ash particle sticking and rebound behavior. Prog. Energy Combust. Sci. 2018, 68, 65–168. [Google Scholar] [CrossRef]
- Fang, M.X.; Shen, D.K.; Li, Y.X.; Yu, C.J.; Luo, Z.Y.; Cen, K.F. Kinetic study on pyrolysis and combustion of wood under different oxygen concentrations by using TG-FTIR analysis. J. Anal. Appl. Pyrolysis 2006, 77, 22–27. [Google Scholar] [CrossRef]
- Yin, C.; Rosendahl, L.A.; Kær, S.K. Grate-firing of biomass for heat and power production. Prog. Energy Combust. Sci. 2008, 34, 725–754. [Google Scholar] [CrossRef]
- Grønli, M.G.; Várhegyi, G.; Di Blasi, C. Thermogravimetric Analysis and Devolatilization Kinetics of Wood. Ind. Eng. Chem. Res. 2002, 41, 4201–4208. [Google Scholar] [CrossRef]
- Saddawi, A.; Jones, J.M.; Williams, A.; Wójtowicz, M.A. Kinetics of the Thermal Decomposition of Biomass. Energy Fuels 2010, 24, 1274–1282. [Google Scholar] [CrossRef]
- Wadhwani, R.; Sutherland, D.; Moinuddin, K.A.M.; Joseph, P. Kinetics of pyrolysis of litter materials from pine and eucalyptus forests. J. Therm. Anal. Calorim. 2017, 130, 2035–2046. [Google Scholar] [CrossRef]
- Xiao, R.; Yang, W.; Cong, X.; Dong, K.; Xu, J.; Wang, D.; Yang, X. Thermogravimetric analysis and reaction kinetics of lignocellulosic biomass pyrolysis. Energy 2020, 201, 117537. [Google Scholar] [CrossRef]
- Alvarado Flores, J.J.; Rutiaga Quiñones, J.G.; Ávalos Rodríguez, M.L.; Alcaraz Vera, J.V.; Espino Valencia, J.; Guevara Martínez, S.J.; Márquez Montesino, F.; Alfaro Rosas, A. Thermal Degradation Kinetics and FT-IR Analysis on the Pyrolysis of Pinus pseudostrobus, Pinus leiophylla and Pinus montezumae as Forest Waste in Western Mexico. Energies 2020, 13, 969. [Google Scholar] [CrossRef]
- Darvell, L.I.; Jones, J.M.; Gudka, B.; Baxter, X.C.; Saddawi, A.; Williams, A.; Malmgren, A. Combustion properties of some power station biomass fuels. Fuel 2010, 89, 2881–2890. [Google Scholar] [CrossRef]
- Kim, S.-S.; Kim, J.; Park, Y.-H.; Park, Y.-K. Pyrolysis kinetics and decomposition characteristics of pine trees. Bioresour. Technol. 2010, 101, 9797–9802. [Google Scholar] [CrossRef]
- Mani, T.; Murugan, P.; Abedi, J.; Mahinpey, N. Pyrolysis of wheat straw in a thermogravimetric analyzer: Effect of particle size and heating rate on devolatilization and estimation of global kinetics. Chem. Eng. Res. Des. 2010, 88, 952–958. [Google Scholar] [CrossRef]
- Seo, D.K.; Park, S.S.; Hwang, J.; Yu, T.-U. Study of the pyrolysis of biomass using thermo-gravimetric analysis (TGA) and concentration measurements of the evolved species. J. Anal. Appl. Pyrolysis 2010, 89, 66–73. [Google Scholar] [CrossRef]
- Slopiecka, K.; Bartocci, P.; Fantozzi, F. Thermogravimetric analysis and kinetic study of poplar wood pyrolysis. Appl. Energy 2012, 97, 491–497. [Google Scholar] [CrossRef]
- Chen, D.; Zheng, Y.; Zhu, X. In-depth investigation on the pyrolysis kinetics of raw biomass. Part I: Kinetic analysis for the drying and devolatilization stages. Bioresour. Technol. 2013, 131, 40–46. [Google Scholar] [CrossRef] [PubMed]
- Parthasarathy, P.; Narayanan, K.S.; Arockiam, L. Study on kinetic parameters of different biomass samples using thermo-gravimetric analysis. Biomass Bioenergy 2013, 58, 58–66. [Google Scholar] [CrossRef]
- Saldarriaga, J.F.; Aguado, R.; Pablos, A.; Amutio, M.; Olazar, M.; Bilbao, J. Fast characterization of biomass fuels by thermogravimetric analysis (TGA). Fuel 2015, 140, 744–751. [Google Scholar] [CrossRef]
- Di Blasi, C. Modeling chemical and physical processes of wood and biomass pyrolysis. Prog. Energy Combust. Sci. 2008, 34, 47–90. [Google Scholar] [CrossRef]
- Bilbao, R.; Mastral, J.F.; Aldea, M.E.; Ceamanos, J. Kinetic study for the thermal decomposition of cellulose and pine sawdust in an air atmosphere. J. Anal. Appl. Pyrolysis 1997, 39, 53–64. [Google Scholar] [CrossRef]
- Shen, D.K.; Gu, S.; Luo, K.H.; Bridgwater, A.V.; Fang, M.X. Kinetic study on thermal decomposition of woods in oxidative environment. Fuel 2009, 88, 1024–1030. [Google Scholar] [CrossRef]
- Yorulmaz, S.Y.; Atimtay, A.T. Investigation of combustion kinetics of treated and untreated waste wood samples with thermogravimetric analysis. Fuel Process. Technol. 2009, 90, 939–946. [Google Scholar] [CrossRef]
- Garcia-Maraver, A.; Perez-Jimenez, J.A.; Serrano-Bernardo, F.; Zamorano, M. Determination and comparison of combustion kinetics parameters of agricultural biomass from olive trees. Renew. Energy 2015, 83, 897–904. [Google Scholar] [CrossRef]
- Ren, X.; Chen, J.; Li, G.; Wang, Y.; Lang, X.; Fan, S. Thermal oxidative degradation kinetics of agricultural residues using distributed activation energy model and global kinetic model. Bioresour. Technol. 2018, 261, 403–411. [Google Scholar] [CrossRef]
- Dhahak, A.; Bounaceur, R.; Le Dreff-Lorimier, C.; Schmidt, G.; Trouve, G.; Battin-Leclerc, F. Development of a detailed kinetic model for the combustion of biomass. Fuel 2019, 242, 756–774. [Google Scholar] [CrossRef]
- Fraga, L.G.; Silva, J.; Teixeira, S.; Soares, D.; Ferreira, M.; Teixeira, J. Influence of Operating Conditions on the Thermal Behavior and Kinetics of Pine Wood Particles using Thermogravimetric Analysis. Energies 2020, 13, 2756. [Google Scholar] [CrossRef]
- Shen, D.K.; Gu, S.; Jin, B.; Fang, M.X. Thermal degradation mechanisms of wood under inert and oxidative environments using DAEM methods. Bioresour. Technol. 2011, 102, 2047–2052. [Google Scholar] [CrossRef] [PubMed]
- Anca-Couce, A.; Zobel, N.; Berger, A.; Behrendt, F. Smouldering of pine wood: Kinetics and reaction heats. Combust. Flame 2012, 159, 1708–1719. [Google Scholar] [CrossRef]
- Munir, S.; Daood, S.S.; Nimmo, W.; Cunliffe, A.M.; Gibbs, B.M. Thermal analysis and devolatilization kinetics of cotton stalk, sugar cane bagasse and shea meal under nitrogen and air atmospheres. Bioresour. Technol. 2009, 100, 1413–1418. [Google Scholar] [CrossRef]
- Yuzbasi, N.S.; Selçuk, N. Air and oxy-fuel combustion characteristics of biomass/lignite blends in TGA-FTIR. Fuel Process. Technol. 2011, 92, 1101–1108. [Google Scholar] [CrossRef]
- Sher, F.; Iqbal, S.Z.; Liu, H.; Imran, M.; Snape, C.E. Thermal and kinetic analysis of diverse biomass fuels under different reaction environment: A way forward to renewable energy sources. Energy Convers. Manag. 2020, 203, 112266. [Google Scholar] [CrossRef]
- Chouchene, A.; Jeguirim, M.; Khiari, B.; Zagrouba, F.; Trouvé, G. Thermal degradation of olive solid waste: Influence of particle size and oxygen concentration. Resour. Conserv. Recycl. 2010, 54, 271–277. [Google Scholar] [CrossRef]
- Su, Y.; Luo, Y.; Wu, W.; Zhang, Y.; Zhao, S. Characteristics of pine wood oxidative pyrolysis: Degradation behavior, carbon oxide production and heat properties. J. Anal. Appl. Pyrolysis 2012, 98, 137–143. [Google Scholar] [CrossRef]
- Amutio, M.; Lopez, G.; Aguado, R.; Artetxe, M.; Bilbao, J.; Olazar, M. Kinetic study of lignocellulosic biomass oxidative pyrolysis. Fuel 2012, 95, 305–311. [Google Scholar] [CrossRef]
- Moreno, A.I.; Font, R.; Conesa, J.A. Combustion of furniture wood waste and solid wood: Kinetic study and evolution of pollutants. Fuel 2017, 192, 169–177. [Google Scholar] [CrossRef]
- Magalhães, D.; Kazanç, F.; Riaza, J.; Erensoy, S.; Kabaklı, Ö.; Chalmers, H. Combustion of Turkish lignites and olive residue: Experiments and kinetic modelling. Fuel 2017, 203, 868–876. [Google Scholar] [CrossRef]
- Lin, Y.; Ma, X.; Ning, X.; Yu, Z. TGA–FTIR analysis of co-combustion characteristics of paper sludge and oil-palm solid wastes. Energy Convers. Manag. 2015, 89, 727–734. [Google Scholar] [CrossRef]
- Sanchez, M.E.; Otero, M.; Gómez, X.; Morán, A. Thermogravimetric kinetic analysis of the combustion of biowastes. Renew. Energy 2009, 34, 1622–1627. [Google Scholar] [CrossRef]
- Fang, X.; Jia, L.; Yin, L. A weighted average global process model based on two−stage kinetic scheme for biomass combustion. Biomass Bioenergy 2013, 48, 43–50. [Google Scholar] [CrossRef]
- Gangavati, P.B.; Safi, M.J.; Singh, A.; Prasad, B.; Mishra, I.M. Pyrolysis and thermal oxidation kinetics of sugar mill press mud. Thermochim. Acta 2005, 428, 63–70. [Google Scholar] [CrossRef]
- Branca, C.; Albano, A.; Di Blasi, C. Critical evaluation of global mechanisms of wood devolatilization. Thermochim. Acta 2005, 429, 133–141. [Google Scholar] [CrossRef]
- Várhegyi, G. Aims and methods in non-isothermal reaction kinetics. J. Anal. Appl. Pyrolysis 2007, 79, 278–288. [Google Scholar] [CrossRef][Green Version]
- Garcia-Maraver, A.; Perez-Jimenez, J.A.; Serrano-Bernardo, F.; Zamorano, M. Determination and comparison of combustion kinetics parameters of agricultural biomass from olive trees. Renew. Energy 2015, 83, 897–904. [Google Scholar] [CrossRef]
- Álvarez, A.; Pizarro, C.; García, R.; Bueno, J.L.; Lavín, A.G. Determination of kinetic parameters for biomass combustion. Bioresour. Technol. 2016, 216, 36–43. [Google Scholar] [CrossRef]
- Yu, D.; Chen, M.; Wei, Y.; Niu, S.; Xue, F. An assessment on co-combustion characteristics of Chinese lignite and eucalyptus bark with TG–MS technique. Powder Technol. 2016, 294, 463–471. [Google Scholar] [CrossRef]
- Mishra, R.K.; Mohanty, K. Pyrolysis kinetics and thermal behavior of waste sawdust biomass using thermogravimetric analysis. Bioresour. Technol. 2018, 251, 63–74. [Google Scholar] [CrossRef] [PubMed]
- Coats, A.W.; Redfern, J.P. Kinetic Parameters from Thermogravimetric Data. Nature 1964, 201, 68–69. [Google Scholar] [CrossRef]

| Proximate Analysis (wt.%, As Received) | Ultimate Analysis (wt.%, Dry Ash Free) | ||
|---|---|---|---|
| Moisture | 6.90 | Carbon | 50.90 |
| Volatile matter | 77.80 | Hydrogen | 5.30 |
| Ash | 0.60 | Nitrogen | 1.55 |
| Fixed carbon | 14.70 | Sulphur | 0.03 |
| Oxygen | 42.22 | ||
| Atmosphere | Flow Rate (mL/min) | 2nd Stage | 3rd Stage | ||||
|---|---|---|---|---|---|---|---|
| T (°C) | A (min-1) | R2 | T (°C) | A (min-1) | R2 | ||
| Air | 2 | 247–370 | 3.75 × 1012 | 0.960 | 370–482 | 1.72 × 1012 | 0.920 |
| 10 | 250–380 | 7.38 × 1011 | 0.957 | 380–499 | 9.03 × 1011 | 0.924 | |
| 50 | 248–380 | 6.52 × 1011 | 0.958 | 380–498 | 8.82 × 1011 | 0.923 | |
| 100 | 250–380 | 6.88 × 1011 | 0.954 | 380–492 | 2.96 × 1012 | 0.925 | |
| 150 | 250–380 | 7.38 × 1011 | 0.957 | 380–497 | 1.04 × 1012 | 0.923 | |
| Argon | 2 | 248–378 | 4.50 × 1011 | 0.956 | 378–499 | 3.99 × 1011 | 0.920 |
| 10 | 249–390 | 1.16 × 1011 | 0.957 | 390–612 | 2.39 × 106 | 0.901 | |
| 50 | 242–380 | 1.13 × 1011 | 0.955 | 380–474 | 1.16 × 1015 | 0.926 | |
| 100 | 239–370 | 2.72 × 1011 | 0.957 | 370–456 | 7.95 × 1014 | 0.896 | |
| 150 | 242–380 | 1.15 × 1011 | 0.954 | 380–473 | 1.61 × 1015 | 0.926 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fraga, L.G.; Silva, J.; Teixeira, S.; Soares, D.; Ferreira, M.; Teixeira, J. Thermal Conversion of Pine Wood and Kinetic Analysis under Oxidative and Non-Oxidative Environments at Low Heating Rate. Proceedings 2020, 58, 23. https://doi.org/10.3390/WEF-06921
Fraga LG, Silva J, Teixeira S, Soares D, Ferreira M, Teixeira J. Thermal Conversion of Pine Wood and Kinetic Analysis under Oxidative and Non-Oxidative Environments at Low Heating Rate. Proceedings. 2020; 58(1):23. https://doi.org/10.3390/WEF-06921
Chicago/Turabian StyleFraga, Lelis Gonzaga, João Silva, Senhorinha Teixeira, Delfim Soares, Manuel Ferreira, and José Teixeira. 2020. "Thermal Conversion of Pine Wood and Kinetic Analysis under Oxidative and Non-Oxidative Environments at Low Heating Rate" Proceedings 58, no. 1: 23. https://doi.org/10.3390/WEF-06921
APA StyleFraga, L. G., Silva, J., Teixeira, S., Soares, D., Ferreira, M., & Teixeira, J. (2020). Thermal Conversion of Pine Wood and Kinetic Analysis under Oxidative and Non-Oxidative Environments at Low Heating Rate. Proceedings, 58(1), 23. https://doi.org/10.3390/WEF-06921








