Fixed Bed Regenerators for HVAC Applications †
Abstract
:1. Introduction
2. Experimental and Theoretical Methods
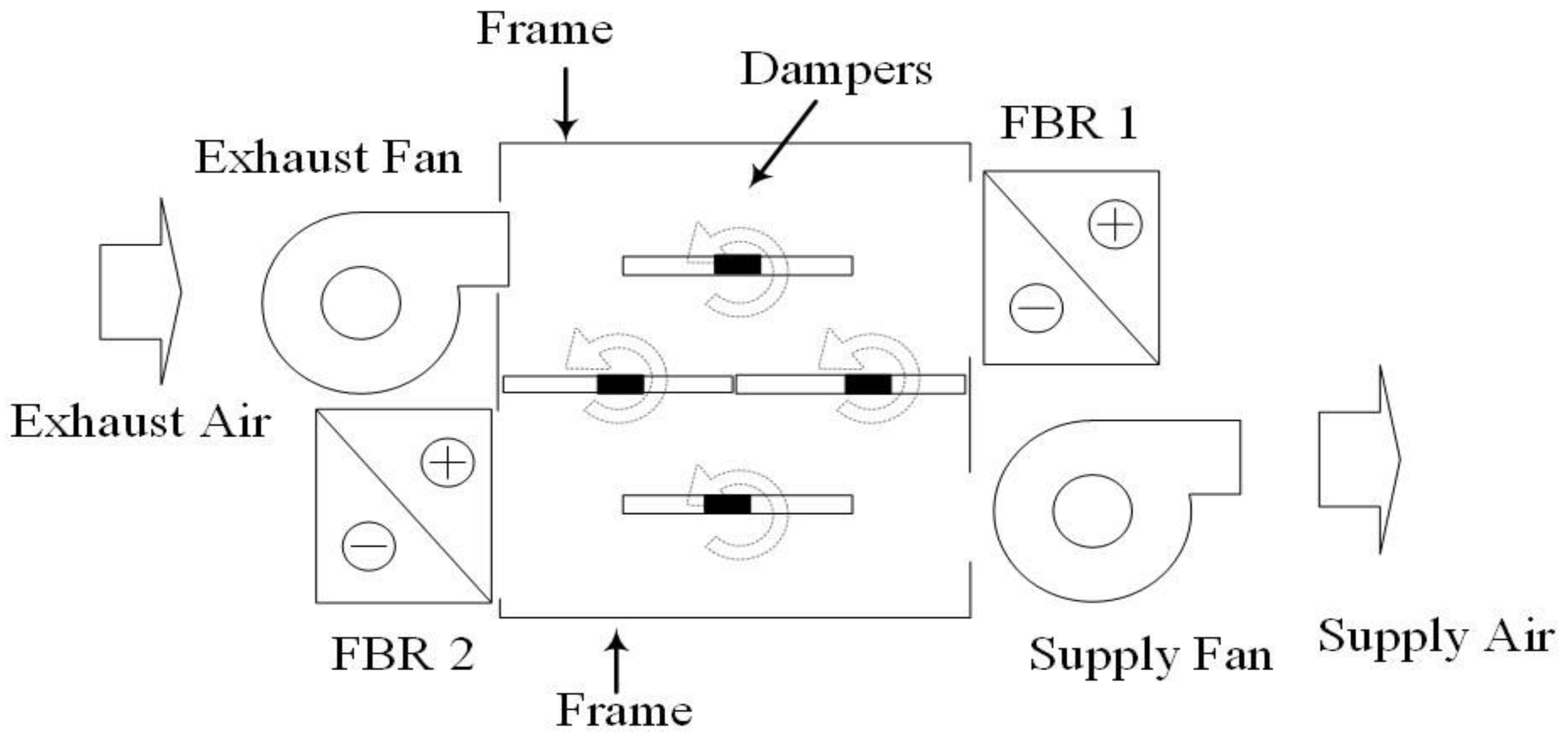
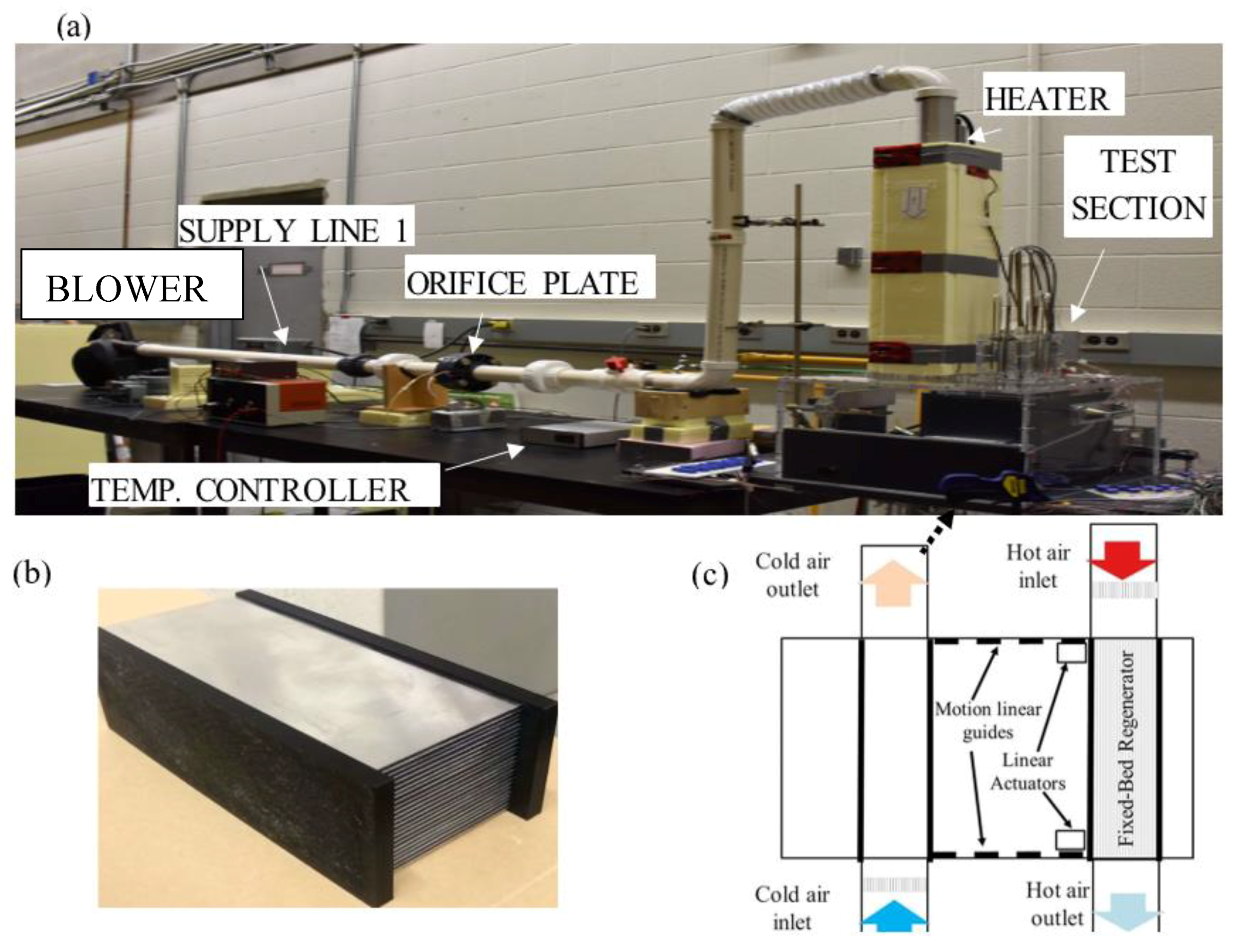
2.1. Experimental Facility
2.2. Numerical Model
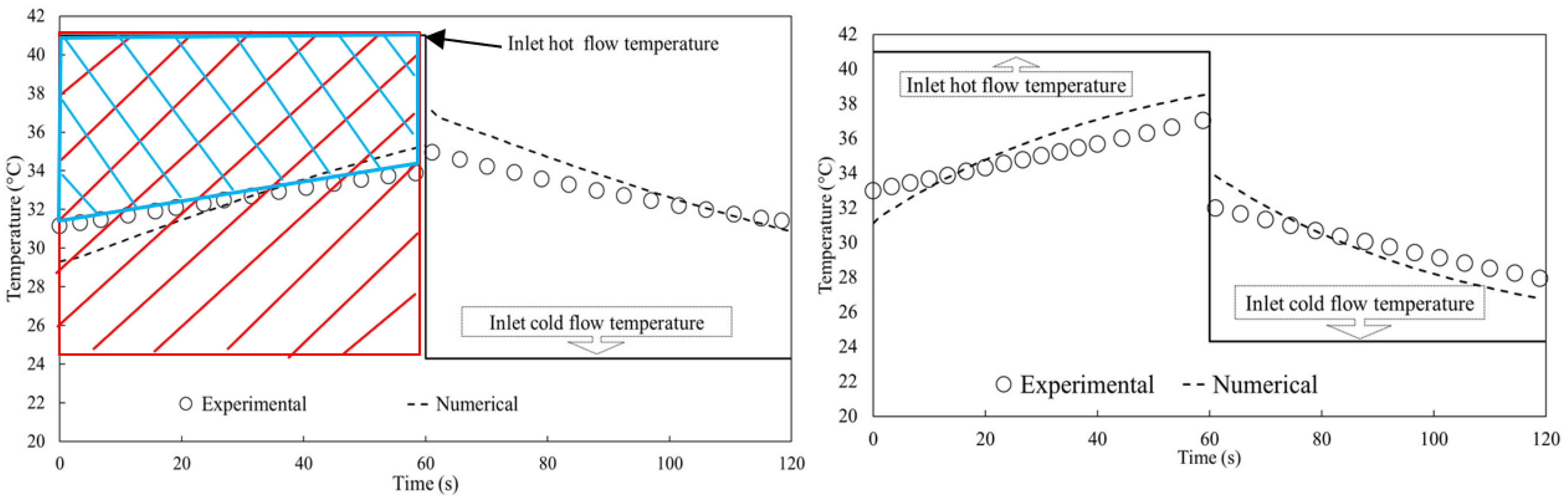
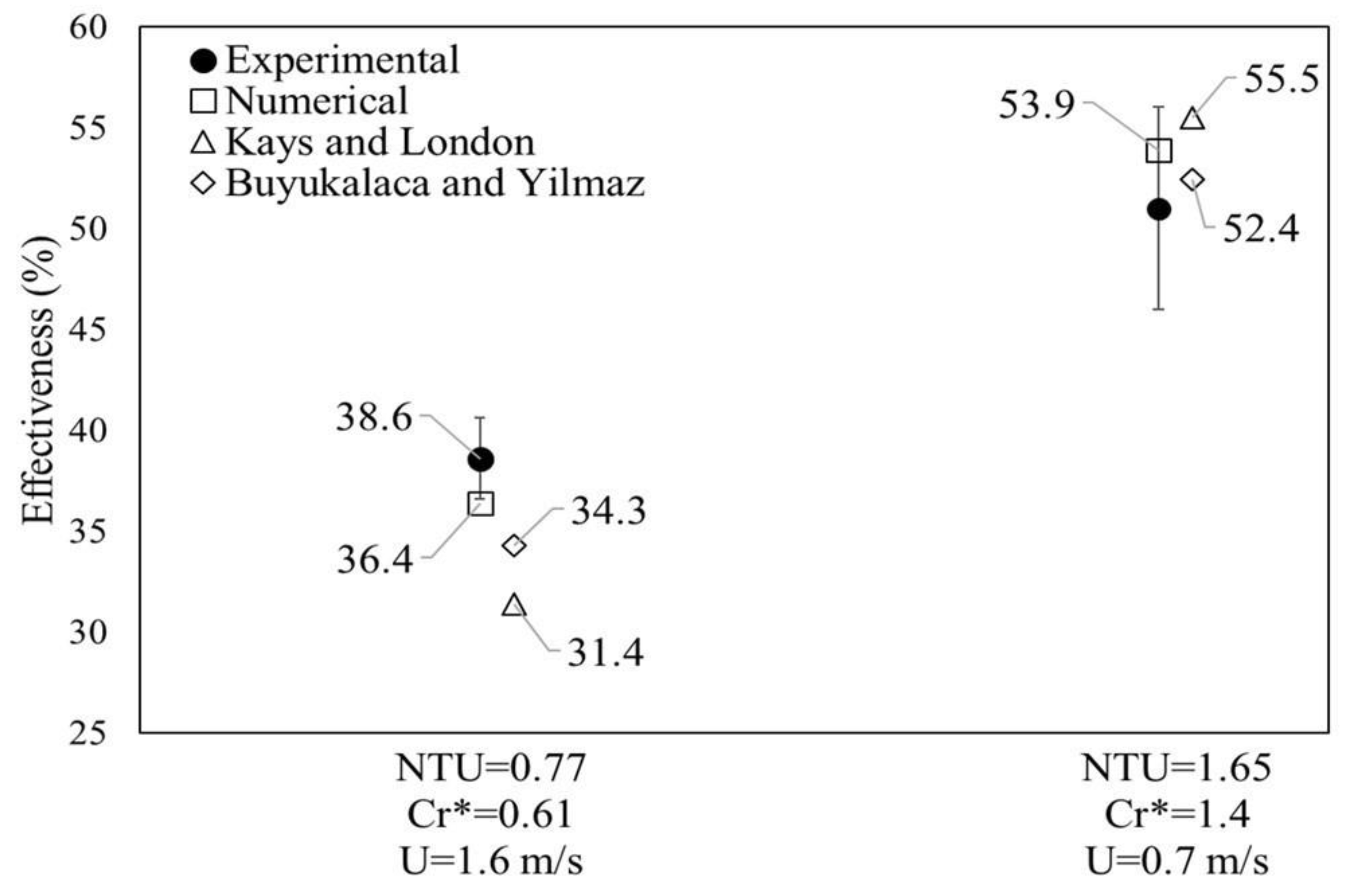
3. Results and Discussions
4. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Natural Resources Canada, Energy Use Data Handbook 1990–2015. Available online: http://oee.nrcan.gc.ca (accessed on 25 July 2018).
- Al-Abidi, A.A.; Bin Mat, S.; Sopian, K.; Sulaiman, M.Y.; Lim, C.H.; Abdulrahman, T. Review of thermal energy storage for air conditioning systems. Renew. Sustain. Energy Rev. 2012, 16, 58025819. [Google Scholar] [CrossRef]
- Singh, J. Impact of indoor air pollution on health, comfort and productivity of the occupants. Int. J. Aerobiol. 1996, 34, 121–127. [Google Scholar] [CrossRef]
- Tempeff Ventilation Technologies. How energy recovery ventilation works. Available online: https://www.tempeffnorthamerica.com/ (accessed on 25 July 2018).
- Sadrameli, S.M. Mathematical models for the simulation of thermal regenerators: A state-of-the-art review. Renew. Sustain. Energy Rev. 2016, 58, 462–476. [Google Scholar] [CrossRef]
- Nizovtsev, M.I.; Borodulin, V.Y.; Letushko, V.N.; Zakharov, A.A. Analysis of the efficiency of air-to-air heat exchanger with a periodic change in the flow direction. Appl. Thermal Eng. 2016, 93, 113–121. [Google Scholar] [CrossRef]
- Nizovtsev, M.I.; Borodulin, V.Y.; Letushko, V.N. Influence of condensation on the efficiency of regenerative heat exchanger for ventilation. Appl. Therm. Eng. 2017, 111, 997–1007. [Google Scholar] [CrossRef]
- Chang, C.C.; Chen, S.L.; Lin, T.Y.; Chiang, Y.C. Experimental and theoretical investigation of regenerative total heat exchanger with periodic flow for air-conditioning systems. Int. J. Refrig. 2017, 81, 123–133. [Google Scholar] [CrossRef]
- Fathieh, F.; Besant, R.W.; Evitts, R.W.; Simonson, C.J. Determination of air-to-air heat wheel sensible effectiveness using temperature step change data. Int. J. Heat Mass Transf. 2015, 87, 312–326. [Google Scholar] [CrossRef]
- Fathieh, F. A Novel Transient Testing Method for Heat/Energy Wheel Components. Ph.D. Thesis, University of Saskatchewan, Saskatoon, SK, Canada, July 2016. [Google Scholar]
- Shah, R.K.; Sekulic, D.P. Fundamentals of heat exchange design; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2003. [Google Scholar]
- Abe, O.O.; Simonson, C.J.; Besant, R.W. Effectiveness of energy wheels from transient measurements. Part 1: Prediction of effectiveness and uncertainty. Int. J. Heat Mass Transfer 2006, 49, 52–62. [Google Scholar] [CrossRef]
- Simonson, C.J.; Besant, R.W. Heat and Moisture Transfer in Desiccant Coated Rotary Energy Exchangers: Part I. Numerical Model. HVAC&R Res. 1997, 3, 325–350. [Google Scholar]
- Kays, A.L.; London, W.M. Compact Heat Exchangers; McGraw-Hill Inc: New York, USA, 1984. [Google Scholar]
- Buyukalaca, O.; Yilmaz, T. Influence of rotational speed on effectiveness of rotary-type heat exchanger. Heat Mass Transfer 2002, 38, 441–447. [Google Scholar] [CrossRef]
| Air channel | Aluminum sheet | ||||||
|---|---|---|---|---|---|---|---|
| Length (cm) | Width (mm) | Height (cm) | Hydraulic diameter (mm) | Thickness (mm) | Density (kg/m3) | Thermal Conductivity (W/m•K) | Specific heat capacity (J/kg•K) |
| 20 | 8 | 4 | 7.6 | 0.62 | 2730 | 162 | 903 |
| Test | Volumetric flowrate (L/min) | Hot inlet temperature (°C)/relative humidity (%) | Cold inlet temperature (°C)/relative humidity (%) | Face velocity (m/s) | Re | NTUo | Cr* |
|---|---|---|---|---|---|---|---|
| 1 | 230 | 41.0/7 | 24.3/12 | 0.7 | 398 | 1.65 | 1.40 |
| 2 | 530 | 41.4/4 | 24.3/13 | 1.6 | 918 | 0.77 | 0.61 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ramin, H.; Krishnan, E.; Simonson, C.J. Fixed Bed Regenerators for HVAC Applications. Proceedings 2019, 23, 4. https://doi.org/10.3390/proceedings2019023004
Ramin H, Krishnan E, Simonson CJ. Fixed Bed Regenerators for HVAC Applications. Proceedings. 2019; 23(1):4. https://doi.org/10.3390/proceedings2019023004
Chicago/Turabian StyleRamin, Hadi, Easwaran Krishnan, and Carey J. Simonson. 2019. "Fixed Bed Regenerators for HVAC Applications" Proceedings 23, no. 1: 4. https://doi.org/10.3390/proceedings2019023004
APA StyleRamin, H., Krishnan, E., & Simonson, C. J. (2019). Fixed Bed Regenerators for HVAC Applications. Proceedings, 23(1), 4. https://doi.org/10.3390/proceedings2019023004




