Optical Infrared Thermography of CFRP with Artificial Defects: Performance of Various Post-Processing Techniques †
Abstract
:1. Introduction
2. Materials and Method
2.1. Samples
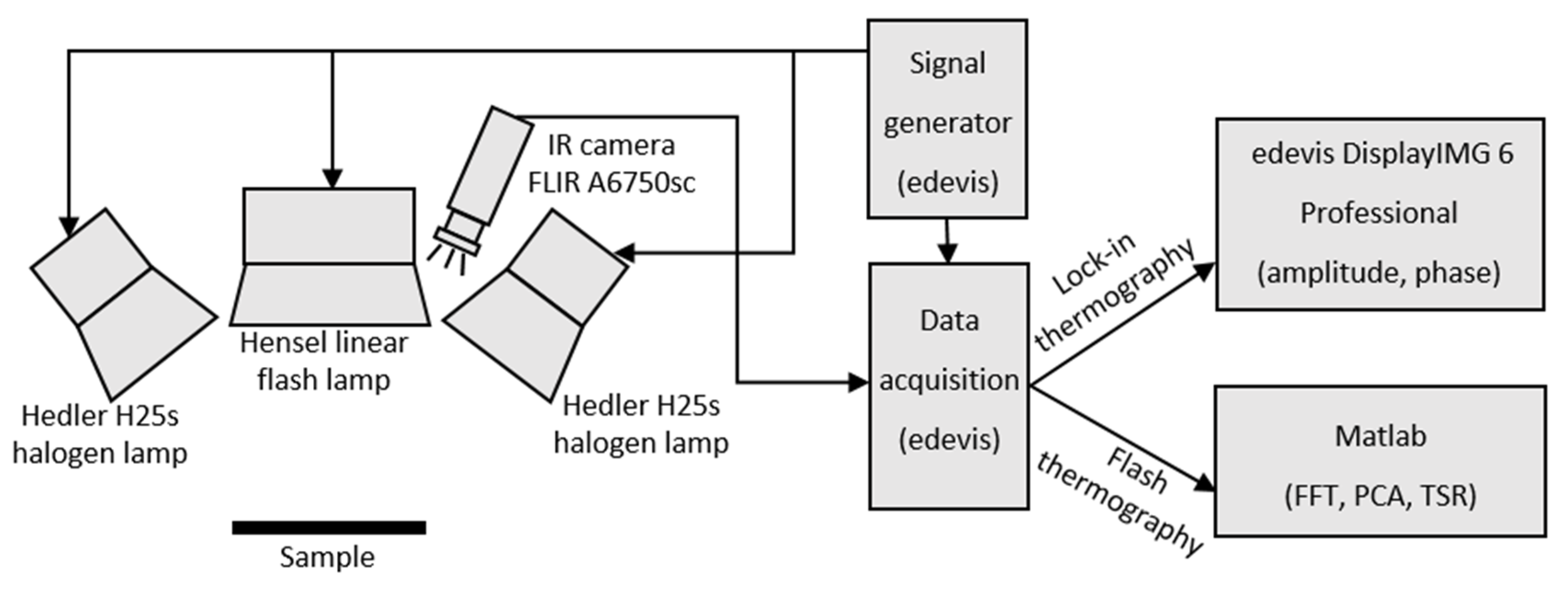
2.2. Experimental Set-Up and Procedure
3. Results
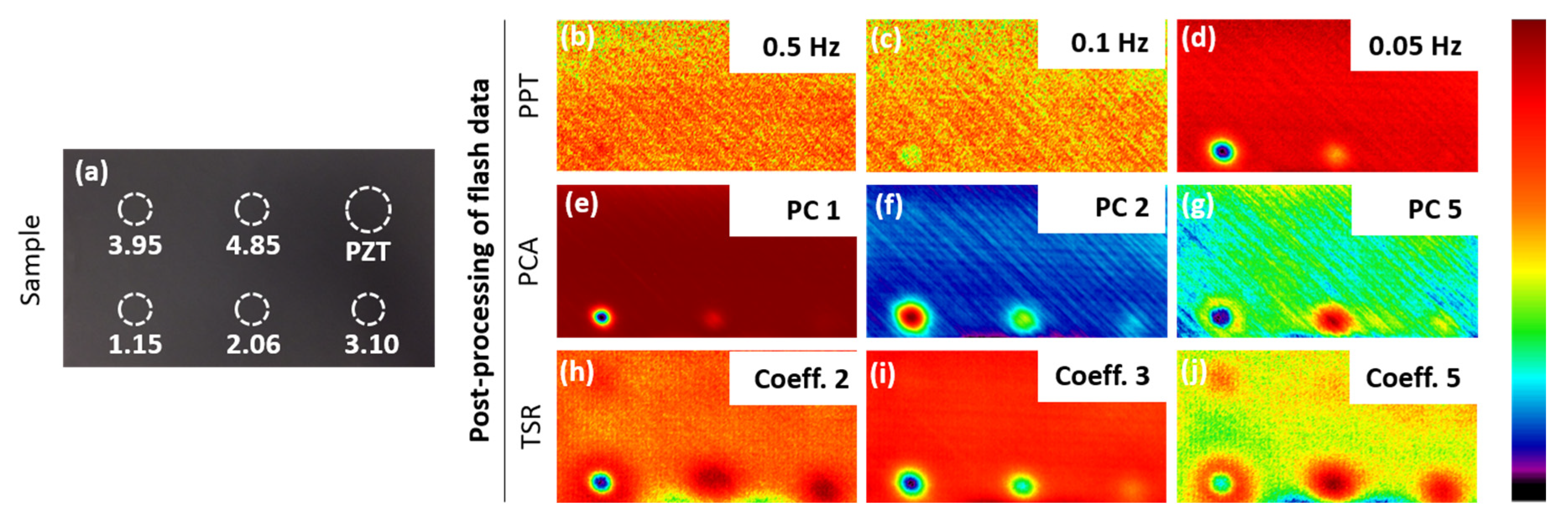
3.1. CFRP Sample with Flat Bottom Holes
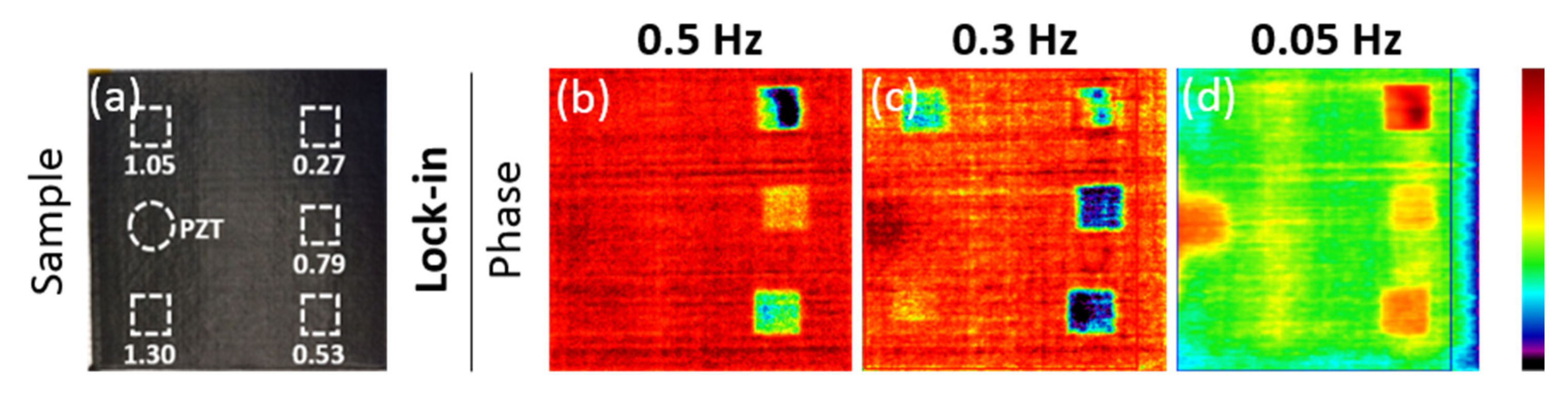
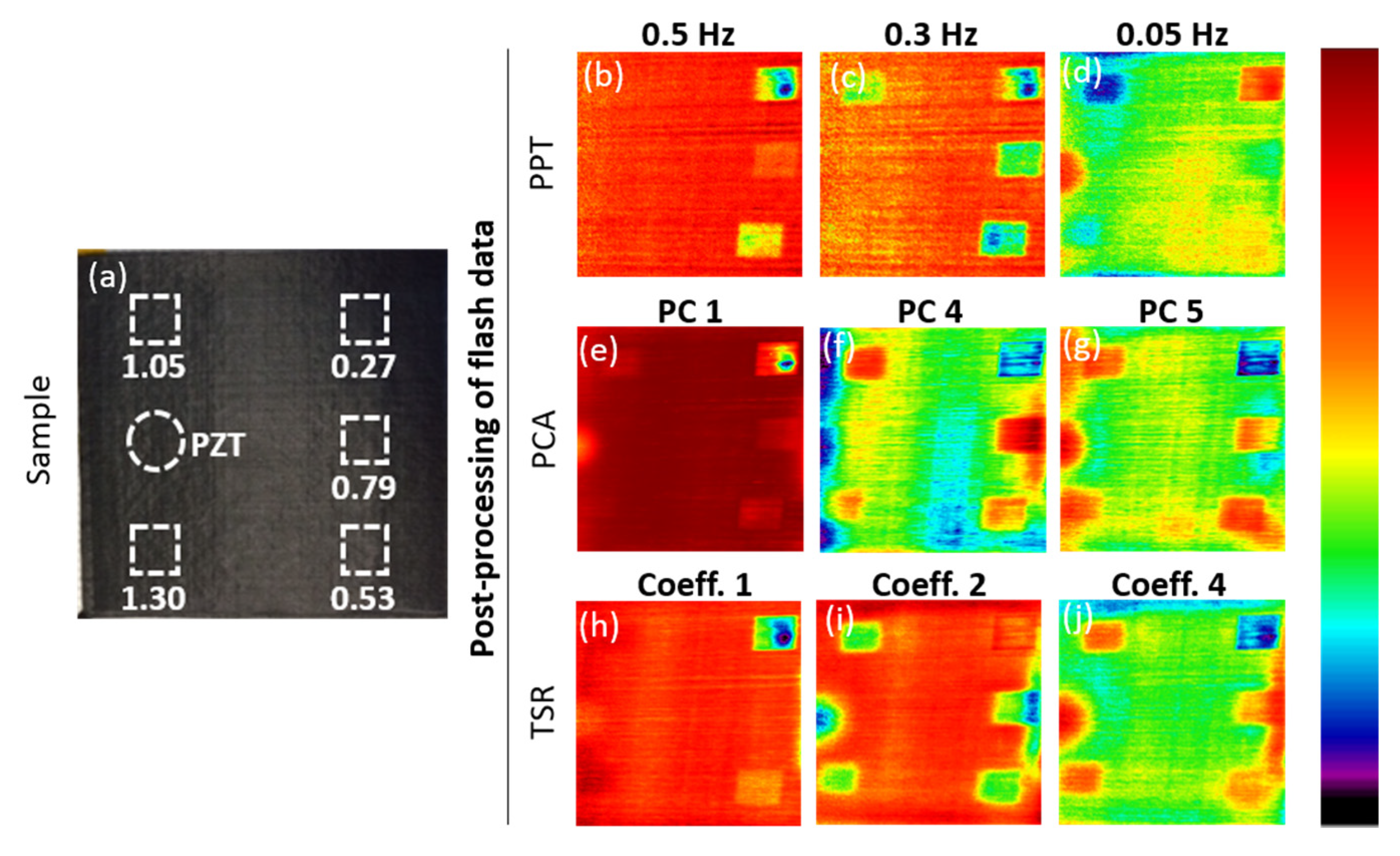
3.2. CFRP Sample with Inserts
4. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Fernandes, H.; Zhang, H.; Maldague, X. An active infrared thermography method for fiber orientation assessment of fiber-reinforced composite materials. Infrared Phys. Technol. 2015, 72, 286–292. [Google Scholar] [CrossRef]
- Beemer, M.F.; Sherpard, S.M. Aspect Ratio Considerations for Flat Bottom Hole Defects in Active Thermography. In Proceedings of the 13th Quantitative Infrared Thermography Conference (QIRT), Gdansk, Poland, 4–8 July 2016; pp. 95–100. [Google Scholar]
- López, F.; Ibarra-Castanedo, C.; Nicolau, V.P.; Maldague, X. Comparative Study of Thermographic Signal Reconstruction and Partial Least Squares Thermography for Detection and Evaluation of Subsurface Defects. In Proceedings of the 12th International Conference on Quantitative Infrared Thermography, Bordeaux, France, 7–11 July 2014. [Google Scholar]
- Rajic, N. Principal Component Thermography for Flaw Contrast Enhancement and Flaw Depth Characterisation in Composite Structures. Compos. Struct. 2002, 58, 521–528. [Google Scholar] [CrossRef]
- Maierhofer, C.; Myrach, P.; Reischel, M.; Steinfurth, H.; Röllig, M.; Kunert, M. Characterizing damage in CFRP structures using flash thermography in reflection and transmission configurations. Compos. Part B Eng. 2014, 57, 35–46. [Google Scholar] [CrossRef]
- Boccardi, S.; Carlomagno, G.M.; Meola, C.; Simeoli, G.; Acierno, D.; Iannace, S.; Sorrentino, L.; Russo, P. Lock-In Thermography for Investigation of Impact Damage in Hybrid Polypropylene/Glass Composites. IEEE A E Syst. Mag. 2016, 31, 26–30. [Google Scholar] [CrossRef]
- Usamentiaga, R.; Ibarra-Castanedo, C.; Klein, M.; Maldague, X.; Peeters, J.; Sanchez-Beato, A. Nondestructive Evaluation of Carbon Fiber Bicycle Frames Using Infrared Thermography. Sensors 2017, 17, 2679. [Google Scholar] [CrossRef] [PubMed]
- Joven, R.; Das, R.; Ahmed, A.; Roozbehjavan, P.; Minaie, B. Thermal Properties of Carbon Fiber-Epoxy Composites with Different Fabric Weaves; SAMPE: Charleston, SC, USA, 2012. [Google Scholar]
- Maldague, X.; Marinetti, S. Pulse Phase Infrared Thermography. J. Appl. Phys. 1996, 79, 2694–2698. [Google Scholar] [CrossRef]
- Shepard, S.M. Temporal Noise Reduction, Compression and Analysis of Thermographic Data Sequences; Thermal Wave Imaging, Inc.: Ferndale, MI, USA, 2003. [Google Scholar]
- Zheng, K.; Chang, Y.-S.; Yao, Y. Defect detection in CFRP structures using pulsed thermographic data enhanced by penalized least squares methods. Compos. Part B Eng. 2015, 79, 351–358. [Google Scholar] [CrossRef]
- Ibarra-Castanedo, C.; Maldague, X. Pulsed phase thermography reviewed. Quant. InfraRed Thermogr. J. 2004, 1, 47–70. [Google Scholar] [CrossRef]

| Sample 1 | Sample 2 | |
|---|---|---|
| Defect type | FBH | Insert |
| Dimensions [mm3] | 150 × 90 × 5.5 | 150 × 150 × 2.6 |
| Layup | [−45/0/45/90]3s | [(0/90)2/0]s |
| Number of defects | 5 | 5 |
| Size of defects | Ø 10 mm | 20 × 20 × 0.06 mm3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Poelman, G.; Hedayatrasa, S.; Segers, J.; Tellez, J.A.C.; Paepegem, W.V.; Kersemans, M. Optical Infrared Thermography of CFRP with Artificial Defects: Performance of Various Post-Processing Techniques. Proceedings 2018, 2, 457. https://doi.org/10.3390/ICEM18-05358
Poelman G, Hedayatrasa S, Segers J, Tellez JAC, Paepegem WV, Kersemans M. Optical Infrared Thermography of CFRP with Artificial Defects: Performance of Various Post-Processing Techniques. Proceedings. 2018; 2(8):457. https://doi.org/10.3390/ICEM18-05358
Chicago/Turabian StylePoelman, Gaétan, Saeid Hedayatrasa, Joost Segers, Javier Andres Calderon Tellez, Wim Van Paepegem, and Mathias Kersemans. 2018. "Optical Infrared Thermography of CFRP with Artificial Defects: Performance of Various Post-Processing Techniques" Proceedings 2, no. 8: 457. https://doi.org/10.3390/ICEM18-05358
APA StylePoelman, G., Hedayatrasa, S., Segers, J., Tellez, J. A. C., Paepegem, W. V., & Kersemans, M. (2018). Optical Infrared Thermography of CFRP with Artificial Defects: Performance of Various Post-Processing Techniques. Proceedings, 2(8), 457. https://doi.org/10.3390/ICEM18-05358






