Aerodynamics of Golf Balls in Still Air †
Abstract
1. Introduction
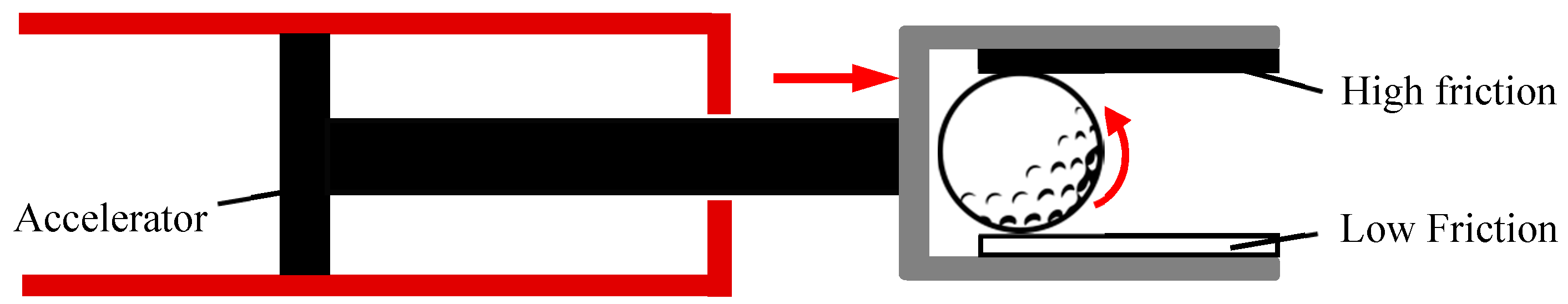
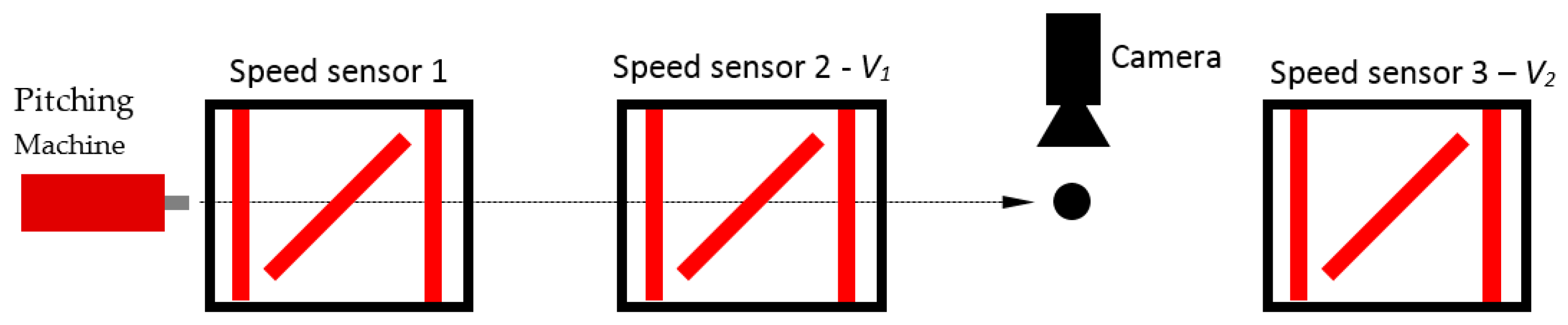
2. Method
3. Results
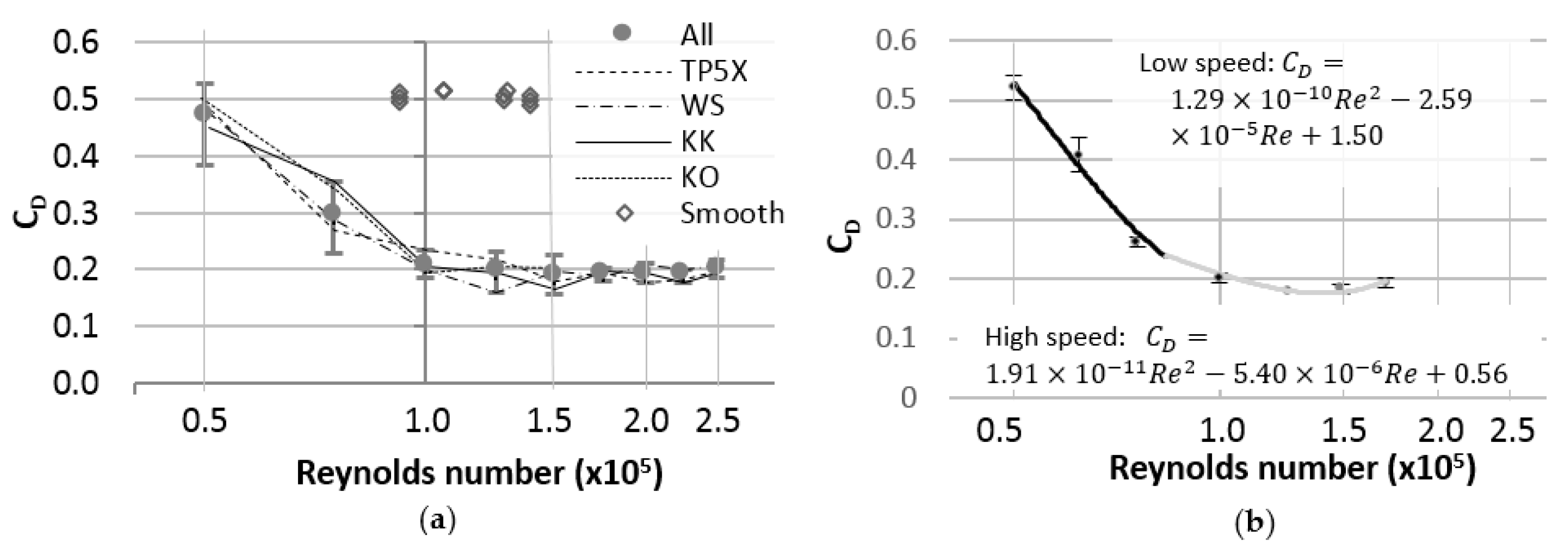
3.1. Drag
3.1.1. Drag—Non-Rotating Projectiles
3.1.2. Drag—Rotating Golf Balls
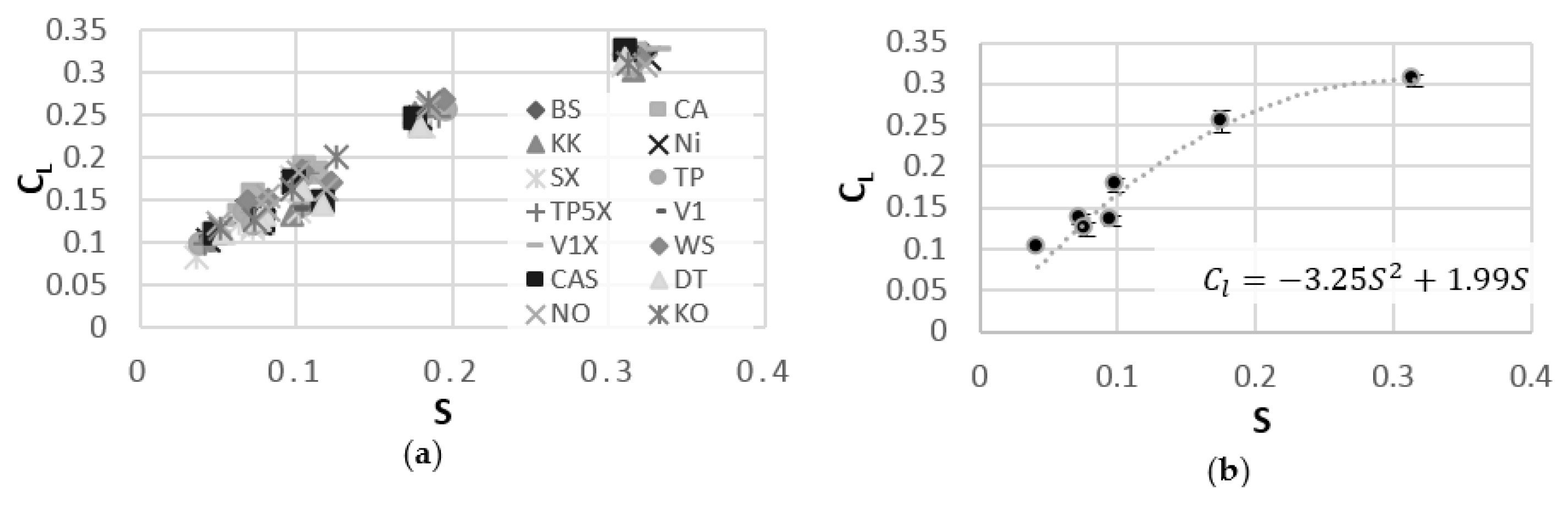
3.2. Lift—Rotating Golf Balls
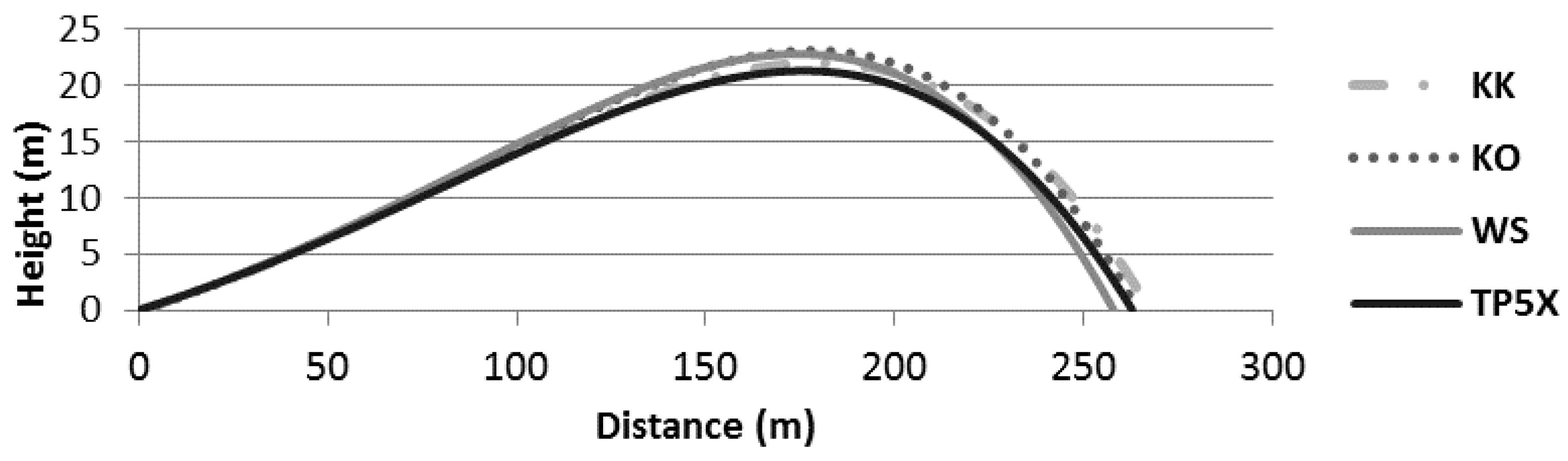
3.3. Golf Ball Trajectory Simulation
4. Conclusions
Conflicts of Interest
References
- PledgeSports. Top 10 Most Popular Sports in the World by Participation. 2017. Available online: https://www.pledgesports.org/2017/06/top-10-most-popular-sports-in-the-world-by-participation/ (accessed on 1 June 2017).
- Davies, J.M. The aerodynamics of golf balls. J. Appl. Phys. 1949, 20, 821–828. [Google Scholar] [CrossRef]
- Hernandez, M.V. USPTO Recognizes Patents on Golf-Related Inventions as Masters Tournament Opens. 2002. Available online: https://www.uspto.gov/about-us/news-updates/uspto-recognizes-patents-golf-related-inventions-masters-tournament-opens (accessed on 4 April 2002).
- Wire, G. Ball Wars: Titleist’s Parent Company Countersues Costco for Patent Infringement, False Advertising over Its Kirkland Ball. 2017. Available online: http://www.golf.com/equipment/2017/08/16/ball-wars-titleists-parent-company-countersues-costco-patent-infringement-false-advertising-over-its (accessed on 16 August 2017).
- Alam, F.; Steiner, T.; Chowdhury, H.; Moria, H.; Khan, I.; Aldawi, F.; Subic, A. A study of golf ball aerodynamic drag. Procedia Eng. 2011, 13, 226–231. [Google Scholar] [CrossRef]
- Elger, D.F.; Roberson, J.A. Engineering Fluid Mechanics; Wiley: Hoboken, NJ, USA, 2013. [Google Scholar]
- Nathan, A.M. The effect of spin on the flight of a baseball. Am. J. Phys. 2008, 76, 119–124. [Google Scholar] [CrossRef]
- Bearman, P.; Harvey, J. Golf ball aerodynamics. Aeronaut. Q. 1976, 27, 112–122. [Google Scholar] [CrossRef]
- Aoki, K.; Muto, K.; Okanaga, H. Aerodynamic characteristics and flow pattern of a golf ball with rotation. Procedia Eng. 2010, 2, 2431–2436. [Google Scholar] [CrossRef]
- Libii, J.N. Dimples and drag: Experimental demonstration of the aerodynamics of golf balls. Am. J. Phys. 2007, 75, 764–767. [Google Scholar] [CrossRef]
- Awbi, H.; Tan, S. Effect of wind-tunnel walls on the drag of a sphere. J. Fluids Eng. 1981, 103, 461–465. [Google Scholar] [CrossRef]
- Cross, R.; Lindsey, C. Measurements of drag and lift on tennis balls in flight. Sports Eng. 2014, 17, 89–96. [Google Scholar] [CrossRef]
- Lieberman, B.B.; Smith, A.J.; Quintavalla, S.J.; Thomas, F.W.; Winfield, D. Method for Determining Coefficients of Lift and Drag of a Golf Ball. US Patent US6186002 B1, 3 February 2001. [Google Scholar]
- Martin, J.; Smith, L.V.; Kensrud, J.R. Drag on sports balls using Doppler radar. Procedia Eng. 2012, 34, 134–139. [Google Scholar] [CrossRef][Green Version]
- Kensrud, J.R.; Smith, L.V. In situ drag measurements of sports balls. Procedia Eng. 2010, 2, 2437–2442. [Google Scholar] [CrossRef]
- Kensrud, J.R.; Smith, L.V. In situ lift measurement of sports balls. Procedia Eng. 2011, 13, 278–283. [Google Scholar] [CrossRef]
- United States Golf Association (USGA). The Ball: Aerodynamics Background Information. Available online: http://www.usga.org/resources/stemfiles/AERO68/aerodynamics_background_info_MS.pdf (accessed on 1 September 2017).
- Kensrud, J.R. Determining Aerodynamic Properties of Sports Balls in Situ; Washington State University: Pullman, WA, USA, 2010. [Google Scholar]
- Achenbach, E. The effects of surface roughness and tunnel blockage on the flow past spheres. J. Fluid Mech. 1974, 65, 113–125. [Google Scholar] [CrossRef]
- Bliss, K. The Average Spin Rates, Launch Angle & Ball Speed for Golf. Available online: http://www.livestrong.com/article/439185-average-spin-rates-launch-angle-ball-speed-golf/ (accessed on 11 September 2017).
- Trackman Newsletter #7. p. 7. Available online: http://www.gavlegolf.com/Media/Get/2610/trackman.pdf (accessed on 1 October 2010).

| Manufacturer | Model | Code | Cost | Manufacturer | Model | Code | Cost |
|---|---|---|---|---|---|---|---|
| Callaway | ChromeSoft | CA | Medium | Bridgestone | B330-RX | BS | Medium |
| Callaway | Supersoft | CAS | Low | Srixon | Z-Star XV | SX | Medium |
| Titleist | Pro V1x | V1X | High | Wilson | Staff Duo | WS | Low |
| Titleist | Pro V1 | V1 | High | Noodle | Long&Soft | NO | Low |
| Titleist | DT TruSoft | DT | Low | Nike | Platinum | NI | Medium |
| TaylorMade | TP5X | TP5X | High | Kirkland | 2017 | KK | Low |
| TaylorMade | TP | TP | Medium | Kirkland | 2016 | KO | Low |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lyu, B.; Kensrud, J.; Smith, L.; Tosaya, T. Aerodynamics of Golf Balls in Still Air. Proceedings 2018, 2, 238. https://doi.org/10.3390/proceedings2060238
Lyu B, Kensrud J, Smith L, Tosaya T. Aerodynamics of Golf Balls in Still Air. Proceedings. 2018; 2(6):238. https://doi.org/10.3390/proceedings2060238
Chicago/Turabian StyleLyu, Bin, Jeff Kensrud, Lloyd Smith, and Taylor Tosaya. 2018. "Aerodynamics of Golf Balls in Still Air" Proceedings 2, no. 6: 238. https://doi.org/10.3390/proceedings2060238
APA StyleLyu, B., Kensrud, J., Smith, L., & Tosaya, T. (2018). Aerodynamics of Golf Balls in Still Air. Proceedings, 2(6), 238. https://doi.org/10.3390/proceedings2060238




