An Experimental Protocol to Model Recovery of Anaerobic Work Capacity †
Abstract
:1. Introduction
2. Models of Critical Power and Anaerobic Work Capacity
3. Materials and Methods
- Exercise intensities below CP draw purely from aerobic stores and result in recharging of AWC, thus increasing the AWC balance.
- The recovery of AWC below CP is not equal to the expenditure of AWC above CP. The rate is a function of the level of power below CP and the time held at that power.
- The 3 min all-out test is robust and CP and AWC can be estimated accurately using the test.
- The power held during recovery interval is constant. The behavior of power versus time below CP is unknown, and hence the power below needs to be constant to mathematically model recovery of AWC.
- A 2 min exertion interval above CP,
- A recovery interval at different recovery powers (β) and recovery durations (trec), and
- A 3 min all-out intensity interval.
4. Discussion
5. Conclusions and Future Work
Acknowledgments
Conflicts of Interest
References
- Rose, E.A.; Parfitt, G. A quantitative analysis and qualitative explanation of the individual differences in affective responses to prescribed and self-selected exercise intensities. J. Sport Exerc. Psychol. 2007, 29, 281–309. [Google Scholar] [CrossRef] [PubMed]
- Hall, E.E.; Ekkekakis, P.; Petruzzello, S.J. The affective beneficence of vigorous exercise revisited. Br. J. Health Psychol. 2002, 7, 47–66. [Google Scholar] [CrossRef]
- Welch, A.S.; Hulley, A.; Ferguson, C.; Beauchamp, M.R. Affective responses of inactive women to a maximal incremental exercise test: A test of the dual-mode model. Psychol. Sport Exerc. 2007, 8, 401–423. [Google Scholar] [CrossRef]
- Monod, H.; Scherrer, J. The Work Capacity of a Synergic Muscular Group. Ergonomics 1965, 329–338. [Google Scholar] [CrossRef]
- Morton, R.H. The critical power and related whole-body bioenergetic models. Eur. J. Appl. Physiol. 2006, 96, 339–354. [Google Scholar] [CrossRef] [PubMed]
- Hill, A.V. The Physiological Basis of Athletic Records. Nature 1925, 116, 544–548. [Google Scholar] [CrossRef]
- Gaesser, G.A.; Carnevale, T.J.; Alan, G.; Womack, C.J. Estimation of critical power with nonlinear and linear models. Med. Sci. Sports Exerc. 1995, 27, 1430–1438. [Google Scholar] [CrossRef] [PubMed]
- Bull, A.J.; Housh, T.J.; Johnson, G.O.; Perry, S.R. Effect of mathematical modeling on the estimation of critical power. Med. Sci. Sports Exerc. 2000, 32, 526–530. [Google Scholar] [CrossRef] [PubMed]
- Bergstrom, H.C.; Housh, T.J.; Zuniga, J.M.; Traylor, D.A.; Lewis, R.W., Jr.; Camic, C.L.; Schmidt, R.J.; Johnson, G.O. Differences Among Estimates of Critical Power and Anaerobic Work Capacity Derived From Five Mathematical Models and the Three-Minute All-Out Test. J. Strength Cond. Res. 2014, 28, 592–600. [Google Scholar] [CrossRef] [PubMed]
- Vanhatalo, A.; Doust, J.H.; Burnley, M. Determination of critical power using a 3-min all-out cycling test. Med. Sci. Sports Exerc. 2007, 39, 548–555. [Google Scholar] [CrossRef]
- Johnson, T.M.; Sexton, P.J.; Placek, A.M.; Murray, S.R.; Pettitt, R.W. Reliability analysis of the 3-min all-out exercise test for cycle ergometry. Med. Sci. Sports Exerc. 2011, 43, 2375–2380. [Google Scholar] [CrossRef] [PubMed]
- Vanhatalo, A.; Doust, J.H.; Burnley, M. Robustness of a 3 min all-out cycling test to manipulations of power profile and cadence in humans. Exp. Physiol. 2008, 93, 383–390. [Google Scholar] [CrossRef]
- Morton, R.H.; Billat, L.V. The critical power model for intermittent exercise. Eur. J. Appl. Physiol. 2004, 91, 303–307. [Google Scholar] [CrossRef]
- Chidnok, W.; Dimenna, F.J.; Bailey, S.J.; Vanhatalo, A.; Morton, R.H.; Wilkerson, D.P.; Jones, A.M. Exercise tolerance in intermittent cycling: Application of the critical power concept. Med. Sci. Sports Exerc. 2012, 44, 966–976. [Google Scholar] [CrossRef] [PubMed]
- Ferguson, C.; Rossiter, H.B.; Whipp, B.J.; Cathcart, A.J.; Murgatroyd, S.R.; Ward, S.A. Effect of recovery duration from prior exhaustive exercise on the parameters of the power-duration relationship. J. Appl. Physiol. 2010, 108, 866–874. [Google Scholar] [CrossRef] [PubMed]
- Skiba, P.F.; Chidnok, W.; Vanhatalo, A.; Jones, A.M. Modeling the Expenditure and Reconstitution of Work Capacity above Critical Power. Med. Sci. Sports Exerc. 2012, 44, 1526–1532. [Google Scholar] [CrossRef] [PubMed]
- Skiba, P.F.; Clarke, D.; Vanhatalo, A.; Jones, A.M. Validation of a novel intermittent w’model for cycling using field data. Int. J. Sports Physiol. Perform. 2014, 9, 900–904. [Google Scholar] [CrossRef]
- Skiba, P.F. The Kinetics of the Work Capacity above Critical Power. Ph.D. Thesis, University of Exeter, Exeter, UK, 2014. [Google Scholar]
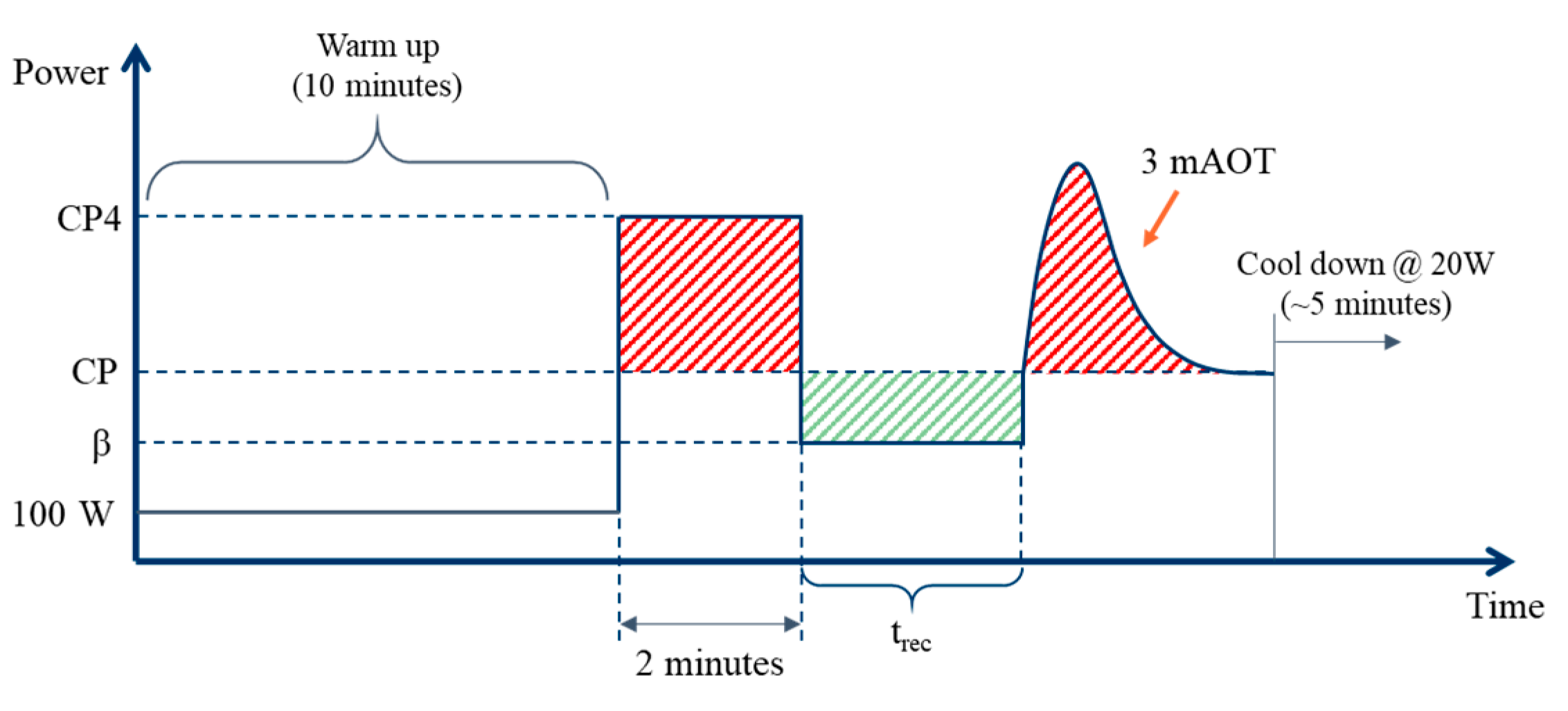
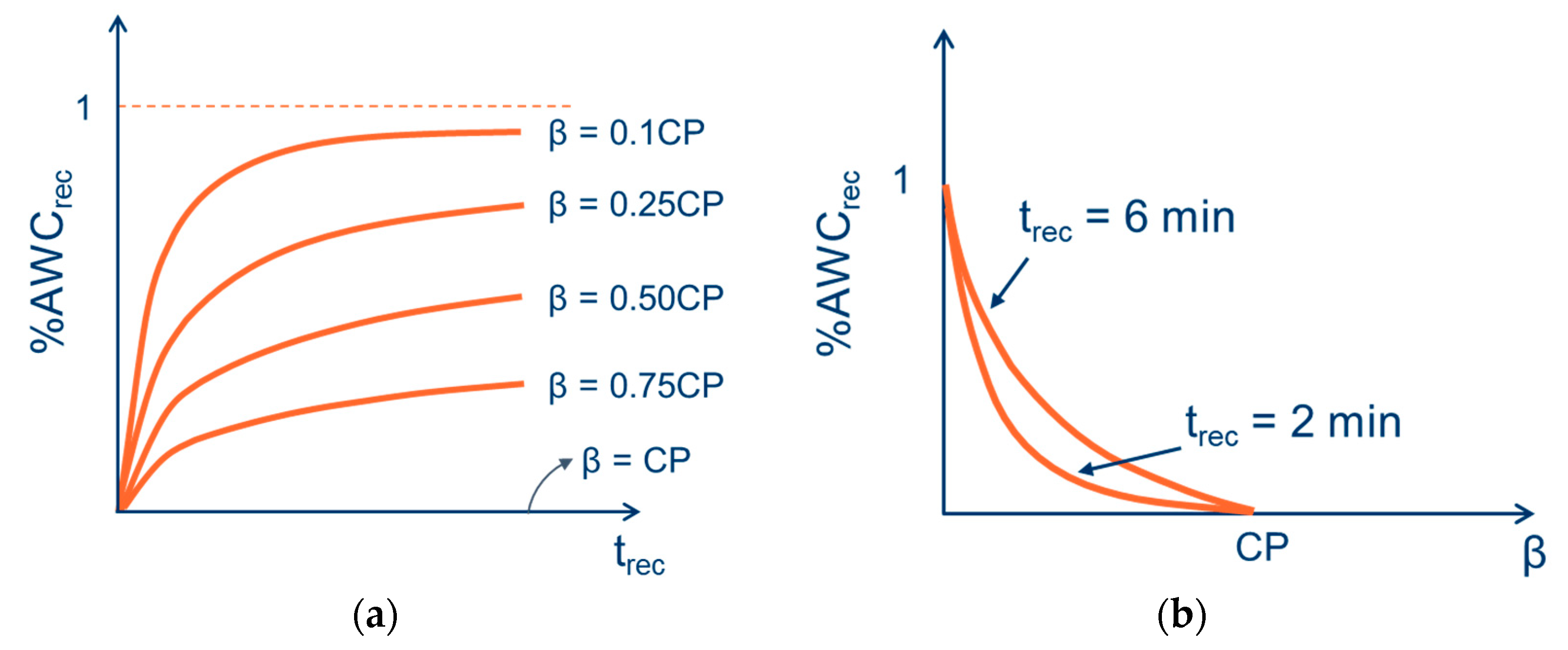
| Session | Test |
|---|---|
| 1 | ramp test, Gas Exchange Threshold (GET) and 3mAOT familiarization |
| 2 | 3mAOT to determine the subject’s CP and AWC |
| 3 | 3mAOT repeatability test and Intermittent Cycling test familiarization |
| 4 | Intermittent cycling test-1 |
| 5 | Intermittent cycling test-1 repeatability |
| 6 | Intermittent cycling test-2 |
| 7 | Intermittent cycling test-3 |
| 8 | Intermittent cycling test-4 |
| 9 | Intermittent cycling test-5 |
| 10 | Intermittent cycling test-6 |
| 11 | Intermittent cycling test-7 |
| 12 | Intermittent cycling test-8 |
| 13 | Intermittent cycling test-9 |
| Recovery Power, β (Watts) | Recovery Duration, trec (minutes) |
|---|---|
| 20 | 2 |
| 0.90 * PGET 1 | 6 |
| PGET + 0.5(CP-PGET) | 15 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sreedhara, V.S.M.; Mocko, G.M.; Hutchison, R.E. An Experimental Protocol to Model Recovery of Anaerobic Work Capacity. Proceedings 2018, 2, 208. https://doi.org/10.3390/proceedings2060208
Sreedhara VSM, Mocko GM, Hutchison RE. An Experimental Protocol to Model Recovery of Anaerobic Work Capacity. Proceedings. 2018; 2(6):208. https://doi.org/10.3390/proceedings2060208
Chicago/Turabian StyleSreedhara, Vijay Sarthy M., Gregory M. Mocko, and Randolph E. Hutchison. 2018. "An Experimental Protocol to Model Recovery of Anaerobic Work Capacity" Proceedings 2, no. 6: 208. https://doi.org/10.3390/proceedings2060208
APA StyleSreedhara, V. S. M., Mocko, G. M., & Hutchison, R. E. (2018). An Experimental Protocol to Model Recovery of Anaerobic Work Capacity. Proceedings, 2(6), 208. https://doi.org/10.3390/proceedings2060208





