Vortex Motion State of the Dry Atmosphere with Nonzero Velocity Divergence †
Abstract
:1. Introduction
2. Main Equations
3. Solution of the Main Equations
4. Conclusions
Author Contributions
Conflicts of Interest
References
- Holton, J.R. An Introduction to Dynamic Meteorology; Academic Press: San Diego, CA, USA, 2004. [Google Scholar]
- Gill, A.E. Atmosphere-Ocean Dynamics; Academic Press: San Diego, CA, USA, 1982. [Google Scholar]
- Pedlosky, J. Geophysical Fluid Dynamics; Springer: New York, NY, USA, 1987. [Google Scholar]
- Lilly, D.K. The development and maintenance of rotation in convective storms. In Intense Atmospheric Vortices; Bengtssoon, L., Lighthill, J., Eds.; Springer: Berlin, Germany, 1982. [Google Scholar]
- Kurihara, Y. Influence of environmental condition on the genesis of tropic storm. In Intense Atmospheric Vortices; Bengtssoon, L., Lighthill, J., Eds.; Springer: Berlin, Germany, 1982. [Google Scholar]
- Burgers, J.M. Application of a model system to illustrate some point of the statistical theory of free turbulence. Proc. Acad. Sci. Amst. 1940, 43, 2–12. [Google Scholar]
- Burgers, J.M. Mathematical model illustrating the theory of turbulence. Adv. Appl. Mech. 1948, 1, 197–199. [Google Scholar]
- Sullivan, R.D. A two-cell vortex solution of the Navier-Stokes equation. J. Aerosp. Sci. 1959, 26, 767–768. [Google Scholar] [CrossRef]
- Liu, S.D.; Xin, G.J.; Liu, S.K.; Liang, F.M. The 3D spiral structure pattern in the atmosphere. Adv. Atmos. Sci. 2000, 17, 519–524. [Google Scholar]
- Liu, S.D.; Liu, S.K.; Fu, Z.T.; Xin, G.J.; Liang, F.M. From 2D Geostrophic Wind to 3D Vortex Motions. Chin. J. Geophys. 2003, 46, 649–656. [Google Scholar] [CrossRef]

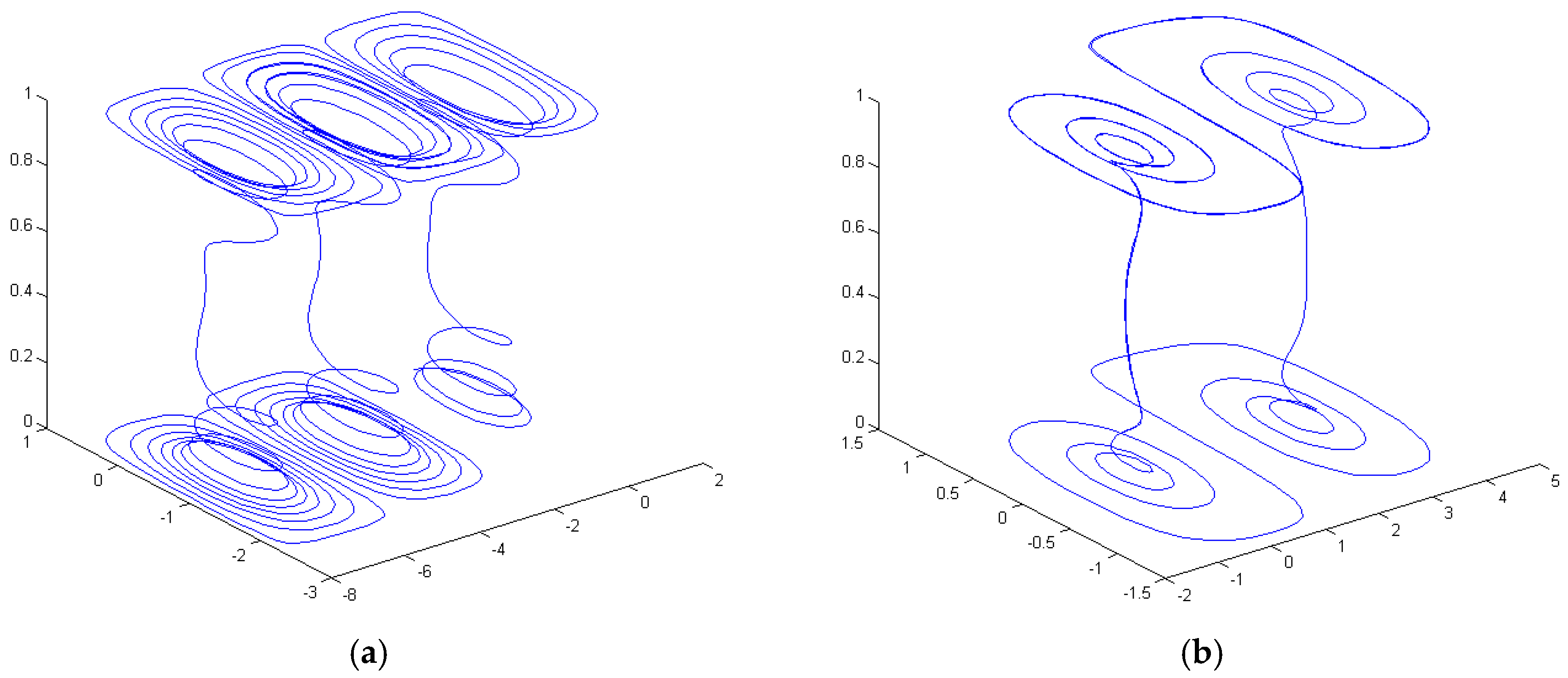
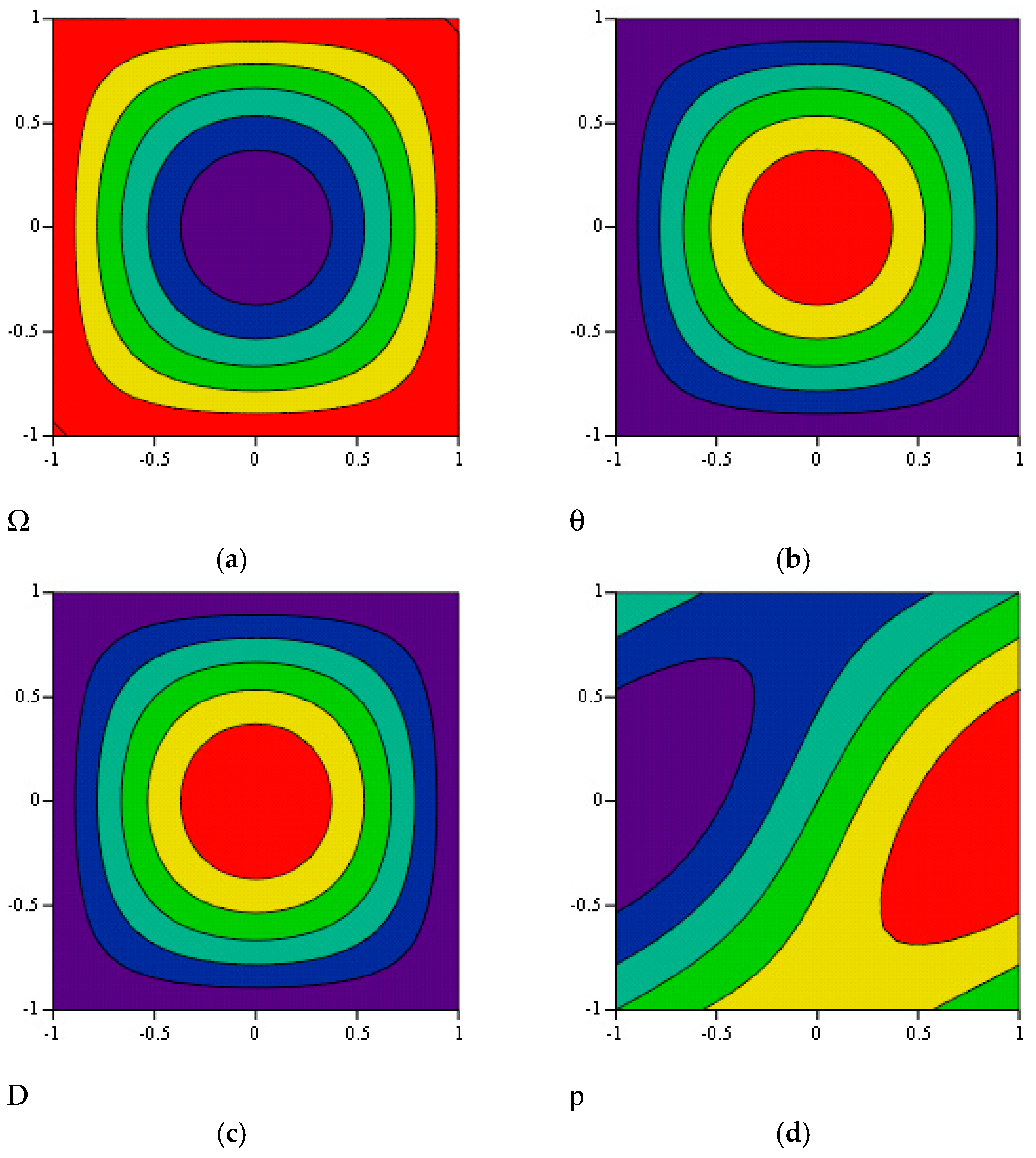
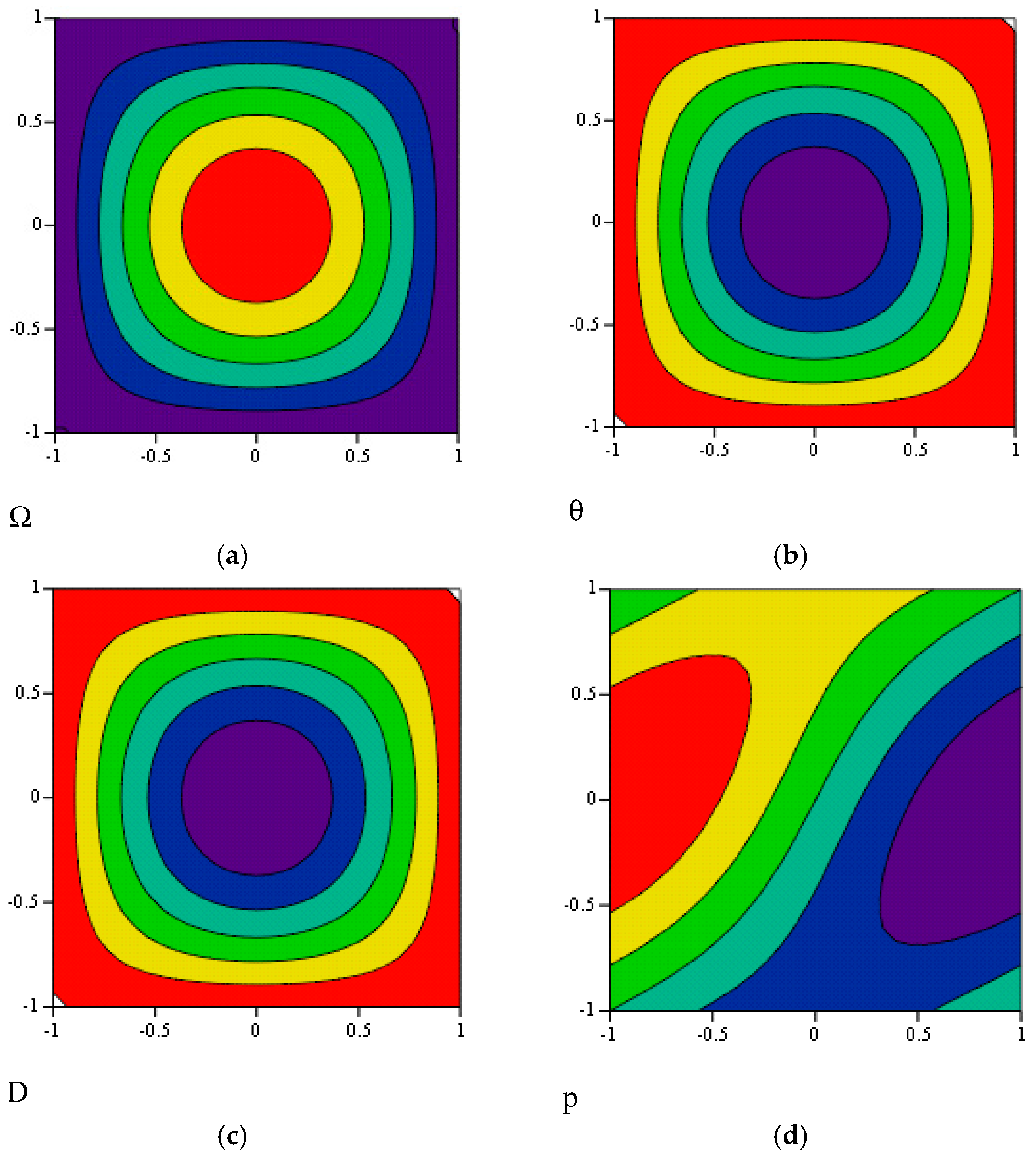
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zakinyan, R.; Zakinyan, A.; Ryzhkov, R.; Semenova, J. Vortex Motion State of the Dry Atmosphere with Nonzero Velocity Divergence. Proceedings 2017, 1, 80. https://doi.org/10.3390/ecas2017-04125
Zakinyan R, Zakinyan A, Ryzhkov R, Semenova J. Vortex Motion State of the Dry Atmosphere with Nonzero Velocity Divergence. Proceedings. 2017; 1(5):80. https://doi.org/10.3390/ecas2017-04125
Chicago/Turabian StyleZakinyan, Robert, Arthur Zakinyan, Roman Ryzhkov, and Julia Semenova. 2017. "Vortex Motion State of the Dry Atmosphere with Nonzero Velocity Divergence" Proceedings 1, no. 5: 80. https://doi.org/10.3390/ecas2017-04125
APA StyleZakinyan, R., Zakinyan, A., Ryzhkov, R., & Semenova, J. (2017). Vortex Motion State of the Dry Atmosphere with Nonzero Velocity Divergence. Proceedings, 1(5), 80. https://doi.org/10.3390/ecas2017-04125




