Uniformity Index as a Universal Air-Cooled Condenser Fan Performance Metric
Abstract
1. Introduction
2. Materials and Methods
2.1. Numerical Model
2.2. Numerical Evaluation Metrics
2.3. Experimental Measurements and Evaluation Metrics
3. Results
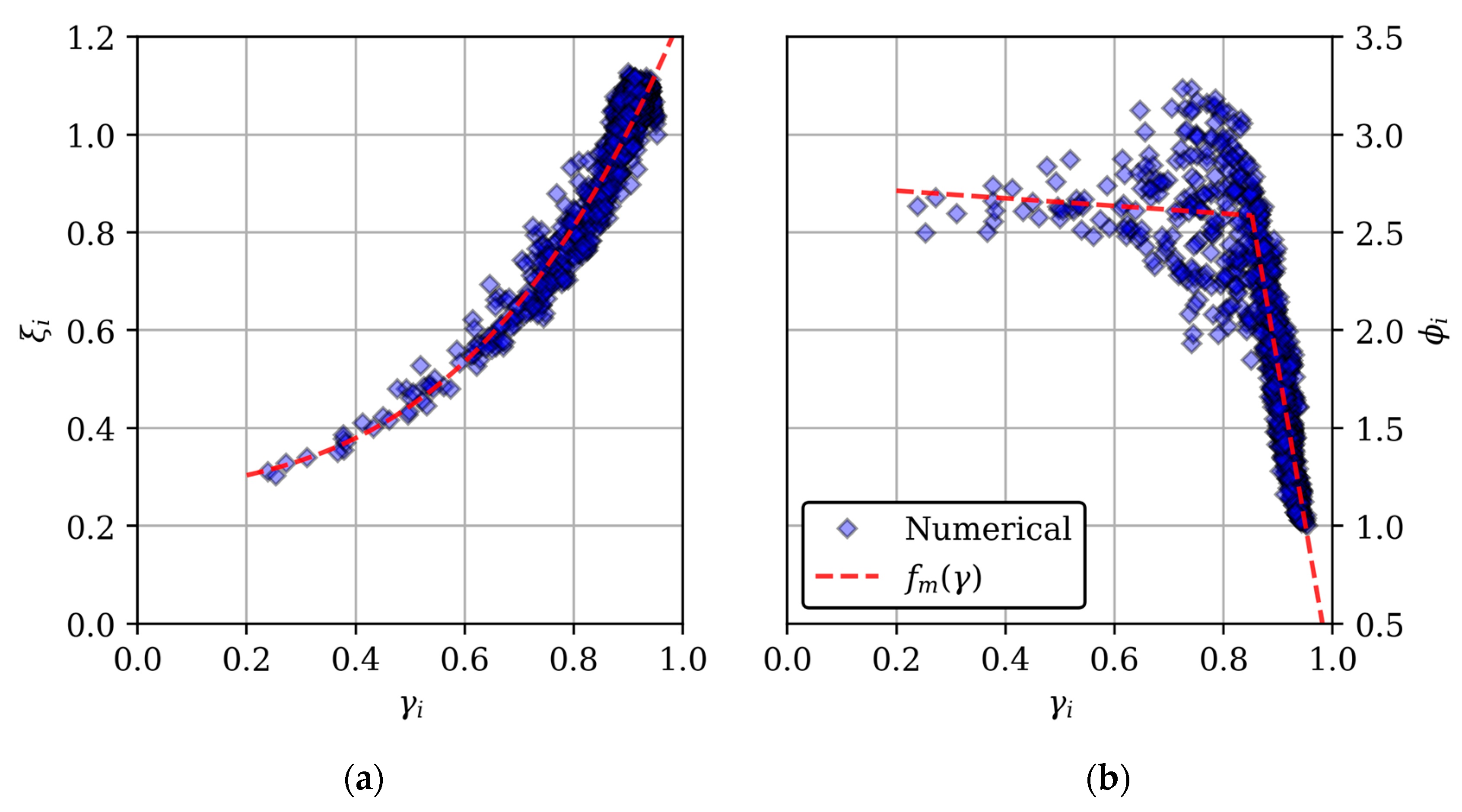
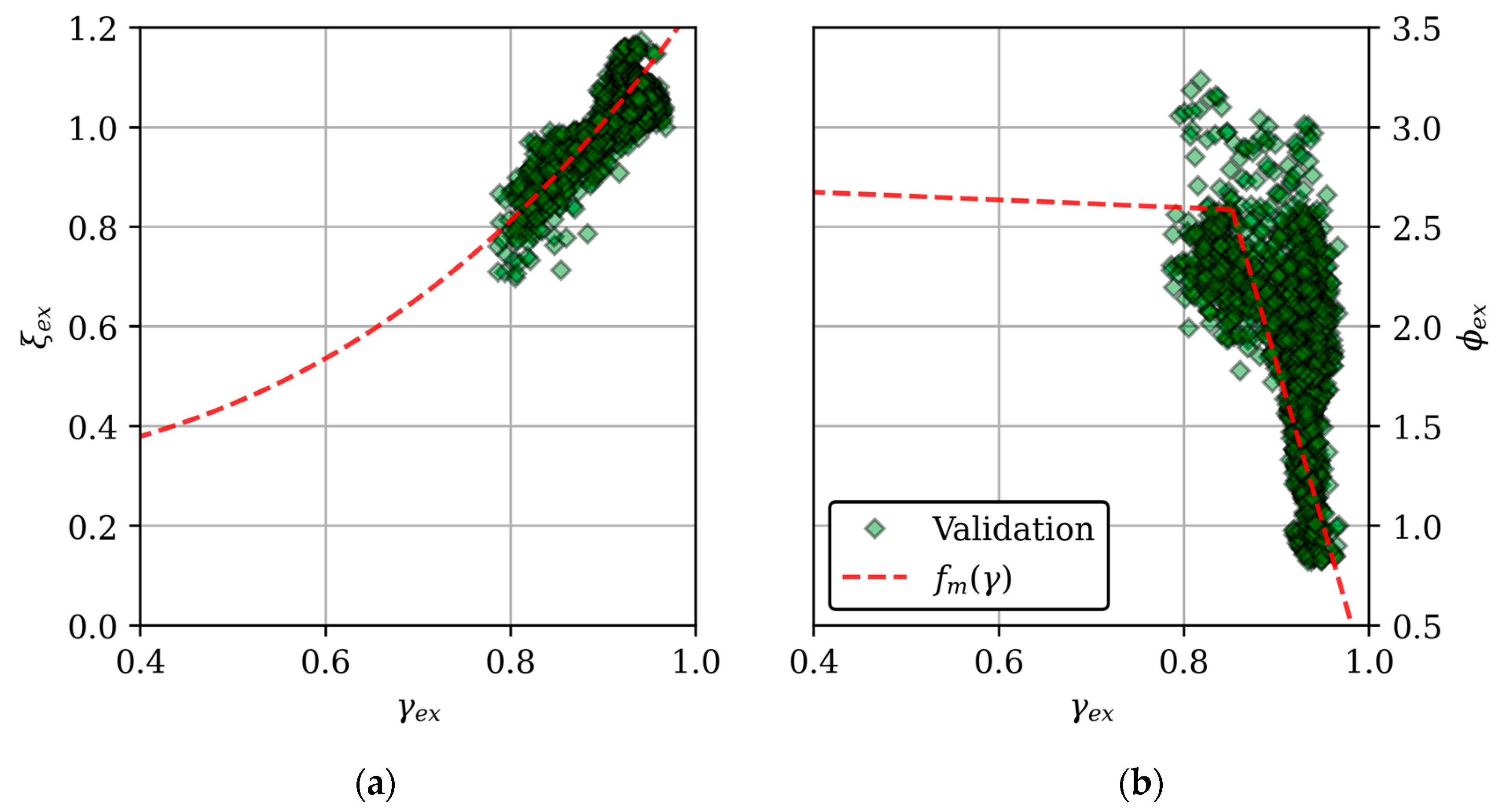
3.1. The Effect of Uniformity Index on Fan Performance and Dynamic Blade Loading
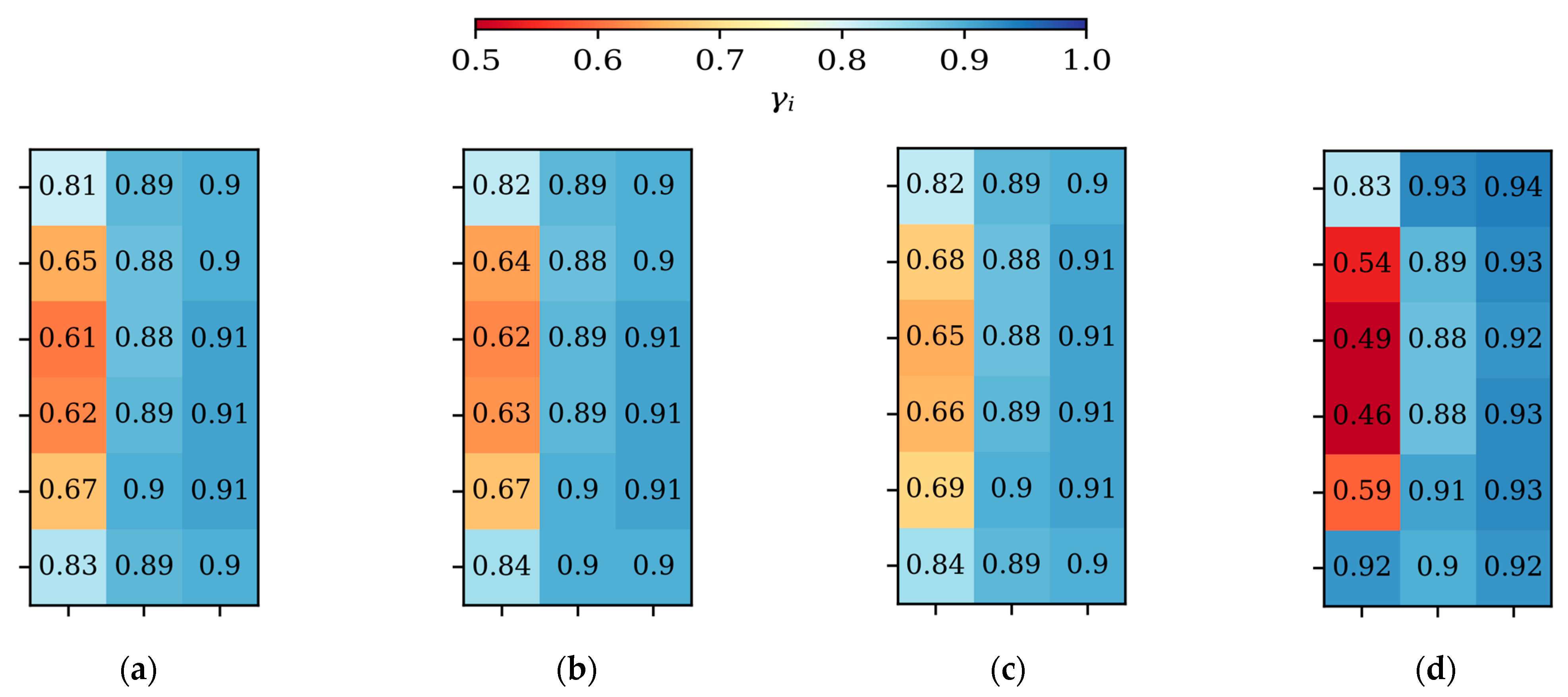
3.2. Sensitivity of Uniformity Index to Sampling Resolution
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Maulbetsch, J.; DiFilippo, M. Effect of Wind Speed and Direction on the Performance of Air-Cooled Condensers; California Energy Commission: Sacramento, CA, USA, 2008; Publication Number CEC-5002013-065-APB. [Google Scholar]
- Maulbetsch, J.; DiFilippo, M. The Use of Wind Barriers to Mitigate the Effect of Wind on Air-Cooled Condensers; California Energy Commission: Sacramento, CA, USA, 2016; Publication number: CEC-500-2016-047. [Google Scholar]
- Bredell, J.R.; Kröger, D.G.; Thiart, G.D. Numerical investigation of fan performance in a forced draft air-cooled steam condenser. Appl. Therm. Eng. 2006, 26, 846–852. [Google Scholar] [CrossRef]
- Thiart, G.D.; Von Backström, T.W. Numerical simulation of the flow field near an axial flow fan operating under distorted inflow conditions. J. Wind Eng. Ind. Aerodyn. 1993, 45, 189–214. [Google Scholar] [CrossRef]
- Meyer, C.J. Numerical investigation of the effect of inlet flow distortions on forced draught air-cooled heat exchanger performance. Appl. Therm. Eng. 2005, 25, 1634–1649. [Google Scholar] [CrossRef]
- Stinnes, W.H.; Von Backström, T.W. Effect of cross-flow on the performance of air-cooled heat exchanger fans. Appl. Therm. Eng. 2002, 22, 1403–1415. [Google Scholar] [CrossRef]
- Hotchkiss, P.J.; Meyer, C.J.; Von Backström, T.W. Numerical investigation into the effect of cross-flow on the performance of axial flow fans in forced draught air-cooled heat exchangers. Appl. Therm. Eng. 2006, 26, 200–208. [Google Scholar] [CrossRef]
- Marincowitz, F.S. Experimental Investigation of the Effects of Windscreens on Air-Cooled Condenser Fan Performance and Dynamic Blade Loading. Master’s Thesis, University of Stellenbosch, Stellenbosch, South Africa, 2019. [Google Scholar]
- Muiyser, J.; Els, D.N.J.; Van der Spuy, S.J.; Zapke, A. Measurement of air flow and blade loading at a large-scale cooling system fan. J. S. Afr. Inst. Mech. Eng. 2014, 30, 30–38. [Google Scholar]
- Yang, L.J.; Du, X.Z.; Yang, Y.P. Wind effect on the thermo-flow performances and its decay characteristics for air-cooled condensers in a power plant. Int. J. Therm. Sci. 2012, 53, 175–187. [Google Scholar] [CrossRef]
- Marincowitz, F.S.; Owen, M.T.F.; Muiyser, J. The Effect of Windscreens and Walkways on Air-cooled Condenser Performance and Fan Blade Dynamic Loading. J. Eng. Gas Turbines Power 2021, 143, 101019. [Google Scholar] [CrossRef]
- Cooling Tower Institute. CTI Standard PTG-143: Recommended Practice For Airflow Testing of Cooling Towers; Cooling Tower Institute: Houston, TX, USA, 1994. [Google Scholar]
- Bredell, J.R.; Kröger, D.G.; Thiart, G.D. Numerical investigation into aerodynamic blade loading in large axial flow fans operating under distorted inflow conditions. J. S. Afr. Inst. Mech. Eng. 2006, 22, 12. [Google Scholar]
- Bianchini, C.; Mirsky, G.; Frumkin, M. Windscreen effect on performance and structure, before and after, and comparison with CFD. In Proceedings of the ACC User Group, Colorado Springs, CO, USA, 8–11 October 2018. [Google Scholar]
- Venter, A.J. Numerical Analysis of Windscreen Effects on Air-Cooled Condenser Fan Performance and Blade Loading. Master’s Thesis, University of Stellenbosch, Stellenbosch, South Africa, 2020. [Google Scholar]
- Engelbrecht, R.A.; Meyer, C.J.; Van der Spuy, S.J. Modeling Strategy for the Analysis of Forced Draft Air-Cooled Condensers Using Rotational Fan Models. J. Therm. Sci. Eng. Appl. 2019, 11, 051011. [Google Scholar] [CrossRef]
- Venter, A.J.; Owen, M.T.F.; Muiyser, J. A numerical analysis of windscreen effects on air-cooled condenser fan performance. Appl. Therm. Eng. 2021, 186, 116416. [Google Scholar] [CrossRef]
- Marincowitz, F.S. Optimisation towards a Wind-Resistant Air-Cooled Condenser for the Modern Energy Sector. Ph.D. Thesis, University of Stellenbosch, Stellenbosch, South Africa, 2021. [Google Scholar]
- Franke, J.; Hirsch, C.; Jensen, G.; Krüs, G.W.; Miles, S.D.; Schatzmann, M.; Westbury, P.S.; Wisse, J.A.; Wright, N. Recommendations on the use of CFD in wind engineering. In Proceedings of the International Conference on Urban Wind Engineering and Buildings Aerodynamics, Sint-Genesius-Rode, Belgium, 5–7 May 2004. [Google Scholar]
- Richards, P.J.; Hoxey, R.P. Appropriate boundary conditions for computational wind engineering models using the k-ε turbulence model. J. Wind Eng. Ind. Aerodyn. 1993, 46–47, 145–153. [Google Scholar] [CrossRef]
- Kröger, D.G. Air-Cooled Heat Exchangers and Cooling Towers: Thermal-Flow Performance Evaluation and Design; PennWell Corporation: Tulsa, OK, USA, 2004. [Google Scholar]
- Kays, W.M.; London, A.L. Compact Heat Exchangers, 3rd ed.; McGraw-Hill: New York, NY, USA, 1984. [Google Scholar]
- Van der Spuy, S.J.; Von Backström, T.W. An evaluation of simplified CFD models applied to perimeter fans in air-cooled steam condensers. Proc. Inst. Mech. Eng. Part A J. Power Energy 2015, 229, 948–967. [Google Scholar] [CrossRef]
- ANSYS Inc. ANSYS Fluent Theory Guide; Release 15.0; ANSYS Inc.: Canonsburg, PA, USA, 2013. [Google Scholar]
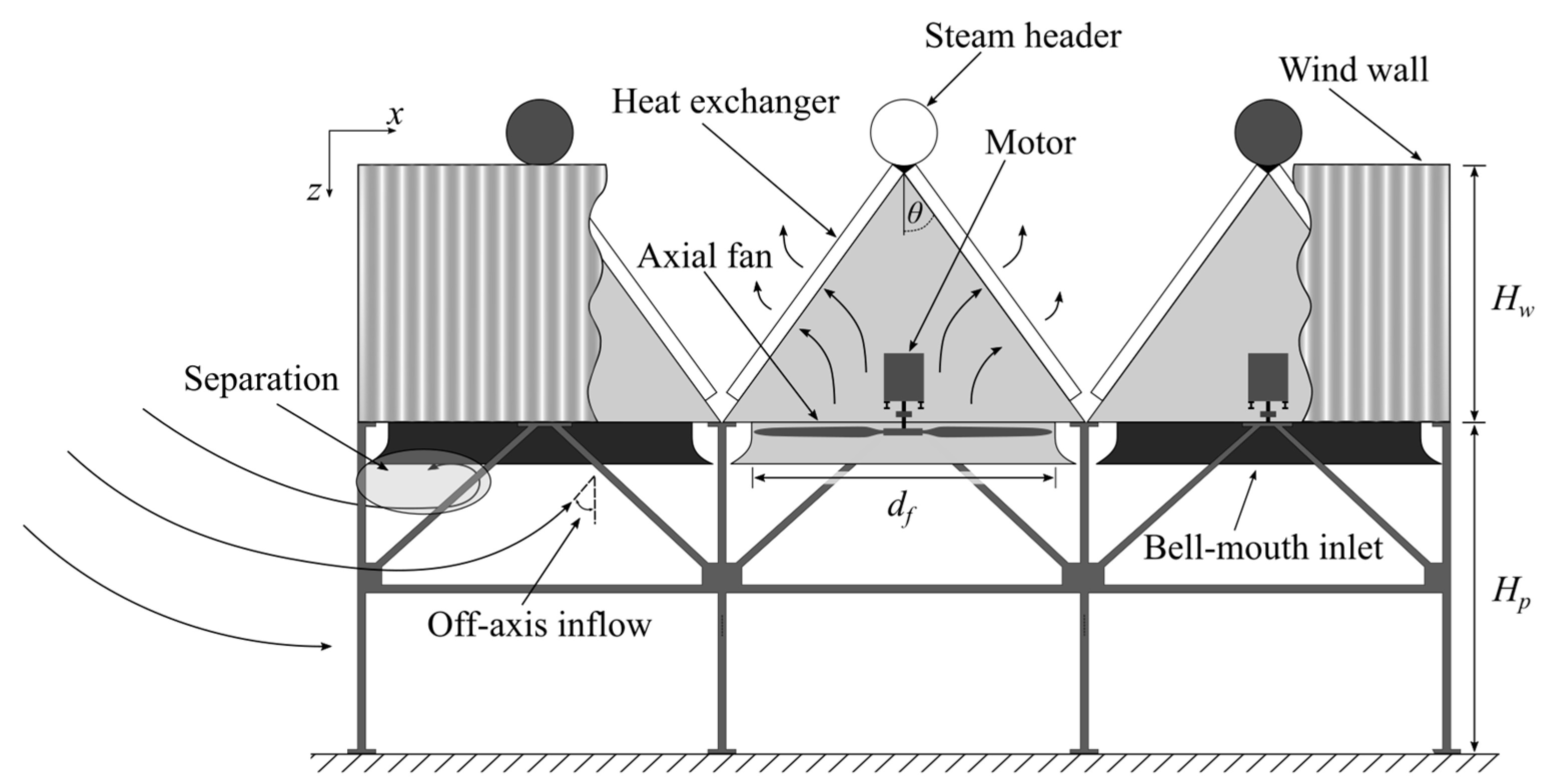
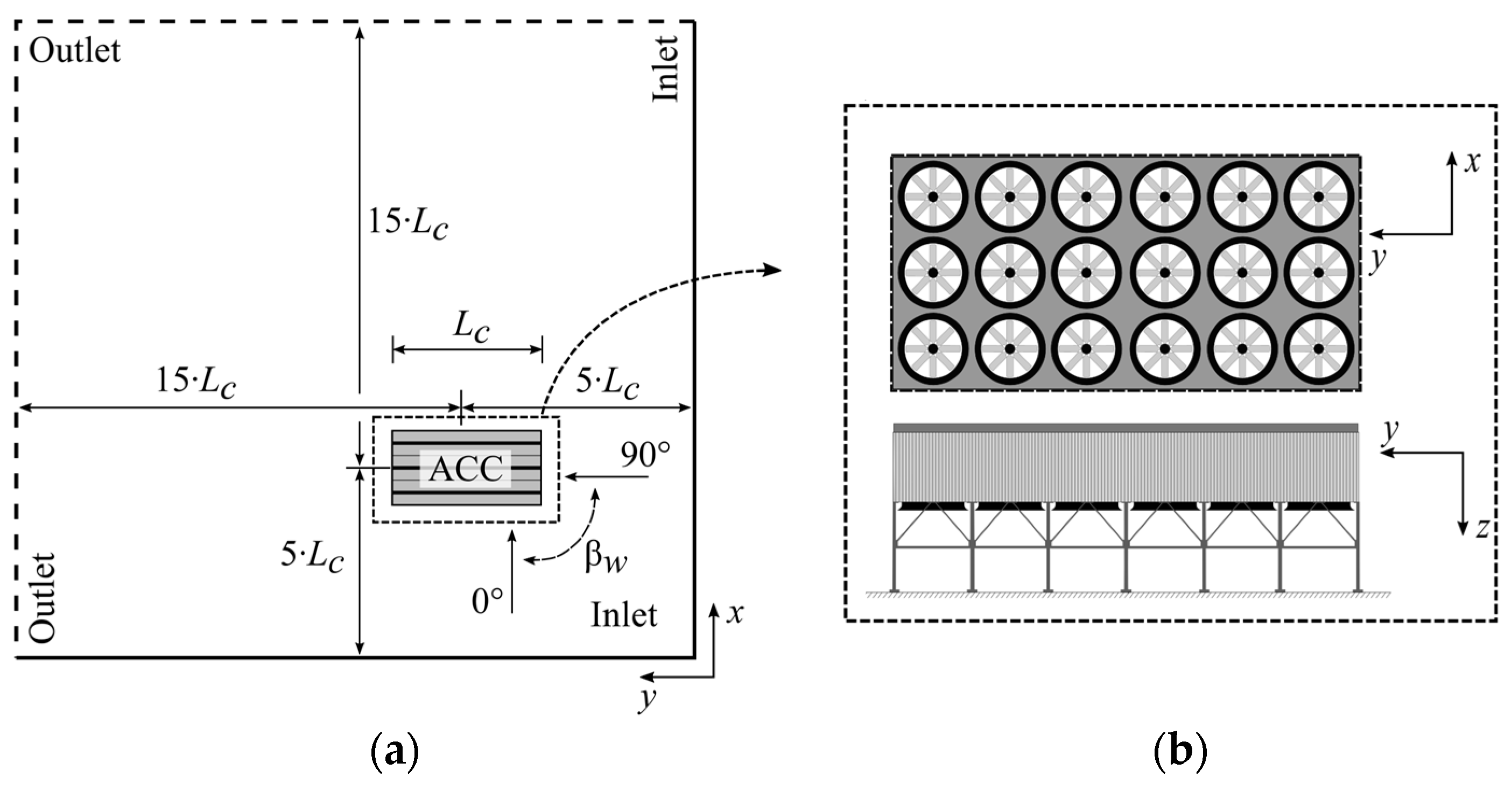
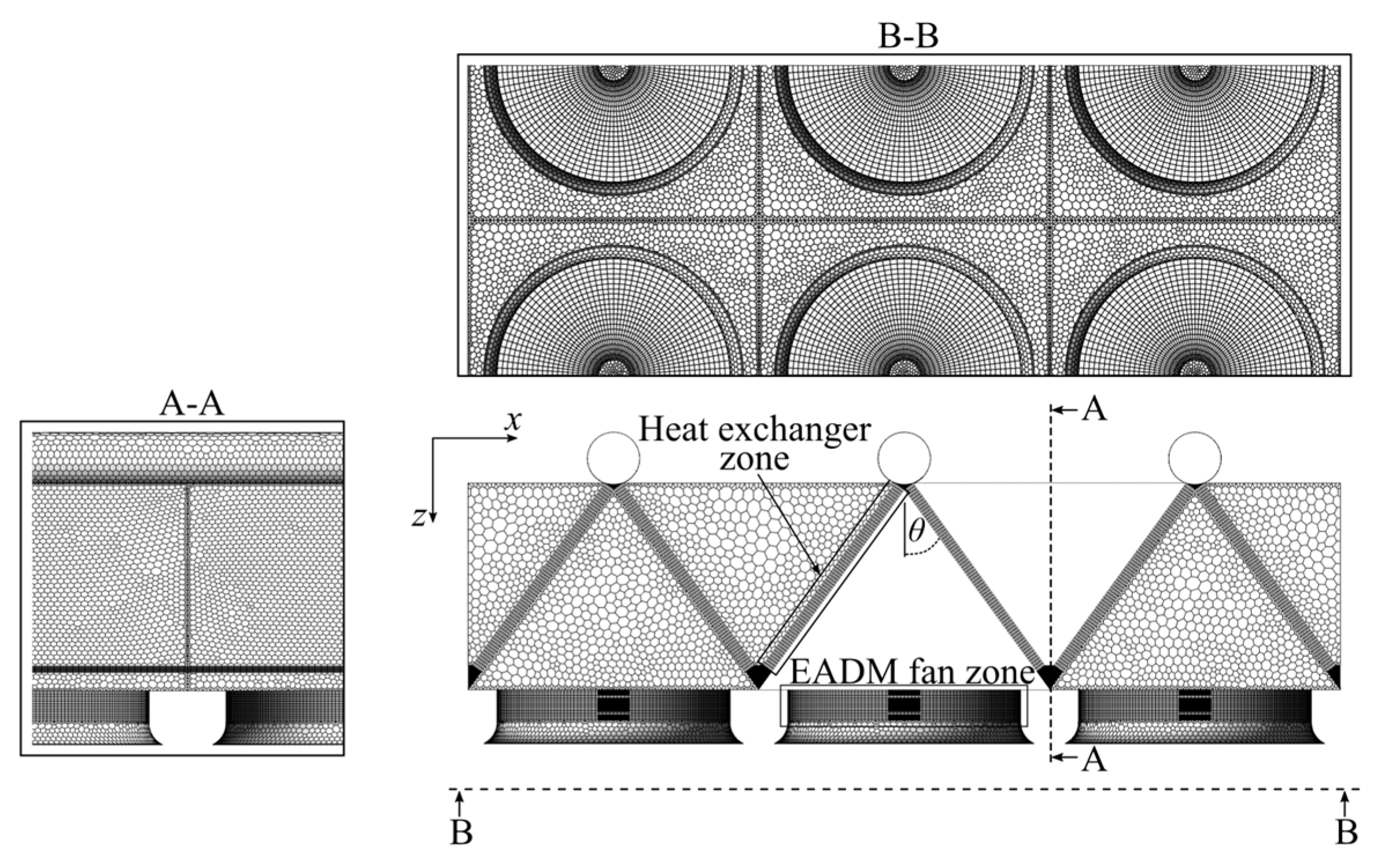

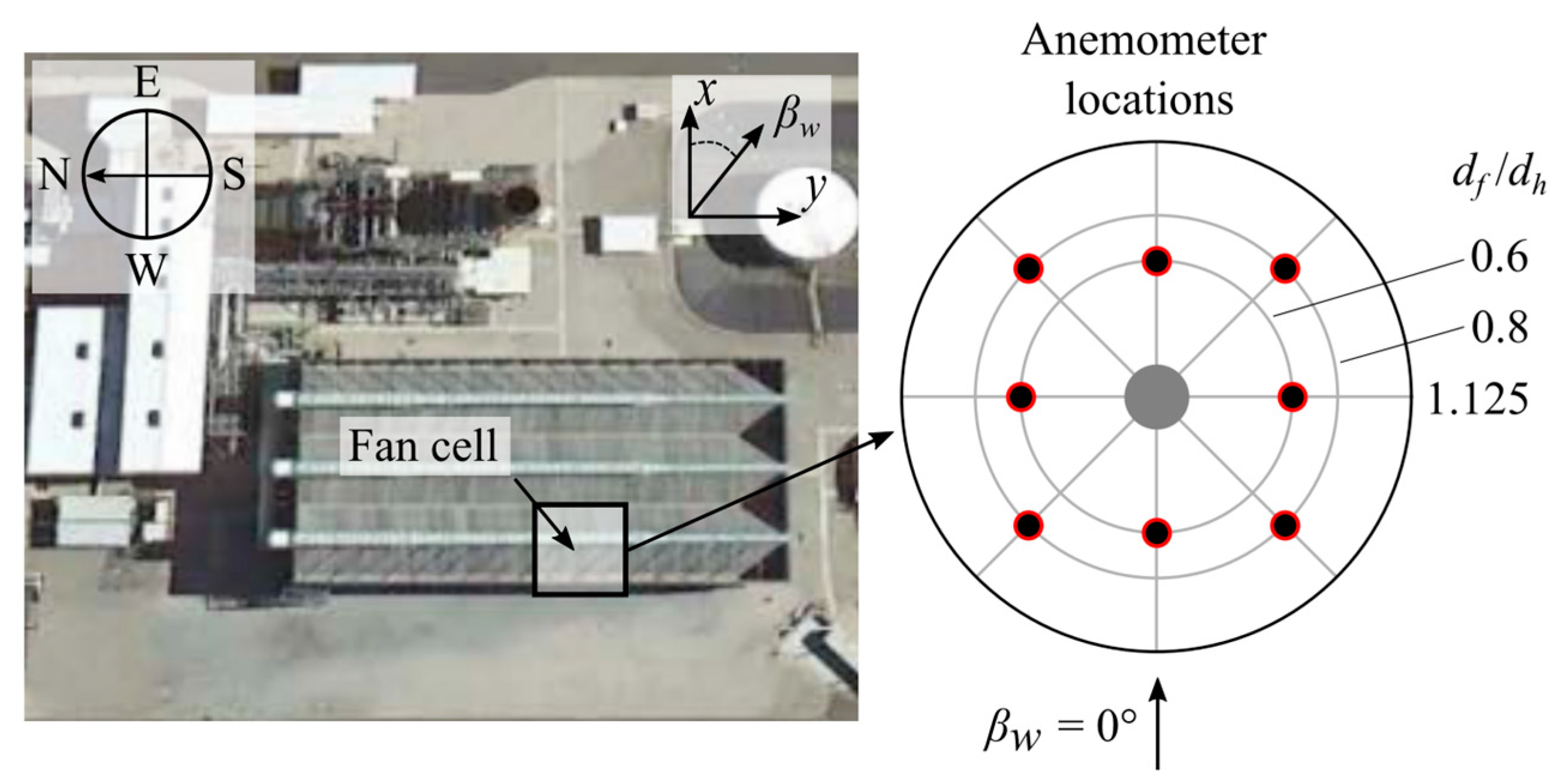


| Parameter Name | Symbol | Value |
|---|---|---|
| Tube length | Lt | 10 m |
| Number of tubes (row 1) | nt1 | 228 |
| Number of tubes (row 2) | nt2 | 232 |
| Inner tube height | Ht | 0.097 m |
| Inner tube width | Wt | 0.017 m |
| Half apex angle | θ | 36° |
| Heat exchanger frontal area | Afr | 137.7 m2 |
| Parameter Name | Symbol | Value |
|---|---|---|
| Fan diameter | df | 10.363 m |
| Hub diameter | dh | 1.4 m |
| Number of blades | nb | 9 |
| Rotational speed | N | 93.2 rpm |
| Design flow rate 1 | 576.54 m3/s | |
| Design pressure rise 1 | ∆p | 119.8 Pa |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY-NC-ND) license (https://creativecommons.org/licenses/by-nc-nd/4.0/).
Share and Cite
Marincowitz, F.; Owen, M.; Muiyser, J.; Holkers, P. Uniformity Index as a Universal Air-Cooled Condenser Fan Performance Metric. Int. J. Turbomach. Propuls. Power 2022, 7, 35. https://doi.org/10.3390/ijtpp7040035
Marincowitz F, Owen M, Muiyser J, Holkers P. Uniformity Index as a Universal Air-Cooled Condenser Fan Performance Metric. International Journal of Turbomachinery, Propulsion and Power. 2022; 7(4):35. https://doi.org/10.3390/ijtpp7040035
Chicago/Turabian StyleMarincowitz, Fredrik, Michael Owen, Jacques Muiyser, and Peter Holkers. 2022. "Uniformity Index as a Universal Air-Cooled Condenser Fan Performance Metric" International Journal of Turbomachinery, Propulsion and Power 7, no. 4: 35. https://doi.org/10.3390/ijtpp7040035
APA StyleMarincowitz, F., Owen, M., Muiyser, J., & Holkers, P. (2022). Uniformity Index as a Universal Air-Cooled Condenser Fan Performance Metric. International Journal of Turbomachinery, Propulsion and Power, 7(4), 35. https://doi.org/10.3390/ijtpp7040035







