Uncovering the Root Causes of Stall Flutter in a Wide Chord Fan Blisk
Abstract
1. Introduction
2. AECC CAE Wide Chord Fan Rig
3. Numerical Simulations and Discussions
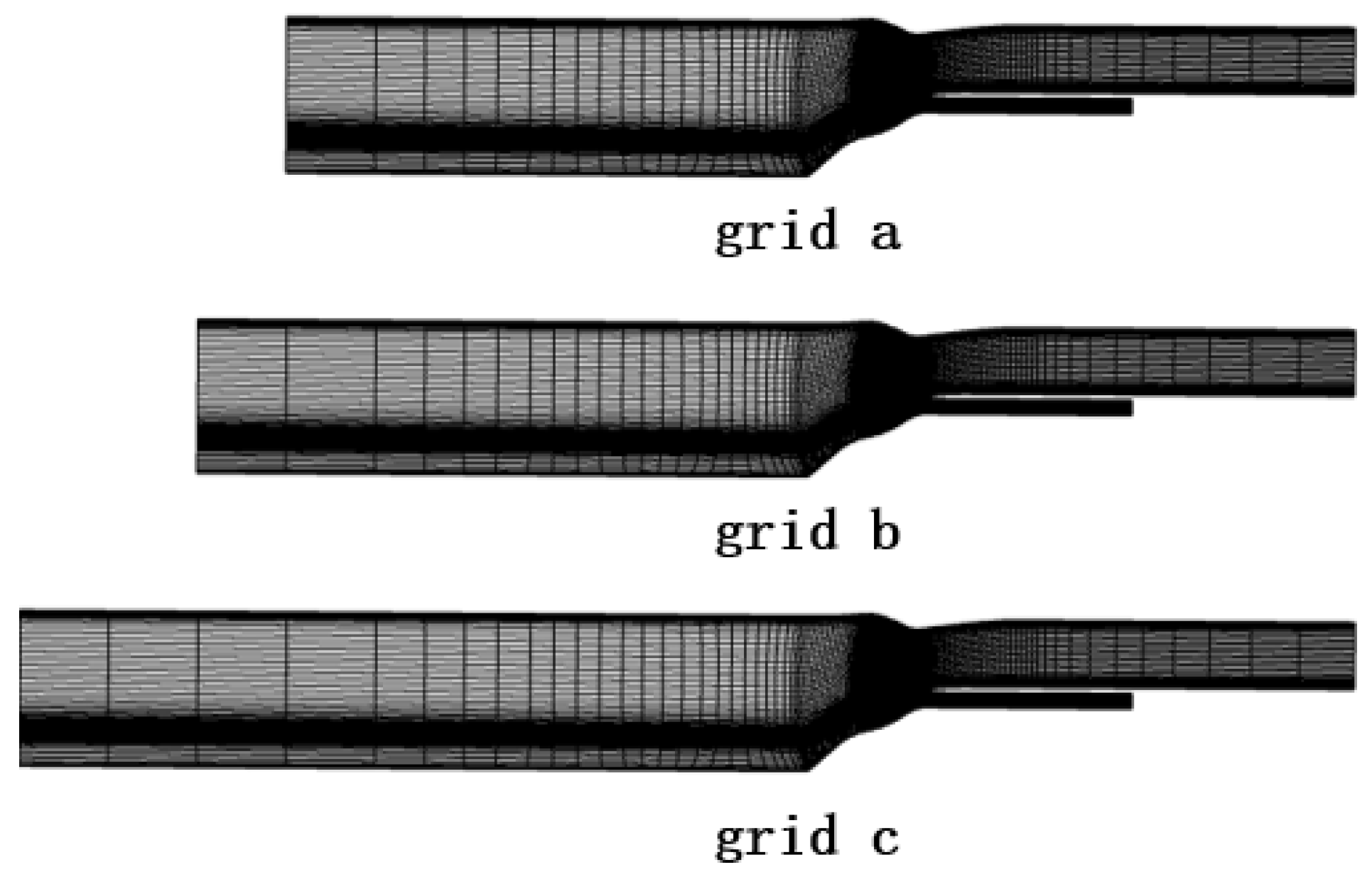
3.1. CFD Mesh with Inflation Treatment
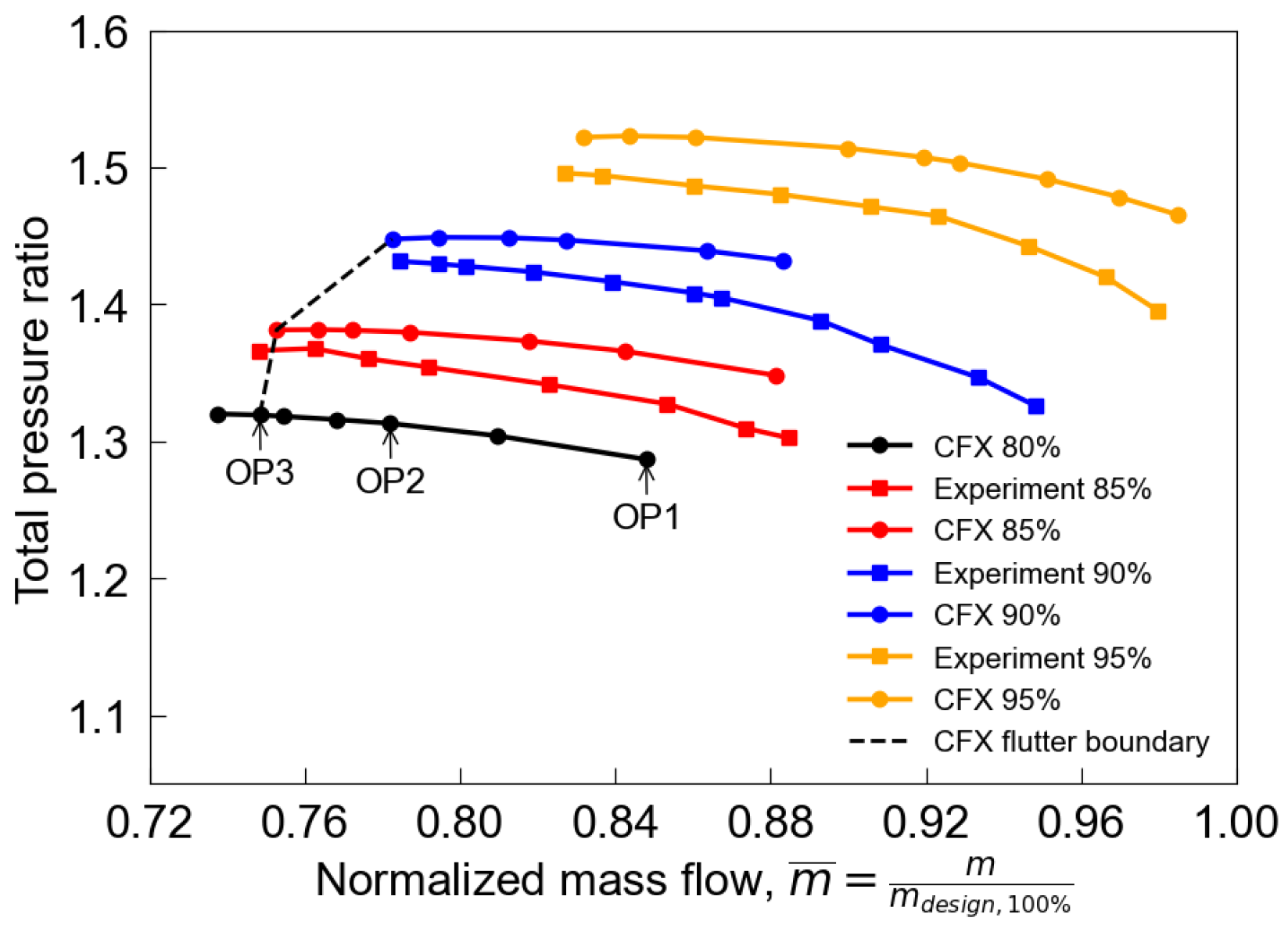
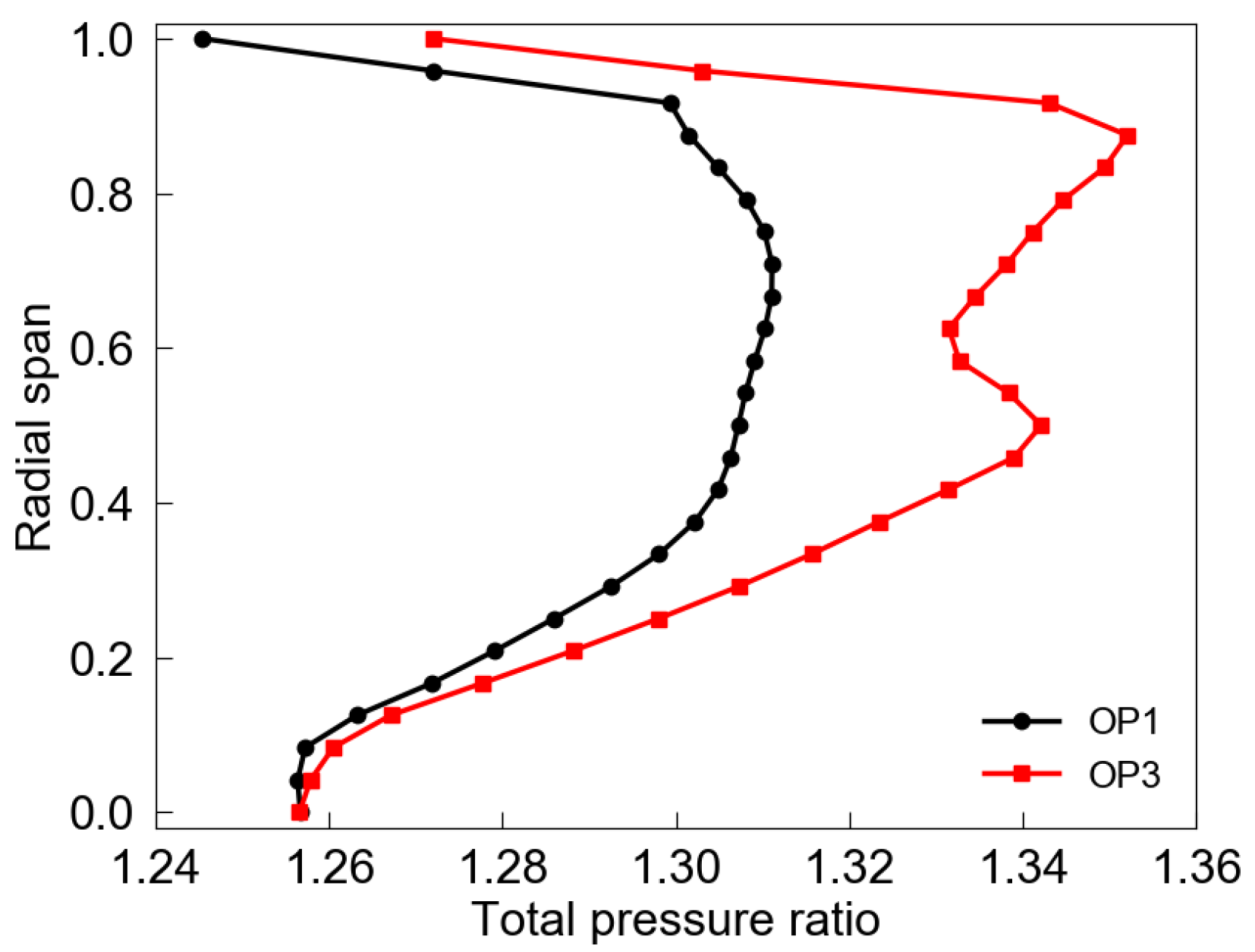
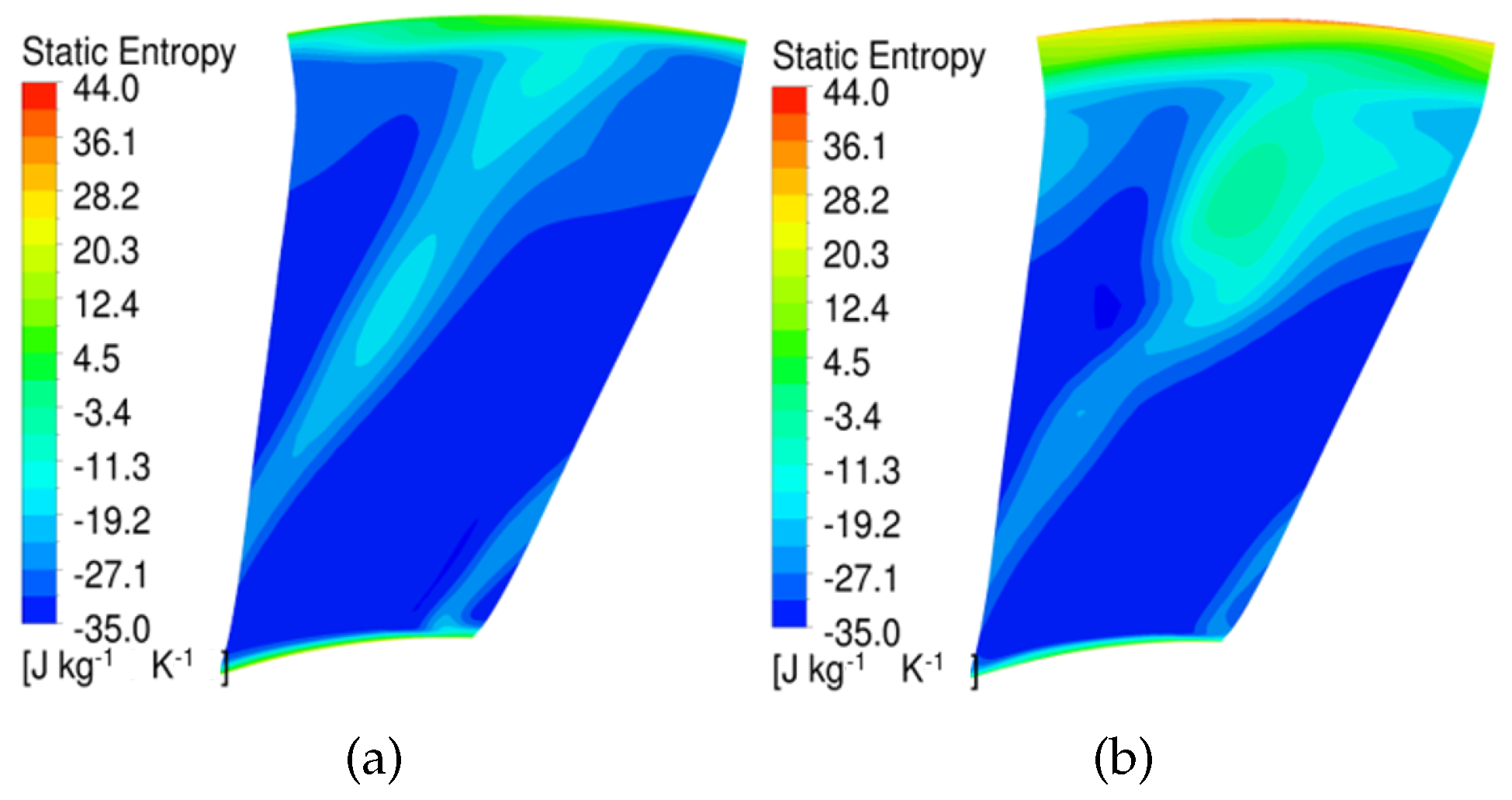
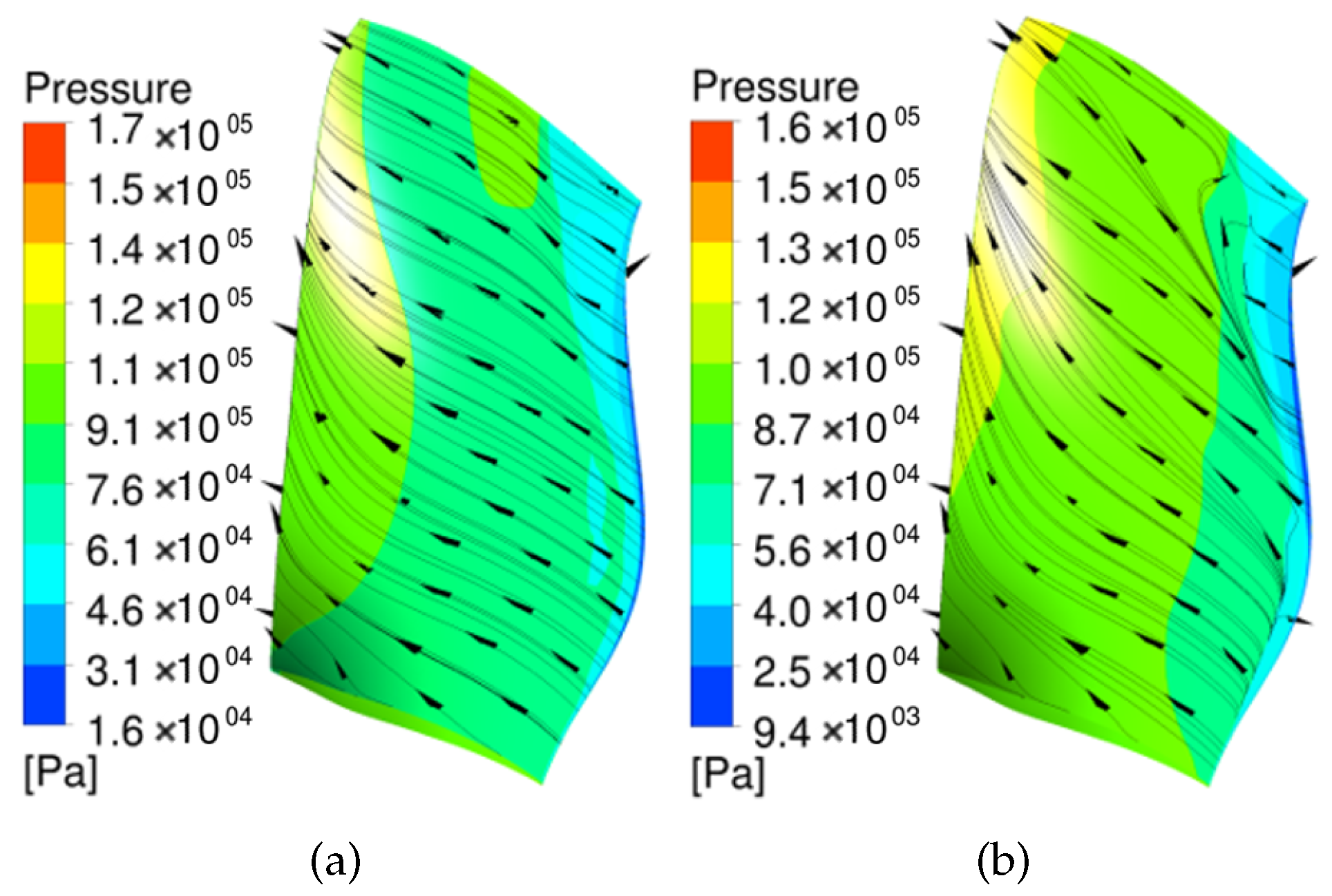
3.2. Steady-State Solutions
3.3. Modal Analysis
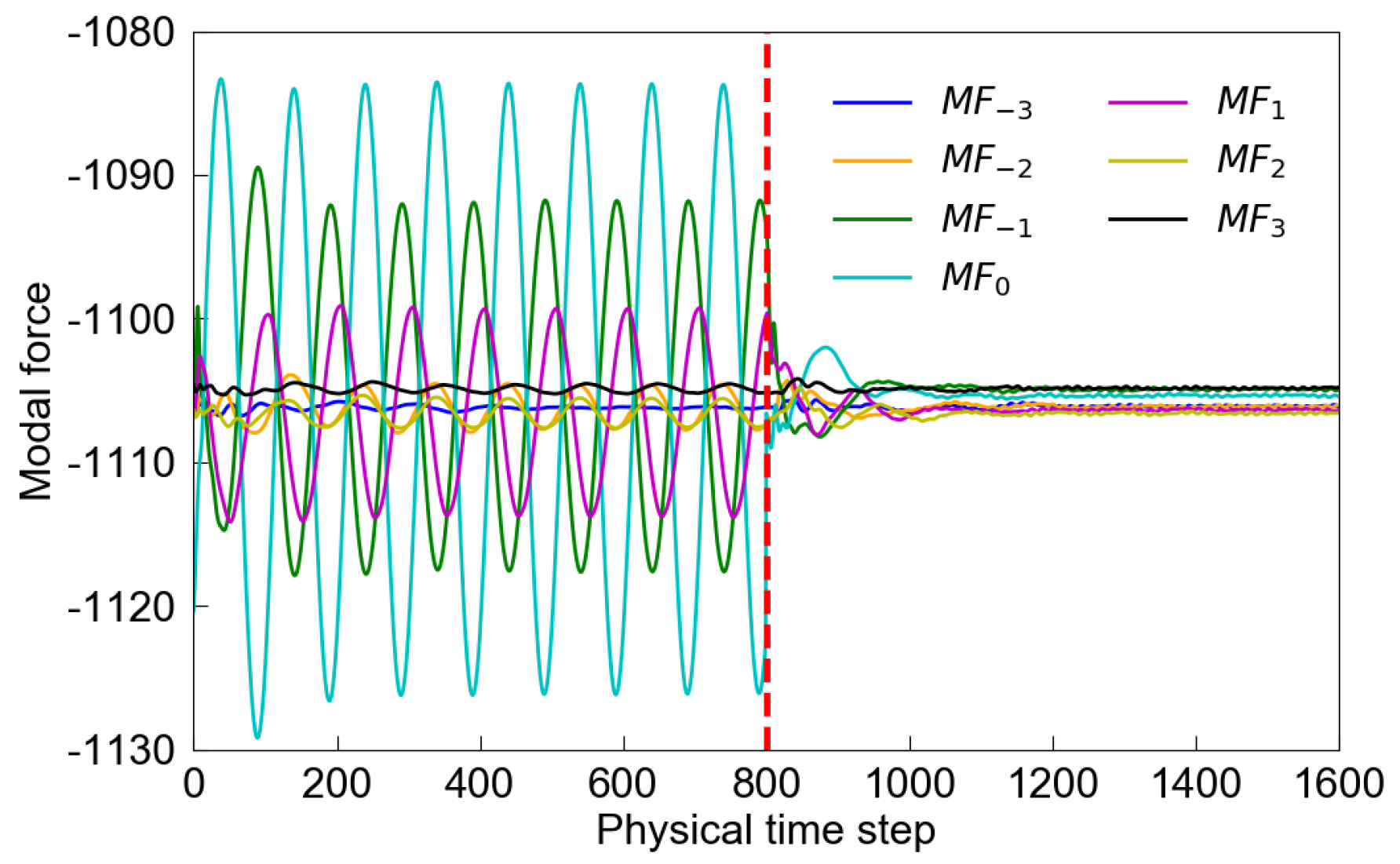
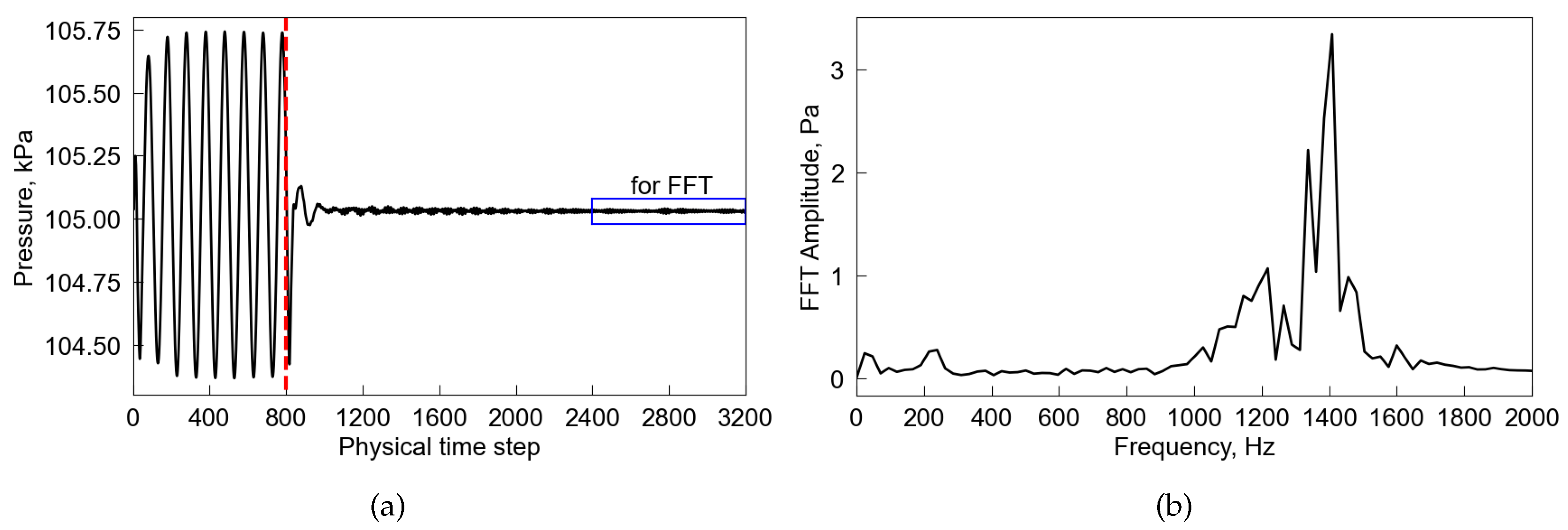
3.4. Aerodynamic Damping Computation
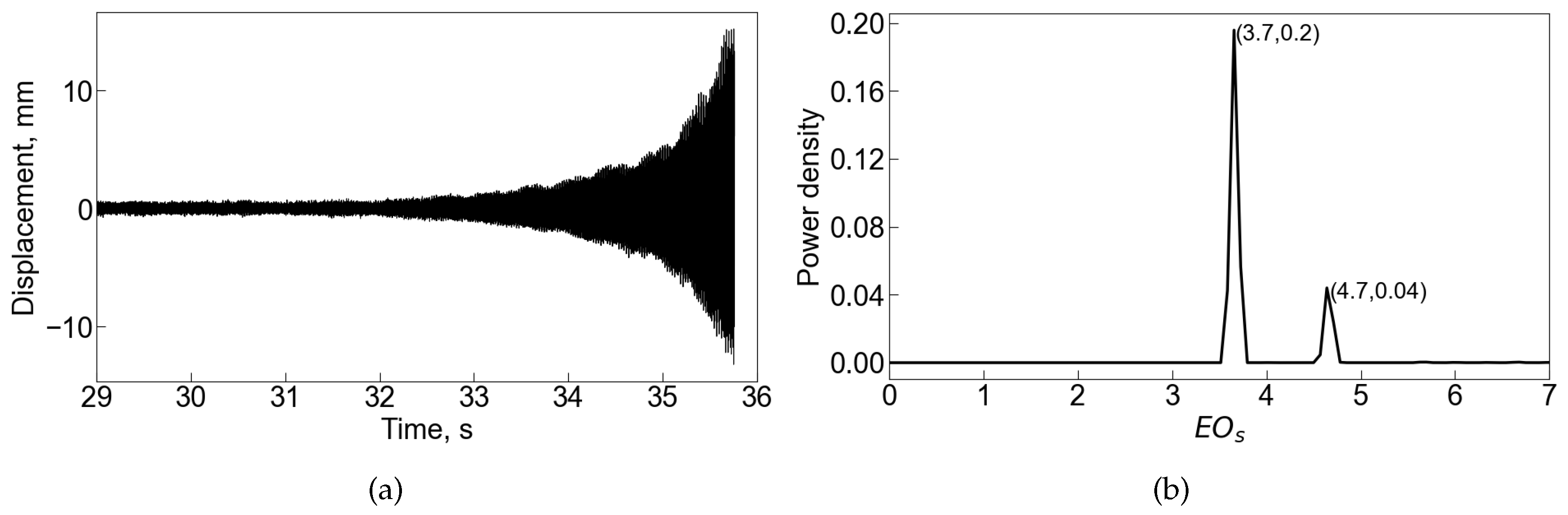
3.5. Flutter Mechanisms
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| c | speed of sound |
| f | blade vibration frequency |
| i | blade index |
| imaginary unit | |
| k | free stream wave number |
| axial wave number | |
| radial–circumferential wave number | |
| l | axial mesh size |
| m | nodal diameter |
| MF | modal force |
| absolute axial Mach number | |
| absolute tangential Mach number | |
| blade surface unit normal | |
| p | static pressure |
| q | generalized displacement |
| r | radial coordinate |
| rotational frame of reference | |
| ℜ | the real part of a complex number |
| stationary frame of reference | |
| t | time |
| T | vibration period |
| u | axial velocity |
| W | aerodynamic work |
| Cartesian coordinates | |
| 1F | first flap mode |
| EO | engine order |
| FFT | fast Fourier transform |
| FSI | fluid–structure interaction |
| ICM | influence coefficient method |
| IBPA | inter blade phase angle |
| NSV | non-synchronous vibration |
| TWM | traveling wave method |
| aerodynamic damping | |
| circumferential coordinate | |
| inter blade phase angle | |
| mode shape vector | |
| blade vibration angular frequency | |
| shaft frequency |
References
- Ren, J.; Huang, H.; Wang, D.; Dong, X.; Cao, B. An efficient coupled-mode flutter analysis method for turbomachinery. Aerosp. Sci. Technol. 2020, 106, 106215. [Google Scholar] [CrossRef]
- Huang, H.; Liu, W.; Petrie-Repar, P.; Wang, D. An efficient aeroelastic eigenvalue method for analyzing coupled-mode flutter in turbomachinery. J. Turbomach. 2021, 143, 021010. [Google Scholar] [CrossRef]
- Dong, X.; Zhang, Y.; Zhang, Y.; Zhang, Z.; Lu, X. Numerical simulations of flutter mechanism for high-speed wide-chord transonic fan. Aerosp. Sci. Technol. 2020, 105, 106009. [Google Scholar] [CrossRef]
- Dong, X.; Zhang, Z.; Zhang, Y.; Zhang, Y.; Lu, X. High-frequency unsteady flow near the tip in a transonic fan rotor with a small clearance. Aerosp. Sci. Technol. 2020, 106, 106211. [Google Scholar] [CrossRef]
- Corral, R.; Beloki, J.; Calza, P.; Elliott, R. Flutter generation and control using mistuning in a turbine rotating rig. AIAA J. 2019, 57, 782–795. [Google Scholar] [CrossRef]
- Rzadkowski, R.; Gnesin, V.; Kolodyazhnaya, L.; Kubitz, L. Aeroelastic behaviour of a 3.5-stage aircraft compressor rotor blades following a bird strike. J. Vib. Eng. Technol. 2018, 6, 281–287. [Google Scholar] [CrossRef]
- Zhao, F.; Nipkau, J.; Vahdati, M. Influence of acoustic reflections on flutter stability of an embedded blade row. Proc. Inst. Mech. Eng. Part A J. Power Energy 2016, 230, 29–43. [Google Scholar] [CrossRef]
- Huang, H.; Mingming, Y.; Dingxi, W. Efficient fan acoustic flutter analysis using the influence coefficient method and a sliding plane. J. Aerosp. Eng. 2023, 36, 06022005. [Google Scholar] [CrossRef]
- Vahdati, M.; Lee, K.B.; Sureshkumar, P. A review of computational aeroelasticity of civil fan blades. Int. J. Gas Turbine Propuls. Power Syst. 2020, 11, 22–35. [Google Scholar] [CrossRef]
- Isomura, K.; Giles, M. A numerical study of flutter in a transonic fan. J. Turbomach. 1998, 120, 500–507. [Google Scholar] [CrossRef]
- Vahdati, M.; Sayma, A.; Marshall, J.; Imregun, M. Mechanisms and prediction methods for fan blade stall flutter. J. Propuls. Power 2001, 17, 1100–1108. [Google Scholar] [CrossRef]
- Carta, F.O. Coupled blade-disk-shroud flutter instabilities in turbojet engine rotors. J. Eng. Power 1967, 89, 419–426. [Google Scholar] [CrossRef]
- Chahine, C.; Verstraete, T.; He, L. A Comparative study of coupled and decoupled fan flutter prediction methods under variation of mass ratio and blade stiffness. J. Fluids Struct. 2019, 85, 110–125. [Google Scholar] [CrossRef]
- Hanamura, Y.; Tanaka, H.; Yamaguchi, K. A simplified method to measure unsteady forces acting on the vibrating blades in cascade. Bull. JSME 1980, 23, 880–887. [Google Scholar] [CrossRef]
- Phan, H.; He, L. Validation studies of linear oscillating compressor cascade and use of influence coefficient method. J. Turbomach. 2020, 142, 051005. [Google Scholar] [CrossRef]
- Kielb, R.E.; Barter, J.W.; Thomas, J.P.; Hall, K.C. Blade excitation by aerodynamic instabilities: A compressor blade study. In Proceedings of the Turbo Expo: Power for Land, Sea, and Air, Atlanta, GA, USA, 16–19 June 2003; Volume 36878, pp. 399–406. [Google Scholar]
- Mao, Z.; Hegde, S.; Pan, T.; Kielb, R.E.; Zori, L.; Campregher, R. Influence of Rotor-Stator Interaction and Reflecting Boundary Conditions on Compressor Forced Response. In Proceedings of the Turbo Expo: Power for Land, Sea, and Air. American Society of Mechanical Engineers, Oslo, Norway, 11–15 June 2018; Volume 50992, p. V02AT45A004. [Google Scholar]
- Brandstetter, C.; Stapelfeldt, S. Analysis of a linear model for non-synchronous vibrations near stall. Int. J. Turbomach. Propuls. Power 2021, 6, 26. [Google Scholar] [CrossRef]
- Mueller, T.R.; Vogt, D.M.; Fischer, M.; Phillipsen, B.A. On the far-field boundary condition treatment in the framework of aeromechanical computations using ANSYS CFX. Proc. Inst. Mech. Eng. Part A J. Power Energy 2018, 235, 0957650920962698. [Google Scholar] [CrossRef]
- Colonius, T.; Lele, S.K.; Moin, P. Boundary conditions for direct computation of aerodynamic sound generation. AIAA J. 1993, 31, 1574–1582. [Google Scholar] [CrossRef]
- Hall, K.C.; Thomas, J.P.; Clark, W.S. Computation of unsteady nonlinear flows in cascades using a harmonic balance technique. AIAA J. 2002, 40, 879–886. [Google Scholar] [CrossRef]
- Vogel, K.; Naidu, A.; Fischer, M. Comparison of the influence coefficient method and travelling wave mode approach for the calculation of aerodynamic damping of centrifugal compressors and axial turbines. In Proceedings of the Turbo Expo: Power for Land, Sea, and Air, Charlotte, NC, USA, 26–30 June 2017; American Society of Mechanical Engineers: New York, NY, USA, 2017; Volume 50930, p. V07BT36A022. [Google Scholar]
- Tyler, J.M.; Sofrin, T.G. Axial Flow Compressor Noise Studies. SAE Trans. 1962, 70, 309–332. [Google Scholar]
- Vahdati, M.; Cumpsty, N. Aeroelastic instability in transonic fans. J. Eng. Gas Turbines Power 2016, 138, 022604. [Google Scholar] [CrossRef]
- Moinier, P.; Giles, M.B. Eigenmode analysis for turbomachinery applications. J. Propuls. Power 2005, 21, 973–978. [Google Scholar] [CrossRef]
- Huang, H. Acoustic eigenmode analysis for swirling flows in ducts. In Proceedings of the Global Power and Propulsion Society, GPPS-TC-2017-0032, Shanghai, China, 30 October–1 November 2017. [Google Scholar]
- Lee, K.B.; Wilson, M.; Vahdati, M. Numerical study on aeroelastic instability for a low-speed fan. J. Turbomach. 2017, 139, 071004. [Google Scholar] [CrossRef]
- Jia, X.; Huang, H.; Wang, D. The effects of blade vibration mode on flutter stability. In Proceedings of the Global Power and Propulsion Society, GPPS-TC-2021-0182, Xi’an, China, 18–20 October 2021. [Google Scholar]




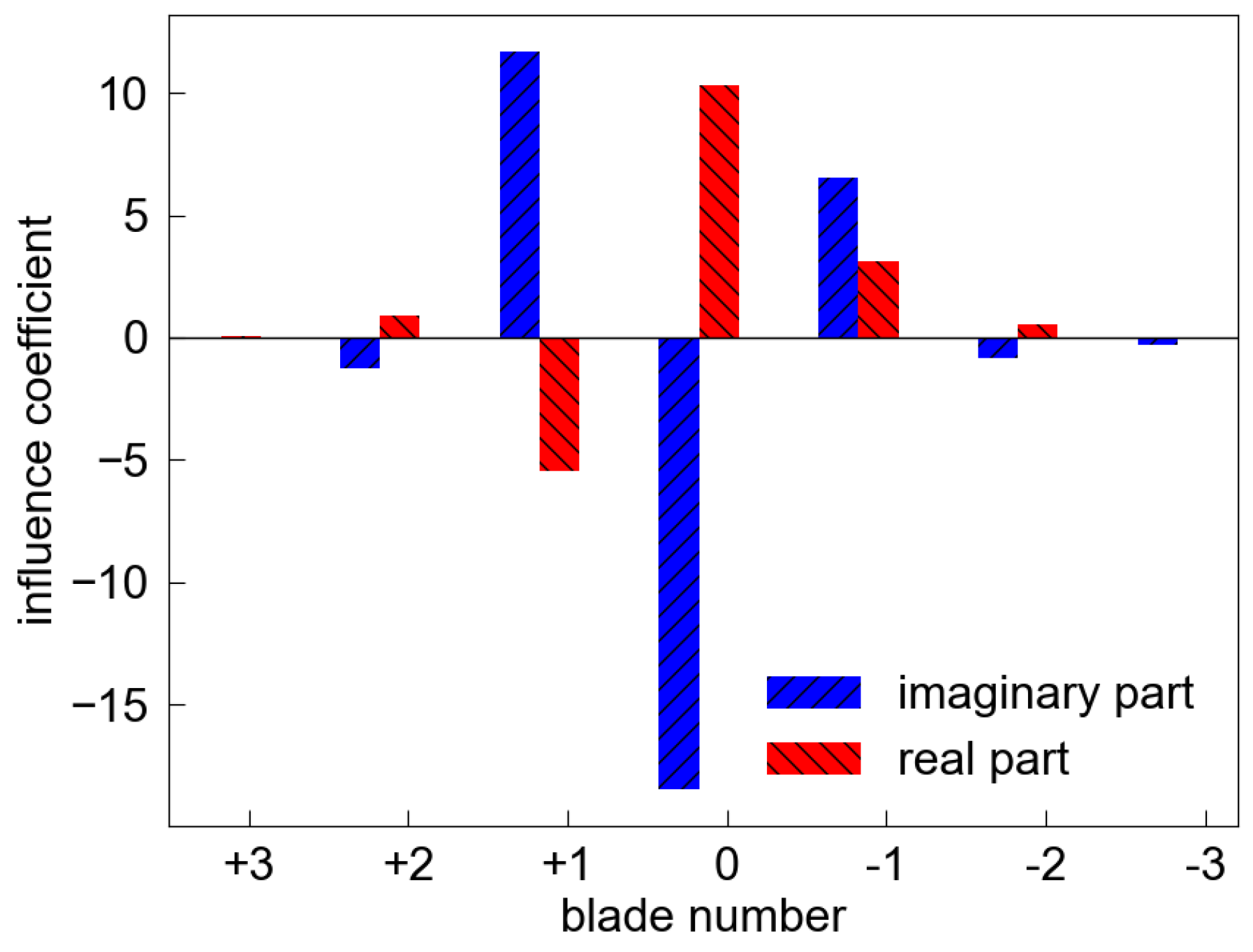
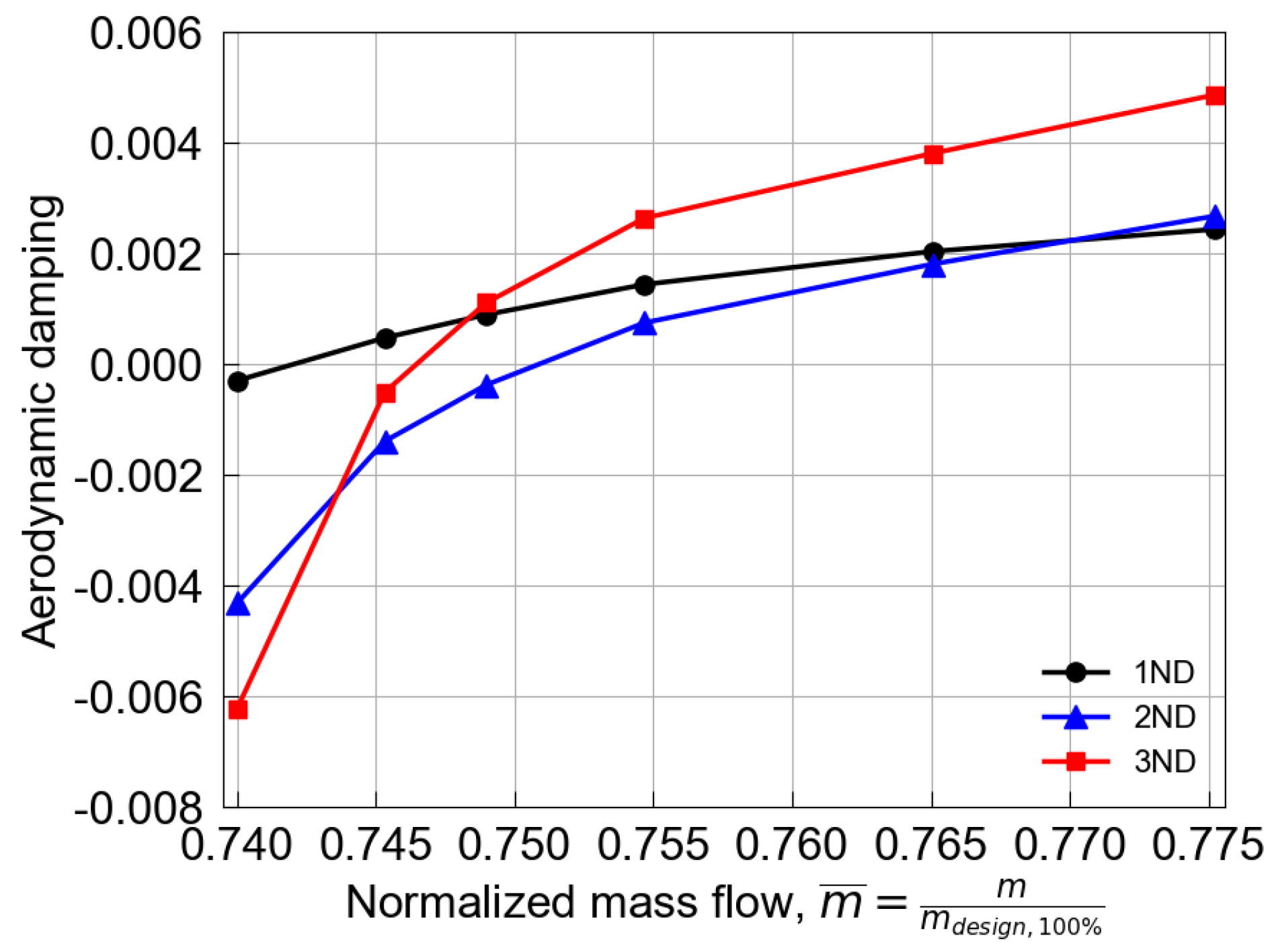
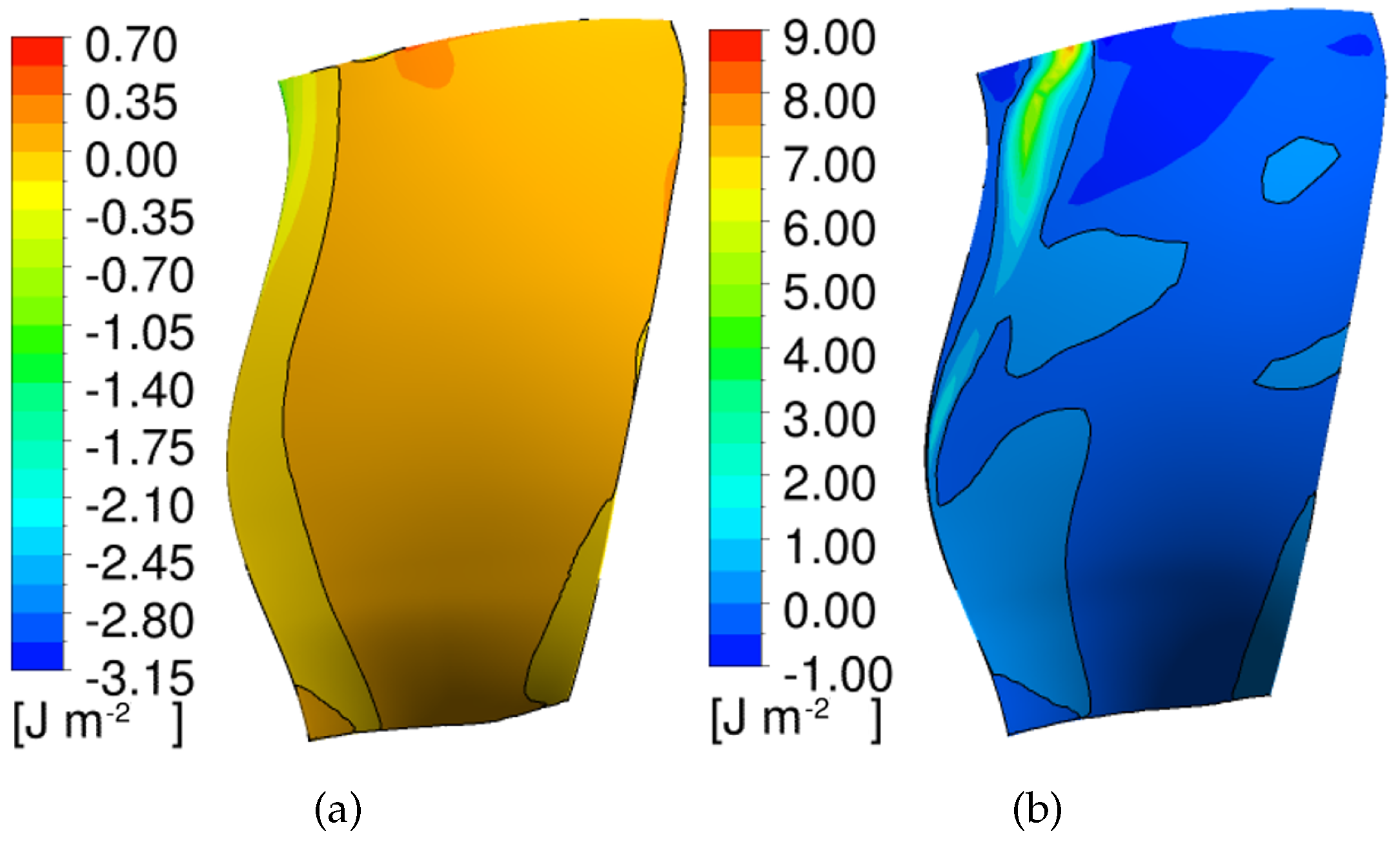


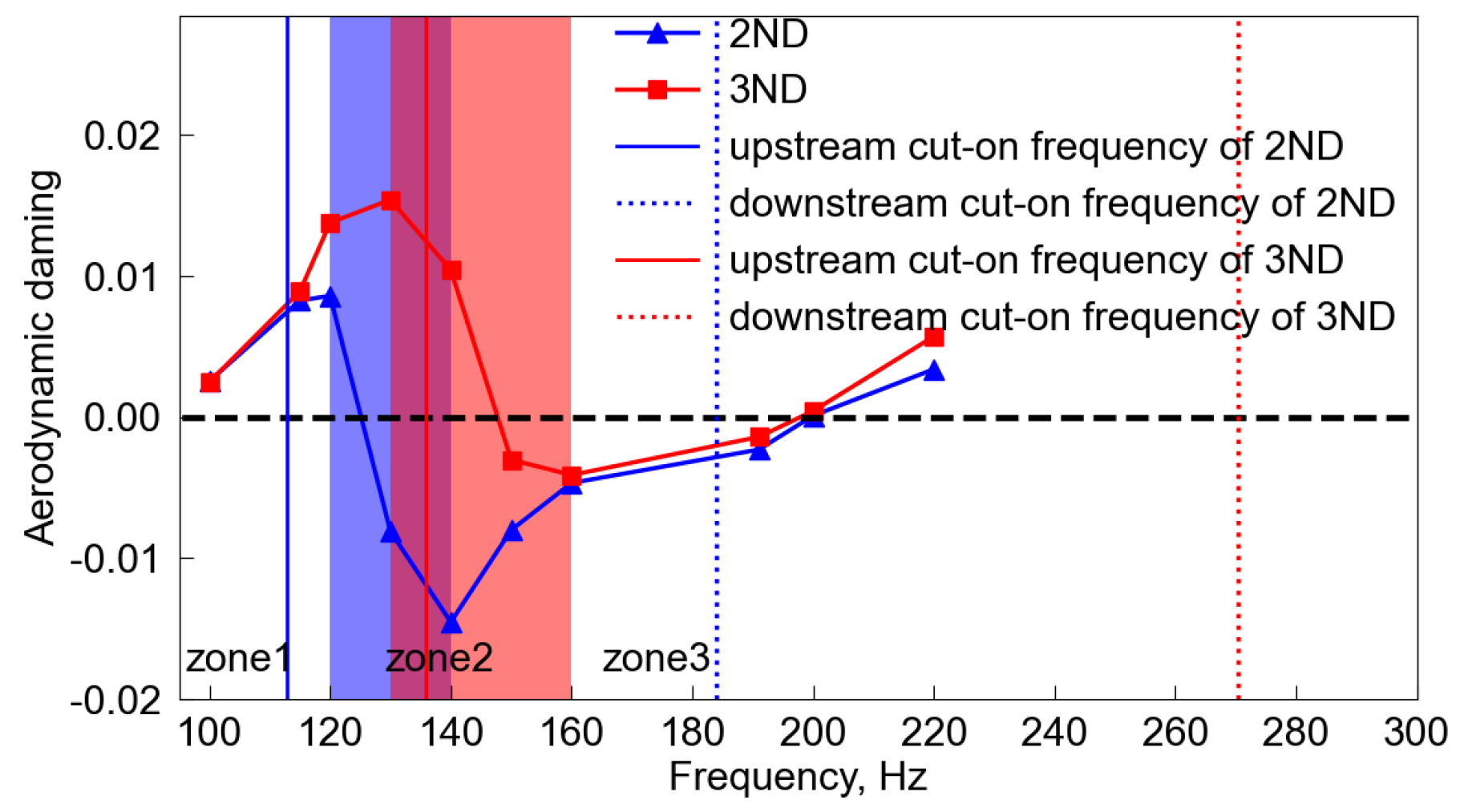
| Type | 2ND | 3ND |
|---|---|---|
| free stream frequency | 415 Hz | 527.5 Hz |
| upstream cut-on frequency | 338 Hz | 473.5 Hz |
| downstream cut-on frequency | 409 Hz | 608 Hz |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY-NC-ND) license (https://creativecommons.org/licenses/by-nc-nd/4.0/).
Share and Cite
Huang, H.; Yang, M.; Wang, D. Uncovering the Root Causes of Stall Flutter in a Wide Chord Fan Blisk. Int. J. Turbomach. Propuls. Power 2022, 7, 30. https://doi.org/10.3390/ijtpp7040030
Huang H, Yang M, Wang D. Uncovering the Root Causes of Stall Flutter in a Wide Chord Fan Blisk. International Journal of Turbomachinery, Propulsion and Power. 2022; 7(4):30. https://doi.org/10.3390/ijtpp7040030
Chicago/Turabian StyleHuang, Huang, Mingming Yang, and Dingxi Wang. 2022. "Uncovering the Root Causes of Stall Flutter in a Wide Chord Fan Blisk" International Journal of Turbomachinery, Propulsion and Power 7, no. 4: 30. https://doi.org/10.3390/ijtpp7040030
APA StyleHuang, H., Yang, M., & Wang, D. (2022). Uncovering the Root Causes of Stall Flutter in a Wide Chord Fan Blisk. International Journal of Turbomachinery, Propulsion and Power, 7(4), 30. https://doi.org/10.3390/ijtpp7040030





