Unsteady Analysis of a Pulsating Alternate Flow Pattern in a Radial Vaned Diffuser
Abstract
:1. Introduction
2. Methodology
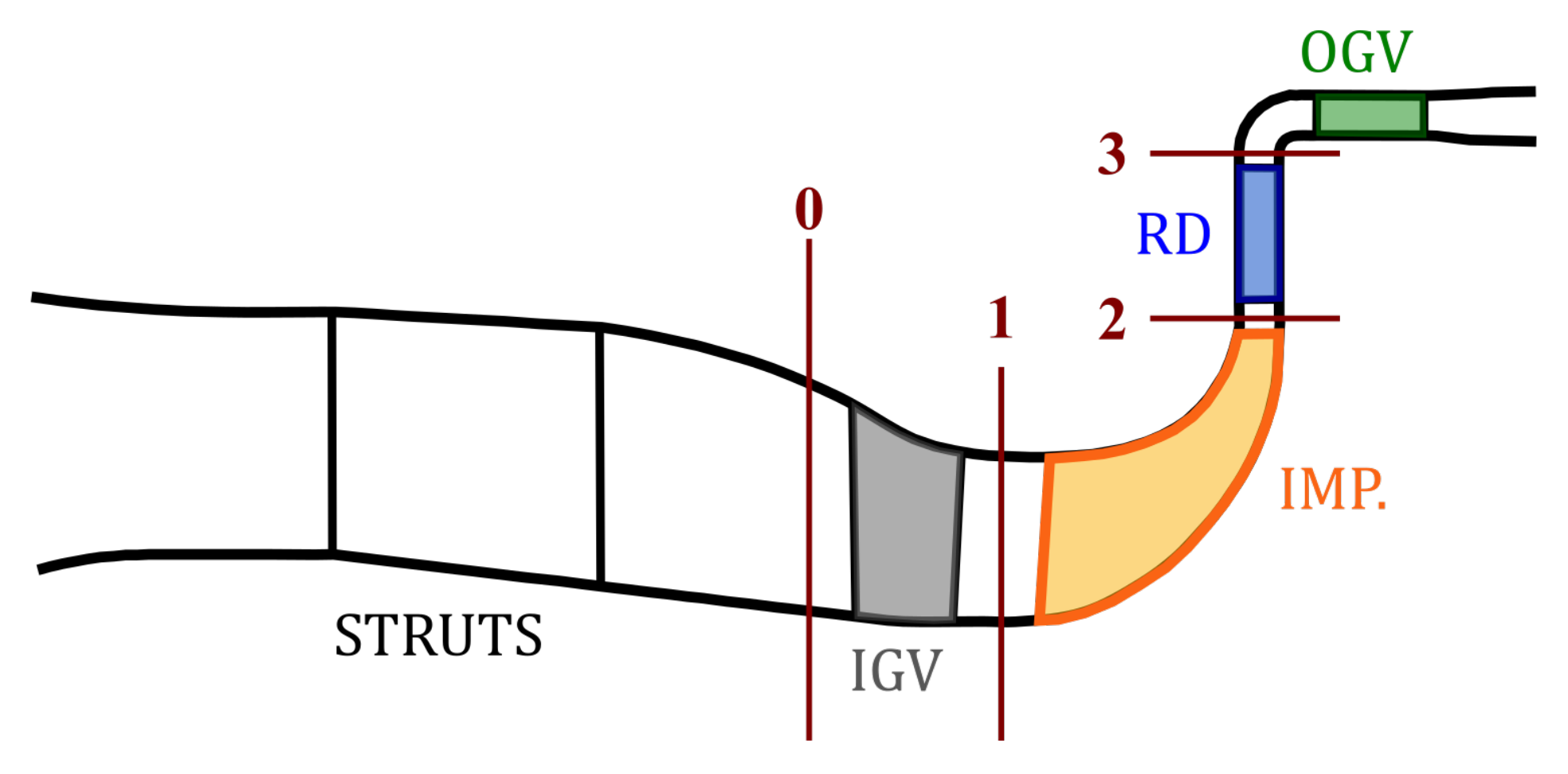
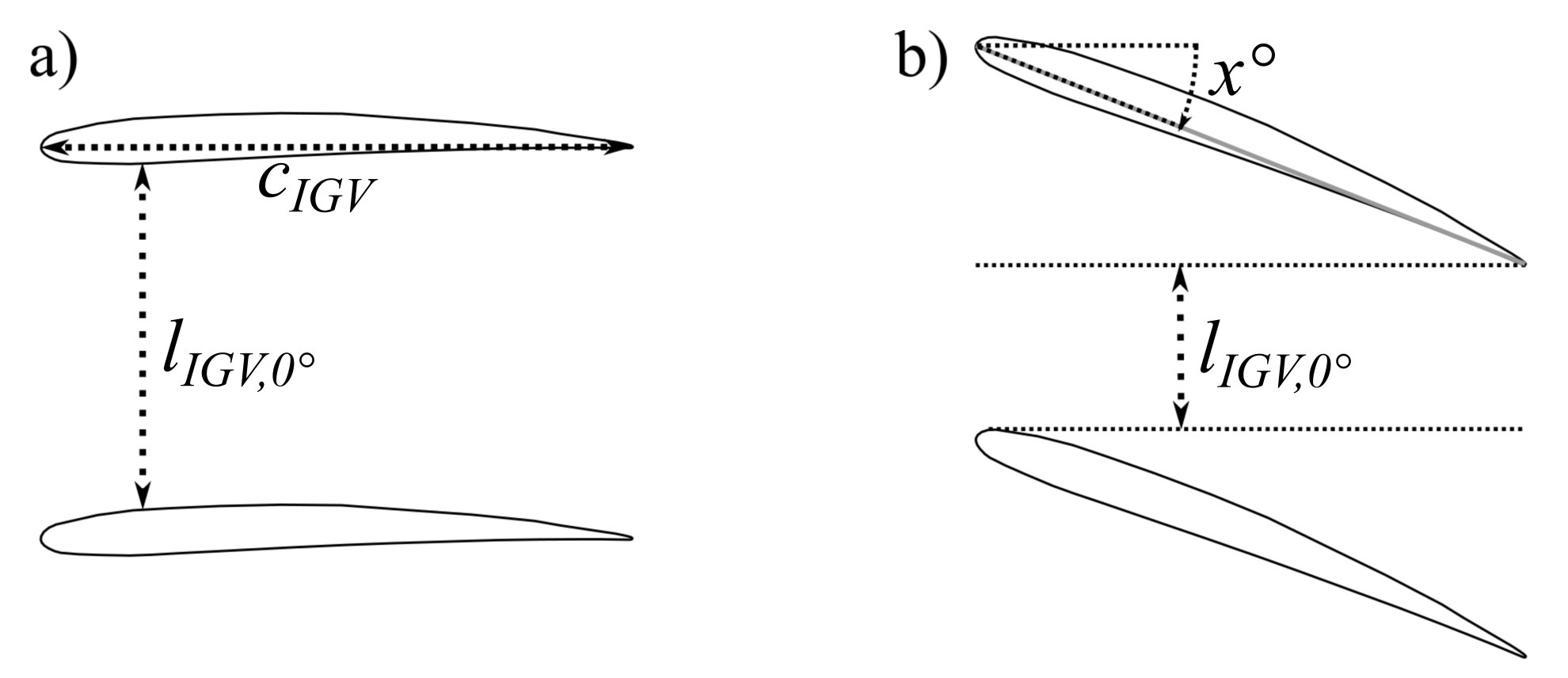
2.1. Test Case
2.2. Experimental Setup
2.3. Numerical Setup
2.4. Topological Analysis
3. Results
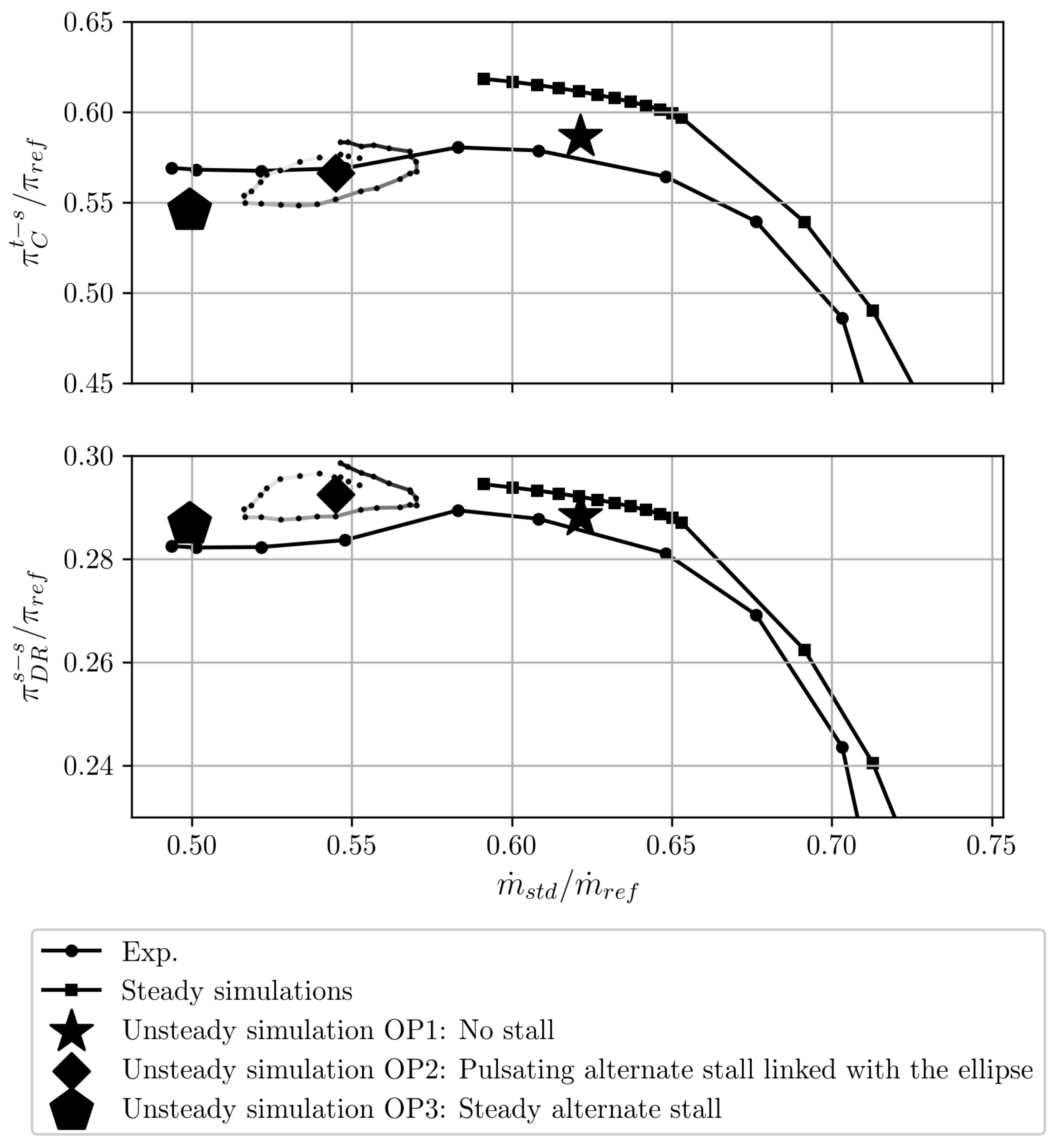
3.1. Experimental Investigation
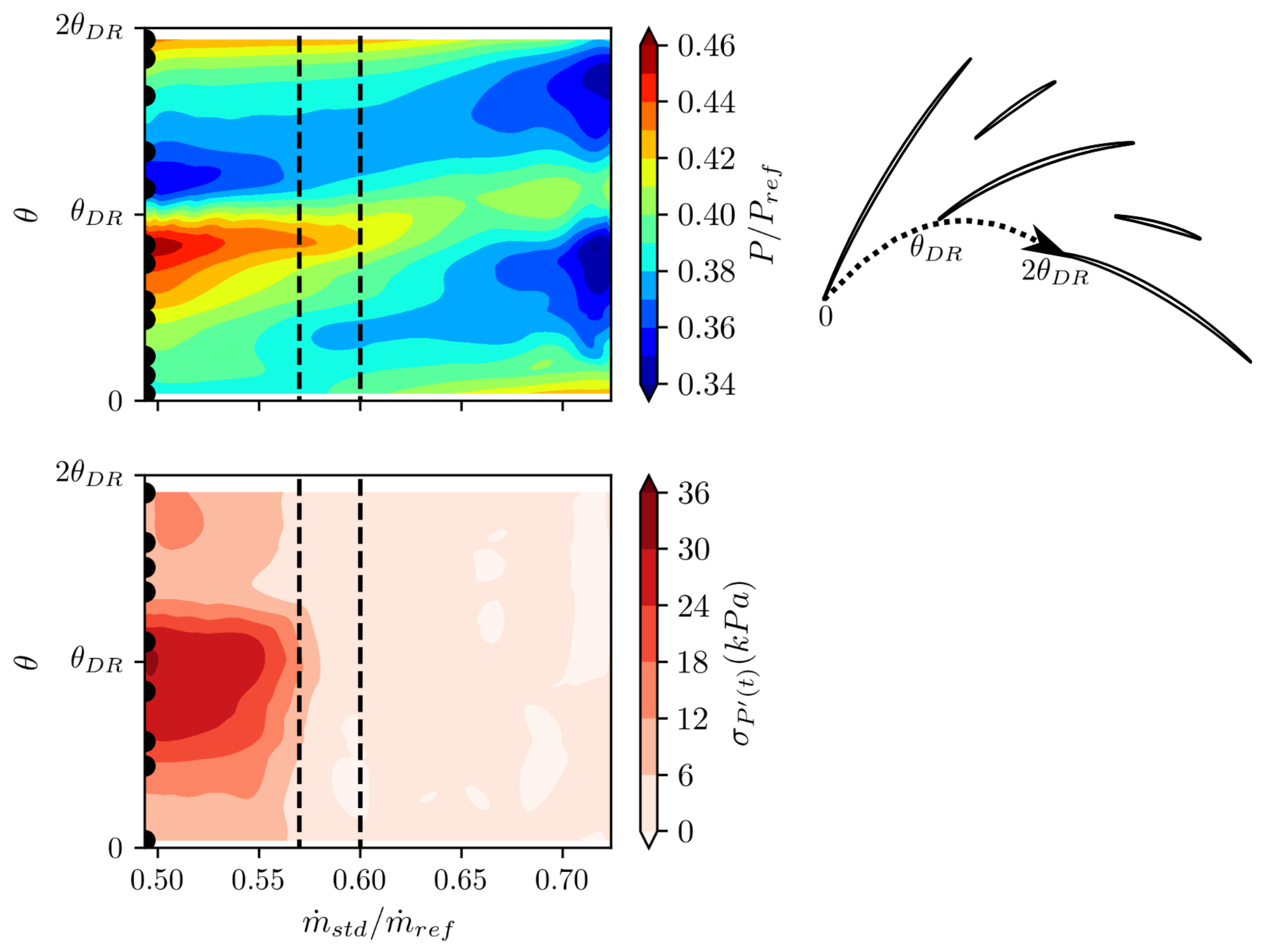
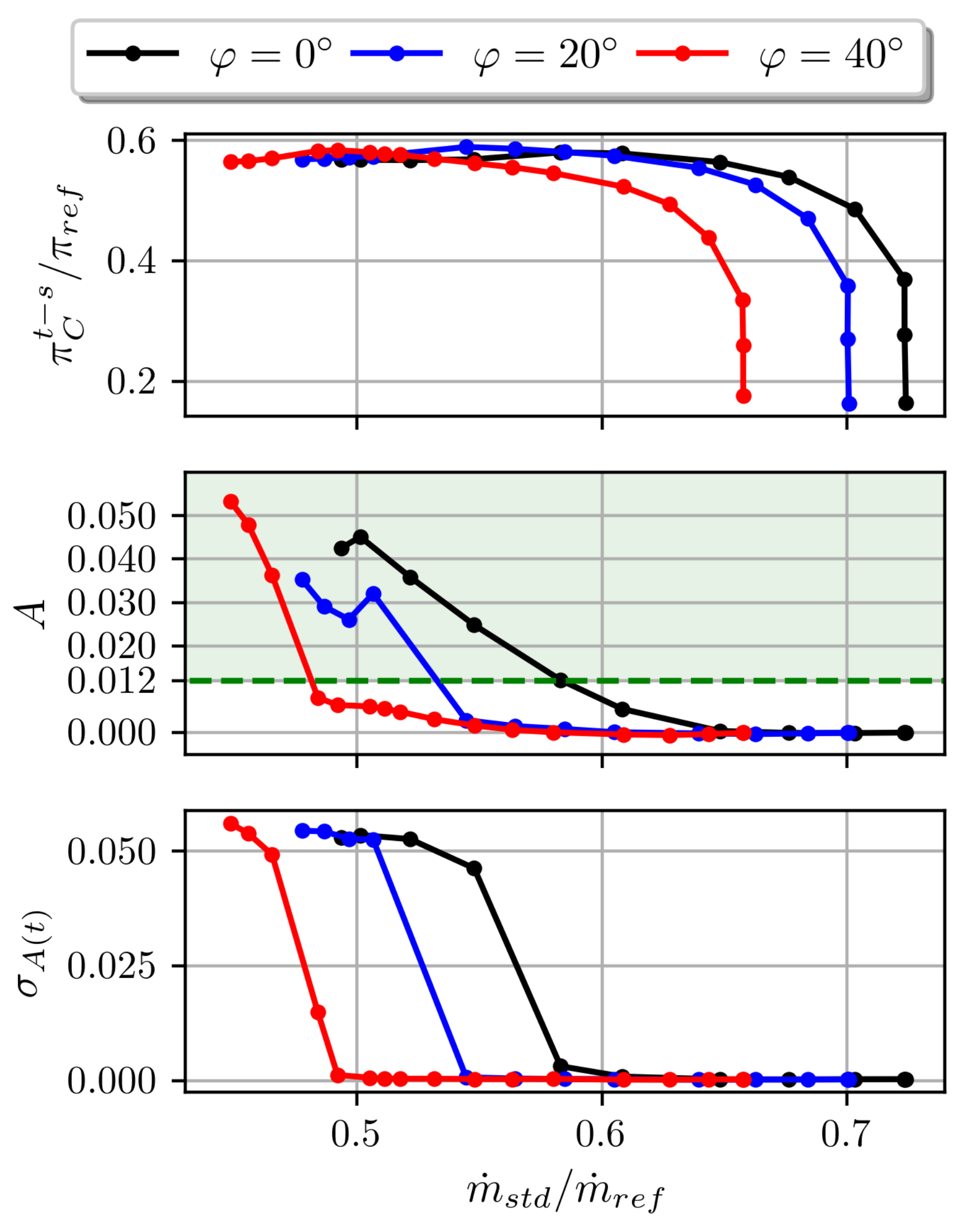
3.1.1. IGV Stagger Angle of
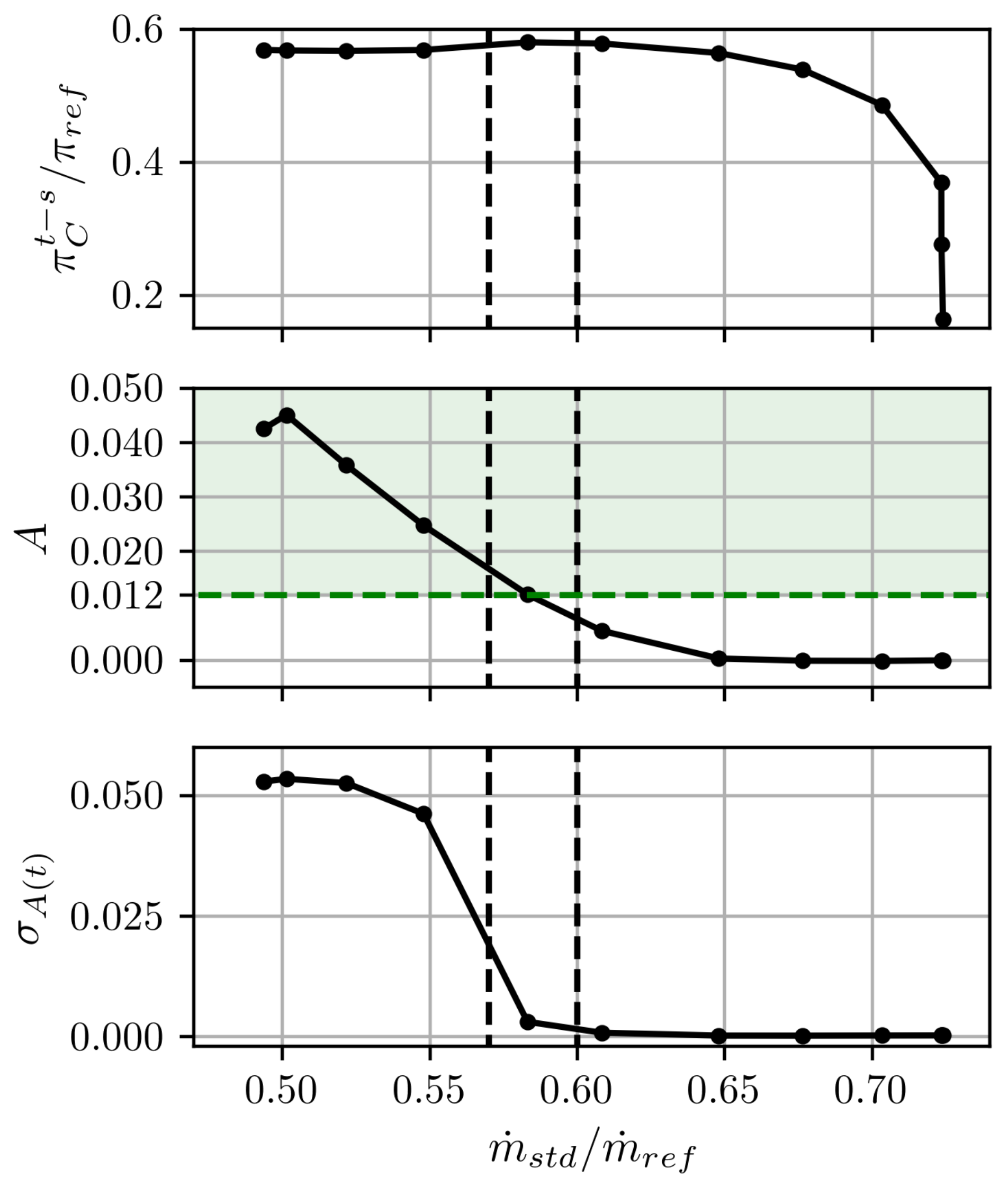
- If , the steady alternate rate is lower than the significance level, meaning that the flow is periodic.
- If , the steady alternate rate is very similar to the significance level, while the unsteady alternate rate standard deviation remains close to zero. Therefore, an alternate flow arises over two channels while remaining steady.
- If , the steady alternate rate becomes higher than the significance level and the unsteady alternate rate standard deviation is of the same order of magnitude as the steady alternate rate. These are the signs of a pulsating alternate flow over two channels. The pressure ratio of the whole stage slightly decreases as well.
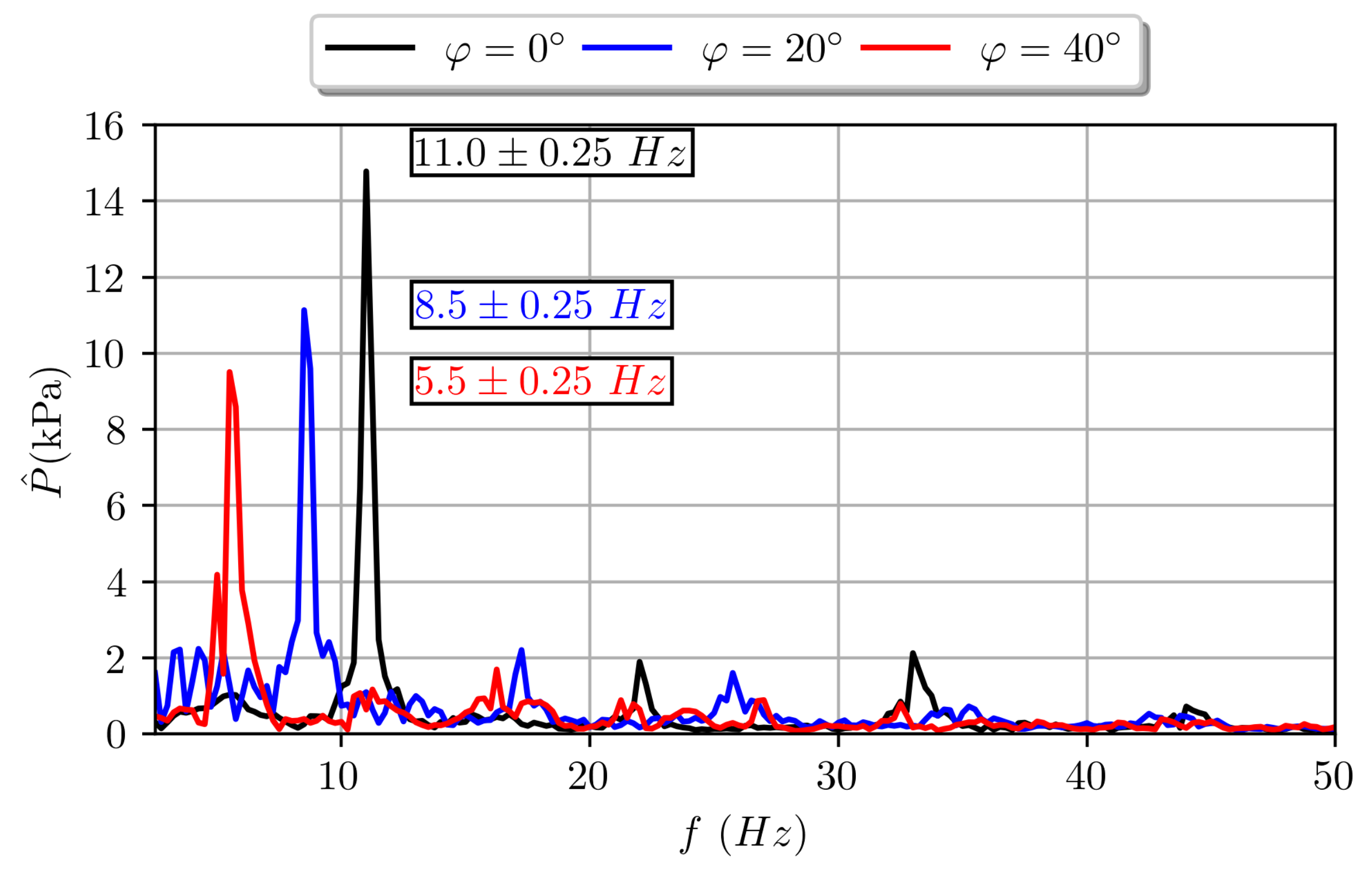
3.1.2. Impact of IGV Stagger Angle on the Helmholtz Frequency
3.2. Validation of Numerical Simulations
3.2.1. OP2: Performance Fluctuations
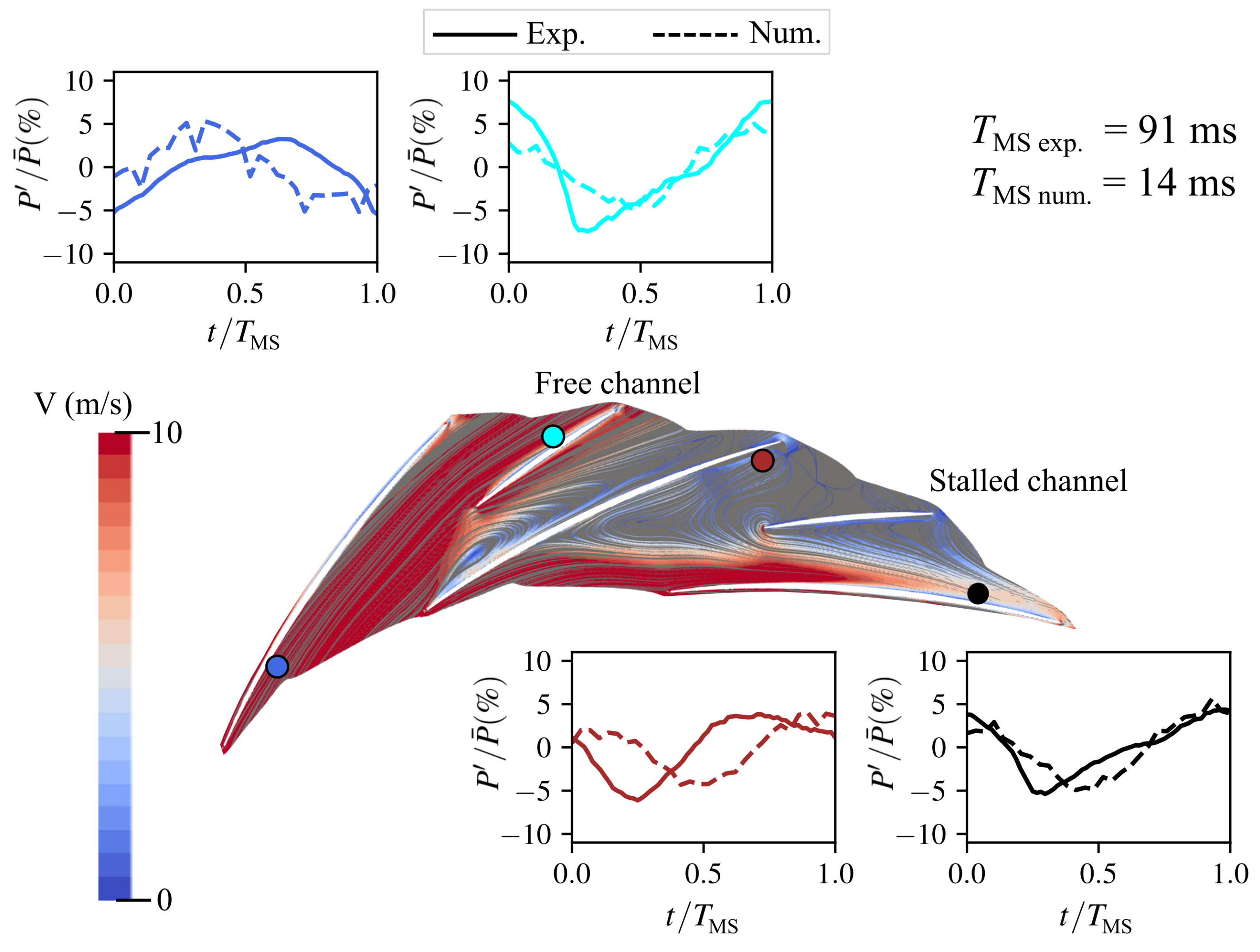
3.2.2. OP2: Pressure Fluctuations in the Radial Diffuser
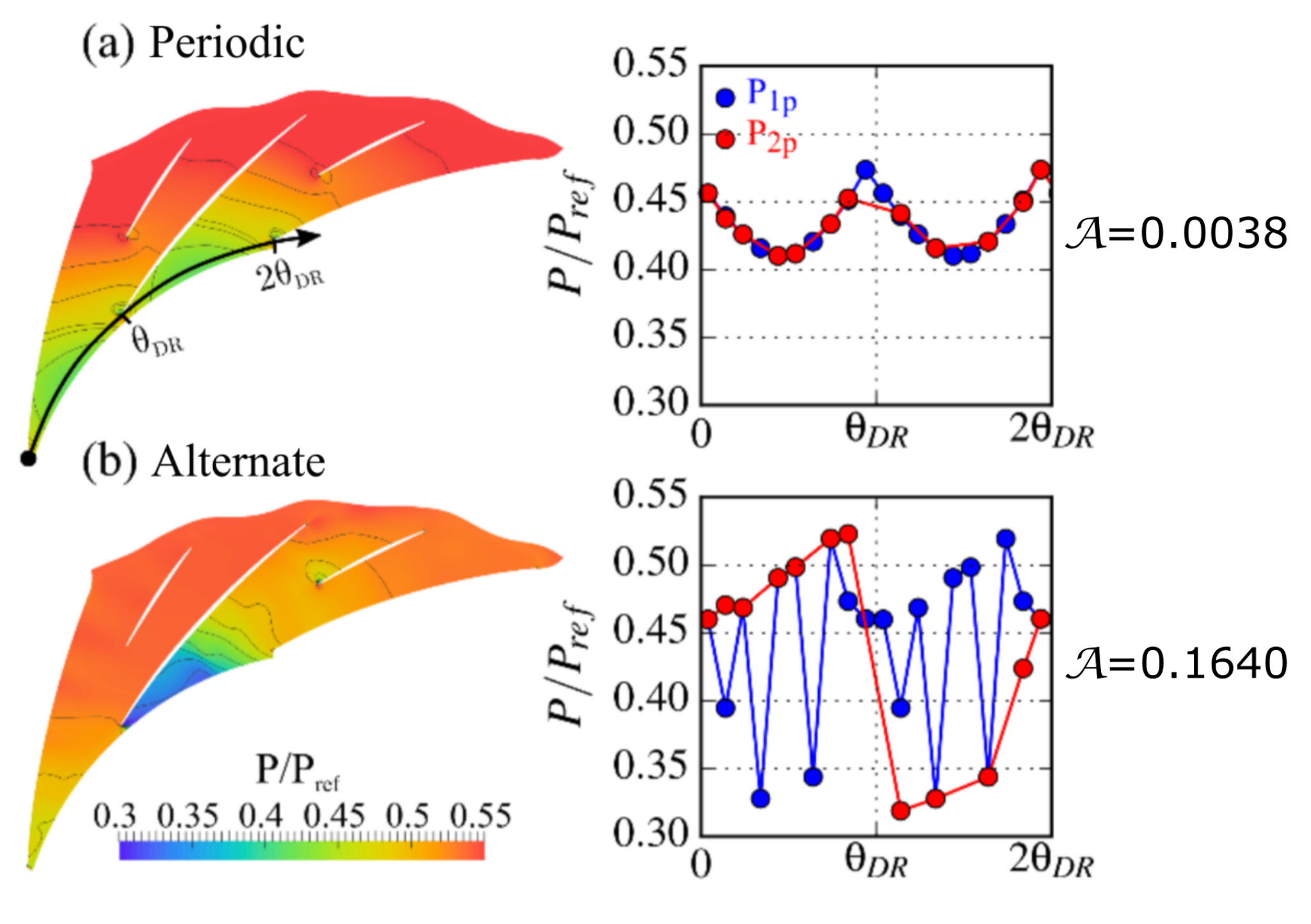
3.3. Average Flow Topology Analysis
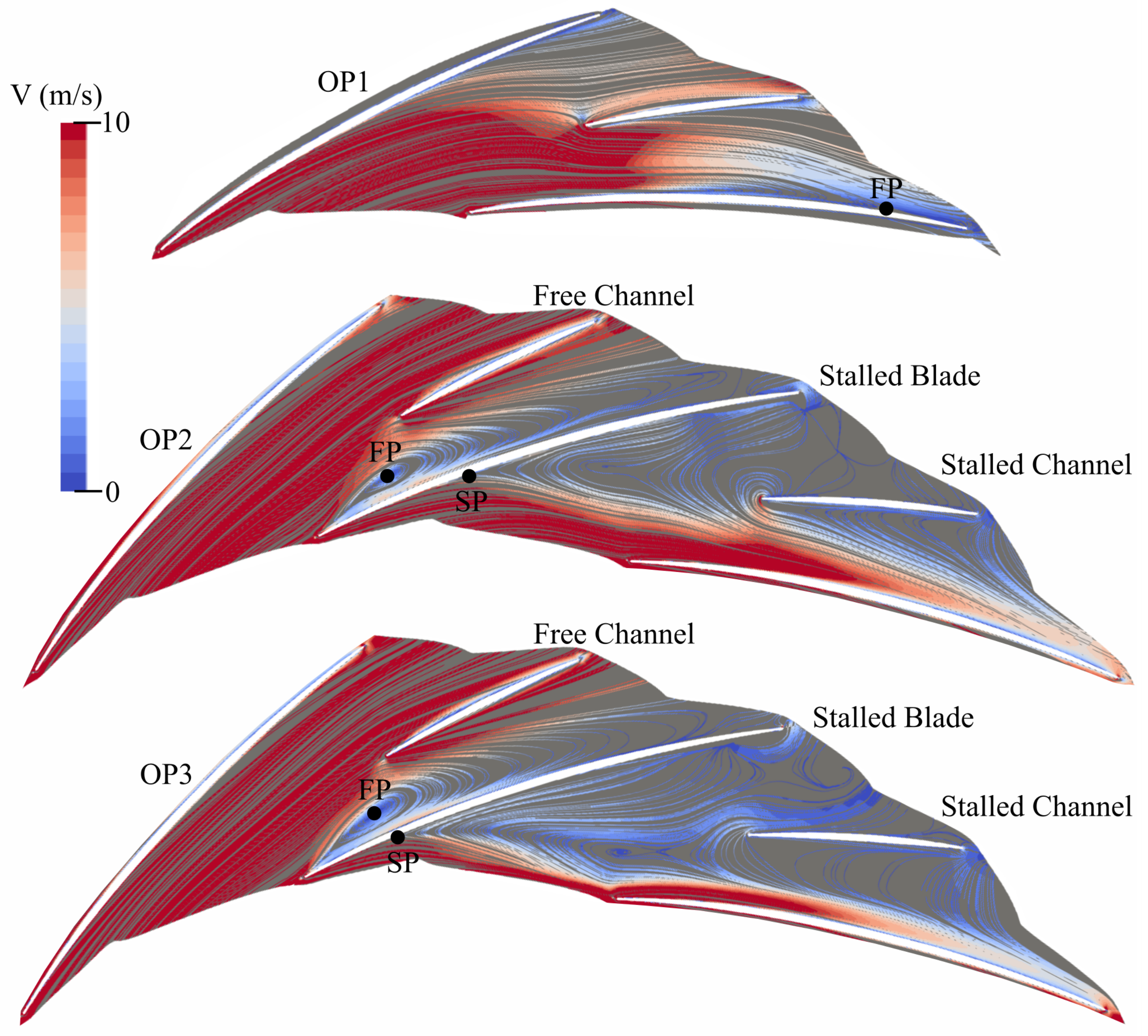
3.3.1. Change in the Average Flow Topology with Decreasing Mass Flow Rate
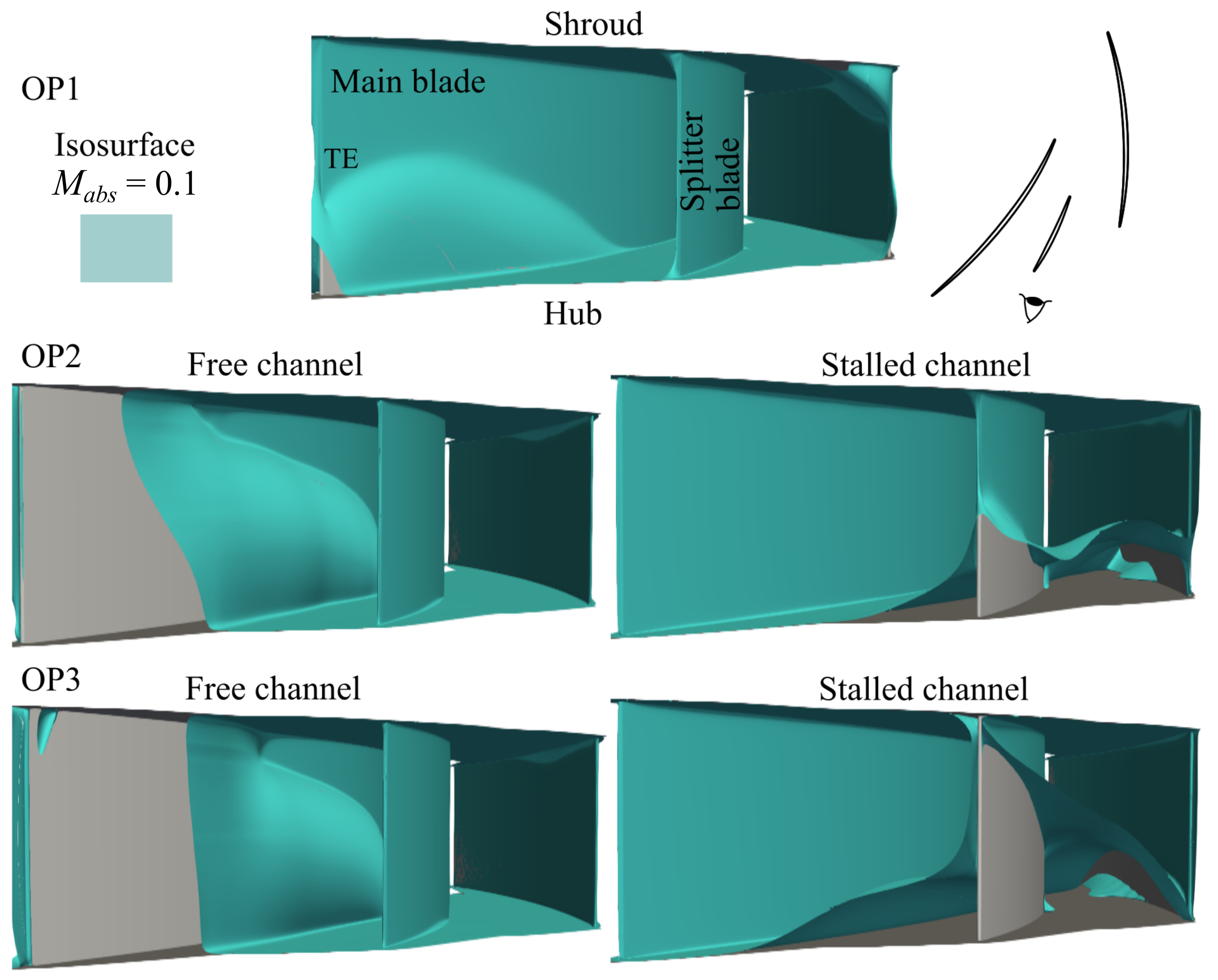
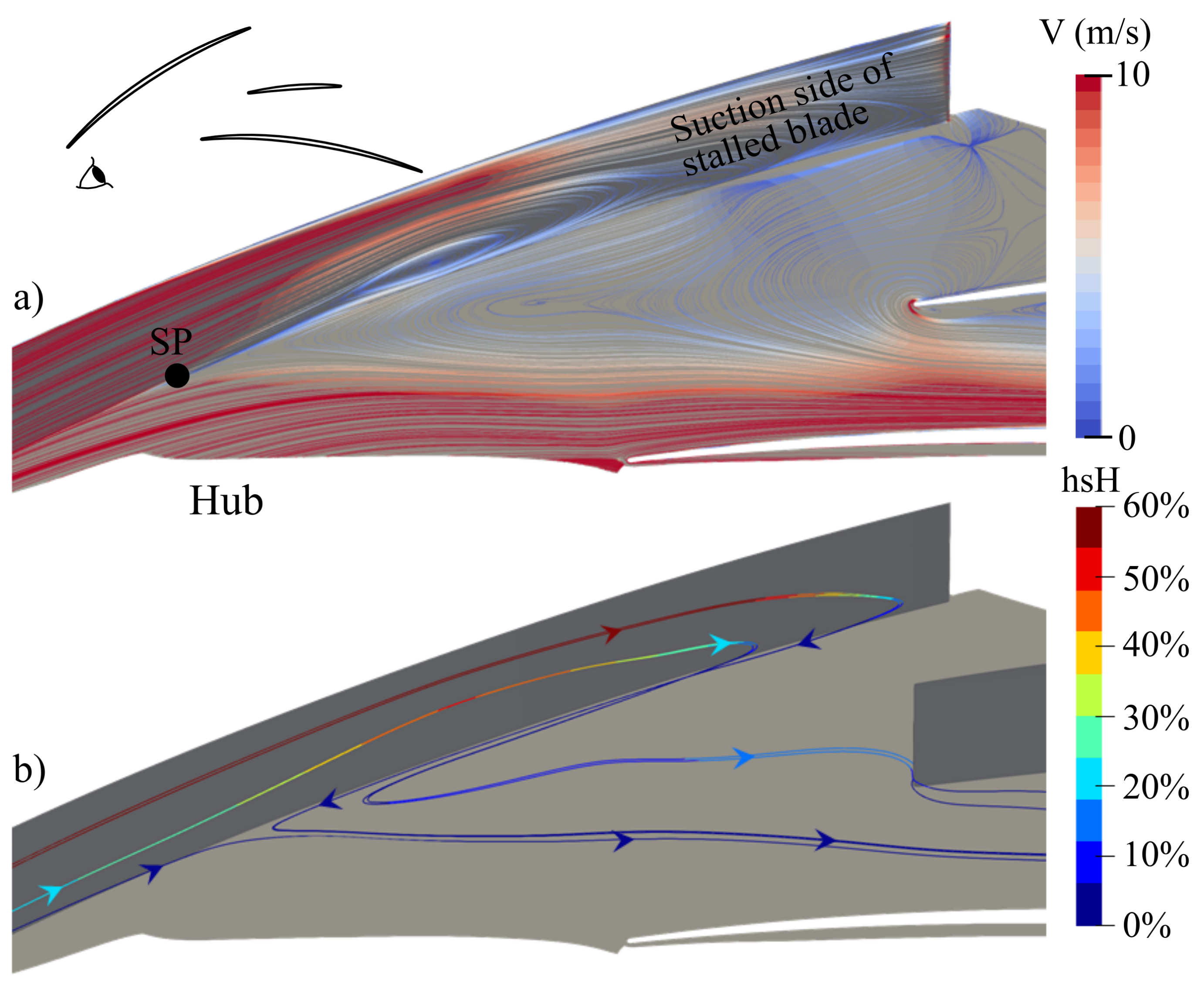
3.3.2. 3D Analysis at OP2
3.3.3. Origins of the Stall on Both Pressure and Suction Sides of a Blade
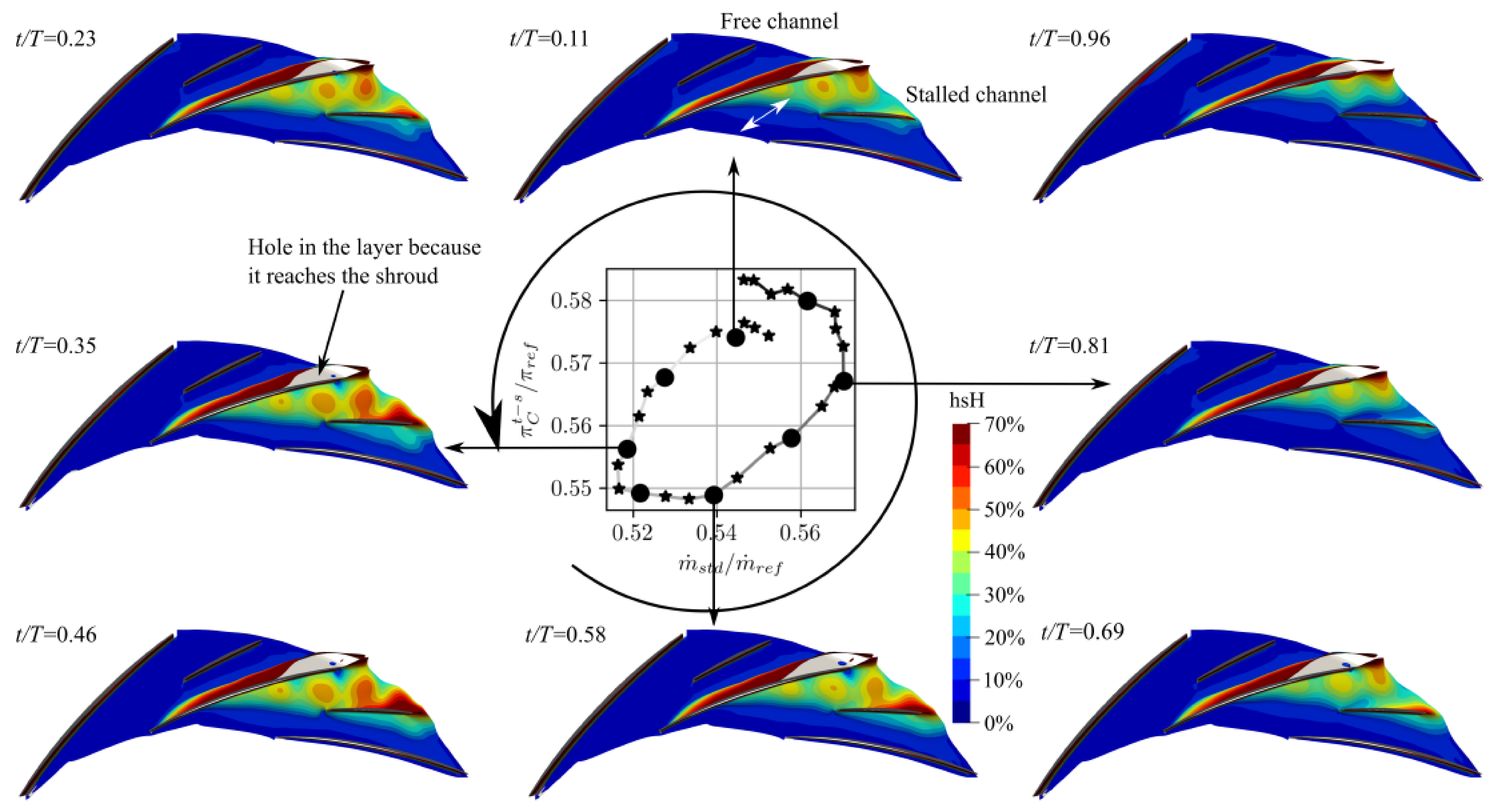
3.4. Pulsating Alternate Flow Pattern at OP2
3.4.1. Assumption of Breathing Stall in URANS Simulation
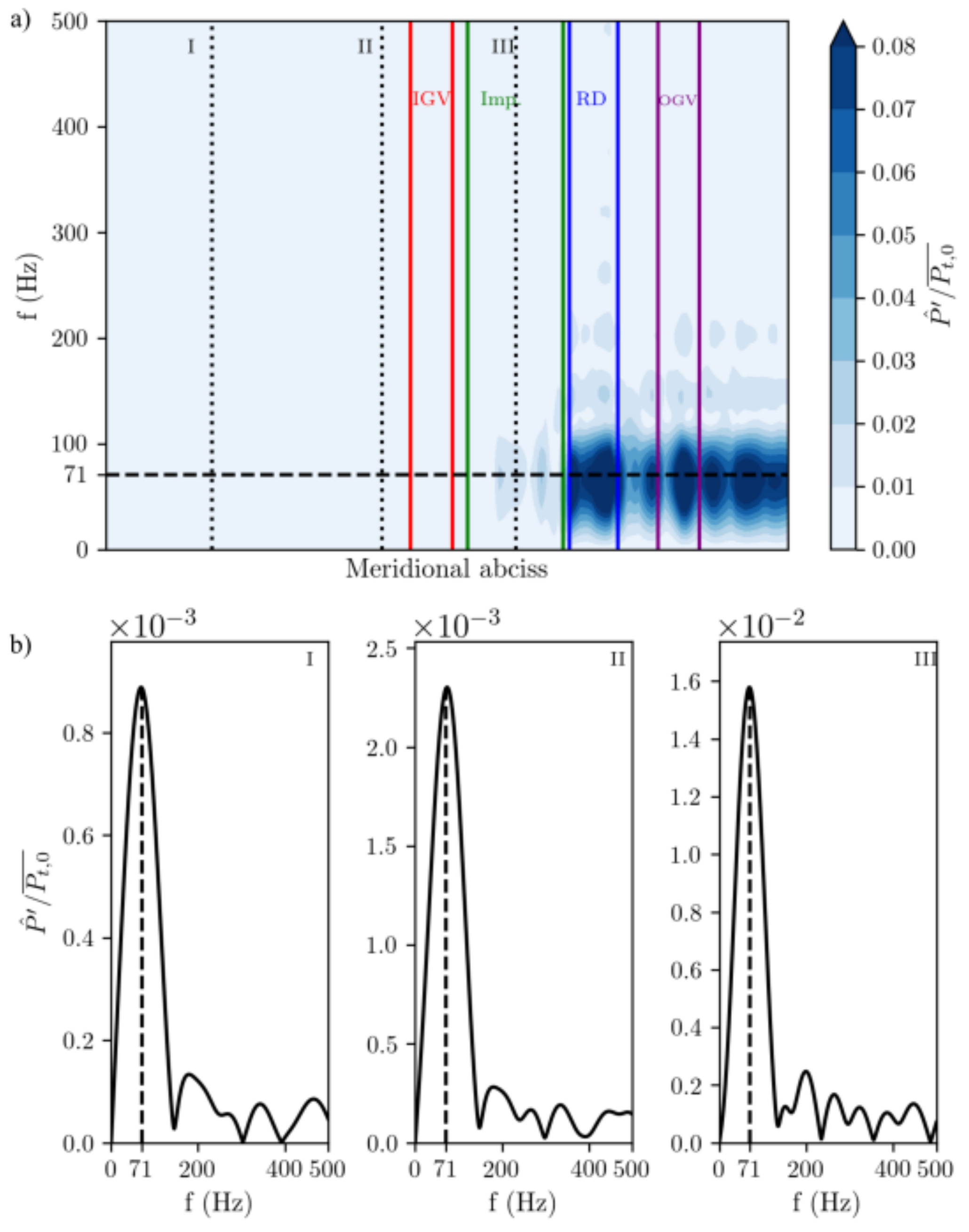
3.4.2. Reflection of Acoustic Waves on the Inlet and Outlet Planes
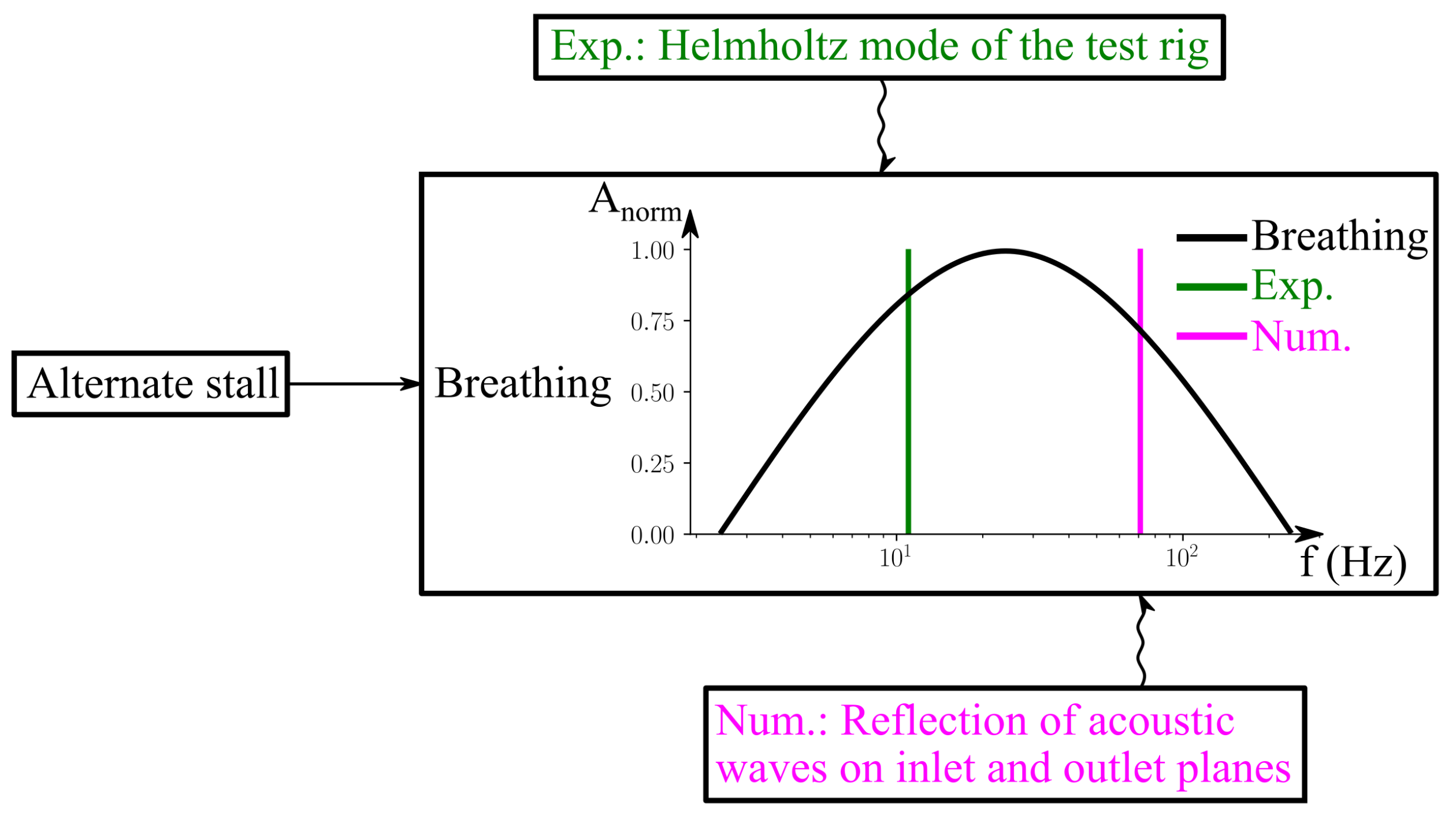
3.4.3. Two Different Resonators: Helmholtz Mode in the Experiments versus Acoustic Planar Mode in the Numerical Simulations
4. Discussion
- Variation of the IGV stagger angle only impacts the pulsation frequency of the alternate flow pattern in the tests. This is explained by the dependence of the Helmholtz frequency on the compressor inlet section.
- Thanks to a topological analysis of the average flow field (computed from URANS simulation) on two radial diffuser adjacent channels, it is observed that the SP (major critical point) in the corner hub/suction side of the stalled blade migrates upstream while staying in the corner if the mass flow rate decreases. One main blade over two is stalled on both sides because the flow originating from this corner separation circumvents the trailing edge and migrates upstream along the pressure side.
- Although the same phenomenon occurs in both the experiment and the numerical simulation, the pulsation frequency of the alternate flow pattern is different. The experimental pulsation is related to the Helmholtz mode of the compressor on the test rig. The numerical pulsation occurs with the reflection of acoustic waves on the inlet and the outlet planes of the simulated domain.
- A coupled CFD simulation, 1D for the test rig and 3D for the compressor stage, might predict the same frequency pulsation of the alternate flow as in the tests.
- URANS turbulence models are deemed to be inaccurate in stalled areas. Therefore, a wall-resolved Large Eddy Simulation (LES) might provide a better description of the flow in stalled areas. However, it should be noted that this simulation is not easily affordable because the mesh should contain at least several billions of cells.
- Finally, stall control using boundary layer suction might extend the operating range of the compressor. These tests will be carried out in 2022 within the framework of the European FLORA project.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Latin Symbols | |
| A | Alternate rate |
| Mass flow | |
| p | Pitch |
| P | Pressure |
| r | Radius |
| V | Absolute velocity |
| W | Relative velocity |
| Greek Symbols | |
| Pressure ratio | |
| IGV stagger angle | |
| Rotation speed | |
| Circumferential direction | |
| Subscripts | |
| ref | Reference (value) |
| std | Standard |
| 0 | IGV inlet |
| 1 | IMP inlet |
| 2 | RD inlet |
| 3 | RD outlet |
| Superscripts | |
| s-s | Static-to-static |
| t | Total |
| t-s | Total-to-static |
| Abbreviations | |
| C | Compressor (IGV + IMP + RD) |
| CCRT | Centre de Calcul Recherche et Technologie |
| FP | Focus Point |
| IGV | Inlet Guide Vane |
| IMP | Impeller |
| LES | Large Eddy Simulation |
| MS | Mild Surge |
| ONERA | Office National d’Etudes et de Recherches Aérospatiales |
| OGV | Outlet Guide Vane |
| RD | Radial Diffuser |
| RANS | Reynolds-Averaged Navier–Stokes |
| SafranHE | Safran Helicopter Engines |
| SP | Saddle Point |
| URANS | Unsteady Reynolds-Averaged Navier–Stokes |
Appendix A. Links between the Centrifugal and Coriolis Forces and the Work Input
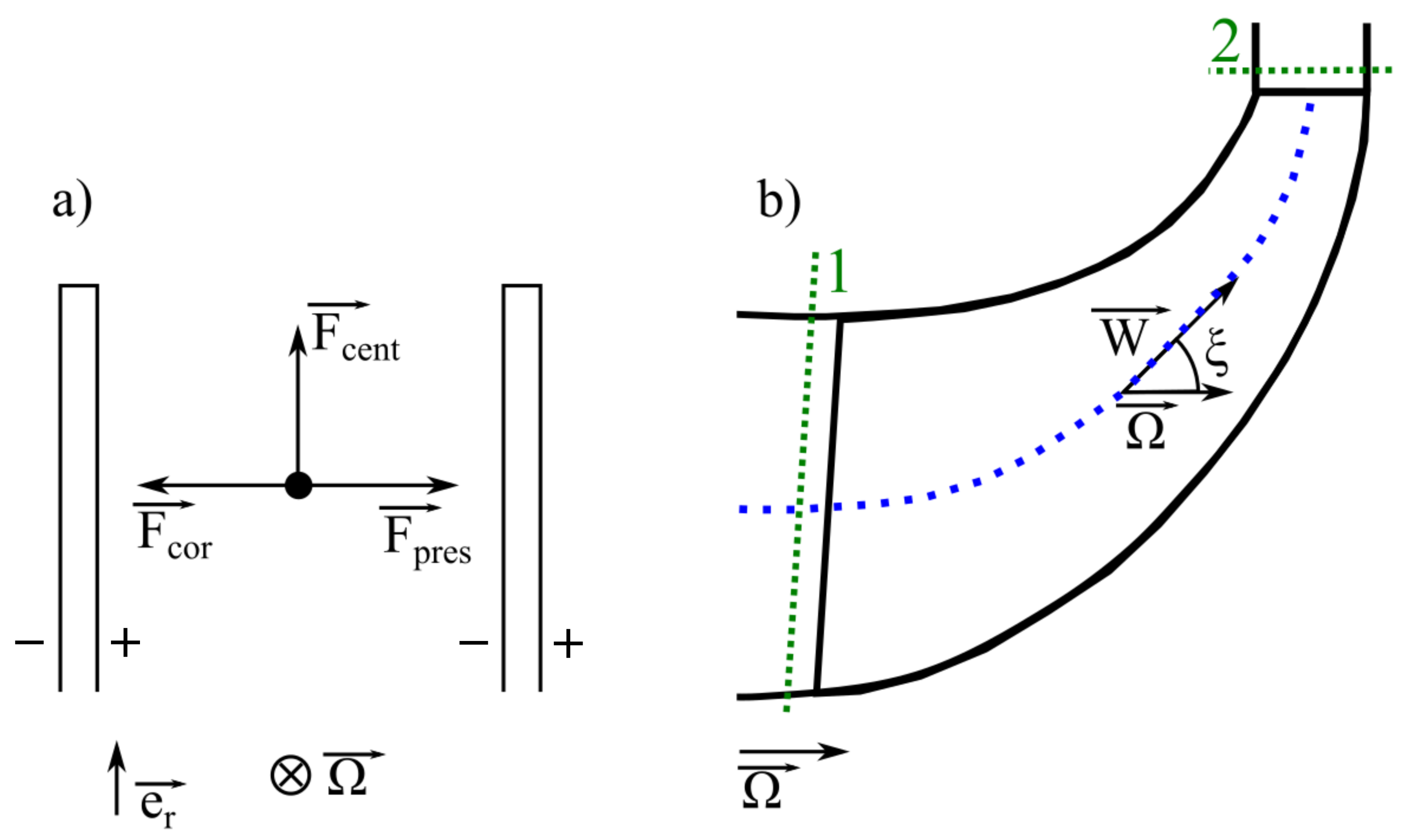
Appendix A.1. Analysis in the Circumferential Direction
Appendix A.2. Analysis in the Rotating Frame of Reference
Appendix A.3. Summary of Forces’ Roles
| Geometry Type | Work | Pressure Increase |
|---|---|---|
| Axial | Deflection | Decrease of relative velocity W |
| Centrifugal | Deflection and Coriolis force | Decrease of relative velocity W and centrifugal force |
References
- Fink, D.A.; Cumpsty, N.A.; Greitzer, E.M. Surge dynamics in a free-spool centrifugal compressor system. J. Turbomach. 1992, 114, 321–332. [Google Scholar] [CrossRef]
- Galindo, J.; Serrano, J.R.; Climent, H.; Tiseira, A. Experiments and modelling of surge in small centrifugal compressor for automotive engines. Exp. Therm Fluid Sci. 2008, 32, 818–826. [Google Scholar] [CrossRef]
- Zheng, X.; Liu, A. Phenomenon and mechanism of two-regime-surge in a centrifugal compressor. J. Turbomach. 2015, 137, 081007. [Google Scholar] [CrossRef]
- Moënne-Loccoz, V.; Trébinjac, I.; Poujol, N.; Duquesne, P. Detection and analysis of an alternate flow pattern in a radial vaned diffuser. Int. J. Turbomach. Propuls. Power 2020, 5, 2. [Google Scholar] [CrossRef] [Green Version]
- Moënne-Loccoz, V.; Trébinjac, I.; Poujol, N.; Duquesne, P. Low frequency stall modes of a radial vaned diffuser flow. Mech. Ind. 2019, 20, 805. [Google Scholar] [CrossRef]
- Sano, T.; Nakamura, Y.; Yoshida, Y.; Tsujimoto, Y. Alternate Blade Stall and Rotating Stall in a Vaned Diffuser. JSME Int. J. Ser. B Fluids Therm. Eng. 2002, 45, 810–819. [Google Scholar] [CrossRef] [Green Version]
- Pedersen, N.; Larsen, P.S.; Jacobsen, C.B. Flow in a Centrifugal Pump Impeller at Design and Off-Design Conditions—Part I: Particle Image Velocimetry (PIV) and Laser Doppler Velocimetry (LDV) Measurements. J. Fluids Eng. 2003, 125, 61–72. [Google Scholar] [CrossRef]
- Poujol, N.; Trébinjac, I.; Duquesne, P. Effects of Inlet Guide Vanes on the Performance and Stability of an Aeronautical Centrifugal Compressor. J. Turbomach. 2021, 143, 101010. [Google Scholar] [CrossRef]
- Cambier, L.; Gazaix, M.; Heib, S.; Plot, S.; Poinot, M.; Veuillot, J.P.; Boussuge, J.F.; Montagnac, M. An Overview of the Multi-Purpose elsAFlow Solver; Aerospace Lab.: Palaiseau, France, 2011; pp. 1–15. [Google Scholar]
- Délery, J. Three-Dimensional Separated Flow Topology: Critical Points, Separation Lines and Vortical Structures; John Wiley & Sons: Hoboken, NJ, USA, 2013. [Google Scholar]
- Duquesne, P.; Chanéac, J.; Mondin, G.; Dombard, J. Guidelines for flow separation analysis in turbomachinery. Int. J. Turbomach. Propuls. Power 2022. under review. [Google Scholar] [CrossRef]
- Benichou, E.; Trébinjac, I. Application of an analytical method to locate a mixing plane in a supersonic compressor. J. Energy Power Eng. 2015, 9, 91–101. [Google Scholar]
- Weiss, J.; Mohammed-Taifour, A.; Schwaab, Q. Unsteady Behavior of a Pressure-Induced Turbulent Separation Bubble. AIAA J. 2015, 53, 2634–2645. [Google Scholar] [CrossRef]
- Mohammed-Taifour, A.; Weiss, J. Unsteadiness in a large turbulent separation bubble. J. Fluid Mech. 2016, 799, 383–412. [Google Scholar] [CrossRef]
- Bontemps, T. Flottement Fan et Couplage Acoustique: Analyse et Modélisation. Ph.D. Thesis, École Centrale de Lyon, Écully, France, 2020. [Google Scholar]



| Boundary Condition | Type |
|---|---|
| Inlet | Stagnation pressure and temperature, velocity angles imposed |
| Outlet | Static pressure at mid-span calculated with a quadratic throttle law and completed by a radial equilibrium |
| Walls | Non-slip and adiabatic conditions |
| Azimuthal borders | Instantaneous periodicity |
| Rotor–Stator interfaces | Two successive 1D interpolations in radius and in azimuthal position |
| Numerical Parameter | Type |
| Spatial scheme | Second-order Roe scheme plus Harten’s entropic correction |
| Temporal scheme | First-order backward Euler scheme with around 10,000 steps by impeller revolution |
| Turbulence model | k-l model of Smith, , , |
| IGV Stagger Angle | |||
|---|---|---|---|
| 11.0 | 8.5 | 5.5 | |
| NA | 0.77 | 0.50 | |
| NA | 0.79 | 0.37 |
| Physical Quantity | Num. | Exp. |
|---|---|---|
| 3.4% | 6.6% | |
| 10% | 16% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY-NC-ND) license (https://creativecommons.org/licenses/by-nc-nd/4.0/).
Share and Cite
Poujol, N.; Buisson, M.; Duquesne, P.; Trébinjac, I. Unsteady Analysis of a Pulsating Alternate Flow Pattern in a Radial Vaned Diffuser. Int. J. Turbomach. Propuls. Power 2022, 7, 23. https://doi.org/10.3390/ijtpp7030023
Poujol N, Buisson M, Duquesne P, Trébinjac I. Unsteady Analysis of a Pulsating Alternate Flow Pattern in a Radial Vaned Diffuser. International Journal of Turbomachinery, Propulsion and Power. 2022; 7(3):23. https://doi.org/10.3390/ijtpp7030023
Chicago/Turabian StylePoujol, Nicolas, Martin Buisson, Pierre Duquesne, and Isabelle Trébinjac. 2022. "Unsteady Analysis of a Pulsating Alternate Flow Pattern in a Radial Vaned Diffuser" International Journal of Turbomachinery, Propulsion and Power 7, no. 3: 23. https://doi.org/10.3390/ijtpp7030023
APA StylePoujol, N., Buisson, M., Duquesne, P., & Trébinjac, I. (2022). Unsteady Analysis of a Pulsating Alternate Flow Pattern in a Radial Vaned Diffuser. International Journal of Turbomachinery, Propulsion and Power, 7(3), 23. https://doi.org/10.3390/ijtpp7030023






