Ground Strength Test Technique of Variable-Camber Wing Leading Edge
Abstract
1. Introduction
2. Variable-Camber Wing Shape Theory
2.1. Theoretical Model of Variable-Camber Wings
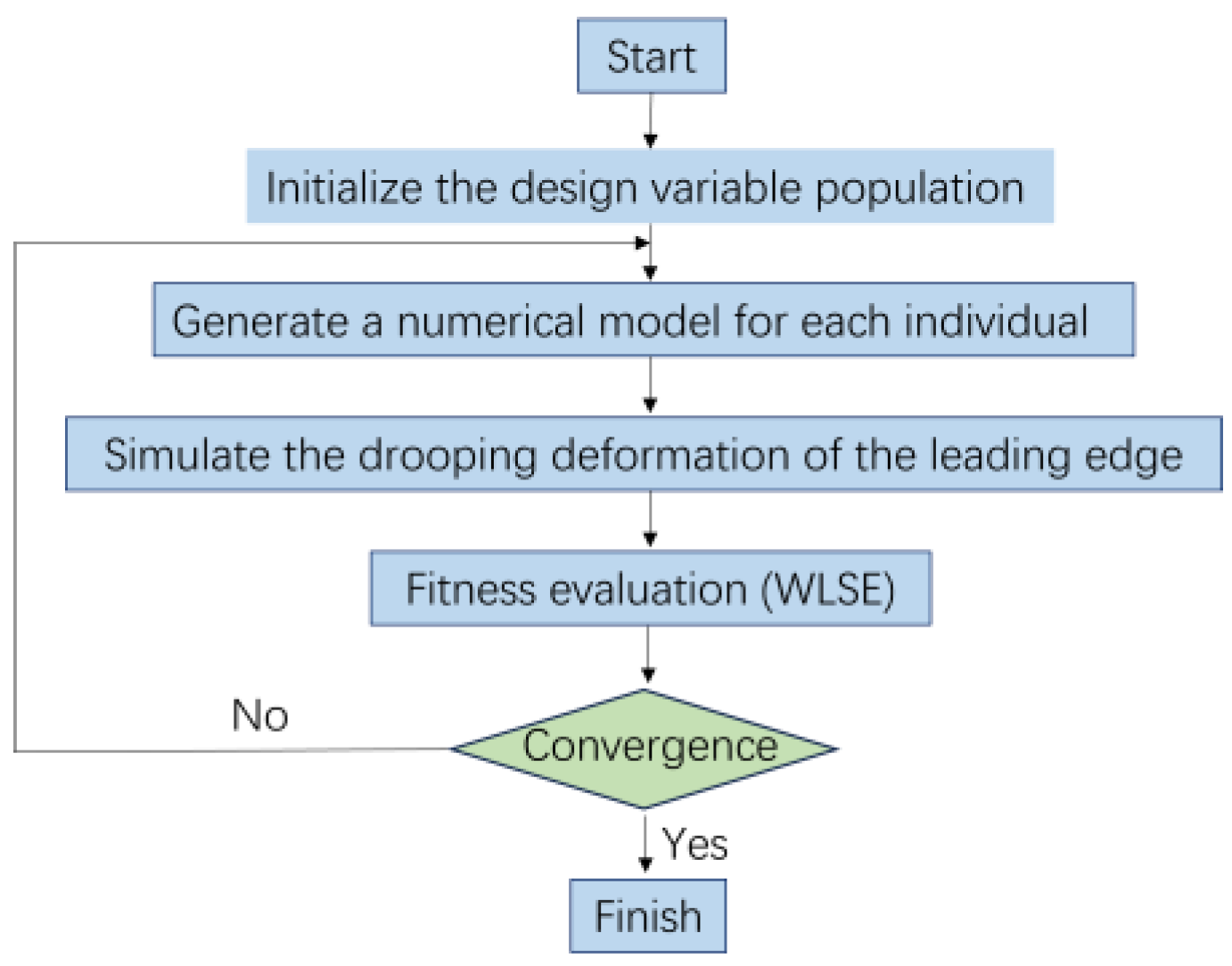
2.2. Theoretical Model Solution Algorithm
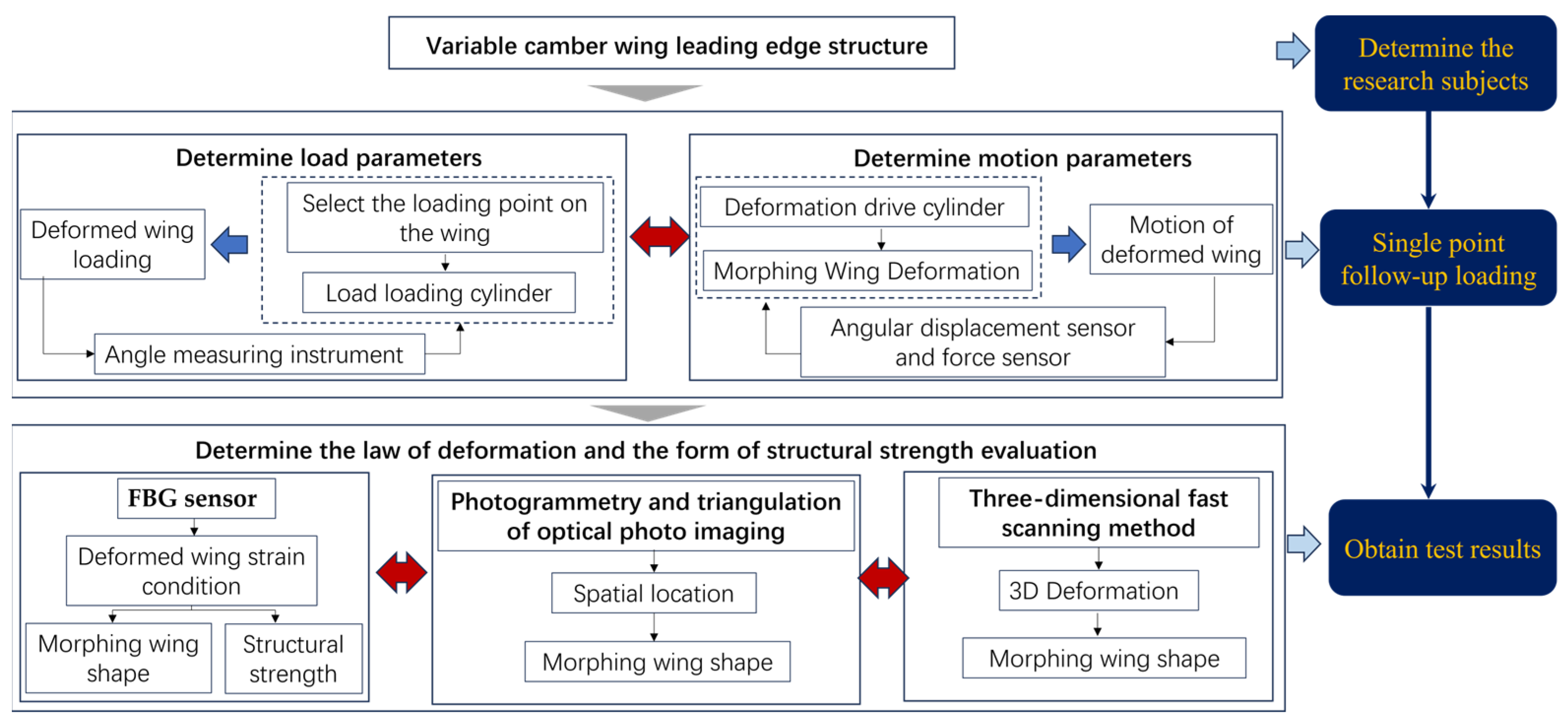
3. Test Scheme Design
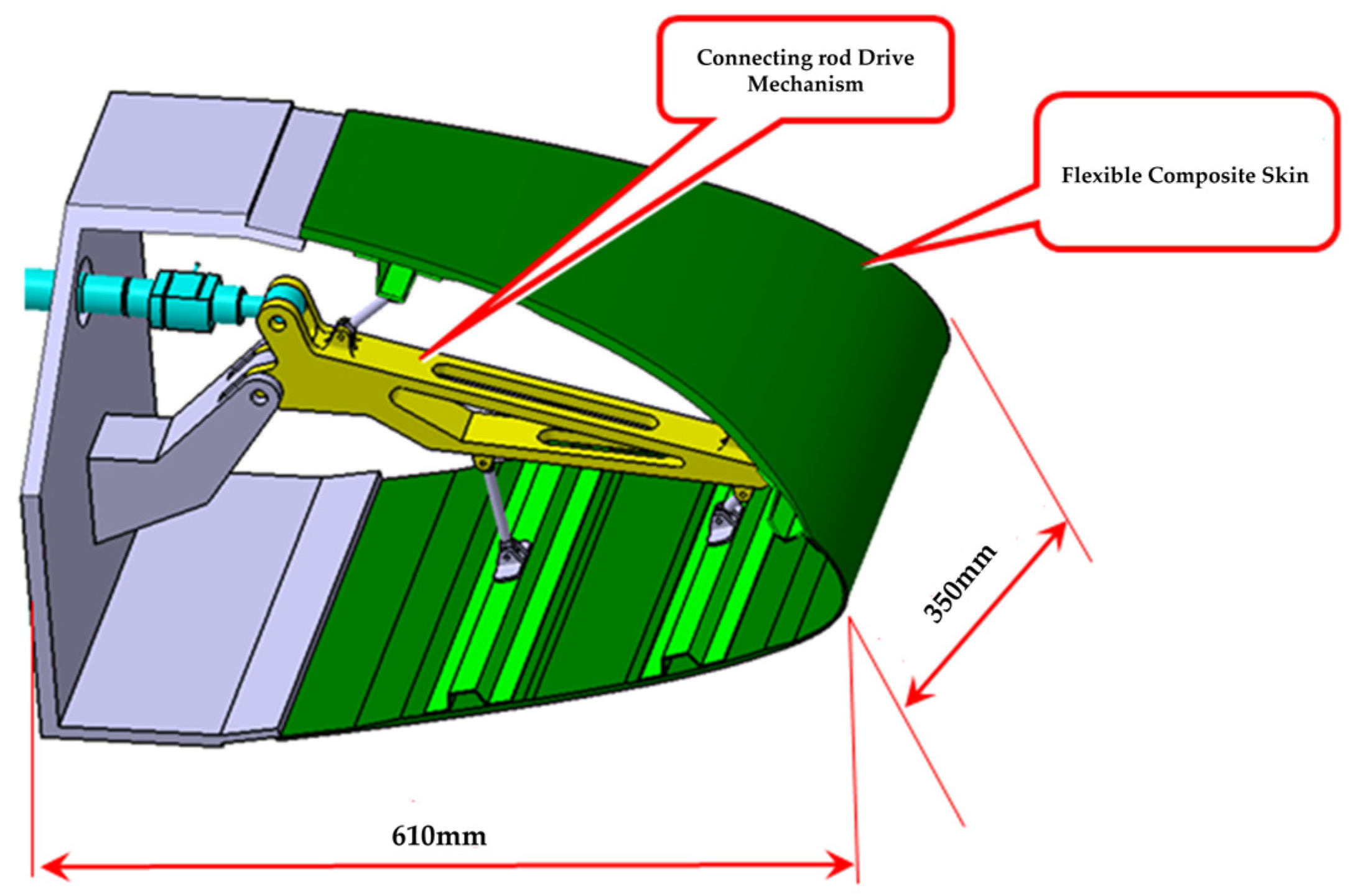
3.1. Study Subjects
3.2. Experimental Device Design
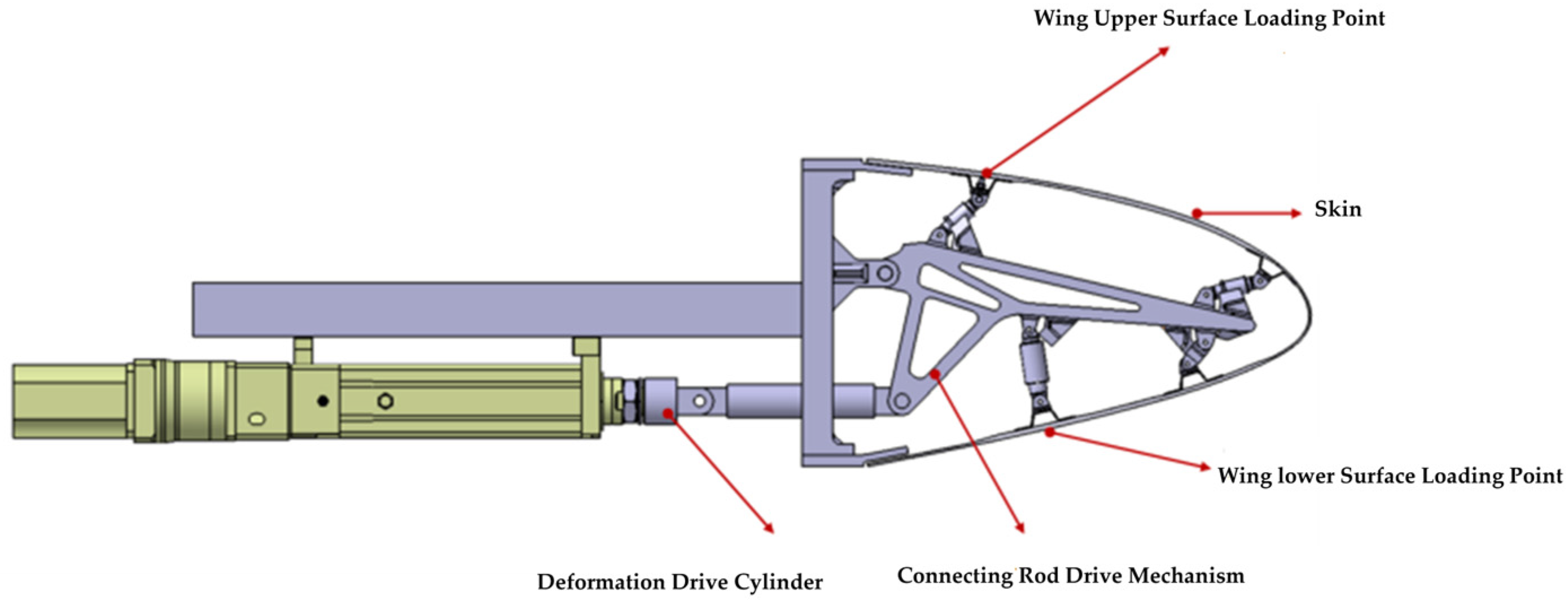
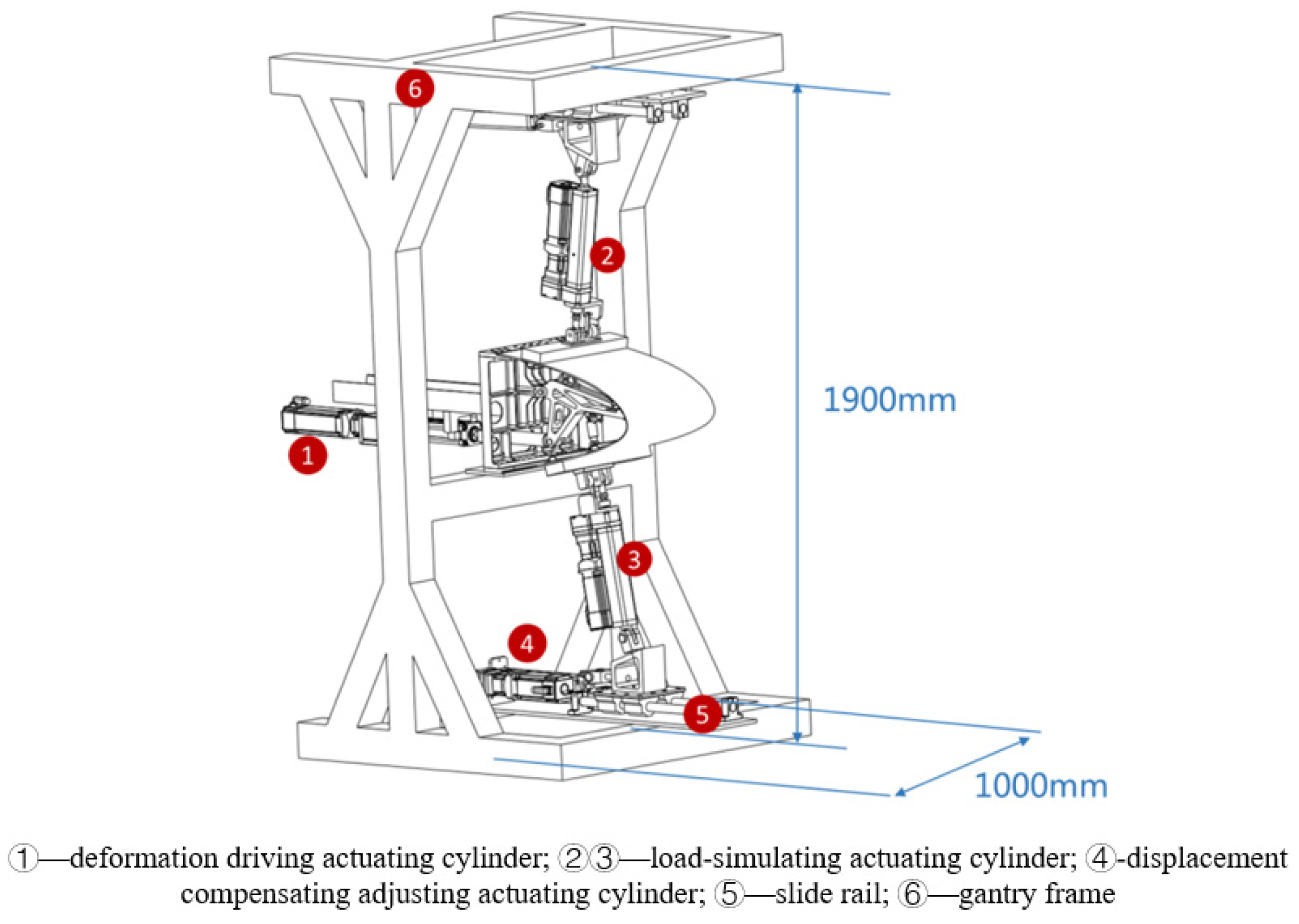
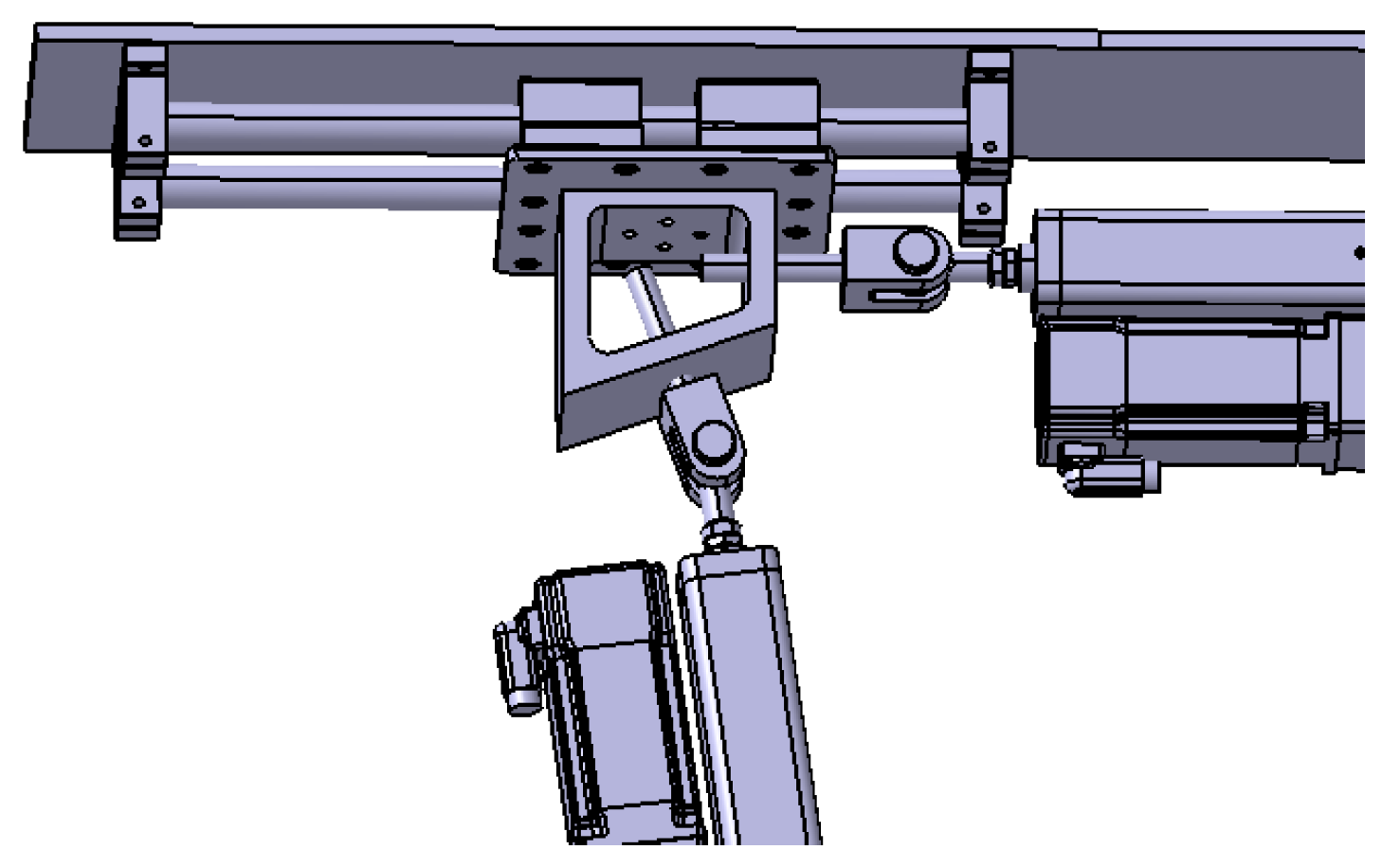
3.2.1. Experimental Loading Device Design
3.2.2. Loading Control Equipment Design
3.3. Experimental Monitoring Equipment Design
4. Leading Edge Strength Test of Variable-Camber Wing
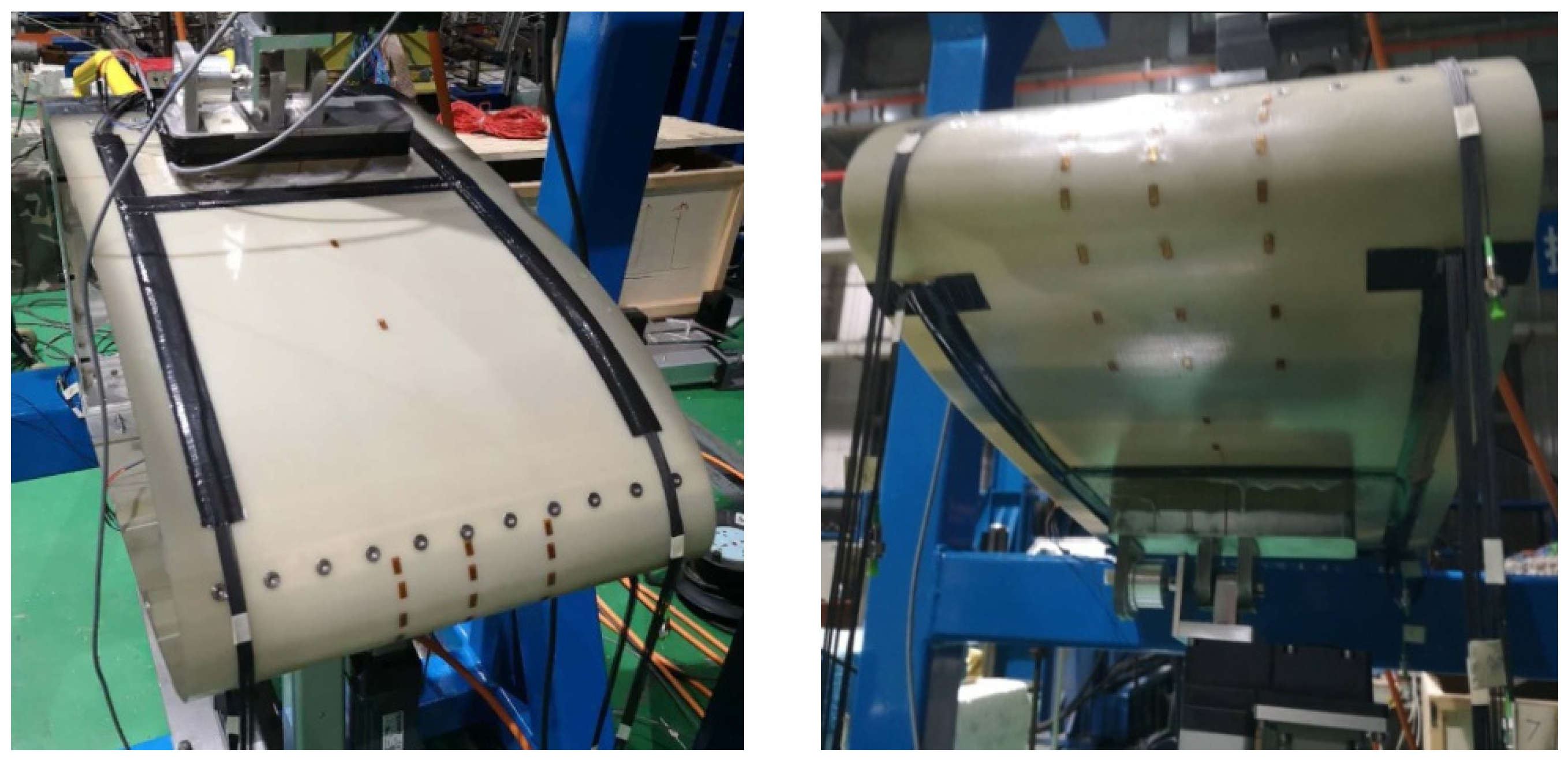
4.1. Test Piece
4.2. Test Load
4.3. Test Results of Loading Condition
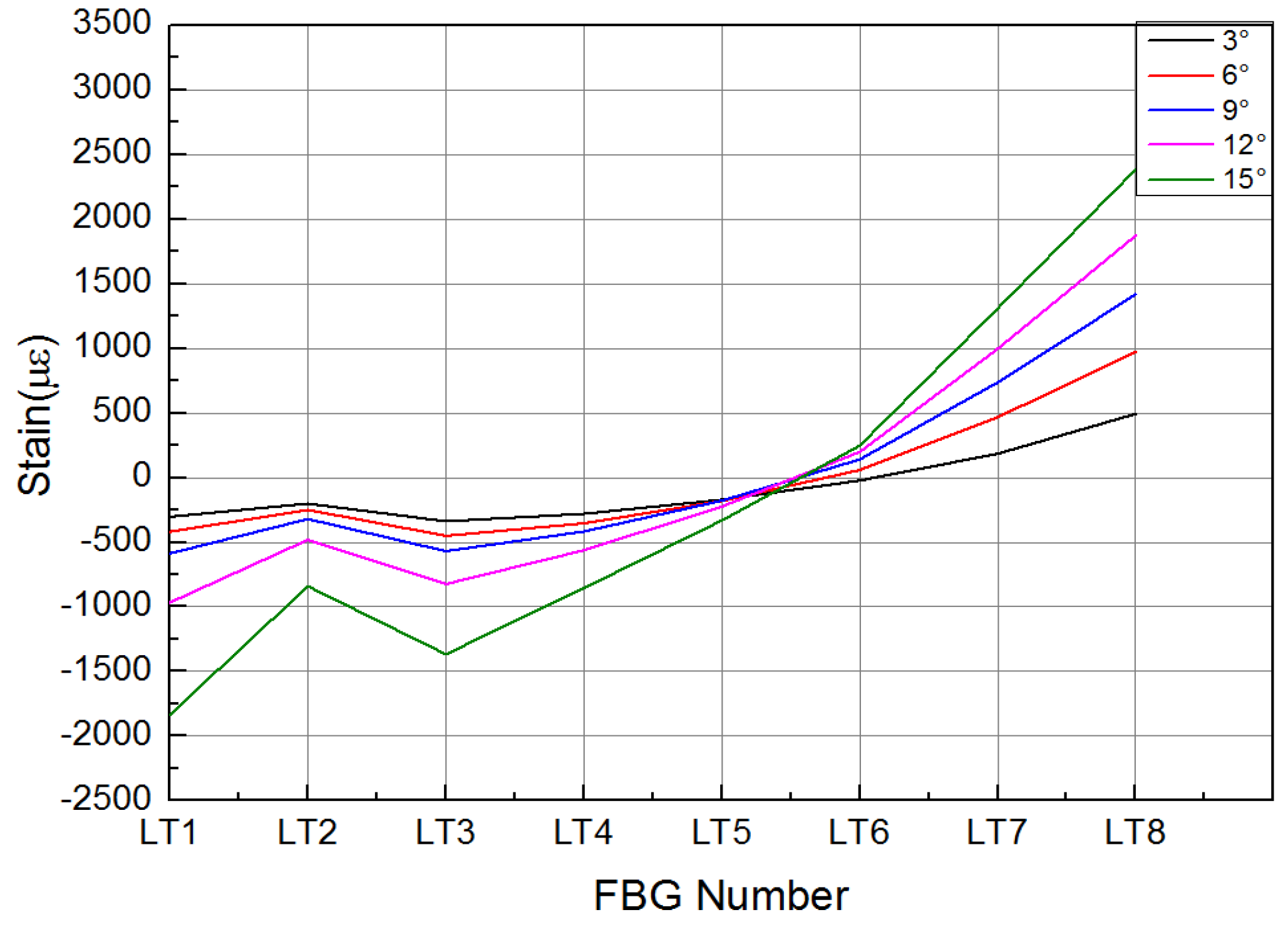
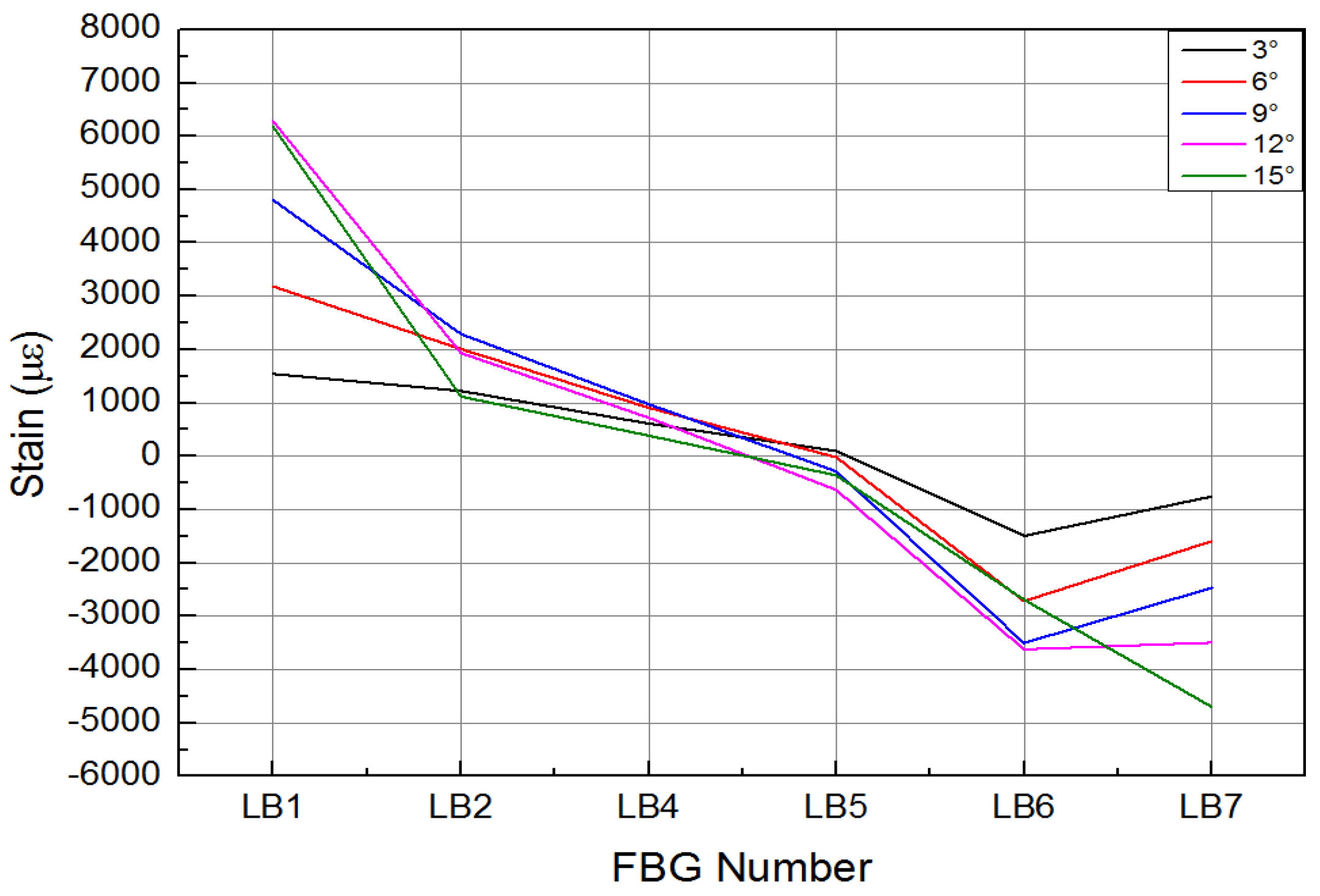
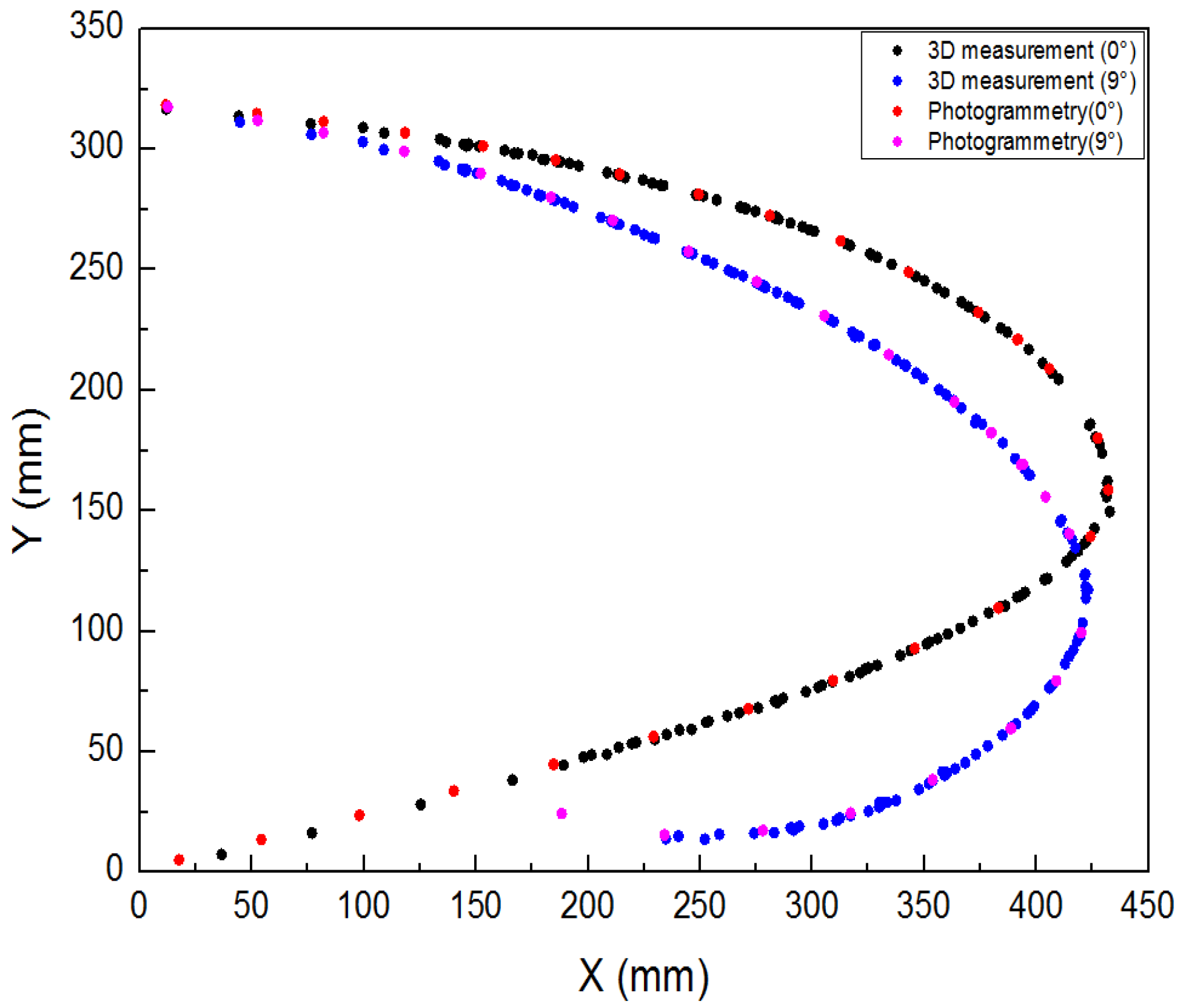
4.4. Test Results and Analysis
5. Conclusions
- The variable-camber wing leading edge is an aeronautical design that mimics the continuous and smooth form of a bird’s wings. In response to the variable-camber wing, this paper introduces a ground-based strength testing technique for the variable-camber wing leading edge. This technique effectively fills the technical gap in monitoring full-scale variable-camber wing leading edges under flight conditions, providing technical support for the testing and evaluation of the leading edge.
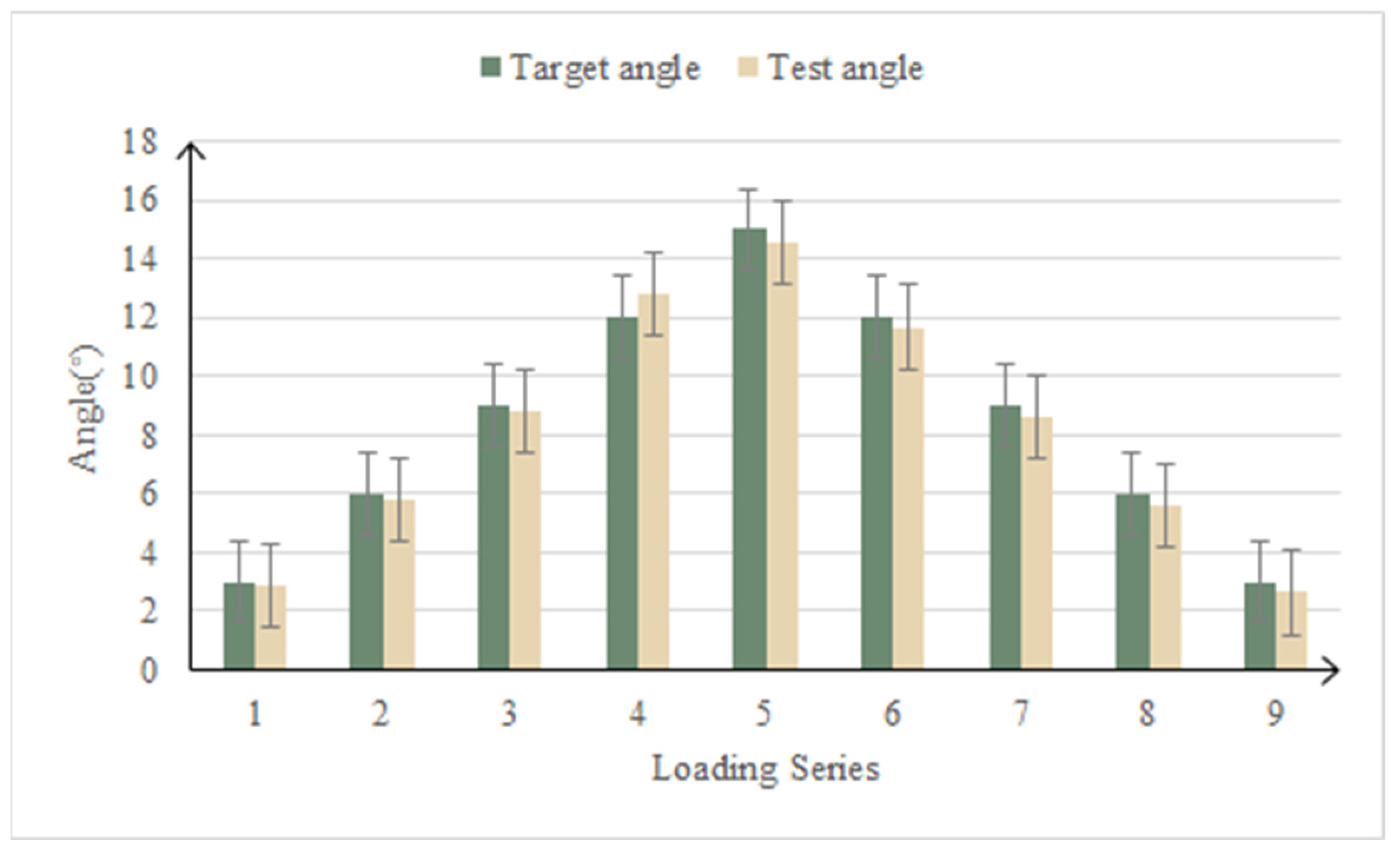
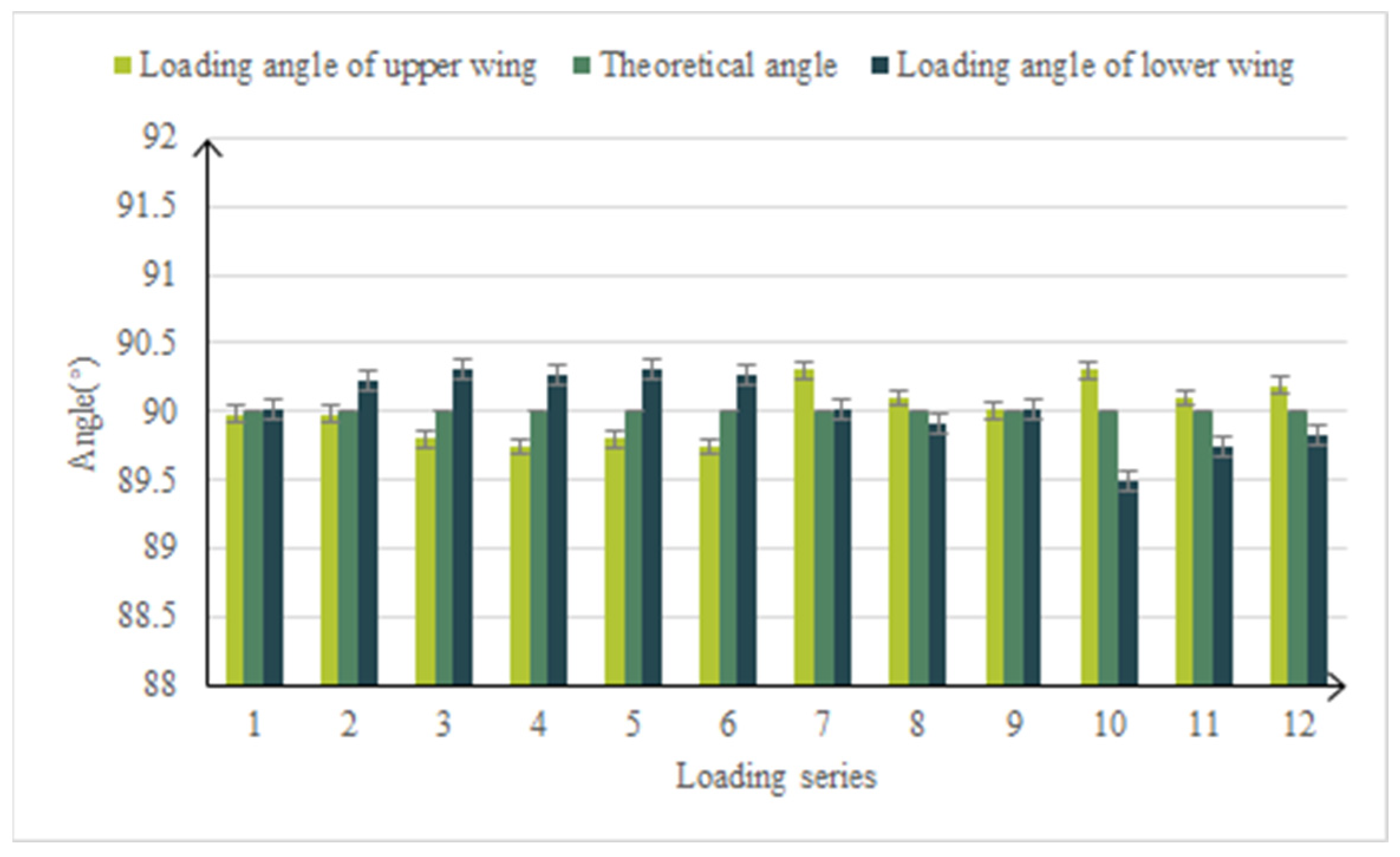
- The deformation process of the leading edge of a full-scale variable-camber wing under real flight load and drive load was accurately simulated. The test results show that the motion function and bearing capacity of the leading edge structure meet the design requirements, and the average deflection angle error is 4.59%.
- A multi-point cooperative control system with precise control, fast response, and stable operation was developed. The feedback results show that the control frequency of the system is as high as 1000 Hz, and the average error of the applied load magnitude and direction is 0.54% and 0.24%, respectively.
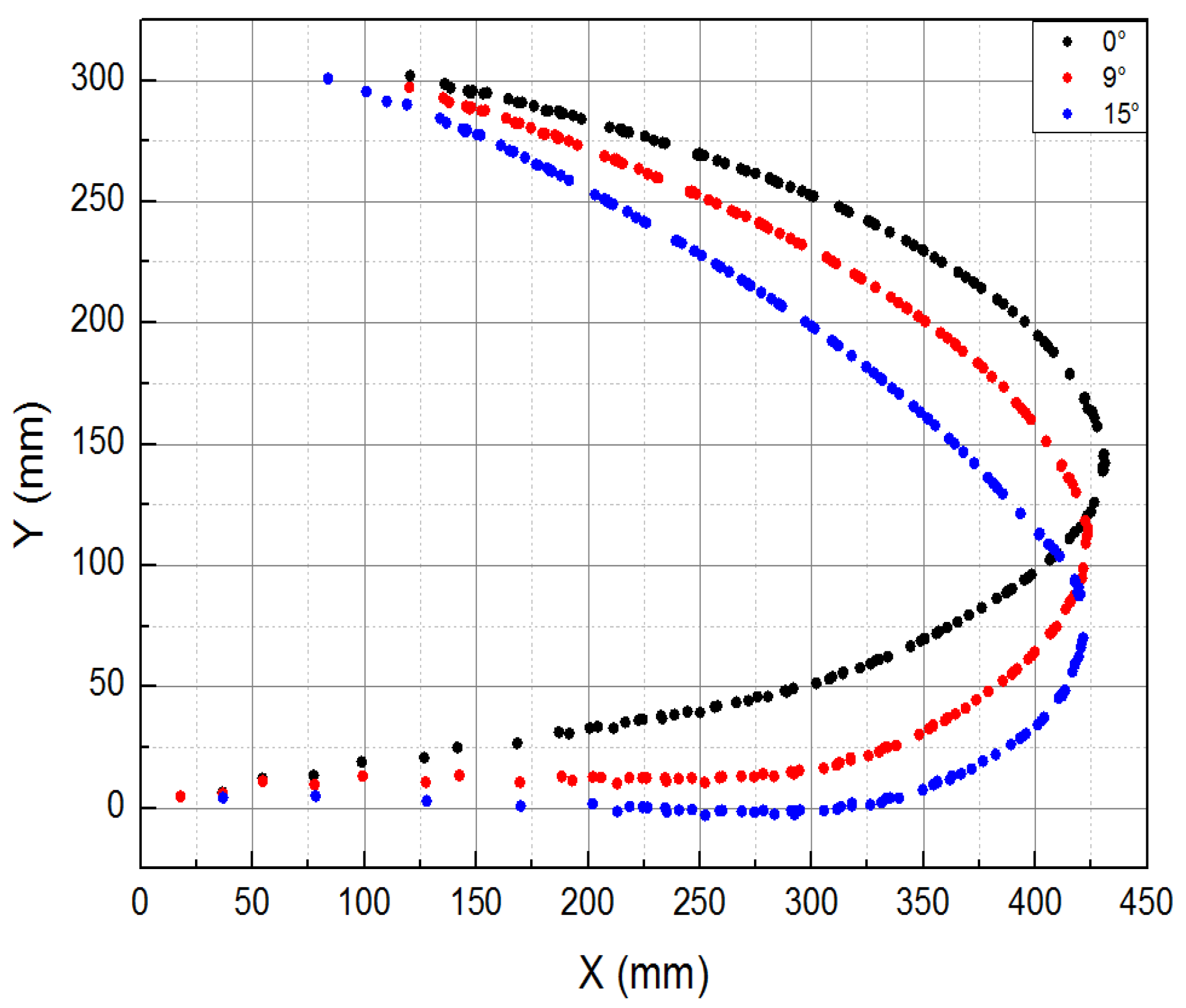
- The distributed sensor monitoring network was reasonably designed to ensure that the entire motion process of the leading edge can be measured and controlled. The measurement results show that the maximum error between the actual deformation and the theoretical deformation is less than 10 mm, and the design target deflection angle was realized.
- While the present study has provided valuable technical support for the testing and evaluation of variable-camber wing leading edges, there are limitations due to manufacturing processes that have restricted the experimental techniques to full-scale measurements in the chordwise direction only, without encompassing the full spanwise extent. Consequently, to more effectively assess the condition of the variable-camber wing system, future endeavors could explore the implementation of distributed control systems to conduct ground strength tests that encompass both the full spanwise and chordwise dimensions.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Li, X.; Zhang, M.; Wang, W.; Zhong, M.; Wang, Z.; Zhou, J.; Xue, J. Research on Variable Camber Wing Technology Development. Aeronaut. Sci. Technol. 2020, 31, 12–24. [Google Scholar] [CrossRef]
- Ni, Y.G.; Yang, Y. Research on the status and key technology in morphing airfoil of adaptive wings. Adv. Aeronatical Sci. Eng. 2018, 9, 297–308. [Google Scholar] [CrossRef]
- Leng, J.S.; Sun, J.; Liu, Y.J. application status and future. Acta Aeronaut. Astronaut. Sin. 2014, 35, 29–45. [Google Scholar]
- Nie, R. Research on Key Technologies of Morphing Wing Structure. Ph.D. Thesis, Nanjing University of Aeronautics and Astronautics, Nanjing, China, 2020. [Google Scholar] [CrossRef]
- Hoa, S.; Abdali, M.; Jasmin, A.; Radeschi, D.; Prats, V.; Faour, H.; Kobaissi, B. Development of a New Flexible Wing Concept for Unmanned Aerial Vehicle Using Corrugated Core Made by 4D Printing of Composites. Compos. Struct. 2022, 290, 115444. [Google Scholar] [CrossRef]
- Sun, J.; Du, L.; Scarpa, F.; Liu, Y.; Leng, J. Morphing Wingtip Structure Based on Active Inflatable Honeycomb and Shape Memory Polymer Composite Skin: A Conceptual Work. Aerosp. Sci. Technol. 2021, 111, 106541. [Google Scholar] [CrossRef]
- Ren, X.; Zhu, G. A Variable Stiffness Morphing Skin: Preparation and Properties. Smart Mater. Struct. 2021, 30, 125016. [Google Scholar] [CrossRef]
- Xu, Y.; Qiu, L.; Yuan, S. Fabrication and Actuation Performance of Selective Laser Melting Additive-Manufactured Active Shape-Memory Alloy Honeycomb Arrays. Actuators 2022, 9, 242. [Google Scholar] [CrossRef]
- Li, T.; Sun, J.; Leng, J.S.; Liu, Y. In-Plane Mechanical Properties of a Novel Cellular Structure for Morphing Applications. Compos. Struct. 2023, 305, 116482. [Google Scholar] [CrossRef]
- Yazik, M.H.M.; Sultan, M.T.H. Shape memory polymer and its composites as morphing materials. In Failure Analysis in Biocomposites, Fibre-Reinforced Composites and Hybrid Composites; Woodhead Publishing: Sawston, UK, 2019; pp. 181–197. [Google Scholar]
- Zhang, X.X.; Yan, B.X.; Sun, G.K.; Song, Y. The Design of Flexible Deformation Wing and Structural Mechanical Property Analysis. In Machinery Design & Manufacture; Tongfang Knowledge Network Technology Co., Ltd.: Beijing, China, 2020; pp. 163–166. [Google Scholar] [CrossRef]
- Zhao, A.M.; Liu, D.S.; Su, Y.; Zou, H. Design and Numerical Simulation of an Innovation Overall Variable Camber Wing. Aeronaut. Comput. Technol. 2018, 48, 111–113+121. [Google Scholar]
- Contell Asins, C.; Landersheim, V.; Laveuve, D.; Adachi, S.; May, M.; Wacker, J.-D.; Decker, J. Analysis and Design of a Leading Edge with Morphing Capabilities for the Wing of a Regional Aircraft—Gapless Chord- and Camber-Increase for High-Lift Performance. Appl. Sci. 2021, 11, 2752. [Google Scholar] [CrossRef]
- Zhang, P.; Zhou, L.; Qiu, T. Mechanical property analysis and structural design of flexible skin based on deformable honeycomb. Chin. J. Solid Mech. 2013, 34, 433–440. [Google Scholar] [CrossRef]
- Liu, L.B. Mechanical Analysis of Trailing Adge with Morphing Skin. Master’s Thesis, Harbin Institute of Technology, Harbin, China, 2012. [Google Scholar]
- Wang, B.W.; Yang, Y.; Qian, Z.S.; Wang, Z.G.; Lyu, S.S.; Sun, X.S. Technical development of variable camber wing: Review. Acta Aeronaut. Et Astronaut. Sin. 2022, 43, 024943. [Google Scholar]
- Martins, J.R.R.A. Fuel burn reduction through wing morphing. Green Aviat. 2016, 9, 73. [Google Scholar]
- Santos, P.; Sousa, J.; Gamboa, P. Variable-span wing development for improved flight performance. J. Intell. Mater. Syst. Struct. 2017, 28, 961–978. [Google Scholar] [CrossRef]
- Xiao, G.H.; Zhou, G.F.; Li, F.G.; Tu, J.Q.; Wang, C.Y. Study on the deformation strategy of reconfigurable lift wing missile based on ballistic simulation. Flight Dyn. 2022, 40, 70–75. [Google Scholar]
- Sadique, M.; Ajaj, R.M.; Khan, K.A. Acompliant polymorphing wing for small UAVs. Chin. J. Aeronaut. 2020, 33, 2575–2588. [Google Scholar]
- Sui, T.; Xu, Z.W. Study on Multi-motor Distributed Driving System of Morphing Wing with Flexible Skin. Mech. Sci. Technol. Aerosp. Eng. 2023, 42, 1551–1558. [Google Scholar]
- Jerković Štil, V.; Varga, T.; Benši, T.; Barukčić, M. A survey of fuzzy algorithms used in multi-motor systems control. Electronics 2020, 9, 1788. [Google Scholar] [CrossRef]
- Geng, Q.; Wang, S.W.; Zhou, Z.Q.; Shi, T.N.; Xia, C.L. Multi-motor speed synchronous control based on improved relative coupling structure. Trans. China Electrotech. Soc. 2019, 34, 474–482. [Google Scholar]
- Shang, X.D.; Liu, Y.L.; Ma, L.T.; Chen, Y.W. Research on coordinated control strategy of multi-motor system based on fuzzy PID. Autom. Instrum. 2021, 13–17. [Google Scholar]
- Zhou, G.F.; Hou, B.C.; Yang, J.H.; Wu, Y.F. Multi-motor control algorithm based on dynamic compensation. Acta Aeronaut. Et Astronaut. Sin. 2020, 41, 723771. [Google Scholar]
- Lyu, S.S.; Wang, B.W.; Yang, Y.; Wang, Z.G. Normal optimization design of flexible skin of airfoil based on genetic algorithm. Chin. J. Appl. Mech. 2020, 37, 617–623. [Google Scholar]
- Lyu, S.S.; Wang, B.W.; Yang, Y. Optimal design of flexible skin on the leading edge of a 3D variable-camber wing. Appl. Math. Mech. 2020, 41, 604–614. [Google Scholar]
- Kotas, S. Future Airplanes Will Fly On Twistable Wings. IEEE Spectrum, 10 December 2015. Available online: https://spectrum.ieee.org/future-airplanes-will-fly-on-twistable-wings (accessed on 1 April 2024).
- Sinapius, M.; Monner, H.P.; Kintscher, M.; Riemenschneider, J. DLR’s morphing wing activities within the European net-work. Procedia IUTAM 2014, 10, 416–426. [Google Scholar] [CrossRef]
- Fortin, F. Shape Optimization of a Stretchable Drooping Leading Edge. In Proceedings of the AIAA Scitech 2019 Forum, San Diego, CA, USA, 7–11 January 2019; AIAA: Reston, Virginia, 2019; p. 2352. [Google Scholar]
- Deb, K.; Pratap, A.; Agarwal, S.; Meyarivan, T.A.M.T. A fast and elitist multi-objective genetic algorithm: NSGA-II. IEEE Trans. Evol. Comput. 2002, 6, 182–197. [Google Scholar] [CrossRef]
- Rudenko, A.; Hannig, A.; Monner, H.P.; Horst, P. Extremely deformable morphing leading edge: Optimization, design and structural testing. J. Intell. Mater. Syst. Struct. 2017, 29, 764–773. [Google Scholar] [CrossRef]
- Vasista, S.; Riemenschneider, J.; Keimer, R.; Monner, H.; Nolte, F.; Horst, P. Morphing Wing Droop Nose with Large Deformation: Ground Tests and Lessons Learned. Aerospace 2019, 6, 111. [Google Scholar] [CrossRef]
- Vasista, S.; Riemenschneider, J.; Monner, H.; Nolte, F.; Horst, P. Manufacture and Testing of a Large-displacement Droop-Nose Morphing Wing Leading Edge. In Proceedings of the AIAA SCITECH 2019 Forum, San Diego, CA, USA, 7–11 January 2019. [Google Scholar] [CrossRef]
- Noviello, M.C.; Pecora, R.; Amoroso, F.; Rea, F.; Arena, M.; Dimino, I. Experimental shape reconstruction of a morphing wing trailing edge in simulated operative conditions. In Proceedings of the 8th International Conference on Mechanical and Aerospace Engineering, Prague, Czech Republic, 22–25 July 2017; pp. 249–256. [Google Scholar] [CrossRef]
- Nie, R.; Qiu, H.J.; Ji, H.L. Flexible skins theoretical and experimental research for one-dimensional wing morphing. Sci. Technol. Eng. 2017, 17, 115–121. [Google Scholar]
- Chen, H.; Wang, B.F.; Lu, J.Y.; Hao, Y.F. Shape optimization and test analysis of flexible skin for morphing wing. Ordnance Mater. Sci. Eng. 2013, 36, 31–34. [Google Scholar] [CrossRef]
- Radespiel, R.; Heinze, W. SFB 880: Fundamentals of high lift for future commercial aircraft. CEAS Aeronaut. J. 2014, 5, 239–251. [Google Scholar] [CrossRef]
- Shi, X.; Yang, Y.; Ge, W.; Wang, Z.; Sun, X. Structure design of variable camber wing trailing edge based on multi-block rotating mechanism. J. Northwestern Polytech. Univ. 2023, 41, 942–949. [Google Scholar] [CrossRef]
- Li, Y.; Pang, B.C.; Zhou, T. Research on the Test Technology of Trailing Edge Structure of Variable Camber Wing. Eng. Test 2024, 64, 42–47. [Google Scholar]




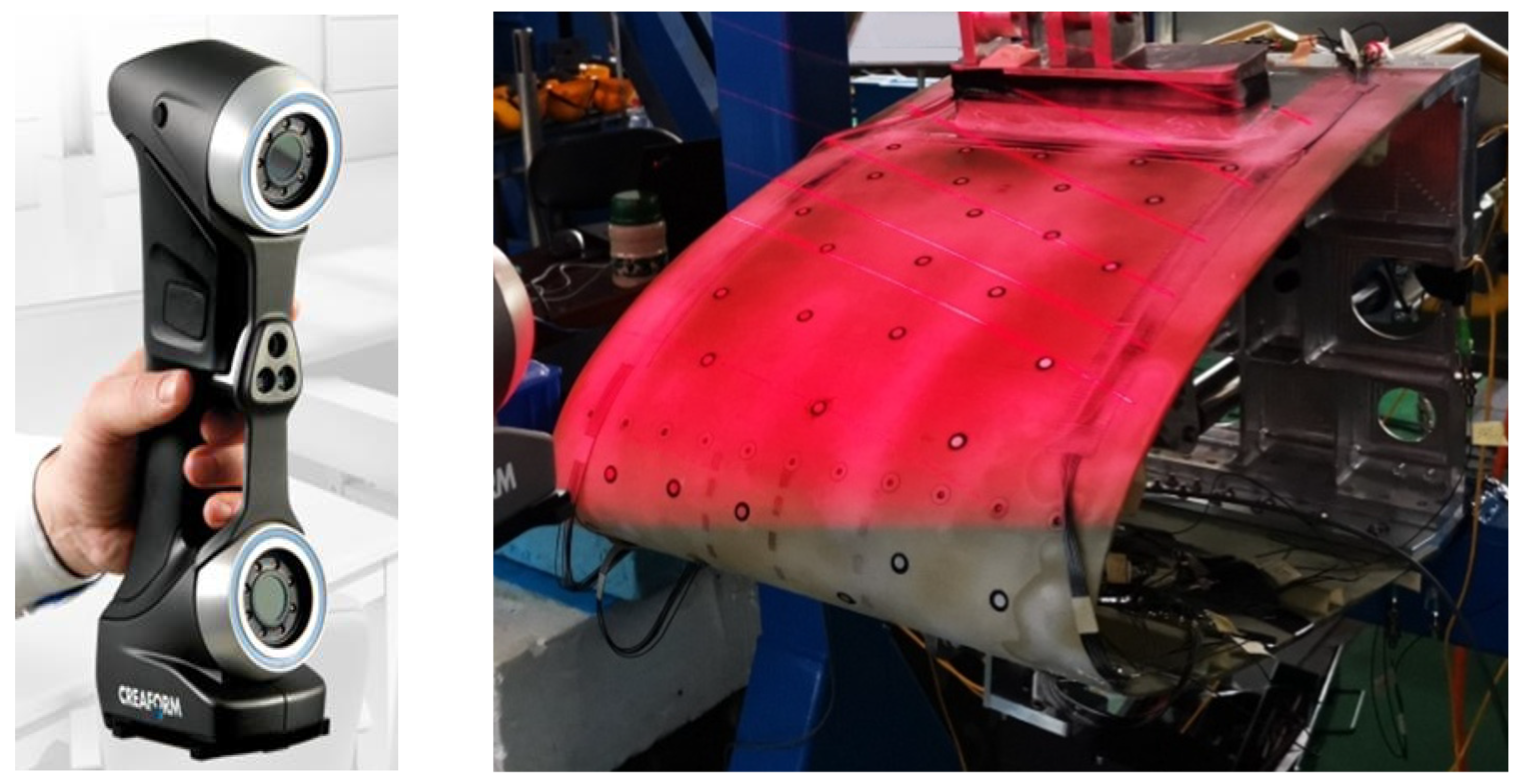




| Structure Deflection Angle (°) | Deformation-Driving Actuating Cylinder Displacement (mm) | Wing Upper Surface Test Load (n) | Wing Lower Surface Test Load (n) |
|---|---|---|---|
| 0 | 0 | −1201.26 | 61.28 |
| 3 | −7.30 | −888.99 | 90.39 |
| 6 | −14.63 | −943.49 | 92.079 |
| 9 | −21.96 | −946.21 | 104.27 |
| 12 | −29.26 | −945.18 | 106.95 |
| 15 | −36.35 | −944.15 | 109.63 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, S.; Chen, X.; Wang, Z.; Liang, Y. Ground Strength Test Technique of Variable-Camber Wing Leading Edge. Biomimetics 2024, 9, 467. https://doi.org/10.3390/biomimetics9080467
Li S, Chen X, Wang Z, Liang Y. Ground Strength Test Technique of Variable-Camber Wing Leading Edge. Biomimetics. 2024; 9(8):467. https://doi.org/10.3390/biomimetics9080467
Chicago/Turabian StyleLi, Shanshan, Xianmin Chen, Zhigang Wang, and Yuanbo Liang. 2024. "Ground Strength Test Technique of Variable-Camber Wing Leading Edge" Biomimetics 9, no. 8: 467. https://doi.org/10.3390/biomimetics9080467
APA StyleLi, S., Chen, X., Wang, Z., & Liang, Y. (2024). Ground Strength Test Technique of Variable-Camber Wing Leading Edge. Biomimetics, 9(8), 467. https://doi.org/10.3390/biomimetics9080467








