Ultimate Strength Study of Structural Bionic CFRP-Sinker Bolt Assemblies Subjected to Preload under Three-Point Bending
Abstract
1. Introduction
2. Experimental Approach
2.1. Experimental Tests
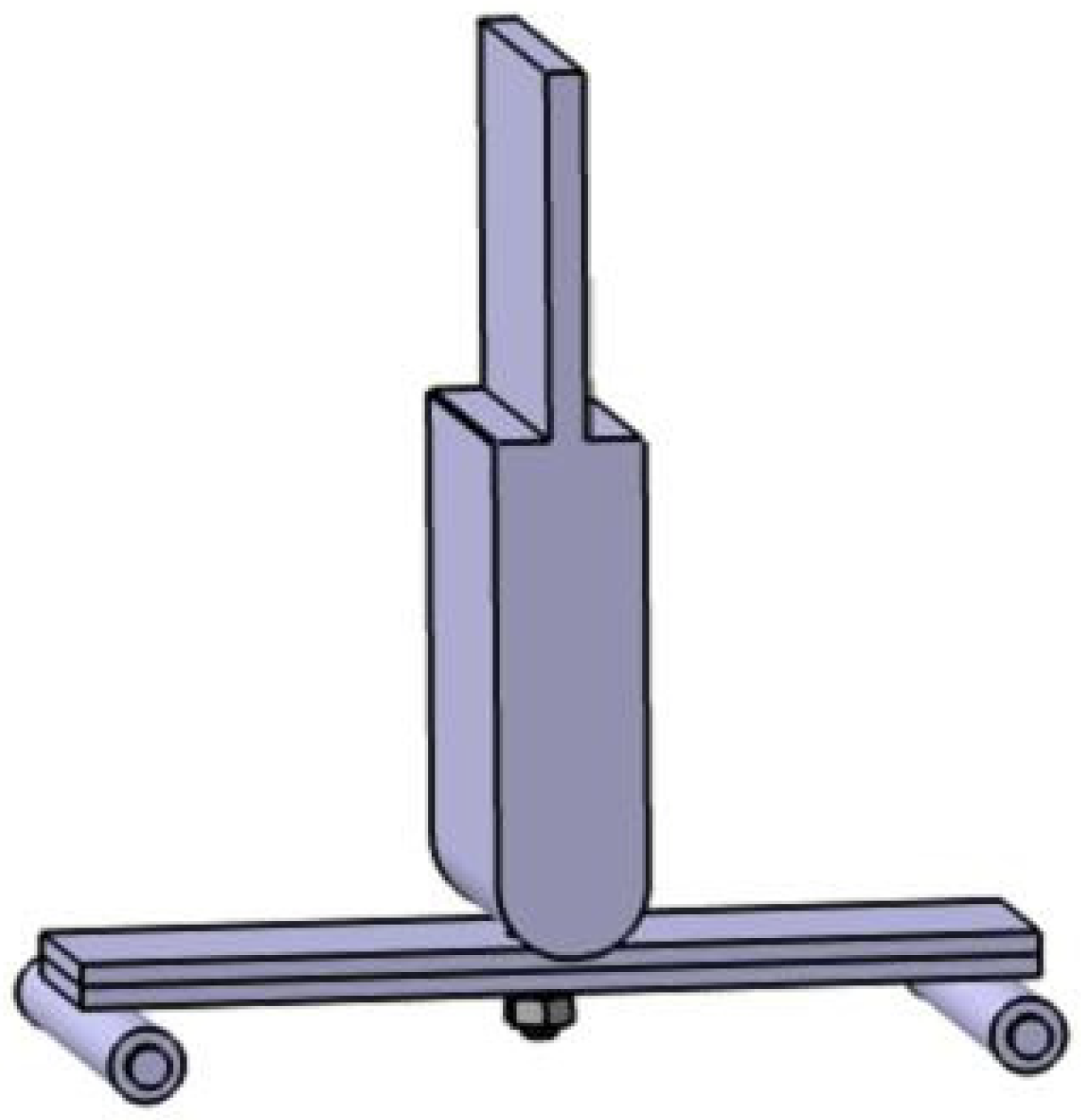
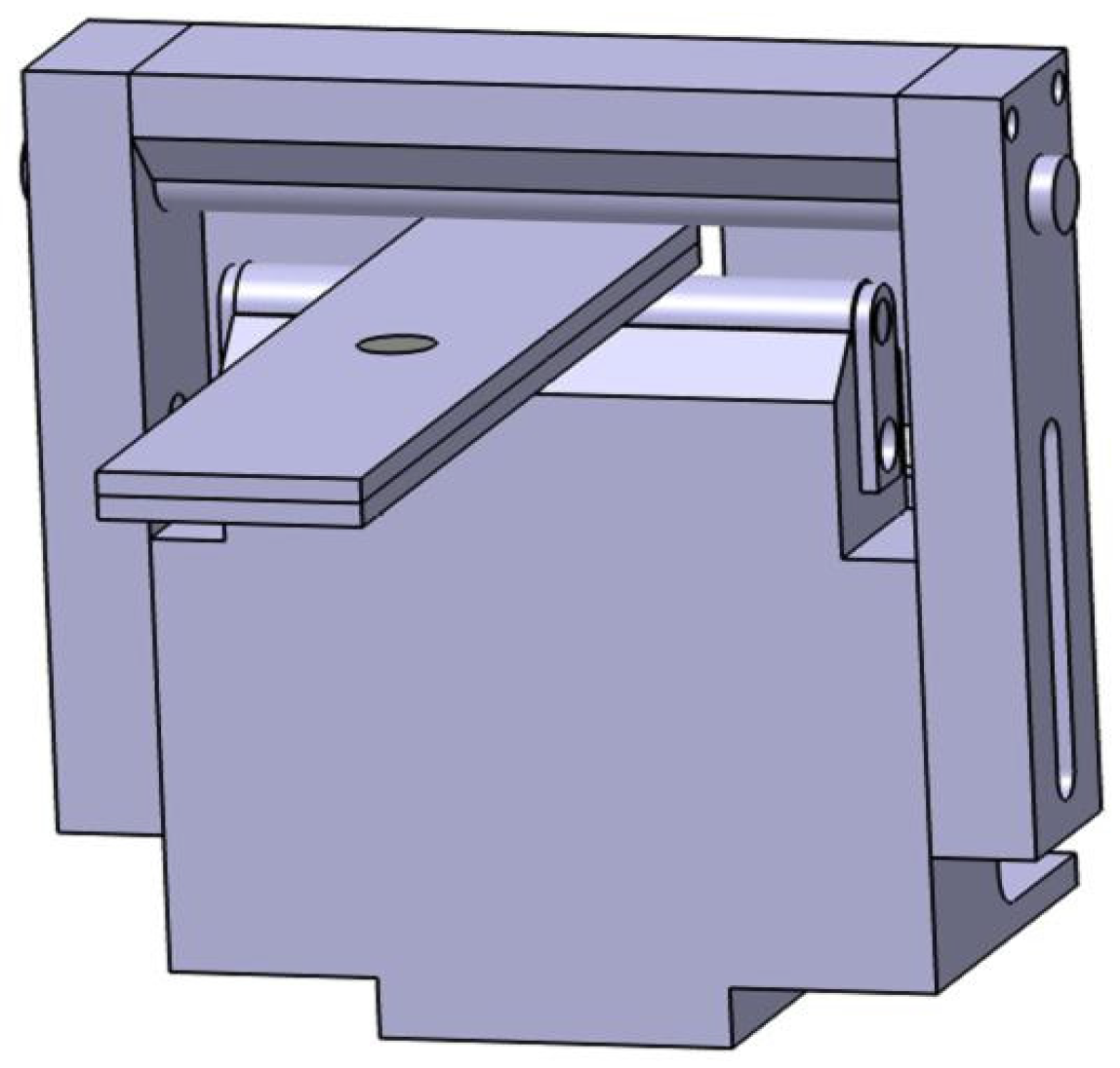
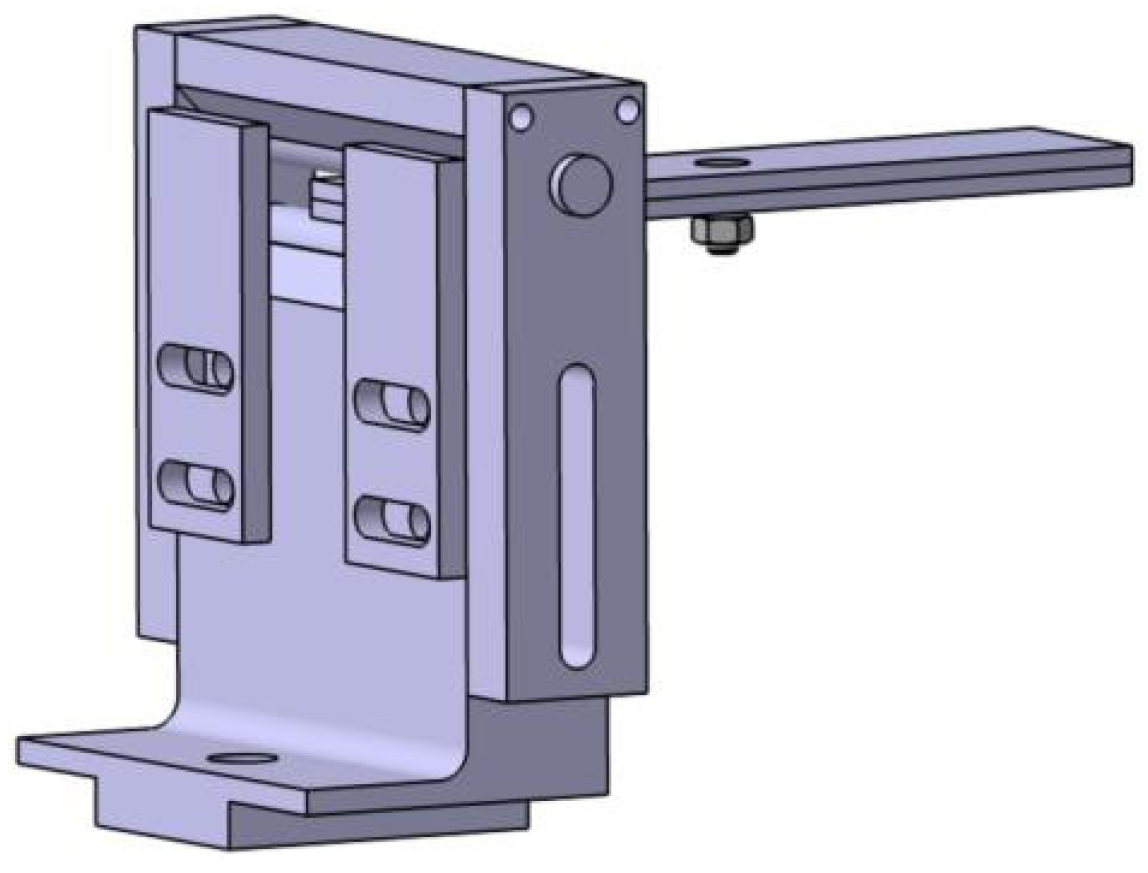
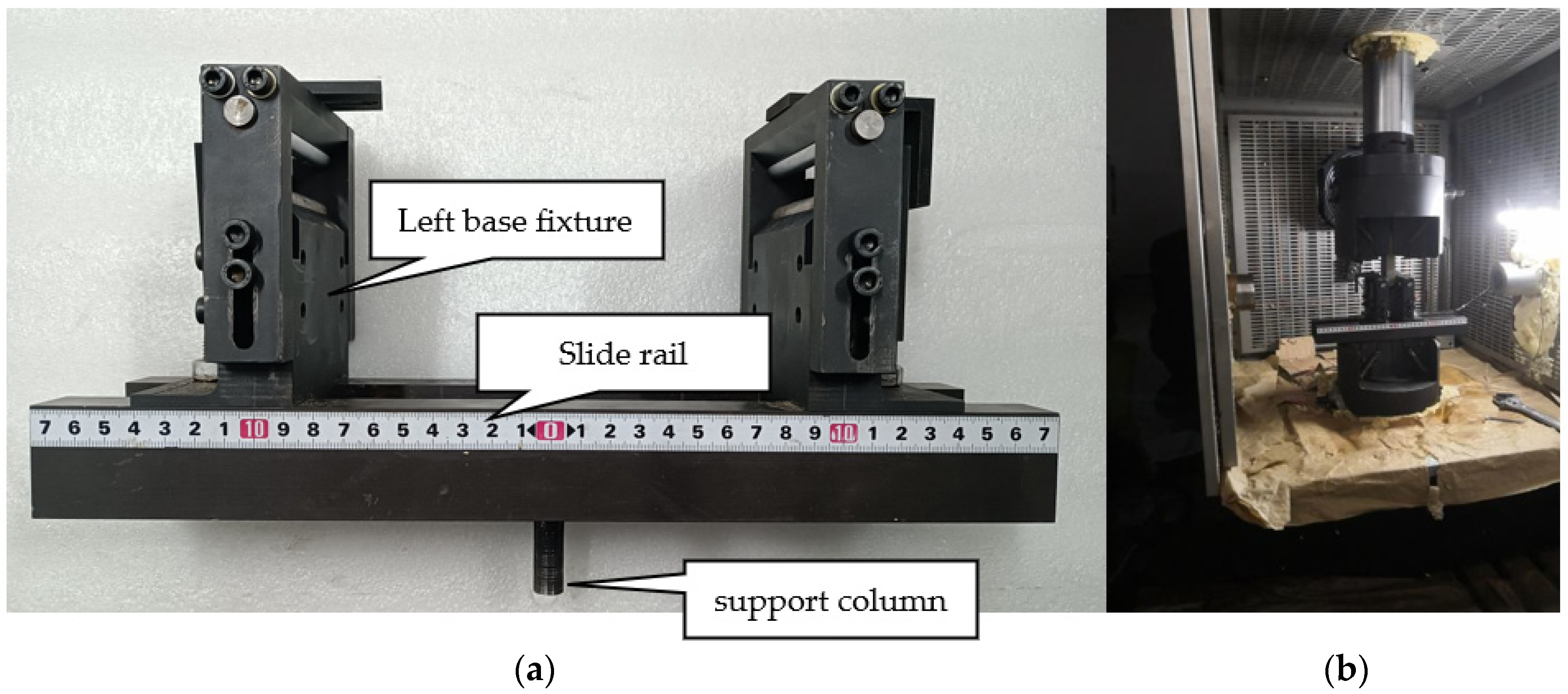
2.1.1. Loading Test System
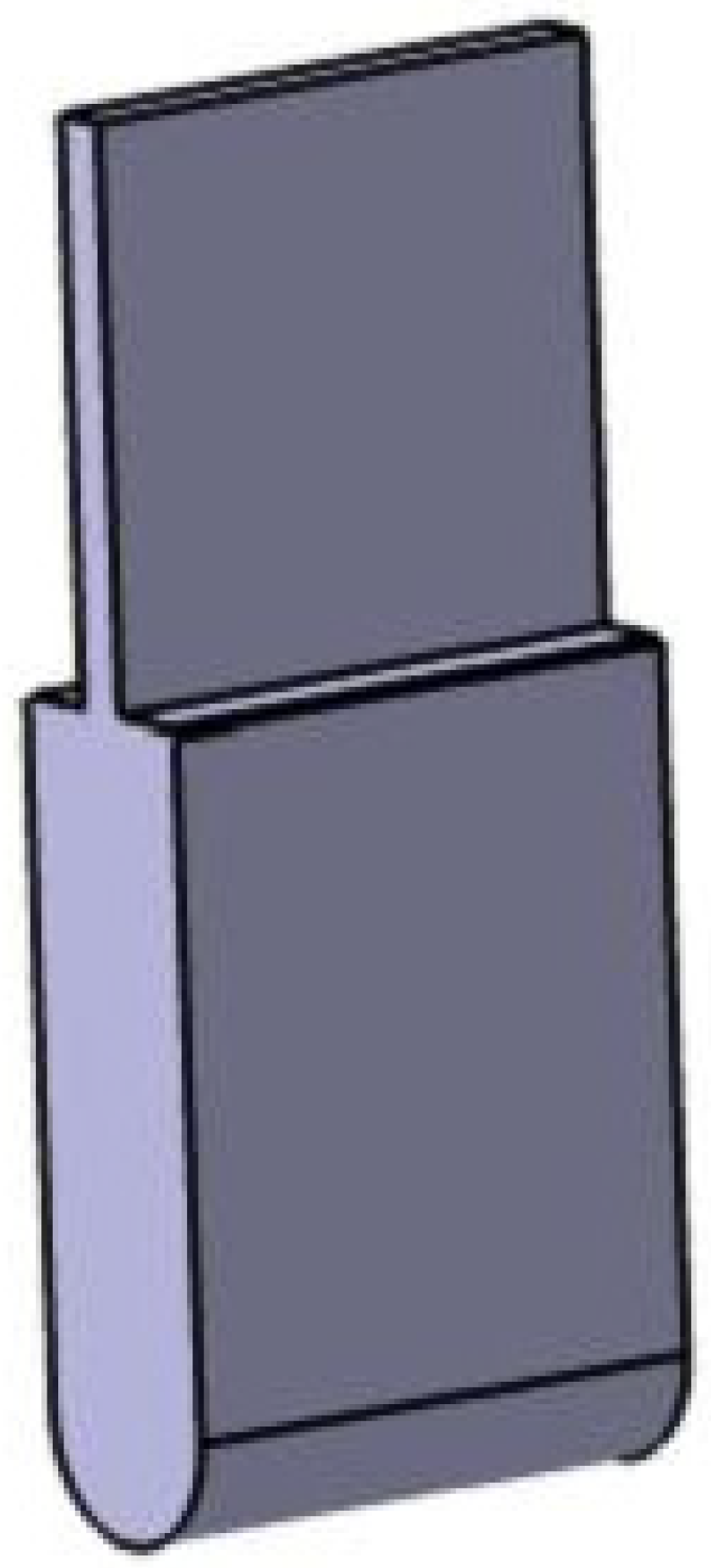
2.1.2. Loading Head and Support Design
2.1.3. Test Specimen Preparation and Load Application
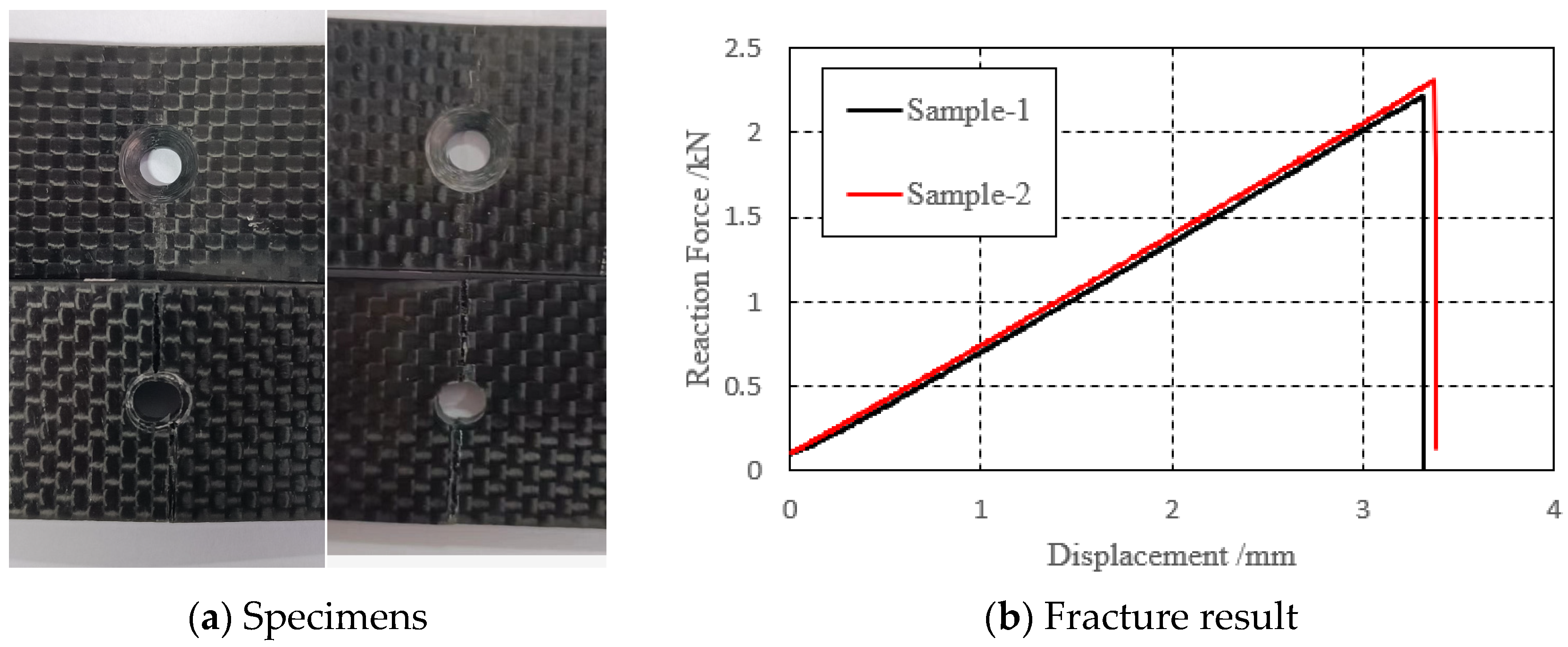
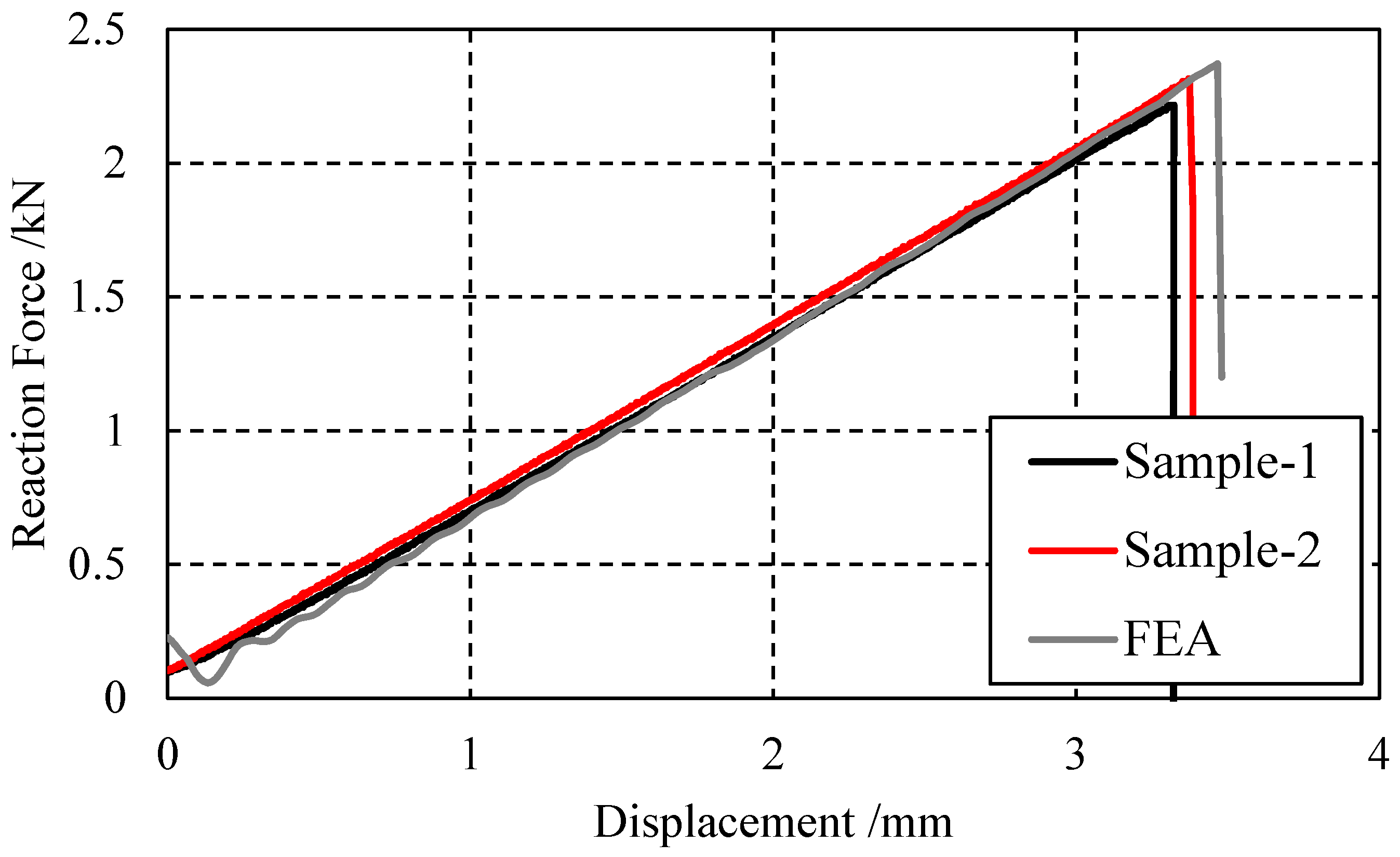
2.2. Loading and Test Results
3. Failure Analysis of CFRP-Countersunk Bolt Assembly
3.1. Hashin Damage Criterion
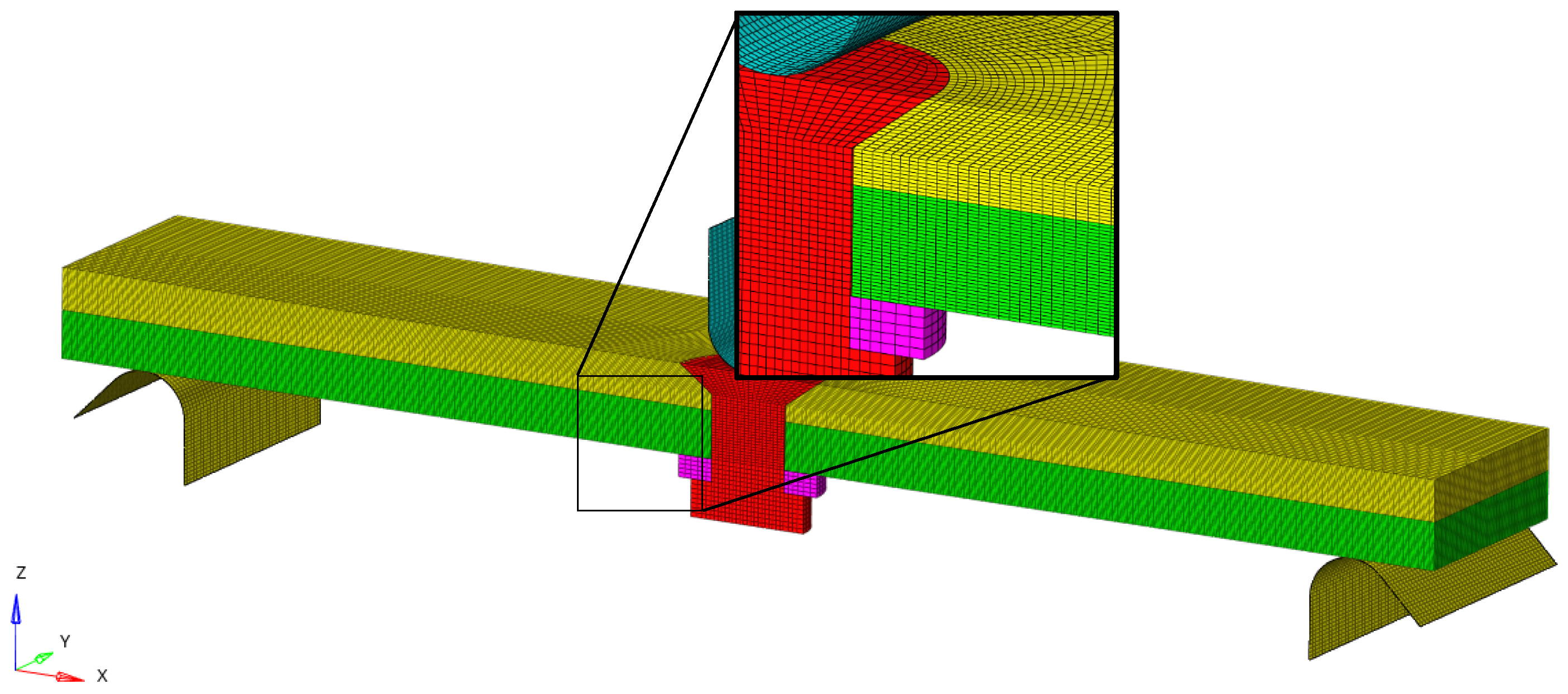
3.2. Geometry and Mesh Model
3.3. Material Properties
3.4. Load and Boundary Conditions
- (1)
- Appling bolt preloading force
- (2)
- Three-point bending
3.5. Meshing
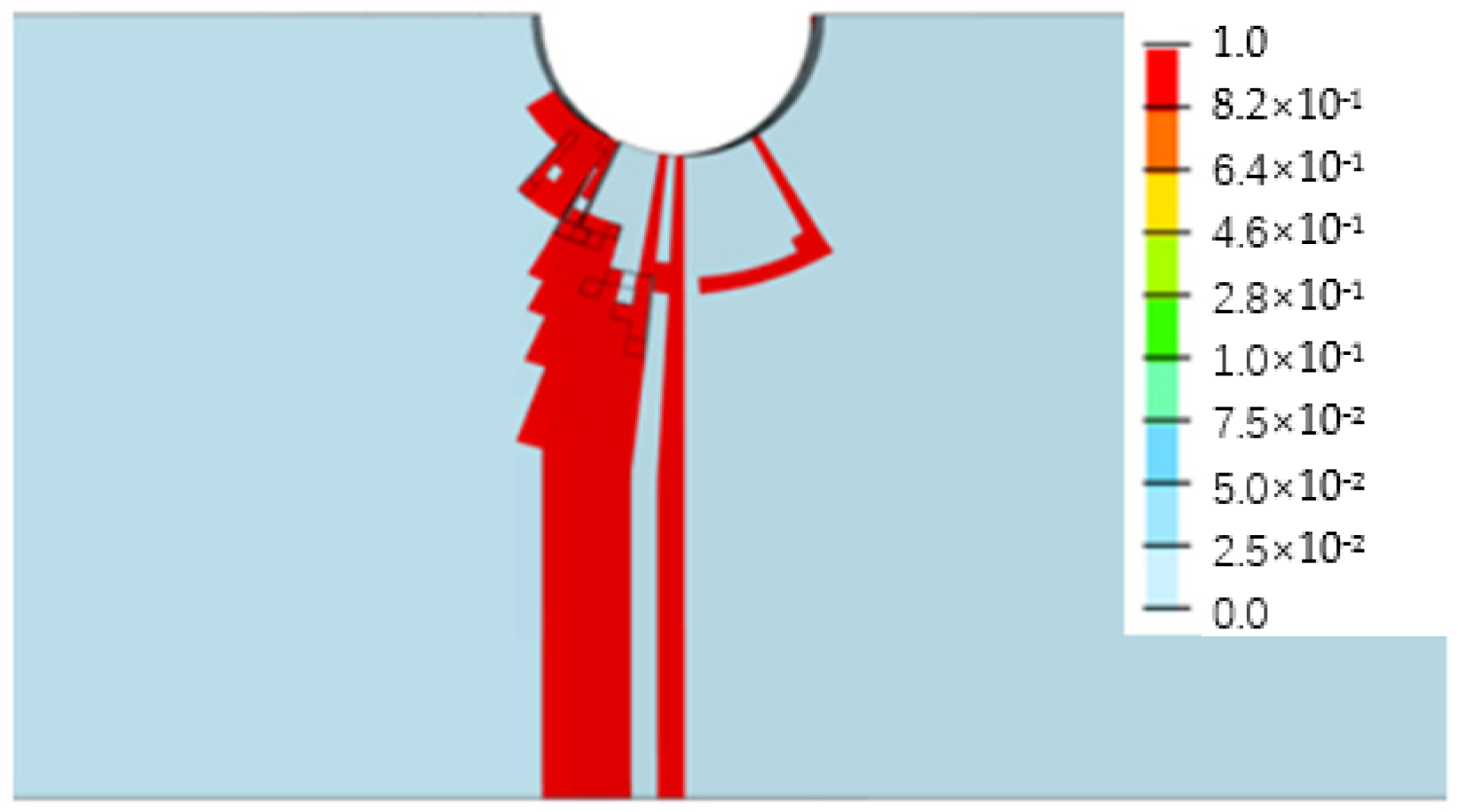
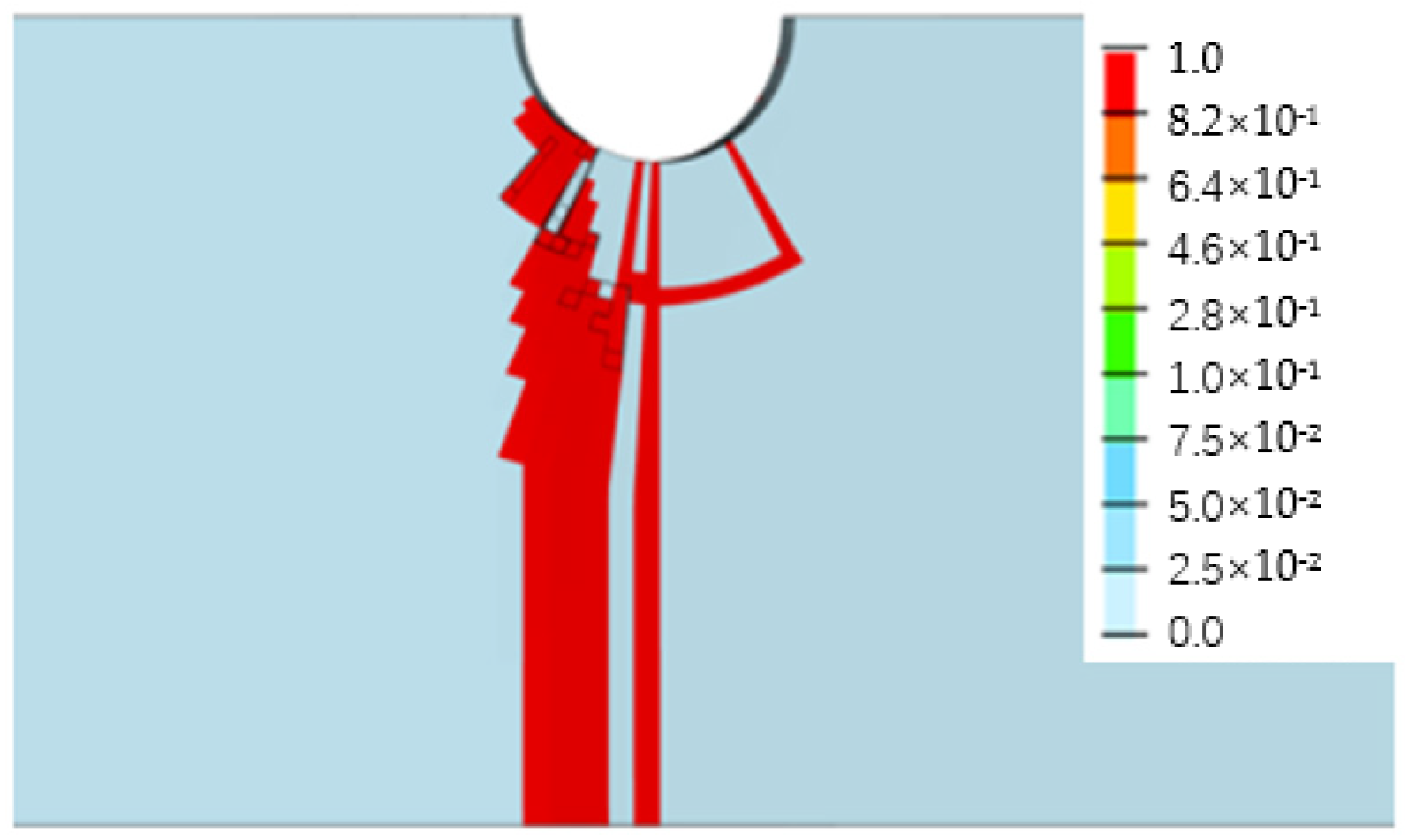
3.6. Analysis Results
4. Effect of Preload on Bending Ultimate Load
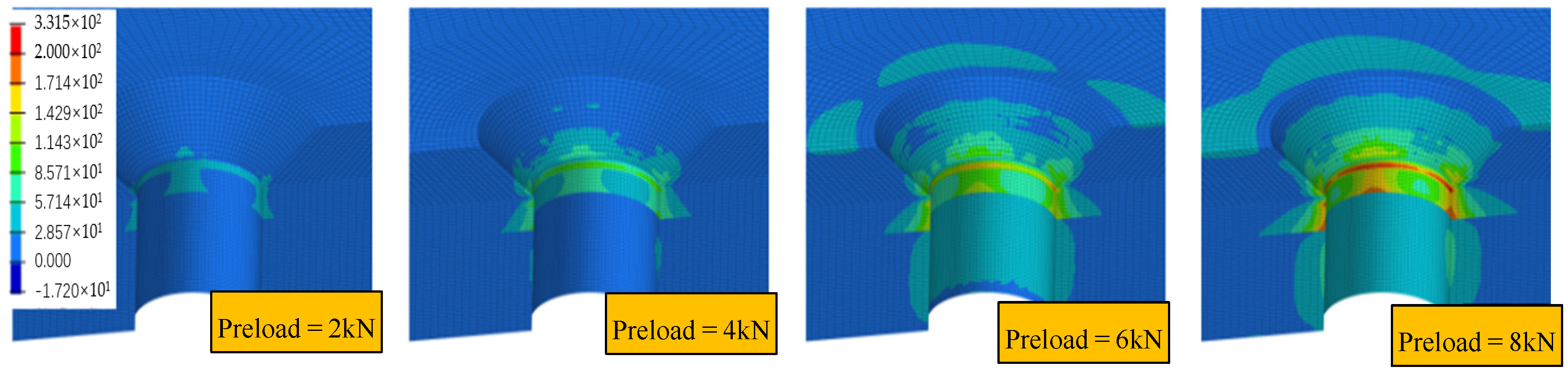
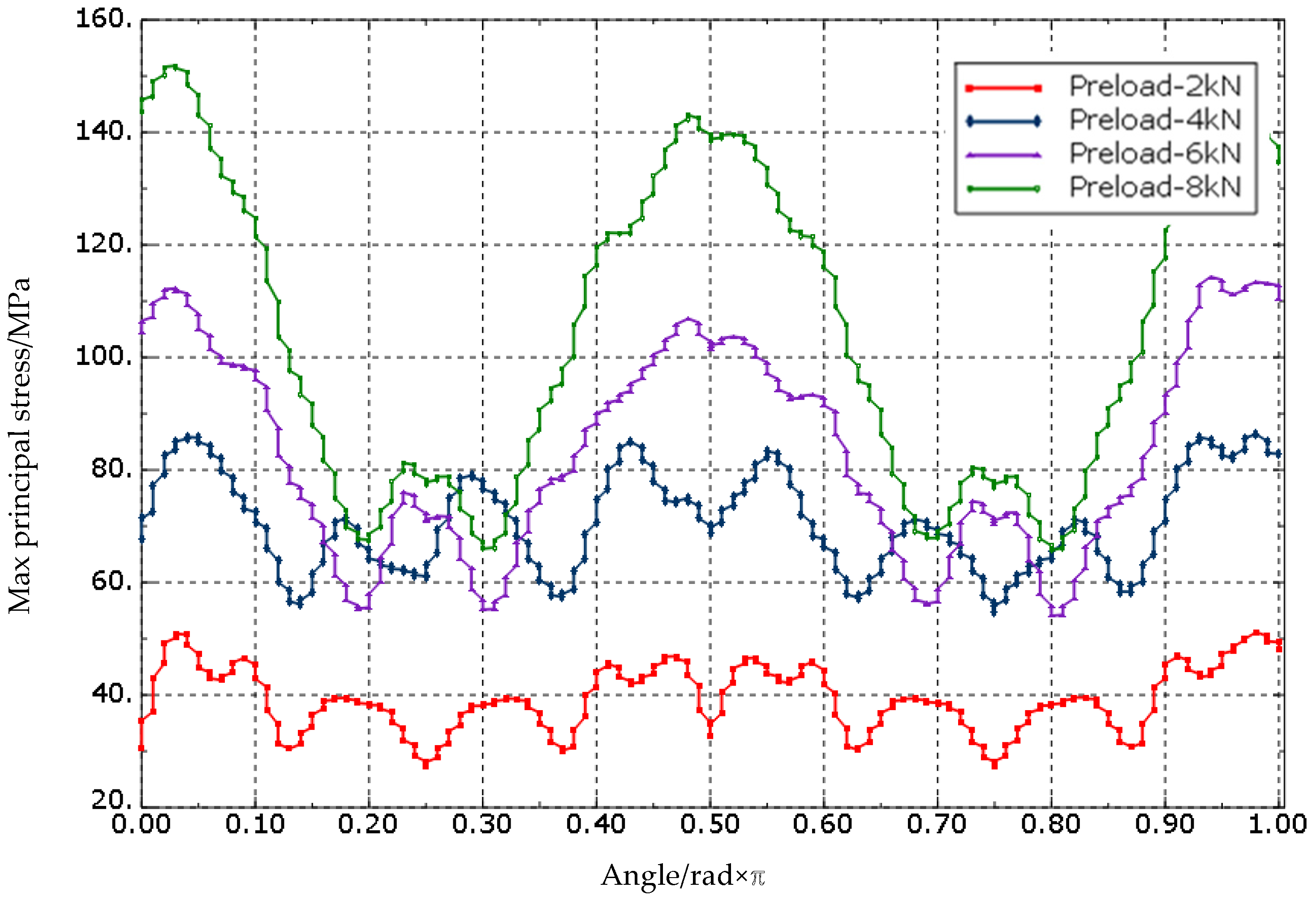
4.1. Counterbore Stress Distribution
4.2. Effect of Preload on Three-Point Bending
5. Conclusions and Outlook
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Ren, L.; Liang, Y. Biological couplings: Function, characteristics and implementation mode. Sci. China Technol. Sci. 2010, 53, 379–387. [Google Scholar] [CrossRef]
- Alaimo, A.; Orlando, C.; Valvano, S. Analytical frequency response solution for composite plates embedding viscoelastic layers. Aerosp. Sci. Technol. 2019, 92, 429–445. [Google Scholar] [CrossRef]
- Shen, G. Mechanics of Composite Materials; Tsinghua University: Beijing, China, 2013. [Google Scholar]
- Zhai, Y.; Li, D.; Li, X.; Wang, L. An experimental study on the effect of joining interface condition on bearing response of single-lap, countersunk composite-aluminum bolted joints. Compos. Struct. 2015, 134, 190–198. [Google Scholar] [CrossRef]
- Zhai, Y.; Li, D.; Li, X.; Wang, L.; Yin, Y. An experimental study on the effect of bolt-hole clearance and bolt torque on single-lap, countersunk composite joints. Compos. Struct. 2015, 127, 411–419. [Google Scholar] [CrossRef]
- Mccarthy, C.T.; Gray, P.J. An analytical model for the prediction of load distribution in highly torqued multi-bolt composite joints. Compos. Struct. 2011, 93, 287–298. [Google Scholar] [CrossRef]
- Kolks, G.; Tserpes, K.I. Efficient progressive damage modeling of hybrid composite/titanium bolted joints. Compos. Part A Appl. Sci. Manuf. 2014, 56, 51–63. [Google Scholar] [CrossRef]
- Liu, L.; Zhang, J.; Chen, K.; Wang, H. Combined and interactive effects of interference fit and preloads on composite joints. Chin. J. Aeronaut. 2014, 27, 716–729. [Google Scholar] [CrossRef]
- Mandal, B.; Chakrabarti, A. Numerical failure assessment of multi-bolt FRP composite joints with varying sizes and preloads of bolts. Compos. Struct. 2018, 187, 169–178. [Google Scholar] [CrossRef]
- Chishti, M.; Wang, C.H.; Thomson, R.S.; Orifici, A.C. Numerical analysis of damage progression and strength of countersunk composite joints. Compos. Struct. 2012, 94, 865–873. [Google Scholar] [CrossRef]
- Liu, Q.; An, L.; Yang, H. Effect of preload on tensile properties of composite single lap joints. Mech. Manuf. Autom. 2022, 51, 5–8. [Google Scholar]
- Sen, F.; Pakdil, M.; Sayman, O.; Benli, S. Experimental failure analysis of mechanically fastened joints with clearance in composite laminates under preload. Mater. Des. 2008, 29, 1159–1169. [Google Scholar] [CrossRef]
- Calin-Dumitru, C. Numerical analysis for the influence of geometrical and mechanical parameters on the stiffness and strength of the composite bolted joints. INCAS Bull. 2018, 10, 21–33. [Google Scholar] [CrossRef]
- Bodjona, K.; Raju, K.; Lim, G.H.; Lessard, L. Load sharing in single-lap bonded/bolted composite joints. Part I: Model development and validation. Compos. Struct. 2015, 129, 268–275. [Google Scholar] [CrossRef]
- Liu, F.; Yao, W.; Zhao, L.; Wu, H.; Zhang, X.; Zhang, J. An improved 2D finite element model for bolt load distribution analysis of composite multi-bolt single-lap joints. Compos. Struct. 2020, 253, 112770. [Google Scholar] [CrossRef]
- Belardi, V.G.; Fanelli, P.; Vivio, F. FE analysis of single-bolt composite bolted joint by means of a simplified modeling technique. Procedia Struct. Integr. 2019, 24, 888–897. [Google Scholar] [CrossRef]
- Hashin, Z. Failure criteria for unidirectional fiber composites. J. Appl. Mech. 1980, 47, 329–335. [Google Scholar] [CrossRef]
- Montagne, B.; Lachaud, F.; Paroissien, E.; Martini, D.; Congourdeau, F. Failure analysis of single lap composite laminate bolted joints: Comparison of experimental and numerical test. Compos. Struct. 2020, 238, 111949. [Google Scholar] [CrossRef]
- Cheng, D. Mechanical Design Manual Chemical Industry; Chemical Industry Press: Beijing, China, 2017. [Google Scholar]



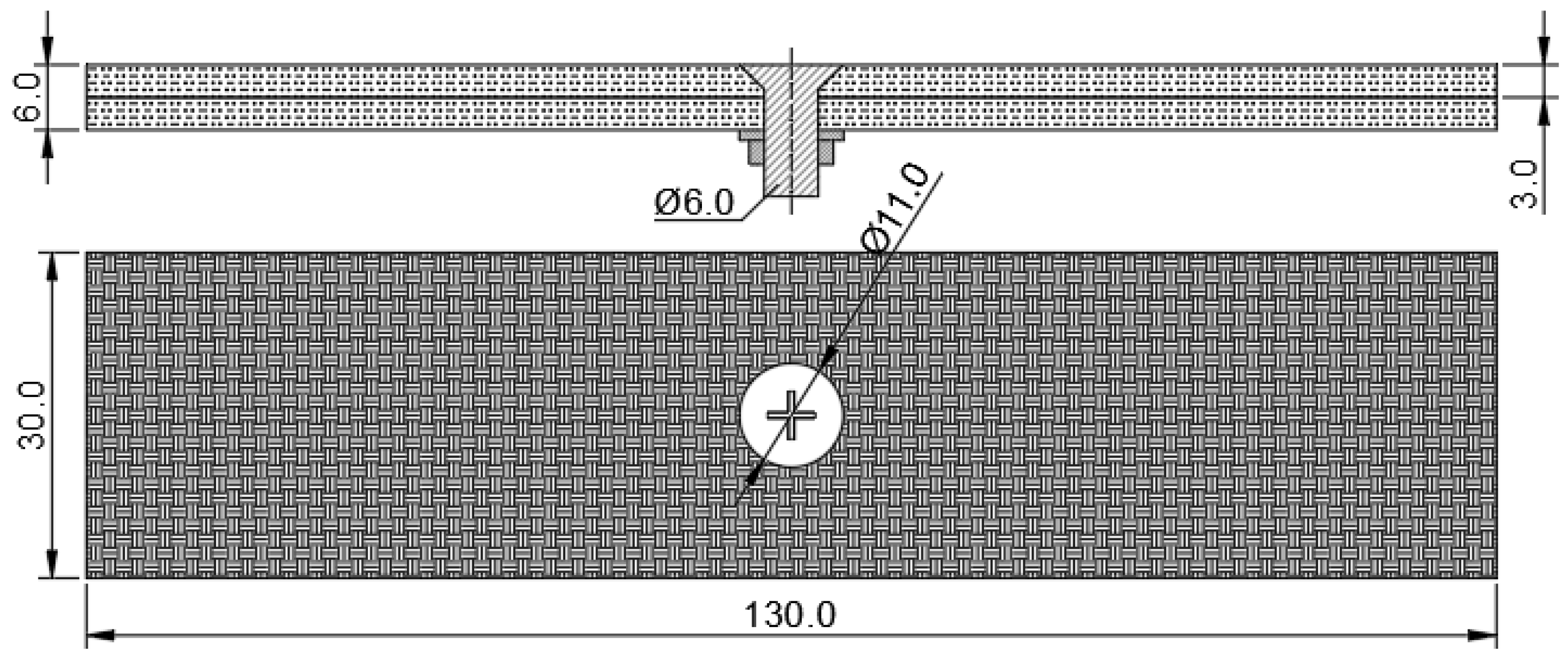




| Parameter Name | Weaving Angle (°) | Number of Plies | Manufacturing Conditions | Size | The Total Thickness |
|---|---|---|---|---|---|
| Numerical value | 0/90 | 30 | High pressure in an autoclave at 0.5 MPa and 120 °C | 130 mm × 30 mm × 6 mm | 3.0 mm |
| E | μ | σb | αt | |
|---|---|---|---|---|
| Countersunk bolt | 110,000 | 0.29 | 950 | 1.2000 × 10−5 |
| Gasket & nut | 210,000 | 0.3 | 235 |
| Yang’s modulus-11 | 69,000 | Longitudinal tensile strength (MPa) | 756 |
| Yang’s modulus-22 | 69,000 | Longitudinal compression strength | 557 |
| Yang’s modulus-33 | 8300 | Lateral tensile strength | 756 |
| Poisson’s ratio-12 plane | 0.064 | Lateral compression strength | 557 |
| Poisson’s ratio-13 plane | 0.064 | Longitudinal shear strength | 118 |
| Poisson’s ratio-23 plane | 0.32 | Lateral compression strength | 118 |
| Shear modulus-12 plane | 4200 | Longitudinal tensile fracture energy | 45 |
| Shear modulus-13 plane | 4200 | Longitudinal compression fracture energy | 0.6 |
| Shear modulus-23 plane | 4200 | Lateral tensile fracture energy | 45 |
| Lateral compression fracture energy | 0.6 |
| Composite–composite | 0.3 |
| Bolt–composite | 0.1 |
| Bolt–gasket | 0.15 |
| Gasket–composite | 0.1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qin, Z.; He, Y.; Wang, S.; Meng, C. Ultimate Strength Study of Structural Bionic CFRP-Sinker Bolt Assemblies Subjected to Preload under Three-Point Bending. Biomimetics 2023, 8, 215. https://doi.org/10.3390/biomimetics8020215
Qin Z, He Y, Wang S, Meng C. Ultimate Strength Study of Structural Bionic CFRP-Sinker Bolt Assemblies Subjected to Preload under Three-Point Bending. Biomimetics. 2023; 8(2):215. https://doi.org/10.3390/biomimetics8020215
Chicago/Turabian StyleQin, Zhengqi, Ying He, Shengwu Wang, and Cunying Meng. 2023. "Ultimate Strength Study of Structural Bionic CFRP-Sinker Bolt Assemblies Subjected to Preload under Three-Point Bending" Biomimetics 8, no. 2: 215. https://doi.org/10.3390/biomimetics8020215
APA StyleQin, Z., He, Y., Wang, S., & Meng, C. (2023). Ultimate Strength Study of Structural Bionic CFRP-Sinker Bolt Assemblies Subjected to Preload under Three-Point Bending. Biomimetics, 8(2), 215. https://doi.org/10.3390/biomimetics8020215






