Three-Dimensional Analysis of Biomimetic Aerofoil in Transonic Flow
Abstract
:1. Introduction
2. Literature Review
3. Computational Procedure
4. Results and Discussion
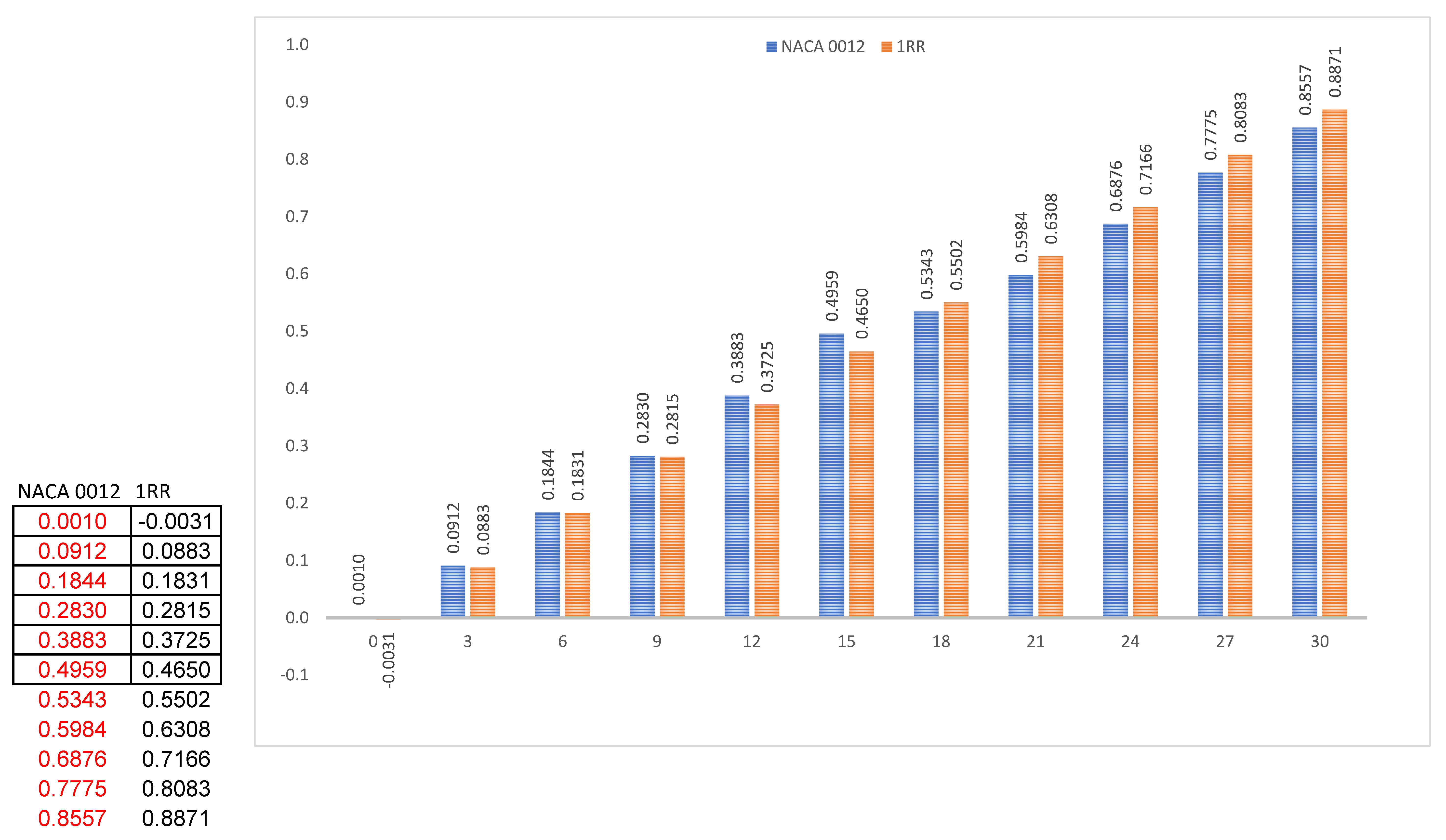
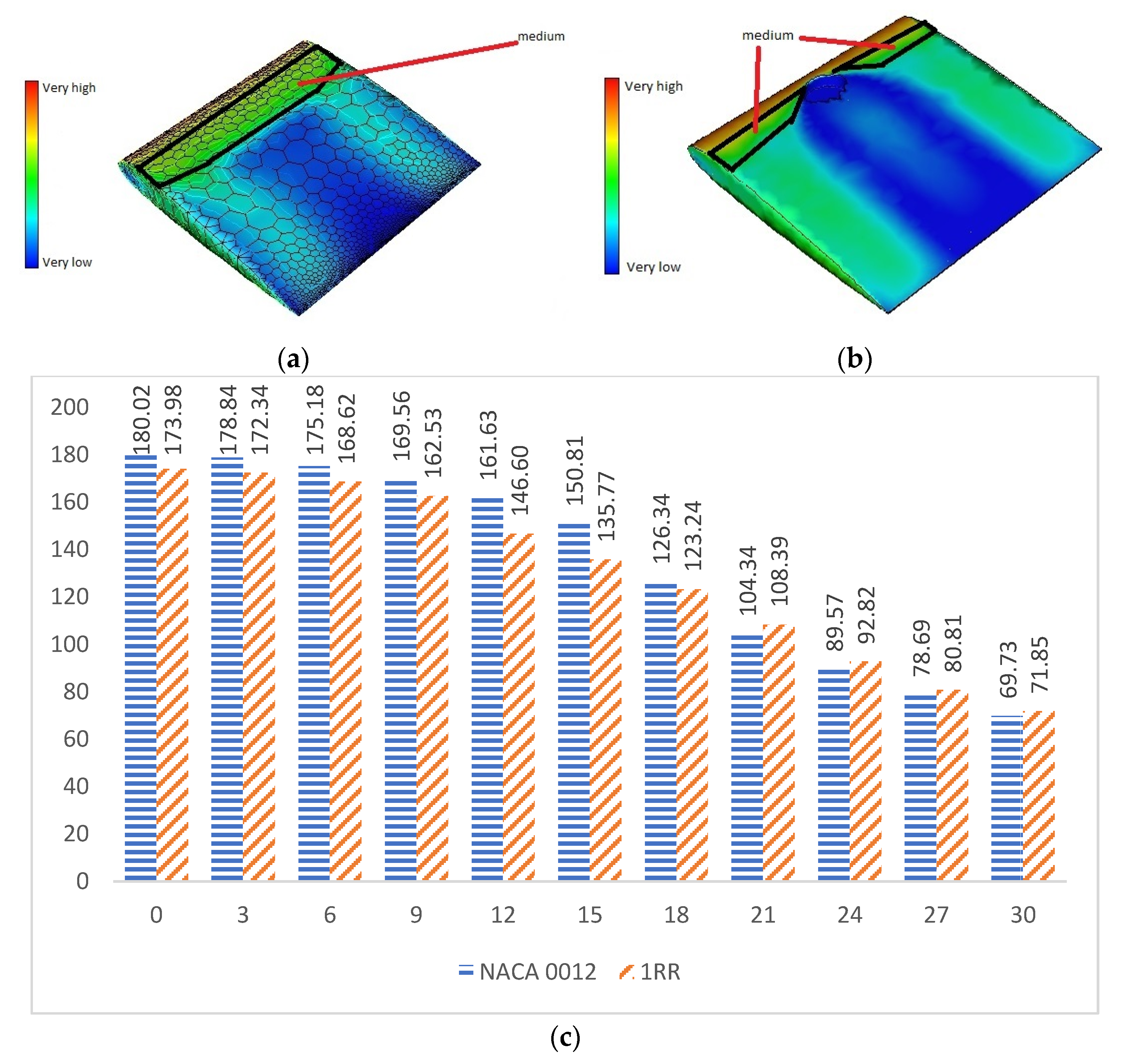
4.1. Lift Force
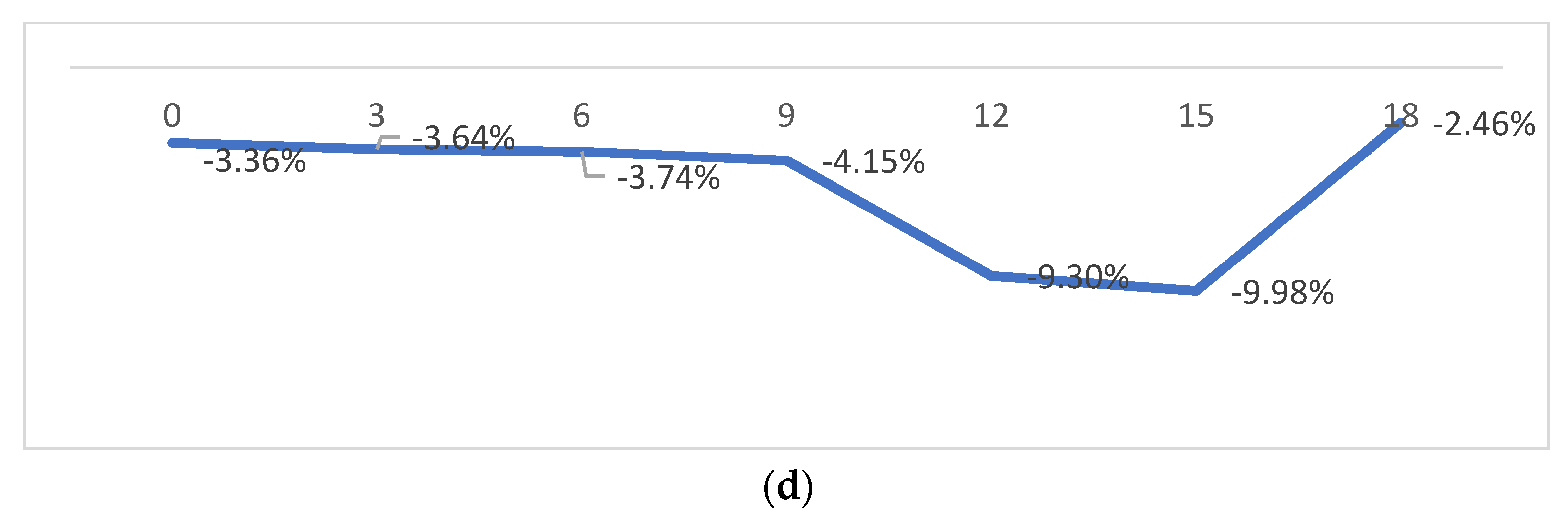
4.2. Viscous Drag
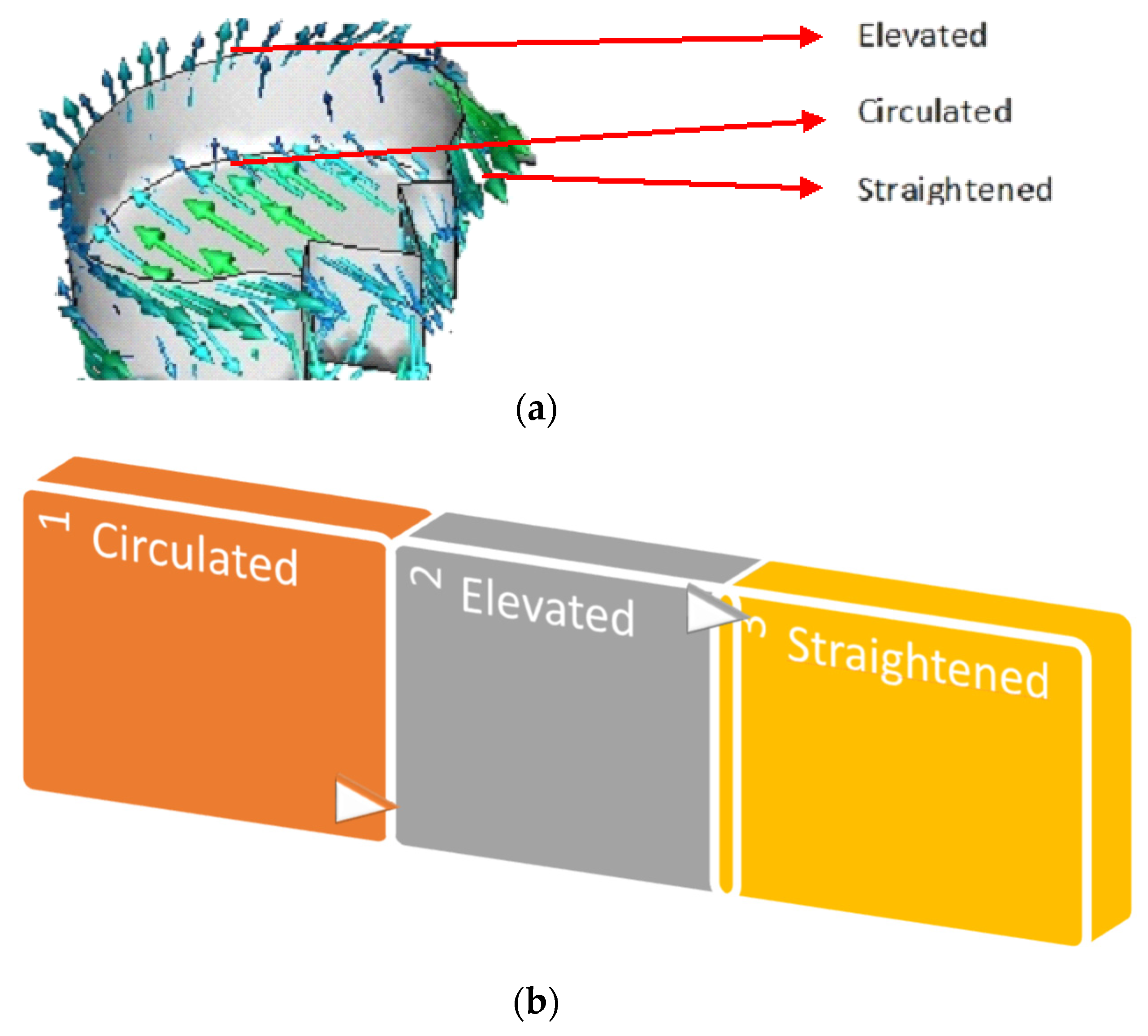
4.3. Influence of RR Pattern in Transonic Flow
5. Validation
6. Conclusions
- (i)
- lift force could be increased up to 5.41% at 21° angle of attack,
- (ii)
- viscous drag could be reduced up to 9.98% at 15° angle of attack,
- (iii)
- developed a new biomimetic pattern called Raw Riblet,
- (iv)
- surface with a little roughness is better than a smooth surface.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
Abbreviations
| HPBA | Hollow Patterned Biomimetic Aerofoil |
| NACA | National Advisory Committee for Aeronautics |
| RR | Raw Riblet |
| SEM | Scanning Electron Microscope |
References
- Tousif, A.M.; Tanjin, A.S.M.; Rafiul, I.; Shabbir, A. Computational Study of Flow around a NACA 0012 Wing Flapped at Different Flap Angles with Varying Mach Numbers. Glob. J. Res. Eng. 2013, 13, 4–15. [Google Scholar]
- Brian, D.; Bharat, B. Shark-skin surfaces for fluid-drag reduction in turbulent flow: A review. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2010, 368, 4775–5737. [Google Scholar]
- Rasuo, B. An Experimental and Theoretical Study of Transonic Flow About the NACA 0012 Airfoil. In Proceedings of the 24th AIAA Applied Aerodynamics Conference, Sanfransisco, CA, USA, 5–8 June 2006; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2012. [Google Scholar]
- Siva, M.; Rajadurai, M.; Pandyrajan, R.; Babu, B. Design of Dragon Fly Aircraft. Res. Inventy Int. J. Eng. Sci. 2013, 2, 40–47. [Google Scholar]
- Siva, M.; Megalingam Murugan, A.; Sivasathya, U.; Dharmalingam, S. Biomimetic in Turbulence Reduction. Recent Developments. J. Appl. Sci. Res. 2015, 11, 123–134. [Google Scholar]
- Siva, M.; Dhavamani, C. Computational Analysis of Biomimetic Butterfly Valve. Bioinspired Biomim. Nanobiomater. 2020, 9, 223–232. [Google Scholar]
- Siva, M.; Dhavamani, C. Computational Analysis to Enhance the Compressible Flow over an Aerofoil surface. Aircr. Eng. Aerosp. Technol. 2021, 93, 925–934. [Google Scholar]
- Feld, K.; Kolberg, A.N.; Nyborg, C.M.; Salewski, M.; Steffensen, J.F.; Berg-Sorensen, K. Dermal Denticles of Three Slowly Swimming Shark Species: Microscopy and Flow Visualization. Biomimetics 2019, 4, 38. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lang, A.; Habegger, M.L.; Motta, P.; Hueter, R.; Afroz, F. Shark Skin Separation Control Mechanisms. Mar. Technol. Soc. J. 2011, 45, 2018–2215. [Google Scholar] [CrossRef]
- Bechert, D.W.; Hoppe, G.; Reif, W.E. On the drag reduction of the shark skin. In Proceedings of the 23rd Aerospace Sciences Meeting, Reno, NV, USA, 14–17 January 1985; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2012. [Google Scholar]
- Bhatia, D.; Zhao, Y.; Yadav, D.; Wang, J. Drag reduction using biomimetic sharkskin denticles. Eng. Technol. Appl. Sci. Res. 2021, 11, 7665–7672. [Google Scholar] [CrossRef]
- Bandyopadhyay, P.; Hellum, A. Modeling how shark and dolphin skin patterns control transitional wall-turbulence vorticity patterns using spatiotemporal phase reset mechanisms. Sci. Rep. 2014, 4, 6650. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Liu, G.; Yuan, Z.; Qiu, Z.; Feng, S.; Xie, Y.; Leng, D.; Tian, X. A brief review of bio-inspired surface technology and application toward underwater drag reduction. Ocean. Eng. 2020, 199, 1–8. [Google Scholar] [CrossRef]
- Rabii, E.M.; Bouchaib, R.; Abdelkhalak, E.H. CFD analysis of the transonic flow over a NACA 0012 airfoil. Uncertain. Reliab. Multiphysical Syst. 2018, 2, 11–17. [Google Scholar]
- Wan, C.; Xisheng, L.; Dongen, M.E.H. On condensation-induced waves. J. Fluid Mech. 2010, 651, 145–164. [Google Scholar]
- Novel, K.S.; Shadab, I. Analysis of transonic flow over an airfoil NACA0012 using CFD. Int. J. Innov. Sci. Eng. Technol. 2015, 2, 379–388. [Google Scholar]
- Lang, A.; Habegger, M.L.; Motta, P. Shark Skin Drag Reduction. In Encyclopedia of Nanotechnology; Bhushan, B., Ed.; Springer: Dordrecht, Germany, 2012; pp. 2394–2400. [Google Scholar]
- Ceballos, J.; Dillon, E. 2015. Available online: https://anatomytoyou.com/2016/01/27/skin-teeth-stories-using-shark-denticles-to-look-to-their-past/ (accessed on 19 December 2021).
- Gregory, N.; Wilby, P.G. NPL 9615 and NACA 0012 A Comparison of Aerodynamic Data; Ministry of Defense, Aerodynamic Division, NPL, Her Majesty’s Stationery Office: London, UK, 1973.
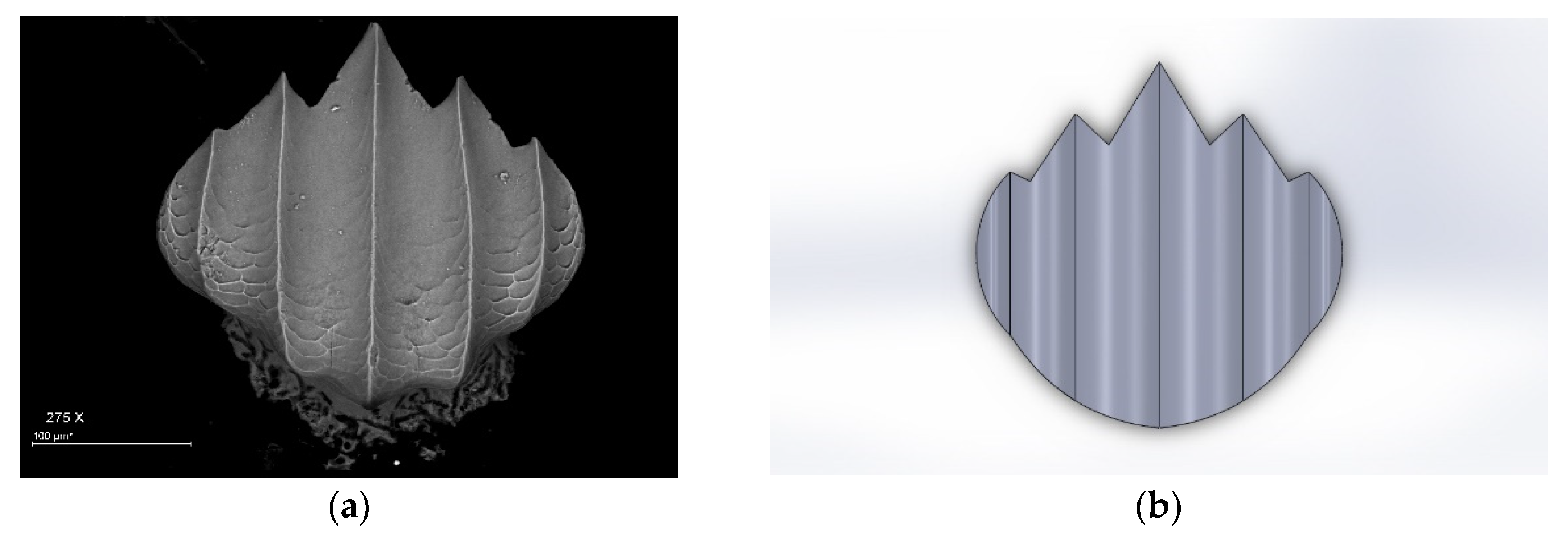
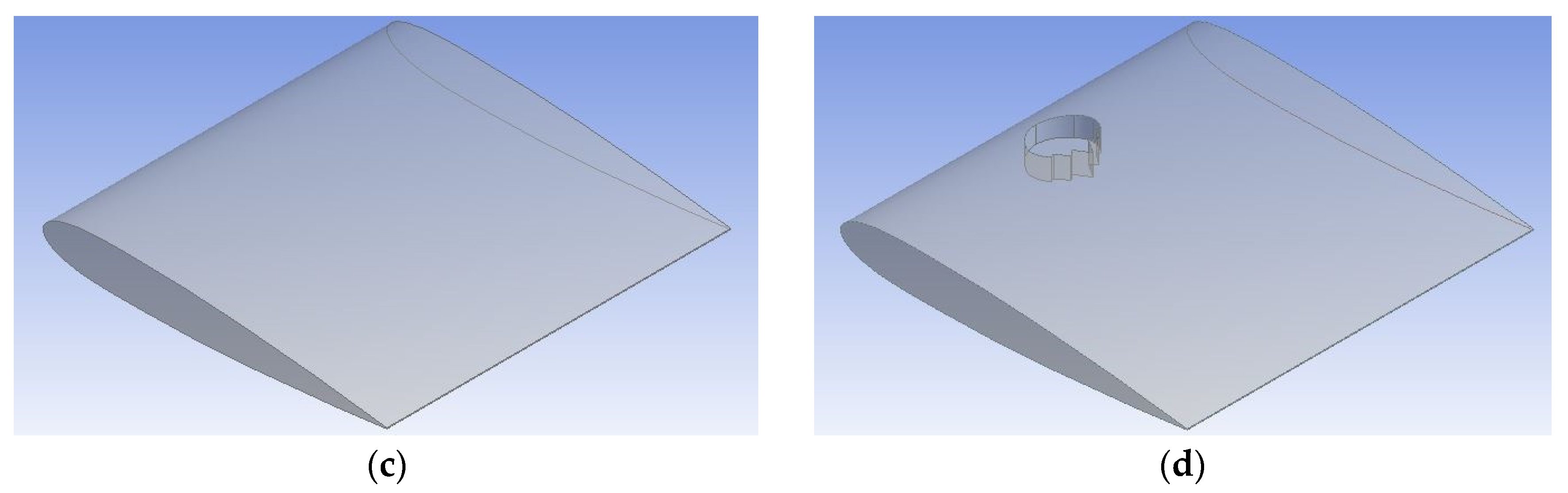
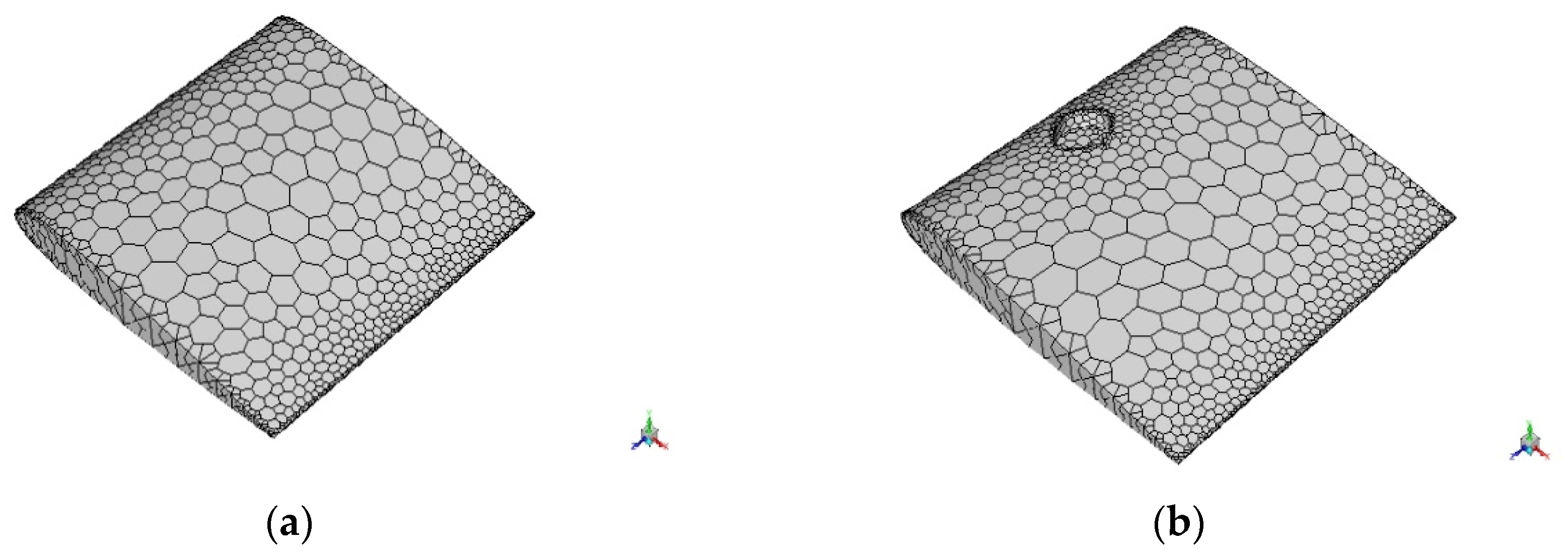

| Element Size | Drag Coefficient |
|---|---|
| 0.3 | 0.019 |
| 0.4 | 0.019 |
| 0.5 | 0.019 |
| 0.6 | 0.019 |
| 0.7 | 0.019 |
| 0.8 | 0.203 |
| 0.9 | 0.205 |
| Consistent Value | 0.019 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Marimuthu, S.; Al-Rabeei, S.; Boha, H.A. Three-Dimensional Analysis of Biomimetic Aerofoil in Transonic Flow. Biomimetics 2022, 7, 20. https://doi.org/10.3390/biomimetics7010020
Marimuthu S, Al-Rabeei S, Boha HA. Three-Dimensional Analysis of Biomimetic Aerofoil in Transonic Flow. Biomimetics. 2022; 7(1):20. https://doi.org/10.3390/biomimetics7010020
Chicago/Turabian StyleMarimuthu, Siva, Samer Al-Rabeei, and Hithim Ahmed Boha. 2022. "Three-Dimensional Analysis of Biomimetic Aerofoil in Transonic Flow" Biomimetics 7, no. 1: 20. https://doi.org/10.3390/biomimetics7010020
APA StyleMarimuthu, S., Al-Rabeei, S., & Boha, H. A. (2022). Three-Dimensional Analysis of Biomimetic Aerofoil in Transonic Flow. Biomimetics, 7(1), 20. https://doi.org/10.3390/biomimetics7010020






