Biomechanical Simulation of Orthodontic En-Bloc Retraction Comparing Compound Technique and Sliding Mechanics Using a HOSEA Robotic Device
Abstract
1. Introduction
2. Materials and Methods
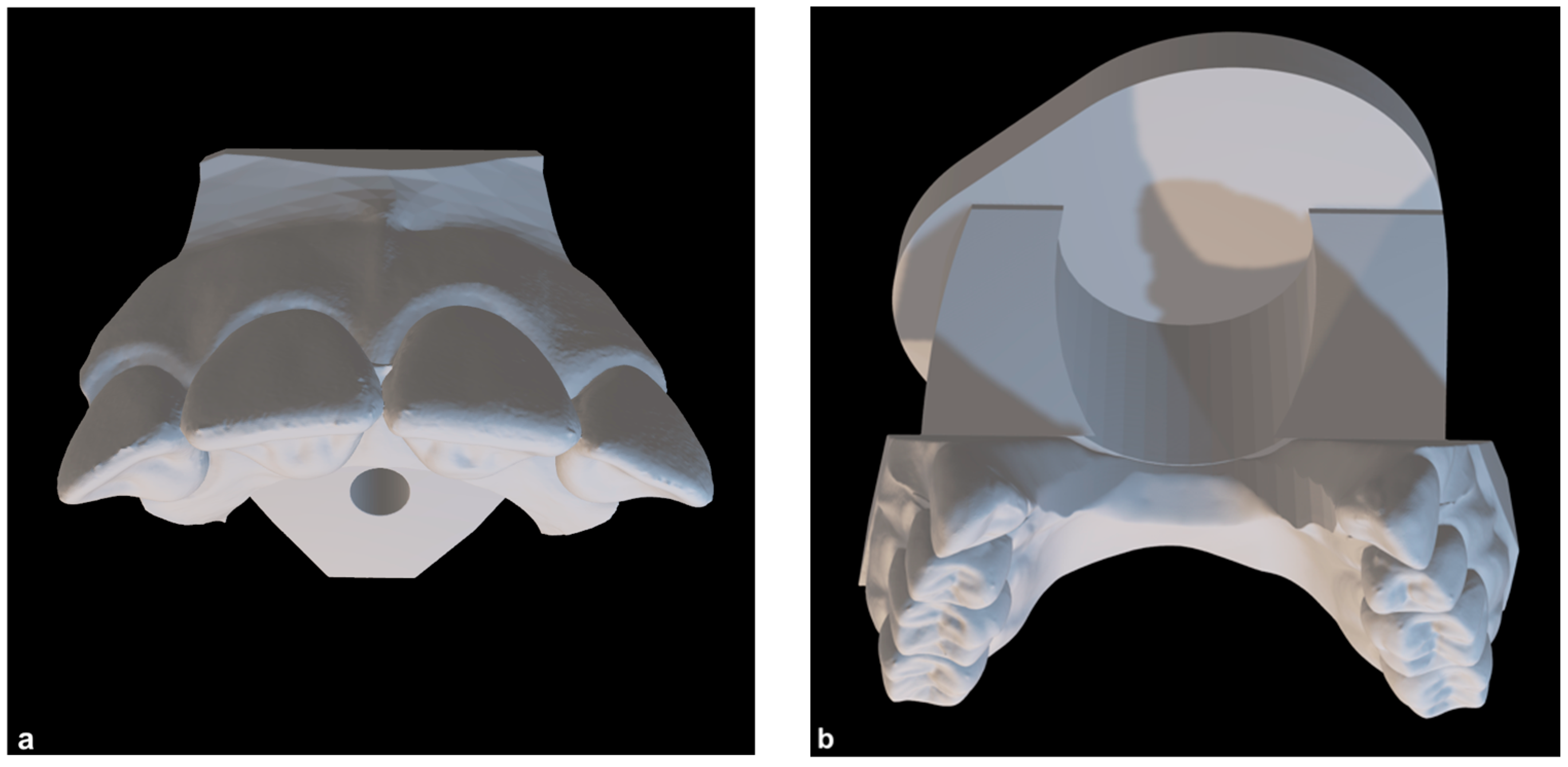
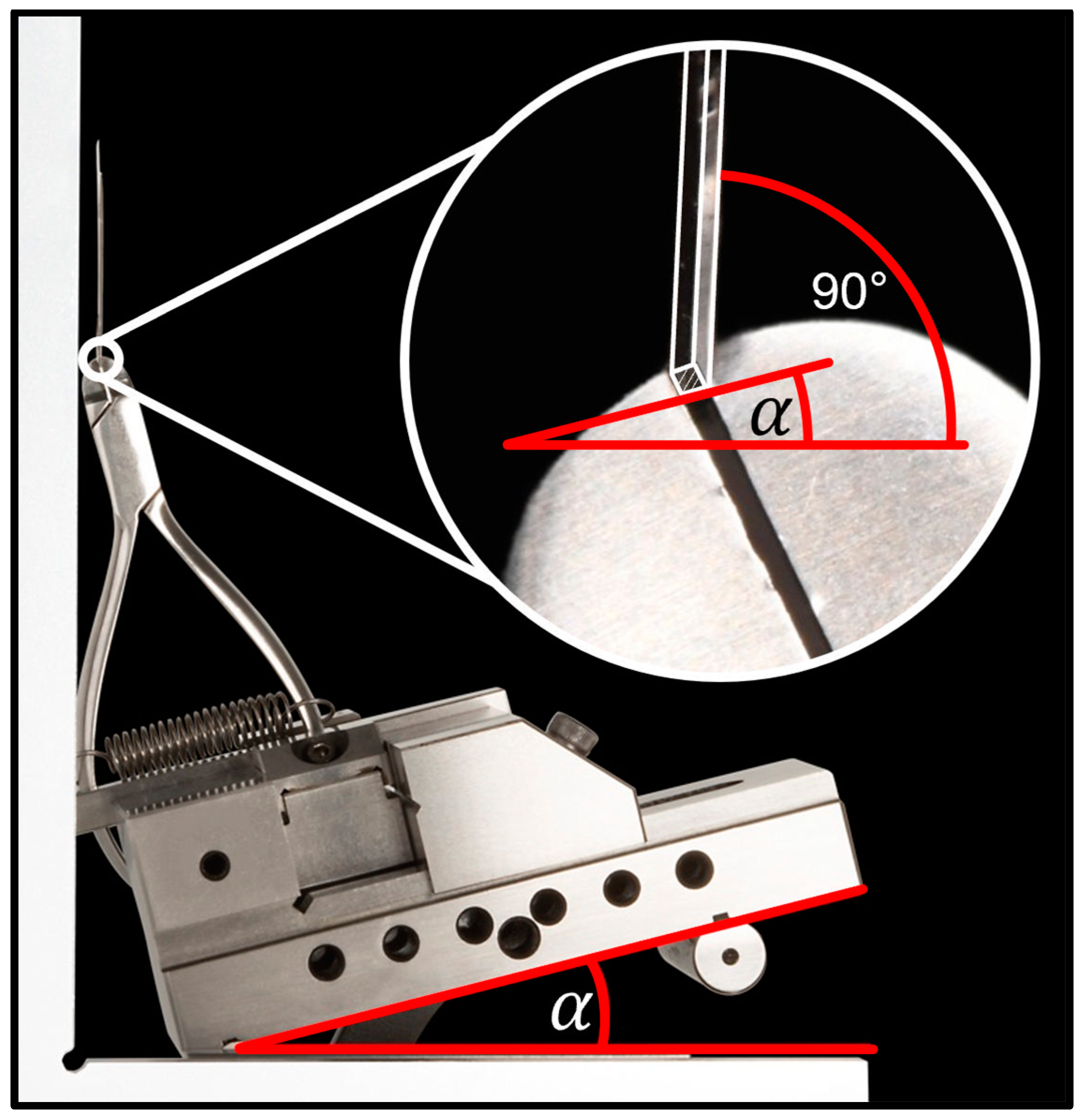
2.1. Development of the Experimental Model for En-Bloc Retraction
2.2. Specimen
- (1)
- Sliding mechanics with SS archwires (Forestadent GmbH, Pforzheim, Germany) and manually bent anterior torque in combination with elastic chains [5].
- (2)
- Compound technique (modified sliding mechanics) with RTAs (Forestadent GmbH, Germany) out of NiTi and SS with prefabricated torque in combination with NiTi coil springs [5].
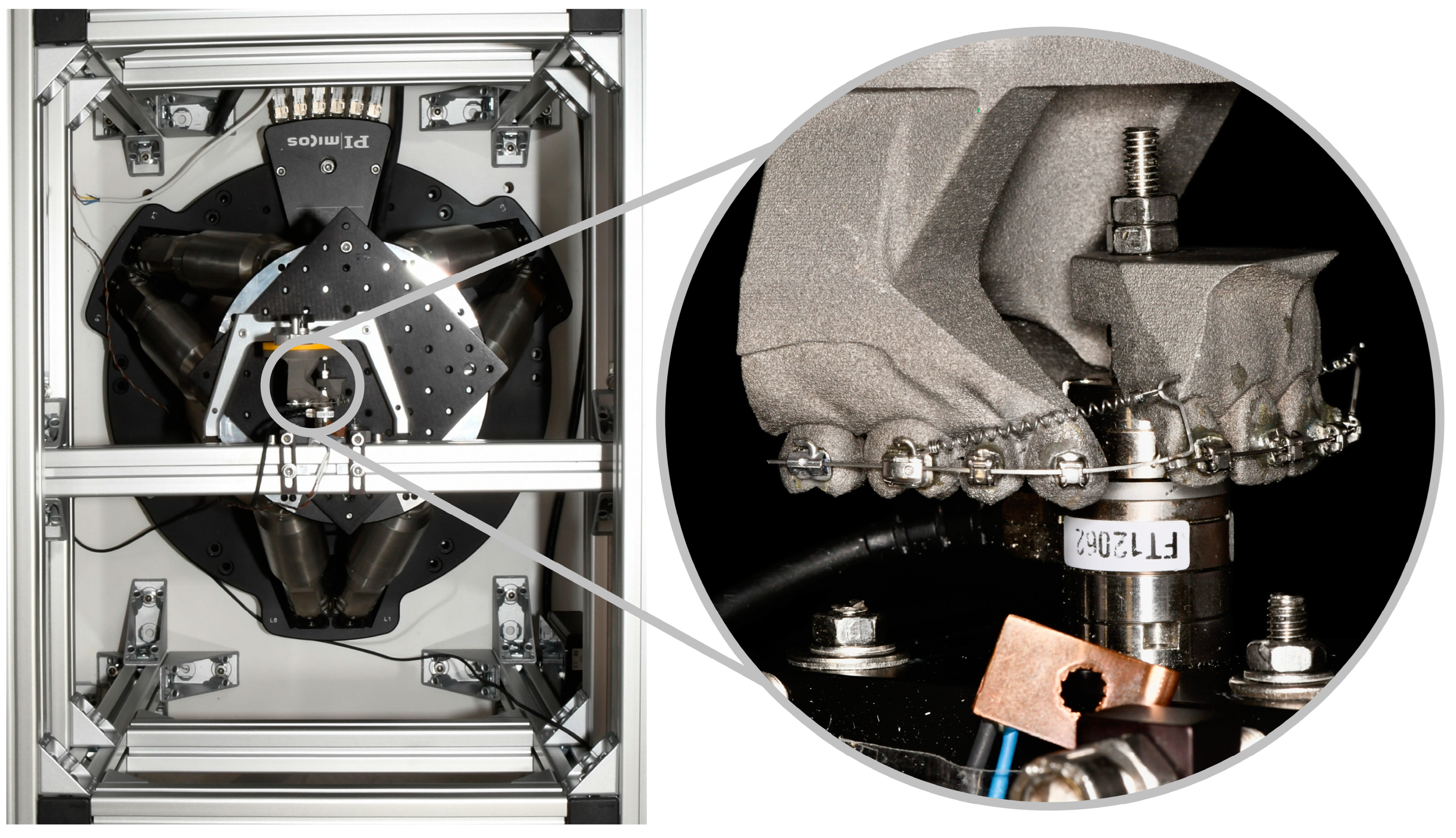
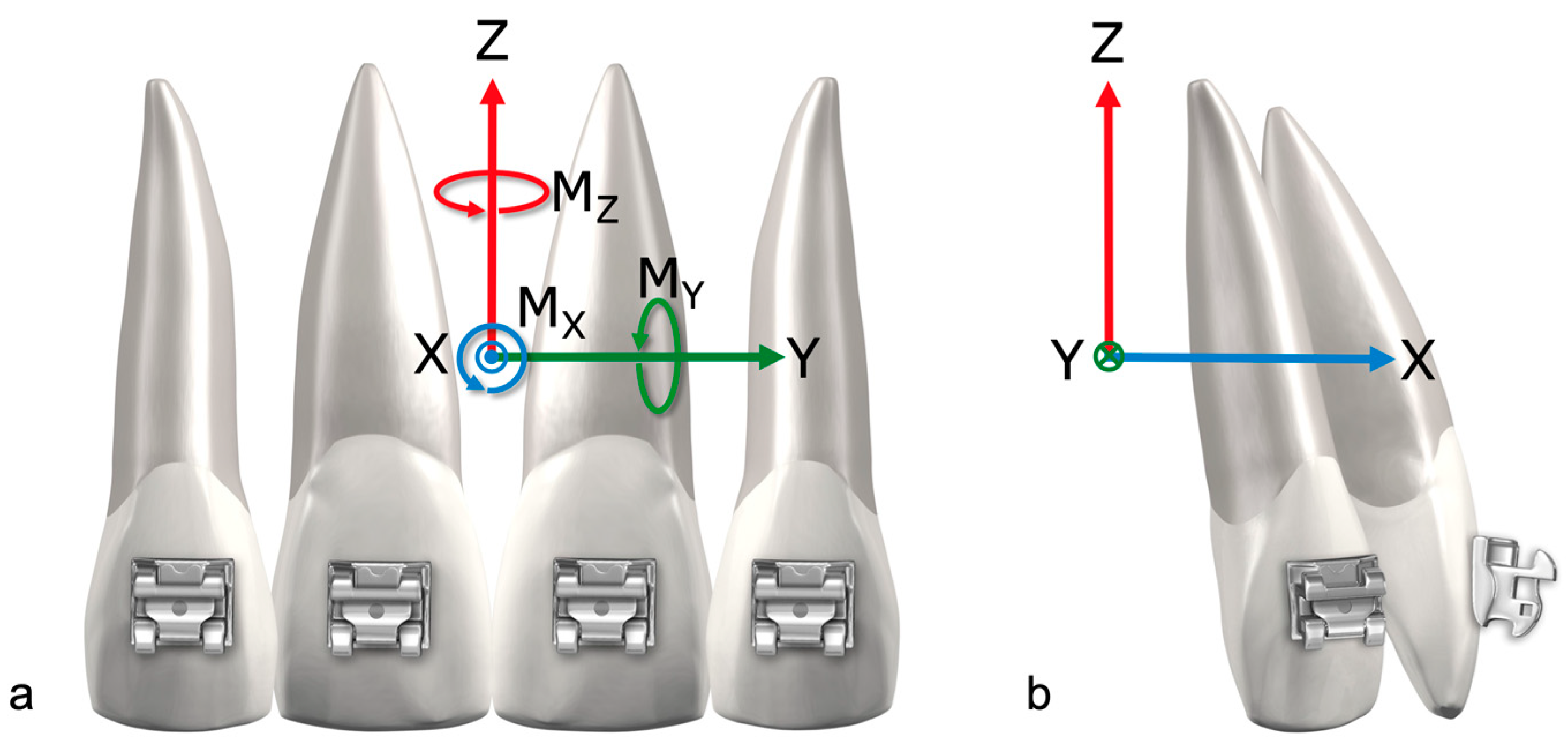
2.3. Biomechanical Simulation with HOSEA
2.4. Statistical Analysis
3. Results
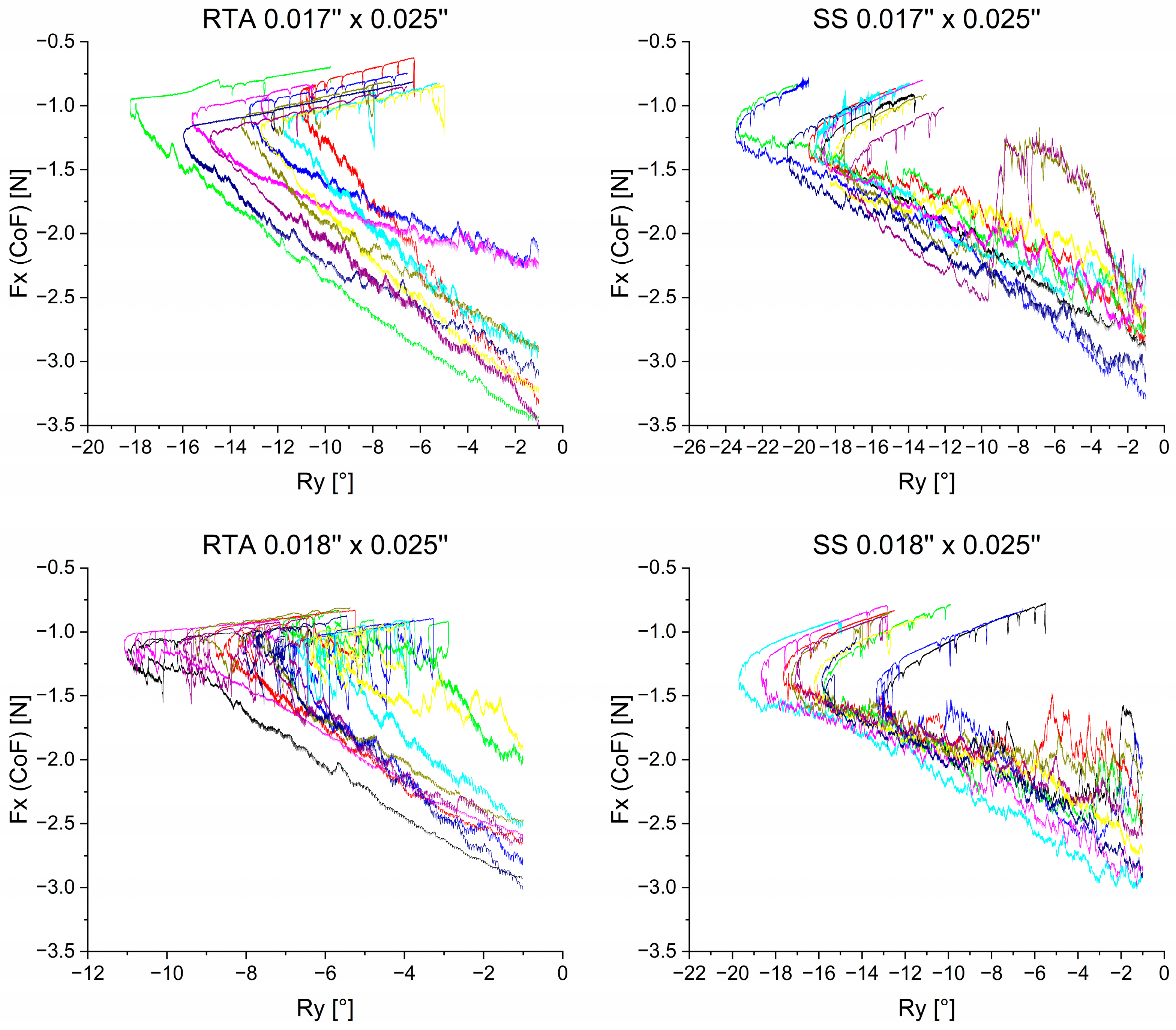
3.1. Retractive Force—Fx
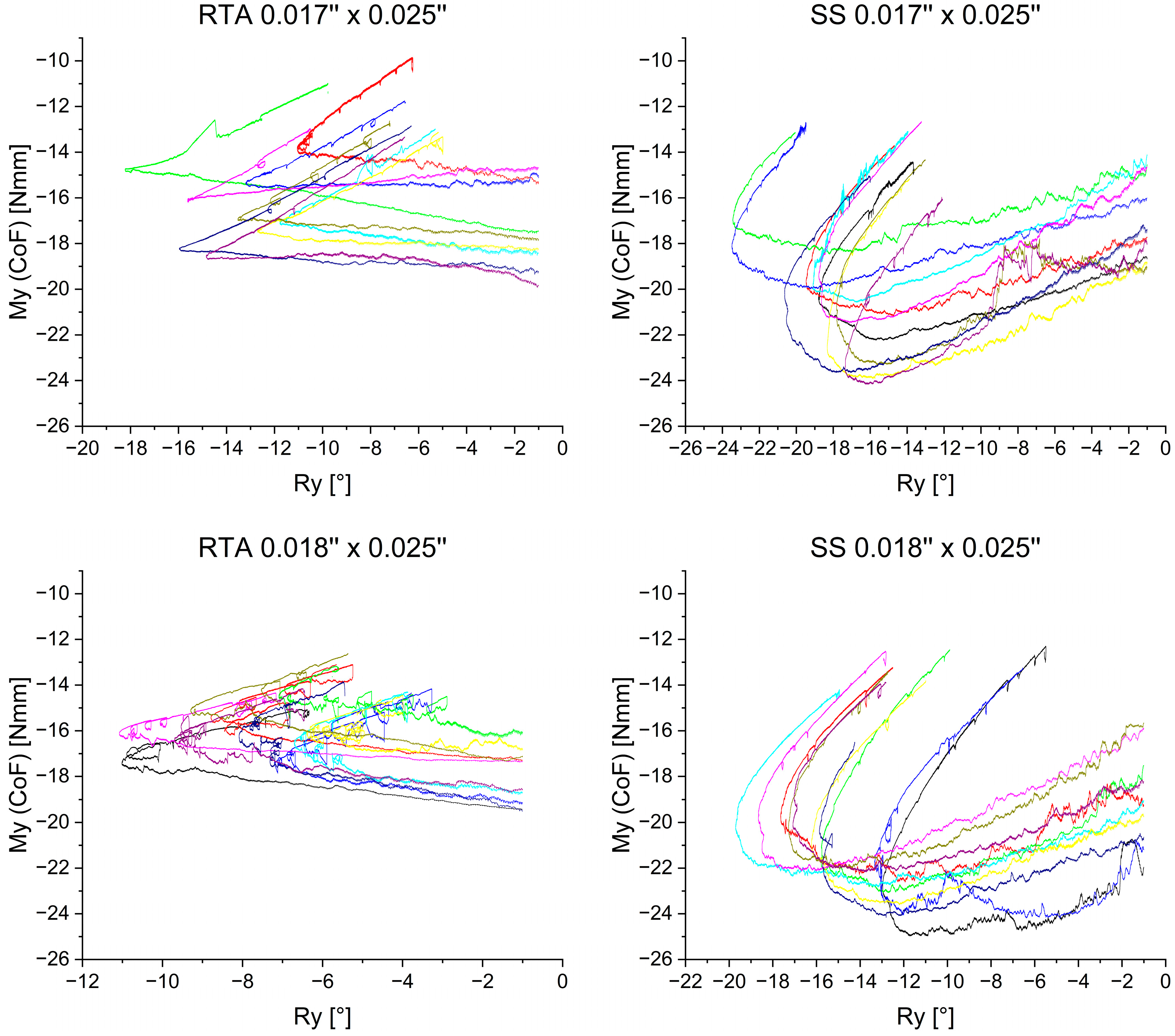
3.2. Rotational Moment—My
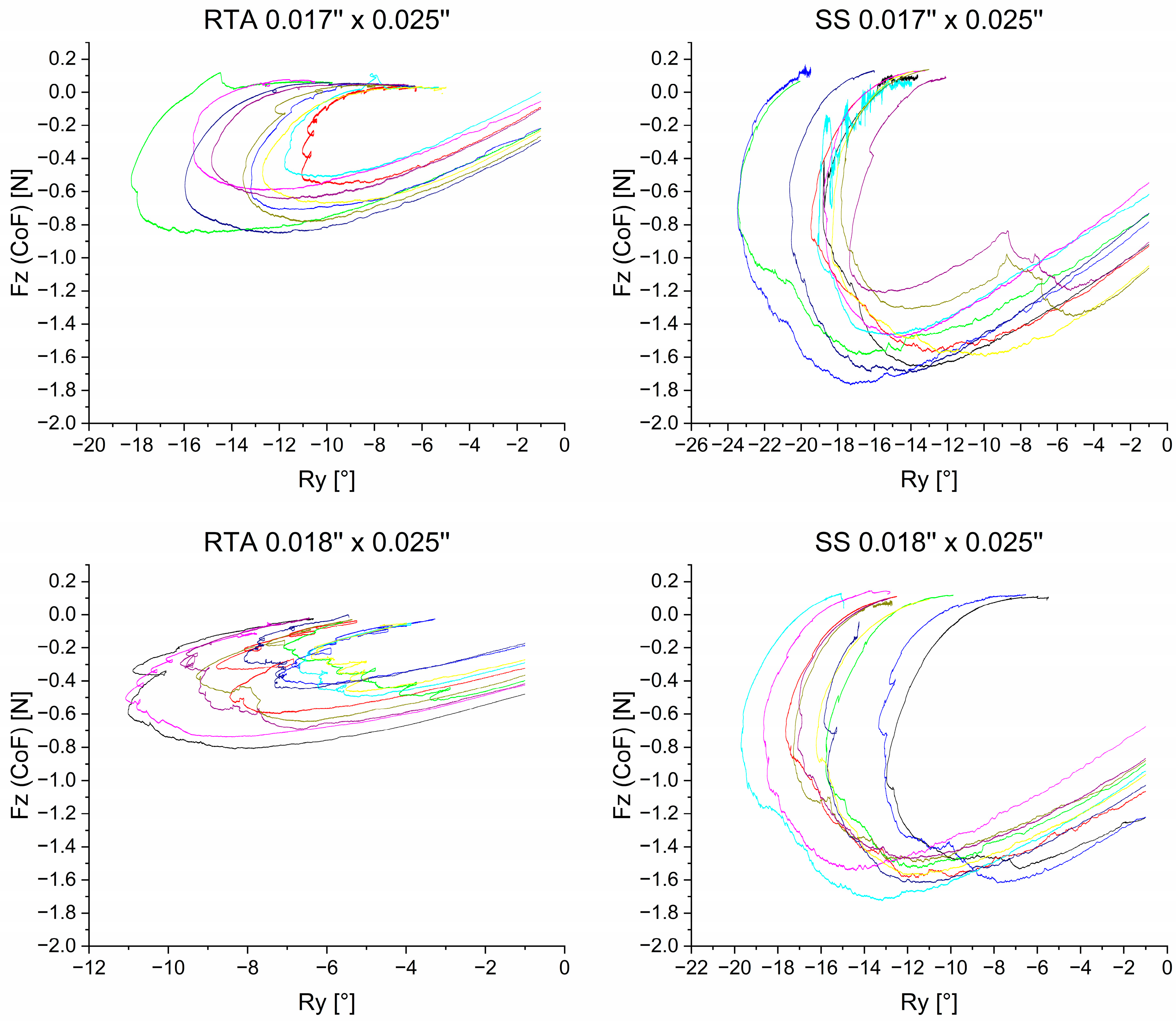
3.3. Extrusive Force—Fz
3.4. Comparison between the Specimen Groups—Statistical Analysis
4. Discussion
- SS archwires with dimensions 0.017” × 0.025” and 0.018” × 0.025” in combination with elastic chains and
- RTAs with dimensions 0.017” × 0.025” and 0.018” × 0.025” in combination with NiTi tension springs.
- The test temperature. The thermocouple shown in Figure 3 measured the temperature close to the sample, but did not directly measure the exact NiTi wire temperature. It is well possible, that the time span between insertion of the archwire (sample setup) and the start of the experiment varied due to the manual application of the mechanics. Even though the experiment didn’t start before the set temperature of 36 °C was reached, the exact temperature in the NiTi wires may have been slightly different, thus causing variations in generated initial forces.
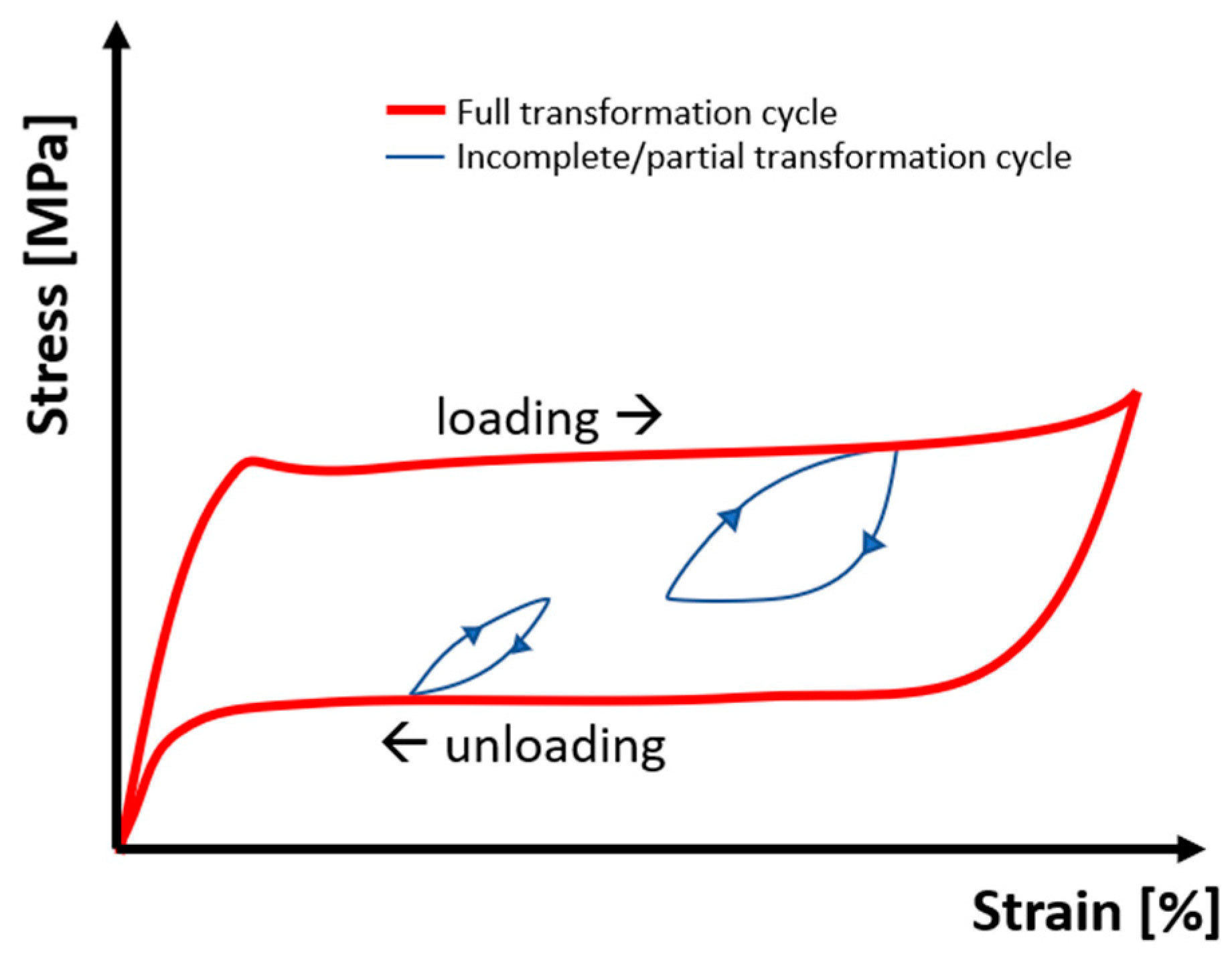
- The over-straining during assembly. It makes a huge difference, if a NiTi spring is strained and immediately attached to the bracket or if it is overstrained and released a bit before attaching to the bracket. The latter procedure yields lower forces with same identical setup and sample. Even though the laboratory operators were made aware upon this effect, it cannot fully be excluded that some differences in attaching the springs may have occurred leading to the variability of the curves shown in Figure 5 for the RTA group.
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Papageorgiou, S.N.; Koletsi, D.; Iliadi, A.; Peltomaki, T.; Eliades, T. Treatment outcome with orthodontic aligners and fixed appliances: A systematic review with meta-analyses. Eur. J. Orthod. 2020, 42, 331–343. [Google Scholar] [CrossRef]
- Tominaga, J.Y.; Tanaka, M.; Koga, Y.; Gonzales, C.; Kobayashi, M.; Yoshida, N. Optimal loading conditions for controlled movement of anterior teeth in sliding mechanics. Angle Orthod. 2009, 79, 1102–1107. [Google Scholar] [CrossRef]
- Kim, T.; Suh, J.; Kim, N.; Lee, M. Optimum conditions for parallel translation of maxillary anterior teeth under retraction force determined with the finite element method. Am. J. Orthod. Dentofac. Orthop. 2010, 137, 639–647. [Google Scholar] [CrossRef]
- Proffit, W.R.; Fields, H.W.; Larson, B.; Sarver, D.M. Contemporary Orthodontics-E-Book; Elsevier Health Sciences: Amsterdam, The Netherlands, 2018. [Google Scholar]
- Wichelhaus, A. Kieferorthopädie—Therapie Band 1: Grundlegende Behandlungskonzepte; Thieme: New York, NY, USA, 2013. [Google Scholar]
- Ribeiro, G.L.; Jacob, H.B. Understanding the basis of space closure in Orthodontics for a more efficient orthodontic treatment. Dent. Press. J. Orthod. 2016, 21, 115–125. [Google Scholar] [CrossRef]
- Sabbagh, H.; Bamidis, E.P.; Keller, A.; Stocker, T.; Baumert, U.; Hoffmann, L.; Wichelhaus, A. Force behaviour of elastic chains during a simulated gap closure in extraction therapy cases. Orthod. Craniofac Res. 2022, 26, 433–441. [Google Scholar] [CrossRef]
- Heo, W.; Nahm, D.S.; Baek, S.H. En masse retraction and two-step retraction of maxillary anterior teeth in adult Class I women. A comparison of anchorage loss. Angle Orthod. 2007, 77, 973–978. [Google Scholar] [CrossRef] [PubMed]
- Burstone, C.J. The segmented arch approach to space closure. Am. J. Orthod. 1982, 82, 361–378. [Google Scholar] [CrossRef] [PubMed]
- Schneider, P.P.; Gandini Júnior, L.G.; Monini, A.D.C.; Pinto, A.D.S.; Kim, K.B. Comparison of anterior retraction and anchorage control between en masse retraction and two-step retraction: A randomized prospective clinical trial. Angle Orthod. 2019, 89, 190–199. [Google Scholar] [CrossRef] [PubMed]
- Schneider, P.P.; Kim, K.B.; da Costa Monini, A.; Dos Santos-Pinto, A.; Gandini, L.G.J. Which one closes extraction spaces faster: En masse retraction or two-step retraction? A randomized prospective clinical trial. Angle Orthod. 2019, 89, 855–861. [Google Scholar] [CrossRef] [PubMed]
- Felemban, N.H.; Al-Sulaimani, F.F.; Murshid, Z.A.; Hassan, A.H. En masse retraction versus two-step retraction of anterior teeth in extraction treatment of bimaxillary protrusion. J. Orthod. Sci. 2013, 2, 28–37. [Google Scholar] [CrossRef] [PubMed]
- Burstone, C.J.; Choy, K. The Biomechanical Foundation of Clinical Orthodontics; Quintessence Publishing Company, Inc.: Berlin, Germany, 2015. [Google Scholar]
- Burstone, C.J.; Koenig, H.A. Optimizing anterior and canine retraction. Am. J. Orthod. 1976, 70, 1–19. [Google Scholar] [CrossRef]
- Kuc, A.E.; Kotuła, J.; Nahajowski, M.; Warnecki, M.; Lis, J.; Amm, E.; Kawala, B.; Sarul, M. Methods of Anterior Torque Control during Retraction: A Systematic Review. Diagnostics 2022, 12, 1611. [Google Scholar] [CrossRef] [PubMed]
- Wichelhaus, A.; Sander, F.G. Biomechanische Prüfung des neuen Torque-Segment-Bogens (TSB). Fortschritte Der Kieferorthopädie 1995, 56, 224–235. [Google Scholar] [CrossRef]
- Sebastian, B.; Bhuvaraghan, A.; Thiruvenkatachari, B. Orthodontic space closure in sliding mechanics: A systematic review and meta-analysis. Eur. J. Orthod. 2022, 44, 210–225. [Google Scholar] [CrossRef] [PubMed]
- Yassir, Y.A.; McIntyre, G.T.; Bearn, D.R. Orthodontic treatment and root resorption: An overview of systematic reviews. Eur. J. Orthod. 2020, 43, 442–456. [Google Scholar] [CrossRef]
- Cuoghi, O.A.; Topolski, F.; de Faria, L.P.; Ervolino, E.; Micheletti, K.R.; Miranda-Zamalloa, Y.M.; Moresca, R.; Moro, A.; de Mendonça, M.R. Correlation between pain and hyalinization during tooth movement induced by different types of force. Angle Orthod. 2019, 89, 788–796. [Google Scholar] [CrossRef] [PubMed]
- Mo, S.S.; Noh, M.K.; Kim, S.H.; Chung, K.R.; Nelson, G. Finite element study of controlling factors of anterior intrusion and torque during Temporary Skeletal Anchorage Device (TSAD) dependent en masse retraction without posterior appliances: Biocreative hybrid retractor (CH-retractor). Angle Orthod. 2020, 90, 255–262. [Google Scholar] [CrossRef]
- Parashar, A.; Aileni, K.R.; Rachala, M.R.; Shashidhar, N.R.; Mallikarjun, V.; Parik, N. Torque Loss in En-Masse Retraction of Maxillary Anterior Teeth Using Miniimplants with Force Vectors at Different Levels: 3D FEM Study. J. Clin. Diagn. Res. 2014, 8, ZC77–ZC80. [Google Scholar] [CrossRef]
- Gajda, S.; Chen, J. Comparison of three-dimensional orthodontic load systems of different commercial archwires for space closure. Angle Orthod. 2012, 82, 333–339. [Google Scholar] [CrossRef]
- Drescher, D.; Bourauel, C.; Thier, M. Application of the orthodontic measurement and simulation system (OMSS) in orthodontics. Eur. J. Orthod. 1991, 13, 169–178. [Google Scholar] [CrossRef]
- Dotzer, B.; Stocker, T.; Wichelhaus, A.; Janjic Rankovic, M.; Sabbagh, H. Biomechanical simulation of forces and moments of initial orthodontic tooth movement in dependence on the used archwire system by ROSS (Robot Orthodontic Measurement & Simulation System). J. Mech. Behav. Biomed. Mater. 2023, 144, 105960. [Google Scholar] [CrossRef]
- Haas, E.; Schmid, A.; Stocker, T.; Wichelhaus, A.; Sabbagh, H. Force-Controlled Biomechanical Simulation of Orthodontic Tooth Movement with Torque Archwires Using HOSEA (Hexapod for Orthodontic Simulation, Evaluation and Analysis). Bioengineering 2023, 10, 1055. [Google Scholar] [CrossRef]
- Wang, J.; Rousso, C.; Christensen, B.I.; Li, P.; Kau, C.H.; MacDougall, M.; Lamani, E. Ethnic differences in the root to crown ratios of the permanent dentition. Orthod. Craniofac. Res. 2019, 22, 99–104. [Google Scholar] [CrossRef] [PubMed]
- Cignoni, P.; Callieri, M.; Corsini, M.; Dellepiane, M.; Ganovelli, F.; Ranzuglia, G. Meshlab: An Open-Source Mesh Processing Tool. In Proceedings of the Eurographics Italian Chapter Conference, Salerno, Italy, 2–4 July 2008; pp. 129–136. [Google Scholar]
- Andrews, L.F. The straight-wire appliance. Br. J. Orthod. 1979, 6, 125–143. [Google Scholar] [CrossRef] [PubMed]
- Kojima, Y.; Fukui, H. Numeric simulations of en-masse space closure with sliding mechanics. Am. J. Orthod. Dentofac. Orthop. 2010, 138, 702.e1–702.e6; discussion 702–704. [Google Scholar] [CrossRef]
- George, S.S.; Reddy, T.R.J.; Kv, S.K.; Chaudhary, G.; Farooq, U.; Cherukuri, V.; Likitha, C. The Influence of Heights of Power Arm for Controlled Anterior Teeth Movement in Sliding Mechanics: A 3D FEM Study. Cureus 2022, 14, e25976. [Google Scholar] [CrossRef]
- Gmyrek, H.; Bourauel, C.; Richter, G.; Harzer, W. Torque capacity of metal and plastic brackets with reference to materials, application, technology and biomechanics. J. Orofac. Orthop. Fortschritte Der Kieferorthopädie 2002, 63, 113–128. [Google Scholar] [CrossRef]
- Weltman, B.; Vig, K.W.; Fields, H.W.; Shanker, S.; Kaizar, E.E. Root resorption associated with orthodontic tooth movement: A systematic review. Am. J. Orthod. Dentofac. Orthop. 2010, 137, 462–476; discussion 412A. [Google Scholar] [CrossRef]
- Sander, F.G.; Schwenzer, N.; Ehrenfeld, M. Kieferorthopädie; Thieme: New York, NY, USA, 2011. [Google Scholar]
- Judith, T.; Mayer, F.S.; Bernd, G. Lapatki. Klinischer Arbeitsbereich von Superelastischen Drähten. In Proceedings of the 95. Jahrestagung der DGKFO, Stuttgart, Germany, 27 September 2023; p. 146. [Google Scholar]
- Rebelo, N.; Gong, X.-Y.; Hall, A.; Pelton, A.; Duerig, T. Finite Element Analysis on the Cyclic Properties of Superelastic Nitinol. In Proceedings of the 2004 ABAQUS Users’ Conference, Boston, MA, USA, 25–27 May 2004. [Google Scholar]
- Frias Cortez, M.A.; Bourauel, C.; Reichert, C.; Jäger, A.; Reimann, S. Numerical and biomechanical analysis of orthodontic treatment of recovered periodontally compromised patients. J. Orofac. Orthop. 2022, 83, 255–268. [Google Scholar] [CrossRef]
- Badawi, H.M.; Toogood, R.W.; Carey, J.P.; Heo, G.; Major, P.W. Three-dimensional orthodontic force measurements. Am. J. Orthod. Dentofac. Orthop. 2009, 136, 518–528. [Google Scholar] [CrossRef]
- Reimann, S.; Keilig, L.; Jager, A.; Bourauel, C. Biomechanical finite-element investigation of the position of the centre of resistance of the upper incisors. Eur. J. Orthod. 2007, 29, 219–224. [Google Scholar] [CrossRef] [PubMed]
- Schmidt, F.; Geiger, M.E.; Jäger, R.; Lapatki, B.G. Comparison of methods to determine the centre of resistance of teeth. Comput. Methods Biomech. Biomed. Eng. 2016, 19, 1673–1682. [Google Scholar] [CrossRef] [PubMed]
- Li, H.; Stocker, T.; Bamidis, E.P.; Sabbagh, H.; Baumert, U.; Mertmann, M.; Wichelhaus, A. Effect of different media on frictional forces between tribological systems made from self-ligating brackets in combination with different stainless steel wire dimensions. Dent. Mater. J. 2021, 40, 1250–1256. [Google Scholar] [CrossRef] [PubMed]
- Bilinska, M.; Golliez, I.M.; Dalstra, M. Force Systems Produced by Different Cantilever Configurations during Deactivation. Materials 2022, 15, 4815. [Google Scholar] [CrossRef]
- Elkholy, F.; Schmidt, F.; Jäger, R.; Lapatki, B.G. Forces and moments delivered by novel, thinner PET-G aligners during labiopalatal bodily movement of a maxillary central incisor: An in vitro study. Angle Orthod. 2016, 86, 883–890. [Google Scholar] [CrossRef]

| Archwire Group | Material Identification | Anterior Torque α (°) | Sample Size | Archwire Size Anterior Segment | Archwire Size Posterior Segments | Retractive Element |
|---|---|---|---|---|---|---|
| SS | X10CrNi 18-8 | 28–32 | 10 | 0.017” × 0.025” | 0.017” × 0.025” | Elastic chain |
| SS | X10CrNi 18-8 | 28–32 | 10 | 0.018” × 0.025” | 0.018” × 0.025” | Elastic chain |
| RTA | X5CrNi18-10 Nickel Titanium | 30 | 10 | 0.017” × 0.025” | 0.017” × 0.022” | Nitinol spring |
| RTA | X5CrNi18-10 Nickel Titanium | 30 | 10 | 0.018” × 0.025” | 0.018” × 0.022” | Nitinol spring |
| Sample Group | Fx,max[N] | Ry,corr [°] | My,max [Nmm] | Ry,corr [°] | Fz,max [N] | Ry,corr [°] | Ry max [°] | ||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| (a) RTA 0.017” × 0.025” | Mean (SD) | −2.942 (0.497) | −1.312 (0.340) | −17.436 (1.645) | c,d | −3.307 (4.573) | −0.691 (0.117) | c,d | −11.716 (1.776) | −14.008 (2.157) | b,c |
| CI [95] | [−3.298; −2.586] | [−1.555; −1.069] | [−18.613; −16.259] | [−6.578; −0.036] | [−0.774; −0.607] | [−12.986; −10.445] | [−15.551; −12.465] | ||||
| (b) RTA 0.018” × 0.025” | Mean (SD) | −2.572 (0.350) | −1.089 (0.114) | −18.099 (1.154) | c,d | 1.410 (0.785 | −0.585 (0.134) | c,d | −6.370 (1.528) | −8.535 (1.718) | c,d |
| CI [95] | [−2.823; −2.322] | [−1.170; −1.008] | [−18.925; −17.274] | [−1.972; −0.849] | [−0.681; −0.489] | [−7.464; −5.277] | [−9.764; −7.305] | ||||
| (c) SS 0.017” × 0.025” | Mean (SD) | −2.839 (0.239) | −1.316 (0.456) | −21.899 (1.897) | a,b | −16.573 (1.371) | −1.540 (0.166) | a,b | −13.492 (3.641) | −19.717 (2.164) | a,b |
| CI [95] | [−3.009; −2.668] | [−1.642; −0.990] | [−23.256; −20.541] | [−17.553; −15.592] | [−1.658; −1.421] | [−16.097; −10.888] | [−21.265; −18.170] | ||||
| (d) SS 0.018” × 0.025” | Mean (SD) | −2.674 (0.247) | −1.551 (0.831) | −23.180 (1.015) | a,b | −12.623 (2.662) | [−1.570 (0.073) | a,b | −11.168 (2.266) | −16.452 (2.126) | b |
| CI [95] | [−2.850; −2.497] | [−2.145; −0.957] | [−23.906; −22.454] | [−14.527; −10.718] | [−1.622; −1.518] | [−12.789; −9.547] | [−17.972; −14.931] |
| Sample Group | Fx [N] | My [Nmm] | Fz [N] | ||
|---|---|---|---|---|---|
| (a) RTA 0.017” × 0.025” | Mean (SD) | −2.891 (0.513) | −17.126 (1.904) | −0.169 (0.099) | c,d |
| CI [95] | [−3.258; −2.525] | [−18.488; −15.764] | [−0.240; −0.098] | ||
| (b) RTA 0.018” × 0.025” | Mean (SD) | −2.549 (0.358) | −17.996 (1.212) | −0.332 (0.104) | c,d |
| CI [95] | [−2.805; −2.293] | [−18.863; −17.129] | [−0.407; −0.258] | ||
| (c) SS 0.017” × 0.025” | Mean (SD) | −2.720 (0.304) | −16.885 (1.898) | −0.827 (0.174) | a,b |
| CI [95] | [−2.937; −2.502] | [−18.243; −15.527] | [−0.951; −0.703] | ||
| (d) SS 0.018” × 0.025” | Mean (SD) | −2.521 (0.316) | −18.920 (2.116) | −0.976 (0.167) | a,b |
| CI [95] | [−2.747; −2.295] | [−20.434; −17.406] | [−1.095; −0.856] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sabbagh, H.; Haas, E.; Baumert, U.; Seidel, C.L.; Hötzel, L.; Wichelhaus, A. Biomechanical Simulation of Orthodontic En-Bloc Retraction Comparing Compound Technique and Sliding Mechanics Using a HOSEA Robotic Device. Bioengineering 2024, 11, 153. https://doi.org/10.3390/bioengineering11020153
Sabbagh H, Haas E, Baumert U, Seidel CL, Hötzel L, Wichelhaus A. Biomechanical Simulation of Orthodontic En-Bloc Retraction Comparing Compound Technique and Sliding Mechanics Using a HOSEA Robotic Device. Bioengineering. 2024; 11(2):153. https://doi.org/10.3390/bioengineering11020153
Chicago/Turabian StyleSabbagh, Hisham, Ellen Haas, Uwe Baumert, Corinna Lesley Seidel, Linus Hötzel, and Andrea Wichelhaus. 2024. "Biomechanical Simulation of Orthodontic En-Bloc Retraction Comparing Compound Technique and Sliding Mechanics Using a HOSEA Robotic Device" Bioengineering 11, no. 2: 153. https://doi.org/10.3390/bioengineering11020153
APA StyleSabbagh, H., Haas, E., Baumert, U., Seidel, C. L., Hötzel, L., & Wichelhaus, A. (2024). Biomechanical Simulation of Orthodontic En-Bloc Retraction Comparing Compound Technique and Sliding Mechanics Using a HOSEA Robotic Device. Bioengineering, 11(2), 153. https://doi.org/10.3390/bioengineering11020153






