Localized Refractive Changes Induced by Symmetric and Progressive Asymmetric Intracorneal Ring Segments Assessed with a 3D Finite-Element Model
Abstract
1. Introduction
2. Materials and Methods
2.1. Finite Element Modelling
2.2. Curvature Analysis
2.3. Optical Aberrations
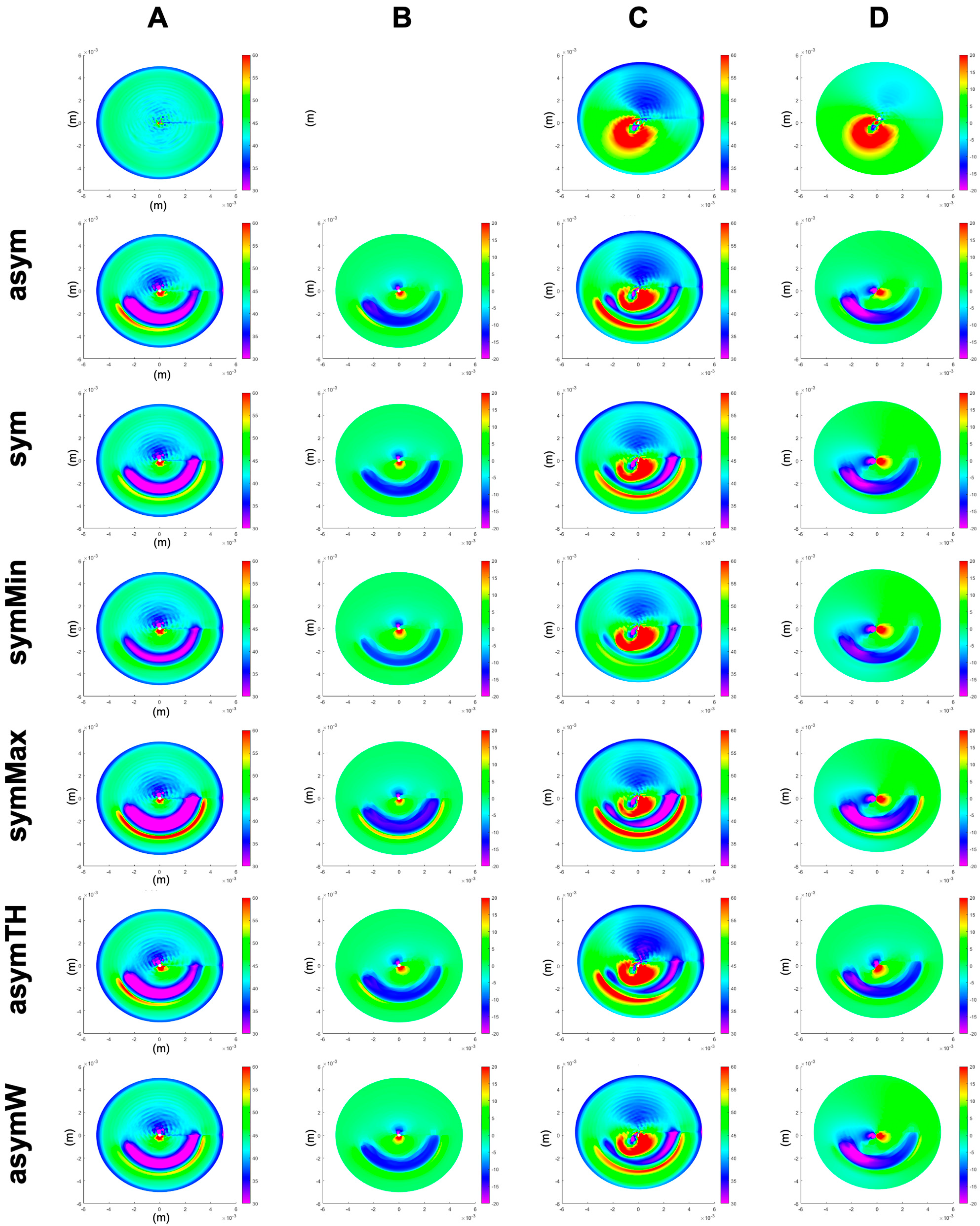
3. Results
3.1. Average Anterior Corneal Curvature
3.2. Local Curvature
3.3. Optical Aberrations
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Piñero, D.P.; Alcón, N. Corneal biomechanics: A review. Clin. Exp. Optom. 2015, 98, 107–116. [Google Scholar] [CrossRef]
- Kling, S.; Hafezi, F. Corneal biomechanics—A review. Ophthalmic Physiol. Opt. 2017, 37, 240–252. [Google Scholar] [CrossRef] [PubMed]
- Roy, A.S.; Dupps, W.J. Patient-specific computational modeling of keratoconus progression and differential responses to collagen cross-linking. Investig. Ophthalmol. Vis. Sci. 2011, 52, 9174–9187. [Google Scholar]
- Pinsky, P.M.; Datye, D.V. A microstructurally-based finite element model of the incised human cornea. J. Biomech. 1991, 24, 907–922. [Google Scholar] [CrossRef] [PubMed]
- Glass, D.H.; Roberts, C.J.; Litsky, A.S.; Weber, P.A. A viscoelastic biomechanical model of the cornea describing the effect of viscosity and elasticity on hysteresis. Investig. Ophthalmol. Vis. Sci. 2008, 49, 3919–3926. [Google Scholar] [CrossRef] [PubMed]
- Pandolfi, A.; Manganiello, F. A model for the human cornea: Constitutive formulation and numerical analysis. Biomech. Model. Mechanobiol. 2006, 5, 237–246. [Google Scholar] [CrossRef] [PubMed]
- Anderson, K. Application of structural analysis to the mechanical behavior of the cornea. J. R. Soc. Interface 2004, 1, 1–13. [Google Scholar] [CrossRef]
- Kling, S.; Marcos, S. Finite-element modeling of intrastromal ring segment implantation into a hyperelastic cornea. Investig. Ophthalmol. Vis. Sci. 2013, 54, 881–889. [Google Scholar] [CrossRef]
- Ariza-Gracia, M.Á.; Flecha-Lescún, J.; Büchler, P.; Calvo, B. Corneal biomechanics after intrastromal ring surgery: Optomechanical in silico assessment. Transl. Vis. Sci. Technol. 2020, 9, 26. [Google Scholar] [CrossRef]
- Lago, M.; Rupérez, M.; Monserrat, C.; Martínez-Martínez, F.; Martínez-Sanchis, S.; Larra, E.; Díez-Ajenjo, M.; Peris-Martínez, C. Patient-specific simulation of the intrastromal ring segment implantation in corneas with keratoconus. J. Mech. Behav. Biomed. Mater. 2015, 51, 260–268. [Google Scholar] [CrossRef]
- Alfonso, J.; Lisa, C.; Fernández-Vega Cueto, L.; Poo, A.; Madrid, D. Clasificación del queratocono basada en fenotipos clínicos. Influencia del astigmatismo congénito en la morfología del queratocono. In Biomecánica y Arquitectura Corneal; Elsevier España: Barcelona, Spain, 2014; pp. 165–184. [Google Scholar] [CrossRef]
- Pinero, D.P.; Alio, J.L.; Teus, M.A.; Barraquer, R.I.; Uceda-Montanés, A. Modeling the intracorneal ring segment effect in keratoconus using refractive, keratometric, and corneal aberrometric data. Investig. Ophthalmol. Vis. Sci. 2010, 51, 5583–5591. [Google Scholar] [CrossRef] [PubMed]
- Prisant, O.; Pottier, E.; Guedj, T.; Xuan, T.H. Clinical outcomes of an asymmetric model of intrastromal corneal ring segments for the correction of keratoconus. Cornea 2020, 39, 155–160. [Google Scholar] [CrossRef] [PubMed]
- Torquetti, L.; Cunha, P.; Luz, A.; Kwitko, S.; Carrion, M.; Rocha, G.; Signorelli, A.; Coscarelli, S.; Ferrara, G.; Bicalho, F.; et al. Clinical outcomes after implantation of 320-arc length intrastromal corneal ring segments in keratoconus. Cornea 2018, 37, 1299–1305. [Google Scholar] [CrossRef]
- Al-Tuwairqi, W.S.; Osuagwu, U.L.; Razzouk, H.; AlHarbi, A.; Ogbuehi, K.C. Clinical evaluation of two types of intracorneal ring segments (ICRS) for keratoconus. Int. Ophthalmol. 2017, 37, 1185–1198. [Google Scholar] [CrossRef][Green Version]
- Vega-Estrada, A.; Chorro, E.; Sewelam, A.; Alio, J.L. Clinical outcomes of a new asymmetric intracorneal ring segment for the treatment of keratoconus. Cornea 2019, 38, 1228–1232. [Google Scholar] [CrossRef] [PubMed]
- Hayes, S.; Boote, C.; Lewis, J.; Sheppard, J.; Abahussin, M.; Quantock, A.J.; Purslow, C.; Votruba, M.; Meek, K.M. Comparative study of fibrillar collagen arrangement in the corneas of primates and other mammals. Anat. Rec. 2007, 290, 1542–1550. [Google Scholar] [CrossRef] [PubMed]
- Meek, K.M.; Tuft, S.J.; Huang, Y.; Gill, P.S.; Hayes, S.; Newton, R.H.; Bron, A.J. Changes in collagen orientation and distribution in keratoconus corneas. Investig. Ophthalmol. Vis. Sci. 2005, 46, 1948–1956. [Google Scholar] [CrossRef]
- Scarcelli, G.; Besner, S.; Pineda, R.; Kalout, P.; Yun, S.H. In vivo biomechanical mapping of normal and keratoconus corneas. JAMA Ophthalmol. 2015, 133, 480–482. [Google Scholar] [CrossRef]
- Flecha-Lescún, J.; Calvo, B.; Zurita, J.; Ariza-Gracia, M.Á. Template-based methodology for the simulation of intracorneal segment ring implantation in human corneas. Biomech. Model. Mechanobiol. 2018, 17, 923–938. [Google Scholar] [CrossRef]
- Atchison, D.A.; Smith, G.; Smith, G. Optics of the Human Eye; Butterworth-Heinemann: Oxford, UK, 2000; Volume 35. [Google Scholar]
- García de Oteyza, G.; Álvarez de Toledo, J.; Barraquer, R.I.; Kling, S. Refractive changes of a new asymmetric intracorneal ring segment with variable thickness and base width: A 2D finite-element model. PLoS ONE 2021, 16, e0257222. [Google Scholar] [CrossRef]
- Piñero, D.P.; Nieto, J.C.; Lopez-Miguel, A. Characterization of corneal structure in keratoconus. J. Cataract. Refract. Surg. 2012, 38, 2167–2183. [Google Scholar] [CrossRef]
- Alió, J.L.; Piñero, D.P.; Alesón, A.; Teus, M.A.; Barraquer, R.I.; Murta, J.; Maldonado, M.J.; de Luna, G.C.; Gutiérrez, R.; Villa, C.; et al. Keratoconus-integrated characterization considering anterior corneal aberrations, internal astigmatism, and corneal biomechanics. J. Cataract. Refract. Surg. 2011, 37, 552–568. [Google Scholar] [CrossRef]
- Alfonso, J.F.; Cueto, L.F.-V.; Baamonde, B.; Merayo-Lloves, J.; Madrid-Costa, D.; Montés-Micó, R. Inferior intrastromal corneal ring segments in paracentral keratoconus with no coincident topographic and coma axis. J. Refract. Surg. 2013, 29, 266–272. [Google Scholar] [CrossRef]
- Kammoun, H.; Piñero, D.P.; Álvarez de Toledo, J.; Barraquer, R.I.; García de Oteyza, G. Clinical outcomes of femtosecond laser-assisted implantation of asymmetric ICRS in keratoconus with No coincidence of topographic and comatic axes. J. Refract. Surg. 2021, 37, 693–699. [Google Scholar] [CrossRef]
- Torres-Netto, E.A.; Kling, S. Corneal Strain Induced by Intracorneal Ring Segment Implantation Visualized With Optical Coherence Elastography. J. Refract. Surg. 2022, 38, 210–216. [Google Scholar] [CrossRef] [PubMed]
- Flecha-Lescún, J. Computational Planning Tools in Ophthalmology: Intrastromal Corneal Ring Surgery. Ph.D. Thesis, Universidad de Zaragoza, Zaragoza, Spain, 2021. [Google Scholar]
| C1 | C2 | C3 | d | th | ||
|---|---|---|---|---|---|---|
| healthy | anterior | 35.5 kPa | 3.2 kPa | 1.9 kPa | 10−5 Pa | 385 µm |
| posterior | 32.0 kPa | 2.9 kPa | 1.7 kPa | 10−5 Pa | 165 µm | |
| KC region1 | anterior | 24.9 kPa | 2.2 kPa | 1.3 kPa | 10−5 Pa | 270 µm |
| posterior | 22.4 kPa | 2.0 kPa | 1.2 kPa | 10−5 Pa | 116 µm | |
| KC region 2 | anterior | 10.7 kPa | 1.0 kPa | 0.57 kPa | 10−5 Pa | 193 µm |
| posterior | 9.6 kPa | 0.86 kPa | 0.51 kPa | 10−5 Pa | 83 µm | |
| sclera | - | 0.8 MPa | 56.1 MPa | 2332 MPa | 10−5 Pa | 1000 µm |
| E | ρ | ν | ||||
| ICRS | - | 3.3 GPa | 1062 kg/m3 | 0.40 | variable |
| ANTERIOR | ||||||||
|---|---|---|---|---|---|---|---|---|
| (mm) | Rx | Ry | ∆Rx | ∆Ry | ∆AL | Qx | Qy | ∆dpt |
| healthy | 7.37 | 7.62 | - | - | - | −0.25 | −0.10 | - |
| healthy asym | 8.05 | 7.95 | 0.68 | 0.33 | −0.04 | 1.21 | −2.07 | −3.17 |
| healthy sym | 7.96 | 8.14 | 0.59 | 0.52 | −0.04 | −0.13 | −0.22 | −3.44 |
| healthy symMax | 8.36 | 8.35 | 0.99 | 0.73 | −0.04 | 0.98 | −1.40 | −5.14 |
| healthy symMin | 7.77 | 7.87 | 0.40 | 0.25 | −0.03 | −0.18 | −0.17 | −2.09 |
| healthy asymW | 7.97 | 8.14 | 0.60 | 0.52 | −0.04 | −0.15 | −0.21 | −3.47 |
| healthy asymTH | 7.96 | 8.13 | 0.59 | 0.50 | −0.04 | 0.65 | −0.94 | −3.40 |
| KC | 6.15 | 7.24 | - | - | 0.11 | −1.55 | 1.33 | - |
| KC asym | 5.88 | 7.72 | −0.26 | 0.48 | −0.04 | −3.83 | 2.19 | −0.89 |
| KC sym | 6.34 | 7.61 | 0.19 | 0.36 | −0.04 | −2.17 | 1.76 | −2.23 |
| KC_symMax | 6.89 | 7.61 | 0.75 | 0.36 | −0.05 | −0.19 | −0.18 | −4.29 |
| KC_symMin | 6.20 | 6.61 | 0.05 | −0.63 | −0.04 | −1.76 | −2.54 | 2.52 |
| KC_asymW | 6.44 | 7.57 | 0.29 | 0.33 | −0.04 | −1.63 | 1.53 | −2.49 |
| KC_asymTH | 6.44 | 6.79 | 0.30 | −0.46 | −0.04 | −0.01 | −3.77 | 0.68 |
| POSTERIOR | ||||||||
| healthy | 7.91 | 7.97 | - | - | - | 0.63 | 0.37 | - |
| healthy asym | 8.35 | 8.70 | 0.99 | 1.08 | - | 5.18 | 7.85 | 0.39 |
| healthy sym | 7.17 | 7.40 | −0.20 | −0.22 | - | −1.39 | 1.03 | −0.51 |
| healthy symMax | 8.74 | 8.69 | 1.37 | 1.07 | - | 8.16 | 9.08 | 0.50 |
| healthy symMin | 7.51 | 7.45 | 0.14 | −0.17 | - | −0.19 | −0.20 | −0.35 |
| healthy asymW | 7.18 | 7.37 | −0.19 | −0.25 | - | −1.48 | 1.08 | −0.52 |
| healthy asymTH | 7.10 | 7.46 | −0.26 | −0.17 | - | −1.53 | 1.10 | −0.52 |
| KC | 6.18 | 6.81 | - | - | - | −1.13 | −1.02 | - |
| KC asym | 6.23 | 7.55 | 0.08 | 0.31 | - | 1.11 | 7.05 | 0.40 |
| KC sym | 6.34 | 7.71 | 0.19 | 0.47 | - | 0.66 | 7.32 | 0.52 |
| KC_symMax | 6.54 | 7.58 | 0.40 | 0.33 | - | 2.88 | 7.59 | 0.56 |
| KC_symMin | 5.86 | 7.18 | −0.28 | −0.07 | - | −2.37 | 4.01 | 0.03 |
| KC_asymW | 6.48 | 7.71 | 0.34 | 0.47 | - | 1.42 | 7.36 | 0.59 |
| KC_asymTH | 5.29 | 6.35 | −0.86 | −0.89 | - | −2.56 | 2.42 | −0.80 |
| Zernike Coefficient | ∆ Healthy | ∆ KC | Description | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| asym | sym | symMax | symMin | varWidth | varTH | pre-op | asym | sym | symMax | symMin | varWidth | varTH | ||
| 1 | 5.11 | 5.52 | 2.85 | 7.69 | 5.54 | 5.10 | 14.25 | 1.37 | −2.04 | −4.19 | −2.04 | −1.74 | 4.77 | vertical tilt |
| 2 | −3.81 | −0.73 | −0.13 | −1.16 | 0.46 | −5.03 | 7.57 | −9.17 | −11.35 | −10.87 | −11.35 | −9.86 | −4.82 | horizontal tilt |
| 3 | 1.37 | −0.12 | −0.27 | −0.03 | −0.48 | 1.72 | 2.60 | 1.28 | −0.19 | −0.45 | −0.19 | −0.55 | 1.51 | oblique primary astigmatism |
| 4 | 1.18 | 1.21 | 2.24 | 0.34 | 1.23 | 1.15 | −4.64 | 2.90 | 2.75 | 3.95 | 2.75 | 2.83 | 3.07 | defocus |
| 5 | −0.16 | 0.40 | −1.18 | 1.29 | 0.48 | −0.37 | 1.61 | −1.41 | −1.57 | −3.35 | −1.57 | −1.28 | −1.01 | vertical/horizontal primary astigmatism |
| 6 | 0.08 | 0.01 | −0.40 | 0.31 | 0.02 | 0.10 | −0.25 | −0.48 | −0.51 | −1.01 | −0.51 | −0.41 | −0.47 | vertical trefoil |
| 7 | −1.89 | −1.81 | −2.72 | −0.99 | −1.86 | −1.84 | 5.29 | −3.42 | −3.13 | −4.31 | −3.13 | −3.22 | −3.38 | vertical coma |
| 8 | −0.28 | 0.28 | 0.42 | 0.16 | 0.05 | −0.06 | 2.37 | −0.67 | 0.36 | 0.44 | 0.36 | 0.00 | −0.62 | horizontal coma |
| 9 | −0.21 | −0.06 | 0.20 | −0.28 | 0.00 | −0.29 | 1.42 | −0.54 | −0.08 | 0.05 | −0.08 | −0.04 | −0.71 | oblique trefoil |
| 10 | 0.25 | −0.16 | −0.31 | −0.05 | −0.20 | 0.28 | −0.40 | 0.63 | −0.04 | −0.03 | −0.04 | −0.01 | 0.75 | oblique quadrafoil |
| 11 | 0.21 | −0.06 | −0.07 | −0.04 | 0.08 | 0.06 | −2.49 | 0.48 | −0.09 | 0.08 | −0.09 | 0.13 | 0.50 | oblique secondary astigmatism |
| 12 | 0.86 | 0.85 | 1.01 | 0.65 | 0.86 | 0.85 | 1.68 | 1.38 | 0.83 | 1.10 | 0.83 | 0.92 | 1.85 | primary spherical |
| 13 | −0.17 | −0.13 | −0.46 | 0.07 | −0.15 | −0.17 | 1.63 | −0.77 | −0.90 | −1.30 | −0.90 | −0.84 | −0.60 | vertical secondary astigmatism |
| 14 | −0.49 | −0.26 | −0.42 | −0.13 | −0.26 | −0.49 | −0.46 | −0.55 | −0.48 | −0.55 | −0.48 | −0.43 | −0.53 | vertical quadrafoil |
| ∑ low order | −20.77 | −21.67 | −22.36 | −21.56 | −21.92 | −20.66 | −22.59 | −20.81 | −22.59 | −23.43 | −22.59 | −22.59 | −20.02 | |
| ∑ high order | 3.16 | 3.47 | 2.06 | 4.52 | 3.37 | 3.26 | 9.58 | 9.69 | 9.58 | 8.08 | 9.58 | 9.70 | 10.41 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
García de Oteyza, G.; Álvarez de Toledo, J.; Barraquer, R.I.; Kling, S. Localized Refractive Changes Induced by Symmetric and Progressive Asymmetric Intracorneal Ring Segments Assessed with a 3D Finite-Element Model. Bioengineering 2023, 10, 1014. https://doi.org/10.3390/bioengineering10091014
García de Oteyza G, Álvarez de Toledo J, Barraquer RI, Kling S. Localized Refractive Changes Induced by Symmetric and Progressive Asymmetric Intracorneal Ring Segments Assessed with a 3D Finite-Element Model. Bioengineering. 2023; 10(9):1014. https://doi.org/10.3390/bioengineering10091014
Chicago/Turabian StyleGarcía de Oteyza, Gonzalo, Juan Álvarez de Toledo, Rafael I. Barraquer, and Sabine Kling. 2023. "Localized Refractive Changes Induced by Symmetric and Progressive Asymmetric Intracorneal Ring Segments Assessed with a 3D Finite-Element Model" Bioengineering 10, no. 9: 1014. https://doi.org/10.3390/bioengineering10091014
APA StyleGarcía de Oteyza, G., Álvarez de Toledo, J., Barraquer, R. I., & Kling, S. (2023). Localized Refractive Changes Induced by Symmetric and Progressive Asymmetric Intracorneal Ring Segments Assessed with a 3D Finite-Element Model. Bioengineering, 10(9), 1014. https://doi.org/10.3390/bioengineering10091014







