In-Plane Free Vibration Analysis of a Scimitar-Type Rotating Curved Beam Using the Adomian Modified Decomposition Method
Abstract
1. Introduction
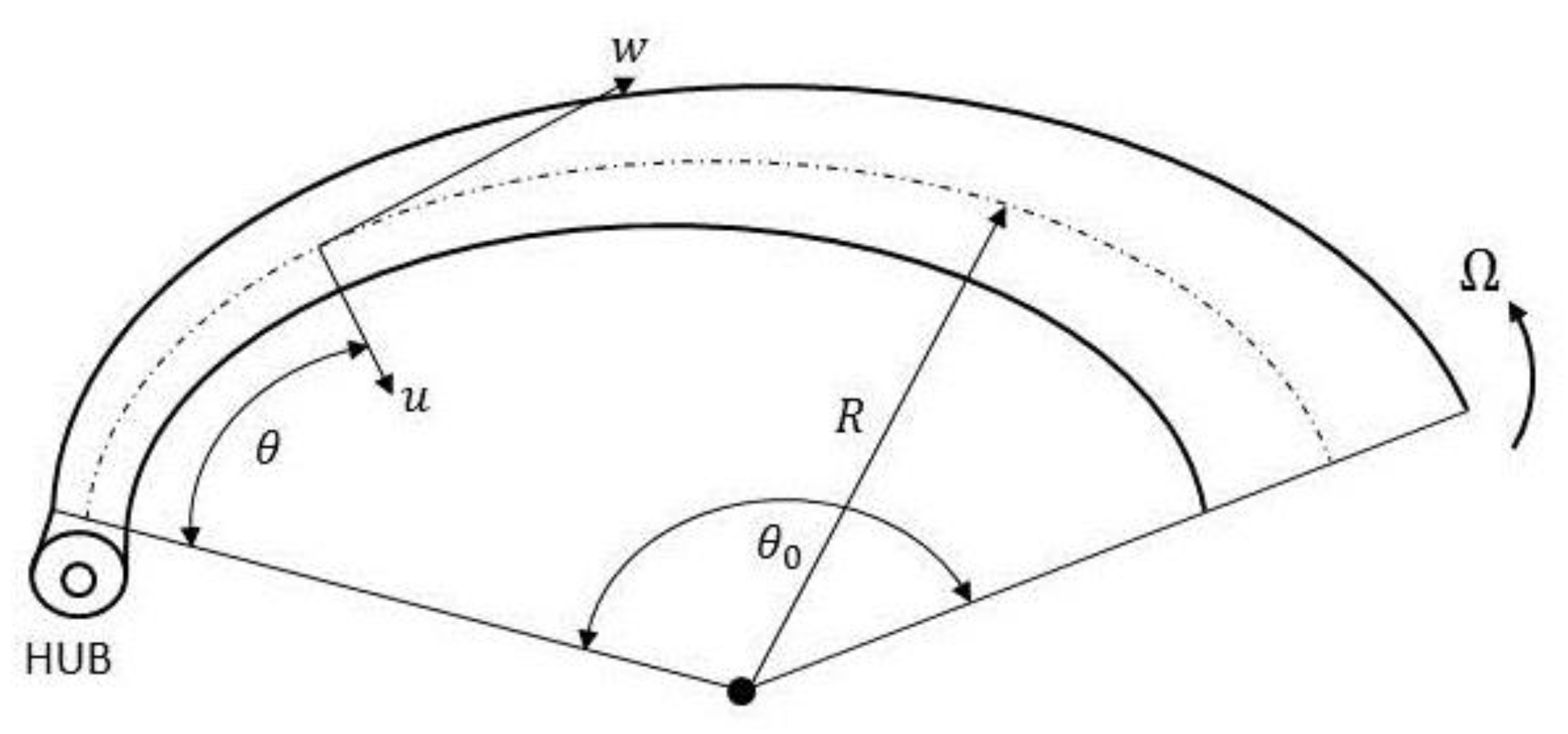
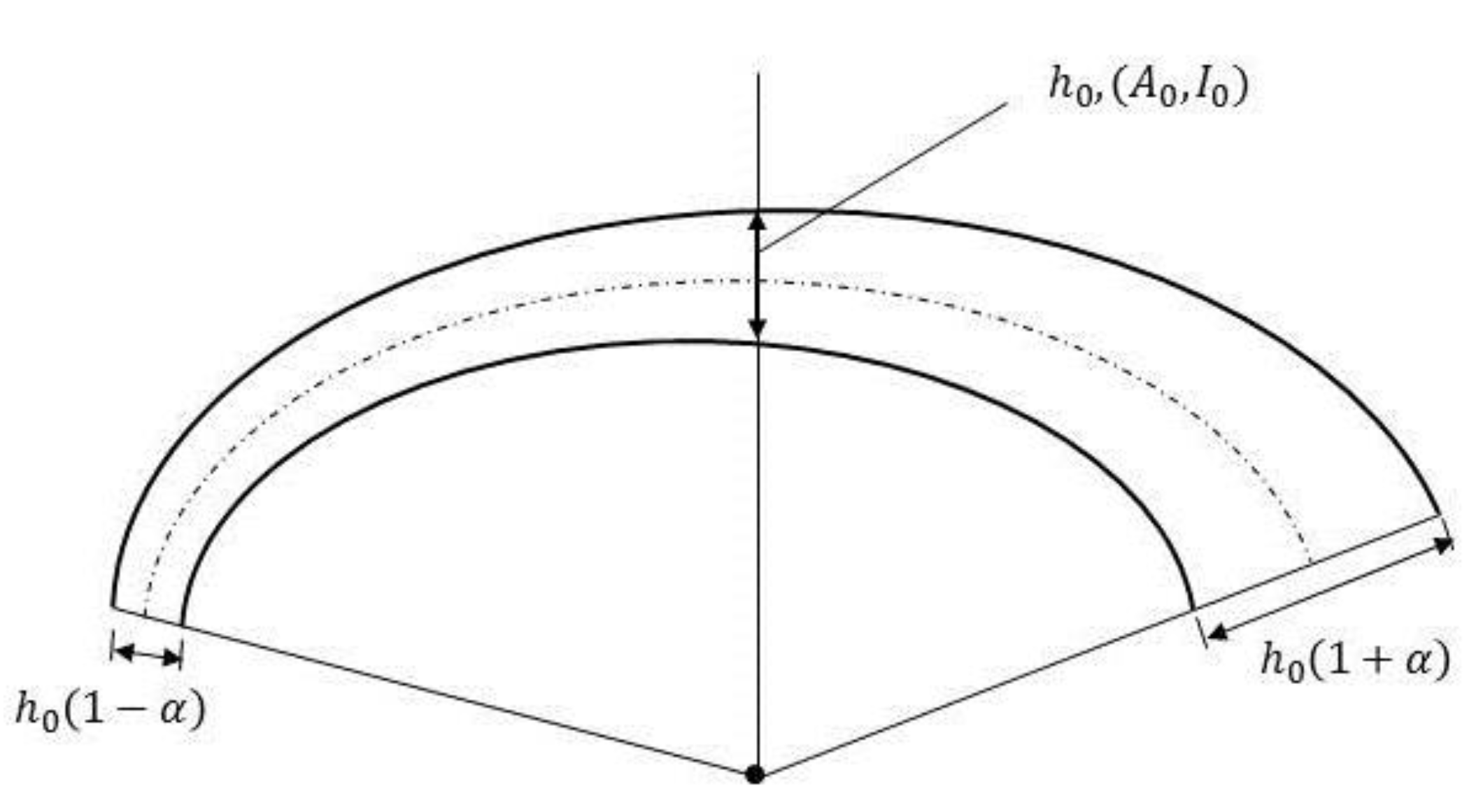
2. Governing Equations
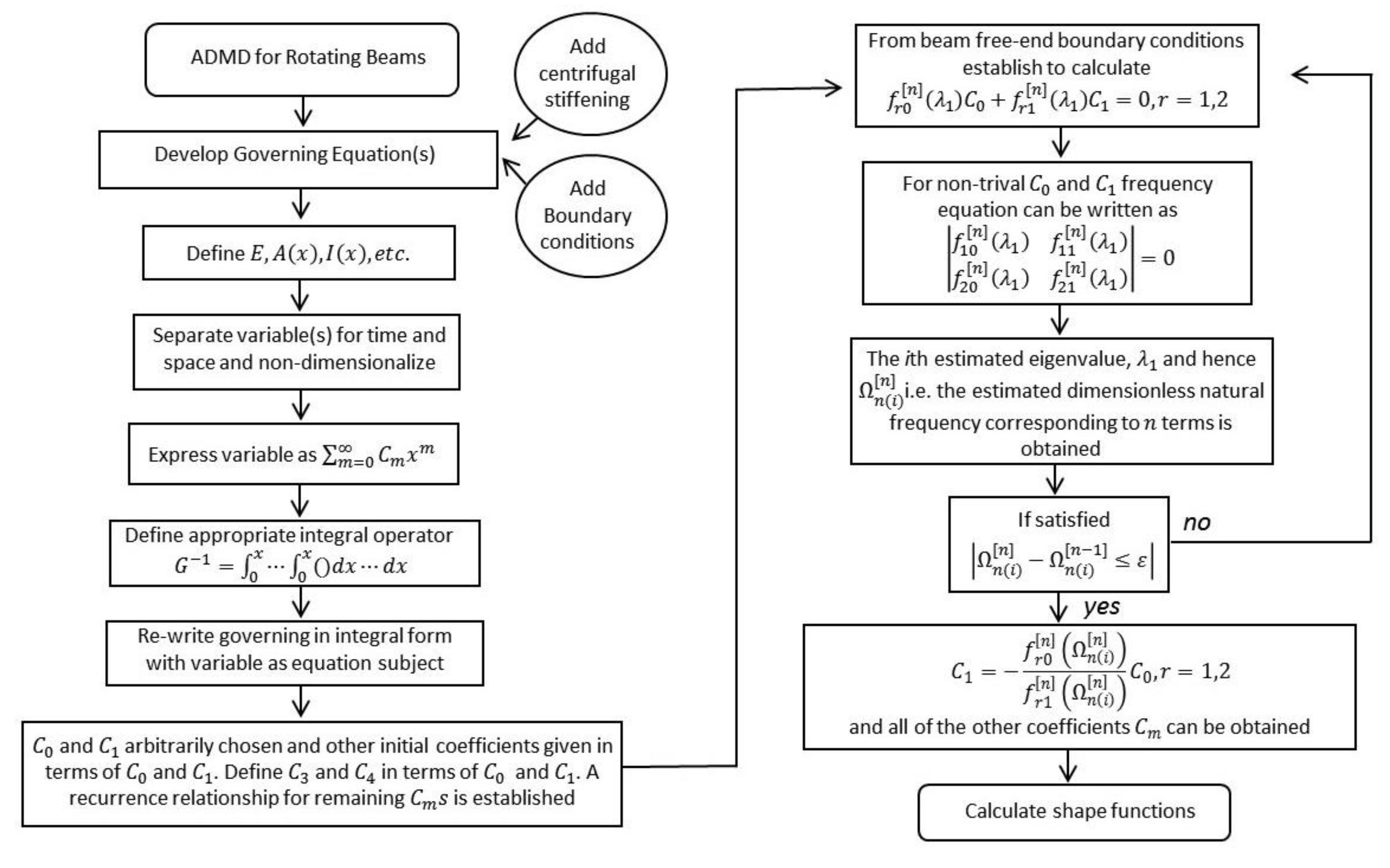
3. Application of the AMDM
4. Numerical Results
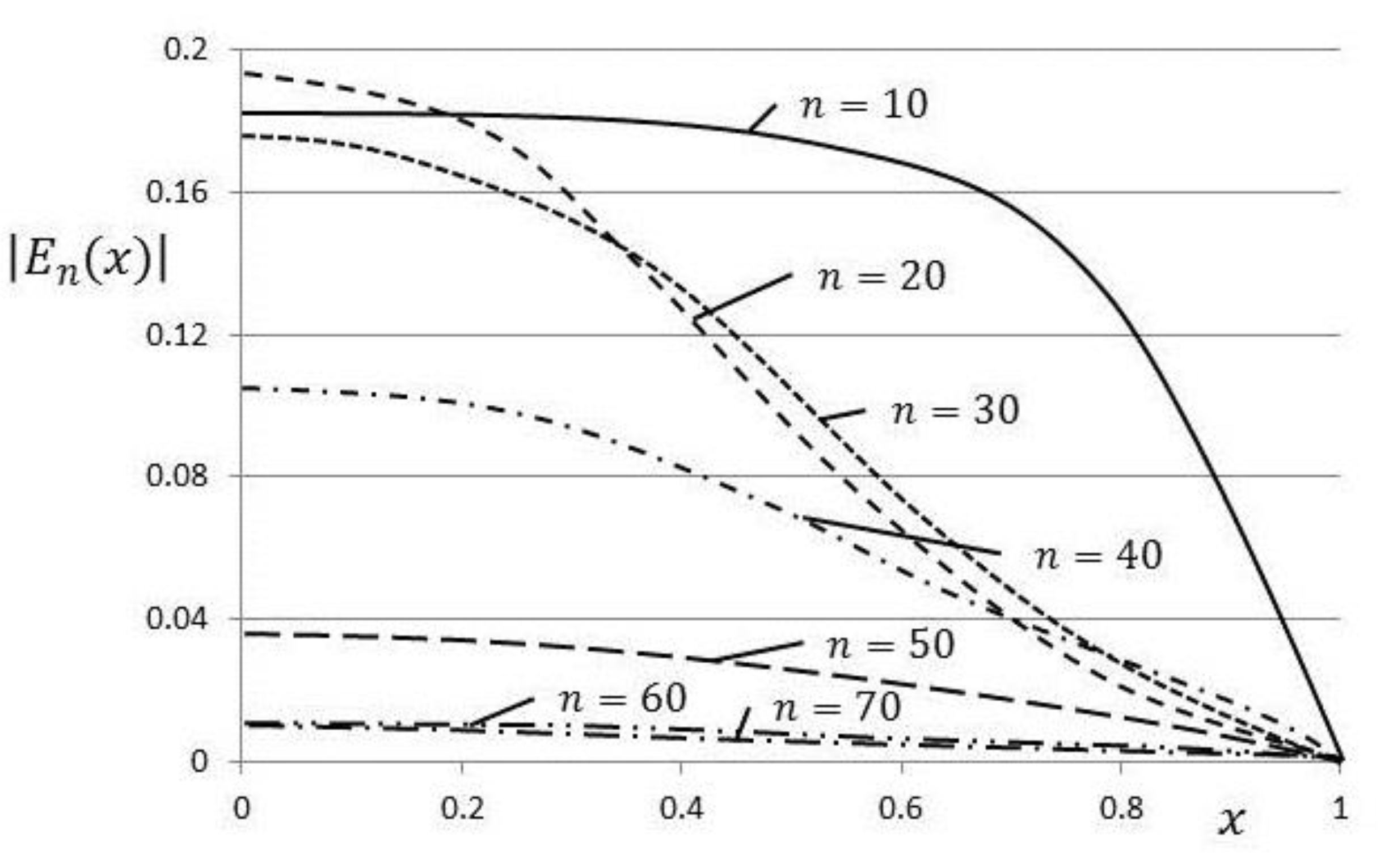
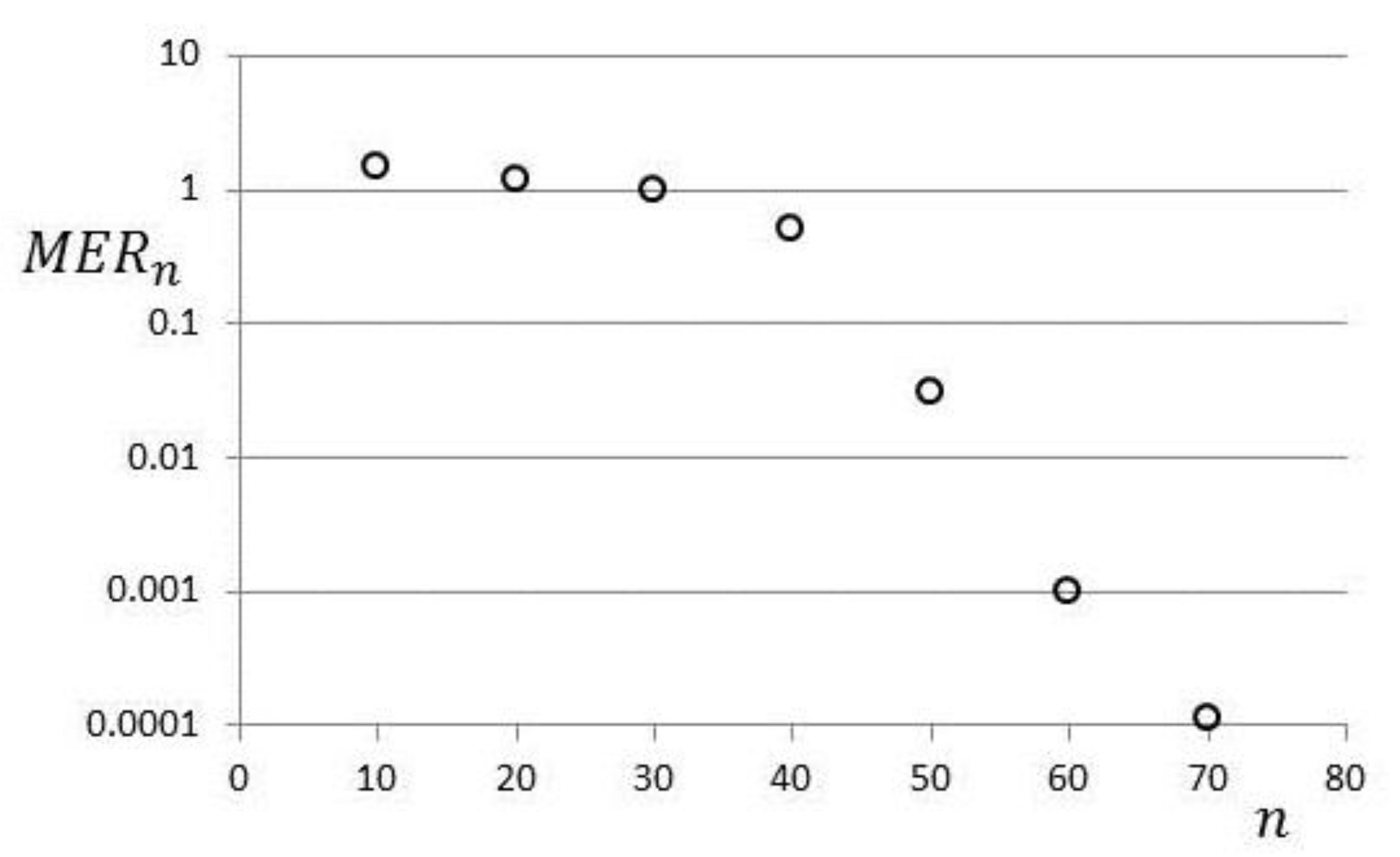
4.1. Unsymmetrical Nonrotating Arch
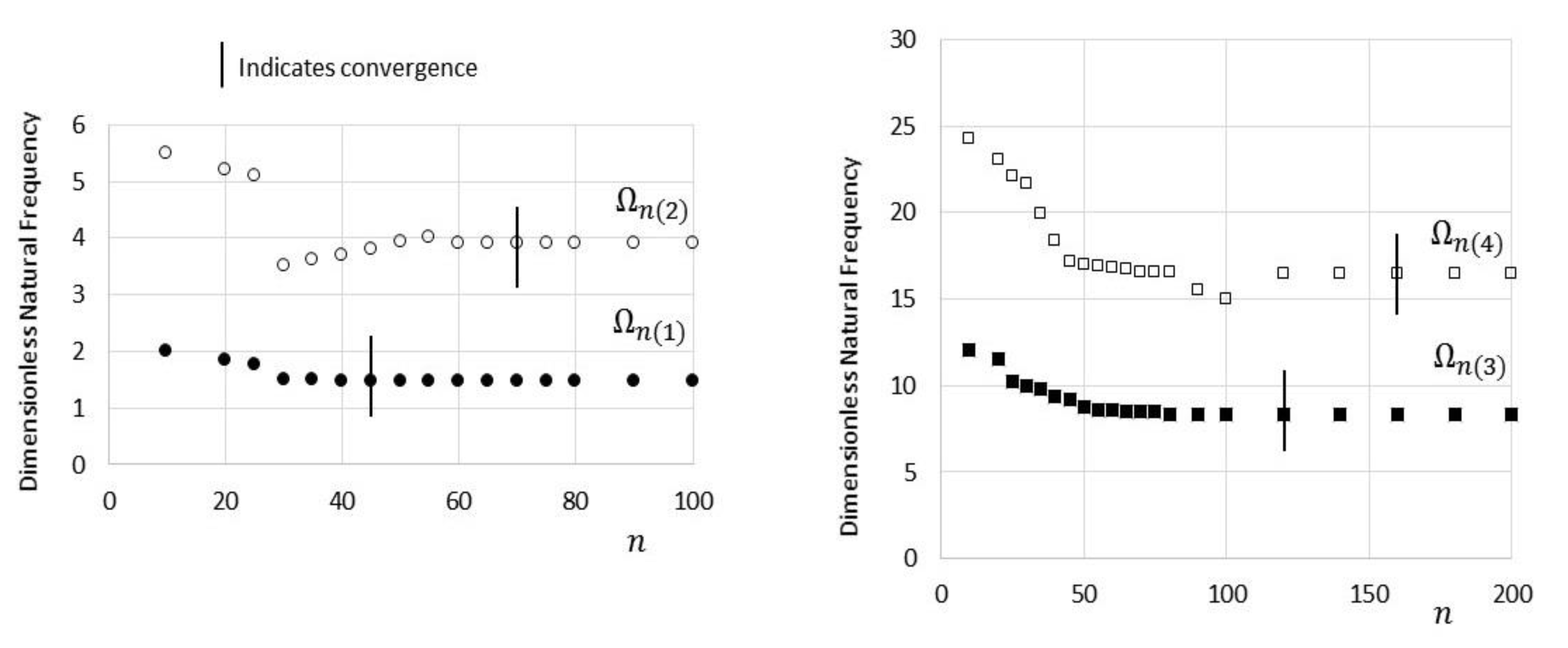
4.2. Unsymmetrical Rotating Scimitar-Type Curved Beam
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
References
- Auciello, N.M.; De Rosa, M.A. Free vibration of circular arches: A review. J. Sound Vib. 1994, 176, 433–458. [Google Scholar] [CrossRef]
- Tong, X.; Mrad, N.; Tabarrok, B. n-plane vibration of circular arches with variable cross-sections. J. Sound Vib. 1998, 212, 121–140. [Google Scholar] [CrossRef]
- Karami, G.; Malekzadeh, G.P. In-plane free vibration analysis of circular arches with varying cross-sections using differential quadrature method. J. Sound Vib. 2004, 274, 777–799. [Google Scholar] [CrossRef]
- Chen, C.N. DQEM analysis of in-plane vibration of curved beam structures. Adv. Eng. Softw. 2005, 36, 412–424. [Google Scholar] [CrossRef]
- Liu, G.R.; Wu, T.Y. In-plane vibration analyses of circular arches by the generalized differential quadrature rule. Int. J. Mech. Sci. 2001, 43, 2597–2611. [Google Scholar] [CrossRef]
- Gimena, F.N.; Gonzaga, P.; Gimena, P.L. 3D-curved beam element with varying cross-sectional area under generalized loads. Eng. Struct. 2008, 30, 404–411. [Google Scholar] [CrossRef]
- Molari, L.; Ubertini, F. A flexibility-based finite element for linear analysis of arbitrarily curved arches. Int. J. Numer. Methods Eng. 2006, 65, 1333–1353. [Google Scholar] [CrossRef]
- Hodges, D.J.; Rutkowski, M.J. Free vibration analysis of rotating beams by a variable order finite element method. AIAA J. 1981, 19, 1459–1466. [Google Scholar] [CrossRef]
- Wright, D.A.; Smith, C.E.; Thresher, R.W.; Wang, J.L.C. Vibration modes of centrifugally stiffened beams. J. Appl. Mech. 1982, 49, 197–201. [Google Scholar] [CrossRef]
- Naguleswaran, S. Lateral vibration of a centrifugally tensioned uniform Euler-Bernoulli beam. J. Sound Vib. 1994, 176, 613–624. [Google Scholar] [CrossRef]
- Yoo, H.H.; Shin, S.H. Vibration analysis of cantilever beams. J. Sound Vib. 1998, 212, 807–828. [Google Scholar] [CrossRef]
- Mao, Q. Application of Adomain modified decomposition method to free vibration analysis of rotating beams. Math. Probl. Eng. 2013, 284720. [Google Scholar]
- Hsu, J.-C.; Lai, H.-Y.; Chen, C.K. An innovative eigenvalue problem solver for free vibration of uniform Timoshenko beams using the Adomian modified decomposition method. J. Sound Vib. 2009, 325, 451–470. [Google Scholar] [CrossRef]
- Adair, D.; Jaeger, M. Simulation of tapered rotating beams with centrifugal stiffening using the Adomian decomposition method. Appl. Math. Model. 2016, 40, 3230–3241. [Google Scholar] [CrossRef]
- Adair, D.; Jaeger, M. Vibration analysis of a uniform pre-twisted rotating Euler-Bernoulli beam using the modified Adomian decomposition method. Math. Mech. Solids 2018, 23, 1345–1363. [Google Scholar] [CrossRef]
- Wang, G.; Wereley, N.M. Free vibration analysis of rotating blades with uniform tapers. AIAA J. 2004, 42, 2429–2437. [Google Scholar] [CrossRef]
- Adair, D.; Jaeger, M. A power series solution for rotating non-uniform Euler-Bernoulli cantilever beams. J. Vib. Control 2018, 24, 3855–3864. [Google Scholar] [CrossRef]
- Banerjee, J.R.; Su, H.; Jackson, D.R. Free vibration of rotating tapered beams using the dynamic stiffness method. J. Sound Vib. 2006, 298, 1034–1054. [Google Scholar] [CrossRef]
- Özdemir, Ö.O.; Kaya, M.O. Flapwise bending vibration analysis of a rotating tapered cantilever Bernoulli-Euler beam by differential transform method. J. Sound Vib. 2006, 289, 413–420. [Google Scholar] [CrossRef]
- Gunda, J.B.; Ganguli, R. Stiff-string basis function for vibration analysis of high speed rotating beams. J. Appl. Mech. 2008, 75, 245021–245025. [Google Scholar] [CrossRef]
- Yang, X.D.; Li, Z.; Zhang, W.; Yang, T.Z.; Lin, C.W. On the gyroscopic and centrifugal effects in the free vibration of rotating beams. J. Vib. Control 2019, 25, 219–227. [Google Scholar] [CrossRef]
- Chen, Q.; Du, J. A Fourier series solution for the transverse vibration of rotating beams with elastic boundary supports. Appl. Acoustics 2019, 155, 1–15. [Google Scholar] [CrossRef]
- Qin, Y.; Li, X.; Yang, E.C.; Li, Y.H. Flapwise free vibration characteristics of a rotating composite thin-walled beam under aerodynamic force and hygrothermal environment. Comp. Struct. 2016, 153, 490–503. [Google Scholar] [CrossRef]
- Jokar, H.; Mahzoon, M.; Vatankhah, R. Dynamic modeling and free vibration analysis of horizontal axis wind turbine blades in the flap-wise direction. Ren. Ener. 2020, 146, 1818–1832. [Google Scholar] [CrossRef]
- Adomian, G. Solving Frontier Problems of Physics: The Decomposition Method; Kluwer Academic Publishers: Boston, MA, USA, 1994. [Google Scholar]
- Das, S. A numerical solution of the vibration equation using modified decomposition method. J. Sound Vib. 2009, 320, 576–583. [Google Scholar] [CrossRef]
- Rach, R.; Duan, J.S.; Wazwaz, A.M. On the solution of non-isothermal reaction-diffusion model equations in a spherical catalyst by the modified Adomian method. Chem. Eng. Commun. 2015, 202, 1081–1088. [Google Scholar] [CrossRef]
- Rach, R.; Duan, J.S.; Wazwaz, A.M. Simulation of large deflections of a flexible cantilever beam fabricated from functionally graded materials by the Adomian decomposition method. Int. J. Dyn. Syst. Differ. Equ. 2019. [Google Scholar] [CrossRef]
- Henrych, J. Dynamics of Arches and Frames; Elsevier: Amsterdam, The Netherlands, 1981. [Google Scholar]
- Wolfram Research Inc. Mathematica, Version 12.0; Wolfram Research Inc.: Champaign, IL, USA, 2019. [Google Scholar]

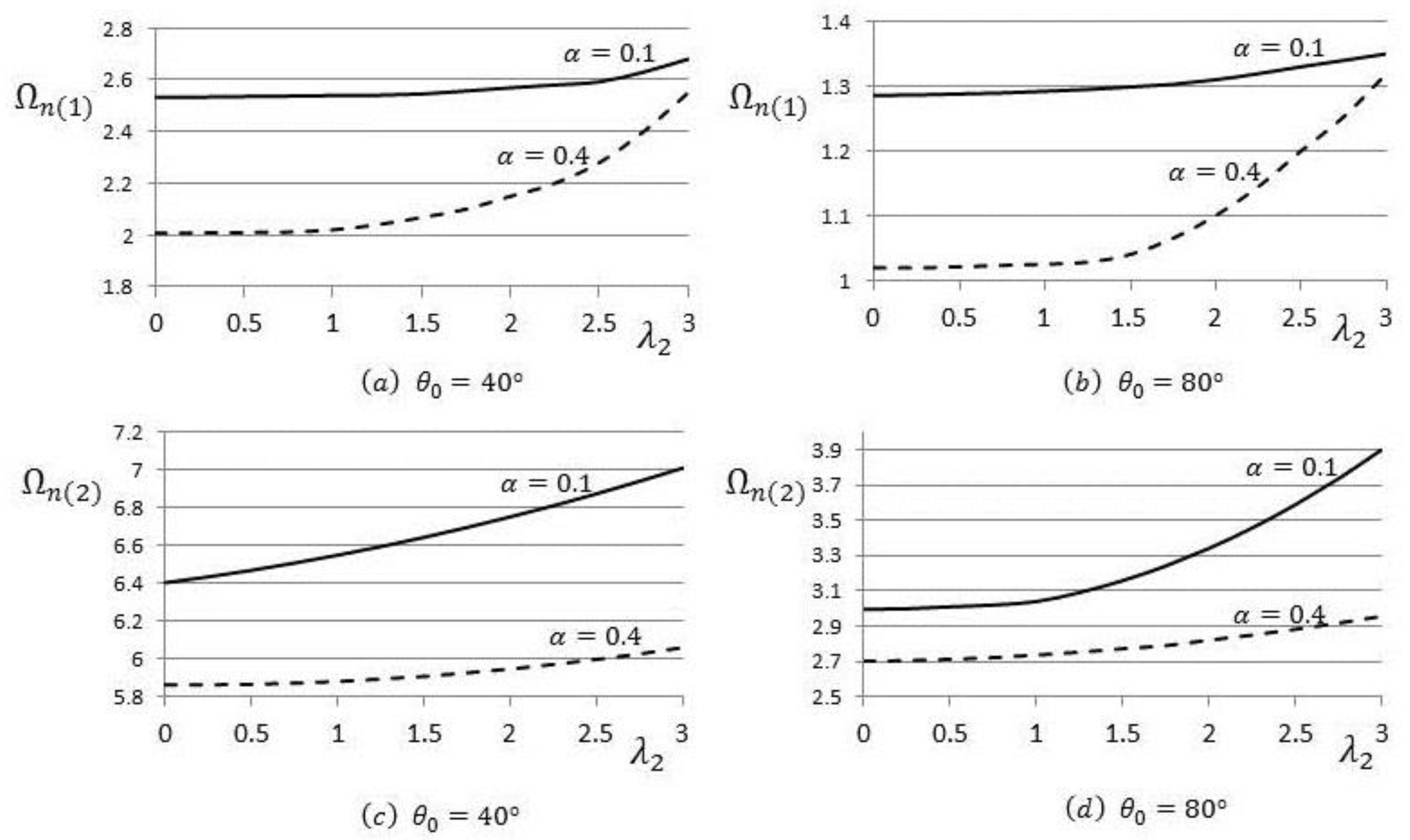
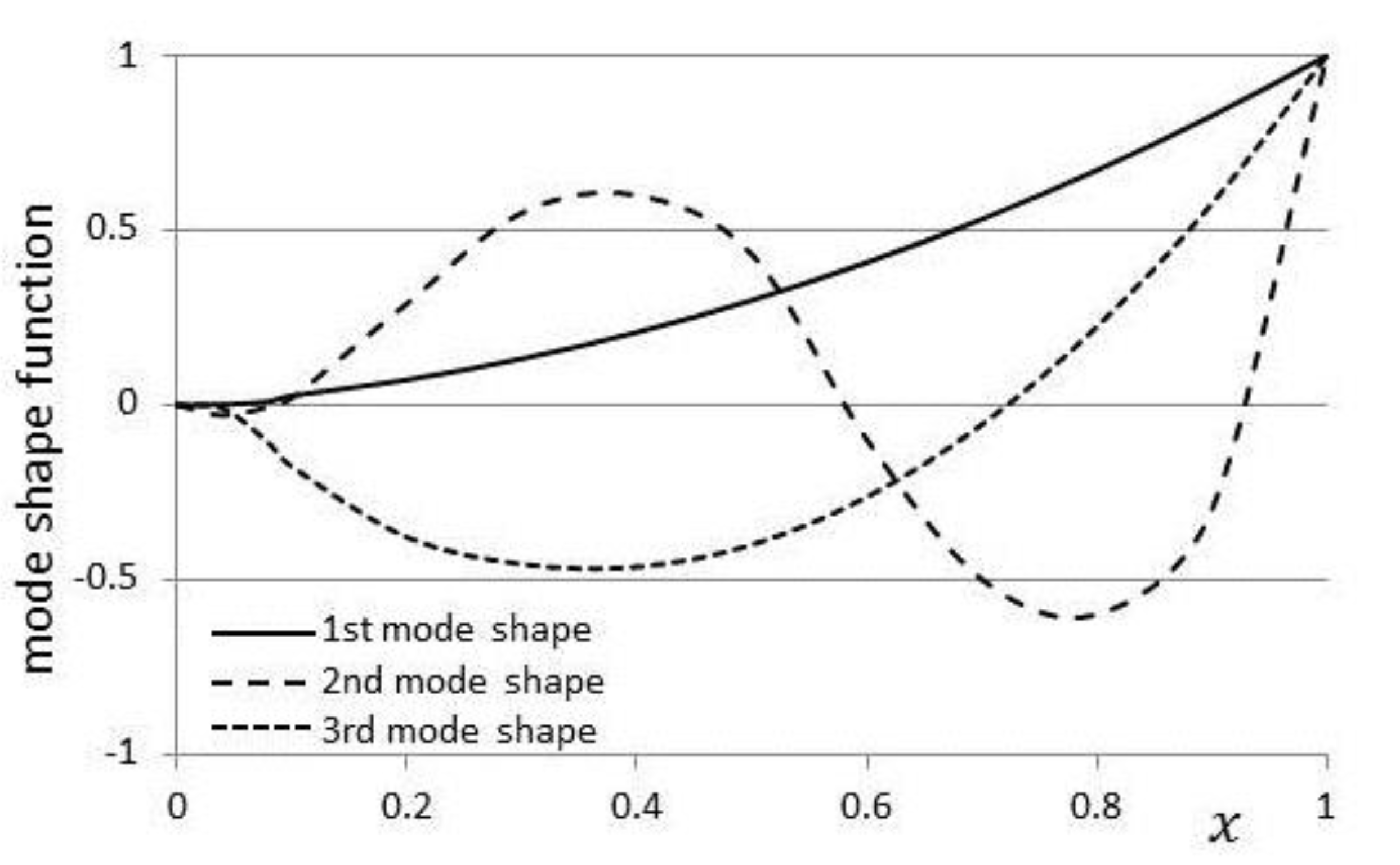
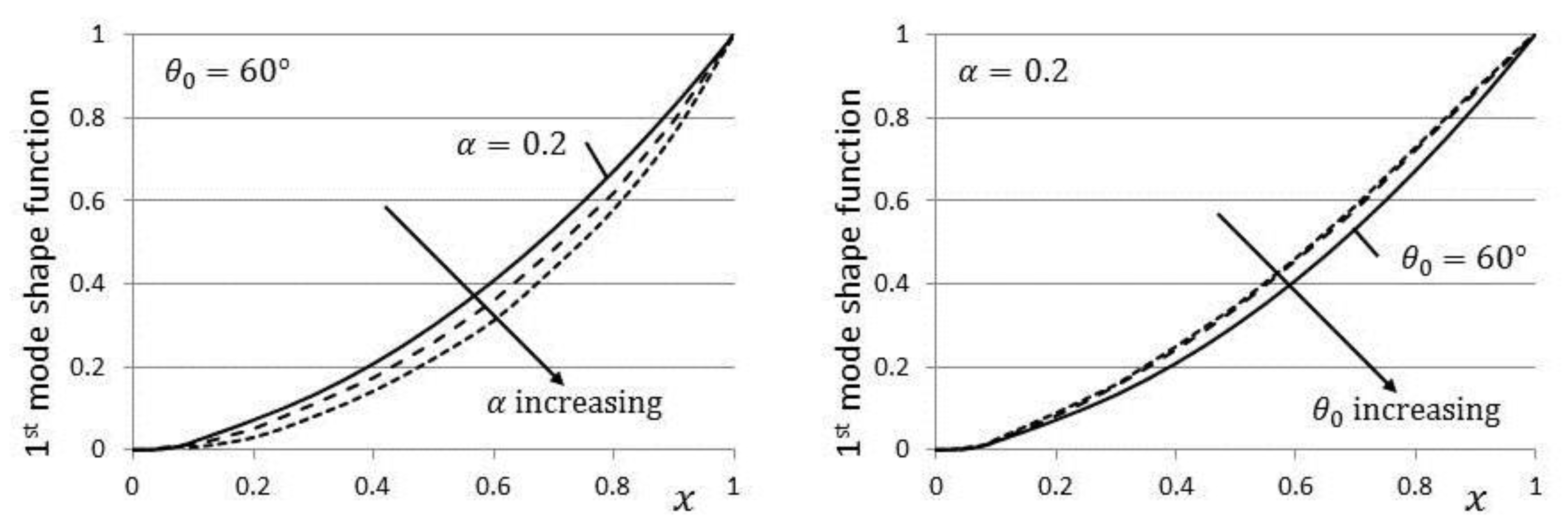
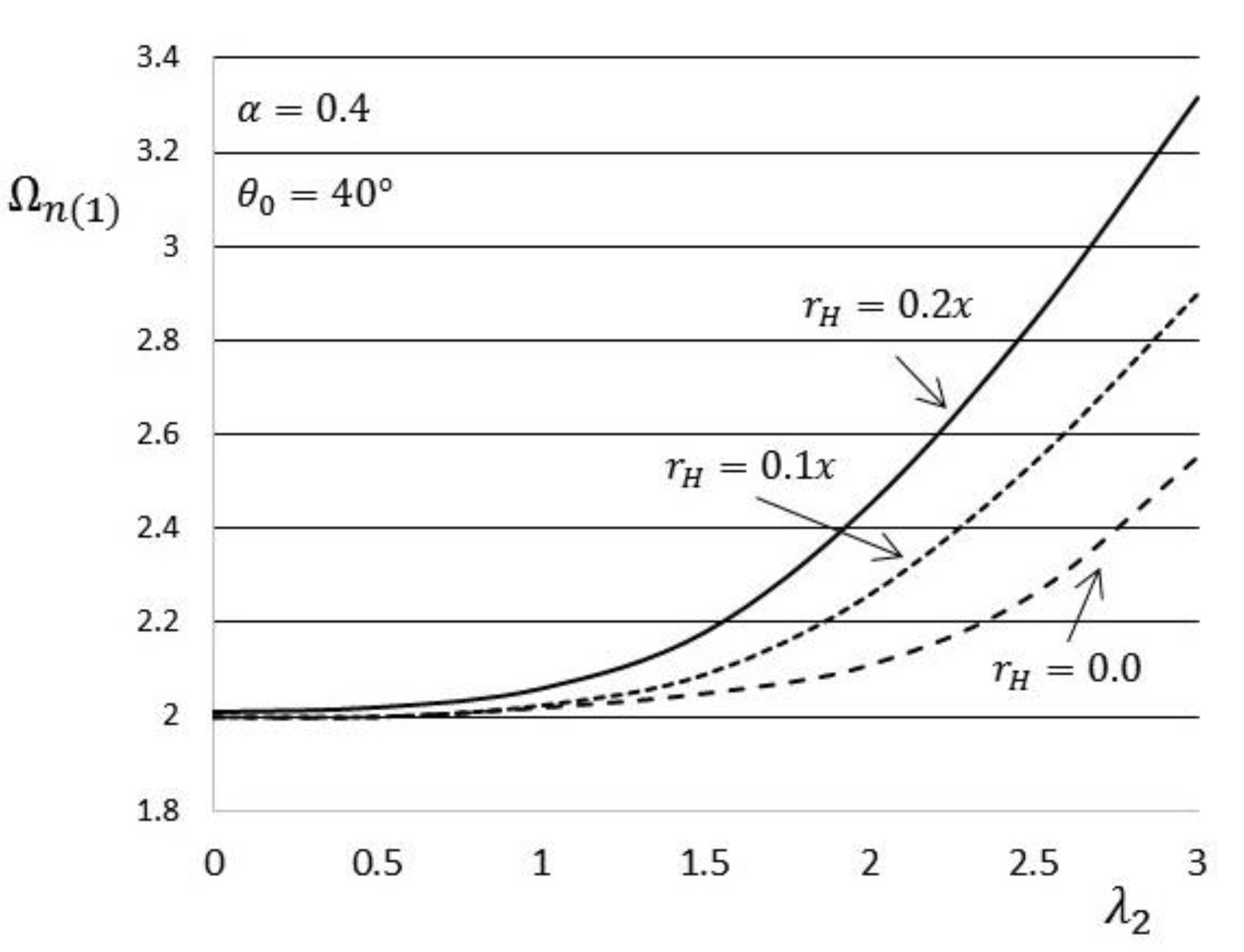
| AMDM | DQ [5] | Dev (%) | AMDM | DQ [5] | Dev (%) | |
|---|---|---|---|---|---|---|
| 10 | 10.0834 | 10.0834 | 0 | 9.4062 | 9.4064 | −0.002 |
| 20 | 5.0465 | 5.0465 | 0 | 4.7077 | 4.7078 | −0.002 |
| 40 | 2.5328 | 2.5328 | 0 | 2.3631 | 2.3630 | +0.004 |
| 60 | 1.6993 | 1.6993 | 0 | 1.5857 | 1.5856 | +0.006 |
| 80 | 1.2858 | 1.2858 | 0 | 1.2000 | 1.1999 | +0.008 |
| 10 | 8.7089 | 8.7087 | +0.002 | 7.9817 | 7.9805 | +0.015 |
| 20 | 4.3588 | 4.3586 | +0.005 | 3.9951 | 3.9943 | +0.020 |
| 40 | 2.1582 | 2.1580 | +0.009 | 2.0059 | 2.0053 | +0.030 |
| 60 | 1.4685 | 1.4683 | +0.014 | 1.3466 | 1.3460 | +0.045 |
| 80 | 1.1117 | 1.1115 | +0.018 | 1.0194 | 1.0191 | +0.030 |
| 10 | 26.3098 | 26.3097 | +0.0004 | 25.7092 | 25.7091 | +0.0003 |
| 20 | 13.0809 | 13.0808 | +0.0008 | 12.7757 | 12.7756 | +0.0008 |
| 40 | 6.4038 | 6.4038 | 0 | 6.2430 | 6.2429 | +0.0016 |
| 60 | 4.1398 | 4.1399 | −0.002 | 4.0264 | 4.0263 | +0.0025 |
| 80 | 2.9956 | 2.9955 | +0.003 | 2.9064 | 2.9062 | +0.0067 |
| 10 | 25.0359 | 25.0358 | +0.0004 | 24.2758 | 24.2742 | +0.0066 |
| 20 | 12.4340 | 12.4338 | +0.0016 | 12.0487 | 12.0479 | +0.0066 |
| 40 | 6.0640 | 6.0638 | +0.0033 | 5.8629 | 5.8625 | +0.0068 |
| 60 | 3.9009 | 3.9007 | +0.0051 | 3.7610 | 3.7608 | +0.0053 |
| 80 | 2.8089 | 2.8086 | +0.0106 | 2.7010 | 2.7005 | +0.0185 |
| S | ||||||
| AMDM | DQ [3] | Dev (%) | AMDM | DQ [3] | Dev (%) | |
| 10 | 1.0704 | 1.0705 | −0.0093 | 2.2911 | 2.2905 | 0.0262 |
| 20 | 1.0718 | 1.0720 | −0.0186 | 2.4867 | 2.4854 | 0.0523 |
| 40 | 1.0722 | 1.0724 | −0.0186 | 2.5228 | 2.5220 | 0.0317 |
| 100 | 1.0723 | 1.0725 | −0.0186 | 2.5321 | 2.5311 | 0.0395 |
| 200 | 1.0724 | 1.0725 | −0.0093 | 2.5334 | 2.5323 | 0.0434 |
| 400 | 1.0724 | 1.0725 | −0.0093 | 2.5340 | 2.5327 | 0.0513 |
| 1000 | 1.0724 | 1.0725 | −0.0093 | 2.5341 | 2.5328 | 0.0513 |
| 4000 | 1.0724 | 1.0725 | −0.0093 | 2.5341 | 2.5328 | 0.0513 |
| 10 | 3.0401 | 3.0399 | 0.0066 | 4.3598 | 4.3602 | −0.0092 |
| 20 | 3.8912 | 3.8907 | 0.0129 | 4.8401 | 4.8671 | −0.5547 |
| 40 | 4.5823 | 4.5818 | 0.0109 | 5.7111 | 5.7200 | −0.1556 |
| 100 | 4.6669 | 4.6660 | 0.0193 | 6.7050 | 6.7160 | −0.1638 |
| 200 | 4.6739 | 4.6736 | 0.0064 | 6.7398 | 6.7427 | −0.0430 |
| 400 | 4.6757 | 4.6754 | 0.0064 | 6.7401 | 6.7454 | −0.0786 |
| 1000 | 4.6758 | 4.6756 | 0.0043 | 6.7456 | 6.7489 | −0.0488 |
| 4000 | 4.6758 | 4.6760 | −0.0043 | 6.7467 | 6.7491 | −0.0356 |
| 10 | 0.9102 | 0.9100 | 0.0220 | 2.1468 | 2.1464 | 0.0186 |
| 20 | 0.9111 | 0.9109 | 0.0219 | 2.3069 | 2.3065 | 0.0173 |
| 40 | 0.9113 | 0.9111 | 0.0219 | 2.3391 | 2.3385 | 0.0256 |
| 100 | 0.9114 | 0.9112 | 0.0219 | 2.3476 | 2.3467 | 0.0384 |
| 200 | 0.9114 | 0.9112 | 0.0219 | 2.3483 | 2.3478 | 0.0213 |
| 400 | 0.9114 | 0.9112 | 0.0219 | 2.3483 | 2.3481 | 0.0085 |
| 1000 | 0.9114 | 0.9112 | 0.0219 | 2.3483 | 2.3481 | 0.0085 |
| 4000 | 0.9114 | 0.9112 | 0.0219 | 2.3483 | 2.3481 | 0.0085 |
| 10 | 2.9215 | 2.9211 | 0.0137 | 4.3236 | 4.3228 | 0.0185 |
| 20 | 3.7675 | 3.7669 | 0.0159 | 4.7479 | 4.7467 | 0.0253 |
| 40 | 4.4269 | 4.4262 | 0.0158 | 5.5915 | 5.5908 | 0.0125 |
| 100 | 4.5160 | 4.5153 | 0.0155 | 6.5627 | 6.5618 | 0.0137 |
| 200 | 4.5243 | 4.5237 | 0.0133 | 6.5904 | 6.5899 | 0.0076 |
| 400 | 4.5269 | 4.5256 | 0.0287 | 6.5981 | 6.5951 | 0.0455 |
| 1000 | 4.5278 | 4.5261 | 0.0376 | 6.5989 | 6.5964 | 0.0380 |
| 4000 | 4.5283 | 4.5263 | 0.0442 | 6.5992 | 6.5967 | 0.0379 |
| S | ||||||
| AMDM | DQ [3] | Dev (%) | AMDM | DQ [3] | Dev (%) | |
| 10 | 2.0366 | 2.0368 | −0.0098 | 2.9081 | 2.9076 | 0.0172 |
| 20 | 2.0905 | 2.0907 | −0.0096 | 3.8750 | 3.8743 | 0.0180 |
| 40 | 2.1014 | 2.1016 | −0.0095 | 4.1963 | 4.1956 | 0.0167 |
| 100 | 2.1045 | 2.1045 | 0 | 4.2241 | 4.2236 | 0.0118 |
| 200 | 2.1047 | 2.1049 | −0.0095 | 4.2272 | 4.2266 | 0.0142 |
| 400 | 2.1049 | 2.1050 | −0.0048 | 4.2278 | 4.2273 | 0.0118 |
| 1000 | 2.1049 | 2.1050 | −0.0048 | 4.2279 | 4.2275 | 0.0095 |
| 4000 | 2.1049 | 2.1050 | −0.0048 | 4.2279 | 4.2276 | 0.0071 |
| 10 | 4.1621 | 4.1619 | 0.0048 | 4.6155 | 4.6160 | −0.0108 |
| 20 | 4.4472 | 4.4466 | 0.0135 | 6.2216 | 6.2220 | −0.0064 |
| 40 | 5.7189 | 5.7184 | 0.0087 | 6.4123 | 6.4127 | −0.0062 |
| 100 | 6.2838 | 6.2832 | 0.0095 | 8.2643 | 8.2648 | −0.0060 |
| 200 | 6.2935 | 6.2930 | 0.0079 | 8.3163 | 8.3169 | −0.0072 |
| 400 | 6.2954 | 6.2950 | 0.0064 | 8.3218 | 8.3220 | −0.0024 |
| 1000 | 6.2958 | 6.2955 | 0.0048 | 8.3230 | 8.3232 | −0.0024 |
| 4000 | 6.2958 | 6.2956 | 0.0032 | 8.3232 | 8.3234 | −00024 |
| 10 | 1.9122 | 1.9121 | 0.0052 | 2.8262 | 2.8258 | 0.0141 |
| 20 | 1.9645 | 1.9645 | 0 | 3.7478 | 3.7475 | 0.0080 |
| 40 | 1.9758 | 1.9757 | 0.0051 | 4.0866 | 4.0861 | 0.0122 |
| 100 | 1.9788 | 1.9786 | 0.0100 | 4.1211 | 4.1208 | 0.0073 |
| 200 | 1.9792 | 1.9791 | 0.0050 | 4.1251 | 4.1246 | 0.0121 |
| 400 | 1.9793 | 1.9792 | 0.0051 | 4.1257 | 4.1255 | 0.0048 |
| 1000 | 1.9793 | 1.9792 | 0.0051 | 4.1259 | 4.1258 | 0.0024 |
| 4000 | 1.9793 | 1.9792 | 0.0051 | 4.1259 | 4.1258 | 0.0024 |
| 10 | 4.1111 | 4.1108 | 0.0073 | 4.5748 | 4.5746 | 0.0044 |
| 20 | 4.3849 | 4.3841 | 0.0182 | 6.1583 | 6.1585 | −0.0032 |
| 40 | 5.5899 | 5.5894 | 0.0089 | 6.3112 | 6.3118 | −0.0095 |
| 100 | 6.1674 | 6.1670 | 0.0065 | 8.1102 | 8.1100 | 0.0025 |
| 200 | 6.1791 | 6.1784 | 0.0113 | 8.1724 | 8.1728 | −0.0049 |
| 400 | 6.1813 | 6.1807 | 0.0097 | 8.1793 | 8.1788 | 0.0061 |
| 1000 | 6.1820 | 6.1814 | 0.0097 | 8.1810 | 8.1802 | 0.0098 |
| 4000 | 6.1823 | 6.1815 | 0.0129 | 8.1812 | 8.1805 | 0.0086 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Adair, D.; Jaeger, M. In-Plane Free Vibration Analysis of a Scimitar-Type Rotating Curved Beam Using the Adomian Modified Decomposition Method. Math. Comput. Appl. 2020, 25, 68. https://doi.org/10.3390/mca25040068
Adair D, Jaeger M. In-Plane Free Vibration Analysis of a Scimitar-Type Rotating Curved Beam Using the Adomian Modified Decomposition Method. Mathematical and Computational Applications. 2020; 25(4):68. https://doi.org/10.3390/mca25040068
Chicago/Turabian StyleAdair, Desmond, and Martin Jaeger. 2020. "In-Plane Free Vibration Analysis of a Scimitar-Type Rotating Curved Beam Using the Adomian Modified Decomposition Method" Mathematical and Computational Applications 25, no. 4: 68. https://doi.org/10.3390/mca25040068
APA StyleAdair, D., & Jaeger, M. (2020). In-Plane Free Vibration Analysis of a Scimitar-Type Rotating Curved Beam Using the Adomian Modified Decomposition Method. Mathematical and Computational Applications, 25(4), 68. https://doi.org/10.3390/mca25040068





