An Adaptive Discrete Integral Terminal Sliding Mode Control Method for a Two-Joint Manipulator
Abstract
1. Introduction
- (1)
- A novel ADITSMC algorithm is proposed, which uses a one-step delay estimation method to compensate for disturbances in the controlled object and adopts the idea of adaptive control to improve the trajectory tracking precision of robotic arm systems and suppress system chattering.
- (2)
- Compared to previous approaches utilizing robust control, neural networks, fuzzy control, etc. [5,6,7,8,9], the proposed scheme features a simpler controller design and higher tracking precision. In comparison to reference [11], the proposed scheme is applicable to multi-input and multi-output systems, thus offering higher system accuracy. In contrast to reference [12], the proposed scheme does not require a state observer, and the data-driven control algorithm is less sensitive to changes in system parameters, maintaining high tracking precision. When compared to references [18,19], the proposed scheme exhibits smaller chattering in the presence of high disturbances. In comparison to references [20,21], the proposed scheme requires fewer parameter adjustments and achieves slightly higher tracking precision.
- (3)
- The primary advantage of the proposed ADITSMC scheme is to provide an easily implemented control method for multi-input and multi-output systems, capable of handling uncertainties and disturbances.
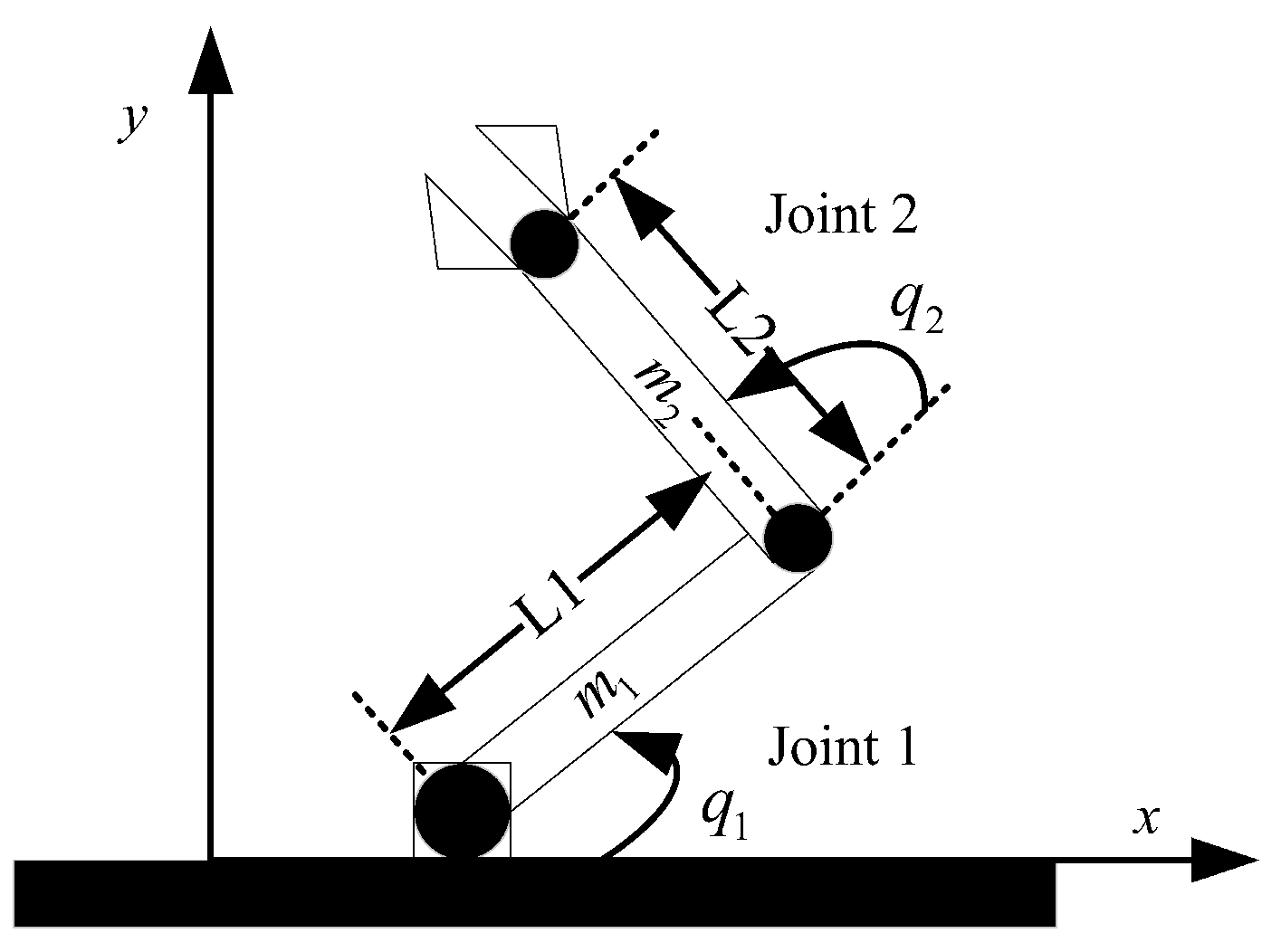
2. Two-Joint Manipulator Model and Its Discrete State Space Expression
2.1. Two-Joint Manipulator Model
2.2. Discrete State Space Expression of Two-Joint Manipulator
3. Design of Adaptive Discrete Integral Terminal Sliding Mode Controller for Two-Joint Manipulator
3.1. Trajectory Tracking Control Structure of Two-Joint Manipulator
3.2. Design of Adaptive Discrete Integral Terminal Sliding Mode Control Law
3.3. Proof of Convergence for the Robotic Arm Adaptive Discrete Integral Terminal Sliding Mode Controller
- (1)
- If
- (2)
- If
4. Simulation Experiment and Analysis
4.1. Related Introduction of Two-Joint Manipulator
4.2. Simulation Parameter Setting
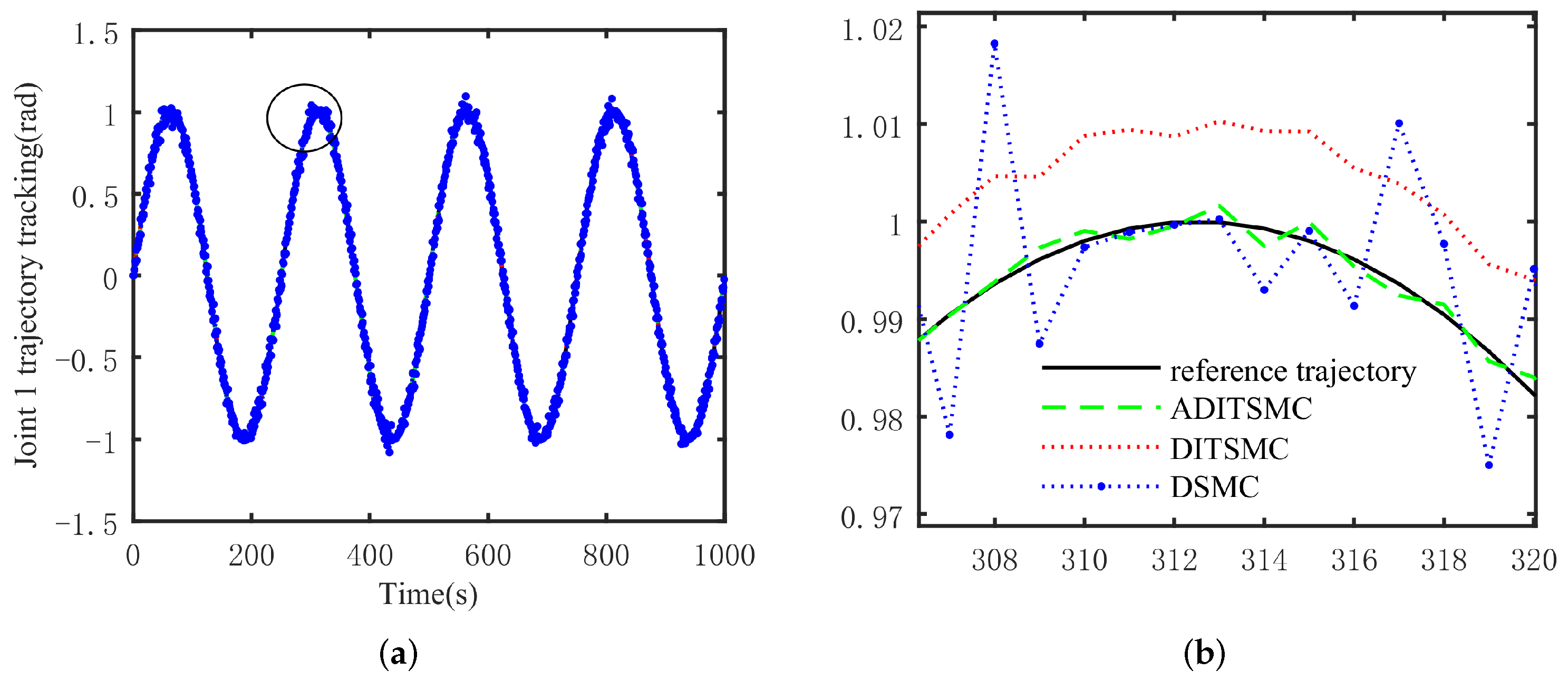
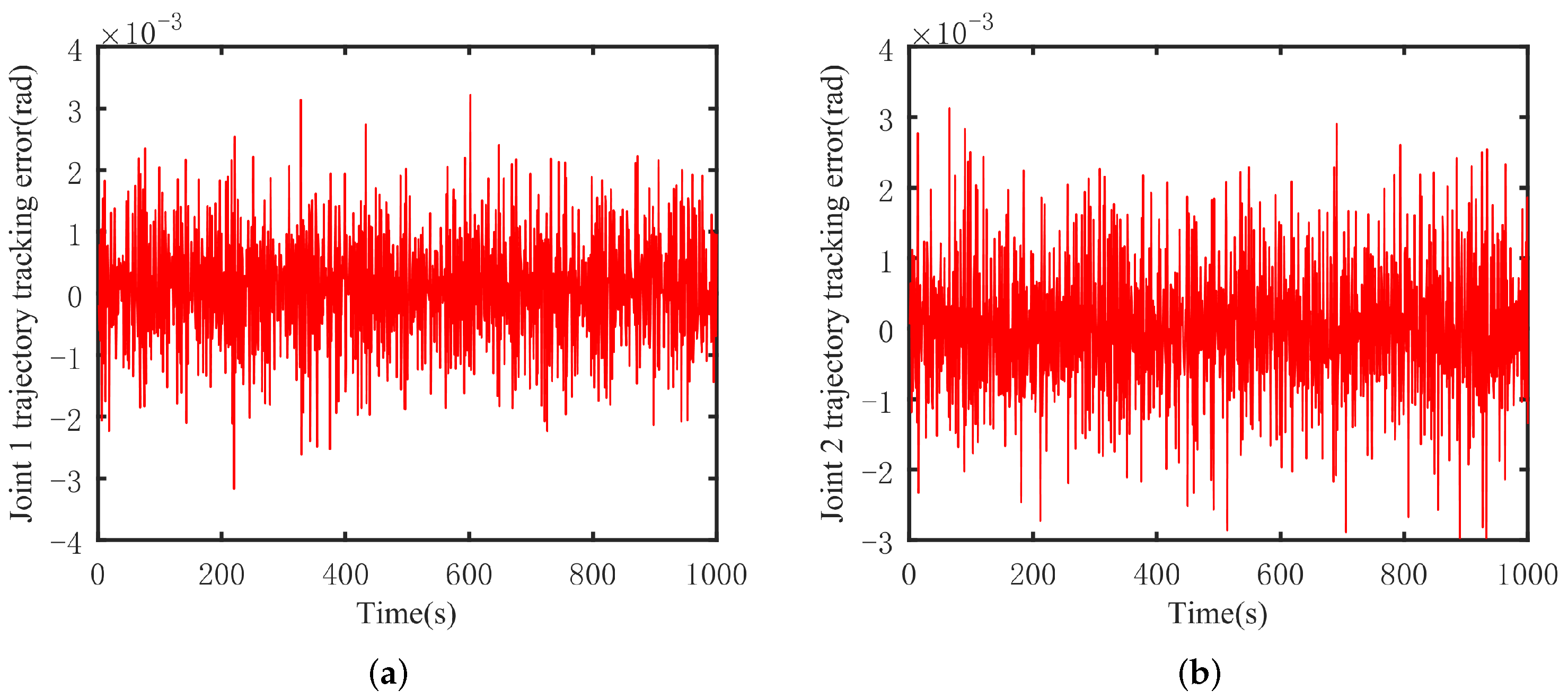
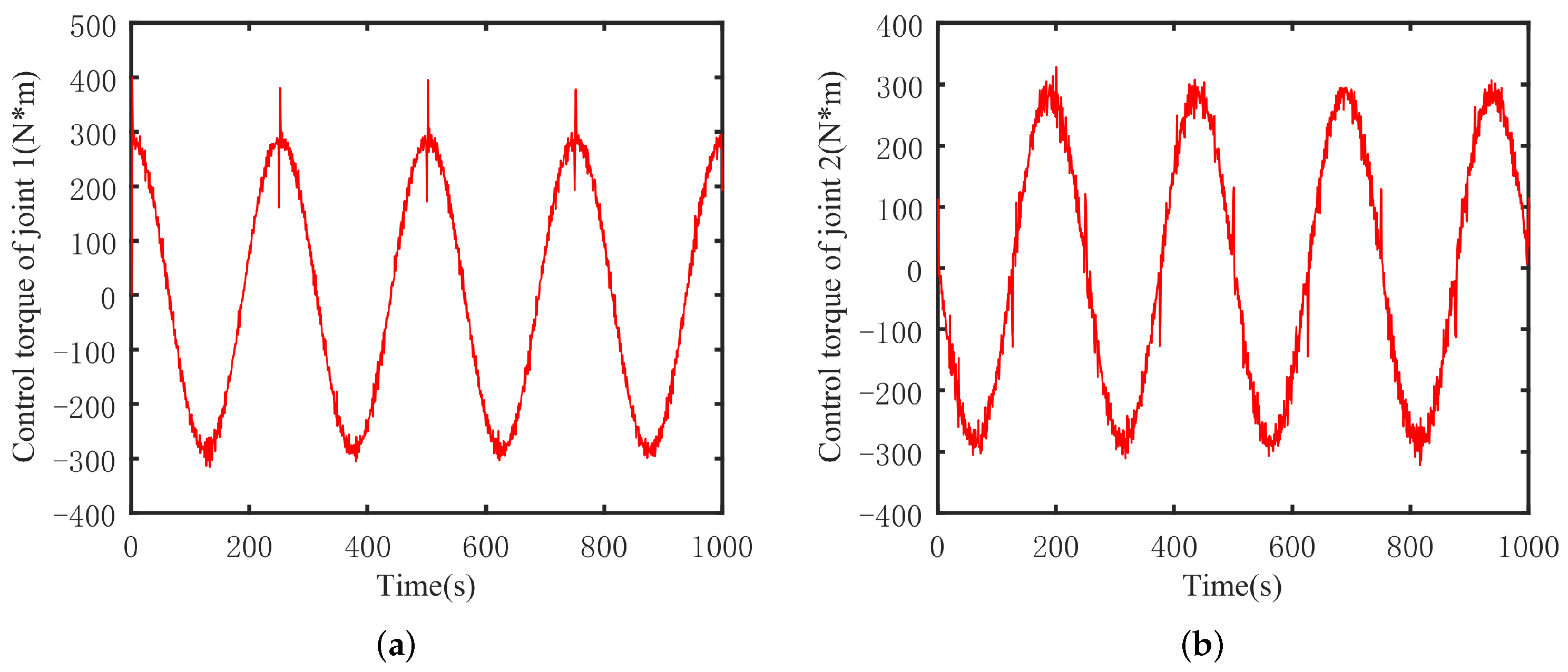
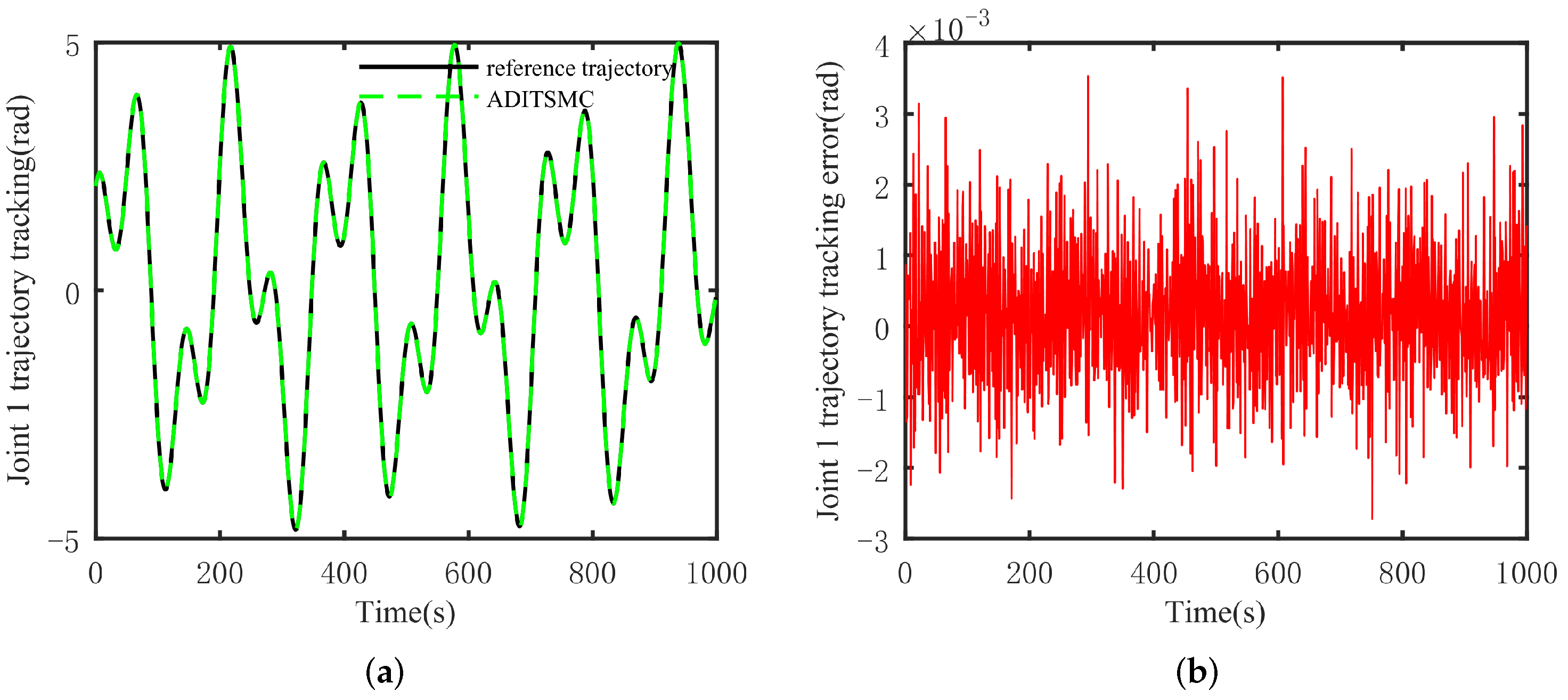
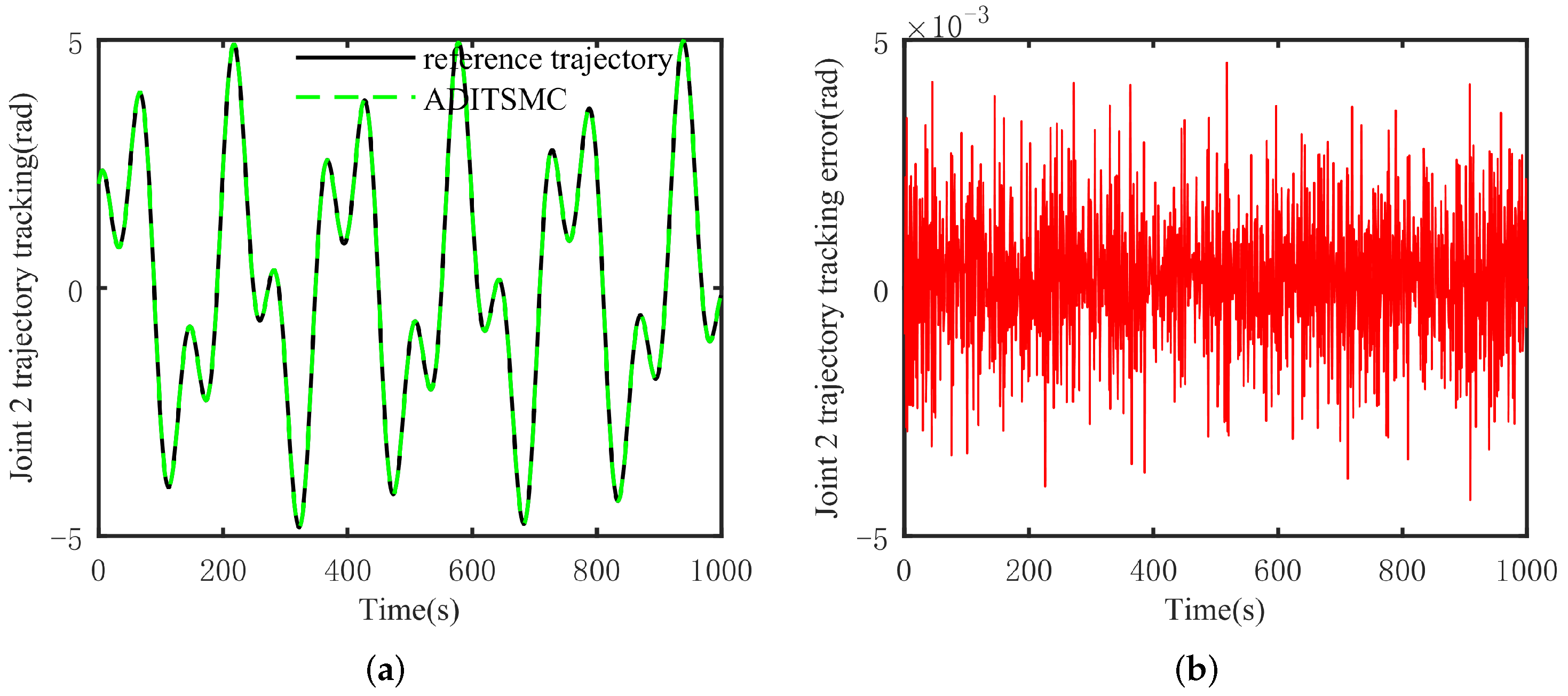
4.3. Simulation Results and Analysis
- (1)
- MSE
- (2)
- IAFV
5. Conclusions
- (1)
- Achieving more precise trajectory tracking control than DITSMC and DSMC, with an error range within [−0.004 rad, 0.004 rad], ensuring the stable operation of the robotic arm in practical engineering applications.
- (2)
- After replacing the disturbances that the robotic arm may encounter in its operational environment with white noise, the control force variation becomes smaller and smoother compared to DITSMC and DSMC.
- (1)
- Considering the multi-degree-of-freedom robotic arm model as the research object, the effectiveness of this control scheme in complex environments will be investigated.
- (2)
- Considering the incorporation of state observers for disturbance estimation, the current one-step delay estimation method in the algorithm may not fully estimate disturbances. Therefore, the use of state observers or disturbance observers for disturbance estimation will be explored to further improve the algorithm’s disturbance rejection capability.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Meng, G.; Han, L.L.; Zhang, C.F. Research progress and technical challenges of space robot. Acta Aeronaut. Astronaut. Sin. 2021, 42, 1–27. [Google Scholar]
- Nash, B.; Walker, A.; Chambers, T. A simulator based on virtual reality to dismantle a research reactor assembly using master-slave manipulators. Ann. Nucl. Energy 2018, 120, 1–7. [Google Scholar] [CrossRef]
- Zhang, X.; Xie, Y.; Jiang, L.; Li, G.; Meng, J.; Huang, Y. Fault-tolerant dynamic control of a four-wheel redundantly-actuated mobile robot. IEEE Access 2019, 7, 157909–157921. [Google Scholar] [CrossRef]
- Sacchi, N.; Incremona, G.P.; Ferrara, A. Sliding mode based fault diagnosis with deep reinforcement learning add-ons for intrinsically redundant manipulators. Int. J. Robust Nonlinear Control 2023, 33, 9109–9127. [Google Scholar] [CrossRef]
- Zhang, X.; Xu, W.; Lu, W. Fractional-order iterative sliding mode control based on the neural network for manipulator. Math. Probl. Eng. 2021, 2021, 1–12. [Google Scholar] [CrossRef]
- Xia, K.; Gao, H.; Ding, L.; Liu, G.; Deng, Z.; Liu, Z.; Ma, C. Trajectory tracking control of wheeled mobile manipulator based on fuzzy neural network and extended Kalman filtering. Neural Comput. Appl. 2018, 30, 447–462. [Google Scholar] [CrossRef]
- Souzanchi-K, M.; Arab, A.; Akbarzadeh-T, M.R.; Fateh, M.M. Robust impedance control of uncertain mobile manipulators using time-delay compensation. IEEE Trans. Control Syst. Technol. 2017, 26, 1942–1953. [Google Scholar] [CrossRef]
- Reboucas Filho, P.P.; da Silva, S.P.P.; Praxedes, V.N.; Hemanth, J.; de Albuquerque, V.H.C. Control of singularity trajectory tracking for robotic manipulator by genetic algorithms. J. Comput. Sci. 2019, 30, 55–64. [Google Scholar] [CrossRef]
- Young, K.D.; Utkin, V.I.; Ozguner, U. A control engineer’s guide to sliding mode control. IEEE Trans. Control Syst. Technol. 1999, 7, 328–342. [Google Scholar] [CrossRef]
- Hou, H.; Yu, X.; Xu, L.; Chuei, R.; Cao, Z. Discrete-time terminal sliding-mode tracking control with alleviated chattering. IEEE/ASME Trans. Mechatronics 2019, 24, 1808–1817. [Google Scholar] [CrossRef]
- Du, H.; Chen, X.; Wen, G.; Yu, X.; Lü, J. Discrete-Time Fast Terminal Sliding Mode Control for Permanent Magnet Linear Motor. IEEE Trans. Ind. Electron. 2018, 65, 9916–9927. [Google Scholar] [CrossRef]
- Huang, X.; Dong, Z.; Zhang, F.; Zhang, L. Discrete-time extended state observer-based model-free adaptive sliding mode control with prescribed performance. Int. J. Robust Nonlinear Control 2022, 32, 4816–4842. [Google Scholar] [CrossRef]
- Zhai, J.; Xu, G. A novel non-singular terminal sliding mode trajectory tracking control for robotic manipulators. IEEE Trans. Circuits Syst. II Express Briefs 2020, 68, 391–395. [Google Scholar] [CrossRef]
- Dachang, Z.; Baolin, D.; Puchen, Z.; Wu, W. Adaptive backstepping sliding mode control of trajectory tracking for robotic manipulators. Complexity 2020, 2020, 1–11. [Google Scholar] [CrossRef]
- He, J.; Yang, X.; Zhang, C.; Liu, J.; Zhang, Q.; Chen, X. Tracking control via sliding mode for heavy-haul trains with input saturation. Meas. Control 2020, 53, 1720–1729. [Google Scholar] [CrossRef]
- Dong, H.; Yang, X.; Gao, H.; Yu, X. Practical terminal sliding-mode control and its applications in servo systems. IEEE Trans. Ind. Electron. 2022, 70, 752–761. [Google Scholar] [CrossRef]
- Xu, D.; Shi, Y.; Ji, Z. Model-Free Adaptive Discrete-Time Integral Sliding-Mode-Constrained-Control for Autonomous 4WMV Parking Systems. IEEE Trans. Ind. Electron. 2017, 65, 834–843. [Google Scholar] [CrossRef]
- Yuan, L.; Li, J. Consensus of discrete-time nonlinear multiagent systems using sliding mode control based on optimal control. IEEE Access 2022, 10, 47275–47283. [Google Scholar] [CrossRef]
- Kang, S.; Wu, H.; Yang, X.; Li, Y.; Yao, J.; Chen, B.; Lu, H. Discrete-time predictive sliding mode control for a constrained parallel micropositioning piezostage. IEEE Trans. Syst. Man Cybern. Syst. 2021, 52, 3025–3036. [Google Scholar] [CrossRef]
- Yao, X.; Park, J.H.; Dong, H.; Guo, L.; Lin, X. Robust adaptive nonsingular terminal sliding mode control for automatic train operation. IEEE Trans. Syst. Man Cybern. Syst. 2018, 49, 2406–2415. [Google Scholar] [CrossRef]
- Xu, Z.; Yang, X.; Zhou, S.; Zhang, W.; Zhang, W.; Yang, S.; Liu, P.X. Extended state observer based adaptive backstepping nonsingular fast terminal sliding-mode control for robotic manipulators with uncertainties. Int. J. Control. Autom. Syst. 2022, 20, 2972–2982. [Google Scholar] [CrossRef]
- Bartoszewicz, A. Discrete-time quasi-sliding-mode control strategies. IEEE Trans. Ind. Electron. 1998, 45, 633–637. [Google Scholar] [CrossRef]
- Abidi, K.; Xu, J.X.; Xinghuo, Y. On the discrete-time integral sliding-mode control. IEEE Trans. Autom. Control 2007, 52, 709–715. [Google Scholar] [CrossRef]
- Xu, Q.S. Digital integral terminal sliding mode predictive control ofpiezoelectric-driven motion system. IEEE Trans. Ind. Electron. 2016, 63, 3976–3984. [Google Scholar] [CrossRef]
- Abidi, K.; Xu, J.X.; She, J.H. A discrete-time terminal sliding-mode control approach applied to a motion control problem. IEEE Trans. Ind. Electron. 2008, 56, 3619–3627. [Google Scholar] [CrossRef]






| Parameter | Parameter Value |
|---|---|
| 0.975 | |
| 1.135 | |
| 100 | |
| 0.05 | |
| 0.01 s |
| Control Method | MSE | IAFV |
|---|---|---|
| ADITSMC | ||
| DITSMC | ||
| DSMC |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, J.; Sui, Z.; Wang, W.; Xu, F. An Adaptive Discrete Integral Terminal Sliding Mode Control Method for a Two-Joint Manipulator. Processes 2024, 12, 1106. https://doi.org/10.3390/pr12061106
Xu J, Sui Z, Wang W, Xu F. An Adaptive Discrete Integral Terminal Sliding Mode Control Method for a Two-Joint Manipulator. Processes. 2024; 12(6):1106. https://doi.org/10.3390/pr12061106
Chicago/Turabian StyleXu, Jianliang, Zhen Sui, Wenduo Wang, and Feng Xu. 2024. "An Adaptive Discrete Integral Terminal Sliding Mode Control Method for a Two-Joint Manipulator" Processes 12, no. 6: 1106. https://doi.org/10.3390/pr12061106
APA StyleXu, J., Sui, Z., Wang, W., & Xu, F. (2024). An Adaptive Discrete Integral Terminal Sliding Mode Control Method for a Two-Joint Manipulator. Processes, 12(6), 1106. https://doi.org/10.3390/pr12061106





