Unified Approach for Prediction of the Volumetric Mass Transfer Coefficients in a Homogeneous and Heterogeneous Bubble Column Based on the Non-Corrected Penetration Theory: Case Studies
Abstract
:1. Introduction
1.1. Correction Factors of the Penetration Theory
1.2. Empirical Correlations for kLa Prediction
2. Experimental Setup and Measurement Technique
3. Results and Discussion
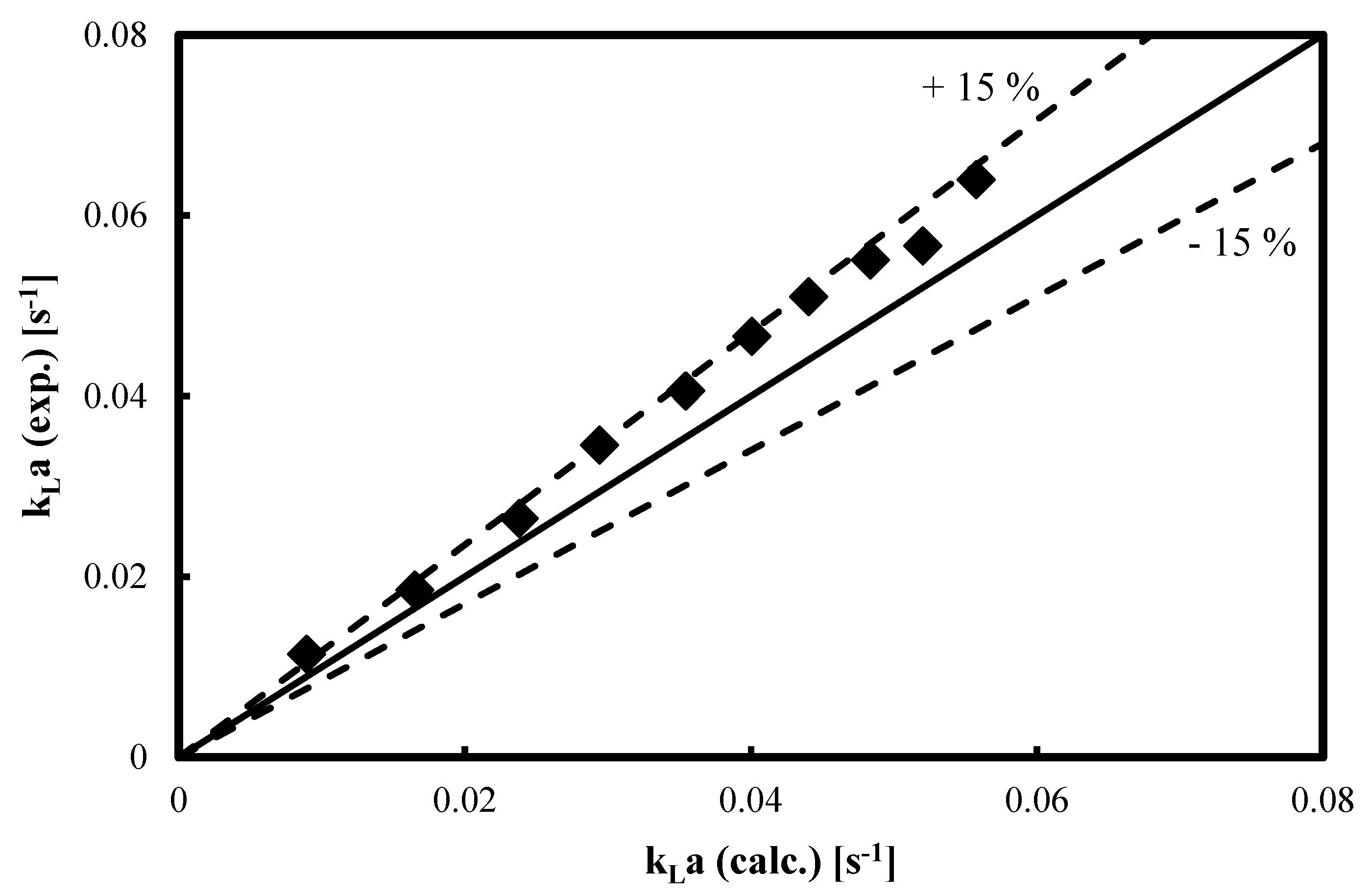
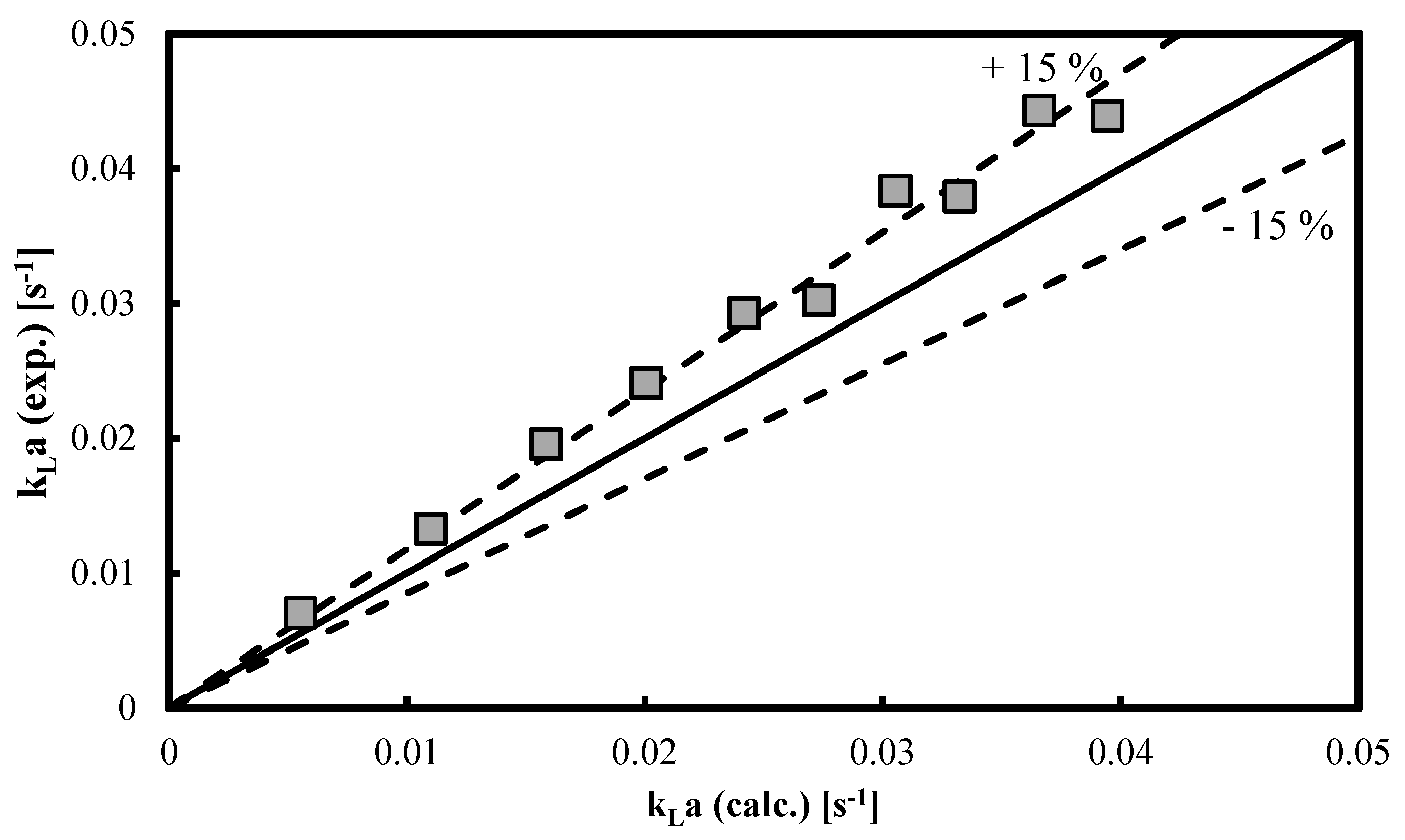
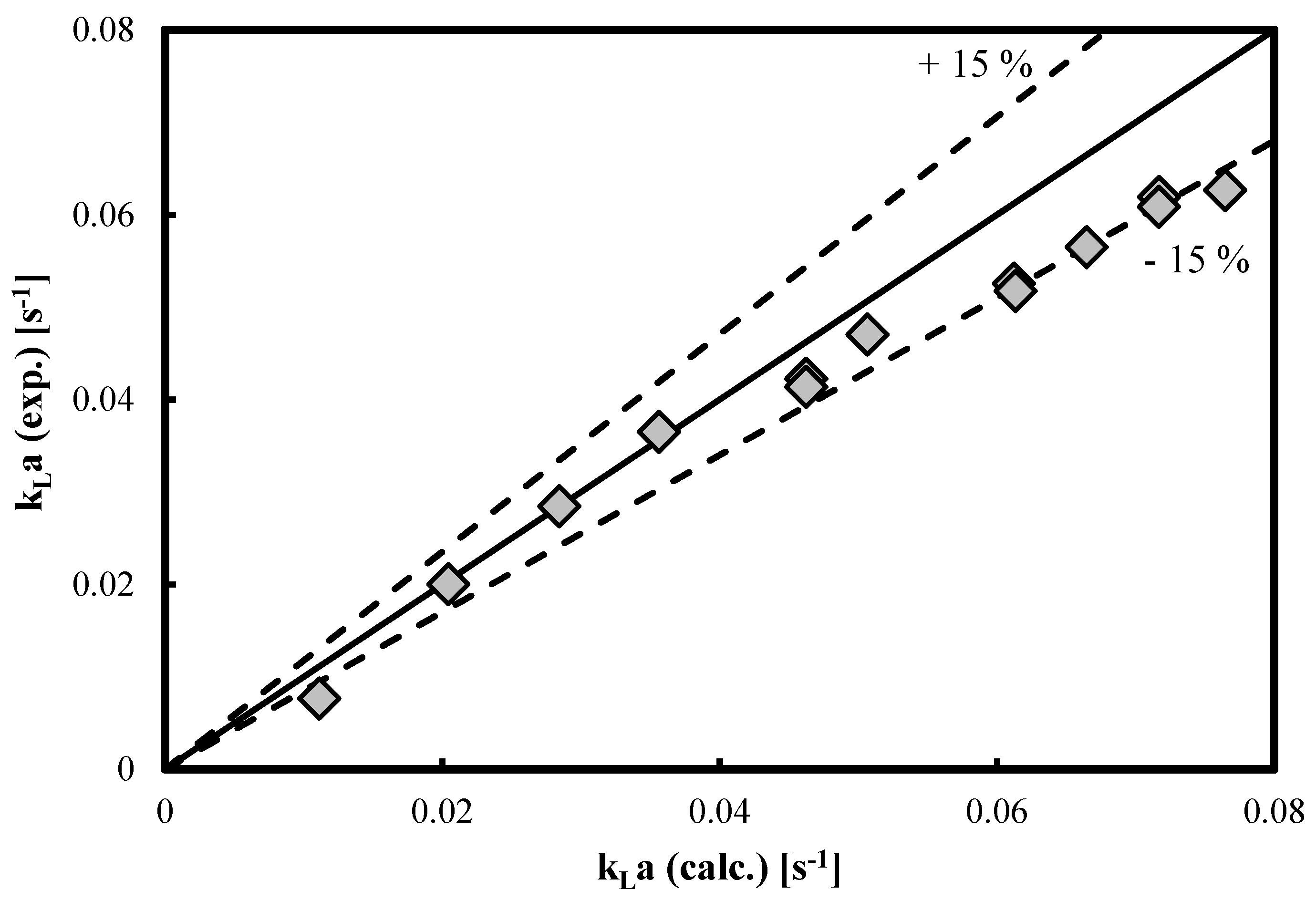
3.1. kLa Predictions in Alcohols
3.2. kLa Predictions in Other Organic Liquids
4. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| a | gas–liquid interfacial area, m−1 |
| a1 | parameter in the gas holdup correlation (see Table 1) [11], − |
| b1 | parameter in the mass transfer correlation (see Table 2) [11], − |
| CL | liquid-phase concentration, arbitrary units |
| CL∞ | liquid-phase concentration at saturation, arbitrary units |
| Dc | column diameter, m |
| DL | molecular diffusivity, m2/s |
| ds | Sauter-mean bubble diameter, m |
| f | parameter in the correlation of Hikita et al. [19,31] |
| fc | correction factor, − |
| I | ionic strength in gas holdup correlation [19], kg ion/m3 |
| kL | liquid-phase mass transfer (MT) coefficient, m/s |
| kLa | volumetric liquid-phase MT coefficient, s−1 |
| P | operating pressure, MPa |
| tc | gas–liquid contact time, s |
| ub | bubble rise velocity, m/s |
| Ug | superficial gas velocity, m/s |
| Greek letters | |
| εg | gas holdup, − |
| εL | liquid holdup, − |
| μL | liquid viscosity, Pa s |
| νL | liquid kinematic viscosity, m2/s |
| ρL | liquid density, kg/m3 |
| σL | surface tension, N/m |
| Dimensionless numbers | |
| Bo | Bond number (=gρLDc2/σL), − |
| Eo | Eötvös number (=gρLds2/σL), − |
| Fr | Froude number (=Ug/(gds)0.5), − |
| Fr’ | Froude number based on Dc (=Ug/(gDc)0.5), − |
| Ga | Galilei number (=gds3/νL2), − |
| Ga’ | Galilei number based on Dc (=gDc3/νL2), − |
| Mo | Morton number (=gμL4/ρLσL3), − |
| Pe | Peclet number, − |
| Re | Reynolds number, − |
| Reb | bubble Reynolds number, − |
| Sc | Schmidt number (=νL/DL), − |
| Sh | Sherwood number based on kLa (=kLads2/DL), − |
| Sh’ | Sherwood number based on kL (=kLds/DL), − |
| Sh” | Sh number based on kLa (liquid volume) (=(kLa/εL)ds2/DL), − |
| Ta | Tadaki number (=Reb0.23Mo), − |
| Abbreviations | |
| ADM | axial dispersion model |
| ARE | average relative error |
| BC | bubble column |
| CSTR | continuous stirred tank reactor |
| MT | mass transfer |
References
- Deckwer, W.-D.; Schumpe, A. Improved tools for bubble column reactor design and scale-up. Chem. Eng. Sci. 1993, 48, 889–911. [Google Scholar] [CrossRef]
- Han, L.; Al-Dahhan, M.H. Gas-liquid mass transfer in a high pressure bubble column reactor with different sparger designs. Chem. Eng. Sci. 2007, 62, 131–139. [Google Scholar] [CrossRef]
- Rubio, F.C.; Garcia, J.L.; Molina, E.; Chisti, Y. Steady-state axial profiles of dissolved oxygen in tall bubble column bioreactors. Chem. Eng. Sci. 1999, 54, 1711–1723. [Google Scholar] [CrossRef]
- Linek, V.; Vacek, V.; Benes, P. A critical review and experimental verification of the correct use of the dynamic method for the determination of oxygen transfer in aerated agitated vessels to water, electrolyte solutions and viscous liquids. Chem. Eng. J. 1987, 34, 11–34. [Google Scholar] [CrossRef]
- Chang, H.N.; Halard, B.; Moo-Young, M. Measurement of kLa by a gasing-in method with oxygen-enriched air. Biotechnol. Bioeng. 1989, 34, 1147–1157. [Google Scholar] [CrossRef]
- Chisti, Y. Airlift Bioreactors; Elsevier: London, UK, 1989. [Google Scholar]
- Jordan, U.; Schumpe, A. The gas density effect on mass transfer in bubble columns with organic liquids. Chem. Eng. Sci. 2001, 56, 6267–6272. [Google Scholar] [CrossRef]
- Deckwer, W.-D.; Hallensleben, J.; Popovic, M. Exclusion of gas sparger influence on mass transfer in bubble columns. Can. J. Chem. Eng. 1980, 58, 190–197. [Google Scholar] [CrossRef]
- Miller, D.N. Scale-up of agitated vessels gas-liquid mass transfer. AIChE J. 1974, 20, 445–453. [Google Scholar] [CrossRef]
- Higbie, R. The rate of absorption of a pure gas into a still liquid during short periods of exposure. Trans. AIChE 1935, 31, 365–389. [Google Scholar]
- Nedeltchev, S.; Jordan, U.; Schumpe, A. Correction of the penetration theory based on mass-transfer data from bubble columns operated in the homogeneous regime under high pressure. Chem. Eng. Sci. 2007, 62, 6263–6273. [Google Scholar] [CrossRef]
- Deckwer, W.-D. On the mechanism of heat transfer in bubble column reactors. Chem. Eng. Sci. 1980, 35, 1341–1346. [Google Scholar] [CrossRef]
- Calderbank, P.H. Gas absorption from bubbles. Chem. Eng. 1967, 45, CE209–CE233. [Google Scholar]
- Xue, J.; Al-Dahhan, M.; Dudukovic, M.P.; Mudde, R.F. Bubble dynamics measurements using four-point optical probe. Can. J. Chem. Eng. 2003, 81, 375–381. [Google Scholar] [CrossRef]
- Jasim, A.; Sultan, A.J.; Al-Dahhan, M. Impact of heat exchanging internals configurations on the gas holdup and bubble properties in a bubble column. Int. J. Multiph. Flow 2019, 112, 63–82. [Google Scholar] [CrossRef]
- Jasim, A.; Sultan, A.J.; Al-Dahhan, M. Influence of heat-exchanging tubes diameter on the gas holdup and bubble dynamics in a bubble column. Fuel 2019, 236, 1191–1203. [Google Scholar] [CrossRef]
- Wilkinson, P.M.; Haringa, H.; Van Dierendonck, L.L. Mass transfer and bubble size in a bubble column under pressure. Chem. Eng. Sci. 1994, 49, 1417–1427. [Google Scholar] [CrossRef]
- Akita, K.; Yoshida, F. Gas holdup and volumetric mass transfer coefficient in bubble columns. Ind. Eng. Chem. Process Des. Dev. 1973, 12, 76–80. [Google Scholar] [CrossRef]
- Hikita, H.; Asai, S.; Tanigawa, K.; Segawa, K.; Kitao, K. Gas hold-ups in bubble columns. Chem. Eng. J. 1980, 20, 59–67. [Google Scholar] [CrossRef]
- Hammer, H.; Schrog, H.; Hektor, K.; Schönau, K.; Küsters, W.; Soemarno, A.; Sahabi, U.; Napp, W. New subfunctions in hydrodynamics, heat and mass transfer for gas/liquid and gas/liquid/solid chemical and biochemical reactors. Front. Chem. React. Eng. 1984, 464–474. [Google Scholar]
- Idogawa, K.; Ikeda, K.; Fukuda, T.; Morooka, S. Effect of gas and liquid properties on the behavior of bubbles in a column under high pressure. Int. Chem. Eng. 1987, 27, 93–99. [Google Scholar] [CrossRef]
- Reilly, J.G.; Scott, D.S.; Bruijn, T.; Jain, A.; Diskorz, J. Correlation for gas holdup in turbulent coalescing bubble columns. Can. J. Chem. Eng. 1986, 64, 705–717. [Google Scholar] [CrossRef]
- Sotelo, J.L.; Benitez, F.J.; Beltran-Heredia, J.; Rodriguez, C. Gas holdup and mass transfer coefficients in bubble columns. 1. Porous gas plate diffusers. Int. Chem. Eng. 1994, 34, 82–91. [Google Scholar]
- Öztürk, S.S.; Schumpe, A.; Deckwer, W.-D. Organic liquids in a bubble column: Holdups and mass transfer coefficients. AIChE J. 1987, 33, 1473–1480. [Google Scholar] [CrossRef]
- Schumpe, A.; Deckwer, W.-D. Viscous media in tower bioreactors: Hydrodynamic characteristics and mass transfer properties. Bioprocess Eng. 1987, 2, 79–94. [Google Scholar] [CrossRef]
- Kemoun, A.; Ong, B.C.; Gupta, P.; Al-Dahhan, M.H.; Dudukovic, M.P. Gas holdup in bubble columns at elevated pressure via computed tomography. Int. J. Multiph. Flow 2001, 27, 929–946. [Google Scholar] [CrossRef]
- Calderbank, P.H.; Lochiel, A.C. Mass transfer coefficients, velocities and shapes of carbon dioxide bubbles in free rise through distilled water. Chem. Eng. Sci. 1964, 19, 485–503. [Google Scholar] [CrossRef]
- Lochiel, A.C.; Calderbank, P.H. Mass transfer in the continuous phase around axisymmetric bodies of revolution. Chem. Eng. Sci. 1964, 19, 471–484. [Google Scholar] [CrossRef]
- Calderbank, P.H.; Johnson, D.S.L.; Loudon, J. Mechanics and mass transfer of single bubbles in free rise through some Newtonian and non-Newtonian liquids. Chem. Eng. Sci. 1970, 25, 235–256. [Google Scholar] [CrossRef]
- Akita, K.; Yoshida, F. Bubble size, interfacial area and liquid-phase mass transfer coefficient in bubble columns. Ind. Eng. Chem. Process Des. Dev. 1974, 13, 84–91. [Google Scholar] [CrossRef]
- Hikita, H.; Asai, S.; Tanigawa, K.; Segawa, K.; Kitao, K. The volumetric mass transfer coefficient in bubble columns. Chem. Eng. J. 1981, 22, 61–67. [Google Scholar] [CrossRef]
- Suh, I.-S.; Schumpe, A.; Deckwer, W.-D.; Kulicke, W.-M. Gas-liquid mass transfer in the bubble column with viscoelastic liquid. Can. J. Chem. Eng. 1991, 69, 506–512. [Google Scholar] [CrossRef]
- Kawase, Y.; Halard, B.; Moo-Young, M. Theoretical prediction of volumetric mass transfer coefficients in bubble columns for Newtonian and non-Newtonian fluids. Chem. Eng. Sci. 1987, 42, 1509–1617. [Google Scholar] [CrossRef]
- Terasaka, K.; Inoue, Y.; Kakizaki, M.; Niwa, M. Simultaneous measurement of three-dimensional shape and behavior of single bubble in liquid using laser sensors. J. Chem. Eng. Jpn. 2004, 37, 921–926. [Google Scholar] [CrossRef]
- Schügerl, K.; Lucke, J.; Oels, U. Bubble column bioreactors. Adv. Biochem. Eng. 1977, 54, 1–84. [Google Scholar]
- Shah, Y.T.; Kelkar, B.J.; Godbole, S.P.; Deckwer, W.-D. Design parameters estimations for bubble column reactors. AIChE J. 1982, 28, 353–379. [Google Scholar] [CrossRef]
- Leonard, C.; Ferrasse, J.-H.; Boutin, O.; Lefevre, S.; Viand, A. Bubble column reactors for high pressures and high temperatures operation. Chem. Eng. Res. Des. 2015, 100, 391–421. [Google Scholar] [CrossRef]




| Authors | Empirical Correlation |
|---|---|
| Jordan and Schumpe [7] | εg/(1 − εg) = a1Eo0.16Ga0.04Fr0.7(1 + 27.0Fr0.52(ρG/ρL)0.58) |
| Akita and Yoshida [18] | εg/(1 − εg) = 0.2Bo0.125(Ga’)0.083Fr’ |
| Hikita et al. [19] | εg = 0.672f(UgμL/σL)0.578Mo−0.131(ρG/ρL)0.062(μG/μL)0.107 f = 1.0 for electrolyte solutions; f = 100.0414I for 0 < I < 1.0 kg ion/m3; f = 1.1 for I > 1.0 kg ion/m3; |
| Hammer et al. [20] | εg/(1 − εg) = 0.4(UgμL/σL)0.87Mo−0.27(ρG/ρL)0.17 |
| Idogawa et al. [21] | εg/(1 − εg) = 0.059Ug0.8ρG0.17(σL/72)−0.22exp(-P) |
| Reilly et al. [22] | εg = 296Ug0.44ρL−0.98ρG0.19σL−0.16 + 0.009 |
| Sotelo et al. [23] | εg = 129(UgμL/σL)0.99Mo−0.123(ρG/ρL)0.187(μG/μL)0.343(ds/Dc)−0.089 |
| Authors | Empirical Correlation |
|---|---|
| Akita and Yoshida [30] | Sh’ = 0.5Sc0.5Ga0.25Eo0.375 |
| Öztürk et al. [24] | Sh = 0.62 Sc0.5Eo0.33Ga0.29Fr0.68(ρG/ρL)0.04 |
| Hikita et al. [31] | kLaUg/g = 14.9f(UgμL/σL)1.76Mo−0.248(μG/μL)0.243Sc−0.604 f = 1 for non-electrolytes; |
| Jordan and Schumpe [7] | Sh” = b1Sc0.5Bo0.34Ga0.27Fr0.72(1 + 13.2Fr0.37(ρG/ρL)0.49) |
| Liquid | ρL (kg/m3) | μL (Pa s) | σL (N/m) | νL (m2/s) | DL (m2/s) | Sc (−) |
|---|---|---|---|---|---|---|
| Anilin | 1022 | 4.4 × 10−3 | 43.5 × 10−3 | 4.31 × 10−6 | 0.97 × 10−9 | 4438.44 |
| 1-Butanol | 809 | 2.94 × 10−3 | 24.6 × 10−3 | 3.63 × 10−6 | 1.29 × 10−9 | 2817.14 |
| Decalin | 884 | 2.66 × 10−3 | 32.5 × 10−3 | 3.01 × 10−6 | 1.60 × 10−9 | 1880.66 |
| Ethyl. glycol | 1113 | 19.94 × 10−3 | 47.4 × 10−3 | 17.92 × 10−6 | 2.60 × 10−10 | 68,905.94 |
| Nitrobenzene | 1203 | 2.02 × 10−3 | 38.1 × 10−3 | 1.68 × 10−6 | 1.63 × 10−9 | 1030.14 |
| 2-Propanol | 785 | 2.42 × 10−3 | 21.1 × 10−3 | 3.08 × 10−6 | 1.44 × 10−9 | 2140.84 |
| Tetralin | 968 | 2.18 × 10−3 | 34.9 × 10−3 | 2.25 × 10−6 | 1.58 × 10−9 | 1425.36 |
| Liquid | ds (m) | Reb (−) | Mo (−) | Ta (−) | Eo (−) |
|---|---|---|---|---|---|
| Anilin | 5.15–5.39 × 10−3 | 244.3–257.2 | 4.371 × 10−8 | 4.96–5.22 | 6.16–6.76 |
| 1-Butanol | 4.29–4.49 × 10−3 | 267.5–281.5 | 6.392 × 10−8 | 5.92–6.24 | 6.07–6.65 |
| Decalin | 4.30–4.50 × 10−3 | 284.4–298.8 | 1.769 × 10−8 | 4.69–4.93 | 5.37–5.89 |
| Ethyl. glycol | 7.11–7.45 × 10−3 | 85.9–91.0 | 1.308 × 10−5 | 6.47–6.86 | 11.75–12.89 |
| Nitrobenzene | 3.85–4.03 × 10−3 | 431.2–452.5 | 2.455 × 10−9 | 4.51–4.73 | 4.64–5.09 |
| 2-Propanol | 3.97–4.14 × 10−3 | 232.8–244.8 | 4.563 × 10−8 | 4.77–5.02 | 5.75–6.14 |
| Tetralin | 4.19–4.39 × 10−3 | 361.6–379.6 | 5.384 × 10−9 | 4.53–4.76 | 4.83–5.29 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nedeltchev, S. Unified Approach for Prediction of the Volumetric Mass Transfer Coefficients in a Homogeneous and Heterogeneous Bubble Column Based on the Non-Corrected Penetration Theory: Case Studies. Processes 2022, 10, 1828. https://doi.org/10.3390/pr10091828
Nedeltchev S. Unified Approach for Prediction of the Volumetric Mass Transfer Coefficients in a Homogeneous and Heterogeneous Bubble Column Based on the Non-Corrected Penetration Theory: Case Studies. Processes. 2022; 10(9):1828. https://doi.org/10.3390/pr10091828
Chicago/Turabian StyleNedeltchev, Stoyan. 2022. "Unified Approach for Prediction of the Volumetric Mass Transfer Coefficients in a Homogeneous and Heterogeneous Bubble Column Based on the Non-Corrected Penetration Theory: Case Studies" Processes 10, no. 9: 1828. https://doi.org/10.3390/pr10091828
APA StyleNedeltchev, S. (2022). Unified Approach for Prediction of the Volumetric Mass Transfer Coefficients in a Homogeneous and Heterogeneous Bubble Column Based on the Non-Corrected Penetration Theory: Case Studies. Processes, 10(9), 1828. https://doi.org/10.3390/pr10091828







