Coherent-Price Systems and Uncertainty-Neutral Valuation

Abstract

This paper considers fundamental questions of arbitrage pricing that arises when the uncertainty model incorporates ambiguity about risk. This additional ambiguity motivates a new principle of risk- and ambiguity-neutral valuation as an extension of the paper by Ross (1976) (Ross, Stephen A. 1976. The arbitrage theory of capital asset pricing. Journal of Economic Theory 13: 341–60). In the spirit of Harrison and Kreps (1979) (Harrison, J. Michael, and David M. Kreps. 1979. Martingales and arbitrage in multiperiod securities markets. Journal of Economic Theory 20: 381–408), the paper establishes a micro-economic foundation of viability in which ambiguity-neutrality imposes a fair-pricing principle via symmetric multiple prior martingales. The resulting equivalent symmetric martingale measure set exists if the uncertain volatility in asset prices is driven by an ambiguous Brownian motion.

1. Introduction

One cornerstone of modern neoclassical finance is the fundamental theorem of asset pricing (FTAP). In its simplest form and if uncertainty is modeled by a probability measure $\mathbb{P}$, the theorem states equivalence between: part (a) the absence of $\mathbb{P}$-arbitrage; and part (b) the existence of a $\mathbb{P}$-equivalent martingale measure (EMM) $\mathbb{Q}$. However, the microeconomic foundation of the FTAP relies on two further pillars: part (c) the price system within a viable equilibrium concept; and part (d) the preference-free valuation perspective.

As masterly elaborated by Harrison and Kreps (1979), part (c) can be directly connected to the infinite-dimensional equilibrium model à la Arrow–Debreu. On the other hand, as explained by Ross (1976), preference-free pricing (part (d)) boils down to risk-neutral pricing, when uncertainty boils down to risk. For an account of all four concepts under single-prior uncertainty, see Dybvig and Ross (2003).

This paper removes the underlying assumption of a given objective, physical, or reference probability measure $\mathbb{P}$. Instead, a set of measures $\mathcal{P}$ describes the (Knightian) uncertainty, which is parameterized by different stochastic volatility models $\sigma$. Such an approach deviates from models in which the term structures of volatilities, including stochastic volatility models such as that of Heston (1993), are described by another stochastic process. Doubts about the modeler’s ability to be aware of all relevant information lead to the view that knowing the true volatility regime is often impossible. As argued by Epstein and Ji (2013), the hypothetical confidence of a universal dependency between past and future is in question and modeling the volatility in terms of a stochastic process, in which the law of motion is exactly known, is avoided. In a similar vein, Carr and Lee (2009) argued that the choice of a particular model describing the short term volatility is problematic because the quantity being modeled is not directly observable. Although an estimate for the initially unobserved state variable can be inferred from market prices of derivative securities, noise in the data generates noise in the estimate, raising doubts that a modeler can correctly select any parametric stochastic process from the menu of consistent alternatives.”

This ambiguity about the true volatility model translates to a set priors $\mathcal{P}$ that is no longer mutually equivalent but happens to be mutually singular. Singular priors $\mathbb{P},{\mathbb{P}}^{\prime }$ live on disjoint supports, $\mathbb{P}\left(A\right)=1$ and ${\mathbb{P}}^{\prime }\left(A\right)=0$, for an event A. This aspect, in particular, requires a reformulation of each of parts (a)–(d) and a careful reconnection of their relations. As such, this paper establishes a FTAP under volatility uncertainty and accounts for a sound microeconomic foundation.

Before introducing the main results of the paper, the new and adjusted parts (a)–(d) deserve some motivation and a detailed account.

(a) Typical costless arbitrage is a non-negative contingent claim, that is with positive probability strictly positive. Such a definition clearly depends on the chosen objective prior that forms the law of the underlying asset price. The situation changes when uncertainty is described by a set of possibly mutually singular priors $\mathcal{P}$. As in Vorbrink (2014), a robust form of arbitrage refers to a positive claim that is strictly positive under some prior in $\mathcal{P}$. Geometrically, the set of arbitrage opportunities can be identified by the positive cone of the $\mathcal{P}$-dependent space of contingent claims with a deleted zero.

(b) Under sole risk and linear expectations, the martingale concept quantifies a fair game. This standard martingale notion can be extended to the multiple-prior uncertainty setting. Following Peng (2006), this paper considers a multiple-prior martingale notion that is based on a time-consistent conditional sublinear expectation $\left({\mathcal{E}}_t\right)$. Such an $\mathcal{E}$-martingale is a supermartingale (unfair game) for all single prior conditional expectations and a martingale (fair game) for some maximizing expected value with respect to the related priors. Only if all the priors in $\mathcal{P}$ are maximal is an $\mathcal{E}$-martingale called symmetric. This corresponds to a uniform notion of a fair game. Instead of the existence of an EMM $\mathbb{Q}$, under which the asset prices are martingales, the present objective multiple-prior uncertainty $\mathcal{P}$ now requires the existence of a set of measures $\mathcal{Q}$. This new set has to define a new multiple prior sublinear expectation ${\mathcal{E}}^{\mathcal{Q}}$ under which the asset price becomes a symmetric martingale. In that case, $\mathcal{Q}$ is called an equivalent symmetric martingale measure set (EsMM-set). The prior-by-prior equivalence to the objective world $\mathcal{P}$, now relies on a family of state prices that creates a risk- and ambiguity-adjusted expectation ${\mathcal{E}}^{\mathcal{Q}}$. The discussion about the role of ${\mathcal{E}}^{\mathcal{Q}}$ continues in part (d). The relation to viability as a model of equilibrium, stated in part (c), requires the notion of a particular nonlinear equilibrium price system that corresponds to ${\mathcal{E}}^{\mathcal{Q}}$.

(c) By accepting the modified notion of arbitrage in part (a) as a weak dominance principle, one crucial issue points to inconsistencies between the linear price system and the present concept of arbitrage. As in the single-prior setup, linear prices on the space of contingent claims are represented by a prior-dependent state-price density. Again, a risk-neutral measure $\mathbb{Q}$ refers to the normalized version of a linear price system in the sense of Arrow–Debreu. However, under volatility uncertainty, priors in $\mathcal{P}$ can be mutually singular. On the one hand, linear and positive prices only capture strictly positive payoffs in events that are in the support of the representing prior. On the other hand, such price systems are blind to an “arbitrage event” outside their support. For this reason, the $\mathbb{P}$-dependent linear prices are aggregated into a robust pricing scheme. The corresponding coherent price system is sublinear, and the new notion of viability (as a model of an economic equilibrium) no longer shares this problematic feature of linear price systems.1

(d) The EMM $\mathbb{Q}$ is often referred to as a description of the risk-neutral world. In fact, under this risk-neutral measure $\mathbb{Q}$, the returns of a risk-free and a risky asset coincide. From this perspective, the valuation of any contingent claim does not depend directly on the agent’s preferences for risk. The obtained valuation is then preference-free. Departing from this basic insight under sole risk, the next natural step is to ask how the preference-free approach can be applied to an objective multiple prior uncertainty model $\mathcal{P}$. To see this connection, consider an EsMM-set $\mathcal{Q}$ from part (b). Preferences for uncertainty consist of risk and ambiguity preferences. The new component, preferences for ambiguity, becomes neutral when we move to the uncertainty-neutral world $\mathcal{Q}$. The symmetry of ${\mathcal{E}}^{\mathcal{Q}}$-martingales, that is, the asset price is a $\mathbb{Q}$-martingale under each $\mathbb{Q}\in \mathcal{Q}$ with no ambiguity about the expected value, exactly corresponds to ambiguity-neutrality (in the mean). From this perspective, an EsMM-set $\mathcal{Q}$ is then a model of a risk- and ambiguity-neutral world. This reasoning qualifies the valuation principle to be called uncertainty neutral.

Based on parts (a)–(d), the FTAP of the present paper is established in a continuous-time setup, in which the risky and ambiguous asset price is driven by a Brownian motion $\mathsf{B}=\left(B^{\sigma }\right)$ with uncertain volatility $\sigma$. This is a zero-mean and stationary process with novel $\mathsf{N}(0,[\underline{\sigma },\overline{\sigma }])$-normally distributed independent increments. Such random variables are characterized by a nonlinear heat PDE. On the other hand, $\mathsf{N}(0,[\underline{\sigma },\overline{\sigma }])$-normally distributed random variables are the outcome of a robust central limit theorem for a given confidence interval $[\underline{\sigma },\overline{\sigma }]$, see Chapter II of Peng (2010). The resulting process is a canonical generalization of the standard Brownian motion, such that the volatility $\sigma =\left({\sigma }_t\right)$ moves almost arbitrarily within $[\underline{\sigma },\overline{\sigma }]$. A Samuelson (1965)-type model incorporates this kind of volatility uncertainty in a risky and ambiguous asset price process that follows the stochastic differential equation

$$\begin{array}{c}\hfill \mathrm{d}{S}_t={\mu }_t\mathrm{d}t+V_t\mathrm{d}{\mathsf{B}}_t.\end{array}$$

(1)

As in the classic single-prior setting, the increment $\mathrm{d}{S}_t$ in Equation (1) is divided into a locally certain part and a locally uncertain part $V_t\mathrm{d}{\mathsf{B}}_t$. The interpretation is

$$\begin{array}{c}\hfill \frac{\mathrm{d}}{\mathrm{d}r}{\mathrm{var}}_r^{\mathbb{P}}\left(S_t\right){|}_{r=t}\in \left[V_t·\underline{\sigma },V_t·\overline{\sigma }\right],\mathbb{P}\text{-}\mathrm{a}.\mathrm{s}.\end{array}$$

(2)

where ${\mathrm{var}}_r^{\mathbb{P}}\left(S_t\right)$ refers to the conditional variance under $\mathbb{P}$. In abuse of notation, the issue in Equation (2) is displayed by ${\mathrm{var}}_t\left(\mathrm{d}{S}_t\right)=V_t^2\mathrm{d}{\langle \mathsf{B}\rangle }_t$. Under a standard Brownian motion, the description in Equation (2) of Equation (1) boils down to the textbook description $\frac{\mathrm{d}}{\mathrm{d}r}{\mathrm{var}}_r\left(S_t\right){|}_{r=t}=V_t$, see Chapter 5 in Duffie (2010).

Section 3 departs from the equivalence in Section 2 of part (a) absence of $\mathcal{P}$-arbitrage; and part (b) existence of an EsMM-set $\mathcal{Q}$. To provide a microeconomic foundation, we discuss the connection between EsMM-sets, coherent price systems and viability as a model of an economic equilibrium from part (c). As mentioned in part (d), the resulting price system is a risk- and ambiguity-neutral valuation.

Related Literature

Harrison and Kreps (1979) described how the arbitrage pricing principle provided an economic foundation. Kreps (1981) continued to lay the foundation. In a later work, Delbaen and Schachermayer (1994) presented a general FTAP in continuous time.

Here, uncertainty and risk are seen as equals. Moving to a dynamic multiple-prior model, the concept of time consistency becomes crucial (see Epstein and Schneider 2003). A common assumption requires mutual equivalence of priors; the resulting drift uncertainty leaves the valuation principle almost unaffected. Cont (2006) noted that such an assumption “means that all models agree on the universe of possible scenarios […], if ${\mathbb{P}}_0$ defines a complete market model, this hypothesis entails that there is no uncertainty on option prices!"

Jouini and Kallal (1995) studied nonlinear pricing caused by bid–ask spreads and transaction costs. In Araujo et al. (2012), pricing rules with finitely many states are considered. An equilibrium with superlinear prices was discussed by Aliprantis et al. (2001). Regarding the consideration of financial markets under volatility uncertainty, see the works of Avellaneda et al. (1995); Denis and Martini (2006) and Peng (2006) on ambiguous Brownian motions. Epstein and Ji (2013) provided a consumption-based asset pricing model. More recently and also under volatility uncertainty, Bouchard and Nutz (2015) discussed the relation between arbitrage and pricing measures in a discrete-time framework.

2. Risk- and Ambiguity-Neutral Asset Pricing

For the whole section, $\mathcal{P}$ is induced by volatility uncertainty about an underlying one-dimensional state process $\left(B_t\right)$. Moreover, assume that $\Omega =C_0\left([0,T]\right)$, the path space of continuous functions $\omega :[0,T]\to \mathbb{R}$ with ${\omega }_0=0$. For the construction of the ambiguous volatility model, consider the Wiener measure ${\mathbb{P}}_0$ that makes the coordinate process $B_t\left(\omega \right)={\omega }_t$ into a Brownian motion. $\mathcal{F}$ denotes the Borel $\sigma$-algebra on $\Omega$. Fix the filtration $\mathbb{F}={\left({\mathcal{F}}_t\right)}_{t\in [0,T]}$ generated by ${\mathcal{F}}_t=\sigma (B_s:s\in [0,t])\vee {\mathcal{N}}_{{\mathbb{P}}_0}$, where ${\mathcal{N}}_{{\mathbb{P}}_0}$ denotes the collection of ${\mathbb{P}}_0$-null sets. Fix an adapted, ${\mathbb{P}}_0\otimes \mathrm{d}t$-square integrable and positive volatility process $\left({\sigma }_t\right)$.

Volatility uncertainty is then based upon martingale laws on $(\Omega ,\mathcal{F})$:

$$\begin{array}{c}\hfill {\mathbb{P}}_{\sigma }:={\mathbb{P}}_0\circ {\left(B^{\sigma }\right)}^{-1},\mathrm{where}{B}_t^{\sigma }={\int }_0^t{\sigma }_s\mathrm{d}{B}_s\end{array}$$

(3)

Via the construction in Equation (3), a set $\mathcal{D}$ of volatility regimes builds the uncertainty model $\mathcal{P}={\left({\mathbb{P}}_{\sigma }\right)}_{\sigma \in \mathcal{D}}$. The quadratic variation ${\langle B^{\sigma }\rangle }_t={\int }_0^t{\sigma }_s^2\mathrm{d}s$ then describes the volatility of $B^{\sigma }$ under ${\mathbb{P}}_0$. The leading Example 1 satisfies the following standing assumption for $\mathcal{P}$. This allows to define in Section 2.2 a sublinear conditional expectation that satisfies the law of iterated expectations. As in Nutz and Soner (2012), for each stopping time $\tau$, define ${\mathcal{P}}_{\tau ,\mathbb{P}}=\{{\mathbb{P}}^{\prime }\in \mathcal{P}:\mathbb{P}={\mathbb{P}}^{\prime }on{\mathcal{F}}_{\tau }\}$, which consists of all the priors in $\mathcal{P}$ that agree with a $\mathbb{P}\in \mathcal{P}$ in the events up to time t.2

Assumption 1.

The set of priors $\mathcal{P}$ isstable under pasting, if for every $P\in \mathcal{P}$, every $\mathbb{F}$-stopping time τ, $B\in {\mathcal{F}}_{\tau }$ and $\mathbb{P},{\mathbb{P}}^{\prime }\in {\mathcal{P}}_{\tau ,\mathbb{P}}$, we have ${\mathbb{P}}_{\tau }\in \mathcal{P}$, where ${\mathbb{P}}_{\tau }\left(A\right)=E^{\mathbb{P}}\left[\mathbb{P}\left(A\right|{\mathcal{F}}_{\tau })1_B+{\mathbb{P}}^{\prime }\left(A\right|{\mathcal{F}}_{\tau })1_{B^c}\right]$, for all $A\in {\mathcal{F}}_{\tau }$.

Example 1.

Suppose that two volatility models ${\sigma }^1$ and ${\sigma }^2$ are consistent with a given dataset. Their respective implications for a trading decision may differ considerably. To address the possibility of different volatility regimes, define the universal extreme cases ${\underline{\sigma }}_t=inf({\sigma }_t^1,{\sigma }_t^2)$ and ${\overline{\sigma }}_t=sup({\sigma }_t^1,{\sigma }_t^2)$. When thinking about reasonable uncertainty management, no scenario between $\underline{\sigma }$ and $\overline{\sigma }$ should be ignored. These boundaries result in:

$$\begin{array}{c}\hfill \mathcal{P}=\left\{{\mathbb{P}}_{\sigma }:{\sigma }_t\in [{\underline{\sigma }}_t,{\overline{\sigma }}_t]\mathrm{for}\mathrm{all}t\in [0,T]\right\}.\end{array}$$

(4)

For $X\in {\mathcal{C}}_b(\Omega )$. define the upper expectation ${\mathcal{E}}^{\mathcal{P}}\left(X\right)={max}_{\mathbb{P}\in \mathcal{P}}{E}^{\mathbb{P}}\left[X\right]$ and the norm ${\parallel ·\parallel }_{\mathcal{P}}$ on ${\mathcal{C}}_b(\Omega )$, the space of all bounded continuous real-valued functions on $\Omega$, given by ${\parallel X\parallel }_{\mathcal{P}}={\mathcal{E}}^{\mathcal{P}}{\left(X^2\right)}^{\frac{1}{2}}$. The completion of ${\mathcal{C}}_b(\Omega )$ under ${\parallel ·\parallel }_{\mathcal{P}}$ is denoted by ${\mathcal{L}}_{\mathcal{P}}$, and let $L_{\mathcal{P}}={\mathcal{L}}_{\mathcal{P}}/\mathcal{N}$ be the quotient space of ${\mathcal{L}}_{\mathcal{P}}$ with respect to the ${\parallel ·\parallel }_{\mathcal{P}}$-null elements $\mathcal{N}$. The elements in $L_{\mathcal{P}}$ have finite variance under every $\mathbb{P}\in \mathcal{P}$ (see Appendix A for a representation of $L_{\mathcal{P}}$). Clearly, $L_{\mathbb{P}}=L^2(\Omega ,\mathcal{F},\mathbb{P})$ is the standard Lebesgue space.

A property holds $\mathcal{P}$-q.s. (quasi surely) if it holds $\mathbb{P}$-almost surely for every $\mathbb{P}\in \mathcal{P}$. A payoff X is positive if $X\ge 0$$\mathcal{P}$-q.s. This induces an order relation ${\ge }_{\mathcal{P}}$, denoted by ≥, on $L_{\mathcal{P}}$ so that the classical arguments prove that $(L_{\mathcal{P}},\parallel ·{\parallel }_{\mathcal{P}},\ge )$ is a Banach lattice (see Appendix A). In addition, set $L_{\mathcal{P},t}=\{X\in L_{\mathcal{P}}:X{\mathcal{F}}_t\text{-}\mathit{measurable}\}$. For questions of asset pricing, $\psi$-deflated claims $\psi X$ play a central role. To guarantee ${\mathcal{E}}^{\mathcal{P}}\left(\psi X\right)<\infty$, it suffices to assume $\psi ,X\in L_{\mathcal{P}}$.

2.1. Arbitrage and Primitives of the Financial Market

For the sake of simplicity, the price of the riskless asset satisfies $S_t^0=1$, that is, the interest rate is zero. Fix a pair $S=(1,S^1)$ on the filtered uncertainty space $(\Omega ,\mathcal{F},\mathcal{P};\mathbb{F})$, where the price process of the uncertain asset $\left(S_t^1\right)$ satisfies $S_t^1\in L_{\mathcal{P}}$ for each t and is $\mathbb{F}$-adapted. As in Harrison and Kreps (1979), trading strategies are simple and omit to exclude doubling strategies under some completion. A simple strategy is an ${\mathcal{F}}_t$-adapted stochastic process $\eta =({\eta }^0,{\eta }^1)$ such that there is a finite sequence of dates $0<t_0\le \cdots \le t_N=T$ and ${\eta }^i$, $i=0,1$, and can be written as ${\eta }_t^i={\sum }_{k=0}^{N-1}{1}_{[t_i,t_{i+1})}\left(t\right){\eta }^{i,k}$, with ${\eta }^{i,k}\in L_{\mathcal{P},t_k}$. The fraction invested in the riskless asset is denoted by ${\eta }_t^0$. A trading strategy $\eta =({\eta }^0,{\eta }^1)$ is self-financing if ${\eta }_{t_{n-1}}{(1,S^1)}_{t_n}={\eta }_{t_n}{(1,S^1)}_{t_n}$ for all dates. The value of the portfolio $X_t^{\eta }$ satisfies $\mathcal{E}\left(X_t^{\eta }\right)<\infty$ for every t. The set of self-financing trading strategies is denoted by $\mathcal{A}$. This financial market $(1,S^1)$, taken together with $\mathcal{A}$, is denoted by $\mathtt{FM}(1,S^1)$.

Under model uncertainty, a robust notion of no-arbitrage notion is a basic step. The following arbitrage concept is a slight generalization of Vorbrink (2014).

Definition 1.

Let $\mathcal{R}\subset \mathcal{P}$. There is an $\mathcal{R}$-arbitrage opportunity in $\mathtt{FM}(1,S^1)$ if an admissible pair $\eta \in \mathcal{A}$ exists such that

${\eta }_0{S}_0\le 0$, ${\eta }_T{S}_T\ge 0$$\mathbb{P}$-a.s.$\forall \mathbb{P}\in \mathcal{R}$ and ${\mathbb{P}}^{*}\left({\eta }_T{S}_T>0\right)>0$ for some ${\mathbb{P}}^{*}\in \mathcal{R}$.

The definition rests on the following thought experiment. An arbitrage strategy is riskless for each $\mathbb{P}\in \mathcal{R}$, and if the prior ${\mathbb{P}}^{*}$ describes the dynamics of asset prices, one would gain a profit with a strictly positive probability. The $\mathcal{P}$-arbitrage notion can be seen as a rather weak arbitrage opportunity and one could argue that no $\mathcal{R}$-arbitrage is consistent with a weak dominance principle based on $\mathcal{R}$. If $\mathcal{R}=\left\{\mathbb{P}\right\}$, the usual arbitrage concept under $\mathbb{P}$ appears.

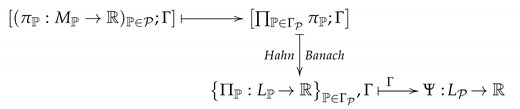
Remark 1.

As originally discussed by Kreps (1981), the order structure of the underlying space of claims determines the natural candidate for a no-arbitrage condition. The order relation ${\ge }_{\mathcal{P}}$ on $L_{\mathcal{P}}$ defines the arbitrage cone $L_{\mathcal{P}+}\setminus \left\{0\right\}$ by deleting the zero of $L_{\mathcal{P}+}:=\{X\in L_{\mathcal{P}}:X{\ge }_{\mathcal{P}}0\}$. Every $\mathcal{R}\subset \mathcal{P}$ defines a weaker order relation ${\ge }_{\mathcal{R}}$ and a larger arbitrage cone. The arbitrage concept in Definition 1 allows any subset $\mathcal{R}\subset \mathcal{P}$ to determine what an arbitrage is.

2.2. EsMM Sets and Ambiguity Neutrality

Let us move to the dynamics of a continuous-time, multiple-prior uncertainty model. The unique existence of sublinear conditional expectations ${\mathcal{E}}_t^{\mathcal{P}}:L_{\mathcal{P}}\to L_{\mathcal{P},t}$ is provided through the following construction, which is based on the dynamic programming principle:

$$\begin{array}{c}\hfill {\mathcal{E}}_t^{\mathcal{P}}\left(X\right)=\mathrm{ess}\underset{{\mathbb{P}}^{\prime }\in \mathcal{P}}{sup}{E}_t^{{\mathbb{P}}^{\prime }}\left[X\right]\mathbb{P}\text{-}\mathrm{a}.\mathrm{s}.\mathrm{for}\mathrm{all}\mathbb{P}\in \mathcal{P}.\end{array}$$

(5)

See Section 2 of Epstein and Ji (2014) for details and a list of properties. The sequence of conditioning satisfies the law of iterated expectations, that is, for every $X\in L_{\mathcal{P}}$ the updating rule ${\mathcal{E}}_t^{\mathcal{P}}\left(X\right)={\mathcal{E}}_t^{\mathcal{P}}\left({\mathcal{E}}_{t+s}^{\mathcal{P}}\left(X\right)\right)$ holds.

A process $\left(X_t\right)$ with $X_t\in L_{\mathcal{P},t}$ for each $t\in [0,T]$, is an ${\mathcal{E}}^{\mathcal{P}}$-martingale if

$$\begin{array}{c}\hfill {\mathcal{E}}_t^{\mathcal{P}}\left(X_{t+s}\right)=X_t\mathrm{for}\mathrm{all}s,t.\end{array}$$

(6)

For an ${\mathcal{E}}^{\mathcal{P}}$-martingale $\left(X_t\right)$, its negative $(-X_t)$ is in general not an ${\mathcal{E}}^{\mathcal{P}}$-martingale again. If this is the case, the process is a symmetric ${\mathcal{E}}^{\mathcal{P}}$-martingale, which is equivalent to the $E^{\mathbb{P}}$-martingale property of $\left(X_t\right)$ for every $\mathbb{P}\in \mathcal{P}$. Symmetry for a process $\left(X_t\right)$ implies no ambiguity about the expected value of $X_t$ under $\mathcal{P}$.

Definition 2.

A set of probability measures $\mathcal{Q}$ on Ω is called an equivalent symmetric martingale measure set (EsMM-set) if the following conditions hold:

1.

For every $\mathbb{Q}\in \mathcal{Q}$, there is a $\mathbb{P}\in \mathcal{P}$ that is equivalent via $\frac{\mathrm{d}\mathbb{Q}}{\mathrm{d}\mathbb{P}}\in L_{\mathbb{P}}$.

2.

$\left(S_t\right)$ is a symmetric ${\mathcal{E}}^{\mathcal{Q}}$-martingale where ${\mathcal{E}}^{\mathcal{Q}}={\{{\mathcal{E}}_t^{\mathcal{Q}}:L_{\mathcal{P}}\to L_{\mathcal{P},t}\}}_{t\in [0,T]}$ is the conditional sublinear expectation under $\mathcal{Q}$ which is stable under pasting.

The collection $\mathtt{EsMM}\left(\mathcal{P}\right)$ denotes the collection of all EsMM-sets $\mathcal{Q}$, as in Equation (14). The case $\mathcal{P}=\left\{\mathbb{P}\right\}$ boils down to the well-known EMM. The first condition states a direct relation between an element $\mathbb{Q}\in \mathcal{Q}$ and a primitive prior in $\mathcal{P}$. Integrability is a technical condition to guarantee the connection to viability and the commodity-price duality. The second part of Definition 2 points to the adjusted martingale condition. The idea of a fair gamble under $\mathcal{Q}$ reflects the neutrality of preferences for both risk and ambiguity. The symmetric martingale property of S implies, as discussed in the Introduction regarding part (c) and part (d), that the (expected) value of the claim is constant with respect to different measure in the EsMM-set. In other words, the valuation is mean-ambiguity free, i.e., preferences for ambiguity under $\mathcal{Q}$ are neutral.

One can think of the ambiguity-neutral part in terms of risk-neutral maxmin preferences of Gilboa and Schmeidler (1989), i.e., the worst-case expected utility under $\mathcal{Q}$. For a claim X on $\left(S_t\right)$, $\mathbb{Q}\mapsto E^{\mathbb{Q}}\left[X\right]$ is then constant on $\mathcal{Q}$. Similarly to pricing under risk, in which risk preferences are irrelevant, analogous reasoning remains valid for preferences for ambiguity. As such, uncertainty neutrality immediately leads to the uncertainty-neutral expectation ${\mathcal{E}}^{\mathcal{Q}}$. The same reasoning remains valid for smooth ambiguity preferences of Klibanoff et al. (2005).

2.3. Existence of EsMM-sets

In this section, asset prices are driven by a G-Brownian motion, in which the volatility uncertainty is contained in the quadratic variation (see Appendix A.2 for an overview). This specific framework allows confirming the existence of EsMM-sets.

Again, the riskless asset $S^0$ has an interest rate $r_t$ of zero. Volatility uncertainty in the asset prices $S:=S^1$ means that every adapted stochastic process $\left({\sigma }_t\right)$ taking values in $[\underline{\sigma },\overline{\sigma }]$ is a possible model for the volatility of the underlying state process. More precisely, S is driven by a G-Brownian motion $\mathsf{B}={\left(B^{{\sigma }_t}\right)}_{\sigma \in \mathcal{D}}$ (see Appendix A.2 for a detailed exposition). As a special case of Example 1, $\mathcal{P}$ is now induced by $[\underline{\sigma },\overline{\sigma }]$. The asset price is determined by the following stochastic differential equation

$$\begin{array}{c}\hfill \mathrm{d}{S}_t={\mu }_t\left(S_t\right)\mathrm{d}{\langle \mathsf{B}\rangle }_t+V_t\left(S_t\right)\mathrm{d}{\mathsf{B}}_t,\end{array}$$

(7)

where ${\langle \mathsf{B}\rangle }_t={\langle B^{\sigma }\rangle }_t={\int }_0^t{\sigma }_s^2\mathrm{d}s$${\mathbb{P}}_{\sigma }$-almost surely. The process $t\mapsto V_t\left(x\right)$ is strictly positive and bounded. Let $\mu :[0,T]\times \Omega \times \mathbb{R}\to \mathbb{R}$ and $V:[0,T]\times \Omega \times \mathbb{R}\to {\mathbb{R}}_{+}$ be processes such that a unique solution of Equation (7) exists (see Part 5 of Peng (2010) for some classical Lipschitz continuity conditions on $\mu$ and V in the state variable).

Remark 2.

As in the classic probabilistic framework, a Girsanov transformation of ${\mathsf{B}}_t+{\int }_0^t{\theta }_r\left(S_r\right)\mathrm{d}{\langle \mathsf{B}\rangle }_r$, with ${\theta }_r\left(S_r\right)=\frac{{\mu }_r\left(S_r\right)}{V_r\left(S_r\right)}$, guarantees the existence of a nontrivial EsMM-set $\mathcal{Q}$. For this approach, define a new sublinear expectation on $L_{\mathcal{P}}$, via $\mathcal{Q}=\psi \mathcal{P}=\{\mathbb{Q}\in \Delta (\Omega ):A\mapsto \mathbb{Q}\left(A\right)={\int }_A\psi \mathrm{d}\mathbb{P}\mathit{and}\mathbb{P}\in \mathcal{P}\}$,

$$\begin{array}{c}\hfill {\mathcal{E}}^{\mathcal{Q}}\left(X\right)=\underset{\mathbb{Q}\in \mathcal{Q}}{max}{E}^{\mathbb{Q}}\left[X\right]=\underset{\mathbb{P}\in \mathcal{P}}{max}{E}^{\mathbb{P}}\left[\psi X\right]={\mathcal{E}}^{\mathcal{P}}\left(\psi X\right).\end{array}$$

(8)

The state price ψ in Equation (8) is now an aggregated object under the uncertainty model, i.e., $\psi ={\psi }_{\mathbb{P}}$$\mathbb{P}$-a.s. for all $\mathbb{P}\in \mathcal{P}$. This universal state-price density $\psi ={\psi }_T^{\theta }$ is the terminal value of a symmetric exponential martingale $\mathrm{d}{\psi }_t^{\theta }={\psi }_t^{\theta }{\theta }_t\left(S_t\right)\mathrm{d}{\mathsf{B}}_t$, with ${\psi }_0^{\theta }=1$. The process $\left({\theta }_t\right)$ is now the market price of risk and ambiguity. Similarities to the single-prior case are apparent. The results in Appendix A.2 allow us to write ${\psi }_T^{\theta }$ explicitly as

$$\begin{array}{c}\hfill {\psi }_T^{\theta }=exp\left(-\frac{1}{2}{\int }_0^T{\theta }_t{\left(S_t\right)}^2\mathrm{d}{\langle \mathsf{B}\rangle }_t-{\int }_0^T{\theta }_t\left(S_t\right)\mathrm{d}{\mathsf{B}}_t\right).\end{array}$$

(9)

Let the pricing kernel in Equation (9) solve $V_t\left(S_t\right){\theta }_t\left(S_t\right)={\mu }_t\left(S_t\right)$. Define the symmetric ${\mathcal{E}}^{\mathcal{P}}$-martingale $S_t^{*}=S_0^{*}+{\int }_0^t{V}_r\left(S_r^{*}\right)\mathrm{d}{\mathsf{B}}_r$ (see the second result in Appendix A.2), and assume ${\mathcal{E}}^{\mathcal{P}}\left(exp\left(\delta ·{\int }_0^T{\theta }_t{\left(S_t\right)}^2\mathrm{d}{\langle \mathsf{B}\rangle }_t\right)\right)<\infty$ for some $\delta >\frac{1}{2}$. Under these conditions, we have the following FTAP under ambiguous volatility. The result corresponds to Theorem 3 of Harrison and Kreps (1979) where uncertainty is captured by a single objective probability measure.

Theorem 1.

An EsMM-set $\mathcal{Q}=\psi \mathcal{P}$ exists if and only if $\mathcal{P}$-arbitrage in $\mathtt{FM}(1,S)$ is absent.

Remark 3.

Theorem 3.3 of Epstein and Ji (2013) obtains analogous state prices by using a consumption-based utility-gradient approach and assuming ${\mu }_t\left(S_t\right)={\mu }_t{S}_t$ and $V_t\left(S_t\right)=V_t{S}_t$. The local form of (7) would then be apparently governed by $\frac{\mathrm{d}{S}_t}{S_t}=b_t\mathrm{d}{\langle \mathsf{B}\rangle }_t+V_t\mathrm{d}{\mathsf{B}}_t$. The relation between the asset price processes, with pricing kernel $\left(\frac{{\mu }_t}{V_t}\right)=\left({\theta }_t\right)$, is as follows ${\int }_0^t{\mu }_s\mathrm{d}{\langle \mathsf{B}\rangle }_s={\int }_0^t{\mu }_s{\widehat{a}}_s\mathrm{d}s={\int }_0^t{b}_s\mathrm{d}s$, where ${\widehat{a}}_s=\frac{\mathrm{d}}{\mathrm{d}s}{\langle \mathsf{B}\rangle }_s$ denotes the derivative of the ambiguous quadratic variation.

We end this section with an example on option pricing under volatility uncertainty.

Example 2.

Let $\theta =\frac{{\mu }_t(·)}{V_t(·)}$ be constant and $X=\phi \left(S_T\right)$ be an option on the uncertain asset price from (7). If φ is convex, such as a European call $\phi \left(x\right)={(x-\mathtt{s})}^{+}$ for some strike price $\mathtt{s}$, the price from (8) then simplifies to

$$\Psi \left(X\right)=\underset{\mathbb{Q}\in \mathcal{Q}}{max}{E}^{\mathbb{Q}}\left[X\right]=E^{{\mathbb{Q}}^{*}}\left[X\right]=E^{P_{\overline{\sigma }}}\left[\psi X\right],$$

where, as a special of Equation (9) under $P_{\overline{\sigma }}$,

$$\psi =exp\left(-\frac{1}{2}{\int }_0^T{\left(\theta \overline{\sigma }\right)}^2\mathrm{d}t-{\int }_0^T\theta \overline{\sigma }\mathrm{d}{B}_t\right).$$

Similarly, for concave φ, such as a European put, we get $\Psi \left(X\right)=E^{P_{\underline{\sigma }}}\left[\psi X\right]$ (see Avellaneda et al. (1995) for a detailed discussion of both cases). In particular, the pricing then coincides with the pricing in a Black–Scholes model.

Nonconvex payoff structures, such as Butterfly options3, yield a maximizing $\mathbb{Q}\in \mathcal{Q}$ with $\mathrm{d}{\mathbb{Q}}^{*}=\psi \mathrm{d}{\mathbb{P}}_{{\sigma }^{*}}$ such that the corresponding ${\sigma }^{*}$ is no longer a constant process. Thus, there is no σ for the Black–Scholes model that matches with the pricing under volatility uncertainty. In Fouque and Ren (2014), with $[\underline{\sigma },\overline{\sigma }]=[0.15,0.2]$, the price of a butterfly option under Ψ is computed through approximation techniques of the corresponding nonlinear heat PDE (see also Appendix A.2 for the relation between PDE’s and G-Brownian motion).

3. A Micro-Foundation of the FTAP under $\mathcal{P}$

Before introducing the economy, see part (c) in the Introduction, the structure of linear price systems on $L_{\mathcal{P}}$ is discussed and the notion of coherent price systems is introduced.

The states of the world, $\Omega$, build a complete separable metric space. $\mathcal{F}=\mathcal{B}(\Omega )$ is the Borel $\sigma$-algebra of $\Omega$. The uncertainty is described by a weakly compact (with respect to ${\mathcal{C}}_b(\Omega )$) subset of Borel probability measures $\mathcal{P}\subset \Delta (\Omega )$ on $(\Omega ,\mathcal{F})$. Assumption A1 in Section 2 is not imposed.

3.1. Linear Price Systems

To realize the problematic aspect of linear equilibrium price systems under ambiguous volatility, consider the dual space of continuous and linear functionals $\Pi :L_{\mathcal{P}}\to \mathbb{R}$ (see Theorem 2 of Beissner and Denis (2018) for a Riesz-type representation theorem). Non-trivial positive payoffs are costless on events outside the domain of the representing equilibrium pricing measure. The reason for this critical aspect relies on the mutual singularity of measures in $\mathcal{P}$. The topological dual space of $L_{\mathcal{P}}$ is denoted by $L_{\mathcal{P}}^{*}$ (see Appendix A.1), and is given by

$$\begin{array}{c}\hfill L_{\mathcal{P}}^{*}=\left\{\Pi :L_{\mathcal{P}}\to \mathbb{R}:\Pi (·)=E^{\mathbb{P}}[{\psi }_{\mathbb{P}}·]|\mathbb{P}\in \mathcal{P},{\psi }_{\mathbb{P}}\in L_{\mathbb{P}}\right\}.\end{array}$$

(10)

Similar to the case with $L_{\mathbb{P}}$ as the commodity space, an analogous interpretation of the dual space in Equation (10) holds true. The state price density ${\psi }_{\mathbb{P}}$ is supported by a prior $\mathbb{P}\in \mathcal{P}$, but the stronger norm ${\parallel x\parallel }_{\mathcal{P}}$, i.e., ${\parallel x\parallel }_{\left\{\mathbb{P}\right\}}\le {\parallel x\parallel }_{\mathcal{P}}$ for any $x\in L_{\mathcal{P}}$, causes a richer dual space that is parameterized by $\mathcal{P}$. In abuse of changing domains with respect to $\Pi$, this means $L_{\mathcal{P}}^{*}={\cup }_{\mathbb{P}\in \mathcal{P}}{L}_{\mathbb{P}}^{*}$. Each $\Pi \in L_{\mathcal{P}}^{*}$ assigns no value to strictly positive payoffs outside the support of the representing prior ${\mathbb{P}}_{\Pi }$.

Any linear expectation $E^{\mathbb{Q}}$ under an EMM $\mathbb{Q}$ can be considered as a normalized, linear, strictly positive and continuous price system. When seeking an FTAP under uncertain volatility that incorporates a notion of market viability, the following question serves to clarify the issue: Is the existence of a single risk neutral measure, $\mathbb{Q}$, equivalent to a certain prior in $\mathcal{P}$ sufficient for the absence of arbitrage?4 As such, the robust arbitrage notion from Definition 1 is inconsistent with a linear theory of valuation. In other words, one pricing measure $\mathbb{Q}$ is incapable of containing all information about what is possible under $\mathcal{P}$. In the same vein, the idea of “no empty promises” of Willard and Dybvig (1999) points to the unpleasant but possible ignorance of payoffs on zero probability events for only some prior. Section 4 continues with a discussion.

3.2. Coherent Price Systems

Under volatility uncertainty the above-mentioned problematic aspect of normalized linear price systems $\Pi (·)=E^{\mathbb{Q}}[·]$ and single EMM’s comes into play, which can be avoided by allowing sublinear prices on $L_{\mathcal{P}}$. For the extension of the price space, this author takes a cue from Aliprantis and Tourky (2002). For each $\mathbb{P}\in \mathcal{P}$, define the set $L_{\mathbb{P}}^{+,1}=\{\psi \in L_{\mathbb{P}}:\psi >0\mathbb{P}\text{-}a.s.an\mathrm{d}{E}^{\mathbb{P}}\left[\psi \right]=1\}$. Similar to Equation (10), the space of coherent price systems is generated by strictly positive and linear functionals and given by

$$\begin{array}{c}\hfill L_{+}^{⊛}=\left\{\Psi :L_{\mathcal{P}}\to \mathbb{R}:\Psi (·)=\underset{\mathbb{P}\in {\Gamma }_{\Psi }}{sup}{E}^{\mathbb{P}}[{\psi }_{\mathbb{P}}·]|{\psi }_{\mathbb{P}}\in L_{\mathbb{P}}^{+,1}\forall \mathbb{P}\in {\Gamma }_{\Psi }\subset \mathcal{P}\right\},\end{array}$$

(11)

where ${\left\{\mathbb{P}\right\}}_{\mathbb{P}\in {\Gamma }_{\Psi }}$ determines a $\Psi$. The set ${\Gamma }_{\Psi }\subset \mathcal{P}$ in Equation (11) then refers to exactly those priors appearing in the representation of $\Psi$ and is called $\Gamma$-relevant. Elements in $L_{+}^{⊛}$ rely on a set of normalized, strictly positive and linear functionals ${\left\{{\Pi }_{\mathbb{P}}\right\}}_{\mathbb{P}\in {\Gamma }_{\Psi }}$ with ${\Pi }_{\mathbb{P}}\in L_{\mathbb{P}}^{*}$, which are consolidated by combining the pointwise maximum and convex combinations of linear price systems. This leads to a consolidation operation

$$\begin{array}{c}\hfill \Gamma :\prod _{\mathbb{P}\in \mathcal{P}}{L}_{\mathbb{P}}^{+,1}\to L_{+}^{⊛},\left\{{\Pi }_{\mathbb{P}}\right\}\mapsto \Gamma \left(\left\{{\Pi }_{\mathbb{P}}\right\}\right)=\Psi \end{array}$$

(12)

to aggregate the linear and prior-dependent price systems $\left\{{\Pi }_{\mathbb{P}}\right\}$. For instance, a linear price system ${\Pi }_{\mathbb{P}}$ corresponds to a consolidation via the (second-order) point measure ${\delta }_{\left\{\mathbb{P}\right\}}\in \Delta \left(\mathcal{P}\right)$, such that only ${\Gamma }_{\mathcal{P}}=\left\{\mathbb{P}\right\}$ is relevant (see Equation (A1) in Appendix A for the general case and an example of how a sublinear functional in $L_{+}^{⊛}$ can be constructed).

Proposition 1.

Each $\Psi \in L_{+}^{⊛}$ satisfies, ∀$X,Y\in L_{\mathcal{P}}$, $c\in \mathbb{R}$ and $\lambda \ge 0$,

1.

Sublinearity $\Psi (\lambda X+Y)\le \lambda \Psi \left(X\right)+\Psi \left(Y\right)$.

2.

Constant preserving $\Psi \left(c\right)=c$.

3.

Strict positivity $X\ge 0$ and $X\ne 0$ on ${\Gamma }_{\Psi }$ implies $\Psi \left(X\right)>0$.

4.

Monotonicity If ${\Gamma }_{\Psi }$ is closed, $X\ge Y$ implies $\Psi \left(X\right)\ge \Psi \left(Y\right)$.

5.

Continuity $X_n\to X$ in ${\parallel ·\parallel }_{\mathcal{P}}$ implies ${lim}_n\Psi \left(X_n\right)=\Psi \left(X\right)$.

The phrase “$X\ne Y$ on ${\Gamma }_{\Psi }$” refers to the presence of a $\mathbb{P}\in {\Gamma }_{\Psi }$ such that $\mathbb{P}(X\ne Y)>0$. In view of the sublinearity of $\Psi$, Corollary 1 in Section 3.3.1 identifies the subspace of $L_{\mathcal{P}}$ where coherent price systems are linear.

3.3. Viable Price Systems

As in Harrison and Kreps (1979), a viable price system is based on a model of an economic equilibrium. To emphasize the key aspects under volatility uncertainty, recall the following.

3.3.1. Viability under Risk

The commodity space is again $L_{\mathbb{P}}$. The price functionals are linear and continuous, and the dual $L_{\mathbb{P}}^{*}$ can be identified with densities in $L_{\mathbb{P}}$.

Fix a linear price system $\pi :M\to \mathbb{R}$, where the marketed space $M\subset L_{\mathbb{P}}$ contains all frictionless achievable claims. ${\mathbb{A}}_{\mathbb{P}}$ is the set of convex, strictly monotone and continuous preference relations on $\mathbb{R}\times L_{\mathbb{P}}$. Viability of $\pi$ means that there is an $\succsim \in {\mathbb{A}}_{\mathbb{P}}$ and a feasible bundle $(\widehat{x},\widehat{X})\in B_{\pi }=\{(y,Y)\in \mathbb{R}\times M:y+\pi \left(Y\right)\le 0\}$ with $(\widehat{x},\widehat{X})\succsim (x,X)$ for all $(x,X)\in B_{\pi }$. Theorem 1 of Harrison and Kreps (1979) shows that π is viable if and only if there is an extension $\Pi \in L_{\mathbb{P}+}^{*}$ of π to $L_{\mathbb{P}}$.

3.3.2. Viability under Ambiguous Volatility

An FTAP with a sound microeconomic foundation contains a third statement (viability) about the existence of an agent (preferring more than less) and being in an optimal state. However, with regard to Remark 1, the notion of strict monotone complete preferences is subtle.5 Beginning with the introduction of marketed spaces $M_{\mathbb{P}}\subset L_{\mathbb{P}}$, $\mathbb{P}\in \mathcal{P}$, any claim in $M_{\mathbb{P}}$ can be achieved frictionless, if $\mathbb{P}$ is the true prior. The deviation from Kreps (1981) in which the market model is described by the quintuple $(L,\parallel ·\parallel ,L_{+}\setminus \left\{0\right\},M,\pi )$, relies on the uncertainty model $\mathcal{P}$ and the resulting effect of ambiguity about the true distribution of payoffs.

The following remark makes this conceptually crucial point precise.

Remark 4.

A marketed space $M\subset L_{\mathcal{P}}$ collects all perfectly replicable claims. Such claims are on a stock with simplified price dynamics $\mathrm{d}{S}_t=V_t\mathrm{d}{\mathtt{B}}_t$ and spans M, via terminal outcomes $X={\eta }_T{S}_T={\int }_0^T{\eta }_t\mathrm{d}{S}_t={\int }_0^T{\eta }_t{V}_t\mathrm{d}{\mathtt{B}}_t$, where η is a feasible and replicating trading strategy. The price of ${\eta }_T{S}_T$ is then the initial cost ${\eta }_0{S}_0$. With ambiguity in prices, e.g., ${\mathtt{B}}_t$ has ambiguous volatility $\sigma \in \mathcal{D}$ as in Equation (1), the uncertainty in S reappears in any claim on S:

$$\begin{array}{c}\hfill X={\int }_0^T{\eta }_t\mathrm{d}{S}_t^{\sigma }={\int }_0^T{\eta }_t{V}_t\mathrm{d}{B}_t^{\sigma }={\int }_0^T{\eta }_t{V}_t\mathrm{d}\left({\int }_0^t{\sigma }_s\mathrm{d}{B}_s\right)={\int }_0^T{\eta }_t{V}_t{\sigma }_t\mathrm{d}{B}_t.\end{array}$$

Here, all the equalities hold only ${\mathbb{P}}_{\sigma }$-a.s. The uncertain volatility σ results in an ambiguous payoff and leads to an ambiguous marketed space and an ambiguous price ${\eta }_0{S}_0^{\sigma }$. To incorporate this ambiguity about replicating portfolio processes, the marketed space $M_{{\mathbb{P}}_{\sigma }}$ and price ${\pi }_{{\mathbb{P}}_{\sigma }}$ now depend on the prior.

In view of Remark 4, ${\pi }_{\mathbb{P}}$ and ${\pi }_{{\mathbb{P}}^{\prime }}$ may have a rich common domain but also different evaluations, namely ${\pi }_{\mathbb{P}}\left(X\right)\ne {\pi }_{{\mathbb{P}}^{\prime }}\left(X\right)$ with $X\in M_{\mathbb{P}}\cap M_{{\mathbb{P}}^{\prime }}$. Coherence is based on sublinear price systems, as illustrated in the following example and discussed by Heath and Ku (2006).

Example 3.

Let $\mathcal{P}=\{\mathbb{P},{\mathbb{P}}^{\prime }\}$. If $\mathbb{P}$ is the true law, each claim in $M_{\mathbb{P}}$ is priced by a linear functional ${\pi }_{\mathbb{P}}$. An agent is unable to choose a portfolio in $M_{{\mathbb{P}}^{\prime }}+M_{\mathbb{P}}$ due to unawareness of the true prior. Equality of prices on the intersection is then less intuitive, since the different priors create different replication costs and consequently different price structures (see Remark 4.) A robust price for $X\in M_{{\mathbb{P}}^{\prime }}\cap M_{\mathbb{P}}$ is $max\{{\pi }_{{\mathbb{P}}^{\prime }}\left(X\right),{\pi }_{\mathbb{P}}\left(X\right)\}$.

The set ${\left({\pi }_{\mathbb{P}}\right)}_{\mathbb{P}\in \mathcal{P}}$ of linear scenario-based price functionals inherits all the information of the underlying financial market. In the single-prior setting under $\mathbb{P}$, the market is incomplete if and only if $M_{\mathbb{P}}\ne L_{\mathbb{P}}$. Let $M_{\mathbb{P}}\prod M_{{\mathbb{P}}^{\prime }}$ denote the Cartesian product of $M_{\mathbb{P}}$ and $M_{{\mathbb{P}}^{\prime }}$. As in Remark 4, the asset span depends on each resulting law ${\mathbb{P}}_{{\sigma }^1}$ and ${\mathbb{P}}_{{\sigma }^2}$ as constructed in Equation (1).

Definition 3.

Fix a set of linear prices ${\{{\pi }_{\mathbb{P}}:M_{\mathbb{P}}\to \mathbb{R}\}}_{\mathbb{P}\in \mathcal{P}}$, where $M_{\mathbb{P}}$ is a closed subspace of $L_{\mathbb{P}}$. A price system $[{\left({\pi }_{\mathbb{P}}\right)}_{\mathbb{P}\in \mathcal{P}};\Gamma ]$ consists of a consolidation operator Γ, see Equation (12), and the product of prices ${\pi }_{\prod \mathcal{P}}:={\prod }_{\mathbb{P}\in {\Gamma }_{\mathcal{P}}}{\pi }_{\mathbb{P}}$ indexed by Γ-relevant priors.

In the economy, there is a single consumption good, a numeraire. Bundles $(x,X)$ are elements in $\mathbb{R}\times L_{\mathbb{P}}$, the units at time 0 and T. ${\mathbb{A}}_{\mathbb{P}}$ denotes the set of rational, convex, strictly monotone, and $L_{\mathbb{P}}$-continuous preference relations ${\succsim }_{\mathbb{P}}$ on $\mathbb{R}\times L_{\mathbb{P}}$. For a price ${\pi }_{\mathbb{P}}:M_{\mathbb{P}}\to \mathbb{R}$, define the budget set by $B_{{\pi }_{\mathbb{P}}}=\{(y,Y)\in \mathbb{R}\times M_{\mathbb{P}}:y+{\pi }_{\mathbb{P}}\left(Y\right)\le 0\}$. An appropriate notion of viability defines a minimal consistency criterion, and can be regarded as an inverse no-trade equilibrium condition. Fix $\mathcal{R}\subset \mathcal{P}$.

Definition 4.

A price system is $\mathcal{R}$-viable if for each $\mathbb{P}\in \mathcal{R}$ there is a preference relation ${\succsim }_{\mathbb{P}}\in {\mathbb{A}}_{\mathbb{P}}$ and a bundle $(x_{\mathbb{P}},X_{\mathbb{P}})\in B_{{\pi }_{\mathbb{P}}}$ such that $(x_{\mathbb{P}},X_{\mathbb{P}})$ is ${\succsim }_{\mathbb{P}}$-maximal on $B_{{\pi }_{\mathbb{P}}}$.

The conditions are necessary and sufficient for a classical economic equilibrium in each relevant scenario in $\mathcal{R}$. Note that Definition 4 has to some extent the preference type of Bewley (2002). When $M_{\mathbb{P}}=M$ for every $\mathbb{P}$, scenario-based viability exactly captures the existence of an agent with Bewley preferences and a maximal consumption bundle $(x,X)$. The concept of scenario-based viability, as a model of an economic equilibrium can be related to Theorem 1.

Example 4.

Let $X\in L_{\mathcal{P}}$ be a contingent claim such that it is priced by $\mathcal{P}$-arbitrage. Then, in view of Section 2, the value at any time t is given by ${\Psi }_t\left(X\right)=\frac{1}{{\psi }_t}{\mathcal{E}}_t^{\mathcal{P}}\left({\psi }_{T}X\right)$, if Γ is assumed to be the maximum operation. In view of Equation (12), this assumption yields $\Gamma \left({\left\{{\Pi }_{\mathbb{P}}\right\}}_{\mathbb{P}\in \mathcal{P}}\right)=\Psi (·)={max}_{\mathbb{P}\in \mathcal{P}}{E}^{\mathbb{P}}[\psi ·]$, where $\psi \in L_{\mathcal{P}}$ is considered as an aggregated collection ${\left({\psi }_{\mathbb{P}}\right)}_{\mathbb{P}\in \mathcal{P}}$. In particular, we can take the deflator $\psi ={\psi }_T^{\theta }$ from Equation (9).

The next result connects viability with price systems in $L_{+}^{⊛}$. For the proof, we redefine the shifted preference relations ${\succsim }_{\mathbb{P}}$ such that every feasible net trade is worse off than $(0,0)\in B_{{\pi }_{\mathbb{P}}}$. Obviously, an agent given by ${\succsim }_{\mathbb{P}}$ does not trade.

Theorem 2.

A price system is $\mathcal{R}$-viable if and only if there is a $\Psi \in L_{+}^{⊛}$ such that ${\pi }_{\mathbb{P}}\le \Psi$ on $L_{\mathcal{P}}\cap M_{\mathbb{P}}$ for each $\mathbb{P}\in {\Gamma }_{\Psi }$ with ${\Gamma }_{\Psi }=\mathcal{R}$.

Apart from the given scenario-based marketed spaces $\left(M_{\mathbb{P}}\right)$, there is an intrinsic subspace of mean-ambiguity free claims in which the price system $\Psi$ acts in a linear manner. In Section 2, the related symmetry property corresponds to a refined martingale notion, which is induced by EsMM-sets.

Corollary 1.

Every $\Psi \in L_{+}^{⊛}$ is linear on the Ψ-marketed space

$$\begin{array}{c}\hfill {\mathbb{M}}_{\Psi }=\left\{X\in L_{\mathcal{P}}:E^{\mathbb{P}}\left[{\psi }_{\mathbb{P}}X\right]\mathrm{is}\mathrm{constant}\mathrm{in}\mathbb{P}\in {\Gamma }_{\Psi }\right\}.\end{array}$$

(13)

For claims in Equation (13), the law of one price holds. In the finite state case, Beissner and Riedel (2019) considered a stronger notion of viability and introduced a general equilibrium concept with coherent price systems $\Psi$. In that case, the state price $\psi ={\psi }_{\mathbb{P}}$ is then the equilibrium outcome and determines the endogenous structure of the marketed space ${\mathbb{M}}_{\Psi }$. Under ambiguous volatility and ${\Gamma }_{\Psi }=\mathcal{P}$, the space ${\mathbb{M}}_{\Psi }$ also determines the implementability of Arrow–Debreu equilibria (with linear prices) via dynamic trading strategies. For a characterization through the net trades of an Arrow–Debreu equilibrium, see the work of Beissner and Riedel (2018).

3.4. Viability, Symmetric Martingales, and the FTAP

Finally, this subsection returns to the setting and results of Section 2. The following theorem justifies the discussion of the connection between viability and symmetric martingales. To guarantee a sublinear conditional expectation ${\mathcal{E}}_t$ under $\mathcal{P}$ that satisfies the dynamic programming principle of Equation (5), Assumption A1 is again imposed. The G-expectation framework of Theorem 1 satisfies Assumption A1 automatically.

The next result connects Theorems 1 and 2.

Theorem 3.

Suppose that the financial market model $\mathtt{FM}(1,S)$ is $\mathcal{P}$-arbitrage free. There is a bijection between viable prices $\Psi \in L_{+}^{⊛}$ and EsMM-sets, such that ${\Gamma }_{\Psi }$ is stable under pasting. $\Psi ={\mathcal{E}}^{\mathcal{Q}}$ holds and the EsMM-set is

$$\begin{array}{c}\hfill \mathcal{Q}=\left\{\mathbb{Q}\in \Delta (\Omega ):\mathrm{d}\mathbb{Q}={\psi }_{\mathbb{P}}\mathrm{d}\mathbb{P},\mathbb{P}\in {\Gamma }_{\mathcal{P}}\mathit{and}{\psi }_{\mathbb{P}}\in L_{\mathbb{P}+}\right\}.\end{array}$$

(14)

Recall the corresponding consolidation $\Gamma$, defined in Equation (12), satisfies ${\Gamma }_{\Psi }=\mathcal{R}\subset \mathcal{P}$. Theorem 3 then establishes a one-to-one mapping between $L_{+}^{⊛}$ and $\mathtt{EsMM}\left(\mathcal{P}\right)$. Analogous to the single-prior setting, this view yields some further insights by combining Theorems 2 and 3. We say that a financial market $\mathtt{FM}(1,S^1)$ is viable if it is ${\Gamma }_{\mathcal{P}}$-arbitrage free, and the associated price system ${\pi }_{\prod \mathcal{P}}$ is viable for a given consolidation operator, as stated in Equation (12).

Corollary 2.

Fix an $\mathcal{R}={\Gamma }_{\Psi }\subset \mathcal{P}$ that is stable under pasting.

1.

$\mathtt{FM}(1,S^1)$ is $\mathcal{R}$-viable if and only if there is an EsMM-set in $\mathtt{EsMM}\left(\mathcal{R}\right)$.

2.

If $\mathtt{EsMM}\left(\mathcal{R}\right)$ is non-empty, then no $\mathcal{R}$-arbitrage exists.

Under the uncertainty neutral expectation ${\mathcal{E}}^{\mathcal{Q}}$, expected returns of the risky and ambiguous asset $S^1$ equals that of the riskless asset $S^0$ and contains no ambiguity.

4. Conclusions

This paper presents a theory of derivative security pricing into which volatility uncertainty is incorporated. The classical notion of equivalent martingale measures changes, and the valuation by means of expectations becomes nonlinear. The results of this paper establish a version of the FTAP under volatility uncertainty.

The present uncertainty model is closely related to that of Epstein and Ji (2013), while the present valuation principle follows the preference-free approach by Ross (1976). The price of a claim is the expected value in an uncertainty-neutral world. Expectations for the security price no longer merely depend on one “risk-neutral” prior; the principle of a risk-neutral valuation is insufficient, as different mutually singular priors deliver completely different linear risk-neutral expectations. The shortcoming of linear prices is rearranged. A single prior, as the output of a linear-price equilibrium, can create an invisible threat of convention and may lead to an illusion of security when faced with a risky and ambiguous future. Within any neoclassical infinite states model, this stems from the dual role of an objective and primitive probability measure $\mathbb{P}$. On the one hand, it quantifies uncertainty. On the other hand, this $\mathbb{P}$ reappears in the representation of any linear equilibrium price system and any EMM.

Under the present type of volatility uncertainty, the focus on a single prior creates a hazard. Payoff-relevant events with a positive probability may be costless under a supporting prior of a linear price. For instance, this can result from the first-order condition in a consumption-based asset pricing model.