High Sensitivity Hydrogen Sensor via the Coupling of Tamm Plasmon Polaritons and Defect Mode
Abstract
1. Introduction
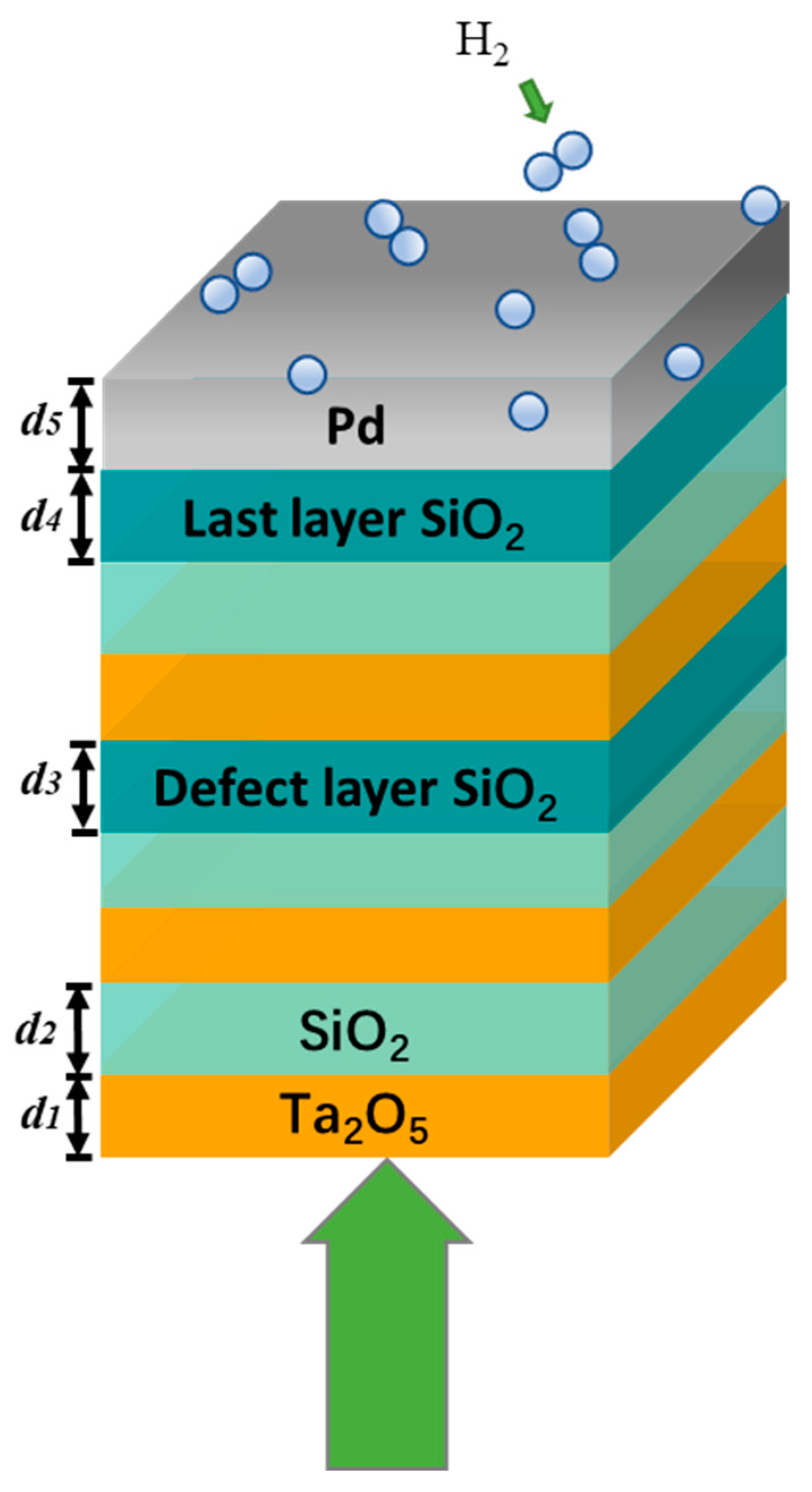
2. Theoretical Model
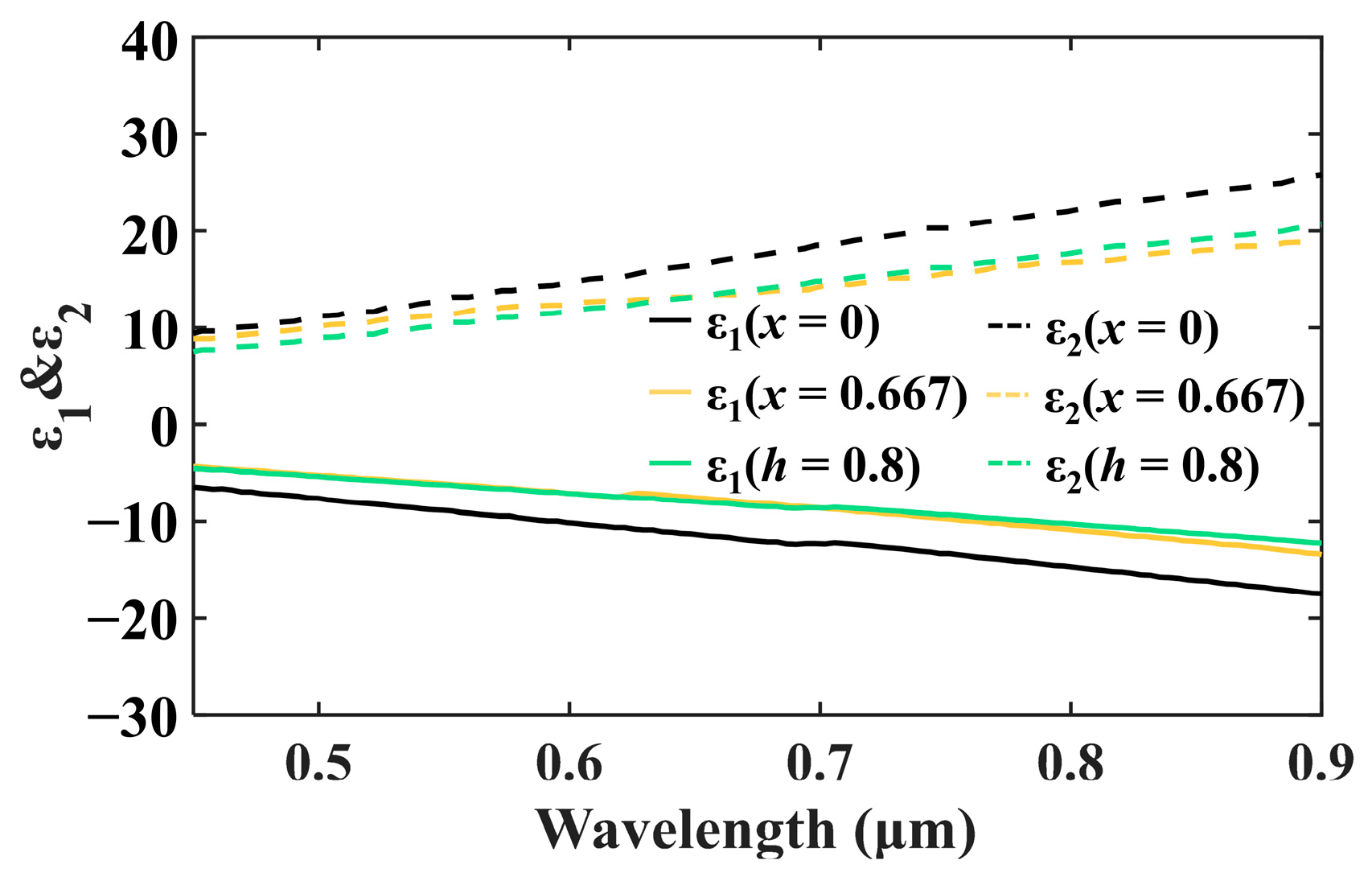
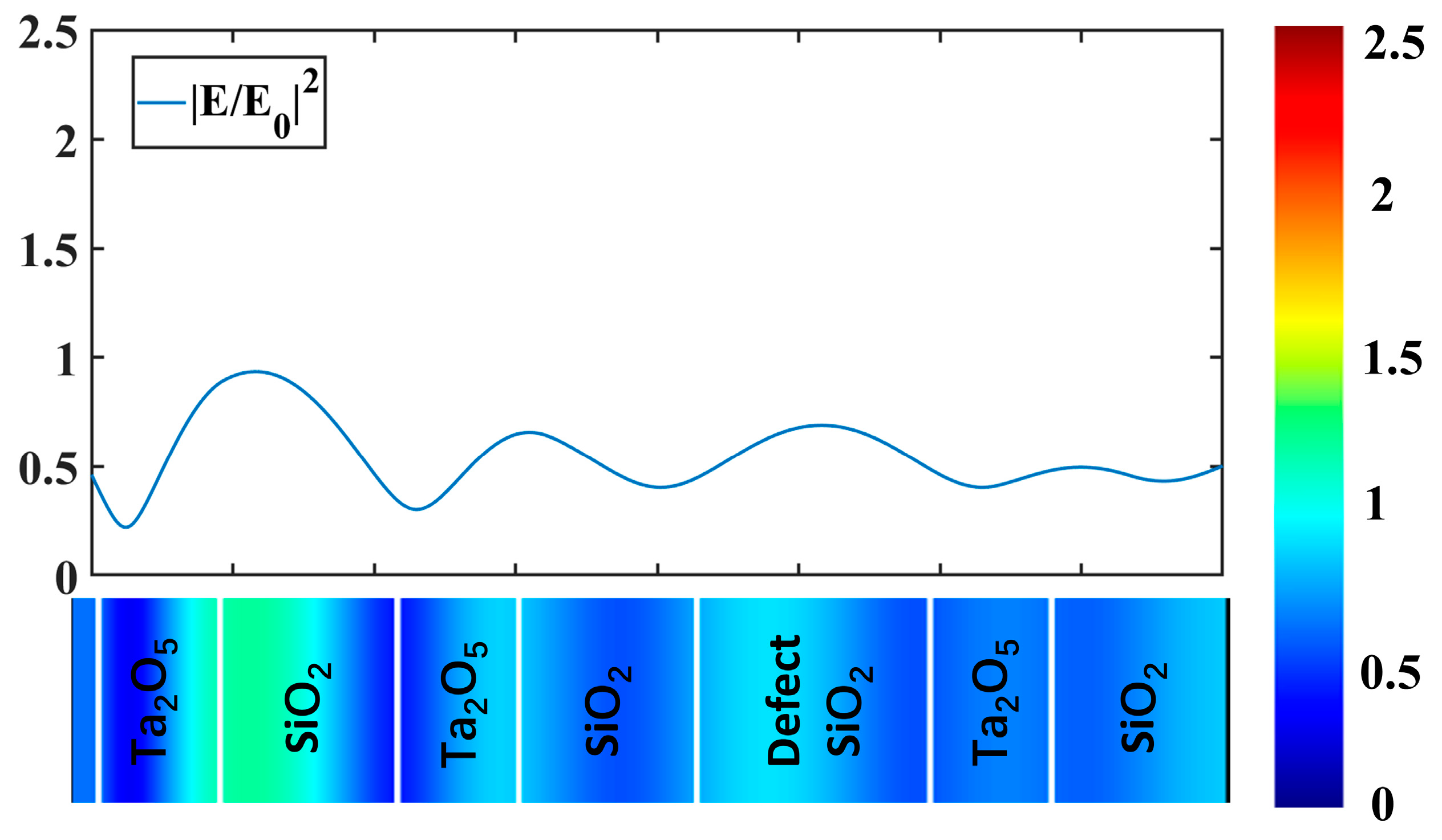
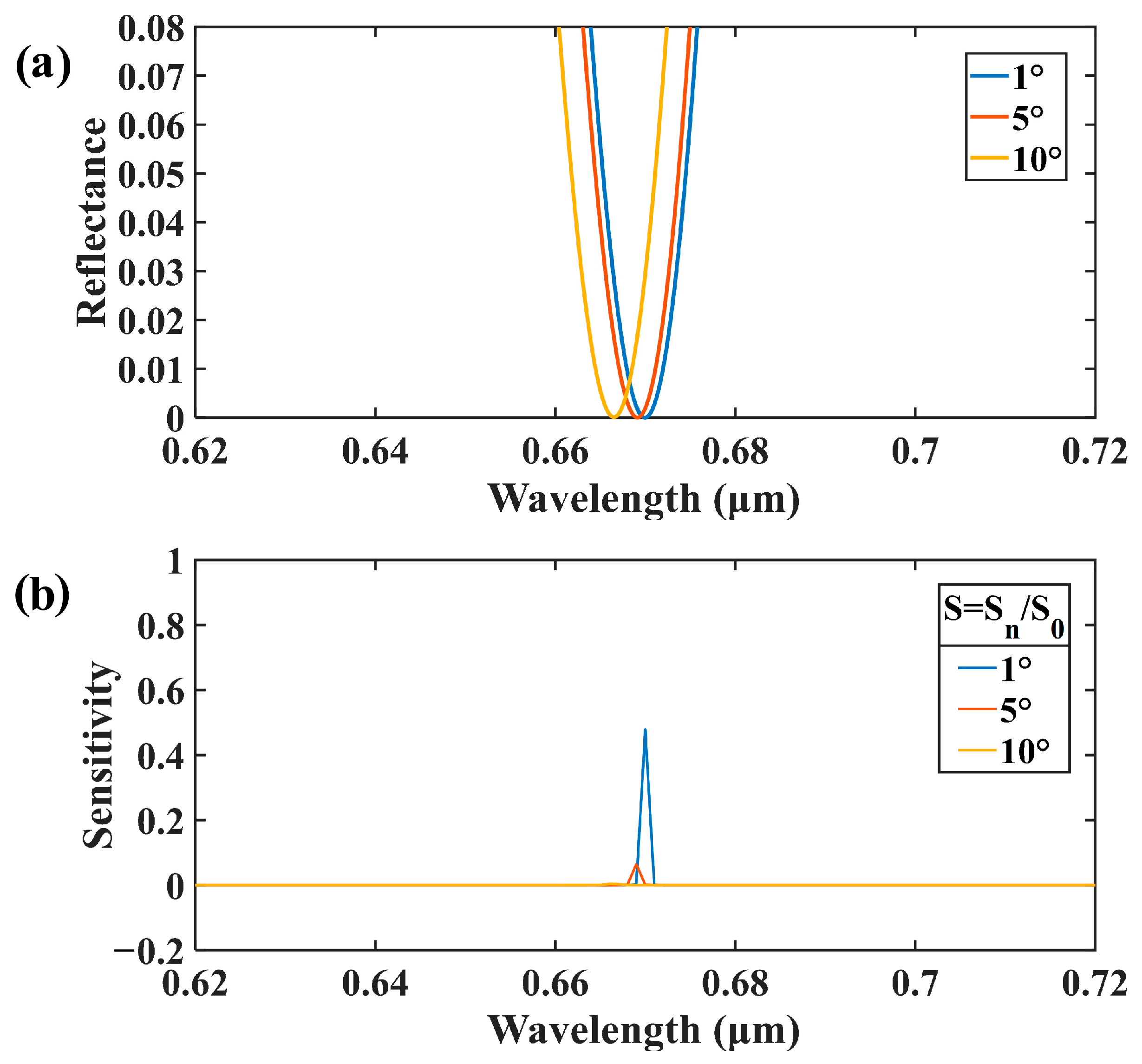
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Dawood, F.; Anda, M.; Shafiullah, G.M. Hydrogen production for energy: An overview. Int. J. Hydrogen Energy 2020, 45, 847–3869. [Google Scholar] [CrossRef]
- Dincer, I. Green methods for hydrogen production. Int. J. Hydrogen Energy 2012, 37, 1954–1971. [Google Scholar] [CrossRef]
- Sakintuna, B.; Lamari-Darkrim, F.; Hirscher, M. Metal hydride materials for solid hydrogen storage: A review. Int. J. Hydrogen Energy 2007, 32, 1121–1140. [Google Scholar] [CrossRef]
- Turner, J.A. Sustainable hydrogen production. Science 2004, 305, 972–974. [Google Scholar] [CrossRef] [PubMed]
- Ball, M.; Wietschel, M. The future of hydrogen-opportunities and challenges. Int. J. Hydrogen Energy 2009, 34, 615–627. [Google Scholar] [CrossRef]
- Hübert, T.; Boon-Brett, L.; Black, G.; Banach, U. Hydrogen sensors—A review. Sens. Actuators B 2011, 157, 329–335. [Google Scholar] [CrossRef]
- Ai, B.; Sun, Y.J.; Zhao, Y.P. Plasmonic hydrogen sensors. Nano-Micro Small 2022, 18, 2107882. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.N.; Peng, H.J.; Qian, X.L.; Zhang, Y.Y.; An, G.W.; Zhao, Y. Recent advancements in optical fiber hydrogen sensors. Sens. Actuators B 2017, 244, 393–416. [Google Scholar] [CrossRef]
- Chen, K.F.; Yuan, D.P.; Zhao, Y.Y. Review of optical hydrogen sensors based on metal hydrides: Recent developments and challenges. Opt. Laser Technol. 2021, 137, 106808. [Google Scholar] [CrossRef]
- Tittl, A.; Mai, P.; Taubert, R.; Dregely, D.; Liu, N.; Giessen, H. Palladium-based plasmonic perfect absorber in the visible wavelength range and its application to hydrogen sensing. Nano Lett. 2011, 11, 4366–4369. [Google Scholar] [CrossRef]
- Xu, F.; Zhang, Z.; Ma, J.; Ma, C.; Guan, B.O.; Chen, K. Large-area ordered Palladium nanostructures by colloidal lithography for hydrogen sensing. Molecules 2022, 27, 6100. [Google Scholar] [CrossRef]
- Luong, H.M.; Pham, M.T.; Guin, T.; Richa, P.M.; Manh, H.P.; George, K.L.; Tho, D.N. Sub-second and ppm-level optical sensing of hydrogen using templated control of nano-hydride geometry and composition. Nat. Commun. 2021, 12, 2414. [Google Scholar] [CrossRef] [PubMed]
- Yang, A.; Huntington, M.D.; Cardinal, M.F.; Masango, S.S.; Van Duyne, R.P.; Odom, T.W. Hetero-oligomer nanoparticle arrays for plasmon-enhanced hydrogen sensing. ACS Nano 2014, 8, 7639–7647. [Google Scholar] [CrossRef] [PubMed]
- Timur, S.; Peter, J.; Christoph, L.; Mikael, K. Directional scattering and hydrogen sensing by bimetallic Pd-Au nanoantennas. Nano Lett. 2012, 12, 2464–2469. [Google Scholar] [CrossRef]
- Wadell, C.; Antosiewicz, T.J.; Langhammer, C. Optical absorption engineering in stacked plasmonic AuSiO2-Pd nanoantennas. Nano Lett. 2012, 12, 4784–4790. [Google Scholar] [CrossRef]
- Silkin, V.M.; Díez Muiño, R.; Chernov, I.P.; Chulkov, E.V.; Echenique, P.M. Tuning the plasmon energy of palladium hydrogen systems by varying the hydrogen concentration. J. Phys. Condens. Matter 2012, 24, 104021. [Google Scholar] [CrossRef] [PubMed]
- Baldi, A.; Narayan, T.C.; Koh, A.L.; Dionne, J.A. In situ detection of hydrogen-induced phase transitions in individual palladium nanocrystals. Nat. Mater. 2014, 13, 1143–1148. [Google Scholar] [CrossRef] [PubMed]
- Strohfeldt, N.; Zhao, J.; Tittl, A.; Giessen, H. Sensitivity engineering in direct contact palladium-gold nano-sandwich hydrogen sensors. Opt. Mater. Express 2015, 5, 2525–2535. [Google Scholar] [CrossRef]
- Poyli, M.A.; Silkin, V.M.; Chernov, I.P.; Echenique, P.M.; Muiño, R.D.; Aizpurua, J. Multiscale theoretical modeling of plasmonic sensing of hydrogen uptake in palladium nanodisks. J. Phys. Chem. Lett. 2012, 3, 2556–2561. [Google Scholar] [CrossRef]
- Sasin, M.E.; Seisyan, R.P.; Kalitteevski, M.A.; Brand, S.; Abram, R.A.; Chamberlain, J.M.; Egorov, A.Y.; Vasil’ev, A.P.; Mikhrin, V.S.; Kavokin, A.V. Tamm plasmon polaritons: Slow and spatially compact light. Appl. Phys. Lett. 2008, 92, 251112. [Google Scholar] [CrossRef]
- Sasin, M.E.; Seisyan, R.P.; Kalitteevski, M.A.; Brand, S.; Abram, R.A.; Chamberlain, J.M.; Egorov, A.Y.; Vasil’ev, A.P.; Mikhrin, V.S.; Kavokin, A.V. RETRACTED: Tamm plasmon-polaritons: First experimental observation. Micro Nanostruct. 2010, 47, 44–49. [Google Scholar] [CrossRef]
- Xu, H.; Wu, P.; Zhu, C.; Elbaz, A.; Zhong, Z.G. Photonic crystal for gas sensing. J. Mater. Chem. C 2013, 1, 6087–6098. [Google Scholar] [CrossRef]
- Zaky, Z.A.; Aly, A.H. Theoretical study of a tunable low-temperature photonic crystal sensor using dielectric superconductor nanocomposite layers. J. Supercond. Nov. Magn. 2020, 33, 2983–2990. [Google Scholar] [CrossRef]
- Zaky, Z.A.; Aly, A.H. Gyroidal graphene/porous silicon array for exciting optical Tamm state as optical sensor. Sci. Rep. 2021, 11, 19389. [Google Scholar] [CrossRef] [PubMed]
- Ye, M.Y.Y.; Xie, Z.; Tang, J.; Ouyang, J.X. Highly sensitive and tunable terahertz biosensor based on optical Tamm states in graphene-based Bragg reflector. Results Phys. 2019, 15, 102779. [Google Scholar] [CrossRef]
- Zaky, Z.A.; Sharma, A.; Alamri, S.; Aly, A.H. Theoretical evaluation of the refractive index sensing capability using the coupling of Tamm-Fano resonance in one-dimensional photonic crystals. Appl. Nanosci. 2021, 11, 2261–2270. [Google Scholar] [CrossRef]
- Wu, F.; Liu, T.; Xiao, S. Polarization-sensitive photonic bandgaps in hybrid one-dimensional photonic crystals composed of all-dielectric elliptical metamaterials and isotropic dielectrics. Appl. Opt. 2023, 62, 706–713. [Google Scholar] [CrossRef] [PubMed]
- Mbakop, F.K.; Djongyang, N.; Ejuh, G.W.; Raïdandi, D.; Woafo, P. Transmission of light through an optical filter of a one-dimensional photonic crystal: Application to the solar thermophotovoltaic system. Phys. B 2017, 516, 92–99. [Google Scholar] [CrossRef]
- Panda, A.; Devi, P.P. Photonic crystal biosensor for refractive index based cancerous cell detection. Opt. Fiber Technol. 2020, 54, 102123. [Google Scholar] [CrossRef]
- Qiao, F.; Zhang, C.; Wan, J.; Zi, J. Photonic quantum-well structures: Multiple channeled filtering phenomena. Appl. Phys. Lett. 2020, 77, 3698. [Google Scholar] [CrossRef]
- Nimtz, G.; Haibel, A.; Vetter, R.M. Pulse reflection by photonic barriers. Phys. Rev. E 2002, 66, 037602. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.S.; Yang, Z.Y.; Ye, M.J.; Wu, W.H.; Chen, L.H.; Shen, H.J.; Ishii, S.; Nagao, T.; Chen, K.P. Tamm plasmon polaritons hydrogen sensors. Adv. Phys. Res. 2023, 2, 2200094. [Google Scholar] [CrossRef]
- Gao, L.; Lemarchand, F.; Lequime, M. Refractive index determination of SiO2 layer in the UV/Vis/NIR range: Spectrophotomtric reverse engineering on single and bi-layer designs. J. Eur. Opt. Soc-Rapid 2013, 8, 13010. [Google Scholar] [CrossRef]
- Rodríguez-de Marcos, L.V.; Larruquert, J.I.; Méndez, J.A.; Aznárez, J.A. Self-consistent optical constants of SiO2 and Ta2O5 films. Opt. Mater. Express 2016, 6, 3622–3637. [Google Scholar] [CrossRef]
- Deng, C.Z.; Ho, Y.L.; Lee, Y.C.; Wang, Z.Y.; Tai, Y.H.; Zyskowski, M.; Daiguji, H.; Delaunay, J.J. Two-pair multilayer Bloch surface wave platform in the near- and mid-infrared regions. Appl. Phys. Lett. 2019, 115, 091102. [Google Scholar] [CrossRef]
- Timofeev, I.V.; Maksimov, D.N.; Sadreev, A.F. Optical defect mode with tunable Q factor in a one-dimensional anisotropic photonic crystal. Phys. Rev. B 2018, 97, 024306. [Google Scholar] [CrossRef]
- Wu, J.Z.; Li, H.J.; Fu, C.J.; Wu, X.H. High quality factor nonreciprocal thermal radiation in a Weyl semimetal film via the strong coupling between Tamm plasmon and defect mode. Int. J. Therm. Sci. 2023, 184, 107902. [Google Scholar] [CrossRef]
- Zaky, Z.A.; Hanafy, H.; Panda, A.; Pukhrambam, P.D.; Aly, A.H. Design and analysis of gassensor using tailorable Fano resonance by coupling between Tamm and defected mode resonance. Plasmonics 2022, 17, 2103–2111. [Google Scholar] [CrossRef]
- Rakić, A.D.; Djurišic, A.B.; Elazar, J.M.; Majewski, M.L. Optical properties of metallic films for vertical-cavity optoelectronic devices. Appl. Opt. 1998, 37, 5271–5283. [Google Scholar] [CrossRef]
- von Rottkay, K.; Rubin, M. Refractive index changes of Pd-coated magnesium lanthanide switchable mirrors upon hydrogen insertion. J. Appl. Phys. 1999, 85, 408–413. [Google Scholar] [CrossRef]
- Aspnes, D.E. Plasmonics and effective-medium theories. Thin Solid Film. 2011, 519, 2571–2574. [Google Scholar] [CrossRef]
- Ahn, J.S.; Kim, K.H.; Noh, T.W.; Riu, D.H.; Boo, K.H.; Kim, H.E. Effective-medium theories for spheroidal particles randomly oriented on a plane: Application to the optical properties of a SiC whisker-Al2O3 composite. Phys. Rev. B 1995, 52, 15244–15252. [Google Scholar] [CrossRef]
- Garnett, J.C.M. Colours in metal glasses and in metallic films. Philos. Trans. R. Soc. A 1904, 203, 385–420. [Google Scholar] [CrossRef]
- Wu, X.H.; Fu, C.J.; Zhang, Z.M. Influence of hBN orientation on the near-field radiative heat transfer between graphene/hBN heterostructures. J. Photonics Energy 2018, 9, 032702. [Google Scholar] [CrossRef]
- Wu, J.; Wang, Z.M.; Wu, B.Y.; Shi, Z.X.; Wu, X.H. The giant enhancement of nonreciprocal radiation in Thue-morse aperiodic structures. Opt. Laser Technol. 2022, 152, 108138. [Google Scholar] [CrossRef]
- Kaliteevski, M.; Iorsh, I.; Brand, S.; Abram, R.A.; Chamberlain, J.M.; Kavokin, A.V.; Shelykh, I.A. Tamm plasmon-polaritons: Possible electromagnetic states at the interface of a metal and a dielectric Bragg mirror. Phys. Rev. B 2007, 76, 165415. [Google Scholar] [CrossRef]
- Brückner, R.; Sudzius, M.; Hintschich, S.I.; Frob, H.; Lyssenko, V.G.; Leo, K. Hybrid optical Tamm states in a planar dielectric microcavity. Phys. Rev. B 2011, 3, 033405. [Google Scholar] [CrossRef]
- Wu, X.H.; Chen, Z.X.; Wu, F. Strong nonreciprocal radiation in a InAs film by critical coupling with a dielectric grating. ES Energy Environ. 2021, 13, 8–12. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, F.; Yin, W.; Zhang, J. High Sensitivity Hydrogen Sensor via the Coupling of Tamm Plasmon Polaritons and Defect Mode. Chemosensors 2024, 12, 67. https://doi.org/10.3390/chemosensors12040067
Zhang F, Yin W, Zhang J. High Sensitivity Hydrogen Sensor via the Coupling of Tamm Plasmon Polaritons and Defect Mode. Chemosensors. 2024; 12(4):67. https://doi.org/10.3390/chemosensors12040067
Chicago/Turabian StyleZhang, Feng, Weifeng Yin, and Jianxia Zhang. 2024. "High Sensitivity Hydrogen Sensor via the Coupling of Tamm Plasmon Polaritons and Defect Mode" Chemosensors 12, no. 4: 67. https://doi.org/10.3390/chemosensors12040067
APA StyleZhang, F., Yin, W., & Zhang, J. (2024). High Sensitivity Hydrogen Sensor via the Coupling of Tamm Plasmon Polaritons and Defect Mode. Chemosensors, 12(4), 67. https://doi.org/10.3390/chemosensors12040067




