A Metafrontier Analysis on the Performance of Grain-Producing Regions in Norway
Abstract
1. Introduction
2. Theoretical Model
3. Empirical Model
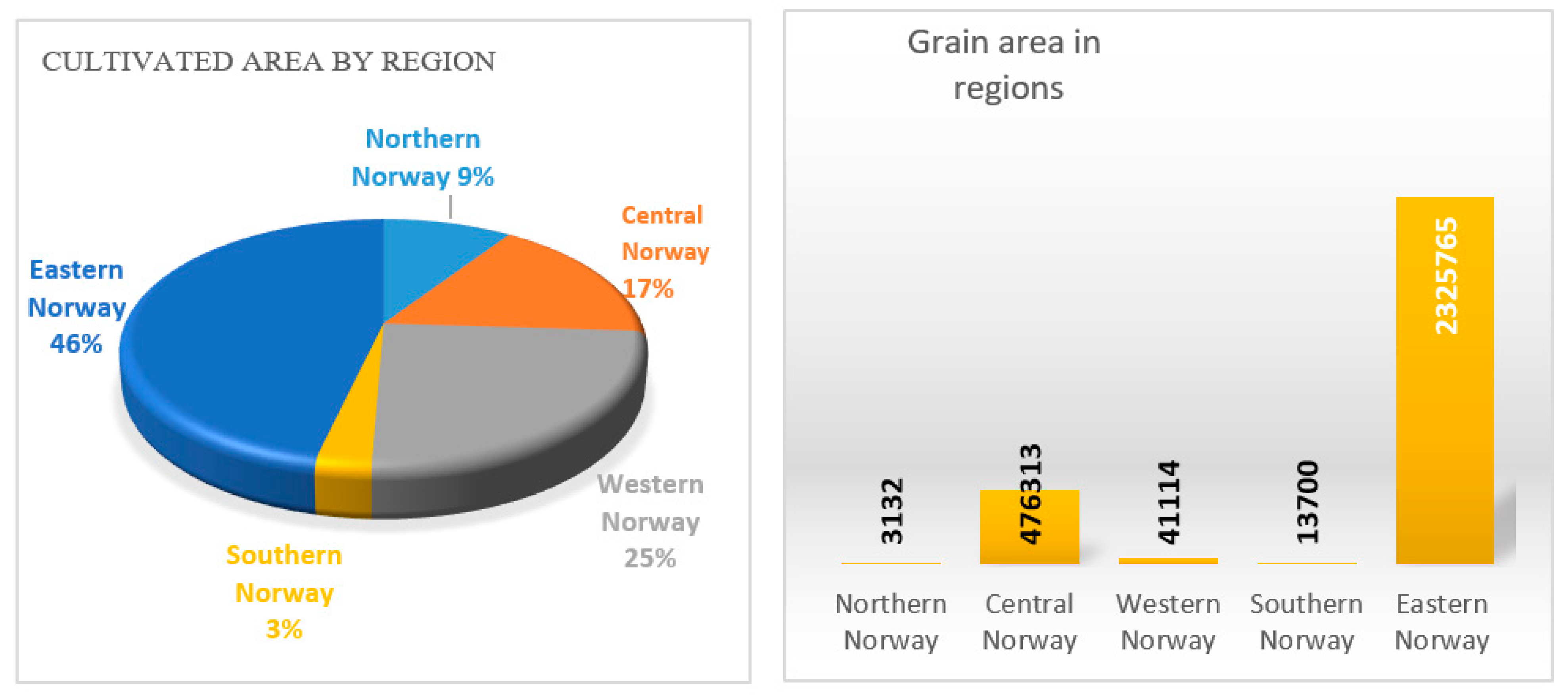
4. Data
5. Estimation Results and Discussion
5.1. Input Elasticity and Technological Change
5.2. Technical Efficiency and Technology Gap Ratio (TGR)
6. Conclusions and Policy Implications
Funding
Acknowledgments
Conflicts of Interest
References
- Alem, Habtamu. 2018. Effects of model specification, short-run, and long-run inefficiency: An empirical analysis of stochastic frontier models. Agricultural Economics 64: 508–16. [Google Scholar] [CrossRef]
- Alem, Habtamu, Gudbrand Lien, J. Brian Hardaker, and Atle Guttormsen. 2019. Regional differences in technical efficiency and technological gap of Norwegian dairy farms: A stochastic meta-frontier model. Applied Economics 51: 409–21. [Google Scholar] [CrossRef]
- Álvarez, Antonio, J. Corral, D. Solís, and A. Pérez. 2008. Does intensification improve the economic efficiency of dairy farms? Journal of Dairy Science 91: 3693–98. [Google Scholar] [CrossRef] [PubMed]
- Baráth, Lajos, and Imre Fertő. 2015. Heterogeneous technology, the scale of land use and technical efficiency: The case of Hungarian crop farms. Land Use Policy 42: 141–50. [Google Scholar] [CrossRef]
- Barnes, A., Dominic Moran, and K. Topp. 2009. The scope for regulatory incentives to encourage increased efficiency of input use by farmers. Journal of Environmental Management 90: 808–14. [Google Scholar] [CrossRef]
- Battese, George E., Prasada Rao, and Christopher J. O’Donnell. 2004. A metafrontier production function for estimation of technical efficiencies and technology gaps for firms operating under different technologies. Journal of Productivity Analysis 21: 91–103. [Google Scholar] [CrossRef]
- Berndt, Ernst R., and Laurits R. Christensen. 1973. The translog function and the substitution of equipment, structures, and labor in US manufacturing 1929–1968. Journal of Econometrics 1: 81–113. [Google Scholar] [CrossRef]
- Boshrabadi, Hossein Mehrabi, Renato Villano, and Euan Fleming. 2008. Technical efficiency and environmental-technological gaps in wheat production in Kerman province of Iran. Agricultural Economics 38: 67–76. [Google Scholar] [CrossRef]
- Coelli, Timothy J., Dodla Sai Prasada Rao, Christopher J. O’Donnell, and George Edward Battese. 2005. An Introduction to Efficiency and Productivity Analysis. New York: Springer. [Google Scholar]
- Greene, William. 2005a. Reconsidering heterogeneity in panel data estimators of the stochastic frontier model. Journal of Econometrics 126: 269–303. [Google Scholar] [CrossRef]
- Greene, William. 2005b. Fixed and Random Effects in Stochastic Frontier Models. Journal of Productivity Analysis 23: 7–32. [Google Scholar] [CrossRef]
- Huang, Zhimin, and Susan X. Li. 2001. Stochastic DEA models with different types of input-output disturbances. Journal of Productivity Analysis 15: 95–113. [Google Scholar] [CrossRef]
- Jondrow, James, C. A. Knox Lovell, Ivan S. Materov, and Peter Schmidt. 1982. On the estimation of technical inefficiency in the stochastic frontier production function model. Journal of Econometrics 19: 233–38. [Google Scholar] [CrossRef]
- Koop, Gary, and Mark F. Steel. 2001. Bayesian analysis of stochastic frontier models. A Companion to Theoretical Econometrics 1: 520–73. [Google Scholar]
- Kumbhakar, Subal C., Gudbrand Lien, and J. Brian Hardaker. 2012. Technical efficiency in competing panel data models: A study of Norwegian grain farming. Journal of Productivity Analysis 41: 321–37. [Google Scholar] [CrossRef]
- Kumbhakar, Subal C., Hongren Wang, and Alan P. Horncastle. 2015. A Practitioner’s Guide to Stochastic Frontier Analysis Using Stata. New York: Cambridge University Press. [Google Scholar]
- Lien, Gudbrand, Subal C. Kumbhakar, and J. Brian Hardaker. 2010. Determinants of off-farm work and its effects on farm performance: The case of Norwegian grain farmers. Agricultural Economics 41: 577–86. [Google Scholar] [CrossRef]
- Løyland, Knut, and Vidar Ringstad. 2001. Gains and structural effects of exploiting scale-economies in Norwegian dairy production. Agricultural Economics 24: 149–66. [Google Scholar] [CrossRef]
- Moreira, Víctor H., and Boris E. Bravo-Ureta. 2010. Technical efficiency and metatechnology ratios for dairy farms in three southern cone countries: A stochastic meta-frontier model. Journal of Productivity Analysis 33: 33–45. [Google Scholar] [CrossRef]
- O’Donnell, Christopher J., D. S. Prasada Rao, and George E. Battese. 2008. Metafrontier frameworks for the study of firm-level efficiencies and technology ratios. Empirical Economics 34: 231–55. [Google Scholar] [CrossRef]
- Odeck, James. 2007. Measuring technical efficiency and productivity growth: A comparison of SFA and DEA on Norwegian grain production data. Applied Economics 39: 2617–30. [Google Scholar] [CrossRef]
- Orea, Luis, and Subal C. Kumbhakar. 2004. Efficiency measurement using a latent class stochastic frontier model. Empirical Economics 29: 169–83. [Google Scholar] [CrossRef]
- Simar, Leopold, and Paul W. Wilson. 2007. Estimation and inference in two-stage, semi-parametric models of production processes. Journal of Econometrics 136: 31–64. [Google Scholar] [CrossRef]
- Statistics Norway. 2016. Agriculture Statistics. Available online: https://www.ssb.no/jord-skog-jakt-og-fiskeri?de=Landbrukstellinger+ (accessed on 9 September 2016).
- Statistics Norway. 2020. Agriculture Statistics. Available online: https://www.ssb.no/jord-skog-jakt-og-fiskeri?de=Landbrukstellinger+ (accessed on 20 May 2020).
- Steinshamn, H., L. Nesheim, and A. K. Bakken. 2016. Grassland production in Norway. Proceedings of the 26th General Meeting of the European Grassland Federation, The Multiple Roles of Grassland in the European Bioeconomy, Trondheim, Norway, September 4–8. [Google Scholar]
- Wang, Hung-Jen, and Chia-Wen Ho. 2010. Estimating fixed-effect panel stochastic frontier models by model transformation. Journal of Econometrics 157: 286–96. [Google Scholar] [CrossRef]
| 1 | There are other approaches, for instance, the Bayesian stochastic frontier (Koop and Steel 2001), semi-parametric (Simar and Wilson 2007), and stochastic DEA (Huang and Li 2001), though these re not commonly used in empirical studies. |
| 2 | Nordic countries include Norway, Sweden, Denmark, Iceland, and Finland. |
| 3 | 3 The data are registered in decare. A decare (daa) is equal to 0.1 hectares (ha). |
| Region | Output (In €2014) | Land (×1) (0.1 Hectare) | Labor (×2) (In Hours) | Var. Cost (×3) (In €2014) | Fixed Cost (×4) (In €2014) | N |
|---|---|---|---|---|---|---|
| Eastern Norway Std. Dev. | 33,527.50 (23,694) | 342 (212) | 991 (634) | 9391.00 (6403) | 15,917.40 (10,372) | 1292 |
| Central Norway Std. Dev. | 28,547.30 (13,664) | 282 (85) | 676 (222) | 6195.50 (2808) | 11,755.20 (5762) | 171 |
| Norway Std. Dev. | 32,945.40 (22,804) | 335 (202) | 954 (606) | 9017.50 (6179) | 15,430.90 (10,032) | 1463 |
| Eastern Norway | Central Norway | Pooled Data | Metafrontier | |||||
|---|---|---|---|---|---|---|---|---|
| S. Frontier | ||||||||
| 1 | 1(land) | 0.64 *** | (0.05) | 0.77 *** | (0.16) | 0.65 *** | (0.04) | 0.36 |
| 2 | 2 (labor) | 0.17 *** | (0.03) | 0.27 *** | (0.03) | 0.15 *** | (0.03) | 0.51 |
| 3 | 3 (V.cost) | 0.12 *** | (0.03) | 0.09 *** | (0.01) | 0.12 *** | (0.03) | 1.35 |
| (F.cost) | 0.12 *** | (0.03) | 0.17 * | (0.08) | 0.12 *** | (0.03) | 0.01 | |
| 11 | 11 | 0.50 ** | (0.18) | 1.05 | (0.90) | 0.12 *** | (0.03) | −0.52 |
| 22 | 22 | 0.11 | (0.06) | 0.25 | (0.22) | 0.57 *** | (0.17) | 0.21 |
| 33 | 33 | 0.04 | (0.05) | 0.001 | (0.08) | 0.06 | (0.05) | 0.01 |
| 44 | 44 | −0.01 | (0.08) | 0.23 | (0.28) | 0.01 | (0.07) | 0.03 |
| 12 | 12 | −0.06 | (0.07) | −0.10 | (0.40) | −0.05 | (0.08) | −0.83 |
| 13 | 13 | −0.14 | (0.09) | −0.25 | (0.33) | −0.17 * | (0.08) | 0.06 |
| 14 | 14 | −0.15 | (0.09) | −0.15 | (0.22) | −0.20 * | (0.08) | −1.30 |
| 23 | 23 | 0.02 | (0.04) | 0.37 | (0.26) | 0.02 | (0.04) | −1.40 |
| 24 | 24 | 0.02 | (0.05) | −0.17 | (0.30) | 0.01 | (0.05) | 1.28 |
| 34 | 34 | 0.09 | (0.07) | 0.01 | (0.10) | 0.11 * | (0.06) | 0.57 |
| t | 0.09 *** | (0.00) | 0.03 *** | (0.00) | 0.03 *** | (0.00) | 0.63 | |
| t ∗ t | 0.01 *** | (0.00) | 0.01 *** | (0.00) | 0.01 *** | (0.00) | 0.64 | |
| 1 | 1 | −0.03 | (0.02) | −0.05 * | (0.02) | −0.03 * | (0.01) | −0.65 |
| 2 | 2 | 0.01 | (0.01) | 0.02 * | (0.01) | 0.02 | (0.01) | −0.22 |
| 3 | 3 | −0.02 * | (0.01) | 0.004 | (0.01) | −0.02 ** | (0.01) | 0.59 |
| 4 | 4 | −0.00 | (0.01) | −0.03 | (0.03) | −0.003 | (0.01) | −0.61 |
| 0 | _cons | −0.39 *** | (0.03) | −0.28 *** | (0.05) | −0.37 *** | (0.03) | 0.89 |
| U-sigma | −3.77 *** | (0.15) | −3.86 *** | (0.59) | −3.79 *** | (0.14) | ||
| V-sigma | −3.06 *** | (0.07) | −4.54 *** | (0.47) | −3.12 *** | (0.07) | ||
| Theta | 0.16 *** | (0.01) | 0.14 *** | (0.03) | 0.16 *** | (0.01) | ||
| Sigma_u | 0.15 *** | (0.01) | 0.14 *** | (0.04) | 0.15 *** | (0.01) | ||
| Sigma_v | 0.22 *** | (0.01) | 0.10 *** | (0.02) | 0.22 *** | (0.01) | ||
| Lambda | 0.70 *** | (0.02) | 1.04 *** | (0.06) | 0.70 *** | (0.02) | ||
| Log-L | −216 *** | 43 *** | −215 *** | |||||
| RTS | 1.06 | 1.33 | 1.04 | |||||
| N | 1292 | 171 | 1463 | |||||
| Region | Mean | Std. Dev. | Minimum | Maximum | N |
|---|---|---|---|---|---|
| Eastern Norway | 0.88 | 0.06 | 0.29 | 0.98 | 1292 |
| Central Norway | 0.87 | 0.10 | 0.39 | 0.98 | 117 |
| All regions | 0.88 | 0.06 | 0.29 | 0.98 | 1463 |
| Regions | TEi | TE* | TGR | N |
|---|---|---|---|---|
| Eastern Norway | 0.88 | 0.52 | 0.59 | 1292 |
| Central Norway | 0.87 | 0.71 | 0.82 | 117 |
| Regions | Mean | SD | Variance | Minimum | Maximum | N |
|---|---|---|---|---|---|---|
| Eastern Norway | 0.59 | 0.30 | 0.09 | 0.39 | 1.000 | 1292 |
| Central Norway | 0.82 | 0.28 | 0.08 | 0.27 | 1.000 | 117 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alem, H. A Metafrontier Analysis on the Performance of Grain-Producing Regions in Norway. Economies 2021, 9, 10. https://doi.org/10.3390/economies9010010
Alem H. A Metafrontier Analysis on the Performance of Grain-Producing Regions in Norway. Economies. 2021; 9(1):10. https://doi.org/10.3390/economies9010010
Chicago/Turabian StyleAlem, Habtamu. 2021. "A Metafrontier Analysis on the Performance of Grain-Producing Regions in Norway" Economies 9, no. 1: 10. https://doi.org/10.3390/economies9010010
APA StyleAlem, H. (2021). A Metafrontier Analysis on the Performance of Grain-Producing Regions in Norway. Economies, 9(1), 10. https://doi.org/10.3390/economies9010010




