Testing the Effects of Real Exchange Rate Pass-Through to Unemployment in Brazil

Abstract

This paper attempts to test the pass-through of the real exchange rate (RERT) to unemployment in Brazil over the period 1981M1–2015M11 using linear and nonlinear Autoregressive Distributed Lag (ARDL) models. The result of the linearity test suggests that the relationship between RERT and unemployment is linear in the short-run and nonlinear in the long-run. Therefore, using the symmetric ARDL model for the short-run analysis, we find that an increase in the RERT decreases the unemployment rate. The result of the nonlinear ARDL for the long-run analysis shows that the unemployment rate reacts to the RERT appreciations and depreciations differently with depreciations having a strong effect. However, the pass-through of the RERT to unemployment is incomplete both in the short- and long-run. These findings have important policy implications for the designing of appropriate monetary policy in response to a rise in unemployment resulting from a change in the real exchange rate.

1. Introduction

In the early 1990s, the economy of Brazil witnessed a radical shift in the exchange rate and trade policy directions toward economic liberalization, which led to high inflows of capital into the country. The existing empirical literature documents said that the policy directions have had success in stabilizing domestic prices and reviving investment and saving decisions, as well as economic growth (see Bogdanski et al. 2000; Frenkel and Ros 2006; Muinhos 2004; Albuquerque and Portugal 2005; Albuquerque and Portugal 2006; Correa and Minella 2010; Fernandes and Novy 2010). Despite these improvements, the Brazilian economy has been rather dwindling and unimpressive, as the appreciation of the real exchange rate ends up hurting the country’s competitiveness, thereby increasing the unemployment rate. According to the economic indicators’ reports on Brazil published by Banco Central do Brasil (2018), the unemployment rate in the first quarter of 2017 was 13.7%. This declined to 13.0% in the second quarter, 12.4% in the third quarter, and 11.8% in the fourth quarter. These rates are much higher than 4.3% and 7.1% in 1990 and 2002, respectively, as reported by Frenkel and Ros (2006). This situation portends worrisome implications for the loss of human capital and an increase in the risk of social exclusion (Nagore and van Soest 2016).

There is vast literature that suggests a relationship between the unemployment rate and real exchange rate (see Belke and Gros 2002; Fan and Song 2006; Frenkel and Ros 2006; Demir 2010; Feldmann 2011). This strand of literature sees the real exchange rate as a major determinant of production costs of the firms, investment and saving decisions, as well as future earnings uncertainty. Theoretically, a depreciation of the real exchange rate (RERT) causes exports to be cheaper and imports to be more expensive and, consequently, the exporting country would gain trade competitiveness. For the exporting country to increase the quantity of exported goods, they have to keep their prices unchanged in the domestic currency. If peradventure, they are facing high adjustment costs, then it may be difficult to increase quantity; rather, they have to increase prices. On the contrary, an appreciation of the RERT causes exports to become expensive and imports to be relatively cheaper; hence, the exporting country will lose trade competitiveness if they do not change their prices in the domestic currency. If the effect of appreciation is significantly large, then reducing export prices may hamper the profit margins. In this sense, Delatte and López-Villavicencio (2012) argue that the exporting country may prefer to absorb the effect of appreciation so as to increase profit margins and pass a significant part of the effect of depreciation to consumers, if only they have the market powers to set prices. Generally, the effect of the real exchange rate on unemployment could be negative or positive, depending on the specific characteristics of the market. If the rigidities in the labor market significantly improve the bargaining powers of the labor, wages will increase and the net return of the firms will fall (see Darby et al. 1999; Belke and Kaas 2004).

The empirical studies on the effects of the real exchange rate on unemployment rate focused mostly on developed economies, while only a few such studies concentrated on emerging and developing economies (see Frenkel and Ros 2006; Demir 2010). In the case of Latin America, Frenkel and Ros (2006) find that the real exchange rate is a major determinant of unemployment in the region. Similarly, Edwards (1989) reveals that an appreciation of the real exchange rate would decrease employment in the manufacturing sector of the developing countries, while Faria and León-Ledesma (2005) show that the long-run equilibrium effect of the real exchange rate on employment occurs through the channel of trade openness. Therefore, they conclude that an appreciation of the real exchange rate would cause employment to decrease. Supporting this strand of literature, Fan and Song (2006), using the manufacturing data in China during the period 1980–2003, reveal that a depreciation of the real exchange rate promotes employment growth in manufacturing industries, but wage growth is not influenced by the real exchange rate. Furthermore, Feldmann (2011), using data on 17 countries for the period 1982–2003, finds that even though the magnitude of the effect is small, high exchange rate volatility increases the unemployment rate. Providing contrasting evidence, Burgess and Knetter (1998) find that the manufacturing employment in Germany and France does not respond to exchange rate volatility; hence, it is slow to adjust itself to the long-run equilibrium rate. Supporting this finding, Galindo et al. (2006) reveal that real exchange rate depreciation has a negative employment effect on the industries with high dollarization liability. This implies that a depreciation of the real exchange rate would increase the unemployment rate. Considering both the short- and long-run, Chang (2011) reveals that exchange rate uncertainty and unemployment have an equilibrium relationship in the long-run, while in the short-run, the impact of exchange rate uncertainty on unemployment is large, irrespective of all the measures of uncertainty applied. Similarly, Nyahokwe and Ncwadi (2013), who assess the impact of exchange rate volatility on unemployment in South Africa, examine the impacts of the real exchange rate, exports, real interest rate, and GDP on unemployment. However, in the short-run, only the real interest rate and exports have a significant impact in explaining unemployment.

In view of the foregoing literature, it is pertinent at this point to state clearly that the extant literature only focused on the linear relationship between the real exchange rate and unemployment (or employment growth) in both the short- and long-run. This relationship, as examined by the previous studies, might exhibit asymmetric effects in either the long-run or short-run, or possibly in both the short- and long-run, especially when prices are particularly rigid downwards and quantities are rigid upwards (Delatte and López-Villavicencio 2012; Apergis 2015). Therefore, the main objective of this paper is to test the effects of the real ERPT on unemployment in Brazil. Given that Brazil is the largest open economy in the Latin American region, based on the size of its gross domestic product (GDP) (The World Bank 2015), and the fact that the economy has been susceptible to the exchange rate shocks on its macroeconomic variables, it is important to understand the dynamics of the real ERPT on unemployment. This will help in designing an appropriate monetary policy framework in response to the effects of the external shocks on the economy of Brazil. Therefore, the contribution of this study can be viewed in two ways: (1) Even though empirical literature on the real ERPT has gained currency over the years, a great deal of it seeks to examine the extent of the ERPT on imports and domestic prices (Usman and Musa 2018) and others on gold price fluctuations (Balcilar et al. 2017). The few empirical studies on the effects of RERT on unemployment extensively applied linear models, mostly based on Johansen cointegration and error correction models (VECM). These models invariably assume a linear relationship between the variables. The findings from those studies could be misleading if there exists a nonlinearity in the relationship. Therefore, to the best of our knowledge, this is the first time an asymmetric effect of the real ERPT on unemployment is examined for Brazil. (2) The paper uses a linear ARDL and nonlinear ARDL model recently proposed by Pesaran et al. (2001) and Shin et al. (2014). These methodologies have the enviable advantages over the multivariate Johansen cointegration and VECM, which are used extensively in previous studies. Specifically, we use bounds testing procedure to establish a long-run relationship between RERT and unemployment for the linear and nonlinear models. Unlike Johansen cointegration and VECM, the ARDL bounds testing approach does not follow the underlying assumption that all the variables must be integrated of the same order. Whether the variables are integrated of order one, I(1) and I(0), or mutually cointegrated, the method would yield accurate and reliable estimates (See Pesaran et al. 2001).

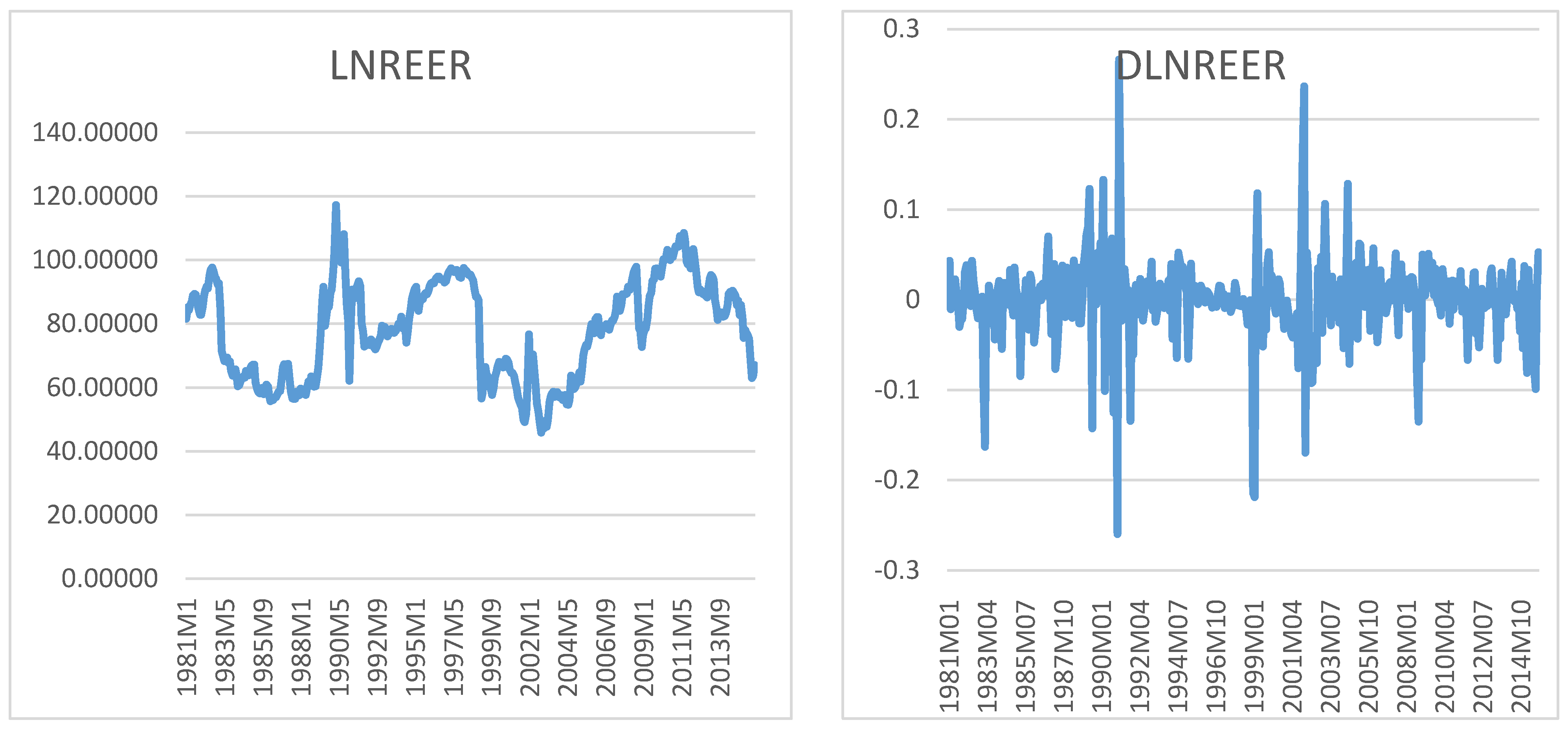
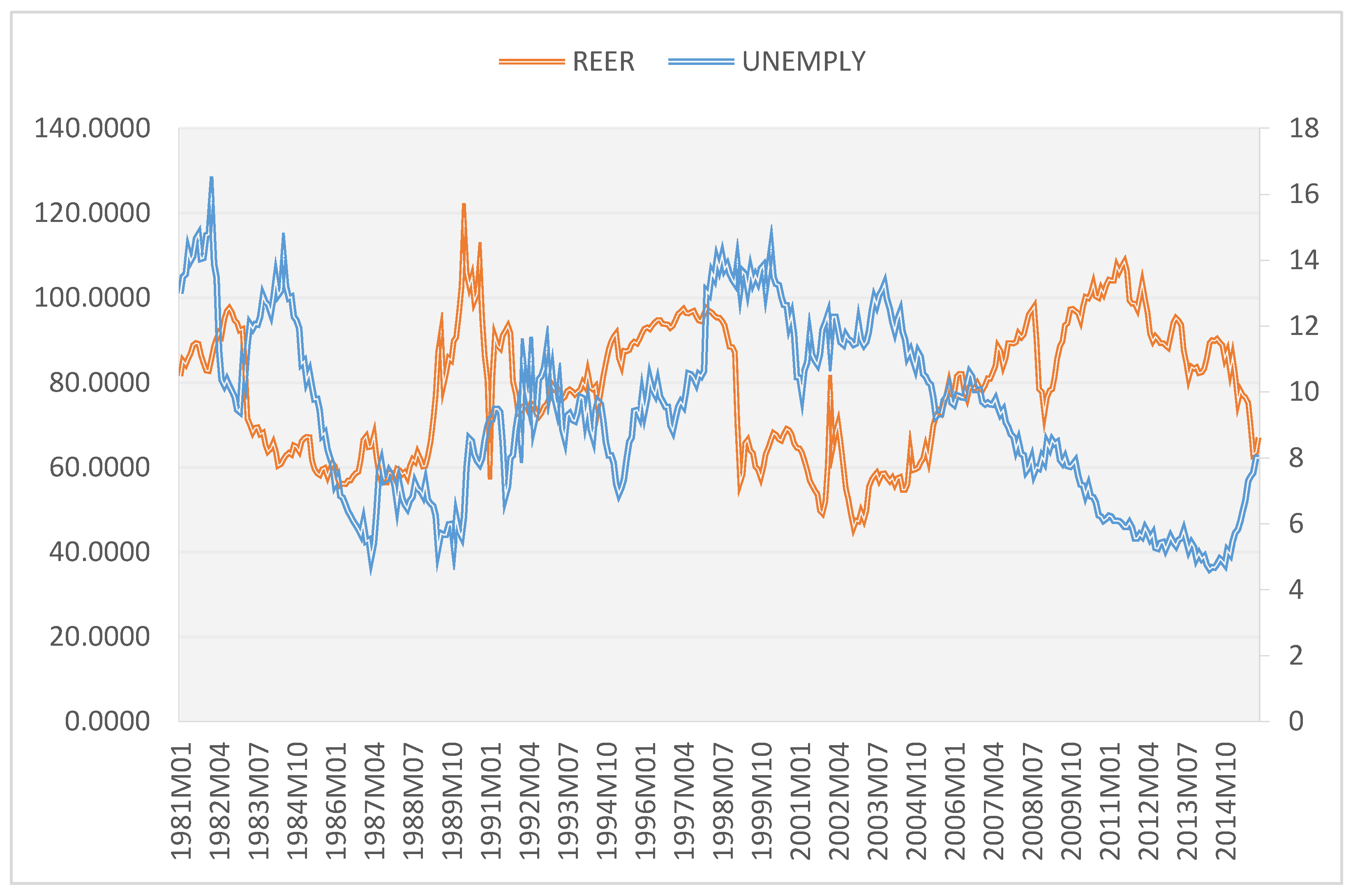
Figure 1 illustrates the behavior of the real effective exchange rate (REERT) and that of the unemployment in Brazil from 1981M01 to 2015M11. The figure shows that the REERT and unemployment rate have witnessed large fluctuations over the years. These fluctuations are attributed to an increase in unemployment and inflation in the 1980s. However, the crawling peg system was very successful in bringing down the rate of inflation over time. The inflation declined, on average, from 2076% in 1994 to 3.2% in 1998, before the system was jettisoned for a more flexible system of exchange rate because of the financial crisis that erupted the economy (Alemán 2011). Furthermore, since the global financial crisis of 2008, and its severe effects on the economies of the world, the REERT has been declining, while the unemployment rate has been increasing in Brazil.

The rest of this paper has been structured as follows. Section 2 presents the data and describes the two methodologies used in this study, which are based on the linear and nonlinear ARDL model. Section 3 is the presentation of results concerning the estimations of the linear and nonlinear ARDL models. Finally, Section 4 provides some concluding remarks and policy implications of the findings.

2. Methodology

2.1. Data

The study uses the real RERT and unemployment rate (UNR) over the period 1981M1 to 2015M11. The monthly frequency data for these variables are generated in order to well capture their asymmetric effects. The data on the unemployment rate is obtained from the database of the Organization for Economic Co-operation and Development (OECD), and it is measured as the total number of unemployed people as a percentage of the labor force1. This data is expressed in percentage, which technically implies its growth impact (see Wooldridge 2009). Following Ghosh and Rajan (2009) and Apergis (2015), the data on the real RERT is measured as the real effective exchange rate (REERT) for the following reasons. Firstly, REERT is broader than the nominal effective exchange rate (NEERT); and secondly, it is more appropriate because of its variations, which leads to robust results. The data is obtained from the International Monetary Fund (IMF) database. It is worth noting that only the RERT is expressed in natural logarithm.

2.2. Unit Root Tests

First, the augmented Dickey-Fuller (ADF) unit root test is applied to check the integrating properties of the time series variables we have used for this study. The null hypothesis for this test is $H_0:\alpha =0$ and the alternative hypothesis is $H_1:\alpha <0$. The null hypothesis, H₀, is rejected if $\alpha$ is less than the asymptotic critical value. Furthermore, the unit root test developed by Zivot and Andrews (1992), which considers the structural breaks, is applied to confirm the results of the ADF in the presence of structural breaks, which are mostly found in the economic and financial time series. If structural breaks are present, then the standard unit root tests will perform poorly (see Zivot and Andrews 1992). Generally, to perform this test, three models considered by Zivot and Andrews (1992) include the model with a break in intercept (Model I), a break in trend (Model II) and a break in intercept and trend (Model III).

$$\mathrm{Model}\mathrm{I}:\Delta y_t={\alpha }_0+{\alpha }_1+\lambda y_{t-1}+\varphi D{U}_t+{\displaystyle \sum _{i=1}^k{\theta }_i\Delta y_{t-i}+{\mu }_t}$$

(1)

$$\mathrm{Model}\mathrm{II}:\Delta y_t={\beta }_0+{\beta }_1+\lambda y_{t-1}+\mathsf{\Phi }D{T}_t+{\displaystyle \sum _{i=1}^k{\theta }_{i}D{y}_{t-i}+{\mu }_t}$$

(2)

$$\mathrm{Model}\mathrm{III}:\Delta y_t={\delta }_0+{\delta }_1+\lambda y_{t-1}+\varphi D{U}_t+\mathsf{\Phi }D{T}_t+{\displaystyle \sum _{i=1}^k{\theta }_{i}D{y}_{t-i}+{\mu }_t}$$

(3)

where $D{U}_t$ is defined as a dummy variable, which indicates a mean shift that occurs at each possible breakpoint ($T_i{}^b$). $D{T}_t$ is the corresponding mean shift in the trend variables. $D{U}_t=1$ if t > $T_i{}^b$, and 0 if otherwise. Similarly, $\mathsf{\Phi }D{T}_t=t-T_i{}^b$ if t > $T_i{}^b$, and 0 if otherwise. It must be noted that $T_i{}^b$ represents the possible break point in the series. The null hypothesis for this test is $H_0:\alpha =0,$ while the alternative hypothesis is $H_1:\alpha$ < 0.

2.3. Model Specification

To estimate the effect of the real ERPT on unemployment in Brazil, the ARDL approach proposed by Pesaran et al. (2001) is applied. This approach allows for jointly investigating the long- and short-run reaction of the unemployment rate to the changes in the real exchange rate and checking for their cointegration. Generally, the unrestricted linear error correction model is presented as follows:

$$\Delta ({\mathrm{UNR}}_t)={\beta }_0+{\beta }_1({\mathrm{UNR}}_{t-1})+{\beta }_{2}ln({\mathrm{RERT}}_{t-1})+{\displaystyle \sum _{i=1}^q{\sigma }_3\Delta }\mathrm{UNR}{(}_{t-i})+{\displaystyle \sum _{i=0}^p{\sigma }_4}\Delta ln({\mathrm{RERT}}_{t-1})+{\mu }_t$$

(4)

where $({\mathrm{UNR}}_t)$ measures the rate of unemployment; $\Delta$ is the difference operator; $ln({\mathrm{RERT}}_{t-1})$ is the lag of the real effective exchange rate; and ${\mu }_t$ is the error term, which is assumed to have zero mean. Theoretically, there are other significant numbers of macroeconomic variables that affect unemployment. For example, increases in the output gap, trade openness, and energy prices are expected to decrease unemployment, while inflation and unemployment are expected to be positively related. However, including these variables into our models increases the fitness of the models, but also decreases the degrees of freedom, which is essential for robustness of our results (Wooldridge 2009; Usman et al. 2016). In this sense, we follow Apergis (2015) by assuming that the effects of control variables not included are captured in the stochastic terms. The coefficients of the long- and short-run real ERPT are expected to be bounded between 0 and 1 $(0<{\beta }_2<1)$ and $(0<{\delta }_4<1)$. If ${\beta }_2=0$ and ${\sigma }_4=0$, then there is no pass-through, indicating that exporting firms, based on the strategy of local currency pricing (LCP) or market share objective, do not vary their export prices in the domestic country’s currency. In this sense, they accommodate the effect of the real exchange rate fluctuations within their mark-ups. If the pass-through is incomplete or partial, that is, ${\beta }_2<1$ and ${\sigma }_4<1$, it indicates that the exporting firms vary their export prices in the domestic country’s currency, but not by the same proportion to the changes in the real exchange rate. Finally, if ${\beta }_2=1$ and ${\sigma }_4=1$, it means that the pass-through is unitary and, therefore, the mark-ups of the exporters do not react to the changes in the real exchange rate, possibly because export prices are set in foreign currency.

As suggested by Apergis (2015), the pass-through of the real exchange rate may possibly exhibit asymmetries. Consequently, to test the asymmetric effects of the real ERPT on unemployment, the recent NARDL model proposed by Shin et al. (2014), which decomposes the real exchange rate into its positive $({\mathrm{RERT}}^{+})$ and negative $({\mathrm{RERT}}^{-})$ partial sums, is applied. Specifically, the nonlinear ARDL model is presented as follows:

$$\begin{array}{l}ln({\mathrm{RERT}}_t^{+})={\displaystyle \sum _{i=1}^t\Delta }ln({\mathrm{RERT}}_i^{+})={\displaystyle \sum _{i=1}^{t}Max(\Delta ln({\mathrm{RERT}}_i),0)}\\ ln({\mathrm{RERT}}_t^{-})={\displaystyle \sum _{i=1}^t\Delta }ln({\mathrm{RERT}}_i^{-})={\displaystyle \sum _{i=1}^{t}Min(\Delta ln({\mathrm{RERT}}_i),0)}\end{array}$$

(5)

where ${\mathrm{RERT}}_t^{+}$ and ${\mathrm{RERT}}_t^{-}$ are the partial sum of the positive (or increases) and negative (or decreases) shocks on the real exchange rate. Equation (5) can be revised to account for an asymmetric level relationship as follows:

$$\begin{array}{l}\Delta ({\mathrm{UNR}}_t)={\phi }_0+{\beta }_1({\mathrm{UNR}}_{t-1})+{\beta }_{2}ln({\mathrm{RERT}}_{t-1}^{+})+{\beta }_{3}ln({\mathrm{RERT}}_{t-1}^{-})+{\displaystyle \sum _{i=1}^q{\sigma }_4\Delta }({\mathrm{UNR}}_{t-i})\\ +{\displaystyle \sum _{i=0}^p{\sigma }_5}\Delta ln({\mathrm{RERT}}_{t-i}^{+})+{\displaystyle \sum _{i=0}^p{\sigma }_6}\Delta ln({\mathrm{RERT}}_{t-i}^{-})+{\nu }_t\end{array}$$

(6)

where $({\mathrm{UNR}}_t)$ and $\Delta$ remain as previously defined in Equation (4). $ln({\mathrm{RERT}}_{t-1})$ is the lag of the real exchange rate, which is decomposed into the partial sums of the positive and negative shocks. ${\nu }_t\sim IN(0,{\sigma }^2).$ The lag orders of the variables are denoted by p and q respectively. The p represents the lag order of exogenous variables while q represents the lag order of the endogenous variable. The first part of Equations (4) and (6) shows the long-run relationship between real ERPT and unemployment, and the second part is the associated short-run of the relationship.

Theoretically, an increase in RERT causes exports to be more expensive and imports to be relatively cheaper, hence domestic currency would lose in trade competitiveness. Therefore, an increase in RERT indicates an appreciation of the domestic currency and a fall in RERT means depreciation of domestic currency. To test for the long-run relationship (cointegration) between RERT and unemployment, a bounds testing approach proposed by Pesaran et al. (2001) is applied. This cointegration test is performed using either F-statistic $(F_{PSS})$ or t-statistic $(t_{BDM})$. The null hypothesis for an F_PSS is $H_0:{\mathrm{RERT}}_t^{+}={\mathrm{RERT}}_i^{-}=0$ against the alternative hypothesis $H_1:{\mathrm{RERT}}_t^{+}\ne 0,{\mathrm{RERT}}_i^{-}\ne 0$. The null hypothesis for a t-BDM on the lagged level dependent variable is $H_0:\mathsf{\Phi }=0$ and the alternative hypothesis is $H_1:\mathsf{\Phi }\ne 0$.

Based on the empirical study by Delatte and López-Villavicencio (2012) and Apergis (2015), this paper estimates the long-run multipliers, that is, the coefficients of the positive and negative changes in the real exchange rate, as $L_{ln(\mathrm{RERT})}^{+}=-{\beta }_2^{+}/{\beta }_1$ and $L_{ln(\mathrm{RERT})}^{+}=-{\beta }_3^{-}/{\beta }_1$. To estimate the long-run symmetric effect of real ERPT, a Wald test is used with the null hypothesis $-{\beta }_2^{+}/{\beta }_1=-{\beta }_3^{-}/{\beta }_1.$ Furthermore, a standard Wald test is used to test for the short-run symmetric effect of real ERPT. The null hypothesis in this test is that ${\displaystyle \sum _{i=0}^p{\sigma }_5}={\displaystyle \sum _{i=0}^p{\sigma }_6}$ for the dynamic coefficients of positive and negative changes in the real effective exchange rate. The short-run estimates of asymmetric real ERPT are given by ${\displaystyle \sum _{i=0}^p{\sigma }_5}\Delta ln({\mathrm{RERT}}_{t-i}^{+})$ and ${\displaystyle \sum _{i=0}^p{\sigma }_6}\Delta ln({\mathrm{RERT}}_{t-i}^{-})$.

3. Empirical Results and Discussion

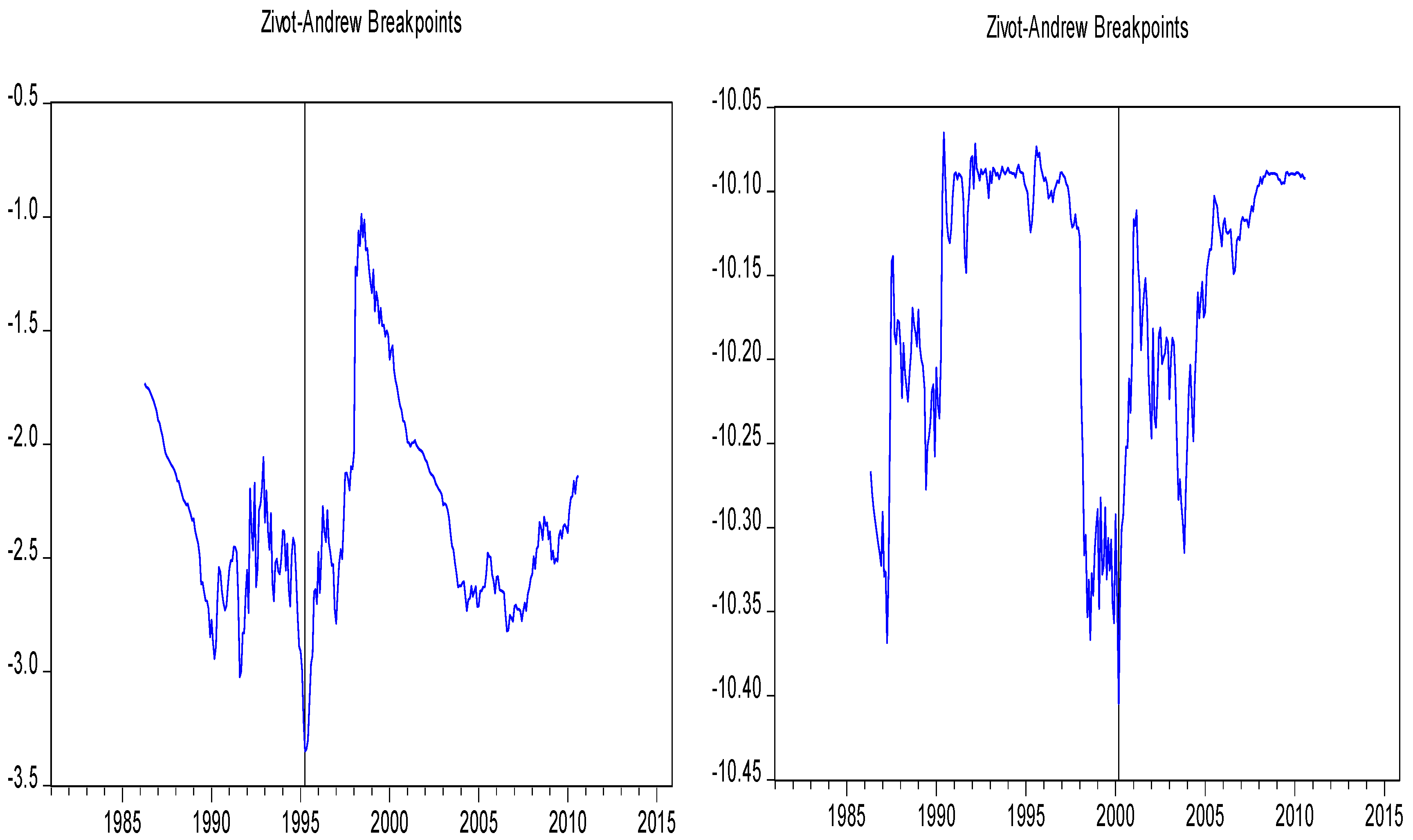
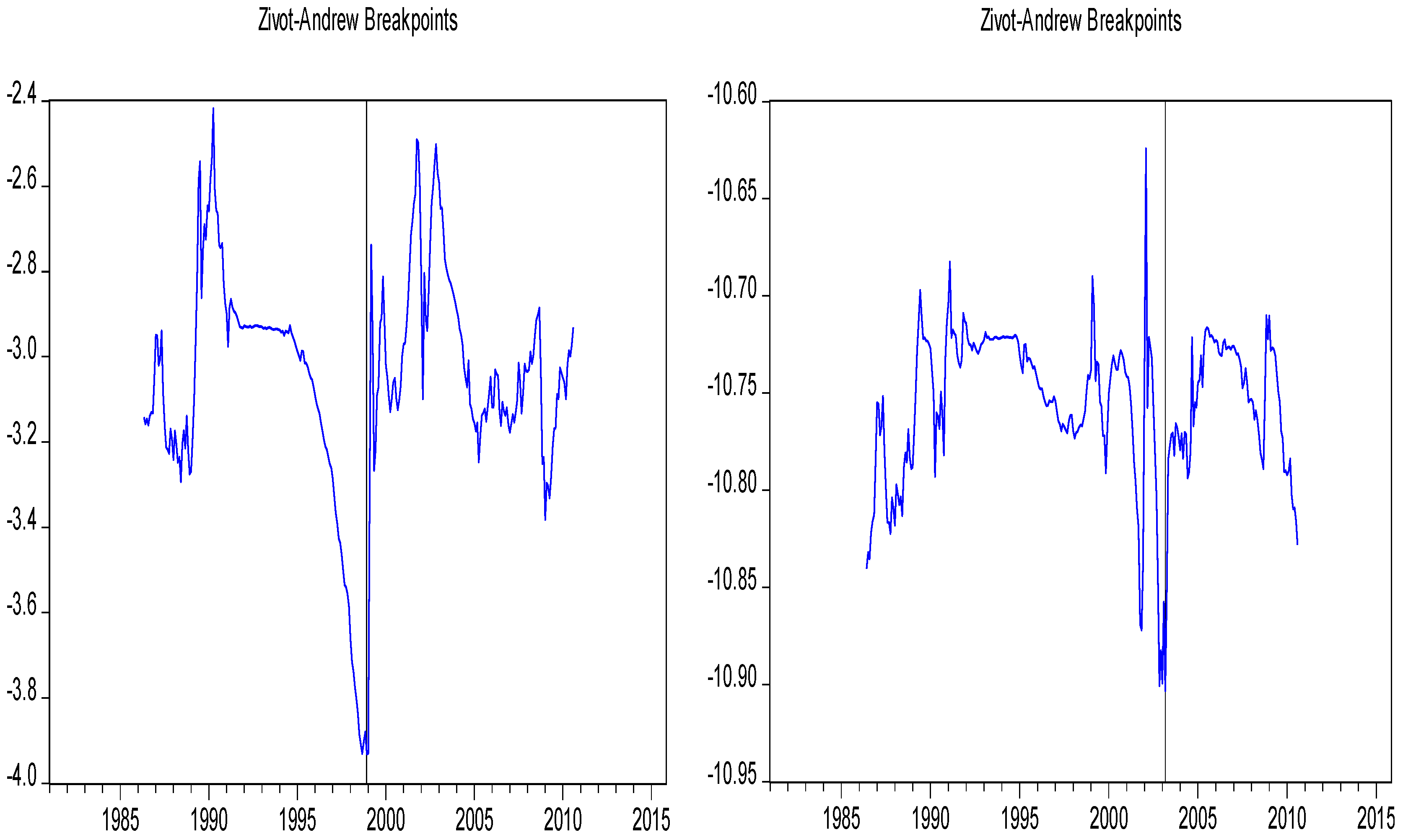
The time plots of the variables in Figure A1 and Figure A2 suggest that there is no trend in the series, but structural breaks in each of the variables are evident. These breaks are more conspicuous in their first differences. The implication of this result is that the nonstationarity tests would be conducted using only the model with intercept. The results of the ADF and Zivot–Andrews unit root tests are presented in

Table 1. Results of ADF and Zivot-Andrews Nonstationarity (Unit Root) Tests.

	At Level		At First Difference
Variable	ADF (Intercept)	Z-A (Intercept)	ADF (Intercept)	Z-A (Intercept)
UNR	−2.073 (0.256)	−3.349	−21.287 (0.000) ***	−10.405 ***
Break-Point	–	1995M04	–	2000M03
Lag Length	(0)	(1)	(0)	(2)
LnRERT	−2.847 (0.049) **	−3.919	−16.984 (0.000) ***	−10.903 ***
Break-Point	–	1998M09	–	2003M03
Lag Length	(1)	(4)	(0)	(4)
Critical Values
1 Percent	−3.45	−5.34	−3.45	−5.34
5 Percent	−2.87	−4.93	−2.87	−4.93
10 Percent	−2.57	−4.58	−2.57	−4.58

Note: *** and ** denote that the null hypothesis, $H_0:\mathsf{\Phi }=0$ is rejected at 1% and 5% level of significance. p-values of the augmented Dickey-Fuller (ADF) test statistics are presented in the brackets ( ). The tests are based only on the model with the intercept. RERT—real exchange rate; UNR—unemployment rate.

. The result of the ADF suggests that the unemployment rate is not stationary at this level. However, after its first difference, the variable becomes stationary at a 1% level of significance. In the case of the real exchange rate, the result indicates the existence of stationarity at a 5% level of significance. After the first difference, it is evident that the real exchange rate is highly significant, easily passing the 1% test of significance in all the models. Furthermore, the Zivot–Andrews test with appropriate lag lengths reveals a slightly different outcome. From the result (see Table 1), it is clear that the unemployment rate and the real exchange rate are stationary in all the models after their first differences. In other words, the null hypothesis of no stationarity is only rejected after their first differences. This signifies that the variables are integrated of order one, I(1), in all the three models with their corresponding break-points2.

Table 2. Long- and short-run symmetry tests.

Variable	Long-Run Asymmetry (WLR)		Short-Run Asymmetry (WSR)
	F-Statistic	p-Value	F-Statistic	p-Value
LnRERT	3.877 **	0.051	2.55	0.112

Note: ** denotes 5% significance level W_SR and W_LR indicate the Wald test for the short- and long-run with p-values in parenthesis. RERT—real exchange rate.

reports the results of long- and short-run symmetry tests using the Wald test. The result clearly shows that the test statistic exceeds the critical value in the long-run, hence the relationship between the RERT and unemployment exhibits asymmetries in the long-run. The implication of this result is that the long-run null hypothesis that $L_{ln(RERT)}^{+}=L_{ln(RERT)}^{-}$ is rejected at a 5 percent level of significance, suggesting that applying linear models in this regard would lead to misspecification of the model. Turning to the short-run symmetric test, the result suggests that the null hypothesis, ${\displaystyle \sum _{i=0}^p\Delta }ln(RER{T}_{t-i}^{+})={\displaystyle \sum _{i=0}^p\Delta }ln(RER{T}_{t-i}^{-})$, cannot be rejected. Therefore, allowing for linear models are most appropriate for analyzing the dynamic interactions between RERT and unemployment for Brazil.

Table 3. Bounds Testing Cointegration.

Model Specification	F-Statistic (FPSS)	Lower Bound I(0)	Upper Bound I(1)
Linear	4.518 **	3.62	4.16
Nonlinear	6.447 **	4.94	5.73

Notes: FPSS denotes the PSS F-statistic. ** denote that the null hypothesis is rejected at 5% level of significance. For K = 1, the upper bounds of the critical values in Pesaran et al. (2001) with unrestricted intercept and no trend are presented at 5% for linear and nonlinear models using F-statistics.

presents the results of the linear and nonlinear bounds testing cointegration using Equations (4) and (6), respectively. The tests clearly show that the $F_{PSS}$ (F-statistic)3 value exceeds the upper critical value of the bounds calculated by Pesaran et al. (2001) at 5% for linear specification and 1% for nonlinear specification. Therefore, the null hypothesis of no cointegration between the unemployment rate and the real exchange rate is rejected. The rejection of the null hypothesis in both cases implies that a valid long-run relationship (cointegration) exists between unemployment and the real exchange rate included in the models.

Table 4. Symmetric ARDL coefficients.

Dependent Variable = UNR
Variables	Coefficient	T-Statistic	p-Value
$LnRERT$	−0.639	−0.09	0.922
$\Delta UN{R}_{t-1}$	−0.018 **	−1.98	0.048
$\Delta LnRERT$	−0.012 ***	−3.96	0.000

Notes: Significance at 1% and 5% level is indicated by *** and ** respectively.

presents the results of the symmetric ARDL model. The results indicate that in the long-run, the effect of the RERT on the unemployment rate is distinctly inelastic and negatively nonsignificant. This confirms the symmetric test result that an asymmetric model performs better in analyzing the long-run relationship between RERT and unemployment. However, in the short-run, the effect of the RERT is inelastic and negatively significant $(\beta =-0.012, \rho <0.01)$. This suggests that a 10% increase in RERT would approximately decrease the unemployment rate by 0.12%. The Wald test of ${\beta }_4=1$ for complete pass-through in the short-run using Equation (4) is further conducted. The result shows that the t-statistic of −13.67 and F-statistic of 60.09 reject the null hypothesis of a complete pass-through at 1% level of significance. The rejection of the null hypothesis in the two test statistics implies that the pass-through effect in the short-run is incomplete. This result aligns with the finding of Ribero et al. (2004) and Demir (2010). Particularly, Ribero’s study finds that the real exchange rate appreciation has a significantly negative effect on employment creation in manufacturing sectors in Brazil, and Demir (2010) reports that the real exchange rate volatility increases unemployment growth of the Turkish manufacturing firms. Our result is similar to Chang (2011) who reports that in the long-run, exchange rate uncertainty has an equilibrium relationship with unemployment, while in the short-run, the impact of the exchange rate uncertainty on unemployment is evident with respect to all the measures of uncertainty.

Table 5. Asymmetric ARDL Coefficients.

Variable	Coefficient	T-Statistic	p-Value
$UN{R}_{t-1}$	−0.236 **	−2.34	0.021
$LnRER{T}^{-}$	0.813 ***	−2.58	0.011
$LnRER{T}^{-}$	−0.667 *	1.82	0.070
$\Delta UN{R}_{t-1}$	−0.131 *	−1.90	0.060
$\Delta LnRER{T}^{+}{}_{t-2}$	−4.272 ***	−2.38	0.019
$\Delta LnRER{T}^{-}{}_{t-1}$	−1.243	−1.04	0.298
Residual Diagnostic Test		F-Statistic	p-Value
Portmanteau test at lag 40 (chi2)		4.859	0.995
Breusch-Pagan heteroscedasticity test		2.939	0.187
Ramsey RESET test (F)		2.213	0.089
Jarque-Bera test on normality (chi2)		2.520	0.284

Notes: Significance at 1%, 5% and 10% level is indicated by ***, **, and *.

presents the results of the dynamic effects of real ERPT to unemployment in Brazil using an appropriate lag length4. Theoretically, the positive change in the real exchange rate (appreciation) implies an increase in the unemployment rate and the negative change in the real exchange rate (depreciation) shows a decrease in the unemployment rate. The coefficient of the long-run asymmetric pass-through described in Equation (6) indicates that the effect of appreciation on unemployment is inelastic and statistically significant, $({\beta }^{+}=0.81, \rho <0.01)$. This finding suggests that a 1% increase in the RERT would lead to approximately 0.81% increase in unemployment rate. On the other hand, a depreciation of RERT exerts a negatively significant and inelastic effect on the unemployment rate, $({\beta }^{-}=-0.67, \rho <0.10)$, suggesting that a 1% increase in RERT would lead to about a 0.67% decrease in unemployment rate. The importance of this result is that it confirms that the RERT depreciation causes the unemployment rate to fall by more than the same magnitude appreciation that will cause it to rise. Therefore, the finding aligns with Fan and Song (2006) that depreciation of the RERT promotes employment growth and reduces the unemployment rate. This finding is also similar to Frenkel and Ros (2006), who find a negative and significant effect of the RERT appreciation on employment growth of 17 Latin American countries.

Turning to the short-term regression associated with the long-run cointegrating model in Equation (2), the result shows that the effect of an appreciation of the RERT on the unemployment rate is elastic and statistically significant $({\beta }^{+}=-4.27, \rho <0.05)$. Specifically, the result shows that a 1% increase in the RERT would inversely change unemployment rate by 4.27%5, while there is no evidence to support the effect of depreciation of the RERT on the unemployment rate. This finding is inconsistent with the earlier finding of Edwards (1989) and Frenkel and Ros (2006). While Edward’s finding points to the fact that appreciation of the RERT decreases employment growth of the manufacturing sector in the developing countries and depreciation increases it, Frenkel and Ros (2006) hold that the real exchange rate is a major determinant of unemployment in the Latin American region. However, our finding echoes the result of Nyahokwe and Ncwadi (2013), who argue that the long-run exchange rate has an impact on unemployment. The only point of departure is that our study focuses on asymmetric effects. Therefore, our finding is novel.

The results of the diagnostic tests conducted on the nonlinear ARDL model used in the study show that the Portmanteau test for autocorrelation, Breusch–Pagan heteroskedasticity test, Ramsey RESET test (F), and Jarque–Bera test on normality could not be rejected. This implies that the nonlinear ARDL model used is correctly specified.

While comparing the results of the symmetric and asymmetric models, it is worth noting that the coefficient of the RERT is not statistically significant in the symmetric ARDL; however, allowing for a long-run asymmetric effect, as suggested by the symmetric test in Table 3, not only increases the magnitude of the pass-through elasticity, but also become statistically significant. Similarly, in the short-run, the pass-through elasticity of RERT in the asymmetric model is possibly beyond the theoretical expectation. However, using the symmetric model, as suggested by the result of the symmetric test in Table 3, gives a sense of theoretical validation.

4. Concluding Remarks and Policy Implications

In this paper, an attempt is made to explore the pass-through of the RERT to unemployment in Brazil using a simple approach of combining the symmetric and asymmetric ARDL models over the period 1981M1–2015M11, as suggested by the symmetric test. Given that most studies simply consider the pass-through of the exchange rate to imports and domestic prices, this study contributes immensely to the bulk of literature by taking into account that positive and negative variations of the real exchange rate have symmetrical and asymmetrical effects on unemployment.

Indeed, the results of the ADF and Zivot–Andrews tests for the stationarity properties of the variables show that unemployment rate and RERT are stationary in their first differences; although under ADF, the RERT is stationary at this level. The results of the symmetric and asymmetric models show that there exists a valid long-run relationship between RERT and unemployment in Brazil.

Based on the symmetric and asymmetric ARDL models, it is clear that there is a role of RERT in the determination of the unemployment, even though the pass-through is incomplete in both the short- and long-run. There is a strong evidence that the pass-through of RERT to unemployment is asymmetrical or nonlinear in the long-run, probably because of downward price rigidities, and symmetrical or linear in the short-run, reflecting the assumption that positive and negative variations of the RERT have equal effects on the unemployment rate. However, the pass-through of appreciation is lower than that of depreciation. In other words, the unemployment rate rises less as a result of an appreciation of domestic currency than the amount that a depreciation of the same magnitude will cause it to fall. Therefore, the findings of this paper are important for the government and its managers, desiring to achieve a lower and stable unemployment rate. In specific terms, the knowledge of the directions (positive and negative) of the RERT movements are essential in the determination of the real exchange rate pass-through to unemployment in the long-run; whereas in the short-run, even though the RERT movements account for the changes in the unemployment rate, its effect is symmetrical. This will assist in designing appropriate monetary policy in response to a rise in unemployment rate resulting from a change in the real exchange rate. Our findings also have policy implication for the timing of current account adjustments, as well as the choice of the exchange rate policy. For further studies, we suggest that a threshold model could be applied in order to capture the effects of not only the direction of the changes in the real exchange rate, but also the effect of the size and magnitude of the changes in the real exchange rate.