The Effect of Government Debt and Other Determinants on Economic Growth: The Greek Experience
Abstract
:1. Introduction
Review of Empirical Literature
2. Empirical Analysis
2.1. Methodology
2.2. Sources and Data
3. Econometric Analysis
3.1. Unit Root Tests
3.2. Cointegration Tests
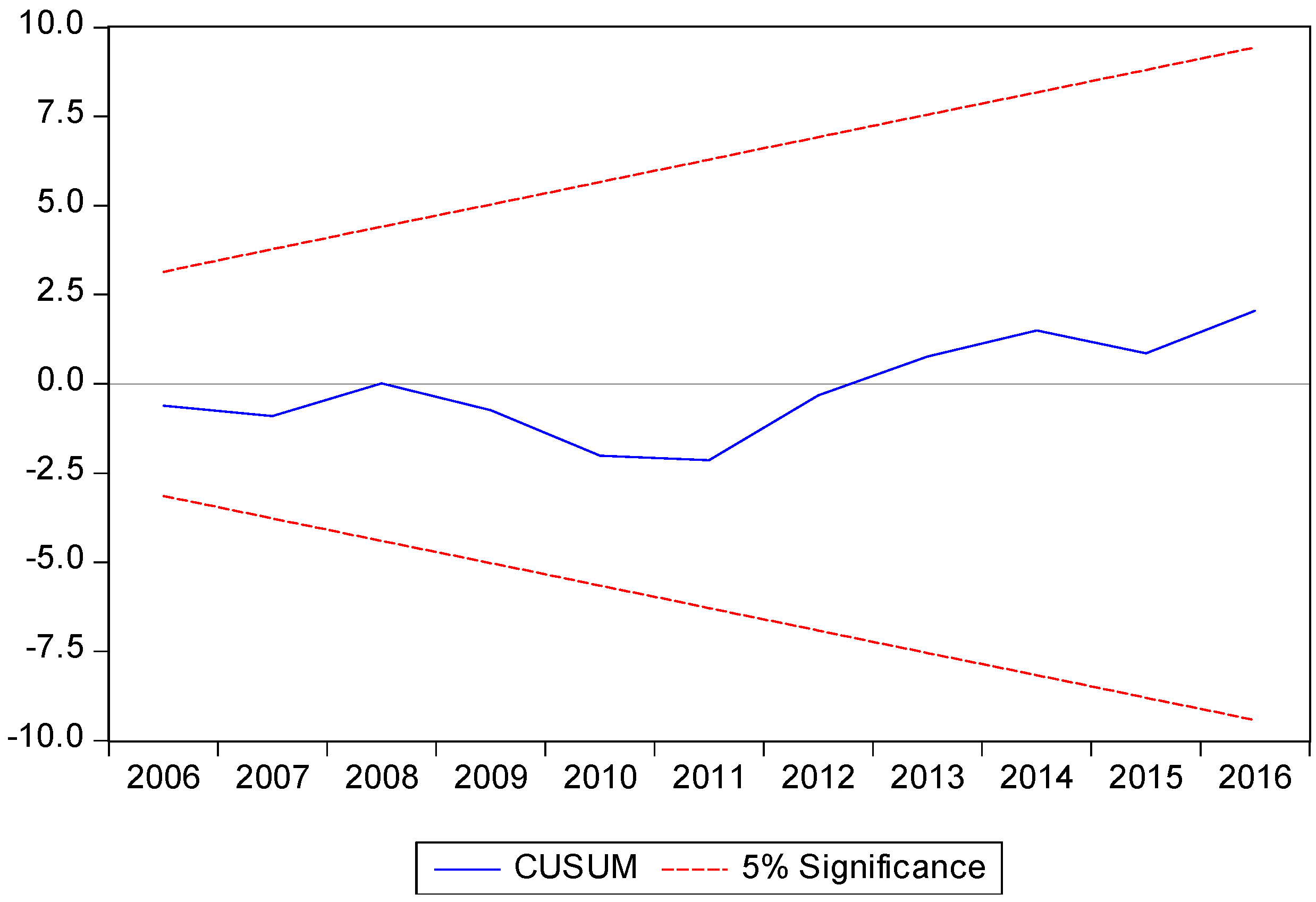
3.3. Robustness Checks
3.4. VAR Estimation
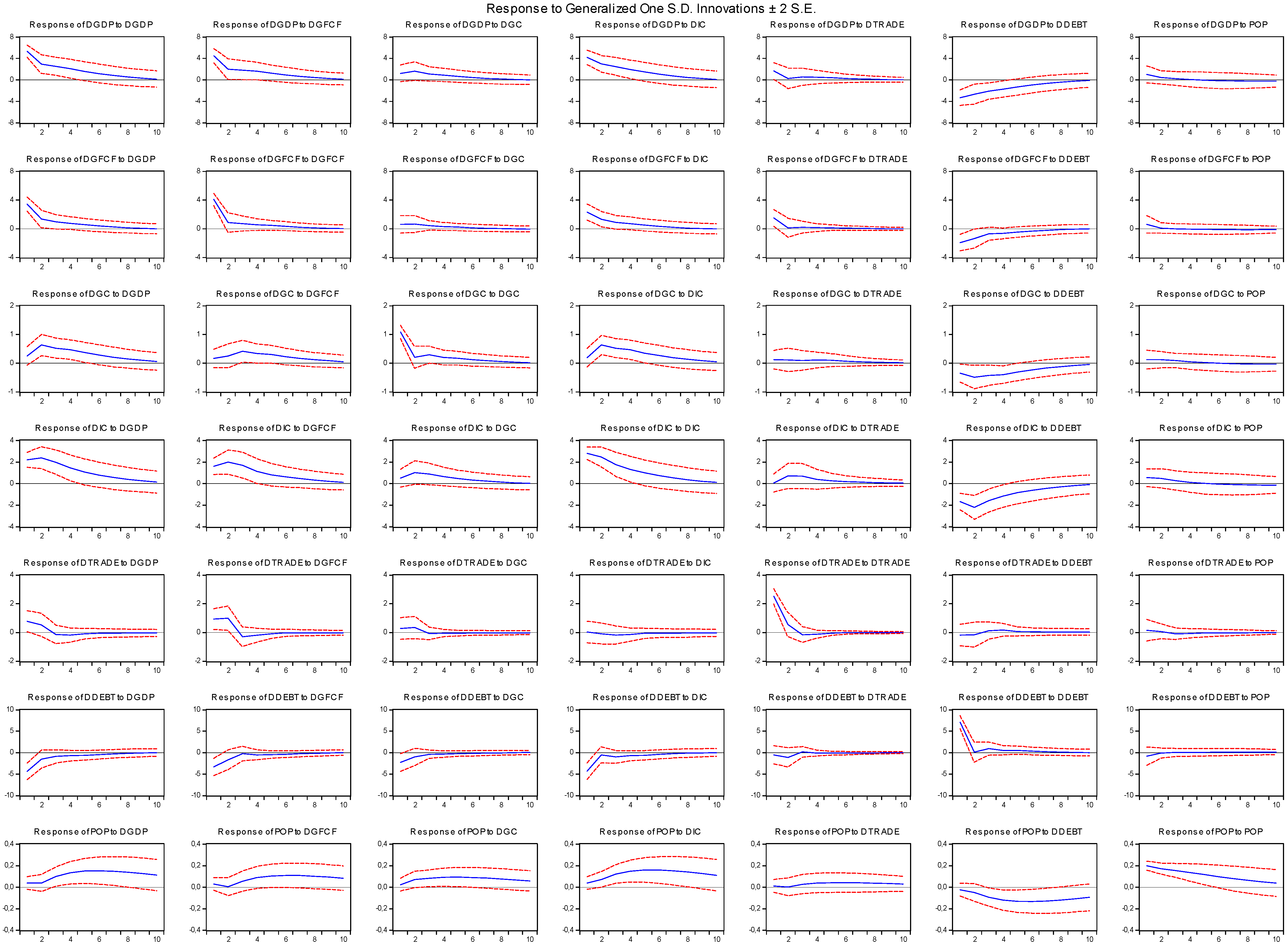
Impulse Response Functions and Variance Decomposition Analysis
3.5. Multiple Structural Breaks Model
3.5.1. Multiple Structural Breaks Tests
3.5.2. Multiple Structural Breaks Estimation Results
4. Discussion of Research Findings
5. Conclusions
Conflicts of Interest
References
- Afonso, Antonio, and José Ricardo Alves. 2015. The Role of Government Debt in Economic Growth. Hacienda Pública Española IEF 215: 9–26. [Google Scholar] [CrossRef]
- Afonso, António, and João Tovar Jalles. 2013. Growth and productivity: The role of government debt. International Review of Economics & Finance 25: 384–407. [Google Scholar]
- Akaike, Hirotugu. 1974. A new look at the statistical model identification. IEEE Transactions on Automatic Control 19: 716–23. [Google Scholar] [CrossRef]
- AMECO Database. 2017. Available online: http://ec.europa.eu/economyfinance/ameco/user/serie/SelectSerie.cfm (accessed on 30 November 2017).
- Anyanwu, John, and Andrew Erhijakpor. 2004. Domestic Debt and Economic Growth: The Nigerian Case. West African Financial and Economic Review 1: 98–128. [Google Scholar]
- Apergis, Nicholas, and Emmanuel Mamatzakis. 2014. What are the driving factors behind the rise of spreads and CDS of euro-area sovereign bonds? A FAVAR model for Greece and Ireland. International Journal of Economics and Business Research 7: 104–20. [Google Scholar] [CrossRef]
- Bai, Jushan, and Pierre Perron. 1998. Estimating and Testing Linear Models with Multiple Structural Changes. Econometrica 66: 47–78. [Google Scholar] [CrossRef]
- Bai, Jushan, and Pierre Perron. 2003. Computation and analysis of multiple structural change models. Journal of Applied Econometrics 18: 1–22. [Google Scholar] [CrossRef]
- Bökemeier, Bettina, and Alfred Greiner. 2015. On the relation between public debt and economic growth: An empirical investigation. Economics and Business Letters 4: 137–50. [Google Scholar]
- Brown, Robert L., James Durbin, and James M. Evans. 1975. Techniques for Testing the Constancy of Regression Relationships over Time. Journal of the Royal Statistical Society, Series B 37: 149–92. [Google Scholar]
- Checherita-Westphal, Cristina, and Philipp Rother. 2012. The impact of high government debt on economic growth and its channels: An empirical investigation for the euro area. European Economic Review 56: 1392–405. [Google Scholar] [CrossRef]
- Cuestas, Juan Carlos, Luis A. Gil-Alana, and Karsten Staehr. 2014. Government debt dynamics and the global financial crisis: Has anything changed in the EA12? Economics Letters 124: 64–66. [Google Scholar] [CrossRef]
- Dickey, David A., and Wayne A. Fuller. 1979. Distributions of the estimators for autoregressive time series with a unit root. Journal of American Statistical Association 74: 427–31. [Google Scholar]
- Dickey, David A., and Wayne A. Fuller. 1981. Likelihood ratio statistics for autoregressive time series with a unit root. Econometrica 49: 1057–72. [Google Scholar] [CrossRef]
- Drine, Imed, and M. Sami Nabi. 2010. Public external debt, informality and production efficiency in developing countries. Economic Modelling 27: 487–95. [Google Scholar] [CrossRef]
- Eberhardt, Markus, and Andrea F. Presbitero. 2015. Public debt and growth: Heterogeneity and non-linearity. Journal of International Economics 97: 45–58. [Google Scholar] [CrossRef]
- Egert, Balázs. 2015. Public debt, economic growth and nonlinear effects: Myth or reality? Journal of Macroeconomics 43: 226–38. [Google Scholar] [CrossRef]
- El-Mahdy, Adel M., and Neveen M. Torayeh. 2009. Debt Sustainability and Economic Growth in Egypt. International Journal of Applied Econometrics and Quantitative Studies 6: 21–55. [Google Scholar]
- Furceri, Davide, and Aleksandra Zdzienicka. 2012. How costly are debt crises? Journal of International Money and Finance 31: 726–42. [Google Scholar] [CrossRef]
- Gómez-Puig, Marta, and Simón Sosvilla-Rivero. 2015. The causal relationship between debt and growth in EMU countries. Journal of Policy Modeling 37: 974–89. [Google Scholar] [CrossRef]
- International Monetary Fund. 2013a. Euro Area Policies. Country Report No. 13/231. Washington: International Monetary Fund. [Google Scholar]
- International Monetary Fund. 2013b. Selected Issues Paper for Greece. Country Report No. 13/155. Washington: International Monetary Fund. [Google Scholar]
- International Monetary Fund. 2016. Fiscal Monitor. Available online: www.imf.org/external/pubs/ft/fm/2016/02/pdf/fm1602.pdf (accessed on 15 January 2018).
- Jiménez-Rodríguez, Rebeca, and Araceli Rodríguez-López. 2015. What Happens to the Relationship between Public Debt and Economic Growth in European Countries? Economics and Business Letters 4: 151–60. [Google Scholar] [CrossRef]
- Kumar, Manmohan, and Jaejoon Woo. 2010. Public Debt and Growth. International Monetary Fund Working Paper No. 10/174. Washington: International Monetary Fund. [Google Scholar]
- Lütkepohl, Helmut. 2006. Structural vector autoregressive analysis for cointegrated variables. Advances in Statistical Analysis, German Statistical Society 90: 75–88. [Google Scholar]
- MacKinnon, James G. 1996. Numerical distribution functions for unit root and cointegration tests. Journal of Applied Econometrics 11: 601–18. [Google Scholar] [CrossRef]
- Mamatzakis, Emmanuel, and Mike Tsionas. 2015. How are market preferences shaped? The case of sovereign debt of stressed euro-area countries. Journal of Banking & Finance 61: 106–16. [Google Scholar]
- Misztal, Piotr. 2010. Public Debt and Economic Growth in the European Union. Journal of Applied Economic Sciences 5: 292–302. [Google Scholar]
- Narayan, Paresh Kumar. 2005. The saving and investment nexus for China: Evidence fromcointegration tests. Applied Economics 37: 1979–90. [Google Scholar] [CrossRef]
- Newey, Whitney K., and Kenneth D. West. 1987. A Simple Positive Semi-Definite, Heteroskedasticity and Autocorrelation Consistent Covariance Matrix. Econometrica 55: 703–8. [Google Scholar] [CrossRef]
- Ogunmuyiwa, Michael Segun. 2011. Does External Debt Promote Economic Growth? Journal of Economic Theory 3: 29–35. [Google Scholar]
- Pattillo, Catherine A., Helene Poirson, and Luca A. Ricci. 2004. What Are the Channels through Which External Debt Affects Growth? International Monetary Fund Working Paper No. 04/15. Washington: International Monetary Fund. [Google Scholar]
- Perron, Pierre. 1989. The great crash, the oil price shock, and the unit root hypothesis. Econometrica 57: 1361–401. [Google Scholar] [CrossRef]
- Perron, Pierre. 1997. Further evidence on breaking trend functions in macroeconomic variables. Journal of Econometrics 80: 355–85. [Google Scholar] [CrossRef]
- Pesaran, M. Hashem, Yongcheol Shin, and Richard J. Smith. 2001. Bounds Testing Approaches to the Analysis of Level Relationships. Journal of Applied Econometrics 16: 289–326. [Google Scholar] [CrossRef]
- Pescatori, Andrea, Damiano Sandri, and John Simon. 2014. Debt and Growth: Is There a Magic Threshold? International Monetary Fund Working Paper No. 14/34. Washington: International Monetary Fund. [Google Scholar]
- Reinhart, Carmen, and Kenneth Rogoff. 2010. Growth in a Time of Debt, American Economic Review. American Economic Association 100: 573–78. [Google Scholar]
- Reinhart, Carmen M., Vincent R. Reinhart, and Kenneth S. Rogoff. 2012. Public Debt Overhangs: Advanced-Economy Episodes since 1800. Journal of Economic Perspectives 26: 69–86. [Google Scholar] [CrossRef]
- Schclarek, Alfredo. 2004. Debt and Economic Growth in Developing and Industrial Countries. Working Papers 2005: 34. Lund: Lund University, Department of Economics. [Google Scholar]
- Schneider, Friedrich. 2011. The Shadow Economy Labour Force. World Economics 12: 53–92. [Google Scholar]
- Sen, Swapan, Krishna M. Kasibhatla, and David B. Stewart. 2007. Debt Overhang and Economic Growth the Asian and the Latin American Experiences. Economic Systems 31: 3–11. [Google Scholar] [CrossRef]
- Shah, Mahmud Hasan, and Pervin Shahida. 2012. External Public Debt and Economic Growth: Empirical Evidence from Bangladesh. Academic Research International 3: 508–15. [Google Scholar]
- Spilimbergo Antonio, Alessandro Prati, and Jonathan David Ostry. 2009. Structural Reforms and Economic Performance in Advanced and Developing Countries. International Monetary Fund Occasional Paper No. 268. Washington: International Monetary Fund. [Google Scholar]
- Swamy, Vighneswara. 2015a. Government Debt and Economic Growth—Decomposing the Cause and Effect Relationship. MPRA Paper 64105. Amsterdam: Elsevier. [Google Scholar]
- Swamy, Vighneswara. 2015b. Government Debt and its Macroeconomic Determinants—An Empirical Investigation. MPRA Paper 64106. Amsterdam: Elsevier. [Google Scholar]
- Tchereni, Betchani, Tshediso Joseph Sekhampu, and Roosevelt Ndovi. 2013. The Impact of Foreign Debt on Economic Growth in Malawi. African Development Review 25: 85–90. [Google Scholar] [CrossRef]
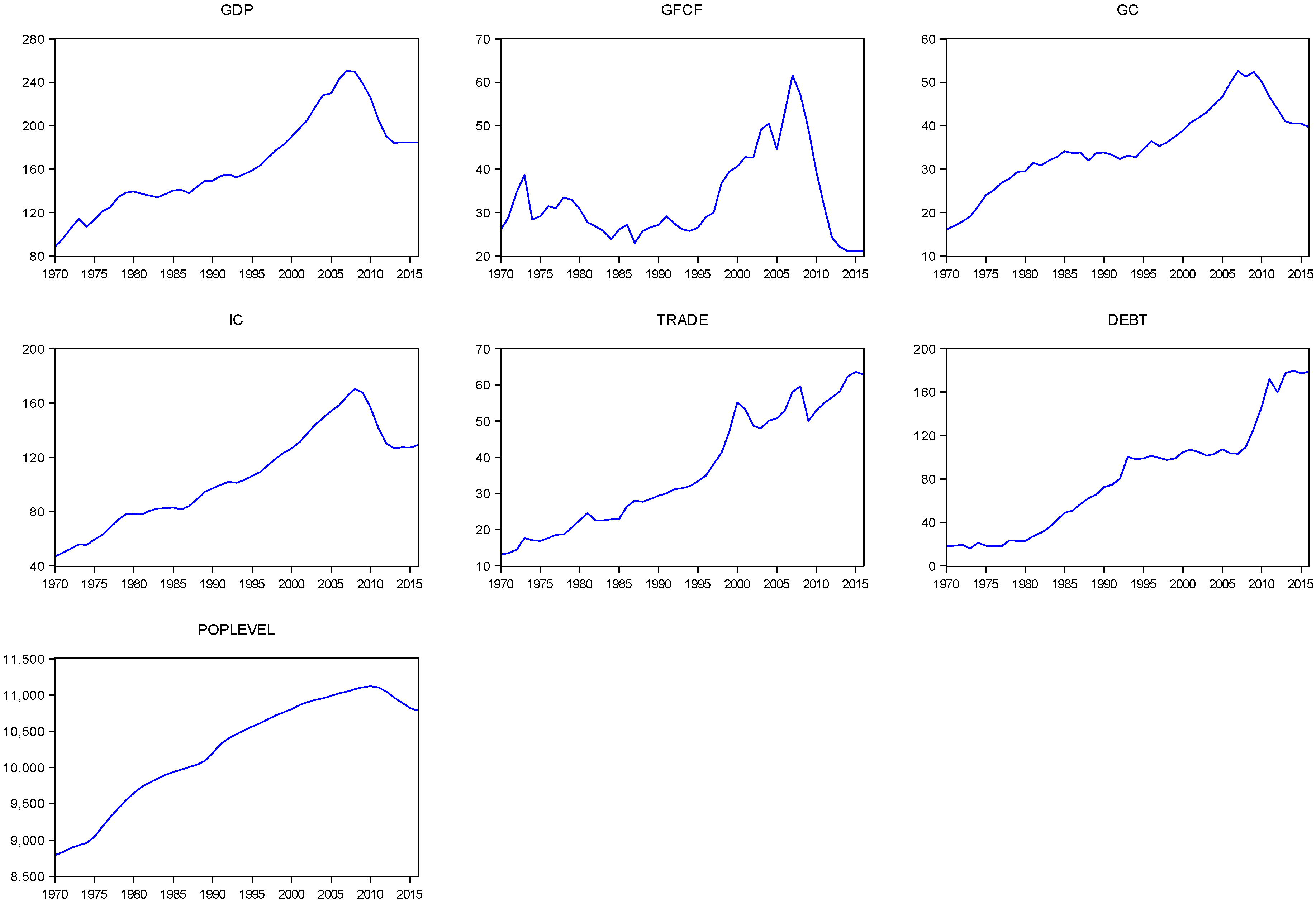
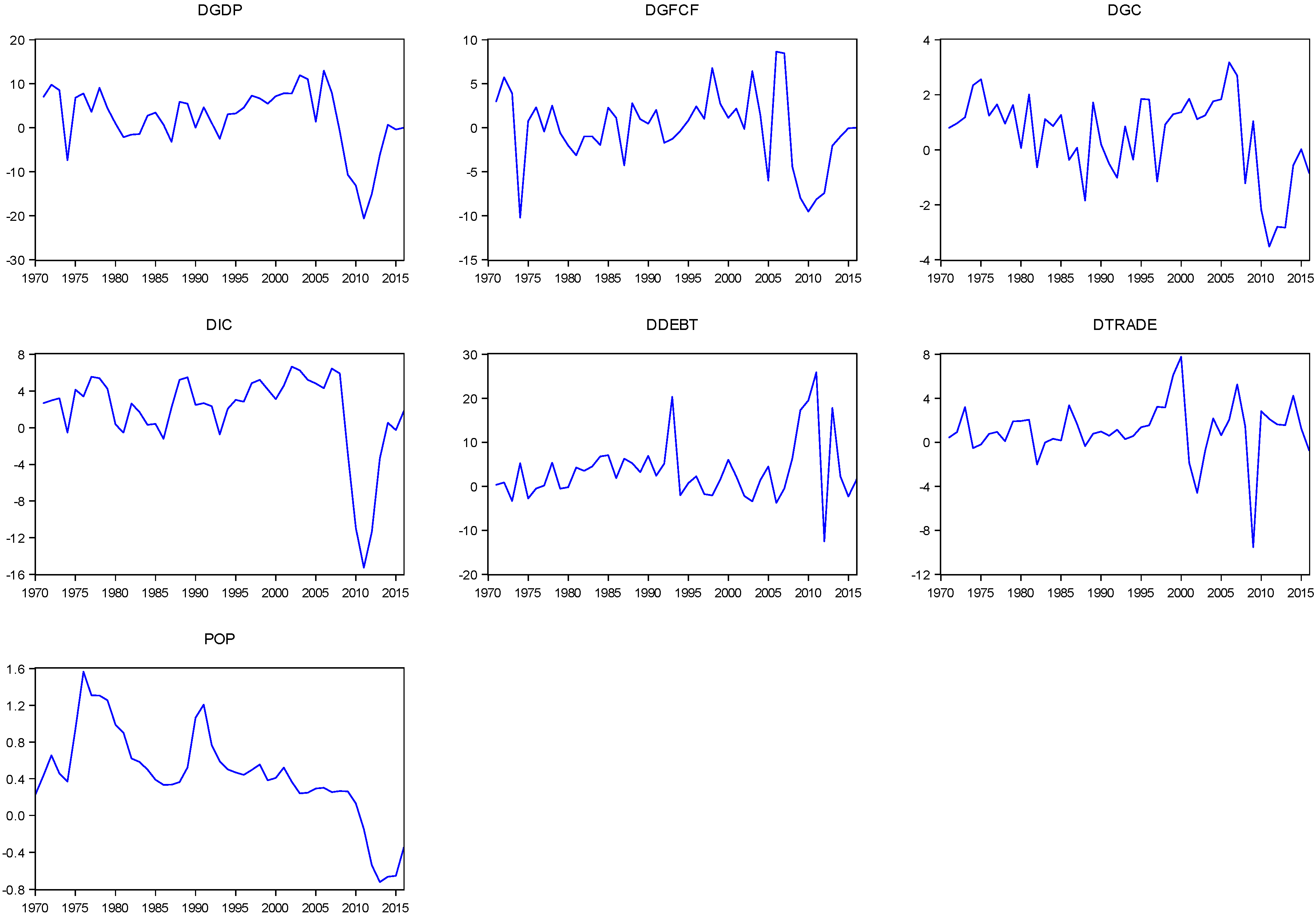

| GDP | Investment | Private | Government | Trade | Population | Debt | |
|---|---|---|---|---|---|---|---|
| Variables Levels | |||||||
| Min | 89 | 21 | 47 | 16 | 13 | 8792 | 16 |
| Max | 250 | 62 | 171 | 53 | 64 | 11,121 | 180 |
| Standard Deviation | 43 | 10 | 35 | 9 | 16 | 743 | 51 |
| Average 1970–2016 | 165 | 33 | 105 | 35 | 36 | 10,244 | 81 |
| Average 1970–1979 | 114 | 31 | 60 | 22 | 17 | 9092 | 19 |
| Average 1980–1989 | 140 | 26 | 83 | 32 | 25 | 9893 | 44 |
| Average 1990–1999 | 162 | 30 | 107 | 35 | 35 | 10,520 | 92 |
| Average 2000–2009 | 225 | 49 | 150 | 46 | 53 | 10,969 | 107 |
| Average 2010–2016 | 194 | 26 | 134 | 43 | 59 | 10,962 | 170 |
| Variables Growth Rates | |||||||
| Min | −9.1 | −26 | −9.7 | −7 | −16 | −0.7 | −17.5 |
| Max | 10 | 22 | 8.8 | 12 | 22 | 1.6 | 33 |
| Standard Deviation | 4.1 | 11.2 | 3.8 | 4.4 | 6.5 | 0.5 | 10.2 |
| Average 1970–2016 | 1.7 | 0.2 | 2.3 | 2.1 | 3.7 | 0.4 | 5.6 |
| Average 1970–1979 | 5.1 | 3.5 | 5.8 | 6.9 | 5.4 | 0.9 | 3.9 |
| Average 1980–1989 | 0.8 | −1.7 | 2.0 | 1.4 | 3.5 | 0.6 | 11.3 |
| Average 1990–1999 | 2.1 | 4.3 | 2.7 | 1.1 | 5.3 | 0.6 | 4.5 |
| Average 2000–2009 | 2.8 | 2.8 | 3.1 | 3.4 | 0.9 | 0.3 | 2.7 |
| Average 2010–2016 | −3.6 | −10.9 | −3.6 | −3.9 | 3.4 | −0.4 | 5.4 |
| Tests | ADF Test | Structural Break ADF Test | |||
|---|---|---|---|---|---|
| Variables | With Intercept | With Intercept and Trend | With Intercept | With Intercept and Trend | |
| lnGDPt | −1.69 | −2.17 | −2.89 | −3.11 | |
| ΔlnGDPt | −2.94 ** | −2.89 *** | −4.50 ** | −5.32 ** | |
| lnGFCFt | −1.92 | −1.78 | −2.89 | −2.60 | |
| ΔlnGFCFt | −4.69 *** | −4.70 *** | −6.42 *** | −6.32 *** | |
| lnICt | −1.46 | −3.23 | −2.47 | −4.77 | |
| ΔlnICt | −3.59 *** | −3.74 ** | −5.89 *** | −6.43 *** | |
| lnGCt | −2.20 | −2.60 | −3.25 | −2.96 | |
| ΔlnGCt | −3.80 *** | −4.23 *** | −5.79 *** | −5.69 *** | |
| lnTRADEt | −0.23 | −3.28 | −2.29 | −7.02 *** | |
| ΔlnTRADEt | −5.89 *** | −5.84 *** | −6.92 *** | −7.25 *** | |
| lnPOPt | −1.98 | −3.58 ** | −3.38 | −5.60 *** | |
| ΔlnPOPt | −4.64 *** | −4.58 *** | −5.95 *** | −5.71 *** | |
| lnDEBTt | 0.73 | −1.94 | −1.53 | −3.41 | |
| ΔlnDEBTt | −6.17 *** | −6.29 *** | −7.65 *** | −7.69 *** | |
| F-Statistic | Significance | I(0) | I(1) |
|---|---|---|---|
| 4.93 | 10% | 2.12 | 3.23 |
| 5% | 2.45 | 3.61 | |
| 2.5% | 2.75 | 3.99 | |
| 1% | 3.15 | 4.43 |
| Variables | Coefficient | p-Value |
|---|---|---|
| Long-Run Estimates | ||
| Investment | 0.48 | 0.00 *** |
| Private consumption | 0.87 | 0.09 * |
| Government consumption | 0.68 | 0.00 *** |
| Trade Openness to GDP ratio | 0.75 | 0.00 *** |
| Government debt to GDP ratio | −0.17 | 0.03 ** |
| Population | 3.55 | 0.00 *** |
| Short−Run Estimates | ||
| ΔInvestment | 0.31 | 0.00 *** |
| ΔPrivate consumption | 1.14 | 0.00 *** |
| ΔGovernment consumption | −0.16 | 0.64 |
| ΔTrade Openness to GDP ratio | 0.59 | 0.00 *** |
| ΔGovernment debt to GDP ratio | −0.09 | 0.00 *** |
| ΔPopulation | −0.83 | 0.39 |
| ECT(−1) | −0.87 | 0.00 *** |
| DGDP | ||||||||
| Period | S.E. | DGDP | DGFCF | DGC | DIC | DTRADE | DDEBT | POP |
| 1 | 5.367109 | 100.0000 | 0.000000 | 0.000000 | 0.000000 | 0.000000 | 0.000000 | 0.000000 |
| 2 | 6.347694 | 92.84623 | 1.760195 | 2.080525 | 2.241954 | 0.126742 | 0.799041 | 0.145312 |
| 3 | 6.927271 | 91.17321 | 2.203039 | 2.269422 | 2.920510 | 0.134816 | 0.961584 | 0.337421 |
| 4 | 7.285171 | 90.58480 | 2.084714 | 2.394973 | 3.156484 | 0.133681 | 1.092130 | 0.553221 |
| 5 | 7.489706 | 90.28286 | 2.024211 | 2.414893 | 3.195489 | 0.130262 | 1.164493 | 0.787794 |
| 6 | 7.599231 | 90.07320 | 1.985235 | 2.410308 | 3.188877 | 0.126673 | 1.194684 | 1.021025 |
| 7 | 7.655610 | 89.89875 | 1.963481 | 2.394957 | 3.173027 | 0.125036 | 1.209285 | 1.235466 |
| 8 | 7.683212 | 89.75038 | 1.950323 | 2.381577 | 3.157980 | 0.125067 | 1.214877 | 1.419797 |
| 9 | 7.696252 | 89.62301 | 1.943778 | 2.373519 | 3.147749 | 0.126214 | 1.216304 | 1.569423 |
| 10 | 7.702634 | 89.51548 | 1.941767 | 2.371086 | 3.143237 | 0.128002 | 1.215795 | 1.684636 |
| DGFCF | ||||||||
| Period | S.E. | DGDP | DGFCF | DGC | DIC | DTRADE | DDEBT | POP |
| 1 | 4.096425 | 71.01870 | 28.98130 | 0.000000 | 0.000000 | 0.000000 | 0.000000 | 0.000000 |
| 2 | 4.402530 | 71.13811 | 26.50064 | 0.487876 | 0.442362 | 0.070642 | 1.175995 | 0.184379 |
| 3 | 4.523193 | 71.87678 | 25.21977 | 0.717022 | 0.599494 | 0.066957 | 1.126364 | 0.393607 |
| 4 | 4.603660 | 72.17613 | 24.45658 | 0.763157 | 0.753317 | 0.064693 | 1.188297 | 0.597819 |
| 5 | 4.647746 | 72.35556 | 24.00109 | 0.788256 | 0.800274 | 0.063606 | 1.204096 | 0.787121 |
| 6 | 4.670818 | 72.39572 | 23.76775 | 0.790546 | 0.810918 | 0.063115 | 1.215984 | 0.955961 |
| 7 | 4.681806 | 72.36387 | 23.65639 | 0.788449 | 0.810316 | 0.063676 | 1.219029 | 1.098270 |
| 8 | 4.686954 | 72.30362 | 23.60462 | 0.786832 | 0.808550 | 0.064968 | 1.219593 | 1.211819 |
| 9 | 4.689577 | 72.24052 | 23.57954 | 0.787814 | 0.808804 | 0.066603 | 1.218931 | 1.297787 |
| 10 | 4.691333 | 72.18645 | 23.56429 | 0.791421 | 0.811713 | 0.068300 | 1.218047 | 1.359778 |
| DIC | ||||||||
| Period | S.E. | DGDP | DGFCF | DGC | DIC | DTRADE | DDEBT | POP |
| 1 | 2.803223 | 61.21621 | 3.012368 | 0.027698 | 35.74373 | 0.000000 | 0.000000 | 0.000000 |
| 2 | 3.917754 | 68.45344 | 1.560286 | 1.420664 | 24.75366 | 0.634272 | 3.142541 | 0.035137 |
| 3 | 4.445631 | 72.88867 | 1.224660 | 2.032387 | 20.02615 | 0.693318 | 2.989842 | 0.144980 |
| 4 | 4.705276 | 74.45848 | 1.202899 | 2.232949 | 18.21958 | 0.630355 | 2.928031 | 0.327705 |
| 5 | 4.842424 | 75.05582 | 1.230080 | 2.295718 | 17.40620 | 0.595937 | 2.874501 | 0.541744 |
| 6 | 4.918858 | 75.29807 | 1.234458 | 2.299914 | 16.98039 | 0.577651 | 2.853146 | 0.756370 |
| 7 | 4.959982 | 75.37546 | 1.224482 | 2.289525 | 16.74479 | 0.568138 | 2.842443 | 0.955158 |
| 8 | 4.980793 | 75.35933 | 1.216066 | 2.277308 | 16.61799 | 0.563854 | 2.836564 | 1.128887 |
| 9 | 4.990710 | 75.29848 | 1.211244 | 2.268723 | 16.55369 | 0.562744 | 2.832539 | 1.272588 |
| 10 | 4.995409 | 75.22463 | 1.209558 | 2.265000 | 16.52266 | 0.563330 | 2.829509 | 1.385316 |
| DGC | ||||||||
| Period | S.E. | DGDP | DGFCF | DGC | DIC | DTRADE | DDEBT | POP |
| 1 | 1.091545 | 5.277675 | 0.572375 | 94.14995 | 0.000000 | 0.000000 | 0.000000 | 0.000000 |
| 2 | 1.370134 | 24.63308 | 14.98146 | 59.77871 | 0.318626 | 0.139451 | 0.130769 | 0.017898 |
| 3 | 1.490187 | 33.17324 | 12.76062 | 51.93148 | 1.727866 | 0.121743 | 0.218722 | 0.066333 |
| 4 | 1.578295 | 38.43564 | 11.88081 | 46.56751 | 2.262088 | 0.142251 | 0.550651 | 0.161050 |
| 5 | 1.629530 | 41.40207 | 11.16799 | 43.94795 | 2.419655 | 0.143281 | 0.634756 | 0.284301 |
| 6 | 1.659358 | 42.95530 | 10.82076 | 42.48031 | 2.471629 | 0.140545 | 0.704282 | 0.427177 |
| 7 | 1.675450 | 43.73099 | 10.62745 | 41.71646 | 2.484716 | 0.137978 | 0.730739 | 0.571668 |
| 8 | 1.683955 | 44.08932 | 10.52712 | 41.31125 | 2.483753 | 0.136654 | 0.746156 | 0.705754 |
| 9 | 1.688184 | 44.22783 | 10.47531 | 41.10817 | 2.478002 | 0.136407 | 0.752533 | 0.821745 |
| 10 | 1.690198 | 44.25800 | 10.45037 | 41.01038 | 2.472811 | 0.136925 | 0.754953 | 0.916561 |
| DTRADE | ||||||||
| Period | S.E. | DGDP | DGFCF | DGC | DIC | DTRADE | DDEBT | POP |
| 1 | 2.521706 | 9.598210 | 4.098258 | 0.297542 | 10.73614 | 75.26985 | 0.000000 | 0.000000 |
| 2 | 2.833599 | 11.04457 | 15.86635 | 1.413901 | 12.04242 | 59.61329 | 0.019415 | 0.000051 |
| 3 | 2.866339 | 11.05231 | 16.78048 | 1.421585 | 12.24731 | 58.44475 | 0.028234 | 0.025338 |
| 4 | 2.875262 | 11.39996 | 16.72113 | 1.414368 | 12.17750 | 58.13479 | 0.115582 | 0.036666 |
| 5 | 2.877253 | 11.48329 | 16.71295 | 1.431068 | 12.16157 | 58.05517 | 0.115468 | 0.040487 |
| 6 | 2.878041 | 11.51318 | 16.71605 | 1.433121 | 12.15625 | 58.02339 | 0.116348 | 0.041656 |
| 7 | 2.878555 | 11.53563 | 16.71236 | 1.435536 | 12.15516 | 58.00269 | 0.116519 | 0.042100 |
| 8 | 2.879006 | 11.55662 | 16.70854 | 1.436634 | 12.15411 | 57.98466 | 0.117223 | 0.042215 |
| 9 | 2.879337 | 11.57312 | 16.70508 | 1.437562 | 12.15289 | 57.97146 | 0.117668 | 0.042216 |
| 10 | 2.879565 | 11.58470 | 16.70275 | 1.438095 | 12.15190 | 57.96233 | 0.118010 | 0.042217 |
| DDEBT | ||||||||
| Period | S.E. | DGDP | DGFCF | DGC | DIC | DTRADE | DDEBT | POP |
| 1 | 7.129703 | 37.69294 | 0.784836 | 3.163484 | 3.313512 | 0.358028 | 54.68720 | 0.000000 |
| 2 | 7.487359 | 38.38591 | 1.642390 | 3.811323 | 4.405577 | 0.383010 | 51.30463 | 0.067159 |
| 3 | 7.618284 | 38.48118 | 3.095898 | 3.701890 | 4.363642 | 0.462141 | 49.72213 | 0.173113 |
| 4 | 7.662429 | 38.92401 | 3.084351 | 3.713509 | 4.402401 | 0.457619 | 49.15138 | 0.266728 |
| 5 | 7.696660 | 39.26898 | 3.076732 | 3.688974 | 4.411359 | 0.453965 | 48.74989 | 0.350096 |
| 6 | 7.714399 | 39.44756 | 3.062861 | 3.679000 | 4.400321 | 0.451893 | 48.53493 | 0.423431 |
| 7 | 7.722931 | 39.50998 | 3.056249 | 3.671556 | 4.392095 | 0.451147 | 48.43297 | 0.486001 |
| 8 | 7.726796 | 39.51780 | 3.053333 | 3.667887 | 4.387709 | 0.451332 | 48.38585 | 0.536090 |
| 9 | 7.728735 | 39.50716 | 3.052182 | 3.666794 | 4.385921 | 0.451854 | 48.36195 | 0.574144 |
| 10 | 7.729997 | 39.49432 | 3.052194 | 3.667284 | 4.385884 | 0.452460 | 48.34618 | 0.601685 |
| DPOP | ||||||||
| Period | S.E. | DGDP | DGFCF | DGC | DIC | DTRADE | DDEBT | POP |
| 1 | 0.198257 | 3.609171 | 0.030834 | 0.502624 | 0.495685 | 0.065973 | 0.106932 | 95.18878 |
| 2 | 0.274738 | 3.898276 | 4.090641 | 4.840830 | 4.088194 | 0.435083 | 0.070723 | 82.57625 |
| 3 | 0.335282 | 11.18868 | 5.000551 | 6.124407 | 6.135559 | 0.976501 | 0.177556 | 70.39675 |
| 4 | 0.389030 | 20.07450 | 4.827256 | 6.936725 | 7.167348 | 1.175895 | 0.379940 | 59.43833 |
| 5 | 0.435668 | 27.75677 | 4.687330 | 7.291766 | 7.613398 | 1.221084 | 0.567148 | 50.86250 |
| 6 | 0.474673 | 33.75991 | 4.554415 | 7.434345 | 7.813776 | 1.201727 | 0.711787 | 44.52404 |
| 7 | 0.506346 | 38.30412 | 4.441218 | 7.454374 | 7.893024 | 1.164485 | 0.826001 | 39.91678 |
| 8 | 0.531274 | 41.68822 | 4.337746 | 7.419969 | 7.906453 | 1.125745 | 0.913284 | 36.60858 |
| 9 | 0.550301 | 44.17069 | 4.249588 | 7.363296 | 7.886021 | 1.091204 | 0.979218 | 34.25998 |
| 10 | 0.564381 | 45.96156 | 4.177453 | 7.302457 | 7.851131 | 1.062615 | 1.027821 | 32.61696 |
| Test for One Break | |||
| Break Test | F-Statistic | Scaled F-Statistic | Critical Value |
| 0 vs. 1 * | 56.92 | 341.57 | 20.08 |
| Estimated Break date: 2007 | |||
| Test for Two Breaks | |||
| Break Test | F-Statistic | Scaled F-Statistic | Critical Value |
| 0 vs. 1 * | 56.92 | 341.57 | 20.08 |
| 1 vs. 2 * | 8.93 | 53.63 | 22.11 |
| Estimated Break date: 1990, 2007 | |||
| Test for Three Breaks | |||
| Break Test | F-Statistic | Scaled F-Statistic | Critical Value |
| 0 vs. 1 * | 56.92 | 341.57 | 20.08 |
| 1 vs. 2 * | 8.93 | 53.63 | 22.11 |
| 2 vs. 3 * | 19.54 | 117.24 | 23.04 |
| Estimated Break date: 1983, 1990, 2007 | |||
| Test for Four Breaks | |||
| Break Test | F-Statistic | Scaled F-Statistic | Critical Value |
| 0 vs. 1 * | 56.92 | 341.57 | 20.08 |
| 1 vs. 2 * | 8.93 | 53.63 | 22.11 |
| 2 vs. 3 * | 19.54 | 117.24 | 23.04 |
| 3 vs. 4 * | 12.62 | 76.09 | 23.77 |
| Estimated Break date: 1983, 1990, 2000, 2007 | |||
| Test for Five Breaks | |||
| Break Test | F-Statistic | Scaled F-Statistic | Critical Value |
| 0 vs. 1 * | 56.92 | 341.57 | 20.08 |
| 1 vs. 2 * | 8.93 | 53.63 | 22.11 |
| 2 vs. 3 * | 19.54 | 117.24 | 23.04 |
| 3 vs. 4 * | 12.62 | 76.09 | 23.77 |
| 4 vs. 5 | 0.00 | 0.00 | 24.43 |
| Variable’s Coefficient | ||||||
|---|---|---|---|---|---|---|
| GFCF | GC | IC | TRADE | DEBT | POP | |
| 4−break model | ||||||
| 1970–1982 | 1.12 *** | 1.17 | 0.63 ** | 0.64 | 0.13 | 1.96 |
| (8.98) | (1.66) | (2.56) | (1.38) | (0.56) | (0.89) | |
| 1983–1989 | 0.83 * | 1.10 *** | 1.21 *** | −0.18 | −0.11 | −2.89 *** |
| (2.02) | (4.84) | (5.59) | (−0.46) | (−1.13) | (−4.84) | |
| 1990–1999 | 0.26 | 0.03 | 1.50 *** | 0.10 | −0.01 | 7.15 *** |
| (1.65) | (0.16) | (20.96) | (0.55) | (−0.31) | (4.6) | |
| 2000–2006 | 0.49 *** | −1.84 | 2.22 *** | 0.66 * | −0.84 ** | 2.84 * |
| (3.79) | (−1.21) | (3.68) | (1.80) | (−2.05) | (1.76) | |
| 2007–2016 | 0.67 *** | 1.55 *** | 0.60 *** | 0.50 *** | 0.01 | 0.01 |
| (4.49) | (6.21) | (7.30) | (5.09) | (0.03) | (0.00) | |
© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pegkas, P. The Effect of Government Debt and Other Determinants on Economic Growth: The Greek Experience. Economies 2018, 6, 10. https://doi.org/10.3390/economies6010010
Pegkas P. The Effect of Government Debt and Other Determinants on Economic Growth: The Greek Experience. Economies. 2018; 6(1):10. https://doi.org/10.3390/economies6010010
Chicago/Turabian StylePegkas, Panagiotis. 2018. "The Effect of Government Debt and Other Determinants on Economic Growth: The Greek Experience" Economies 6, no. 1: 10. https://doi.org/10.3390/economies6010010
APA StylePegkas, P. (2018). The Effect of Government Debt and Other Determinants on Economic Growth: The Greek Experience. Economies, 6(1), 10. https://doi.org/10.3390/economies6010010




