Chipless Radio Frequency Identification (RFID) Sensor for Angular Rotation Monitoring
Abstract
1. Introduction
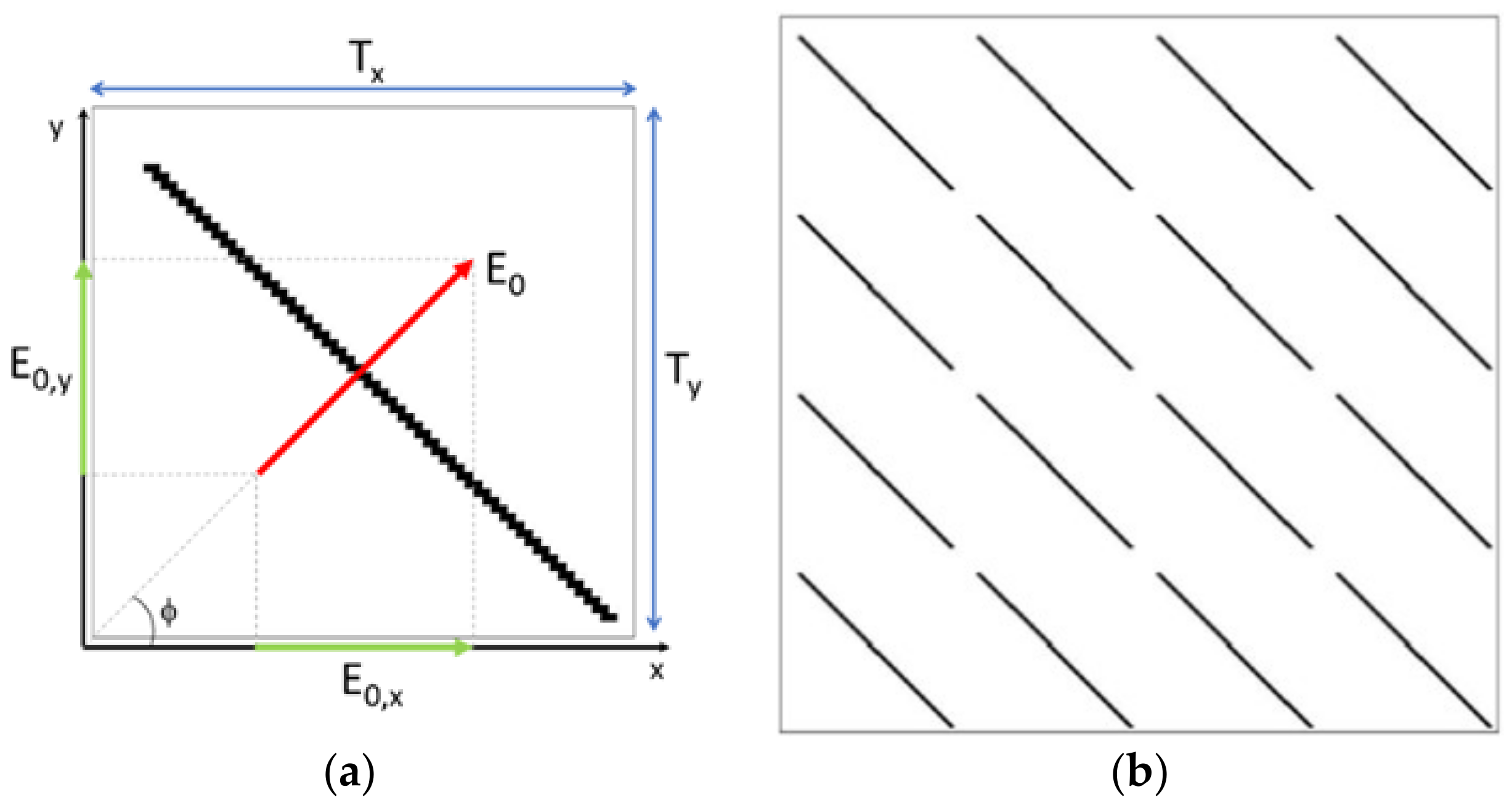
2. Cross Polar Features and Chipless RFID Sensor Design
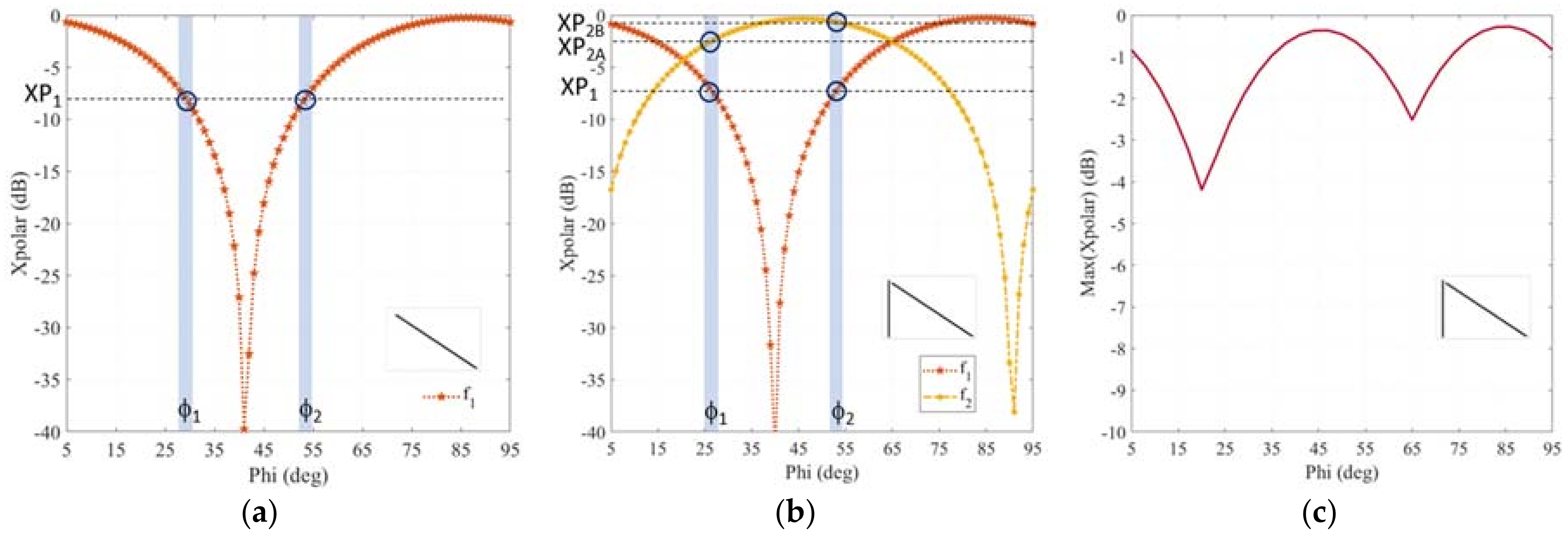
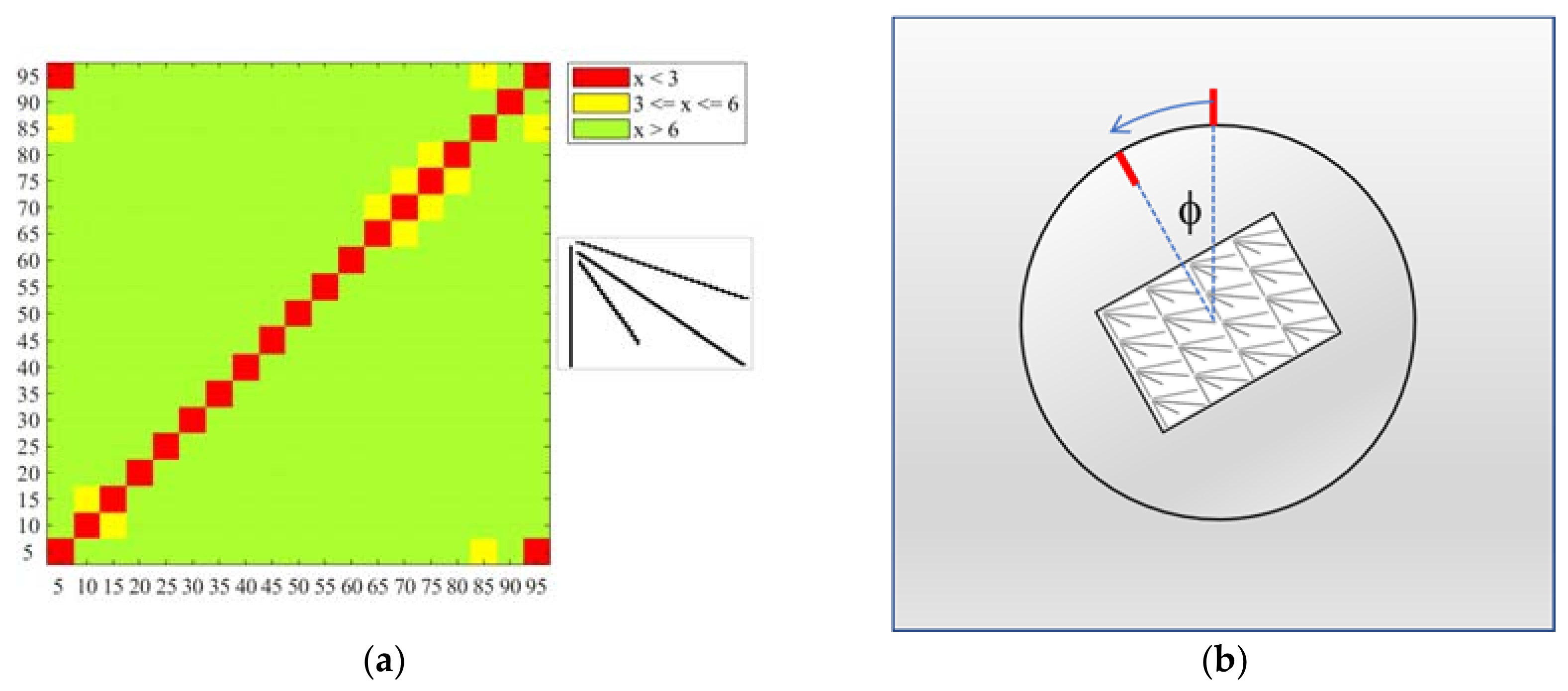
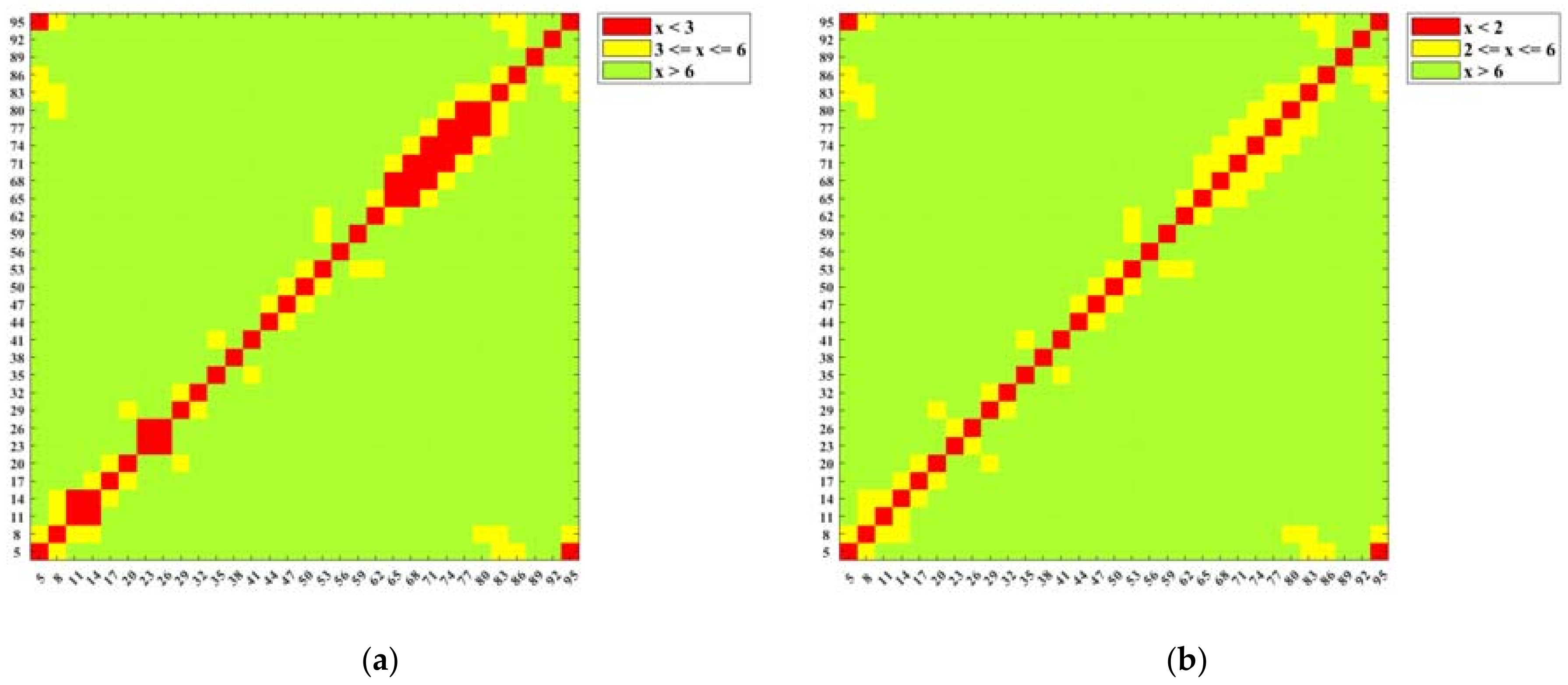
3. Performance Assessment and Design Improvement
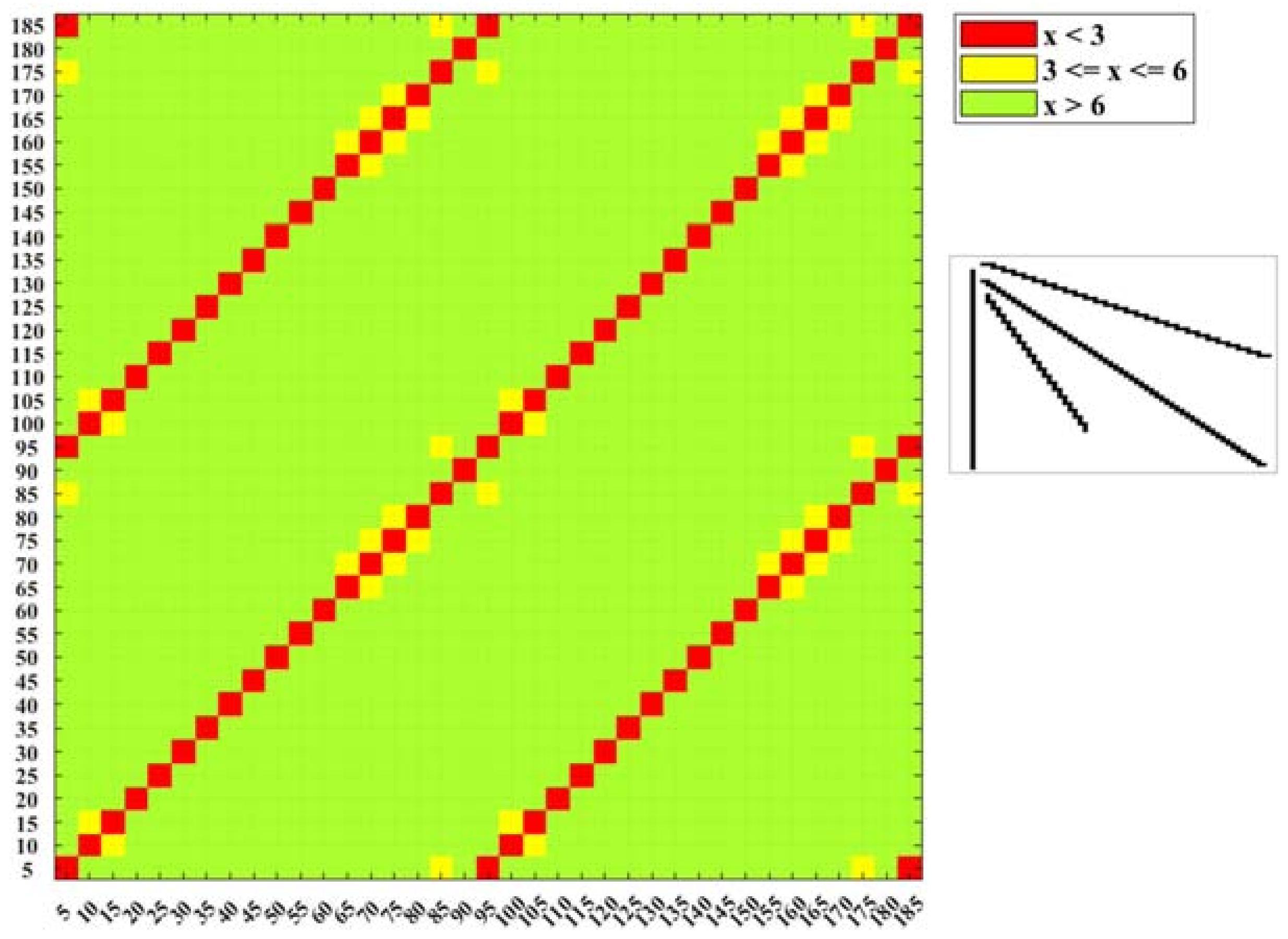
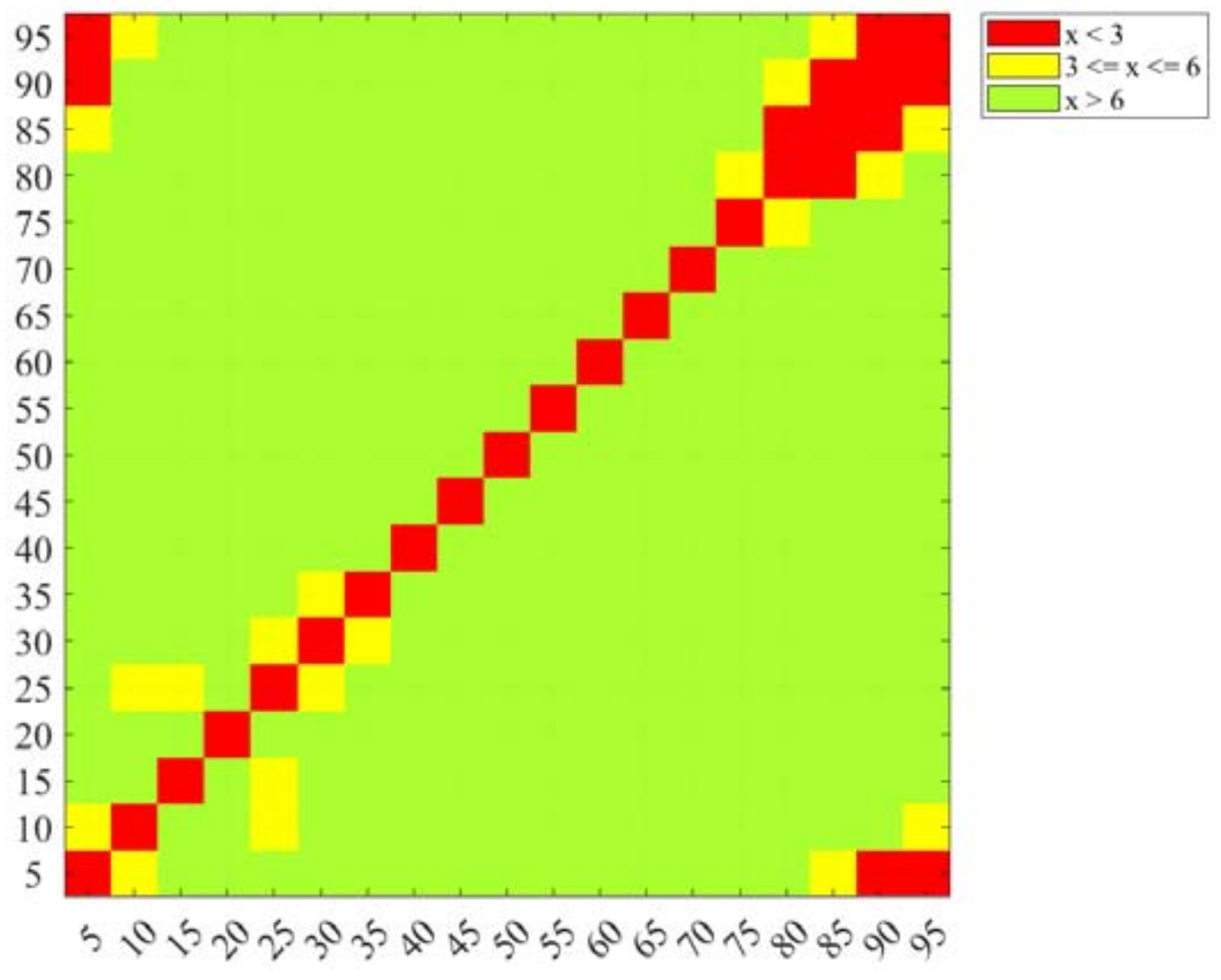
4. Measurements
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Finkenzeller, K. Fundamentals and Applications in Contactless Smart Cards, Radio Frequency Identification and Near-Field Communication, 3rd ed.; Wiley: Chichester, UK; Hoboken, NJ, USA, 2010. [Google Scholar]
- Angeles, R. Rfid Technologies: Supply-Chain Applications and Implementation Issues. Inf. Syst. Manag. 2005, 22, 51–65. [Google Scholar] [CrossRef]
- Liu, G.; Mao, L.; Chen, L.; Xie, S. Locatable-Body Temperature Monitoring Based on Semi-Active UHF RFID Tags. Sensors 2014, 14, 5952–5966. [Google Scholar] [CrossRef] [PubMed]
- Vaz, A.; Ubarretxena, A.; Zalbide, I.; Pardo, D.; Solar, H.; Garcia-Alonso, A.; Berenguer, R. Full Passive UHF Tag With a Temperature Sensor Suitable for Human Body Temperature Monitoring. IEEE Trans. Circuits Syst. II Express Briefs 2010, 57, 95–99. [Google Scholar] [CrossRef]
- Ramos, A.; Clement, P.; Lazaro, A.; Llobet, E.; Girbau, D. Nitrogen Dioxide Wireless Sensor Based on Carbon Nanotubes and UWB RFID Technology. IEEE Antennas Wirel. Propag. Lett. 2015, 14, 1145–1148. [Google Scholar] [CrossRef]
- Vyas, R.; Lakafosis, V.; Lee, H.; Shaker, G.; Yang, L.; Orecchini, G.; Traille, A.; Tentzeris, M.M.; Roselli, L. Inkjet Printed, Self Powered, Wireless Sensors for Environmental, Gas, and Authentication-Based Sensing. IEEE Sens. J. 2011, 11, 3139–3152. [Google Scholar] [CrossRef]
- Occhiuzzi, C.; Marrocco, G. Human body sensing: A pervasive approach by implanted RFID tags. In Proceedings of the 2010 3rd International Symposium on Applied Sciences in Biomedical and Communication Technologies (ISABEL 2010), Rome, Italy, 7–10 November 2010; pp. 1–5. [Google Scholar]
- Jiang, Z.; Fu, Z.; Yang, F. RFID tag antenna based wireless sensing method for medical transfusion applications. In Proceedings of the 2012 IEEE International Conference on RFID-Technologies and Applications (RFID-TA), Nice, France, 5–7 November 2012; pp. 126–130. [Google Scholar]
- Leon-Salas, W.D.; Halmen, C. A RFID Sensor for Corrosion Monitoring in Concrete. IEEE Sens. J. 2016, 16, 32–42. [Google Scholar] [CrossRef]
- Zhang, J.; Tian, G.; Marindra, A.; Sunny, A.; Zhao, A. A Review of Passive RFID Tag Antenna-Based Sensors and Systems for Structural Health Monitoring Applications. Sensors 2017, 17, 265. [Google Scholar] [CrossRef] [PubMed]
- Buffi, A.; Nepa, P.; Lombardini, F. A Phase-Based Technique for Localization of UHF-RFID Tags Moving on a Conveyor Belt: Performance Analysis and Test-Case Measurements. IEEE Sens. J. 2015, 15, 387–396. [Google Scholar] [CrossRef]
- Krigslund, R.; Dosen, S.; Popovski, P.; Dideriksen, J.L.; Pedersen, G.F.; Farina, D. A Novel Technology for Motion Capture Using Passive UHF RFID Tags. IEEE Trans. Biomed. Eng. 2013, 60, 1453–1457. [Google Scholar] [CrossRef] [PubMed]
- Bhattacharyya, R.; Floerkemeier, C.; Sarma, S. Low-Cost, Ubiquitous RFID-Tag-Antenna-Based Sensing. Proc. IEEE 2010, 98, 1593–1600. [Google Scholar] [CrossRef]
- Ruiz-Garcia, L.; Lunadei, L.; Barreiro, P.; Robla, I. A Review of Wireless Sensor Technologies and Applications in Agriculture and Food Industry: State of the Art and Current Trends. Sensors 2009, 9, 4728–4750. [Google Scholar] [CrossRef] [PubMed]
- Potyrailo, R.A.; Nagraj, N.; Tang, Z.; Mondello, F.J.; Surman, C.; Morris, W. Battery-free Radio Frequency Identification (RFID) Sensors for Food Quality and Safety. J. Agric. Food Chem. 2012, 60, 8535–8543. [Google Scholar] [CrossRef] [PubMed]
- Costa, F.; Genovesi, S.; Monorchio, A. A Chipless RFID Based on Multiresonant High-Impedance Surfaces. IEEE Trans. Microw. Theory Tech. 2013, 61, 146–153. [Google Scholar] [CrossRef]
- Vena, A.; Perret, E.; Tedjini, S. Design of Compact and Auto-Compensated Single-Layer Chipless RFID Tag. IEEE Trans. Microw. Theory Tech. 2012, 60, 2913–2924. [Google Scholar] [CrossRef]
- Chamarti, A.; Varahramyan, K. Transmission Delay Line Based ID Generation Circuit for RFID Applications. IEEE Microw. Wirel. Compon. Lett. 2006, 16, 588–590. [Google Scholar] [CrossRef]
- Harma, S.; Arthur, W.G.; Hartmann, C.S.; Maev, R.G.; Plessky, V.P. Inline SAW RFID tag using time position and phase encoding. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2008, 55, 1840–1846. [Google Scholar] [CrossRef] [PubMed]
- Genovesi, S.; Costa, F.; Monorchio, A.; Manara, G. Chipless RFID Tag Exploiting Multifrequency Delta-Phase Quantization Encoding. IEEE Antennas Wirel. Propag. Lett. 2016, 15, 738–741. [Google Scholar] [CrossRef]
- Balbin, I.; Karmakar, N.C. Phase-Encoded Chipless RFID Transponder for Large-Scale Low-Cost Applications. IEEE Microw. Wirel. Compon. Lett. 2009, 19, 509–511. [Google Scholar] [CrossRef]
- Costa, F.; Genovesi, S.; Monorchio, A. Chipless RFIDs for Metallic Objects by Using Cross Polarization Encoding. IEEE Trans. Antennas Propag. 2014, 62, 4402–4407. [Google Scholar] [CrossRef]
- Vena, A.; Perret, E.; Tedjini, S. A compact chipless RFID tag using polarization diversity for encoding and sensing. In Proceedings of the 2012 IEEE International Conference on RFID (RFID), Orlando, FL, USA, 3–5 April 2012; pp. 191–197. [Google Scholar]
- Borgese, M.; Dicandia, F.A.; Costa, F.; Genovesi, S.; Manara, G. An Inkjet Printed Chipless RFID Sensor for Wireless Humidity Monitoring. IEEE Sens. J. 2017, 17, 4699–4707. [Google Scholar] [CrossRef]
- Sauer, S.; Fischer, W.-J. A Passive Wireless Humidity Threshold Monitoring Sensor Principle Based on Deliquescent Salts and a Diffusion Based Irreversible State Change. IEEE Sens. J. 2014, 14, 971–978. [Google Scholar] [CrossRef]
- Nair, R.S.; Perret, E.; Tedjini, S.; Baron, T. A Group-Delay-Based Chipless RFID Humidity Tag Sensor Using Silicon Nanowires. IEEE Antennas Wirel. Propag. Lett. 2013, 12, 729–732. [Google Scholar] [CrossRef]
- Schussler, M.; Kohler, C.; Wiens, A.; Kubina, B.; Mandel, C.; Friedrich, A.; Binder, J.; Jakoby, R. Screen printed chipless wireless temperature sensor tag based on Barium Strontium Titanate thick film capacitor. In Proceedings of the 2014 IEEE SENSORS, Valencia, Spain, 2–5 November 2014; pp. 2223–2226. [Google Scholar]
- Vena, A.; Sydänheimo, L.; Tentzeris, M.M.; Ukkonen, L. A Fully Inkjet-Printed Wireless and Chipless Sensor for CO2 and Temperature Detection. IEEE Sens. J. 2015, 15, 89–99. [Google Scholar] [CrossRef]
- Pöpperl, M.; Adametz, J.; Vossiek, M. Extremely temperature-resistant, polarimetric radar barcode. In Proceedings of the 2017 47th European Microwave Conference (EuMC), Nuremberg, Germany, 10–12 October 2017; pp. 97–100. [Google Scholar]
- Horestani, A.K.; Abbott, D.; Fumeaux, C. Rotation Sensor Based on Horn-Shaped Split Ring Resonator. IEEE Sens. J. 2013, 13, 3014–3015. [Google Scholar] [CrossRef]
- Ebrahimi, A.; Withayachumnankul, W.; Al-Sarawi, S.F.; Abbott, D. Metamaterial-Inspired Rotation Sensor with Wide Dynamic Range. IEEE Sens. J. 2014, 14, 2609–2614. [Google Scholar] [CrossRef]
- Sipal, V.; Narbudowicz, A.Z.; Ammann, M.J. Contactless Measurement of Angular Velocity Using Circularly Polarized Antennas. IEEE Sens. J. 2015, 15, 3459–3466. [Google Scholar] [CrossRef]
- Matbouly, H.E.; Zannas, K.; Duroc, Y.; Tedjini, S. Analysis and Assessments of Time Delay Constrains for Passive RFID Tag-Sensor Communication Link: Application for Rotation Speed Sensing. IEEE Sens. J. 2017, 17, 2174–2181. [Google Scholar] [CrossRef]
- Genovesi, S.; Costa, F.; Borgese, M.; Monorchio, A.; Manara, G. Chipless RFID tag exploiting cross polarization for angular rotation sensing. In Proceedings of the 2016 IEEE International Conference on Wireless for Space and Extreme Environments (WiSEE), Aachen, Germany, 26–28 September 2016; pp. 158–160. [Google Scholar]
- Genovesi, S.; Costa, F.; Borgese, M.; Dicandia, F.A.; Monorchio, A.; Manara, G. Chipless RFID sensor for rotation monitoring. In Proceedings of the 2017 IEEE International Conference on RFID Technology Application (RFID-TA), Warsaw, Poland, 20–22 September 2017; pp. 233–236. [Google Scholar]
- Mata-Contreras, J.; Herrojo, C.; Martín, F. Application of Split Ring Resonator (SRR) Loaded Transmission Lines to the Design of Angular Displacement and Velocity Sensors for Space Applications. IEEE Trans. Microw. Theory Tech. 2017, 65, 4450–4460. [Google Scholar] [CrossRef]
- Borgese, M.; Costa, F.; Genovesi, S.; Monorchio, A.; Manara, G. Multi-frequency polarizarition converter with enhanced angular robustness. In Proceedings of the 2016 IEEE International Symposium on Antennas and Propagation (APSURSI), Fajardo, Puerto Rico, 26 June–1 July 2016; pp. 669–670. [Google Scholar]
- Costa, F.; Gentile, A.; Genovesi, S.; Buoncristiani, L.; Lazaro, A.; Villarino, R.; Girbau, D. A Depolarizing Chipless RF Label for Dielectric Permittivity Sensing. IEEE Microw. Wirel. Compon. Lett. 2018, 28, 371–373. [Google Scholar] [CrossRef]
- Mandel, C.; Jiménez-Sáez, A.; Polat, E.; Schüßler, M.; Kubina, B.; Scherer, T.; Lautenschläger, N.; Jakoby, R. Dielectric ring resonators as chipless temperature sensors for wireless machine tool monitoring. In Proceedings of the 2017 11th European Conference on Antennas and Propagation (EUCAP), Paris, France, 19–24 March 2017; pp. 3912–3916. [Google Scholar]
- Costa, F.; Borgese, M.; Gentile, A.; Buoncristiani, L.; Genovesi, S.; Dicandia, F.A.; Bianchi, D.; Monorchio, A.; Manara, G. Robust Reading Approach for Moving Chipless RFID Tags by Using ISAR Processing. IEEE Trans. Microw. Theory Techn. 2018, 66, 2442–2451. [Google Scholar] [CrossRef]
- Mittra, R.; Chan, C.H.; Cwik, T. Techniques for analyzing frequency selective surfaces—A review. Proc. IEEE 1988, 76, 1593–1615. [Google Scholar] [CrossRef]



© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Genovesi, S.; Costa, F.; Borgese, M.; Dicandia, F.A.; Manara, G. Chipless Radio Frequency Identification (RFID) Sensor for Angular Rotation Monitoring. Technologies 2018, 6, 61. https://doi.org/10.3390/technologies6030061
Genovesi S, Costa F, Borgese M, Dicandia FA, Manara G. Chipless Radio Frequency Identification (RFID) Sensor for Angular Rotation Monitoring. Technologies. 2018; 6(3):61. https://doi.org/10.3390/technologies6030061
Chicago/Turabian StyleGenovesi, Simone, Filippo Costa, Michele Borgese, Francesco Alessio Dicandia, and Giuliano Manara. 2018. "Chipless Radio Frequency Identification (RFID) Sensor for Angular Rotation Monitoring" Technologies 6, no. 3: 61. https://doi.org/10.3390/technologies6030061
APA StyleGenovesi, S., Costa, F., Borgese, M., Dicandia, F. A., & Manara, G. (2018). Chipless Radio Frequency Identification (RFID) Sensor for Angular Rotation Monitoring. Technologies, 6(3), 61. https://doi.org/10.3390/technologies6030061






