Magnetic Stirling Cycle for Qubits with Anisotropy near the Quantum Critical Point
Abstract
1. Introduction
2. Model
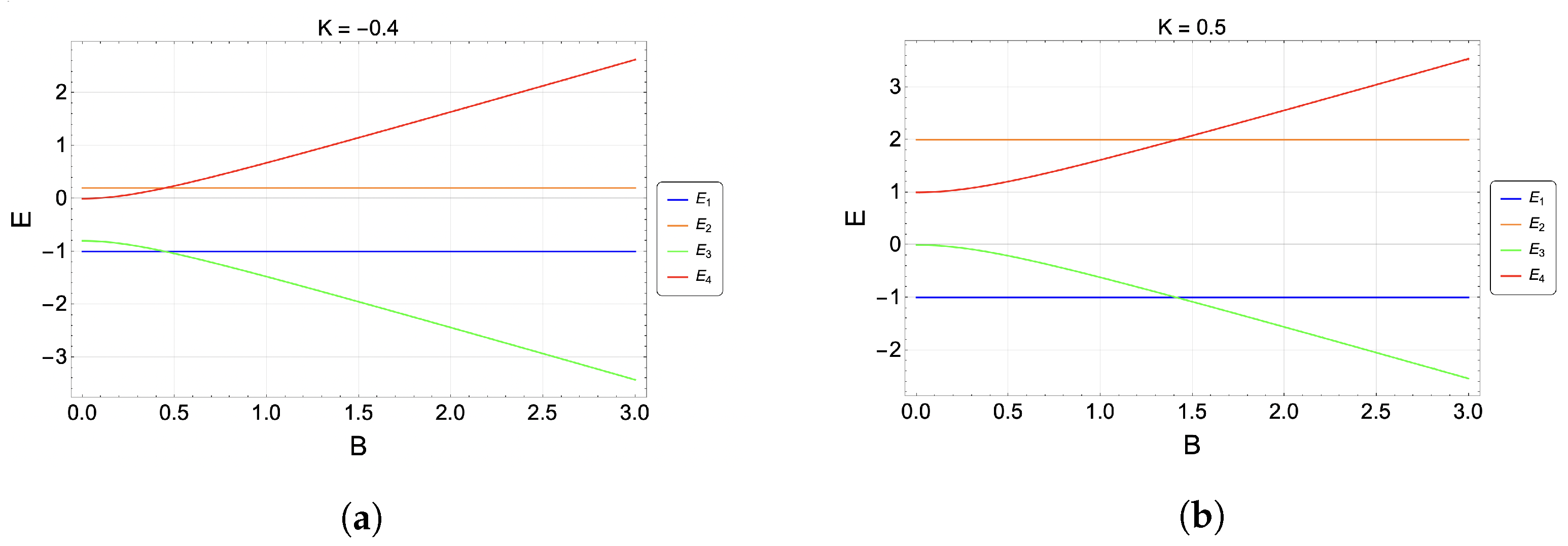
3. Spin Correlations and Quantum Phase Transition
3.1. Spin Correlations
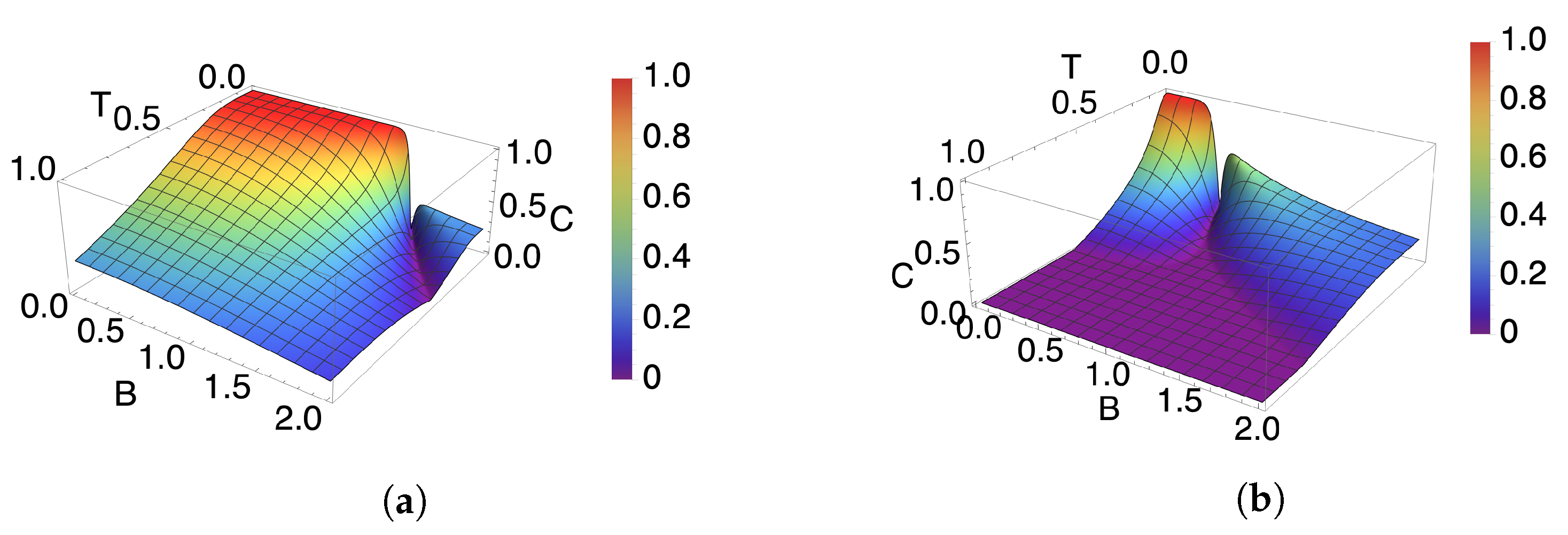
3.2. Quantum Entanglement
3.3. Linking Correlation with Thermodynamics
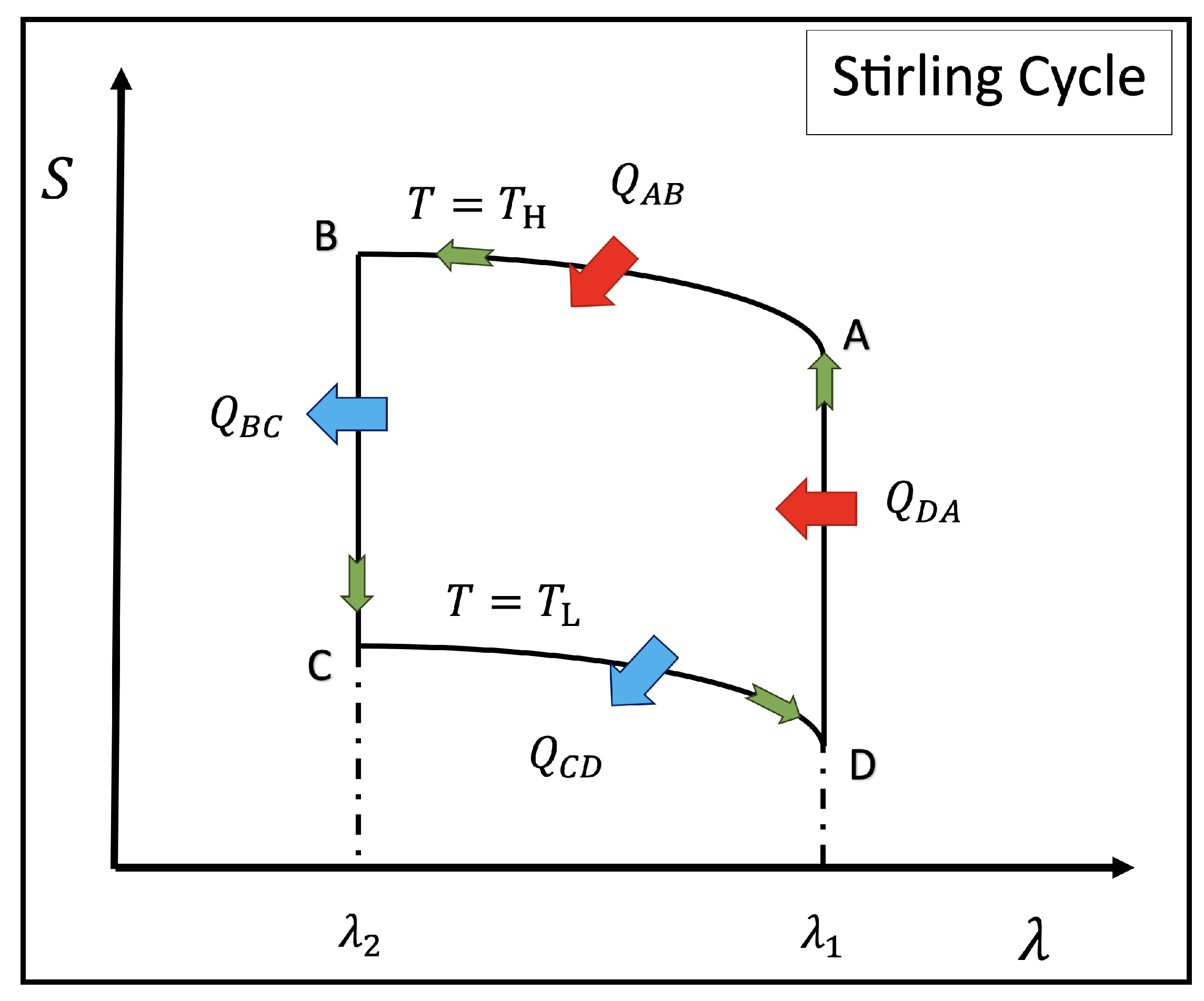
4. Quantum Stirling Cycle
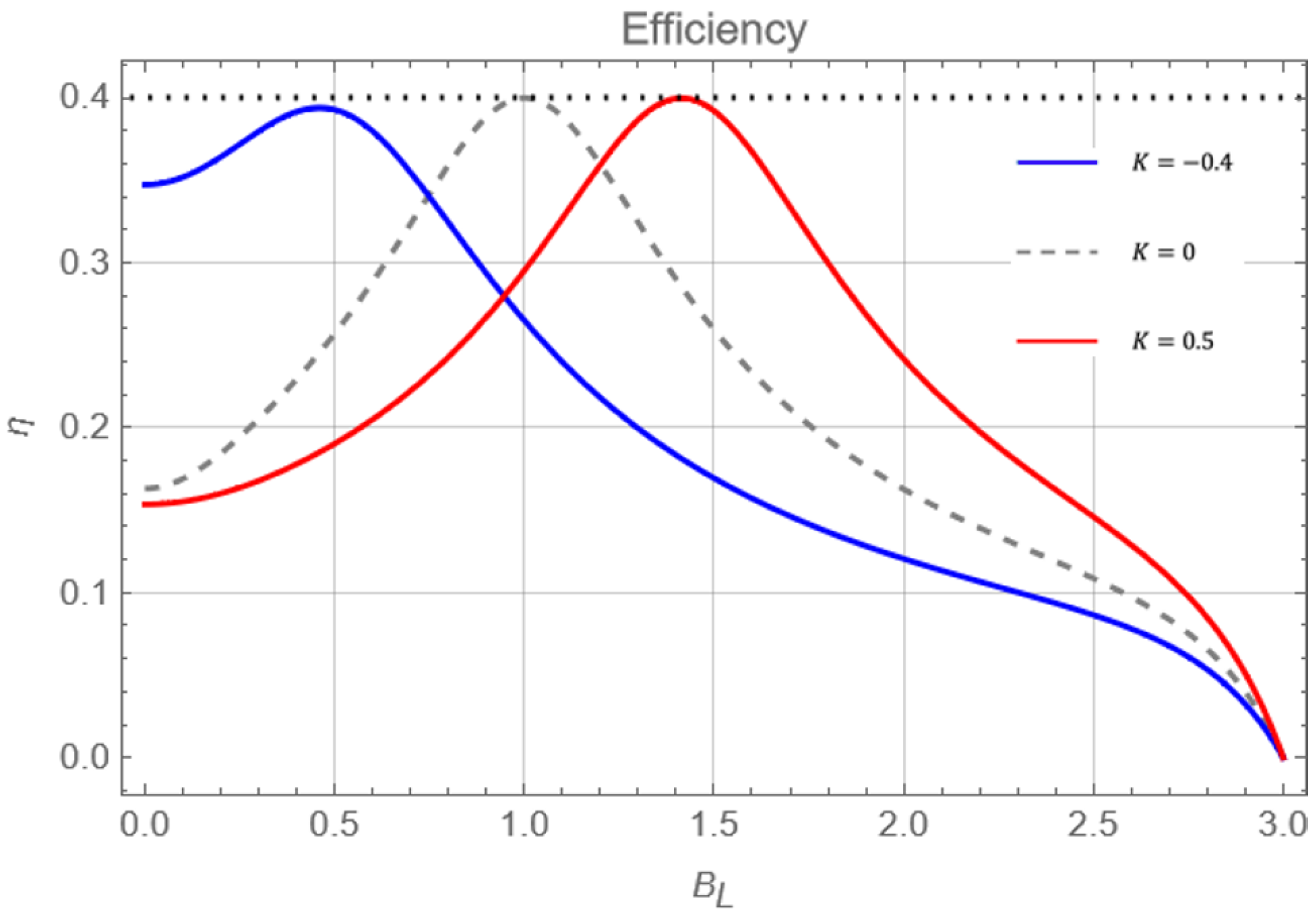
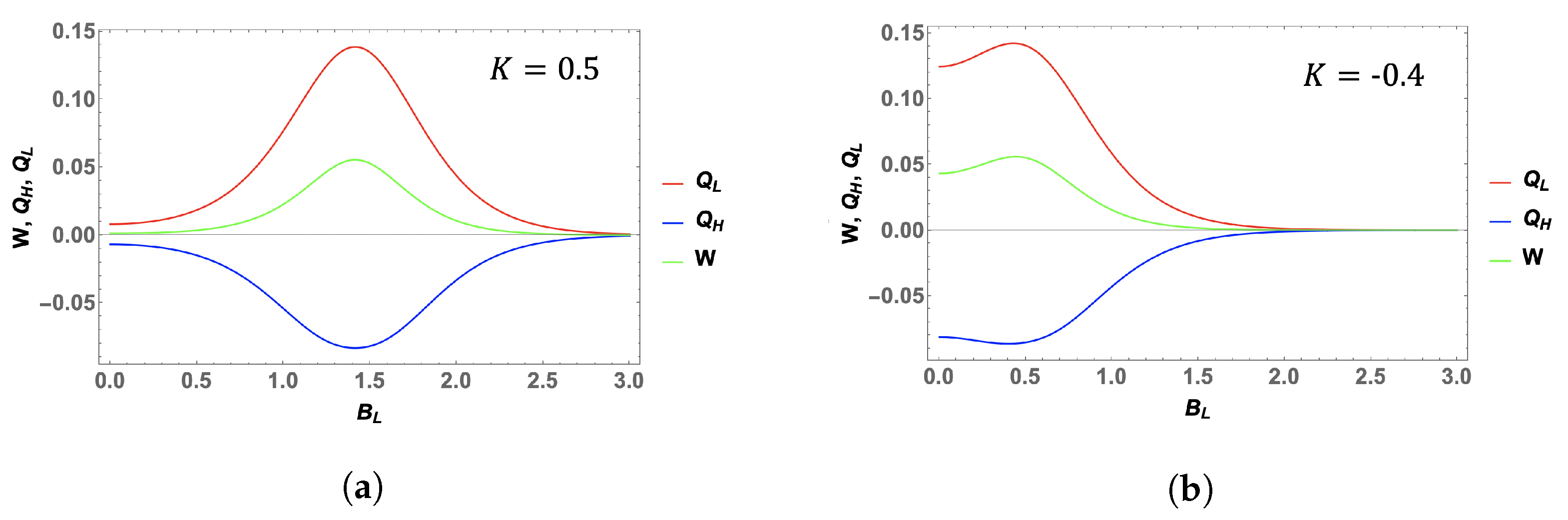
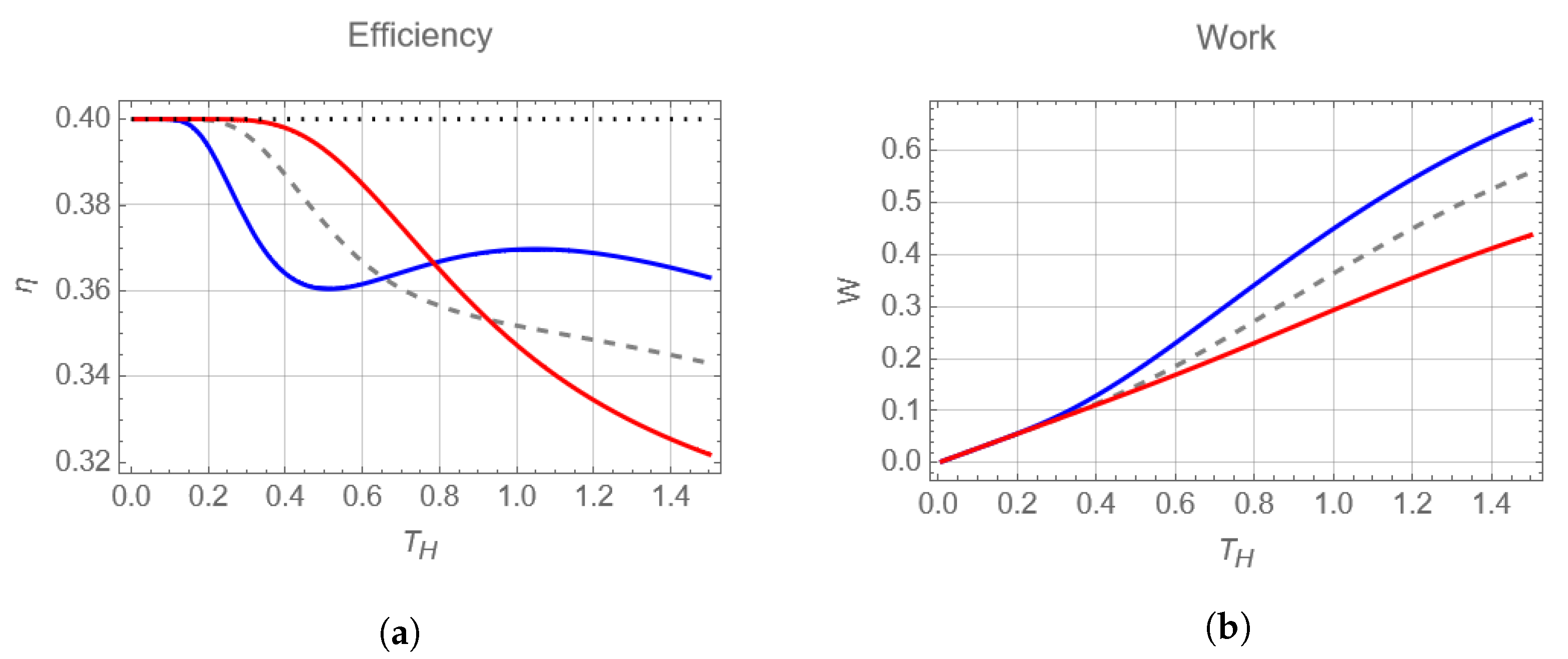
5. Results and Discussion
6. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Geva, E.; Kosloff, R. A quantum-mechanical heat engine operating in finite-time, a model consisting of Spin-1/2 systems as the working fluid. J. Chem. Phys. 1992, 96, 3054–3067. [Google Scholar] [CrossRef]
- Geva, E.; Kosloff, R. On the classical limit of quantum thermodynamics in finite-time. J. Chem. Phys. 1992, 97, 4398–4412. [Google Scholar] [CrossRef]
- Peterson, J.P.S.; Batalhao, T.B.; Herrera, M.; Souza, A.M.; Sarthour, R.S.; Oliveira, I.S.; Serra, R.M. Experimental Characterization of a Spin Quantum Heat Engine. Phys. Rev. Lett. 2019, 123, 240601. [Google Scholar] [CrossRef]
- He, J.; Chen, J. Quantum refrigeration cycles using spin-(1)/(2) systems as the working substance. Phys. Rev. E 2002, 65, 036145. [Google Scholar] [CrossRef]
- Zhang, T.; Liu, W.T.; Chen, P.X.; Li, C.Z. Four-level entangled quantum heat engines. Phys. Rev. A 2007, 75, 062102. [Google Scholar] [CrossRef]
- Henrich, M.J.; Mahler, G.; Michel, M. Driven spin systems as quantum thermodynamic machines: Fundamental limits. Phys. Rev. E 2007, 75, 051118. [Google Scholar] [CrossRef] [PubMed]
- Saygin, H.; Sisman, A. Quantum degeneracy effect on the work output from a Stirling cycle. J. Appl. Phys. 2001, 90, 3086–3089. [Google Scholar] [CrossRef]
- Zhang, G.F. Entangled quantum heat engines based on two two-spin systems with Dzyaloshinski-Moriya anisotropic antisymmetric interaction. Eur. Phys. J. D 2008, 49, 123–128. [Google Scholar] [CrossRef]
- Cakmak, B.; Mustecaplioglu, O.E. Spin quantum heat engines with shortcuts to adiabaticity. Phys. Rev. E 2019, 99, 032108. [Google Scholar] [CrossRef]
- Wu, F.; Chen, L.; Wu, S.; Sun, F.; Wu, C. Performance of an irreversible quantum Carnot engine with spin 1/2. J. Chem. Phys. 2006, 124, 214702. [Google Scholar] [CrossRef]
- Azimi, M.; Chotorlishvili, L.; Mishra, S.K.; Vekua, T.; Huebner, W.; Berakdar, J. Quantum Otto heat engine based on a multiferroic chain working substance. New J. Phys. 2014, 16, 063018. [Google Scholar] [CrossRef]
- Allahverdyan, A.; Gracia, R.; Nieuwenhuizen, T. Work extraction in the spin-boson model. Phys. Rev. E 2005, 71, 046106. [Google Scholar] [CrossRef] [PubMed]
- Henrich, M.J.; Rempp, F.; Mahler, G. Quantum thermodynamic Otto machines: A spin-system approach. Eur. Phys. J. Spec. Top. 2007, 151, 157–165. [Google Scholar] [CrossRef]
- Wang, J.; He, J.; Xin, Y. Performance analysis of a spin quantum heat engine cycle with internal friction. Phys. Scr. 2007, 75, 227–234. [Google Scholar] [CrossRef]
- Chen, J.; Lin, B.; Hua, B. The performance of a quantum heat engine working with spin systems. J. Phys. D-Appl. Phys. 2002, 35, 2051–2057. [Google Scholar] [CrossRef]
- Ono, K.; Shevchenko, S.N.; Mori, T.; Moriyama, S.; Nori, F. Analog of a Quantum Heat Engine Using a Single-Spin Qubit. Phys. Rev. Lett. 2020, 125, 166802. [Google Scholar] [CrossRef]
- Altintas, F.; Mustecaplioglu, O.E. General formalism of local thermodynamics with an example: Quantum Otto engine with a spin-1/2 coupled to an arbitrary spin. Phys. Rev. E 2015, 92, 022142. [Google Scholar] [CrossRef]
- Wu, F.; Chen, L.; Sun, F.; Wu, C.; Hua, P. Optimum performance parameters for a quantum carnot heat pump with spin-1/2. Energy Convers. Manag. 1998, 39, 1161–1167. [Google Scholar] [CrossRef]
- Alecce, A.; Galve, F.; Lo Gullo, N.; Dell’Anna, L.; Plastina, F.; Zambrini, R. Quantum Otto cycle with inner friction: Finite-time and disorder effects. New J. Phys. 2015, 17, 075007. [Google Scholar] [CrossRef]
- Kosloff, R.; Feldmann, T. Optimal performance of reciprocating demagnetization quantum refrigerators. Phys. Rev. E 2010, 82, 011134. [Google Scholar] [CrossRef]
- Katz, G.; Kosloff, R. Quantum Thermodynamics in Strong Coupling: Heat Transport and Refrigeration. Entropy 2016, 18, 186. [Google Scholar] [CrossRef]
- Altintas, F.; Hardal, A.U.C.; Mustecaplioglu, O.E. Quantum correlated heat engine with spin squeezing. Phys. Rev. E 2014, 90, 032102. [Google Scholar] [CrossRef] [PubMed]
- Myers, N.M.; McCready, J.; Deffner, S. Quantum heat engines with singular interactions. Symmetry 2021, 13, 978. [Google Scholar] [CrossRef]
- Purkait, C.; Biswas, A. Performance of Heisenberg-coupled spins as quantum Stirling heat machine near quantum critical point. Phys. Lett. A 2022, 442. [Google Scholar] [CrossRef]
- Zhao, L.-M.; Zhang, G.-F. Entangled quantum Otto and quantum Stirling heat engine based on two-spin systems with Dzyaloshinski-Moriya interaction. Acta Phys. Sin. 2017, 66, 240502. [Google Scholar] [CrossRef]
- Cakmak, S. Benchmarking quantum Stirling and Otto cycles for an interacting spin system. J. Opt. Soc. Am. B-Opt. Phys. 2022, 39, 1209–1215. [Google Scholar] [CrossRef]
- He, J.Z.; He, X.; Zheng, J. Thermal Entangled Quantum Heat Engine Working with a Three-Qubit Heisenberg XX Model. Int. J. Theor. Phys. 2012, 51, 2066–2076. [Google Scholar] [CrossRef]
- Kuznetsova, E.I.; Yurischev, M.A.; Haddadi, S. Quantum Otto heat engines on XYZ spin working medium with DM and KSEA interactions: Operating modes and efficiency at maximal work output. Quantum Inf. Process. 2023, 22, 192. [Google Scholar] [CrossRef]
- Kamta, G.L.; Starace, A.F. Anisotropy and Magnetic Field Effects on the Entanglement of a Two Qubit Heisenberg XY Chain. Phys. Rev. Lett. 2002, 88, 107901. [Google Scholar] [CrossRef]
- Werlang, T.G.; Trippe, C.; Ribeiro, G.A.P.; Rigolin, G. Quantum Correlations in Spin Chains at Finite Temperatures and Quantum Phase Transitions. Phys. Rev. Lett. 2010, 105, 095702. [Google Scholar] [CrossRef]
- Vidal, G.; Latorre, J.; Rico, E.; Kitaev, A. Entanglement in quantum critical phenomena. Phys. Rev. Lett. 2003, 90, 227902. [Google Scholar] [CrossRef] [PubMed]
- Throckmorton, R.E.; Sarma, S.D. Studying many-body localization in exchange-coupled electron spin qubits using spin-spin correlations. Phys. Rev. B 2023, 103, 165431. [Google Scholar] [CrossRef]
- O’Connor, K.M.; Wootters, W.K. Entanglent rings. Phys. Rev. A 2001, 63, 052302. [Google Scholar] [CrossRef]
- Mzaouali, Z.; El Baz, M. Long range quantum coherence, quantum & classical correlations in Heisenberg XX chain. Phys. A-Stat. Mech. Its Appl. 2019, 518, 119–130. [Google Scholar] [CrossRef]
- Vidal, J.; Mosseri, R.; Dukelsky, J. Entanglement in a first-order quantum phase transition. Phys. Rev. A 2004, 69, 054101. [Google Scholar] [CrossRef]
- Leviatan, A. First-order quantum phase transition in a finite system. Phys. Rev. C 2006, 74, 051301. [Google Scholar] [CrossRef]
- Wootters, W.K. Entanglement of formation of an arbitrary state of two qubits. Phys. Rev. Lett. 1998, 80, 2245. [Google Scholar] [CrossRef]
- Norambuena, A.; Franco, A.; Coto, R. From the open generalized Heisenberg model to the Landau–Lifshitz equation. New J. Phys. 2020, 22, 103029. [Google Scholar] [CrossRef]
- Goold, J.; Huber, M.; Riera, A.; del Rio, L.; Skrzypczyk, P. The role of quantum information in thermodynamics—A topical review. J. Phys. A Math. Theor. 2016, 49, 143001. [Google Scholar] [CrossRef]
- Watanabe, S. Information Theoretical Analysis of Multivariate Correlation. IBM J. Res. Dev. 1960, 4, 66–82. [Google Scholar] [CrossRef]
- Horodecki, M.; Oppenheim, J. Fundamental limitations for quantum and nanoscale thermodynamics. Nat. Commun. 2013, 4, 2059. [Google Scholar] [CrossRef] [PubMed]
- Sapienza, F.; Cerisola, F.; Roncaglia, A.J. Correlations as a resource in quantum thermodynamics. Nat. Commun. 2019, 10, 2492. [Google Scholar] [CrossRef] [PubMed]

| Heat and Work | Engine | Refrigerator |
|---|---|---|
| >0 | <0 | |
| <0 | >0 | |
| W | >0 | >0 |
| <1 | - - - - | |
| COP | - - - - | >1 (expected) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Araya, C.; Peña, F.J.; Norambuena, A.; Castorene, B.; Vargas, P. Magnetic Stirling Cycle for Qubits with Anisotropy near the Quantum Critical Point. Technologies 2023, 11, 169. https://doi.org/10.3390/technologies11060169
Araya C, Peña FJ, Norambuena A, Castorene B, Vargas P. Magnetic Stirling Cycle for Qubits with Anisotropy near the Quantum Critical Point. Technologies. 2023; 11(6):169. https://doi.org/10.3390/technologies11060169
Chicago/Turabian StyleAraya, Cristóbal, Francisco J. Peña, Ariel Norambuena, Bastián Castorene, and Patricio Vargas. 2023. "Magnetic Stirling Cycle for Qubits with Anisotropy near the Quantum Critical Point" Technologies 11, no. 6: 169. https://doi.org/10.3390/technologies11060169
APA StyleAraya, C., Peña, F. J., Norambuena, A., Castorene, B., & Vargas, P. (2023). Magnetic Stirling Cycle for Qubits with Anisotropy near the Quantum Critical Point. Technologies, 11(6), 169. https://doi.org/10.3390/technologies11060169








