Aspects of Quantum Gravity Phenomenology and Astrophysics
Abstract
1. Introduction
2. Graviton
2.1. The Linearized Theory of the Graviton
2.2. Gravitons in Loop Quantum Gravity
2.3. Gravitons in Semiclassical Gravity
- (i)
- As the coherent states are non-Abelian in nature, the expectation values of operators have semiclassical corrections which originate due to self-interactions. These can be detected for high-frequency gravitational waves.
- (ii)
- The search for individual “gravitons” or quanta of geometry would require much more precise instruments, able to resolve the coarse-graining of geometry itself.
2.4. Summary
3. Search for Hawking Radiation and Primordial Black Holes
3.1. Formation of Primordial Black Holes (PBH)
3.2. Jean’s Instability
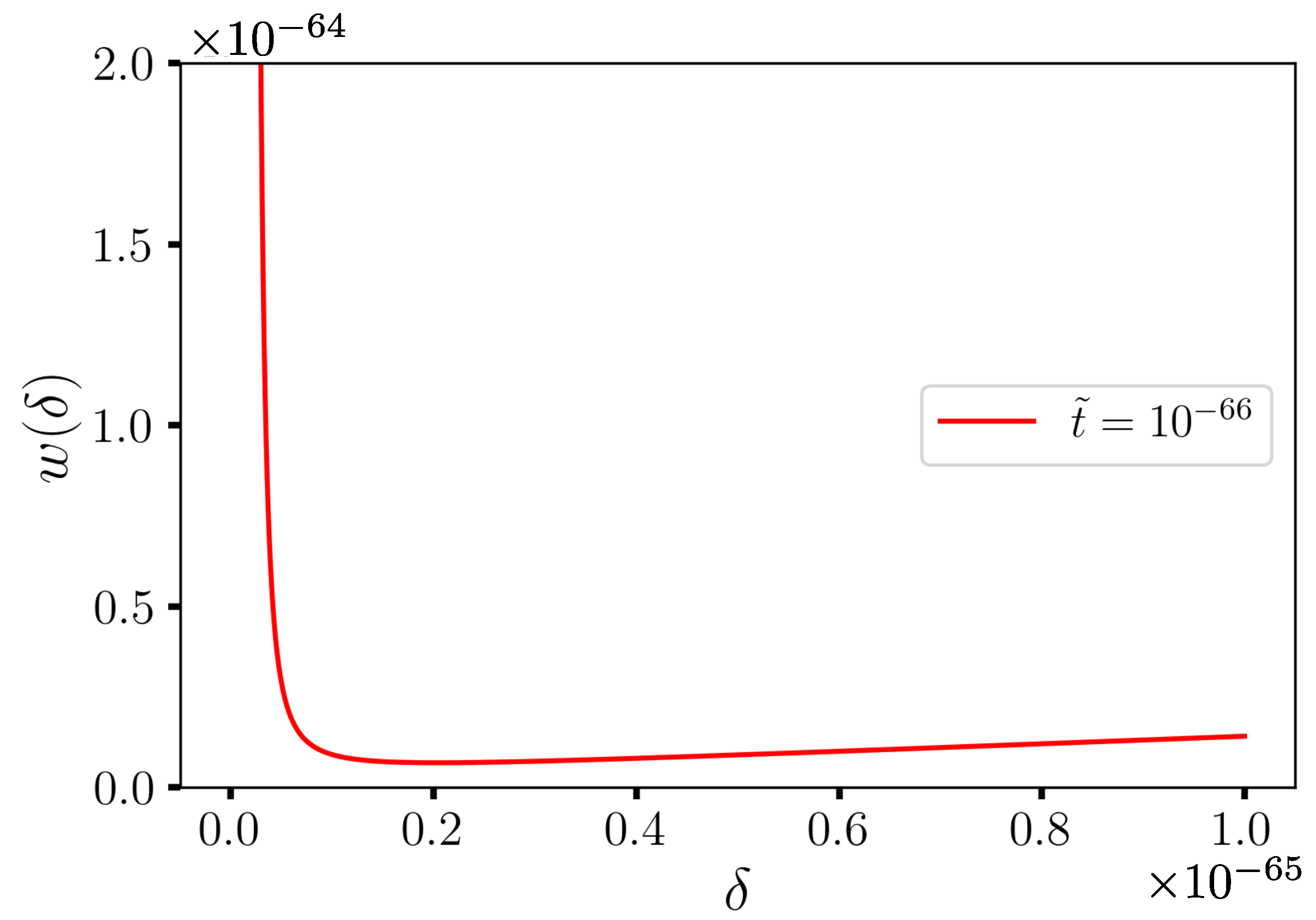
3.3. A Quantum Entropy Production Fluid and Jean’s Instability
3.4. PBH Formation
3.5. Evaporation of PBH
3.6. Archived Data
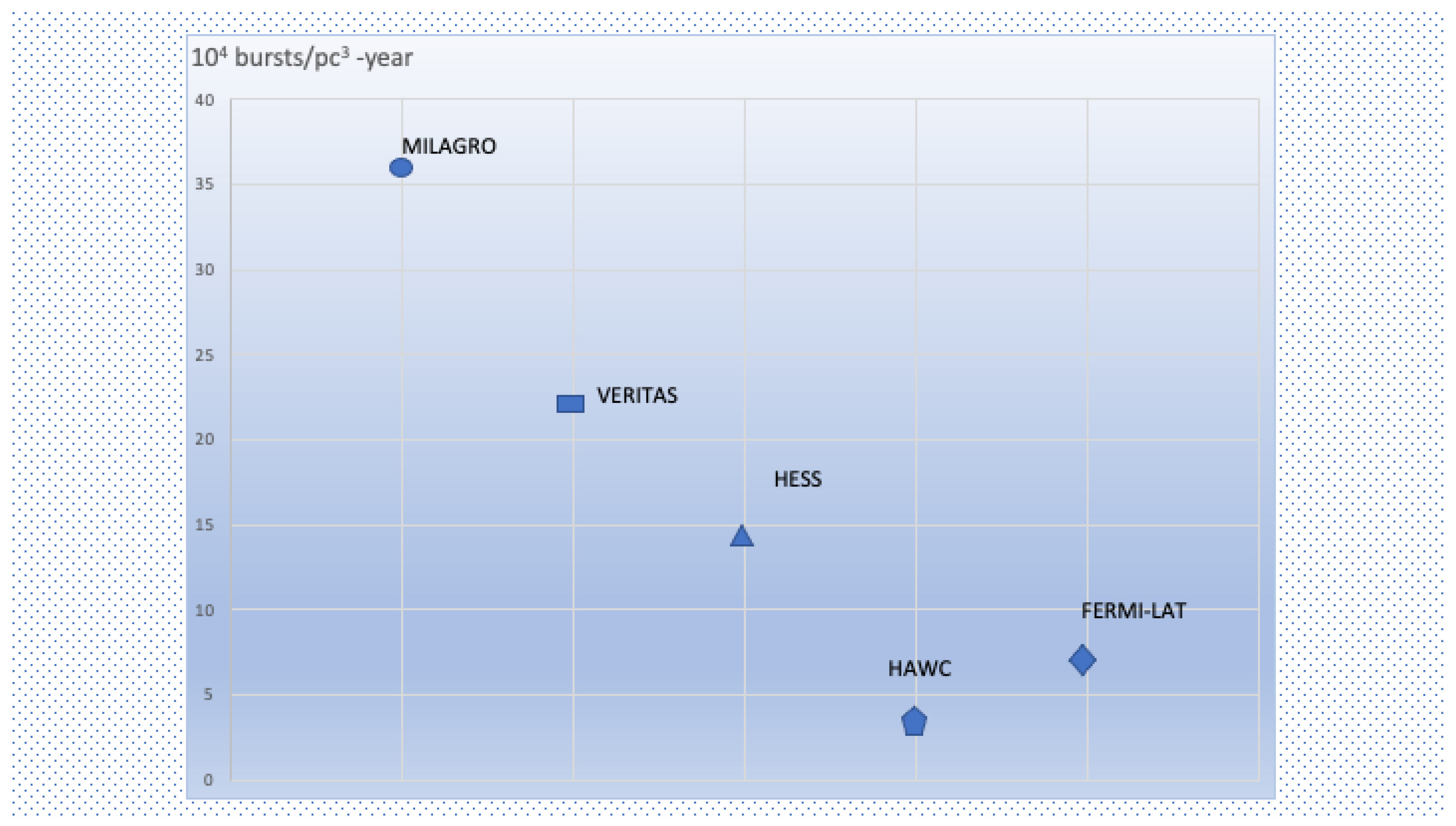
3.7. Gamma-Ray Bursts
3.8. HESS
3.9. Neutrino Experiments
4. Event Horizon
5. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abernathy, M.R.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; et al. LIGO Scientific collaboration and Virgo Collaboration, Observation of Gravitational Waves from a Binary Black Hole Merger. Phys. Rev. Lett. 2016, 116, 061102. [Google Scholar] [CrossRef] [PubMed]
- Shao, L.; Wex, N.; Zhou, S. New graviton mass bound from binary pulsars. Phys. Rev. 2020, D102, 024069. [Google Scholar] [CrossRef]
- Bern, Z. Perturbative Quantum Gravity and its Relation to Gauge Theory, Living Reviews in Relativity. Living Rev. Relativ. 2002, 5, 5. [Google Scholar] [CrossRef] [PubMed]
- Rovelli, C. Loop Quantum Gravity. Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Isi, M.; Farr, W.M.; Giesler, M.; Scheel, M.A.; Teukolsky, S. Testing the Black-Hole Area Law with GW150914. Phys. Rev. Lett. 2021, 121, 011103. [Google Scholar] [CrossRef]
- Escriva, A. PBH formation from spherically symmetric hydrodynamical perturbations: A review. Universe 2022, 8, 66. [Google Scholar] [CrossRef]
- Carr, B.; Kohri, K.; Sendouda, Y.; Yokoyama, J. Constraints on Primordial Black Holes. Rep. Prog. Phys. 2021, 84, 116902. [Google Scholar] [CrossRef]
- Escriva, A.; Kuehnel, F.; Tada, Y. Primordial Black Holes. arXiv 2022, arXiv:2211.05767v3. [Google Scholar]
- Laha, R. Primordial Black Holes as a Dark Matter Candidate Are Severely Constrained by the Galactic Center 511 keV γ-Ray Line. Phys. Rev. Lett. 2019, 123, 251101. [Google Scholar] [CrossRef]
- Dasgupta, B.; Laha, R.; Ray, A. Neutrino and Positron Constraints on Spinning Primordial Black Hole Dark Matter. Phys. Rev. Lett. 2020, 125, 101101. [Google Scholar] [CrossRef]
- Cappelutti, N.; Hasinger, G.; Natarajan, P. Exploring the High-redshift PBH-ΛCDM Universe: Early Black Hole Seeding, the First Stars and Cosmic Radiation Backgrounds. Astro. J. 2022, 926, 205. [Google Scholar] [CrossRef]
- Dasgupta, B.; Laha, R.; Ray, A. Low Mass Black Holes from Dark Core Collapse. Phys. Rev. Lett. 2021, 126, 141105. [Google Scholar] [CrossRef] [PubMed]
- Dasgupta, A. Quantum Gravity Effects on Unstable Orbits in Schwarzschild Space-time. Journ. Cosm. Astro. Phys. 2010, 5, 011. [Google Scholar] [CrossRef]
- Maharana, S.; Dasgupta, A. Semiclassical corrections to the photon orbits of a non-rotating black hole. arXiv 2011, arXiv:2011.00676. [Google Scholar]
- Addazi, A.; Alvarez-Muniz, J.; Alves Batista, R.; Amelino-Camelia, G.; Antonelli, V.; Arzano, M.; Asorey, M.; Atteia, J.L.; Bahamonde, S.; Bajardi, F.; et al. Quantum gravity phenomenology at the dawn of the multi-messenger era—A review. Prog. Part. Nucl. Phys. 2022, 125, 103948. [Google Scholar] [CrossRef]
- Amelino-Camelina, G. Are We at the Dawn of Quantum Gravity Phenomenology. Lect. Notes. Phys. 2000, 549, 1–49. [Google Scholar]
- Alcubierre, M.; Allen, G.; Brügmann, B.; Lanfermann, G.; Seidel, E.; Suen, W.-M.; Tobias, M. Gravitational Collapse of Gravitational Waves in 3D Numerical Relativity. Phys. Rev. 2000, D61, 041501. [Google Scholar] [CrossRef]
- Bishop, N.T.; Rezzolla, L. Extraction of Gravitational Waves in Numerical Relativity. Liv. Rev. Relat. 2016, 19, 2. [Google Scholar] [CrossRef]
- Dewitt, B.S. Quantum Theory of Gravity. III. Applications of the Covariant Theory. Phys. Rev. 1967, 162, 1239. [Google Scholar] [CrossRef]
- Lauscher, O.; Reuter, M. Is Quantum Einstein Gravity Nonperturbatively Renormalizable? Class. Quant. Grav. 2002, 19, 483. [Google Scholar] [CrossRef]
- Ashtekar, A.; Rovelli, C.; Smolin, L. Gravitons and loops. Phys. Rev. 1991, D44, 1740. [Google Scholar] [CrossRef] [PubMed]
- Thiemann, T.; Winkler, O. Gauge field theory coherent states (GCS): II. Peakedness properties. Class. Quant. Grav. 2001, 18, 2561–2636. [Google Scholar] [CrossRef]
- Sahlmann, H. Coupling Matter to Loop Quantum Gravity. Ph.D. Thesis, University of Potsdam, Potsdam, Germany, 2002. [Google Scholar]
- Dasgupta, A. Coherent states for black holes. J. Cosmol. Astropart. Phys. 2003, 8, 004. [Google Scholar] [CrossRef]
- Varadarajan, M. Gravitons from a loop representation of linearized gravity. Phys. Rev. 2002, 66, 024017. [Google Scholar] [CrossRef]
- Varadarajan, M. The graviton vacuum as a distributional state in kinematic loop quantum gravity. Class. Quant. Grav. 2005, 22, 1207. [Google Scholar] [CrossRef]
- Mortuza-Hossain, G. Large volume quantum correction in loop quantum cosmology: Graviton illusion? arXiv 2005, arXiv:gr-qc/0504125. [Google Scholar]
- Hinterleitner, F.; Major, S. Toward loop quantization of plane gravitational waves. Class. Quant. Grav. 2012, 29, 065019. [Google Scholar] [CrossRef]
- Garcia-Chung, A.; Mertens, J.B.; Rastgoo, S.; Tavakoli, Y.; Moniz, P.V. Propagation of quantum gravity-modified gravitational waves on a classical FLRW spacetime. Phys. Rev. D 2021, 103, 084053. [Google Scholar] [CrossRef]
- Fajardo-Montenegro, J.L.; Dasgupta, A. Semiclassical States for Gravitons, work in progress.
- Hall, B. The Segal-Bargmann “Coherent State” Transform for Compact Lie Groups. J. Funct. Anal. 1994, 122, 103. [Google Scholar] [CrossRef]
- Dasgupta, A. Semiclassical Loop Quantum Gravity and Black Hole Thermodynamics. SIGMA 2013, 19, 013. [Google Scholar] [CrossRef]
- Hawking, S.W. Particle creation by black holes. Comm. Math. Phys. 1975, 43, 199. [Google Scholar] [CrossRef]
- Choptuik, M.W. Universality and scaling in gravitational collapse of a massless scalar field. Phys. Rev. Lett. 1993, 70, 12. [Google Scholar] [CrossRef] [PubMed]
- Mcmillan, S. Available online: http://www.physics.drexel.edu/~steve/Courses/Physics-431/jeans_instability.pdf (accessed on 31 January 2023).
- Tomisaka, K. Lecture Notes. Available online: http://th.nao.ac.jp/MEMBER/tomisaka/Lecture_Notes/StarFormation/6/node36.html (accessed on 31 January 2023).
- Prigogine, I.; Geheniau, J.; Gunzig, E.; Nardone, P. Thermodynamics of cosmological matter creation. Proc. Natl. Acad. Sci. USA 1988, 85, 7428. [Google Scholar] [CrossRef] [PubMed]
- Kremer, G.M.; Richarte, M.G.; Teston, F. Jeans instability in a universe with dissipation. Phys. Rev. 2018, D97, 023515. [Google Scholar] [CrossRef]
- Dwivedee, D.; Nayak, B.; Jamil, M.; Singh, L.P.; Myrzakulov, R. Evolution of Primordial Black Holes in Loop Quantum Cosmology. J. Astrophys. Astron. 2014, 35, 97. [Google Scholar] [CrossRef]
- Page, D. Particle emission rates from a black hole: Massless particles from an uncharged, non-rotating hole. Phys. Rev. 1976, D13, 198. [Google Scholar] [CrossRef]
- Page, D.; Hawking, S.W. Gamma rays from primordial black holes. Astrophys. J. 1976, 206, 1–7. [Google Scholar] [CrossRef]
- COMPTEL Data Source. Available online: http://cta.irap.omp.eu/ctools/users/tutorials/howto/comptel/index.html (accessed on 31 January 2023).
- Coogan, A.; Morrison, L. Profumo, Direct Detection of Hawking Radiation from Asteroid-Mass Primordial Black Holes. Phys. Rev. Lett. 2021, 126, 171101. [Google Scholar] [CrossRef]
- AMEGO Collaboration. Available online: https://asd.gsfc.nasa.gov/amego/ (accessed on 31 January 2023).
- Tavernier, T.; Glicenstein, J.-F.; Brun, F. Search for Primordial Black Hole evaporations with H.E.S.S. arXiv 2019, arXiv:1909.01620. [Google Scholar]
- Feldman, G.; Cousins, R.J. A Unified Approach to the Classical Statistical Analysis of Small Signals. Phys. Rev. 1998, D57, 3873. [Google Scholar] [CrossRef]
- Lopez-Coto, R.; Doro, M.; de Angelis, A.; Mariotti, M.; Harding, J.P. Prospects for the observation of Primordial Black Hole evaporation with the Southern Wide field of view Gamma-ray Observatory. J. Cosmol. Astropart. Phys. 2021, 8, 040. [Google Scholar] [CrossRef]
- Engel, K.; Peisker, A.; Harding, P.; Wood, J.; Martinez-Castellanos, I.; Albert, A.; Tollefson, K.; on behalf of HAWC Collaboration. Setting Upper Limits on the Local Burst Rate Density of Primordial Black Holes Using HAWC, PoS ICRC2019 516 (2021). Available online: https://inspirehep.net/literature/1820186 (accessed on 31 January 2023).
- Korwar, M.; Profumo, S. Updated Constraints on Primordial Black Hole Evaporation. arXiv 2023, arXiv:2302.04408. [Google Scholar]
- HESS Experiment. Available online: https://www.mpi-hd.mpg.de/hfm/HESS/ (accessed on 31 January 2023).
- Bernal, N.; Chu, X.; Garcia-Cely, C.; Hambye, T.; Zaldivar, B. Production Regimes for Self-Interacting Dark Matter. J. Cosmol. Astropart. Phys. 2022, 10, 68. [Google Scholar] [CrossRef]
- Zhang, Z.H.; Yang, L.T.; Yue, Q.; Kang, K.J.; Li, Y.J.; An, H.P.; Greeshma, C.; Chang, J.P.; Chen, Y.H.; Cheng, J.P.; et al. Search for keV–MeV Light Dark Matter from Evaporating Primordial Black Holes at the CDEX-10 Experiment. arXiv 2022, arXiv:2211.07477v1. [Google Scholar]
- Barrau, A.; Martineau, K.; Moulin, F. A Status Report on the Phenomenology of Black Holes in Loop Quantum Gravity: Evaporation, Tunneling to White Holes, Dark Matter and Gravitational Waves. Universe 2018, 4, 102. [Google Scholar] [CrossRef]
- Event Horizon Telescope Collaboration. Available online: http://eventhorizontelescope.org/ (accessed on 31 January 2023).
- Event Horizon Telescope Collaboration. First M87 Event Horizon Telescope Results. I. The Shadow of the Supermassive Black Hole. Astrophys. Phys. J. Lett. 2019, 875. [Google Scholar] [CrossRef]
- Cardoso, V.; Pani, P. Testing the nature of dark compact objects: A status report. Living Rev. Relativ. 2019, 22, 4. [Google Scholar] [CrossRef]
- The Event Horizon Telescope Collaboration. First M87 Event Horizon Telescope Results. VIII. Magnetic Field Structure near The Event Horizon. Astrophys. J. Lett. 2021, 910, L12. [Google Scholar] [CrossRef]
- The LISA Space Telescope. Available online: https://lisa.nasa.gov/ (accessed on 31 January 2023).


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dasgupta, A.; Fajardo-Montenegro, J. Aspects of Quantum Gravity Phenomenology and Astrophysics. Universe 2023, 9, 128. https://doi.org/10.3390/universe9030128
Dasgupta A, Fajardo-Montenegro J. Aspects of Quantum Gravity Phenomenology and Astrophysics. Universe. 2023; 9(3):128. https://doi.org/10.3390/universe9030128
Chicago/Turabian StyleDasgupta, Arundhati, and José Fajardo-Montenegro. 2023. "Aspects of Quantum Gravity Phenomenology and Astrophysics" Universe 9, no. 3: 128. https://doi.org/10.3390/universe9030128
APA StyleDasgupta, A., & Fajardo-Montenegro, J. (2023). Aspects of Quantum Gravity Phenomenology and Astrophysics. Universe, 9(3), 128. https://doi.org/10.3390/universe9030128






