1. Introduction
Radio radiation has been detected in all star types across the Hertzsprung–Russell diagram [
1]. There exist approximately 150 eclipsing binaries that displayed radio emission. Stellar radio radiation provides the most direct probes of physical processes, including magnetic activity, particle acceleration, stellar disk and mass transport [
2]. Early astronomy studies were based on the observation of visible bands because the study of astrophysics is dependent on the capabilities of the detection equipment. Radio technology, such as Very Large Array (VLA) [
3], is improving with the advent technology, which allows the nature of radio to be studied. Early radio observations were influenced by many factors, for example, instrumentation and background radiation. Kellermann and Pauliny-Toth [
4] observed that the radio signal of the star named
Ori about 0.11 ± 0.03 Jy in the 2.8 cm band under such condition. Then, Seaquist [
5] obtained the radio intensity of four stars in the 2.85 cm band. Güdel [
1] presented a radio Hertzsprung-Russell diagram between 1 and 10 GHz based on a radio catalog with 3021 radio stars [
6]. Later, Wendker [
7] updated it to 3699 radio stars in 2001, and revealed the ubiquity of stellar radio emissions.
Obtaining the parameters of the eclipsing binaries with radio emissions is required for optical and radio observations. We selected three eclipsing binaries and revised their stellar parameters. The introduction of these three binaries (V Crt, WY Cnc, and CG Cyg) with radio radiations is as follows:
V Crt is a conventional semi-detached eclipsing binary with a period of 0.702 d, and was listed as a variable star [
8]. Parthasarathy and Sanwal [
9] obtained the light curve of photoelectric photometry in the B and V bands. V Crt was detected at a radio intensity close to 25 mJy at 10,600 MHz [
10]. Liu [
11] has studied more detail and obtained the orbit parameter of V Crt. Sarma and Vivekananda Rao [
12] updated its stellar parameters with a mass ratio of q = 0.4, which is smaller than the result of 0.683 [
11].
WY Cnc is an RS CVn type detached eclipsing binary with a period of 0.8293686 d [
13], which shows strong photospheric starspot activity and chromospheric activity emissions in the H
, and CaII HK lines. It exhibits radio emissions of 0.4 mJy at 4860 MHz and 0.23 mJy at 4885 MHz [
7]. It was first defined as an Algol eclipsing system [
14]. Rao and Sarma [
15] performed preliminary statistics on the total brightness and discovered light curve distortion outside the eclipse. Subsequently, a systematic photometric study was conducted [
16,
17,
18]. Xie et al. [
19] analyzed the position of a spot on the photospheric surface, and found a starspot variation outside the eclipse. Helfand et al. [
20] obtained a radio intensity of 0.4 to 0.5 mJy using the VLA FIRST survey. Hall et al. [
21] observed a decreasing orbital period. Later, Albayrak et al. [
22] found that WY Cnc has a third body with a possible light time effect of approximately 85 yr. Recently, many astronomers have obtained more light minimum [
23,
24,
25,
26,
27,
28,
29,
30,
31,
32,
33,
34]. Kjurkchieva et al. [
35] revised the orbital parameters based on spectroscopic and photometric observations. Tian et al. [
36] analyzed O-C and argued that the periodic change was because of the angular momentum loss of magnetic braking. Chen [
37] analyzed the angular momentum loss and suggested that the period change may be due to magnetic braking or circum-binary disk, but also requires too strong magnetic field strength. Long-term photometric monitoring is therefore required to discuss the orbital period of WY Cnc.
CG Cyg is an RS CVn binary consisting of two dwarf components and has a period of 0.6311 d, which was discovered to be a variable star [
38]. There is a radio emission of 25 mJy at 10,600 MHz [
7]. Naftilan and Milone [
39] obtained the spectral type of CG Cyg with G9V and K3V. The remarkable intrinsic variations was observed both outside and within the eclipse [
40,
41,
42,
43,
44,
45,
46,
47,
48,
49,
50,
51], which were explained the starspot models [
42,
51,
52,
53,
54]. The period of CG Cyg was increased using light curve minima [
44]. Later, Albayrak et al. [
22] calculated the period variation using a model of a third-body orbit with period of 46.54 yr by fitting the light curve minimum. Afsar et al. [
55] revised the period cycle of 51 or 22.5 yrs, which was caused by magnetic activity. Shi et al. [
56] estimated that the period increased by 2.48 × 10
d yr
.
Because V Crt, WY Cnc and CG Cyg are short period eclipsing binaries with magnetic activity and radio radiation, we performed photometric observations using an optical telescope, and revise their orbital parameters. Further, we also collected the light curve minimum times of eclipsing binaries to analyze their period variation and discussed their physical mechanisms.
2. Radio Eclipsing Binaries and Photometric Follow-Up Observations
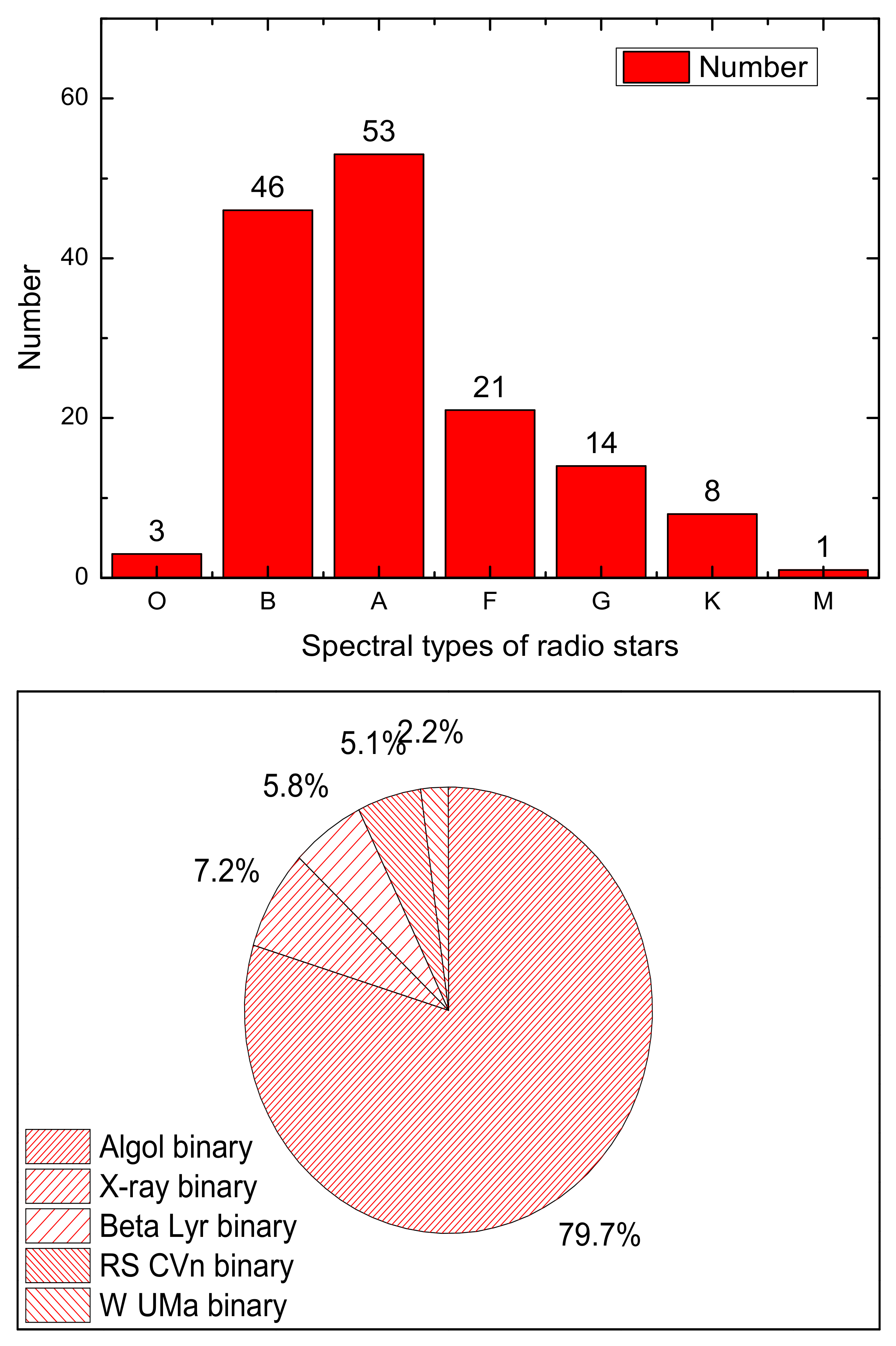
We found 150 eclipsing binaries from the updated catalogue of 3699 radio stars [
7]. We collected their respective stellar parameters, including the coordinate, spectral types, stellar type, radio intensities at different wavelengths (1465 MHz, 1490 MHz, and 4860 MHz) and listed them in
Table 1. The full table will be published in electric format. There are different type eclipsing binaries, such as Algol eclipsing binary, X-ray binaries, RS CVn binary, and so on). V Crt is one of the semi-detached binaries and belonged to the Beta Lyr binary type. We calculated the number of spectral types and binary types using the eclipse binaries exhibiting radio emission. The results are shown in
Figure 1. As shown in
Figure 1, most of the eclipsing binaries with radio emission are B and A spectral-type stars, whose radio radiation might be explained using the stellar wind. By contrast, the radio radiations of eclipsing binaries with spectral-types FGKM are likely caused by stellar magnetic activity [
1,
2].
From the radio eclipsing binaries, we selected three objects (V Crt, CG Cyg, and WY Cnc) with short orbital periods to discuss their physical properties. We analyzed their stellar physical parameters via photometric method using an optical telescope. Our photometric observation for V Crt was conducted using the 60 cm optical telescope at the Southeastern Association for Research in Astronomy (SARA) in Chile on 27 February, 28 May, and 2 March 2020. They were conducted in four BVRI bands using a charge-coupled device (CCD) with resolution of a 1024 × 1024 pixels with BVRI filters of a Bessel system with a 13’ × 13’ field. The limit magnitude with an S/N of 10 is approximately 19 mag of V filter in 10 min [
57]. For WY Cnc and CG Cyg, the CCD photometric observation data in BVRI were obtained using 85 cm optical telescope at the Xinglong station of the National Astronomical Observatories of China (NAOC). The detector used 1024 × 1024 pixels [
58]. The photometric data of WY Cnc was observed on 25 and 30 December 2009, and 4, 6 and 7 January 2010, while CG Cyg was observed on 1, 2, and 3 October 2009. The CCD photometric images were reduced using the IRAF(IRAF is distributed by the National Optical Astronomy Observatories, which are operated by the Association of Universities for Research in Astronomy, Inc., under cooperative agreement with the National Science Foundation.) package. The photometric data obtained after processing are listed in
Table 2. TYC 6085-1462-1, 2-MASS J11240146-1639367 and NSV 25409 are the comparison stars of V Crt, WY Cnc and CG Cyg, respectively. The
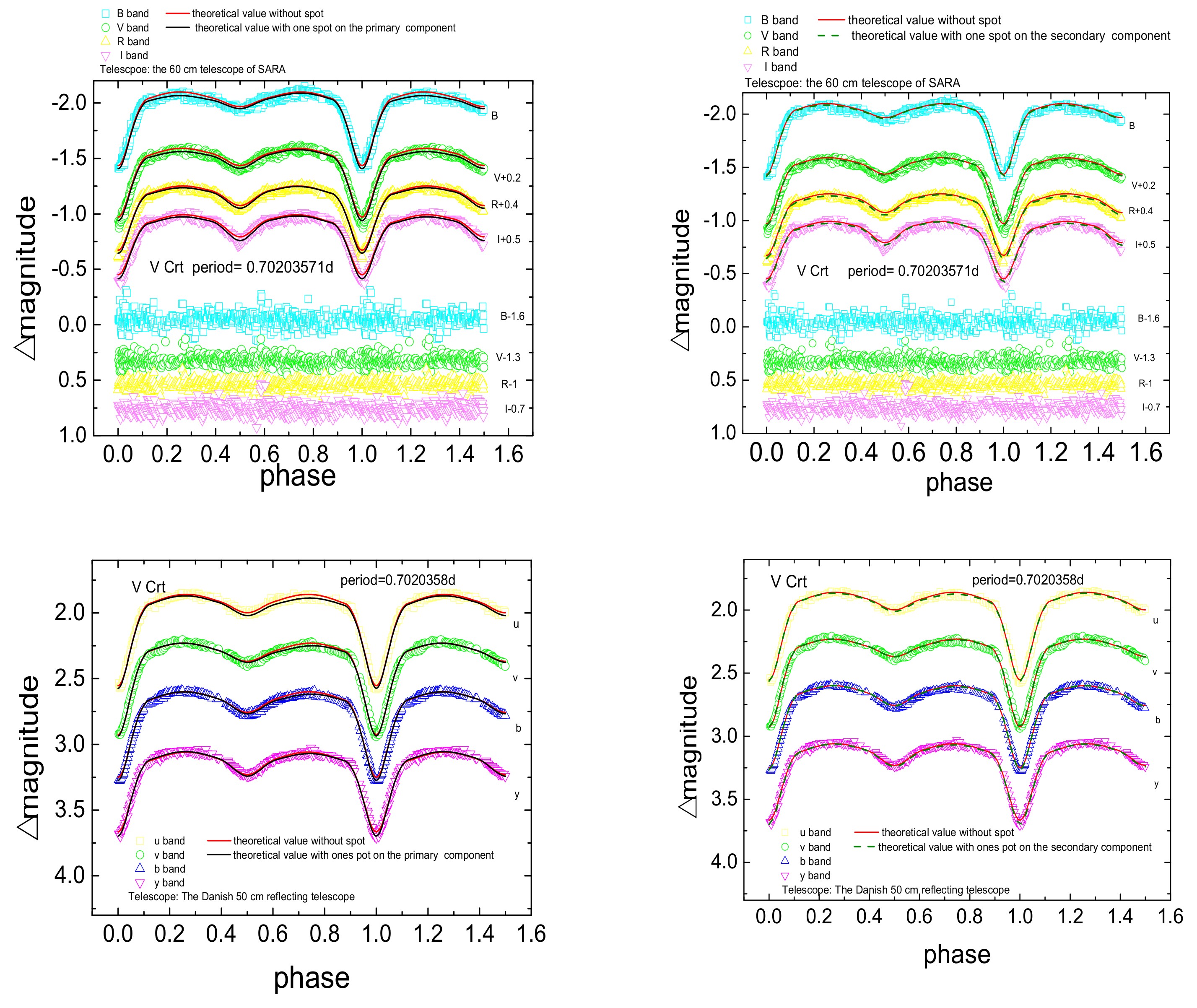
magnitude of the light curves of V Crt, CG Cyg, and WY Cnc obtained from our observations are plotted in
Figure 2,
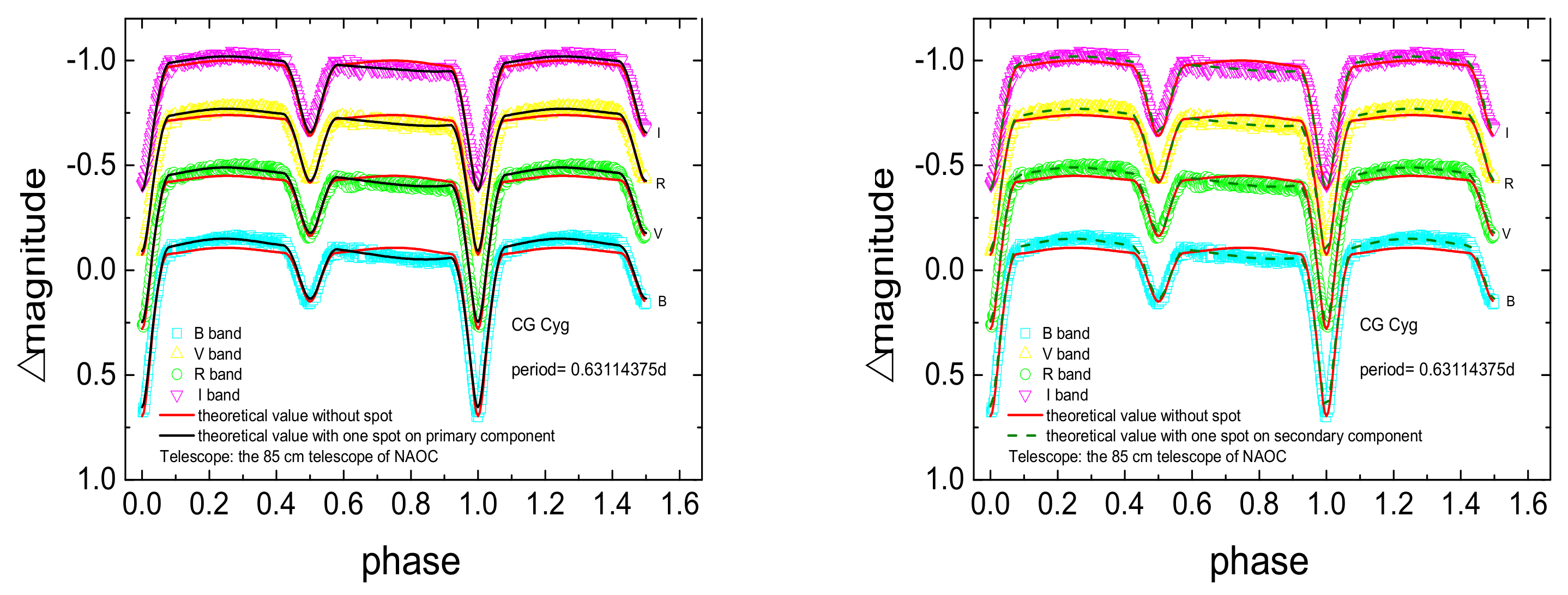
Figure 3 and
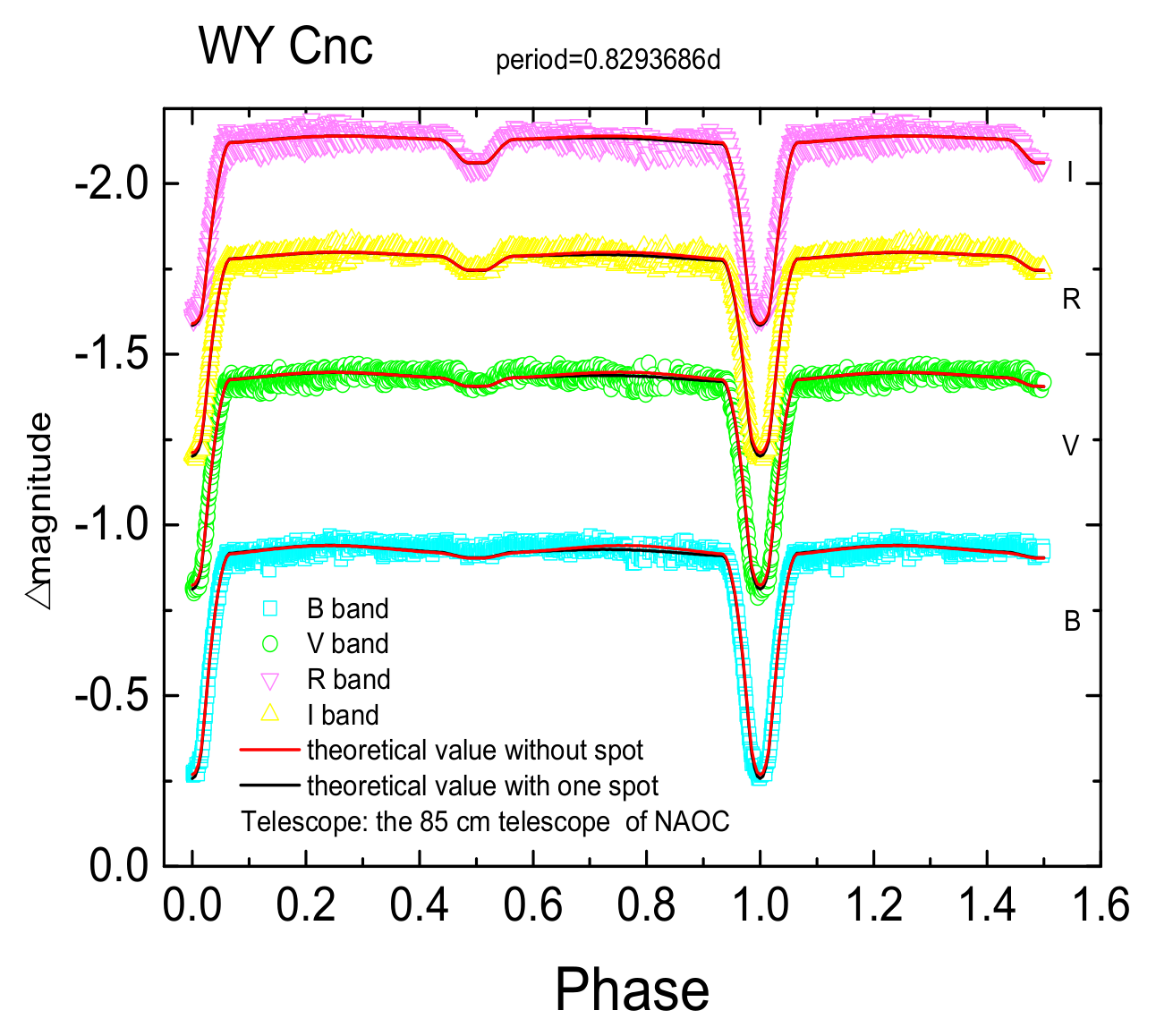
Figure 4, respectively.
4. Orbital Parameters and Starspots
We used the updated 2014 Wilson-Devinney program [
128,
129,
130,
131] to revise the orbital parameters of the eclipsing binaries. The Wilson-Devinney program is the most widely used for light curve modeling code to obtain the stellar and starspot parameters of eclipsing binary. This program uses a different correction method for parameter adjustment of the observed light and velocity curves by the lease squares criterion, and produced the corresponding theoretical curves. We set V Crt as a semi-detached binary in the WD program according to previous research [
11]. We set 7500 K for the primary component, 5000 K for the secondary component, a mass ratio of the secondary component and primary component (q) of 0.683 as the initial value. Further, we also set the bolometric albedo coefficient
=
= 0.5 [
132] and gravity-darkening coefficients
=
= 0.32 [
133]. The bolometric limb-darkening coefficients
and limb-darkening coefficients for the four BVRI bands are
= 0.469,
= 0.429;
= 0.558,
= 0.864,
= 0.471,
= 0.725,
= 0.375,
= 0.599,
= 0.294,
= 0.489, respectively based on the stellar temperature [
134]. We attempted the calculation using several sets of mass ratios to obtain the best rate if the mass ratio was unknown. The temperature was obtained using J-H color index relation [
135] or spectral type. We simultaneously adjusted with other parameters: orbital inclination (
i), temperature of secondary component (
), monochromatic luminosity of the primary component (
,
,
and
), and dimensionless potentials of the two components (
and
). We adjusted each parameters for converge to obtain the best solution by several steps. We used the weighted sum of squares of the residual between the theoretical and observed light curves to judge the best results.
The corresponding orbital parameters obtained from several calculations are listed in
Table 5. The theoretical and observed light curves are demonstrated in
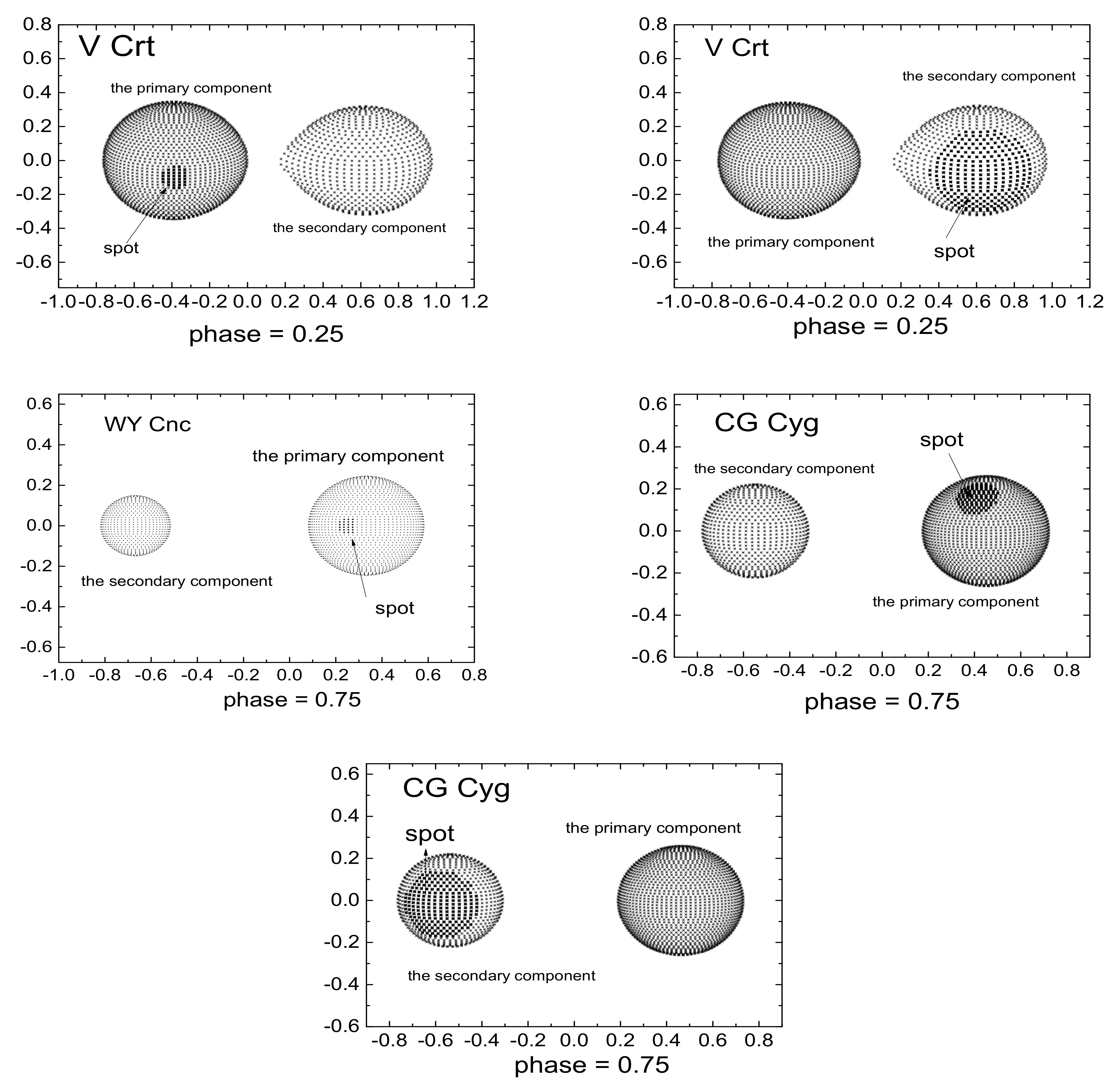
Figure 2. The stellar structure is displayed in
Figure 6. An obvious asymmetry was observed in the light curve. We attempted to add a cold spot on the primary or secondary components to explain this. We assumed the spot shape is circular. The spot longitude, radius and temperature are the free parameters. The starspot parameters were appropriately adjusted until they converge. The weighted sum of squares of the residual between the theoretical and observed light curves was used to judge the best results. Before fitting the spot parameters, we must set the initial value, generally, the latitude is fixed at 90
to simplify the model. The spot temperature factor (Tspot/Tstar) was set to 0.8 because the cold spot was approximately 1000 K below the stellar total surface temperature, and the radius was set equal to the phase range where the deviation of the light curves occurs. The spot longitude was determined using the center of the light curve distortion. The spot radius was estimated by the fitting the observed light curve. Because the spot size is correlated with stellar temperature, and the spot latitude is correlated with spot radius. We have to adjust the spot parameters for many runs to determine the converge solutions. We obtained the best solution with the lowest sum of squares of the residual between the theoretical and observed light curves. The details of the fitting of these parameters have been described in the previous paper [
136]. Initially, we added a cold spot at the secondary component but the result is not very good. We suppose that that the primary star is gaining mass because the secondary Roche lobe was filled as a semi-detached binary system. Hence, we changed the spot at primary component. Finally, we obtained the two solutions of a spot on the primary and secondary component. The
was 6.614 for the spot on primary component and 7.0947 for the spot on the secondary component. We found that there are no significantly different for the two solutions, as shown in
Figure 2. We also attempted to use two spots to explain the light curve distortion. However, there are no significantly improvements of the residual. For our light curve, there were no significant improvements of the residuals. The longitude of the spot on the primary component was
. The spot radius was
and the spot temperature was 6763 K ± 909 K. The spot parameters are listed in
Table 6.
We used a similar method to obtain orbital and starspot parameters for WY Cnc and CG Cyg. We set the temperature of the primary component of WY Cnc to 5500 K and set the secondary temperature to 4000 K [
35]. Based on the temperatures of both components, we determined the bolometric limb-darkening coefficients
and limb-darkening coefficients for BVRI four bands to be
= 0.520,
= 0.429;
= 0.781,
= 0.817,
= 0.644,
= 0.705,
= 0.533,
= 0.612,
= 0.438,
= 0.476, respectively. For CG Cyg, we set the temperature of the primary component to 5200 K and that of the secondary component to 4400 K [
51]. The corresponding bolometric limb-darkening coefficients
and limb-darkening coefficients for the BVRI bands were
= 0.530,
= 0.532;
= 0.822,
= 0.944,
= 0.684,
= 0.795,
= 0.566,
= 0.658,
= 0.464,
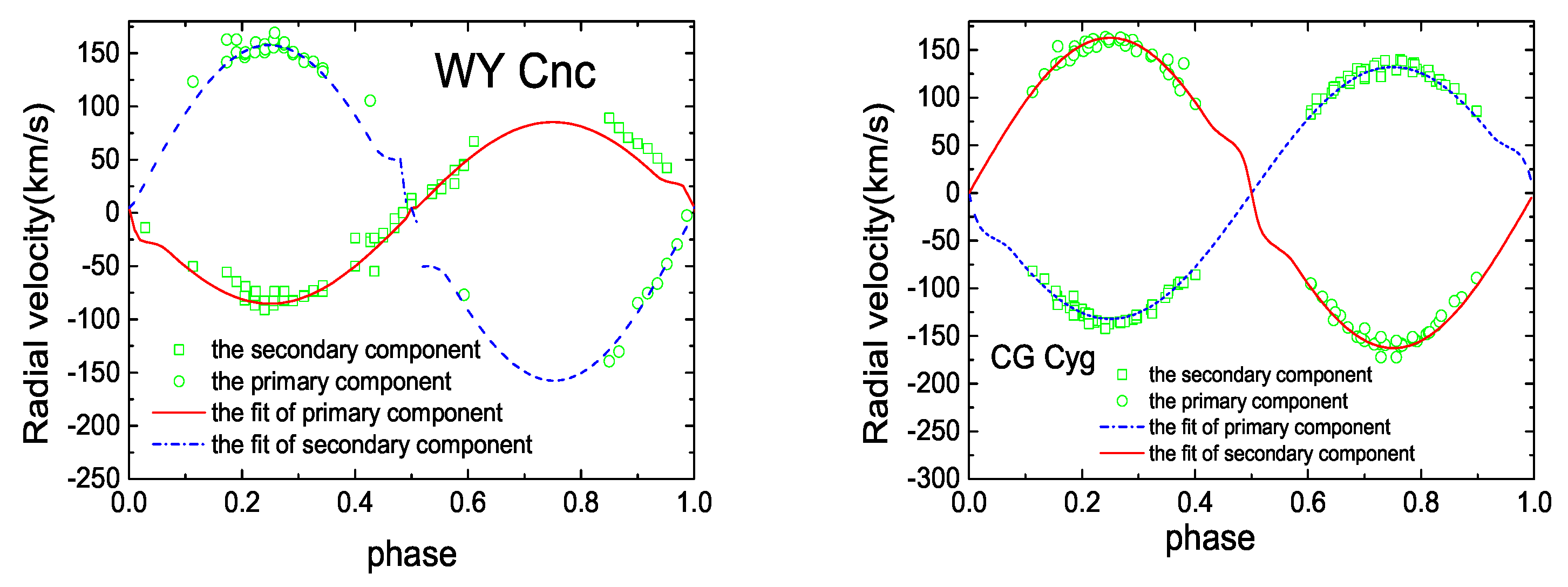
= 0.532, respectively. We collected radial velocity values of WY Cnc [
35] and CG Cyg [
51]. We analyzed the published radial velocities and light curve to obtain orbital parameters using the WD program. The resultant light curves are shown in
Figure 3 and
Figure 4 for WY Cnc and CG Cyg. The orbital parameters are listed in
Table 5 and the spot parameters are listed in
Table 6. Further, we plotted the theoretical and observational radial velocity curves in
Figure 7. The starspot structures of WY Cnc and CG Cyg are displayed in
Figure 6.
5. Discussions and Conclusions
The photometric orbital parameters of V Crt were obtained using the WD program. The mass ratio and orbital inclination were 0.637 ± 0.004 and 73.629
± 0.067, respectively, which are similar to the results of the mass ratio of 0.683 ± 0.002 and inclination of 73.08
± 0.05 [
11]. The luminosity ratio of the primary component and total intensity of V Crt in the difference BVRI bands were 0.9263 ± 0.0026, 0.8721 ± 0.0035, 0.8221 ± 0.0042 and 0.7759 ± 0.0046, respectively. The dimensionless potential of V Crt was
=
= 3.489 ± 0.014. The mass of primary component of V Crt was obtained as
= 1.68 M
based on the relationship between mass and color index. The mass of the secondary component
was 1.07 M
. The semi-major axis
a was obtained using the Kepler’s third law (
). We calculated the radius (R) of each component using
, where r
is the weighted average value of r
, r
and r
. The luminosity was obtained by
= (
/
(
/
, where T
= 5770 K. We calculated the period change of dP/dt = 2.80 (
) ×
d yr
using the change of parabolic fit with quadratic coefficient terms greater than zero, which may means that the mass was transferred mass from the secondary component to the primary component [
137] or magnetic breaking [
138]. Because of the semi-detached system, we calculated the mass transfer rate using the following formula [
139]:
Finally, we obtained the rate of mass transfer of d/dt = 3.92 () × M yr.
We obtained a rate of mass decrease for WY Cnc as
d/
dt= −7.31 (
) ×
yr
. Because WY Cnc is a detached binary system, we opine that the period variation can not explained by the mass transfer. If the change in period contributes to magnetic breaking, we can calculate the angular momentum loss as follows:
where the angular momentum is:
where G is the gravitational constant. We calculated
J as
, which is similar to the result reported by Chen [
37]. The period change of WY Cnc may be caused by the magnetic braking effect [
37].
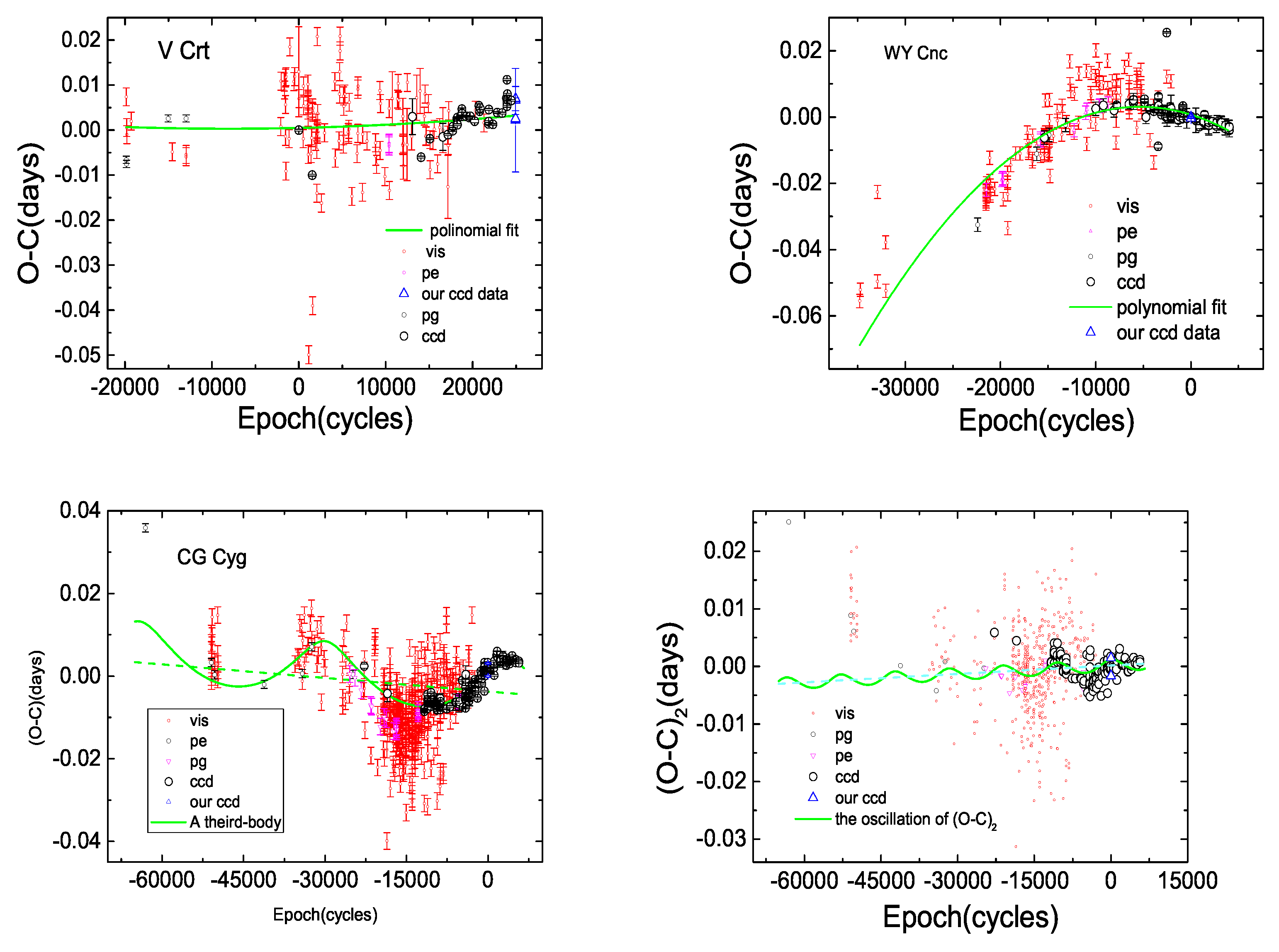
For CG Cyg, a cyclic variation of O-C was observed, as shown in the lower left panel of
Figure 5, which might be explained by the light-time effect with a third body. Firstly, the semi-axis
of the binary must be calculated:
where
i is the orbital inclination,
A is the amplitude, and
c is the speed of light. The amplitude of the oscillation of the systemic velocity can be calculated as follows [
140]:
where
,
, and
are the velocity (km/s), distance from the sun to the earth (Au), and period of third body (yr), respectively. Subsequently, we can calculate the mass (
) of the third-body as followings:
The value of the third body was smallest when
i = 90, as illustrated in
Figure 8. Therefore, we obtained the minimum mass of
as 0.14 M
, which is close to the result of 0.12 M
[
55]. The parameters of the periodic oscillation are listed in
Table 7.
Except for the third body, the period oscillation in lower left panel of
Figure 5 might be caused by magnetic cycles. The change in quadrupole moment due to magnetic activity in the internal constitution can also cause cyclic oscillations (
P/
P). Therefore, we considered the magnetic activity cycles of this system, as following:
where
is the period of magnetic activity. The
P/P of
P of 59.20 yr was calculated to be 1.918 ×
. This value is larger than the traditional value of
P/P−
∼
for magnetic activity in RS CVn and Algol binaries [
141]. Therefore, we can conclude that the periodic oscillations might have been caused by the LITE of a third body, not the magnetic cycle. For the second cycle of
P of 18.31 yr, the
P/P was calculated to be 9.398 ×
. It is in the range of traditional values, which might be caused by the magnetic activity. There is no direct evidence for the third based on our observation of the telescopes. Therefore, we prefer the magnetic activity cycle than the third body to explain the cyclic variations. However, we cannot rule out that these variations might be caused by the third body. New telescope imaging technology are required to check that in the future.
WY Cnc and CG Cyg are detached and RS CVn eclipsing binaries. Using the obtained light curves, we revised the orbital parameters using the WD program. The derived mass ratio of 0.501 ± 0.002 and orbit inclination of 89.759 ± 0.002
are similar to that obtained in the previous results in regions for a mass ratio of 0.38–0.59 and for an inclination of 86–90
for WY Cnc [
35]. The mass ratio of 0.820 ± 0.001 for CG Cyg is similar to 0.862 [
53] and 0.825 [
51]. The orbit inclination of 82.890 ± 0.029
for CG Cyg is similar to the previous results of 83 ± 0.2
[
51] and 82.602 ± 0.099
[
35]. We obtained the starspot parameters of WY Cnc and CG Cyg at our observational times, which were different from the previous starspot parameters [
35]. We confirm that variation in the light curves variations owing to varying starspot in different seasons [
53,
142]. Further photometric and spectroscopic observations are required to determine the magnetic activity cycle.
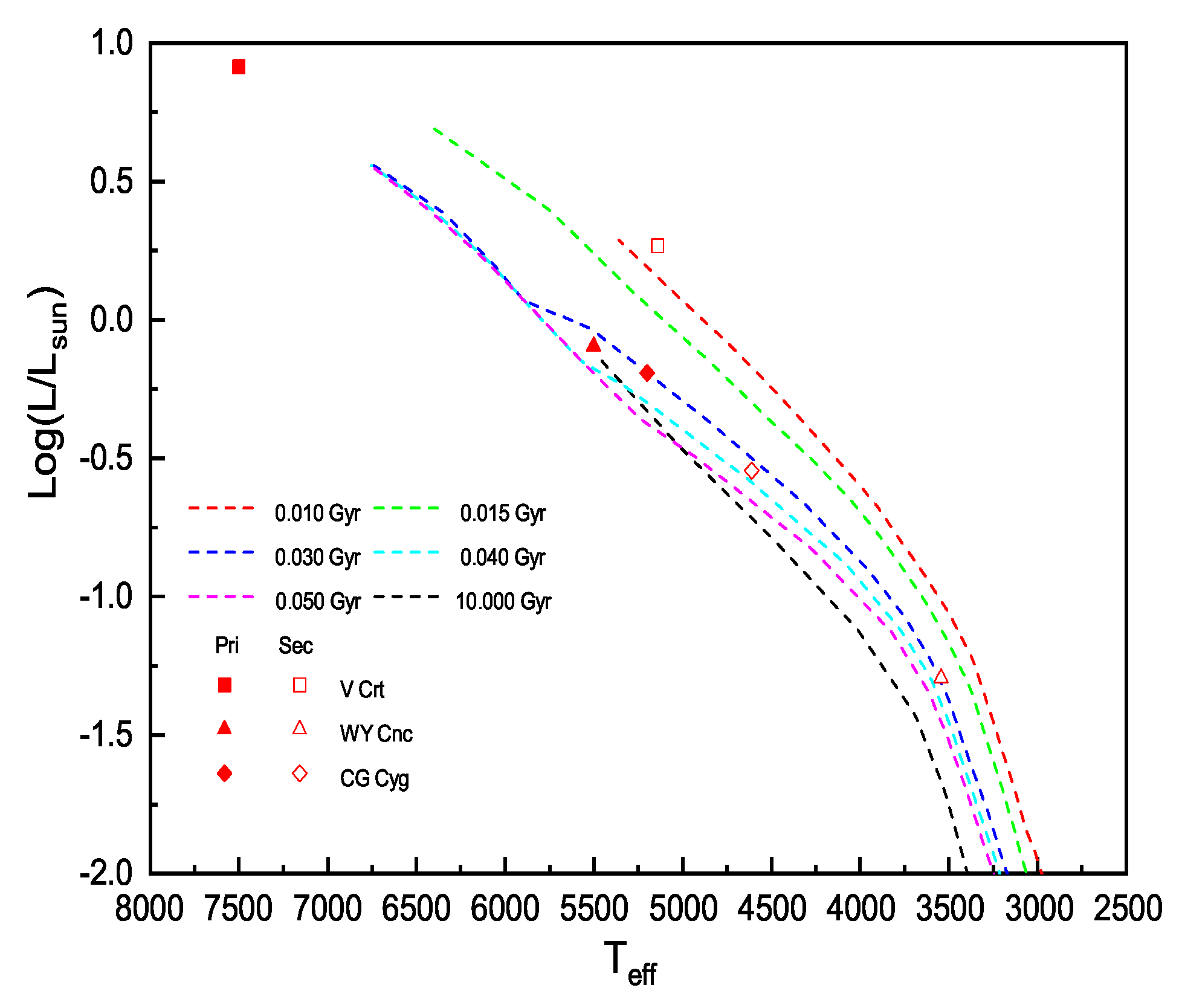
In
Figure 9, we plotted the
vs.
diagrams with many theoretical isochrone [
143], and indicated the positions of both primary and second components of V Crt, WY Cnc and CG Cyg. We found that the parameters of the primary and secondary components of WY Cnc and CG Cyg are in agreement with the 0.030 Gyr tracks of the theoretical model. The secondary component of V Crt are in the region of 0.03–0.05 Gyr. Because V Crt is a semi-detached eclipsing binary and the primary component of V Crt is approximately 7500 K, it is difficult to determine its age. However, its age can be determined by investigating additional observations and theoretical models.