Relativistic Cosmology with an Introduction to Inflation

Abstract

In this review article, the study of the development of relativistic cosmology and the introduction of inflation in it as an exponentially expanding early phase of the universe is carried out. We study the properties of the standard cosmological model developed in the framework of relativistic cosmology and the geometric structure of spacetime connected coherently with it. The geometric properties of space and spacetime ingrained into the standard model of cosmology are investigated in addition. The big bang model of the beginning of the universe is based on the standard model which succumbed to failure in explaining the flatness and the large-scale homogeneity of the universe as demonstrated by observational evidence. These cosmological problems were resolved by introducing a brief acceleratedly expanding phase in the very early universe known as inflation. The cosmic inflation by setting the initial conditions of the standard big bang model resolves these problems of the theory. We discuss how the inflationary paradigm solves these problems by proposing the fast expansion period in the early universe. Further inflation and dark energy in $f\left(R\right)$ modified gravity are also reviewed.

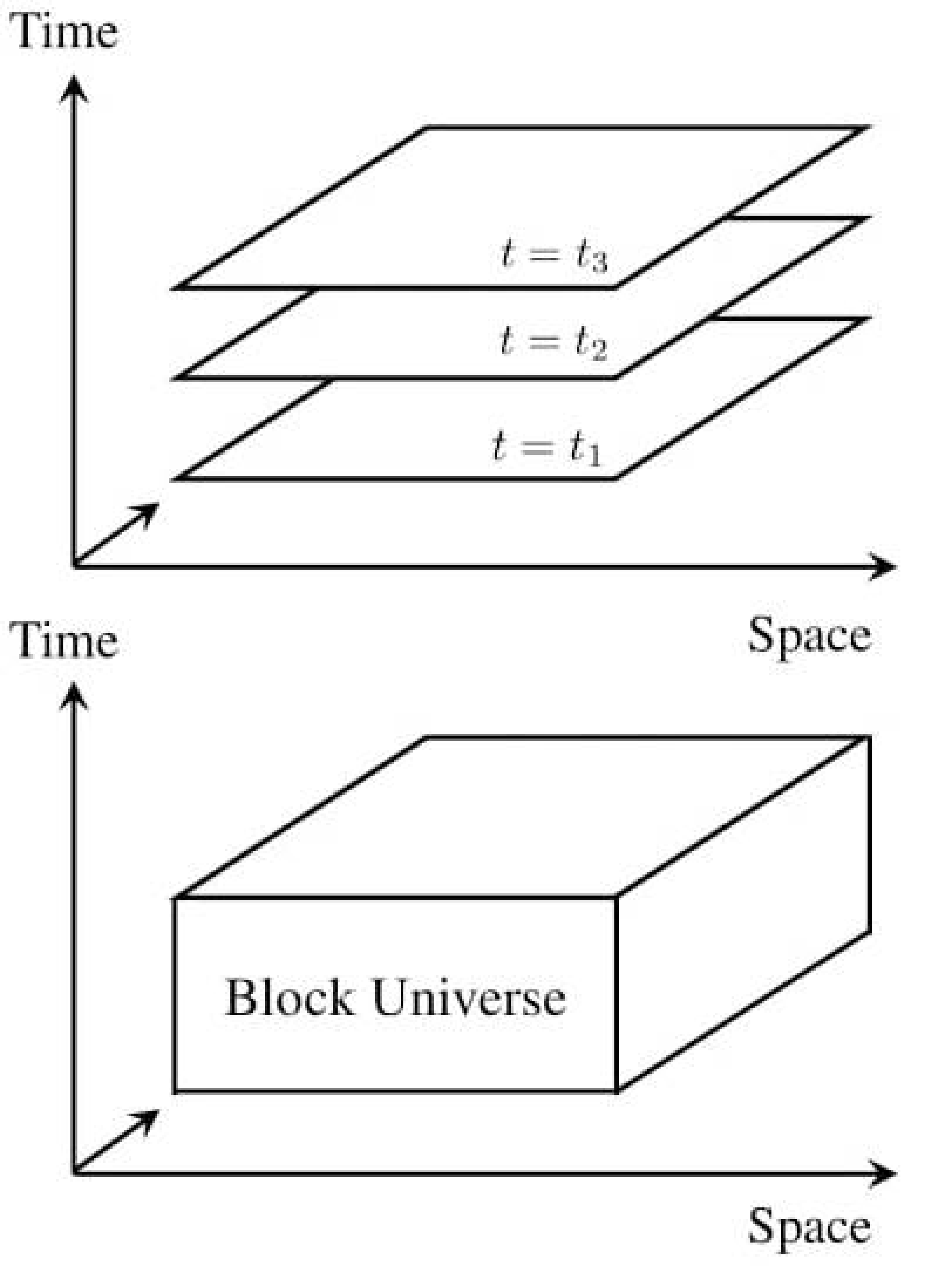
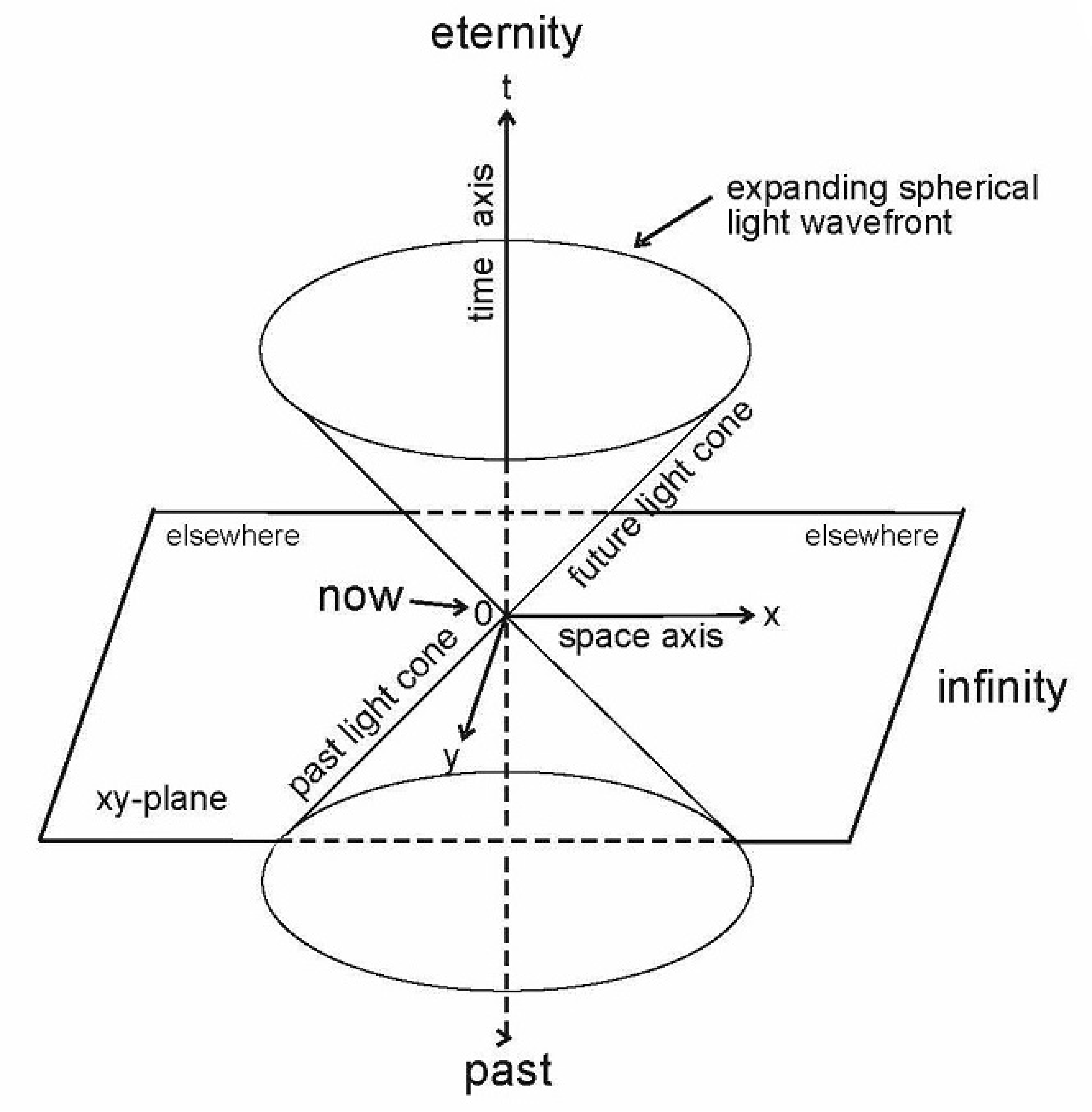
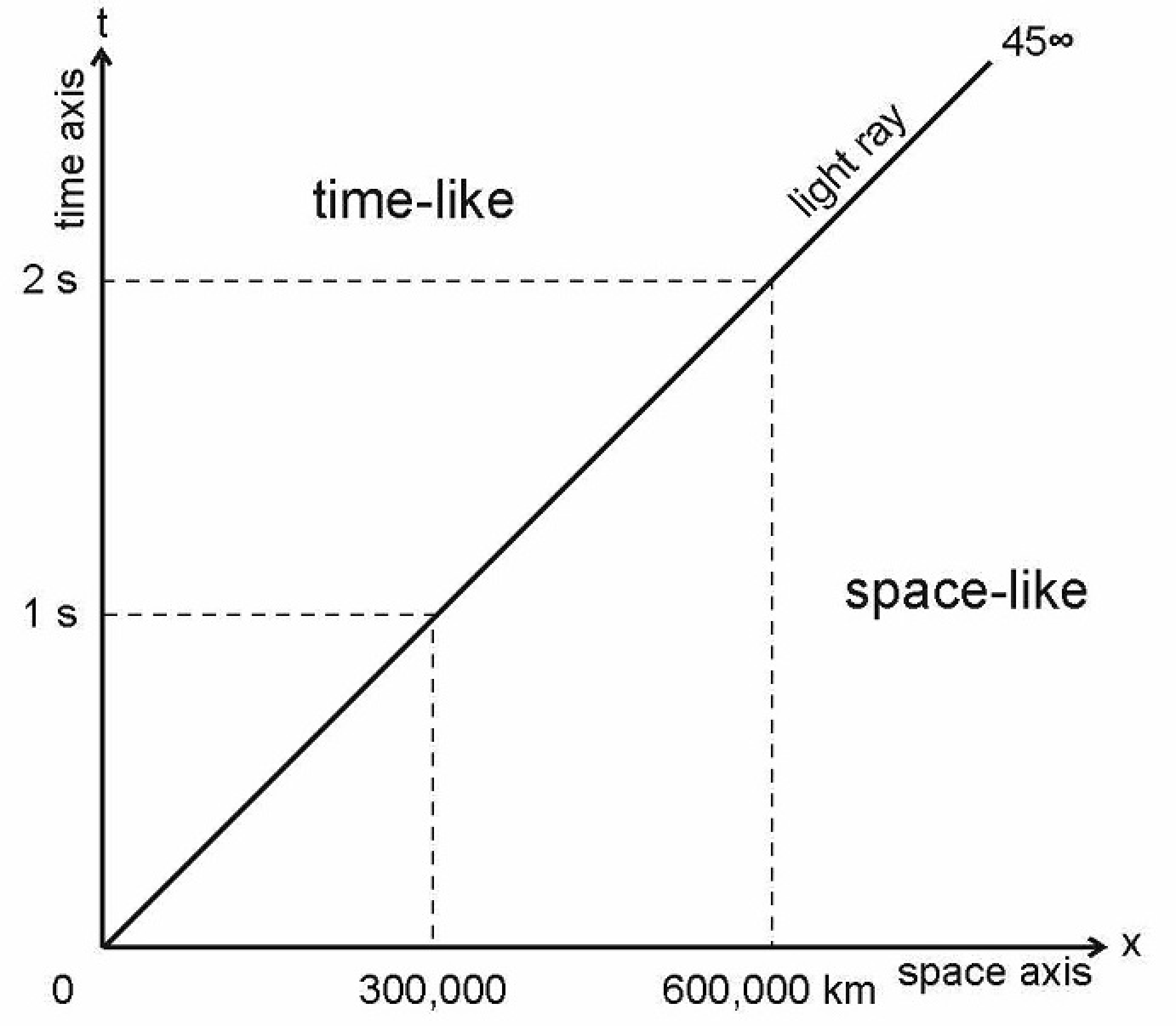
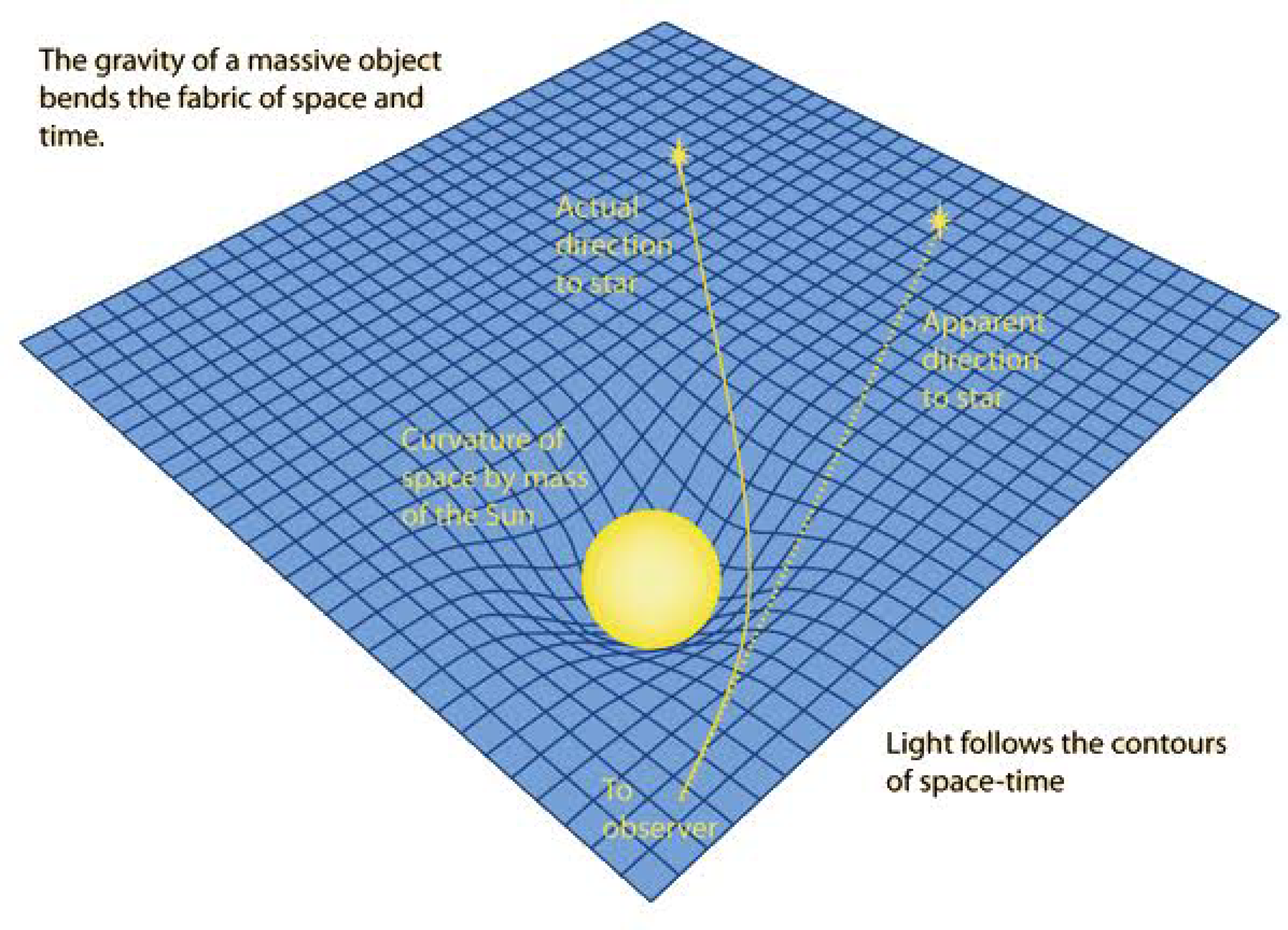
1. Introduction

With the advent of general relativity in 1916, spacetime transformed itself into one of the four fundamental interactions of the universe and the geometrical structure attached to it was taken to demonstrate gravity in a dynamical way [1]. The force of gravity was replaced by the curvature of spacetime that is mirrored through the geometric structure of metric tensor $g_{\mu \nu }$. The spacetime became an integral part of the universe and a dynamical medium where the whole phenomenal universe exists. Any solution of the field equations of general relativity entails a certain structural geometry of spacetime or just a spacetime that represents a universe itself, therefore determining a solution of the field equations is similar to coming across a specific model of the universe.

Cosmology studies the universe as a whole [2] encompassing its beginning in spacetime or as spacetime itself, its evolution, and its eventual ultimate fate. The history of cosmology dates back to ancient Greeks, Indians, and Iranians with its roots at that time in philosophy and religion. Before modern scientific cosmology emerges, it has been nurtured in the womb of Ibrahamic religions especially Judaism, Christianity, and Islam. Cosmology as modern science begins with the surfacing of general relativity when Einstein first himself put it to use to formulate a cosmological model of the universe mathematically. The model brought about a dynamic universe but was rendered to be static as there was no cosmological evidence of its contraction or expansion at that time [3]. Einstein’s static model was afterward proved to be inconsistent with cosmological observations and was discarded; however, its formulation as the first mathematical model based on the field equations of general relativity laid the foundational stone for the inception of modern relativistic cosmology as science.

Cosmology takes into account the largest scale of spacetime that is the causally connected maximal patch of the cosmos from the perspective of its origin, evolution, and futuristic eventual fate. It gives the universe a mathematical description as large as the cosmological observational parameters reveal and allow consequently. The modern relativistic cosmology was established on general relativity which brought forth the big bang model of the universe. The big bang model was marred with some inward problems related to it, which were removed by introducing an exponentially expanding phase in the early universe known as inflation. de Sitter presented a model of the universe devoid of ordinary matter, however with the cosmological constant term retained. The geometry of the model was proved to be accelerating [4]. The de Sitter universe corresponds to the specific case related to one of the very early solutions of Einstein’s Field Equations (EFE). The importance of the de Sitter model was not recognized until the introduction of inflation in the late 20th century, as the actual universe must be considered as a local set of perturbations in the geometry of de Sitter having validity at large. de Sitter geometry represents Euclidean space with a metric that depends on time. It was found that the inflation could be the de Sitter in general or quasi-de Sitter geometry which has an innate impact on the evolution of the geometry of FLRW spacetimes. It further bears its relation with the late-time accelerated expansion of the universe and to the dynamic geometry of the spacetime intrinsically cohered with it. The paradigm of inflation, as it is propounded, has a profound impact on the evolution of the universe as the geometry of spacetime. The de Sitter universe represents the inflationary phase of the universe with slightly broken time translational symmetry.

Alexander Friedmann predicted theoretically the universe to be dynamic, the one which can expand, contract, or even be born out of a singularity [5]. George Lemaitre, unaware of Friedmann’s work at that time, independently reached the same conclusion. In 1931, he also proposed a theory of the primeval atom which came to be known later on as the big bang theory by Fred Hoyle accidentally [6]. Edwin Hubble first proved the existence of other galaxies besides the Milky Way and afterward in 1929 discovered based on observational evidence that the universe is actually expanding [7]. This was actually discovering what Friedmann already had predicted theoretically in 1922. In the late 1940s, George Gamow (1904–1968) and his collaborators, Ralph Alpher (1921–2007) and Robert Herman (1914–1997), independently worked on Lemaître’s hypothesis and transformed it into a model of the early universe. They made supposition about the initial state of the universe comprising of a very hot, compressed mixture of nucleons and photons, thereby introducing the big bang model on the basis of comparatively strong evidences. They did not associate a particular name with the early state of the universe. Based on this model they were successful in calculating the amount of helium in the universe, but unfortunately, there was no authentic observational evidence through which their calculations could be compared [8].

The standard relativistic model of cosmology underpinning big bang theory could not explain the global structure of the universe and the origin of matter in it. The distribution of matter in it homogeneously on large scales and the spatial flatness also remained enigmatic. The big bang model just made an assumption about these but could not solve them. In the framework of effective field theory, the aspects of nonsingular cosmology were explored by Yong Cai et al. It is shown that the effective field theory assists in having the clarification about the origin of no-go-theorem and helps to resolve this theorem [9].

The inflationary era was proposed in the standard model of cosmology which propounds the big bang theory of the creation of the universe. Inflation solves the problems encountered in the big bang cosmology. Gliner, in 1965, hypothesized an era of exponential expansion for the universe earlier than any significant inflationary model surfaced [10]. It was found that the scalar fields are dynamic in nature, and in 1972 it was proposed that during phase transitions the energy density of the universe as a scalar field changes [11]. Andrei Linde, in 1974, realized that scalar fields can play an important role in describing the phases of the very early universe. He speculated that the energy density of a scalar field can play the role of vacuum energy dubbed as a cosmological constant [12].

In 1978, Englert, Brout, and Gunzig [13] forwarded a proposal of “fireball” hypothesis attempting to resolve the primordial singularity problem. They based their investigations on the entropy contained in the universe and approached the issue of early evolution of the universe by introducing particle production in it. They inquired deep down into it and on the basis of their hypothesis inferred that a universe undergoing a quantum mechanical effect would itself appear in a state of negative pressure and would be subject to a phase of exponential expansion. A work was mentioned by Linde in his review article [14] where he sought, in collaboration with Chibisov, to develop a cosmological model based upon the facts known in connection with the scalar fields. Considering the supercooled vacuum as a self-contained source for entropy, they tried to bring about the exponential expansion of the universe to be concerned with it. They, however discovered instantly that the universe becomes very inhomogeneous after the bubble wall collisions take place.

Slightly before Alan Guth’s original proposal of inflation surfaced, Alexei Starobinsky in 1980 proposed a model of inflation on the base of a conformal anomaly in quantum gravity. His proposal was presented in the framework of general relativity where slight modification of the equations of general relativity in matter sector was proposed and quantum corrections were employed to it in order to have a phase of the early universe. Starobinsky’s model can be considered as the first model of inflation which is of semi-realistic nature and evades from the graceful exit problem [15]. It was hardly concerned with the problems of homogeneity and isotropy which occur in the relativistic cosmological model of the big bang. His model, as he himself accentuated, can be considered the extreme opposite of chaos in Misner’s model. The model is found to agree with cosmological observations with slight deviations form recent measurements. Tensor perturbations that represent gravitational waves have also been predicted in Starobinsky’s model with a spectrum that is flat.

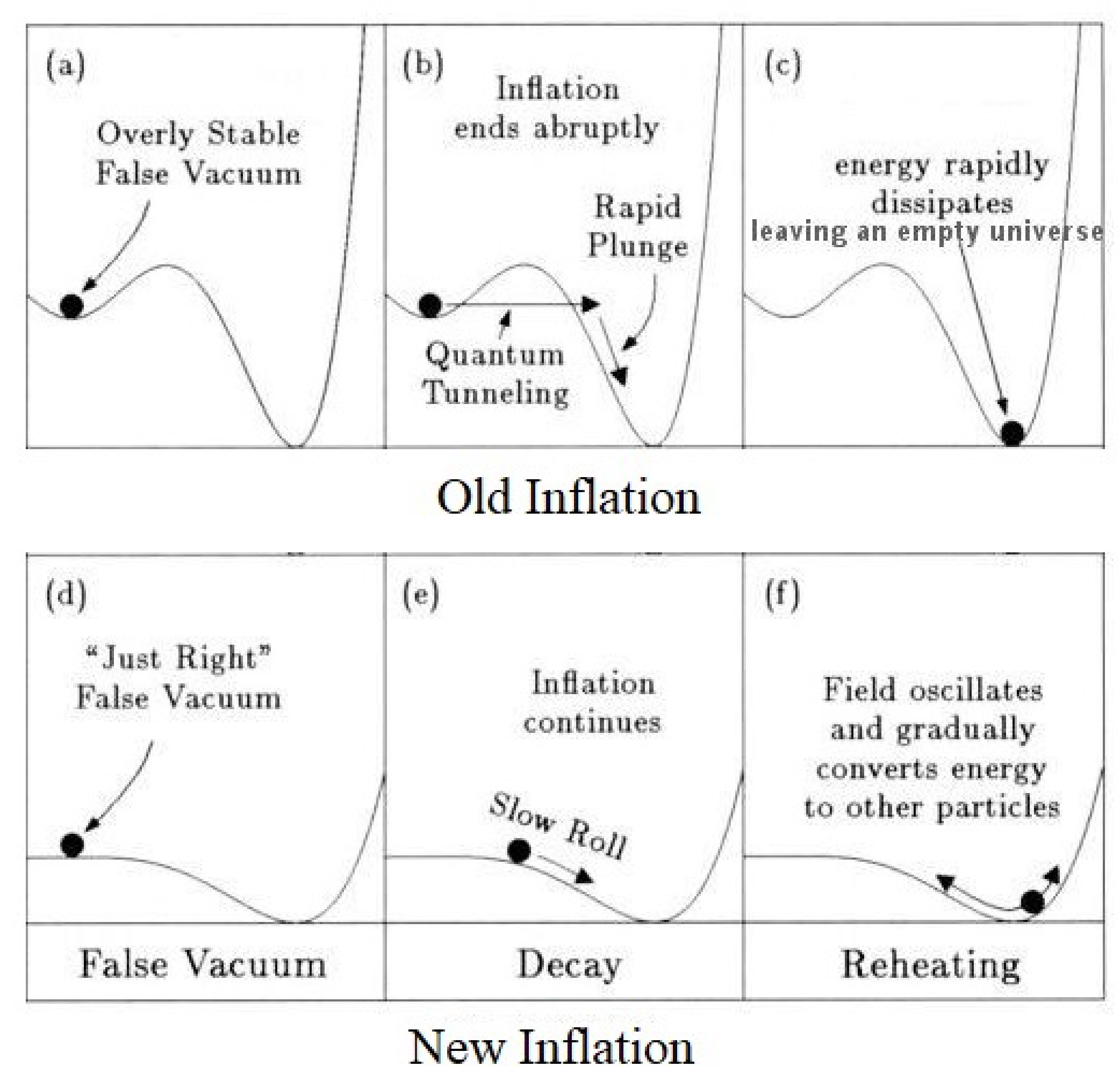
Alan Guth employed the dynamics of a scalar field and with a clear physical motivation presented an inflationary model [16] in 1981 on the base of supercooling theory during the cosmic phase transitions where the universe expands in a supercooled false vacuum state. A false vacuum is a metastable state containing a huge energy density without any field or particle so that when the universe expands from this heavy nothingness state its energy density does not change and empty space remains empty so that the inflation occurs in false vacuum [17]. The duration of inflationary phase in Guth’s original scenario is too short to resolve any problem, although was supposed to solve these problems and consequently the universe becomes very inhomogeneous which leads to the graceful exit problem [18,19]. The problem prevents the universe from evolving to later stages and is inherently existing in the originally proposed version of Guth.

The graceful exit problem was addressed independently by Linde, Steinhard, and Albrecht [20,21,22,23,24,25], where they introduced a phase of slow roll inflation at the end of the normal inflationary phase inclusively known as new inflation. The resolution of the problem was sought by constructing a new inflationary paradigm where the inflation can have its inception either in an unstable state at the top of the effective potential or in the state of false vacuum. In this scenario, the dynamics of the scalar field is such that it rolls gradually down to the lowest of its effective potential. It is of great importance to note that the shifting away of the scalar field from the false vacuum state to other later states has remarkable consequences. When the scalar field rolls slowly towards its lowest so-called slow roll inflation, the density perturbations are generated which seed the structure formation of the universe [26,27,28]. The production of density perturbations during the phase of slow roll inflation is inversely proportional to the motion of the scalar field [29,30]. The basic difference between the new inflationary scenario and that of the old one is that the advantageous portion of the inflation in the new scenario, which is responsible for the large scale homogeneity of the universe, does not take place in the false vacuum state, where the scalar field vanishes. This means that the new inflation could explain why our universe was so large only if it was very large initially and contained many particles from the very beginning.

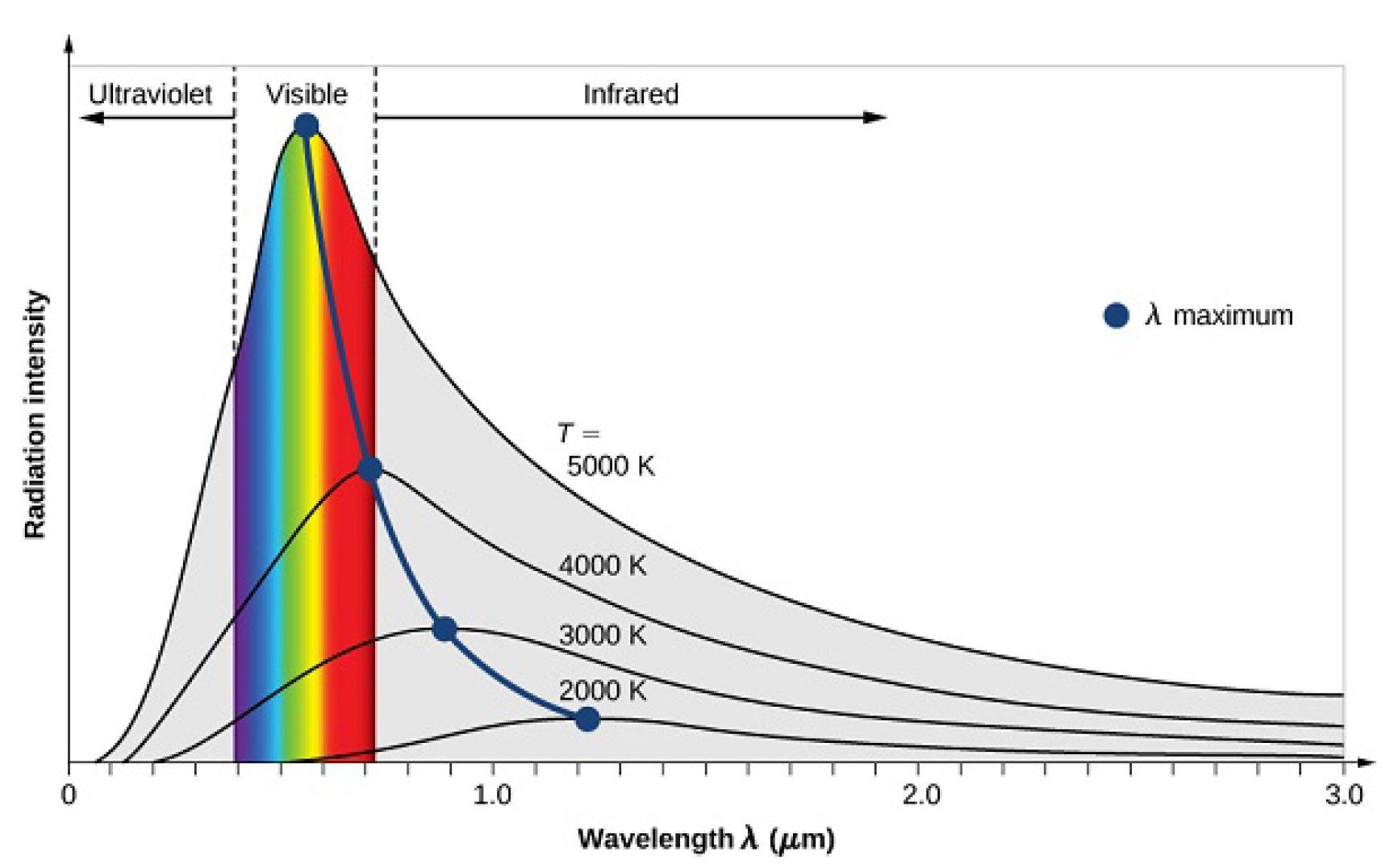
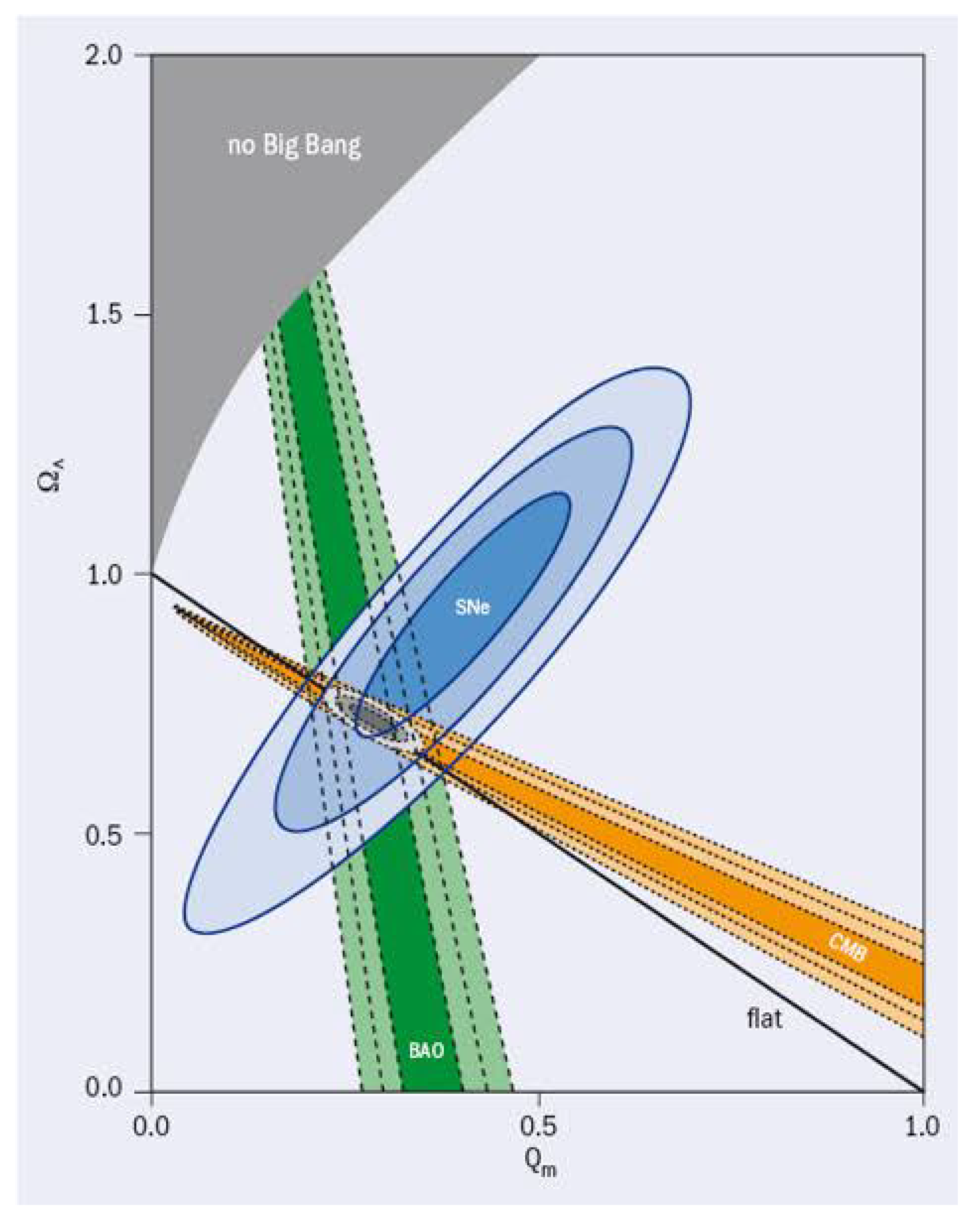
The course of 20th century has presented many challenges to the standard cosmology. In the framework of the standard model, in addition to inflation, another breakthrough came forth in 1998 when the observation-based accelerated expansion of the universe was discovered [31,32,33]. Before this discovery, however, it was thought that in the perspective of all known forms of matter and energy that obey the strong energy condition $\rho +3p>0$, the expansion of the universe would slow down with the passage of time. This was a natural consequence of Friedmann equations that play a central role in the evolution of the universe. From the acceleration equation $\frac{\ddot{a}}{a}=-\frac{4\pi G}{3}\left(\rho +3p\right)$, the universe must be undergoing deceleration characterized by deceleration parameter $q_0=-\frac{a\ddot{a}}{{\dot{a}}^2}$; however, astoundingly the value of $q_0<0$ was observationally determined, meaning that the expansion of the universe is accelerating rather to be decelerating. The discovery of accelerated expansion has won Noble prize in 2011. To explain the cause of accelerated expansion an exotic form of energy density was introduced hypothetically known usually as dark energy. The present budget of the universe from the observational data is contributed by dark energy $70\%$, dark matter $25\%$ and $5\%$ ordinary baryon matter [34,35]. Dark energy is effective on the largest scales of intra galaxies and does not affect gravitationally bound systems. To explain the origin of dark energy, there is a large number of proposed models. Many independent observations lend support to the existence of dark energy such as CMB, SN Ia, BAO, etc. Today dark energy constitutes a very significant subject of relativistic cosmology with observational data by providing information about its basic nature, for reviews see in [36,37,38]. In this article, we study the standard model of cosmology by investigating the geometric structure of spacetime related with it in the framework of general relativity. Beginning with Euclidean space, we study spacetime in special and general theory of relativity. We discuss problems encountered in the standard big bang cosmology, and the inflationary solutions introduced into it, by proposing a phase of accelerated expansion in the early universe. A discussion of $f\left(R\right)$ modified gravity is also presented with discussion of how inflation and dark energy can be described in its framework.

The layout of the paper is as follows. In Section 2, we discuss the structure of Euclidean space beginning with the axioms of Euclidean geometry and the significant role played by the Pythagoras theorem in its development. It has four subsections discussing space, time, and spacetime in relativity and pre-relativity physics. Section 3 begins with relativistic cosmology with a discussion on its underlying principles. The standard model of cosmology is discussed in Section 4 with nine subsections about its geometric structure. In Section 5, the derivation of Friedmann equations is carried out. Section 6 describes different aspects of embedding a geometrical object in a space of higher dimensions. It is has four subsections. Section 7 presents the very first relativistic model developed by Einstein himself. It has two subsections that discuss the instability of Einstein’s universe and de Sitter’s empty universe model respectively. In Section 8, a discussion on conformal FLRW line elements is presented in addition to the vacuum, radiation, and matter-dominated eras. It has 12 subsections covering related topics. Section 9 with its four subsections is devoted to the discussion of cosmological problems faced by the standard model. In Section 10, we embark on inflation and discuss its dynamics. Section 11 describes how the proposal of exponential expansion in the early universe solves the cosmological problems. $\mathsf{\Lambda }$CDM and $f\left(R\right)$ are discussed in this section. In the last Section, we provide a summary of the paper. Four indices are added in the end.

2. Euclidean Space

Euclidean geometry is established on a set of simple axioms and the definitions derived from these axioms. These axioms were first stated by Euclid in about 300 B.C. [39]. A space at the level of mathematical abstraction is the set of points where each point represents a specific position in it. When an abstract space is mapped onto a physical space, each point of it represents a physical location in it. Euclidean space is what entails on the base of axioms of Euclidean geometry. Geometrically, a space can be described by reducing it to a certain specification of the distance between each pair of its neighboring points. In order to reduce all of the geometry of a space to a certain specification of the distance between each pair of neighboring points we use the metric or line element which measures the space and describes its nature. A line element specifies a certain geometry and its form varies corresponding to different coordinate systems. Five basic postulate lie at the core of Euclidean space and are the basis of standard laws of geometry:

1.

Any two points can be joined by a straight line, i.e., the shortest distance between two points is a straight line.

2.

A straight line can be extended to any length.

3.

A circle can be drawn with a given a line segment as radius and one end as center of the circle.

4.

All right angles are congruent.

5.

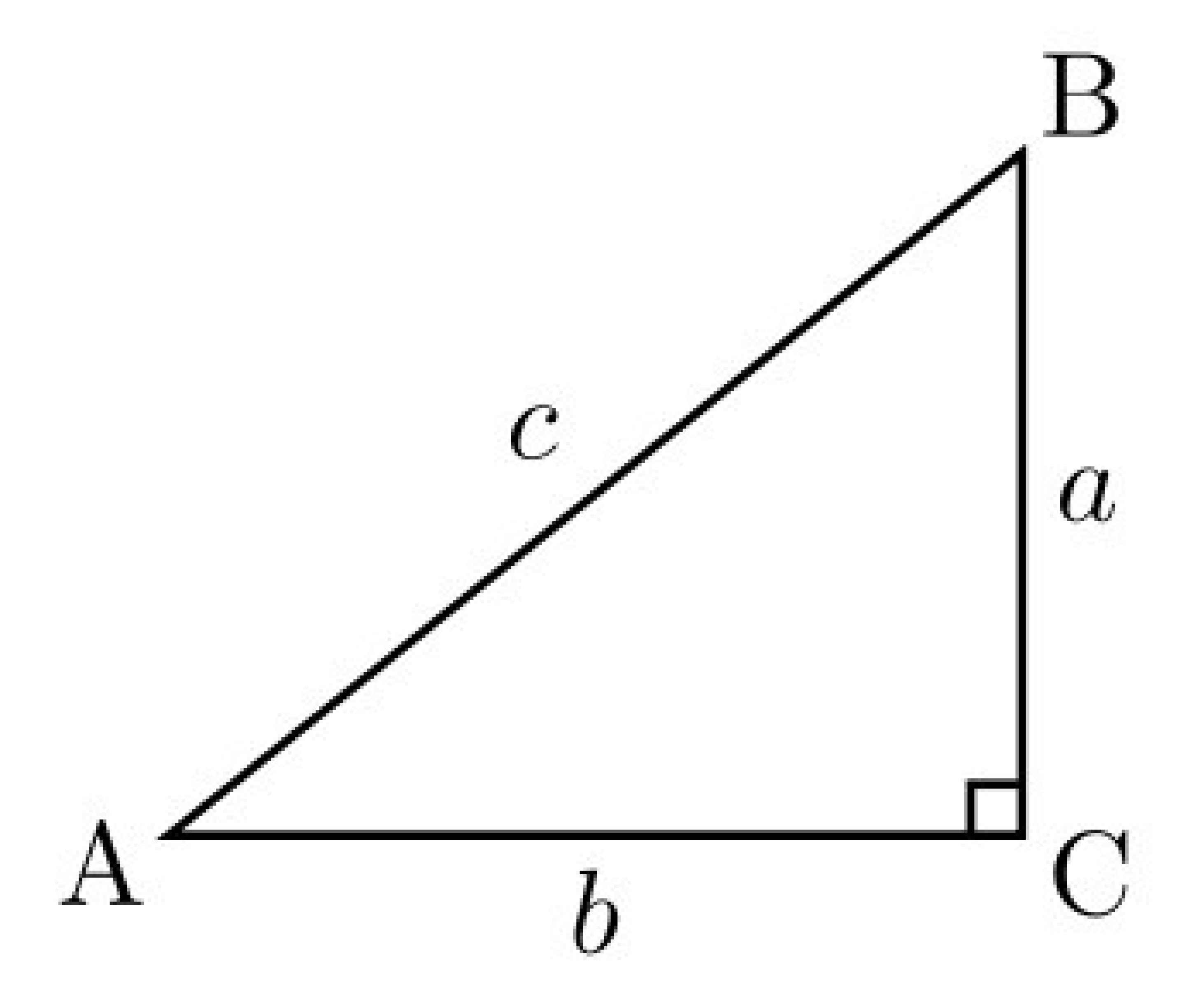
Given a line and a point not on the line, it is possible to draw exactly one line through the given point parallel to the line, i.e., parallel lines remain a constant distance apart. Pythagoras theorem was known before Euclid and can also be derived from the five postulates and is used to find distance between any two points in Euclidean space. A mathematical space is an abstraction used to model the physical space of the universe. The Euclidean space consists of geometric points and has three dimensions. Now the Pythagorean theorem for a right triangle describes how to calculate the length of hypotenuse when the lengths of other two sides namely base and altitude are given. The length of hypotenuse gives distance between two points. 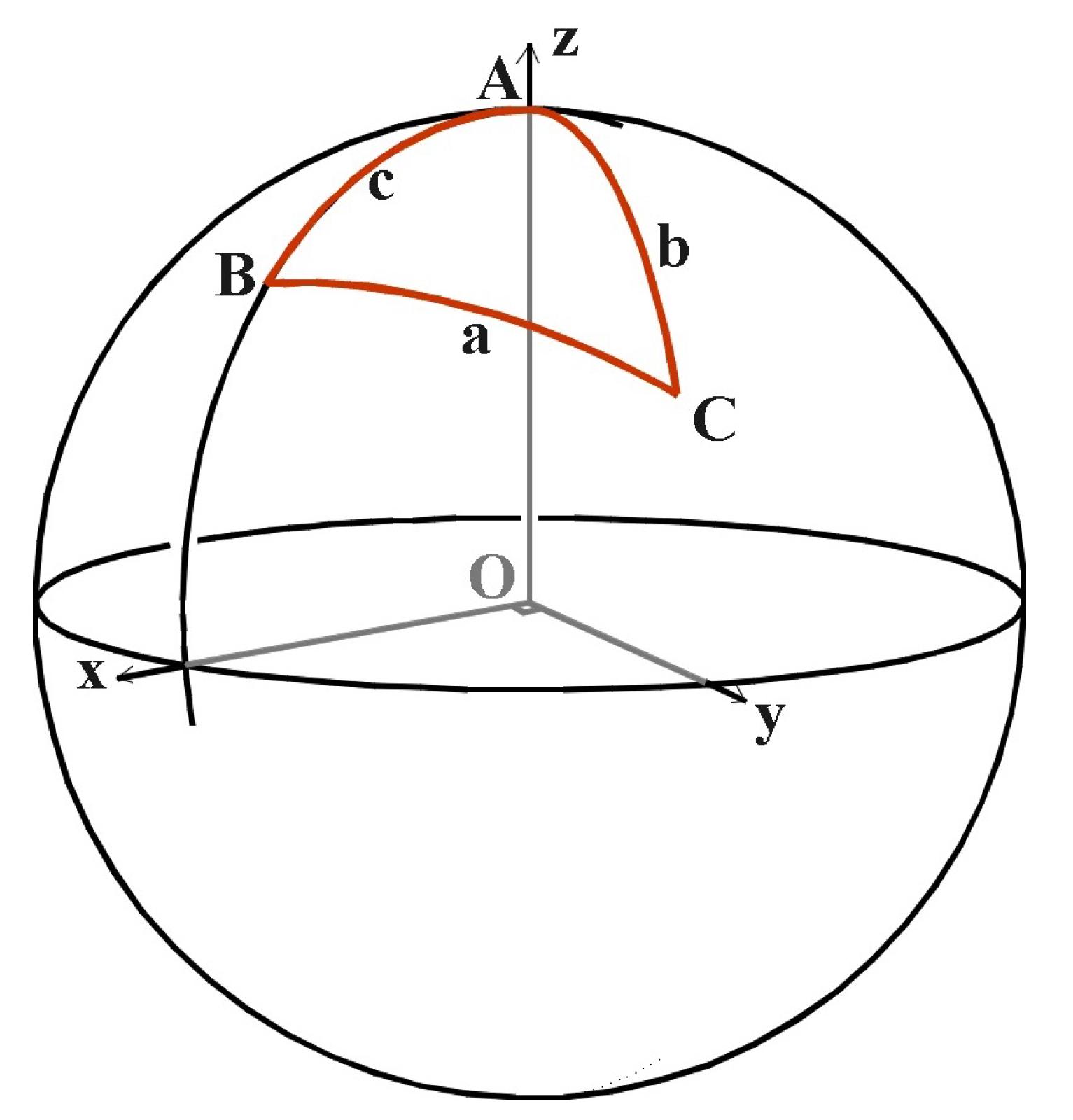Figure 1 below shows Pythagoras theorem:

$$d^2=x^2+y^2$$

(1)

Now, as the space can be described everywhere consisting of geometric points, we can define mutual relation for every infinitesimally close three points of the space forming a right triangle so that we can determine the element of distance between any two points with the help of Pythagoras theorem. Using rectangular Cartesian coordinate system we can express distance between two points in differential form as

$${dl}^2={dx}^2+{dy}^2$$

(2)

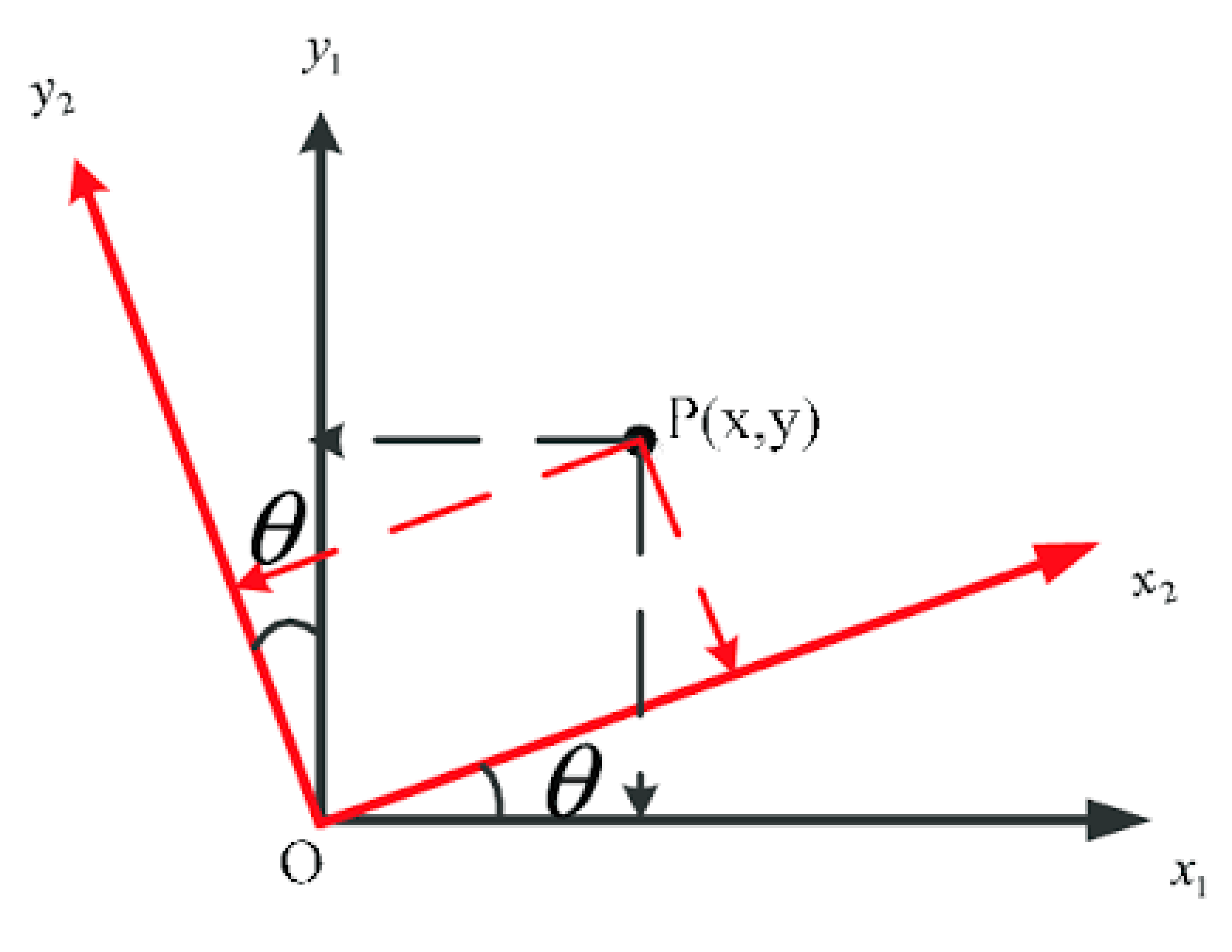
The distance-measure by Pythagoras theorem in Equation (2) will be known as metric or line element in two dimensions and defines Euclidean metric for two dimensional space. The distance measured between two points by the metric in Equation (2) does not change on rotating the coordinate system in which these two points are specified as Figure 2 manifests it:

$$\left(\begin{array}{cc}& x^{\prime }\\ & y^{\prime }\end{array}\right)=\left(\begin{array}{cc}cos\theta & sin\theta \\ -sin\theta & cos\theta \end{array}\right)\left(\begin{array}{cc}& x\\ & y\end{array}\right)$$

(3)

or

$$\begin{array}{c}\hfill \begin{array}{cc}\hfill & x^{\prime }=xcos\theta +ysin\theta \hfill \\ \hfill & y^{\prime }=-xsin\theta +ycos\theta \hfill \end{array}\end{array}$$

(4)

The distance between two points remains invariant which means that

$$d{x^{\prime }}^2+d{y^{\prime }}^2=d{x}^2+d{y}^2$$

(5)

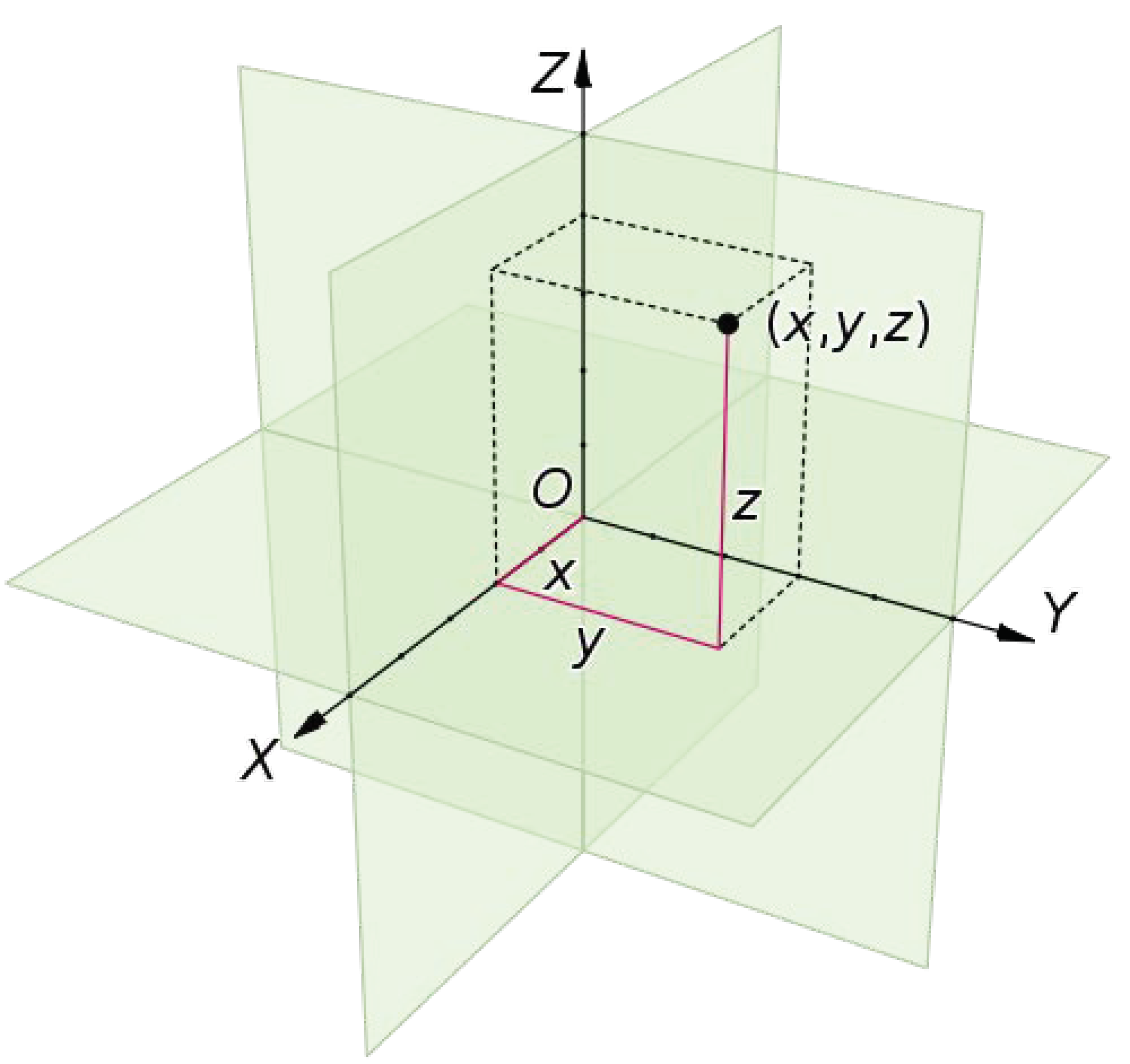
The Pythagorean theorem in three dimensions can be described as

$$d^2=x^2+y^2+z^2$$

(6)

Three mutually perpendicular planes along three dimensions of the Cartesian coordinate system divide it in 3-planes as is shown in Figure 3:

Now, in reference to a coordinate system each point of this space will have three coordinates $\left(x,y,z\right)$ if we approach its structure through Cartesian scheme, i.e., in Cartesian coordinates each point of it is represented by three coordinates which are the distances measured starting from the origin of the coordinate axes along the corresponding axes, i.e., x-axis, y-axis, and z-axis, respectively. These three axes stand for three dimensions of space. We find the distance between two points with Cartesian coordinate for three points separated infinitesimally

$${dl}^2={dx}^2+{dy}^2+{dz}^2$$

(7)

which gives the metric of three-dimensional space The distance between two points with Cartesian coordinates $\left(x,y,z\right)$ and $\left(p,q,r\right)$ will be

$$d{s}^2={\left(x-p\right)}^2+{\left(y-q\right)}^2+{\left(z-r\right)}^2$$

(8)

The infinitesimal distance between any two points $\left(x,y,z\right)$ and $\left(x+dx,y+dy,z+dz\right)$ can be had using the metric written above in Equation (7) in three dimensional Euclidean space.

$$d{s}^2={\left[x-\left(x+dx\right)\right]}^2+{\left[y-\left(y+dy\right)\right]}^2+{\left[z-\left(z+dz\right)\right]}^2$$

(9)

$$d{s}^2={\left(-dx\right)}^2+{\left(-dy\right)}^2+{\left(-dz\right)}^2=d{x}^2+d{y}^2+d{z}^2$$

(10)

or in tensor form

$$d{s}^2={\delta }_{\mu \nu }d{x}^{\mu }d{x}^{\nu }$$

(11)

where ${\delta }_{\mu \nu }$ is the Kronecker delta function representing a symmetric tensor of rank two and can be expressed as a $3\times 3$ matrix form

$${\delta }_{\mu \nu }=\left(\begin{array}{ccc}{\delta }_{11}& {\delta }_{12}& {\delta }_{13}\\ {\delta }_{21}& {\delta }_{22}& {\delta }_{23}\\ {\delta }_{31}& {\delta }_{32}& {\delta }_{33}\end{array}\right)=\left(\begin{array}{ccc}1& 0& 0\\ 0& 1& 0\\ 0& 0& 1\end{array}\right)$$

(12)

diagonal of

$${\delta }_{\mu \nu }=diag\left[{\delta }_{\mu \nu }\right]=\left[+1,+1,+1\right]$$

(13)

and trace of

$${\delta }_{\mu \nu }=\sum _{\mu =\nu }\left[{\delta }_{\mu \nu }\right]=1+1+1=3$$

(14)

therefore, ${\delta }_{\mu \nu }=diag\left(+1,+1\dots \dots \dots \dots +1\right)$ in Equation (13) defines an n-dimensional Euclidean space.

Now Equation (11) can be expanded using Einstein summation convention

$$d{s}^2={\delta }_{1\nu }d{x}^{1}d{x}^{\nu }+{\delta }_{2\nu }d{x}^{2}d{x}^{\nu }+{\delta }_{3\nu }d{x}^{3}d{x}^{\nu }$$

(15)

$$\begin{array}{c}d{s}^2=\left({\delta }_{11}d{x}^{1}d{x}^1+{\delta }_{12}d{x}^{1}d{x}^2+{\delta }_{13}d{x}^{1}d{x}^3\right)\hfill \\ +\left({\delta }_{21}d{x}^{2}d{x}^1+{\delta }_{22}d{x}^{2}d{x}^2+{\delta }_{23}d{x}^{2}d{x}^3\right)\hfill \\ +\left({\delta }_{31}d{x}^{3}d{x}^1+{\delta }_{32}d{x}^{3}d{x}^2+{\delta }_{33}d{x}^{3}d{x}^3\right)\hfill \end{array}$$

(16)

$$\begin{array}{c}d{s}^2=\left(\left(1\right)d{x}^{1}d{x}^1+\left(0\right)d{x}^{1}d{x}^2+\left(0\right)d{x}^{1}d{x}^3\right)\hfill \\ +\left(\left(0\right)d{x}^{2}d{x}^1+\left(1\right)d{x}^{2}d{x}^2+\left(0\right)d{x}^{2}d{x}^3\right)\hfill \\ +\left(\left(0\right)d{x}^{3}d{x}^1+\left(0\right)d{x}^{3}d{x}^2+\left(1\right)d{x}^{3}d{x}^3\right)\hfill \end{array}$$

(17)

$$d{s}^2=\left(d{x}^{1}d{x}^1+0+0\right)+\left(0+d{x}^{2}d{x}^2+0\right)+\left(0+0+d{x}^{3}d{x}^3\right)$$

(18)

$$d{s}^2=\left(d{x}^{1}d{x}^1\right)+\left(d{x}^{2}d{x}^2\right)+\left(d{x}^{3}d{x}^3\right)$$

(19)

$$d{s}^2={\left(d{x}^1\right)}^2+{\left(d{x}^2\right)}^2+{\left(d{x}^3\right)}^2$$

(20)

$$d{s}^2=d{x}^2+d{y}^2+d{z}^2$$

(21)

Equation (21) can also be written in the form

$$ds·ds=dx·dx+dy·dy+dz·dz$$

(22)

From Equation (22), we can see that the inner product in three dimensional Euclidean space can be perfectly described, that is why three dimensional Euclidean space is an example of a complete inner product space. An explanatory discussion of maximally symmetric 3 space can be consulted in Appendix B.

2.1. Newtonian Mechanics: The Structure of Space and Time

Space and time are absolute structures in classical physics and can be distinguished from one another in an independent way. Newton’s Mechanics is based specifically on three laws of motion, a law of gravitation and Galilean principle of relativity which are inherently related with the properties of space and time. Newtonian space is a three-dimensional extension around us which constitutes absolute space. Absolute space in Newton’s own words is described as “Absolute space, in its own nature, without relation to anything external remains always similar and immovable”, therefore space is rigid, motionless, and can be viewed as colossally empty three-dimensional cubic or cuboidal box where material objects reside and all physical phenomena take place. Newtonian space has the properties of Euclidean pace where infinitesimal distance between any two points is a straight line and if three points constitute a right angled triangle, then three sides are related by Pythagoras theorem which ascribes to it the properties of a flat space. Sum of angles in a triangle in such space is ${180}^{\circ }$. Newtonian space is homogeneous and isotropic which entails Newtonian Mechanics. Homogeneity implies translational invariance of the properties of space which means that it has similar properties at every point contained in it. The property of being homogeneous is called homogeneity that leads to the invariance of physical laws performed in two or more coordinate systems. Newton’s 3rd law, law of conservation of momentum and energy, etc. come out as a consequence of homogeneity of space. It is also an isotropic that implies rotational invariance of the properties of space. It means that it has similar properties in all directions and is therefore direction-independent. Thus, isotropy implies homogeneity but the converse is not true. The absolute time has been enunciated as follows “Absolute time, and mathematical time of itself and from its own nature flows equably without relation to anything external, and is otherwise called duration” such time exists independent of space and whatever dynamically happens in it and flows uniformly in one direction. An interval of time possesses always unchanging meaning for all times. This is presented figuratively in Figure 4 below:

According to Newtonian Mechanics, gravitation and relative motion do not affect the rate at which time flows. From Newton’s 2nd law $F=ma$, the isotropy of time can be viewed in case of a dynamic system that does not change from perpetrating transition from $+t$ to $-t$. This is because it does not incorporate the element of time explicitly which implies that past and future are indistinguishable but this is paradoxical because time is unidirectional and flows always from past to future. Two observers in two inertial frames of reference in relative motion and equipped with standard measuring clocks record the spacetime coordinates of an event written as $\left(t,x,y,z\right)$ and $\left(t^{\prime },x^{\prime },y^{\prime },z^{\prime }\right)$, respectively. According to Galilean principle of relativity, the coordinate transformations are

$$\begin{array}{c}\hfill \begin{array}{cc}\hfill & x^{\prime }=x-vt\hfill \\ \hfill & y^{\prime }=y\hfill \\ \hfill & z^{\prime }=z\hfill \\ \hfill & t^{\prime }=t\hfill \end{array}\end{array}$$

(23)

We can calculate the addition of velocities according to these transformations by differentiating the spatial parts of Equation (23) with respect to time t, we have

$$\begin{array}{c}\hfill \begin{array}{cc}\hfill & \frac{d{x}^{\prime }}{dt}=\frac{dx}{dt}-v\hfill \\ \hfill & \frac{d{y}^{\prime }}{dt}=\frac{dy}{dt}\hfill \\ \hfill & \frac{d{z}^{\prime }}{dt}=\frac{dz}{dt}\hfill \end{array}\end{array}$$

(24)

As $t=t^{\prime }$, we infer that $\frac{d{x}^{\prime }}{dt}=\frac{d{x}^{\prime }}{d{t}^{\prime }}$. Likewise, acceleration can also be differentiated once again from Equation (24), which gives

$$\begin{array}{c}\hfill \begin{array}{cc}\hfill & \frac{d^2{x}^{\prime }}{d{t}^2}=\frac{d^{2}x}{d{t}^2}\hfill \\ \hfill & \frac{d^2{y}^{\prime }}{d{t}^2}=\frac{d^{2}y}{d{t}^2}\hfill \\ \hfill & \frac{d^2{z}^{\prime }}{d{t}^2}=\frac{d^{2}z}{d{t}^2}\hfill \end{array}\end{array}$$

(25)

We can observe from Equation (25) that the accelerations in both frames are same. The time-coordinate $t^{\prime }$ of one inertial frame remains unaffected during transformation to another inertial frame of reference in classical physics and does not depend on spatial coordinates x, y, and z. The set of equations in Equation (23) are known as Galilean transformations. The motion along y and z spatial dimensions remains unaffected and the time coordinates in the two frames are equivalent which implies that time is absolute as Newton believed meaning that for all the inertial observers the time interval between any two events would be invariant. We notice that the two events having coordinates $\left(t,x,y,z\right)$ and $\left(t^{\prime },x^{\prime },y^{\prime },z^{\prime }\right)$, respectively, with differential of the distance as Euclidean spatial interval described in Equation (21) as $d{s}^2=d{x}^2+d{y}^2+d{z}^2$ and the time interval $\Delta t=t^{\prime }-t$ both remain separately invariant under the Galilean transformations in Equation (23). This fact makes us consider the nature of space and time as absolute entities in Newtonian Mechanics. We identify the quantity $d{s}^2$ as square of the distance between points of three-dimensional Euclidean space and invariance of this differential of distance alludes to the fact that it is geometrical structural property of the space itself in its own right. This describes the geometry of space and time according to Newton’s views.

2.2. Special Theory of Relativity: The Structure of Spacetime

Special relativity is a theory of the structure of spacetime and in this way constitutes a geometric theory [40]. The fields and particles grow over this spacetime structure and relativistic mechanics is developed according to this structure which corresponds to the postulates of special relativity. According to the Lorentz transformations implied by it, space and time are not distinguishable quantities but constitute innately a single continuum to be known as spacetime. One of the Einstein’s 1905 papers brought forward this theory founded upon two postulates [41].

1.

The principle of special covariance.

2.

The principle of invariance of the velocity of light (c). As the laws of physics remain form-invariant, i.e., covariant according to a privileged class of observers known as inertial frames. This is also called principle of relativity. These two principles overthrew the pre-relativity notions of absolute space and absolute time proposing instead relative concepts. In classical physics as we saw earlier the coordinates of two observers are related by Galilean transformations, whereas according to the special relativity, the coordinates in two frames are related using Lorentz transformations.

$$\begin{array}{c}\hfill \begin{array}{cc}\hfill & x^{\prime }=\frac{x-vt}{\sqrt{1-\frac{v^2}{c^2}}}\hfill \\ \hfill & y^{\prime }=y\hfill \\ \hfill & z^{\prime }=z\hfill \\ \hfill & t^{\prime }=\frac{t-\frac{vx}{c^2}}{\sqrt{1-\frac{v^2}{c^2}}}\hfill \end{array}\end{array}$$

(26)

Lorentz transformations contain all the geometric information about space and time, and describe the structure of spacetime. Further, we can see that space and time coordinates are absolute according to the Galilean transformations for two inertial observers which move relative to each other and are connected through space and time coordinates. Time coordinate has the same magnitude in pre-relativity physics; however, according to the special relativity, which obeys Lorentz transformations, the time coordinate in one coordinate system is connected to the time coordinate of the second coordinate system through both time and space coordinates, which alludes to the fact that space and time coordinates are now to be dealt on equal footings. It is obvious from the Lorentz transformations that the time coordinates are not equivalent in two frames, i.e., $t\ne t^{\prime }$ rather $t^{\prime }$ is innately cohered with both of the coordinates of time and space t and x respectively. It means that time $t^{\prime }$ of one coordinate frame converts partially in space and partially in time coordinates. Therefore, $t^{\prime }$ does not remain independent but has partially coalesced with space coordinates losing its absolute nature and the principle of relativity forbade us to locate a preferred frame of reference ensuing that absolute notion of time disappears logically. This fact was first perceived by Minkowski when he was recasting the special relativity in the language of geometry. He has presented a very profound and significant geometrical structure underlying special relativity. While delivering a lecture at the meeting of the Göttingen Mathematical Society on 5 November 1907, he introduced the concept of spacetime continuum whereby he asserted that independent space and time have to doom away into mere shadows and only a union of the two can preserve an independent reality. Minkowski viewed that the principle of special relativity can be described by the metric $-d{t}^2+d{x}^2+d{y}^2+d{z}^2$ on the four-dimensional space $R^4$ which familiarized the concept of spacetime continuum and paved the way for the formulation of general relativity. A Minkowski metric g on the linear space $R^4$ is a symmetric non-degenerate bilinear form with signature $\left(-,+,+,+\right)$. It means that there exists a basis $\left\{e_0,e_1,e_2,e_3\right\}$ such that $g\left(e_{\mu },e_{\nu }\right)=g_{\mu \nu }$ where $\mu ,\nu \in \left\{0,1,2,3\right\}$ and $g_{\mu \nu }$ is expressed in the form

$$\begin{array}{c}\hfill \begin{array}{c}\hfill g_{\mu \nu }=\left(\begin{array}{cccc}{g}_{00}& g_{01}& g_{02}& g_{03}\\ g_{10}& g_{11}& g_{12}& g_{13}\\ g_{20}& g_{21}& g_{22}& g_{23}\\ g_{30}& g_{31}& g_{32}& g_{33}\end{array}\right)=\left(\begin{array}{cccc}-1& 0& 0& 0\\ 0& 1& 0& 0\\ 0& 0& 1& 0\\ 0& 0& 0& 1\end{array}\right)\end{array}\end{array}$$

(27)

so that an we have orthonormal basis and can construct a system of coordinates of $R^4$ as $\left(x^0,x^1,x^2,x^3\right)$ such that at each point we can have $e_0={\partial }_t$ and ${\partial }_{x^j}$ where $j=1,2,3$. Now, with respect to this coordinate system, we can write the metric tensor $\left(0,2\right)$ in the form $g=g_{\mu \nu }d{x}^{\mu }d{x}^{\nu }=-d{t}^2+{\displaystyle \sum _1^3}d{x}^j$ or $d{s}^2=-d{t}^2+d{x}^2+d{y}^2+d{z}^2$ The negative sign with one time component term in the metric indicates that it is not Euclidean space but represents a pseudo-Euclidean known as Minkowski space and also guarantees that the speed of light is same in all inertial frames. An expanding Minkowskian spacetime can be described in the form as written below which represents the simplest of all dynamic spacetimes $d{s}^2=-d{t}^2+a^2\left(t\right)\left[d{x}^2+d{y}^2+d{z}^2\right]$. It was thought convenient on the dimensional grounds to introduce the coordinates in the form $\left(x^0,x^1,x^2,x^3\right)=\left(ct,x,y,z\right)$. Pythagoras theorem applied in Euclidean space $R^3$ of three spatial dimensions gives the distance of two points as an invariant as we observed in previous section.

$$d{s}^2=+d{x}^2+d{y}^2+d{z}^2$$

(28)

here $ds$ the length element is a scalar quantity which means that in certain frame of references all the observers will agree upon the length of the measured object. In 1905, Einstein speculated that the measurement of the spacetime interval

$$d{s}^2=-d{x}^2-d{y}^2-d{z}^2+{\left(cdt\right)}^2={\eta }_{\mu \nu }d{x}^{\mu }d{x}^{\nu }$$

(29)

where

$$\begin{array}{c}\hfill \begin{array}{c}\hfill {\eta }_{\mu \nu }=\left(\begin{array}{cccc}{g}_{00}& g_{01}& g_{02}& g_{03}\\ g_{10}& g_{11}& g_{12}& g_{13}\\ g_{20}& g_{21}& g_{22}& g_{23}\\ g_{30}& g_{31}& g_{32}& g_{33}\end{array}\right)=\left(\begin{array}{cccc}-1& 0& 0& 0\\ 0& -1& 0& 0\\ 0& 0& -1& 0\\ 0& 0& 0& 1\end{array}\right)\end{array}\end{array}$$

(30)

would not result in identical either in space or in time [42] for the observers in relative uniform motion. However, Minkowski noted that the four-dimensional entity in Equation (29) would remain invariant for all such observers. The basic significant idea which Minkowski took notice of was that the spacetime interval remains invariant for all the observers in uniform relative motion meaning that it is also a scalar upon which they all will agree. The metric of Minkowski space which is homogenous and isotropic is given by

$$g_{\mu \nu }={\eta }_{\mu \nu }=diag\left(-1,+1,+1,+1\right)$$

(31)

thus the geometry of spacetime is flat in special relativity. It is notable here that it is spacetime that is flat, however in classical mechanics, it is space rather than spacetime. If the Minkowskian geometry of spacetime is required to be expanding, it can be made so. However, in the framework of special relativity, it does not need to expand. Figure 5 gives the structure of Minkowskian spacetime as a null cone structure. In the Figure 6, it is shown how the dimension time is converted in as space.

$$g_{\mu \nu }={\eta }_{\mu \nu }=diag\left(-1,a^2\left(t\right),a^2\left(t\right),a^2\left(t\right)\right)$$

(32)

2.3. General Theory of Relativity: The Structure of Spacetime

The essence of general relativity is that geometry is gravity which comes from Equivalence principle. It models gravity into the dynamic structure of spacetime. In general relativity, the structure of spacetime is described by a fundamental quantity called the spacetime metric $g_{\mu \nu }$ or line element which gives the nature of the geometry of spacetime by finding the distance between two infinitesimally neighboring points in it. The geometrical structure of spacetime is incarnated [43] in two basic principles.

1.

Principle of general covariance.

2.

The spacetime continuum has, at each of its points, a quadratic structure of coordinate differentials $d{s}^2=g=g_{\mu \nu }d{x}^{\mu }d{x}^{\nu }$ known as “square of the interval” between the two points under consideration.

We consider a four-dimensional continuum every point of which is distinct from the other with four coordinates-a quadruplet $x_1,x_2,x_3,x_4$ assigned consecutively to each of them

$$\begin{array}{c}\hfill \begin{array}{cc}\hfill & {x^{\prime }}_1={x^{\prime }}_1\left(x_1,x_2,x_3,x_4\right)\hfill \\ \hfill & {x^{\prime }}_2={x^{\prime }}_2\left(x_1,x_2,x_3,x_4\right)\hfill \\ \hfill & {x^{\prime }}_3={x^{\prime }}_3\left(x_1,x_2,x_3,x_4\right)\hfill \\ \hfill & {x^{\prime }}_4={x^{\prime }}_4\left(x_1,x_2,x_3,x_4\right)\hfill \end{array}\end{array}$$

(33)

It is denoted by $g_{\mu \nu }$. In matrix form with components, it is written as

$$\begin{array}{c}\hfill \begin{array}{c}\hfill g_{\mu \nu }=\left(\begin{array}{cccc}{g}_{00}& g_{01}& g_{02}& g_{03}\\ g_{10}& g_{11}& g_{12}& g_{13}\\ g_{20}& g_{21}& g_{22}& g_{23}\\ g_{30}& a_{31}& g_{32}& g_{33}\end{array}\right)\end{array}\end{array}$$

(34)

The properties of spacetime that are intrinsically related to it, are completely determined by the spacetime metric. An example of the local curved spacetime around the Sun in two dimensions is displayed in Figure 7:

A detailed discussion of space, time and spacetime is presented in Appendix A.

2.4. The Basics of General Relativity

It would be convenient to have a retrospective look into the basics of general relativity whose role has been very fundamental to the modern cosmology. We briefly review the structure of the theory specifically in connection with the geometrical structure of spacetime in it. General relativity in its core describes that gravity is the geometry of four-dimensional spacetime manifested through its curvature. It is a theory of spacetime and gravitation that are the very basic components of the universe. Einstein’s journey towards general relativity in order to introduce gravity in his previous theory sought the fascinating geometry of the structure of spacetime, such that gravity as a field force disappeared and was assimilated in the very geometric structure of spacetime. In constructing the framework of new theory, Einstein was influenced and governed by Mach’s principle, which states that it is a priori existence and distribution of matter which determines the geometry of spacetime, and in the absence of it, there shall be no geometric structure of a spacetime in the universe. Therefore, there will be no inertial properties in an, otherwise, empty universe. In general, relativity gravitation and inertia are essentially indistinguishable. The metric tensor $g_{\mu \nu }$ describes the effect of both combinedly, and it is arbitrary to ask which one contributes its effect more and which less, therefore to call it with a single name is suitable either inertia or gravitation [4]. In general relativity gravitation, inertia and the geometry of spacetime are coalesced into a single entity represented by a symmetric tensor of second rank $g_{\mu \nu }$ which owes its existence due to presence and distribution of matter which is represented by an other symmetric tensor $T_{\mu \nu }$ known as energy-momentum tensor. The metric tensor $g_{\mu \nu }$ is the fundamental object of study in general relativity and takes into consideration all the causal and geometrical structure of spacetime. General relativity underlies five fundamental principles connotated by it implicitly or explicitly manner:

• Mach’s principle
• principle of equivalence
• principle of covariance
• principle of minimal gravitational coupling
• correspondence principle

In the light of the principle of general covariance, the theory requires that the laws of physics might be formulated in a coordinate-independent style. The coordinate independence requires the replacement of partial derivatives by covariant derivatives which introduces connection coefficients ${\mathsf{\Gamma }}_{\mu \nu }^{\lambda }$ as the 2nd kind of Christoffel symbols. All the geometric structure of spacetime is based on the existence of these connection coefficients. The field equations of general relativity read as $G_{\mu \nu }=8\pi T_{\mu \nu }$, where $G_{\mu \nu }=R_{\mu \nu }-\frac{1}{2}{g}_{\mu \nu }R$ is the Einstein tensor and is expressed in terms of Ricci tensor, metric tensor, and Ricci scalar, and $T_{\mu \nu }$ is energy momentum tensor. The spacetime continuum of general relativity is postulated as a 4-dimensional Lorentzian manifold (M, g), where M denotes the Manifold and g is metric defined over it. The geometry of a spacetime is encoded in its metric which has a geodesic structure, though complex and frequently solved numerically for a specific bunch of geodesics. These geodesics specify the physical properties of the geometry of spacetime which are interpreted by drawing graphically in a certain spacelike volume. Gravity is the geometry of spacetime itself which is described through its dynamic structure in the framework of general relativity. The interaction between spacetime and the content it contains which mutually form and the universe is the pith and marrow of general relativity. Matter tells spacetime how to curve and spacetime tells the matter how to move. General relativity thus transforms gravitation from being a force to being it a property of spacetime, so that gravity does remain a force but curvature of the geometric structure of spacetime. Einstein worked out a relation between matter–energy content of the universe and its gravitating effects in the form of geometry of spacetime. He employed the language of tensors to describe it. The invariant interval between two events separated infinitesimally with coordinates $\left(t,x,y,z\right)$ and $\left(t+dt,x+dx,y+dy,z+dz\right)$ has been defined according to special relativity

$$d{s}^2={\eta }_{\mu \nu }d{x}^{\mu }d{x}^{\nu }$$

(35)

Which defines a Lorentz invariant Minkowski flat spacetime whose geometry of spacetime is encoded in ${\eta }_{\mu \nu }$. Under the change of coordinates $d{s}^2$ remains invariant and is spacelike for $d{s}^2>0$, timelike for $d{s}^2<0$ and light-like for $d{s}^2=0$. Photon path is described by $ds=0$ and baryonic matter follows a path between two events for which

$$\int ds=0$$

(36)

i.e., it generates stationary values and conforms to the shortest distance between two points to be straight line which means that there are no external forces to set their path deviated. General relativity was based on five principles incorporated in it explicitly or implicitly, namely, equivalence principle, relativity principle, Mach’s principle, and Correspondence principle. Tensors are geometric objects defined on a manifold M, which remain invariant under the change of coordinates. It is composed of a set of quantities which are called its components, therefore a it is the generalization of a vector which means that it has more than three components. They represent mathematical entities which conform to certain laws of transformations. The properties of components of a tensor do not depend on a coordinate system which is used to describe the tensors. Transformation laws of a tensor relate its components in two different coordinate systems. The mathematical representation of a tensor is displayed through considering usually a bold face alphabetical letter like A, B, T, P, etc. with an index or a set of indices in the form of superscripts or subscripts or both in mixed form. These superscripts and subscripts in case of a tensor are called contravariant and covariant indices. Contravariant indices of a tensor are used to give the meaning of a contravariant components of it like $A^{\mu }$, $A^{\mu \nu }$, $A^{\mu \nu \xi \dots ..}$. Covariant indices of a tensor are used to signify the meaning of a contravariant components of it like $A_{\mu }$, $A_{\mu \nu }$, $A_{\mu \nu \xi \dots ..}$. The indices of both types namely contravariant and covariant are used to specify the components of a mixed tensor like $A_{\mu }^{\nu }$, $A_{\mu \xi }^{\nu }$, $A_{\mu }^{\nu \sigma }$, $A_{\mu \xi \upsilon \dots }^{\nu \sigma \dots }$. A mixed tensor is a tensor which has contravariant as well as covariant components. The number of indices appearing in the symbol representing certain type of a tensor is known as its rank. The appearing indices in the symbol representing a tensor can be contravariant or covariant or both type of indices in it. The order of a tensor is the same thing as rank, only the name differs. The number of components of a tensor is related with its rank or order and the dimensions of the space in which the is being described. In an n-dimensional space, a tensor of rank, say, k will have number of components equal to number of components of a tensor in n-dimensional space is equivalent to $n^k=$${\left(numberofdimensionsofspace\right)}^{rank}$. However, the spacetime of general relativity is pseudo-Riemannian having four dimensions, three spatial and one temporal. Coordinate patches are necessarily considered to map whole of the spacetime. Each point-event of a coordinate patch in the four-dimensional pseudo-Riemannian spacetime is labeled by a general coordinate system, which conventionally runs over 0, 1, 2, and 3, where 0 stands for time and the rest for space coordinates. An inertial or otherwise frame of reference characterized by a coordinate system can be attached to every point event of the spacetime and coordinate transformations between any two coordinate systems can be found. These can be written as

$$\begin{array}{c}\hfill \begin{array}{cc}\hfill & {A^{\prime }}_{\mu }=\frac{\partial x^{\nu }}{\partial {x^{\prime }}^{\mu }}{A}_{\nu }\hfill \\ \hfill & {B^{\prime }}^{\mu }=\frac{\partial {x^{\prime }}^{\mu }}{\partial x^{\nu }}{B}^{\nu }\hfill \\ \hfill & A_{\nu }^{{}^{\prime }\mu }=\frac{\partial x^{{}^{\prime }\mu }}{\partial x^{\zeta }}\frac{\partial x^{\sigma }}{\partial x^{{}^{\prime }\nu }}{A}_{\sigma }^{\zeta }\hfill \end{array}\end{array}$$

(37)

while switching to Riemannian geometry for non-Euclidean spaces ordinary partial differentiation is generalized to covariant differentiation and is defined using a semi-colon; as

$$\begin{array}{c}{B}_{\nu ;\mu }={\partial }_{,\mu }{B}_{\nu }-{\mathsf{\Gamma }}_{\nu \mu }^{\sigma }{B}_{\sigma }\hfill \\ {B^{\nu }}_{;\mu }={\partial }_{,\mu }{B}^{\nu }+{\mathsf{\Gamma }}_{\mu \sigma }^{\nu }{B}^{\sigma }\hfill \end{array}$$

(38)

where comma, denotes an ordinary partial differentiation with respect to the corresponding variable and; signifies covariant differentiation. In the covariant differentiation, indices can also be raised or lowered with metric tensor, however the covariant differentiation of it vanishes, i.e., $g_{\mu \nu ;\alpha }=0$. The interval between infinitesimally separated events $x^{\mu }$ and $x^{\mu }+d{x}^{\mu }$ is given by

$$d{s}^2=g_{\mu \nu }d{x}^{\mu }d{x}^{\nu }$$

(39)

The corresponding contravariant tensor of $g_{\mu \nu }$ is given by $g^{\mu \nu }$ and they result in Kronecker delta. Moreover, indices can be lowered or raised using the metric tensor in either form as

$$\begin{array}{c}\hfill \begin{array}{cc}\hfill & g_{\mu \nu }{g}^{\mu \zeta }={\delta }_{\nu }^{\zeta }\hfill \\ \hfill & g_{\mu \nu }{B}^{\nu }=B_{\mu }\hfill \\ \hfill & g^{\mu \nu }{B}_{\nu }=B^{\mu }\hfill \end{array}\end{array}$$

(40)

In general relativity, all the geometry of curved spacetime is contained in the second-rank symmetric tensor $g_{\mu \nu }$ known as fundamental or metric tensor and is the function of four coordinates $g_{\mu \nu }=g_{\mu \nu }\left(x_0,x_1,x_2,x_3\right)$ and $g_{\mu \nu }$ encodes all the information about gravitational field induced by presence of matter. It governs the other matter as a response mimicking the role of gravitational potential similar to that of Newtonian gravity so that the paths remain no more straight, and the action in Equation (36) determines the path of a free particle known as geodesic

$$\frac{d^2{x}^{\mu }}{d{s}^2}+{\mathsf{\Gamma }}_{\nu \zeta }^{\mu }\frac{d{x}^{\nu }}{ds}\frac{d{x}^{\zeta }}{ds}=0$$

(41)

where

$${\mathsf{\Gamma }}_{\nu \zeta }^{\mu }=g^{\mu \lambda }{\mathsf{\Gamma }}_{\nu \zeta \lambda }=\frac{1}{2}{g}^{\mu \lambda }\left(\frac{\partial g_{\nu \lambda }}{\partial x^{\zeta }}+\frac{\partial g_{\zeta \lambda }}{\partial x^{\nu }}+\frac{\partial g_{\nu \zeta }}{\partial x^{\lambda }}\right)$$

(42)

are the Christoffel symbols which through the geodesic equation specify the world lines of free particles. The “acceleration due to gravity” in Newtonian gravitation law is described by these symbols in Einstein’s picture of gravity as the geometric properties of spacetime encoding the similar information. Locally these symbols vanish in the inertial frame of reference in free fall and under coordinate transformation from $x^{\mu }$ and ${x^{\prime }}^{\mu }$ do not constitute components of a tensor and therefore do not represent a tensor.

$${{\mathsf{\Gamma }}^{\prime }}_{\mu \nu }^{\sigma }=\frac{\partial {x^{\prime }}^{\sigma }}{\partial x^{\lambda }}\frac{\partial x^{\zeta }}{\partial {x^{\prime }}^{\mu }}\frac{\partial x^{\rho }}{\partial {x^{\prime }}^{\nu }}{\mathsf{\Gamma }}_{\zeta \rho }^{\lambda }+\frac{{\partial }^2{x}^{\zeta }}{\partial {x^{\prime }}^{\mu }\partial {x^{\prime }}^{\nu }}\frac{\partial {x^{\prime }}^{\sigma }}{\partial x^{\zeta }}$$

(43)

The Riemann tensor is defined as

$$R_{\mu \nu \lambda }^{\sigma }=\frac{\partial }{\partial x^{\nu }}{\mathsf{\Gamma }}_{\mu \lambda }^{\sigma }-\frac{\partial }{\partial x^{\lambda }}{\mathsf{\Gamma }}_{\mu \nu }^{\sigma }+{\mathsf{\Gamma }}_{\mu \lambda }^n{\mathsf{\Gamma }}_{n\nu }^{\sigma }-{\mathsf{\Gamma }}_{\mu \nu }^n{\mathsf{\Gamma }}_{n\lambda }^{\sigma }$$

(44)

It has symmetry properties and satisfies the following Bianchi identity:

$$R_{\mu \nu \lambda ;\zeta }^{\sigma }+R_{\mu \zeta \nu ;\lambda }^{\sigma }+R_{\mu \lambda \zeta ;\nu }^{\sigma }=0$$

(45)

The Ricci tensor is obtained from Riemann tensor contracting

$$R_{\mu \nu }=g_{\lambda \sigma }{R}_{\mu \nu \lambda }^{\sigma }=\frac{\partial }{\partial x^{\nu }}{\mathsf{\Gamma }}_{\mu \lambda }^{\sigma }-\frac{\partial }{\partial x^{\lambda }}{\mathsf{\Gamma }}_{\mu \nu }^{\sigma }+{\mathsf{\Gamma }}_{\mu \lambda }^n{\mathsf{\Gamma }}_{n\nu }^{\sigma }-{\mathsf{\Gamma }}_{\mu \nu }^n{\mathsf{\Gamma }}_{n\lambda }^{\sigma }$$

(46)

Another expression of Ricci tensor is written in the form given below when determinant of the metric tensor $g_{\mu \nu }$ is envisaged as a matrix and denoted by g

$$R_{\mu \nu }={\mathsf{\Gamma }}_{\mu \nu ,\lambda }^{\lambda }-{\left(ln\sqrt{-g}\right)}_{,\mu \nu }+{\left(ln\sqrt{-g}\right)}_{,\lambda }{\mathsf{\Gamma }}_{\mu \nu }^{\lambda }-{\mathsf{\Gamma }}_{\pi \mu }^{\lambda }{\mathsf{\Gamma }}_{\lambda \nu }^{\pi }$$

(47)

The Ricci scalar or scalar curvature is described as

$$R=g^{\mu \nu }{R}_{\mu \nu }$$

(48)

Contraction of the Bianchi Identity in Equation (45) gives

$$R_{\mu \nu }-\frac{1}{2}{g}_{\mu \nu }R$$

(49)

which is the Einstein tensor. Now we can write basic equations of general relativity

$$R_{\mu \nu }-\frac{1}{2}{g}_{\mu \nu }R=8\pi T_{\mu \nu }$$

(50)

or

$$G_{\mu \nu }=8\pi T_{\mu \nu }$$

(51)

$$G_{\mu \nu }\propto T_{\mu \nu }$$

(52)

These are written with cosmological constant also. From Equation (52)

$$G_{\mu \nu }+\mathsf{\Lambda }{g}_{\mu \nu }=8\pi T_{\mu \nu }$$

(53)

Energy-momentum tensor $T_{\mu \nu }$ is the source term for the metric tensor $g_{\mu \nu }$ which for a most general matter-energy fluid that is consistent with the assumption of homogeneity and isotropy represents a perfect fluid and has the form

$$T_{\mu \nu }=\left(\rho +p\right)u_{\mu }{u}_{\nu }-p{g}_{\mu \nu }$$

(54)

where $u^{\mu }=\left(1,0,0,0\right)$ is the four velocity in a comoving frame of reference and

$$T_{\mu \nu }=\left(\begin{array}{cccc}\rho & 0& 0& 0\\ 0& p& 0& 0\\ 0& 0& p& 0\\ 0& 0& 0& p\end{array}\right)$$

(55)

3. Relativistic Cosmology

Relativistic cosmology was founded on three fundamental principles

• Cosmological principle;
• Weyl’s principle;
• General relativity.

These are illustrated in the following subsections.

3.1. Cosmological Principle

The cosmological principle states that on sufficiently large scale, the universe is homogenous and isotropic at any time. Therefore, it is the same for all observers and has similar properties on larger scales. The principle is the generalization of Copernican principle and almost all the standard cosmological models of the spacetime underpin it. It has two forms:

(1)

Cosmological principle with respect to spatial invariance

(2)

Cosmological principle with respect to temporal invariance

In special invariance, we suppose the invariance of space with respect to translational and rotational properties known as homogeneity and isotropy, respectively, and the principle may be regarded as cosmological principle. Under both the invariant properties the space remains isomorphic. A perfect cosmological principle incorporates temporal homogeneity and isotropy which was employed by the steady state theory of the eternal universe and was not supported by the observation and was disfavored. For a local observer the principle might not be satisfied as the Earth and the solar system are not homogeneous and isotropic since the matter clumps together to form objects like planets, stars, galaxies with voids of vacuum-like in between them but on the larger scales of about MP > 1000 Pc the universe obeys the cosmological principle. The uniformity of CMBR in all directions (homogeneity and isotropy) provides the confirmatory proof of the cosmological principle. It is the generalization of Copernican Principle which incorporates homogeneity and isotropy. Homogeneity means location independence, i.e., all places in the universe at galactic scales are indistinguishable. Isotropy gives direction independence, i.e., in whatever direction we look in the universe it appears same. Certainly Isotropy connotes homogeneity, but this not true vice versa. To better understand its geometric properties, we begin with 1-dimensional spaces and revise to the four-dimensional spaces and then observe how the four-dimensional spacetime geometrical properties can be understood in this perspective. It is necessary to understand what we mean by embedding of a geometric object in an n-dimensional space because of the reason FLRW metric incorporates example of embedding three dimensional spaces in four dimensional spacetime. Figure 8 delineates homogeneity and isotropy properties of space:

3.2. Weyl’s Principle

Weyl’s principle helps us consider the universal stuff as consisting of a fluid, the particles of which are constituted by galaxies. Therefore, what we name “the universe” is just cosmic fluid. In the cosmological spacetime, the world lines of the fundamental observers form a smooth bundle of time-like geodesics which would never meet except in the past singularity from where the universe emerged or at the future singularity if it would happen. The fundamental observers are those who comove with the cosmic fluid. The world lines of galaxies as fluid particles are always and everywhere orthogonal to the family of spatial hypersurfaces. The postulate was presented by Hermann Weyl (1885–1955) in 1923 which is essentially about the nature of matter in the universe [44]. He regarded the material content of the universe in the form of fluid whose constituent particles make a substratum in the cosmic fluid.

It means that in the substratum of spacetime it allows us to consider the structure of the universe as fluid. The Weyl principle introduces further symmetry in the structure of spacetime described by the metric tensor by considering the galaxies as test particles and postulates that the geodesics on which these galaxies move do not intersect. It states that the world lines of galaxies considered as “test particles” form a 3-bundle of non-intersecting geodesics orthogonal to a series of spacelike hypersurfaces. A simple illustration of the Weyl’s principle is given in the Figure 9:

3.3. General Relativity

General relativity provides the best existing theory of gravitation on cosmological scales and models it structured into the geometric structure of spacetime. In Section 3, we discussed its basic ingredients.

4. The Standard Model of Cosmology

The standard model in cosmology has been established on the most general homogeneous and isotropic spacetime. The standard model that propounds the hot big bang model of the universe is known as Friedmann–Lemaitre–Robertson–Walker (FLRW) line element which reads as in the Cartesian coordinates

$$d{s}^2=-d{t}^2+d{x}^2+d{y}^2+d{z}^2$$

(56)

and in the spherical coordinates, we have

$$\begin{array}{c}\hfill \begin{array}{cc}\hfill & d{s}^2=g=g_{\mu \nu }d{x}^{\mu }d{x}^{\nu }=-d{t}^2\hfill \\ \hfill & +a{\left(t\right)}^2\left(\frac{1}{1-k{r}^2}d{r}^2+r^{2}d{\theta }^2+r^2{sin}^2\theta d{\varphi }^2\right)\hfill \end{array}\end{array}$$

(57)

Or equivalently

$$\begin{array}{c}\hfill \begin{array}{cc}\hfill d{s}^2=\left(\begin{array}{cccc}{g}_{00}& g_{01}& g_{02}& g_{03}\\ g_{10}& g_{11}& g_{12}& g_{13}\\ g_{20}& g_{21}& g_{22}& g_{23}\\ g_{30}& g_{31}& g_{32}& g_{33}\end{array}\right)& =\left(\begin{array}{cccc}-1& 0& 0& 0\\ 0& \frac{a^2\left(t\right)}{1-k{r}^2}& 0& 0\\ 0& 0& a^2\left(t\right)r^2& 0\\ 0& 0& 0& a^2\left(t\right)r^2{sin}^2\theta \end{array}\right)\hfill \end{array}\end{array}$$

(58)

The predictions for the quantitative behavior of the expanding universe is enunciated suitably by the metric tensor and the scale factor as a function of time, i.e., $a\left(t\right)$ describes the scale of coordinate grid interrelating the coordinate distance with physical distance, i.e., in a smooth and homogeneously expanding universe.

4.1. Geometric Properties of the FLRW Line Element

From the line element in Equation (57)

$$d{s}^2=-d{t}^2+a{\left(t\right)}^2\left[\frac{1}{1-k{r}^2}d{r}^2+r^{2}d{\theta }^2+r^2{sin}^2\theta d{\varphi }^2\right]$$

(59)

As time flows only in one direction and the space obeys cosmological principle, therefore we are allowed to separate the metric in temporal and spatial parts. To understand the four dimensional spacetime geometry of FLRW universe we begin with the geometry of spatial part of the line element that is

$$a{\left(t\right)}^2\left(\frac{1}{1-k{r}^2}d{r}^2+r^{2}d{\theta }^2+r^2{sin}^2\theta d{\varphi }^2\right)$$

(60)

This is the spatial part of the metric in Equation (59) and is characterized by the scale factor $a\left(t\right)$, which is the function of time and 2nd curvature of the space k. These are obviously determined by the self-gravitating properties of the matter–energy content in the universe. The spatial part of the metric incorporates cosmological principle implying homogeneity and isotropy which provides the kinematics for the geometry of spacetime while we will observe afterwards that Einstein equations provide the dynamics into it through the scale factor $a\left(t\right)$.

4.2. Comoving Coordinates and Peculiar Velocities

The coordinates $\left(r,\theta ,\varphi \right)$ form the cosmological rest frame and are known as comoving coordinates. They can be considered constant because the particles remain at rest in these coordinates. Peculiar velocity is the motion of the particles with respect to comoving coordinates. Peculiar velocities of the galaxies and supernovae are ignored in cosmology in the expanding spacetime. As $p\left(a\right)\propto \frac{1}{a\left(t\right)}$, therefore momentum in expanding spacetime is red-shifted and freely moving particles come to rest in comoving coordinates. Physical distance between two points is calculated as thee scale factor $a\left(t\right)$ times the coordinate distance. The expression without scale factor inside the bracket is the pure kinematical statement of the geometry of spacetime

$$\frac{1}{1-k{r}^2}d{r}^2+r^{2}d{\theta }^2+r^2{sin}^2\theta d{\varphi }^2$$

(61)

and represents the line element of the three-dimensional space with hidden symmetry of being homogeneous and isotropic. It represents three geometries for three values of k.

4.3. The Geometry of Spherical World

For $k=+1$, the hypersurface is

$$\frac{1}{1-r^2}d{r}^2+r^{2}d{\theta }^2+r^2{sin}^2\theta d{\varphi }^2$$

(62)

and represents a three dimensional sphere embedded in a four dimensional Euclidean space. This space is finite and closed.

4.4. The Geometry of Hyperbolic World

For $k=-1$, the hypersurface is

$$\frac{1}{1+r^2}d{r}^2+r^{2}d{\theta }^2+r^2{sin}^2\theta d{\varphi }^2$$

(63)

and represents a three-dimensional hypersphere or hyperbola embedded in a four-dimensional pseud-Euclidean space. This space is infinite and open.

4.5. The Geometry of Euclidean World

For $k=0$, the hypersurface is

$$d{r}^2+r^{2}d{\theta }^2+r^2{sin}^2\theta d{\varphi }^2$$

(64)

and represents a three-dimensional Euclidean flat space. This space is also infinite and open. Now to determine Friedmann equations, we write first the components of the metric tensor, since the metric is diagonal due to homogeneity and isotropy therefore we have these diagonal components

$$\begin{array}{c}\hfill \begin{array}{cc}\hfill & g_{00}=g_{tt}=-1\hfill \\ \hfill & g_{11}=g_{rr}=\frac{a^2\left(t\right)}{1-k{r}^2}\hfill \\ \hfill & g_{22}=g_{\theta \theta }=a^2\left(t\right)r^2\hfill \\ \hfill & g_{33}=g_{\varphi \varphi }=a^2\left(t\right)r^2{sin}^2\theta \hfill \end{array}\end{array}$$

(65)

Now, we turn to solve the FLRW metric and begins with finding Christoffel symbols of 2nd kind or the affine connections which are given by

$${\mathsf{\Gamma }}_{\mu \nu }^{\sigma }=g^{\sigma \lambda }{\mathsf{\Gamma }}_{\mu \nu \lambda }=\frac{1}{2}{g}^{\sigma \lambda }\left(\frac{\partial g_{\mu \lambda }}{\partial x^{\nu }}+\frac{\partial g_{\nu \lambda }}{\partial x^{\mu }}+\frac{\partial g_{\mu \nu }}{\partial x^{\lambda }}\right)$$

(66)

In four dimensions these will have ${\left(4\right)}^3=64$ components. The four generalized cases emerge in four dimensions for $\mu$, $\nu$, $\lambda$, and $\sigma$.

Case I: $\mu =\nu =\lambda$

$${\mathsf{\Gamma }}_{\mu \mu }^{\mu }=\frac{1}{2}\frac{\partial }{\partial x^{\mu }}log{g}_{\mu \mu }$$

(67)

In four dimensions

$$\begin{array}{c}{\mathsf{\Gamma }}_{00}^0{\mathsf{\Gamma }}_{11}^1\hfill \\ {\mathsf{\Gamma }}_{22}^2{\mathsf{\Gamma }}_{33}^3\hfill \end{array}$$

(68)

will emerge.

Case II: $\sigma =\mu$, $\mu \ne \nu$

$${\mathsf{\Gamma }}_{\mu \lambda }^{\mu }=\frac{1}{2}\frac{\partial }{\partial x^{\lambda }}log{g}_{\mu \mu }$$

(69)

In four dimensions the following twelve cases

$$\begin{array}{c}{\mathsf{\Gamma }}_{01}^0{\mathsf{\Gamma }}_{02}^0{\mathsf{\Gamma }}_{03}^0{\mathsf{\Gamma }}_{10}^1\hfill \\ {\mathsf{\Gamma }}_{12}^1{\mathsf{\Gamma }}_{13}^1{\mathsf{\Gamma }}_{20}^2{\mathsf{\Gamma }}_{21}^2\hfill \\ {\mathsf{\Gamma }}_{23}^2{\mathsf{\Gamma }}_{30}^3{\mathsf{\Gamma }}_{31}^3{\mathsf{\Gamma }}_{32}^3\hfill \end{array}$$

(70)

will emerge.

Case III: $\sigma =\mu$, $\mu =\lambda$

$${\mathsf{\Gamma }}_{\lambda \lambda }^{\mu }=-\frac{1}{2g_{\mu \mu }}\frac{\partial g_{\lambda \lambda }}{\partial x^{\mu }}$$

(71)

In four dimensions the following twelve cases

$$\begin{array}{c}{\mathsf{\Gamma }}_{11}^0{\mathsf{\Gamma }}_{22}^0{\mathsf{\Gamma }}_{33}^0{\mathsf{\Gamma }}_{00}^1\hfill \\ {\mathsf{\Gamma }}_{22}^1{\mathsf{\Gamma }}_{33}^1{\mathsf{\Gamma }}_{00}^2{\mathsf{\Gamma }}_{11}^2\hfill \\ {\mathsf{\Gamma }}_{33}^2{\mathsf{\Gamma }}_{00}^3{\mathsf{\Gamma }}_{11}^3{\mathsf{\Gamma }}_{22}^3\hfill \end{array}$$

(72)

will emerge.

Case IV: $\sigma \ne \mu \ne \nu$

$${\mathsf{\Gamma }}_{\mu \nu }^{\sigma }=g^{\sigma \lambda }{\mathsf{\Gamma }}_{\mu \nu \lambda }=\frac{1}{2}{g}^{\sigma \lambda }\left(\frac{\partial g_{\mu \lambda }}{\partial x^{\nu }}+\frac{\partial g_{\nu \lambda }}{\partial x^{\mu }}+\frac{\partial g_{\mu \nu }}{\partial x^{\lambda }}\right)=0$$

(73)

In four dimensions the following twenty four cases:

$$\begin{array}{c}{\mathsf{\Gamma }}_{12}^0{\mathsf{\Gamma }}_{21}^0{\mathsf{\Gamma }}_{13}^0{\mathsf{\Gamma }}_{31}^0\hfill \\ {\mathsf{\Gamma }}_{23}^0{\mathsf{\Gamma }}_{32}^0{\mathsf{\Gamma }}_{02}^1{\mathsf{\Gamma }}_{20}^1\hfill \\ {\mathsf{\Gamma }}_{03}^1{\mathsf{\Gamma }}_{30}^1{\mathsf{\Gamma }}_{23}^1{\mathsf{\Gamma }}_{32}^1\hfill \\ {\mathsf{\Gamma }}_{01}^2{\mathsf{\Gamma }}_{10}^2{\mathsf{\Gamma }}_{03}^2{\mathsf{\Gamma }}_{30}^2\hfill \\ {\mathsf{\Gamma }}_{13}^2{\mathsf{\Gamma }}_{31}^2{\mathsf{\Gamma }}_{01}^3{\mathsf{\Gamma }}_{10}^3\hfill \\ {\mathsf{\Gamma }}_{12}^3{\mathsf{\Gamma }}_{21}^3{\mathsf{\Gamma }}_{20}^3{\mathsf{\Gamma }}_{02}^3\hfill \end{array}$$

(74)

emerge and vanish.

4.6. Non-Vanishing Christoffel Symbols

We determine the following non-vanishing Christoffel symbols of the 2nd kind with the help of formula given in Equation (66) for the metric in Equation (57), which is the metric of universe in standard cosmology.

$$\begin{array}{c}{\mathsf{\Gamma }}_{11}^1=\frac{kr}{1-k{r}^2}\hfill \\ {\mathsf{\Gamma }}_{10}^1={\mathsf{\Gamma }}_{01}^1={\mathsf{\Gamma }}_{20}^2={\mathsf{\Gamma }}_{02}^2={\mathsf{\Gamma }}_{30}^3={\mathsf{\Gamma }}_{03}^3=\frac{\dot{a}\left(t\right)}{a\left(t\right)}\hfill \\ {\mathsf{\Gamma }}_{21}^2={\mathsf{\Gamma }}_{12}^2={\mathsf{\Gamma }}_{31}^3={\mathsf{\Gamma }}_{13}^3=\frac{1}{r}\hfill \\ {\mathsf{\Gamma }}_{32}^3={\mathsf{\Gamma }}_{23}^3=\frac{sin\theta }{cos\theta }\hfill \\ {\mathsf{\Gamma }}_{11}^0=\frac{a\left(t\right)\dot{a}\left(t\right)}{1-k{r}^2}\hfill \\ {\mathsf{\Gamma }}_{22}^0=a\left(t\right)\dot{a}\left(t\right)r^2\hfill \\ {\mathsf{\Gamma }}_{33}^0=a\left(t\right)\dot{a}\left(t\right)r^2{sin}^2\theta \hfill \\ {\mathsf{\Gamma }}_{22}^1=-r\left(1-k{r}^2\right)\hfill \\ {\mathsf{\Gamma }}_{33}^1=-r{sin}^2\theta \left(1-k{r}^2\right)\hfill \\ {\mathsf{\Gamma }}_{33}^2=-sin\theta cos\theta \hfill \end{array}$$

(75)

4.7. Riemann Curvature Tensor

The Riemann curvature tensor $R_{\mu \nu \lambda }^{\sigma }$ has ${\left(4\right)}^4=256$ components in four dimensions from which only twenty components can possibly be non-vanishing. The Riemann tensor is given by

$$R_{\mu \nu \lambda }^{\sigma }=\frac{\partial }{\partial x^{\nu }}{\mathsf{\Gamma }}_{\mu \lambda }^{\sigma }-\frac{\partial }{\partial x^{\lambda }}{\mathsf{\Gamma }}_{\mu \nu }^{\sigma }+{\mathsf{\Gamma }}_{\mu \lambda }^n{\mathsf{\Gamma }}_{n\nu }^{\sigma }-{\mathsf{\Gamma }}_{\mu \nu }^n{\mathsf{\Gamma }}_{n\lambda }^{\sigma }$$

(76)

The possibly non-vanishing twenty components are given by

$$\begin{array}{c}{R}_{110}^0{R}_{220}^0{R}_{330}^0{R}_{221}^0\hfill \\ R_{331}^0{R}_{001}^1{R}_{221}^1{R}_{331}^1\hfill \\ R_{332}^1{R}_{002}^2{R}_{112}^2{R}_{332}^2\hfill \\ R_{021}^2{R}_{003}^3{R}_{113}^3{R}_{223}^3\hfill \\ R_{031}^3\hfill \end{array}$$

(77)

The non-vanishing components are

$$\begin{array}{c}{R}_{110}^0=-\frac{a\left(t\right)\ddot{a}\left(t\right)}{1-k{r}^2}\hfill \\ R_{101}^0=\frac{a\left(t\right)\ddot{a}\left(t\right)}{1-k{r}^2}\hfill \\ R_{010}^1=-\frac{\ddot{a}\left(t\right)}{a\left(t\right)}\hfill \\ R_{001}^1=\frac{\ddot{a}\left(t\right)}{a\left(t\right)}\hfill \\ R_{220}^0=-a\left(t\right)\ddot{a}\left(t\right)r^2\hfill \\ R_{202}^0=a\left(t\right)\ddot{a}\left(t\right)r^2\hfill \\ R_{020}^2=R_{030}^3=-\frac{\ddot{a}\left(t\right)}{a\left(t\right)}\hfill \\ R_{002}^2=R_{003}^3=\frac{\ddot{a}\left(t\right)}{a\left(t\right)}\hfill \\ R_{330}^0=-a\left(t\right)\ddot{a}\left(t\right)r^2{sin}^2\theta \hfill \\ R_{303}^0=a\left(t\right)\ddot{a}\left(t\right)r^2{sin}^2\theta \hfill \\ R_{221}^1=R_{223}^3=-r^2\left(k+{\dot{a}}^2\left(t\right)\right)\hfill \\ R_{212}^1=R_{232}^3=r^2\left(k+{\dot{a}}^2\left(t\right)\right)\hfill \\ R_{121}^2=R_{131}^3=\frac{k+{\dot{a}}^2\left(t\right)}{1-k{r}^2}\hfill \\ R_{112}^2=R_{113}^3=-\frac{k+{\dot{a}}^2\left(t\right)}{1-k{r}^2}\hfill \\ R_{331}^1=R_{332}^2=-r^2{sin}^2\theta \left(k+{\dot{a}}^2\left(t\right)\right)\hfill \\ R_{313}^1=R_{323}^2=r^2{sin}^2\theta \left(k+{\dot{a}}^2\left(t\right)\right)\hfill \end{array}$$

(78)

4.8. Ricci Curvature Tensor and Ricci Scalar

Ricci tensor $\left(R_{\mu \nu }\right)$ is obtained by contracting Riemann tensor $R_{\mu \nu \lambda }^{\sigma }$. We contract it by placing $\lambda =\sigma$, so that $R_{\mu \nu \lambda }^{\sigma }=R_{\mu \nu \lambda }^{\lambda }=R_{\mu \nu }$ In four dimensions it has ${\left(4\right)}^2=16$ components:

$$\begin{array}{c}{R}_{00}{R}_{11}{R}_{22}{R}_{33}{R}_{01}\hfill \\ R_{10}{R}_{02}{R}_{20}{R}_{03}{R}_{30}\hfill \\ R_{12}{R}_{21}{R}_{31}{R}_{13}{R}_{23}\hfill \\ R_{32}\hfill \end{array}$$

(79)

The non-vanishing components are

$$\begin{array}{c}{R}_{00}=3\frac{\ddot{a}}{a}\hfill \\ R_{11}=-\frac{a\left(t\right)\ddot{a}\left(t\right)+2k+2{\dot{a}}^2}{1-k{r}^2}\hfill \\ R_{22}=-r^2\left(a\left(t\right)\ddot{a}\left(t\right)+2k+2{\dot{a}}^2\right)\hfill \\ R_{33}=-r^2{sin}^2\theta \left(a\left(t\right)\ddot{a}\left(t\right)+2k+2{\dot{a}}^2\right)\hfill \end{array}$$

(80)

Ricci scalar $\left(R\right)$ is obtained by contracting Ricci tensor

$$R=g^{\mu \nu }{R}_{\mu \nu }$$

(81)

Using double sums and simplifying in four dimensions, we have

$$R=g^{00}{R}_{00}+g^{11}{R}_{11}+g^{22}{R}_{22}+g^{33}{R}_{33}$$

(82)

$$R=-6\left[\frac{\ddot{a}\left(t\right)}{a\left(t\right)}+{\left(\frac{\dot{a}\left(t\right)}{a\left(t\right)}\right)}^2+\frac{k}{a^2\left(t\right)}\right]$$

(83)

4.9. Einstein Tensor $\left(G_{\mu \nu }\right)$

Einstein tensor is defined in terms of Ricci tensor $R_{\mu \nu }$, Ricci scalar R, and the metric tensor $g_{\mu \nu }$. It is expressed as

$$G_{\mu \nu }=R_{\mu \nu }-\frac{1}{2}{g}_{\mu \nu }R$$

(84)

In four dimensions it has ${\left(4\right)}^2=16$, components. These are

$$\begin{array}{c}{G}_{00}{G}_{11}{G}_{22}{G}_{33}{G}_{01}\hfill \\ G_{10}{G}_{02}{G}_{20}{G}_{03}{G}_{30}\hfill \\ G_{12}{G}_{21}{G}_{31}{G}_{13}{G}_{23}\hfill \\ G_{32}\hfill \end{array}$$

(85)

The non-vanishing components are

$$\begin{array}{c}{G}_{00}=-3\left[{\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}\right]\hfill \\ G_{11}=g_{11}\left[2\frac{\ddot{a}}{a}+{\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}\right]\hfill \\ G_{22}=g_{22}\left[2\frac{\ddot{a}}{a}+{\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}\right]\hfill \\ G_{33}=g_{33}\left[2\frac{\ddot{a}}{a}+{\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}\right]\hfill \end{array}$$

(86)

In Equation (86), the spatial components of Einstein tensor can be written in a single equation of tensorial nature.

$$G_{\mu \nu }=g_{\mu \nu }\left[2\frac{\ddot{a}}{a}+{\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}\right]$$

(87)

where $\mu =\nu =1,2,3$, and mixed Einstein tensor can be found by $g^{\zeta \nu }{G}_{\mu \nu }=G_{\mu }^{\zeta }$

$$\begin{array}{c}{G}_0^0=3\left[{\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}\right]\hfill \\ G_1^1=g_{11}\left[2\frac{\ddot{a}}{a}+{\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}\right]\hfill \\ G_2^2=g_{22}\left[2\frac{\ddot{a}}{a}+{\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}\right]\hfill \\ G_3^3=g_{33}\left[2\frac{\ddot{a}}{a}+{\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}\right]\hfill \end{array}$$

(88)

Now, we calculate the energy-momentum tensor of a perfect fluid in mixed form. Cosmological principle and Weyl’s postulate imply the material content of the universe to be regarded as perfect fluid [1,2,3].

$$g^{\zeta \nu }{T}_{\mu \nu }=T_{\mu }^{\zeta }=\left(\begin{array}{cccc}{T}_{00}& T_{01}& T_{02}& T_{03}\\ T_{10}& T_{11}& T_{12}& T_{13}\\ T_{20}& T_{21}& T_{22}& T_{23}\\ T_{30}& T_{31}& T_{32}& T_{33}\end{array}\right)=\left(\begin{array}{cccc}\rho & 0& 0& 0\\ 0& -p& 0& 0\\ 0& 0& -p& 0\\ 0& 0& 0& -p\end{array}\right)$$

(89)

The non-vanishing components of energy-momentum tensor are

$$\begin{array}{c}{T}_0^0=\rho T_1^1=-p\hfill \\ T_2^2=-p{T}_3^3=-p\hfill \end{array}$$

(90)

Putting the values of Einstein tensor $G_{\mu \nu }$ and energy-momentum tensor $T_{\mu \nu }$ from Equations (88) and (89), respectively, in Einstein field equations

$$G_{\mu }^{\zeta }=8\pi T_{\mu }^{\zeta }$$

(91)

$$\begin{array}{c}3\left[{\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}\right]=8\pi G\rho \hfill \\ g_{11}\left[2\frac{\ddot{a}}{a}+{\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}\right]=-8\pi Gp\hfill \\ g_{22}\left[2\frac{\ddot{a}}{a}+{\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}\right]=-8\pi Gp\hfill \\ g_{33}\left[2\frac{\ddot{a}}{a}+{\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}\right]=-8\pi Gp\hfill \end{array}$$

(92)

5. Derivation of Friedmann’s Equations

Now, using the Einstein field equations, we set to derive the Friedmann’s Equations that describe the evolution of the universe by relating the large-scale geometrical characteristics of spacetime to the large-scale distribution of matter–energy and momentum. From Equation (92), we can write

$$3\left[{\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}\right]=8\pi G\rho$$

(93)

$$2\frac{\ddot{a}}{a}+{\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}=-8\pi Gp$$

(94)

For other two components listed in Equation (92) the 2nd and 3rd components repeat, therefore we will write only one time from the three components. From Equations (93) and (94) we derive the Friedmann’s Equations and an equation for the conservation of matter. Substituting Equation (93) in Equation (94) and performing simplification we get

$$\frac{\ddot{a}}{a}=-\frac{4\pi G}{3}\left(\rho +3p\right)$$

(95)

and from Equation (93) which is the time-time component of the Einstein Equations.

$${\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}=\frac{8\pi G}{3}\rho$$

(96)

for

$$\frac{\dot{a}}{a}=H$$

(97)

which is Hubble parameter and gives expansion rate. The above Equation (96) can be written as

$$H^2+\frac{k}{a^2}=\frac{8\pi G}{3}\rho$$

(98)

differentiating Equation (97) with respect to time ‘t’

$${\partial }_{t}H={\partial }_t\left(\frac{\dot{a}}{a}\right)$$

(99)

we obtain

$$\dot{H}=\frac{\ddot{a}}{a}-H^2$$

(100)

which gives

$$\dot{H}+H^2=\frac{\ddot{a}}{a}$$

(101)

Therefore, that Equation (95) takes the form in terms of Hubble parameter.

$$\dot{H}+H^2=-\frac{4\pi G}{3}\left(\rho +3p\right)$$

(102)

we can also find

$$\dot{H}=-\frac{4\pi G}{3}\left(\rho +3p\right)-H^2$$

(103)

From Equation (98) $H^2=\frac{8\pi G}{3}\rho$ with $k=0$, for flat universe substituting it in Equation (103) above

$$\dot{H}=-\frac{4\pi G}{3}\left(\rho +3p\right)-\frac{8\pi G}{3}\rho$$

(104)

which results in

$${\partial }_{t}H=-4\pi G\left(\rho +p\right)$$

(105)

Now, differentiating Equation (93) with respect to time after shifting the factor 3 on the right side, we have

$$\frac{\dot{a}}{a}\left[2\frac{\ddot{a}}{a}-2{\left(\frac{\dot{a}}{a}\right)}^2-2\frac{k}{a^2}\right]=\frac{8\pi G}{3}\dot{\rho }$$

(106)

subtracting now Equation (93) from Equation (94), we obtain

$$2\frac{\ddot{a}}{a}-2{\left(\frac{\dot{a}}{a}\right)}^2-2\frac{k}{a^2}=-8\pi G\left(\rho +p\right)$$

(107)

substituting Equation (107) in Equation (106), after simplification we have

$$\dot{\rho }+3\frac{\dot{a}}{a}\left(\rho +p\right)=0$$

(108)

Cosmological principle compels us to consider a fluid in which inhomogeneities will be considered smoothed out and evolution of the universe shall be considered in the form of perfect fluid characterized by energy density $\rho$ and isotropic pressure p. Further we consider that the pressure of the fluid depends only on the density neglecting its impact on the volume and the temperature, i.e., $p=p\left(\rho \right)$ which defines a barotropic fluid. In addition, pressure and density bear a linear relationship

$$p\propto \rho \Rightarrow p=w\rho$$

(109)

where $w=\frac{p}{\rho }$ is a dimensionless constant known as equation of state parameter. Substituting Equation (109) in Equation (108), we have another form of energy conservation for the equation of state parameter w,

$$\frac{\dot{\rho }}{\rho }+3\frac{\dot{a}}{a}\left(1+w\right)=0$$

(110)

Now, Equations (95), (96), and (108) represent two Friedmann’s Equations, namely, acceleration and evolution equations, and the equation of conservation, respectively. According to this equation, the evolution of all kinds of matter is determined by the conservation of energy and momentum.

Friedmann Equations with Cosmological Constant $\mathsf{\Lambda }$

We have to incorporate dark matter and dark energy in the matter–energy content due to the significance of their role in current accelerated expansion and the present Minkowskian flat geometry of the universe. Therefore, their role is however unavoidable in the evolution of the universe. The solution of FLRW line element gives the Friedman’s equations using Einstein field equations with cosmological constant $\mathsf{\Lambda }$ written usually in the form

$$G_{\mu \nu }+\mathsf{\Lambda }{g}_{\mu \nu }=8\pi T_{\mu \nu }$$

(111)

and Friedmann’s equations with cosmological constant $\mathsf{\Lambda }$ can be worked out

$$\frac{{\dot{a}}^2}{a^2}+\frac{k}{a^2}=\frac{8\pi G}{3}\rho +\frac{\mathsf{\Lambda }}{3}$$

(112)

$$\frac{\ddot{a}}{a}=-\frac{4\pi G}{3}\left(\rho +3p\right)+\frac{\mathsf{\Lambda }}{3}$$

(113)

The equation of energy conservation can also be calculated from these Friedman equations in the presence of cosmological constant $\mathsf{\Lambda }$. Multiplying Equation (112) with $3a^2$, differentiating it with respect to time and then dividing by $\dot{a}$, we have

$$6\ddot{a}=8\pi Ga\left(2\rho +\frac{a}{\dot{a}}\dot{\rho }\right)+2\mathsf{\Lambda }a$$

(114)

dividing Equation (114) by a.

$$6\frac{\ddot{a}}{a}=8\pi G\left(2\rho +\frac{a}{\dot{a}}\dot{\rho }\right)+2\mathsf{\Lambda }$$

(115)

Substituting now the 2nd Friedman Equation from Equation (113) in it, we have

$$6\left(-\frac{4\pi G}{3}\left(\rho +3p\right)+\frac{\mathsf{\Lambda }}{3}\right)=8\pi G\left(2\rho +\frac{a}{\dot{a}}\dot{\rho }\right)+2\mathsf{\Lambda }$$

(116)

after simplification, we obtain

$$\dot{\rho }+3\frac{\dot{a}}{a}\left(\rho +p\right)=0$$

(117)

where $\rho$ and p are contributed by all whatever exists and constitutes the universe.

6. A Geometric Object Embedded in an n-Dimensional Space

An object cannot be placed in a space whose dimensions are equal or less than the object to be placed, rather the space must have larger number of dimensions in order to let the object allow rest in it. The presence of an object in a space having larger dimensions than the object is called embedding of it in that space.

6.1. Intrinsic Geometry

The properties of the geometry that we have access to, based on visualization of the two dimensional beings are called intrinsic because two dimensional beings cannot observe how surfaces are shaped in three or higher dimensional spaces.

6.2. Extrinsic Geometry

The properties of the geometry that we have access to, based on visualization of higher dimensional creature are called extrinsic because higher-dimensional creature can observe how surfaces are shaped in three- or higher-dimensional spaces. The geometrical properties related to an object describing how it has been embedded in some higher dimensional space. Extrinsic geometrical properties depend on how the bodies are placed in the space and how they affect it. The geometry which comes into existence due to interaction between space and the body placed in it describes the extrinsic properties. General relativity considers the geometry of spacetime as the extrinsic property of an object and owes its existence due to the body being present in it.

6.3. The Geometry of 2-Sphere Embedded in Three-Dimensional Space

We consider a three-dimensional Euclidean space where three dimensions namely length, width, and height are represented by three coordinate axes, respectively, as we know this space consists of points separate from time, and therefore we do not call its points as events. We assign the triplet of three Cartesian coordinates $\left(x,y,z\right)$ to each point of it, where x, y, and z are measured along the three axes of it. The sketch of embedding the geometry of 2-sphere in three dimensional space is drawn in Figure 10:

The line element in this space is given by

$$d{s}^2=d{x}^2+d{y}^2+d{z}^2$$

(118)

Considering now a sphere with its center at the origin of this coordinate system and envisaging its radius to be a, the surface in Cartesian coordinates $\left(x,y,z\right)$ where x, y, and z are along the three axes of three-dimensional Euclidean space. The equation of sphere of this sphere is

$$x^2+y^2+z^2=a^2$$

(119)

Differentiating Equation (119) with respect to time

$$2x\frac{dx}{dt}+2x\frac{dx}{dt}+2x\frac{dx}{dt}=0$$

(120)

Moreover, in differential form

$$2xdx+2ydy+2zdz=0$$

(121)

Solving Equation (121) for $dz$, we have

$$dz=-\frac{xdx+ydy}{z}$$

(122)

Finding the value of z from Equation (119)

$$z=\sqrt{a^2-(x^2+y^2)}$$

(123)

Substituting in Equation (122)

$$dz=-\frac{xdx+ydy}{\sqrt{a^2-(x^2+y^2)}}$$

(124)

The value of $dz=-\frac{xdx+ydy}{{\left[a^2-(x^2+y^2)\right]}^{\frac{1}{2}}}$ comes up with a sort of constraint on $dz$ which despite of being displaced by infinitesimally small amounts $dx$ and $dy$ from an arbitrary point on the surface of the sphere holds us on the surface of the sphere. Squaring $dz$ in Equation (124)

$$d{z}^2=\frac{{(xdx+ydy)}^2}{a^2-(x^2+y^2)}$$

(125)

Putting in Equation (118), the line element takes the form by substituting for $d{z}^2$

$$d{s}^2=d{x}^2+d{y}^2+\frac{{(xdx+ydy)}^2}{a^2-(x^2+y^2)}$$

(126)

The value of the line element in Equation (126) represents the line element for a sphere in terms of Cartesian coordinates $\left(x,y,z\right)$. We further observe that the line element in Equation (126) has a coordinate singularity at $a^2=x^2+y^2$ in correspondence with the equator of the sphere and in relation to the point A, otherwise at the equator in the intrinsic geometry of 2-sphere there exists no such physical situation. The embedding scenario manifests how the coordinates $(x,y)$ cover the whole surface of the sphere uniquely up to this point A. The geometry of 2-sphere in these coordinates becomes geometrically meaningful in three-dimensional Euclidean space. We can transform the line element in Equation (126) above into spherical polar coordinates by taking

$$\begin{array}{c}x=rsin\theta cos\varphi \hfill \\ y=rsin\theta sin\varphi \hfill \end{array}$$

(127)

where we differentiate each of x and y with respect to $\theta$ and $\varphi$ alternately to find

$$\begin{array}{c}dx=sin\theta cos\varphi dr+rcos\varphi cos\theta d\theta -rsin\theta sin\varphi d\varphi \hfill \\ dy=sin\theta sin\varphi dr+rsin\varphi cos\theta d\theta +rsin\theta cos\varphi d\varphi \hfill \end{array}$$

(128)

adding the values of x, y given in Equation (127) after taking square of both equations in it, we get

$$x^2+y^2=r^2{sin}^2\theta$$

(129)

adding $dx$ and $dy$ in Equation (128) after taking square of both equations in it, we possess

$$d{x}^2+d{y}^2={\left(sin\theta dr+rcos\theta d\theta \right)}^2+r^2{sin}^2\theta d{\varphi }^2$$

(130)

we find the expression

$$\begin{array}{c}xdx+ydy=\left(sin\theta dr+rcos\theta d\theta \right)\left(xcos\varphi +ysin\varphi \right)\hfill \\ -rsin\theta d\varphi \left(xsin\varphi -ycos\varphi \right)\hfill \end{array}$$

(131)

squaring Equation (131), we have

$${\left(xdx+ydy\right)}^2={\left(\begin{array}{c}\left(sin\theta dr+rcos\theta d\theta \right)\left(xcos\varphi +ysin\varphi \right)\hfill \\ -rsin\theta d\varphi \left(xsin\varphi -ycos\varphi \right)\hfill \end{array}\right)}^2$$

(132)

Now substituting Equations (129), (130), and (132) in Equation (126) and simplifying to have the following form:

$$d{s}^2=d{r}^2+r^{2}d{\theta }^2+r^2{sin}^2\theta d{\varphi }^2$$

(133)

The value of the line element in Equation (133) gives the line element for a sphere in terms of Spherical polar coordinates $\left(r,\theta ,\varphi \right)$. The line element in Equation (126) results in an alternative form for

$$\begin{array}{c}x=\xi cos\varphi \hfill \\ y=\xi sin\varphi \hfill \end{array}$$

(134)

$$d{s}^2=\frac{a^2}{a^2-{\xi }^2)}d{\xi }^2+{\xi }^{2}d\varphi$$

(135)

The line element in Equation (135) above gives us, in addition, freedom to choose an arbitrary point on the surface of the sphere by $\xi =0$ as the origin of the coordinate system. This freedom connotes in it as a hidden symmetry. We can develop $\xi$ and $\varphi$ coordinate curves on the surface of the sphere by generating a standard coordinate system $(\xi ,\varphi )$ on the tangent plane at the point A that projects vertically downward onto the surface of the sphere. We further observe that the line element in Equation (135) has a coordinate singularity at $a=\xi$ in correspondence with the equator of the sphere in relation to the point A, otherwise at the equator in the intrinsic geometry of 2-sphere there exists no shade of occurrence of such situation. The embedding picture manifests how the coordinates $(\xi ,\varphi )$ cover the whole surface of the sphere uniquely up to this point A. The geometry of 2-sphere in these coordinates becomes geometrically meaningful in three dimensional Euclidean space.

6.4. The Geometry of 3-Sphere Embedded in Four Dimensional Euclidean Space

Spaces with dimensions higher than three are now significant in mathematical sciences to have proper description of the physical universe. We consider a four-dimensional Euclidean space which can be considered mathematical extension of three-dimensional Euclidean space. Minkowski used a four-dimensional spacetime to explain the phenomena of the physical world as required by special relativity. The structure of Euclidean four-dimensional space is simple as compared to the Minkowskian structure of spacetime. Minkowskian four dimensional spacetime is pseudo-Euclidean space. In four-dimensional Euclidean space, we assign the quadruplet of four Cartesian coordinates $\left(x,y,z,w\right)$ to each point of it, where x, y, z, and w are along the four axes of it. The line element in this space is given by

$$d{s}^2=d{x}^2+d{y}^2+d{z}^2+d{w}^2$$

(136)

Considering now a sphere with its center at the origin of this coordinate system with radius a, the surface in Cartesian coordinates $\left(x,y,z,w\right)$ where x, y, z and w are along the four axes of four dimensional Euclidean space. The equation of the sphere reads as

$$x^2+y^2+z^2+w^2=a^2$$

(137)

Differentiating Equation (137) with respect to time,

$$2x\frac{dx}{dt}+2y\frac{dy}{dt}+2z\frac{dz}{dt}+2w\frac{dw}{dt}=0$$

(138)

And in differential form

$$2xdx+2ydy+2zdz+2wdw=0$$

(139)

Finding out the value of $dw$ from Equation (138), we get

$$dw=-\frac{xdx+ydy+zdz}{w}$$

(140)

Now finding the value of w from Equation (137)

$$w=\sqrt{a^2-(x^2+y^2+z^2)}$$

(141)

Substituting in Equation (140), we obtain

$$dw=-\frac{xdx+ydy+zdz}{\sqrt{a^2-(x^2+y^2+z^2)}}$$

(142)

The value of $dw=-\frac{xdx+ydy+zdz}{{\left[a^2-(x^2+y^2+z^2)\right]}^{\frac{1}{2}}}$ provides a sort of constraint on $dw$ which, though displaced by infinitesimally small amounts $dx$, $dy$, $dz$ from an arbitrary point on the surface of the sphere holds us stuck on the surface of the sphere. squaring Equation (142)

$$d{w}^2=-\frac{{\left(xdx+ydy+zdz\right)}^2}{a^2-(x^2+y^2+z^2)}$$

(143)

substituting now in Equation (136), the line element takes the form for the value of $d{w}^2$

$$d{s}^2=d{x}^2+d{y}^2+d{z}^2+\frac{{\left(xdx+ydy+zdz\right)}^2}{a^2-(x^2+y^2+z^2)}$$

(144)

The value of the line element in Equation (144) gives the line element for a sphere in terms of Cartesian coordinates $\left(x,y,z,w\right)$, We further observe that the line elements in Equation (144) has a coordinate singularity at $a^2=x^2+y^2+z^2$ in correspondence with the equator of the sphere relative to the point A; otherwise, at the equator in the intrinsic geometry of the 3-sphere, there does not exist any situation like this. The embedding picture manifests how the coordinates $(x,y,z)$ cover the whole surface of the sphere uniquely up to this point A. The geometry of 3-sphere in these coordinates becomes geometrically meaningful in four dimensional Euclidean space. We transform the line element in Equation (144) into spherical polar coordinates which are given below,

$$\begin{array}{c}x=rsin\theta cos\varphi \hfill \\ y=rsin\theta sin\varphi \hfill \\ z=rcos\theta \hfill \end{array}$$

(145)

where we differentiate x, and y with respect to $\theta$ and with respect to $\varphi$ each and differentiate z with respect to $\theta$ only to find

$$\begin{array}{c}dx=sin\theta cos\varphi dr+rcos\varphi cos\theta d\theta -rsin\theta sin\varphi d\varphi \hfill \\ dy=sin\theta sin\varphi dr+rsin\varphi cos\theta d\theta +rsin\theta cos\varphi d\varphi \hfill \\ dz=rcos\theta \hfill \end{array}$$

(146)

Adding x, y, and z in Equation (145) after taking square of all three equations in it to obtain

$$x^2+y^2+z^2=r^2$$

(147)

Adding now $dx$, $dy$, and $dz$ in Equation (146) after taking square of all three equations in it to have

$$d{x}^2+d{y}^2+d{z}^2=d{r}^2+r^{2}d{\theta }^2+r^2{sin}^2\theta d{\varphi }^2$$

(148)

and the expression we calculate

$$\begin{array}{c}xdx+ydy+zdz=\left(sin\theta dr+rcos\theta d\theta \right)\left(xcos\varphi +ysin\varphi \right)\hfill \\ -rsin\theta d\varphi \left(xsin\varphi -ycos\varphi \right)+rcos\theta \left(cos\theta dr-rsin\theta d\theta \right)\hfill \end{array}$$

(149)

Squaring Equation (149), we have

$${\left(xdx+ydy+zdz\right)}^2={\left(\begin{array}{c}\left(sin\theta dr+rcos\theta d\theta \right)\left(xcos\varphi +ysin\varphi \right)\hfill \\ -rsin\theta d\varphi \left(xsin\varphi -ycos\varphi \right)\hfill \\ +rcos\theta \left(cos\theta dr-rsin\theta d\theta \right)\hfill \end{array}\right)}^2$$

(150)

Now, substituting Equations (147), (148), and (150) in Equation (144), after simplification we get

$$d{s}^2=\frac{a^2}{a^2-r^2}d{r}^2+r^{2}d{\theta }^2+r^2{sin}^2\theta d{\varphi }^2$$

(151)

It can further be expressed in the form

$$d{s}^2=\frac{1}{\left(1-\frac{r^2}{a^2}\right)}d{r}^2+r^{2}d{\theta }^2+r^2{sin}^2\theta d{\varphi }^2$$

(152)

It is important to note here that for $a\to \infty$ in the Equation (152) above. It reduces to the metric of ordinary three-dimensional Euclidean space

$$d{s}^2=d{r}^2+r^{2}d{\theta }^2+r^2{sin}^2\theta d{\varphi }^2$$

(153)

which we calculated in Equation (133). The metric in Equation (151) has a singularity at $r=a$, which is just a coordinate singularity and has nothing to do with physical reality of the sphere as we can observe. The line element in Equation (152) results in an alternative form for

$$\begin{array}{c}x=\xi cos\varphi \hfill \\ y=\xi sin\varphi \hfill \end{array}$$

(154)

$$d{s}^2=\frac{a^2}{(a^2-{\xi }^2)}d{\xi }^2+{\xi }^{2}d\varphi$$

(155)

The line element in Equation (155) gives us, in addition, freedom to choose an arbitrary point on the surface of the sphere by $\xi =0$ as the origin of the coordinate system. This freedom is implied by it as a hidden symmetry in it. We can develop $\xi$ and $\varphi$ coordinate curves on the surface of the sphere by generating a standard coordinate system $(\xi ,\varphi )$ on the tangent plane at the point A that projects vertically downward onto the surface of the sphere. We further observe that the line element in Equation (155) has a coordinate singularity at $a=\xi$ with respect to the equator of the sphere in relation with the point A, otherwise at the equator in the intrinsic geometry of 2-sphere there exists no such situation. The embedding picture manifests how the coordinates $(\xi ,\varphi )$ cover the whole surface of the sphere uniquely up to the point A. The geometry of the 2-sphere in these coordinates becomes geometrically meaningful in three dimensional Euclidean space.

7. Einstein’s Static Universe

Albert Einstein himself applied general relativity to the largest scale of spacetime [3] and presented the very first relativistic model of the universe laying the foundations of modern theoretical cosmology. The model was later on called as Einstein world or universe. For this purpose, Einstein modified his field equations by proposing an inbuilt energy density known as cosmological constant $\mathsf{\Lambda }$ in the geometrical structure of spacetime itself that provides repulsive gravity to keep the universe from expanding

$$G_{\mu \nu }+\mathsf{\Lambda }{g}_{\mu \nu }=8\pi T_{\mu \nu }$$

(156)

Equation (156) when solved for the most homogeneous and isotropic geometry of FLRW spacetime produces Friedmann equations as we derived earlier

$${\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}=\frac{8\pi G}{3}\rho +\frac{\mathsf{\Lambda }}{3}$$

(157)

$$\frac{\ddot{a}}{a}=-\frac{4\pi G}{3}\left(\rho +3p\right)+\frac{\mathsf{\Lambda }}{3}$$

(158)

As for a static universe $H=0$, which implies that $\frac{\ddot{a}}{a}=0$. Now a static universe possesses cold matter which means it does not has pressure, i.e., $p=0$, so Equations (157) and (158) reduce to the form, respectively,

$$\frac{8\pi G}{3}\rho +\frac{\mathsf{\Lambda }}{3}-\frac{k}{a^2}=0$$

(159)

and

$$-\frac{4\pi G}{3}\rho +\frac{\mathsf{\Lambda }}{3}=0$$

(160)

From above Equation (160), we have

$$\mathsf{\Lambda }=4\pi G\rho$$

(161)

Substituting this value of $\mathsf{\Lambda }$ in Equation (159), and having the equation simplified, we again get the value of $\mathsf{\Lambda }$ in terms of the curvature term k and the scalar factor $a\left(t\right)$, that is

$$\mathsf{\Lambda }=\frac{k}{a^2}$$

(162)

The line element for the static Einstein universe can be written now using FLRW metric. From above Equation (162) for $k=+1$, we have $a^2\left(t\right)={\mathsf{\Lambda }}^{-1}$, substituting in Equation (59), the static solution for closed universe becomes

$$d{s}^2=-d{t}^2+{\mathsf{\Lambda }}^{-1}\left(\frac{1}{1-k{r}^2}d{r}^2+r^{2}d{\theta }^2+r^2{sin}^2\theta d{\varphi }^2\right)$$

Using the Schwarzschild coordinates with the re-scale of radial coordinate and by defining $R=ra$, we have

$$d{s}^2=-d{t}^2+\frac{1}{1-\mathsf{\Lambda }{R}^2}d{R}^2+R^{2}d{\theta }^2+R^2{sin}^2\theta d{\varphi }^2$$

Case-I (empty universe)

substituting $\mathsf{\Lambda }=0$ in Equation (161) gives $4\pi G\rho =0\Rightarrow \rho =0$ which implies that $k=0$ a Euclidean flat universe. It does not belong to Einstein static universe because it is empty.

Case II (non-empty universe)

Einstein universe belongs to $\mathsf{\Lambda }\ne 0$ and $\rho \ne 0$ implying that $k>0$ which represents a universe with hypersurface of Riemannian geometry. In Einstein’s universe $\rho >0$, therefore a positive cosmological constant $\mathsf{\Lambda }>0$ would be allowed which also implies $k>0$.

7.1. Instability of Einstein’s Universe

Equation of energy conservation can be had from Equations (157) and (158) by multiplying Equation (158) with $3a^2$, differentiating it with respect to time and then dividing by $\dot{a}$, we have

$$6\ddot{a}=8\pi Ga\left(2\rho +\frac{a}{\dot{a}}\dot{\rho }\right)+2\mathsf{\Lambda }a$$

(163)

Dividing Equation (163) by a and substituting the 2nd Friedman Equation from Equation (159) in it, we have

$$6\frac{\ddot{a}}{a}=8\pi G\left(2\rho +\frac{a}{\dot{a}}\dot{\rho }\right)+2\mathsf{\Lambda }$$

(164)

$$6\left(-\frac{4\pi G}{3}\left(\rho +3p\right)+\frac{\mathsf{\Lambda }}{3}\right)=8\pi G\left(2\rho +\frac{a}{\dot{a}}\dot{\rho }\right)+2\mathsf{\Lambda }$$

(165)

after simplification, we get

$$\dot{\rho }+3\frac{\dot{a}}{a}\left(\rho +p\right)=0$$

(166)

For the cold matter universe $p=0$, with this the resulting equation is a separable universe

$$\dot{\rho }+3\frac{\dot{a}}{a}\rho =0$$

(167)

$$\int \frac{\dot{\rho }}{\rho }dt=-3\int \frac{\dot{a}}{a}dt$$

(168)

$$ln\rho =-3lna+lnZ$$

(169)

$$ln\rho =ln{a}^{-3}+lnZ$$

(170)

$$ln\rho -ln{a}^{-3}=lnZ$$

(171)

$$ln\frac{\rho }{a^{-3}}=lnZ$$

(172)

which gives

$$\rho a^3=Z$$

(173)

where Z is some positive constant of integration $Z>0$

$$\rho =\frac{Z}{a^3}$$

(174)

Further, as the universe does not expand so that $a\left(t\right)=a\left(t_0\right)=a_0$, therefore replacing $a\left(t\right)$ with $a_0$ in Equation (174)

$$\rho =\frac{Z}{a_0^3}$$

(175)

Substituting the value of $\rho$ from Equation (176) in Equation (158), i.e., 2nd Friedmann Equation with $p=0$, we obtain

$$\frac{\ddot{a}}{a}=-\frac{4\pi GZ}{3{a_0}^3}+\frac{\mathsf{\Lambda }}{3}$$

(176)

and substituting in Equation (161) gives

$$\mathsf{\Lambda }=4\pi G\rho =4\pi G\frac{Z}{a_0^3}$$

(177)

where $4\pi G\frac{Z}{a_0^3}>0$ since $Z>0$, Now, we perturb the solution slightly with the following perturbation

$$\epsilon <<1$$

(178)

$$a\left(t\right)=a\left(t_0\right)+\epsilon \left(t\right)a\left(t_0\right)=a_0(1+\epsilon \left(t\right))$$

(179)

substituting this in Equation (175), we have

$$\frac{\frac{d^2}{d{t}^2}\left(a_0(1+\epsilon \left(t\right))\right)}{a_0(1+\epsilon \left(t\right))}=-\frac{4\pi GZ}{3{\left(a_0(1+\epsilon \left(t\right))\right)}^3}+\frac{\mathsf{\Lambda }}{3}$$

(180)

Or

$$\frac{d^2}{d{t}^2}\left(a_0(1+\epsilon \left(t\right))\right)=-\frac{4\pi GZ}{3a_0^2}{(1+\epsilon (t\left)\right)}^{-2}+\frac{\mathsf{\Lambda }}{3}{a}_0(1+\epsilon \left(t\right))$$

(181)

Using the Maclaurin series expansion as $\epsilon <<1$, and ${(1+\epsilon (t\left)\right)}^{-2}$$=1-2\epsilon +O\left({\epsilon }^2\right)$, Now Equation (181) becomes by neglecting $O\left({\epsilon }^2\right)$ as $\epsilon <<1$, so that

$$a_0\frac{d^2\epsilon }{d{t}^2}=-\frac{4\pi GZ}{3a_0^2}(1-2\epsilon \left(t\right))+\frac{\mathsf{\Lambda }}{3}{a}_0(1+\epsilon \left(t\right))+O\left({\epsilon }^2\right)$$

(182)

$$\ddot{\epsilon }=-\frac{4\pi GZ}{3a_0^3}+\frac{8\pi GZ}{3a_0^3}\epsilon +\frac{\mathsf{\Lambda }}{3}\epsilon +\frac{\mathsf{\Lambda }}{3}=\left(\frac{8\pi GZ}{3a_0^3}+\frac{\mathsf{\Lambda }}{3}\right)\epsilon +\frac{\mathsf{\Lambda }}{3}-\frac{4\pi GZ}{3a_0^3}$$

(183)

Using the value of $\mathsf{\Lambda }=\frac{4\pi GZ}{a_0^3}$ from Equation (177) in Equation (183), it can be expressed in the form

$$\frac{d^2\epsilon }{d{t}^2}-\mathsf{\Lambda }\epsilon =0$$

(184)

As the cosmological constant is $\mathsf{\Lambda }>0$, the solution of above equation will read as

$$\epsilon =Pexp\left(\sqrt{\mathsf{\Lambda }}t\right)+Qexp(-\sqrt{\mathsf{\Lambda }}t)$$

(185)

Due to existence of the 1st term in the above solution as positive and in the case of an arbitrary perturbation considered initially, both of the constants $P\ne 0$, $Q\ne 0$ will help the perturbation grow and it will not remain small which will imply that the static solution is unstable, although $P=0$ can be possible only for specialized initial conditions such as singular one.

7.2. De Sitter Universe

In Einstein’s static model with positive cosmological constant when energy density of the matter is removed de Sitter model results. The de Sitter model of the universe presented in 1917 was proposed just after Einstein presented his static closed model of the universe. Einstein resorting to the Mach’s principle was of the view that it is merely matter density in universe that is the cause of inertia and gravitation. For checking the status of this Einstein’s belief de Sitter posed the 2nd model of the universe devoid of matter density $T_{\mu \nu }=0$, however retaining the cosmological constant that is $G_{\mu \nu }=g_{\mu \nu }\mathsf{\Lambda }$. The de Sitter model is the maximally symmetric solution of Einstein’s field equations with vanishing matter density. The geometric theoretic structure of spacetime of the de Sitter model is comparatively more complicated than that of Einstein’s model of the universe. The characteristic of the de Sitter model is that it predicts redshift despite it contains neither matter density nor radiation. We review de Sitter model using Fiedmann’s equations, however it is important to note that these equations were worked out after the development of de Sitter model. We derived Friedmann equations above in the presence of cosmological constant term $\mathsf{\Lambda }$, which are

$${\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}=\frac{8\pi G}{3}\rho +\frac{\mathsf{\Lambda }}{3}$$

(186)

$$\frac{\ddot{a}}{a}=-\frac{4\pi G}{3}\left(\rho +3p\right)+\frac{\mathsf{\Lambda }}{3}$$

(187)

de Sitter universe corresponds to $\rho =0$, so that $k\left(\rho \right)=0$, Equation (186) takes the form

$$\frac{\dot{a}}{a}=\sqrt{\frac{\mathsf{\Lambda }}{3}}$$

(188)

Integrating with respect to time

$$\int \frac{\dot{a}\left(t\right)}{a\left(t\right)}dt=\sqrt{\frac{\mathsf{\Lambda }}{3}}\int dt$$

(189)

$$a\left(t\right)=e^{\sqrt{\frac{\mathsf{\Lambda }}{3}}t}$$

(190)

From Equation (188), $H=\frac{\dot{a}}{a}$, so the Equation (190) can be expressed as

$$a\left(t\right)=e^{Ht}$$

(191)

Which corresponds to the modified Einstein field equations

$$G_{\mu \nu }=-g_{\mu \nu }\mathsf{\Lambda }$$

(192)

8. The Conformal FLRW Line Element

The metric in Equation (57) can be conformally recast by defining conformal time as

$$d\tau =\frac{dt}{a\left(t\right)}$$

(193)

so that

$$dt=a\left(t\right)d\tau$$

(194)

After substituting Equation (194) in Equation (57) and simplifying, we get the line element in the form

$$d{s}^2=-a{\left(\tau \right)}^2\left[-d{\tau }^2-{\left(1-k{r}^2\right)}^{-1}d{r}^2-r^2{\mathsf{\Omega }}^2\right]$$

(195)

Due to conformal time the scale factor $a\left(\tau \right)$ becomes a factor of spatial as well as temporal components in the metric. Now, a function $f\left(t\right)$ which depends upon time can be differentiated as

$$\begin{array}{c}\dot{f}\left(t\right)=\frac{f^{\prime }\left(\tau \right)}{a\left(\tau \right)}\hfill \\ \ddot{f}\left(t\right)=\frac{f^{″}\left(\tau \right)}{a^2\left(\tau \right)}-\frac{f^{\prime }\left(\tau \right)}{a^2\left(\tau \right)}\mathcal{H}\hfill \end{array}$$

(196)

where dot “.” and “,” represent derivatives with respect to cosmic and conformal times, respectively, and $\mathcal{H}=\frac{a^{\prime }\left(\tau \right)}{a\left(\tau \right)}$. Now, replacing $f\left(t\right)$ and its derivatives with $a\left(t\right)$ both in correspondence with cosmic time ‘t’ and conformal time ‘$\tau$’

$$\dot{a}\left(t\right)=\frac{a^{\prime }\left(\tau \right)}{a\left(\tau \right)}$$

(197)

$$\ddot{a}\left(t\right)=\frac{a^{″}\left(\tau \right)}{a^2\left(\tau \right)}-\frac{{\mathcal{H}}^2}{a\left(\tau \right)}$$

(198)

and

$$H=\frac{\dot{a}}{a}=\frac{\mathcal{H}}{a\left(\tau \right)}$$

(199)

$$\dot{H}=\frac{{\mathcal{H}}^{\prime }}{a^2\left(\tau \right)}-\frac{{\mathcal{H}}^2}{a^2\left(\tau \right)}$$

(200)

Similarly

$${\mathcal{H}}^2=\frac{8\pi G{a}^2}{3}\rho -k$$

(201)

and

$${\rho }^{\prime }+3\mathcal{H}\left(\rho +p\right)=0$$

(202)

Now we solve the energy conservation equation From Equation (108)

$$\dot{\rho }+3\frac{\dot{a}}{a}\left(\rho +p\right)=0$$

(203)

in order to get the relation between energy density $\rho$, scale factor a and equation of state parameter $w=\frac{p}{\rho }$ we solve

$$\dot{\rho }=-3\frac{\dot{a}}{a}\left(\rho +p\right)=-3\frac{\dot{a}}{a}\rho \left(1+\frac{p}{\rho }\right)$$

(204)

$$\Rightarrow \frac{\dot{\rho }}{\rho }=-3\frac{\dot{a}}{a}\left(1+w\right)$$

(205)

where $\frac{p}{\rho }=w$. Integrating Equation (205)

$$\int \frac{1}{\rho }d\rho =-3\left(1+w\right)\int \frac{1}{a}da$$

(206)

which gives

$$\rho =a^{-3\left(1+w\right)}$$

(207)

Now from 1st Friedmann equation, after simplification and doing integration, we find

$$a=t^{\frac{2}{3\left(1+w\right)}}$$

(208)

For $w=-1$, 0, $\frac{1}{3}$, we find pressure, energy density and scale factor characterizing the expansion of the universe which depicts three phases of the universe namely vacuum dominated, radiation dominated and matter dominated, respectively.

8.1. Vacuum Domination ($\mathsf{\Lambda }$-Dominated Era)

For $w=-1$

$$\rho =a^{-3\left(1+w\right)}=a^0$$

(209)

and

$$a=t^{\frac{2}{3\left(1-1\right)}}=t^{\infty }$$

(210)

8.2. Radiation Domination

For $w=\frac{1}{3}$

$$\rho =a^{-3\left(1+\frac{1}{3}\right)}=a^{-4}$$

(211)

and

$$a=t^{\frac{2}{3\left(1+\frac{1}{3}\right)}}=t^{\frac{1}{2}}$$

(212)

8.3. Matter Domination

For $w=0$

$$\rho =a^{-3\left(1+0\right)}=a^{-3}$$

(213)

and

$$a=t^{\frac{2}{3\left(1+0\right)}}=t^{\frac{2}{3}}$$

(214)

8.4. Critical Density (${\rho }_c$) and Density Parameter ($\mathsf{\Omega }$)

Now from 1st Friedman Equation (112) with $\mathsf{\Lambda }=0$ and $H={\partial }_{t}lna$, we relate the curvature of spacetime k and the expansion characterized by the scale factor $a\left(t\right)$ to the energy density $\rho \left(t\right)$ of the universe and find the expression for the critical density required to keep the current rate of the expansion.

$$H^2=\frac{8\pi G}{3}\rho -\frac{k}{a^2}$$

(215)

For critical density ${\rho }_c$ the curvature of spacetime geometry k must vanish, so that Equation (215) reduces to

$$H^2=\frac{8\pi G}{3}\rho$$

(216)

where we obtain the expression for critical density

$$\rho ={\rho }_c=\frac{3H^2}{8\pi G}$$

(217)

From Equation (215) dividing both sides by $H^2$ and rearranging

$$1=\frac{8\pi G}{3H^2}\rho -\frac{k}{a^2{H}^2}=\frac{\rho }{\left(\frac{3H^2}{8\pi G}\right)}-\frac{k}{a^2{H}^2}$$

(218)

where $\frac{3H^2}{8\pi G}={\rho }_c$, therefore Equation (218) becomes

$$1=\frac{\rho }{{\rho }_c}-\frac{k}{a^2{H}^2}=\mathsf{\Omega }-\frac{k}{a^2{H}^2}$$

(219)

$$\Rightarrow \mathsf{\Omega }-1=\frac{k}{a^2{H}^2}$$

(220)

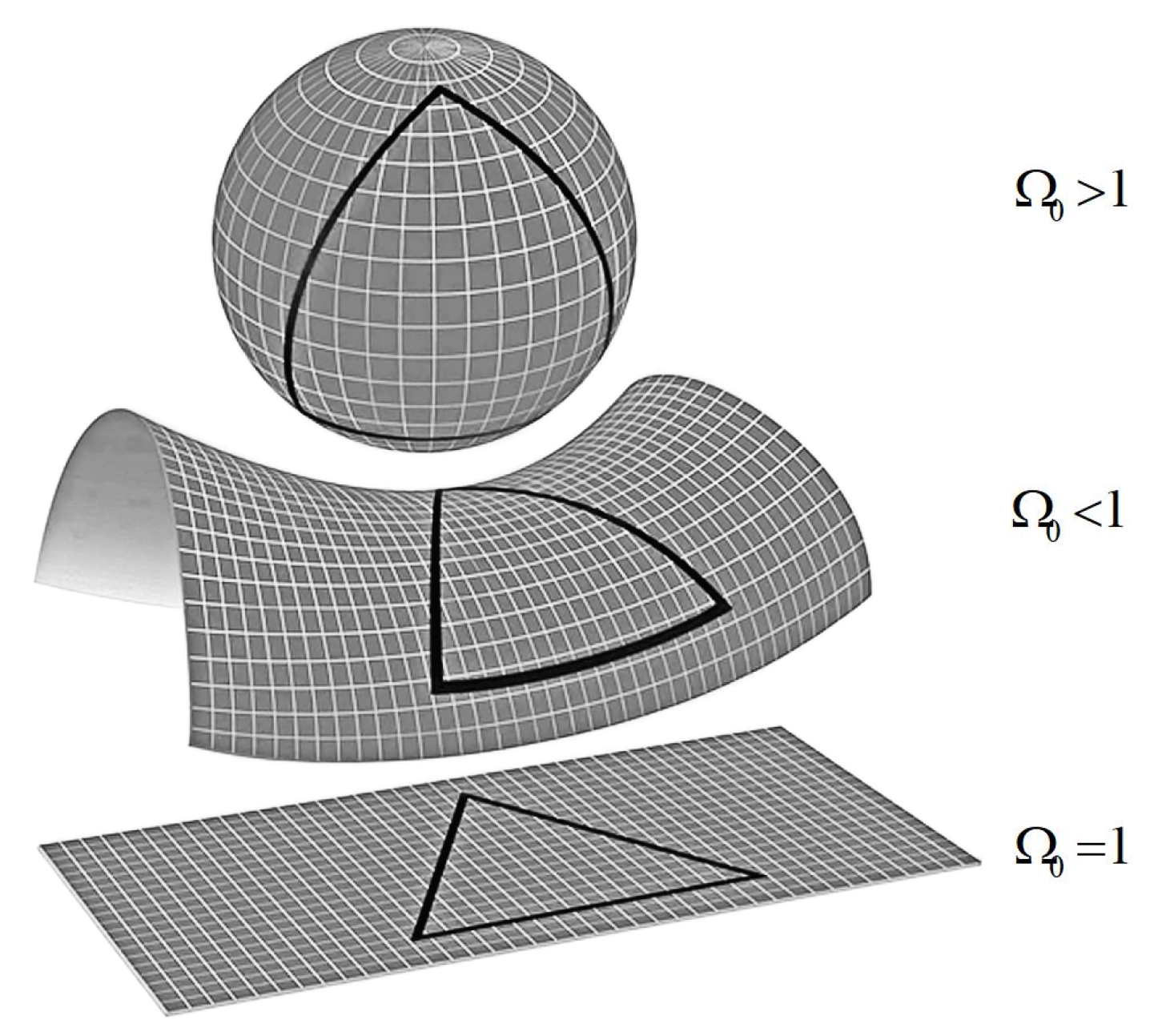
where $\mathsf{\Omega }=\frac{\rho }{{\rho }_c}$ is the density parameter and we can predict in terms of it about the geometry of universe. The local geometry of the universe is investigated by this parameter by observing whether the relative density is smaller than unity, greater than or equal to it. In the Figure 11 all three geometries are represented as the density parameter would allow:

Equation (220) can also be derived from Equation (215) in an alternative style. Writing Equation (215) by multiplying and dividing the 1st term on the right side with ${\rho }_c$

$$H^2=\frac{8\pi G\rho }{3}\frac{{\rho }_c}{{\rho }_c}-\frac{k}{a^2}$$

(221)

Using the density parameter $\mathsf{\Omega }=\frac{\rho }{{\rho }_c}$, in Equation (221) we can write

$$H^2=\frac{8\pi G}{3}{\rho }_c\mathsf{\Omega }-\frac{k}{a^2}$$

(222)

Now, from the critical density expression in Equation (217),

$$\begin{array}{c}\Rightarrow \frac{3}{8\pi G}=\frac{{\rho }_c}{H^2}\hfill \\ \Rightarrow \frac{8\pi G}{3}=\frac{H^2}{{\rho }_c}\hfill \end{array}$$

(223)

Substituting the 2nd part in Equation (223) in Equation (222) and using the density parameter, we get

$$H^2=H^2\mathsf{\Omega }-\frac{k}{a^2}$$

(224)

which gives the following form similar to Equation (220)

$$\mathsf{\Omega }-1=\frac{k}{a^2{H}^2}$$

(225)

Now

$$\mathsf{\Omega }=\frac{\rho }{{\rho }_c}$$

(226)

is considered decisive in describing the evolution of the universe. The present value of it is denoted by ${\mathsf{\Omega }}_0$ and it gives following three geometries of the universe

$${\mathsf{\Omega }}_0>1$$

(227)

a closed universe implying the universe with spherical geometry

$${\mathsf{\Omega }}_0<1$$

(228)

an open universe implying the universe with hyperbolic geometry and

$${\mathsf{\Omega }}_0=1$$

(229)

a flat universe implying the universe with Euclidean or Minkowskian geometry. The present value of critical density can be calculated with present value of Hubble constant $H_0$, gravitational constant G and $\pi$.

$$\begin{array}{c}{\rho }_{c,0}=\frac{3H_0^2}{8\pi G}\hfill \\ =\frac{3{\left(73.8\right)}^2}{8\left(3.14\right)\left(6.67\times {10}^{-11}\right)}\hfill \\ =1.1\times {10}^{-5}{h}^2\hfill \end{array}$$

(230)

where the scaled Hubble parameter h is defined by $H=100$ hkm ${\mathrm{s}}^{-1}$$\mathrm{Mp}{\mathrm{c}}^{-1}$ and $H^{-1}=9.778$${\mathrm{h}}^{-1}$ Gyr $H^{-1}=2998$ ${\mathrm{h}}^{-1}$ Mpc.

8.5. Particle Horizon

When the scale factor $a\left(t\right)$ is multiplied with the co-moving coordinates we get the proper distance. In cosmology causality is one directional since we just receive photons from the outer world that serves to be self-sufficient approach. The horizon or horizon distance of the universe is defined as the maximum distance that light could have traveled to our reference Earth as the time after the beginning of the universe when for the first time it became exposed to electromagnetic radiation [45], thus horizon represents the causal distance in the universe.

$$d_H\left(t\right)=a\left(t\right){\int }_0^t\frac{d{t}^{\prime }}{a\left(t^{\prime }\right)}$$

(231)

Such that $d_H\left(t\right)\sim H^{-1}\left(t\right)$ Particle horizon is defined to be the distance traveled by a photon from the time of big bang up to a certain later time, t. Particle horizon puts limits on communication from the deep inward past.

8.6. Event Horizon

An event horizon defines such a set of points from which light signals sent at some given time will never be received by an observer in the future. It sets limits on the horizon distance and on communication to the future so that as long as it exists, the size of the causal patch of the universe will be finite.

8.7. Deceleration Parameter ($q_0$)

A Taylor series is a series expansion of a function about a given point. We require here a one dimensional Taylor series which is the expansion of a real function $f\left(x\right)$ about a point $x=a$ and is given by

$$\begin{array}{c}f\left(t\right)={\left.f\left(x\right)\right|}_{x=a}=f\left(a\right)+f^{\prime }\left(a\right)\left(x-a\right)+\frac{f^{″}\left(a\right)}{2!}{\left(x-a\right)}^2\hfill \\ +\frac{f^{″\prime }\left(a\right)}{3!}{\left(x-a\right)}^3+···+\frac{f^n\left(a\right)}{n!}{\left(x-a\right)}^n+···\hfill \end{array}$$

(232)

We take the function $f\left(x\right)=a\left(t\right)$ which is scale factor and find its Taylor series expansion about the present time $t=t_0$

$$\begin{array}{c}a\left(t\right)={\left.a\left(t\right)\right|}_{t=t_0}=a\left(t_0\right)+\dot{a}\left(t_0\right)\left(t-t_0\right)+\frac{\ddot{a}\left(t_0\right)}{2!}{\left(t-t_0\right)}^2\hfill \\ +\frac{\left(t_0\right)}{3!}{\left(t-t_0\right)}^3+···+\frac{a^n\left(t_0\right)}{n!}{\left(t-t_0\right)}^n+···\hfill \end{array}$$

(233)

dividing Equation (233) by $a\left(t_0\right)$ throughout, we have

$$\begin{array}{c}\frac{a\left(t\right)}{a\left(t_0\right)}=\frac{a\left(t_0\right)}{a\left(t_0\right)}+\frac{\dot{a}\left(t_0\right)}{a\left(t_0\right)}\left(t-t_0\right)+\frac{1}{2}\frac{\ddot{a}\left(t_0\right)}{a\left(t_0\right)}{\left(t-t_0\right)}^2\hfill \\ +\frac{1}{6}\frac{\left(t_0\right)}{a\left(t_0\right)}{\left(t-t_0\right)}^3+···+\frac{1}{n!}\frac{a^n\left(t_0\right)}{a\left(t_0\right)}{\left(t-t_0\right)}^n+···\hfill \end{array}$$

(234)

ignoring the higher terms we have the following remaining expression

$$\frac{a\left(t\right)}{a\left(t_0\right)}=1+\frac{\dot{a}\left(t_0\right)}{a\left(t_0\right)}\left(t-t_0\right)+\frac{1}{2}\frac{\ddot{a}\left(t_0\right)}{a\left(t_0\right)}{\left(t-t_0\right)}^2$$

(235)

multiplying and dividing now by $\dot{a}\left(t\right)$ with 3rd term of Equation (235) on the right hand side:

$$\frac{a\left(t\right)}{a\left(t_0\right)}=1+\frac{\dot{a}\left(t_0\right)}{a\left(t_0\right)}\left(t-t_0\right)+\frac{1}{2}\frac{\dot{a}\left(t_0\right)}{a\left(t\right)}\frac{\ddot{a}\left(t_0\right)}{\dot{a}\left(t_0\right)}{\left(t-t_0\right)}^2$$

(236)

Multiplying again the 3rd term on the right hand side of Equation (236) with $\frac{\dot{a}\left(t_0\right)}{a\left(t_0\right)}$ and its reciprocal $\frac{a\left(t_0\right)}{\dot{a}\left(t_0\right)}$, we have

$$\frac{a\left(t\right)}{a\left(t_0\right)}=1+\frac{\dot{a}\left(t_0\right)}{a\left(t_0\right)}\left(t-t_0\right)+\frac{1}{2}\left(\frac{\dot{a}\left(t_0\right)}{a\left(t_0\right)}\times \frac{a\left(t_0\right)}{\dot{a}\left(t_0\right)}\right)\frac{\dot{a}\left(t_0\right)}{a\left(t\right)}\frac{\ddot{a}\left(t_0\right)}{\dot{a}\left(t_0\right)}{\left(t-t_0\right)}^2$$

(237)

Putting for $\frac{\dot{a}\left(t_0\right)}{a\left(t_0\right)}=H_0$, the present value of Hubble parameter and $\frac{a\left(t_0\right)\ddot{a}\left(t_0\right)}{{\left[\dot{a}\left(t_0\right)\right]}^2}=-q_0$, Equation (237) reduces to the following:

$$\frac{a\left(t\right)}{a\left(t_0\right)}=1+H_0\left(t-t_0\right)+\frac{1}{2}{H}_0^2\left(-q_0\right){\left(t-t_0\right)}^2$$

(238)

where

$$q_0=-\frac{a\left(t_0\right)\ddot{a}\left(t_0\right)}{{\left[\dot{a}\left(t_0\right)\right]}^2}=-\frac{\ddot{a}\left(t_0\right)}{\dot{a}\left(t_0\right)}{H}_0^{-1}=-\frac{\ddot{a}\left(t_0\right)}{a\left(t_0\right)}{H}_0^{-2}$$

(239)

is called the deceleration parameter. It tells us that greater the value of $q_0$, the faster will be speed of deceleration. It can be further related with the acceleration equation

$$\frac{\ddot{a}\left(t\right)}{a\left(t\right)}=-\frac{4\pi G}{3}\left(\rho +3p\right)$$

(240)

Putting Equation (240) in Equation (239)

$$q_0=-\left(-\frac{4\pi G}{3}\left(\rho +3p\right)\right)H_0^{-2}$$

(241)

With $p=0$ for a universe having matter domination and present energy density $\rho ={\rho }_0$ with dividing and multiplying by 2, we possess

$$q_0=\frac{1}{2}\frac{8\pi G}{3H_0^2}{\rho }_0$$

(242)

Now, as the critical density is given by ${\rho }_c=\frac{3H_0^2}{8\pi G}$ from the 1st Friedmann equation. Therefore Equation (242) takes the form

$$q_0=\frac{1}{2}\left(\frac{1}{{\rho }_c}\right){\rho }_0=\frac{1}{2}\frac{{\rho }_0}{{\rho }_c}=\frac{1}{2}{\mathsf{\Omega }}_0$$

(243)

The measurement of deceleration parameter $q_0$ determines how much bigger the universe was in earlier times. The explorations of redshift measures of supernovae of Type $SNIa$ to measure the value of $q_0$ has shown astoundingly that $q_0<0$ at the present which means that the expansion of the universe is accelerating rather than to be decelerating which affirms that the concept of dark energy must be acknowledged. Accelerated expansion of the universe corresponds to $q_0<0$, whereas $q_0>0$ corresponds decelerated expansion. It is interesting to notice that for all of these components we have $H>0$, i.e., an increasing scale factor which gives the expansion rate of the universe. Moreover, to get a better understanding of the properties of each species, it is useful to introduce the deceleration parameter $q_0$ as

$$\begin{array}{c}{q}_0=-\frac{\ddot{a}\dot{a}}{{\dot{a}}^2}\hfill \\ =-\frac{\ddot{a}}{\dot{a}}\frac{a}{\dot{a}}\hfill \\ =-\frac{\ddot{a}}{\dot{a}}{H}^{-1}\hfill \end{array}$$

(244)

such that for both matter-dominated or radiation-dominated universe the expansion is decelerating. It is also interesting to notice that components with $w<-\frac{1}{3}$ give an accelerated expansion.

8.8. Friedmann Equations in Terms of Density Parameter

We found earlier Friedmann equations

$${\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}=\frac{8\pi G}{3}\rho$$

(245)

$$\frac{\ddot{a}}{a}=-\frac{4\pi G}{3}\left(\rho +3p\right)$$

(246)

In Equation (245) in order to incorporate vacuum energy we can write energy density as the sum of all energy components $\rho ={\rho }_m+{\rho }_r+{\rho }_{\mathsf{\Lambda }}$ such that the equation can be written as

$$H^2=\frac{8\pi G}{3}\left({\rho }_m+{\rho }_r+{\rho }_{\mathsf{\Lambda }}\right)-\frac{k}{a^2}$$

(247)

where $\frac{\dot{a}}{a}=H$ is the Hubble parameter, writing ${\rho }_{\mathsf{\Lambda }}$ as ${\rho }_{\mathsf{\Lambda }}=\mathsf{\Lambda }=\frac{\mathsf{\Lambda }}{8\pi G}$ and $\rho ={\rho }_m+{\rho }_r$ which further can be written as the contributing ingredients ${\rho }_m={\rho }_b+{\rho }_{CDM}$ and ${\rho }_r={\rho }_{\gamma }+{\rho }_{\nu }$, also we found earlier the critical density to be $\frac{3H^2}{8\pi G}={\rho }_{cd}$, which for the present value can be expressed as ${\rho }_{c,0}=\frac{3H_0^2}{8\pi G}$ we find from it the value of $8\pi G=\frac{3H_0^2}{{\rho }_{cd,0}}$ and substitute in Equation (247) which comes to be

$$H^2=H_0^2\left(\frac{{\rho }_m}{{\rho }_{c,0}}+\frac{{\rho }_r}{{\rho }_{c,0}}+\frac{{\rho }_{\mathsf{\Lambda }}}{{\rho }_{c,0}}\right)-\frac{k}{a^2}$$

(248)

or

$$H^2=H_0^2\left({\mathsf{\Omega }}_{m,0}+{\mathsf{\Omega }}_{r,0}+{\mathsf{\Omega }}_{\mathsf{\Lambda },0}\right)-\frac{k}{a^2}$$

(249)

where

$${\mathsf{\Omega }}_{m,0}=\frac{{\rho }_m}{{\rho }_{c,0}},{\mathsf{\Omega }}_{r,0}=\frac{{\rho }_r}{{\rho }_{c,0}},{\mathsf{\Omega }}_{\mathsf{\Lambda },0}=\frac{{\rho }_{\mathsf{\Lambda }}}{{\rho }_{c,0}}$$

(250)

It might be suitable to write the curvature term k in terms of density parameter $k={\mathsf{\Omega }}_{k,0}=\frac{{\rho }_k}{{\rho }_{c,0}}$, further the present value of the scale factor $a\left(t\right)=1$ so that Equation (249) takes the form

$$H^2=H_0^2\left({\mathsf{\Omega }}_{m,0}+{\mathsf{\Omega }}_{r,0}+{\mathsf{\Omega }}_{\mathsf{\Lambda },0}\right)-{\mathsf{\Omega }}_{k,0}$$

(251)

Now, for the present value of Hubble parameter, i.e., $H=H_0$, Equation (251) can be written for the curvature density parameter

$${\mathsf{\Omega }}_{k,0}=H_0^2\left({\mathsf{\Omega }}_{m,0}+{\mathsf{\Omega }}_{r,0}+{\mathsf{\Omega }}_{\mathsf{\Lambda },0}-1\right)$$

(252)

Equation (249) can be written in general form, i.e., $H\ne H_0$ and $a\ne a_0=1$

$$H^2=H_0^2\left({\mathsf{\Omega }}_m+{\mathsf{\Omega }}_r+{\mathsf{\Omega }}_{\mathsf{\Lambda }}\right)-\frac{{\mathsf{\Omega }}_k}{a^2}$$

(253)

Equation (253) can also be written for the present values of all the energy density parameters

$$H^2=H_0^2\left({\mathsf{\Omega }}_{m,0}+{\mathsf{\Omega }}_{r,0}+{\mathsf{\Omega }}_{\mathsf{\Lambda },0}\right)-\frac{{\mathsf{\Omega }}_{k,0}}{a^2}$$

(254)

We know that energy density $\rho$ for matter, radiation and vacuum domination eras changes with the scale factor that characterizes the expansion of the universe according to

$$\begin{array}{c}\rho \propto a^{-3}\hfill \\ \rho \propto a^{-4}\hfill \\ \rho \propto a^0\hfill \end{array}$$

(255)

respectively. Thus, Equation (254) takes the following form using Equation (255):

$$H^2=H_0^2\left({\mathsf{\Omega }}_{m,0}{a}^{-3}+{\mathsf{\Omega }}_{r,0}{a}^{-4}+{\mathsf{\Omega }}_{\mathsf{\Lambda },0}{a}^0\right)-{\mathsf{\Omega }}_{k,0}{a}^{-2}$$

(256)

The Equation (256) represents Friedmann equation in terms of density parameters.

For $a=\frac{a\left(t\right)}{a\left(t_0\right)}=\frac{1}{1+z}$, Equation (256) can be expressed in terms of redshift as follows

$$\begin{array}{c}{H}^2=H_0^2\left({\mathsf{\Omega }}_{m,0}{\left(1+z\right)}^3+{\mathsf{\Omega }}_{r,0}{\left(1+z\right)}^4+{\mathsf{\Omega }}_{\mathsf{\Lambda },0}{\left(1+z\right)}^0\right)\hfill \\ -{\mathsf{\Omega }}_{k,0}{\left(1+z\right)}^2\hfill \end{array}$$

(257)

We can discuss various models for the universe using Equation (256) for matter, radiation, $\mathsf{\Lambda }$ and curvature-dominated eras.

For matter domination, Equation (256) with ${\mathsf{\Omega }}_{m,0}=1$ and with the rest of terms vanishing gives

$$\begin{array}{c}a={\left(\frac{3}{2}{H}_{0}t\right)}^{\frac{2}{3}}\hfill \\ t=\frac{2a^{\frac{3}{2}}}{3H_0}\hfill \end{array}$$

(258)

which gives an expanding universe with expansion rate inversely proportional to time, i.e., $H=\frac{2}{3}{t}^{-1}$ and age of the universe would be $t_0=\frac{2}{3}{H}_0^{-1}$. Such model must be subject to deceleration as the time goes on.

For radiation domination, Equation (256) with ${\mathsf{\Omega }}_{r,0}=1$ and with the rest of terms vanishing, gives

$$\begin{array}{c}a=\sqrt{2H_{0}t}\hfill \\ t=\frac{a^2}{2H_0}\hfill \end{array}$$

(259)

which gives an expanding universe with expansion rate inversely proportional to time, i.e., $H=\frac{1}{2}{t}^{-1}$ and age of the universe would be $t_0=\frac{1}{2}{H}_0^{-1}$. The expansion is subject to deceleration in this radiation-dominated era.

For $\mathsf{\Lambda }$ domination, Equation (256) with ${\mathsf{\Omega }}_{\mathsf{\Lambda },0}=1$ and the rest of terms vanishing gives

$$\begin{array}{c}a=e^{H_{0}t}\hfill \\ t=\infty \hfill \end{array}$$

(260)

which gives an exponentially expanding universe with expansion rate inversely proportional to time, i.e., $H=\left(lna\right)t^{-1}$ and infinite age.

For k domination or the otherwise empty universe, Equation (256) with ${\mathsf{\Omega }}_{k,0}=1$ and with the rest of terms vanishing gives

$$\begin{array}{c}a=H_{0}t\hfill \\ t=\infty \hfill \end{array}$$

(261)

which gives an expanding universe with expansion rate inversely proportional to the time, i.e., “t”.

8.9. Cosmological Redshift

we considering the FLRW geometry

$$d{s}^2=-d{t}^2+a{\left(t\right)}^2\left[{\left(1-k{r}^2\right)}^{-1}d{r}^2+r^{2}d{\theta }^2+r^2{sin}^2\theta d{\varphi }^2\right]$$

(262)

Note here that the coordinates $\left(r,\theta ,\varphi \right)$ in the metric Equation (262) are comoving spatial coordinates; therefore, galaxies which are considered as point particles constituting the particles of cosmological fluid in cosmology remain at fixed coordinates and it is the geometry of the spacetime that expands itself and is characterized by the scale factor $a\left(t\right)$ completely. Three intervals—spacelike, timelike and lightlike, or null—expressed in the form $d{s}^2>0$, $d{s}^2<0$, or $d{s}^2=0$, respectively. In the spacetime geometry light propagates following the interval $d{s}^2=0$ or $ds=0$ which means that it does not travel at all any distance through the spacetime. We consider a ray of light propagating along the radius as all the points in space are equivalent at a given time from some zero value radius to some certain value of it in later times. As the light ray travels radially therefore only one spatial dimension is retained and the vanishing of time dimension follows from $ds=0$ and other two spatial dimensions vanish due to radial propagation of light therefore $dt=d\theta =d\varphi =0$, then Equation (262) gives

$$0=-d{t}^2+a{\left(t\right)}^2{\left(1-k{r}^2\right)}^{-1}d{r}^2$$

(263)

or

$$\frac{dt}{a\left(t\right)}=\frac{1}{\sqrt{\left(1-k{r}^2\right)}}dr$$

(264)

In order to calculate the total time elapsed from $r=0$ to some certain later time value $r=r_0$, we shall integrate Equation (264) between emission and reception times $t_e$ and $t_r$ respectively.

$${\int }_{t=t_{emi}}^{t=t_{rec}}\left(\frac{1}{a\left(t\right)}\right)dt={\int }_{r=0}^{r=r_0}\left(\frac{1}{\sqrt{1-k{r}^2}}\right)dr$$

(265)

A ray of light, now, given off after a short interval of time $d{t}_{emi}$ so that time of emission of light ray becomes $t_{emi}+d{t}_{emi}$ and accordingly we can have the time of reception to be $t_{rec}+d{t}_{rec}$ from an integral of the same nature given in Equation (265) because of comoving coordinates the galaxies remain at the same coordinates, so that

$${\int }_{t=t_{emi}}^{t=t_{rec}}\left(\frac{1}{a\left(t\right)}\right)dt={\int }_{t=t_{emi}+d{t}_{emi}}^{t=t_{rec}+d{t}_{rec}}\left(\frac{1}{a\left(t\right)}\right)dt$$

(266)

now

$${\int }_{t=t_{emi}}^{t=t_{emi}+d{t}_{emi}}\left(\frac{1}{a\left(t\right)}\right)dt={\int }_{t=t_{rec}}^{t=t_{rec}+d{t}_{rec}}\left(\frac{1}{a\left(t\right)}\right)dt$$

(267)

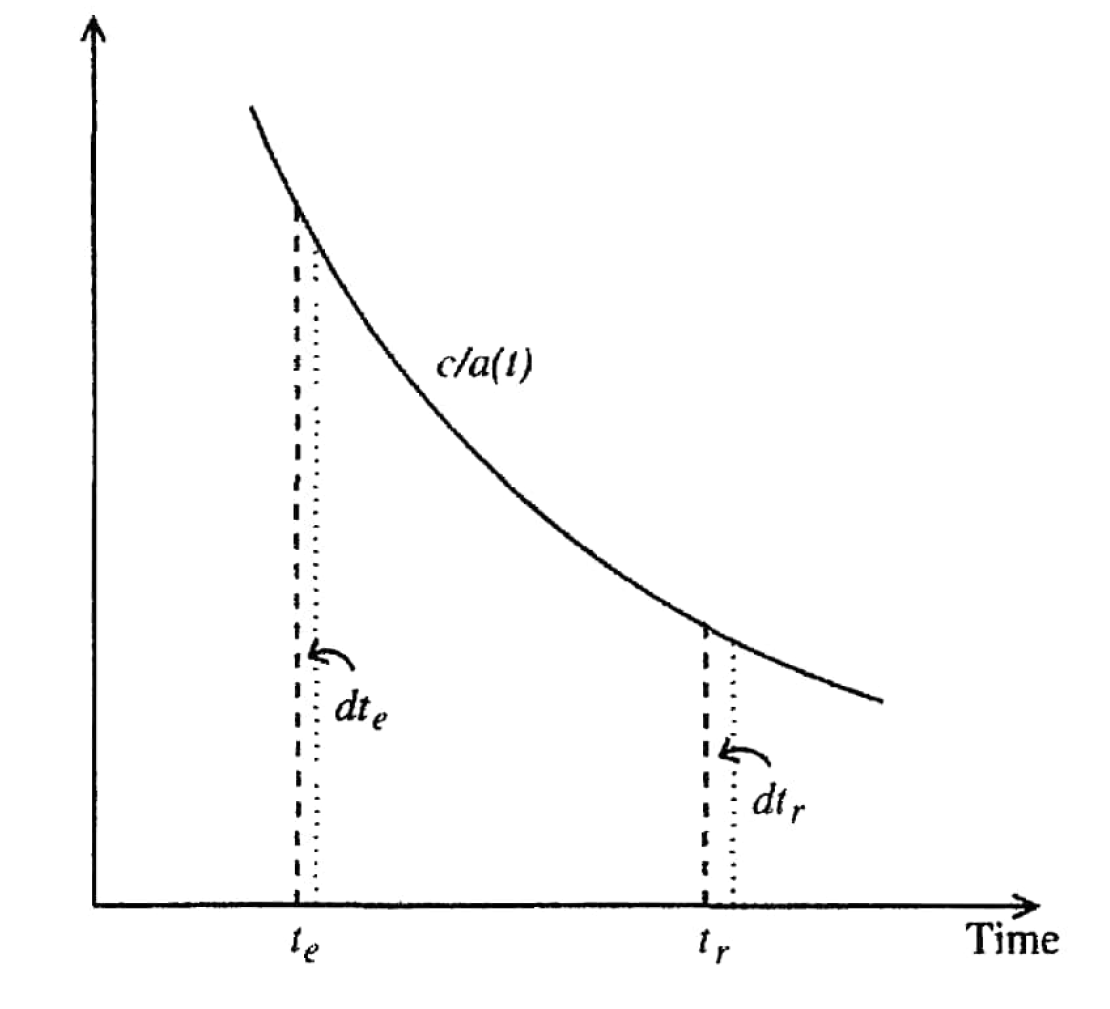
For deriving the redshift relation as the universe expands we use Figure 12 that is given below:

The slices are very narrow, so the area is just the area of a rectangle, i.e., width times height, i.e.,

$$\frac{d{t}_{rec}}{a\left(t_{rec}\right)}=\frac{d{t}_{emi}}{a\left(t_{emi}\right)}$$

(268)

For an expanding universe

$$a\left(t_{rec}\right)>a\left(t_{emi}\right),$$

(269)

it implies from Equation (268) $d{t}_{rec}>d{t}_{emi}$ that as the universe expands the time interval between two rays increases. We consider now successive crests or troughs of a single ray instead of two rays as we did earlier so that wave length $\lambda$ is directly proportional to the time interval between two successive crests or troughs $\lambda \propto dt$ and $dt\propto a\left(t\right)$ and we have

$$\frac{{\lambda }_{rec}}{{\lambda }_{emi}}=\frac{a\left(t_{rec}\right)}{a\left(t_{emi}\right)}$$

(270)

We define now the redshift

$$1+z\equiv \frac{a\left(t_{rec}\right)}{a\left(t_{emi}\right)}$$

(271)

8.10. Luminosity ($\left(L\right)$), Brightness, Luminosity Distance ($\left(d_L\right)$) and Angular Diameter Distance $\left(d_A\right)$

We can deduce relations from the properties of electromagnetic radiation and the quantities contained in FLRW line element. The velocity of electromagnetic waves is constant and finite. Light and electromagnetic radiation acts as cosmological messenger and all the distances measured cosmologically are extracted from the properties of it. The velocity of light being finite has to take time to reach us and universe might have expanded significantly during this time.

8.11. Luminosity L

Luminosity is defined as the absolute measure of the electromagnetic power or energy radiated per unit time by an astronomical object like star, galaxy or cluster of galaxies. It is denoted by L and is measure in Joule per second $\left(J{s}^{-1}\right)$ which is also known as watts. Usually luminosity is measured in terms of the luminosity of the sun denoted by $L_{\odot }$.

8.12. Brightness

It refers to how bright an object appears to an observer and depends upon luminosity, distance between the observer and the object and absorption of light along the path between observer and the object.

8.13. Luminosity Distance ($\left(d_L\right)$)

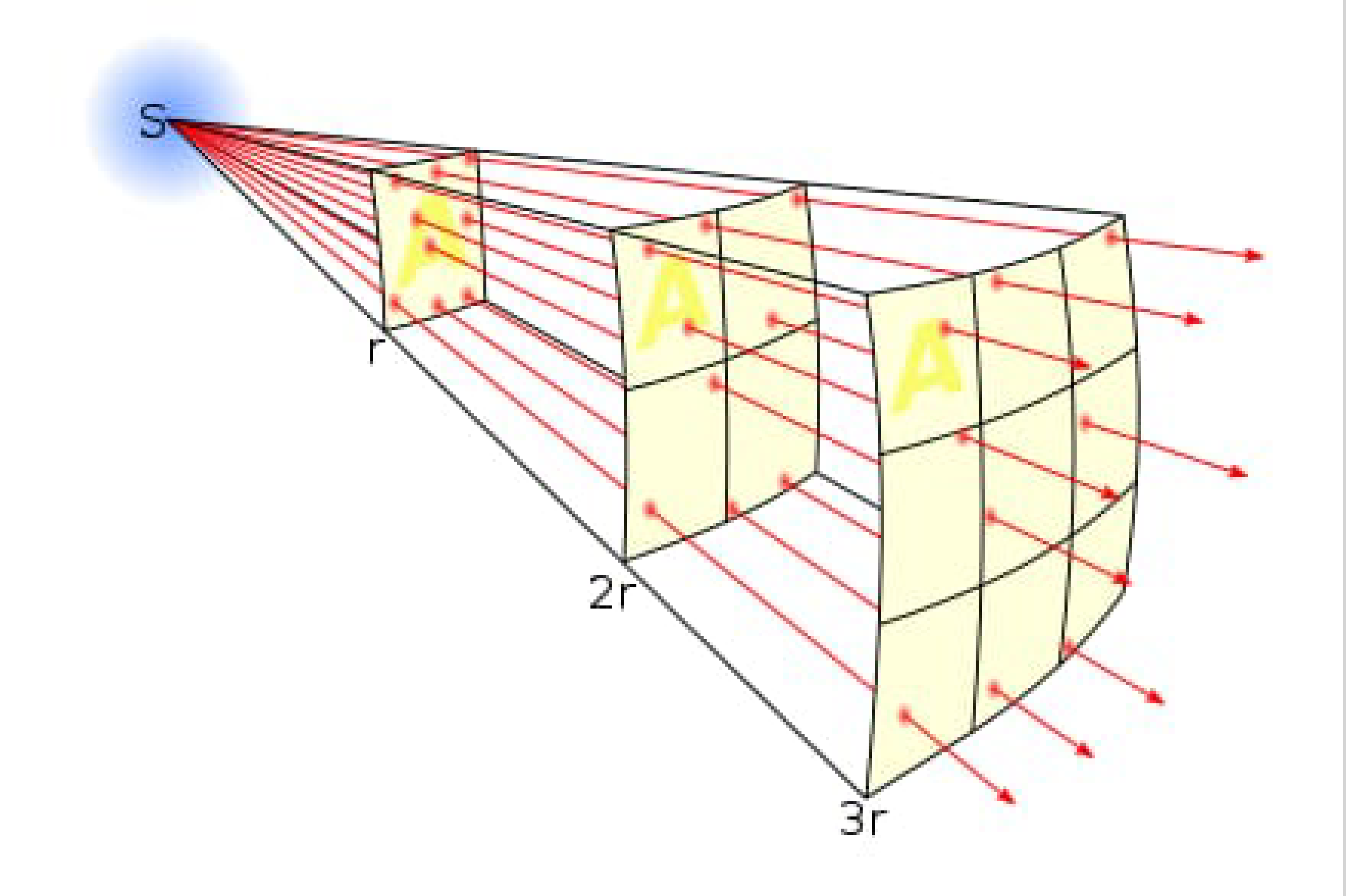
We consider a point source S radiating electromagnetic light equally in all directions spherically; the amount of light passing through elements of surface areas varies with the distance of it from the light source.

Given below in Figure 13, the light of luminosity L is being radiated. We consider a spherical hollow centered on the point source S as shown in the Figure 13 below The interior of a hollow sphere gets illuminated thoroughly. As the radius of the sphere increases, the surface area of the imagined hollow sphere also increases, such that a constant or absolute measure of luminosity has to spread in expanding sphere illuminating it, i.e., as the radius increases the constant luminosity has more and more surface area to illuminate which leads to decrease in the observed brightness. If an observer at a distance equivalent to the radius of sphere receives the electromagnetic radiation L per unit time and F be the energy flux per unit time per unit area from the source or the point source, say O, then in Euclidean geometry we will have

$$F=\frac{L}{A}=\frac{L}{4\pi r^2}$$

(272)

where $F=$ Flux density of the illuminated sphere, $L=$ luminosity, and $A=$ area of the illuminated sphere From Equation (272) for $r=d_L$

$$F=\frac{L}{4\pi {d_L}^2}$$

(273)

which gives

$$d_L=\sqrt{\frac{L}{4\pi F}}$$

(274)

We next look how the luminosity distance is related with expansion of the universe. In expanding sphere we might have its radius as the product of scale factor and the radius, i.e., $a\left(t\right)r=a\left(t\right)d_L$, so that the energy emitted gets diluted

$$4\pi r^2\to 4\pi {\left(a\left(t\right)r\right)}^2$$

(275)

and a photon loses energy as $F\propto \frac{a\left(t_e\right)}{a\left(t_0\right)}$ and redshift relation we have $1+z=\frac{\lambda \left(t_0\right)}{\lambda \left(t_e\right)}=\frac{a\left(t_0\right)}{a\left(t_e\right)}$ which implies $F\propto \frac{a\left(t_e\right)}{a\left(t_0\right)}\propto \frac{1}{1+z}$ Equation (273) becomes

$$F=\frac{L}{4\pi {\left(a\left(t\right)r\right)}^2}$$

(276)

further

$$\frac{a\left(t_e\right)}{a\left(t_0\right)}=\frac{L}{4\pi {\left(a\left(t\right)d_L\right)}^2}$$

(277)

If L is known for a source, it is known as standard candle. Supernovae type $Ia$ were used as standard candles for larger cosmic redshifts which led to accelerated expansion.

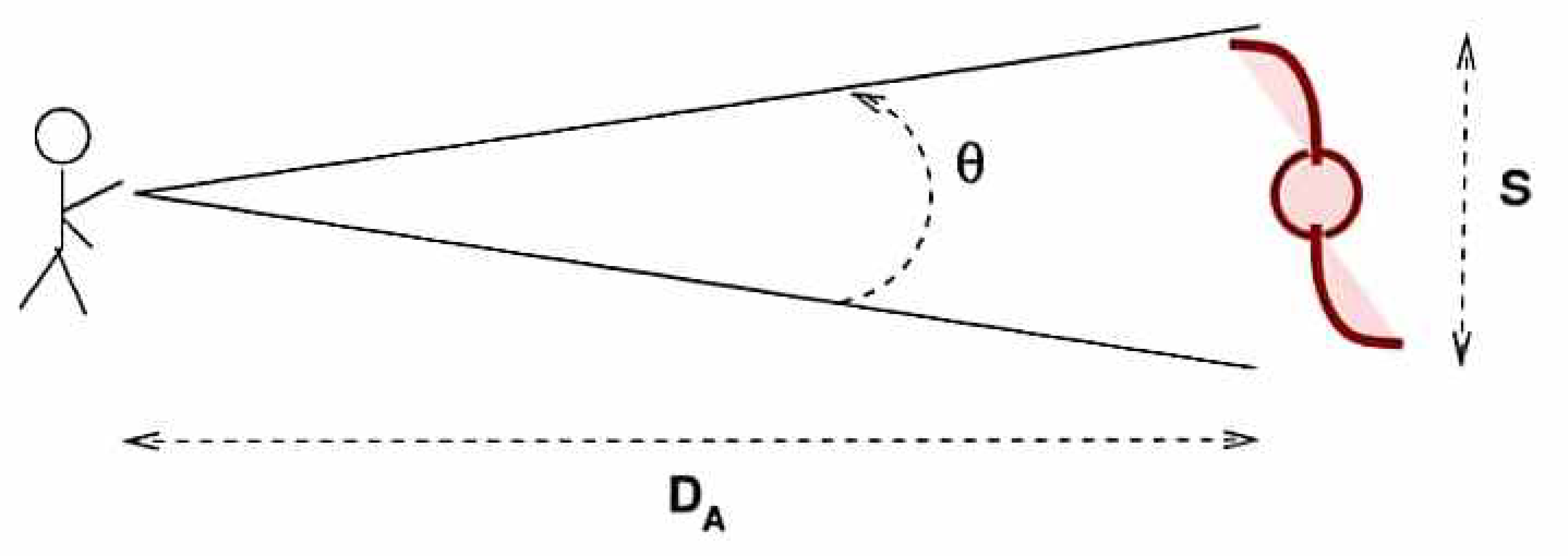
8.14. Angular Diameter Distance $\left(d_A\right)$

It is the ratio of the proper distance measured when the light left the surface of an object to the later measured distance by redshifting of light in some later time. Certainly the redshift of light measured would be smaller measured at the time when the light left the surface of the object to be measured in later times. The schematic diagram is shown in Figure 14 for angular diameter distance:

It is defined in terms of objects physical distance known as proper distance and the angular size of the object seen from the surface of earth. If size of the source be S and angular size $\theta$, then

$$\theta =\frac{S}{D_A}$$

(278)

where $D_A$ is the angular diameter distance of the source. From FLRW line element for photons $d{r}^2\approx d{\varphi }^2\approx 0$, we have

$$d{s}^2=a^2\left(t\right)\left(r^{2}d{\theta }^2\right)$$

(279)

$$ds=D_A=a\left(t\right)rd\theta$$

(280)

$$d\theta =\frac{D_A}{ra\left(t\right)}=\frac{d_A}{ra\left(t\right)}$$

(281)

9. Problems Faced by the Standard Model of Cosmology

From 1st Friedmann equation

$${\left(\frac{\dot{a}}{a}\right)}^2+\frac{k}{a^2}=\frac{8\pi G}{3}\rho$$

(282)

we see that curvature k is negligible depending on observation and $\mathsf{\Omega }\simeq 1$ which means it would have been created tuned finely in the very early universe. From 2nd Friedmann equation

$$\frac{\ddot{a}}{a}=-\frac{4\pi G}{3}\left(\rho +3p\right)$$

(283)

we see that if $\left(\rho +3p\right)$ remains positive, the acceleration is negative which means that the expansion of the universe will go on slowing down. Further, far flung parts of the universe display the same properties as observation evidence despite the fact that they have not been in causal contact with each other.

9.1. Monopole Problem

The problem is about the question of why do we not observe magnetic monopoles in the universe today. It results from combining the big bang model with GUT in particle physics, thus it is related to particle cosmology where during symmetry breaking phase transitions are considered. In the very early universe, when the phase transitions are considered to occur, it is expected that these phase transitions will create magnetic monopoles with enormous energy density which might dominate the total energy density of the universe. During symmetry breaking when phase transitions take place, these give rise to flaws known to be as topological defects. GUT predict that during GUT phase transitions these point-like topological defects are created which act as magnetic monopoles. It is considered that the radiation and matter dominated eras could not take place as these monopoles do not get diluted as they are supposed to be non-relativistic and their energy density would decay like $a^{-3}$ [46], but as we observe the universe evolved to the later eras so question arises how this occurred which is at the heart of this problem.

9.2. Horizon Problem

On the basis of the standard big bang model it is difficult to understand the uniform distribution of the temperature of CMB to 1 part in ${10}^5$. The horizon problem is related with the issue of the causal contact as it has been revealed by the uniform distribution of temperature of the cosmic background radiation (CMB) across all parts of the universe. In order to understand the problem we have to understand the horizon size and causal contact or communication. At any instant of time horizon size is defined as the largest distance, i.e., maximal distance over which two events could be in causal with each other. Therefore it is the maximum distance a photon could have traveled since the birth of the universe or since the time when the universe became transparent. It can be found from the FLRW metric to be $d{s}^2=R_H=c{\int }_0^t\frac{dt}{a\left(t\right)}$ which reveals the fact that size of the horizon depends upon the history of the universe as it evolves through time. It is also called comoving horizon as causal contact develops between two events and the universe is expanding so that they are getting separated apart mutually. In the standard big bang theory the universe was matter dominated at the time of last scattering ($t_{ls}$) so that the horizon distance at that time can be approximated by the value $d_H\left(t_{ls}\right)=2c{H}^{-1}\left(t_{ls}\right)$. Now, the Hubble distance at the time of last scattering was $c{H}^{-1}\left(t_{ls}\right)\approx 0.2\mathrm{Mpc}$ and the horizon distance at last scattering was $d_H\left(t_{ls}\right)\approx 0.4\mathrm{Mpc}$. Therefore, the points which were separated more than 0.4 Mpc distance apart at the time of last scattering ($t_{ls}$) were not connected causally in the big bang scenario. Further, angular diameter distance $\left(d_A\right)$ to the last scattering surface is 13 Mpc; therefore. points on the last scattering surface that were separated by a horizon distance shall have angular separation ${\theta }_H=\frac{d_H\left(t_{ls}\right)}{d_A}\approx \frac{0.4\mathrm{Mpc}}{13\mathrm{Mpc}}\approx 0.03\mathrm{rad}\approx 2^0$ as viewed today from the Earth. It means that the points separated by an angle as small as ∼$2^0$ on the last scattering surface were not in causal contact with each other when CMB emitted with temperature fluctuations. However, we come to know that $\frac{\delta T}{T}$ is as small as ${10}^{-5}$ on the scales with angular separation ${\theta }_H>2^0$. So here we state the problem that the regions which were not connected through causal contact with each other at the time of last scattering have similar properties homogeneously.

9.3. Flatness Problem

When we consider Friedmann’s equations evolve in a universe where only radiation and baryonic matter exist without vacuum energy density present there, then flatness problem arises in such a universe [47]. From the 1st Friedmann Equation

$$H^2=\frac{8\pi G}{3}\rho -\frac{k}{a^2}$$

(284)

$$1=\frac{8\pi G}{3H^2}\rho -\frac{k}{a^2{H}^2}=\frac{\rho }{\left(\frac{3H^2}{8\pi G}\right)}-\frac{k}{a^2{H}^2}$$

(285)

where $\frac{3H^2}{8\pi G}={\rho }_c$, therefore Equation (285) becomes

$$1=\frac{\rho }{{\rho }_c}-\frac{k}{a^2{H}^2}=\mathsf{\Omega }-\frac{k}{a^2{H}^2}$$

(286)

$$\Rightarrow \mathsf{\Omega }-1=\frac{k}{a^2{H}^2}$$

(287)

so that the spatial curvature of the universe is related to the density parameter $\mathsf{\Omega }$ through Friedmann’s equation. Observational evidence shows that the universe is nearly flat today, i.e., $\rho ={\rho }_c$, $\Rightarrow \mathsf{\Omega }=\frac{\rho }{{\rho }_c}\approx 1$. This means that the value of $\mathsf{\Omega }$ would have to be very close to 1 at Planck era $t_{pl}$. This means that the initial conditions of the universe were tuned finely. Because of this, the flatness problem is also known as the fine-tuning problem and the flatness problem arises because in a comoving volume the entropy remains conserved. Further, from Equation (284) above, the energy density of the universe without considerations of vacuum energy as is the case of big bang model is $\rho ={\rho }_R+{\rho }_M$ and we can also write

$$H^2=\frac{8\pi G}{3}\left({\rho }_R+{\rho }_M\right)-\frac{k}{a^2}$$

(288)

The term $-\frac{k}{a^2}$ is clearly proportional to $a^{-2}$, while the energy density terms ${\rho }_R$ and ${\rho }_M$ fall off faster than scale factor $a\left(t\right)$, i.e., ${\rho }_R\propto \frac{1}{a^3\left(t\right)}$ and ${\rho }_M\propto \frac{1}{a^4\left(t\right)}$. This ratio $\frac{\left(\frac{k}{a^2\left(t\right)}\right)}{\left(\frac{8\pi G}{3}({\rho }_R+{\rho }_M)\right)}=\frac{\left(\frac{k}{a^2\left(t\right)}\right)}{\left(\frac{\rho }{3M_{pl}^2}\right)}$ then is much smaller than unity when the scale factor $a\left(t\right)$ has increased by a factor of ${10}^{30}$ since the Planck era.

9.4. Entropy Problem

The adiabatic expansion of the universe following the first law of thermodynamics is related to the flatness problem [48] discussed above. Temperature plays a significant role in the early universe because at early epochs the age and expansion rate $H={\partial }_{t}lna$ are described in terms of it with the number of relativistic degrees of freedom. From the 1st Friedmann’s equation we have the expression for density parameter $\mathsf{\Omega }-1=\frac{k}{a^2\left(t\right)H^2}$ and expansion rate in radiation-dominated era in terms of temperature is $H_{{\rho }_R}^2\approx 8\pi G{T}^4=\frac{T^4}{M_{pl}^2}$, so that the density parameter expression becomes $\mathsf{\Omega }-1=\frac{k{M}_{pl}^2}{a^2\left(t\right)T^4}$. Now the entropy density is $s\sim T^3$ and the entropy per commoving volume $S\propto a^3\left(t\right)s\propto a^3\left(t\right)T^3$ and we have $\mathsf{\Omega }-1=\frac{k{M}_{pl}^2}{S^{\frac{2}{3}}{T}^2}$. The entropy per co-moving volume S remains constant throughout the evolution of the universe as the hypothesis of adiabaticity requires so that we ${\left|\mathsf{\Omega }-1\right|}_{t=t_{pl}}=\frac{\left(1\right)M_{pl}^2}{S_U^{\frac{2}{3}}{T}_{pl}^2}\approx {10}^{-60}$. It comes clear that at early epochs the value of $\mathsf{\Omega }-1$ is very close to zero as the total entropy of the universe is very large.

10. Introduction to Inflation

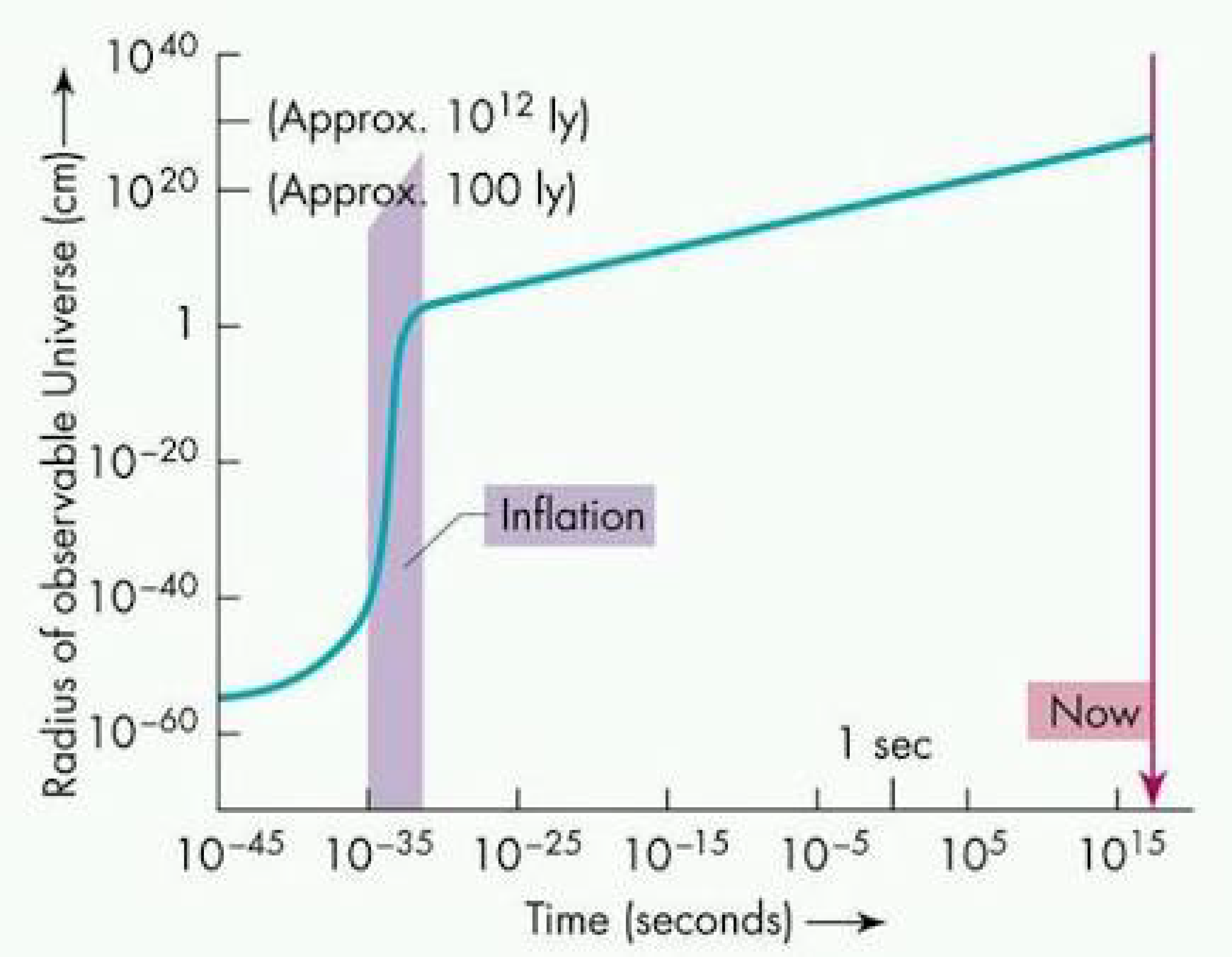
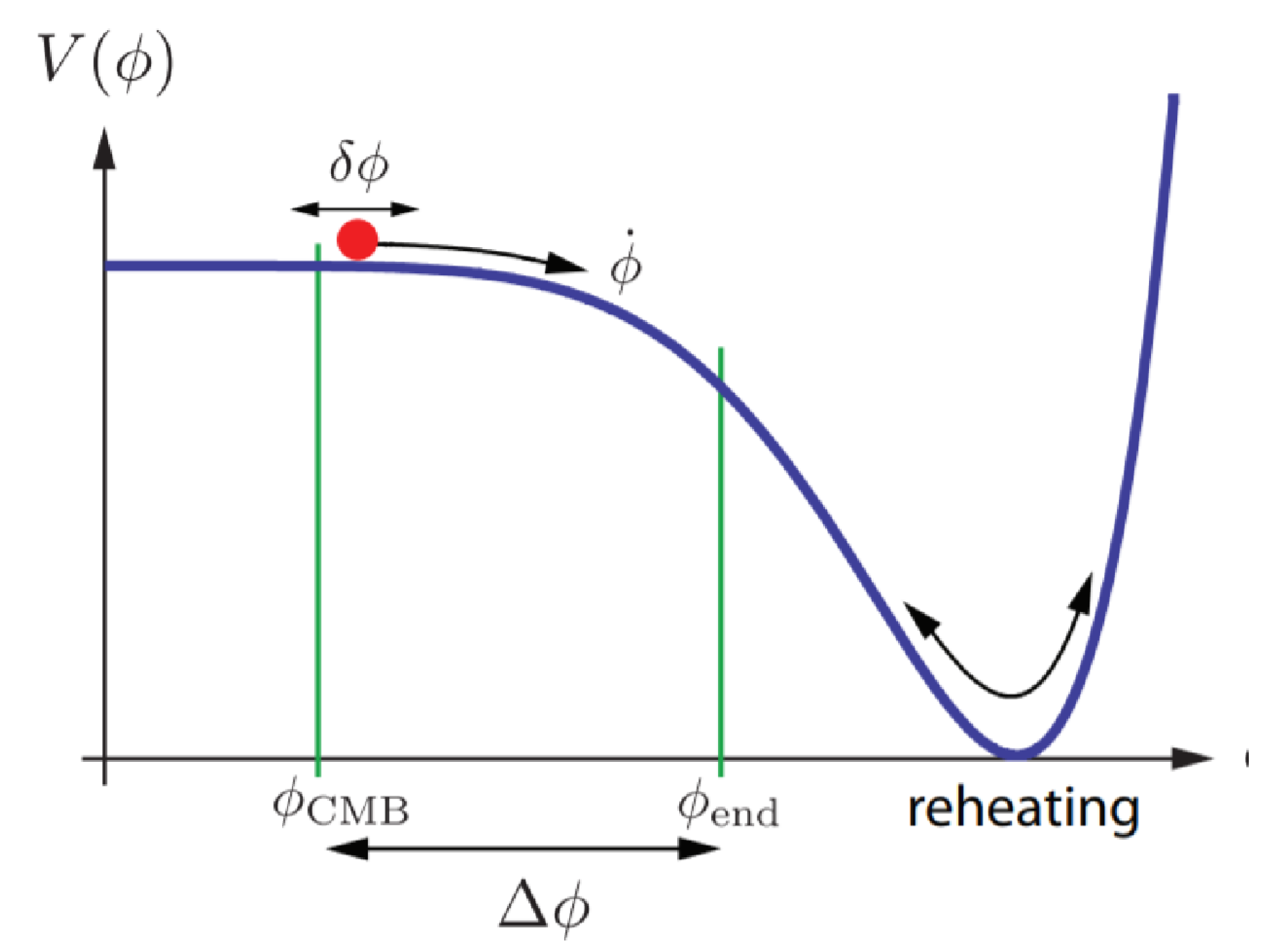
Inflation is the period of superluminally accelerated expansion of the universe taking place sometime in the very early history of the universe. It is now a widely accepted paradigm which is described as the monumental outgrowth gushing out during the tiniest fraction of the first second between (${10}^{-36}$–${10}^{-32}$) s. Inflation maintains that just after the occurrence of the big bang, exponential stretching of spacetime geometry took place, i.e., becoming twice in size again and again at least about (60–70) times over before slowing down. Alexei Strobinsky approached the exponentially expanding phase in the early universe by modifying Einstein Field Equations whereas Alan Guth approached the scenario in the realm of particle physics proposing a new picture of the time elapsed in the very small fraction of the first second in the 1980. He suggested that the universe spent its earliest moments growing exponentially faster than it does today. There is a large number of inflation models in hand today but every model has its own limitations to draw the true picture of what happened actually in the early universe.

As the theory of inflation is recognized today, it has myriad models describing inflationary phase in the early universe. From among the heap of these competing models which differ slightly from one to the other, no model can claim a complete and all-embracing prospectus of what happened actually in the universe so that the fast expansion of or in spacetime takes place. All the energy density that can be adhered to the early exponentially expanding phase of the universe was in the very fabric of spacetime itself despite ti being in the form of radiation or particles. The early accelerating phase can be now best described with de Sitter model with slightly broken time symmetry. With the creation of spacetime that purports to be the earliest patch of the universe that comes to being would be stretched apart in an incredibly small time span of the order of a tiniest fraction of first second to such a colossally larger size that its geometry and topology would be hardly indiscernible from Euclidean geometry. It will logically ensue similar initial conditions for the energy density to be dispersed at every point in the fabric of spacetime and the same will be the condition of temperature in this early phase. That’s why the quantum fluctuations which seed in later times the structure formation in the universe impart the uniform temperature to all parts of the universe thereby resolving the homogeneity problem of the universe. This is because all the quantum fluctuations which cause the observable universe were once causally connected in the deep past of the universe. It might have attained a highest temperature which was within or lesser than the limits of Planck scale (${10}^{19}$ GeV). The energy scale mentioned earlier when the inflation comes to an end and transforms into the uniform, very hot, largely dense that is a cooling and expanding state we ascribe to the hot big bang. This will take place for a universe inflating from a lower entropy state to an entropy state at higher level in the panorama of the hot big bang, where the entropy would carry on to get larger as it happens in our observed universe. The point of time in the earliest where the universe can be viewed approximately and hardly as classical is known as the Planck Era. It is thought that prior to this era the universe might be described as the hitherto unsuspected theory of certain quantum nature like quantum gravity etc. This era corresponds to $E_P\sim {10}^{19}\mathrm{GeV}>E>E_{GUT}\sim {10}^{15}\mathrm{GeV}$ and the energies, temperature and times of particles are $E_P\sim {10}^{19}\mathrm{GeV}$, $T_P\sim {10}^{32}\mathrm{K}$, $t_P\sim {10}^{-43}\mathrm{s}$, respectively. Grand unified theories describe that at high energies as described above the Electroweak and strong force are unified into a single force and that these interactions bring the particles present into thermal equilibrium Electroweak Era corresponds to phase transitions that occur through spontaneous symmetry breaking (SSB) which can be characterized by the acquisition of certain non-zero values by scalar parameters known as Higgs fields. Until the Higgs field has zero values, symmetry remains observable and spontaneously breaks at the moment the Higgs field becomes non-zero. The idea of phase transitions in the very early universe suggests the existence of the scalar fields and provides the motivation for considering their effect on the expansion of the universe.

The power spectrum of CMB is calculated by measuring the magnitude of temperature variations versus the angular size of hot and cold spots. To understand the nature of CMB the spectrum of a perfect blackbody is given in Appendix C. During these measurements, a series of peaks with different strengths and frequencies are determined which conforms to the predictions of inflation theory which confirms that all sound waves were indeed produced at the same moment by inflation. It is believed that inflation might have given rise to sound waves-the waves traveling in the primordial vacuum-like medium with different frequencies after the big bang at ${10}^{-35}\mathrm{s}$ starting in phase and would have been oscillating in radiation era for 380,000 years. Now, in the acoustic oscillations of the early universe, these must be measurable as power spectrum similar to that of measuring the sound spectrum of a musical instrument. The history of evolution of the universe to the present epoch is sketched in Figure 15, Figure 16 and Figure 17 show how the inflationary period is driven by the inflaton field:

10.1. Starobinsky $R^2$-Inflation

Alexei Starobinsky proposed a cosmological inflationary phase of the universe shortly before Alan Guth in 1980 working in the framework of general relativity. The model is founded on the semiclassical Einstein field equations which provide a self-consistent solution for an exponentially accelerating era [49]. Starobinsky modified the general relativity to describe the behavior of very early universe undergoing an exponential period by suggesting quantum corrections to the energy momentum tensor $T_{\mu \nu }$. The quantum corrections are calculated by taking the expectation value of the energy momentum tensor. Beginning with Einstein equations

$$G_{\mu \nu }=8\pi T_{\mu \nu }$$

(289)

$$R_{\mu \nu }-\frac{1}{2}{g}_{\mu \nu }R=-8\pi <T_{\mu \nu }>$$

(290)

where $<T_{\mu \nu }>$ represents the expectation value of the energy momentum tensor. The expectation value of energy momentum tensor is the probabilistic value of a result or measurement which is fundamentally rooted in all quantum mechanical systems. Intuitively, it is the arithmetic mean of a large number of independent values of a variable under consideration. The energy momentum tensor $T_{\mu \nu }$ usually takes care of classical components of the universe in the form of matter and radiation in the context of flat spacetime as the parametric observations evidence in the recent data. In the case of curved spacetimes, nonetheless $T_{\mu \nu }$ might be vanishing gradually and $<T_{\mu \nu }>$ must be imparted contributions from quantum regime non-trivially. In the absence of classical components of the universe in the form of matter and radiation, the curvature of spacetime from quantum fluctuations of matter fields contribute to $<T_{\mu \nu }>$ non-trivially which Starobinsky utilized. These are known as quantum corrections to the energy-momentum tensor $T_{\mu \nu }$. The quantum fluctuations of matter fields give non-trivial contributions to the expectation value of the energy momentum tensor $<T_{\mu \nu }>$ in the presence of cosmologically curved spacetime, regardless, matter and radian do not exist in classical style. In the background we consider FLRW spacetime

$$d{s}^2=-d{t}^2+a{\left(t\right)}^2\left({\left(1-k{r}^2\right)}^{-1}d{r}^2+r^{2}d{\theta }^2+r^2{sin}^2\theta d{\varphi }^2\right)$$

(291)

The spatial part $\left(\frac{1}{1-k{r}^2}d{r}^2+r^{2}d{\theta }^2+r^2{sin}^2\theta d{\varphi }^2\right)$ of the metric represents the three geometries depending on the values of k. For $k=+1$, it represents a spherical geometry of 3-sphere which is finite, closed, and without boundary. For $k=0$, it represents a flat Euclidean geometry of 3-planes which is, in principle, infinite in extent, open, and without boundary. $k=-1$, it represents a hyperbolic geometry of 3-hyperboloids which is infinite, open and without boundary. In the presence of conformally-invariant, free and massless fields, the quantum corrections adapt a simple form such that we can describe the expectation value of energy momentum tensor as

$$<T_{\mu \nu }>=k_1{H}_{\mu \nu }^{\left(1\right)}+k_2{H}_{\mu \nu }^{\left(2\right)}$$

(292)

where $k_1$ and $k_2$ are numerical coefficients in standard notation. In order to find $<T_{\mu \nu }>$ we have to compute these constants $k_1$ and $k_2$ and $H_{\mu \nu }^{\left(1\right)}$ and $H_{\mu \nu }^{\left(2\right)}$. The coefficient $k_1$ is determined experimentally and can assume any value. The $H_{\mu \nu }^{\left(1\right)}$ is a tensor and is conserved identically when expressed as the action given below and varied with respect to metric tensor $\sqrt{-g}$, i.e.,

$$H_{\mu \nu }^{\left(1\right)}=\frac{2}{\sqrt{-g}}\frac{\delta }{\delta g^{\mu \nu }}\int \left(d^{4}x\sqrt{-g}\right)R^2=0$$

(293)

and

$$H_{\mu \nu }^{\left(2\right)}=2R{,}_{\mu ,\nu }-2g_{\mu \nu }{R}_{,\lambda }^{,\lambda }+2R{R}_{\mu \nu }-\frac{1}{2}{g}_{\mu \nu }{R}^2$$

(294)

The coefficient $k_2$ of $H_{\mu \nu }$ is defined uniquely in the following form

$$k_2=\left(N_0+\frac{11}{2}{N}_{{}^1/{}_2}+31N_1\right)\frac{1}{1440{\pi }^2}$$

(295)

where $N_0$, $N_{{}^1/{}_2}$ and $N_1$ denote the number of quantum fields with the subscripts of all three N’s 0, ${}^1/{}_2$ and 1 representing spins of zero, half, and one respectively. In certain GUT theories due to larger multiplier factor of $N_1$, the value of $k_2$ is majorly contributed by vector fields. Now, $H_{\mu \nu }$ is also a tensor and it does not conserve generally but conserves only in those spacetimes which are conformally flat like FLRW spacetimes in particular and cannot be obtained by varying a local action as in the case of $H_{\mu \nu }$. The Equation (292) multiplying with $8\pi G$ to both sides can be written as

$$8\pi G<T_{\mu \nu }>=8\pi G{k}_1{H}_{\mu \nu }^{\left(1\right)}+8\pi G{k}_2{H}_{\mu \nu }^{\left(2\right)}$$

(296)

or

$$8\pi G<T_{\mu \nu }>=\frac{48\pi G}{6}{k}_1{H}_{\mu \nu }^{\left(1\right)}+8\pi G{k}_2{H}_{\mu \nu }^{\left(2\right)}$$

(297)

Now, we introduce the following parameters for convenience

$$\begin{array}{c}M=\sqrt{\frac{1}{48\pi G{k}_1}}\hfill \\ H_0=\sqrt{\frac{1}{8\pi G{k}_2}}\hfill \end{array}$$

(298)

where both the parameters are positive i.e., $H_0>0$ and $M>0$. Now Equation (297) takes the form

$$8\pi G<T_{\mu \nu }>=\frac{1}{6}{M}^{-2}{H}_{\mu \nu }^{\left(1\right)}+H_0^{-2}{H}_{\mu \nu }^{\left(2\right)}$$

(299)

Equation (299) can serve as the reasonable approximation in case of certain GUT models for the limit $R>{\mu }^2$, where $\mu$ represents the unified energy scale. Conformally invariant field equations usually describe the spinor and massless vector fields and contribute to $<T_{\mu \nu }>$ in the form of Equation (299). Further, if the number of matter fields is sufficiently bigger, then the corrections to Einstein’s field equations due to gravitons can also be ignored.

10.2. Trace Anomaly

The trace of expectation value of energy-momentum tensor $<T_{\mu \nu }>$ does not vanish rather it has a non-zero anomalous trace and this is what we call as trace anomaly. It is, however, interesting to note here that the trace of energy-momentum tensor without expectation value, i.e., $T_{\mu \nu }$, vanishes for all those classical fields which are conformally invariant. Therefore, the trace of $<T_{\mu \nu }>$ is given by

$$<T_{\nu }^{\nu }>=M_{pl}^{-2}\left[H_0^{-2}\left(\frac{1}{3}{R}^2-R_{\nu \sigma }{R}^{\nu \sigma }\right)-M^{-2}{R}_{;\nu }^{;\nu }\right]$$

(300)

The masses of the fields can be looked over in the limit of higher curvature, i.e., when $R\gg m^2$ and in the same limit it remains true for the case of asymptotically free gauge theories where interactions between the fields become negligible. In de sitter space we can have

$$R_{\mu \nu }=\frac{1}{4}R{g}_{\mu \nu }$$

(301)

where R is constant curvature term i.e., Ricci scalar. Substituting now Equations (300) and (301) in Equation (290), we have $R=12H_0^2$ for non-trivial solution and the corresponding de Sitter solutions come about for $k=0$, $+1$, $-1$, respectively,

$$a\left(t\right)=a_0{e}^{t{H}_0}$$

(302)

$$a\left(t\right)=\frac{1}{H_0}cosh\left(t{H}_0\right)$$

(303)

$$a\left(t\right)=\frac{1}{H_0}sinh\left(t{H}_0\right)$$

(304)

Equation (302) corresponds to $k=0$ and gives a flat universe, Equation (303) gives a closed solution for $k=+1$, and the 3rd Equation (304) for $k=-1$ propounds the open de Sitter model of the universe. These solutions are impelled completely by the quantum corrections rendered to classical EFE and serve the purpose of inflationary epoch in the very early universe. Starobinsky inflation corresponds to a potential parameterized in terms of scalar field $\varphi$ is $V\left(\varphi \right)=\frac{3}{4}{\left(1-e^{-\sqrt{\frac{2}{3}\varphi }}\right)}^2$.

10.3. Inflation and de Sitter Universe

In a very shorter period of time about ${10}^{-35}$ after spacetime came into being, the inflationary era of accelerating superluminal expansion known to be de Sitter phase took place. de Sitter phase removed all the wrinkles of curvature and warpage of spacetime so that the universe is to be observed flat. It further smoothed out all energy density stuff for the distribution of radiation and matter. One significant remnant as the traces of this fast expansion remains there known later on to be cosmic background radiation. In de Sitter universe there exists no ordinary matter, however, de Sitter retained cosmological constant which represents vacuum energy smeared out into the structure of spacetime. We can define the energy density of this non-relativistic matter

$${\rho }_{\mathsf{\Lambda }}=\frac{\mathsf{\Lambda }}{8\pi G}$$

(305)

As $p_{\mathsf{\Lambda }}=-{\rho }_{\mathsf{\Lambda }}$ which gives an exotic form of matter with negative pressure, that is where the scale factor $a\left(t\right)$ goes on increasing but $\dot{a}\left(t\right)$ is decreasing. We write

$${\left(\frac{\dot{a}}{a}\right)}^2=\frac{8\pi G}{3}{\rho }_{\mathsf{\Lambda }}-\frac{k}{a^2}$$

(306)

$$\frac{\ddot{a}}{a}=-\frac{4\pi G}{3}\left({\rho }_{\mathsf{\Lambda }}+3p_{\mathsf{\Lambda }}\right)$$

(307)

and

$${\dot{\rho }}_{\mathsf{\Lambda }}+3H\left({\rho }_{\mathsf{\Lambda }}+p_{\mathsf{\Lambda }}\right)=0$$

(308)

From Equation (308) with ${\dot{\rho }}_{\mathsf{\Lambda }}=\frac{d{\rho }_{\mathsf{\Lambda }}}{dt}=0$ and $\frac{p_{\mathsf{\Lambda }}}{{\rho }_{\mathsf{\Lambda }}}=w$

$$3H\left({\rho }_{\mathsf{\Lambda }}+p_{\mathsf{\Lambda }}\right)=0$$

(309)

or

$$3H{\rho }_{\mathsf{\Lambda }}\left(1+w\right)=0$$

(310)

Now from Equation (307) for $\frac{p_{\mathsf{\Lambda }}}{{\rho }_{\mathsf{\Lambda }}}=w$

$$\frac{\ddot{a}}{a}=-\frac{4\pi G}{3}{\rho }_{\mathsf{\Lambda }}\left(1+3w\right)$$

(311)

For $w=-1$, Equation (311) becomes

$$\frac{\ddot{a}}{a}=-\frac{4\pi G}{3}{\rho }_{\mathsf{\Lambda }}\left(1-3\right)=\frac{8\pi G}{3}{\rho }_{\mathsf{\Lambda }}$$

(312)

or

$$\frac{d^{2}a}{d{t}^2}-\frac{8\pi G}{3}{\rho }_{\mathsf{\Lambda }}a=0$$

(313)

Equation (313) is the equation of a harmonic oscillator. From Equation (306) for vanishing curvature, i.e., $k=0$ where $\mathsf{\Lambda }$ dominates and $\frac{\dot{a}}{a}=H$

$$H_{\mathsf{\Lambda }}^2=\frac{8\pi G}{3}{\rho }_{\mathsf{\Lambda }}$$

(314)

Finding the value of ${\rho }_{\mathsf{\Lambda }}$

$${\rho }_{\mathsf{\Lambda }}=\frac{3H_{\mathsf{\Lambda }}^2}{8\pi G}$$

(315)

Substituting Equation (315) in Equation (313), and simplifying we have

$$\frac{d^{2}a}{d{t}^2}-\frac{8\pi G}{3}\left(\frac{3H_{\mathsf{\Lambda }}^2}{8\pi G}\right)a=0$$

(316)

or

$$\frac{d^{2}a}{d{t}^2}-3H_{\mathsf{\Lambda }}^{2}a=0$$

(317)

We can write the solution of above Equation (317) as

$$a\left(t\right)=C_{1}exp\left(H_{\mathsf{\Lambda }}t\right)+C_{2}exp\left(-H_{\mathsf{\Lambda }}t\right)$$

(318)

differentiating Equation (318) twice with respect to time ${}^{\prime }{t}^{\prime }$

$$\dot{a}\left(t\right)=C_1{H}_{\mathsf{\Lambda }}exp\left(H_{\mathsf{\Lambda }}t\right)-C_2{H}_{\mathsf{\Lambda }}exp\left(-H_{\mathsf{\Lambda }}t\right)$$

(319)

again

$$\ddot{a}\left(t\right)=C_1{H}_{\mathsf{\Lambda }}^{2}exp\left(H_{\mathsf{\Lambda }}t\right)+C_2{H}_{\mathsf{\Lambda }}^{2}exp\left(-H_{\mathsf{\Lambda }}t\right)$$

(320)

using Equation (318) in Equation (320), we can write

$$\ddot{a}\left(t\right)=H_{\mathsf{\Lambda }}^2\left(C_{1}exp\left(H_{\mathsf{\Lambda }}t\right)+C_{2}exp\left(-H_{\mathsf{\Lambda }}t\right)\right)=H_{\mathsf{\Lambda }}^{2}a\left(t\right)$$

(321)

substituting the value of ${\rho }_{\mathsf{\Lambda }}$ from Equation (315) in Equation (306), we have

$${\left(\frac{\dot{a}}{a}\right)}^2=\frac{8\pi G}{3}\left(\frac{3H_{\mathsf{\Lambda }}^2}{8\pi G}\right)-\frac{k}{a^2}=H_{\mathsf{\Lambda }}^2-\frac{k}{a^2}$$

(322)

simplifying Equation (322) gives

$$k=H_{\mathsf{\Lambda }}^2{a}^2-{\dot{a}}^2$$

(323)

substituting the values of $a\left(t\right)$ and $\dot{a}\left(t\right)$ from Equations (318) and (319) in above Equation (323)

$$\begin{array}{c}k=H_{\mathsf{\Lambda }}^2{\left(C_{1}exp\left(H_{\mathsf{\Lambda }}t\right)+C_{2}exp\left(-H_{\mathsf{\Lambda }}t\right)\right)}^2\hfill \\ -{\left(C_1{H}_{\mathsf{\Lambda }}exp\left(H_{\mathsf{\Lambda }}t\right)-C_2{H}_{\mathsf{\Lambda }}exp\left(-H_{\mathsf{\Lambda }}t\right)\right)}^2\hfill \end{array}$$

(324)

simplification gives

$$k=4H_{\mathsf{\Lambda }}^2{C}_1{C}_2$$

(325)

Equation (325) means that the curvature term k depends upon the constants of integration $C_1$ and $C_2$. For flat universe either $C_1=0$ or $C_2=0$. The solution in Equation (318) becomes accordingly

$$a\left(t\right)=C_2{e}^{-H_{\mathsf{\Lambda }}t}$$

(326)

and

$$a\left(t\right)=C_1{e}^{H_{\mathsf{\Lambda }}t}$$

(327)

Further Einstein equations are given by

$$G_{\mu \nu }+\mathsf{\Lambda }{g}_{\mu \nu }=8\pi T_{\mu \nu }$$

(328)

where

$$G_{\mu \nu }=R_{\mu \nu }-\frac{1}{2}{g}_{\mu \nu }R$$

(329)

and the form of solution of these equations upon which big bang standard cosmology is based, as worked out by Alexander Friedman (1922), George Lemaitre (1927), and afterwards by Robertson and Walker (1935) independently on the base of cosmological principle which put to use the homogeneity and isotropy, is

$$d{s}^2=-d{t}^2+a{\left(t\right)}^2\left({\left(1-k{r}^2\right)}^{-1}d{r}^2+r^{2}d{\theta }^2+r^2{sin}^2\theta d{\varphi }^2\right)$$

(330)

where ${\mathsf{\Omega }}^2=d{\theta }^2+{sin}^2\theta d{\varphi }^2$. The metric in Equation (330) is characterized by scale factor $a\left(t\right)$ and the curvature of spacetime k which are obviously determined by the self-gravitation of all the matter–energy content in the universe. We have incorporated dark matter and dark energy in the matter–energy content because their role is not avoidable at all in accelerated expansion and the present Minkowskian flat geometry of the universe. The solution of this line element gives Friedman equations using Einstein field equations that govern the time evolution of the universe and are given as

$$\frac{{\dot{a}}^2}{a^2}+\frac{k}{a^2}=\frac{8\pi G}{3}\rho +\frac{\mathsf{\Lambda }}{3}$$

(331)

and

$$\frac{\ddot{a}}{a}=-\frac{4\pi G}{3}\left(\rho +3p\right)+\frac{\mathsf{\Lambda }}{3}$$

(332)

The presence of cosmological term $\mathsf{\Lambda }$ in the above equations would be equivalent to that of a fluid having an equation of state $p=-\rho$ which is satisfied by

$$\rho +3p>0$$

(333)

Looking at the things classically, we may approach the classical period of exponential expansion by using the first Friedmann equation by vanishing density $\rho$ of radiation and baryons and the entailing curvature k in $\mathsf{\Lambda }$-dominated Era which corresponds to equivalently having a fluid with $p=-\rho$, thus Equation (331) becomes

$$\begin{array}{c}\frac{{\dot{a}}^2}{a^2}+\frac{\left(0\right)}{a^2}=\frac{8\pi G}{3}\left(0\right)+\frac{\mathsf{\Lambda }}{3}\hfill \\ \frac{{\dot{a}}^2}{a^2}=\frac{\mathsf{\Lambda }}{3}\hfill \\ \dot{a}=\frac{da}{dt}=\sqrt{\frac{\mathsf{\Lambda }}{3}}a\hfill \\ \frac{da}{a}=\sqrt{\frac{\mathsf{\Lambda }}{3}}dt\hfill \end{array}$$

(334)

after integrating and simplifying, we get

$$a=e^{\sqrt{\frac{\mathsf{\Lambda }}{3}}t}$$

(335)

Equation (335) gives the exponential expansion of the scale factor. It describes the fact that when the universe was dominated by cosmological constant $\mathsf{\Lambda }$, the rate expansion was much faster than the present day scenario. From Equation (332)

$$\ddot{a}=-\frac{4\pi G}{3}\left(\rho +3p\right)a+\frac{\mathsf{\Lambda }}{3}a$$

(336)

Considering a closed volume with energy $U=\rho V=\rho \frac{4\pi }{3}{a}^3$ and now we see how inflationary period is obtained in the perspective of particle physics where a negative pressure is achieved for it to take place. Friedmann solved EFE with $\mathsf{\Lambda }=0$, so

$$\frac{{\dot{a}}^2}{a^2}+\frac{k}{a^2}=\frac{8\pi G}{3}\rho$$

(337)

$$\frac{\ddot{a}}{a}=-\frac{4\pi G}{3}\left(\rho +3p\right)$$

(338)

Equation (338) is known as acceleration equation. The inflationary period, as its definition implies, is the acceleratingly expanding phase of the universe in a very small fraction of first second, as the expansion is characterized by the scale factor a; therefore, we have such an era as

$$\ddot{a}>0$$

(339)

thus Inflationary era

$$\iff \ddot{a}>0$$

(340)

dividing both sides of Equation (317) by scale factor a

$$\frac{\ddot{a}}{a}>0$$

(341)

which is LHS of Equations (338) and (341) imposes the condition on RHS of Equation (338)

$$\begin{array}{c}-\frac{4\pi G}{3}\left(\rho +3p\right)>0\hfill \\ \Rightarrow \rho +3p<0\hfill \\ \Rightarrow p<-\frac{1}{3}\rho \hfill \\ \Rightarrow \rho >-3p\hfill \end{array}$$

(342)

For the inflation to occur and set the universe in an accelerating phase, we require the matter to possess an equation of state with negative pressure. The possibility of this negative pressure p which is less than negative of one-third of density is in perspective of symmetry breaking in modern models of particle physics. From

$$\frac{{\dot{a}}^2}{a^2}+\frac{k}{a^2}=\frac{8\pi G}{3}\rho$$

(343)

$${\dot{a}}^2=\frac{8\pi G}{3}\rho a^2-k$$

(344)

For $\ddot{a}>o$, the scale factor shall increase faster than $a\left(t\right)\propto t$ and the term $\frac{8\pi G}{3}\rho a^2$ shall increase during this accelerated era such that the curvature term k will become negligibly small and shall vanish. Inflationary era is also defined by considering the shrinking of Hubble Sphere [43] due to its direct linkage to the horizon problem and because it provides a fundamental role in producing of quantum fluctuations. Shrinking Hubble Sphere is defined as

$$\frac{d\left[{\left(aH\right)}^{-1}\right]}{dt}<0$$

(345)

$$\frac{d\left[{\left(aH\right)}^{-1}\right]}{dt}=\frac{d\left[{\left(a\frac{\dot{a}}{a}\right)}^{-1}\right]}{dt}=\frac{d\left[{\left(\dot{a}\right)}^{-1}\right]}{dt}=-\frac{\ddot{a}}{a^2}$$

(346)

$$-\frac{\ddot{a}}{a^2}<0$$

(347)

which will imply accelerated expansion

$$\ddot{a}>0$$

(348)

At $t=0$, the scale factor a characterizing expansion of the universe comes out to be of a specific value. In Equation (337), when $\rho ={\rho }_{\varphi }$ is of very larger value and the scale factor a dominates over the curvature term k, then we have

$${\left(\frac{\dot{a}}{a}\right)}^2=H^2=\frac{8\pi G}{3}{\rho }_{\varphi }$$

(349)

$$a=a_0{e}^{Ht}$$

(350)

de Sitter line element is given by

$$d{s}^2=-d{t}^2+e^{2Ht}\left(d{x}^2+d{y}^2+d{z}^2\right)$$

(351)

inflation has to terminate and H is constant, meaning that the de Sitter phase cannot give perfect inflationary era, however for $\frac{\dot{H}}{H^2}$, it would compensate. It would be interesting here to note that Z. G. Lie and Y.S. Piao have shown that the universe we observe today may have emerged from a de Sitter background without having the requirement of a large tunneling in potential and with low energy scale [50].

10.4. The Conditions under Which the Inflation Occurs

Shrinking Hubble sphere has been considered as basic definition of inflationary era due to its direct connection to the horizon problem and with mechanism of quantum fluctuation generations [51]. differentiating the comoving Hubble radius ${\left(aH\right)}^{-1}$ with respect to time we find the acceleratedly expanding Hubble sphere

$${\partial }_t{\left(aH\right)}^{-1}=-\frac{\ddot{a}}{{\dot{a}}^2}$$

(352)

We see that $-\frac{\ddot{a}}{{\dot{a}}^2}<0$, multiplying the inequality by $-1$ and simplifying, we have

$$\ddot{a}>0$$

(353)

which means that shrinking comoving Hubble sphere ${\left(aH\right)}^{-1}$ points toward accelerated expansion $\ddot{a}>0$. As Hubble sphere H remains nearly constant, in order to understand the meaning of nearly constant we see how its slow roll variation takes place, so taking H as variable

$${\partial }_t\left(\frac{1}{aH}\right)=-\frac{\dot{a}H+a\dot{H}}{{\left(aH\right)}^2}=-\frac{1}{a}\left(1+\frac{\dot{H}}{H^2}\right)$$

(354)

where $\frac{\dot{H}}{H^2}=-\epsilon$ known as slow roll parameter. It can be inferred that $\frac{\dot{H}}{H^2}<0$ implies shrinking Hubble sphere.

10.5. Slow Roll Inflation—The Dynamics of Scalar Field

Elementary particles in modern physics are represented by quantum fields and oscillations of these fields are translated as particles. Scalar fields represent spin zero particles in field theories and look like vacuum states because they have same quantum numbers as vacuum. The matter with negative pressure $\rho =-p$ represents physical vacuum-like state where the quantum fluctuations of all types of physical fields exist. These fluctuations can be considered as waves of all possible wavelengths related with physical fields, i.e., wavy physical fields moving freely in all directions. The negative pressure violates the strong energy condition which is necessary for the inflation to occur. To keep things simpler a single scalar field namely inflaton $\varphi =\varphi \left(x,t\right)$ is considered present in the very early universe, as the value of the scalar field depends upon position x in space which assigns potential energy to each field value. It is also dynamical due to being function of time t and has kinetic energy as well, i.e., energy density $\rho \left(\varphi \right)$ associated with the inflaton $\varphi$ is $\rho \left(\varphi \right)={\rho }_p+{\rho }_k$. The ratio of the potential and kinetic energy terms of $\varphi =\varphi \left(x,t\right)$, decides the evolution of the universe. The Lagrangian of the scalar inflaton field $\varphi$ is expressed as the energy difference between its kinetic and potential terms, that is

$$\mathcal{L}=\frac{1}{2}\left(g_{\mu \nu }{\partial }^2\varphi -V\left(\varphi \right)\right)$$

(355)

It is assumed that the background of FLRW universe has been sourced by energy-momentum associated with the inflaton that dominates the universe in the beginning. We shall observe under what conditions this causes accelerated expansion of the FLRW universe.

$$S=\int d^{4}x\sqrt{-g}\mathcal{L}=\int d^{4}x\sqrt{-g}\left[\frac{1}{2}\left(g_{\mu \nu }{\partial }^2\varphi -V\left(\varphi \right)\right)\right]$$

(356)

The energy-momentum tensor of the inflaton field is given as

$$T_{\mu \nu }={\partial }_{\mu }\varphi {\partial }_{\nu }\varphi -g_{\mu \nu }\mathcal{L}$$

(357)

$$T_{\mu \nu }={\partial }_{\mu }\varphi {\partial }_{\nu }\varphi -g_{\mu \nu }\left(L\right)$$

(358)

which for $\mu =0$, $\nu =0$ results as

$$T_{00}=\frac{1}{2}{\dot{\varphi }}^2+\frac{1}{2a^2}{\nabla }^2\varphi +V\left(\varphi \right)$$

(359)

and for $\mu =\nu =j$

$$T_{jj}=\frac{1}{2}{\dot{\varphi }}^2-\frac{1}{6a^2}{\nabla }^2\varphi -V\left(\varphi \right)$$

(360)

The gradient term vanishes, in the otherwise condition, the pressure gained is much less than the required value to impart impetus for inflation to take place, therefore we obtain the following values for energy density and pressure

$${\rho }_{\varphi }=T_{00}=\frac{1}{2}{\dot{\varphi }}^2+V\left(\varphi \right)$$

(361)

and

$$p_{\varphi }=T_{jj}=\frac{1}{2}{\dot{\varphi }}^2-V\left(\varphi \right)$$

(362)

The condition $V\left(\varphi \right)>>{\dot{\varphi }}^2$ corresponds to the negative pressure condition ${\rho }_{\varphi }=-p_{\varphi }$ which means that the potential (vacuum) energy of the inflaton derives inflation. Now using Euler–Lagrange equations

$${\partial }^{\mu }\frac{\delta \left(\sqrt{-g}L\right)}{\delta {\partial }^{\mu }\varphi }-\frac{\delta \left(\sqrt{-g}L\right)}{\delta \varphi }=0$$

(363)

we can find equation for inflaton field that comes to be

$$\ddot{\varphi }+3\frac{\dot{a}}{a}\dot{\varphi }-\frac{1}{a^2\left(t\right)}{\nabla }^2\varphi +V_{,\varphi }\left(\varphi \right)=0$$

(364)

It can also be computed from the energy density and the pressure terms given in Equations (361) and (362) respectively by substituting in equation of energy conservation

$$\frac{d\rho }{dt}+3H\left(\rho +p\right)=0$$

(365)

Equation (365) in terms of inflation field $\varphi$

$$\frac{d{\rho }_{\varphi }}{dt}+3H\left({\rho }_{\varphi }+p_{\varphi }\right)=0$$

(366)

By substituting Equations (361) and (362) in Equation (366), we have

$$\frac{d\left(\frac{1}{2}{\dot{\varphi }}^2+V\left(\varphi \right)\right)}{dt}+3H\left(\frac{1}{2}{\dot{\varphi }}^2+V\left(\varphi \right)+\frac{1}{2}{\dot{\varphi }}^2-V\left(\varphi \right)\right)=0$$

(367)

$$\left(\ddot{\varphi }+V^{\prime }\left(\varphi \right)+3H\dot{\varphi }\right)\dot{\varphi }=0$$

(368)

$$\ddot{\varphi }+V^{\prime }\left(\varphi \right)+3H\dot{\varphi }=0$$

(369)

where $V^{\prime }\left(\varphi \right)=\frac{dV\left(\varphi \right)}{d\varphi }$ and the term $3H\dot{\varphi }$ is known as friction term and offers friction to the inflaton field when it rolls down $\left(\dot{\varphi }\right)$ its potential during expansion of the universe $H=\frac{\dot{a}}{a}$. Figure 18 and Figure 19 manifest how the scalar field drives the universe evolution in the beginning and how does it slow roll afterward respectively:

10.6. Conditions of the Slow Roll Inflation

According to the big bang model, that is, the currently accepted model, the universe is about 14 billion years old. At the point of existence the curvature of spacetime was very large or equivalently can be described in other words that space was largely warped and curved where only quantum effects can prevail and the question of time to exist is likely to become absurd. From this state how the very brief era of exponential expansion can be had is fulfilled by assumption of scalar field which take the responsibility of such state mentioned. We know from the 2nd Friedmann’s equation which is acceleration equation

$$\frac{\ddot{a}}{a}=-\frac{4\pi G}{3}\left({\rho }_{\varphi }+3p_{\varphi }\right)$$

(370)

For $\ddot{a}>0$

$${\rho }_{\varphi }+3p_{\varphi }<0\Rightarrow p_{\varphi }<\frac{1}{3}{\rho }_{\varphi }$$

(371)

From Equations (361) and (362), substituting for $p_{\varphi }$ and ${\rho }_{\varphi }$ in Equation (371)

$$\left(\frac{1}{2}{\dot{\varphi }}^2-V\left(\varphi \right)\right)<-\frac{1}{3}\left(\frac{1}{2}{\dot{\varphi }}^2+V\left(\varphi \right)\right)$$

(372)

solving the inequality and keeping in mind that $\dot{\varphi }$ is a squared term, we have

$${\dot{\varphi }}^2<<V\left(\varphi \right)$$

(373)

which means that the inflaton field is slowly rolling down its potential. Differentiating Equation (373) with respect to time, we have

$$\ddot{\varphi }<\frac{1}{2}{V}^{\prime }\left(\varphi \right)$$

(374)

Now from Equation (369), we obtain

$$\ddot{\varphi }+V^{\prime }\left(\varphi \right)=-3H\dot{\varphi }$$

(375)

We neglect the acceleration providing term $\ddot{\varphi }=\frac{d^2\varphi }{d{t}^2}$ as the inflaton field has to roll now slowly to escape from graceful exit problem in inflation i.e., it is decelerating, so we write

$$V^{\prime }\left(\varphi \right)=-3H\dot{\varphi }$$

(376)

plugging Equation (376) in Equation (374)

$$\ddot{\varphi }<\frac{1}{2}(-3H\dot{\varphi })$$

(377)

On neglecting the constant factor, it gives

$$\ddot{\varphi }<<3H\dot{\varphi }$$

(378)

differentiating now Equation (376) with respect to time,

$$3\left(\dot{H}\dot{\varphi }+H\ddot{\varphi }\right)=-V^{″}\left(\varphi \right)\dot{\varphi }$$

(379)

As H remains constant during inflation, therefore $\dot{H}$ vanishes and we have

$$\ddot{\varphi }=-\frac{V^{″}\left(\varphi \right)\dot{\varphi }}{3H}$$

(380)

Putting Equation (380) in Equation (378), we have

$$-\frac{V^{″}\left(\varphi \right)\dot{\varphi }}{3H}<<3H\dot{\varphi }$$

(381)

It gives

$$V^{″}\left(\varphi \right)<<H^2$$

(382)

10.7. Parameters for the Slow Roll Inflation

Two slow roll parameters $\epsilon$ and $\eta$ are defined in terms of Hubble parameter H as well as potential V which quantify slow roll inflation.

$${\epsilon }_H=-\frac{\dot{H}}{H^2}$$

(383)

Using the relation $a\left(t\right)\propto e^{-N}\Rightarrow N=lna$, it can also be expressed in the form

$${\epsilon }_H=-\frac{d\left(lnH\right)}{dN}$$

(384)

where N is the number of e-folds and 2nd is defined as

$${\eta }_H=-\frac{1}{2}\frac{\ddot{H}}{\dot{H}H}$$

(385)

From 1st Friedmann equation

$${\left(\frac{\dot{a}}{a}\right)}^2-\frac{k}{a^2}=\frac{8\pi G}{3}\rho$$

(386)

For $\rho ={\rho }_{\varphi }$ and from Equation (361) ${\rho }_{\varphi }=\frac{1}{2}{\dot{\varphi }}^2+V\left(\varphi \right)$, as during inflation $V\left(\varphi \right)>>{\dot{\varphi }}^2$, so that ${\rho }_{\varphi }=V\left(\varphi \right)$ also curvature term k is negligibly small, so that Equation (386) becomes

$$H^2=\frac{8\pi G}{3}V\left(\varphi \right)$$

(387)

differentiating Equation (387) with respect to time and simplifying

$$\dot{H}=\frac{4\pi G}{3H}{V}^{\prime }\left(\varphi \right)\left(\dot{\varphi }\right)$$

(388)

And from Equation (376) substituting in Equation (388), we have

$$\dot{H}=-4\pi G\left({\dot{\varphi }}^2\right)$$

(389)

Substituting above in Equation (383), we have

$${\epsilon }_H=-\frac{\dot{H}}{H^2}=-\frac{-4\pi G\left({\dot{\varphi }}^2\right)}{H^2}=\frac{4\pi G}{H^2}{\dot{\varphi }}^2$$

(390)

Again from Equation (376), we have

$$\dot{\varphi }=-\frac{V^{\prime }\left(\varphi \right)}{3H}$$

(391)

squaring

$${\dot{\varphi }}^2=-\frac{{V^{\prime }}^2\left(\varphi \right)}{9H^2}$$

(392)

substituting in Equation (390)

$${\epsilon }_H=\frac{4\pi G}{H^2}\left(-\frac{{V^{\prime }}^2\left(\varphi \right)}{9H^2}\right)=\frac{4\pi G{V^{\prime }}^2\left(\varphi \right)}{9{\left(H^2\right)}^2}$$

(393)

From Equation (387) putting for $H^2$

$${\epsilon }_V=\frac{4\pi G{V^{\prime }}^2\left(\varphi \right)}{9{\left(\frac{8\pi G}{3}V\left(\varphi \right)\right)}^2}=\frac{1}{16\pi G}{\left(\frac{V^{\prime }\left(\varphi \right)}{V\left(\varphi \right)}\right)}^2=\frac{M_{pl}^2}{2}{\left(\frac{V^{\prime }\left(\varphi \right)}{V\left(\varphi \right)}\right)}^2$$

(394)

${\eta }_H$ can also be expressed as

$${\eta }_H=-\frac{\ddot{\varphi }}{H\dot{\varphi }}$$

(395)

$${\eta }_V=\frac{1}{8\pi G}\left(\frac{V^{″}\left(\varphi \right)}{V\left(\varphi \right)}\right)=M_{pl}^2\left(\frac{V^{″}\left(\varphi \right)}{V\left(\varphi \right)}\right)$$

(396)

From Equation (387) $H^2=\frac{8\pi G}{3}V\left(\varphi \right)$, which gives $8\pi GV\left(\varphi \right)=3H^2$ substituting above in Equation (396), we have

$${\eta }_V=\frac{V^{″}\left(\varphi \right)}{3H^2}$$

(397)

10.8. Number of e-Folds

It is usual practice to have the inflation quantified and the quantity which does this is called number of e-fold denoted by N before the inflation ends. As the time goes by N goes on decreasing and becomes zero when inflation ends. It is counted or measured backwards in time from the end of inflation which means that $N=0$ at the end of inflation grows to maximal value towards the beginning of inflation. It measures the number of times the space grows during inflationary period. The amount of e-folds necessarily required to resolve the big bang problems of Horizon, Flatness, Monopole, Entropy, etc. is $N\sim$ 60–75 depending upon the different models and on the reasonable estimation of the observational parameters. To find the number of e-folds between beginning and end of inflation we know that during inflation the scale factor evolves as

$$a\left(t\right)=a\left(t_0\right)e^{Ht}$$

(398)

or

$$a\left(t\right)=a\left(t_0\right)e^{H\left(t-t_i\right)}$$

(399)

The factor $Ht$ constitute the number of e-folds denoted by N, i.e.,

$$N=Ht$$

(400)

differentiating Equation (400) with respect to time

$$\frac{dN}{dt}=H={\partial }_{t}lna$$

(401)

$$N={\int }_{t_i}^{t_f}Hdt={\int }_{t_i}^{t_f}\frac{\dot{a}}{a}dt=ln\left(\frac{a_{t_f}}{a_{t_i}}\right)$$

(402)

Further, the relation between Hubble parameter H and the number of e-folds N can be written. we have derived earlier the evolution equation for inflaton field that reads

$$\ddot{\varphi }+3H\dot{\varphi }+V_{,\varphi }=0$$

(403)

During slow roll inflation $\ddot{\varphi }=0$, so that Equation (403) becomes

$$3H\dot{\varphi }+V_{,\varphi }=0$$

(404)

$$3H\dot{\varphi }=-V_{,\varphi }$$

(405)

Moreover, during slow roll the Friedmann’s 1st equation evolves as with $k=0$ and $\rho =V\left(\varphi \right)+\frac{1}{2}{\dot{\varphi }}^2$

$$H^2=\frac{8\pi G}{3}\left(V\left(\varphi \right)+\frac{1}{2}{\dot{\varphi }}^2\right)$$

(406)

During slow roll ${\left(\dot{\varphi }\right)}^2<<V\left(\varphi \right)$and only $\dot{\varphi }$ works, thus Equation (406) becomes

$$H^2=\frac{8\pi G}{3}V\left(\varphi \right)$$

(407)

Dividing Equation (405) by Equation (407)

$$\frac{\dot{\varphi }}{H}=-\frac{V_{,\varphi }}{8\pi GV\left(\varphi \right)}$$

(408)

Now from Equation (400), we can write because $t=t_f-t_i$, so $t={\int }_{t_i}^{t_f}dt$ and with dividing and multiplying by $d\varphi$

$$N=Ht={\int }_{t_i}^{t_f}Hdt={\int }_{t_i}^{t_f}H\frac{dt}{d\varphi }d\varphi$$

(409)

where $\dot{\varphi }=\frac{d\varphi }{dt}$, Equation (409) takes the form

$$N={\int }_{{\varphi }_i}^{{\varphi }_f}\frac{H}{\dot{\varphi }}d\varphi$$

(410)

Substituting from Equation (408) after inverting

$$N={\int }_{{\varphi }_i}^{{\varphi }_f}\left(-\frac{8\pi GV\left(\varphi \right)}{V_{,\varphi }}\right)d\varphi =-8\pi G{\int }_{{\varphi }_i}^{{\varphi }_f}\frac{V\left(\varphi \right)}{V_{,\varphi }}d\varphi$$

(411)

or

$$N=8\pi G{\int }_{{\varphi }_f}^{{\varphi }_i}\frac{V\left(\varphi \right)}{V_{,\varphi }}d\varphi$$

(412)

Thus number of e-folds can be found in terms of potential of the inflaton field. Further slow roll parameter ${\epsilon }_H$ can be described in terms of number of e-fold N, we know

$${\epsilon }_H=-\frac{\dot{H}}{H^2}=-\frac{1}{H^2}\frac{dH}{dt}=-\frac{1}{H^2}\frac{dH}{dN}\frac{dN}{dt}$$

(413)

$${\epsilon }_H=-\frac{1}{H^2}\frac{dlnN}{dt}$$

(414)

11. Inflationary Solutions to the Big Bang Problems

Horizon, flatness, entropy, and monopole problems are initial value problems which inflation solves in one go. Inflation explains why the observable universe is spatially flat, isotropically homogeneous and so large in size.

11.1. Inflation and Horizon Problem

We consider that the inflation begins at a time $\left(t_i\right)$ and comes to an end at some time $\left(t_f\right)$ and the expansion rate $H={\partial }_{t}lna$, curvature term k and energy density of matter and radiation $\rho ={\rho }_M+{\rho }_R$ during inflation vanishes, we know that

$$a\left(t\right)=a\left(t\right)e^{Ht}=a\left(t\right)e^{H(t-t_i)}$$

(415)

and

$$a\left(t\right)=a\left(t\right)e^{Ht}=a\left(t\right)e^{H(t_f-t)}$$

(416)

We will find how long the inflation must sustain to resolve the horizon problem. We can find the corresponding e-folding number N that is

$$N=Ht={\int }_{t_i}^{t_f}Hdt$$

(417)

As $H=\frac{\dot{a}}{a}$,

$$N={\int }_{t_i}^{t_f}\frac{\dot{a}}{a}dt={\int }_{t_i}^{t_f}\frac{da}{a}$$

(418)

$$N={\left.lna\right|}_{t_i}^{t_f}=ln\left(a_f-a_i\right)$$

(419)

or

$$\frac{a_f}{a_i}=e^N$$

(420)

or

$$\frac{a_i}{a_f}=e^{-N}$$

(421)

Now the horizon scale observed today $H_0^{-1}$ was reduced during inflation to a value of ${\lambda }_{H_0}\left(t_i\right)$ which is smaller than the horizon length during inflation.

$${\lambda }_{H_0}\left(t_i\right)=R_{H_0}\left(\frac{a_{t_i}}{a_{t_0}}\right)$$

(422)

Dividing and multiplying Equation (422) by $a_{t_f}$

$${\lambda }_{H_0}\left(t_i\right)=R_{H_0}\left(\frac{a_{t_i}}{a_{t_0}}\times \frac{a_{t_f}}{a_{t_f}}\right)$$

(423)

Now, from Equation (421) $\frac{a_i}{a_f}=e^{-N}$ and using the relation between scale factor and temperature during this phase $a\sim \frac{1}{T}$$\Rightarrow a_i\sim \frac{1}{T_i}$ and $\Rightarrow a_f\sim \frac{1}{T_f}$ so that we have

$${\lambda }_{H_0}\left(t_i\right)=H_0^{-1}\frac{T_0}{T_f}{e}^{-N}$$

(424)

where $R_{H_0}=H_0^{-1}$. Now ${\lambda }_{H_0}\left(t_i\right)<H_I^{-1}$ where $H_I^{-1}$ is the horizon length during inflation. Therefore, Equation (424) can be expressed as

$$H_0^{-1}\frac{T_0}{T_f}{e}^{-N}\le H_I^{-1}$$

(425)

$$\frac{H_0^{-1}}{H_I^{-1}}\frac{T_0}{T_f}\le e^N$$

(426)

or

$$e^N\ge \frac{\left(\frac{T_0}{H_0}\right)}{\left(\frac{T_f}{H_I}\right)}\Rightarrow N\ge ln\frac{\left(\frac{T_0}{H_0}\right)}{\left(\frac{T_f}{H_I}\right)}$$

(427)

$$N\ge ln\left(\frac{T_0}{H_0}\right)-ln\left(\frac{T_f}{H_I}\right)$$

(428)

or

$$N\approx 67+ln\left(\frac{H_I}{T_f}\right)$$

(429)

$$N\ge 70$$

(430)

11.2. Inflation and Flatness Problem

From 1st Friedmann equation,

$$H^2+\frac{k}{a^2}=\frac{8\pi G}{3}\rho$$

(431)

We found the density parameter expression

$$\mathsf{\Omega }-1=\frac{k}{H^2{a}^2\left(t\right)}$$

(432)

during the inflationary period Hubble parameter giving expansion rate remains almost constant so that Equation (432) is

$$\mathsf{\Omega }-1=\frac{k}{H^2{a}^2\left(t\right)}\propto \frac{1}{a^2\left(t\right)}$$

(433)

We observed earlier that

$${\left|\mathsf{\Omega }-1\right|}_{t=t_{pl}}\approx {10}^{-60}$$

(434)

which means that to have the value of the density parameter as observed today, i.e., ${\mathsf{\Omega }}_0$ to be of the order of unity, the initial value of $\mathsf{\Omega }$ at the beginning of the radiation-dominated era must be same as given in Equation (434) above, and from Equation (432), we can write for the time at the beginning of inflationary era

$${\left|\mathsf{\Omega }-1\right|}_{t=t_i}=\frac{k}{H_I^2{a}_i^2\left(t\right)}$$

(435)

and for the time when inflationary period comes to end

$${\left|\mathsf{\Omega }-1\right|}_{t=t_f}=\frac{k}{H_I^2{a}_f^2\left(t\right)}$$

(436)

Further, the beginning of the radiation-dominated era can be recognized with the beginning of inflationary phase such that it is required

$${\left|\mathsf{\Omega }-1\right|}_{t=t_f}={10}^{-60}$$

(437)

dividing now Equation (436) by Equation (435)

$$\frac{{\left|\mathsf{\Omega }-1\right|}_{t=t_f}}{{\left|\mathsf{\Omega }-1\right|}_{t=t_i}}=\frac{\frac{k}{H_I^2{a}_f^2\left(t\right)}}{\frac{k}{H_I^2{a}_i^2\left(t\right)}}=\left(\frac{a_i^2\left(t\right)}{a_f^2\left(t\right)}\right)$$

(438)

We calculated $\frac{a_i}{a_f}=e^{-N}$, the above Equation (438) takes the form

$$\frac{{\left|\mathsf{\Omega }-1\right|}_{t=t_f}}{{\left|\mathsf{\Omega }-1\right|}_{t=t_i}}={\left(e^{-N}\right)}^2$$

(439)

$$e^{-2N}=\frac{{\left|\mathsf{\Omega }-1\right|}_{t=t_f}}{{\left|\mathsf{\Omega }-1\right|}_{t=t_i}}$$

(440)

With taking ${\left|\mathsf{\Omega }-1\right|}_{t=t_i}\approx 1$,

$$N\approx -\frac{1}{2}ln{\left|\mathsf{\Omega }-1\right|}_{t=t_f}$$

(441)

$$N\simeq 70$$

(442)

11.3. Inflation and Entropy Problem

The entropy problem can be resolved if a large amount of entropy is created in the very early universe non-adiabatically [48,51] which is accomplished by inflationary era in a finite time in the early history of the universe. Let the entropy at the end of inflation is $S_f$ and in the beginning it was $S_i$ such that $S_f\propto S_i$, then

$$S_f=M^3{S}_i$$

(443)

where M is the numerical factor with value $M^3={10}^{10}\Rightarrow M={10}^{30}$. Now $S_f=S_U$. We know that $S\sim {\left(aT\right)}^3$, so that we can write for

$$S_i\sim {\left(a_i{T}_i\right)}^3$$

(444)

and for

$$S_f\sim {\left(a_f{T}_f\right)}^3$$

(445)

where $T_i$ and $T_f$ are the measures of temperature at the beginning and end of the inflationary period. dividing Equation (445) by Equation (444) we have

$$\frac{S_f}{S_i}\approx {\left(\frac{a_f}{a_i}\right)}^3{\left(\frac{T_f}{T_i}\right)}^3$$

(446)

or

$${\left(\frac{a_f}{a_i}\right)}^3\approx \frac{S_f}{S_i}{\left(\frac{T_i}{T_f}\right)}^3$$

(447)

$$\frac{a_f}{a_i}\approx {\left(\frac{S_f}{S_i}\right)}^3\frac{T_i}{T_f}$$

(448)

Now $\frac{a_f}{a_i}=e^N$, and considering that at the beginning of inflationary phase the total entropy of the universe was of the order 1, i.e., $S_i\sim 1$ and $S_f=S_U$, thus Equation (448) takes the form

$$e^N\approx {\left(S_U\right)}^3\frac{T_i}{T_f}$$

(449)

$$N\approx ln{\left(S_U\right)}^3\frac{T_i}{T_f}$$

(450)

$$N\sim 70$$

(451)

therefore, entropy problem is resolved by inflationary period.

11.4. Inflation and Monopole Problem

In grand unified theories (GUT), the standard model $SU\left(3\right)\times SU\left(2\right)\times U\left(1\right)$ in particle physics emerges out of a simple symmetry group breaking. In these theories, heavy particles of very high density are predicted to be created which are known as magnetic monopoles. The cosmological monopoles prior to the period of inflation are considered to take place and are allowed supposedly to exist. It means that inflation allows the existence of magnetic monopoles, that is to say that as they are created earlier to era of inflation, these magnetic monopoles are supposed to form in symmetry breaking during phase transitions and where the inflationary era is considered to take place just after it. Inflation dilutes the density of these magnetic monopoles $n_{mp}\propto \frac{N_{mp}}{a^3}\to 0$ to the negligibly small size such that these become so small to be detected today [52]. During inflation monopoles collapse in an exponential way and their abundant presence falls to the level of being hardly detectable.

11.5. Inflation and Observations

Cosmological perturbations are an important relic of the inflation used to describe the anisotropies of the cosmic microwave background (CMB) and structure evolution and formation of the universe. The seeds of inhomogeneities, which represent all the structure in the universe were produced during inflationary phase and were stretched to the astronomical scales with the exponential expansion. These inhomogeneities are what we see today as stars, galaxies, etc. in the form of baryonic matter. From the theory of linear perturbations and from the relation $\delta \varphi \iff \delta g_{\mu \nu }$ we know how to categorize FLRW metric perturbations at the first order in the form of scalar, vector, and tensor perturbations of spins 0, 1, and 2, respectively. A very important parameter which determines the properties of the perturbations of the scalar field is power spectrum $p_{\varphi }\left(k\right)$. The scalar field power spectrum at the time of horizon crossing comes out to be

$$p_{\varphi }\left(k\right)={\left(\frac{H}{2\pi }\right)}_{k=aH}^2$$

(452)

and the curvature power spectrum is calculated to be

$$p_R\left(k\right)={\left[\left(\frac{H}{\dot{\varphi }}\right)\frac{H}{2\pi }\right]}_{k=aH}^2$$

(453)

and the power spectrum for tensor perturbations is given by

$$p_T\left(k\right)=64\pi G{\left(\frac{H}{2\pi }\right)}_{k=aH}^2$$

(454)

The tensor-to-scalar ratio of the spectrum is defined in the following expression and is found in terms of the first slow roll parameter:

$$r=\frac{p_T\left(k\right)}{p_R\left(k\right)}=16{\epsilon }_V$$

(455)

We can now define scalar and tensor spectral indices as

$$n_s-1=\frac{dln{p}_R\left(k\right)}{dlnk}$$

(456)

and

$$n_T=\frac{dln{p}_T\left(k\right)}{dlnk}$$

(457)

scale invariance of the scalar power spectrum is characterized by $n_s-1=0\Rightarrow n_s=1$. Deviations from the scale invariance in an inflationary model gives it specific features. In this case, the spectral indices to their lowest order can be described in terms of potential slow roll parameters ${\epsilon }_V$ and ${\eta }_V$. In case of large field model, a general polynomial potential is

$$V\left(\varphi \right)={\mathsf{\Lambda }}^4{\left(\frac{\varphi }{\mu }\right)}^p$$

(458)

and we find

$$r=4\left(\frac{p}{N}\right)$$

(459)

$$n_s-1=-\frac{1}{N}\left(1+\frac{p}{2}\right)$$

(460)

For a particular inflationary model p must be assigned a value greater than unity [53].

11.6. $\mathsf{\Lambda }$CDM

The standard model of cosmology describes a universe that evolves from a singularity at $t=0$. This singularity is known as the big bang and marks the instant when the universe begins its evolution in time. A detailed discussion of big bang theory of creation is presented in Appendix D. The kinematics of it is described by FLRW spacetime, and its dynamics is governed by the Friedmann equations in the framework of general relativity. The standard model is usually known as big bang model [54] due to extrapolation of redshift towards big bang singularity. The observational parameters are not fixed by the standard model or big bang which means that the big bang is parameterizable. $\mathsf{\Lambda }$CDM model constitutes one of such parameterizations and shows remarkable consistency with the recent observations. That is why it has gathered support by majority of cosmologists. It incorporates the ingredients, namely, cosmological constant $\mathsf{\Lambda }$ and cold matter (CDM), in addition to ordinary matter. It is interesting to note that the nature of both ingredients contained in it are colossally unknown for which theoretical and observational developments are underway. It is also called the Concordance model for being it in agreement with the recent measurements of parameters. Obviously the dynamics of $\mathsf{\Lambda }$CDM is governed by general relativity. $\mathsf{\Lambda }$ was introduced by Einstein himself [3] to balance the gravitational effect of the ordinary matter in order to show a static model of the universe. Therefore, the energy density of $\mathsf{\Lambda }$ is contained in the structure of spacetime itself or in other words it is vacuum energy of space. However, it was dropped by Einstein after the expansion was confirmed in 1929 calling it as the biggest blunder of his life ever made. After the accelerated expansion was discovered in 1998, $\mathsf{\Lambda }$ is coming back once again to accommodate the effect of accelerated expansion, but this time it is expected to have a dynamic nature. According to the recent observations [35,36], dark energy is ~$70\%$, dark matter constitutes $25\%$, and the ordinary matter (baryonic) is $5\%$ only. In the framework of the $\mathsf{\Lambda }$CDM model, the nature of dark energy presents one of the most challenging issues to the present day cosmology. In $\mathsf{\Lambda }$CDM, space is spatially flat and the radius of curvature, therefore becomes infinitely large. The $\mathsf{\Lambda }$CDM adapts a minimum number of parameters to describe the universe that is six. The recent measurements of these parameters from different sources are given [35,55] below in the

Table 1. Values of six parameters of $\mathsf{\Lambda }$CDM from different sources.

Sr. No	Parameters	$\mathit{\Lambda }$CDM
		Planck-2018	Planck-Lowℓ + SPT	Planck-Lowℓ + SPT + BOSS	Planck-Lowℓ + SPT + BOSS + SHOES
1	${\mathsf{\Omega }}_b{h}^2$	0.02233 ± 0.00015	0.02269 ± 0.00025	0.02250 ± 0.00021	0.02255 ± 0.00020
2	${\mathsf{\Omega }}_c{h}^2$	0.1198 ± 0.0012	0.1143 ± 0.0020	0.1166 ± 0.0012	0.1159 ± 0.0010
3	$n_s$	0.9652 ± 0.0042	0.9785 ± 0.0074	0.9716 ± 0.0056	0.9735 ± 0.0054
4	$ln\left({10}^{10}{A}_S\right)$	3.043 ± 0.014	3.021 ± 0.017	3.014 ± 0.017	3.008 ± 0.017
5	$\tau$	0.0540 ± 0.0074	0.0510 ± 0.0086	0.0456 ± 0.0082	0.0437 ± 0.0087
6	$100{\theta }_{MC}$	1.04089 ± 0.00031	-	-	-

.

From these six free parameters, we can deduce other parameters like Hubble constant with some assumption about the cosmological model. The detail can be found in [35]. In the context of $\mathsf{\Lambda }$CDM we can find how the energy densities is related with the parameters like scale factor $a_t$, time t, Hubble parameter, etc. in the corresponding sectors namely radiation, matter (cold matter), and dark energy. Using Equations (96) and (108), we find for the radiation sector for which $\rho =3p\Rightarrow w=\frac{1}{3}$

$${\rho }_r\left(a\right)={\rho }_{r_0}{a}^{-4}\left(t\right)=3M_{pl}^2{H}_0^2{\mathsf{\Omega }}_{r_0}{a}^{-4}\left(t\right)$$

(461)

$${\mathsf{\Omega }}_r\left(a\right)={\left(\frac{H_0}{H}\right)}^2{\mathsf{\Omega }}_{r_0}{a}^{-4}\left(t\right)$$

(462)

and

$$a\left(t\right)\propto t^{\frac{1}{2}}$$

(463)

for the matter sector incorporating baryonic and cold dark matter for which $\rho =3p\Rightarrow w=\frac{1}{3}$ we have

$${\rho }_m\left(a\right)={\rho }_{m_0}{a}^{-3}\left(t\right)=3M_{pl}^2{H}_0^2{\mathsf{\Omega }}_{m_0}{a}^{-3}\left(t\right)$$

(464)

$${\mathsf{\Omega }}_m\left(a\right)={\left(\frac{H_0}{H}\right)}^2{\mathsf{\Omega }}_{m_0}{a}^{-3}\left(t\right)$$

(465)

and

$$a\left(t\right)\propto t^{\frac{2}{3}}$$

(466)

for the dark energy sector for which ${\rho }_{\mathsf{\Lambda }}=-p\Rightarrow w_{\mathsf{\Lambda }}=-1$, we have

$${\rho }_{\mathsf{\Lambda }}=constant$$

(467)

and

$$a\left(t\right)\propto exp\sqrt{\frac{\mathsf{\Lambda }}{3}}t$$

(468)

Using Friedmann equation and total energy density of all forms, we can determine the background dynamics in the form of two very significant parameters

$$H^2\left(a\right)=H_0^2\left(\frac{{\mathsf{\Omega }}_{m0}}{a^3}+\frac{{\mathsf{\Omega }}_{r0}}{a^4}+\frac{{\mathsf{\Omega }}_{\mathsf{\Lambda }0}}{a^0}\right)$$

(469)

$$q\left(z\right)=\frac{1}{2}\left\{{\mathsf{\Omega }}_m\left(z\right)+2{\mathsf{\Omega }}_r\left(z\right)-{\mathsf{\Omega }}_{\mathsf{\Lambda }}\left(z\right)\right\}$$

(470)

The observations of Planck collaboration on cosmic microwave background radiation (CMB) give [35] the values of these parameters to be ${\mathsf{\Omega }}_{m0}=0.3089$, ${\mathsf{\Omega }}_{r0}=5.38916\times {10}^{-5}$ and ${\mathsf{\Omega }}_{\mathsf{\Lambda }0}=0.691046$ and for a flat $\mathsf{\Lambda }$CDM model, the observations from Type SNe Ia supernovae give [3] ${\mathsf{\Omega }}_{m0}=0.295$ and for other we can estimate from ${\mathsf{\Omega }}_{\mathsf{\Lambda }}=1-{\mathsf{\Omega }}_{DM}-{\mathsf{\Omega }}_b$. The Figure 20 gives the dark energy and cold matter densities [56] in terms of density parameters ${\mathsf{\Omega }}_{\mathsf{\Lambda }}$ and ${\mathsf{\Omega }}_m$.

11.7. Inflation and Dark Energy in $f\left(R\right)$ Modified Gravity

Einstein Field Equation (EFE) of general relativity is well known

$$G_{\mu \nu }=R_{\mu \nu }-\frac{1}{2}{g}_{\mu \nu }R=8\pi T_{\mu \nu }$$

(471)

Equation (471) corresponds to Einstein-Hilbert action

$$S_{EH}=\frac{1}{16\pi G}\int d^{4}x\sqrt{-g}R+\int d^{4}x\sqrt{-g}{\mathcal{L}}_m$$

(472)

In scalar field models we usually modify RHS, i.e., energy-momentum tensor (matter sector) and accordingly add some terms for a scalar field. If the RHS is kept unaltered and LHS is modified that stands for the geometry of spacetime mimicking the role of gravity. Due to this reason, it is called the model of modified gravity. The LHS of EFE is derived merely from the curvature term, i.e., Ricci Scalar R, however in the modified gravity we replace it by a general function of it [57,58,59,60]. Replacing the Ricci scalar R in the Einstein–Hilbert action given in Equation (471) by a general function of R, that is, $f\left(R\right)$, i.e., $R\to f\left(R\right)$, we have

$$S_{EH\left(f\left(R\right)\right)}=\frac{1}{16\pi G}\int d^{4}x\sqrt{-g}f\left(R\right)+\int d^{4}x\sqrt{-g}{\mathcal{L}}_M$$

(473)

The variation of Equation (473) would be

$$\delta S_{EH\left(f\left(R\right)\right)}=\int d^{4}x\delta \left(\sqrt{-g}f\left(R\right)\right)+\int d^{4}x\delta \left(L\right)$$

(474)

Equation (474) yields through tedious calculations the following modified gravity equation

$$F\left(R\right)R_{\mu \nu }-\frac{1}{2}f\left(R\right)g_{\mu \nu }-{\nabla }_{\mu }{\nabla }_{\nu }F\left(R\right)+g_{\mu \nu }\square F\left(R\right)=k{T}_{\mu \nu }$$

(475)

where $\square ={\nabla }_{\nu }{\nabla }^{\nu }$ and $F\left(R\right)=f_{,R}\left(R\right)$ In Equation (474), the LHS is the modified form of $R_{\mu \nu }-\frac{1}{2}{g}_{\mu \nu }R=G_{\mu \nu }$. We contract Equation (475) with $g^{\mu \nu }$ to find the trace of modified EFE

$$F\left(R\right)R+3\square F\left(R\right)-2f\left(R\right)=kT$$

(476)

For a vacuum solution $T=0$, the de Sitter space with curvature term R to be constant

$$F\left(R\right)R+3\square F\left(R\right)-2f\left(R\right)=0$$

(477)

Equation (477) represents an inflationary solution with the term $3\square F\left(R\right)=0$.

$$F\left(R\right)R-2f\left(R\right)=0$$

(478)

If the condition in Equation (478) is fulfilled, the late time de Sitter solution can be obtained in a $f\left(R\right)$-based dark energy model. The Friedmann Equations for the modified gravity can be determined in the following way by a spatially flat expanding FLRW universe

$$d{s}^2=-d{t}^2+a^2\left(t\right){\eta }_{\mu \nu }d{x}^{\mu }d{x}^{\nu }$$

(479)

now going through the lengthy calculations, we find

$$R_{00}=-3\left(\dot{H}+H^2\right)$$

(480)

$$R_{jj}=2{\dot{a}}^2+a\ddot{a}$$

(481)

and

$$R=6\left(\dot{H}+2H^2\right)$$

(482)

Equation (480) can be further re-expressed using Equation (482)

$$R_{00}=-\frac{1}{2}R+3H^2$$

(483)

for

$$T_{\mu \nu }=diag\left(-{\rho }_M,p_M,p_M,p_M\right)$$

(484)

where the trace of $T_{\mu \nu }$ is $\left(-{\rho }_M+3p_M\right)$. Now $\mu =\nu =0$ in Equation (475) gives

$$F\left(R\right)R_{00}-\frac{1}{2}f\left(R\right)g_{00}-{\nabla }_0{\nabla }_{0}F\left(R\right)+g_{00}\square F\left(R\right)=k{T}_{00}$$

(485)

solving through tedious calculations by making use of Equation (480) and Equation (484), we reach at the result

$$3H^{2}F=\frac{1}{2}\left(FR-f\right)-3H\dot{F}+k{\rho }_M$$

(486)

again for $\mu =\nu =j$ in Equation (475), we have

$$F\left(R\right)R_{jj}-\frac{1}{2}f\left(R\right)g_{jj}-{\nabla }_j{\nabla }_{j}F\left(R\right)+g_{jj}\square F\left(R\right)=k^2{T}_{jj}$$

(487)

we find

$$g_{jj}\square F\left(R\right)=-2a^{2}H\dot{F}-a^2\ddot{F}+{\nabla }_j{\nabla }_{j}F$$

(488)

Using Equations (481), (484) and (488) in Equation (487), we obtain

$$2\dot{H}F=-\ddot{F}+H\dot{F}-k\left({\rho }_M+p_M\right)$$

(489)

Equations (486) and (489) together determine the background dynamics of a flat FLRW universe governed by $f\left(R\right)$. From Equation (486), dividing by $3H^{2}F$ and for $\rho ={\rho }_R+{\rho }_M$, we can construct a dynamical system in the framework of $f\left(R\right)$, that is,

$$-\frac{\dot{F}}{HF}+\left(-\frac{f}{6H^{2}F}\right)+\frac{R}{6H^2}+\frac{k{\rho }_R}{3H^{2}F}+\frac{k{\rho }_M}{3H^{2}F}=1$$

(490)

here we can define the following parameters

$$x_1=-\frac{\dot{F}}{HF}$$

(491)

$$x_2=-\frac{f}{6H^{2}F}$$

(492)

$$x_3=\frac{R}{6H^2}$$

(493)

$$x_4={\mathsf{\Omega }}_R=\frac{k{\rho }_R}{3H^{2}F}$$

(494)

$$x_5={\mathsf{\Omega }}_M=\frac{k{\rho }_M}{3H^{2}F}$$

(495)

Equation (493) can be recast by using Equation (482)

$$x_3=\frac{\dot{H}}{H^2}+2=\frac{R}{6H^2}$$

(496)

we have

$$x_1+x_2+x_3+x_4+x_5=1$$

(497)

Now for $p_M=\frac{4}{3}{\rho }_R$, $N=lna$ and $\dot{H}=H^{\prime }H$, we can determine the following dynamical system:

$$\frac{d{x}_1}{dN}=x_1^2-x_1{x}_3-3x_2-x_3+x_4-1$$

(498)

$$\frac{d{x}_2}{dN}=x_1{x}_2-2x_2\left(x_3-2\right)+\frac{x_1}{m}$$

(499)

where

$$m=\frac{R{f}_{,RR}}{f_{,R}}$$

(500)

$$\frac{d{x}_3}{dN}=-2x_3\left(x_3-2\right)-\frac{x_1{x}_3}{m}$$

(501)

$$\frac{d{x}_4}{dN}=x_4\left(x_1-2x_3\right)$$

(502)

$$\frac{d{x}_5}{dN}=x_5\left(x_1-2x_3\right)$$

(503)

The effective equation of state can also be written form Equations (486) and (489) by division

$$w_{eff}=-1-\frac{2\dot{H}F}{3H^{2}F}$$

(504)

Or using Equation (496)

$$w_{eff}=-\frac{1}{3}\left(-1+2x_3\right)$$

(505)

Another form can also be written as

$$w_{eff}=-1+\frac{\ddot{F}-H\dot{F}+k\left(\rho +p\right)}{\frac{1}{2}\left(FR-f\right)-3H\dot{F}+k{\rho }_M}$$

(506)

Now we find $f\left(R\right)$ inflation by considering first a general form of $f\left(R\right)$ and determine its dynamics. Afterwards, Starobinsky inflation in $f\left(R\right)$ will be discussed. Let us consider

$$f\left(R\right)=R+b{R}^n$$

(507)

we have

$$F=f_{,R}\left(R\right)=1+nb{R}^{n-1}$$

(508)

and

$$\dot{F}=\frac{\partial F}{\partial t}=n\left(n-1\right)b{R}^{n-2}\frac{\partial R}{\partial t}$$

(509)

and

$$FR=R+nb{R}^n$$

(510)

substituting Equations (507)–(510) in Equation (486) where the $k{\rho }_M$ vanishes during inflationary phase, we have

$$3H^2\left(1+nb{R}^{n-1}\right)=\frac{1}{2}\left(nb-1\right)R^n-3n\left(n-1\right)Hb{R}^{n-2}\frac{\partial R}{\partial t}$$

(511)

The cosmological acceleration could be realized in the regime $F>>1\Rightarrow 1+nb{R}^{n-1}>>1$ and $nb{R}^{n-1}>>0$, which implies $1+nb{R}^{n-1}\approx nb{R}^{n-1}$. Dividing Equation (511) by $3nb{R}^{n-1}$, we obtain after simplification

$$H^2=\frac{1}{6}\left(\frac{n-1}{n}\right)\left(R-\frac{6Hn}{R}\frac{\partial R}{\partial t}\right)$$

(512)

also taking the time derivative of Equation (482)

$$\dot{R}=6\left(\ddot{H}+4H\dot{H}\right)$$

(513)

finding the ratio

$$\frac{1}{R}\frac{\partial R}{\partial t}=\frac{H\dot{H}}{H^2}\left(\frac{4+\frac{\ddot{H}}{H\dot{H}}}{2+\frac{\dot{H}}{H^2}}\right)$$

(514)

In Equation (514), the following approximations are validated during the inflation era

$$\frac{\dot{H}}{H^2}<<1\Rightarrow \frac{\dot{H}}{H^2}\to 0$$

(515)

and

$$\frac{\ddot{H}}{H\dot{H}}<<1\Rightarrow \frac{\ddot{H}}{H\dot{H}}\to 0$$

(516)

Equation (514) takes the form

$$\frac{1}{R}\frac{\partial R}{\partial t}=2\frac{\dot{H}}{H}$$

(517)

Using Equation (482) and Equation (517) in Equation (512), we get

$$H^2=\frac{n-1}{n}\left[H^2\left(2+\left(1-2n\right)\frac{\dot{H}}{H^2}\right)\right]$$

(518)

Or

$$\frac{\dot{H}}{H^2}=-\frac{2-n}{\left(n-1\right)\left(2n-1\right)}$$

(519)

Let

$$\frac{2-n}{\left(n-1\right)\left(2n-1\right)}=\epsilon$$

(520)

then Equation (519) becomes

$$\frac{\dot{H}}{H^2}=-\epsilon$$

(521)

Equation (519) gives the slow roll parameter. Integrating Equation (521), we get

$$H=\frac{1}{\epsilon t}$$

(522)

Equation (522) gives the expansion rate. We can determine the evolution of the scale factor $a\left(t\right)$

$$a\left(t\right)={\left(t\right)}^{\frac{1}{\epsilon }}$$

(523)

Equation (523) implies that the inflationary period is the one during which cosmic expansion is very fast in a very short span of time. Now to find out the condition on n, we use the condition $\epsilon <1$. From Equation (520) we have

$$\frac{2-n}{\left(n-1\right)\left(2n-1\right)}<1$$

(524)

which gives

$$n>\frac{1}{2}\left(1\pm \sqrt{3}\right)$$

(525)

We observe here that for $n=2$, the slow roll parameter $\epsilon =0$, which means the Hubble parameter H remains nearly constant in the limit $F>>1$. For $n>2$ it implies super-inflation with $\dot{H}>0$. It means that the viable condition to achieve a phase of standard inflation must be

$$\frac{1}{2}\left(1+\sqrt{3}\right)<n<2$$

(526)

For the Starobinsky $R^2$-inflation, we put $n=2$ and $b=\frac{1}{6M^2}$ in Equation (507)

$$f\left(R\right)=R+\frac{1}{6M^2}{R}^2$$

(527)

using Equations (482) and (513), after substituting for n and b in Equation (511), we get after simplification

$$\ddot{H}-\frac{1}{2}\frac{{\dot{H}}^2}{H}+\frac{1}{2}{M}^{2}H+3H\dot{H}=0$$

(528)

as the Hubble parameter remains almost constant during inflation or varies very slowly, therefore the first two terms $\ddot{H}$ and $-\frac{1}{2}\frac{{\dot{H}}^2}{H}$ can be neglected, and we get

$$\dot{H}=-\frac{1}{6}{M}^2$$

(529)

Integration of above Equation (529) between the limits $H_i$, $H_f$ and $t_i$, $t_f$ gives

$$H_f-H_i=-\frac{1}{6}{M}^2\left(t_f-t_i\right)$$

(530)

For $H_f=H={\partial }_{t}a$, we have on integrating again

$$a\left(t\right)=a_i\left(t\right)exp\left(H_i\left(t-t_i\right)-\frac{1}{12}{\left(t-t_i\right)}^2{M}^2\right)$$

(531)

Equation (482) becomes on making use of Equation (529)

$$R=-M^2+12H^2$$

(532)

The slow roll parameter on using Equation (529) takes the form

$$\epsilon =\frac{M^2}{6H^2}<1$$

(533)

for $\epsilon \le 1$, we have from Equation (533)

$$H^2\ge \frac{1}{6}{M}^2\Rightarrow 6H^2\ge M^2\Rightarrow H^2\ge M^2$$

(534)

for $t=t_f\Rightarrow \epsilon \sim 1$, from Equations (521) and (529) for $H=H_f$ we obtain

$$H_f=\frac{1}{\sqrt{6}}M$$

(535)

substituting Equation (535) in Equation (530) we have

$$t_f-t_i=\frac{6H_i}{M^2}$$

(536)

where the term $\frac{1}{6}{M}^2$ is ignored. In addition, Equation (532) reduces to $R\simeq -M^2$ when the inflationary phase approaches towards its ending. The number of e-folds for the inflationary phase turns out to be

$$N={\int }_{t_i}^{t_f}Hdt=\frac{1}{2\epsilon }$$

(537)

12. The Line Element of the Perturbed Universe

When the perturbations of inflaton field $\varphi$ are considered the energy momentum tensor $T_{\mu \nu }$ is also perturbed. The perturbations of the inflaton field are thus consequently reflected through the metric tensor $g_{\mu \nu }$ such that $\delta \varphi \iff \delta g_{\mu \nu }$. We discuss here only the scalar perturbations for which the metric takes the form as

$$d{s}^2=a^2\left(t\right)\left[\begin{array}{c}\left(-1-2A\right)d{\tau }^2+2{\partial }_{i}Bd\tau d{x}^i+\left(1-2\psi \right){\delta }_{ij}\hfill \\ +D_{ij}E)d{x}^{i}d{x}^j\hfill \end{array}\right]$$

(538)

$$\delta g_{\mu \nu }=a^2\left(t\right)\left(\begin{array}{cc}\delta g_{00}& \delta g_{0i}\\ \delta g_{i0}& \delta g_{ij}\end{array}\right)=a^2\left(t\right)\left(\begin{array}{cc}1-2A& {\partial }_{i}B\\ {\partial }_{i}B& \left(\left(1-2\psi \right){\delta }_{ij}+D_{ij}E\right)\end{array}\right)$$

(539)

12.1. Inverse of $\delta g_{\mu \nu }$

Let the inverse of $\delta g_{\mu \nu }$ be $\delta g^{\mu \nu }$ and we write

$$\begin{array}{c}\delta g^{\mu \nu }=\frac{1}{a^2\left(t\right)}\left(\begin{array}{cc}\delta g^{00}& \delta g^{0i}\\ \delta g^{i0}& \delta g^{ij}\end{array}\right)\hfill \\ =\left(\begin{array}{cc}\frac{1}{a^2\left(t\right)}\left(-1+X\right)& \frac{1}{a^2\left(t\right)}{\partial }^{i}Y\\ \frac{1}{a^2\left(t\right)}{\partial }^{i}Y& \frac{1}{a^2\left(t\right)}\left(\left(1+2Z\right){\delta }^{ij}+D^{ij}K\right)\end{array}\right)\hfill \end{array}$$

(540)

We find the inverse of Equation (454) that is $g^{\mu \nu }$, so that we have

$$\begin{array}{c}{g}^{\mu \zeta }{g}_{\zeta \nu }=g_0^{\mu \zeta }{g}_{\zeta \nu }^0={\delta }_{\nu }^{\mu }\hfill \\ \left(g_0^{\mu \zeta }+\delta g^{\mu \zeta }\right)\left(g_{\zeta \nu }^0+\delta g_{\zeta \nu }\right)={\delta }_{\nu }^{\mu }\hfill \\ g_0^{\mu \zeta }{g}_{\zeta \nu }^0+g_0^{\mu \zeta }\delta g_{\zeta \nu }+\delta g^{\mu \zeta }{g}_{\zeta \nu }^0+\delta g^{\mu \zeta }\delta g_{\zeta \nu }={\delta }_{\nu }^{\mu }\hfill \end{array}$$

(541)

where ${\delta }_{\nu }^{\mu }$ is the Kronecker delta function and is defined as

$${\delta }_{\nu }^{\mu }=\left\{\begin{array}{cc}1,\hfill & \mathrm{if}\mu =\nu \\ 0,\hfill & \mathrm{if}\mu \ne \nu \end{array}\right.$$

(542)

and $g_{\zeta \nu }$ is the simply unperturbed FLRW line element described as

$$d{s}^2=a^2\left(t\right)\left[-d{t}^2+{\delta }_{ij}d{x}^{i}d{x}^j\right]$$

(543)

$$g_{\mu \nu }^0=g_{\mu \nu }=a^2\left(t\right)\left(\begin{array}{cc}{g}_{00}& g_{0i}\\ g_{i0}& g_{ij}\end{array}\right)=a^2\left(t\right)\left(\begin{array}{cc}-1& 0\\ 0& {\delta }_{ij}\end{array}\right)$$

(544)

The inverse of $g_{\mu \nu }^0=g_{\mu \nu }$ is simply $g_0^{\mu \nu }=g^{\mu \nu }$ since for diagonal unperturbed metric $g_{\mu \nu }=\frac{1}{g_{\mu \nu }}$, so that we can write

$$g_0^{\mu \nu }=g^{\mu \nu }=\frac{1}{a^2\left(t\right)}\left(\begin{array}{cc}{g}^{00}& g^{0i}\\ g^{i0}& g^{ij}\end{array}\right)=\left(\begin{array}{cc}\frac{1}{a^2\left(t\right)}& 0\\ 0& \frac{1}{a^2\left(t\right)}{\delta }_{ij}\end{array}\right)$$

(545)

For $\mu =0$, $\nu =0$ in Equation (541), we have

$$\begin{array}{c}\left(g_0^{00}{g}_{00}^{\left(0\right)}+g_0^{0i}{g}_{i0}^{\left(0\right)}\right)+\left(g_0^{00}\delta g_{00}+g_0^{0i}\delta g_{i0}\right)+\left(\delta g^{00}{g}_{00}^{\left(0\right)}+\delta g^{0i}{g}_{i0}^{\left(0\right)}\right)\hfill \\ +\left(\delta g^{00}\delta g_{00}+\delta g^{0i}\delta g_{0i}\right)={\delta }_0^0\hfill \end{array}$$

(546)

which give

$$\delta g^{00}\delta g_{00}+\delta g^{0i}\delta g_{i0}=1$$

(547)

substituting the values, we have

$$-\frac{1}{a^2\left(t\right)}\left(-1+X\right)a^2\left(t\right)\left(-1-2A\right)+\frac{1}{a^2\left(t\right)}{\partial }^{i}Y\left(a^2\left(t\right){\partial }_{i}B\right)=1$$

(548)

Simplifying and neglecting second order product terms $-2AX$ and ${\partial }^{i}Y·{\partial }_{i}B$, we get

$$X=2A$$

(549)

Again from Equation (541) for $\mu =0$, $\nu =i$, we have after simplification

$$\begin{array}{c}\left(g_0^{00}{g}_{0i}^{\left(0\right)}+g_0^{0i}{g}_{ji}^{\left(0\right)}\right)+\left(g_0^{00}\delta g_{0i}+g_0^{0i}\delta g_{ji}\right)+\left(\delta g^{00}{g}_{0i}^{\left(0\right)}+\delta g^{0i}{g}_{ji}^{\left(0\right)}\right)\hfill \\ +\left(\delta g^{00}\delta g_{0i}+\delta g^{0i}\delta g_{ji}\right)={\delta }_i^0\hfill \end{array}$$

(550)

$$\delta g^{00}\delta g_{0i}+\delta g^{0j}\delta g_{ji}=0$$

(551)

Substituting the values

$$\frac{1}{a^2\left(t\right)}\left(-1+X\right)a^2\left(t\right){\partial }_{i}B+\frac{1}{a^2\left(t\right)}{\partial }^{j}Y\left(a^2\left(t\right)\left(1-2\psi \right){\delta }_{ji}+D_{ji}E\right)=0$$

(552)

neglecting the higher product terms $2A·{\partial }_{i}B$, ${\partial }_{i}Y·2\psi$ and ${\partial }^j{D}_{ji}Y·E$, we have

$$-{\partial }_{i}B+{\partial }_{i}Y=0$$

(553)

On integrating, we get

$$Y=B$$

(554)

Now from Equation (541) for $\mu =i$, $\nu =j$, we have

$$\begin{array}{c}\left(g_0^{i0}{g}_{0j}^{\left(0\right)}+g_0^{ij}{g}_{ij}^{\left(0\right)}\right)+\left(g_0^{i0}\delta g_{0j}^{\left(0\right)}+g_0^{ij}\delta g_{ij}^{\left(0\right)}\right)+\left(\delta g^{i0}{g}_{0j}^{\left(0\right)}+\delta g^{ij}{g}_{ij}^{\left(0\right)}\right)\hfill \\ +\left(\delta g^{i0}\delta g_{0j}+\delta g^{ij}\delta g_{ij}\right)={\delta }_j^i\hfill \end{array}$$

(555)

The non-vanishing terms are

$$\delta g^{i0}\delta g_{0j}+\delta g^{ik}\delta g_{kj}={\delta }_j^i$$

(556)

substituting values suitable change of indices

$$\begin{array}{c}\frac{1}{a^2\left(t\right)}\left({\partial }^{i}Y\right)a^2\left(t\right){\partial }_{i}B+\frac{1}{a^2\left(t\right)}\left(\left(1+2z\right){\delta }^{ik}+D^{ik}E\right)\hfill \\ ·a^2\left(t\right)\left(\left(1-2\psi \right){\delta }_{kj}+D_{kj}E\right)={\delta }_j^i\hfill \end{array}$$

(557)

using properties ${\delta }^{ik}{\delta }_{kj}={\delta }_j^i$, ${\delta }^{ik}{D}_{kj}=D_j^i$, ${\delta }_{kj}{D}^{ik}=D_k^i$ and neglecting the higher order product terms ${\partial }^{i}B·{\partial }_{j}B$, $-4Z\psi$, $2Z{D}_j^{i}E$, $-2\psi ·D_j^{i}K$ and $D_j^{i}KE$, we have

$$\left(1-2\psi +2Z\right){\delta }_j^i+\left(E+K\right)D_j^i={\delta }_j^i+0D_j^i$$

(558)

Comparing the coefficients of ${\delta }_j^i$ and $D_j^i$, we get $Z=\psi$ and $K=-E$, so that inverse metric of the perturbed line element becomes

$$\begin{array}{c}\delta g^{\mu \nu }=\frac{1}{a^2\left(t\right)}\left(\begin{array}{cc}\delta g^{00}& \delta g^{0i}\\ \delta g^{i0}& \delta g^{ij}\end{array}\right)\hfill \\ =\left(\begin{array}{cc}\frac{1}{a^2\left(t\right)}\left(-1+2A\right)& \frac{1}{a^2\left(t\right)}{\partial }^{i}B\\ \frac{1}{a^2\left(t\right)}{\partial }^{i}B& \frac{1}{a^2\left(t\right)}\left(\left(1+2\psi \right){\delta }^{ij}-D^{ij}E\right)\end{array}\right)\hfill \end{array}$$

(559)

12.2. The Unperturbed Line Element

The unperturbed line element is given by

$$d{s}^2=a^2\left(t\right)\left[-d{t}^2+{\delta }_{ij}d{x}^{i}d{x}^j\right]$$

(560)

or

$$g_{\mu \nu }^0=g_{\mu \nu }=a^2\left(t\right)\left(\begin{array}{cc}{g}_{00}& g_{0i}\\ g_{i0}& g_{ij}\end{array}\right)=a^2\left(t\right)\left(\begin{array}{cc}-1& 0\\ 0& {\delta }_{ij}\end{array}\right)$$

(561)

The inverse of $g_{\mu \nu }^0=g_{\mu \nu }$ is simply $g_0^{\mu \nu }=g^{\mu \nu }$ as for diagonal unperturbed metric $g_{\mu \nu }=\frac{1}{g_{\mu \nu }}$, so that we can write

$$g_0^{\mu \nu }=g^{\mu \nu }=\frac{1}{a^2\left(t\right)}\left(\begin{array}{cc}{g}^{00}& g^{0i}\\ g^{i0}& g^{ij}\end{array}\right)=\left(\begin{array}{cc}\frac{1}{a^2\left(t\right)}& 0\\ 0& \frac{1}{a^2\left(t\right)}{\delta }_{ij}\end{array}\right)$$

(562)

We calculate now affine connections-the 2nd kind of Christoffel symbols

$${\mathsf{\Gamma }}_{\mu \nu }^{\sigma }=g^{\sigma \lambda }{\mathsf{\Gamma }}_{\mu \nu \lambda }=\frac{1}{2}{g}^{\sigma \lambda }\left({g_{\mu \lambda }}_{,\nu }+{g_{\nu \lambda }}_{,\mu }+{g_{\mu \nu }}_{,\lambda }\right)$$

(563)

We can compute the following possible components

$$\begin{array}{c}{\mathsf{\Gamma }}_{00}^0{\mathsf{\Gamma }}_{ii}^i{\mathsf{\Gamma }}_{jj}^j{\mathsf{\Gamma }}_{00}^i\hfill \\ {\mathsf{\Gamma }}_{00}^j{\mathsf{\Gamma }}_{0j}^i{\mathsf{\Gamma }}_{i0}^j{\mathsf{\Gamma }}_{ij}^0\hfill \\ {\mathsf{\Gamma }}_{0i}^0{\mathsf{\Gamma }}_{0j}^0{\mathsf{\Gamma }}_{jk}^i{\mathsf{\Gamma }}_{ik}^j\hfill \\ {\mathsf{\Gamma }}_{ij}^k\hfill \end{array}$$

(564)

For $\sigma =\mu =\nu =0$, we have

$${\mathsf{\Gamma }}_{00}^0=-\frac{1}{2a^2\left(t\right)}{\partial }_{,0}{g}_{00}=\frac{\dot{a}}{a}$$

(565)

For $\sigma =i$, $\mu =0$, $\nu =j$, we have

$${\mathsf{\Gamma }}_{0j}^i=\frac{1}{2a^2\left(t\right)}{\delta }^{ik}{\partial }_{,0}\left(a^2\left(t\right){\delta }_{jk}\right)=\frac{\dot{a}}{a}{\delta }_j^i$$

(566)

For $\sigma =0$, $\mu =i$, $\nu =j$, we have

$${\mathsf{\Gamma }}_{ij}^0=\frac{1}{2a^2\left(t\right)}{\partial }_{,0}\left(a^2\left(t\right){\delta }_{ij}\right)=\frac{\dot{a}}{a}{\delta }_{ij}$$

(567)

For $\sigma =i$, $\mu =0$, $\nu =0$, we have

$${\mathsf{\Gamma }}_{00}^i=0$$

(568)

and

For $\sigma =0$, $\mu =0$, $\nu =i$, we have

$${\mathsf{\Gamma }}_{0i}^0=0$$

(569)

and for $\sigma =i$, $\mu =j$, $\nu =k$, we have

$${\mathsf{\Gamma }}_{jk}^i=0$$

(570)

Now, we can calculate perturbed element when we have necessary components of perturbed and unperturbed metric and its inverse metric tensor components,

$$\begin{array}{c}\delta {\mathsf{\Gamma }}_{\mu \nu }^{\sigma }=\delta \left(g^{\sigma \lambda }{\mathsf{\Gamma }}_{\mu \nu \lambda }\right)=\frac{1}{2}\delta \left(g^{\sigma \lambda }\left({g_{\mu \lambda }}_{,\nu }+{g_{\nu \lambda }}_{,\mu }+{g_{\mu \nu }}_{,\lambda }\right)\right)\hfill \\ =\frac{1}{2}\delta g^{\sigma \lambda }\left({g_{\mu \lambda }}_{,\nu }+{g_{\nu \lambda }}_{,\mu }-{g_{\mu \nu }}_{,\lambda }\right)\hfill \\ +\frac{1}{2}{g}^{\sigma \lambda }\left({\left(\delta g_{\mu \lambda }\right)}_{,\nu }+{\left(\delta g_{\nu \lambda }\right)}_{,\mu }+{\left(\delta g_{\mu \nu }\right)}_{,\lambda }\right)\hfill \end{array}$$

(571)

We are on our stake now to calculate the following components:

$$\begin{array}{c}\delta {\mathsf{\Gamma }}_{00}^0\delta {\mathsf{\Gamma }}_{ii}^i\delta {\mathsf{\Gamma }}_{jj}^j\hfill \\ \delta {\mathsf{\Gamma }}_{00}^i\delta {\mathsf{\Gamma }}_{00}^j\delta {\mathsf{\Gamma }}_{0j}^i\hfill \\ \delta {\mathsf{\Gamma }}_{i0}^j\delta {\mathsf{\Gamma }}_{ij}^0\delta {\mathsf{\Gamma }}_{0i}^0\hfill \\ \delta {\mathsf{\Gamma }}_{0j}^0\delta {\mathsf{\Gamma }}_{jk}^i\delta {\mathsf{\Gamma }}_{ik}^j\hfill \\ \delta {\mathsf{\Gamma }}_{ij}^k\hfill \end{array}$$

(572)

The non-vanishing components are For $\sigma =\mu =\nu =0$, we have

$$\delta {\mathsf{\Gamma }}_{00}^0=\dot{A}$$

(573)

For $\sigma =i$, $\mu =0$, $\nu =j$, we have

$$\delta {\mathsf{\Gamma }}_{0j}^i=-\dot{\psi }{\delta }_j^i+\frac{1}{2}{D}_{ij}\dot{E}$$

(574)

For $\sigma =0$, $\mu =i$, $\nu =j$, we have

$$\delta {\mathsf{\Gamma }}_{ij}^0=-2\frac{\dot{a}}{a}A{\delta }_{ij}-{\partial }_i{\partial }_{j}B-2\frac{\dot{a}}{a}\psi {\delta }_{ij}-{\psi }^{\prime }{\delta }_{ij}-\frac{\dot{a}}{a}{D}_{ij}E+\frac{1}{2}{D}_{ij}\dot{E}$$

(575)

For $\sigma =i$, $\mu =0$, $\nu =0$, we have

$$\delta {\mathsf{\Gamma }}_{00}^i=\frac{\dot{a}}{a}{\partial }^{i}B+{\partial }^i\dot{B}+{\partial }^{i}A$$

(576)

and For $\sigma =0$, $\mu =0$, $\nu =i$, we have

$$\delta {\mathsf{\Gamma }}_{0i}^0={\partial }_{i}A+\frac{\dot{a}}{a}{\partial }_{i}B$$

(577)

and for $\sigma =i$, $\mu =j$, $\nu =k$, we have

$$\begin{array}{c}\delta {\mathsf{\Gamma }}_{jk}^i=-{\partial }_j\psi {\delta }_k^i-{\partial }_k\psi {\delta }_j^i+{\partial }^i\psi {\delta }_{jk}-\frac{\dot{a}}{a}{\partial }^{i}B{\delta }_{jk}+\frac{1}{2}{\partial }_j{D}_k^{i}E\hfill \\ +\frac{1}{2}{\partial }_k{D}_j^{i}E-+\frac{1}{2}{\partial }^i{D}_{jk}E\hfill \end{array}$$

(578)

Now the unperturbed Ricci tensor is given

$$R_{\mu \nu }=g_{\lambda \sigma }{R}_{\mu \nu \lambda }^{\sigma }={\partial }_{,\nu }{\mathsf{\Gamma }}_{\mu \lambda }^{\sigma }-{\partial }_{,\lambda }{\mathsf{\Gamma }}_{\mu \nu }^{\sigma }+{\mathsf{\Gamma }}_{\mu \lambda }^n{\mathsf{\Gamma }}_{n\nu }^{\sigma }-{\mathsf{\Gamma }}_{\mu \nu }^n{\mathsf{\Gamma }}_{n\lambda }^{\sigma }$$

(579)

For $\mu =0$, $\nu =0$, we have

$$R_{00}={\partial }_{,\sigma }{\mathsf{\Gamma }}_{00}^{\sigma }-{\partial }_{,0}{\mathsf{\Gamma }}_{0\sigma }^{\sigma }+{\mathsf{\Gamma }}_{\mu \lambda }^n{\mathsf{\Gamma }}_{n\nu }^{\sigma }-{\mathsf{\Gamma }}_{\mu \nu }^n{\mathsf{\Gamma }}_{n\lambda }^{\sigma }=0$$

(580)

For $\mu =0$, $\nu =i$, we have

$$R_{0i}={\partial }_{,\sigma }{\mathsf{\Gamma }}_{00}^{\sigma }-{\partial }_{,0}{\mathsf{\Gamma }}_{0\sigma }^{\sigma }+{\mathsf{\Gamma }}_{\mu \lambda }^n{\mathsf{\Gamma }}_{n\nu }^{\sigma }-{\mathsf{\Gamma }}_{\mu \nu }^n{\mathsf{\Gamma }}_{n\lambda }^{\sigma }=0$$

(581)

For $\mu =i$, $\nu =j$, we have

$$R_{ij}={\partial }_{,\sigma }{\mathsf{\Gamma }}_{00}^{\sigma }-{\partial }_{,0}{\mathsf{\Gamma }}_{0\sigma }^{\sigma }+{\mathsf{\Gamma }}_{\mu \lambda }^n{\mathsf{\Gamma }}_{n\nu }^{\sigma }-{\mathsf{\Gamma }}_{\mu \nu }^n{\mathsf{\Gamma }}_{n\lambda }^{\sigma }=0$$

(582)

Now. we calculate the perturbed Ricci tensor components:

$$\begin{array}{c}\delta R_{\mu \nu }={\partial }_{,\nu }\delta {\mathsf{\Gamma }}_{\mu \lambda }^{\sigma }-{\partial }_{,\lambda }\delta {\mathsf{\Gamma }}_{\mu \nu }^{\sigma }+\delta {\mathsf{\Gamma }}_{\mu \lambda }^n{\mathsf{\Gamma }}_{n\nu }^{\sigma }+{\mathsf{\Gamma }}_{\mu \lambda }^n\delta {\mathsf{\Gamma }}_{n\nu }^{\sigma }-\delta {\mathsf{\Gamma }}_{\mu \nu }^n{\mathsf{\Gamma }}_{n\lambda }^{\sigma }\hfill \\ -{\mathsf{\Gamma }}_{\mu \nu }^n\delta {\mathsf{\Gamma }}_{n\lambda }^{\sigma }\hfill \end{array}$$

(583)

For $\mu =0$, $\nu =0$, we have

$$\begin{array}{c}\delta R_{00}={\partial }_{,\nu }\delta {\mathsf{\Gamma }}_{\mu \lambda }^{\sigma }-{\partial }_{,\lambda }\delta {\mathsf{\Gamma }}_{\mu \nu }^{\sigma }+\delta {\mathsf{\Gamma }}_{\mu \lambda }^n{\mathsf{\Gamma }}_{n\nu }^{\sigma }+{\mathsf{\Gamma }}_{\mu \lambda }^n\delta {\mathsf{\Gamma }}_{n\nu }^{\sigma }-\delta {\mathsf{\Gamma }}_{\mu \nu }^n{\mathsf{\Gamma }}_{n\lambda }^{\sigma }\hfill \\ -{\mathsf{\Gamma }}_{\mu \nu }^n\delta {\mathsf{\Gamma }}_{n\lambda }^{\sigma }=\frac{\dot{a}}{a}{\partial }_i{\partial }^{i}B+{\partial }_i{\partial }^i{B}^{\prime }+{\partial }_i{\partial }^{i}A+3{\psi }^{″}+3\frac{\dot{a}}{a}{\psi }^{\prime }+3\frac{\dot{a}}{a}{A}^{\prime }\hfill \end{array}$$

(584)

For $\mu =0$, $\nu =i$, we have

$$\begin{array}{c}\delta R_{0i}={\partial }_{,\nu }\delta {\mathsf{\Gamma }}_{\mu \lambda }^{\sigma }-{\partial }_{,\lambda }\delta {\mathsf{\Gamma }}_{\mu \nu }^{\sigma }+\delta {\mathsf{\Gamma }}_{\mu \lambda }^n{\mathsf{\Gamma }}_{n\nu }^{\sigma }+{\mathsf{\Gamma }}_{\mu \lambda }^n\delta {\mathsf{\Gamma }}_{n\nu }^{\sigma }-\delta {\mathsf{\Gamma }}_{\mu \nu }^n{\mathsf{\Gamma }}_{n\lambda }^{\sigma }\hfill \\ -{\mathsf{\Gamma }}_{\mu \nu }^n\delta {\mathsf{\Gamma }}_{n\lambda }^{\sigma }=\frac{\ddot{a}}{a}{\partial }_{i}B+{\left(\frac{\dot{a}}{a}\right)}^2{\partial }_{i}B+2{\partial }_i{\psi }^{\prime }+2\frac{\dot{a}}{a}{\partial }_{i}A+\frac{1}{2}{\partial }_k{D}_i^k{E}^{\prime }\hfill \end{array}$$

(585)

For $\mu =i$, $\nu =j$, we have

$$\begin{array}{c}\delta R_{ij}={\partial }_{,\nu }\delta {\mathsf{\Gamma }}_{\mu \lambda }^{\sigma }-{\partial }_{,\lambda }\delta {\mathsf{\Gamma }}_{\mu \nu }^{\sigma }+\delta {\mathsf{\Gamma }}_{\mu \lambda }^n{\mathsf{\Gamma }}_{n\nu }^{\sigma }+{\mathsf{\Gamma }}_{\mu \lambda }^n\delta {\mathsf{\Gamma }}_{n\nu }^{\sigma }-\delta {\mathsf{\Gamma }}_{\mu \nu }^n{\mathsf{\Gamma }}_{n\lambda }^{\sigma }-{\mathsf{\Gamma }}_{\mu \nu }^n\delta {\mathsf{\Gamma }}_{n\lambda }^{\sigma }\hfill \\ =\left(\begin{array}{c}-\frac{\dot{a}}{a}\dot{A}-5\frac{\dot{a}}{a}\dot{\psi }-2\frac{\ddot{a}}{a}A-2{\left(\frac{\dot{a}}{a}\right)}^{2}A-2\frac{\ddot{a}}{a}\psi -2{\left(\frac{\dot{a}}{a}\right)}^2\psi -\ddot{\psi }\hfill \\ +{\partial }_k{\partial }^k\psi -\frac{\dot{a}}{a}{\partial }_k{\partial }^{k}B\hfill \end{array}\right){\delta }_{ij}\hfill \\ -{\partial }_i{\partial }_j\dot{B}+\frac{\dot{a}}{a}{D}_{ij}\dot{E}+\frac{\ddot{a}}{a}{D}_{ij}E+{\left(\frac{\dot{a}}{a}\right)}^2{D}_{ij}E+\frac{1}{2}{D}_{ij}\ddot{E}+{\partial }_i{\partial }_j\psi -{\partial }_i{\partial }_{j}A\hfill \\ -2\frac{\dot{a}}{a}{\partial }_i{\partial }_{j}B+\frac{1}{2}{\partial }_k{\partial }_i{D}_j^{k}E+\frac{1}{2}{\partial }_k{\partial }_j{D}_i^{k}E-\frac{1}{2}{\partial }_k{\partial }^k{D}_{ij}E\hfill \end{array}$$

(586)

Unperturbed Ricci scalar is obtained by contracting the unperturbed Ricci tensor

$$R=g^{\mu \nu }{R}_{\mu \nu }$$

(587)

Using double sum and simplifying, we have

$$R=g^{00}{R}_{00}+g^{11}{R}_{11}+g^{22}{R}_{22}+g^{33}{R}_{33}=6\frac{\ddot{a}}{a^3}$$

(588)

and perturbed Ricci scalar, using double sum and simplifying, we have

$$\begin{array}{c}\delta R=\delta g^{\mu \nu }{R}_{\mu \nu }+g^{\mu \nu }\delta R_{\mu \nu }=\delta g^{00}{R}_{00}+\delta g^{11}{R}_{11}+\delta g^{22}{R}_{22}\hfill \\ +\delta g^{33}{R}_{33}+g^{00}\delta R_{00}+g^{11}\delta R_{11}+g^{22}\delta R_{22}+g^{33}\delta R_{33}\hfill \\ =-6\frac{\dot{a}}{a^3}{\partial }_i{\partial }^{i}B-\frac{2}{a^2}{\partial }_i{\partial }^{i}B-\frac{2}{a^2}{\partial }_i{\partial }^{i}A-\frac{6}{a^2}\ddot{\psi }-6\frac{\dot{a}}{a^3}\dot{A}-18\frac{\dot{a}}{a^3}\dot{\psi }\hfill \\ -12\frac{\ddot{a}}{a^3}A+\frac{4}{a^2}{\partial }_i{\partial }^i\psi +\frac{1}{a^2}{\partial }_k{\partial }^i{D}_i^{k}E\hfill \end{array}$$

(589)

now unperturbed Einstein tensor is

$$G_{\mu \nu }=R_{\mu \nu }+\frac{1}{2}{g}_{\mu \nu }R$$

(590)

For $\mu =0$, $\nu =0$, we have

$$G_{00}=R_{00}+\frac{1}{2}{g}_{00}R=3{\left(\frac{\dot{a}}{a}\right)}^2$$

(591)

For $\mu =0$, $\nu =i$, we have

$$G_{0i}=R_{0i}+\frac{1}{2}{g}_{0i}R=0$$

(592)

For $\mu =i$, $\nu =j$, we have

$$G_{ij}=R_{ij}+\frac{1}{2}{g}_{ij}R=\left(-2\frac{\ddot{a}}{a}+{\left(\frac{\dot{a}}{a}\right)}^2\right){\delta }_{ij}$$

(593)

Now, the perturbed Einstein tensor at first order of perturbation is

$$\delta G_{\mu \nu }=\delta R_{\mu \nu }-\frac{1}{2}\left(\delta g_{\mu \nu }R+g_{\mu \nu }\delta R\right)$$

(594)

For $\mu =0$, $\nu =0$, we have

$$\begin{array}{c}\delta G_{00}=\delta R_{00}-\frac{1}{2}\left(\delta g_{00}R+g_{00}\delta R\right)=-2\frac{\dot{a}}{a}{\partial }_i{\partial }^{i}B-6\frac{\dot{a}}{a}\dot{\psi }\hfill \\ +2{\partial }_i{\partial }^i\psi +\frac{1}{2}{\partial }_k{\partial }^i{D}_i^{k}E\hfill \end{array}$$

(595)

For $\mu =0$, $\nu =i$, we have

$$\begin{array}{c}\delta G_{0i}=\delta R_{0i}-\frac{1}{2}\left(\delta g_{0i}R+g_{0i}\delta R\right)=-2\frac{\ddot{a}}{a}{\partial }_{i}B+{\left(\frac{\dot{a}}{a}\right)}^2{\partial }_{i}B\hfill \\ +2{\partial }_i\dot{\psi }+\frac{1}{2}{\partial }_k{D}_i^k\dot{E}\hfill \\ +2\frac{\dot{a}}{a}{\partial }_{i}A\hfill \end{array}$$

(596)

For $\mu =i$, $\nu =j$, we have

$$\begin{array}{c}\delta G_{ij}=\delta R_{ij}-\frac{1}{2}\left(\delta g_{ij}R+g_{ij}\delta R\right)\hfill \\ =\left(\begin{array}{c}2\frac{\dot{a}}{a}\dot{A}+4\frac{\dot{a}}{a}\dot{\psi }+4\frac{\ddot{a}}{a}A-2{\left(\frac{\dot{a}}{a}\right)}^{2}A+4\frac{\ddot{a}}{a}\psi -2{\left(\frac{\dot{a}}{a}\right)}^2\psi +2\ddot{\psi }\hfill \\ -{\partial }_k{\partial }^k\psi +2\frac{\dot{a}}{a}{\partial }_k{\partial }^{k}B+{\partial }_k{\partial }^k\dot{B}+{\partial }_k{\partial }^{k}A+\frac{1}{2}{\partial }_k{\partial }^{\rho }{D}_{\rho }^{k}E\hfill \end{array}\right){\delta }_{ij}\hfill \\ -{\partial }_i{\partial }_j\dot{B}+\frac{\dot{a}}{a}{D}_{ij}\dot{E}-2\frac{\ddot{a}}{a}{D}_{ij}E-{\partial }_i{\partial }_{j}A+{\partial }_i{\partial }_j\psi +{\left(\frac{\dot{a}}{a}\right)}^2{D}_{ij}E\hfill \\ +\frac{1}{2}{D}_{ij}\ddot{E}-2\frac{\dot{a}}{a}{\partial }_i{\partial }_{j}B+\frac{1}{2}{\partial }_k{\partial }_i{D}_j^{k}E+\frac{1}{2}{\partial }^k{\partial }_j{D}_{ik}E-\frac{1}{2}{\partial }_k{\partial }^k{D}_{ij}E\hfill \end{array}$$

(597)

Now, the unperturbed stress energy tensor is

$$T_{\mu \nu }={\partial }_{\mu }\varphi {\partial }_{\nu }\varphi -g_{\mu \nu }\left(\frac{1}{2}{g}^{\sigma \rho }{\partial }_{\sigma }\varphi {\partial }_{\rho }\varphi -V\left(\varphi \right)\right)$$

(598)

For $\mu =0$, $\nu =0$, we have

$$T_{00}={\partial }_0\varphi {\partial }_0\varphi -g_{00}\left(\frac{1}{2}{g}^{\sigma \rho }{\partial }_{\sigma }\varphi {\partial }_{\rho }\varphi -V\left(\varphi \right)\right)=\frac{1}{2}{\dot{\varphi }}^2+a^2\left(t\right)V\left(\varphi \right)$$

(599)

For $\mu =0$, $\nu =i$, we have

$$T_{0i}={\partial }_0\varphi {\partial }_i\varphi -g_{0i}\left(\frac{1}{2}{g}^{\sigma \rho }{\partial }_{\sigma }\varphi {\partial }_{\rho }\varphi -V\left(\varphi \right)\right)=0$$

(600)

For $\mu =i$, $\nu =j$, we have

$$T_{ij}={\partial }_i\varphi {\partial }_j\varphi -g_{ij}\left(\frac{1}{2}{g}^{\sigma \rho }{\partial }_{\sigma }\varphi {\partial }_{\rho }\varphi -V\left(\varphi \right)\right)=\left(\frac{1}{2}{\dot{\varphi }}^2-a^2\left(t\right)V\left(\varphi \right)\right){\delta }_{ij}$$

(601)

Now, the perturbed stress energy tensor at first order of perturbation is

$$\delta T_{\mu \nu }=\begin{array}{c}{\partial }_{\mu }\left(\delta \varphi \right){\partial }_{\nu }\varphi +{\partial }_{\mu }\varphi {\partial }_{\nu }\left(\delta \varphi \right)-\delta g_{\mu \nu }\left(\frac{1}{2}{g}^{\sigma \rho }{\partial }_{\sigma }\varphi {\partial }_{\rho }\varphi +V\left(\varphi \right)\right)\hfill \\ -g_{\mu \nu }\left(\begin{array}{c}\frac{1}{2}\delta g^{\sigma \rho }{\partial }_{\sigma }\varphi {\partial }_{\rho }\varphi +g^{\sigma \rho }{\partial }_{\sigma }\left(\delta \varphi \right){\partial }_{\rho }\varphi +g^{\sigma \rho }{\partial }_{\sigma }\varphi {\partial }_{\rho }\left(\delta \varphi \right)\hfill \\ +{\partial }_{\varphi }\delta V\left(\varphi \right)+{\partial }_{\varphi }V\left(\varphi \right)\delta \varphi \hfill \end{array}\right)\hfill \end{array}$$

(602)

For $\mu =0$, $\nu =0$, we have

$$\delta T_{00}=\dot{\varphi }\delta \dot{\varphi }+2a^2\left(t\right)AV\left(\varphi \right)+\delta \varphi a^2\left(t\right)V_{\varphi }\left(\varphi \right)$$

(603)

For $\mu =0$, $\nu =i$, we have

$$\delta T_{0i}=\dot{\varphi }{\partial }_i\left(\delta \varphi \right)+\frac{{\dot{\varphi }}^2}{2}{\partial }_{i}B-a^2\left(t\right)V\left(\varphi \right){\partial }_{i}B$$

(604)

For $\mu =i$, $\nu =j$, we have

$$\begin{array}{c}\delta T_{ij}=\left(\dot{\varphi }\delta \dot{\varphi }-{\dot{\varphi }}^{2}A-a^2\left(t\right)V_{\varphi }\left(\varphi \right)\delta \varphi -{\dot{\varphi }}^2\psi +2a^2\left(t\right)V\left(\varphi \right)\psi \right){\delta }_{ij}\hfill \\ -a^2\left(t\right)V\left(\varphi \right)D_{ij}E+\frac{1}{2}{\dot{\varphi }}^2{D}_{ij}E\hfill \end{array}$$

(605)

Therefore, the perturbed Einstein field equations are

$$\delta G_{\mu \nu }=8\pi \delta T_{\mu \nu }$$

(606)

comparing the corresponding components, we have

$$\begin{array}{c}-2\frac{\dot{a}}{a}{\partial }_i{\partial }^{i}B-6\frac{\dot{a}}{a}\dot{\psi }+2{\partial }_i{\partial }^i\psi +\frac{1}{2}{\partial }_k{\partial }^i{D}_i^{k}E\hfill \\ =8\pi \dot{\varphi }\delta \dot{\varphi }+2a^2\left(t\right)AV\left(\varphi \right)+\delta \varphi a^2\left(t\right)V_{\varphi }\left(\varphi \right)\hfill \end{array}$$

(607)

$$\begin{array}{c}-2\frac{\ddot{a}}{a}{\partial }_{i}B+{\left(\frac{\dot{a}}{a}\right)}^2{\partial }_{i}B+2{\partial }_i\dot{\psi }+\frac{1}{2}{\partial }_k{D}_i^k\dot{E}+2\frac{\dot{a}}{a}{\partial }_{i}A\hfill \\ =8\pi \left(\dot{\varphi }{\partial }_i\left(\delta \varphi \right)+\frac{{\dot{\varphi }}^2}{2}{\partial }_{i}B-a^2\left(t\right)V\left(\varphi \right){\partial }_{i}B\right)\hfill \end{array}$$

(608)

$$\begin{array}{c}\left(\begin{array}{c}2\frac{\dot{a}}{a}\dot{A}+4\frac{\dot{a}}{a}\dot{\psi }+4\frac{\ddot{a}}{a}A-2{\left(\frac{\dot{a}}{a}\right)}^{2}A+4\frac{\ddot{a}}{a}\psi -2{\left(\frac{\dot{a}}{a}\right)}^2\psi +2\ddot{\psi }\hfill \\ -{\partial }_k{\partial }^k\psi +2\frac{\dot{a}}{a}{\partial }_k{\partial }^{k}B+{\partial }_k{\partial }^k\dot{B}+{\partial }_k{\partial }^{k}A+\frac{1}{2}{\partial }_k{\partial }^{\rho }{D}_{\rho }^{k}E\hfill \end{array}\right){\delta }_{ij}\hfill \\ \begin{array}{c}-{\partial }_i{\partial }_j\dot{B}+\frac{\dot{a}}{a}{D}_{ij}\dot{E}-2\frac{\ddot{a}}{a}{D}_{ij}E-{\partial }_i{\partial }_{j}A+{\partial }_i{\partial }_j\psi +{\left(\frac{\dot{a}}{a}\right)}^2{D}_{ij}E\hfill \\ +\frac{1}{2}{D}_{ij}\ddot{E}-2\frac{\dot{a}}{a}{\partial }_i{\partial }_{j}B+\frac{1}{2}{\partial }_k{\partial }_i{D}_j^{k}E+\frac{1}{2}{\partial }^k{\partial }_j{D}_{ik}E-\frac{1}{2}{\partial }_k{\partial }^k{D}_{ij}E\hfill \end{array}\hfill \\ \begin{array}{c}=8\pi \left(\begin{array}{c}\left(\dot{\varphi }\delta \dot{\varphi }-{\dot{\varphi }}^{2}A-a^2\left(t\right)V_{\varphi }\left(\varphi \right)\delta \varphi -{\dot{\varphi }}^2\psi +2a^2\left(t\right)V\left(\varphi \right)\psi \right){\delta }_{ij}\hfill \\ -a^2\left(t\right)V\left(\varphi \right)D_{ij}E\hfill \end{array}\right)\hfill \\ +\frac{1}{2}{\dot{\varphi }}^2{D}_{ij}E\hfill \end{array}\hfill \end{array}$$

(609)

perturbed equations can also be determined in mixed tensor form. Expressing Equation (606) in mixed form and working out on the same lines as we did earlier

$$\delta G_{\mu }^{\nu }=8\pi \delta T_{\mu }^{\nu }$$

(610)

13. Summary

Relativistic cosmology was founded on the general theory of relativity with the introduction of the cosmological principle and Weyl’s principle implicitly implied. In the beginning, Einstein’s and de Sitter’s cosmological models were presented, though now of historical interest, yet they both are very significant as the first initiates the modern cosmology relativistically and scientifically and the latter, later on, was used to provide the initial conditions of the big bang model with a slight change. The first theoretical models for the possibility of dynamic universe evolved beginning with Friedmann, Lemaitre and were observationally determined by E. Hubble. In 1929, E. Hubble found exactly the same expanding universe that Friedmann did theoretically in 1922. Therefore, it was Friedmann who championed the cause of dynamical universes; however, his work was recognized later when he was no more in the world. The theory of big bang based on the standard cosmological model faces Horizon, Flatness, Entropy problems etc. To resolve these problems a phase of exponentially expanding universe was introduced in its very early history which occurred in a very small fraction of time (about $\frac{1}{{10}^{43}}$ s of the very 1st second after time creation) known as inflation. The inflation is identified as the initial conditions under which the big bang might have taken place. The introduction of inflation caused the name inflationary cosmology and it is about forty years since its birth to date. The inflationary paradigm stands now on firm observational footing and is accepted irrevocably in cosmology as the viable description for the early universe. Starobinsky, Guth, and Linde are credited with setting the foundations of inflationary cosmology. The inflationary cosmology is being hailed as successful in explaining the origin of structure formation through cosmological quantum fluctuations as relicts of cosmic inflation. The observations conducted on microwave background radian and the recent discoveries of gravitational waves and black holes lend the confirmatory support to the underlying principles of the inflationary cosmology. Dark energy is the one of most challenging issues of the standard cosmology both on theoretical and observational grounds. In the framework of $\mathsf{\Lambda }$CDM it has equation of state (EoS) $w=-1$, however $\delta$ is facing fine-tuning problem. An alternative remedy to tackle the problems of $\delta$ are the model consisting of canonical and non-canonical scalar fields. The scalar field models modify matter sector of EFE on the right hand side, nonetheless in $f\left(R\right)$ geometry is modified as curvature of spacetime. $\mathsf{\Lambda }$CDM model is accepted for its being in good agreement with the recent observations. Note that there exists well-elaborated scenario to unify inflation with Dark Energy in modified gravity which was first proposed in S. Nojiri and S.D. Odintsov [61].