Many Aspects of Magnetic Fields in Neutron Stars
Abstract
:1. Introduction
2. Microscopic Aspects
3. Macroscopic Aspects
3.1. Formal Aspects of the Magnetic Field on the Structure of Neutron Stars
3.2. Global Structural Properties
4. Evolution Aspects
4.1. Magnetic-Thermal Evolution
4.2. Complex Magnetic Field Evolution
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Duncan, R.C.; Thompson, C. Formation of very strongly magnetized neutron stars—Implications for gamma-ray bursts. Astrophys. J. 1992, 392, L9–L13. [Google Scholar] [CrossRef]
- Thompson, C.; Duncan, R.C. Neutron star dynamos and the origins of pulsar magnetism. Astrophys. J. 1993, 408, 194–217. [Google Scholar] [CrossRef]
- Olausen, S.A.; Kaspi, V.M. The McGill Magnetar Catalog. Astrophys. J. Suppl. 2014, 212, 6. [Google Scholar] [CrossRef]
- Thompson, C.; Duncan, R.C. The soft gamma repeaters as very strongly magnetized neutron stars—I. Radiative mechanism for outbursts. Mon. Not. R. Astron. Soc. 1995, 275, 255–300. [Google Scholar] [CrossRef]
- Thompson, C.; Duncan, R.C. The Soft Gamma Repeaters as Very Strongly Magnetized Neutron Stars. II. Quiescent Neutrino, X-ray, and Alfven Wave Emission. Astrophys. J. 1996, 473, 322. [Google Scholar] [CrossRef]
- Kaspi, V.M.; Kramer, M. Radio Pulsars: The Neutron Star Population Fundamental Physics. ArXiv, 2016; arXiv:astro-ph.HE/1602.07738. [Google Scholar]
- Melatos, A. Bumpy spindown of anomalous X-ray pulsars: The link with magnetars. Astrophys. J. 1999, 519, L77. [Google Scholar] [CrossRef]
- Makishima, K.; Enoto, T.; Hiraga, J.S.; Nakano, T.; Nakazawa, K.; Sakurai, S.; Sasano, M.; Murakami, H. Possible Evidence for Free Precession of a Strongly Magnetized Neutron Star in the Magnetar 4U 0142+61. Phys. Rev. Lett. 2014, 112, 171102. [Google Scholar] [CrossRef] [PubMed]
- Landau, L.D.; Lifshitz, E.M. Quantum Mechanics: Non-Relativistic Theory; Elsevier: Amsterdam, The Netherlands, 1977. [Google Scholar]
- Lai, D.; Shapiro, S.L. Cold equation of state in a strong magnetic field—Effects of inverse beta-decay. Astrophys. J. 1991, 383, 745–751. [Google Scholar] [CrossRef]
- Chakrabarty, S. Quark matter in strong magnetic field. Phys. Rev. D 1996, 54, 1306–1316. [Google Scholar] [CrossRef]
- Chakrabarty, S.; Bandyopadhyay, D.; Pal, S. Dense nuclear matter in a strong magnetic field. Phys. Rev. Lett. 1997, 78, 2898–2901. [Google Scholar] [CrossRef]
- Yuan, Y.F.; Zhang, J.L. The Effects of Interior Magnetic Fields on the Properties of Neutron Stars in the Relativistic Mean-Field Theory. Astrophys. J. 1999, 525, 950–958. [Google Scholar] [CrossRef]
- Broderick, A.; Prakash, M.; Lattimer, J.M. The Equation of state of neutron star matter in strong magnetic fields. Astrophys. J. 2000, 537, 351. [Google Scholar] [CrossRef]
- Dexheimer, V.; Negreiros, R.; Schramm, S. Hybrid stars in a strong magnetic field. Eur. Phys. J. A 2012, 48, 189. [Google Scholar] [CrossRef]
- Canuto, V.; Chiu, H.Y. Quantum theory of an electron gas in intense magnetic fields. Phys. Rev. 1968, 173, 1210–1219. [Google Scholar] [CrossRef]
- Canuto, V.; Chiu, H.Y. Thermodynamic properties of a magnetized fermi gas. Phys. Rev. 1968, 173, 1220–1228. [Google Scholar] [CrossRef]
- Strickland, M.; Dexheimer, V.; Menezes, D.P. Bulk Properties of a Fermi Gas in a Magnetic Field. Phys. Rev. D 2012, 86, 125032. [Google Scholar] [CrossRef]
- Dexheimer, V.; Menezes, D.P.; Strickland, M. The influence of strong magnetic fields on proto-quark stars. J. Phys. G Nucl. Part. Phys. 2014, 41, 015203. [Google Scholar] [CrossRef]
- Andersen, J.O.; Naylor, W.R.; Tranberg, A. Phase diagram of QCD in a magnetic field. Rev. Mod. Phys. 2016, 88, 025001. [Google Scholar] [CrossRef]
- Broderick, A.E.; Prakash, M.; Lattimer, J.M. Effects of strong magnetic fields in strange baryonic matter. Phys. Lett. B 2002, 531, 167–174. [Google Scholar] [CrossRef]
- Bordbar, G.H.; Rezaei, Z. Magnetized hot neutron matter: Lowest order constrained variational calculations. Phys. Lett. B 2013, 718, 1125–1131. [Google Scholar] [CrossRef]
- Dexheimer, V.; Negreiros, R.; Schramm, S.; Hempel, M. Deconfinement to Quark Matter in Neutron Stars—The Influence of Strong Magnetic Fields. AIP Conf. Proc. 2013, 1520, 264–269. [Google Scholar]
- Weinberg, S. Why do quarks behave like bare Dirac particles? Phys. Rev. Lett. 1990, 65, 1181–1183. [Google Scholar] [CrossRef] [PubMed]
- Ferrer, E.J.; de la Incera, V.; Manreza Paret, D.; Pérez Martínez, A.; Sanchez, A. Insignificance of the anomalous magnetic moment of charged fermions for the equation of state of a magnetized and dense medium. Phys. Rev. D 2015, 91, 085041. [Google Scholar] [CrossRef]
- Chatterjee, D.; Elghozi, T.; Novak, J.; Oertel, M. Consistent neutron star models with magnetic field dependent equations of state. Mon. Not. Roy. Astron. Soc. 2015, 447, 3785–3796. [Google Scholar] [CrossRef]
- Franzon, B.; Dexheimer, V.; Schramm, S. A self-consistent study of magnetic field effects on hybrid stars. Mon. Not. Roy. Astron. Soc. 2016, 456, 2937–2945. [Google Scholar] [CrossRef]
- Dexheimer, V.; Negreiros, R.; Schramm, S. Hybrid Stars in a Strong Magnetic Field. Eur. Phys. J. A 2012, 48, 189. [Google Scholar] [CrossRef]
- Alloy, M.D.; Menezes, D.P. Maxwell equation violation by density dependent magnetic fields in neutron stars. Int. J. Mod. Phys. Conf. Ser. 2017, 45, 1760031. [Google Scholar] [CrossRef]
- Dexheimer, V.; Franzon, B.; Gomes, R.O.; Farias, R.L.S.; Avancini, S.S.; Schramm, S. What is the magnetic field distribution for the equation of state of magnetized neutron stars? Phys. Lett. B 2017, 773, 487–491. [Google Scholar] [CrossRef]
- Oppenheimer, J.R.; Snyder, H. On Continued Gravitational Contraction. Phys. Rev. 1939, 56, 455–459. [Google Scholar] [CrossRef]
- Hurley, K.; Dingus, B.L.; Mukherjee, R.; Sreekumar, P.; Kouveliotou, C.; Meegan, C.; Fishman, G.J.; Band, D.; Ford, L.; Bertsch, D.; et al. Detection of a γ-ray burst of very long duration and very high energy. Nature 1994, 372, 652–654. [Google Scholar] [CrossRef]
- Galama, T.J.; Vreeswijk, P.M.; van Paradijs, J.; Kouveliotou, C.; Augusteijn, T.; Böhnhardt, H.; Brewer, J.P.; Doublier, V.; Gonzalez, J.F.; Leibundgut, B.; et al. An unusual supernova in the error box of the γ-ray burst of 25 April 1998. Nature 1998, 395, 670–672. [Google Scholar] [CrossRef]
- Popov, S.B.; Prokhorov, M.E. Progenitors with enhanced rotation and the origin of magnetars. Mon. Not. R. Astron. Soc. 2006, 367, 732–736. [Google Scholar] [CrossRef]
- Herrera, L.; di Prisco, A.; Martin, J.; Ospino, J.; Santos, N.O.; Troconis, O. Spherically symmetric dissipative anisotropic fluids: A general study. Phys. Rev. D 2004, 69, 084026. [Google Scholar] [CrossRef]
- Herrera, L.; di Prisco, A.; Fuenmayor, E.; Troconis, O. Dynamics of Viscous Dissipative Gravitational Collapse: A Full Causal Approach. Int. J. Mod. Phys. D 2009, 18, 129–145. [Google Scholar] [CrossRef]
- Bonazzola, S.; Gourgoulhon, E.; Salgado, M.; Marck, J.A. Axisymmetric rotating relativistic bodies: A new numerical approach for ‘exact’ solutions. Astron. Astrophys. 1993, 278, 421–443. [Google Scholar]
- Misner, C.; Thorne, K.S.; Wheeler, J.A. Gravitation; Princeton University Press: Princeton, NJ, USA, 1973. [Google Scholar]
- Herrera, L.; Di Prisco, A.; Ibáñez, J.; Ospino, J. Axially symmetric static sources: A general framework and some analytical solutions. Phys. Rev. D 2013, 87, 024014. [Google Scholar] [CrossRef]
- Tolman, R.C. Effect of Inhomogeneity on Cosmological Models. Proc. Natl. Acad. Sci. USA 1934, 20, 169–176. [Google Scholar] [CrossRef] [PubMed]
- Oppenheimer, J.R.; Volkoff, G.M. On Massive Neutron Cores. Phys. Rev. 1939, 55, 374–381. [Google Scholar] [CrossRef]
- Tolman, R.C. Static Solutions of Einstein’s Field Equations for Spheres of Fluid. Phys. Rev. 1939, 55, 364–373. [Google Scholar] [CrossRef]
- Bondi, H. The contraction of gravitating spheres. Proc. R. Soc. A 1964, 281, 39–48. [Google Scholar] [CrossRef]
- Bocquet, M.; Bonazzola, S.; Gourgoulhon, E.; Novak, J. Rotating neutron star models with magnetic field. Astron. Astrophys. 1995, 301, 757–775. [Google Scholar]
- Cardall, C.Y.; Prakash, M.; Lattimer, J.M. Effects of strong magnetic fields on neutron star structure. Astrophys. J. 2001, 554, 322–339. [Google Scholar] [CrossRef]
- Frieben, J.; Rezzolla, L. Equilibrium models of relativistic stars with a toroidal magnetic field. Mon. Not. Roy. Astron. Soc. 2012, 427, 3406–3426. [Google Scholar] [CrossRef]
- Pili, A.G.; Bucciantini, N.; Del Zanna, L. Axisymmetric equilibrium models for magnetized neutron stars in General Relativity under the Conformally Flat Condition. Mon. Not. Roy. Astron. Soc. 2014, 439, 3541–3563. [Google Scholar] [CrossRef]
- Gomes, R.O.; Franzon, B.; Dexheimer, V.; Schramm, S. Many-body forces in magnetic neutron stars. Astrophys. J. 2017, 850, 20. [Google Scholar] [CrossRef]
- Pili, A.G.; Bucciantini, N.; Del Zanna, L. General relativistic models for rotating magnetized neutron stars in conformally flat space-time. Mon. Not. Roy. Astron. Soc. 2017, 470, 2469–2493. [Google Scholar] [CrossRef]
- Kaplan, D.L.; Bassa, C.; Wang, Z.; Cumming, A.; Kaspi, V.M. Nearby, Thermally Emitting Neutron Stars. AIP Conf. Proc. 2008, 983, 331–339. [Google Scholar]
- Haberl, F. AXPs and X-ray dim neutron stars: Recent XMM-Newton and Chandra results. In Proceedings of the 34th COSPAR Scientific Assembly, Houston, TX, USA, 10–19 October 2002. [Google Scholar]
- Kaplan, D.L.; van Kerkwijk, M.H. A Coherent Timing Solution for the Nearby Isolated Neutron Star RX J0720.4-3125. Astrophys. J. Lett. 2005, 628, L45–L48. [Google Scholar] [CrossRef]
- Gill, R.; Heyl, J.S. Statistical ages and the cooling rate of X-ray dim isolated neutron stars. Mon. Not. R. Astron. Soc. 2013, 435, 3243–3250. [Google Scholar] [CrossRef]
- Kaplan, D.L.; van Kerkwijk, M.H. Constraining the Spin-down of the Nearby Isolated Neutron Star RX J0806.4-4123, and Implications for the Population of Nearby Neutron Stars. Astrophys. J. 2009, 705, 798–808. [Google Scholar] [CrossRef]
- Kaplan, D.L.; van Kerkwijk, M.H. A Coherent Timing Solution for the Nearby Isolated Neutron Star RX J1308.6+2127/RBS 1223. Astrophys. J. Lett. 2005, 635, L65–L68. [Google Scholar] [CrossRef]
- Walter, F.M.; Wolk, S.J.; Neuhäuser, R. Discovery of a nearby isolated neutron star. Nature 1996, 379, 233–235. [Google Scholar] [CrossRef]
- Van Kerkwijk, M.H.; Kaplan, D.L. Timing the Nearby Isolated Neutron Star RX J1856.5-3754. Astrophys. J. Lett. 2008, 673, L163–L166. [Google Scholar] [CrossRef]
- Zane, S.; Cropper, M.; Turolla, R.; Zampieri, L.; Chieregato, M.; Drake, J.J.; Treves, A. XMM-Newton Detection of Pulsations and a Spectral Feature in the X-ray Emission of the Isolated Neutron Star 1RXS J214303.7+065419/RBS 1774. Astrophys. J. 2005, 627, 397–403. [Google Scholar] [CrossRef]
- Kaplan, D.L.; van Kerkwijk, M.H. Constraining the Spin-Down of the Nearby Isolated Neutron Star RX J2143.0+0654. Astrophys. J. Lett. 2009, 692, L62–L66. [Google Scholar] [CrossRef]
- Van Kerkwijk, M.H.; Kaplan, D.L.; Durant, M.; Kulkarni, S.R.; Paerels, F. A Strong, Broad Absorption Feature in the X-ray Spectrum of the Nearby Neutron Star RX J1605.3+3249. Astrophys. J. 2004, 608, 432–443. [Google Scholar] [CrossRef]
- Pires, A.M.; Haberl, F.; Zavlin, V.E.; Motch, C.; Zane, S.; Hohle, M.M. XMM-Newton reveals a candidate period for the spin of the “Magnificent Seven” neutron star RX J1605.3+3249. Astron. Astrophys. 2014, 563, A50. [Google Scholar] [CrossRef]
- Negreiros, R.; Schramm, S.; Weber, F. Impact of Rotation-Driven Particle Repopulation on the Thermal Evolution of Pulsars. Phys. Lett. B 2011, 718, 1–5. [Google Scholar]
- Negreiros, R.; Schramm, S.; Weber, F. Thermal evolution of neutron stars in two dimensions. Phys. Rev. D 2012, 85, 104019. [Google Scholar] [CrossRef]
- De Carvalho, S.M.; Negreiros, R.; Orsaria, M.; Contrera, G.A.; Weber, F.; Spinella, W. Thermal evolution of hybrid stars within the framework of a nonlocal Nambu-Jona-Lasinio model. Phys. Rev. C 2015, 92, 035810. [Google Scholar] [CrossRef]
- Shternin, P.S.; Yakovlev, D.G.; Heinke, C.O.; Ho, W.C.G.; Patnaude, D.J. Cooling neutron star in the Cassiopeia A supernova remnant: evidence for superfluidity in the core. Mon. Not. R. Astron. Soc. Lett. 2011, 412, L108–L112. [Google Scholar] [CrossRef]
- Yakovlev, D.G.; Ho, W.C.G.; Shternin, P.S.; Heinke, C.O.; Potekhin, A.Y. Cooling rates of neutron stars and the young neutron star in the Cassiopeia A supernova remnant. Mon. Not. R. Astron. Soc. 2011, 411, 1977–1988. [Google Scholar] [CrossRef]
- Page, D.; Prakash, M.; Lattimer, J.M.; Steiner, A.W. Rapid Cooling of the Neutron Star in Cassiopeia A Triggered by Neutron Superfluidity in Dense Matter. Phys. Rev. Lett. 2011, 106, 081101. [Google Scholar] [CrossRef] [PubMed]
- Muslimov, A.; Page, D. Delayed switch-on of pulsars. Astrophys. J. Lett. 1995, 440, L77–L80. [Google Scholar] [CrossRef]
- Geppert, U.; Page, D.; Zannias, T. Submergence and re-diffusion of the neutron star magnetic field after the supernova. Astron. Astrophys. 1999, 345, 847–854. [Google Scholar]
- Viganò, D.; Pons, J.A. Central compact objects and the hidden magnetic field scenario. Mon. Not. R. Astron. Soc. 2012, 425, 2487–2492. [Google Scholar] [CrossRef] [Green Version]
- Bernal, C.G.; Page, D.; Lee, W.H. Hypercritical Accretion onto a Newborn Neutron Star and Magnetic Field Submergence. Astrophys. J. 2013, 770, 106. [Google Scholar] [CrossRef]
- Heiselberg, H.; Hjorth-Jensen, M. Phase Transitions in Rotating Neutron Stars. Phys. Rev. Lett. 1998, 80, 5485–5488. [Google Scholar] [CrossRef]
- Page, D.; Lattimer, J.M.; Prakash, M.; Steiner, A.W. Minimal Cooling of Neutron Stars: A New Paradigm. Astrophys. J. Suppl. Ser. 2004, 155, 623–650. [Google Scholar] [CrossRef]
- Kaplan, D.L.; van Kerkwijk, M.H.; Anderson, J. The Parallax and Proper Motion of RX J1856.53754 Revisited. Astrophys. J. 2002, 571, 447–457. [Google Scholar] [CrossRef]
- Chevalier, R.A. Neutron star accretion in a supernova. Astrophys. J. 1989, 346, 847–859. [Google Scholar] [CrossRef]
- Bernal, C.G.; Lee, W.H.; Page, D. Hypercritical accretion onto a magnetized neutron star surface: A numerical approach. Revista Mexicana de Astronomía y Astrofísica 2010, 46, 309–322. [Google Scholar]
- Muslimov, A.; Page, D. Magnetic and Spin History of Very Young Pulsars. Astrophys. J. 1996, 458, 347. [Google Scholar] [CrossRef]
- Brown, G.E.; Weingartner, J.C. Accretion onto and radiation from the compact object formed in SN 1987A. Astrophys. J. 1994, 436, 843–847. [Google Scholar] [CrossRef]
- Pons, J.A.; Miralles, J.A.; Geppert, U. Magneto-thermal evolution of neutron stars. Astron. Astrophys. 2009, 496, 207–216. [Google Scholar] [CrossRef]
- Viganò, D.; Rea, N.; Pons, J.A.; Perna, R.; Aguilera, D.N.; Miralles, J.A. Unifying the observational diversity of isolated neutron stars via magneto-thermal evolution models. Mon. Not. R. Astron. Soc. 2013, 434, 123–141. [Google Scholar] [CrossRef] [Green Version]
- Gourgouliatos, K.N.; Wood, T.S.; Hollerbach, R. Magnetic field evolution in magnetar crusts through three-dimensional simulations. Proc. Natl. Acad. Sci. USA 2016, 113, 3944–3949. [Google Scholar] [CrossRef] [PubMed]
- Kaspi, V.M. Grand unification of neutron stars. Proc. Natl. Acad. Sci. USA 2010, 107, 7147–7152. [Google Scholar] [CrossRef] [PubMed]
- Harding, A.K. The neutron star zoo. Front. Phys. 2013, 8, 679–692. [Google Scholar] [CrossRef]
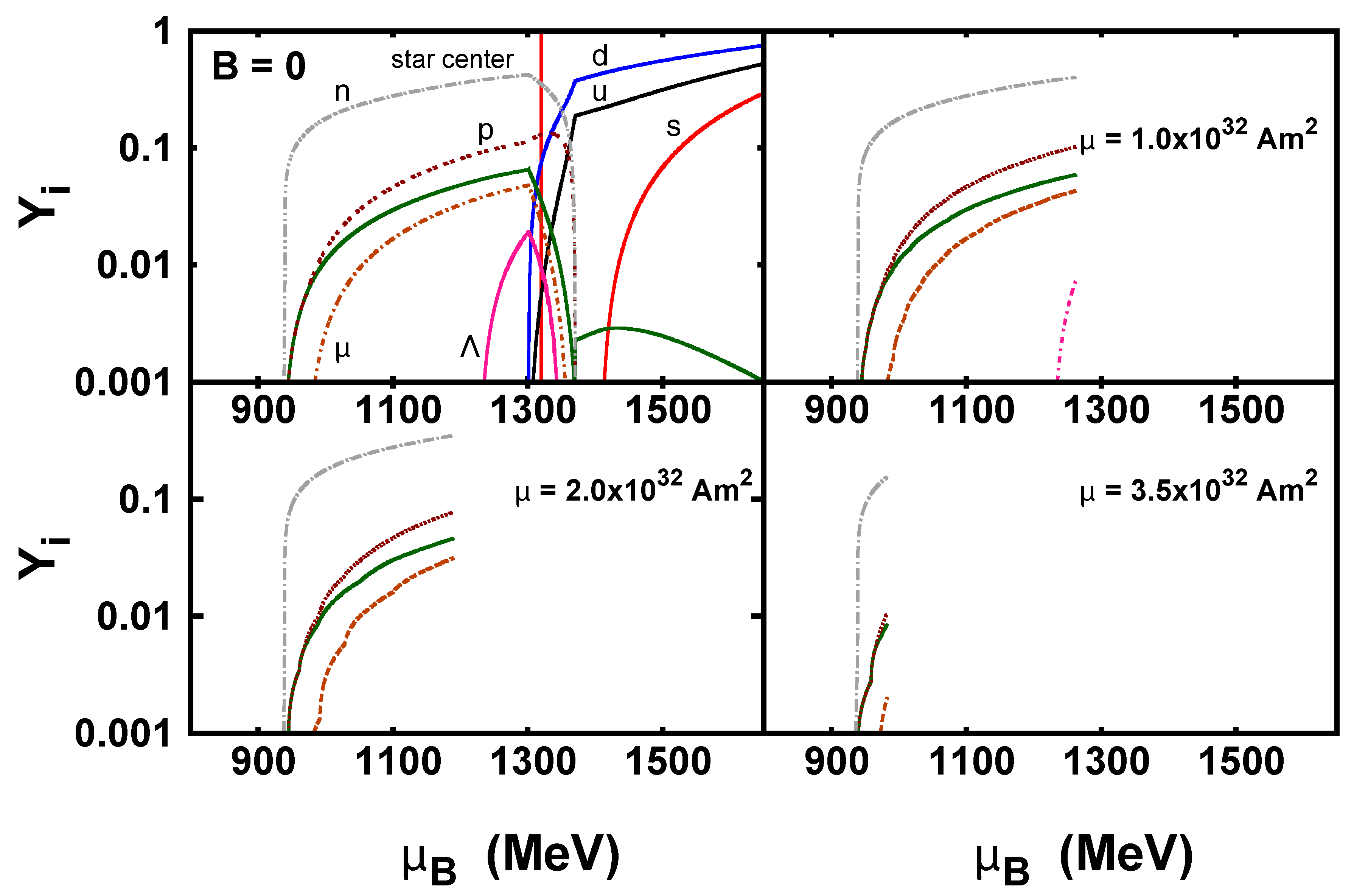
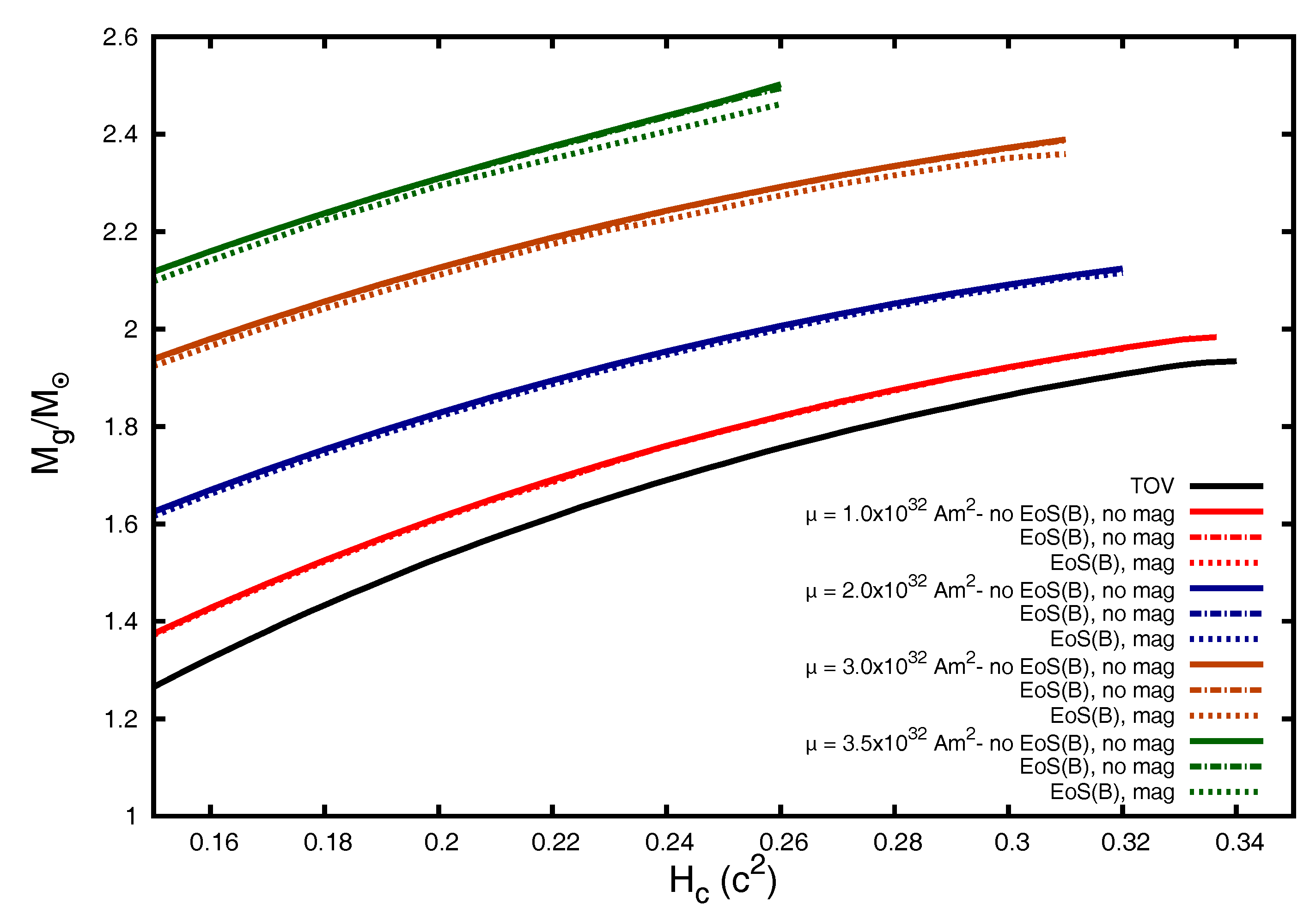
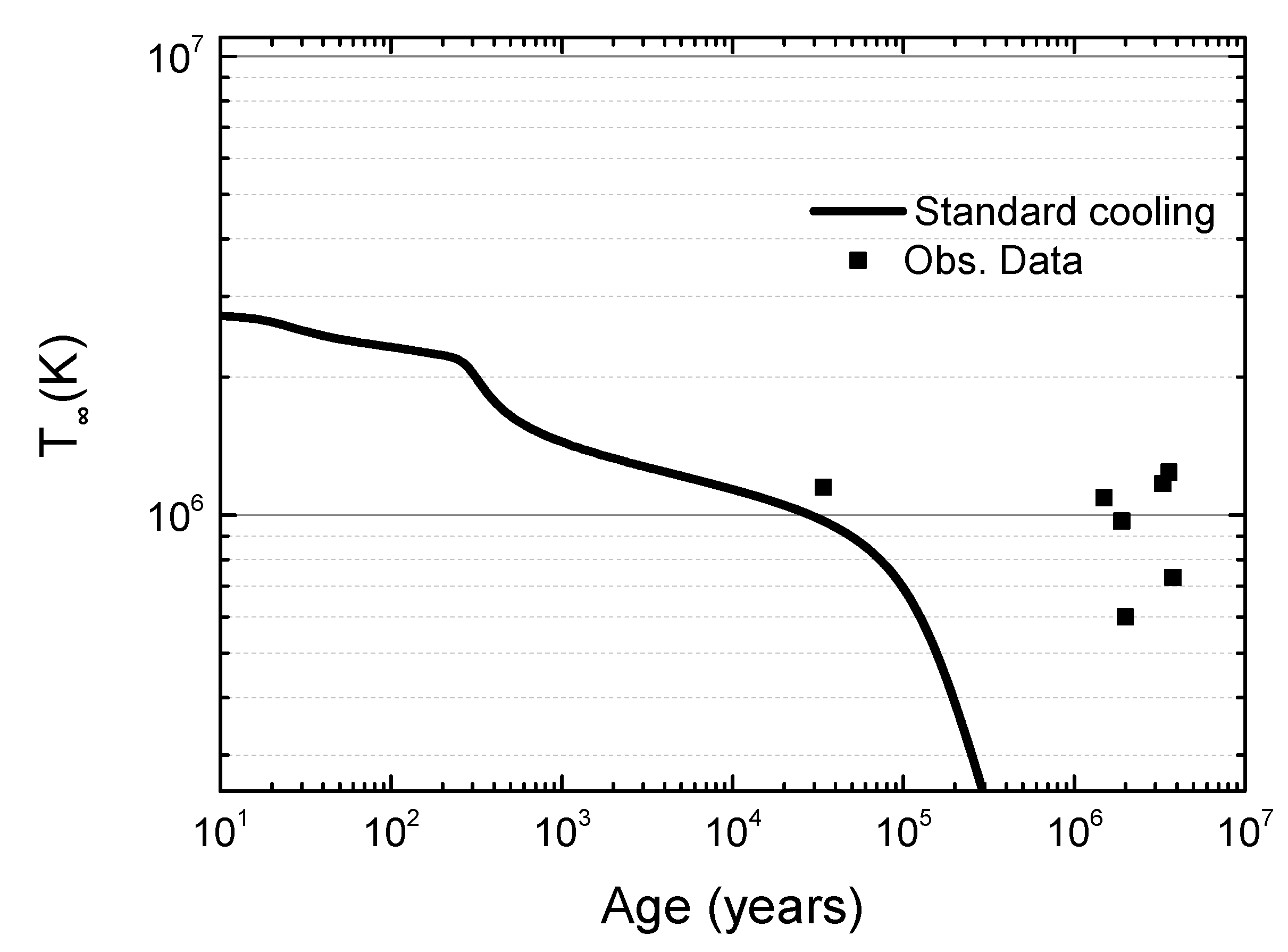
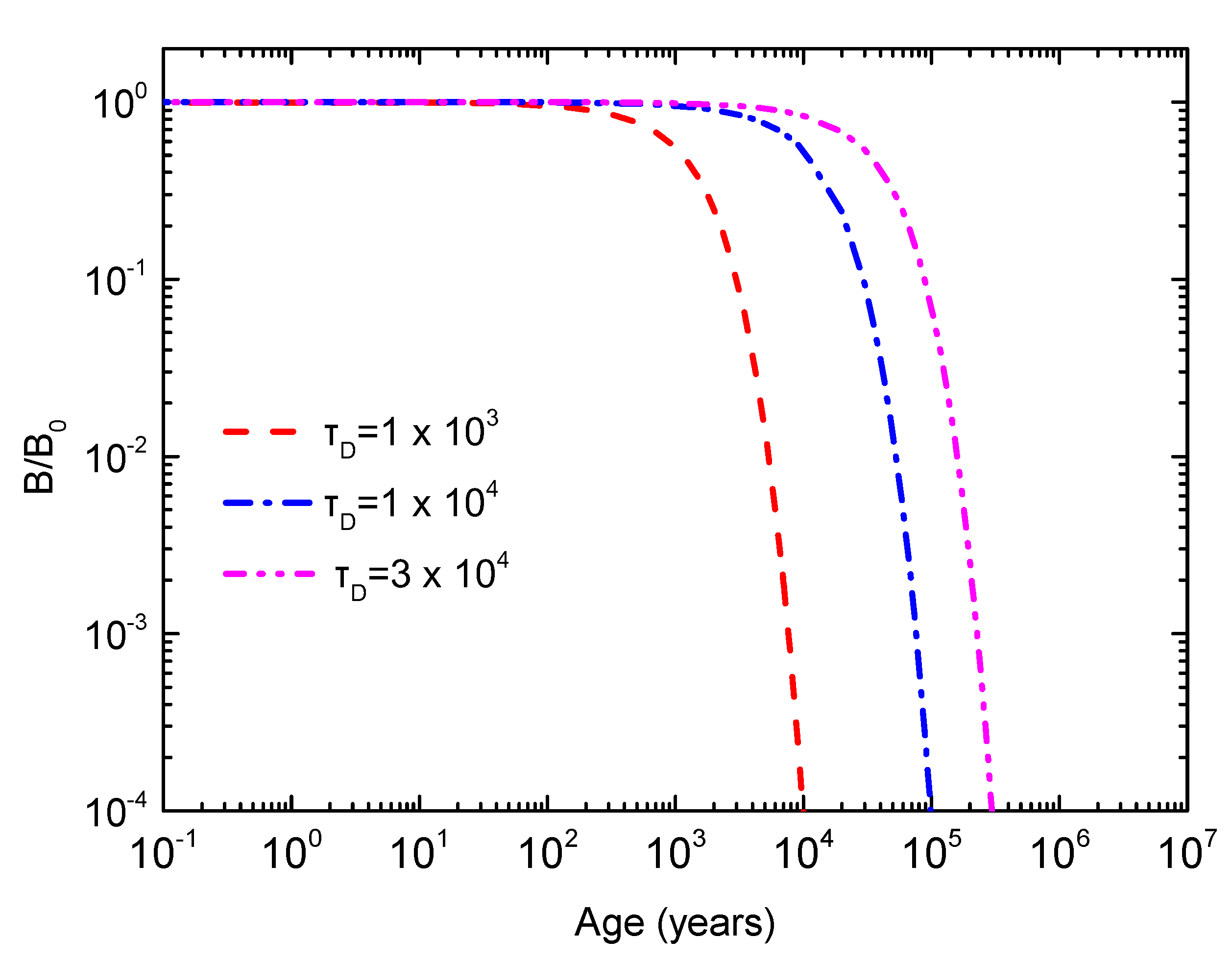
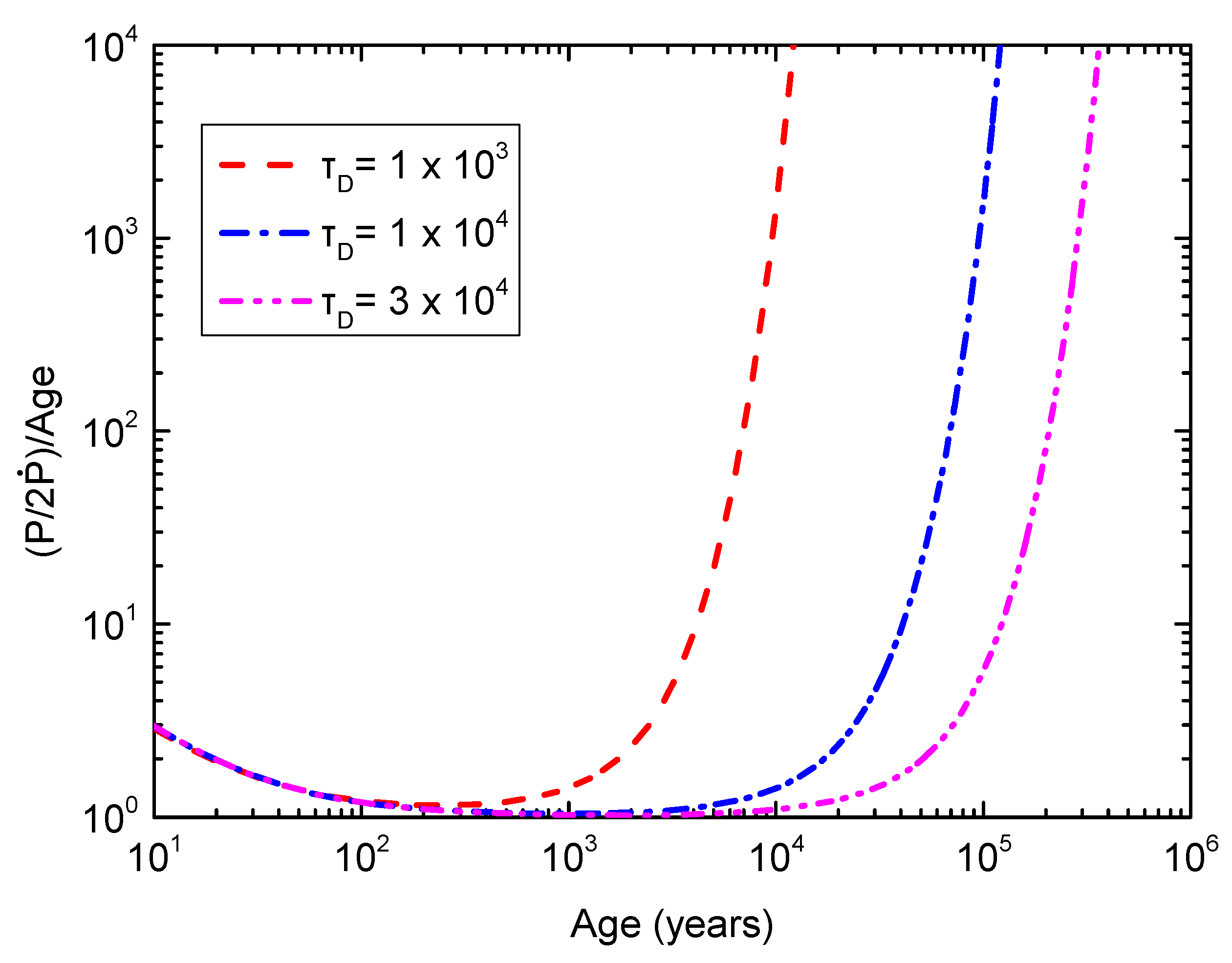
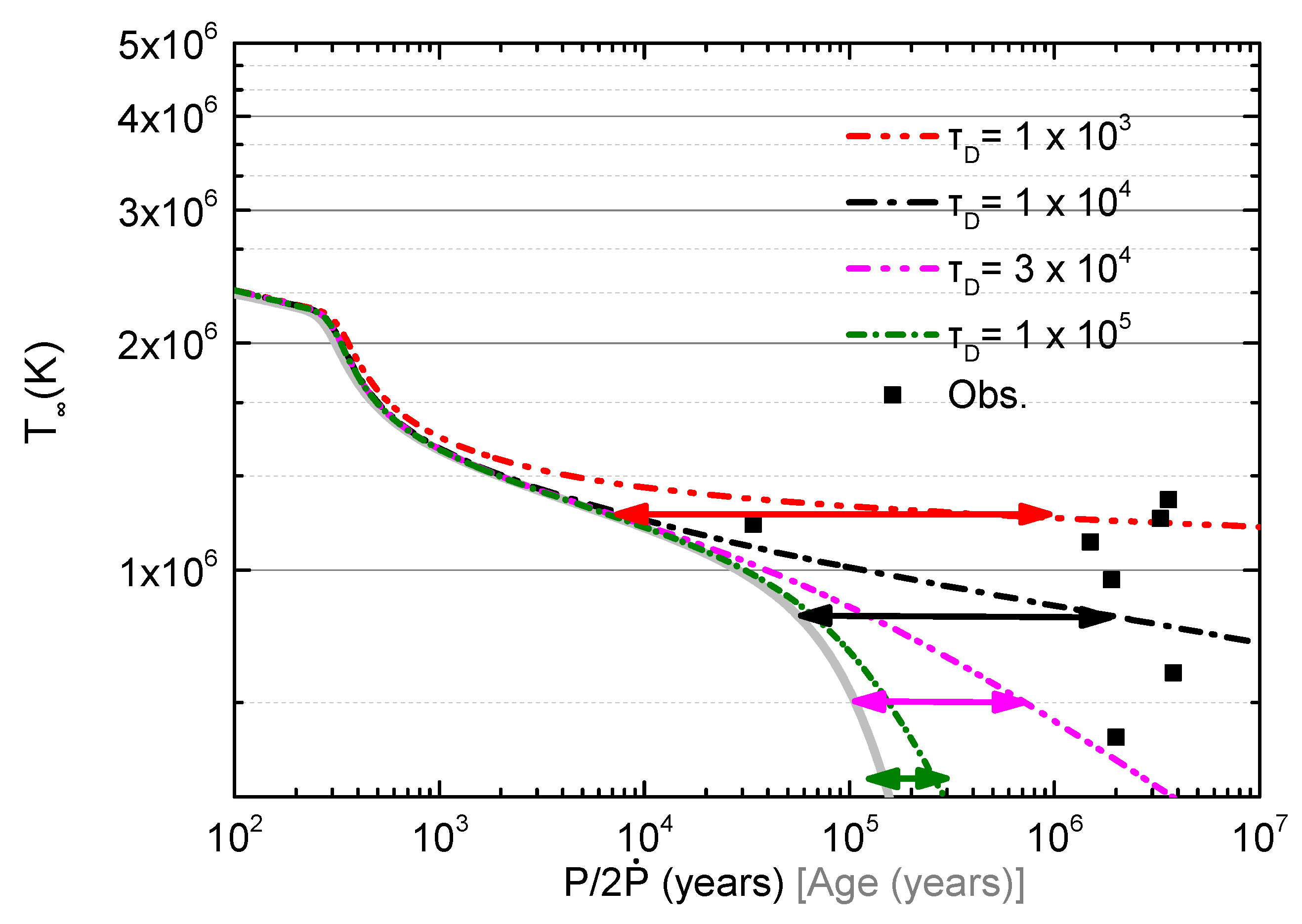
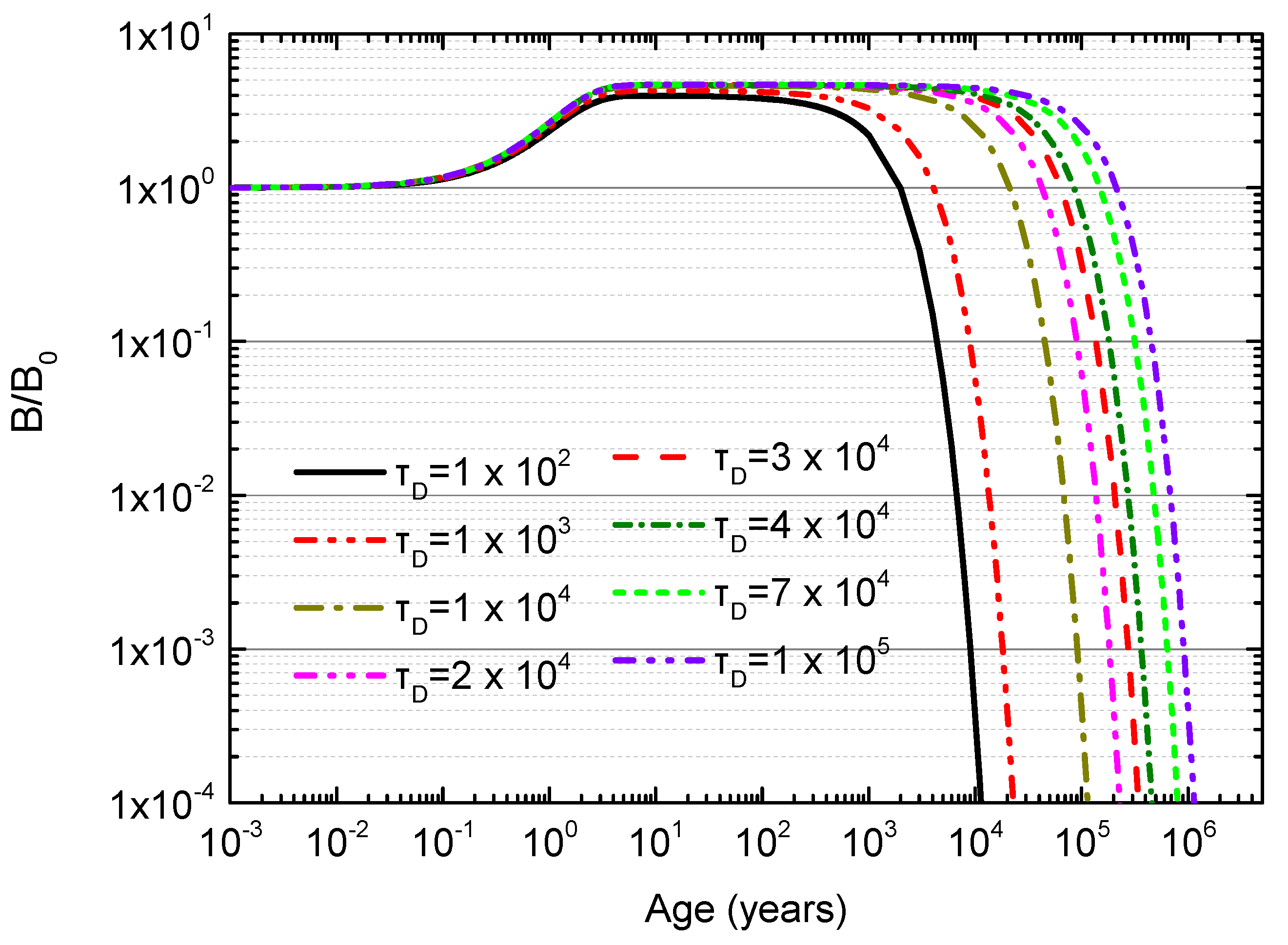
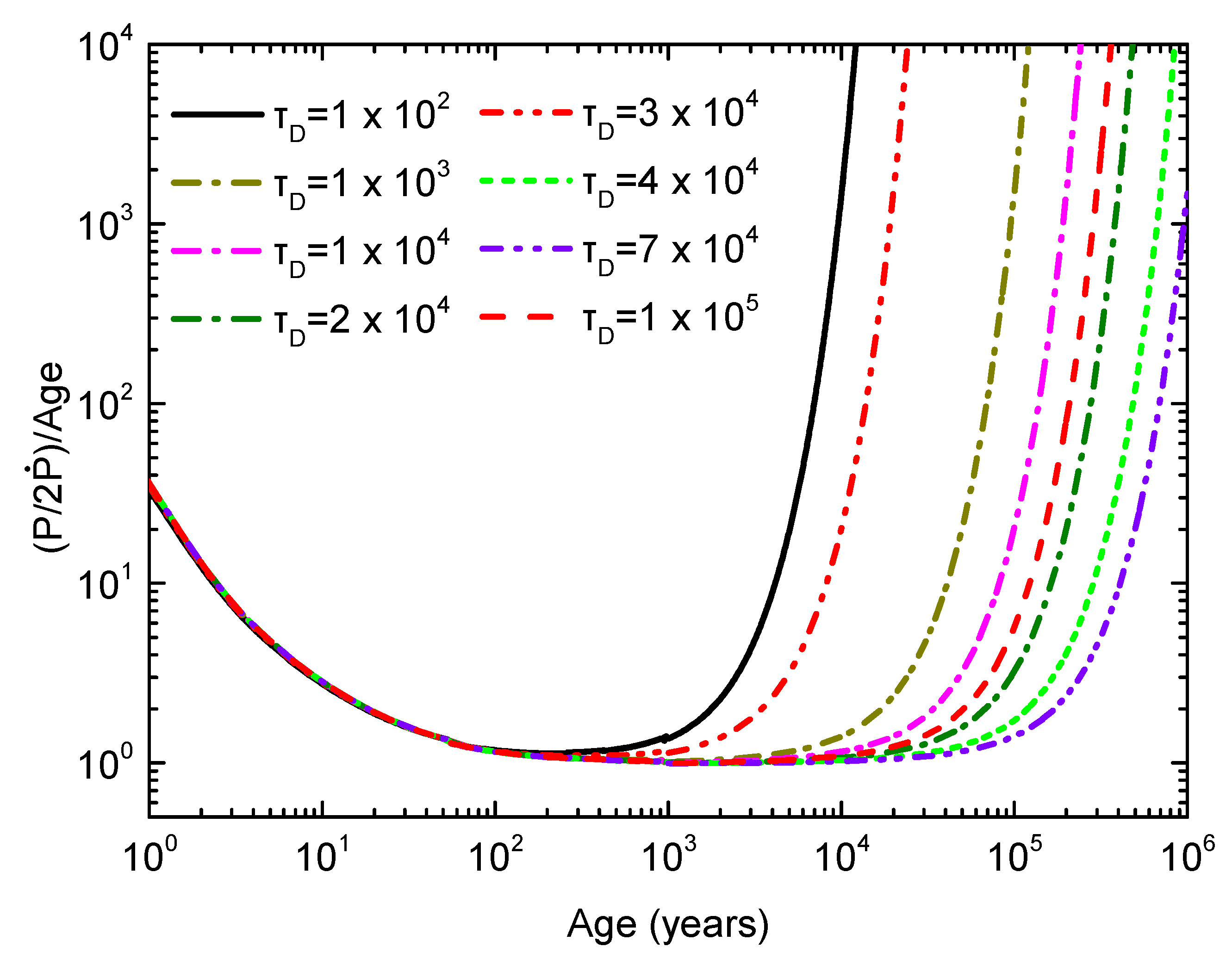
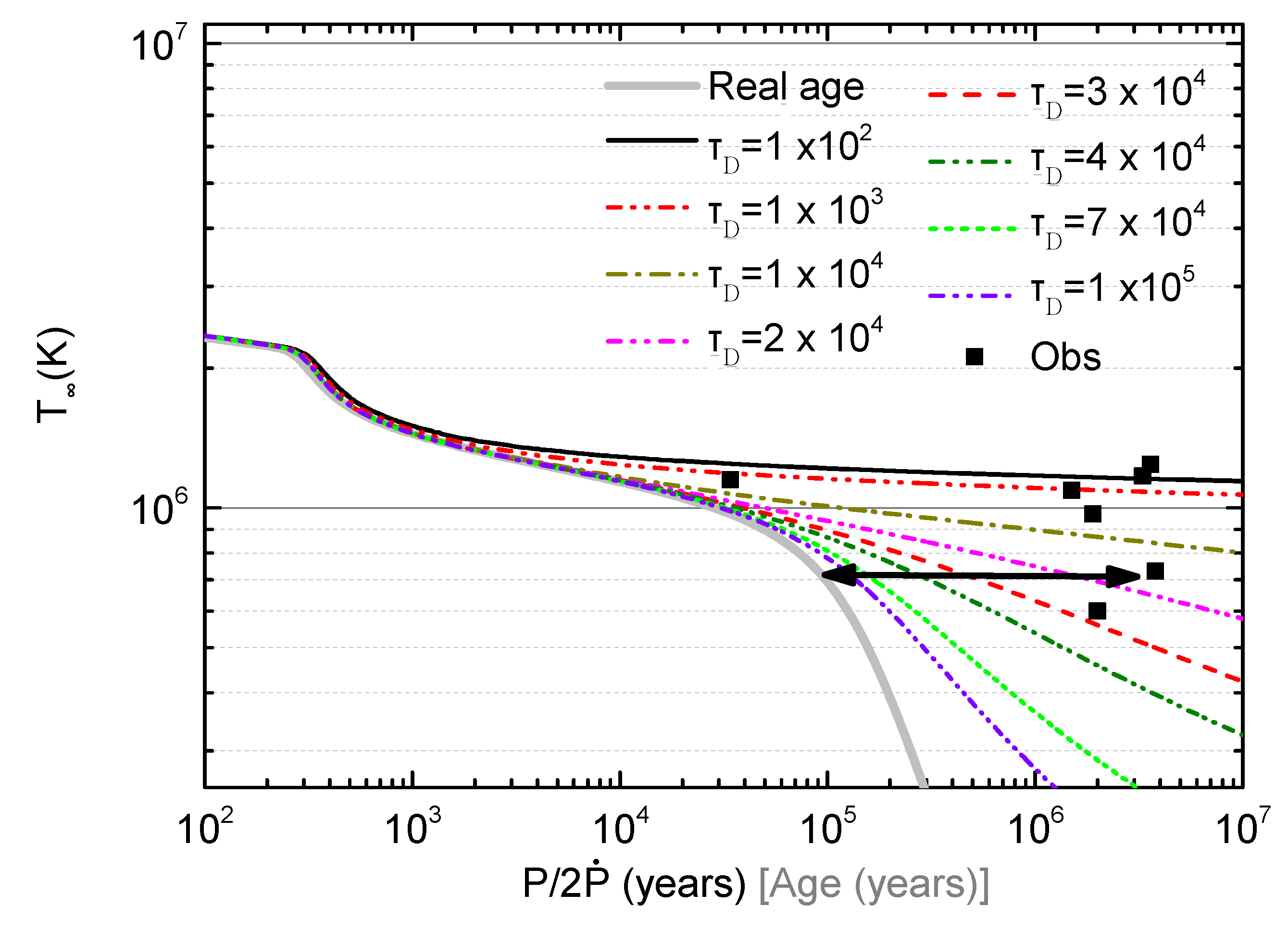
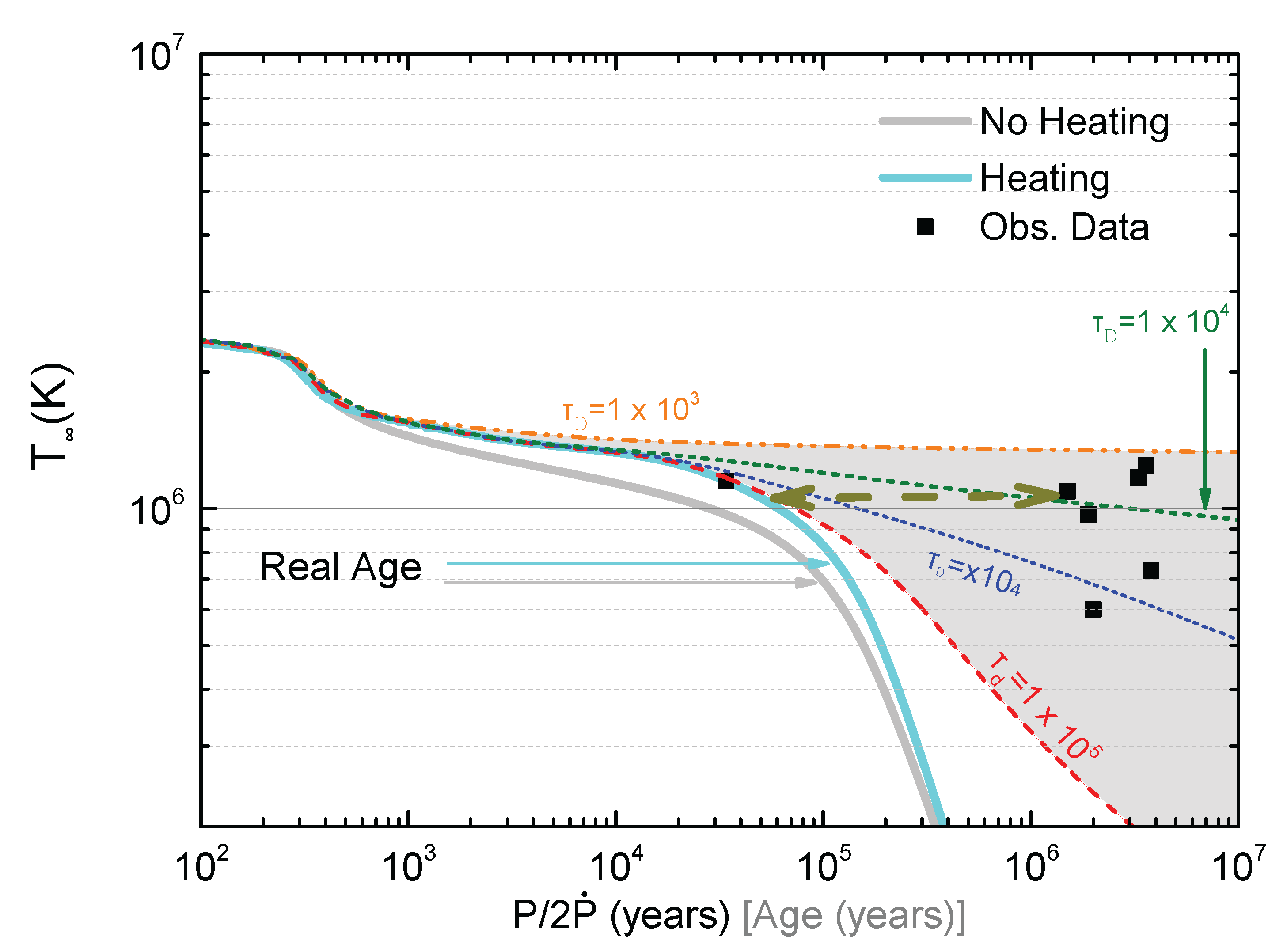
| XDIN Name | Period (s) P (s) | Period Derivative (s s) | Blackbody Temperature (K) | References | Spin-Down Age (Years) t (yr) |
|---|---|---|---|---|---|
| RX J0720.4-3125 | 8.39 | [52,53] | |||
| RX J0806.4-4123 | 11.37 | [53,54] | |||
| RX J1308.6+2127 | 10.31 | [53,55] | |||
| RX J1856.5-3754 | 7.055 | [56,57] | |||
| RX J2143.0+0654 | 9.437 | [58,59] | |||
| RX J0420.0-5022 | 3.45 | [58,59] | |||
| RX J1605.3+3249 | 3.39 | [60,61] |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Negreiros, R.; Bernal, C.; Dexheimer, V.; Troconis, O. Many Aspects of Magnetic Fields in Neutron Stars. Universe 2018, 4, 43. https://doi.org/10.3390/universe4030043
Negreiros R, Bernal C, Dexheimer V, Troconis O. Many Aspects of Magnetic Fields in Neutron Stars. Universe. 2018; 4(3):43. https://doi.org/10.3390/universe4030043
Chicago/Turabian StyleNegreiros, Rodrigo, Cristian Bernal, Veronica Dexheimer, and Orlenys Troconis. 2018. "Many Aspects of Magnetic Fields in Neutron Stars" Universe 4, no. 3: 43. https://doi.org/10.3390/universe4030043
APA StyleNegreiros, R., Bernal, C., Dexheimer, V., & Troconis, O. (2018). Many Aspects of Magnetic Fields in Neutron Stars. Universe, 4(3), 43. https://doi.org/10.3390/universe4030043






