On the Hypothesis of Exact Conservation of Charged Weak Hadronic Vector Current in the Standard Model
Abstract
1. Introduction
2. Precision Analysis of Neutron Lifetime to Order
3. Fierz Interference Term
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. The Amplitude of the Rate of the n Radiative β− Decay
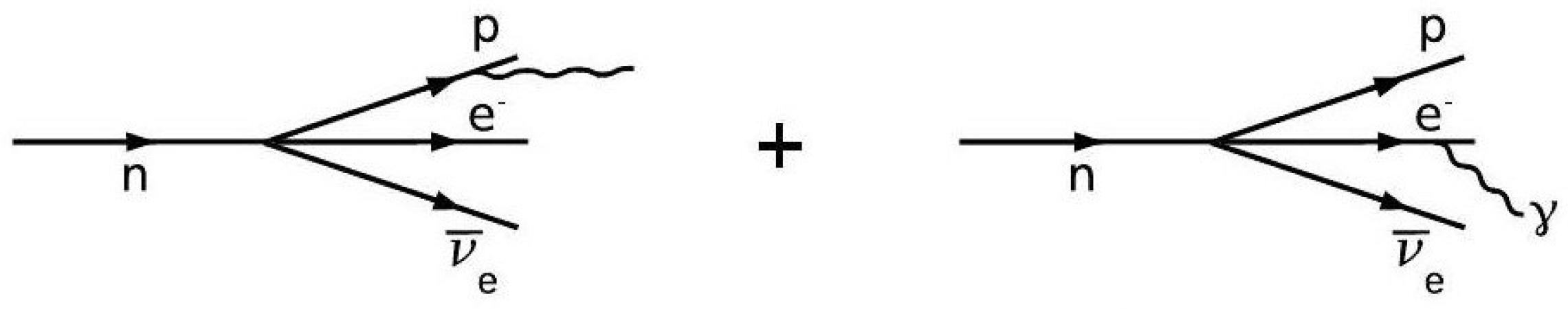
| [keV] | (Experiment) | (Theory) | (Theory) | |
|---|---|---|---|---|
| [4] | ||||
| [1] | ||||
| [1] | ||||
Appendix B. Numerical Analysis of the Branching Ratio in Equation (A5)
Appendix C. Numerical Analysis of Equation (A7)
References
- Ivanov, A.N.; Pitschmann, M.; Troitskaya, N.I. Neutron beta-decay as laboratory for test of standard model. Phys. Rev. D 2013, arXiv:1212.0332v4. [Google Scholar] [CrossRef]
- Partignani, C.; et al. [Particle Data Group] Review of Particle Physics. Chin. Phys. C 2016, 40, 100001. [Google Scholar]
- Arzumanov, S.; Bondarenko, L.; Chernyavsky, S.; Geltenbort, P.; Morozov, V.; Nesvizhevsky, V.V.; Panin, Y.; Strepetov, A. A measurement of the neutron lifetime using the method of storage of ultracold neutrons and detection of inelastically up-scattered neutrons. Phys. Lett. B 2015, 745, 79. [Google Scholar] [CrossRef]
- Abele, H. The neutron. Its properties and basic interactions. Prog. Part. Nucl. Phys. 2008, 60, 1–81. [Google Scholar] [CrossRef]
- Olive, K.A.; et al. [Particle Data Group] Review of Particle Physics. Chin. Phys. A 2014, 38, 090001. [Google Scholar] [CrossRef]
- Hardy, J.C.; Tower, I.S. Superallowed 0+–0+ nuclear Beta decays: 2014 critical survey, with precise results for V ud and CKM unitarity. Phys. Rev. D 2015, 91, 025501. [Google Scholar]
- Severijns, N.; Naviliat-Cuncic, O. Symmetry tests in nuclear beta decay. Annu. Rev. Nucl. Part. Sci. 2011, 61, 23. [Google Scholar] [CrossRef]
- Naviliat-Cuncic, O.; Severijns, N. Test of the Conserved Vector Current Hypothesis in T = 1/2 Mirror Transitions and New Determination of |Vud|. Phys. Rev. Lett. 2009, 102, 142302. [Google Scholar]
- Ankowski, A.M. Improved estimate of the cross section for inverse beta decay. J. Phys. Conf. Ser. 2019, arXiv:1601.06169v1. [Google Scholar] [CrossRef]
- Giunti, C. On the implementation of CVC in weak charged-current proton-neutron transitions. arXiv 2016, arXiv:1602.00215. [Google Scholar]
- Ivanov, A.N.; Pitschmann, M.; Troitskaya, N.I.; Berdnikov, Y.A. Bound-state β− decay of the neutron re-examined. Phys. Rev. C 2014, 89, 055502. [Google Scholar] [CrossRef]
- Nowakowski, M.; Paschos, E.A.; Rodriguez, J.M. All electromagnetic form factors. Eur. J. Phys. 2005, 26, 545. [Google Scholar] [CrossRef]
- Leitner, T.; Alvarez-Ruso, L.; Mosel, U. Charged current neutrino-nucleus interactions at intermediate energies. Phys. Rev. C 2006, 73, 065502. [Google Scholar] [CrossRef]
- Adler, S.L.; Dashen, R. Current Algebras; Benjamin: New York, NY, USA, 1968. [Google Scholar]
- Alfaro, V.D.; Fubini, S.; Furlan, G.; Rossetti, C. Currents in Hadron Physics; North-Holland Publishing Co.: Amsterdam, The Netherlands; London, UK; American Elsevier Publishing Co., Inc.: New York, NY, USA, 1973. [Google Scholar]
- Sirlin, A. General properties of the electromagnetic corrections to the beta decay of a physical nucleon. Phys. Rev. 1967, 164, 1767. [Google Scholar] [CrossRef]
- Abers, E.S.; Dicus, D.A.; Norton, R.E.; Queen, H.R. Radiative Corrections to the Fermi Part of Strangeness-Conserving β Decay. Phys. Rev. 1968, 167, 1461. [Google Scholar] [CrossRef]
- Serebrov, A.P.; Varlamov, V.E.; Kharitonov, A.G.; Fomin, A.K.; Pokotilovski, Y.N.; Geltenbort, P.; Krasnoschekova, I.A.; Lasakov, M.S.; Taldaev, R.R.; Vassiljev, A.V.; et al. Neutron lifetime measurements using gravitationally trapped ultracold neutrons. Phys. Rev. C 2008, 78, 035505. [Google Scholar] [CrossRef]
- Ebel, M.E.; Feldman, G. Further remarks on Coulomb corrections in allowed beta transitions. Nucl. Phys. 1957, 4, 213. [Google Scholar] [CrossRef]
- Herczeg, P. Beta decay beyond the standard model. Prog. Part. Nucl. Phys. 2001, 46, 413. [Google Scholar] [CrossRef]
- Severijns, N.; Beck, M.; Naviliat-Cuncic, O. Tests of the standard electroweak model in nuclear beta decay. Rev. Mod. Phys. 2006, 78, 991–1040. [Google Scholar] [CrossRef]
- Nico, J.S. Neutron beta decay. J. Phys. G Nucl. Part. Phys. 2009, 36, 104001. [Google Scholar] [CrossRef]
- Jackson, J.D.; Treiman, S.B.; Wyld, H.W., Jr. Possible Tests of Time Reversal Invariance in Beta Decay. Phys. Rev. 1957, 106, 517. [Google Scholar] [CrossRef]
- Herczeg, P. Beta decay and muon decay beyond the Standard Model. In Precision Tests of the Standard Electroweak Model; Langacker, P., Ed.; Advanced Series on Directions in High Energy Physics; World Scientific: Singapore, 1995; Volume 14, p. 7851998. [Google Scholar]
- Faber, M.; Ivanov, A.N.; Ivanova, V.A.; Marton, J.; Pitschmann, M.; Serebrov, A.P.; Troitskaya, N.I.; Wellenzohn, M. Continuum-state and bound-state β-decay rates of the neutron. Phys. Rev. C 2009, 80, 035503. [Google Scholar] [CrossRef]
- Saul, H. Measurement of the Beta Asymmetry in Neutron Decay with PERKEO III. In Proceedings of the (the PERKEO Collaboration), Talk on 20th of January 2017; Institute of Atomic and Subatomic Physics, Technische Universität Wien: Wien, Austria, 2017. [Google Scholar]
- Ivanov, A.N.; Höllwieser, R.; Troitskaya, N.I.; Wellenzohn, M.; Berdnikov, Y.A. Precision theoretical analysis of neutron radiative beta decay to order O(α2/π2). Phys. Rev. D 2017, arXiv:1701.04613. [Google Scholar] [CrossRef]
- Ivanov, A.N.; Höllwieser, R.; Troitskaya, N.I.; Wellenzohn, M.; Zherebtsov, O.M.; Serebrov, A.P. Deficit of reactor antineutrinos at distances smaller than 100 m and inverse β decay. Phys. Rev. C 2013, 88, 055501. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Altarawneh, D.; Höllwieser, R.; Wellenzohn, M. On the Hypothesis of Exact Conservation of Charged Weak Hadronic Vector Current in the Standard Model. Universe 2024, 10, 436. https://doi.org/10.3390/universe10120436
Altarawneh D, Höllwieser R, Wellenzohn M. On the Hypothesis of Exact Conservation of Charged Weak Hadronic Vector Current in the Standard Model. Universe. 2024; 10(12):436. https://doi.org/10.3390/universe10120436
Chicago/Turabian StyleAltarawneh, Derar, Roman Höllwieser, and Markus Wellenzohn. 2024. "On the Hypothesis of Exact Conservation of Charged Weak Hadronic Vector Current in the Standard Model" Universe 10, no. 12: 436. https://doi.org/10.3390/universe10120436
APA StyleAltarawneh, D., Höllwieser, R., & Wellenzohn, M. (2024). On the Hypothesis of Exact Conservation of Charged Weak Hadronic Vector Current in the Standard Model. Universe, 10(12), 436. https://doi.org/10.3390/universe10120436






